CO2 Capture and Enhanced Hydrogen Production Enabled by Low-Temperature Separation of PSA Tail Gas: A Detailed Exergy Analysis
Abstract
1. Background and Motivation
- Quantification of the exergy efficiency of a novel process for CO2 separation, purification, and compression, unambiguously defined as the ratio between the actual power requirement and the minimum exergy requirement for a reversible process;
- Allocation and quantification of all thermodynamic losses in the process;
- Presentation of a procedure to verify the correctness of the results through a transparent consistency check, where two independent calculations of the total irreversibility are compared.
2. Literature Review
2.1. Low-Temperature and Cryogenic Post-Combustion CO2 Capture
2.2. CO2 Capture from Oxyfuel Combustion
2.3. CO2 Capture in Pre-Combustion Syngas and Hydrogen Production Processes
2.4. Exergy Analysis of Low-Temperature CO2 Separation Processes
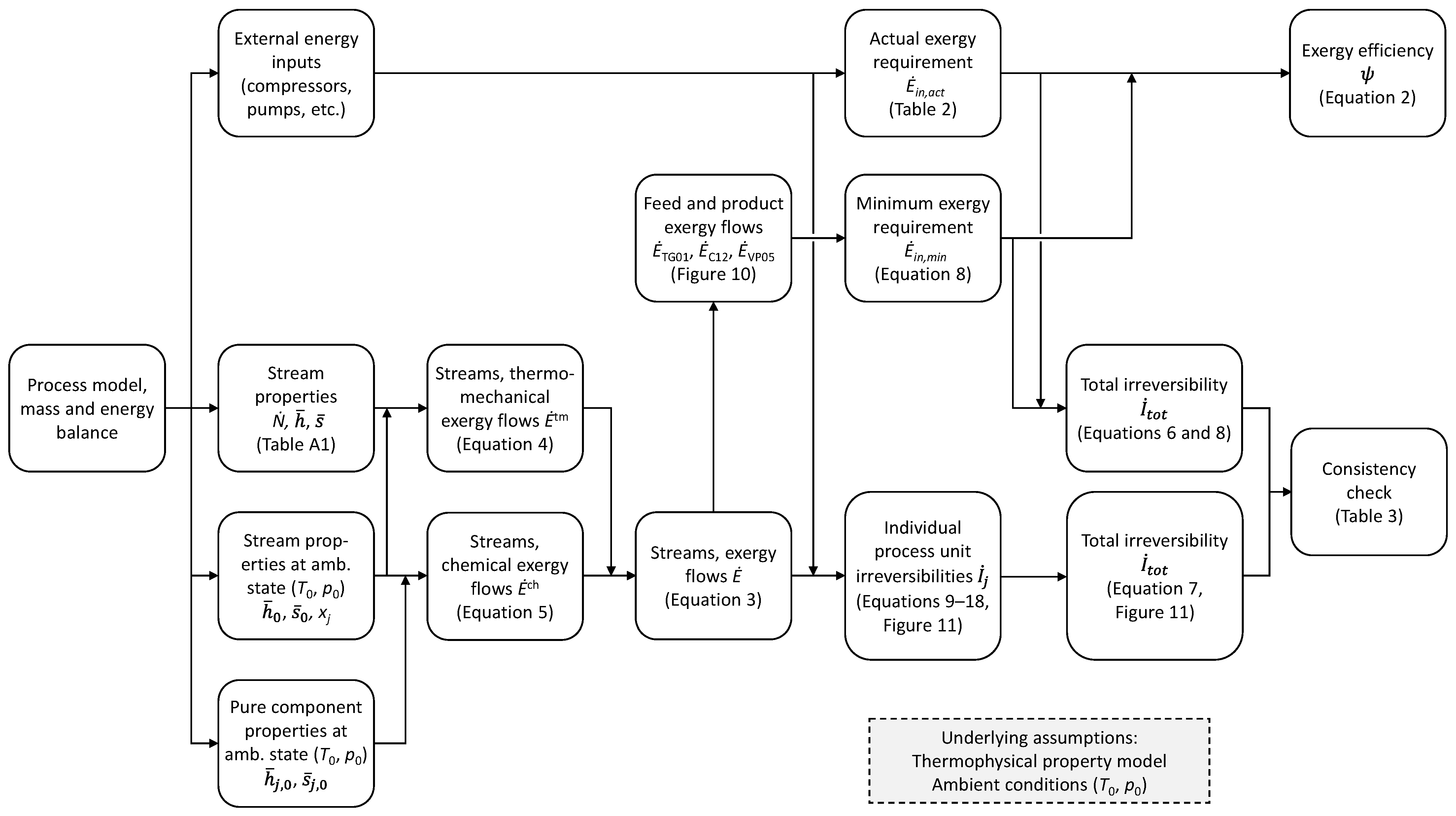
3. Method: Exergy Analysis
3.1. Exergy Components
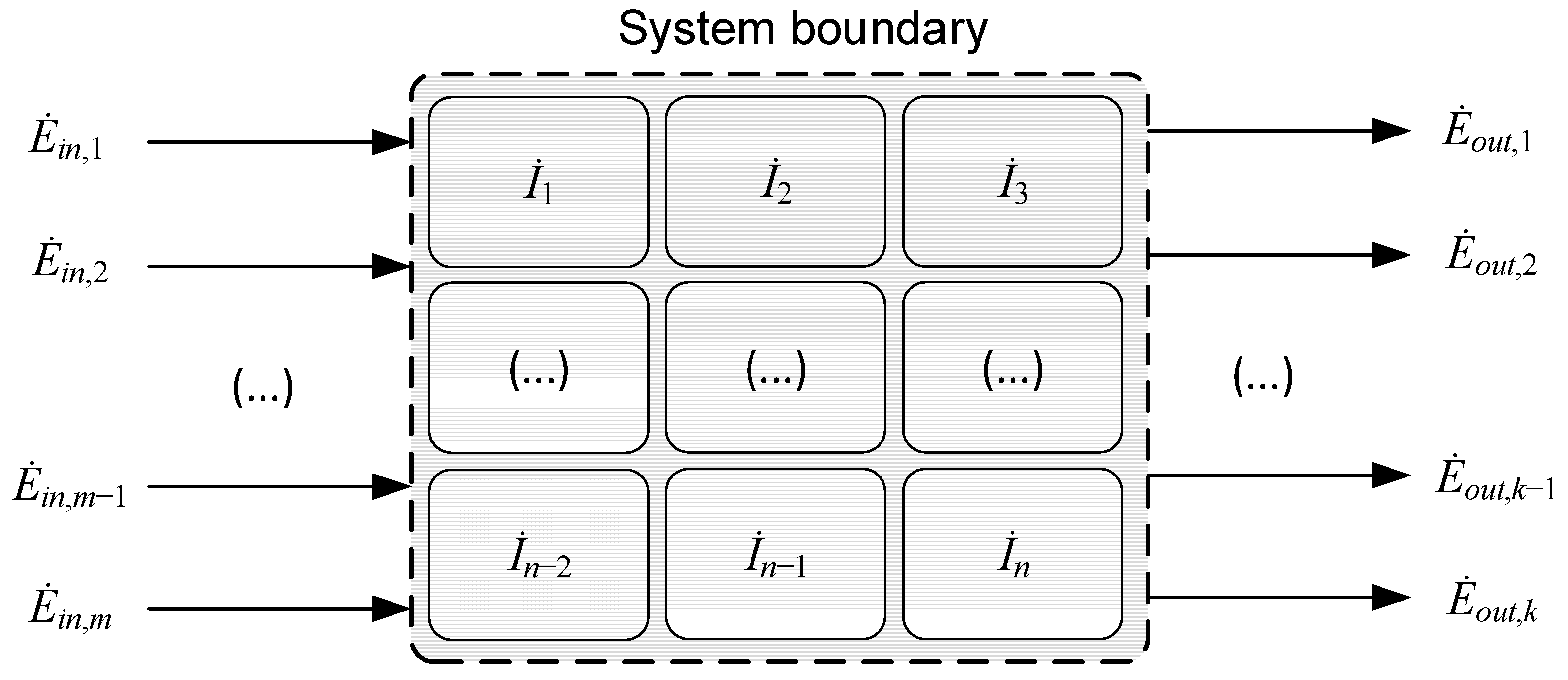
3.2. Exergy Balance of a Control Volume
3.3. Minimum Exergy Requirement
3.4. Irreversibilities in Process Components
3.4.1. Heat Exchangers
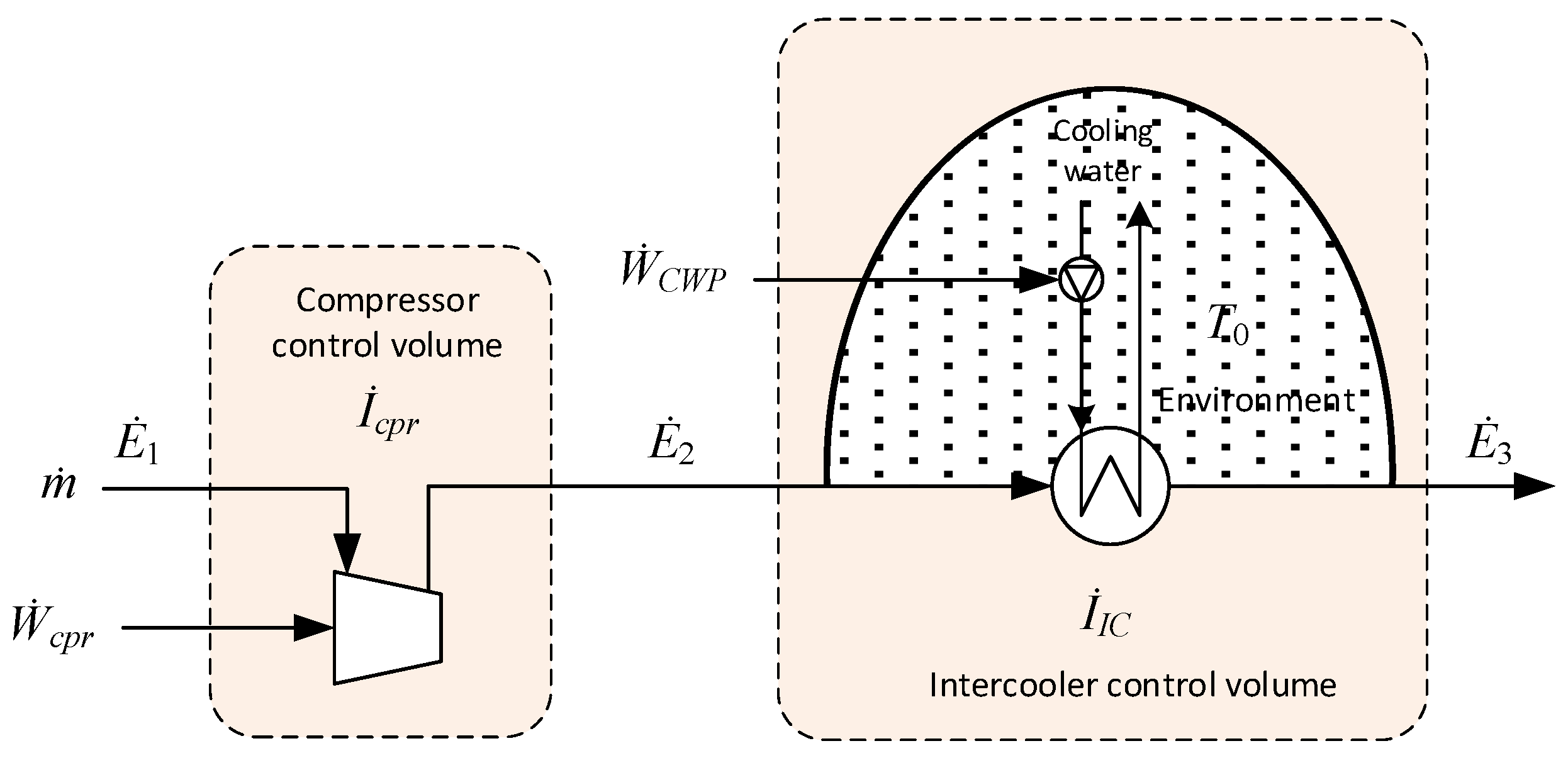
3.4.2. Compressors and Intercoolers
3.4.3. Expanders
3.4.4. Regenerative Solid Desiccant Dehydration Unit
3.4.5. Other Components: Mixers, Separators, and Valves
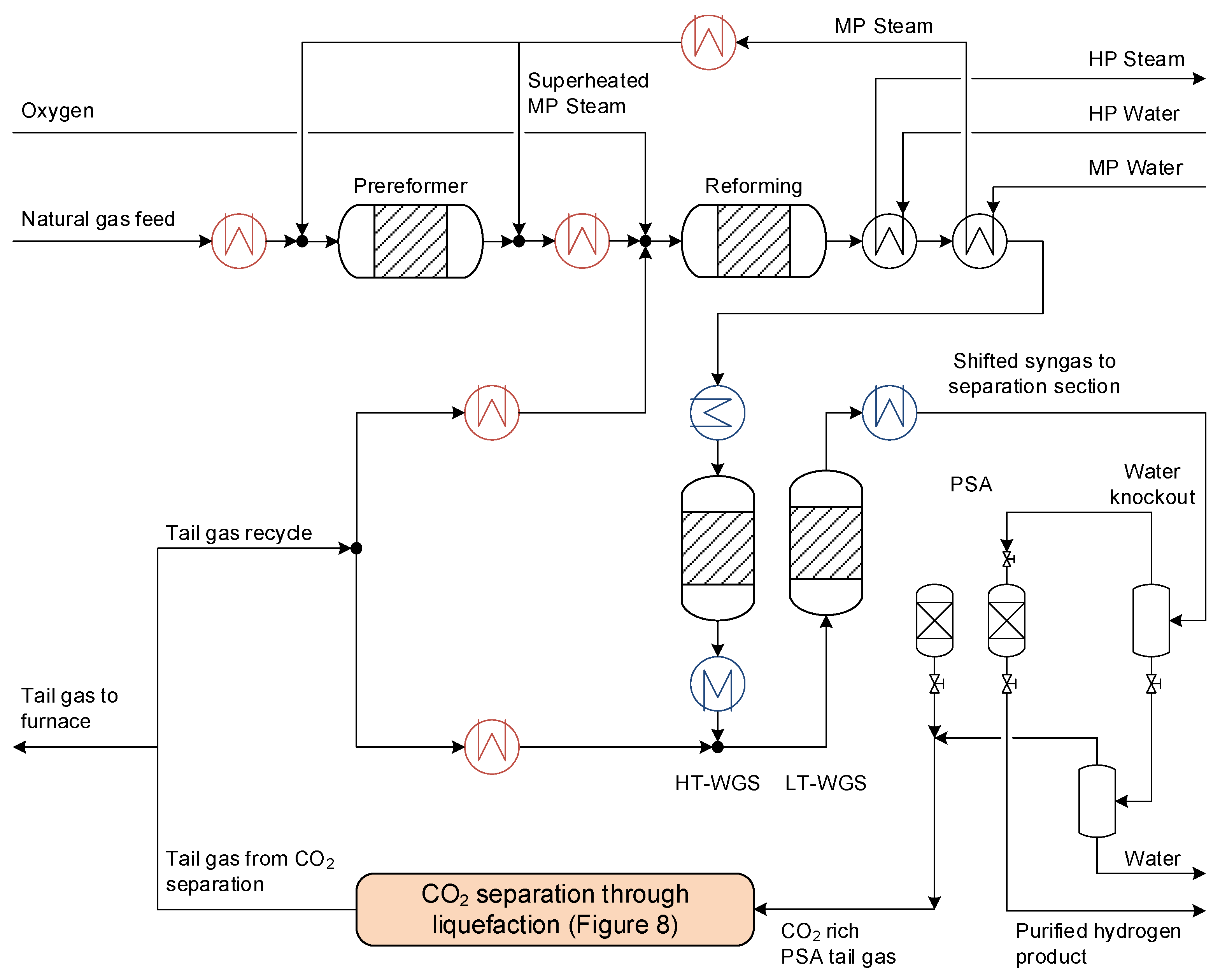
4. Process Description
4.1. Hydrogen Production Plant with CO2 Capture
- 1.1 bar(a) tail gas discharge pressure;
- 85% hydrogen recovery ratio.
4.2. Low-Temperature Unit for CO2 Capture and Tail Gas Recovery and Recycling
- Multi-stage tail gas compression with intercoolers and dehydration unit;
- Heat exchanger network for partial condensation of the compressed tail gas;
- Main separation vessel for phase separation of the partially condensed tail gas;
- Three-stage flash separation for CO2 purification and flash gas recycling to compressors;
- Auxiliary cascade refrigeration cycle with propane and ethane as refrigerants;
- Pressurisation of the purified CO2 by liquid pumping.

5. Results and Discussion
- To derive the minimum exergy requirement for transforming the tail gas feed stream into the specified separation products;
- To calculate the exergy efficiency of this process;
- To provide full transparency of the causes of exergy losses,
- A detailed explanation of the required power input to the process;
- Transparent checks and balances to verify that all irreversibilities are accounted for by disclosing the relative difference in the independent irreversibility calculations based on the bottom-up and top-down approaches (see Equations (6) and (7));
- A basis for assessing further opportunities for improving the process efficiency.
5.1. Minimum Exergy Requirement for the Tail Gas Separation Unit
5.2. Distribution of Process Irreversibilities
5.3. Irreversibility Components in Process Heat Exchangers
5.4. Consistency of Irreversibility Calculations
5.5. Exergy Efficiency
5.6. Sensitivity Analysis
6. Discussion
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| ED | exergy destruction |
| EF | “fuel exergy” |
| EL | exergy loss |
| exergy flow rate | |
| minimum exergy requirement | |
| actual exergy input | |
| molar exergy | |
| h | specific enthalpy |
| molar enthalpy | |
| irreversibility rate | |
| mass flow rate | |
| molar flow rate | |
| p | pressure |
| heat rate | |
| s | specific entropy |
| molar entropy | |
| T | temperature |
| power, rate of work | |
| x | molar fraction |
| Greek symbols | |
| η | efficiency |
| Δ | difference |
| ρ | mass density |
| ψ | exergy efficiency, rational efficiency |
| Subscripts | |
| 0 | ambient |
| C | cold |
| cpr | compressor, compression |
| CWP | cooling water pump |
| dry | drying, dehydration |
| el | electric |
| exp | expander, expansion |
| H | hot |
| HX | heat exchanger |
| IC | intercooler |
| j | integer counter |
| p | power |
| prod | product |
| REC | recycle |
| th | thermal |
| tot | total |
| Superscripts | |
| ch | chemical |
| kin | kinetic |
| pot | potential |
| Q | heat transfer |
| tm | thermomechanical |
| Δp | pressure loss |
Appendix A
| Stream ID | Temperature | Pressure | Molar Flow | Enthalpy | Entropy | Chemical Composition [mol%] | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| °C | bar | kmol/h | kJ/kmol | kJ/(kmol-K) | CH4 | CO2 | N2 | CO | H2O | H2 | C3H8 | C2H6 | |
| C01 | −53.00 | 55.00 | 5049.9 | −395,822 | 78.15 | 1.09 | 95.61 | 1.65 | 0.63 | 0.00 | 1.02 | — | — |
| C02 | −22.50 | 54.70 | 5049.9 | −393,152 | 89.49 | 1.09 | 95.61 | 1.65 | 0.63 | 0.00 | 1.02 | — | — |
| C03 | −25.43 | 31.80 | 5049.9 | −393,152 | 89.95 | 1.09 | 95.61 | 1.65 | 0.63 | 0.00 | 1.02 | — | — |
| C04 | −25.43 | 31.80 | 4802.7 | −400,800 | 87.71 | 0.84 | 97.51 | 0.97 | 0.38 | 0.00 | 0.30 | — | — |
| C05 | −32.87 | 17.10 | 4802.7 | −400,800 | 88.08 | 0.84 | 97.51 | 0.97 | 0.38 | 0.00 | 0.30 | — | — |
| C06 | −32.87 | 17.10 | 4463.6 | −407,318 | 84.00 | 0.45 | 99.06 | 0.32 | 0.13 | 0.00 | 0.03 | — | — |
| C07 | −50.72 | 6.94 | 4463.6 | −407,318 | 84.52 | 0.45 | 99.06 | 0.32 | 0.13 | 0.00 | 0.03 | — | — |
| C08 | −50.72 | 6.94 | 3966.0 | −411,740 | 76.72 | 0.10 | 99.85 | 0.03 | 0.01 | 0.00 | 0.00 | — | — |
| C09 | −47.46 | 70.00 | 3966.0 | −411,419 | 77.13 | 0.10 | 99.85 | 0.03 | 0.01 | 0.00 | 0.00 | — | — |
| C10 | −22.00 | 69.70 | 3966.0 | −409,219 | 86.36 | 0.10 | 99.85 | 0.03 | 0.01 | 0.00 | 0.00 | — | — |
| C11 | 11.21 | 69.40 | 3966.0 | −405,634 | 99.72 | 0.10 | 99.85 | 0.03 | 0.01 | 0.00 | 0.00 | — | — |
| C12 | 16.56 | 110.00 | 3966.0 | −405,365 | 99.94 | 0.10 | 99.85 | 0.03 | 0.01 | 0.00 | 0.00 | — | — |
| HR01 | −25.43 | 31.80 | 247.2 | −244,539 | 133.45 | 6.06 | 58.70 | 14.75 | 5.48 | 0.00 | 15.01 | — | — |
| HR02 | −25.51 | 31.70 | 247.2 | −244,539 | 133.47 | 6.06 | 58.70 | 14.75 | 5.48 | 0.00 | 15.01 | — | — |
| LR01 | −50.72 | 6.94 | 497.6 | −372,078 | 146.72 | 3.24 | 92.75 | 2.64 | 1.07 | 0.00 | 0.30 | — | — |
| LR02 | −50.90 | 6.84 | 497.6 | −372,078 | 146.83 | 3.24 | 92.75 | 2.64 | 1.07 | 0.00 | 0.30 | — | — |
| MR01 | −32.87 | 17.10 | 339.1 | −315,003 | 141.79 | 5.89 | 77.11 | 9.51 | 3.64 | 0.00 | 3.85 | — | — |
| MR02 | −33.01 | 17.00 | 339.1 | −315,003 | 141.83 | 5.89 | 77.11 | 9.51 | 3.64 | 0.00 | 3.85 | — | — |
| REC01 | 23.00 | 31.70 | 522.6 | −256,736 | 137.15 | 2.60 | 63.68 | 8.35 | 2.91 | 0.00 | 22.45 | — | — |
| REC02 | 23.00 | 17.00 | 522.6 | −256,387 | 143.17 | 2.60 | 63.68 | 8.35 | 2.91 | 0.00 | 22.45 | — | — |
| TG01 | 23.00 | 1.10 | 7369.3 | −245,773 | 165.91 | 2.40 | 60.93 | 8.66 | 2.99 | 0.26 | 24.75 | — | — |
| TG02 | 116.93 | 3.05 | 7369.3 | −242,442 | 167.22 | 2.40 | 60.93 | 8.66 | 2.99 | 0.26 | 24.75 | — | — |
| TG03 | 23.00 | 2.75 | 7369.3 | −245,809 | 158.21 | 2.40 | 60.93 | 8.66 | 2.99 | 0.26 | 24.75 | — | — |
| TG04 | 110.65 | 7.14 | 7369.3 | −242,727 | 159.44 | 2.40 | 60.93 | 8.66 | 2.99 | 0.26 | 24.75 | — | — |
| TG05 | 23.00 | 6.84 | 7369.3 | −245,896 | 150.42 | 2.40 | 60.93 | 8.66 | 2.99 | 0.26 | 24.75 | — | — |
| TG06 | 17.87 | 6.84 | 7866.9 | −253,878 | 150.45 | 2.46 | 62.94 | 8.28 | 2.87 | 0.24 | 23.20 | — | — |
| TG07 | 101.80 | 17.30 | 7866.9 | −250,982 | 151.63 | 2.46 | 62.94 | 8.28 | 2.87 | 0.24 | 23.20 | — | — |
| TG08 | 23.00 | 17.00 | 7866.9 | −253,944 | 142.90 | 2.46 | 62.94 | 8.28 | 2.87 | 0.24 | 23.20 | — | — |
| TG09 | 20.68 | 17.00 | 8728.6 | −256,462 | 142.96 | 2.60 | 63.54 | 8.33 | 2.91 | 0.22 | 22.41 | — | — |
| TG10 | 79.87 | 33.00 | 8728.6 | −254,496 | 143.80 | 2.60 | 63.54 | 8.33 | 2.91 | 0.22 | 22.41 | — | — |
| TG11 | 23.00 | 32.70 | 8728.6 | −256,769 | 136.84 | 2.60 | 63.54 | 8.33 | 2.91 | 0.22 | 22.41 | — | — |
| TG12 | 23.00 | 31.70 | 8186.8 | −256,736 | 137.15 | 2.60 | 63.68 | 8.35 | 2.91 | 0.00 | 22.45 | — | — |
| TG13 | 21.54 | 31.70 | 8434.0 | −256,378 | 137.07 | 2.70 | 63.53 | 8.54 | 2.99 | 0.00 | 22.24 | — | — |
| TG14 | 74.00 | 56.50 | 8434.0 | −254,741 | 137.78 | 2.70 | 63.53 | 8.54 | 2.99 | 0.00 | 22.24 | — | — |
| TG15 | 23.00 | 56.20 | 8434.0 | −256,928 | 131.00 | 2.70 | 63.53 | 8.54 | 2.99 | 0.00 | 22.24 | — | — |
| TG16 | −8.40 | 55.90 | 8434.0 | −259,051 | 123.33 | 2.70 | 63.53 | 8.54 | 2.99 | 0.00 | 22.24 | — | — |
| TG17 | −19.00 | 55.60 | 8434.0 | −262,272 | 110.96 | 2.70 | 63.53 | 8.54 | 2.99 | 0.00 | 22.24 | — | — |
| TG18 | −37.44 | 55.30 | 8434.0 | −265,519 | 97.77 | 2.70 | 63.53 | 8.54 | 2.99 | 0.00 | 22.24 | — | — |
| TG19 | −53.00 | 55.00 | 8434.0 | −267,322 | 89.89 | 2.70 | 63.53 | 8.54 | 2.99 | 0.00 | 22.24 | — | — |
| VP01 | −53.00 | 55.00 | 3384.1 | −75,570 | 107.41 | 5.11 | 15.67 | 18.83 | 6.50 | 0.00 | 53.89 | — | — |
| VP02 | −22.50 | 54.70 | 3384.1 | −74,546 | 111.81 | 5.11 | 15.67 | 18.83 | 6.50 | 0.00 | 53.89 | — | — |
| VP03 | −38.03 | 42.31 | 3384.1 | −74,969 | 112.13 | 5.11 | 15.67 | 18.83 | 6.50 | 0.00 | 53.89 | — | — |
| VP04 | −22.50 | 42.01 | 3384.1 | −74,462 | 114.28 | 5.11 | 15.67 | 18.83 | 6.50 | 0.00 | 53.89 | — | — |
| VP05 | 11.21 | 41.70 | 3384.1 | −73,374 | 118.41 | 5.11 | 15.67 | 18.83 | 6.50 | 0.00 | 53.89 | — | — |
| C3-LP01 | −22.00 | 2.27 | 2796.1 | −124,068 | 76.46 | — | — | — | — | — | — | 100 | — |
| C3-LP02 | −22.00 | 2.27 | 1150.6 | −124,068 | 76.46 | — | — | — | — | — | — | 100 | — |
| C3-LP03 | −19.49 | 2.23 | 1150.6 | −107,355 | 143.14 | — | — | — | — | — | — | 100 | — |
| C3-LP04 | −22.00 | 2.27 | 1645.5 | −124,068 | 76.46 | — | — | — | — | — | — | 100 | — |
| C3-LP05 | −22.49 | 2.23 | 1645.5 | −107,559 | 142.33 | — | — | — | — | — | — | 100 | — |
| C3-LP06 | −21.25 | 2.23 | 2796.1 | −107,475 | 142.67 | — | — | — | — | — | — | 100 | — |
| C3-IP01 | −3.82 | 3.46 | 2796.1 | −106,440 | 143.25 | — | — | — | — | — | — | 100 | — |
| C3-IP02 | −9.87 | 3.46 | 2796.1 | −124,068 | 76.30 | — | — | — | — | — | — | 100 | — |
| C3-IP03 | −9.87 | 3.46 | 3675.6 | −106,873 | 141.62 | — | — | — | — | — | — | 100 | — |
| C3-IP04 | 33.21 | 9.50 | 3675.6 | −104,430 | 142.82 | — | — | — | — | — | — | 100 | — |
| C3-IP05 | 23.00 | 9.20 | 3675.6 | −120,284 | 89.67 | — | — | — | — | — | — | 100 | — |
| C3-IP06 | −9.88 | 3.46 | 3675.6 | −120,284 | 90.68 | — | — | — | — | — | — | 100 | — |
| C2-01 | 17.04 | 15.50 | 1488.5 | −86,207 | 168.83 | — | — | — | — | — | — | — | 100 |
| C2-02 | −18.71 | 15.20 | 1488.5 | −99,126 | 118.88 | — | — | — | — | — | — | — | 100 |
| C2-03 | −55.00 | 4.60 | 1488.5 | −99,126 | 120.28 | — | — | — | — | — | — | — | 100 |
| C2-04 | −52.00 | 4.52 | 1488.5 | −88,913 | 167.22 | — | — | — | — | — | — | — | 100 |
| Isentropic Efficiency | Polytropic Efficiency | Shaft Power | |
|---|---|---|---|
| % | % | kW | |
| Tail gas compressor, stage 1 | 85.0 | 86.6 | 6819.9 |
| Tail gas compressor, stage 2 | 85.0 | 86.5 | 6307.8 |
| Tail gas compressor, stage 3 | 85.0 | 86.5 | 6329.7 |
| Tail gas compressor, stage 4 | 85.0 | 86.1 | 4765.9 |
| Tail gas compressor, stage 5 | 85.0 | 86.0 | 3836.4 |
| Propane compressor, stage 1 | 85.0 | 85.4 | 804.4 |
| Propane compressor, stage 2 | 85.0 | 86.0 | 2495.3 |
| Ethane compressor | 83.0 | 84.9 | 1118.8 |
| Expander | 85.0 | 84.5 | 397.7 |
| LT CO2 pump | 75.0 | 353.6 | |
| HT CO2 pump | 75.0 | 297.0 |
| Heat Exchanger ID | Minimum Temperature Approach | Pressure Drop per Pass | Thermal Duty |
|---|---|---|---|
| °C | kPa | kW | |
| MS-HX1 | 3.0 | 30 | 4972.3 |
| AUX-HX1 | 3.0 | 4–30 | 7546.2 |
| MS-HX2 | 3.0 | 30 | 7608.5 |
| AUX-HX2 | 2.0 | 8–30 | 4222.6 |
| C2 Condenser | 3.3 | 4–30 | 5341.5 |
| Dehydration, specific power | MJp/kg H2O | 8 |
| Molsieve bed, pressure drop | kPa | 100 |
| Molsieve regeneration recycle ratio | % | 6 |
| Ambient coolers, outlet temperature | °C | 23 |
| Ambient coolers, pressure drop | kPa | 30 |
| Ambient coolers, specific pumping power | MJp/MJth | 0.007 |
References
- Seck, G.S.; Hache, E.; Sabathier, J.; Guedes, F.; Reigstad, G.A.; Straus, J.; Wolfgang, O.; Ouassou, J.A.; Askeland, M.; Hjorth, I.; et al. Hydrogen and the decarbonization of the energy system in europe in 2050: A detailed model-based analysis. Renew. Sustain. Energy Rev. 2022, 167, 112779. [Google Scholar] [CrossRef]
- Pruvost, F.; Cloete, S.; del Pozo, C.A.; Zaabout, A. Blue, green, and turquoise pathways for minimizing hydrogen production costs from steam methane reforming with CO2 capture. Energy Convers. Manag. 2022, 274, 116458. [Google Scholar] [CrossRef]
- Berstad, D.; Gundersen, T. On the exergy efficiency of CO2 capture: The relation between sub-process and overall efficiencies. Carbon Capture Sci. Technol. 2023, 7, 100111. [Google Scholar] [CrossRef]
- Straus, J.; Skjervold, V.T.; Anantharaman, R.; Berstad, D. Novel approach for low CO2 intensity hydrogen production from natural gas. Sustain. Energy Fuels 2022, 6, 4948–4961. [Google Scholar] [CrossRef]
- Tuinier, M.; Annaland, M.v.S.; Kuipers, J. A novel process for cryogenic CO2 capture using dynamically operated packed beds—An experimental and numerical study. Int. J. Greenh. Gas Control 2011, 5, 694–701. [Google Scholar] [CrossRef]
- Tuinier, M.; Hamers, H.; Annaland, M.v.S. Techno-Economic evaluation of cryogenic CO2 capture—A comparison with absorption and membrane technology. Int. J. Greenh. Gas Control 2011, 5, 1559–1565. [Google Scholar] [CrossRef]
- Clodic, D.; Hitti, R.; Younes, M.; Bill, A.; Casier, F. CO2 Capture by Anti-Sublimation Thermo-Economic Process Evaluation. In Proceedings of the Fourth Annual Conference Carbon Capture Sequestration, Alexandria, VA, USA, 2–5 May 2005; pp. 1–11. [Google Scholar]
- Clodic, D.; Younes, M.; Bill, A. Test Results of CO2 Capture by Anti-Sublimation Capture Efficiency and Energy Consumption for Boiler Plants. In Greenhouse Gas Control Technologies; Elsevier Science Ltd.: Amsterdam, The Netherlands, 2005; pp. 1775–1780. ISBN 978-0-08-044704-9. [Google Scholar]
- Clodic, D.; Younes, M.; Riachi, Y.; El Hitti, R.; Boulawz Ksayer, E.; Pan, X.; Yu, Y. CO2 Capture by Anti-Sublimation Using Integrated Cascade System. Available online: https://iifiir.org/en/fridoc/co2-capture-by-anti-sublimation-using-integrated-cascade-system-27838 (accessed on 16 January 2024).
- Tuinier, M.; Annaland, M.v.S.; Kramer, G.; Kuipers, J. Cryogenic CO2 capture using dynamically operated packed beds. Chem. Eng. Sci. 2010, 65, 114–119. [Google Scholar] [CrossRef]
- Cann, D.; Font-Palma, C.; Willson, P. Moving packed beds for cryogenic CO2 capture: Analysis of packing material and bed precooling. Carbon Capture Sci. Technol. 2021, 1, 100017. [Google Scholar] [CrossRef]
- Cann, D.; Font-Palma, C.; Willson, P. Experimental analysis of CO2 frost front behaviour in moving packed beds for cryogenic CO2 capture. Int. J. Greenh. Gas Control 2021, 107, 103291. [Google Scholar] [CrossRef]
- Jensen, M.J.; Russell, C.S.; Bergeson, D.; Hoeger, C.D.; Frankman, D.J.; Bence, C.S.; Baxter, L.L. Prediction and validation of external cooling loop cryogenic carbon capture (CCC-ECL) for full-scale coal-fired power plant retrofit. Int. J. Greenh. Gas Control 2015, 42, 200–212. [Google Scholar] [CrossRef]
- De, D.K.; Oduniyi, I.A.; Sam, A.A. A novel cryogenic technology for low-cost carbon capture from NGCC power plants for climate change mitigation. Therm. Sci. Eng. Prog. 2022, 36, 101495. [Google Scholar] [CrossRef]
- Babar, M.; Mukhtar, A.; Mubashir, M.; Saqib, S.; Ullah, S.; Quddusi, A.H.A.; Bustam, M.A.; Show, P.L. Development of a novel switched packed bed process for cryogenic CO2 capture from natural gas. Process. Saf. Environ. Prot. 2021, 147, 878–887. [Google Scholar] [CrossRef]
- Song, C.; Liu, Q.; Ji, N.; Deng, S.; Zhao, J.; Li, Y.; Kitamura, Y. Reducing the energy consumption of membrane-cryogenic hybrid CO2 capture by process optimization. Energy 2017, 124, 29–39. [Google Scholar] [CrossRef]
- Bouma, R.; Vercauteren, F.; van Os, P.; Goetheer, E.; Berstad, D.; Anantharaman, R. Membrane-assisted CO2 Liquefaction: Performance Modelling of CO2 Capture from Flue Gas in Cement Production. Energy Procedia 2017, 114, 72–80. [Google Scholar] [CrossRef]
- Anantharaman, R.; Berstad, D.; Roussanaly, S. Techno-Economic Performance of a Hybrid Membrane—Liquefaction Process for Post-combustion CO2 Capture. Energy Procedia 2014, 61, 1244–1247. [Google Scholar] [CrossRef]
- Song, C.; Fan, Z.; Li, R.; Liu, Q.; Sun, Y.; Kitamura, Y. Intensification of CO2 separation performance via cryogenic and membrane hybrid process—Comparison of polyimide and polysulfone hollow fiber membrane. Chem. Eng. Process.-Process. Intensif. 2018, 133, 83–89. [Google Scholar] [CrossRef]
- Li, R.; Lian, S.; Zhang, Z.; Deng, S.; Song, C. Simulation of a novel hybrid membrane-cryogenic process for post-combustion carbon capture. Carbon Capture Sci. Technol. 2022, 5, 100075. [Google Scholar] [CrossRef]
- Berstad, D.; Anantharaman, R.; Nekså, P. Low-temperature CO2 capture technologies—Applications and potential. Int. J. Refrig. 2013, 36, 1403–1416. [Google Scholar] [CrossRef]
- Magli, F.; Spinelli, M.; Fantini, M.; Romano, M.C.; Gatti, M. Techno-Economic optimization and off-design analysis of CO2 purification units for cement plants with oxyfuel-based CO2 capture. Int. J. Greenh. Gas Control 2022, 115, 103591. [Google Scholar] [CrossRef]
- Jin, B.; Zhao, H.; Zheng, C. Optimization and control for CO2 compression and purification unit in oxy-combustion power plants. Energy 2015, 83, 416–430. [Google Scholar] [CrossRef]
- White, V.; Wright, A.; Tappe, S.; Yan, J. The Air Products Vattenfall Oxyfuel CO2 Compression and Purification Pilot Plant at Schwarze Pumpe. Energy Procedia 2013, 37, 1490–1499. [Google Scholar] [CrossRef]
- Font-Palma, C.; Errey, O.; Corden, C.; Chalmers, H.; Lucquiaud, M.; del Rio, M.S.; Jackson, S.; Medcalf, D.; Livesey, B.; Gibbins, J.; et al. Integrated oxyfuel power plant with improved CO2 separation and compression technology for EOR application. Process. Saf. Environ. Prot. 2016, 103, 455–465. [Google Scholar] [CrossRef]
- Kim, S.-I.; Lee, H.; Lee, Y.W.; Yang, W. Performance evaluation of a pressurized oxy-combustion power plant according to wet and dry flue gas recirculation. Int. J. Greenh. Gas Control 2021, 107, 103277. [Google Scholar] [CrossRef]
- van der Spek, M.; Eldrup, N.H.; Skagestad, R.; Ramirez, A. Techno-Economic Performance of State-of-the-Art Oxyfuel Technology for Low-CO2 Coal-fired Electricity Production. Energy Procedia 2017, 114, 6432–6439. [Google Scholar] [CrossRef]
- Consonni, S.; Viganò, F.; Kreutz, T.; De Lorenzo, L. CO2 Capture in IGCC Plants via Cryogenic Separation. In Proceedings of the Sixth Annual Conference on Carbon Capture & Sequestration, Pittsburgh, PA, USA, 7–10 May 2007; pp. 7–10. [Google Scholar]
- Berstad, D.; Anantharaman, R.; Nekså, P. Low-temperature CCS from an IGCC Power Plant and Comparison with Physical Solvents. Energy Procedia 2013, 37, 2204–2211. [Google Scholar] [CrossRef][Green Version]
- Berstad, D.; Skaugen, G.; Roussanaly, S.; Anantharaman, R.; Nekså, P.; Jordal, K.; Trædal, S.; Gundersen, T. CO2 Capture from IGCC by Low-Temperature Synthesis Gas Separation. Energies 2022, 15, 515. [Google Scholar] [CrossRef]
- Kim, D.; Berstad, D.; Anantharaman, R.; Straus, J.; Peters, T.A.; Gundersen, T. Low Temperature Applications for CO2 Capture in Hydrogen Production. In Computer Aided Chemical Engineering; Pierucci, S., Manenti, F., Bozzano, G.L., Manca, D., Eds.; Elsevier: Amsterdam, The Netherlands, 2020; Volume 48, pp. 445–450. [Google Scholar]
- Kim, D.; Liu, Z.; Anantharaman, R.; Riboldi, L.; Odsæter, L.; Berstad, D.; Peters, T.A.; Polfus, J.M.; Malerød-Fjeld, H.; Gundersen, T. Design of a Novel Hybrid Process for Membrane Assisted Clean Hydrogen Production with CO2 Capture through Liquefaction. In Computer Aided Chemical Engineering; Yamashita, Y., Kano, M., Eds.; Elsevier: Amsterdam, The Netherlands, 2022; Volume 49, pp. 127–132. [Google Scholar]
- Leclerc, M.; Rodrigues, G.; Dubettier, R.; Ruban, S. Optimized configuration to reduce H2 carbon footprint in a refinery. Int. J. Greenh. Gas Control 2022, 118, 103671. [Google Scholar] [CrossRef]
- Marmolejo-Correa, D.; Gundersen, T. A comparison of exergy efficiency definitions with focus on low temperature processes. Energy 2012, 44, 477–489. [Google Scholar] [CrossRef]
- Dodge, B.F. Chemical Engineering Thermodynamics; Chemical Engineering Series; McGraw-Hill Book Company: New York, NY, USA, 1944. [Google Scholar]
- Gjennestad, M.A.; Aursand, E.; Magnanelli, E.; Pharoah, J. Performance analysis of heat and energy recovery ventilators using exergy analysis and nonequilibrium thermodynamics. Energy Build. 2018, 170, 195–205. [Google Scholar] [CrossRef]
- Berstad, D.; Skaugen, G.; Wilhelmsen, Ø. Dissecting the exergy balance of a hydrogen liquefier: Analysis of a scaled-up claude hydrogen liquefier with mixed refrigerant pre-cooling. Int. J. Hydrogen Energy 2020, 46, 8014–8029. [Google Scholar] [CrossRef]
- Kotas, T.J. The Exergy Method of Thermal Plant Analysis; Krieger Publishing: Malabar, FL, USA, 1995; ISBN 978-0-89464-946-2. [Google Scholar]
- Pichot, D.; Granados, L.; Morel, T.; Schuller, A.; Dubettier, R.; Lockwood, F. Start-up of Port-Jérôme CRYOCAP™ Plant: Optimized Cryogenic CO2 Capture from H2 Plants. Energy Procedia 2017, 114, 2682–2689. [Google Scholar] [CrossRef]
- Fandiño, O.; Trusler, J.M.; Vega-Maza, D. Phase behavior of (CO2 + H2) and (CO2 + N2) at temperatures between (218.15 and 303.15) K at pressures up to 15 MPa. Int. J. Greenh. Gas Control 2015, 36, 78–92. [Google Scholar] [CrossRef]



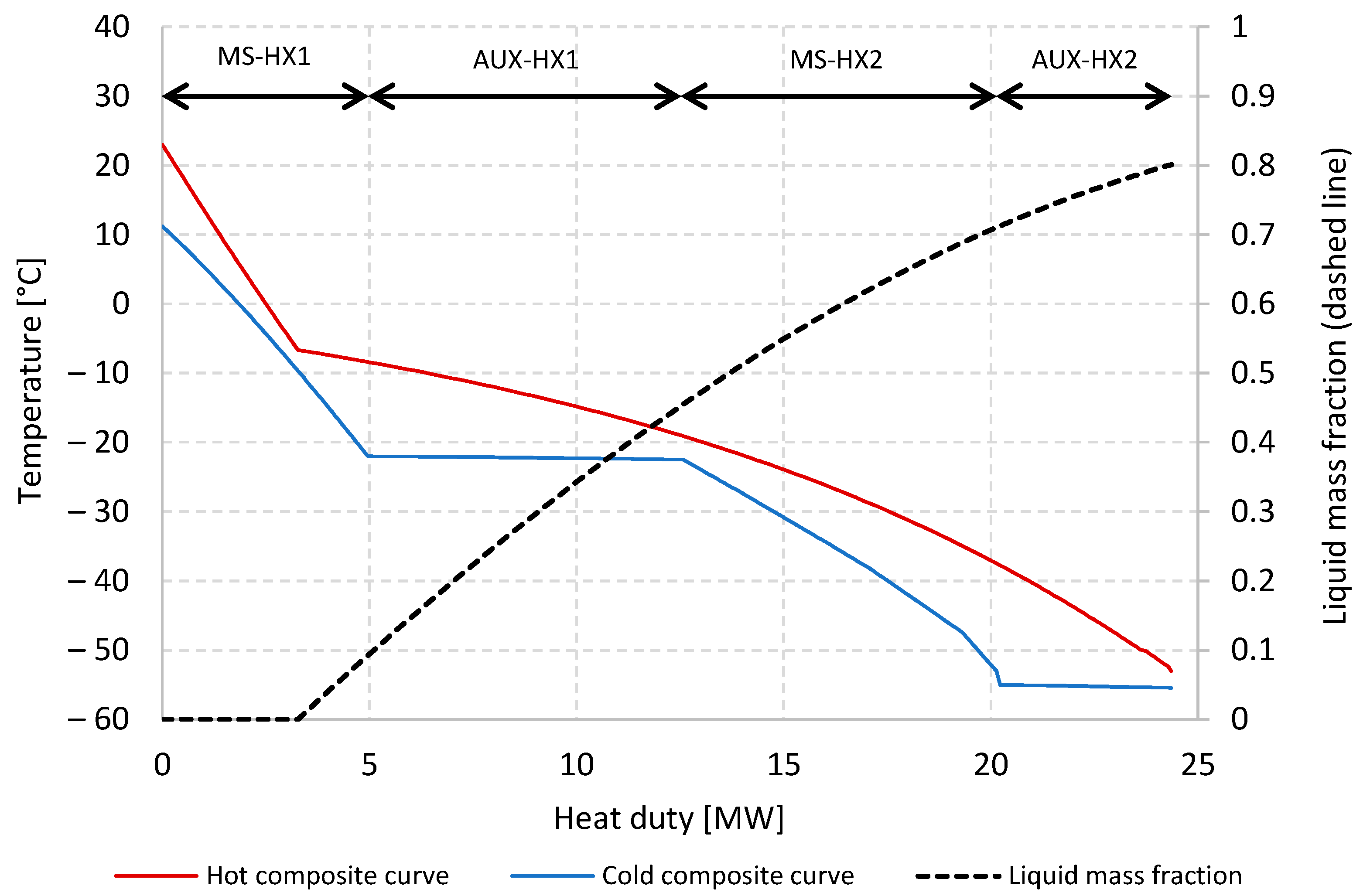
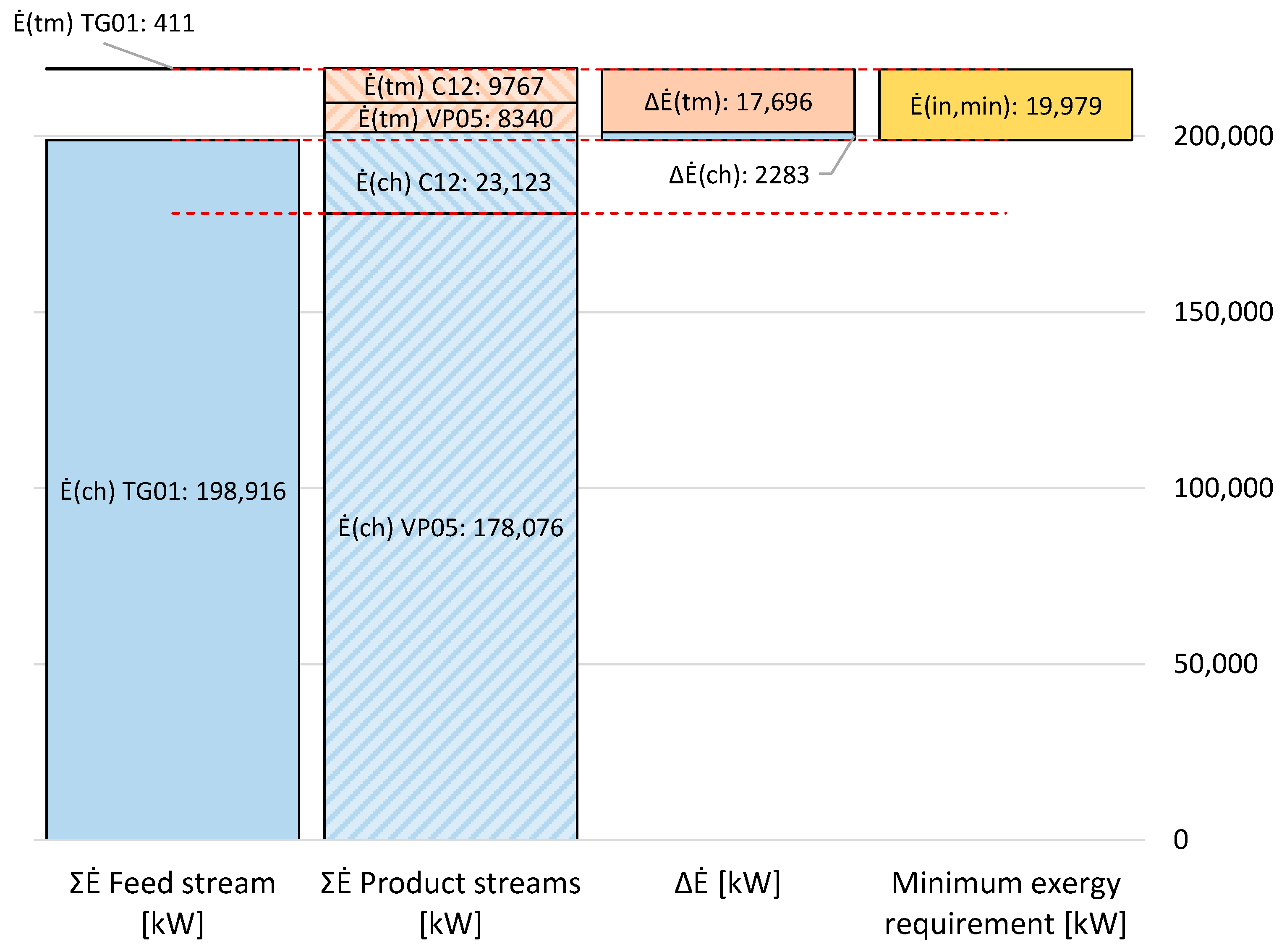


| Parameter/KPI | Unit | Value |
|---|---|---|
| Tail gas recycle | % | 93 |
| Fraction of tail gas recycled to ATR | % | 25 |
| TFeed,LT-WGS | °C | 200 |
| Carbon capture rate | % | 98.24 |
| Higher heating value efficiency | % | 84.44 |
| Lower heating value efficiency | % | 78.34 |
| Power [kW] | Specific Power [MJ/ton CO2 Captured] | Percentage of Total | |
|---|---|---|---|
| Tail gas compression | 28,059.7 | 579.6 | 82.0% |
| Auxiliary refrigeration | 4418.4 | 91.3 | 12.9% |
| Molsieve regeneration | 767.9 | 15.9 | 2.2% |
| Liquid CO2 pumping | 650.6 | 13.4 | 1.9% |
| Cooling water pumping | 326.7 | 6.7 | 1.0% |
| Total power requirement | 34,223.3 | 706.9 | 100% |
| Top-Down Calculation, Equation (6) | Bottom-Up Calculation, Equation (7) | Relative Difference | |
|---|---|---|---|
| Irreversibility [kW] | 14,243.970 | 14,243.975 |
| Sensitivity Variable | Variable Change | Irreversibilities [kW] | Power Requirement [kW] | Exergy Efficiency | Consistency of Exergy Balance Convergence 1 |
|---|---|---|---|---|---|
| Compressor efficiencies [%] | −5% points | 16,320 | 36,299 | 55.04% | 9·10−10 |
| HX pressure losses | +100% | 15,766 | 35,713 | 55.85% | 2·10−7 |
| HX minimum ΔT [°C] | +2 °C | 14,830 | 34,809 | 57.40% | 1·10−6 |
| Ambient coolers outlet T [°C] | +7 °C | 14,664 | 34,651 | 57.68% | 6·10−7 |
| Dehydration, recycle ratio | +4% points | 14,460 | 34,439 | 58.01% | 2·10−7 |
| Separator heat leakage [W] | +1000 W 2 | 14,247 | 34,226 | 58.37% | 2·10−7 |
| Baseline results | — | 14,244 | 34,223 | 58.38% | 4·10−7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Berstad, D.; Straus, J.; Gundersen, T. CO2 Capture and Enhanced Hydrogen Production Enabled by Low-Temperature Separation of PSA Tail Gas: A Detailed Exergy Analysis. Energies 2024, 17, 1072. https://doi.org/10.3390/en17051072
Berstad D, Straus J, Gundersen T. CO2 Capture and Enhanced Hydrogen Production Enabled by Low-Temperature Separation of PSA Tail Gas: A Detailed Exergy Analysis. Energies. 2024; 17(5):1072. https://doi.org/10.3390/en17051072
Chicago/Turabian StyleBerstad, David, Julian Straus, and Truls Gundersen. 2024. "CO2 Capture and Enhanced Hydrogen Production Enabled by Low-Temperature Separation of PSA Tail Gas: A Detailed Exergy Analysis" Energies 17, no. 5: 1072. https://doi.org/10.3390/en17051072
APA StyleBerstad, D., Straus, J., & Gundersen, T. (2024). CO2 Capture and Enhanced Hydrogen Production Enabled by Low-Temperature Separation of PSA Tail Gas: A Detailed Exergy Analysis. Energies, 17(5), 1072. https://doi.org/10.3390/en17051072





