Autonomous Gate Drivers Tailored for Triangular Current Mode-Based Zero-Voltage Switching Two-Level Three-Phase Inverters for Electric Vehicle Drive Systems
Abstract
1. Introduction
- Achieve turn-on ZVS by sensing the on-state of the power device on the gate driver.
- High-speed peak current control by measuring the current of the power device on the gate driver.
- Analyzing the intended operation of the proposed AGDs for a 10 kW power-rated inverter.
- Examining ZVS at both turn-on and turn-off by computing the switching losses.
- Analyzing the sinusoidal output waveforms of currents and voltages.
- Evaluating the inverter efficiency based on the proposed AGDs.
- Assessing the adaptive control features of the proposed AGDs.
2. Soft-Switched Inverter
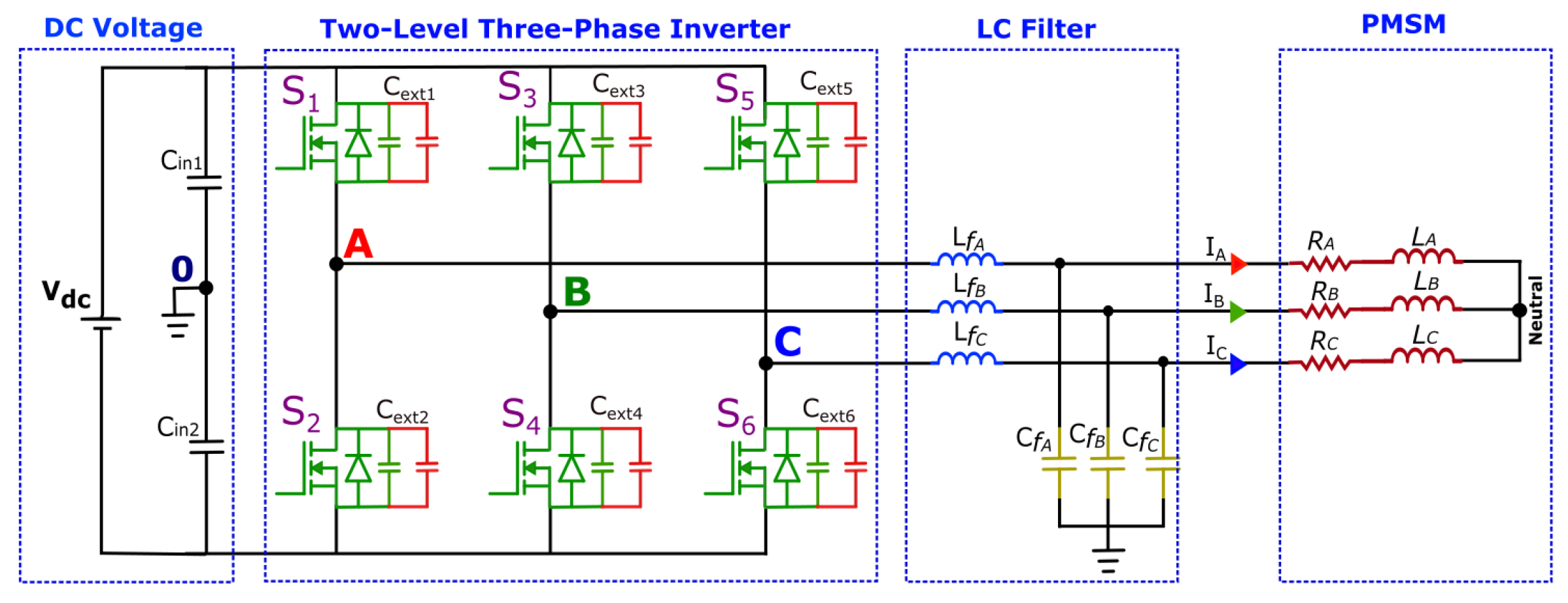
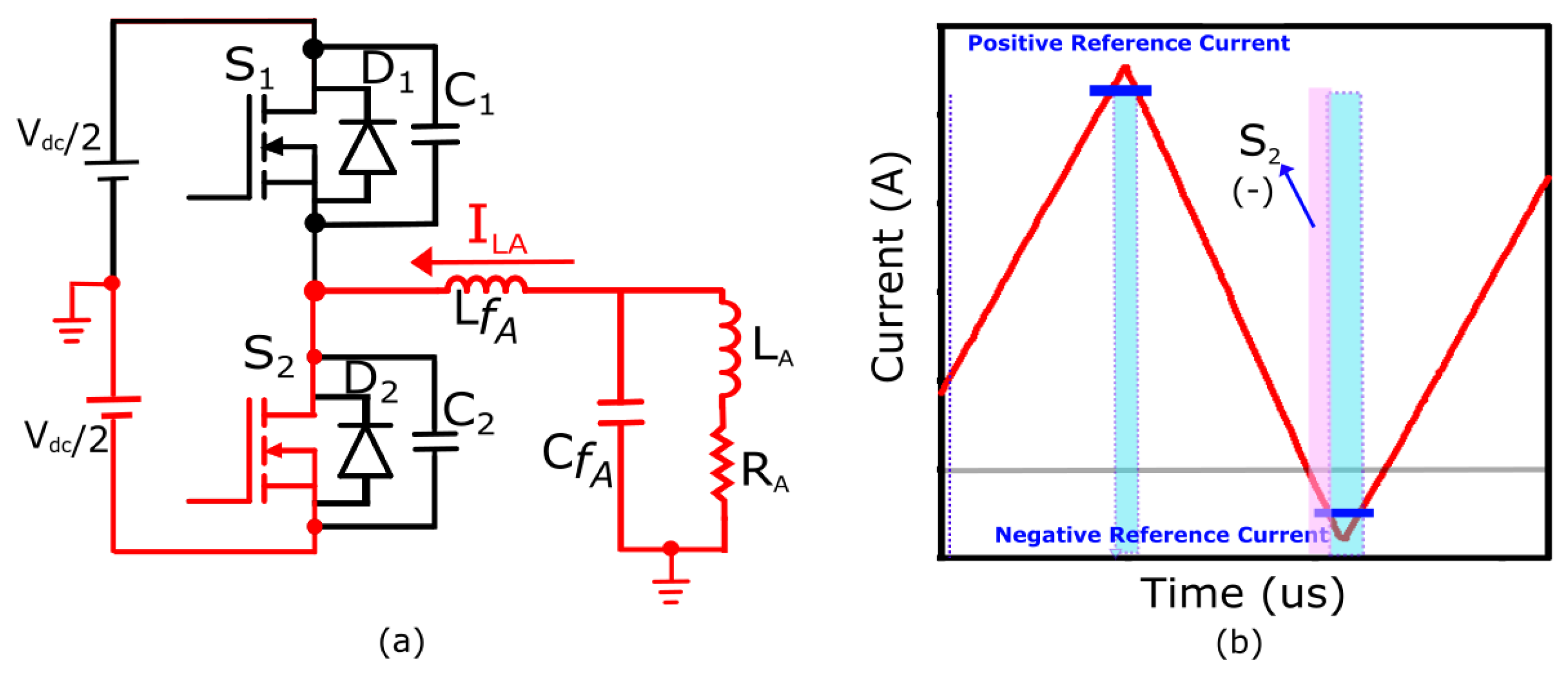
2.1. Circuit Topology
- Each switch is equipped with an external snubber capacitance (). The objective of this capacitance is to increase the overall capacitance across the switch. This increased capacitance plays an important role in achieving turn-off ZVS as outlined in [38]. The intentional presence of this enhanced capacitance serves to deliberately slow down the rise time of during the turn-off transient, effectively reducing the overlap between non-zero and by distributing it over time.
- The star point of the filter capacitors is connected to the mid-point of the dc-bus. This setup provides low-impedance pathways for high-frequency components within the inductor currents (, , ). Additionally, the wire connecting the dc-bus and filter capacitors serves as a pseudo fourth wire within the system, making it possible for the non-zero sum of the filter inductor currents to circulate back to the DC bus [39]. This connection helps in controlling the common-mode voltages of the filter capacitor. By managing the common-mode voltage in this manner, the EMI issues and leaking current [40] can be reduced. In motor-drive applications, this strategy can be employed to effectively reduce bearing currents [41,42]. It is noteworthy that harmonics could be introduced into this common mode [39], presenting an opportunity to lower both the required DC link voltage [43] and capacitance [44].
2.2. Principle of Operation
2.2.1. Operating Modes of a Single-Phase Half-Bridge Inverter
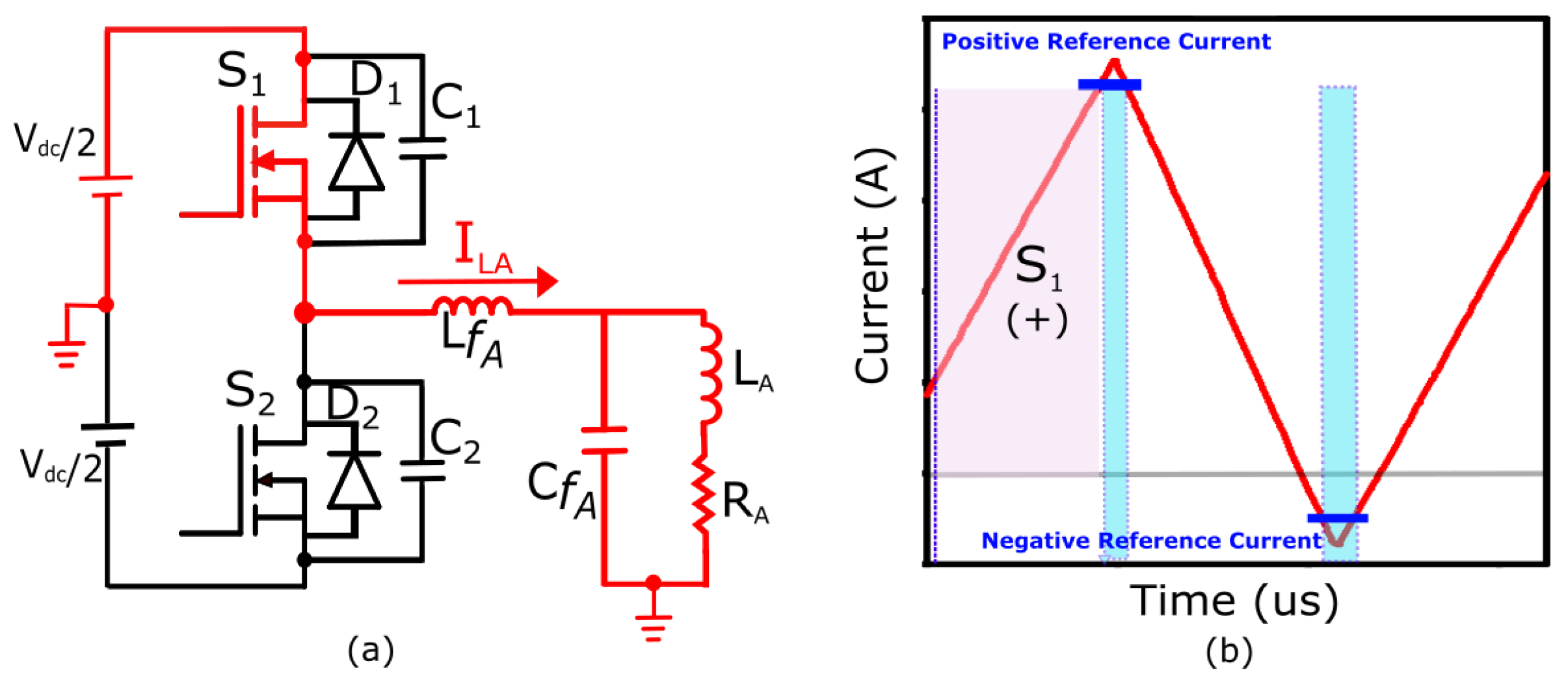
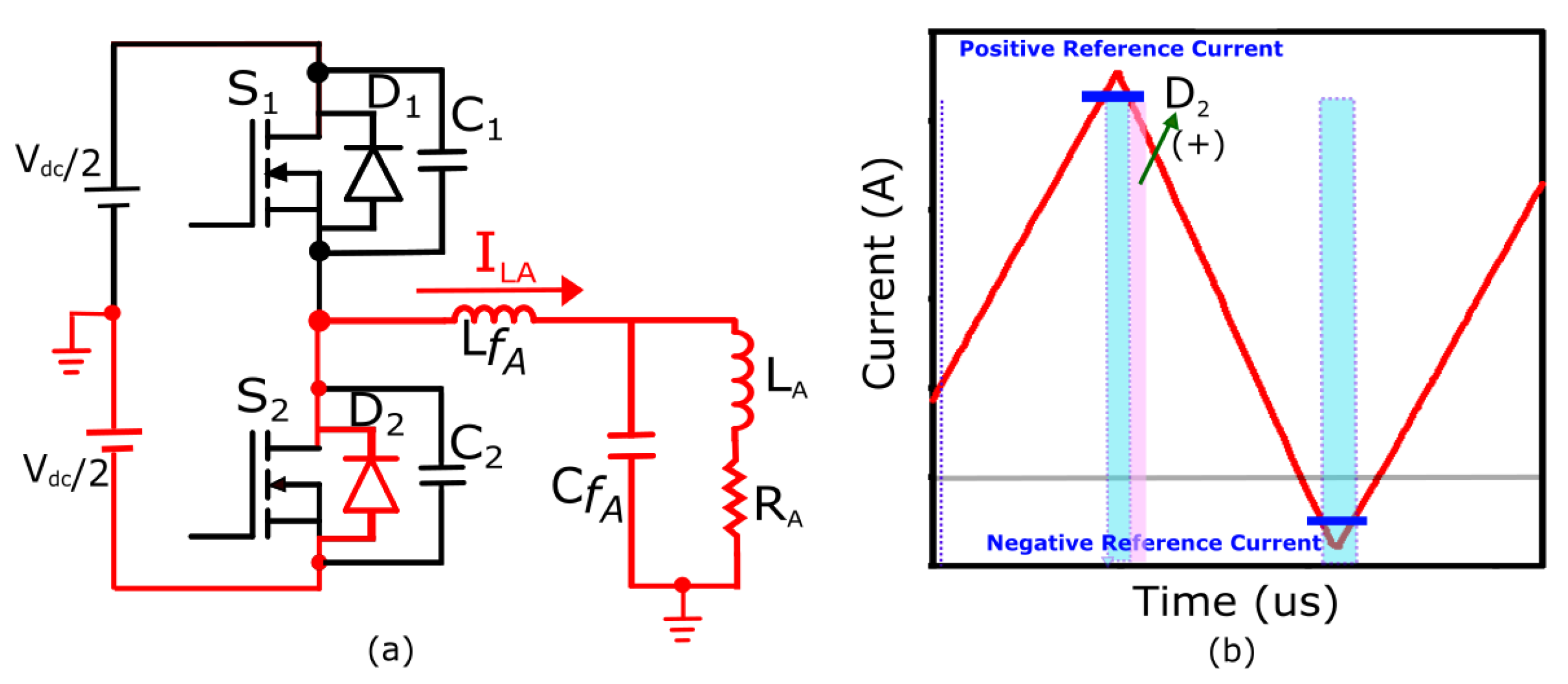
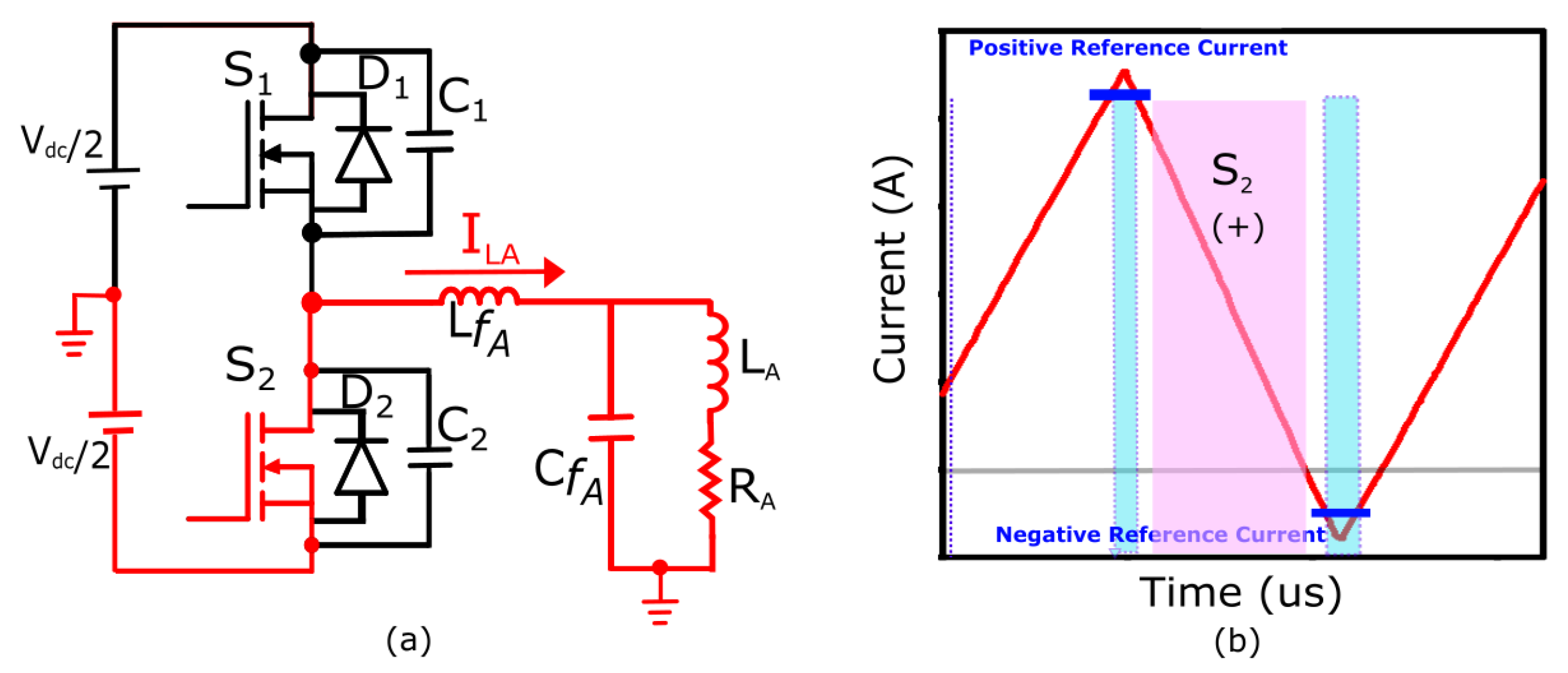
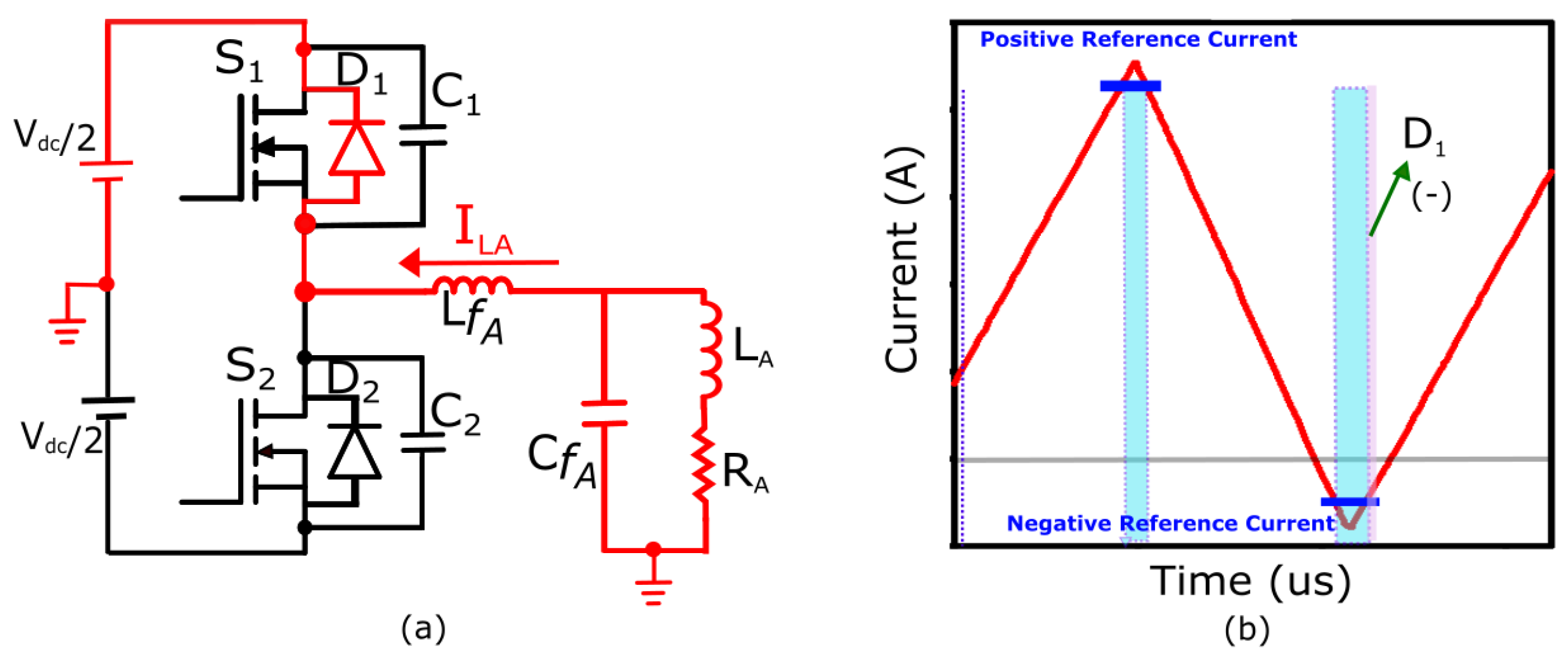
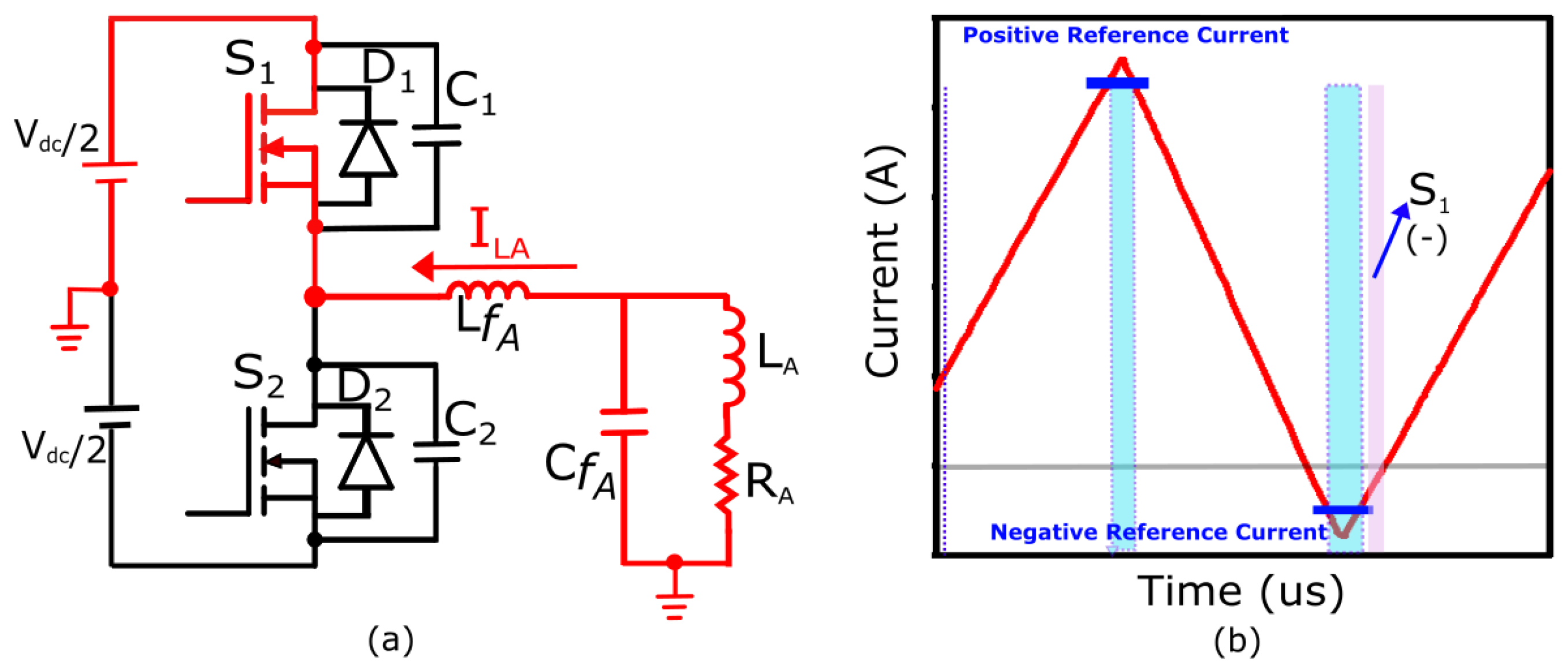
Mode 1
Mode 2
Mode 3
Mode 4
Mode 5
Mode 6
Mode 7
Mode 8
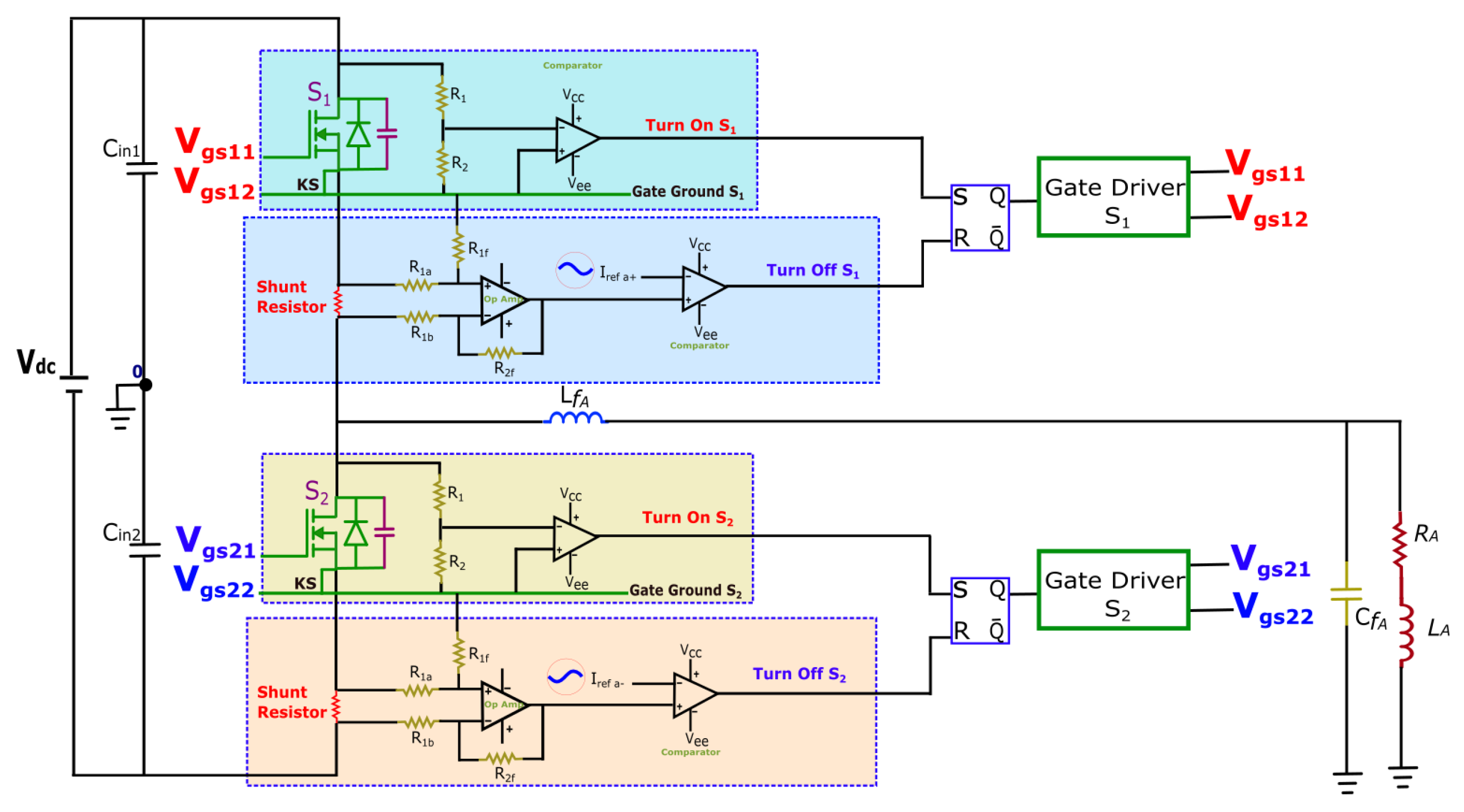
3. Autonomous Gate Drivers (AGDs)
3.1. Generation of Turn-on Signal
3.2. Generation of Turn-off Signal
3.3. Generation of Reference Current
4. Simulation Results and Discussion
4.1. Model Parameters
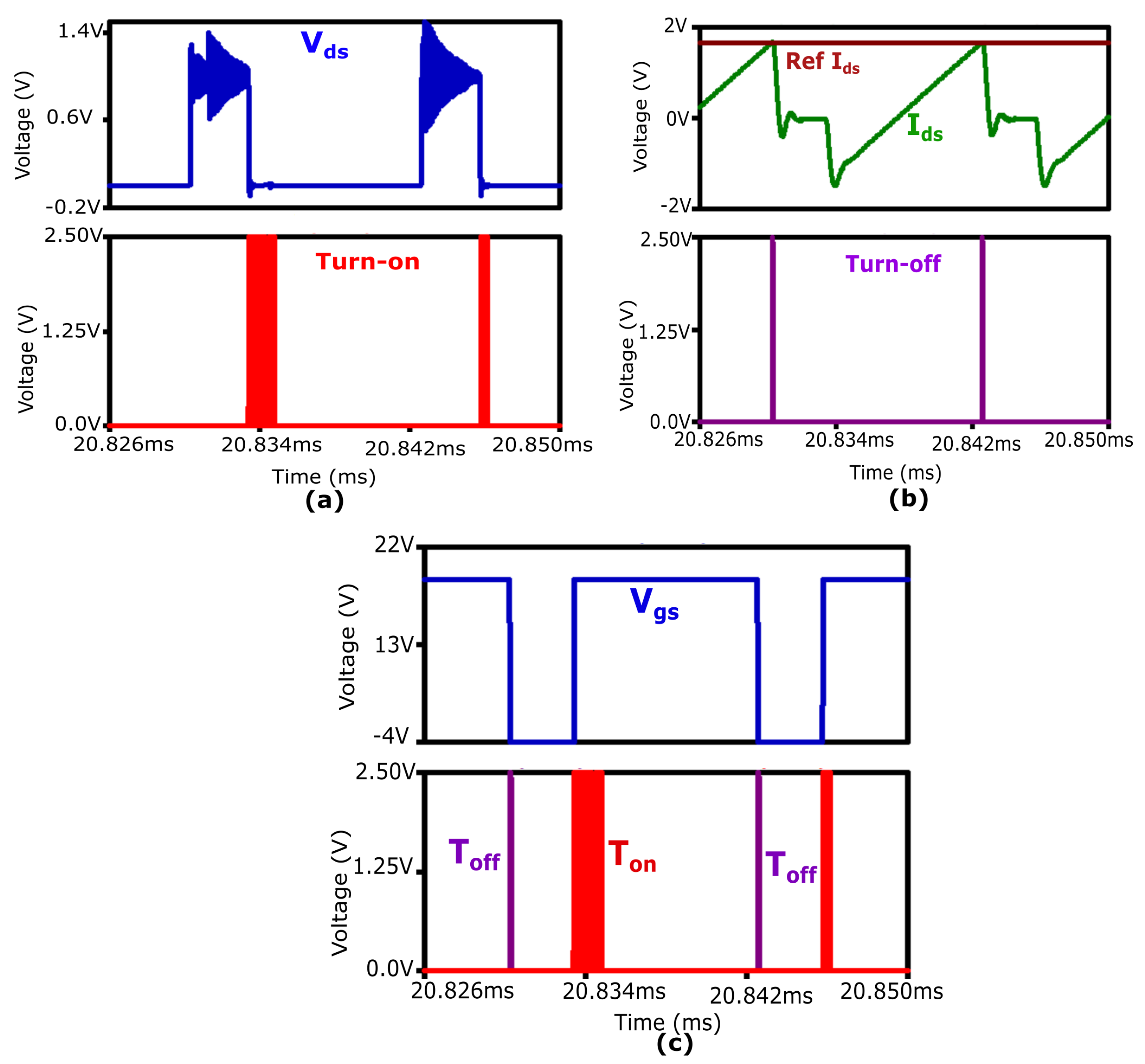
4.2. Operation of the Proposed AGD
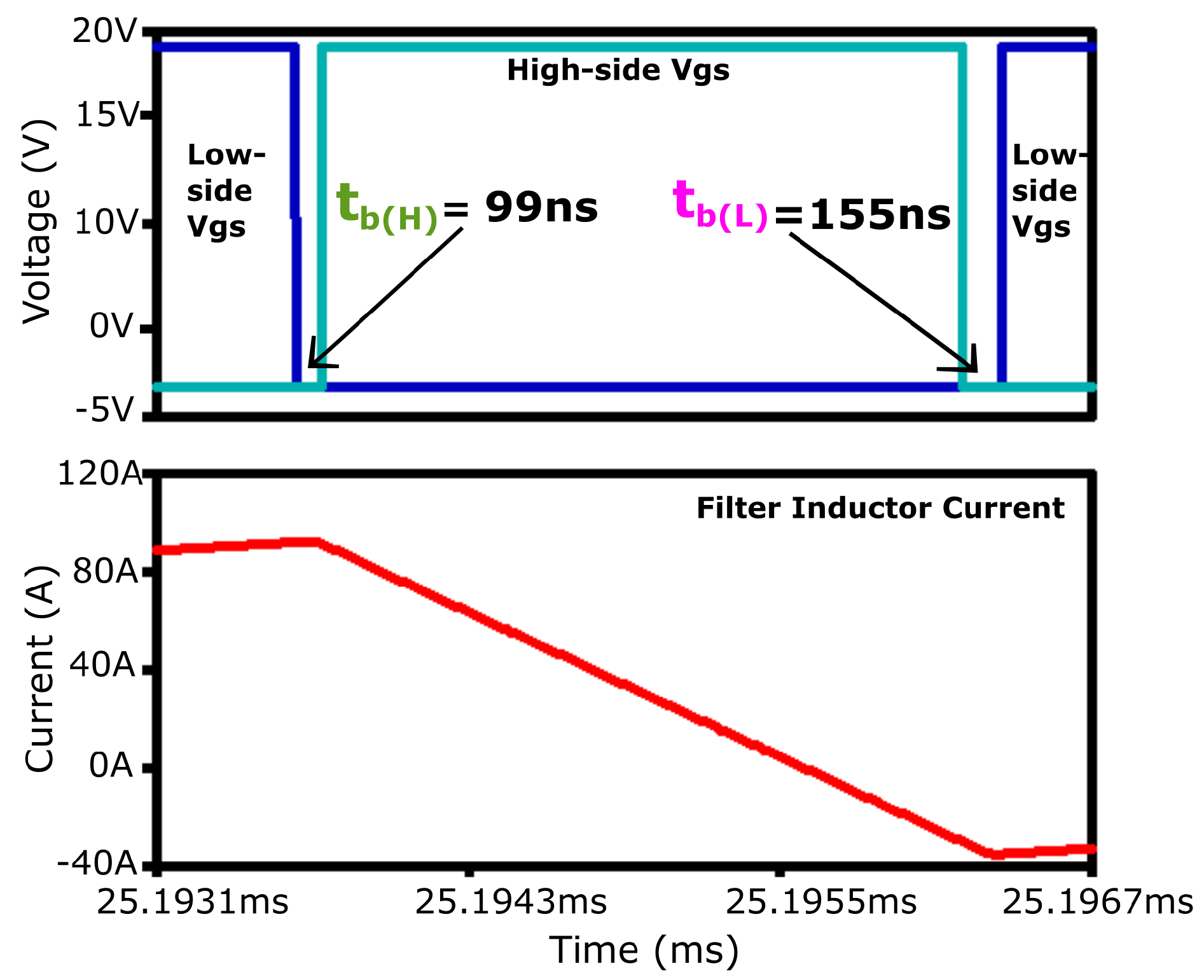
4.3. Impact of Inductor Current on Blanking Time
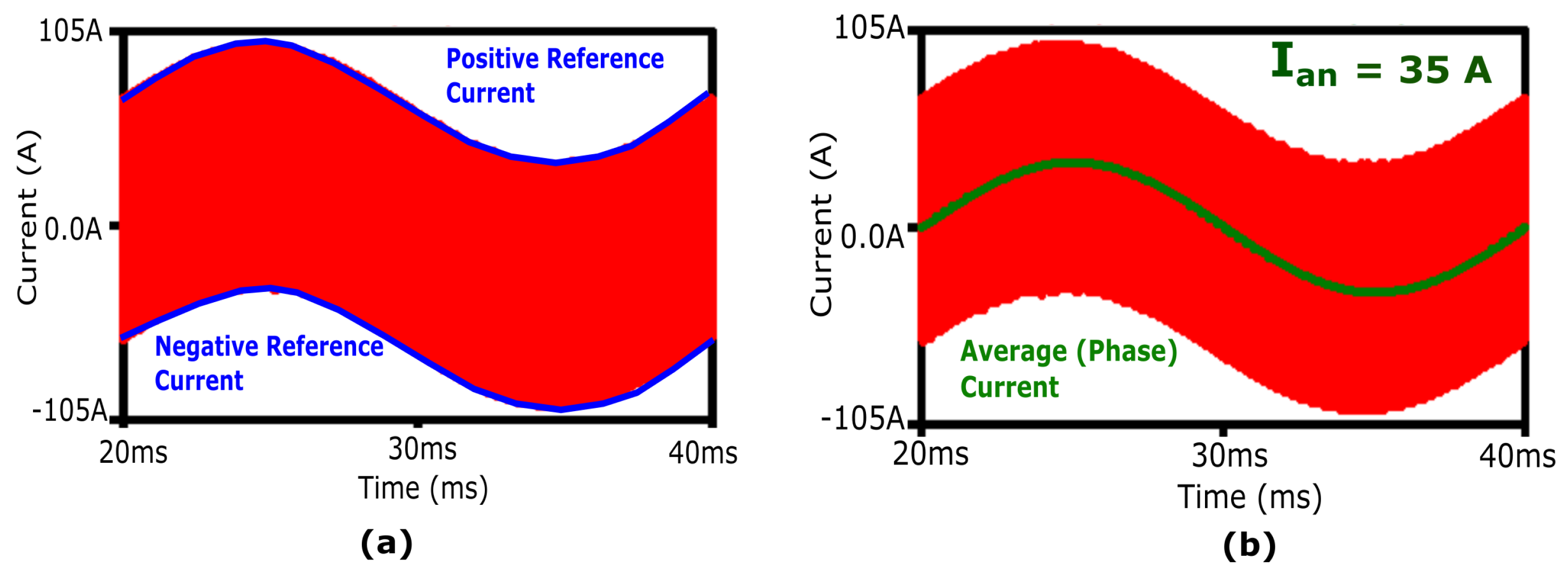
4.4. Reference Current and Inductor Current
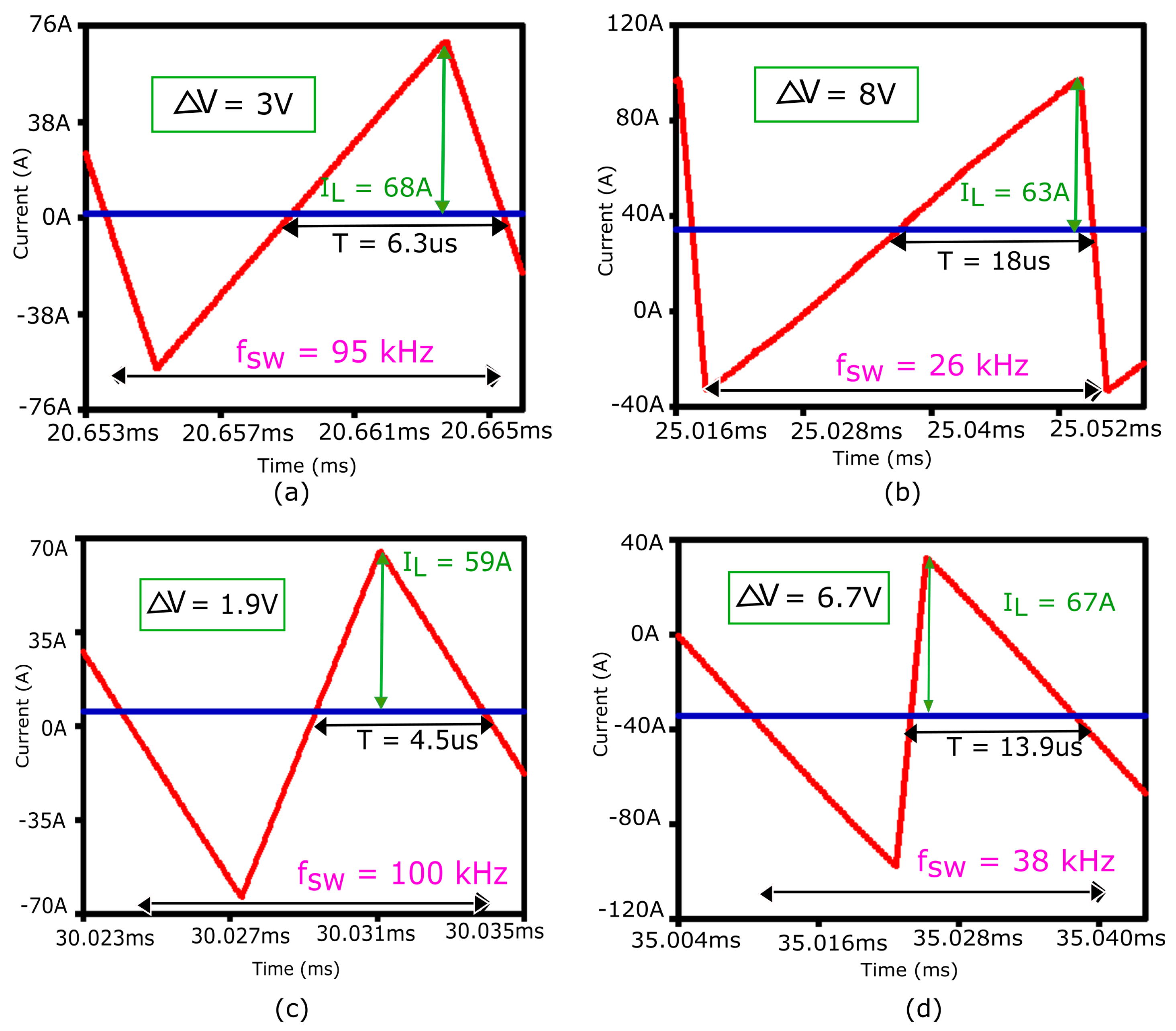
4.5. Effect of Variation in Switching Frequency on LC Filter
- At the zero crossing point, the switching frequency is maximum [46].
- At the peak of the fundamental line cycle, the switching frequency is minimum.
4.5.1. Ripple in Inductor Current
4.5.2. Ripple in Filter Voltage
- The minimum voltage ripple is at a high switching frequency which is at the zero crossings. The reason for this is the short time available to charge/discharge the capacitor by the inductor current.
- The maximum voltage ripple is at low switching and it is less than the desired voltage ripple.
4.6. LC Filter Power Losses
4.6.1. Filter Inductor Power Losses
- The copper loss arises from the resistance of the winding and is determined as follows:where represents the AC resistance and denotes the root mean square (rms) value of the inductor current.
- Iron loss, resulting from fluctuations in the magnetic field within the core, is divided into hysteresis loss and eddy current loss. Both hysteresis and eddy current losses are present, complicating accurate calculations. The hysteresis loop changes over the fundamental cycle of current, while eddy currents vary accordingly. Moreover, eddy currents are partly dependent on hysteresis, further complicating the calculation of iron losses. Therefore, for precise estimation of losses, it is preferable to measure them at various operating conditions and construct a model based on these measurements.
4.6.2. Filter Capacitor Power Losses
- Resistive losses: These losses occur as a result of the internal resistance of the capacitor. When current flows through the capacitor, it encounters resistance, leading to power dissipation in the form of heat. The formula to calculate power dissipation due to ESR is:where is the power dissipated due to ESR, and is the root mean square inductor current flowing through the capacitor.
- Dielectric losses: These losses occur due to the imperfect insulation properties of the dielectric material within the capacitor.
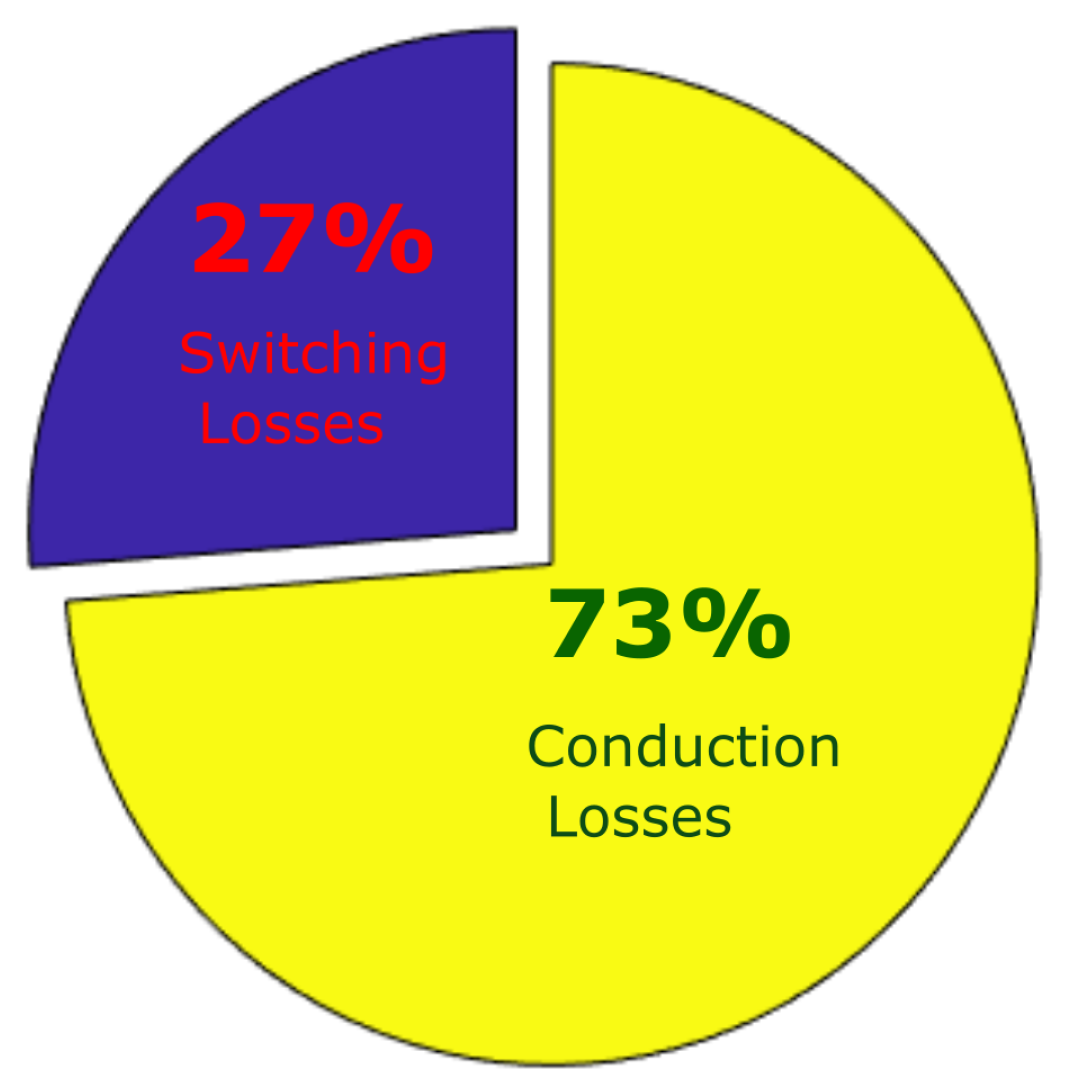
4.7. Power Losses in Inverter
4.7.1. Switching Losses
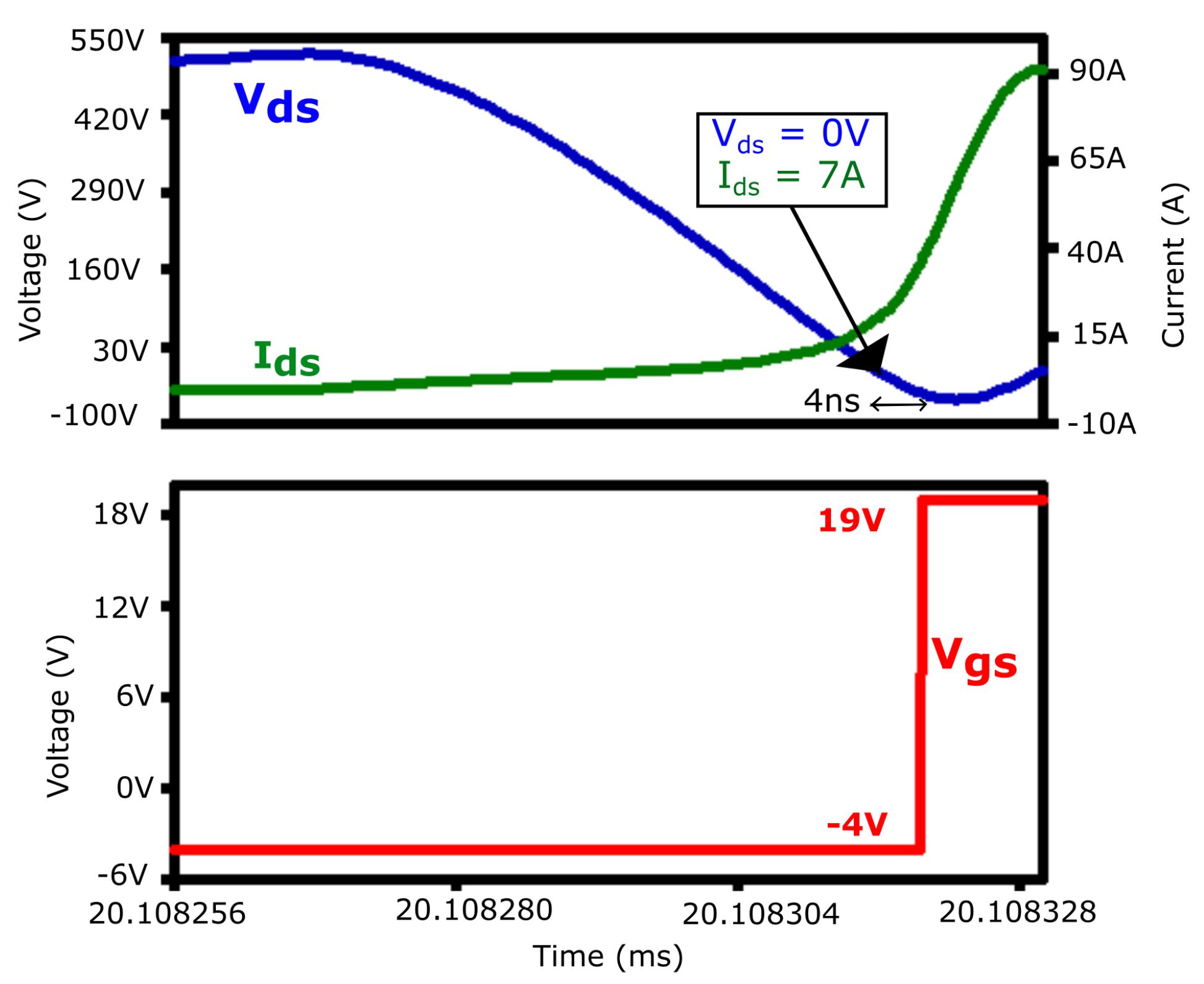
- Turn-On Switching LossesIn the proposed inverter, the turn-on switching losses are almost negligible due to the ZVS turn-on. Figure 16 illustrates that when the voltage across the switch is less than zero, the high-speed comparator of AGD detects it. After a delay of 4 nanoseconds, the AGD turns on the switch at zero voltage. Following the ZVS turn-on, the current starts flowing through the channel of the MOSFET.
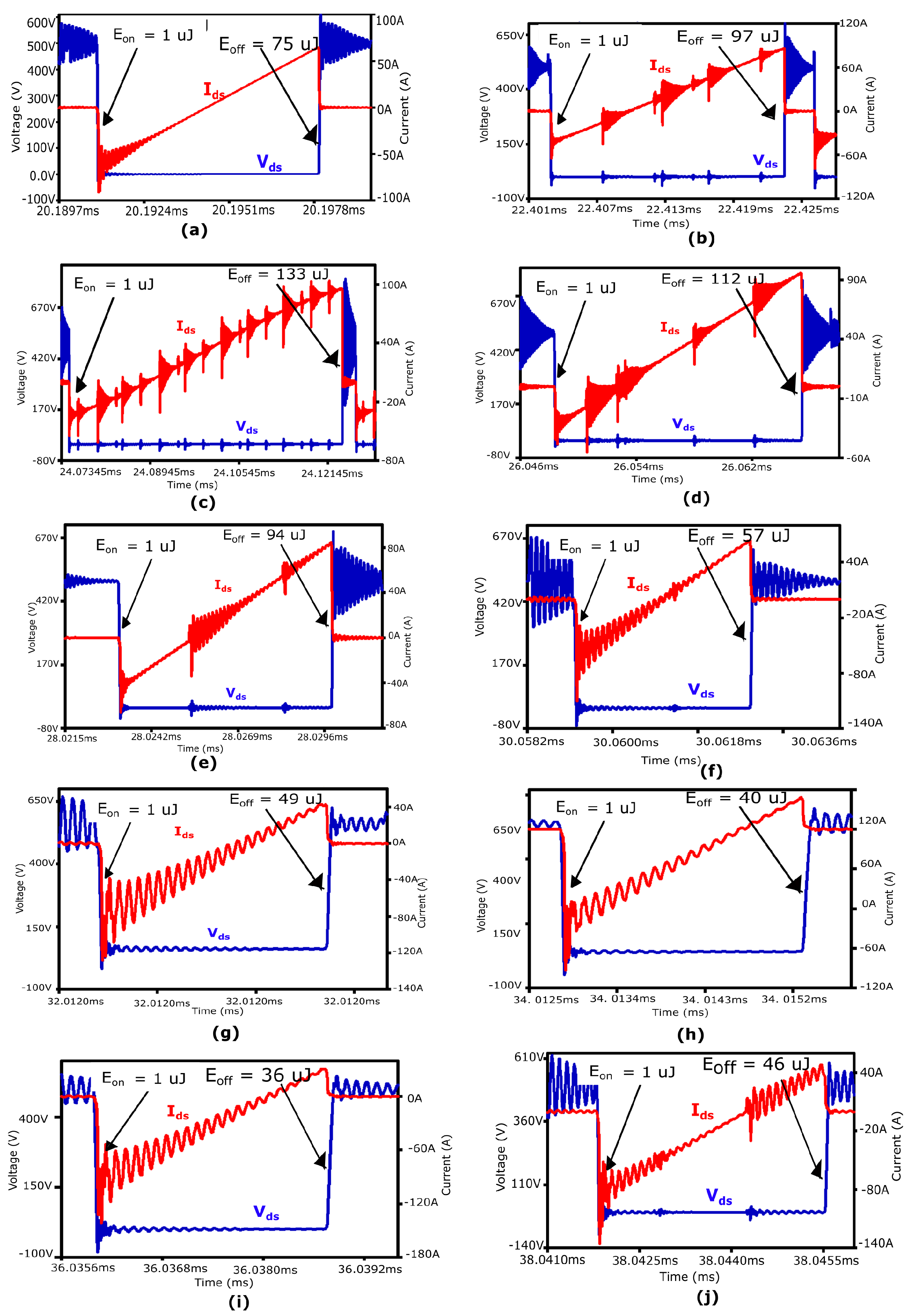
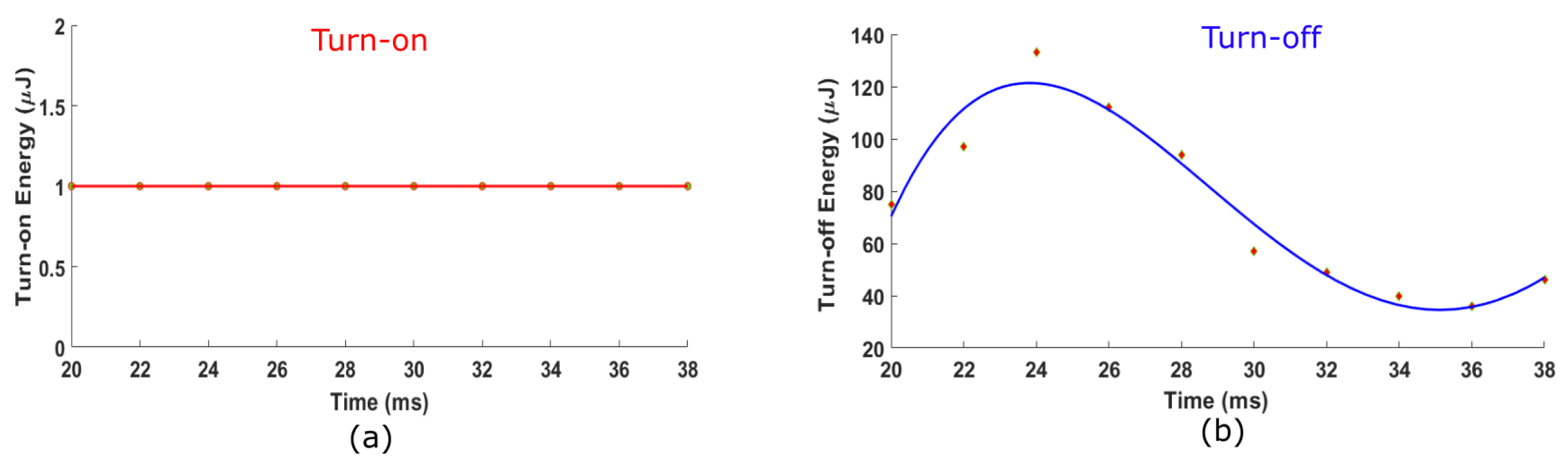
- Turn-Off Switching Losses
- The average switching energy for one switch is 75 J.
- The average switching frequency for one line cycle = 64 kHz
4.7.2. Conduction Losses
4.7.3. Total Inverter Power Losses
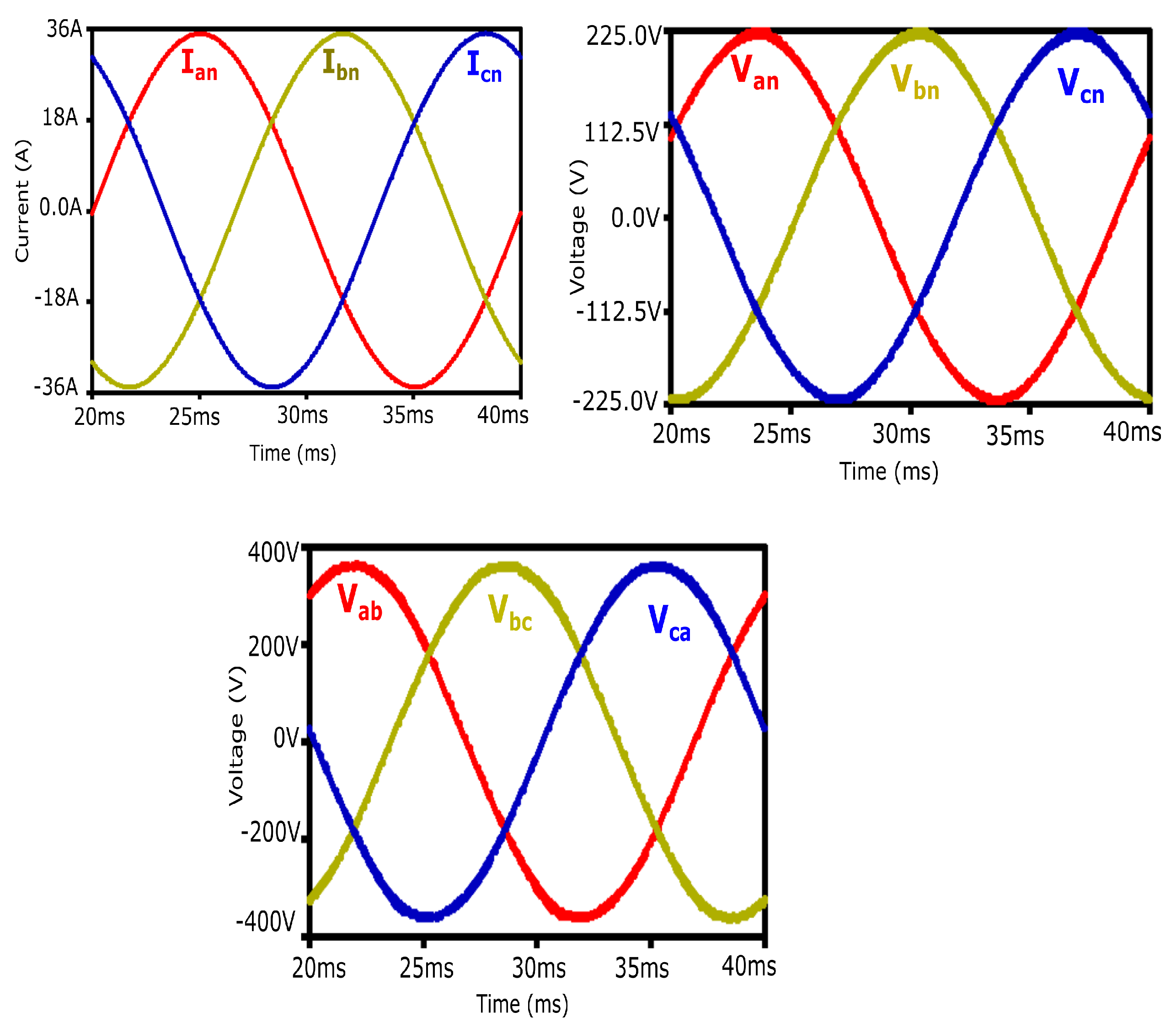
4.8. Sinusoidal Output Waveforms
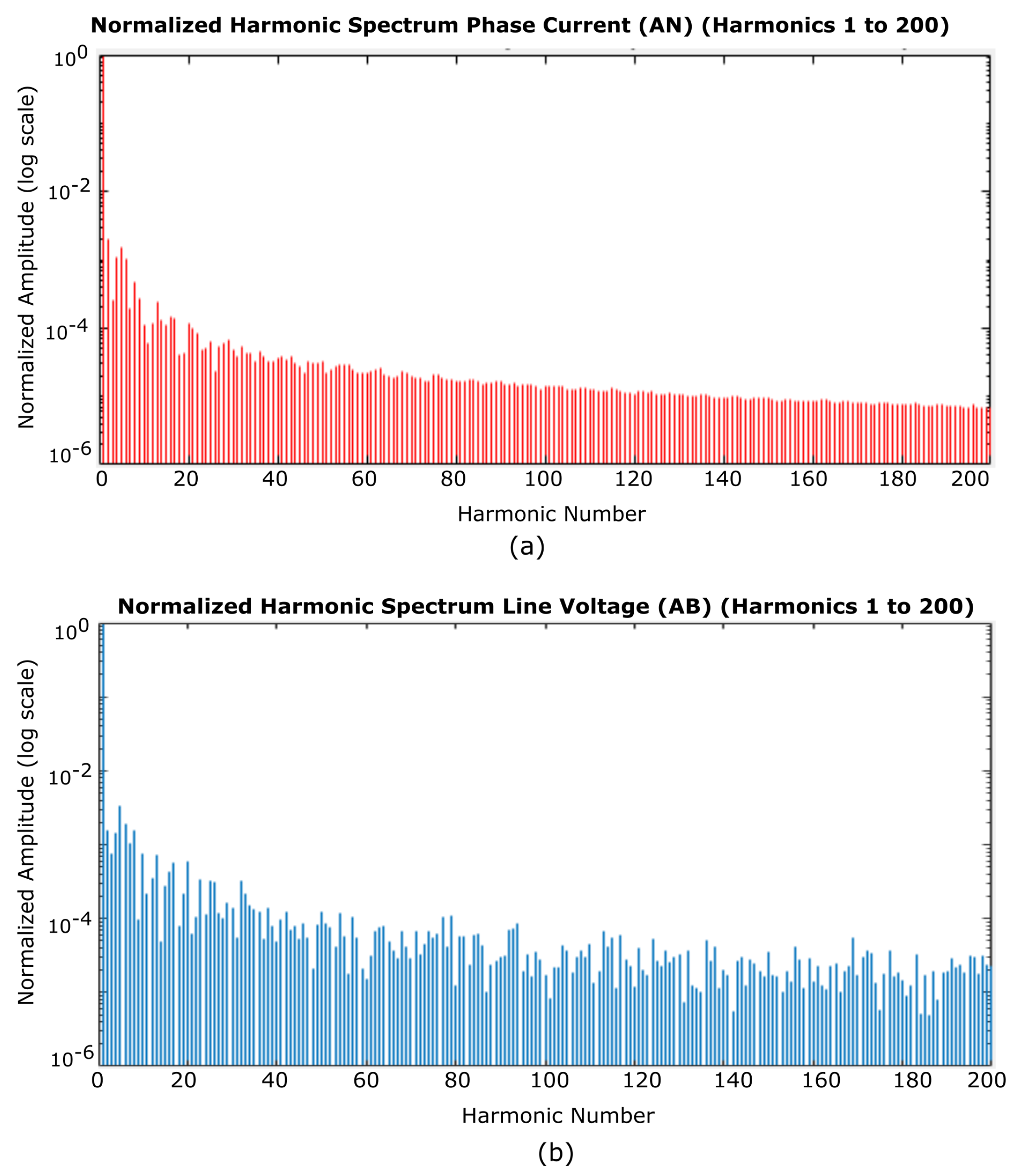
- For phase current (), the THD is 0.3%.
- For line voltage (), the THD is 0.5%.
4.9. Adaptive Control Features of Proposed AGDs
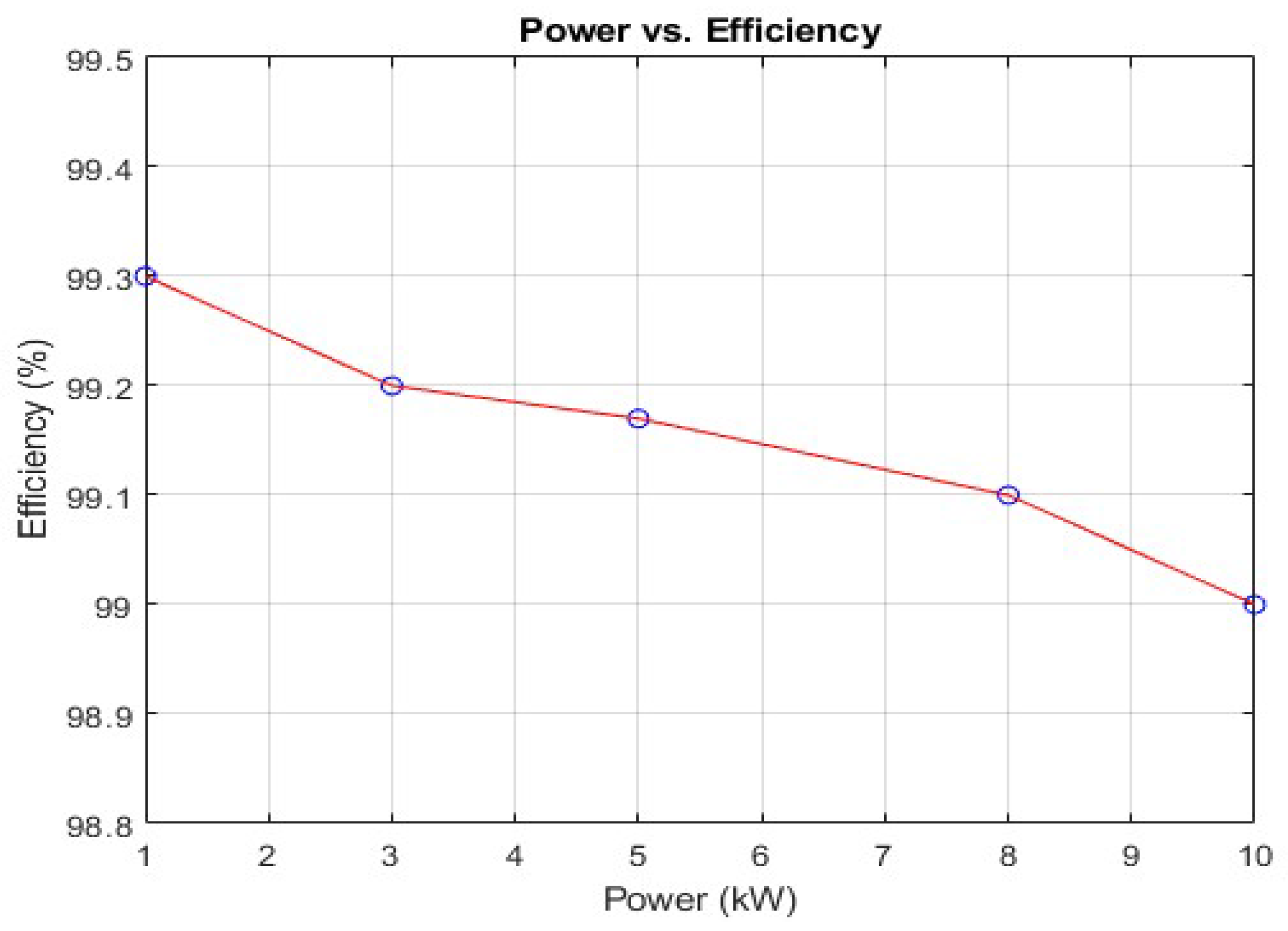
4.10. Efficiency
- By decreasing the reference value for the inductor current, the average value of the phase current decreases.
- The efficiency increases with a low reference value. This is due to a reduction in conduction losses as the filter inductor ripple is small now. There is a probability of reduction in turn-off losses as the current ripple is small and there is less overlapping of voltage and current at turn-off.
4.11. EMI Mitigation
4.12. Hardware Design Recommendations
4.12.1. New Capacitor Technology: Enhanced Inverter Performance
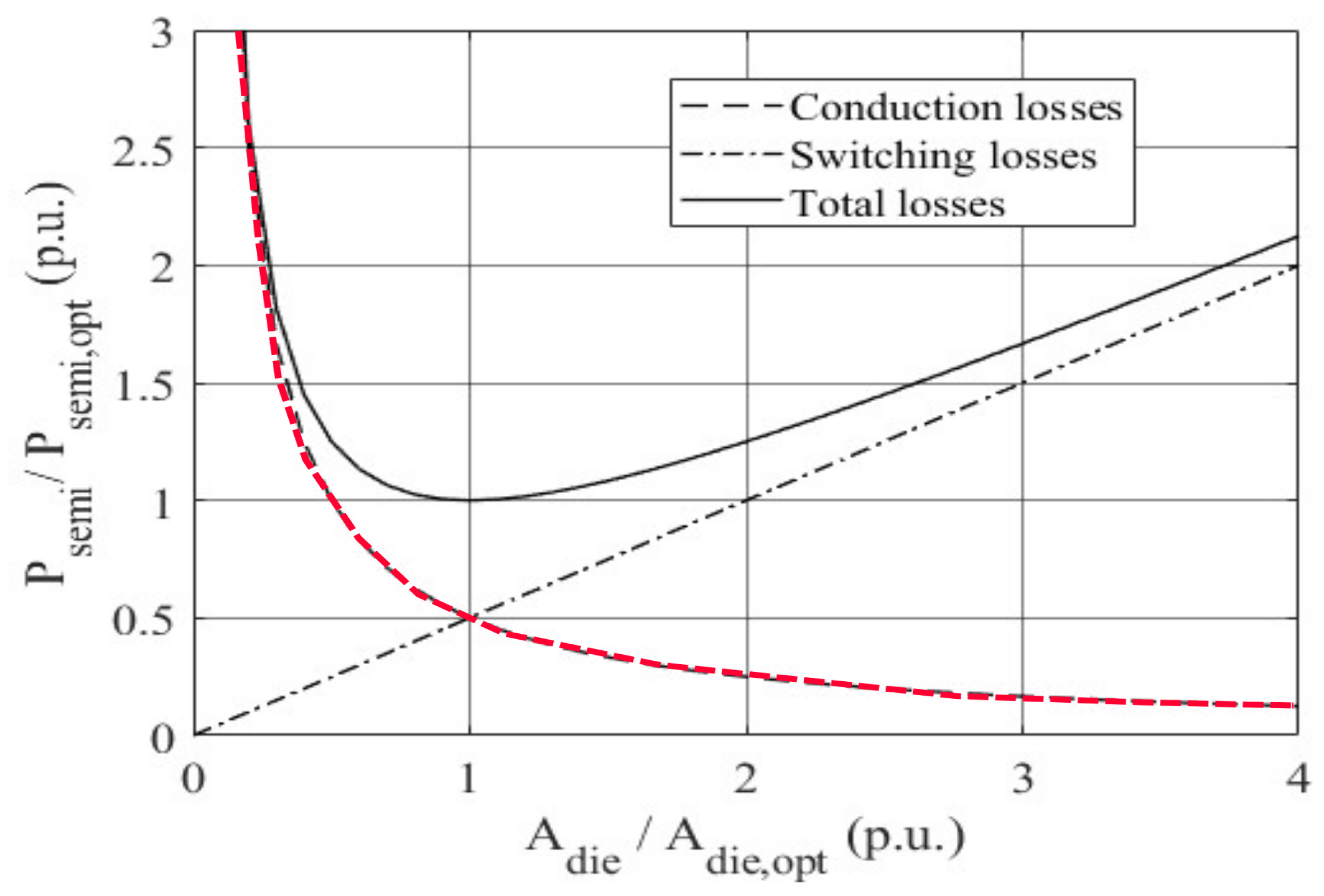
4.12.2. Impact of Increased Die Area on SiC MOSFET Performance and Losses
- Input Capacitance Increase: By increasing the input capacitance, the turn-on losses will increase for hard-switched converters. This is due to the large oscillations produced by the LC loop involving the die input capacitance and inductance of the circuit. These oscillations will increase the EMI issues. To dampen these LC oscillations, the gate resistance needs to be increased. However, it will reduce the switching speed of the converter and increase the turn-on switching losses. But for soft-switched converters, this enhanced input capacitance will not affect the turn-on switching losses as the switch turns on at ZVS.
- Output Capacitance Increase: By increasing the output capacitance, the turn-off losses will be reduced due to the less overlapping of and . Then there will be no need to use the external snubber capacitance as the elevated output capacitance will serve as snubber capacitance.
- Die Area Increase: By increasing the die area, the resistance of the channel decreases and it will reduce the conduction losses. Another way to reduce the conduction losses is to use multiple parallel-connected MOSFETs that will reduce the .
4.12.3. Filter Inductor Design
4.12.4. Mitigating EMI in EVs: Shielding Considerations
5. Conclusions
6. Future Work
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| AGDs | autonomous gate drivers |
| BCM | boundary conduction mode |
| drain-source capacitance | |
| CM | common mode |
| CCM | continuous conduction mode |
| CRM | critical conduction mode |
| DCM | discontinuous conduction mode |
| EMI | electromagnetic interference |
| ESR | equivalent series resistance |
| EVs | electric vehicles |
| IGBTs | insulated-gate bipolar transistors |
| MOSFETs | metal-oxide semiconductor field-effect transistors |
| PMSM | Permanent Magnet Synchronous Motor |
| SiC | silicon carbide |
| SSW | soft switching |
| TCM | triangular-current mode |
| THD | total harmonic distortion |
| drain-source voltage | |
| gate-source voltage | |
| ZCS | zero-current switching |
| ZVS | zero-voltage switching |
References
- Nitta, N.; Wu, F.; Lee, J.T.; Yushin, G. Li-ion battery materials: Present and future. Mater. Today 2015, 18, 252–264. [Google Scholar] [CrossRef]
- Kong, L.; Wang, L.; Zhu, J.; Bian, J.; Xia, W.; Zhao, R.; Lin, H.; Zhao, Y. Configuring solid-state batteries to power electric vehicles: A deliberation on technology, chemistry and energy. Chem. Commun. 2021, 57, 12587–12594. [Google Scholar] [CrossRef]
- Jan, W.; Khan, A.D.; Iftikhar, F.J.; Ali, G. Recent advancements and challenges in deploying lithium-sulfur batteries as economical energy storage devices. J. Energy Storage 2023, 72, 108559. [Google Scholar] [CrossRef]
- Mazumder, H.; Ektesabi, M.; Kapoor, A. Effect of mass distribution on cornering dynamics of retrofitted EV. In Proceedings of the 2012 IEEE International Electric Vehicle Conference, Greenville, SC, USA, 4–8 March 2012; pp. 1–6. [Google Scholar] [CrossRef]
- Kretschmar, K.; Nee, H.-P. An AC Converter with a Small DC link Capacitor for a 15 kW Permanent Magnet Synchronous Integral Motor. In Proceedings of the International Conference on Power Electronics and Variable Speed Drives, London, UK, 21–23 September 1998; pp. 622–625. [Google Scholar] [CrossRef]
- Miric, S.; Niklaus, P.S.; Huber, J.; Stäger, C.; Haider, M.; Kolar, J.W. Analysis and Experimental Verification of the EMI Signature of Three-Phase Three-Level TCM Soft-Switching Converter Systems. IEEE Access 2023, 11, 57391–57400. [Google Scholar] [CrossRef]
- Ranstad, P.; Nee, H.-P. On Dynamic Effects Influencing IGBT Losses in Soft-Switching Converters. IEEE Trans. Power Electron. 2011, 26, 260–271. [Google Scholar] [CrossRef]
- Shirabe, K.; Swamy, M.; Kang, J.-K.; Hisatsune, M.; Wu, Y.; Kebort, D.; Honea, J. Advantages of high frequency PWM in AC motor drive applications. In Proceedings of the 2012 IEEE Energy Conversion Congress and Exposition (ECCE), Raleigh, NC, USA, 15–20 September 2012; pp. 2977–2984. [Google Scholar] [CrossRef]
- Nee, H.-P.; Kolar, J.W.; Friedrichs, P.; Rabkowski, J. Editorial: Special Issue on Wide Bandgap Power Devices and Their Applications. IEEE Trans. Power Electron. 2014, 29, 2153–2154. [Google Scholar] [CrossRef]
- Ranstad, P.; Nee, H.-P.; Linner, J.; Peftitsis, D. An Experimental Evaluation of SiC Switches in Soft-Switching Converters. IEEE Trans. Power Electron. 2014, 29, 2527–2538. [Google Scholar] [CrossRef]
- Zhang, L.; Yuan, X.; Wu, X.; Shi, C.; Zhang, J.; Zhang, Y. Performance evaluation of high-power SiC MOSFET modules in comparison to si IGBT modules. IEEE Trans. Power Electron. 2019, 34, 1181–1196. [Google Scholar] [CrossRef]
- Yan, Q.; Yuan, X.; Geng, Y.; Charalambous, A.; Wu, X. Performance evaluation of split output converters with SiC MOSFETs and SiC schottky diodes. IEEE Trans. Power Electron. 2017, 32, 406–422. [Google Scholar] [CrossRef]
- Haider, M.; Niklaus, P.S.; Madlener, M.; Rohner, G.; Kolar, J.W. Comparative Evaluation of Gate Driver and LC-Filter Based dv/dt-Limitation for SiC-Based Motor-Integrated Variable Speed Drive Inverters. IEEE Open J. Power Electron. 2023, 4, 450–462. [Google Scholar] [CrossRef]
- Jia, X.; Hu, C.; Dong, B.; He, F.; Wang, H.; Xu, D. Influence of system layout on CM EMI noise of SiC electric vehicle powertrains. CPSS Trans. Power Electron. Appl. 2021, 6, 298–309. [Google Scholar] [CrossRef]
- He, Y.; Zhu, J.; Zhou, B.; Zhao, X. EMI Issues and Reduction in 800 V Electrical Vehicle Systems by Incorporating Cable Effects. In Proceedings of the 2023 IEEE 6th Student Conference on Electric Machines and Systems (SCEMS), Huzhou, China, 7–9 December 2023; pp. 1–5. [Google Scholar] [CrossRef]
- Bosi, M.; Campanini, A.; Peretto, L.; Sanchez, A.M.; Pajares, F.J. Measurement equipment and optimal approach for power line filter design for automotive. In Proceedings of the 2022 IEEE International Workshop on Metrology for Automotive (MetroAutomotive), Modena, Italy, 4–6 July 2022; pp. 53–58. [Google Scholar] [CrossRef]
- Rao, H.; Zhou, B.; Zhang, F.; Xu, Y.; Hong, C.; Yang, J. Electromagnetic Compatibility of Power Electronic Devices in Electric Vehicle Drive System. In Proceedings of the 2021 IEEE 4th International Conference on Electronics Technology (ICET), Chengdu, China, 7–10 May 2021; pp. 509–512. [Google Scholar] [CrossRef]
- Xu, D.; Li, R.; He, N.; Deng, J.; Wu, Y. Soft-Switching Technology for Three-Phase Power Electronics Converters; Wiley: Hoboken, NJ, USA, 2021. [Google Scholar]
- Henze, C.; Martin, H.; Parsley, D. Zero-voltage switching in high frequency power converters using pulse width modulation. In Proceedings of the 3rd Annual IEEE Applied Power Electronics Conference and Exposition, New Orleans, LA, USA, 1–5 February 1988; pp. 33–40. [Google Scholar] [CrossRef]
- Xu, S.; Cao, B.; Chang, L.; Shao, R. Pulse Energy Modulation for a Single-Phase Bridge Inverter with Active Power Decoupling Capability. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 9, 2014–2026. [Google Scholar] [CrossRef]
- Su, B.; Zhang, J.; Lu, Z. Totem-Pole Boost Bridgeless PFC Rectifier with Simple Zero-Current Detection and Full-Range ZVS Operating at the Boundary of DCM/CCM. IEEE Trans. Power Electron. 2011, 26, 427–435. [Google Scholar] [CrossRef]
- Cho, J.; Hu, D.; Cho, G. Three phase sine wave voltage source inverter using the soft switched resonant poles. In Proceedings of the 15th Annual Conference IEEE Industrial Electronics Society, Philadelphia, PA, USA, 6–10 November 1989; pp. 48–53. [Google Scholar] [CrossRef]
- Haryani, N.; Ohn, S.J.; Hu, J.; Rankin, P.; Burgos, R.; Boroyevich, D. A Novel ZVS Turn-on Triangular Current Mode Control with Phase Synchronization for Three Level Inverters. In Proceedings of the 2018 IEEE Energy Conversion Congress and Exposition (ECCE), Portland, OR, USA, 23–27 September 2018; pp. 2207–2214. [Google Scholar] [CrossRef]
- Cimendag, A.; Duman, T.; Yilmaz, M. 250W GaN Hybrid BCM Micro-inverter Design for Avionic Applications. In Proceedings of the 2022 IEEE Applied Power Electronics Conference and Exposition (APEC), Houston, TX, USA, 20–24 March 2022; pp. 838–842. [Google Scholar] [CrossRef]
- Marxgut, C.; Biela, J.; Kolar, J.W. Interleaved Triangular Current Mode (TCM) resonant transition single phase PFC rectifier with high efficiency and high power density. In Proceedings of the 2010 International Power Electronics Conference—ECCE Asia—IPEC, Sapporo, Japan, 21–24 June 2010; pp. 1725–1732. [Google Scholar] [CrossRef]
- Marxgut, C.; Krismer, F.; Bortis, D.; Kolar, J.W. Ultraflat Interleaved Triangular Current Mode (TCM) Single-Phase PFC Rectifier. IEEE Trans. Power Electron. 2014, 29, 873–882. [Google Scholar] [CrossRef]
- Haryani, N.; Sun, B.; Burgos, R. A novel soft switching ZVS sinusoidal input boundary current mode control of 6-switch three phase 2-level boost rectifier for active and active + reactive power generation. In Proceedings of the IEEE Applied Power Electronics Conference and Exposition (APEC), San Antonio, TX, USA, 4–8 March 2018; pp. 8–15. [Google Scholar] [CrossRef]
- Amirahmadi, A.; Hu, H.; Grishina, A.; Zhang, Q.; Chen, L.; Somani, U.; Batarseh, I. Hybrid ZVS BCM current controlled three-phase microinverter. IEEE Trans. Power Electron. 2014, 29, 2124–2134. [Google Scholar] [CrossRef]
- Chen, J.; Sha, D.; Zhang, J.; Liao, X. An SiC MOSFET based three-phase ZVS inverter employing variable switching frequency space vector PWM control. IEEE Trans. Power Electron. 2019, 34, 6320–6331. [Google Scholar] [CrossRef]
- Haryani, N.; Sun, B.; Burgos, R. ZVS turn-on triangular current mode (TCM) control for three phase 2-level inverters with reactive power control. In Proceedings of the IEEE Energy Conversion Congress and Exposition (ECCE), Portland, OR, USA, 23–27 September 2018; pp. 4940–4947. [Google Scholar] [CrossRef]
- Huang, Z.; Li, Q.; Lee, F.C. Digital-based soft-switching modulation for high-frequency three-phase inverters with reactive power transfer capability. In Proceedings of the IEEE Energy Conversion Congress and Exposition (ECCE), Portland, OR, USA, 23–27 September 2018; pp. 6751–6758. [Google Scholar] [CrossRef]
- Huang, Z.; Liu, Z.; Lee, F.C.; Li, Q. Critical-mode-based soft-switching modulation for high-frequency three-phase bidirectional AC–DC converters. IEEE Trans. Power Electron. 2019, 34, 3888–3898. [Google Scholar] [CrossRef]
- De Doncker, R.W.; Lyons, J. The auxiliary resonant commutated pole converter. In Proceedings of the Conference Record of the 1990 IEEE—Industry Applications Society Annual Meeting 1990, Seattle, WA, USA, 7–12 October 1990; Volume 2, pp. 1228–1235. [Google Scholar] [CrossRef]
- Haider, M.; Anderson, J.A.; Nain, N.; Zulauf, G.; Kolar, J.W.; Xu, D.M.; Deboy, G. Analytical Calculation of the Residual ZVS Losses of TCM-Operated Single-Phase PFC Rectifiers. IEEE Open J. Power Electron. 2021, 2, 250–264. [Google Scholar] [CrossRef]
- Anderson, J.A.; Zulauf, G.; Kolar, J.W.; Deboy, G. New Figure-of-Merit Combining Semiconductor and Multi-Level Converter Properties. IEEE Open J. Power Electron. 2020, 1, 322–338. [Google Scholar] [CrossRef]
- Zhang, D.; Zhang, Q.; Hu, H.; Grishina, A.; Shen, J.; Batarseh, I. High efficiency current mode control for three-phase micro-inverters. In Proceedings of the 2012 Twenty-Seventh Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Orlando, FL, USA, 5–9 February 2012; pp. 892–897. [Google Scholar] [CrossRef]
- Sadik, D.-P.; Heinig, S.; Jacobs, K.; Johannesson, D.; Lim, J.-K.; Nawaz, M.; Dijkhuizen, F.; Bakowski, M.; Norrga, S.; Nee, H.-P. Investigation of the surge current capability of the body diode of SiC MOSFETs for HVDC applications. In Proceedings of the 2016 18th European Conference on Power Electronics and Applications (EPE’16 ECCE Europe), Karlsruhe, Germany, 5–9 September 2016; pp. 1–10. [Google Scholar]
- Agrawal, B.; Preindl, M.; Emadi, A. Turn-off energy minimization for soft-switching power converters with wide bandgap devices. In Proceedings of the 2017 IEEE International Conference on Industrial Technology (ICIT), Toronto, ON, Canada, 22–25 March 2017; pp. 236–241. [Google Scholar] [CrossRef]
- Wang, Q.; Burgos, R. A Method for Increasing Modulation Index of Three Phase Triangular Conduction Mode Converter. In Proceedings of the 2018 IEEE 19th Workshop on Control and Modeling for Power Electronics (COMPEL), Padua, Italy, 25–28 June 2018; pp. 1–5. [Google Scholar] [CrossRef]
- Serban, I. Power Decoupling Method for Single-Phase H-Bridge Inverters with No Additional Power Electronics. IEEE Trans. Ind. Electron. 2015, 62, 4805–4813. [Google Scholar] [CrossRef]
- Eull, M.; Preindl, M. A Soft Switching Inverter Minimizing Bearing Currents in 800V Electric Vehicle Drives. In Proceedings of the 2021 IEEE 13th International Symposium on Diagnostics for Electrical Machines, Power Electronics and Drives (SDEMPED), Dallas, TX, USA, 22–25 August 2021; pp. 457–463. [Google Scholar] [CrossRef]
- Eull, M.; Zhou, L.; Jahnes, M.; Preindl, M. Bidirectional Nonisolated Fast Charger Integrated in the Electric Vehicle Traction Drivetrain. IEEE Trans. Transp. Electrif. 2022, 8, 180–195. [Google Scholar] [CrossRef]
- Zhou, L.; Eull, M.; Wang, W.; Preindl, M. Modular Model-Predictive Control with Regulated Third-Harmonic Injection for Zero-Sequence Stabilized Inverter. IEEE Trans. Ind. Appl. 2022, 58, 7634–7647. [Google Scholar] [CrossRef]
- Tang, Y.; Yao, W.; Loh, P.C.; Blaabjerg, F. Highly Reliable Transformerless Photovoltaic Inverters with Leakage Current and Pulsating Power Elimination. IEEE Trans. Ind. Electron. 2016, 63, 1016–1026. [Google Scholar] [CrossRef]
- Abbas, K.; Nee, H.-P.; Kostov, K. Autonomously Modulating Gate Drivers for Triangular-Current Mode (TCM) Zero-Voltage Switching (ZVS) Buck Converter. In Proceedings of the 2023 22nd International Symposium on Power Electronics (Ee), Novi Sad, Serbia, 25–28 October 2023; pp. 1–6. [Google Scholar] [CrossRef]
- Fan, B.; Wang, Q.; Burgos, R.; Ismail, A.; Boroyevich, D. Adaptive hysteresis current based ZVS modulation and voltage gain compensation for high-frequency three-phase converters. IEEE Trans. Power Electron. 2021, 36, 1143–1156. [Google Scholar] [CrossRef]
- Mohan, N.; Undeland, T.M.; Robbins, W.P. Power Electronics: Converters, Applications, and Design; John Wiley & Sons: Hoboken, NJ, USA, 2003. [Google Scholar]
- Rabkowski, J.; Peftitsis, D.; Nee, H.-P. Design Steps Toward a 40-kVA SiC JFET Inverter with Natural-Convection Cooling and an Efficiency Exceeding 99.5%. IEEE Trans. Ind. Appl. 2013, 49, 1589–1598. [Google Scholar] [CrossRef]
- Neumayr, D.; Bortis, D.; Kolar, J.W.; Koini, M.; Konrad, J. Comprehensive Large-Signal Performance Analysis of Ceramic Capacitors for Power Pulsation Buffers. In Proceedings of the 17th IEEE Workshop on Control and Modeling of Power Electronics (COMPEL), Trondheim, Norway, 27–30 June 2016. [Google Scholar] [CrossRef]
- Burkart, R.M.; Uemura, H.; Kolar, J.W. Optimal inductor design for 3-phase voltage-source PWM converters considering different magnetic materials and a wide switching frequency range. In Proceedings of the 2014 International Power Electronics Conference (IPEC-Hiroshima 2014—ECCE ASIA), Hiroshima, Japan, 18–21 May 2014; pp. 891–898. [Google Scholar] [CrossRef]
- Nielsen, A.B.; Davari, P.; Blaabjerg, F.; Nielsen, B.V. Power Density and Loss Optimization Design Methodology of a 10 kW 2-Level 3-Phase SiC Inverter. In Proceedings of the 2020 IEEE 21st Workshop on Control and Modeling for Power Electronics (COMPEL), Aalborg, Denmark, 9–12 November 2020; pp. 1–7. [Google Scholar] [CrossRef]






| Parameters | Values |
|---|---|
| SiC MOSFET | C3M0016120K |
| On-state resistance () | 16 m |
| Fundamental frequency () | 50 Hz |
| Input voltage | 500 |
| Power rating | 10 kW |
| Modulation index (m) | 0.9 |
| Filter inductance | 9 H |
| Filter capacitance | 80 F |
| Snubber capacitance | 2 nF |
| Phase voltage (peak) | 225 V |
| Phase current (peak) | 35 A |
| Parameters | Values |
|---|---|
| Power factor () | 0.9 |
| Resistance (R) | 5.63 |
| Inductance (L) | 8.83 mH |
| Time (ms) | Switching Frequency (kHz ) |
|---|---|
| 20 | 95 |
| 25 | 26 |
| 30 | 100 |
| 35 | 38 |
| Phase Current (A) | (W) | (W) | (W) | Efficiency |
|---|---|---|---|---|
| 35 | 10,287 | 10,185 | 102 | 99.00% |
| 31 | 8451 | 8375 | 76 | 99.10% |
| 26 | 5838 | 5790 | 48 | 99.17% |
| 20 | 3690 | 3660 | 30 | 99.20% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abbas, K.; Nee, H.-P. Autonomous Gate Drivers Tailored for Triangular Current Mode-Based Zero-Voltage Switching Two-Level Three-Phase Inverters for Electric Vehicle Drive Systems. Energies 2024, 17, 1060. https://doi.org/10.3390/en17051060
Abbas K, Nee H-P. Autonomous Gate Drivers Tailored for Triangular Current Mode-Based Zero-Voltage Switching Two-Level Three-Phase Inverters for Electric Vehicle Drive Systems. Energies. 2024; 17(5):1060. https://doi.org/10.3390/en17051060
Chicago/Turabian StyleAbbas, Khizra, and Hans-Peter Nee. 2024. "Autonomous Gate Drivers Tailored for Triangular Current Mode-Based Zero-Voltage Switching Two-Level Three-Phase Inverters for Electric Vehicle Drive Systems" Energies 17, no. 5: 1060. https://doi.org/10.3390/en17051060
APA StyleAbbas, K., & Nee, H.-P. (2024). Autonomous Gate Drivers Tailored for Triangular Current Mode-Based Zero-Voltage Switching Two-Level Three-Phase Inverters for Electric Vehicle Drive Systems. Energies, 17(5), 1060. https://doi.org/10.3390/en17051060






