Measurement Error in Thermoelectric Generator Induced by Temperature Fluctuation
Abstract
1. Introduction
2. Theoretical Analysis and Experimental Method
2.1. Theoretical Solution
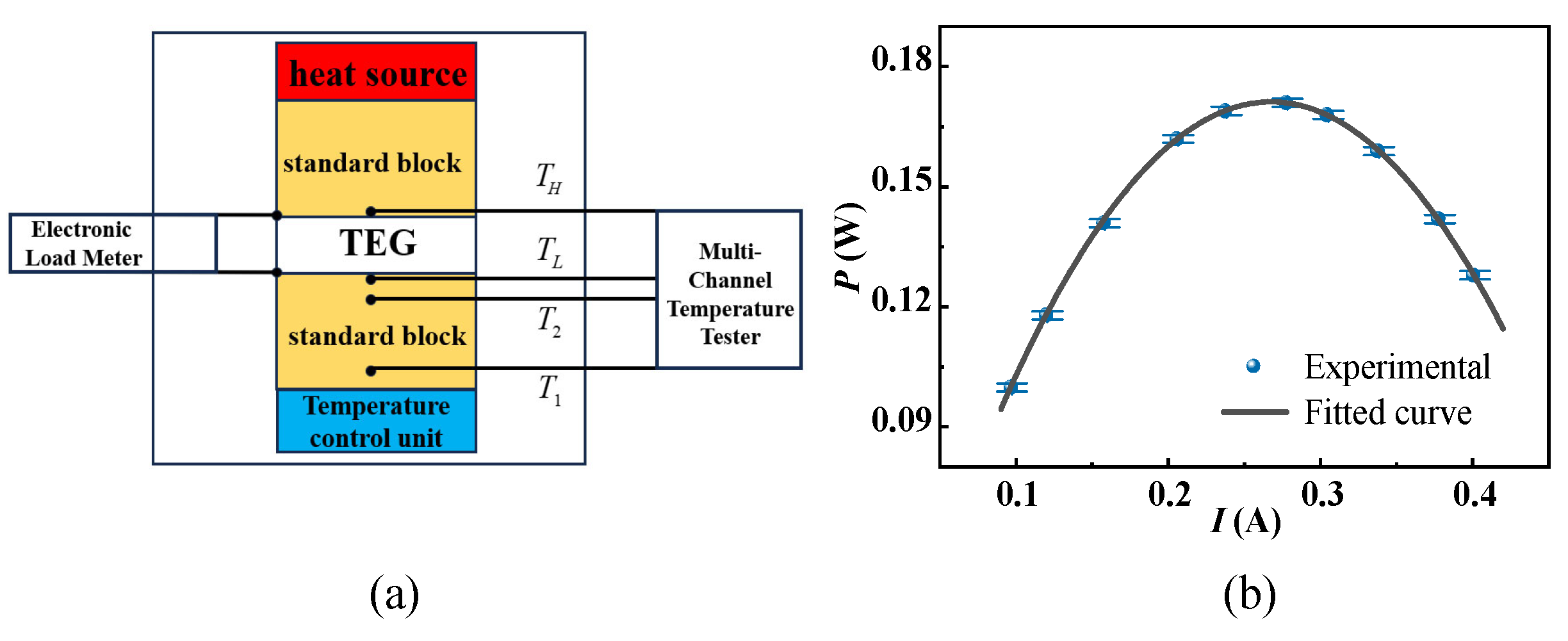
2.2. Experimental Method
3. Results and Discussion
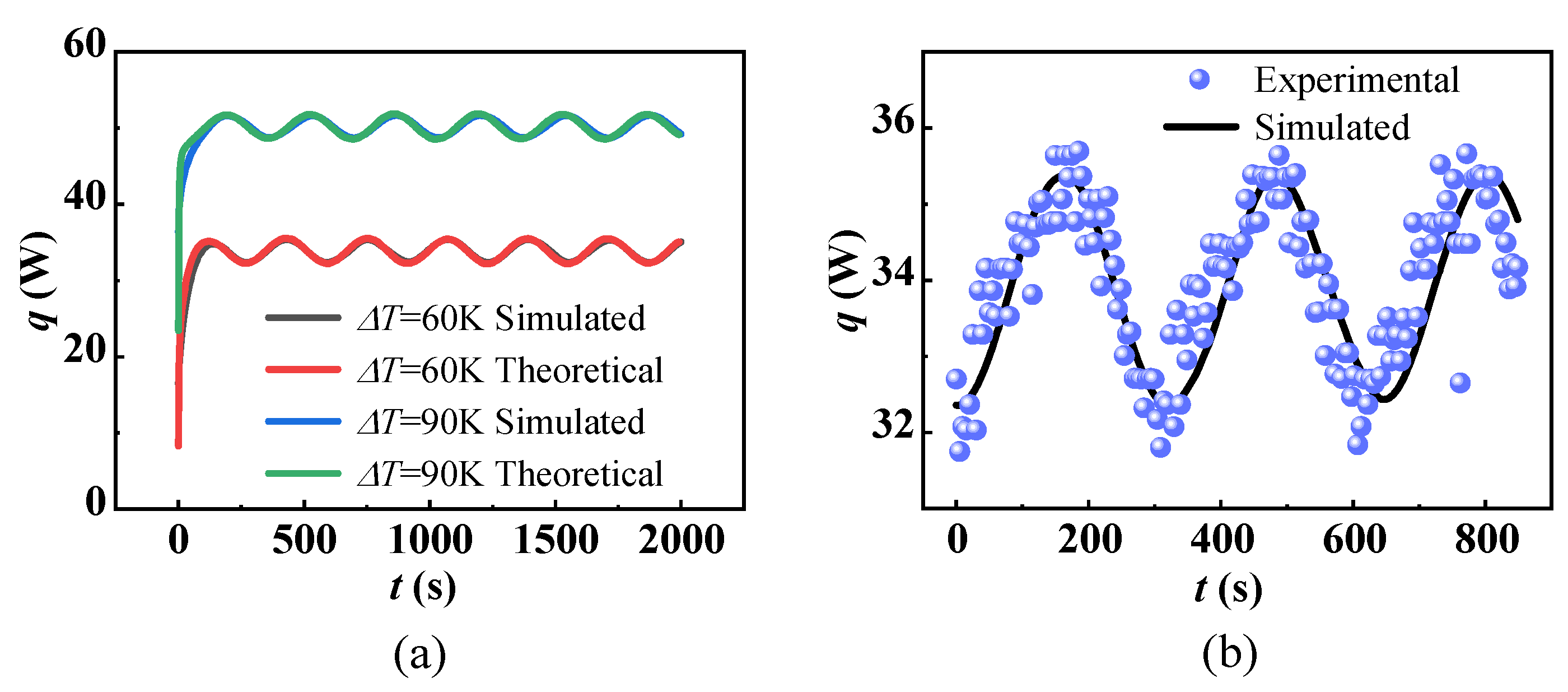
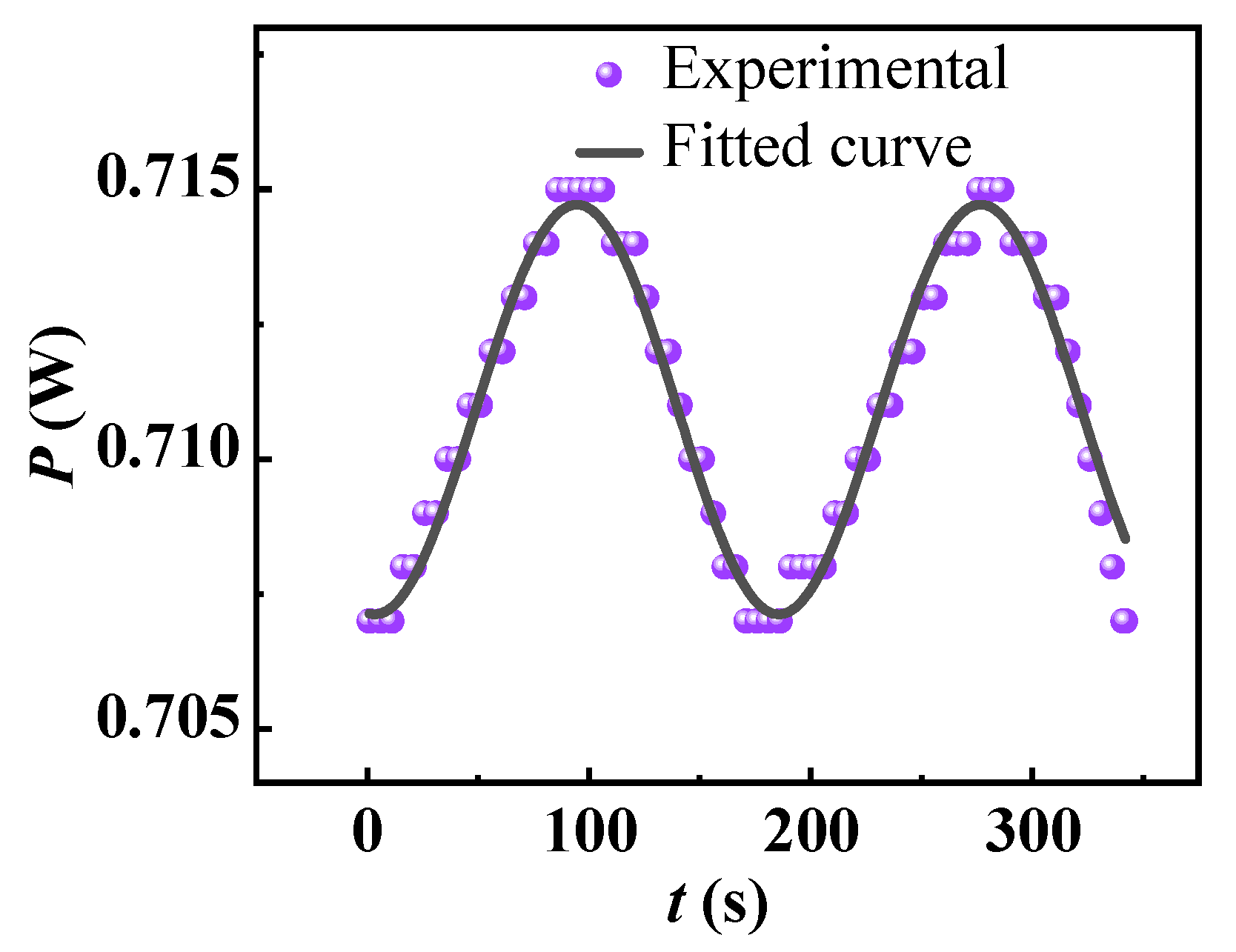
3.1. Theoretical Model Validation
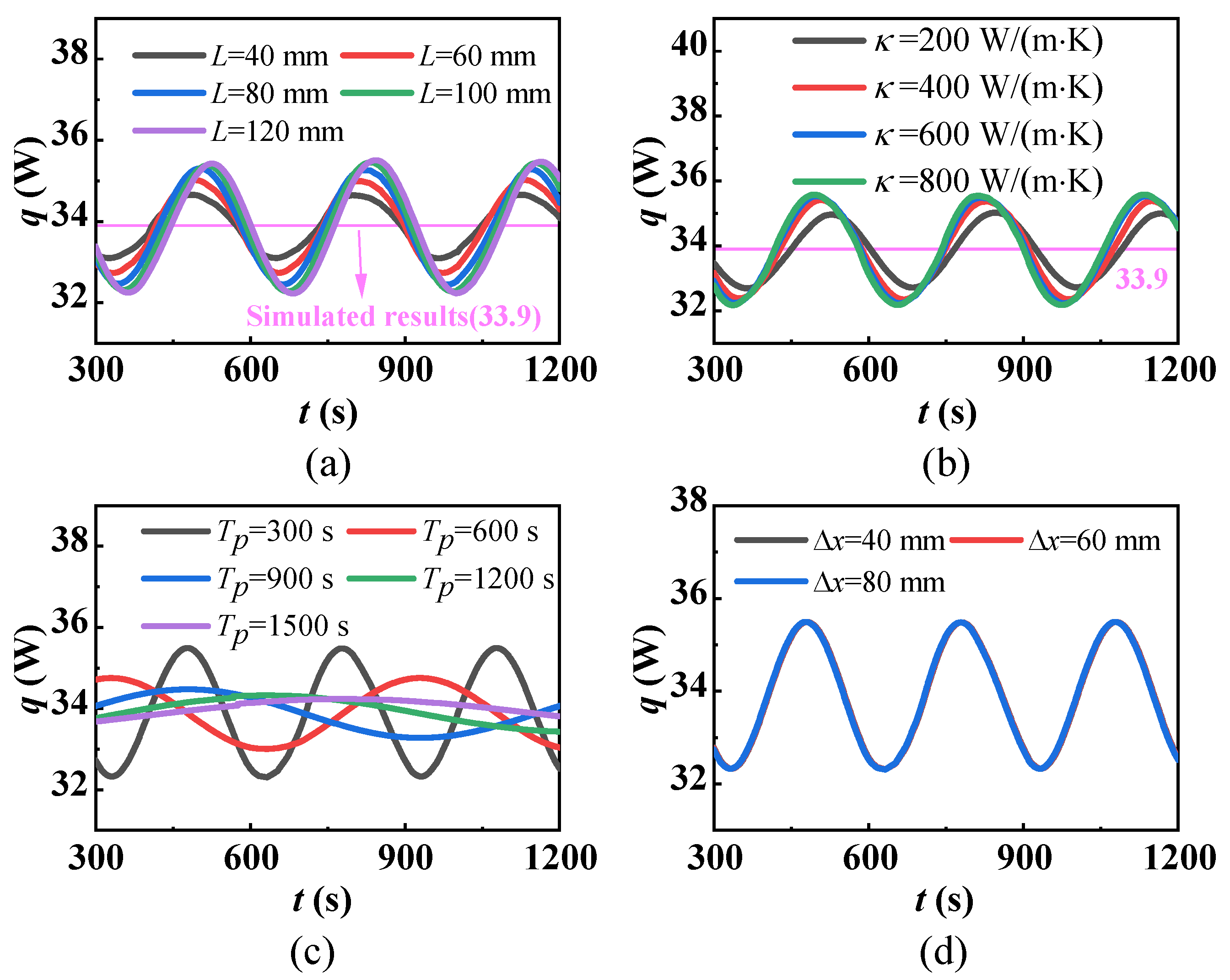
3.2. Parametric Analysis
3.3. Error Correction
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wu, Z.; Zhang, S.; Liu, Z.; Mu, E.; Hu, Z. Thermoelectric converter: Strategies from materials to device application. Nano Energy 2022, 91, 106692. [Google Scholar] [CrossRef]
- Champier, D. Thermoelectric generators: A review of applications. Energy Convers. Manag. 2017, 140, 167–181. [Google Scholar] [CrossRef]
- Singh, Y.; Singh, S.K.; Hazra, P. The Quest for High-Efficiency Thermoelectric Generators for Extracting Electricity from Waste Heat. JOM 2021, 73, 4070–4084. [Google Scholar] [CrossRef]
- Song, K.; Wang, S.; Duan, Y.; Ling, X.; Schiavone, P. Effect of Inevitable Heat Leap on the Conversion Efficiency of Thermoelectric Generators. Phys. Rev. Lett. 2023, 131, 207001. [Google Scholar] [CrossRef]
- Kim, S.I.; Lee, K.H.; Mun, H.A.; Kim, H.S.; Hwang, S.W.; Roh, J.W.; Yang, D.J.; Shin, W.H.; Li, X.S.; Lee, Y.H.; et al. Dense dislocation arrays embedded in grain boundaries for high-performance bulk thermoelectrics. Science 2015, 348, 109–114. [Google Scholar] [CrossRef] [PubMed]
- Zhu, B.; Liu, X.; Wang, Q.; Qiu, Y.; Shu, Z.; Guo, Z.; Tong, Y.; Cui, J.; Gu, M.; He, J. Realizing record high performance in n-type Bi2Te3-based thermoelectric materials. Energy Environ. Sci. 2020, 13, 2106–2114. [Google Scholar] [CrossRef]
- Tsai, Y.-F.; Wei, P.-C.; Chang, L.; Wang, K.-K.; Yang, C.-C.; Lai, Y.-C.; Hsing, C.-R.; Wei, C.-M.; He, J.; Snyder, G.J.; et al. Compositional Fluctuations Locked by Athermal Transformation Yielding High Thermoelectric Performance in GeTe. Adv. Mater. 2021, 33, 2008808. [Google Scholar] [CrossRef] [PubMed]
- Liu, D.; Wang, D.; Hong, T.; Wang, Z.; Wang, Y.; Qin, Y.; Su, L.; Yang, T.; Gao, X.; Ge, Z.; et al. Lattice plainification advances highly effective SnSe crystalline thermoelectrics. Science 2023, 380, 841–846. [Google Scholar] [CrossRef] [PubMed]
- Xu, H.; Zhang, Q.; Yi, L.; Huang, S.; Yang, H.; Li, Y.; Guo, Z.; Hu, H.; Sun, P.; Tan, X.; et al. High performance of Bi2Te3-based thermoelectric generator owing to pressure in fabrication process. Appl. Energy 2022, 326, 119959. [Google Scholar] [CrossRef]
- Xie, L.; Yin, L.; Yu, Y.; Peng, G.; Song, S.; Ying, P.; Cai, S.; Sun, Y.; Shi, W.; Wu, H.; et al. Screening strategy for developing thermoelectric interface materials. Science 2023, 382, 921–928. [Google Scholar] [CrossRef] [PubMed]
- Gao, G.; Chen, S.-Y.; Ai, L.-Q.; Liu, N.; Gou, J.-J.; Gong, C.-L. A thermal contact model encompassing near-field effects of multi-interface TEGs in hypersonic conditions. Int. J. Heat Mass Transf. 2023, 216, 124543. [Google Scholar] [CrossRef]
- Yi, L.; Xu, H.; Yang, H.; Huang, S.; Yang, H.; Li, Y.; Zhang, Q.; Guo, Z.; Hu, H.; Sun, P.; et al. Design of Bi2Te3-based thermoelectric generator in a widely applicable system. J. Power Sources 2023, 559, 232661. [Google Scholar] [CrossRef]
- Wang, P.; Wang, B.; Wang, K.; Gao, R.; Xi, L. An analytical model for performance prediction and optimization of thermoelectric generators with varied leg cross-sections. Int. J. Heat Mass Transf. 2021, 174, 121292. [Google Scholar] [CrossRef]
- Huang, S.; Yang, H.; Li, Y.; Guo, Z.; Zhang, Q.; Cai, J.; Wu, J.; Tan, X.; Liu, G.; Song, K.; et al. Optimizing GeTe-based thermoelectric generator for low-grade heat recovery. Appl. Energy 2023, 349, 121584. [Google Scholar] [CrossRef]
- Ahiska, R.; Mamur, H. A test system and supervisory control and data acquisition application with programmable logic controller for thermoelectric generators. Energy Convers. Manag. 2012, 64, 15–22. [Google Scholar] [CrossRef]
- Rao, A.; Joshi, G.; Poudel, B.; Ballinger, C. A custom designed modular, scalable test system for an efficient performance evaluation of thermoelectric devices. Energy Convers. Manag. X 2022, 14, 100228. [Google Scholar] [CrossRef]
- He, H.; Liu, W.; Wu, Y.; Rong, M.; Zhao, P.; Tang, X. An approximate and efficient characterization method for temperature-dependent parameters of thermoelectric modules. Energy Convers. Manag. 2019, 180, 584–597. [Google Scholar] [CrossRef]
- Guo, Z.; Song, K.; Yan, Z.; Sun, P.; Tan, X.; Wu, G.; Zhang, Q.; Liu, G.-Q.; Yu, B.; Jiang, J. Broadening the optimum thermoelectric power generation range of p-type sintered Bi0.4Sb1.6Te3 by suppressing bipolar effect. Chem. Eng. J. 2021, 426, 131853. [Google Scholar] [CrossRef]
- Luo, D.; Zhao, Y.; Yan, Y.; Chen, H.; Chen, W.-H.; Wang, R.; Li, Y.; Yang, X. Development of two transient models for predicting dynamic response characteristics of an automobile thermoelectric generator system. Appl. Therm. Eng. 2023, 221, 119793. [Google Scholar] [CrossRef]
- Khattak, Z.; Ali, H.M. Air cooled heat sink geometries subjected to forced flow: A critical review. Int. J. Heat Mass Transf. 2019, 130, 141–161. [Google Scholar] [CrossRef]
- Lv, S.; He, W.; Jiang, Q.; Hu, Z.; Liu, X.; Chen, H.; Liu, M. Study of different heat exchange technologies influence on the performance of thermoelectric generators. Energy Convers. Manag. 2018, 156, 167–177. [Google Scholar] [CrossRef]
- Wang, X.; Deng, W.; Tang, X.; He, H. Experiment and simulation study on the specification parameters of finned heat sink for thermoelectric system in consideration of radiation among fins. Int. J. Therm. Sci. 2023, 185, 108097. [Google Scholar] [CrossRef]
- Zheng, L.J.; Kang, D.H.; Kim, N.K.; Youn, Y.J.; Kang, H.W. Theoretical analysis of natural evaporative cooling to enhance the efficiency of thermoelectric devices. Int. J. Heat Mass Transf. 2019, 143, 118512. [Google Scholar] [CrossRef]
- Zeng, Z.; Zhang, W.; Yu, H.; Cao, D.; Li, X. Dynamic thermal model and control performance analysis over frequency domain of temperature control unit. Case Stud. Therm. Eng. 2023, 45, 102975. [Google Scholar] [CrossRef]
- Želi, V.; Zorica, D. Analytical and numerical treatment of the heat conduction equation obtained via time-fractional distributed-order heat conduction law. Physica A 2018, 492, 2316–2335. [Google Scholar] [CrossRef]
- Song, K.; Song, H.-P.; Gao, C.-F. Macro-performance of multilayered thermoelectric medium*. Chin. Phys. B 2017, 26, 127307. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Yang, H.; Yu, C.; Zhou, W.; Zhang, Q.; Hu, H.; Sun, P.; Wu, J.; Tan, X.; Song, K.; et al. Measurement Error in Thermoelectric Generator Induced by Temperature Fluctuation. Energies 2024, 17, 1036. https://doi.org/10.3390/en17051036
Li Y, Yang H, Yu C, Zhou W, Zhang Q, Hu H, Sun P, Wu J, Tan X, Song K, et al. Measurement Error in Thermoelectric Generator Induced by Temperature Fluctuation. Energies. 2024; 17(5):1036. https://doi.org/10.3390/en17051036
Chicago/Turabian StyleLi, Yanan, Hao Yang, Chuanbin Yu, Wenjie Zhou, Qiang Zhang, Haoyang Hu, Peng Sun, Jiehua Wu, Xiaojian Tan, Kun Song, and et al. 2024. "Measurement Error in Thermoelectric Generator Induced by Temperature Fluctuation" Energies 17, no. 5: 1036. https://doi.org/10.3390/en17051036
APA StyleLi, Y., Yang, H., Yu, C., Zhou, W., Zhang, Q., Hu, H., Sun, P., Wu, J., Tan, X., Song, K., Liu, G., & Jiang, J. (2024). Measurement Error in Thermoelectric Generator Induced by Temperature Fluctuation. Energies, 17(5), 1036. https://doi.org/10.3390/en17051036






