Numerical Study on the Characteristics and Control Method of Coal Leakage between Supports in Integrated Mining of Extremely Loose and Soft Coal Seams
Abstract
1. Introduction
2. Numerical Modeling of Coal Leakage between Hydraulic Supports
2.1. Geological Conditions of Study Mines
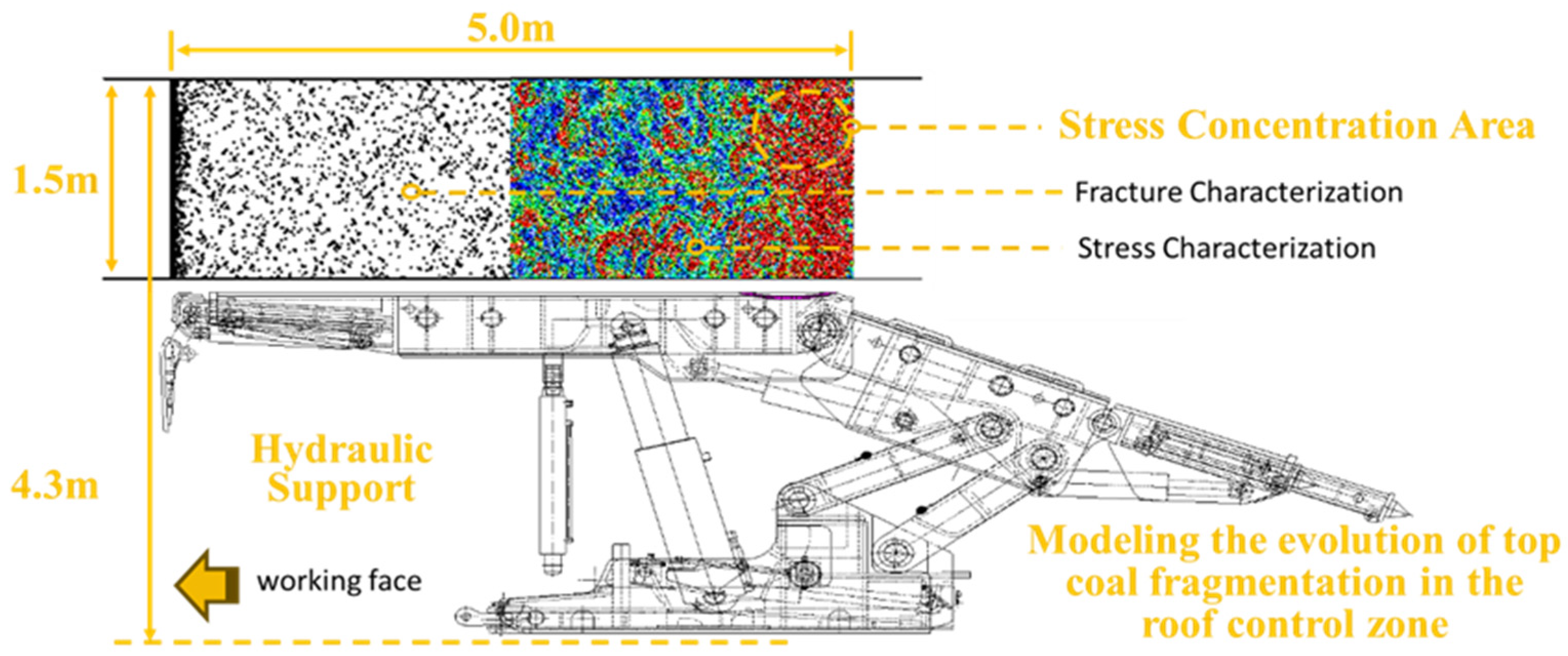
2.2. Development of Numerical Models
3. Numerical Simulation Results and Analysis
3.1. Top Coal Crushing Characteristics
3.2. Characteristics of Coal Leakage between Hydraulic Supports
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wu, F.F.; Zhang, S.R.; Yue, X.; Liu, C.; Liu, Q.; Zhang, J.; Lv, B.; Gao, Z. Study on mechanism and improvement technology of Top coal loss in horizontal sublevel fully mechanized caving mining. Energy Explor. Exploit. 2022, 40, 1394–1408. [Google Scholar] [CrossRef]
- Yang, L.; Wang, J.C.; Yang, S.L.; Li, L.H.; Wu, S.W. Improving the top coal recovery ratio in longwall top coal caving mining using drawing balance analysis. Int. J. Min. Reclam. Environ. 2023, 37, 319–337. [Google Scholar] [CrossRef]
- Song, X.H.; Zhu, D.F.; Wang, Z.L.; Huo, Y.M.; Liu, Y.Y.; Liu, G.F.; Cao, J.J.; Li, H.C. Forty years of integrated mining in China’s coal mines: Progress in theory and technical equipment research. Coal Sci. Technol. 2021, 49, 1–29. [Google Scholar]
- Wang, J.C. 40 years and prospects of integrated mining in China. J. China Coal Soc. 2023, 48, 83–99. [Google Scholar] [CrossRef]
- Wang, J. Research on the Coal Rock Release Pattern and Coal Release Process Parameters of Large-Height Generalized Mining of Thick Coal Seams. Master’s Thesis, China University of Mining and Technology, Xuzhou, China, 2008. [Google Scholar]
- Wei, J.P. Research on the Fracturing Pattern and Arching Mechanism of Top Coal in the Integrated Face. Doctoral Dissertation, Taiyuan University of Technology, Taiyuan, China, 2004. [Google Scholar]
- Xue, B.; Zhang, Y.; Li, J.; Wang, Y. A review of coal gangue identification research-application to China’s top coal release process. Environ. Sci. Pollut. Res. 2023, 30, 14091–14103. [Google Scholar] [CrossRef] [PubMed]
- Zhang, N.B.; Liu, C.Y.; Chen, B.B.; Zhu, C.Q.; Zhou, J.F. The flow pattern of coal gangue release and determination of parameters of coal release process in thick coal seam under extremely close coal seam. J. Min. Saf. Eng. 2021, 38, 911–918. [Google Scholar]
- Wang, J.C.; Fu, Q. Theory and application of bulk medium flow for top coal release in low-level integrated mining. J. China Coal Soc. 2002, 1, 337–341. [Google Scholar]
- Wang, J.C.; Li, Z.G.; Chen, Y.J.; Zheng, H.F. Experimental study on the theory of bulk media flow in top coal release of integrated mining. J. China Coal Soc. 2004, 3, 260–263. [Google Scholar]
- Zhang, J.W. Simulation Study on Three-Dimensional Release Law of Bulk Top Coal in Integrated Mining. Doctoral Dissertation, China University of Mining and Technology (Beijing), Beijing, China, 2017. [Google Scholar]
- He, G.R.; Wang, N.; Zhang, J. Key technology and management of circular arc section of large inclination angle “three soft” extra thick coal seam working face. Coal Sci. Technol. 2023, 1, 1–12. [Google Scholar]
- Wang, L.; Wang, M.Y.; Yan, H.B.; Yan, W.T.; Chen, J.J. Research on the mode and mechanism of overburden subsidence in “three soft” coal seams. Coal Technol. 2023, 42, 28–31. [Google Scholar]
- Wang, X.F.; Wang, Y.; Zhang, D.S. Research on strengthening support technology for key parts of large inclination angle “three soft” coal seam roadway. J. Min. Saf. Eng. 2017, 34, 208–213. [Google Scholar]
- He, X. Research on the Breakage and Release Pattern of Thick Hard Top Coal and Cracking to Promote Release in Shenshubian Coal Mine. Master’s Thesis, China University of Mining and Technology, Xuzhou, China, 2020. [Google Scholar]
- Wang, J.C.; Zhang, J.W. BBR study on top coal release pattern of integrated mining. J. China Coal Soc. 2015, 40, 487–493. [Google Scholar]
- Zhang, N.B.; Liu, C.Y.; Chen, Y. Numerical simulation study of gangue flow field law in unstable thick coal seam roof coal mining. Coal Technol. 2014, 33, 1–4. [Google Scholar]
- Du, L.F. Research on Coal Releasing Process of Group Coal Releasing in Integrated Mining of Extra-Thick Coal Seam in Tashan Coal Mine. Master’s Thesis, Research Institute of Coal Science, Beijing, China, 2020. [Google Scholar]
- Du, L.F.; Xie, X.Z.; Zhao, T.L. Analysis of spatial and temporal field coupling of coal top coal at the beginning of multiple coal discharge port comprehensive mining. Coal Sci. Technol. 2019, 47, 56–62. [Google Scholar]
- Wang, X.F.; Chen, X.Y.; Wang, J.Y.; Chang, Z.C.; Qin, D.D. Creep damage mechanism and control of perimeter rock in deep soft rock tunnel in Pingdingshan mine. J. Min. Saf. Eng. 2023, 40, 1139–1150. [Google Scholar]
- Wang, X.F.; Jia, J.F.; Yang, Z.B.; Zhao, W.L.; Wang, J.Y.; Yang, P.J.; Yang, Q. Determination of the strength of high-foam filler at the top of ultra-high roadway in very soft and thick coal seams. J. Min. Rock Control. Eng. 2023, 5, 77–87. [Google Scholar]
- Skrzypkowski, K.; Korzeniowski, W.; Nguyen Duc, T. Choice of Powered Roof Support FAZOS-15/31-POz for Vang Danh Hard Coal Mine. Inżynieria Miner. 2022, 2, 2–13. [Google Scholar] [CrossRef]
- Zeng, Q.; Li, Z.; Wan, L.; Ma, D. Study on Roof Instability Effect and Bearing Characteristics of Hydraulic Support in Longwall Top Coal Caving. Appl. Sci. 2023, 13, 8102. [Google Scholar] [CrossRef]
- Huo, Y.M. Mechanism of Top Coal Crushing and Intelligent Coal Release Control in Integrated Mining of Thick Coal Seams. Doctoral Dissertation, Taiyuan University of Technology, Taiyuan, China, 2021. [Google Scholar]
- Mao, H.J.; Guo, Y.T.; Wang, G.J.; Yang, C.H. Evaluation of the effect of clay mineralogical composition on hydration. Geotechnics 2010, 31, 2723–2728. [Google Scholar]
- He, X.; Liu, C.Y.; Wu, F.F.; Peng, K.P.; Yang, J.X. Study on the influence of elevation angle on top coal release pattern in upward sloping generalized mining coal seams. Coal Sci. Technol. 2021, 49, 25–31. [Google Scholar]
- Han, Y.F.; Wang, Z.H.; Tang, Y.S. Characterization of zonal damage of top coal in integrated mining of thick coal seams. Coal Sci. Technol. 2022, 50, 99–107. [Google Scholar]
- Liu, C.Y.; Huang, B.X.; Wu, F.F.; Wan, Z.J.; Yang, P.J. Block size theory and application for breaking and venting top coal in generalized mining. J. Min. Saf. Eng. 2006, 1, 56–61. [Google Scholar]
- Liu, C.Y.; Zhang, N.B.; Guo, F.Q.; An, S.; Chen, B.B. Temporal pattern and identification method of coal-gangue-rock release flow in a thick coal seam. J. China Coal Soc. 2022, 47, 137–151. [Google Scholar]
- Huang, S.Y. Bulk Mechanics; Mechanical Industry Press: Beijing, China, 1993. [Google Scholar]
- Song, X.M. Correlation study on the distribution and blockiness of top coal fissures in top coal mining. J. China Coal Soc. 1998, 2, 40–44. [Google Scholar]
- Jin, Z.M.; Wei, J.P.; Jin, W.W. Characteristics of support pressure distribution in front of a roof coal mine. J. Taiyuan Univ. Technol. 2001, 3, 216–218. [Google Scholar]
- Jin, Z.M.; Kang, T.H.; Gong, P.L.; Song, X.M. Correlation between fractal fracture and top coal emplacement in coal bodies. J. Rock Mech. Eng. 1996, 2, 48–54. [Google Scholar]
- Jin, Z.M.; Zhang, H.X.; Song, X.G.; Kang, T.H. Study on the deformation and motion law of top coal in general-purpose mining field. Mine Press. Roof Manag. 1992, 1, 26–31. [Google Scholar]
- Song, X.H.; Qian, M.G.; Jin, Z.M. Study on the distribution law of top coal lumpiness in roof coal mining. J. China Coal Soc. 1999, 1, 39–43. [Google Scholar]
- Xu, Y.X.; Wang, G.F.; Li, M.Z.; He, M.; Zhang, J.H.; Zhou, C.T.; Han, H.J. Numerical simulation study of integrated mining of extra-thick hard coal seams based on bonded particle model. J. China Coal Soc. 2019, 44, 3317–3328. [Google Scholar]















| Density/(kg/m3) | Normal to Tangential Stiffness Ratio/(kn/ks) | Contact Modulus/GPa | Friction Coefficient/f | Normal Bond Strength/MPa | Tangential Bond Strength/MPa |
|---|---|---|---|---|---|
| 1400 | 1.5 | 50 | 0.5 | 10 | 50 |
| Rock Stratum | Thickness/m | Normal Stiffness/GPa | Tangential Stiffness/GPa | Capacity/N/m3 | Equivalent Diameter/m | Porosity | Coefficient of Friction |
|---|---|---|---|---|---|---|---|
| gangue | 1 | 4 | 6 | 2500 | 0.05~0.06 | 0.2 | 0.55 |
| top coal | 0.5 | 4 | 4 | 1400 | 0.0095~0.0105 | 0.1 | 0.30 |
| Width of Hydraulic Support | Deduce the Highest Level | Minimum Roof Control Distance Porosity | Height of Coal Discharge Opening | Coal Miner Cut-off Depth | |||||
|---|---|---|---|---|---|---|---|---|---|
| Actual/m | Model/m | Actual/m | Model/m | Actual/m | Model/m | Actual/m | Model/m | Actual/m | Model/m |
| 1.5 | 6 | 2.3 | 9.2 | 4.59 | 18.36 | 1.1 | 4.4 | 0.6 | 2.4 |
| Stratum | Actual | Model | |||
|---|---|---|---|---|---|
| Thicknesses/m | Lump Size/mm | Thicknesses/cm | Lump Size/mm | ||
| top coal | lower part | 1.0 | 125 | 3.10 | 5 |
| upper section | 2.0 | 437.5 | 6.20 | 17.5 | |
| immediate roof | lower part | 2.5 | 187.5 | 4.99 | 7.5 |
| upper section | 3.5 | 500 | 6.74 | 20 | |
| main roof | lower part | 2.0 | 250 | 4.58 | 10 |
| central section | 2.0 | 300 | 5.2 | 12.5 | |
| upper section | 2.0 | 375 | 5.2 | 15 | |
| overlying strata | full | 3.5 | 1000 | 14.5 | 40 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, P.; Zhang, S.; Wang, X. Numerical Study on the Characteristics and Control Method of Coal Leakage between Supports in Integrated Mining of Extremely Loose and Soft Coal Seams. Energies 2024, 17, 1013. https://doi.org/10.3390/en17051013
Yang P, Zhang S, Wang X. Numerical Study on the Characteristics and Control Method of Coal Leakage between Supports in Integrated Mining of Extremely Loose and Soft Coal Seams. Energies. 2024; 17(5):1013. https://doi.org/10.3390/en17051013
Chicago/Turabian StyleYang, Peiju, Shurong Zhang, and Xufeng Wang. 2024. "Numerical Study on the Characteristics and Control Method of Coal Leakage between Supports in Integrated Mining of Extremely Loose and Soft Coal Seams" Energies 17, no. 5: 1013. https://doi.org/10.3390/en17051013
APA StyleYang, P., Zhang, S., & Wang, X. (2024). Numerical Study on the Characteristics and Control Method of Coal Leakage between Supports in Integrated Mining of Extremely Loose and Soft Coal Seams. Energies, 17(5), 1013. https://doi.org/10.3390/en17051013






