Dynamics of a Rail Vehicle in Transition Curve above Critical Velocity with Focus on Hunting Motion Considering the Review of History of the Stability Studies
Abstract
1. Introduction
2. Rail Vehicle Motion Stability Tests
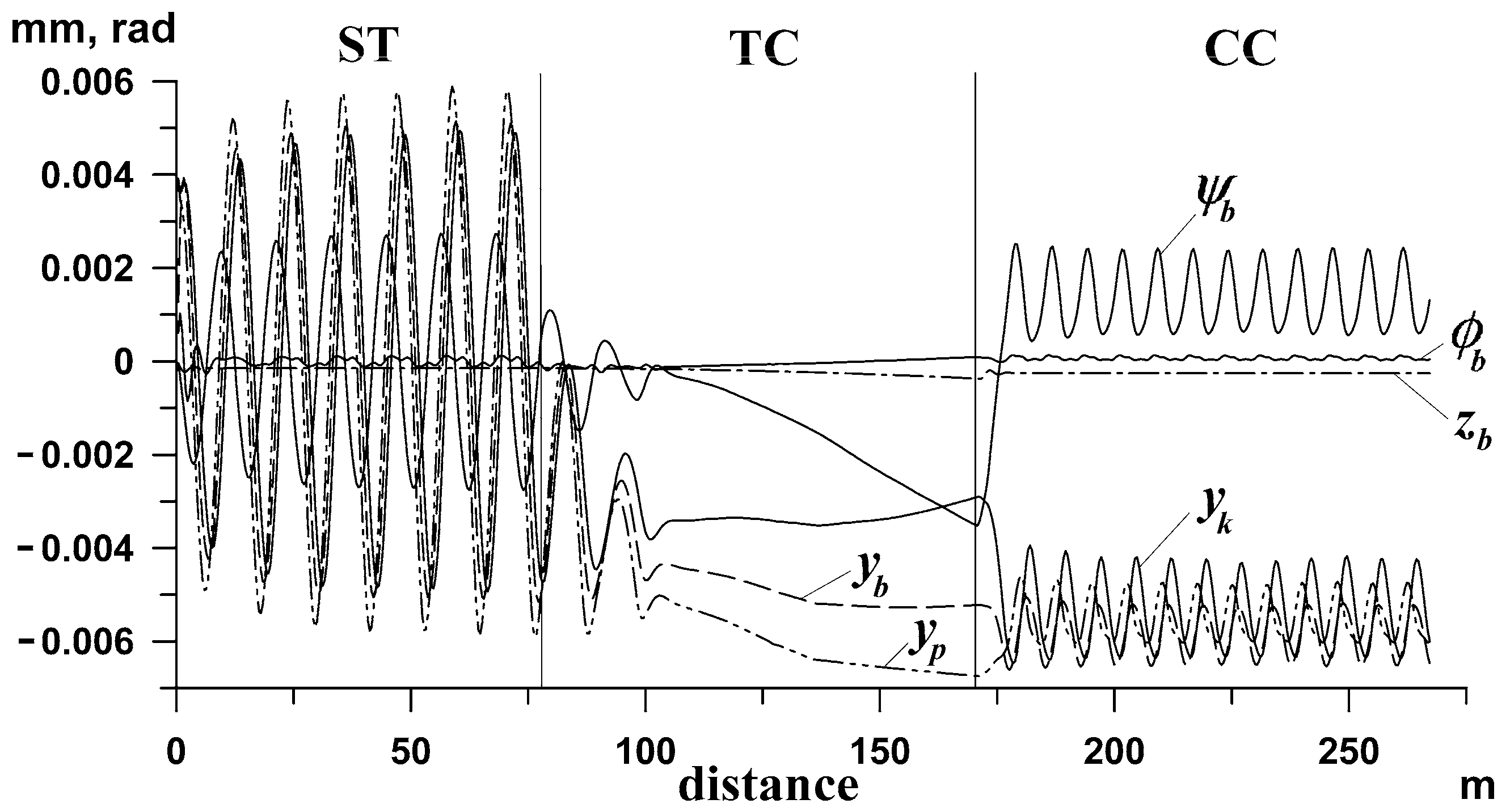
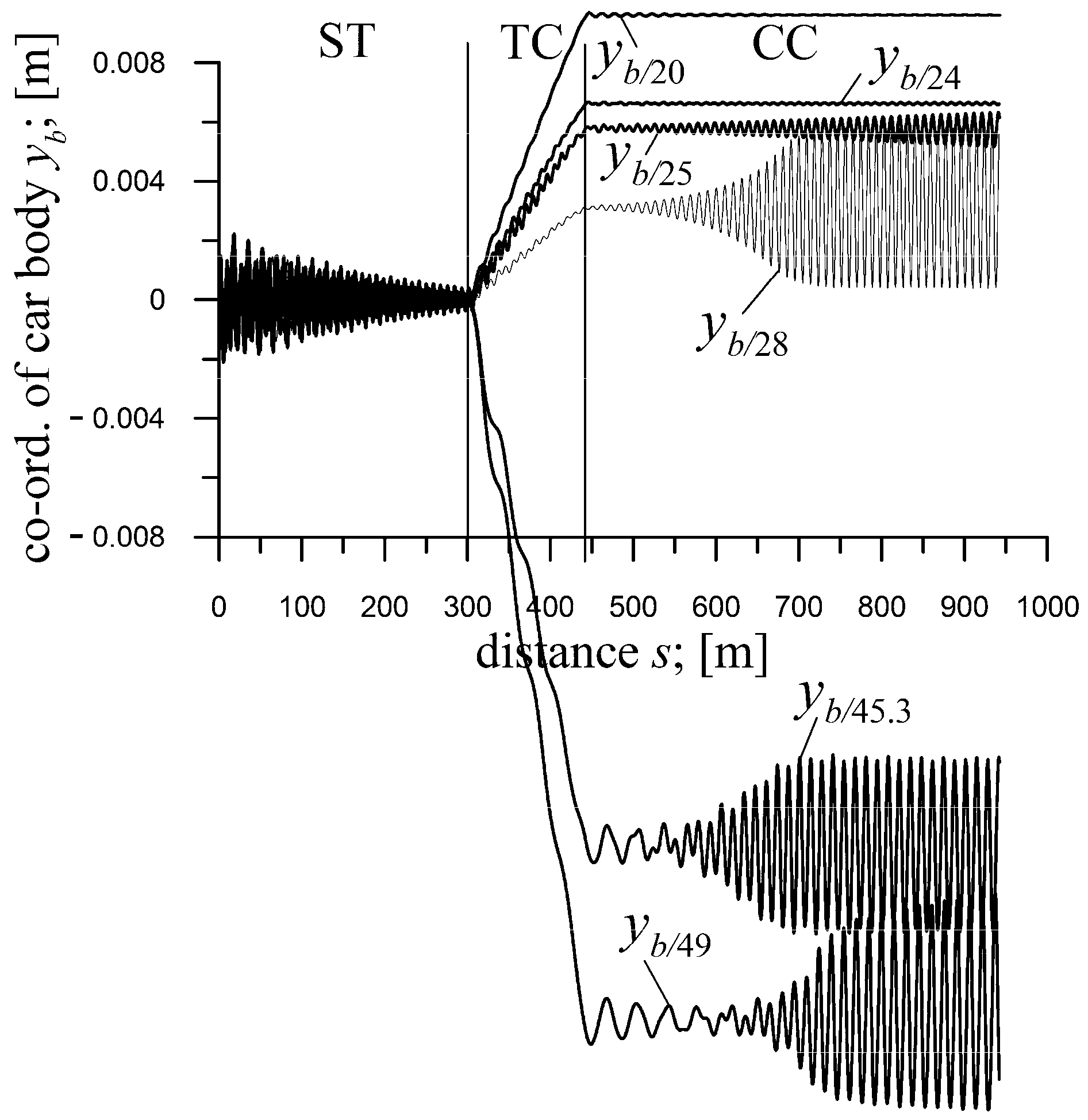
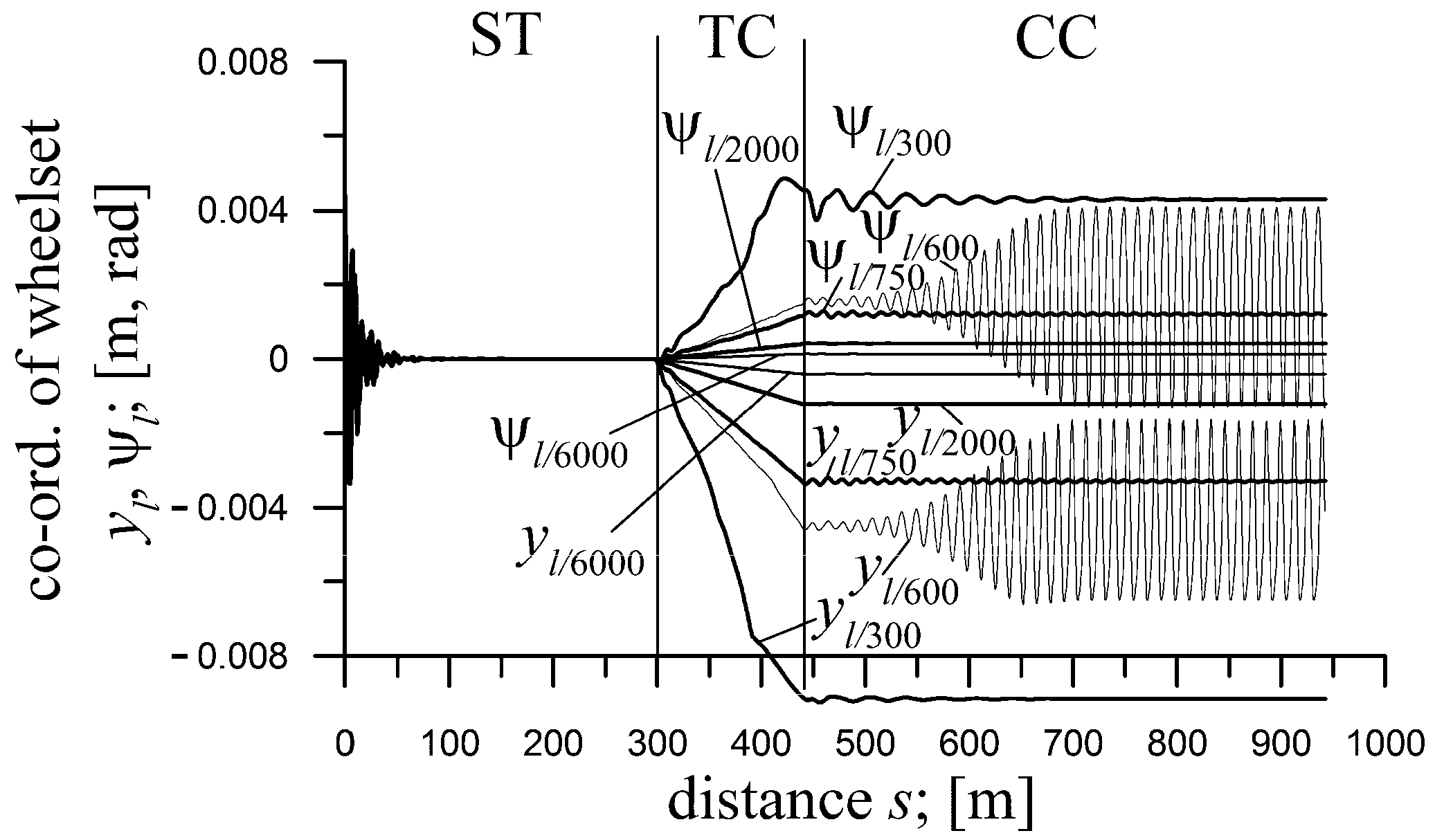
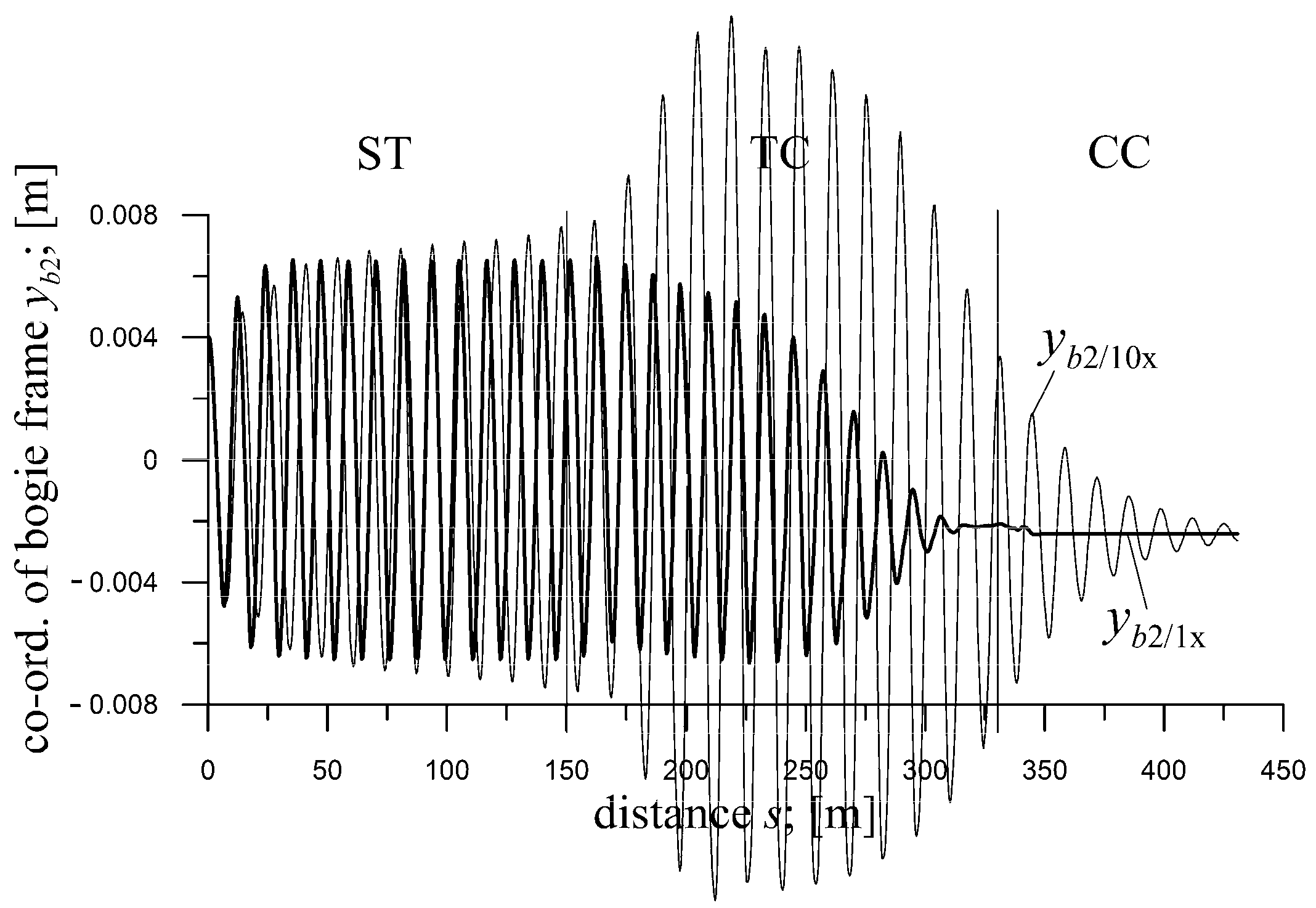
3. Motion of a Rail Vehicle in a Transition Curve above the Critical Velocity
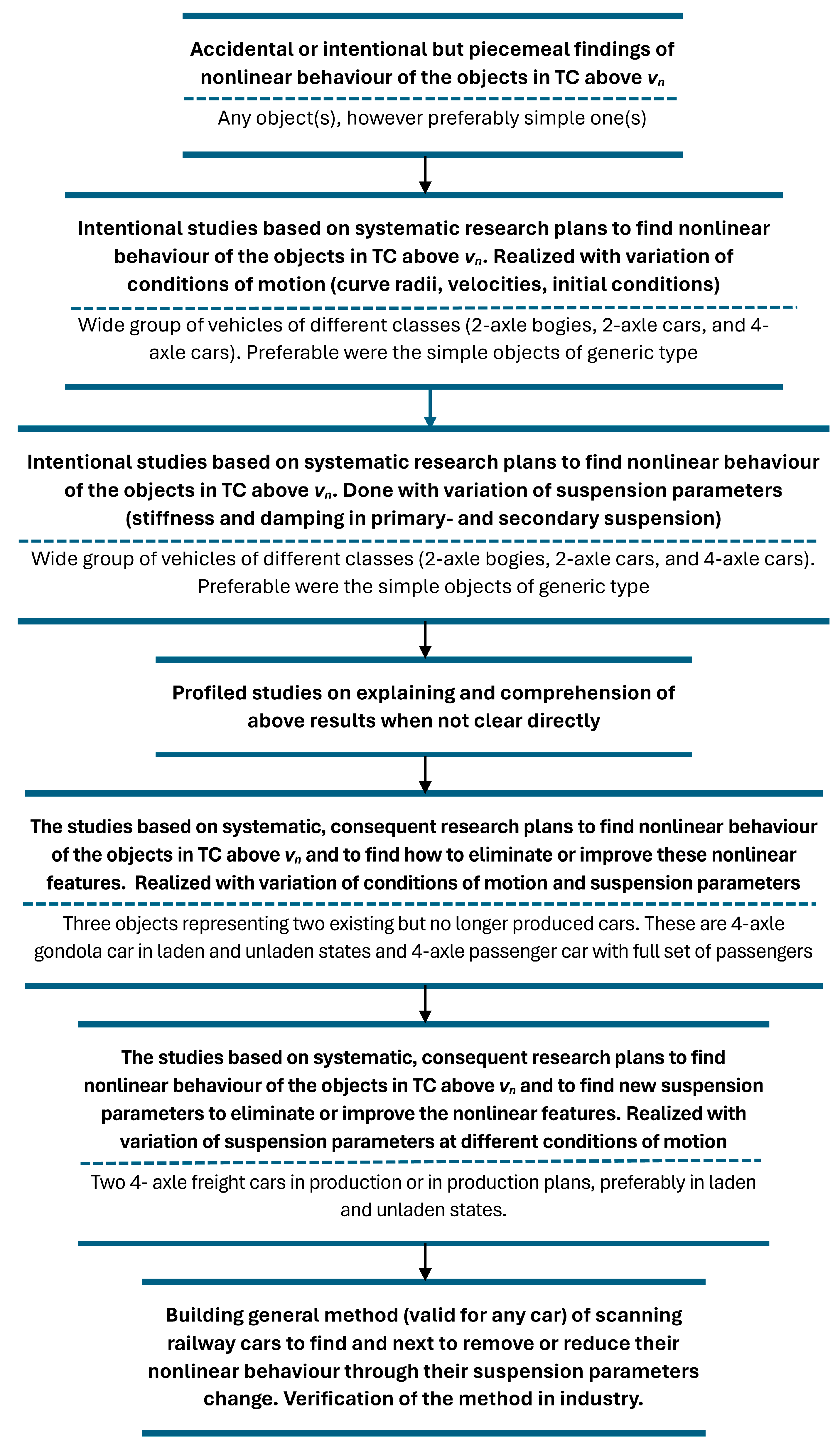
4. Information on Approach to the Problem by the Present Authors
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zboiński, K. Dynamical investigation of railway vehicles on a curved track. Eur. J. Mech. Part A Solids 1998, 17, 1001–1020. [Google Scholar] [CrossRef]
- Zboiński, K. Selected problems of non-linear (non-smooth) dynamics of rail vehicles in curved track. In Non-Smooth Problems in Vehicle System Dynamics; Thomsen, P.G., True, H., Eds.; Springer: Berlin/Heidelberg, Germany, 2010; pp. 87–99. [Google Scholar] [CrossRef]
- Hoffmann, M. Dynamics of European Two-Axle Freight Wagons. Ph.D. Thesis, Technical University of Denmark, Informatics and Mathematical Modelling, Lyngby, Denmark, 2006; 159p. [Google Scholar]
- Hoffmann, M.; True, H. The dynamics of European two-axle railway freight wagons with UIC standard suspension. Veh. Syst. Dyn. 2008, 46 (Suppl. S1), 225–236. [Google Scholar] [CrossRef]
- Hoffmann, M.; True, H. The dynamics of two-axle freight wagons with UIC standard suspension. In Proceedings of the 10th VSDIA Conference on Vehicle System Dynamics, Identification and Anomalies, Budapest, Hungary, 6–8 November 2006; Zobory, I., Ed.; Budapest University of Technology and Economics: Budapest, Hungary, 2006; pp. 183–190, ISBN 978-963-420-968-3. [Google Scholar]
- Knothe, K.; Bohm, F. History of Stability of Railway and Road Vehicles. Veh. Syst. Dyn. 1999, 31, 283–323. [Google Scholar] [CrossRef]
- Knothe, K.; Stichel, S. Rail Vehicle Dynamics; Springer: Cham, Switzerland, 2017; 321p, ISBN 978-3-319-45374-3. [Google Scholar]
- Wickens, A.H. Static and Dynamic Stability of Unsymmetric Two-Axle Railway Vehicles Possessing Perfect Steering. Veh. Syst. Dyn. 1982, 11, 89–106. [Google Scholar] [CrossRef]
- Wickens, A.H. The dynamic stability of railway vehicle wheelsets and bogies having profiled wheels. Int. J. Solid Struct. 1965, 1, 319–341. [Google Scholar] [CrossRef]
- Wickens, A.H. The dynamics of railway vehicles on straight track: Fundamental consideration of lateral stability. Proc. IMechE Part F J. Rail Rapid Transit 1965, 180, 1–16. [Google Scholar] [CrossRef]
- Wickens, A.H. The dynamics stability of a simplified four-wheeled vehicle having profiled wheels. Int. J. Solid Struct. 1965, 1, 385–406. [Google Scholar] [CrossRef]
- Dukkipati, R.V. Modelling and simulation of the hunting of a three-piece railway truck on NCR curved track simulator. Veh. Syst. Dyn. 1994, 23 (Suppl. S1), 105–115. [Google Scholar] [CrossRef]
- Shen, Z. On principles and methods to reduce the wheel/rail forces for rail freight vehicles. Veh. Syst. Dyn. 1992, 20 (Suppl. S1), 584–595. [Google Scholar] [CrossRef]
- Liu, M. A Nonlinear Dynamic Model for Single-axle Wheelset with Profiled Wheels and Rails. In Proceedings of the International Conference of Control, Dynamic Systems, and Robotics, Ottawa, ON, Canada, 7–8 May 2014. Paper No. 60(1-8). [Google Scholar]
- Kalker, J.J. Simplified theory of rolling contact. Mech. Aeronaut. Eng. Shipbuild. 1873, 1, 1–10. [Google Scholar]
- Kalker, J.J. Three-Dimensional Elastic Bodies in Rolling Contact; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1990; 314p, ISBN 978-0-7923-0712-9. [Google Scholar]
- Kortum, W.; Sharp, R.S.; De Pater, A.D. Application of Multibody Computer Codes to Vehicle System Dynamics—Progress Report to 12th IAVSD Symposium on a Workshop and Resulting Activities; Soc. for Eng. and Scientific Education Oberpfaffenhofen: Lyon, France, 1991. [Google Scholar]
- Kass-Petersen, C.; True, H. A bifurcation analysis of nonlinear oscillation in railway vehicles. Veh. Syst. Dyn. 1983, 12, 5–6. [Google Scholar] [CrossRef]
- Kass-Petersen, C.; True, H. A bifurcation analysis of nonlinear oscillations in railway vehicles. In Proceedings of the 8th IAVSD Symposium on Dynamics of Vehicles on Roads and Tracks, Cambridge, MA, USA, 15–19 August 1983; Hedrick, K., Ed.; Swets & Zeitlinger: Amsterdam, The Netherlands; Lisse, The Netherlands, 1984; pp. 655–665. [Google Scholar]
- Gasch, R.; Kik, W.; Moelle, D. Non-Linear Bogie Hunting. Veh. Syst. Dyn. 1981, 10, 145–148. [Google Scholar] [CrossRef]
- Gasch, R.; Moelle, D. Nonlinear bogie hunting. In Proceedings of the 7th IAVSD Symposium on Dynamics of Vehicles on Roads and Tracks, Cambridge, UK, 7–11 September 1981; Wickens, A.H., Ed.; Swets & Zeitlinger: Lisse, The Netherlands, 1982; pp. 455–467. [Google Scholar]
- Gasch, R.; Moelle, D.; Knothe, K. The effect of non-linearities on the limit-cycles of railway vehicles. In Proceedings of the 8th IAVSD Symposium on Dynamics of Vehicles on Roads and Tracks, Cambridge, MA, USA, 15–19 August 1983; Hedrick, K., Ed.; Swets & Zeitlinger: Amsterdam, The Netherlands; Lisse, The Netherlands, 1984; pp. 207–224. [Google Scholar]
- Aizpun, M.; Vinolas, J. Illustrating the implications of choices at the vehicle model building stage in simulation results. In The Dynamics of Vehicles on Roads and Tracks—Proceeding of the 24th IAVSD Symp; Rosenberger, M., Plochl, M., Six, K., Edelmann, J., Eds.; Taylor & Francis: London, UK, 2016; pp. 1033–1042. ISBN 978-1-138-02885-2. [Google Scholar]
- Cheng, Y.C.; Lee, S.Y.; Chen, H.H. Modelling and nonlinear hunting stability analysis of high-speed railway vehicle on curved tracks. J. Sound Vib. 2009, 324, 139–160. [Google Scholar] [CrossRef]
- Choi, Y.-S.; Shin, B.-S. Critical speed of high-speed trains considering wheel-rail contact. J. Mech. Sci. Technol. 2015, 29, 4593–4600. [Google Scholar] [CrossRef]
- Choromański, W.; Kisilowski, J.; Łopata, H. Investigation of technical stochastic stability of lateral vibrations of mathematical model of railway vehicle. Rozpr. Inż. 1985, 3, 219–234. [Google Scholar]
- Di Gialleonardo, E.; Bruni, S.; True, H. Analysis of the non-linear dynamics of a 2-axle freight wagon in curves. Veh. Syst. Dyn. 2014, 52, 125–141. [Google Scholar] [CrossRef]
- Gao, X.-J.; Li, Y.-H.; Yue, Y. The “resultant bifurcation diagram” method and its application to bifurcation behaviours of a symmetric railway bogie system. Nonlinear Dyn. 2012, 70, 363–380. [Google Scholar] [CrossRef]
- Goodall, R.M.; Iwnicki, S. Non-linear dynamic techniques v. equivalent conicity methods for rail vehicle stability assessment. In Proceedings of the 18th IAVSD Symposium on Dynamics of Vehicles on Roads and Tracks, Vehicle System Dynamics, Kanagawa, Japan, 24–30 August 2003; Abe, M., Ed.; Taylor & Francis: London, UK, 2004; Volume 41, pp. 791–799, ISBN 9026519729. [Google Scholar]
- Huilgol, R.R. Hopf-Friedrichs bifurcation and the hunting of a railway axle. Q. Appl. Math. 1978, 36, 85–94. [Google Scholar] [CrossRef]
- Iwnicki, S.; Stichel, S.; Orlova, A.; Hecht, M. Dynamics of railway freight vehicles. Veh. Syst. Dyn. 2015, 53, 995–1033. [Google Scholar] [CrossRef]
- Jensen, J.C.; True, H. On the new route to chaos in railway dynamics. Nonlinear Dyn. 1997, 13, 117–129. [Google Scholar] [CrossRef]
- Kardas-Cinal, E. Some Problems Related to Investigations of Wheelset Model Stability. In 2nd Polish-German Workshop; IPPT PAN: Warsaw, Poland, 1991; pp. 119–130. [Google Scholar]
- Kardas-Cinal, E.; Kisilowski, J. The influence of riding speed on vehicle track dynamics in the presence of track random irregularities. Mach. Dyn. Probl. 1995, 11, 55–67. [Google Scholar]
- Kisilowski, J.; Kardas-Cinal, E. On a certain method of examining stability of mathematical models of railway vehicle with disturbances occurring in real object. Veh. Syst. Dyn. 1994, 23 (Suppl. S1), 262–273. [Google Scholar] [CrossRef]
- Kisilowski, J.; Knothe, K. Advanced Railway Vehicle System Dynamics; WNT: Warszawa, Poland, 1991; 442p, ISBN 83-204-1348-6. [Google Scholar]
- Kumar, V.; Rastogi, V.; Pathak, P.M. Modelling and evaluation of the hunting behaviour of a high-speed railway vehicle on curved track. Proc. IMechE Part F J. Rail Rapid Transit 2018, 233, 220–236. [Google Scholar] [CrossRef]
- Lee, S.Y.; Cheng, Y.C. A new dynamic model of high-speed railway vehicle moving on curved tracks. ASME J. Vib. Acoust. 2008, 130, 011009. [Google Scholar] [CrossRef]
- Lee, S.Y.; Cheng, Y.C. Hunting stability analysis of a new dynamic model of high-speed railway vehicle moving on curved tracks. In Proceedings of the 15th IASTED International Conference on Applied Simulation and Modelling, Rhodes, Greece, 26–28 June 2006; pp. 8–14. [Google Scholar]
- Lee, S.Y.; Cheng, Y.C. Influence of the vertical and the roll motions of frames on the hunting stability of trucks moving on curved tracks. J. Sound Vib. 2006, 294, 441–453. [Google Scholar] [CrossRef]
- Lee, S.Y.; Cheng, Y.C. Nonlinear analysis on hunting stability for high-speed railway vehicle truck on curved tracks. ASME J. Vib. Acoust. 2005, 127, 324–332. [Google Scholar] [CrossRef]
- Lee, S.Y.; Cheng, Y.C. Nonlinear hunting stability analysis of high-speed railway vehicles on curved tracks. Int. J. Heavy Veh. Syst. 2003, 10, 344–361. [Google Scholar] [CrossRef]
- Lee, S.Y.; Cheng, Y.C.; Kuo, C.M. Non-linear modelling and analysis on the hunting stability of trucks moving on curved tracks. In Proceedings of the 14th IASTED International Conference on Applied Simulation and Modelling, Benalmadena, Spain, 15–17 June 2005; pp. 422–426. [Google Scholar]
- Luo, G.W.; Shi, Y.Q.; Zhu, X.F.; Du, S.S. Hunting patterns and bifurcation characteristics of a three-axle locomotive bogie system in the presence of the flange contact nonlinearity. Int. J. Mech. Sci. 2018, 136, 321–338. [Google Scholar] [CrossRef]
- Mazzola, L.; Alfi, S.; Bruni, S. Evaluation on the hunting behaviour of a railway vehicle in a curve. Proc. IMechE Part F J. Rail Rapid Transit 2015, 229, 530–541. [Google Scholar] [CrossRef]
- Park, J.-H.; Koh, H.-I.; Kim, N.-P. Parametric study of lateral stability for a railway vehicle. J. Mech. Sci. Technol. 2011, 25, 1657–1666. [Google Scholar] [CrossRef][Green Version]
- Polach, O. Characteristic parameters of nonlinear wheel/rail contact geometry. Veh. Syst. Dyn. 2010, 48 (Suppl. S1), 19–36. [Google Scholar] [CrossRef]
- Polach, O. On non-linear methods of bogie stability assessment using computer simulations. Proc. IMechE Part F J. Rail Rapid Transit 2006, 220, 13–27. [Google Scholar] [CrossRef]
- Rezvani, M.A.; Mazraeh, A. Dynamics and stability analysis of a freight wagon subjective to the railway track and wheelset operational conditions. Eur. J. Mech. A-Solids 2017, 61, 22–34. [Google Scholar] [CrossRef]
- Schupp, G. Computational bifurcation analysis of mechanical systems with applications to railway vehicles. In Proceedings of the 18th IAVSD Symposium on Dynamics of Vehicles on Roads and Tracks, Vehicle System Dynamics, Atsugi, Japan, 24–30 August 2003; Abe, M., Ed.; Taylor & Francis: London, UK, 2004; Volume 41, pp. 458–467, ISBN 9026519729. [Google Scholar]
- Stichel, S. Limit cycle behaviour and chaotic motions of two-axle freight wagons with friction damping. Multibody Syst. Dyn. 2002, 8, 243–255. [Google Scholar] [CrossRef]
- Toumi, M.; Chollet, H.; Yin, H. Effect of elasto-plasticity on the creep force characteristic and the railway vehicle stability. In The Dynamics of Vehicles on Roads and Tracks—Proceeding 24th IAVSD Symp; Rosenberger, M., Plochl, M., Six, K., Edelmann, J., Eds.; Taylor & Francis: London, UK, 2016; pp. 1015–1025. ISBN 978-1-138-02885-2. [Google Scholar]
- True, H. Does a critical speed for railroad vehicles exist? In Proceedings of the ASME/IEEE Joint Railroad Conference, Chicago, IL, USA, 22–24 March 1994; Hawthorne, K.L., Hill, R.J., Eds.; IEEE: Piscataway, NJ, USA, 1994; pp. 125–131. [Google Scholar] [CrossRef]
- True, H. Dynamics of a rolling wheelset. Appl. Mech. Rev. 1993, 46, 438–444. [Google Scholar] [CrossRef]
- True, H. Multiple attractors and critical parameters and how to find them numerically: The right, the wrong and the gambling way. Veh. Syst. Dyn. 2013, 51, 443–459. [Google Scholar] [CrossRef]
- True, H. On the theory of nonlinear dynamics and its applications in vehicle systems dynamics. Veh. Syst. Dyn. 1999, 31, 393–421. [Google Scholar] [CrossRef]
- True, H. Railway vehicle chaos and asymmetric hunting. Veh. Syst. Dyn. 1992, 20 (Suppl. S1), 625–637. [Google Scholar] [CrossRef]
- True, H. Recent advances in the fundamental understanding of railway vehicle dynamics. Int. J. Veh. Des. 2006, 40, 251–264. [Google Scholar] [CrossRef]
- True HBirkedal Nielsen, J. On the Dynamics of Steady Curving of Railway Vehicles. In Proceedings of the 6th Mini Conference on Vehicles System Dynamics, Identification and Anomalies, Budapest, Hungary, 9–11 November 1998; Zobory, I., Ed.; Technical University of Budapest: Budapest, Hungary, 1998; pp. 73–81, ISBN 9634206352. [Google Scholar]
- True, H.; Hansen, T.G.; Lundell, H. On the Quasi-Stationary Curving Dynamics of a Railroad Truck. In Proceedings of the 2005 ASME/IEEE Joint Rail Conference, Pueblo, CO, USA, 16–18 March 2005; IEEE: Piscataway, NJ, USA, 2005; pp. 131–138. [Google Scholar] [CrossRef]
- True, H.; Jensen, J.C. Chaos and asymmetry in railway vehicle dynamics. Period. Polytech. Ser. Transp. Eng. 1994, 22, 55–68. [Google Scholar]
- True, H.; Jensen, J.C. Parameter study of hunting and chaos in railway vehicle dynamics. Veh. Syst. Dyn. 1994, 23 (Suppl. S1), 508–520. [Google Scholar] [CrossRef]
- True, H.; Jensen, J.C.; Slivsgaard, E. Non-linear Railway Dynamics and Chaos. In Proceedings of the 5th Mini Conference on Vehicles System Dynamics, Identification and Anomalies, Budapest, Hungary, 11–13 November 1996; Zobory, I., Ed.; Technical University of Budapest: Budapest, Hungary, 1996; pp. 51–60. [Google Scholar]
- True, H.; Trzepacz, L. The dynamics of railway freight wagon wheelset with dry friction damping in the suspension. Veh. Syst. Dyn. 2004, 41, 587–596. [Google Scholar]
- Uyulan, C.; Gokasan, M.; Bogosyan, S. Dynamic Investigation of the Hunting Motion of a Railway Bogie in a Curved Track via Bifurcation Analysis. Math. Probl. Eng. 2017, 2017, 8276245. [Google Scholar] [CrossRef]
- Xu, G.; Steindl, A.; Troger, H. Nonlinear stability analysis of a bogie of a low-platform wagon. Veh. Syst. Dyn. 1992, 20 (Suppl. S1), 653–665. [Google Scholar] [CrossRef]
- Zeng, J.; Wu, P. Stability analysis of high speed railway vehicles. JSME Int. J. Ser. C Mech. Syst. Mach. Elem. Manuf. 2004, 47, 464–470. [Google Scholar] [CrossRef]
- Zeng, J.; Wu, P. Stability of high-speed train. J. Traffic Transp. Eng. 2005, 5, 464–470. [Google Scholar]
- Zhang, T.; True, H.; Dai, H. The influence of the perturbation on wheel rotation speed on the stability of a railway bogie on steady curve sections of a track. Veh. Syst. Dyn. 2019, 57, 425–443. [Google Scholar] [CrossRef]
- Zhang, T.; True, H.; Dai, H. The lateral dynamics of a nonsmooth railway wheelset model. Int. J. Bifurc. Chaos 2018, 28, 1850095. [Google Scholar] [CrossRef]
- Ebeling, K. High-speed Railways in Germany. Jpn. Railw. Transp. Rev. 2005, 40, 36–45. [Google Scholar]
- Yu, C.W.; Menz, N.; Hunter, C.; Chen, Y.Y.; Farias, P. An analysis of the Chinese High-Speed Rail from the American perspective. In Proceedings of the 53rd Annual Transportation Research Forum, Tampa, FL, USA, 15–17 March 2012; p. 207119. [Google Scholar]
- Jiao, J.; Harbin, J.; Li, Y. Fast Tracks: A Comparison of High Speed Rail in China, Europe and the United States. J. Transp. Technol. 2013, 3, 57–62. [Google Scholar] [CrossRef]
- Sone, S. Comparison of the technologies of the Japanese Shinkansen and Chinese High-speed Railways. J. Zhejiang Univ. Sci. A 2015, 16, 769–780. [Google Scholar] [CrossRef]
- Purba, A.; Nakamura, F.; Niken, C.; Jafri, M.; Pratomo, P. A current review of high speed railways experiences in Asia and Europe. AIP Conf. Proc. 2017, 1903, 060004. [Google Scholar] [CrossRef]
- Zembri, P.; Libourel, E. Towards oversized high-speed rail systems? Some lessons from France and Spain. Transp. Res. Procedia 2017, 25, 368–385. [Google Scholar] [CrossRef]
- Wang, K.; Liu, P. Lateral stability Analysis of Heavy-Haul Vehicle on Curved Track Based on Wheel/Rail Coupled Dynamics. J. Transp. Technol. 2012, 2, 150–157. [Google Scholar] [CrossRef]
- Dusza, M. The study of track gauge influence on lateral stability of 4-axle rail vehicle model. Arch. Transp. 2014, 30, 7–20. [Google Scholar] [CrossRef]
- Zboiński, K.; Gołofit-Stawińska, M. The impact of primary suspension stiffness of 2-axle bogie of MKIII passenger car on its dynamical behaviour. Transp. Probl. 2018, 13, 135–146. [Google Scholar] [CrossRef]
- Zboinski, K.; Golofit-Stawinska, M. Investigation into nonlinear phenomena for various railway vehicles in transition curves at velocities close to critical one. Nonlinear Dyn. 2019, 98, 1555–1601. [Google Scholar] [CrossRef]
- Carballeira, J.; Baeza, L.; Rovira, A.; Garcia, E. Technical characteristics and dynamic modelling of Talgo trains. Veh. Syst. Dyn. 2008, 46 (Suppl. S1), 301–316. [Google Scholar] [CrossRef]
- Droździel, J.; Sowiński, B. Railway car dynamic response to track transition curve and single standard turnout. In Computers in Railways X. Computer System Design and Operation in the Railway and Other Transit Systems; Allen, J., Brebbia, C.A., Rumsey, A.F., Sciutto, G., Sone, S., Goodman, C.J., Eds.; WIT Transactions on The Built Environment; WIT Press: Billerica, MA, USA, 2006; Volume 88, pp. 849–858. [Google Scholar] [CrossRef][Green Version]
- Kondo, O.; Yamazaki, Y. Simulation Technology for Railway Vehicle Dynamics; Nippon Steel & Sumitomo Metal Technical Report, Report No. 105; Nippon Steel & Sumitomo Metal: Tokyo, Japan, 2013. [Google Scholar]
- Kuba, T.; Lugner, P. Dynamic behaviour of tramways with different kinds of bogies. Veh. Syst. Dyn. 2012, 50, 277–289. [Google Scholar] [CrossRef]
- Kurzeck, B.; Hecht, M. Dynamic simulation of friction-induced vibrations in a light railway bogie while curving compared with measurement results. Veh. Syst. Dyn. 2010, 48 (Suppl. S1), 121–138. [Google Scholar] [CrossRef]
- Lau, A.; Kassa, E. Simulation of Vehicle-Track Interaction in Small Radius Curves and Switches and Crossings. In Proceedings of the Third International Conference on Railway Technology: Research, Development and Maintenance, Cagliari, Italy, 5–8 April 2016; Civil-Comp Press: Edinburgh, Scotland, 2016; p. 126. [Google Scholar] [CrossRef]
- Matsumoto, A.; Michitsuji, Y. Flange-Climb derailments of Freight Trains on Curved Tracks Due to Rolling. In Proceedings of the 10th International Conference on Railway Bogies and Running Gears, Budapest, Hungary, 12–15 September 2016; Scientific Society of Mechanical Engineers: New York, NY, USA, 2016; pp. 91–100. [Google Scholar]
- Prandi, D. Railway Bogie Stability Control From Secondary Yaw Actuators. Master’s Thesis, Politecnico di Milano, Scuola di Ingegneria Industriale e dell’Informazione, Milan, Italy, 2014; 64p. [Google Scholar]
- Shaltout, R.; Ulianov, C.; Baeza, L. Development of a Simulation Tool for the Dynamic Analysis of Railway Vehicle-Track Interaction. Transp. Probl. 2015, 10, 47–58. [Google Scholar] [CrossRef]
- Zhai, W.M.; Wang, K.Y. Lateral interactions of trains and tracks on small radius curves: Simulation and experiment. Veh. Syst. Dyn. 2006, 44 (Suppl. S1), 520–530. [Google Scholar] [CrossRef]
- Xia, F.; True, H. On the dynamics of the three-piece-freight truck. In Proceedings of the 2003 ASME/IEEE Joint Railroad Conference, Chicago, IL, USA, 24 April 2003; pp. 149–159. [Google Scholar] [CrossRef]
- Ahmadian, M.; Yang, S. Hopf bifurcation and hunting behavior in a rail wheelset with flange contact. Nonlinear Dyn. 1998, 15, 15–30. [Google Scholar] [CrossRef]
- Chudzikiewicz, A.; Sowiński, B. Modelling and simulation of trams bogies with fully independently rotating wheels. In The Dynamics of Vehicles on Roads and Tracs—Proceeding of the 24th IAVSD Symp; Rosenberger, M., Plochl, M., Six, K., Edelmann, J., Eds.; CRC Press: Boca Raton, FL, USA; Taylor & Francis Group: London, UK, 2016; pp. 1427–1434. ISBN 978-1-138-02885-2. [Google Scholar]
- Dumitriu, M. Influence of the longitudinal and lateral suspension damping on the vibration behaviour in the railway vehicles. Arch. Mech. Eng. 2015, 62, 115–140. [Google Scholar] [CrossRef]
- Froment, O.; Aubry, D.; Castel, L. Analysis of the stability of nonlinear railways dynamics. In Computational Mechanics, New Trends and Applications; Idelsohn, S., Onate, E., Dvorkin, E., Eds.; CIMNE: Barcelona, Spain, 1998; pp. 1–9. [Google Scholar]
- Sedighi, H.M.; Shirazi, K.H. Bifurcation analysis in hunting dynamical behaviour in a railway bogie: Using novel exact equivalent functions for discontinuous nonlinearities. Sci. Iran. Trans. B Mech. Eng. 2012, 19, 1493–1501. [Google Scholar] [CrossRef]
- Warczek, J.; Burdzik, R.; Peruń, G. The method for identification of damping coefficient of the trucks suspension. Key Eng. Mater. 2014, 588, 281–289. [Google Scholar] [CrossRef]
- Bustos, A.; Tomas-Rodriguez, M.; Rubio, H.; Castejon, C. On the nonlinear hunting stability of a high-speed train boogie. Nonlinear Dyn. 2023, 111, 2059–2078. [Google Scholar] [CrossRef]
- Chernysheva, Y.; Gorskiy, A. Methods Proposed for Analysis of Vibrations of Railway Cars. In International Scientific SiberianTransport Forum TransSiberia—2021. TransSiberia 2021; Manakov, A., Edigarian, A., Eds.; Lecture Notes in Networks and Systems; Springer: Cham, Switzerland, 2022; Volume 402. [Google Scholar] [CrossRef]
- Ge, P.; Wie, X.; Liu, J.; Cao, H. Bifurcation of a modified railway wheelset model with nonlinear equivalent conicity and wheel–rail force. Nonlinear Dyn. 2020, 102, 79–100. [Google Scholar] [CrossRef]
- Guo, J.; Shi, H.; Luo, R.; Zeng, J. Bifurcation analysis of a railway wheelset with nonlinear wheel–rail contact. Nonlinear Dyn. 2021, 104, 989–1005. [Google Scholar] [CrossRef]
- Guo, J.; Zhang, G.; Shi, H.; Zeng, J. Small amplitude bogie hunting identification method for high-speed trains based on machine learning. Veh. Syst. Dyn. 2023. [Google Scholar] [CrossRef]
- Pandey, M.; BBhattacharya, B. Effect of bolster suspension parameters of three-piece freight bogie on the lateral frame force. Int. J. Rail Transp. 2020, 8, 45–65. [Google Scholar] [CrossRef]
- Shvets, A.O. Dynamic interaction of a freight car body and a three-piece bogie during axle load increase. Veh. Syst. Dyn. 2022, 60, 3291–3313. [Google Scholar] [CrossRef]
- Skerman, D.; Colin Cole, C.; Spiryagin, M. Determining the critical speed for hunting of three-piece freight bogies: Practice versus simulation approaches. Veh. Syst. Dyn. 2022, 60, 3314–3335. [Google Scholar] [CrossRef]
- Sun, J.; Chi, M.; Jin, X.; Liang, S.; Wang, J.; Li, W. Experimental and numerical study on carbody hunting of electric locomotive induced by low wheel–rail contact conicity. Veh. Syst. Dyn. 2021, 59, 203–223. [Google Scholar] [CrossRef]
- Sun, J.; Meli, E.; Song, X.; Chi, M.; Jiao, W.; Jiang, Y. A novel measuring system for high-speed railway vehicles hunting monitoring able to predict wheelset motion and wheel/rail contact characteristics. Veh. Syst. Dyn. 2023, 61, 1621–1643. [Google Scholar] [CrossRef]
- Umemoto, J.; Yabuno, H. Parametric and self-excited oscillation produced in railway wheelset due to mass imbalance and large wheel tread angle. Nonlinear Dyn. 2023, 111, 4087–4106. [Google Scholar] [CrossRef]
- Wang, X.; Lu, Z.; Wen, J.; Wie, J.; Wang, Z. Kinematics modelling and numerical investigation on the hunting oscillation of wheel-rail nonlinear geometric contact system. Nonlinear Dyn. 2022, 107, 2075–2097. [Google Scholar] [CrossRef]
- Yang, C.; Huang, Y.; Li, F. Influence of Curve Geometric Parameters on Dynamic Interactions of Side-Frame Cross-Braced Bogie. In Proceedings of the ICRT 2021, Second International Conference on Rail Transportation, Chengdu, China, 5–6 July 2021. [Google Scholar]
- Zboinski, K. Modelling dynamics of certain class of discrete multi-body systems based on direct method of the dynamics of relative motion. Meccanica 2012, 47, 1527–1551. [Google Scholar] [CrossRef][Green Version]
- Zboinski, K. Modeling, Simulation, and Results of Their Use in Railway Vehicle Dynamics Studies. In Railway Research—Selected topics on Development, Safety and Technology; Zboinski, K., Ed.; InTech: Rijeka, Croatia, 2015; pp. 165–195. [Google Scholar] [CrossRef][Green Version]

| No. | Problem Name | Initial Date |
|---|---|---|
| 1 | The first documented research on the kinematics of rail vehicle conical wheelset | Beginning of 19th century |
| 2 | Derivation of the formula for the wavelength of conical wheelset lateral vibrations referred to as sinusoidal or hunting motion | 1883 |
| 3 | The first stability research of rail vehicles with kinematic relations in the wheel–rail contact of conical wheels with flanges, taking account of Culomb’s friction in wheel–rail contact | 1887 |
| 4 | Explanation of the hunting motion with account taken of linear and then non-linear creep-force law in the contact | ~1926 |
| 5 | Start of research into the stability of locomotives | 1928 |
| 6 | The first research into high-speed rail in Japan | ~1945 |
| 7 | The research on high-speed rail in Europe | 1950s |
| 8 | Non-linear contact theory of rolling contact of an elastic spherical surface on a flat surface | 1958 |
| 9 | Research into the stability of high-speed rail bogie (and vehicle) with flexibly guided wheelsets, and comprehension of the difference between forced vibrations and self-exciting vibrations | 1960, 1965 |
| 10 | Stability studies of linear systems and then the appearance of the critical velocity notion | 1965 |
| 11 | Development of programs for the Automatic Generation of Equations of Motion (AGEM): simulation and analysis of the rail vehicle dynamics (stability) | 1970s |
| 12 | Stability studies with account taken of non-linearities: “quasi-linearization” technique | 1976 |
| 13 | Developing the MEDYNA program (based on the theory of one-wheel contact with one rail) | 1976 |
| 14 | Bifurcation methods in the stability study: first mature study | 1978 |
| 15 | Simulation methods to calculate the limit cycles in dynamics of rail vehicles; introduction of non-linear critical velocity into stability analysis thanks to self-exciting vibrations and bifurcation theories | 1875, 1981 |
| 16 | Developing the FASTSIM program (based on a simplified contact theory) | 1982 |
| 17 | Developing the SIMPACK program (enabling research on driving comfort, stability, and curving performance) | 1980s |
| 18 | Developing the non-linear CONTACT program (which takes into account non-elliptical contact areas) | 1986 |
| 19 | Development of methods and simulation studies on the lateral non-linear stability with use of the bifurcation approach | ~1990 |
| 20 | Application and determination of the non-linear critical velocity as a certain contrast to the linear critical velocity | ~1992 |
| 21 | Study of chaotic solutions presented together with stable and unstable stationary and periodic solutions on bifurcation diagrams | ~1992 |
| 22 | Development of methods and studies of stability in CC, including the bifurcation approach | 1998 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zboinski, K.; Golofit-Stawinska, M. Dynamics of a Rail Vehicle in Transition Curve above Critical Velocity with Focus on Hunting Motion Considering the Review of History of the Stability Studies. Energies 2024, 17, 967. https://doi.org/10.3390/en17040967
Zboinski K, Golofit-Stawinska M. Dynamics of a Rail Vehicle in Transition Curve above Critical Velocity with Focus on Hunting Motion Considering the Review of History of the Stability Studies. Energies. 2024; 17(4):967. https://doi.org/10.3390/en17040967
Chicago/Turabian StyleZboinski, Krzysztof, and Milena Golofit-Stawinska. 2024. "Dynamics of a Rail Vehicle in Transition Curve above Critical Velocity with Focus on Hunting Motion Considering the Review of History of the Stability Studies" Energies 17, no. 4: 967. https://doi.org/10.3390/en17040967
APA StyleZboinski, K., & Golofit-Stawinska, M. (2024). Dynamics of a Rail Vehicle in Transition Curve above Critical Velocity with Focus on Hunting Motion Considering the Review of History of the Stability Studies. Energies, 17(4), 967. https://doi.org/10.3390/en17040967






