Effects of Near-Critical Condensation and Cavitation on the Performance of S-CO2 Compressor
Abstract
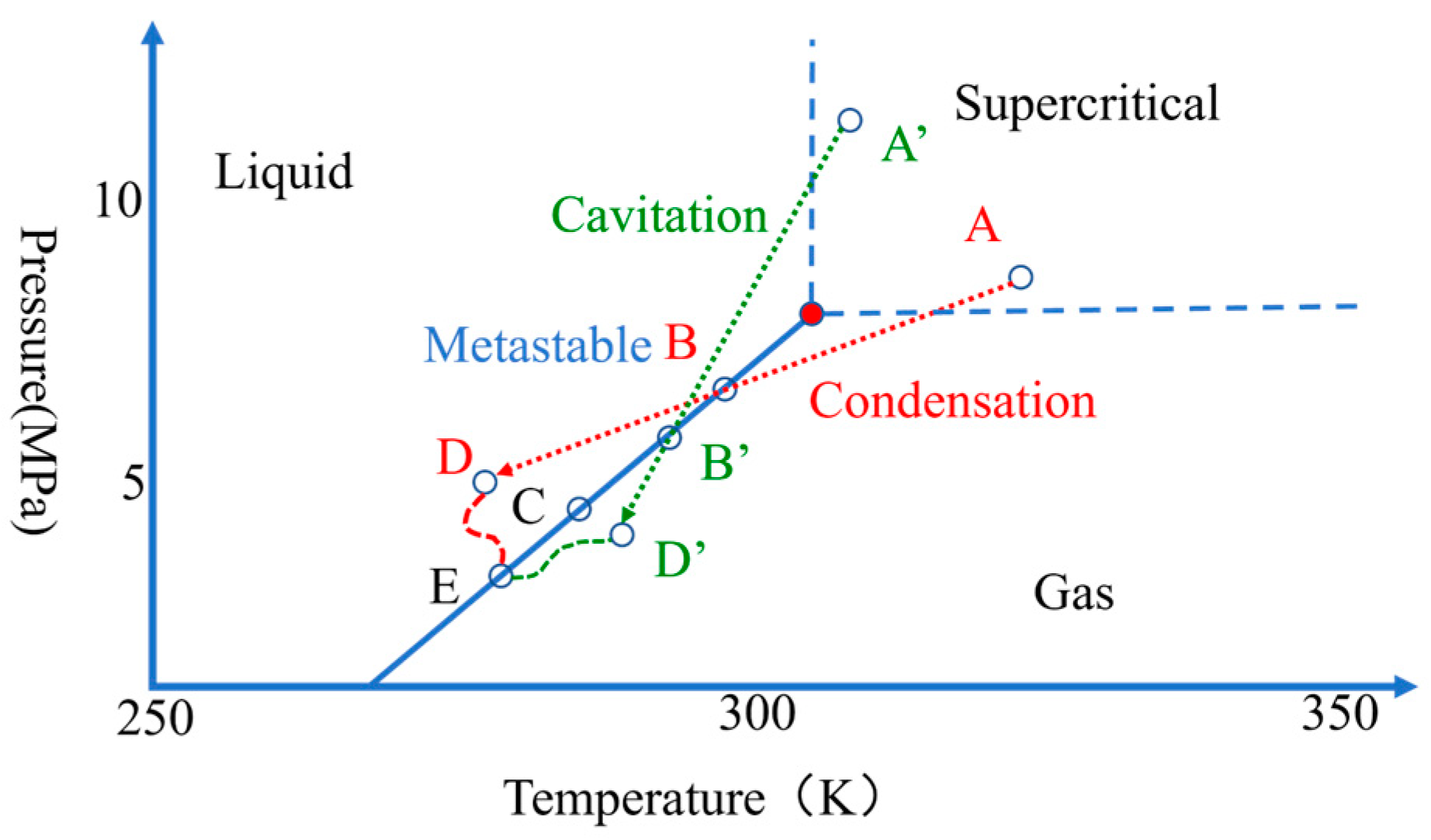
1. Introduction
2. Numerical Investigation
2.1. CO2 Condensation in Lettieri Nozzle
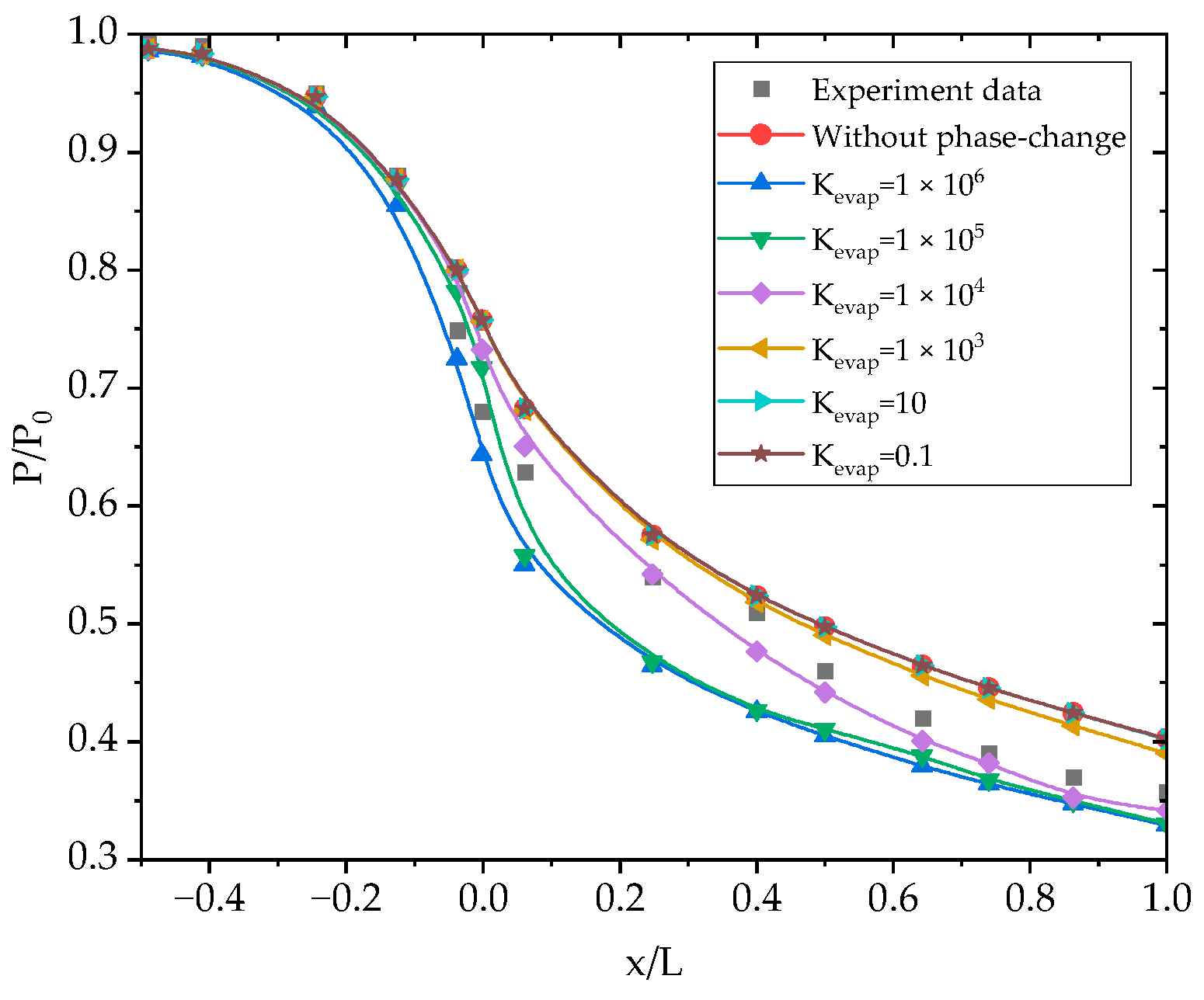
2.1.1. Condensation Model
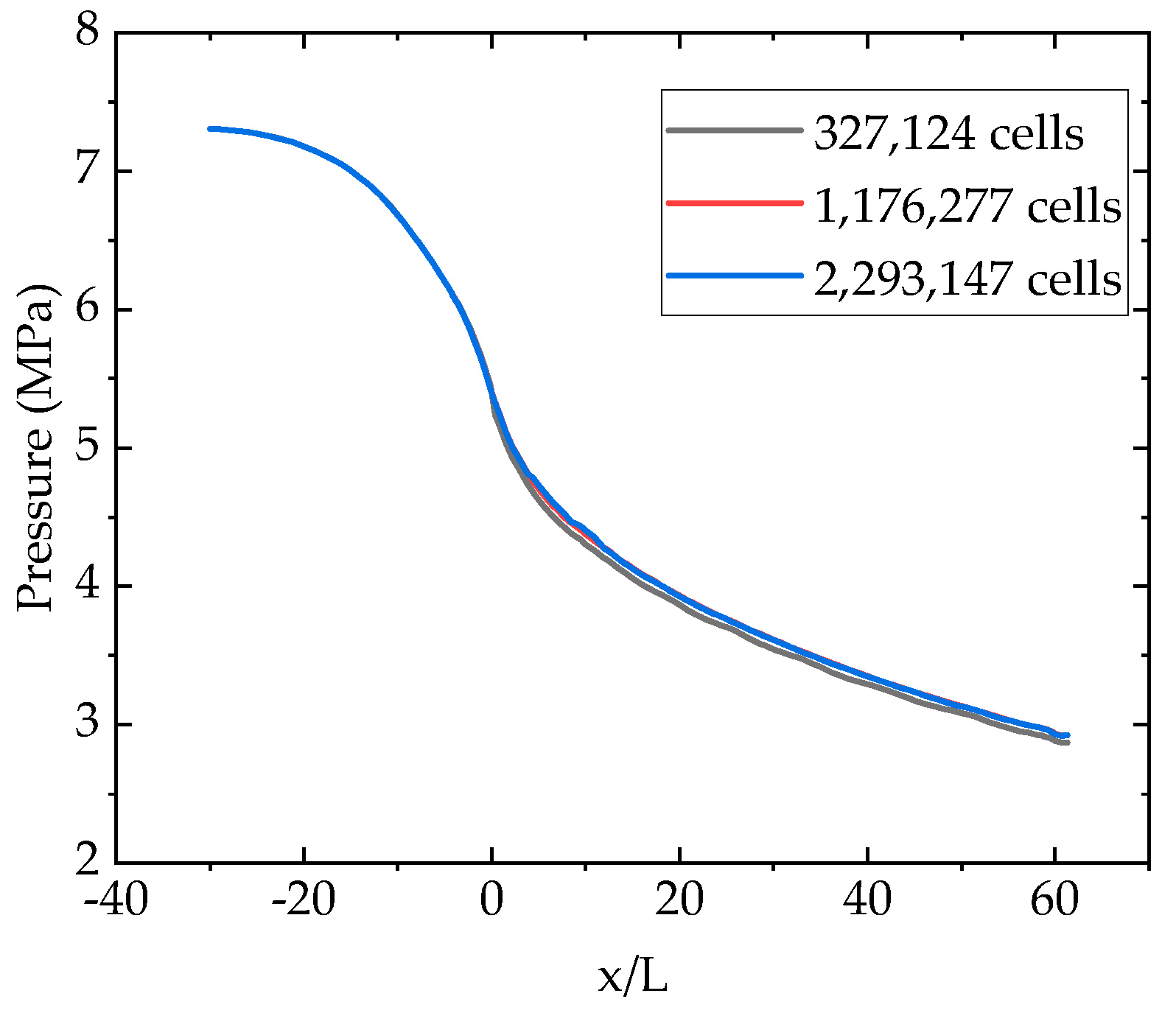
2.1.2. Simulation in Lettieri Nozzle
2.2. Verification of Sandia Compressor Simulation
3. Main Compressor Simulation
3.1. Geometry of Main Compressor
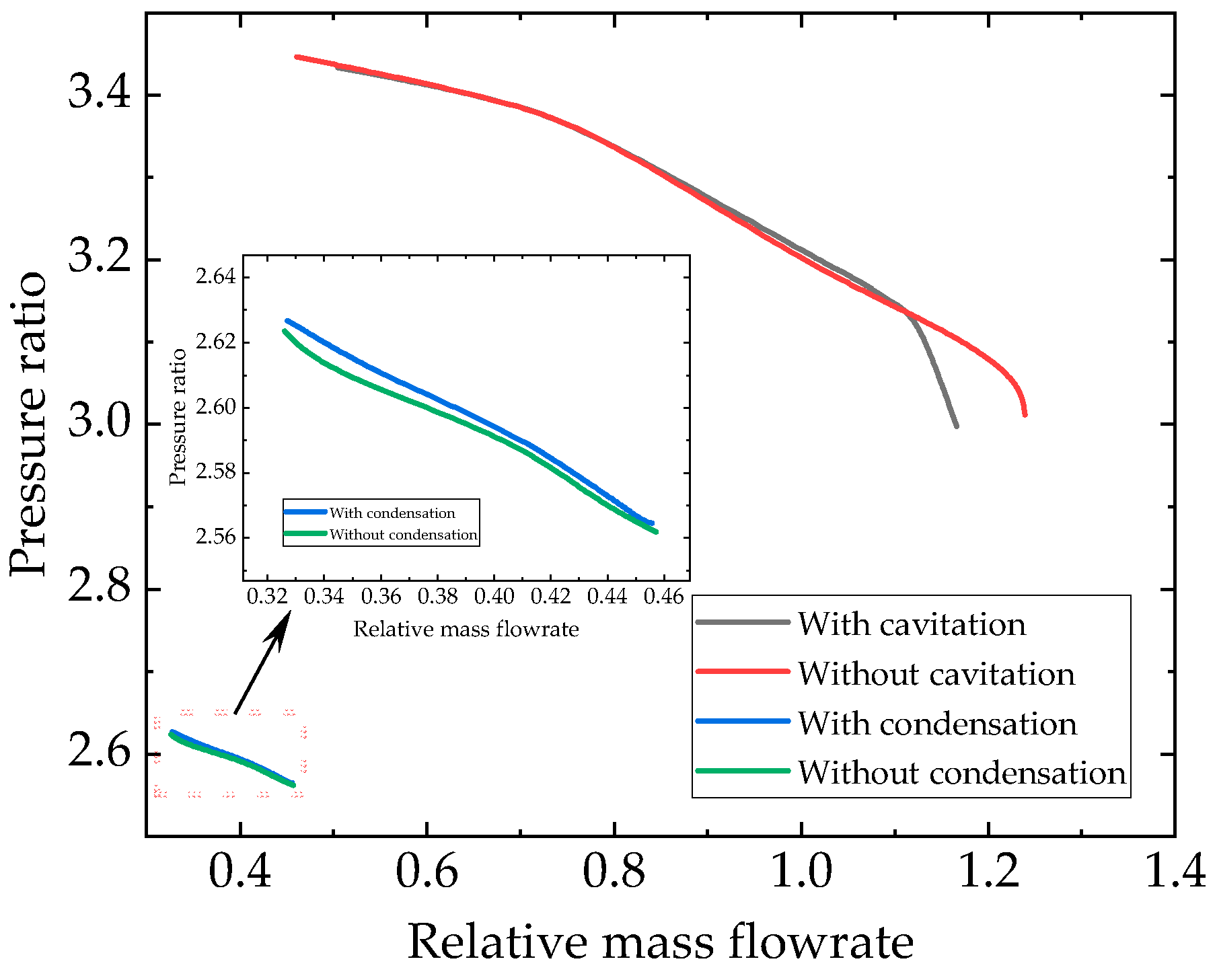
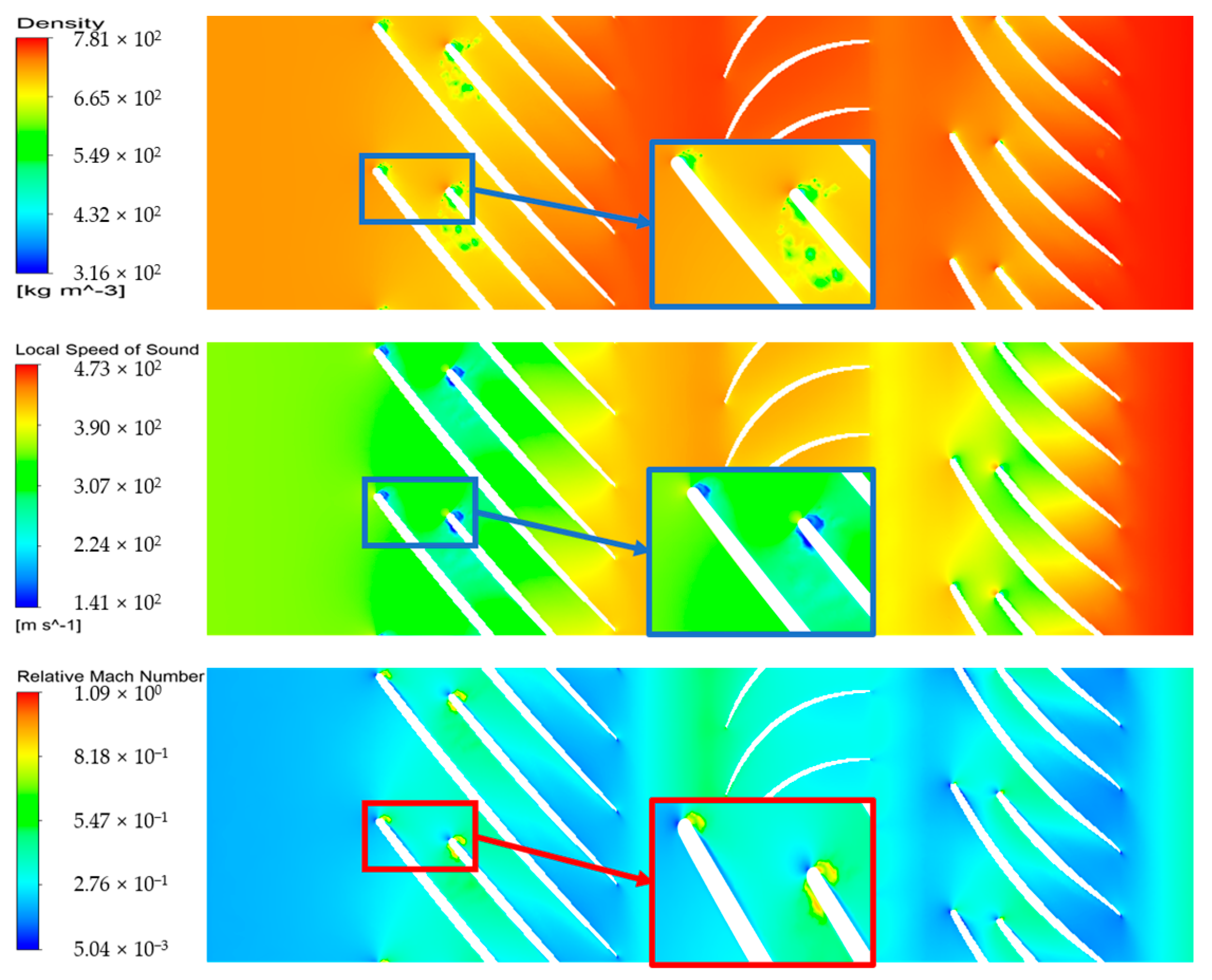
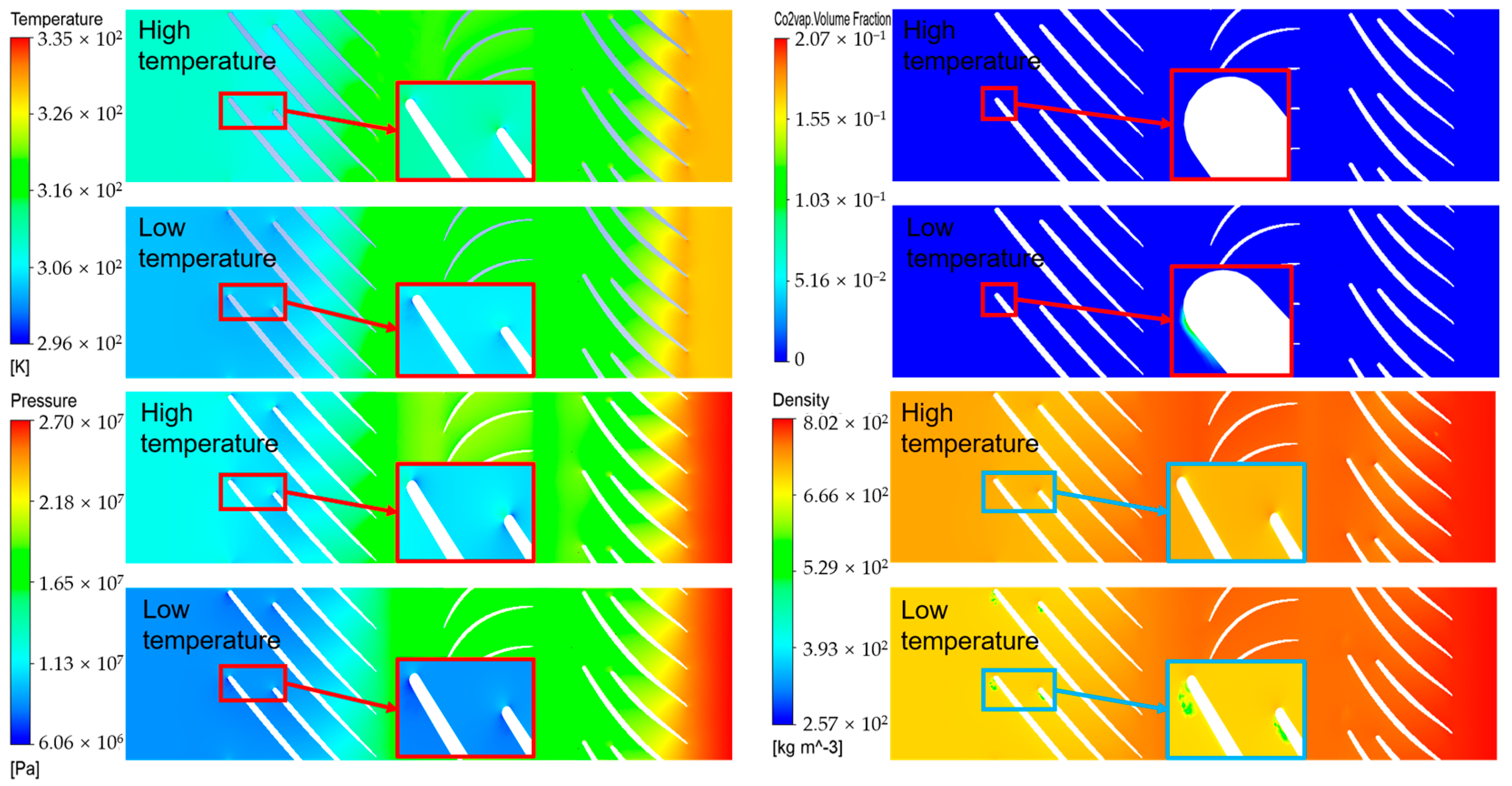
3.2. Numerical Simulation for Different Inlet Conditions
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Pt | total pressure |
| Tt | total temperature |
| S | entropy |
| Pc | pressure of CO2 critical point |
| Tc | temperature of CO2 critical point |
| Sc | entropy of CO2 critical point |
| P0 | inlet pressure of nozzle |
| L | total length of nozzle |
| N | shaft speed |
| mr | relative mass flow rate |
| CFD | computational fluid dynamics |
| RGP | real gas properties |
References
- Cho, J.; Shin, H.; Cho, J.; Kang, Y.S.; Ra, H.S.; Roh, C.; Lee, B.; Lee, G.; Kim, B.; Baik, Y.J. Preliminary experimental study of a supercritical CO2 power cycle test loop with a high-speed turbo-generator using R134a under similarity conditions. Front. Energy 2017, 11, 452–460. [Google Scholar] [CrossRef]
- Cheng, W.L.; Huang, W.X.; Nian, Y.L. Global parameter optimization and criterion formula of supercritical carbon dioxide Brayton cycle with recompression. Energy Convers. Manag. 2017, 150, 669–677. [Google Scholar] [CrossRef]
- Feher, E.G. The supercritical thermodynamic power cycle. Energy Convers. 1968, 8, 85–90. [Google Scholar] [CrossRef]
- Dostal, V.; Driscoll, M.J.; Hejzlar, P. A Supercritical Carbon Dioxide Cycle for Next Generation Nuclear Reactors. 2004. Available online: https://web.mit.edu/22.33/www/dostal.pdf (accessed on 29 December 2023).
- Poerner, M.; Musgrove, G.; Beck, G. Liquid CO2 Formation, Impact, and Mitigation at the Inlet to a Supercritical CO2 Compressor. In Proceedings of the ASME Turbo Expo: Turbine Technical Conference and Exposition, Seoul, Republic of Korea, 13–17 June 2016; pp. 351–360. [Google Scholar]
- Liu, C.; Zhang, L. Research Progress of Supercritical Carbon Dioxide Centrifugal Compressor. Therm. Power Gener. 2021, 50, 34–42. [Google Scholar]
- Baltadjiev, N.D.; Lettieri, C.; Spakovszky, Z.S. An Investigation of Real Gas Effects in Supercritical CO2 Centrifugal Compressors. J. Turbomach. Trans. ASME 2015, 137, 13. [Google Scholar] [CrossRef]
- Pei, J.; Zhao, Y.; Zhao, M.; Liu, G.; Yang, Q.; Li, L. Effects of leading edge profiles on flow behavior and performance of supercritical CO2 centrifugal compressor. Int. J. Mech. Sci. 2022, 229, 107520. [Google Scholar] [CrossRef]
- Rinaldi, E.; Pecnik, R.; Colonna, P. Computational Fluid Dynamic Simulation of a Supercritical CO2 Compressor Performance Map. J. Eng. Gas. Turbines Power-Trans. ASME 2015, 137, 7. [Google Scholar] [CrossRef]
- Lettieri, C.; Spakovszky, Z.; Paxson, D.; Bryanston-Cross, P. Characterization of non-equilibrium condensation of supercritical carbon dioxide in a de Laval nozzle. In Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017. [Google Scholar]
- Gyarmathy, G. Nucleation of steam in high-pressure nozzle experiments. Proc. Inst. Mech. Eng. Part A-J. Power Energy 2005, 219, 511–521. [Google Scholar] [CrossRef]
- Shao, W.Y.; Yang, J.G.; Wang, X.F.; Ma, Z.Y. A real gas-based throughflow method for the analysis of SCO2 centrifugal compressors. Proc. Inst. Mech. Eng. Part C-J. Eng. Mech. Eng. Sci. 2020, 234, 1943–1958. [Google Scholar] [CrossRef]
- Melissa Poerner, P.E. Understanding Wet Gas in A Supercritical Carbon Dioxide Cycle. In Proceedings of the 5th International Symposium—Supercritical CO2 Power Cycles, San Antonio, TX, USA, 28–31 March 2016. [Google Scholar]
- Furusawa, T.; Miyazawa, H.; Moriguchi, S.; Yamamoto, S. NUMERICAL METHOD for SIMULATING HIGH PRESSURE CO2 FLOWS with NONEQUILIBRIUM CONDENSATION. In Proceedings of the ASME Turbo Expo: Turbomachinery Technical Conference and Exposition, Oslo, Norway, 11–15 June 2018. [Google Scholar]
- Anderson, M.R. Atypical Fluid Behavior on the Liquid Side of the Saturation Line of CO2 with Implications for Compressor Design. In Proceedings of the 6th International Supercritical CO2 Power Cycles Symposium, Pittsburgh, PA, USA, 27–29 March 2018. [Google Scholar]
- Persico, G.; Gaetani, P.; Romei, A.; Toni, L.; Bellobuono, E.F.; Valente, R. Implications of phase change on the aerodynamics of centrifugal compressors for supercritical carbon dioxide applications. In Proceedings of the ASME Turbo Expo 2020: Turbomachinery Technical Conference and Exposition, Virtual, 21–25 September 2020; International Gas Turbine Institute: Houston, TX, USA. [Google Scholar]
- Hosangadi, A.; Liu, Z.S.; Weathers, T.; Ahuja, V.; Busby, J. Modeling Multiphase Effects in CO2 Compressors at Subcritical Inlet Conditions. J. Eng. Gas. Turbines Power-Trans. ASME 2019, 141, 13. [Google Scholar] [CrossRef]
- Conboy, T.M.; Wright, S.A. Experimental Investigation of the S-CO2 Condensing Cycle; Sandia National Lab.: Albuquerque, NM, USA, 2011. [Google Scholar]
- Wright, S.; Conboy, T.; Radel, R.; Rochau, G. Modeling and Experimental Results for Condensing Supercritical CO2 Power Cycles; Office of Scientific and Technical Information (OSTI): Oak Ridge, TN, USA, 2011.
- Wright, S.; Radel, R.; Vernon, M.; Pickard, P.; Rochau, G. Operation and Analysis of a Supercritical CO2 Brayton Cycle; Office of Scientific and Technical Information (OSTI): Oak Ridge, TN, USA, 2010.
- Noall, J.S.; Nichols, B.; Pasch, J.J. Achievable Efficiency and Stability of Supercritical CO2 Compression Systems. 2014. Available online: https://sco2symposium.com/papers2014/turbomachinery/51PPT-Noall.pdf (accessed on 29 December 2023).
- Allison, T.C.; McClung, A. Limiting Inlet Conditions for Phase Change Avoidance in Supercritical CO2 Compressors. In Proceedings of the ASME Turbo Expo: Turbomachinery Technical Conference and Exposition, Phoenix, AZ, USA, 17–21 June 2019. [Google Scholar]
- Ansys Inc. Ansys Fluent Theory Guide; Ansys Inc.: Canonsburg, PA, USA, 2022. [Google Scholar]
- Lettieri, C.; Yang, D.; Spakovszky, Z. An Investigation of Condensation Effects in Supercritical Carbon Dioxide Compressors. J. Eng. Gas. Turbines Power-Trans. ASME 2015, 137, 8. [Google Scholar] [CrossRef]
- Brinckman, K.W.; Hosangadi, A.; Liu, Z.S.; Weathers, T. NUMERICAL SIMULATION of NON-EQUILIBRIUM CONDENSATION in SUPERCRITICAL CO2 COMPRESSORS. In Proceedings of the ASME Turbo Expo: Turbomachinery Technical Conference and Exposition, Phoenix, AZ, USA, 17–21 June 2019. [Google Scholar]

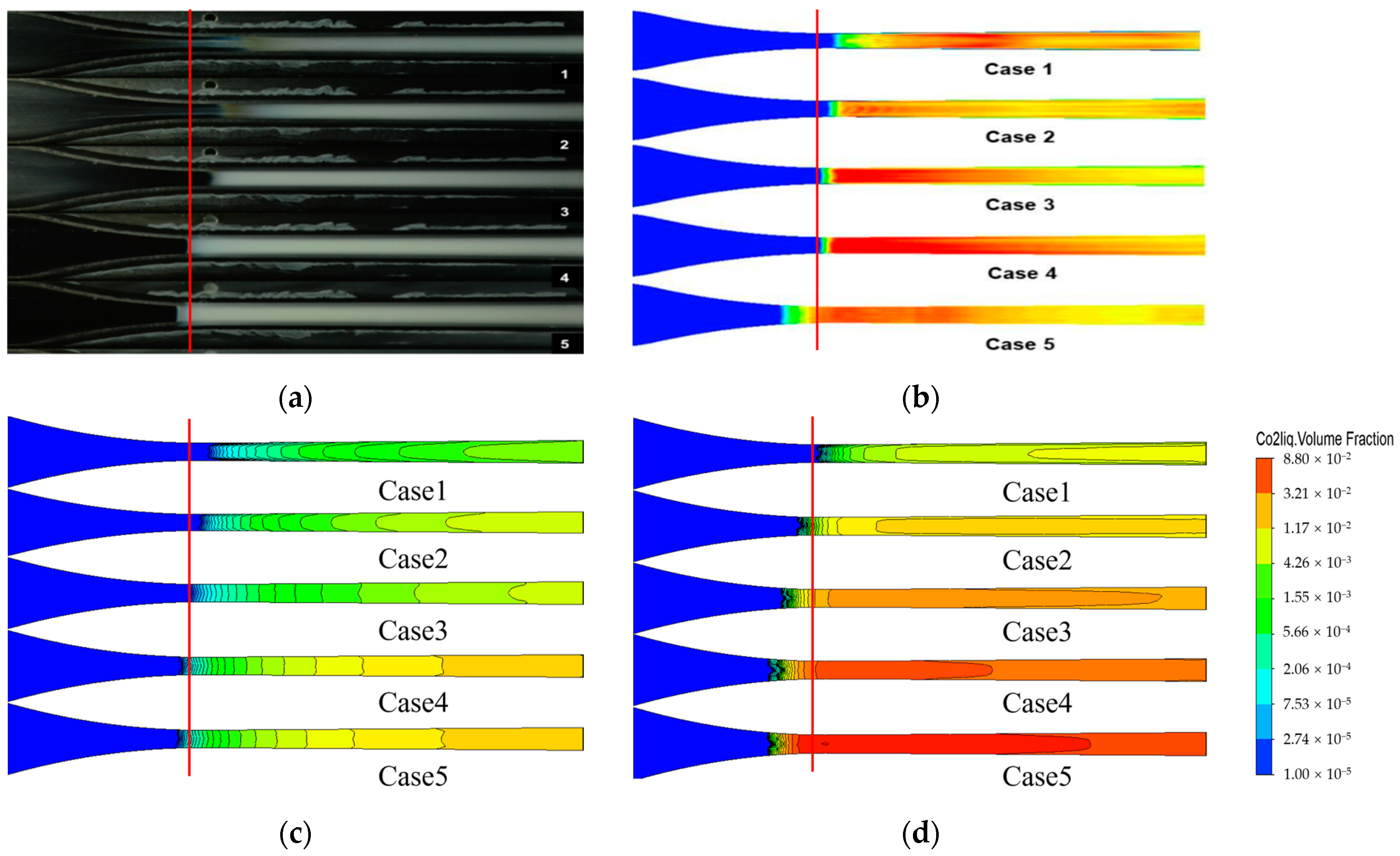
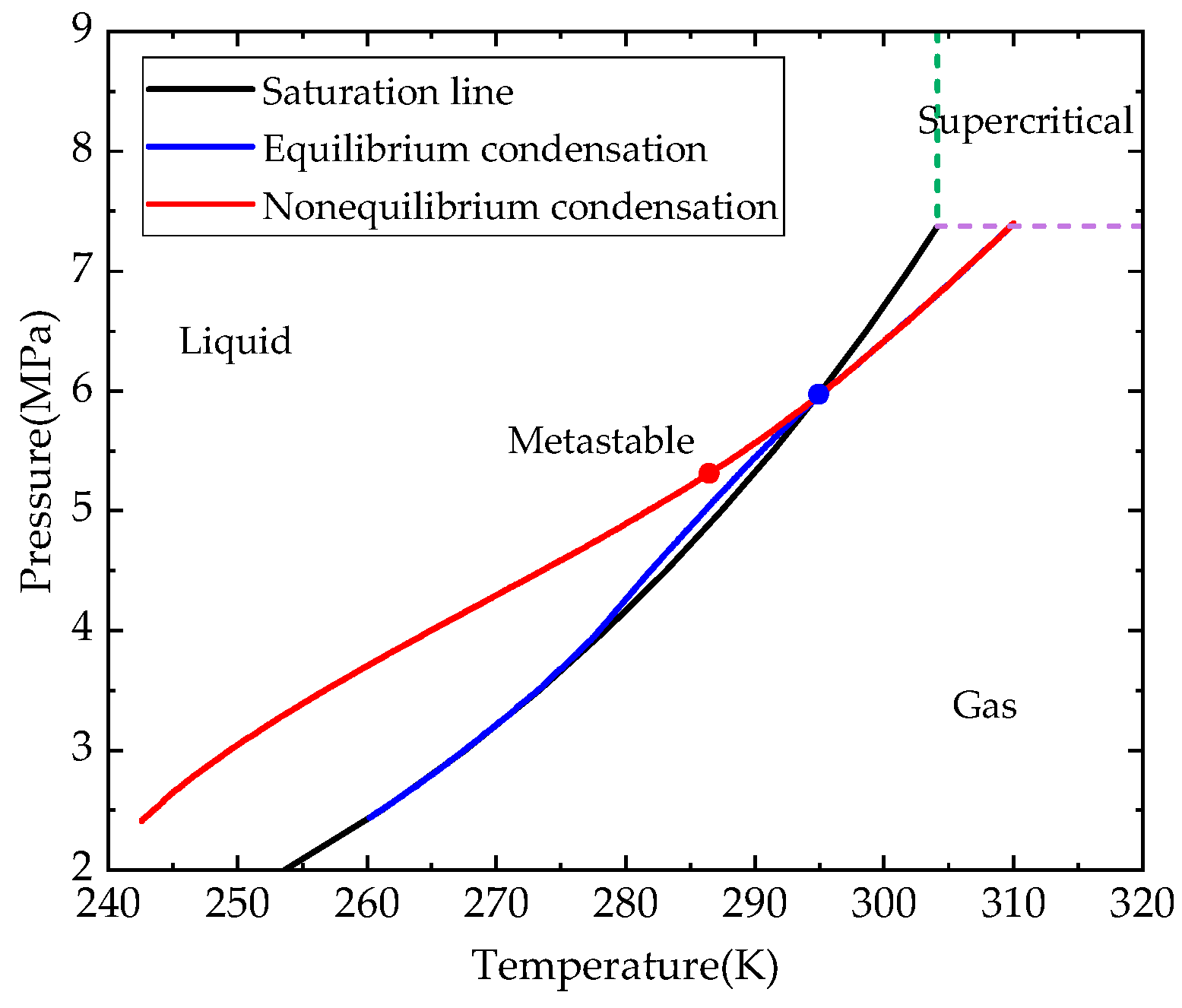
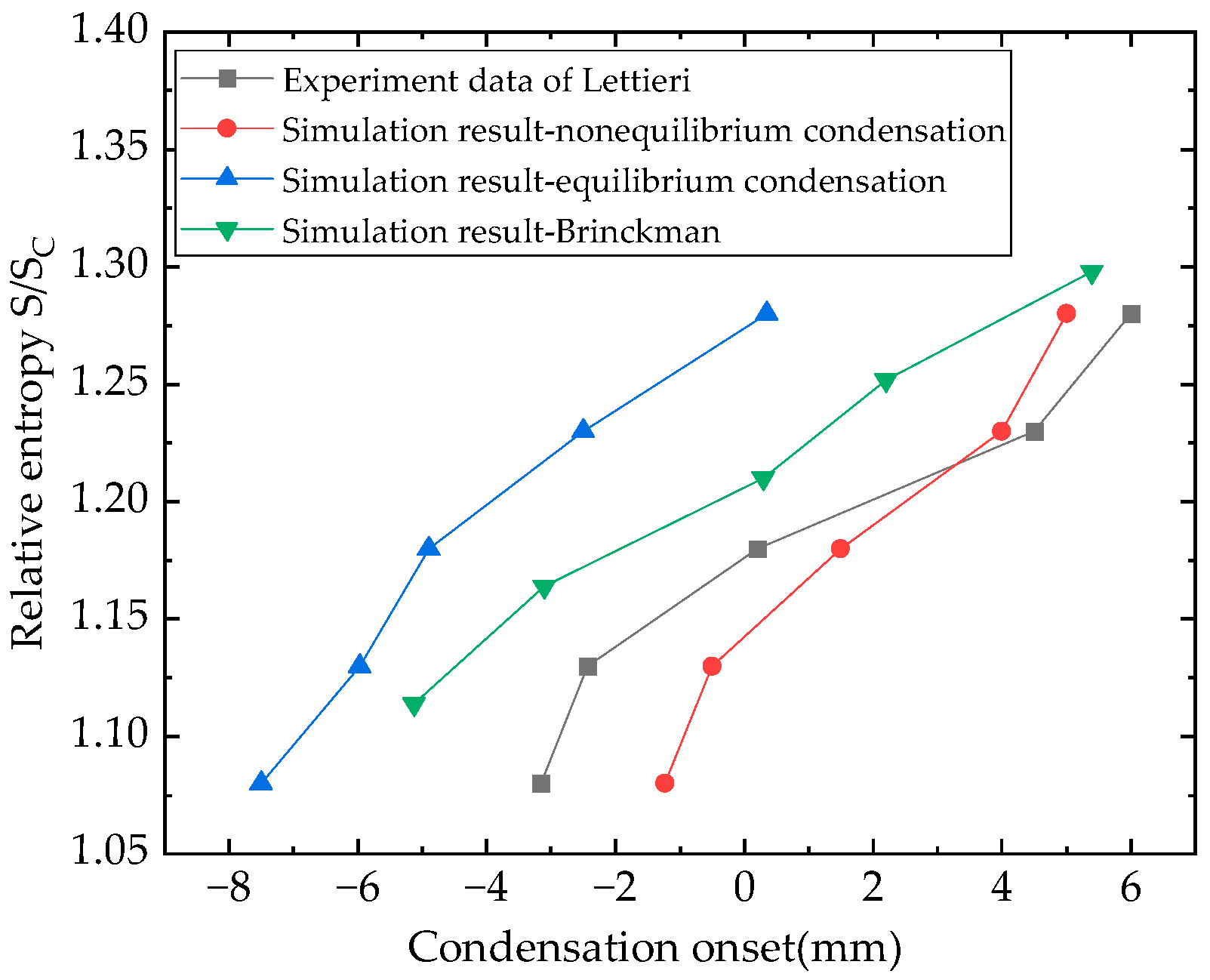


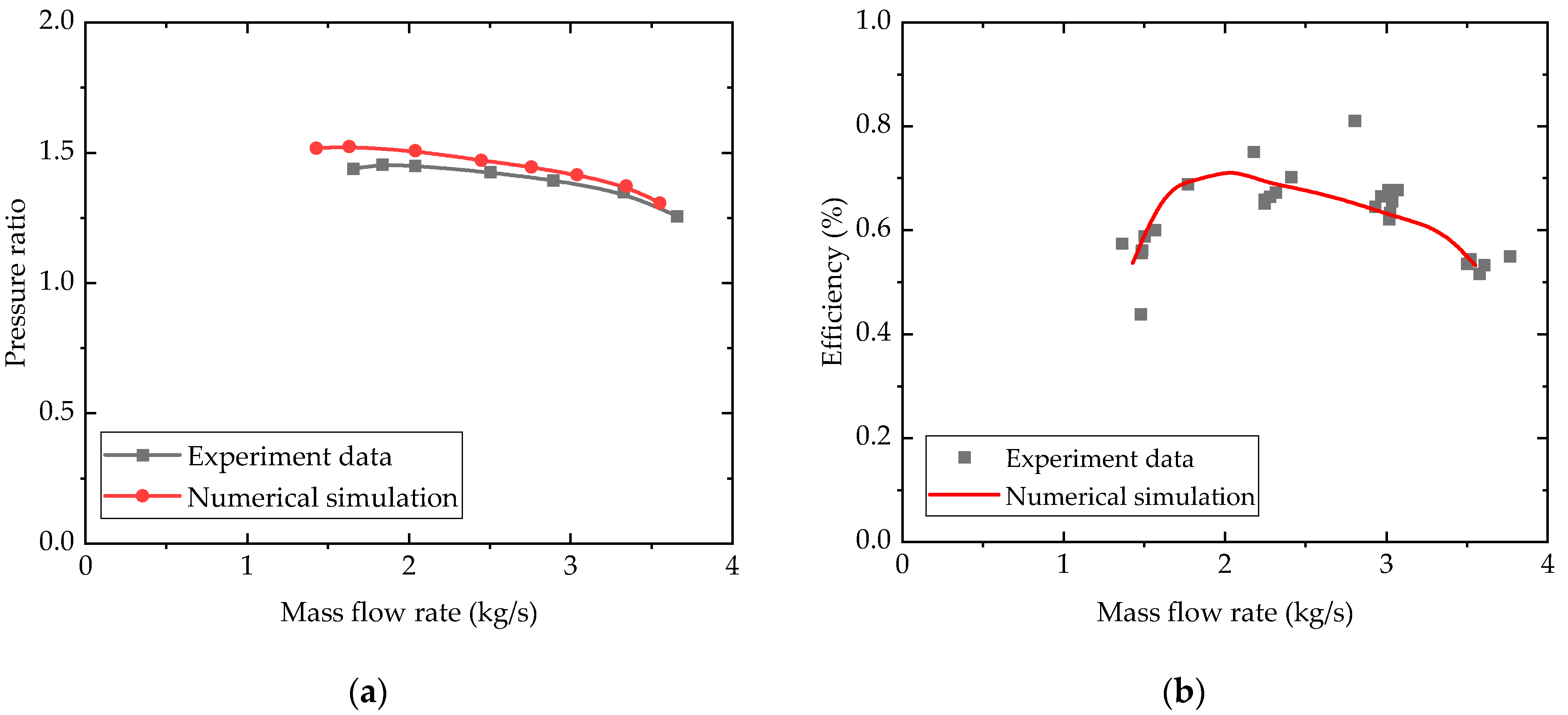


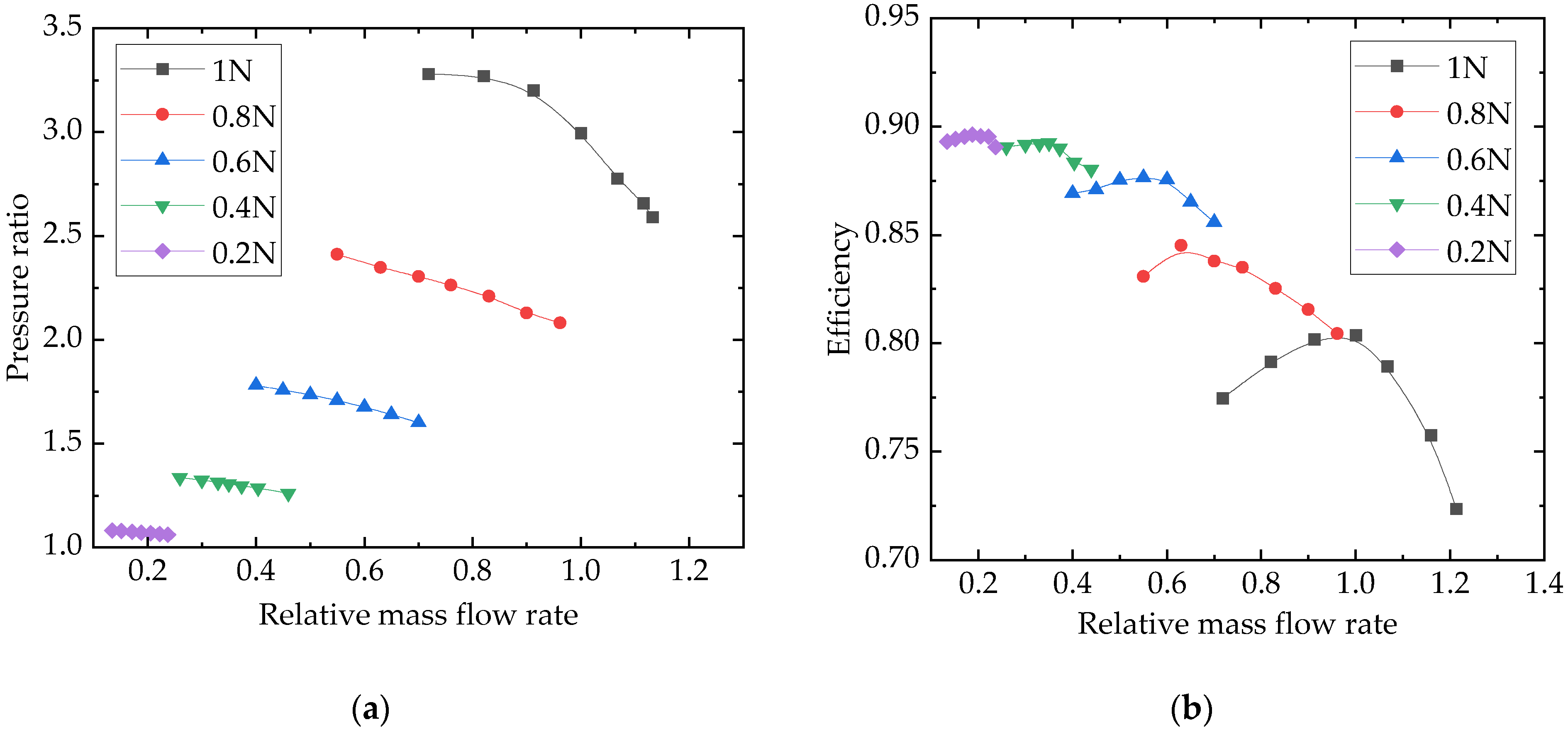
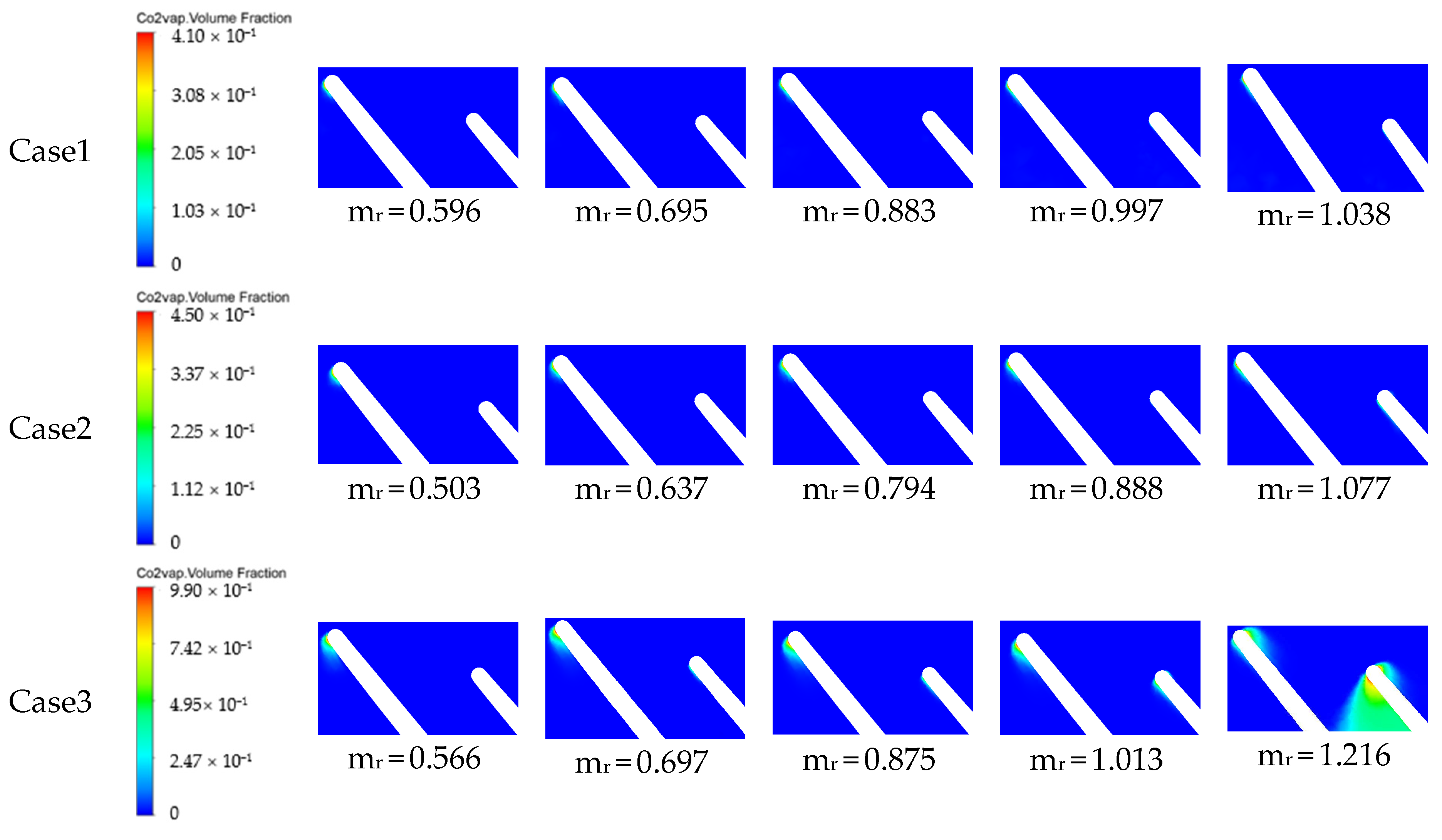
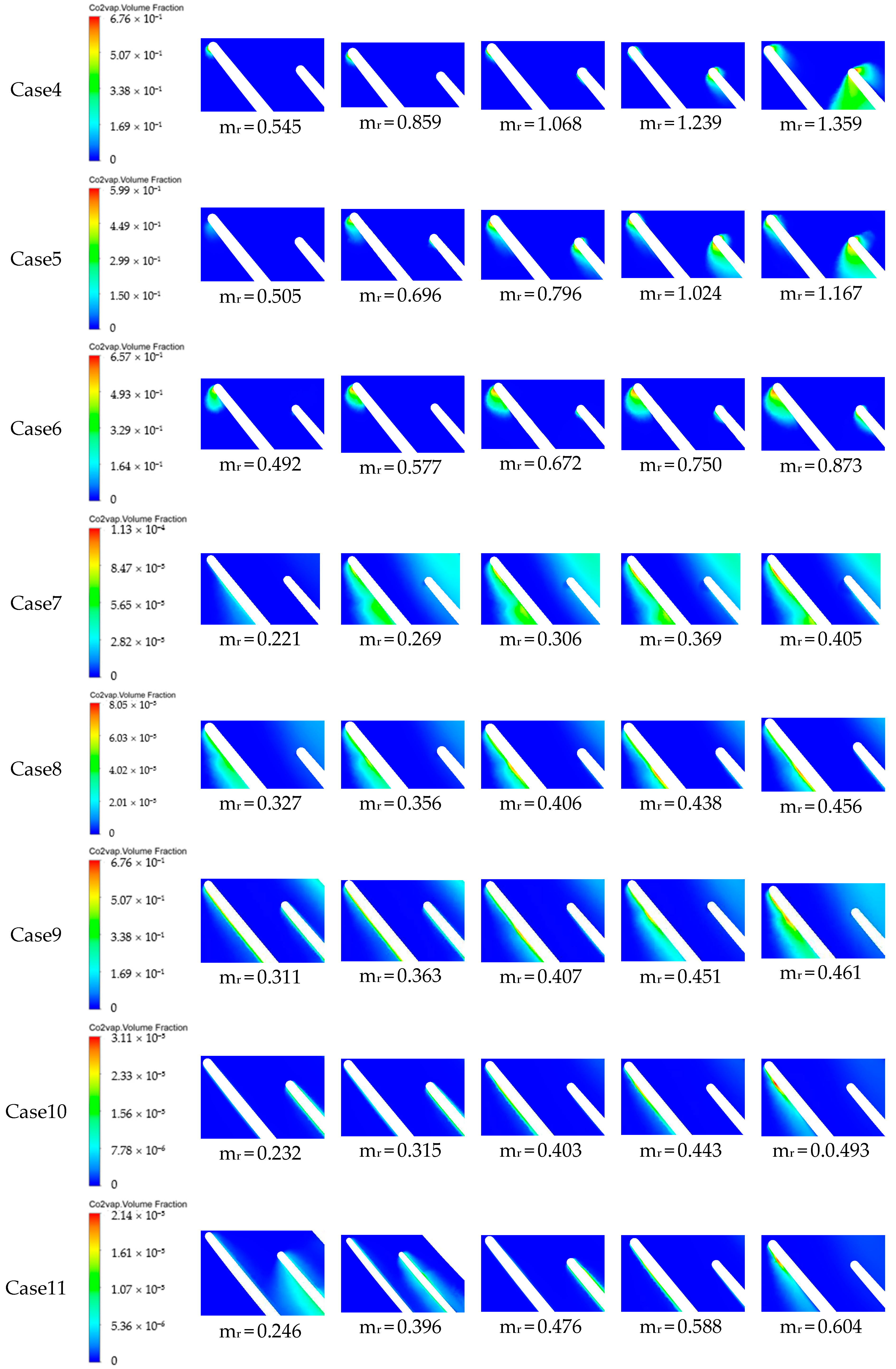
| Case | Pt (MPa) | Tt (K) | S (J/Kg·K) | Pt/PC | Tt/TC | S/SC |
|---|---|---|---|---|---|---|
| 1 | 5.9 | 310 | 1830 | 0.8 | 1.02 | 1.28 |
| 2 | 6.7 | 310 | 1766 | 0.9 | 1.02 | 1.23 |
| 3 | 7.4 | 310 | 1695 | 1.0 | 1.02 | 1.18 |
| 4 | 8.0 | 311 | 1634 | 1.1 | 1.025 | 1.13 |
| 5 | 8.4 | 312 | 1548 | 1.2 | 1.027 | 1.08 |
| Parameters | Value |
|---|---|
| Number of main blades | 6 |
| Inlet hub radius (mm) | 2.537 |
| Inlet hub angle at tip (°) | 17.88 |
| Exit blade radius (mm) | 18.682 |
| Tip clearance (mm) | 0.254 |
| Exit blade back-sweep angle (°) | −50 |
| Number of splitters | 6 |
| Inlet shroud radius (mm) | 9.372 |
| Inlet blade angle at tip (°) | 50 |
| Exit blade width (mm) | 1.954 |
| Blade thickness (mm) | 0.762 |
| Shaft length (mm) | 15.9 |
| Parameters | Value |
|---|---|
| Mass flow rate (kg/s) | 26.0 |
| Inlet pressure (MPa) | 8.0 |
| Inlet temperature (K) | 306.15 |
| Outlet pressure (MPa) | 24.0 |
| Rotation speed (rpm) | 25,000 |
| Pressure ratio | 3.0 |
| Choke mass flow rate at rated rotation speed (kg/s) | 30.5 |
| Surge mass flow rate at rated rotation speed (kg/s) | 17.5 |
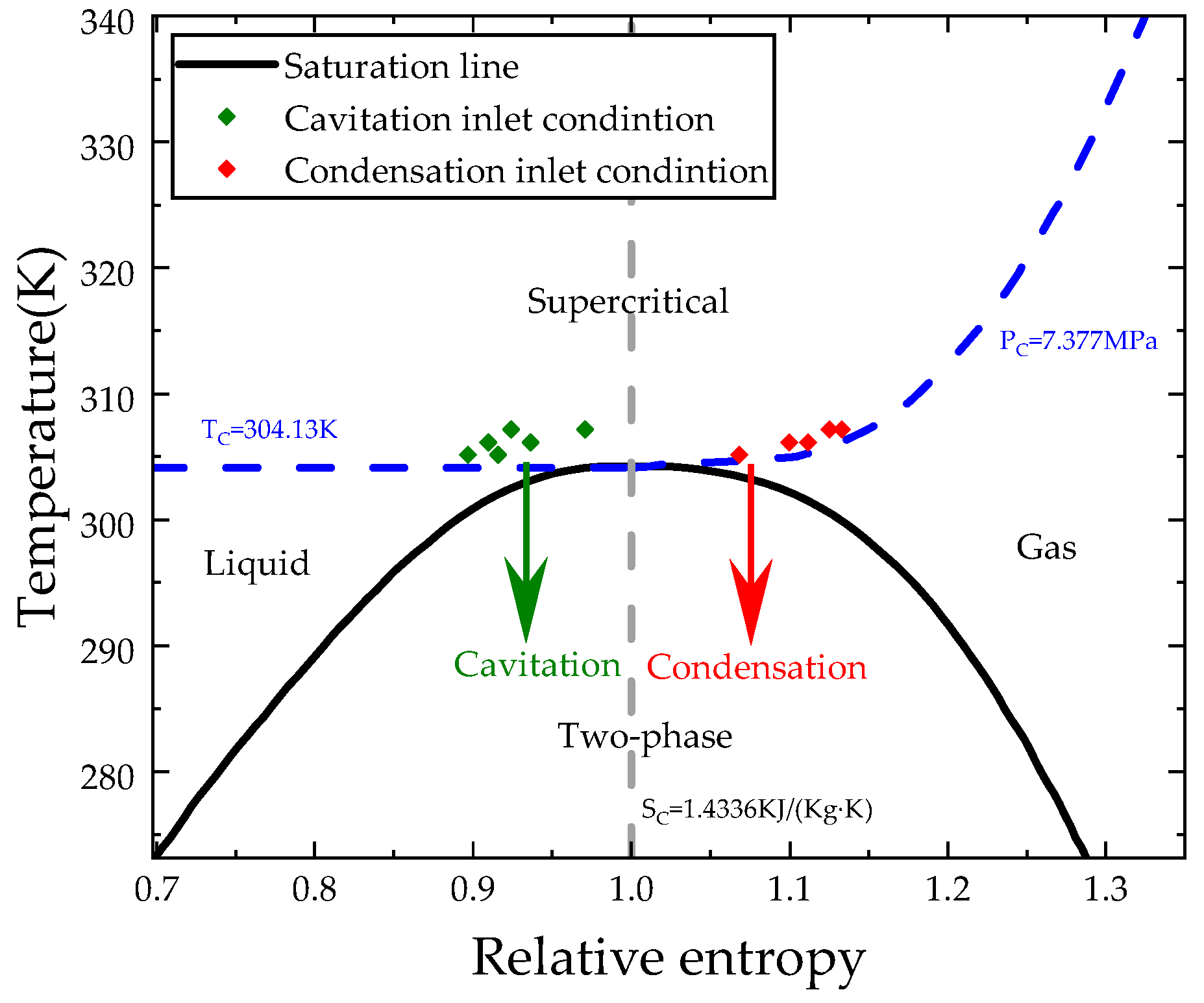
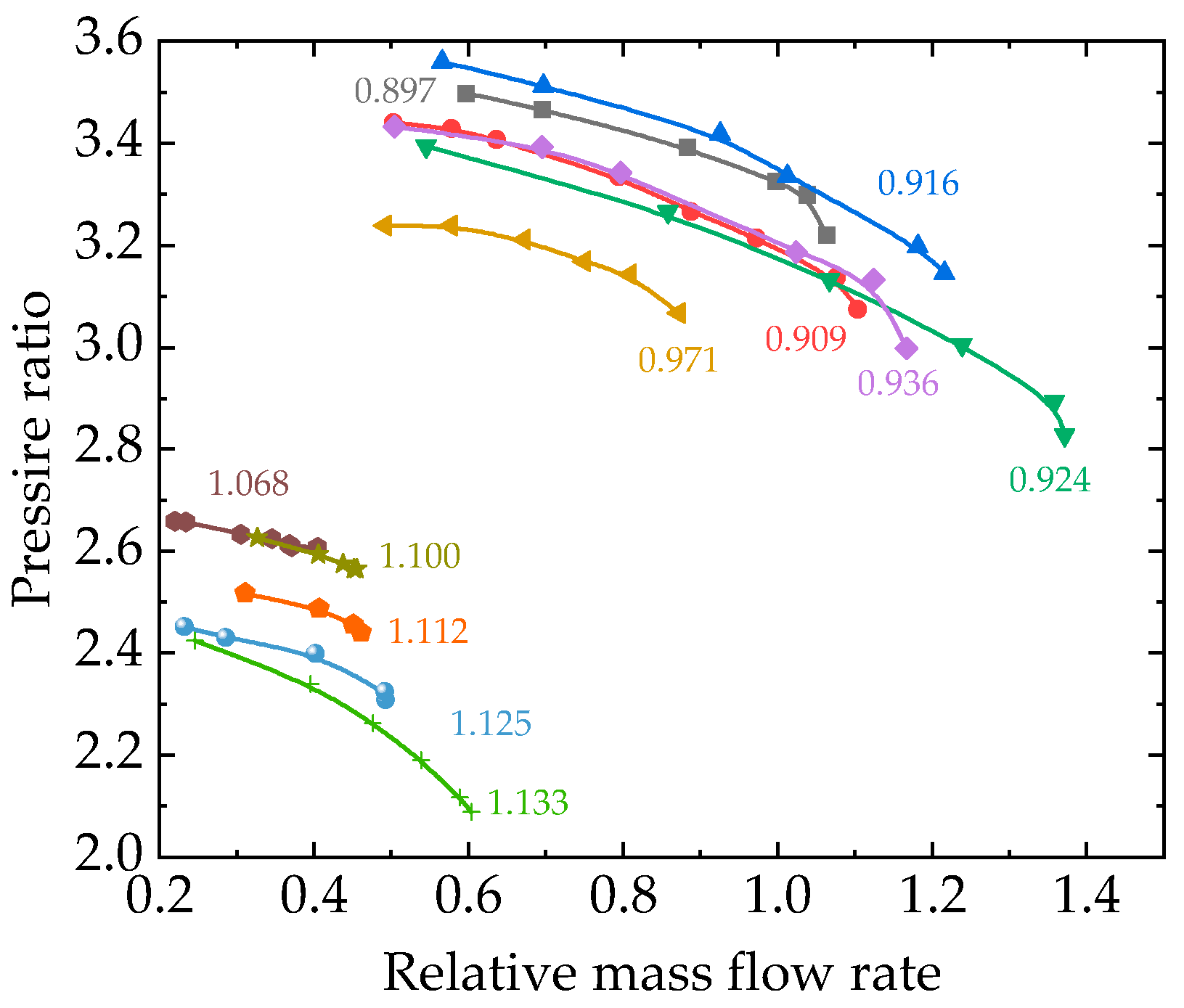
| Case | Pt (MPa) | Tt (K) | S (J/kg·K) | St/Sc |
|---|---|---|---|---|
| 1 | 8.50 | 305.15 | 1.286 | 0.897 |
| 2 | 8.50 | 306.15 | 1.304 | 0.909 |
| 3 | 8.00 | 305.15 | 1.313 | 0.916 |
| 4 | 8.50 | 307.15 | 1.325 | 0.924 |
| 5 | 8.00 | 306.15 | 1.324 | 0.936 |
| 6 | 8.00 | 307.15 | 1.392 | 0.971 |
| 7 | 7.50 | 305.15 | 1.531 | 1.068 |
| 8 | 7.55 | 305.15 | 1.577 | 1.100 |
| 9 | 7.50 | 306.15 | 1.594 | 1.112 |
| 10 | 7.50 | 307.15 | 1.613 | 1.125 |
| 11 | 7.55 | 307.15 | 1.625 | 1.133 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, W.; Tian, Y.; Jiang, P.; Wang, B.; Xu, X. Effects of Near-Critical Condensation and Cavitation on the Performance of S-CO2 Compressor. Energies 2024, 17, 854. https://doi.org/10.3390/en17040854
Xie W, Tian Y, Jiang P, Wang B, Xu X. Effects of Near-Critical Condensation and Cavitation on the Performance of S-CO2 Compressor. Energies. 2024; 17(4):854. https://doi.org/10.3390/en17040854
Chicago/Turabian StyleXie, Wenlin, Yong Tian, Peng Jiang, Bo Wang, and Xiang Xu. 2024. "Effects of Near-Critical Condensation and Cavitation on the Performance of S-CO2 Compressor" Energies 17, no. 4: 854. https://doi.org/10.3390/en17040854
APA StyleXie, W., Tian, Y., Jiang, P., Wang, B., & Xu, X. (2024). Effects of Near-Critical Condensation and Cavitation on the Performance of S-CO2 Compressor. Energies, 17(4), 854. https://doi.org/10.3390/en17040854





