Modeling and Control of a Modular Multilevel Converter Based on a Battery Energy Storage System with Soft Arm State-of-Charge Balancing Control
Abstract
1. Introduction
2. MMC-BESS Modeling and Analysis
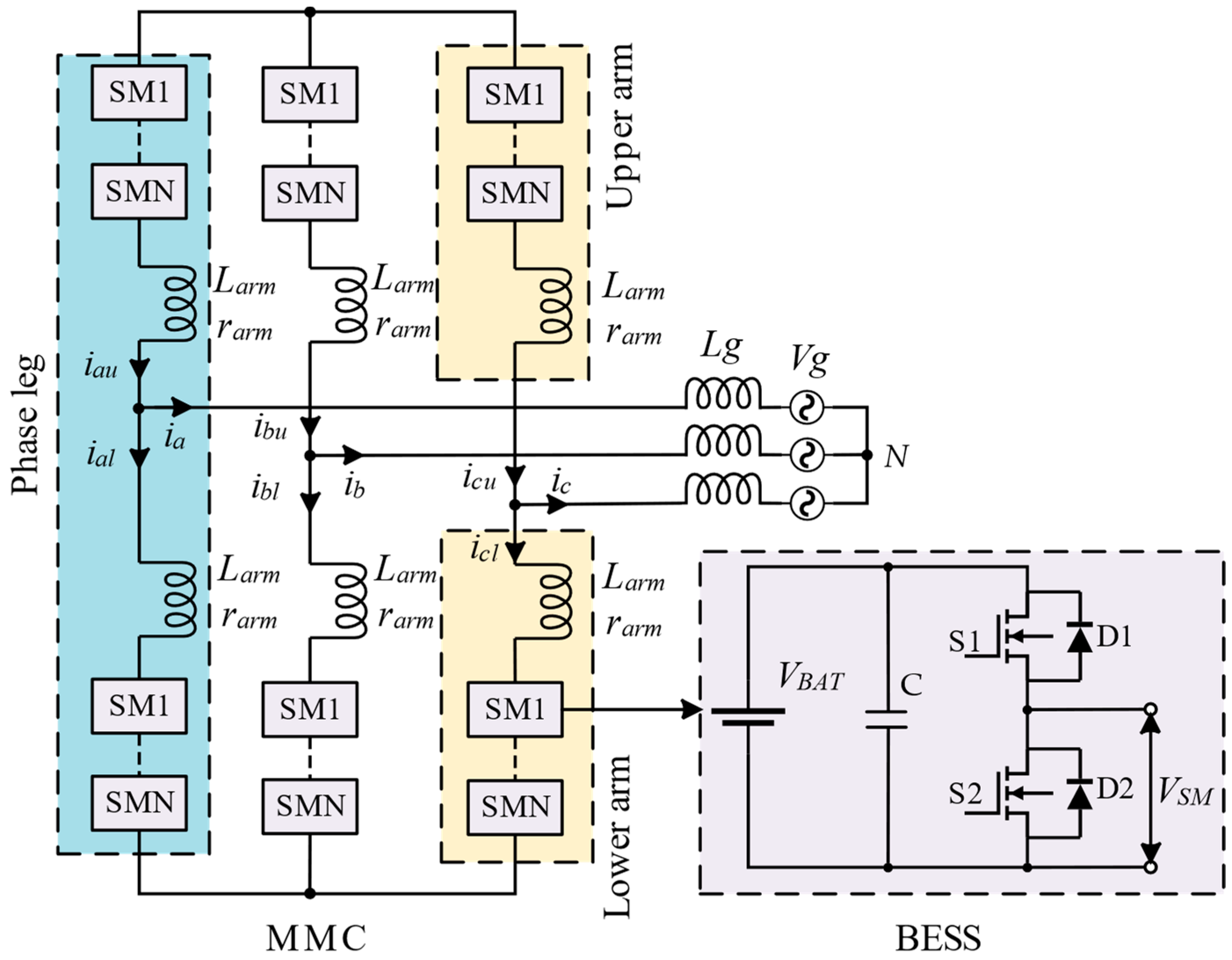
2.1. MMC-BESS Configuration
2.2. MMC-BESS Modeling and Analysis
2.2.1. MMC-BESS System Modeling
2.2.2. MMC-BESS SoC Balancing Analysis
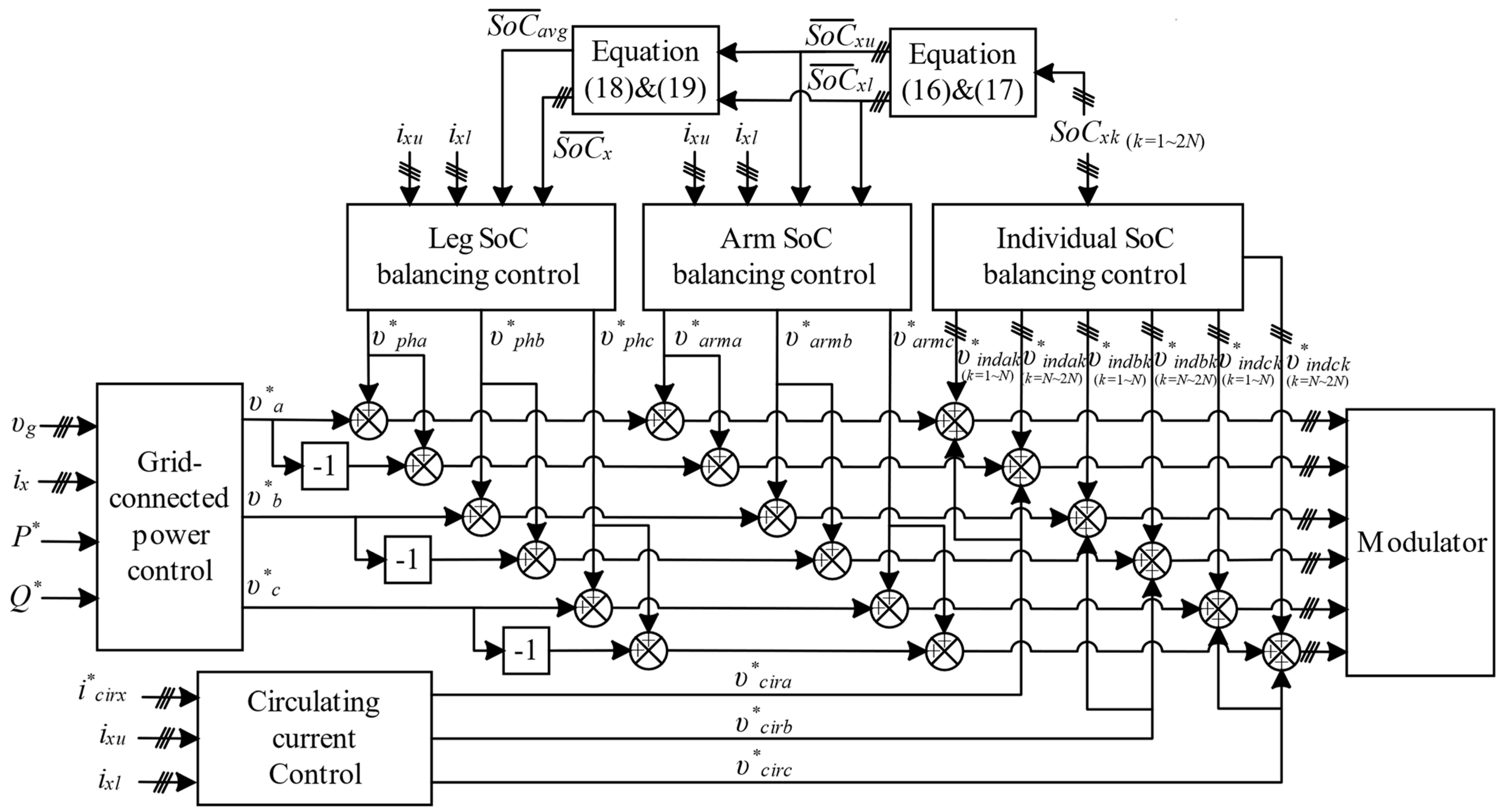
3. MMC-BESS Control Strategy
3.1. Grid-Connected Power Control
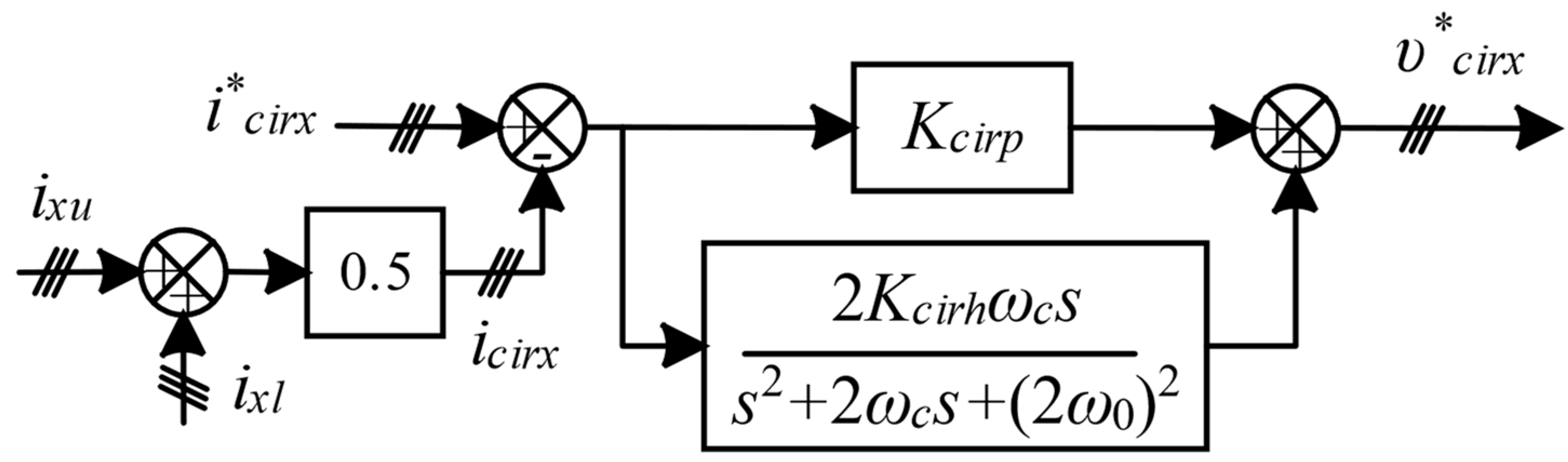
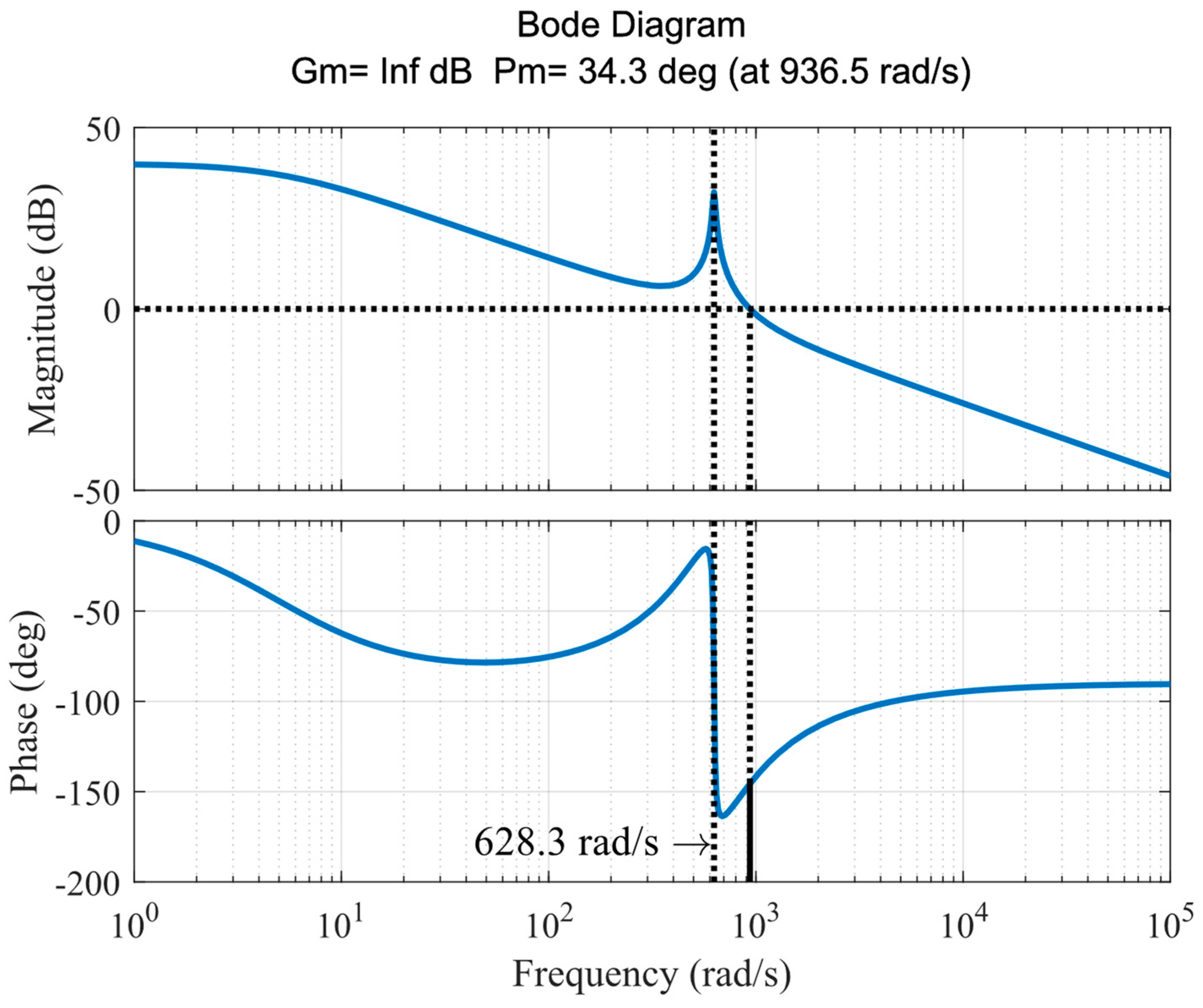
3.2. Circulating Current Control
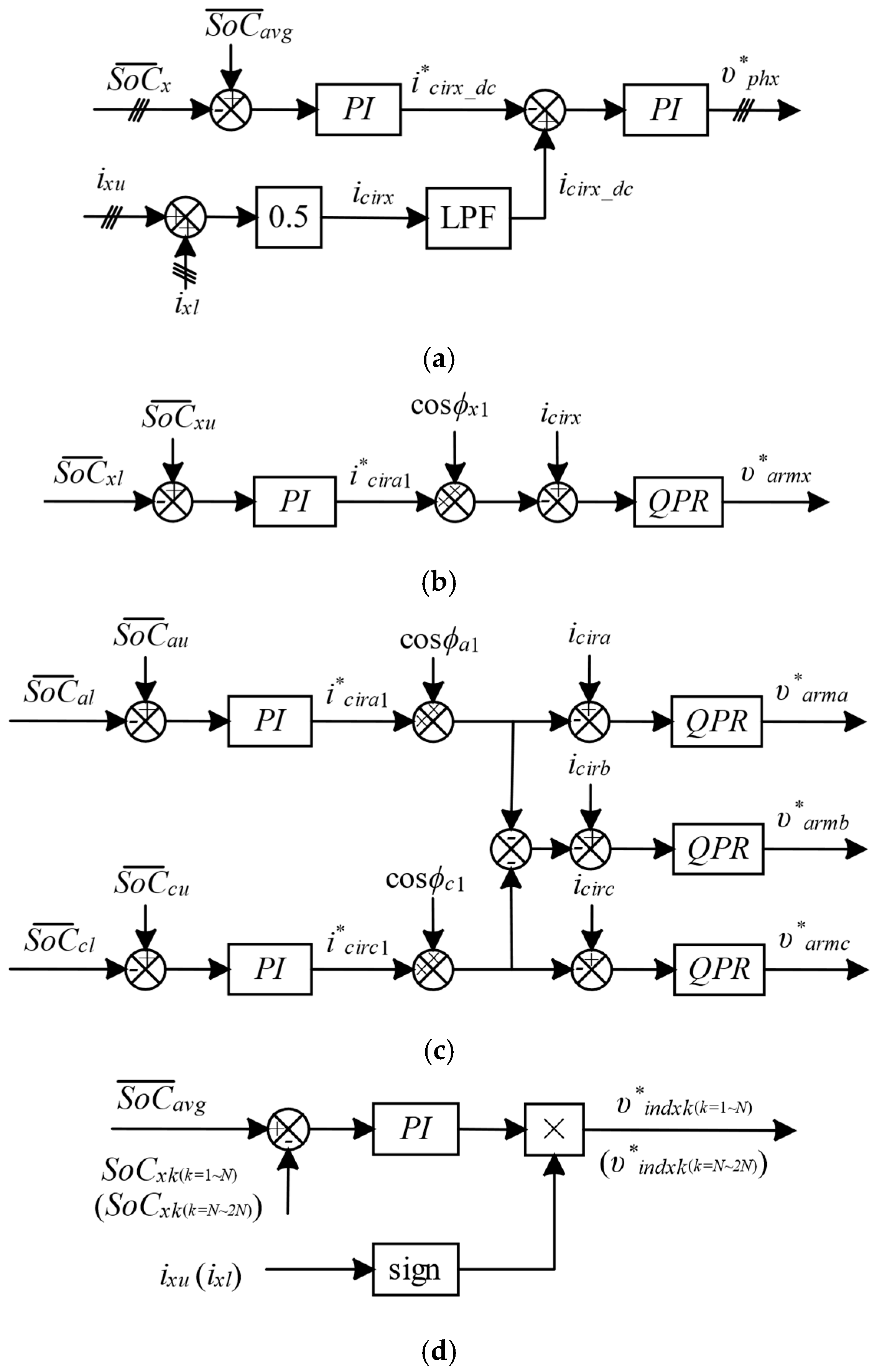
3.3. SoC Balancing Control
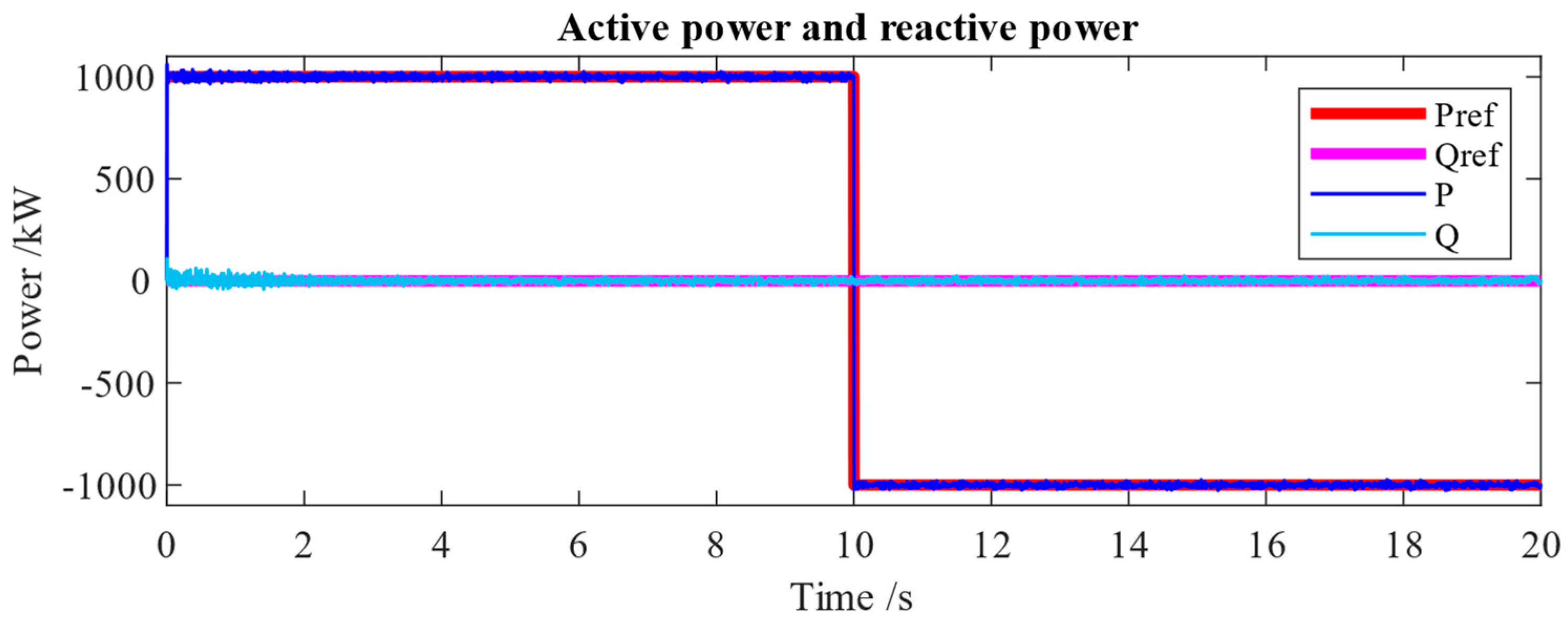
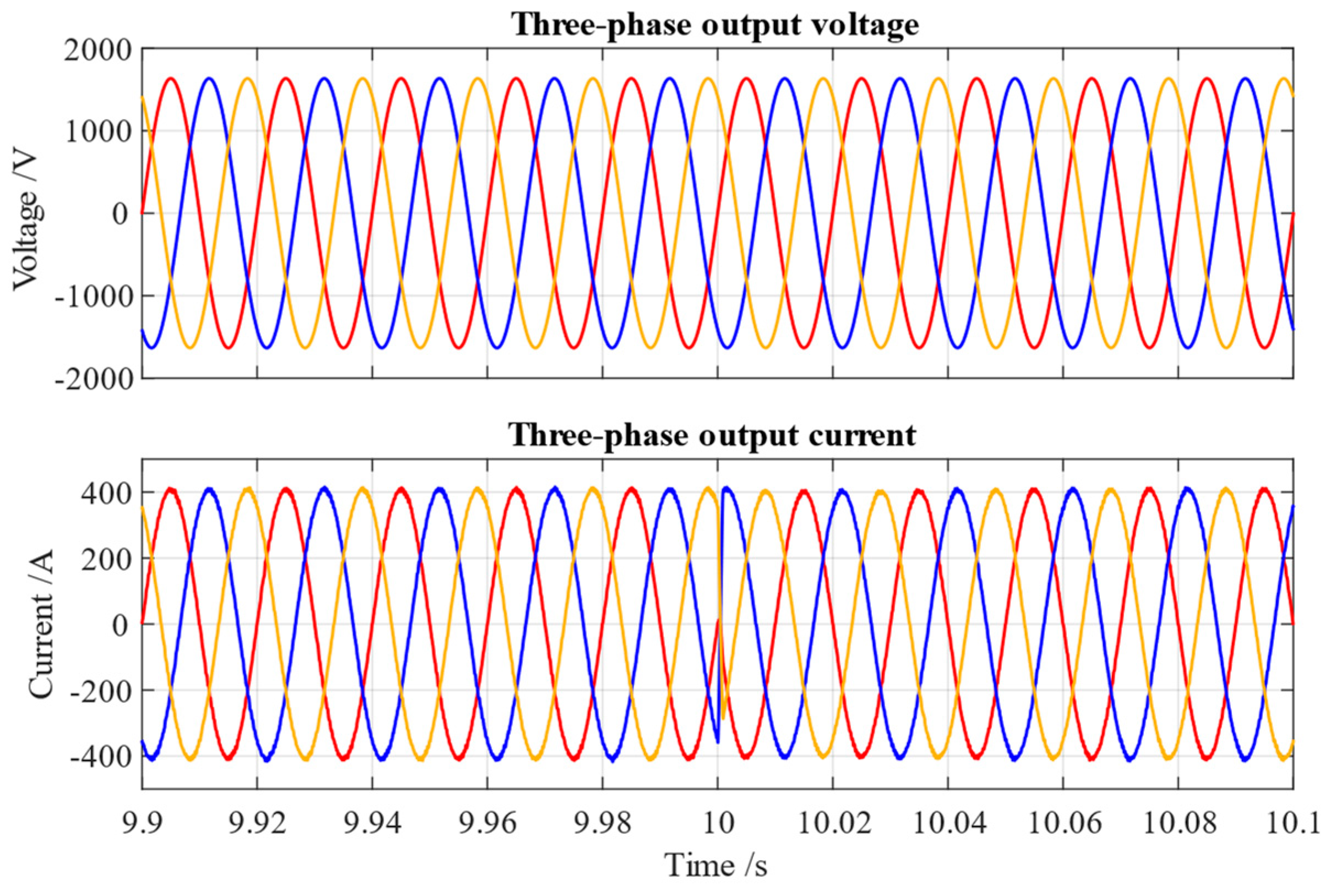
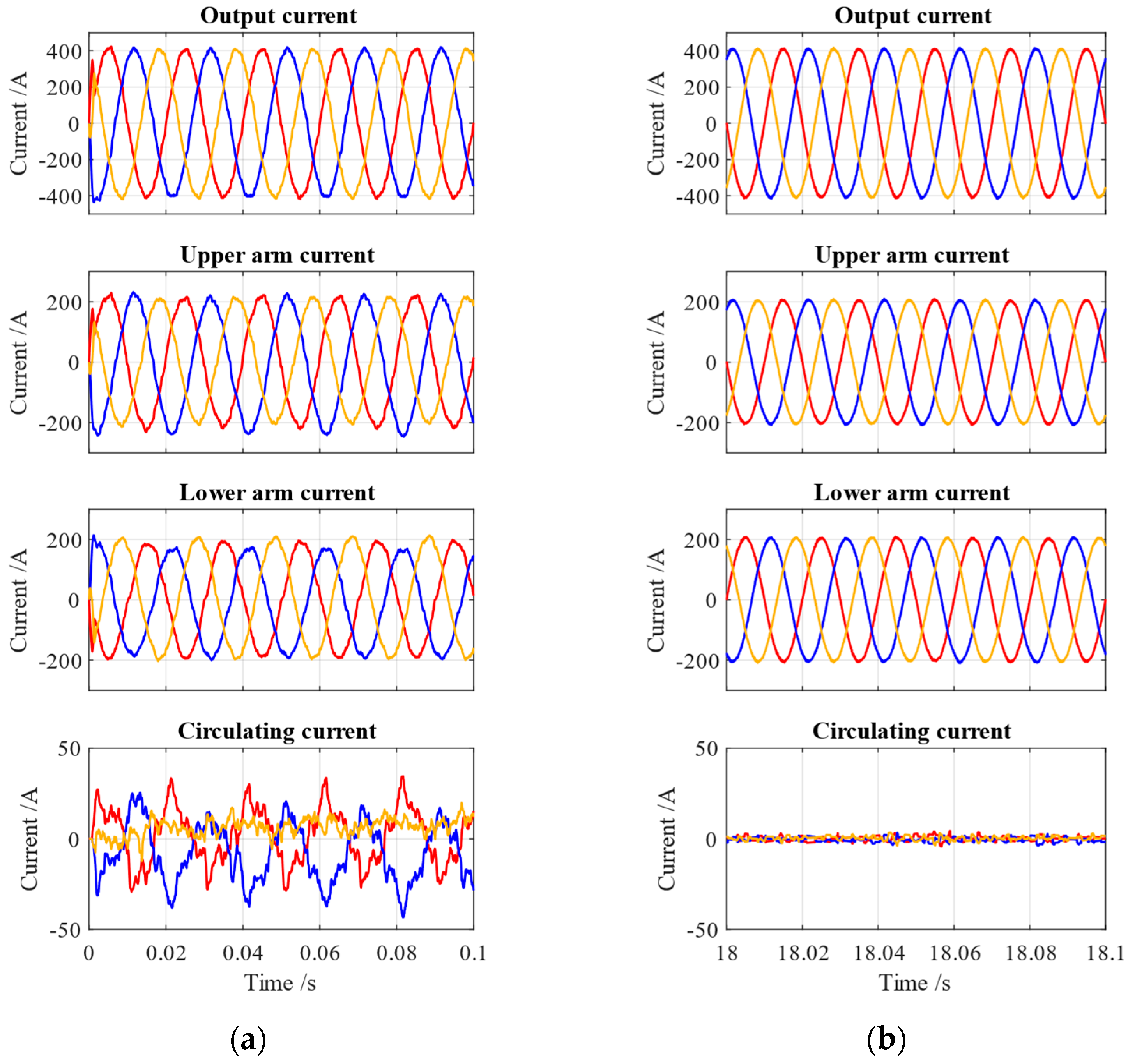
4. Results and Analysis
5. Conclusions
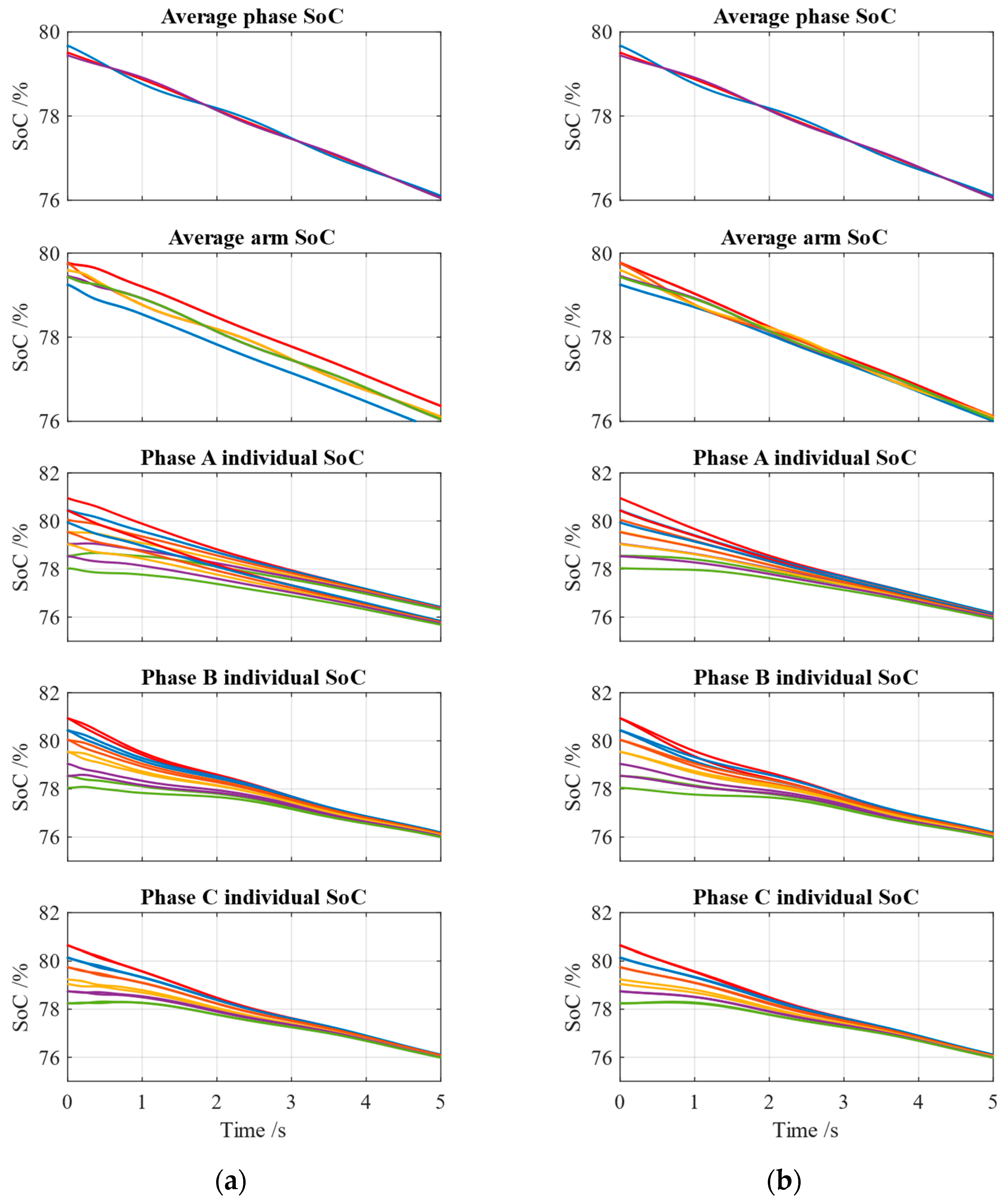
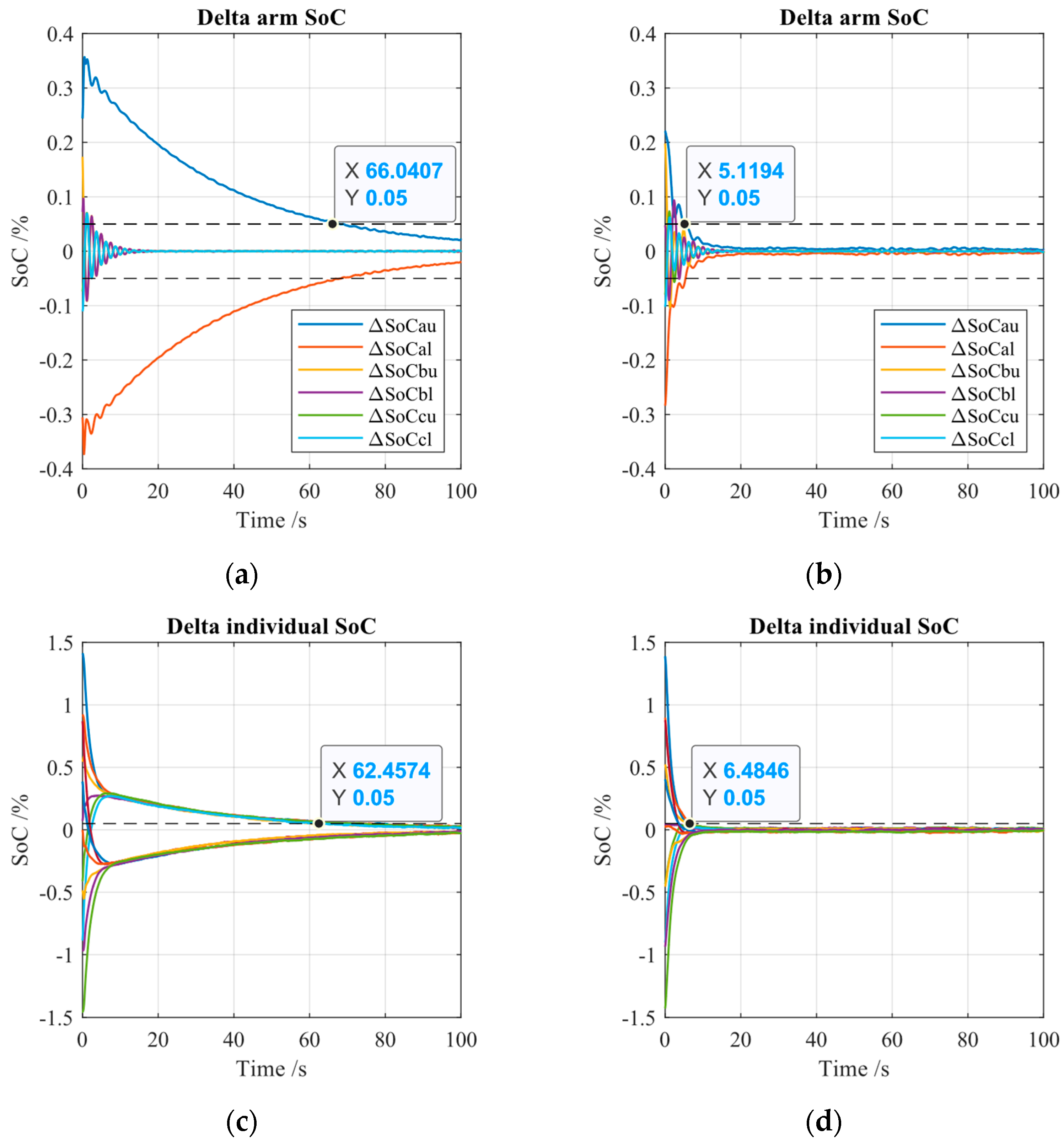
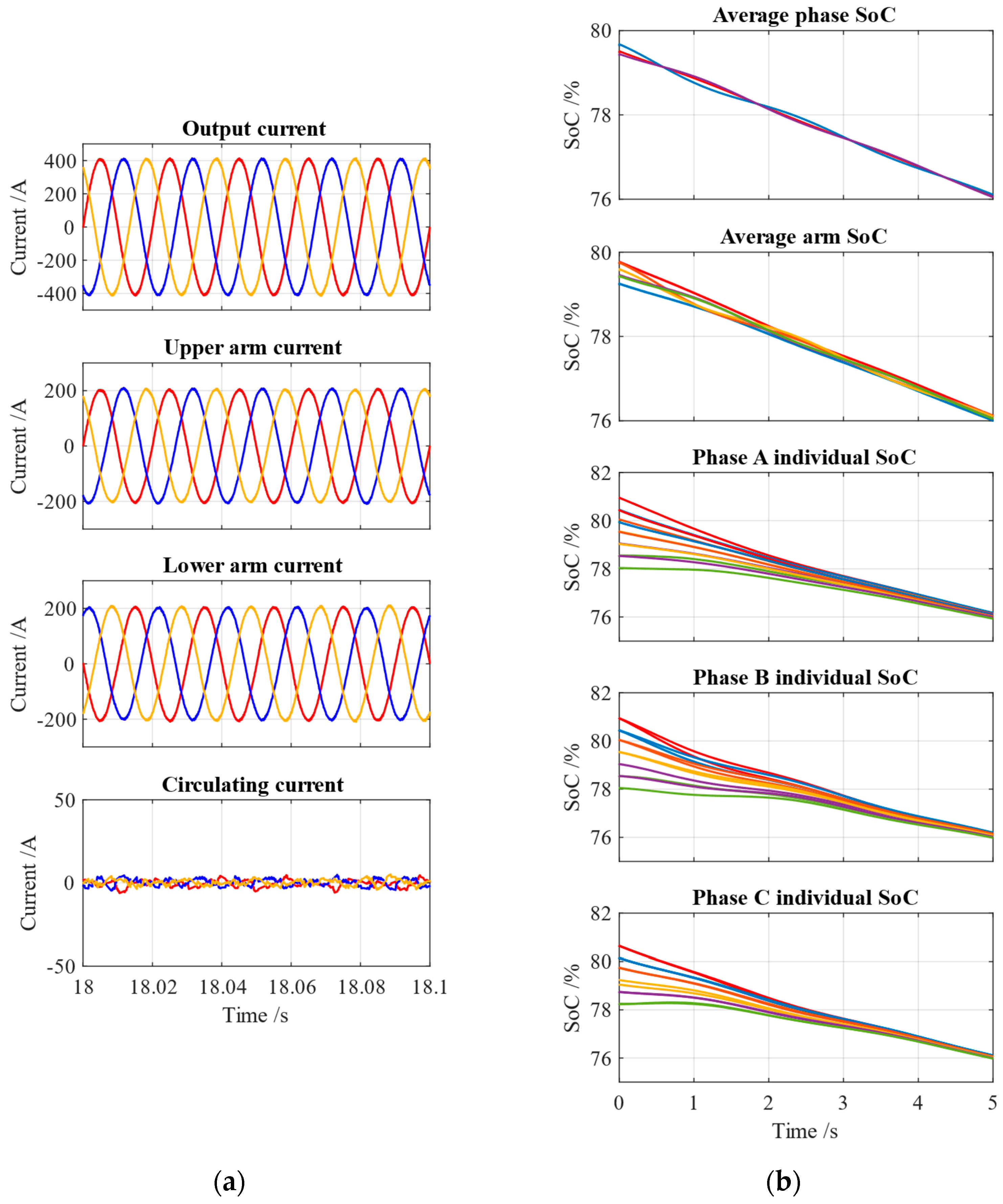
- Compared to HASBC, SASBC provides better performance in balancing the SoC between and within the arms. In this case, under the control of HASBC, the arm SoCs of the phase A upper arm and lower arm are not able to be equalized before 100 s, and it takes 66 s to reduce the delta SoC to 0.05% with reference to the average arm SoC. In contrast, all the arm SoCs in the three phases/six arms can be balanced fast, and it takes 5.1 s to fall into the 0.05% zone under the control of SASBC. Besides, individual SoCs of phase A under the control of HASBC are split into two groups and exhibit a protracted convergence exceeding 100 s toward zero, requiring 62.5 s to reach the 0.05% deviation range. However, they are forced to converge at zero rapidly and only take 6.5 s to reach the margin of 0.05% under the SASBC.
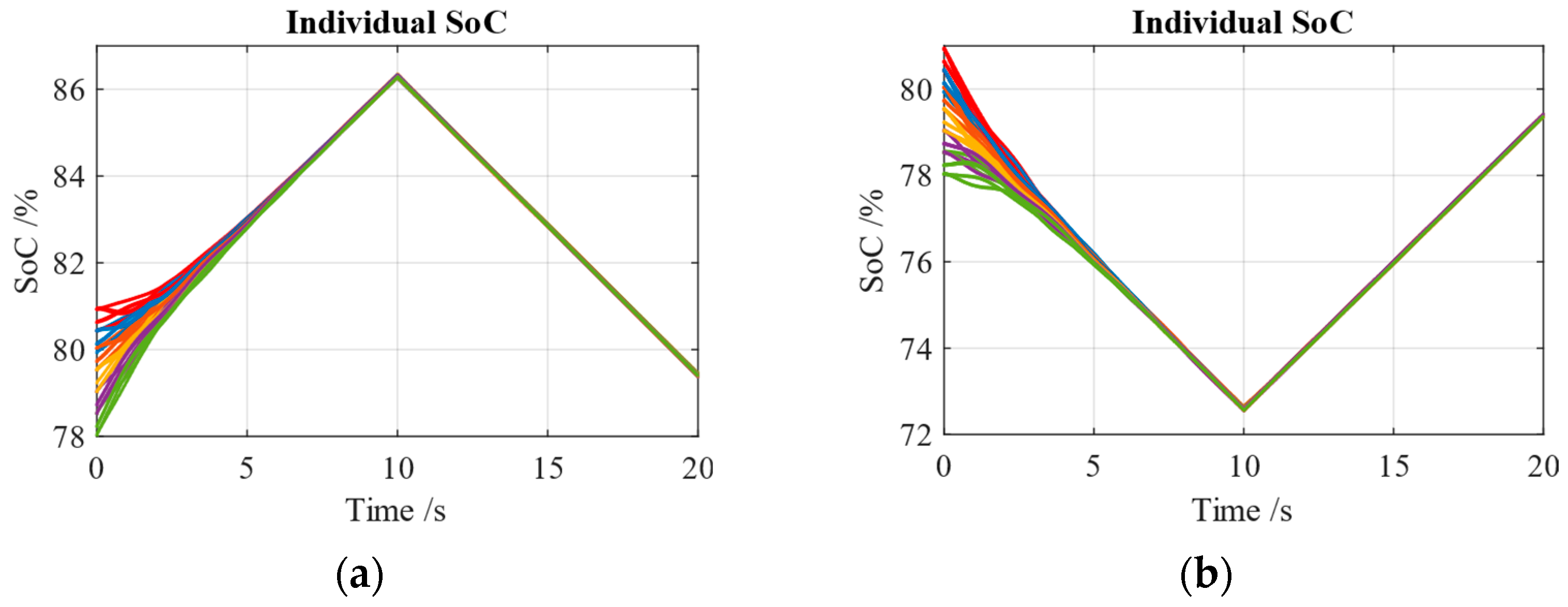
- The MMC-BESS power fluctuates between phases, arms, and individual submodules to balance the SoC of batteries, and after the accomplishment of the SoC equalization, the power is equally distributed and the circulating current is well eliminated.
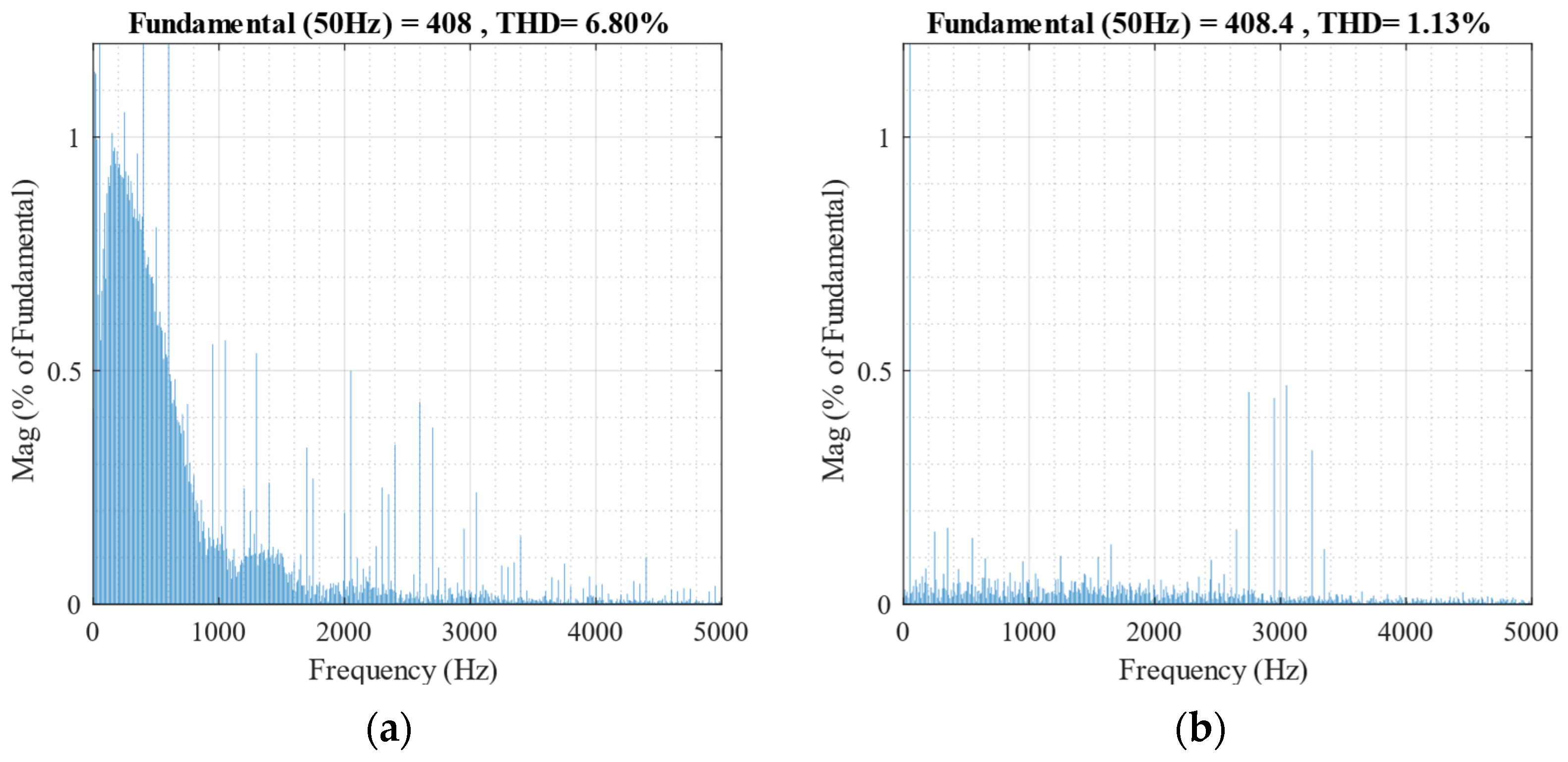
- The MMC-BESS can operate in both charging and discharging modes, and the THD of the output current is reduced from 6.80% to 1.13% after SoC balancing is achieved.
- The robustness test shows the control system’s effective performance in handling component variations.
Author Contributions
Funding

Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Derivation of Equations
Appendix A.1. Equation (30)
Appendix A.2. Equation (31)
References
- Market Strategy Board. Grid Integration of Large-Capacity Renewable Energy Sources and Use of Large-Capacity Electrical Energy Storage. Available online: https://webstore.ansi.org/Documents/Grid-integration-Large-Capacity-Renewable-Energy.pdf (accessed on 15 June 2023).
- Mahmoud, A.A.; Hafez, A.A.; Yousef, A.M. Modular Multilevel Converters for Renewable Energies Interfacing: Comparative Review. In Proceedings of the 2019 IEEE Conference on Power Electronics and Renewable Energy (CPERE), Aswan City, Egypt, 23–25 October 2019; pp. 397–406. [Google Scholar]
- Nami, A.; Zare, F. Multilevel Converters in Renewable Energy Systems. In Renew Energy; IntechOpen: London, UK, 2009. [Google Scholar] [CrossRef][Green Version]
- Soong, T.; Lehn, P.W. Evaluation of Emerging Modular Multilevel Converters for BESS Applications. IEEE Trans. Power Deliv. 2014, 29, 2086–2094. [Google Scholar] [CrossRef]
- Wang, Y.; Aksoz, A.; Geury, T.; El Baghdadi, M. Omar Hegazy Performance Enhancement of a Battery-Grid Connected SiC MMC for DC Microgrid Systems. In Proceedings of the 2021 Sixteenth International Conference on Ecological Vehicles and Renewable Energies (EVER), Monte-Carlo, Monaco, 5–7 May 2021; pp. 1–6. [Google Scholar]
- Lesnicar, A.; Marquardt, R. An Innovative Modular Multilevel Converter Topology Suitable for a Wide Power Range. In Proceedings of the 2003 IEEE Bologna Power Tech Conference Proceedings, Bologna, Italy, 23–26 June 2003; Volume 3, pp. 272–277. [Google Scholar]
- Wang, Y.; Aksoz, A.; Geury, T.; Ozturk, S.B.; Kivanc, O.C.; Hegazy, O. A Review of Modular Multilevel Converters for Stationary Applications. Appl. Sci. 2020, 10, 7719. [Google Scholar] [CrossRef]
- Ma, Z.; Jia, M.; Koltermann, L.; Blömeke, A.; De Doncker, R.W.; Li, W.; Sauer, D.U. Review on Grid-Tied Modular Battery Energy Storage Systems: Configuration Classifications, Control Advances, and Performance Evaluations. J. Energy Storage 2023, 74, 109272. [Google Scholar] [CrossRef]
- Shen, K.; Xiao, B.; Mei, J.; Tolbert, L.M.; Wang, J.; Cai, X.; Ji, Y. A Modulation Reconfiguration Based Fault-Tolerant Control Scheme for Modular Multilevel Converters. In Proceedings of the 2013 Twenty-Eighth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 17–21 March 2013; pp. 3251–3255. [Google Scholar]
- Zheng, Z.; Wang, K.; Xu, L.; Li, Y. A Hybrid Cascaded Multilevel Converter for Battery Energy Management Applied in Electric Vehicles. IEEE Trans. Power Electron. 2014, 29, 3537–3546. [Google Scholar] [CrossRef]
- Liang, G.; Tafti, H.D.; Farivar, G.G.; Pou, J.; Townsend, C.D.; Konstantinou, G.; Ceballos, S. Analytical Derivation of Intersubmodule Active Power Disparity Limits in Modular Multilevel Converter-Based Battery Energy Storage Systems. IEEE Trans. Power Electron. 2021, 36, 2864–2874. [Google Scholar] [CrossRef]
- Mashayekh, A.; Kersten, A.; Kuder, M.; Estaller, J.; Khorasani, M.; Buberger, J.; Eckerle, R.; Weyh, T. Proactive SoC Balancing Strategy for Battery Modular Multilevel Management (BM3) Converter Systems and Reconfigurable Batteries. In Proceedings of the 2021 23rd European Conference on Power Electronics and Applications, EPE 2021 ECCE Europe, Virtual, 6–10 September 2021. [Google Scholar]
- Omar, A.; Wood, A.; Laird, H.; Gaynor, P. Simplified SOC Balancing of an MMC with Embedded Storage in an EV System. In Proceedings of the TENCON 2021—2021 IEEE Region 10 Conference (TENCON), Auckland, New Zealand, 7–10 December 2021; Volume 2021, pp. 929–934. [Google Scholar]
- Mukherjee, N.; De, D. A New State-of-Charge Control Derivation Method for Hybrid Battery Type Integration. IEEE Trans. Energy Convers. 2017, 32, 866–875. [Google Scholar] [CrossRef]
- Maharjan, L.; Inoue, S.; Akagi, H.; Asakura, J. State-of-Charge (SOC)-Balancing Control of a Battery Energy Storage System Based on a Cascade PWM Converter. IEEE Trans. Power Electron. 2009, 24, 1628–1636. [Google Scholar] [CrossRef]
- Lachichi, A. Modular Multilevel Converters with Integrated Batteries Energy Storage. In Proceedings of the 2014 International Conference on Renewable Energy Research and Application (ICRERA), Milwaukee, WI, USA, 19–22 October 2014; pp. 828–832. [Google Scholar]
- Hatano, N.; Ise, T. Control Scheme of Cascaded H-Bridge STATCOM Using Zero-Sequence Voltage and Negative-Sequence Current. IEEE Trans. Power Deliv. 2010, 25, 543–550. [Google Scholar] [CrossRef]
- Li, N.; Gao, F.; Shang, X.; Jia, H.; Li, S.; Guo, B.; Hou, K.; Zhao, X. Operation Principles of Modular Multilevel Conversion System for Electric Vehicles. In Proceedings of the 2020 5th Asia Conference on Power and Electrical Engineering, ACPEE 2020, Chengdu, China, 4–7 June 2020; pp. 1199–1203. [Google Scholar] [CrossRef]
- Quraan, M.; Yeo, T.; Tricoli, P. Design and Control of Modular Multilevel Converters for Battery Electric Vehicles. IEEE Trans. Power Electron. 2016, 31, 507–517. [Google Scholar] [CrossRef]
- D’Arco, S.; Quraan, M.; Tricoli, P.; Piegari, L. Low Frequency Operation of Modular Multilevel Converters with Embedded Battery Cells for Traction Drives. In Proceedings of the 2016 International Symposium on Power Electronics, Electrical Drives, Automation and Motion (SPEEDAM), Capri, Italy, 22–24 June 2016; pp. 1375–1382. [Google Scholar]
- Cai, W.; Hu, P.; Jiang, D.; Liang, Y. An MMC Based Hybrid Energy Storage System: Concept, Topology, and Control. In Proceedings of the 2020 IEEE Student Conference on Electric Machines and Systems, SCEMS 2020, Jinan, China, 4–6 December 2020; pp. 680–685. [Google Scholar]
- Luo, H.; Xu, C.; Dai, K.; Cheng, C.; Huang, Y.; Pan, F. Balance Control of SOC for MMC-BESS With Power Fluctuation Suppression, PCC Voltage Regulation, and Harmonic Mitigation in Grid-Connected Wind Farm. IEEE Access 2022, 10, 117732–117744. [Google Scholar] [CrossRef]
- Li, N.; Gao, F. Linearized Operation of MMC Battery Energy Storage System. In Proceedings of the 2016 IEEE 2nd Annual Southern Power Electronics Conference (SPEC), Auckland, New Zealand, 5–8 December 2016; pp. 1–5. [Google Scholar]
- Vasiladiotis, M.; Rufer, A. Analysis and Control of Modular Multilevel Converters with Integrated Battery Energy Storage. IEEE Trans. Power Electron. 2015, 30, 163–175. [Google Scholar] [CrossRef]
- Chen, Q.; Li, R.; Cai, X. Analysis and Fault Control of Hybrid Modular Multilevel Converter with Integrated Battery Energy Storage System. IEEE J. Emerg. Sel. Top. Power Electron. 2017, 5, 64–78. [Google Scholar] [CrossRef]
- Liu, C.; Cai, X.; Chen, Q. Self-Adaptation Control of Second-Life Battery Energy Storage System Based on Cascaded H-Bridge Converter. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 1428–1441. [Google Scholar] [CrossRef]
- Ma, Y.; Lin, H.; Wang, Z.; Ze, Z. Modified State-of-Charge Balancing Control of Modular Multilevel Converter with Integrated Battery Energy Storage System. Energies 2018, 12, 96. [Google Scholar] [CrossRef]
- Ma, Y.; Lin, H.; Wang, Z.; Wang, T. Capacitor Voltage Balancing Control of Modular Multilevel Converters with Energy Storage System by Using Carrier Phase-Shifted Modulation. In Proceedings of the 2017 IEEE Applied Power Electronics Conference and Exposition (APEC), Tampa, FL, USA, 26–30 March 2017; pp. 1821–1828. [Google Scholar]
- Wang, Y.; Chakraborty, S.; Tran, D.D.; Geury, T.; Hegazy, O. Design and Optimization of the Arm Inductor for Modular Multilevel Converter. In Proceedings of the 2022 International Symposium on Power Electronics, Electrical Drives, Automation and Motion, SPEEDAM 2022, Sorrento, Italy, 22–24 June 2022; pp. 354–359. [Google Scholar]
- An, Q.; Zhang, J.; An, Q.; Shamekov, A. Quasi-Proportional-Resonant Controller Based Adaptive Position Observer for Sensorless Control of PMSM Drives Under Low Carrier Ratio. IEEE Trans. Ind. Electron. 2020, 67, 2564–2573. [Google Scholar] [CrossRef]
- Qiu, J.; Hang, L.; Liu, D.; Geng, S.; Ma, X.; Li, Z. Novel Method for Circulating Current Suppression in MMCs Based on Multiple Quasi-PR Controller. In Proceedings of the 2016 IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 20–24 March 2016; Volume 18, pp. 3560–3565. [Google Scholar]
- She, X.; Huang, A.; Ni, X.; Burgos, R. AC Circulating Currents Suppression in Modular Multilevel Converter. In Proceedings of the IECON Proceedings (Industrial Electronics Conference), Montreal, QC, Canada, 25–28 October 2012; pp. 191–196. [Google Scholar] [CrossRef]
- Ye, T.; Dai, N.; Lam, C.-S.; Wong, M.-C.; Guerrero, J.M. Analysis, Design, and Implementation of a Quasi-Proportional-Resonant Controller for a Multifunctional Capacitive-Coupling Grid-Connected Inverter. IEEE Trans. Ind. Appl. 2016, 52, 4269–4280. [Google Scholar] [CrossRef]
- Parikh, H.R.; Martin-loeches, R.S. Control of Modular Multilevel Converter(MMC) Based HVDC Application under Unbalanced Conditions. Master’s Thesis, Aalborg University, Aalborg, Denmark, 2016. Available online: https://projekter.aau.dk/projekter/files/239473549/PED4_940.pdf (accessed on 25 September 2021).
- Holmes, D.G.; Lipo, T.A.; McGrath, B.P.; Kong, W.Y. Optimized Design of Stationary Frame Three Phase AC Current Regulators. IEEE Trans. Power Electron. 2009, 24, 2417–2426. [Google Scholar] [CrossRef]
- Kolb, J.; Kammerer, F.; Braun, M. Dimensioning and Design of a Modular Multilevel Converter for Drive Applications. In Proceedings of the 15th International Power Electronics and Motion Control Conference and Exposition, EPE-PEMC 2012 ECCE Europe, Novi Sad, Serbia, 4–6 September 2012. [Google Scholar]



| Parameters | Values |
|---|---|
| No. of submodules per arm | 6 |
| Nominal voltage of battery banks | 1000 V |
| RMS grid phase-to-phase voltage | 2000 V |
| Grid frequency | 50 Hz |
| Carrier frequency | 1 kHz |
| Nominal power level | ±1 MW |
| Submodule capacitance | |
| Arm inductance | 10 mH |
| SoCs | Hard Arm SoC Balancing Control | Soft Arm SoC Balancing Control |
|---|---|---|
| Arm SoC balancing | Split s (66 s ()) | Balanced (5.1 s ()) |
| Phase A individual SoC | Split s () | Balanced () |
| Phase B individual SoC | Balanced | Balanced |
| Phase C individual SoC | Balanced | Balanced |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Chakraborty, S.; Geury, T.; Hegazy, O. Modeling and Control of a Modular Multilevel Converter Based on a Battery Energy Storage System with Soft Arm State-of-Charge Balancing Control. Energies 2024, 17, 740. https://doi.org/10.3390/en17030740
Wang Y, Chakraborty S, Geury T, Hegazy O. Modeling and Control of a Modular Multilevel Converter Based on a Battery Energy Storage System with Soft Arm State-of-Charge Balancing Control. Energies. 2024; 17(3):740. https://doi.org/10.3390/en17030740
Chicago/Turabian StyleWang, Yang, Sajib Chakraborty, Thomas Geury, and Omar Hegazy. 2024. "Modeling and Control of a Modular Multilevel Converter Based on a Battery Energy Storage System with Soft Arm State-of-Charge Balancing Control" Energies 17, no. 3: 740. https://doi.org/10.3390/en17030740
APA StyleWang, Y., Chakraborty, S., Geury, T., & Hegazy, O. (2024). Modeling and Control of a Modular Multilevel Converter Based on a Battery Energy Storage System with Soft Arm State-of-Charge Balancing Control. Energies, 17(3), 740. https://doi.org/10.3390/en17030740










