A Comprehensive Review of Compensation Control Techniques Suitable for Cascaded H-Bridge Multilevel Inverter Operation with Unequal DC Sources or Faulty Cells
Abstract
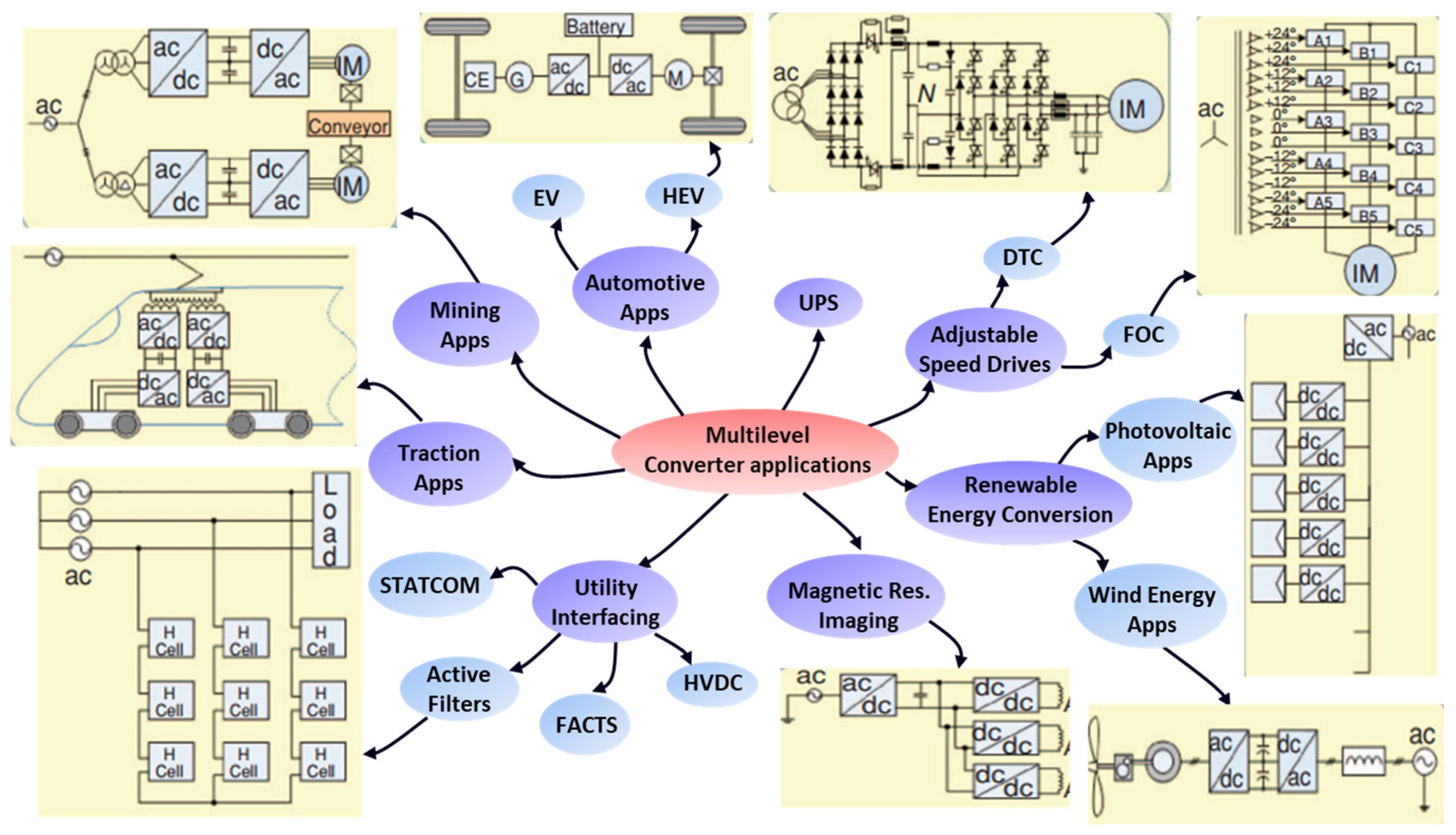
1. Introduction
1.1. Background and Context
- (1)
- (2)
- (3)
- (4)
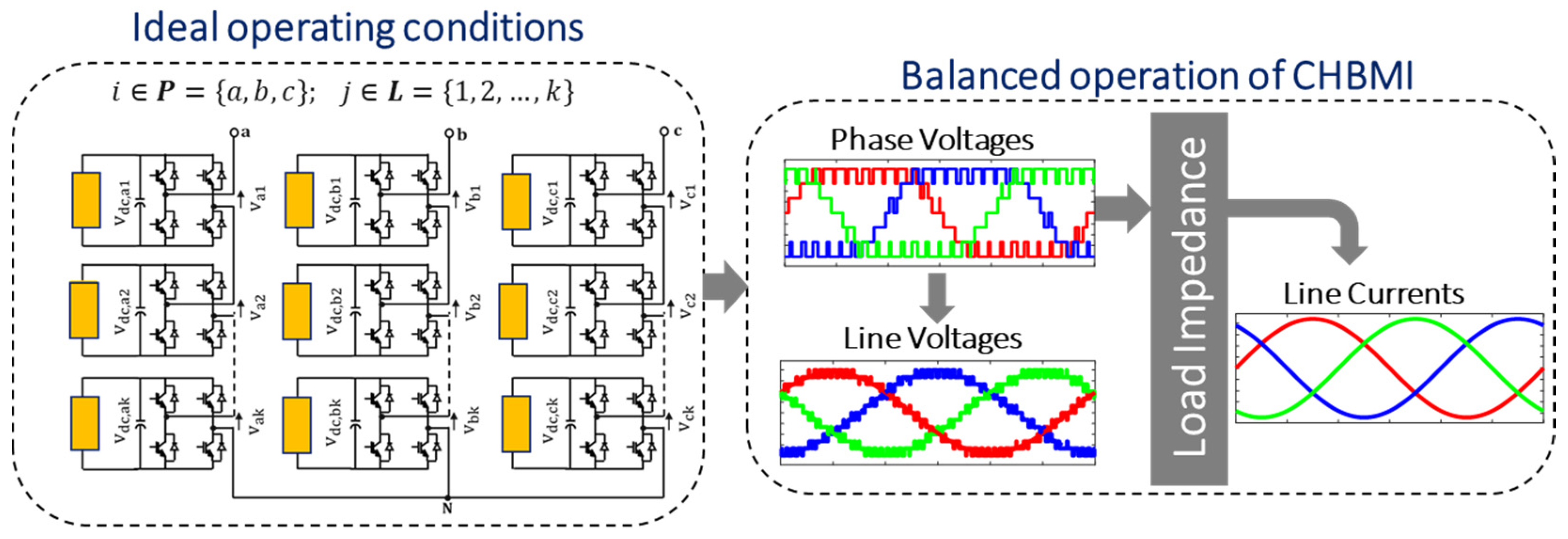
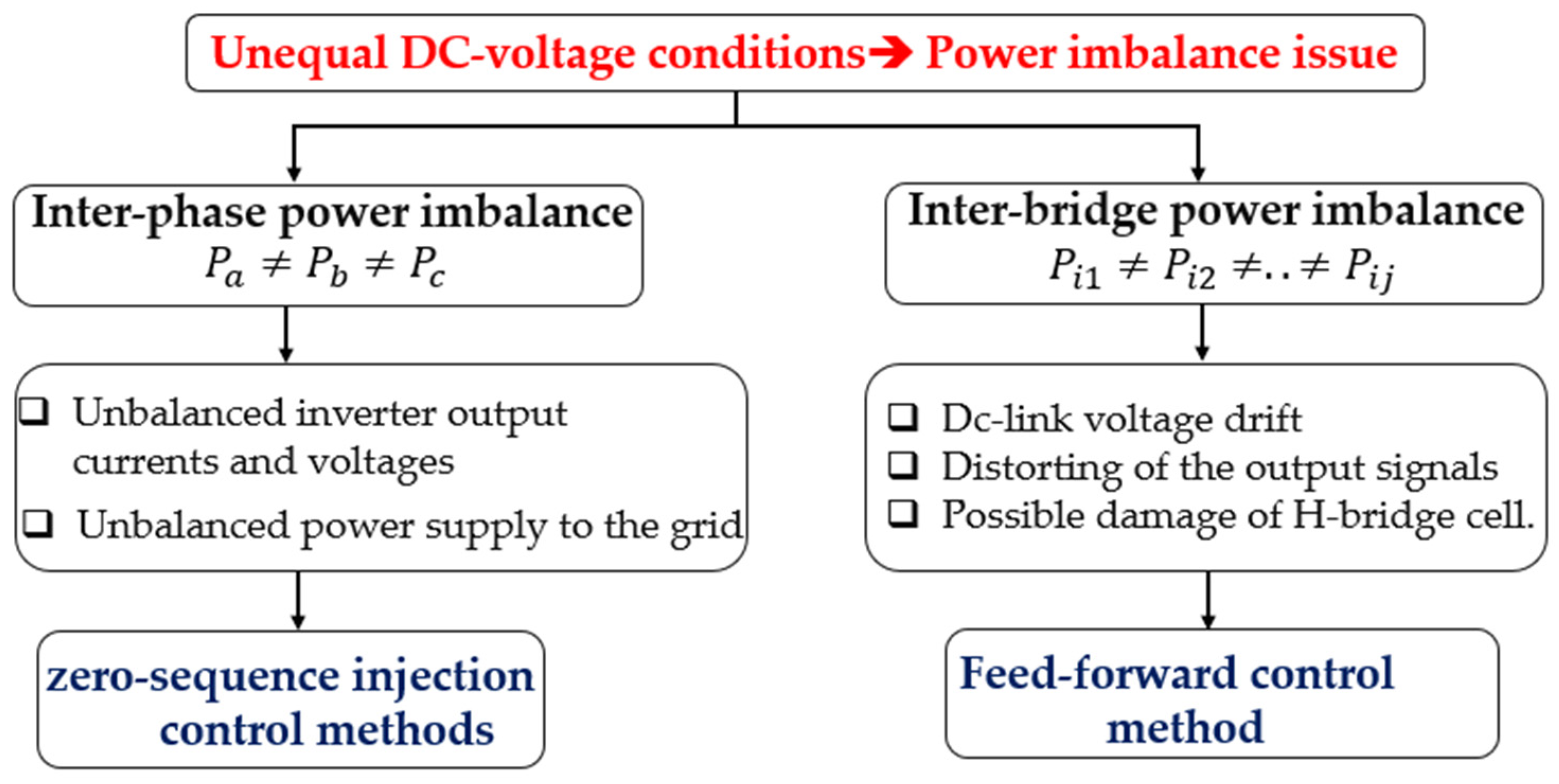
1.2. Major Operational Issue in CHBMI
1.3. Main Contribution
2. Review of Existing Control Methods for CHBMIs with Unequal DC Sources or Failed Cells
2.1. Existing Control Methods for CHBMIs with Unequal DC Voltage Sources
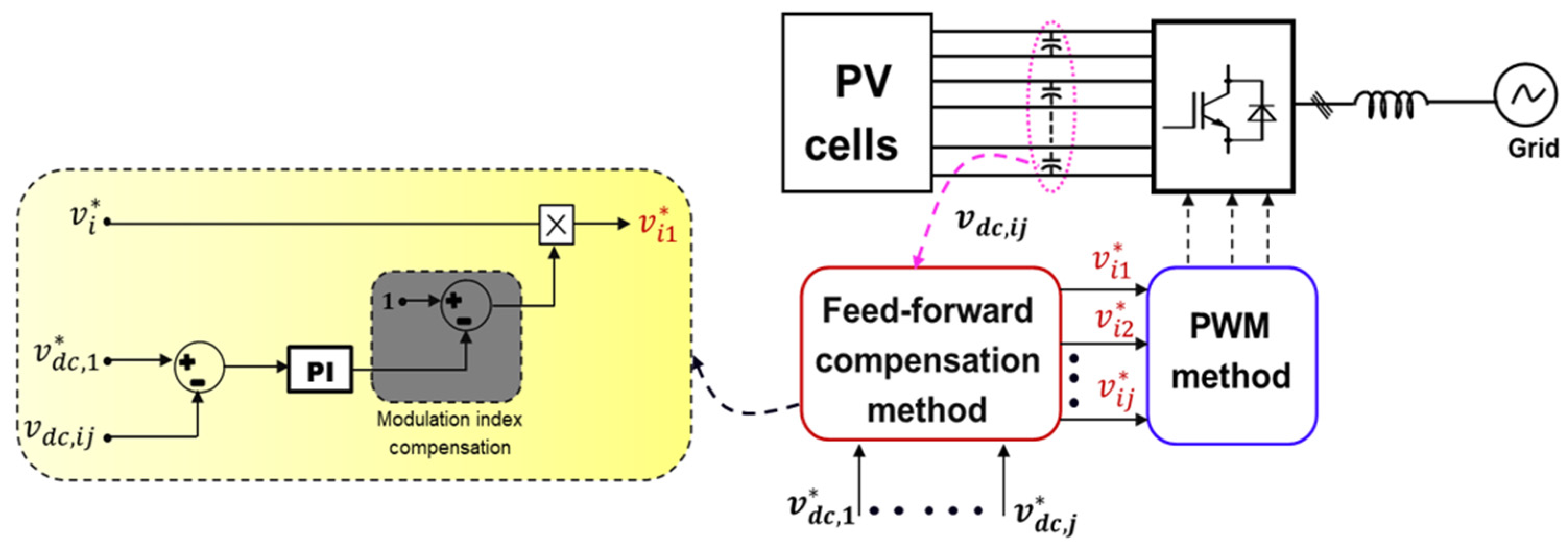
2.1.1. Feedforward Compensation Control Method
- (a)
- Operating principle
- (b)
- Advantages and disadvantages
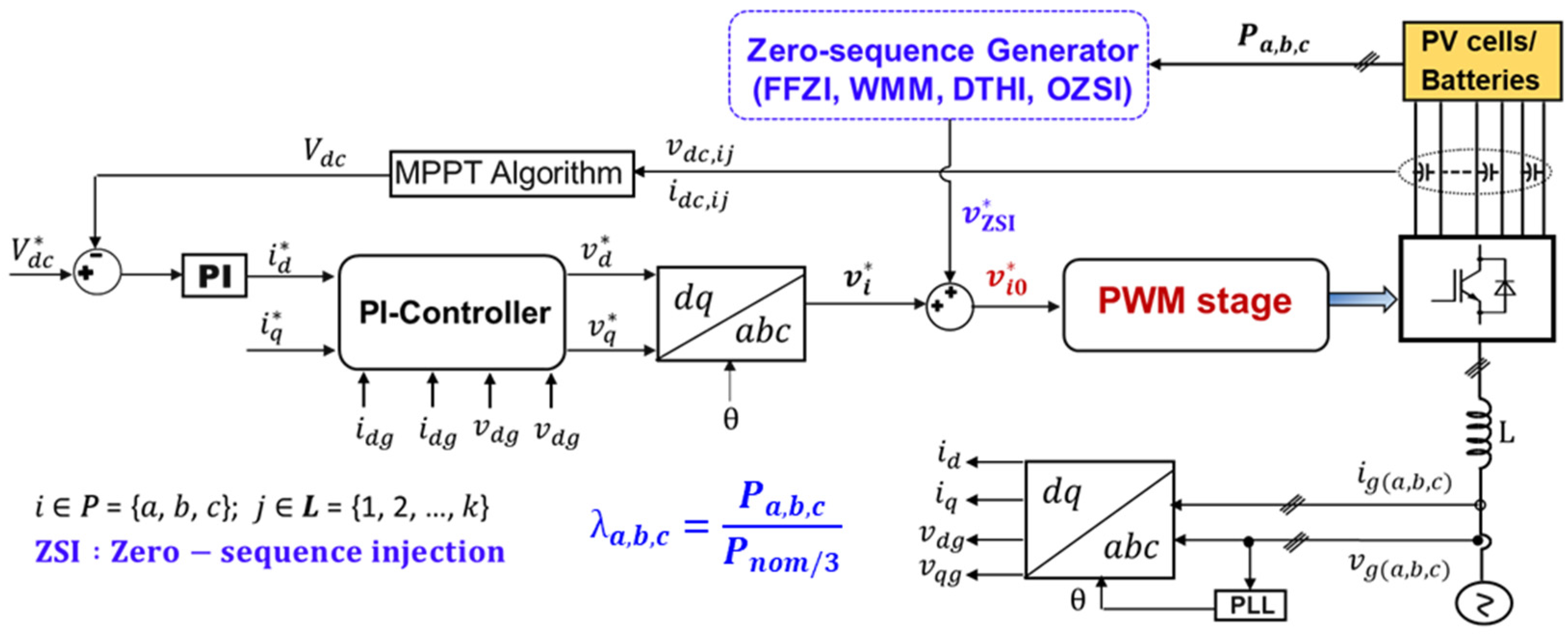
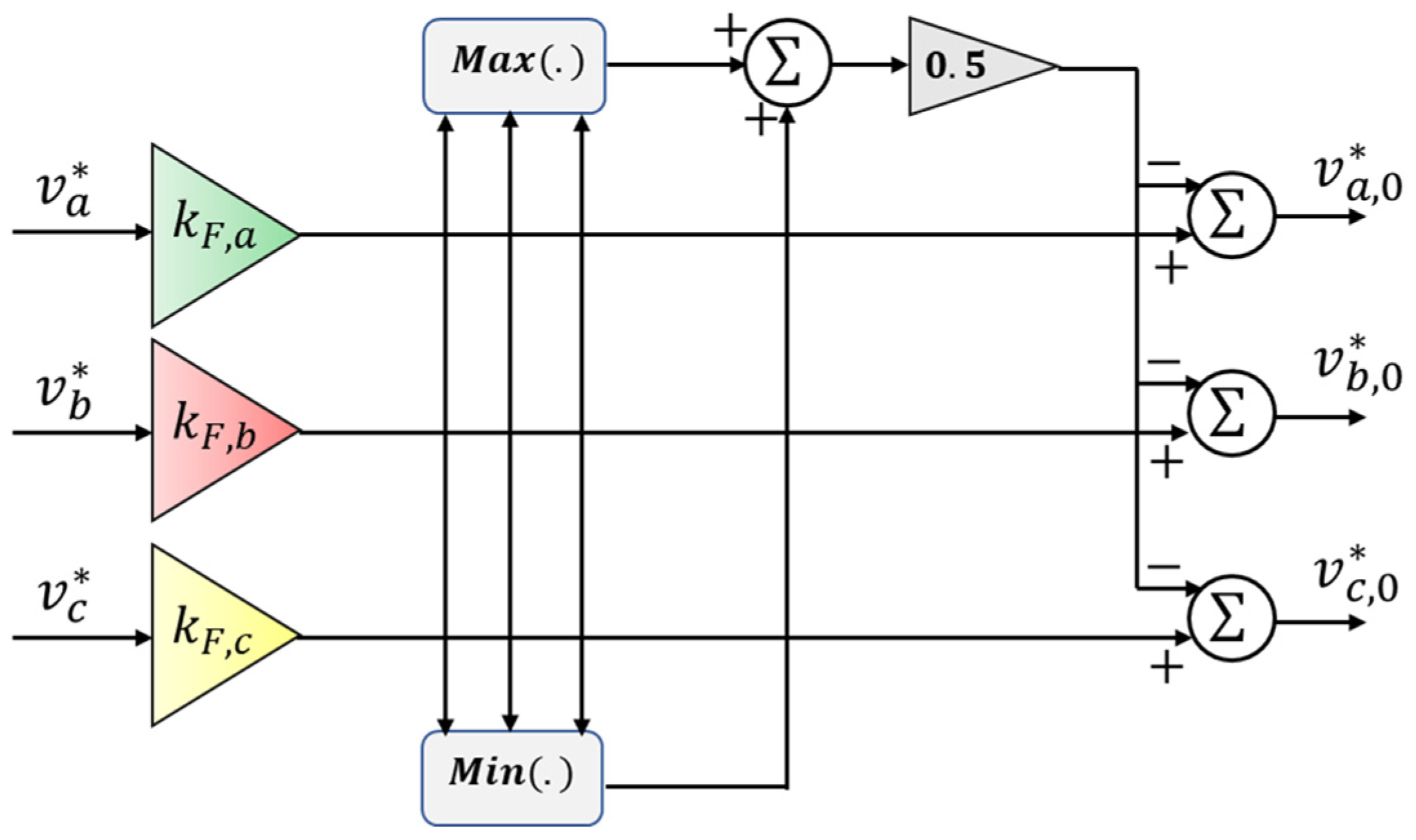
2.1.2. Zero-Sequence Injection Control Method
- (a)
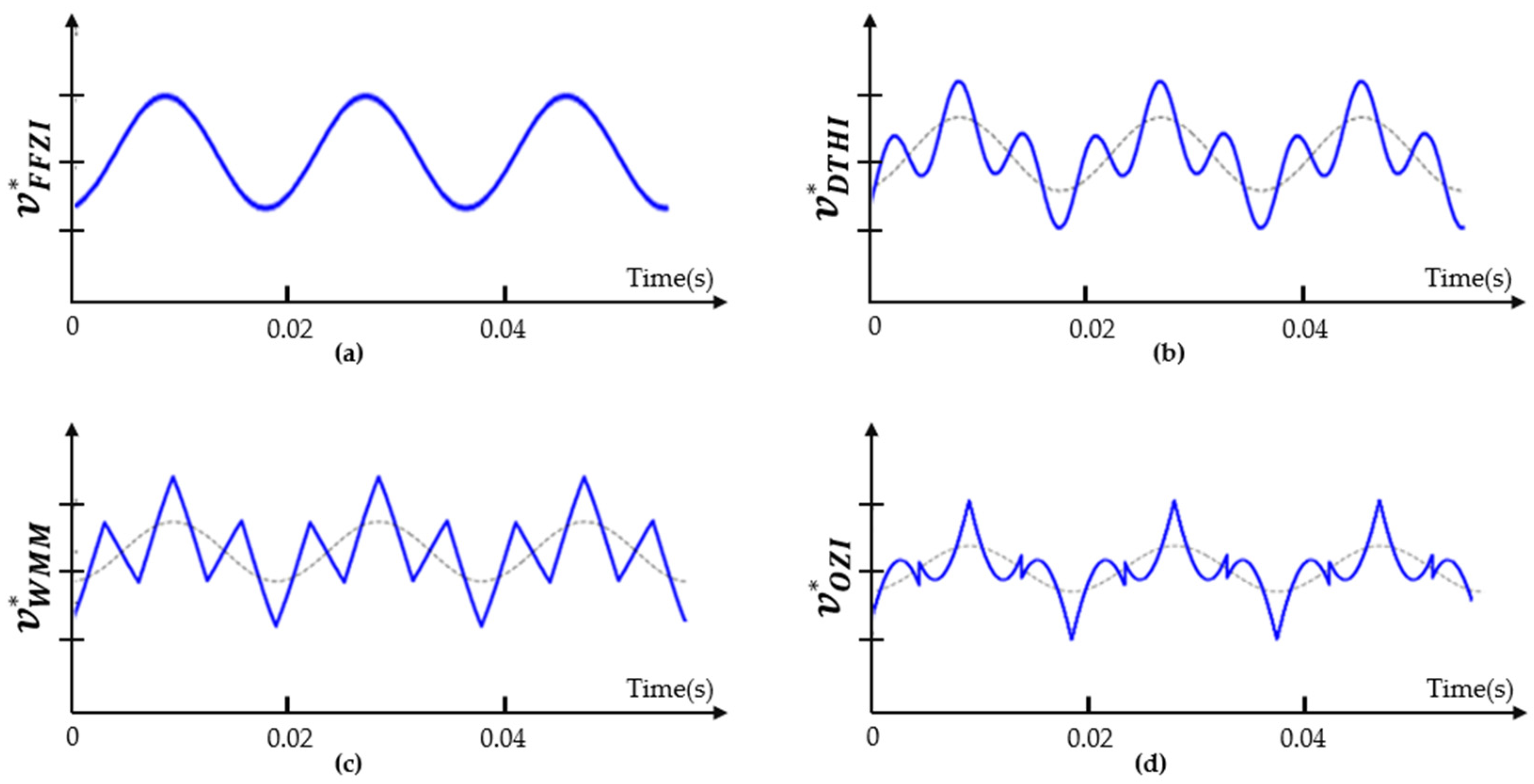
- Operating principle and implementation scheme
- A fundamental frequency zero-sequence injection (FFZSI) method;
- A weighted min–max (WMM) method zero-sequence injection method;
- Double third harmonic (DTHI) injection method;
- Optimal zero-sequence injection (OZSI) method.
- (b)
- Advantages and disadvantages
2.1.3. State-of-the-Art Other Compensation Control Approaches for Unbalanced CHBMI Operation with Unequal DC Voltage Sources
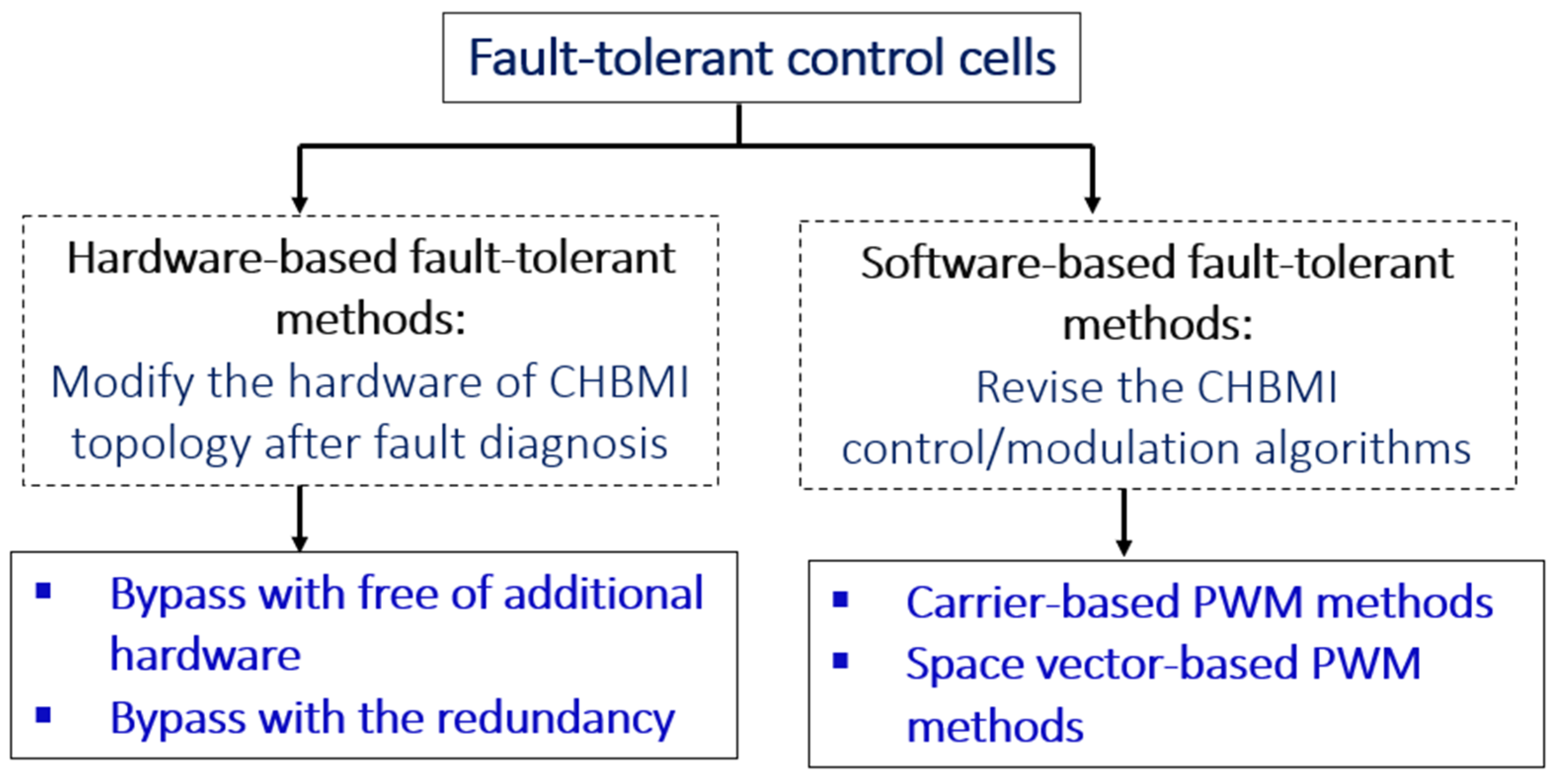
2.2. Existing Control Methods for CHBMI Operation with Failed Cells
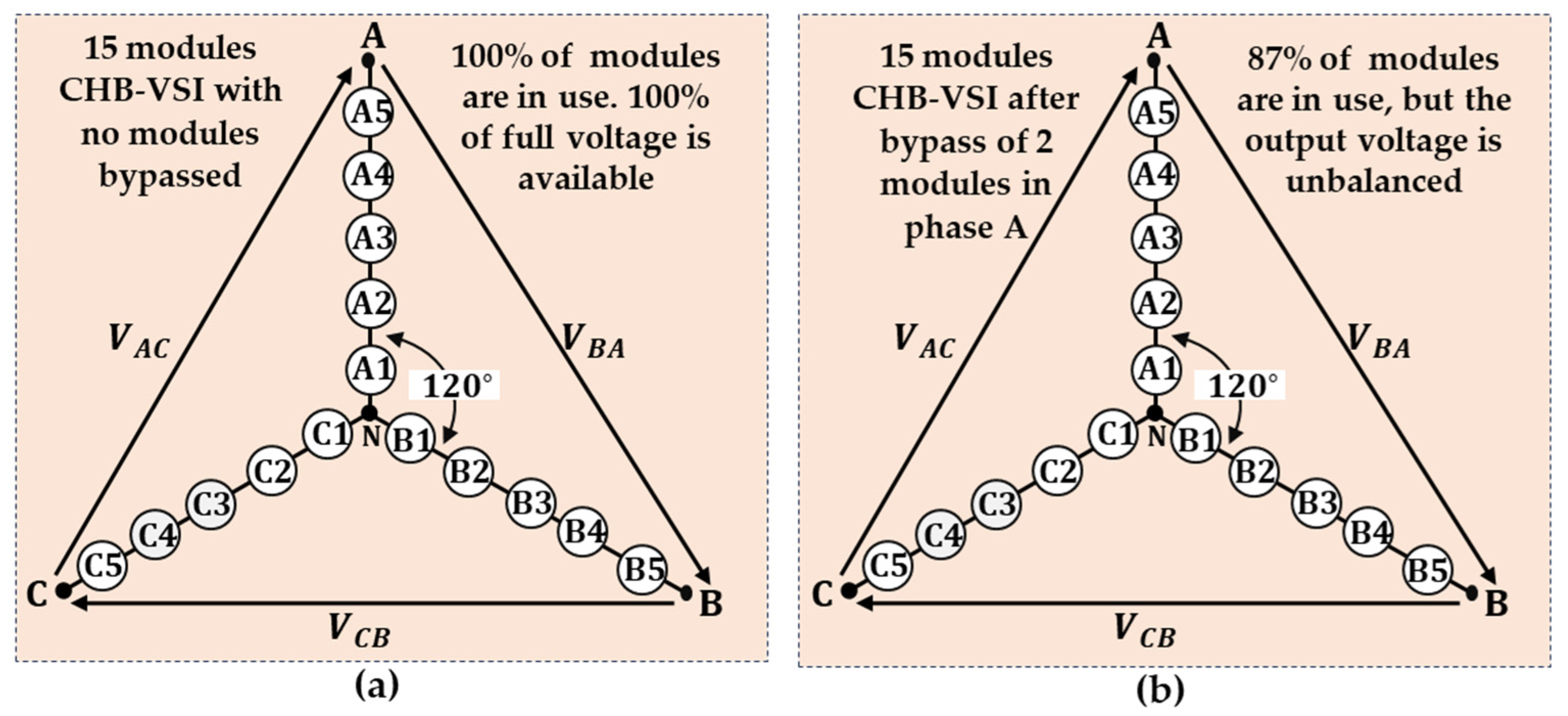
2.2.1. Basic Considerations
2.2.2. Conventional Neutral-Shift Control Method
- (a)
- Operating principle and implementation scheme
- (b)
- Advantages and disadvantages
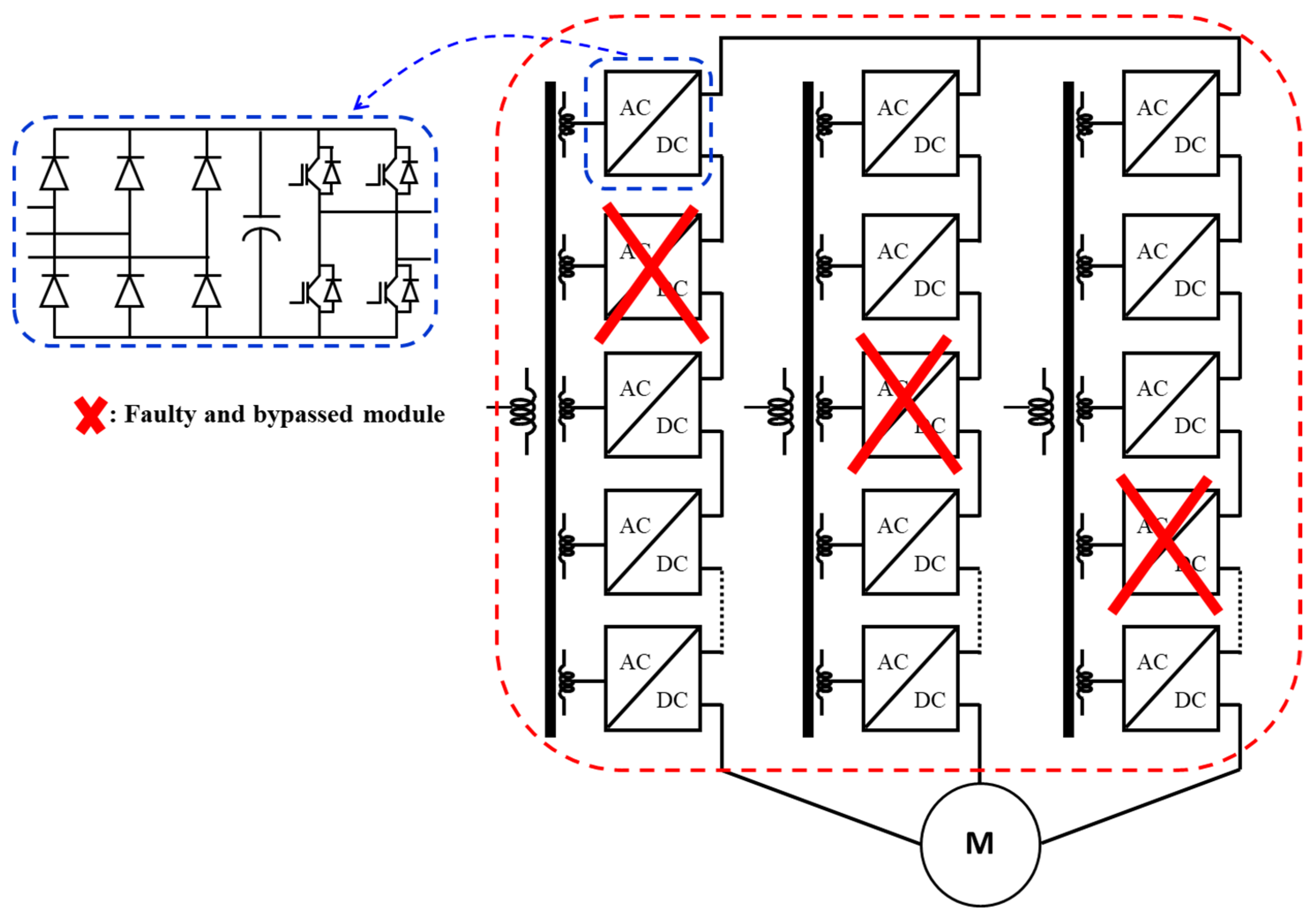
2.2.3. Peak-Reduction Fault-Tolerant Control Strategy
- (a)
- Operating principle and implementation scheme
- (b)
- Advantages and disadvantages
3. Suggested Future Trends
- (i)
- Universal compensation control technique: There is a gap in the existing literature regarding control methods that simultaneously address unequal DC voltage sources and failed cells in CHBMI topologies. A search of the literature did not release a unified and generalized compensation control technique that can rebalance both the CHBMI output LL voltages and currents at any unequal DC source conditions and simultaneously deal with the failed cells.
- (ii)
- Improved neutral-shift compensation control schemes with SVPWM approach: A thorough search of the literature has revealed the lack of a neutral-shift-based space vector pulse-width modulation (SVPWM) control scheme for the control of a cascaded H-bridge multilevel inverter (CHBMI) operating with fractional DC source voltages and where the inverter space vector diagram in -plan is formed with non-integer phase leg magnitudes ( ⅅ). This results in a highly complex control problem that existing neutral-shift-based SVPWM control methods cannot effectively address. This critical research gap must be addressed in the future by developing new analyses and control/modulation approaches optimized for such scenarios.
- (iii)
- Advanced control schemes: Recently, fault-tolerant and compensation strategies using model predictive control, including data-driven methods, have been suggested in the literature for CHBMIs under unbalanced operation [67,68,69]. The model predictive control methods are becoming increasingly popular in the advanced control of inverters. These methods have proven effective in preventing and compensating for issues related to unequal DC sources or failed cells. At the same time, they remain immune to system dynamics, such as changes in input and other parameters. However, a search of the literature did not release a model predictive control scheme with fault-tolerant capabilities considering the inverter operation with random fluctuations of DC source voltages (. Under these conditions, the inverter phasor in the -plan may have non-integer entries, and some states may disappear or slightly move within the phasor limits, depending on the severity of the unequal condition of DC sources. This adds high complexity to the control process. Existing model predictive-based compensation control methods may not be directly applicable or optimized for such scenarios.
- (iv)
- Harmonics and disturbance rejection: A harmonic analysis of existing compensation control methods for cascaded H-bridge inverter operation under unequal DC voltage sources and failed cells should be better conducted. The CHBMI output voltage and current spectra are adjusted using the investigated compensation control methods. Because the locations of the modified harmonics are unknown, it is crucial for the completeness of the design to analytically calculate these harmonics in the frequency domain, assess their effects on the power transmission line, and evaluate the type of stresses they are generating into the system where the CHBMI is involved. Mitigating harmonics and their related disturbances under the operation of CHBMIs with unequal DC voltage sources or failed cells is crucial for the overall system’s output power quality performances. Improving the existing compensation control methods to address this aspect more effectively is an ongoing research area.
- (v)
- Efficiency and thermal management: Achieving high efficiency and effective thermal management under unequal DC voltage sources or failed cells is critical for CHBMI systems, especially in high-power applications. Further work is needed to optimize existing compensation control methods for efficiency and thermal performance. In addition, inverter switching, and conduction losses are greatly influenced by using a given compensation control method. Consequently, power device losses and the overall CHBMI efficiency should be analyzed in depth in the view of practical applications.
- (vi)
- Simple and optimized switching strategy: The existing compensation control strategies are typically implemented using multicarrier-based or space-vector-based PWM techniques. Multi-carrier-based techniques are easy to implement but must guarantee optimized switching patterns and have high computation costs. On the other hand, SV PWM-based techniques offer flexibility in optimizing the switching patterns but require significant development efforts when the number of voltage levels produced by the inverter is large. The use of simplified and optimized modulation techniques during the system’s unbalanced operation will be a promising control solution. In recent years, single-carrier (SC-PWM) PWM methods have become more prevalent in multilevel converter topology control systems [70,71,72,73]. Compared to traditional multi-carrier PWM techniques or space vector-based techniques, they present a straightforward real-time implementation while achieving acceptable harmonic performances [73]. Developing compensation control methods based on an SC-PWM algorithms is needed.
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bose, B.K. The past, present and future of power electronics. IEEE Ind. Electron. Mag. 2009, 3, 7–14. [Google Scholar] [CrossRef]
- Rodriguez, J.; Bernet, S.; Wu, B.; Pont, J.; Kouro, S. Multilevel Voltage-Source-Converter Topologies for Industrial Medium-Voltage Drives. IEEE Trans. Ind. Electron. 2007, 54, 2930–2945. [Google Scholar] [CrossRef]
- Bana, P.R.; Panda, K.P.; Naayagi, R.T.; Panda, G. Recently developed reduced switch multilevel inverter for renewable energy integration and drives application: Topologies, comprehensive analysis, and comparative evaluation. IEEE Access 2019, 7, 54888–54909. [Google Scholar] [CrossRef]
- Salem, A.; Khang, H.V.; Robbersmyr, K.G.; Norambuena, M.; Rodriguez, J. Voltage source multilevel inverters with reduced device count: Topological review and novel comparative factors. IEEE Trans. Power Electron. 2021, 36, 2720–2747. [Google Scholar] [CrossRef]
- Kouro, S.; Malinowski, M.; Gopakumar, K.; Pou, J.; Franquelo, L.G.; Wu, B.; Rodriguez, J.; Pérez, M.A.; Leon, J.I. Recent advances and industrial applications of multilevel converters. IEEE Trans. Ind. Electron. 2010, 57, 2553–2580. [Google Scholar] [CrossRef]
- Carrasco, J.M.; Franquelo, L.G.; Bialasiewicz, J.T.; Galvan, E.; Portillo Guisado, R.C.; Prats, M.M.; Leon, J.I.; Moreno Alfonso, N. Power-electronic systems for the grid integration of renewable energy sources: A survey. IEEE Trans. Ind. Electron. 2006, 53, 1002–1016. [Google Scholar] [CrossRef]
- Perez, M.A.; Bernet, S.; Rodriguez, J.; Kouro, S.; Lizana, R. Circuit topologies, modeling, control schemes, and applications of modular multilevel converters. IEEE Trans. Power Electron. 2015, 30, 4–17. [Google Scholar] [CrossRef]
- Franquelo, L.G.; Rodriguez, J.; Leon, J.I.; Kouro, S.; Portillo, R.; Prats, M.A. The age of multilevel converters arrives. IEEE Trans Ind Electron. 2008, 2, 28–39. [Google Scholar] [CrossRef]
- Wang, C.; Li, Y. A Survey on Topologies of Multilevel Converters and Study of Two Novel Topologies. In Proceedings of the IEEE 6th International Power Electronics and Motion Control Conference, Wuhan, China, 17–20 May 2009; pp. 860–865. [Google Scholar]
- Rahman, S.; Meraj, M.; Iqbal, A.; Prathap-Reddy, B.; Khan, I. A Combinational Level-Shifted and Phase-Shifted PWM Technique for Symmetrical Power Distribution in CHB Inverters. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 932–941. [Google Scholar] [CrossRef]
- Peng, X.; He, X.; Han, P.; Lin, X.; Shu, Z.; Gao, S. Sequence pulse modulation for voltage balance in a cascaded H-bridge rectifier. J. Power Electron. 2017, 17, 664–673. [Google Scholar] [CrossRef]
- Li, X.; Chai, J.; Li, M.; Li, L.; You, R. A Grid Frequency Support Control Strategy of the Three Phase Cascaded H-Bridge Based Photovoltaic Generation System. IEEE Access 2022, 10, 56974–56984. [Google Scholar] [CrossRef]
- Tian, J.; Hu, D.; Zhou, C.; Yang, Y.; Wu, W.; Mao, C.; Wang, D. Individual DC voltage balance control for cascaded Hbridge electronic power transformer with separated DC-link topology. IEEE Access 2019, 7, 38558–38567. [Google Scholar] [CrossRef]
- Rauf, A.M.; Abdel-Monem, M.; Hegazy, G.O. A Review on Multilevel Converters for Efficient Integration of Battery Systems in Stationary Applications. Energies 2023, 16, 4133. [Google Scholar] [CrossRef]
- Mouselinos, T.P.; Tatakis, E.C. Multilevel inverters: A survey of limitations and recommended problem-solving techniques. In Proceedings of the 21st European Conference on Power Electronics and Applications (EPE ’19 ECCE Europe), Genova, Italy, 3–5 September 2019; pp. 1–10. [Google Scholar]
- Admed, M. Classical Control for Unequal DC Sources Five-Level Inverter-Based SHE Technique. Energies 2020, 13, 4715. [Google Scholar] [CrossRef]
- Akhmetov, Z.; Hammami, M.; Grandi, G.; Ruderman, A. On PWM Strategies and Current THD for Single- and Three-Phase Cascade H-Bridge Inverters with Non-Equal DC Sources. Energies 2019, 12, 441. [Google Scholar] [CrossRef]
- Hamza, H.; Song-Manguelle, J.; Lingom, P.M.; Nyobe-Yome, J.; Doumbia, M.L. A Review of Fault-Tolerant Control Methods for Cascaded H-Bridge Multilevel Inverters. In Proceedings of the 14th IEEE International Conference on Power Electronics and Drive Systems (PEDS), Montreal, QC, Canada, 7–10 August 2023. [Google Scholar]
- Xiong, J.; Zhou, F.; Li, Q. Soft-switching modulation of the multilevel cascaded H-bridge inverter under DC source fault. IET Gener. Transm. Distrib. 2021, 15, 1337–1347. [Google Scholar] [CrossRef]
- Yang, K.; Lan, X.; Zhang, Q.; Tang, X. Unified selective harmonic elimination for cascaded Hbridge asymmetric multilevel inverter. IEEE J. Emerg. Sel. Top. Power Electron. 2018, 6, 2138–2146. [Google Scholar] [CrossRef]
- Ghazanfari, A.; Mokhtari, H.; Firouzi, M. Simple voltage balancing approach for CHB multilevel inverter considering low harmonic content based on a hybrid optimal modulation strategy. IEEE Trans. Power Deliv. 2012, 27, 2150–2158. [Google Scholar] [CrossRef]
- Townsend, C.D.; Summers, T.J.; Betz, R.E. Impact of practical issues on the harmonic performance of phase-shifted modulation strategies for a cascaded H-bridge statcom. IEEE Trans. Ind. Electron. 2013, 61, 2655–2664. [Google Scholar] [CrossRef]
- Kim, I.; Chan, R.; Kwak, S. Model predictive control method for CHB multi-level inverter with reduced calculation complexity and fast dynamics. IET Electr. Power Appl. 2017, 11, 784–792. [Google Scholar] [CrossRef]
- Zhang, Y.; Wu, X.; Yuan, X.; Wang, Y.; Dai, P. Fast model predictive control for multilevel cascaded Hbridge STATCOM with polynomial computation time. IEEE Trans. Ind. Electron. 2016, 63, 5231–5243. [Google Scholar]
- Chan, R.; Kwak, S. Improved Finite-Control-Set Model Predictive Control for Cascaded H-Bridge Inverters. Energies 2018, 11, 355. [Google Scholar] [CrossRef]
- Shu, Z.; He, X.; Wang, Z.; Qiu, D.; Jing, Y. Voltage balancing approaches for diode-clamped multilevel converters using auxiliary capacitor-based circuits. IEEE Trans. Power Electron. 2013, 28, 2111–2124. [Google Scholar] [CrossRef]
- Wang, Q.; Liu, K.; Wang, K.; Qin, L.; Zhang, J.; Pu, Q. Fast capacitor voltage balancing strategy based on system history operation information for MMC. IET Gener. Transm. Distrib. 2019, 13, 1104–1109. [Google Scholar] [CrossRef]
- Wang, L.; Wu, Q.; Tang, W. Energy Balance Control of a Cascaded Multilevel Inverter for Standalone Solar Photovoltaic Applications. Energies 2017, 10, 1805. [Google Scholar] [CrossRef]
- Lingom, P.M.; Song-Manguelle, J.; Betoka-Onyama, S.P.; Nyobe-Yome, J.M.; Doumbia, M.L. A Power Quality Assessment of Electric Submersible Pumps Fed by Variable Frequency Drives under Normal and Failure Modes. Energies 2023, 16, 5121. [Google Scholar] [CrossRef]
- Mirafzal, B. Survey of Fault-Tolerance Techniques for Three-Phase Voltage Source Inverters. IEEE Trans. Ind. Electron. 2014, 61, 5192–5202. [Google Scholar] [CrossRef]
- Gao, Z.; Cecati, C.; Ding, S.X. A Survey of Fault Diagnosis and Fault-Tolerant Techniques—Part I: Fault Diagnosis with Model-Based and Signal-Based Approaches. IEEE Trans. Ind. Electron. 2015, 62, 3757–3767. [Google Scholar] [CrossRef]
- Gautam, S.P.; Jalhotra, M.; Sahu, L.K.; Kumar, M.R.; Gupta, K.K. A Survey on Fault Tolerant and Diagnostic Techniques of Multilevel Inverter. IEEE Access 2023, 11, 60866–60888. [Google Scholar] [CrossRef]
- Lingom, P.; Song-Manguelle, J.; Mon-Nzongo, D.L.; Lingom, P.; Flesch, R.C.C.; Jin, T. Analysis and control of PV cascaded H-bridge multilevel inverter with failed cells and changing meteorological conditions. IEEE Trans. Power Electron. 2021, 36, 1777–1789. [Google Scholar] [CrossRef]
- Song, Y.; Wang, B. Survey on reliability of power electronic systems. IEEE Trans. Power Electron. 2013, 28, 591–604. [Google Scholar] [CrossRef]
- Lezana, P.; Ortiz, G. Extended operation of cascade multicell converters under fault condition. IEEE Trans. Ind. Electron. 2009, 56, 2697–2703. [Google Scholar] [CrossRef]
- Hammond, P.W. Enhancing the reliability of modular medium voltage drives. IEEE Trans. Ind. Electron. 2002, 49, 948–954. [Google Scholar] [CrossRef]
- Lingom, P.M.; Song-Manguelle, J.; Nyobe-Yome, J.M.; Mon-Nzongo, D.L.; Jin, T.; Doumbia, M.L. Control of a Cascaded H-bride Multilevel Inverter with Failed cells for Grid-Connected Application. In Proceedings of the 10th IEEE International Symposium on Power Electronics for Distributed Generation Systems, Xi’an, China, 3–6 June 2019. [Google Scholar]
- Lingom, P.; Song-Manguelle, J.; Betoka-Onyama, S.P.; Doumbia, M.L.; Nyobe-Yome, J.M.; Jin, T. A generalized modulation strategy for cascaded H-bridge Multilevel Inverter under Unequal DC Sources. In Proceedings of the IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 9–13 October 2022. [Google Scholar]
- Kumar, A.; Verma, V. Performance enhancement of single-phase grid-connected PV system under partial shading using cascaded multilevel converter. IEEE Trans. Ind. Appl. 2018, 54, 2665–2676. [Google Scholar] [CrossRef]
- Tolbert, L.M.; Chiasson, J.N.; McKenzie, K.J.; Zhong, D. Control of cascaded multilevel converters with unequal voltage sources for HEVs. In Electric Machines and Drives Conference, Proceedings of the IEMDC’03, Xi’an, China, 3–6 June 2019; IEEE International: New York, NY, USA, 2003; pp. 663–669. [Google Scholar]
- Marzo, I.; Sanchez-Ruiz, A.; Barrena, J.A.; Abad, G.; Muguruza, I. Power Balancing in Cascaded H-Bridge and Modular Multilevel Converters Under Unbalanced Operation: A Review. IEEE Access 2021, 9, 110525–110543. [Google Scholar] [CrossRef]
- Lamb, J.; Mirafzal, B. An Adaptive SPWM Technique for Cascaded Multilevel Converters with Time-Variant DC Sources. IEEE Trans. Ind. Appl. 2016, 52, 4146–4156. [Google Scholar] [CrossRef]
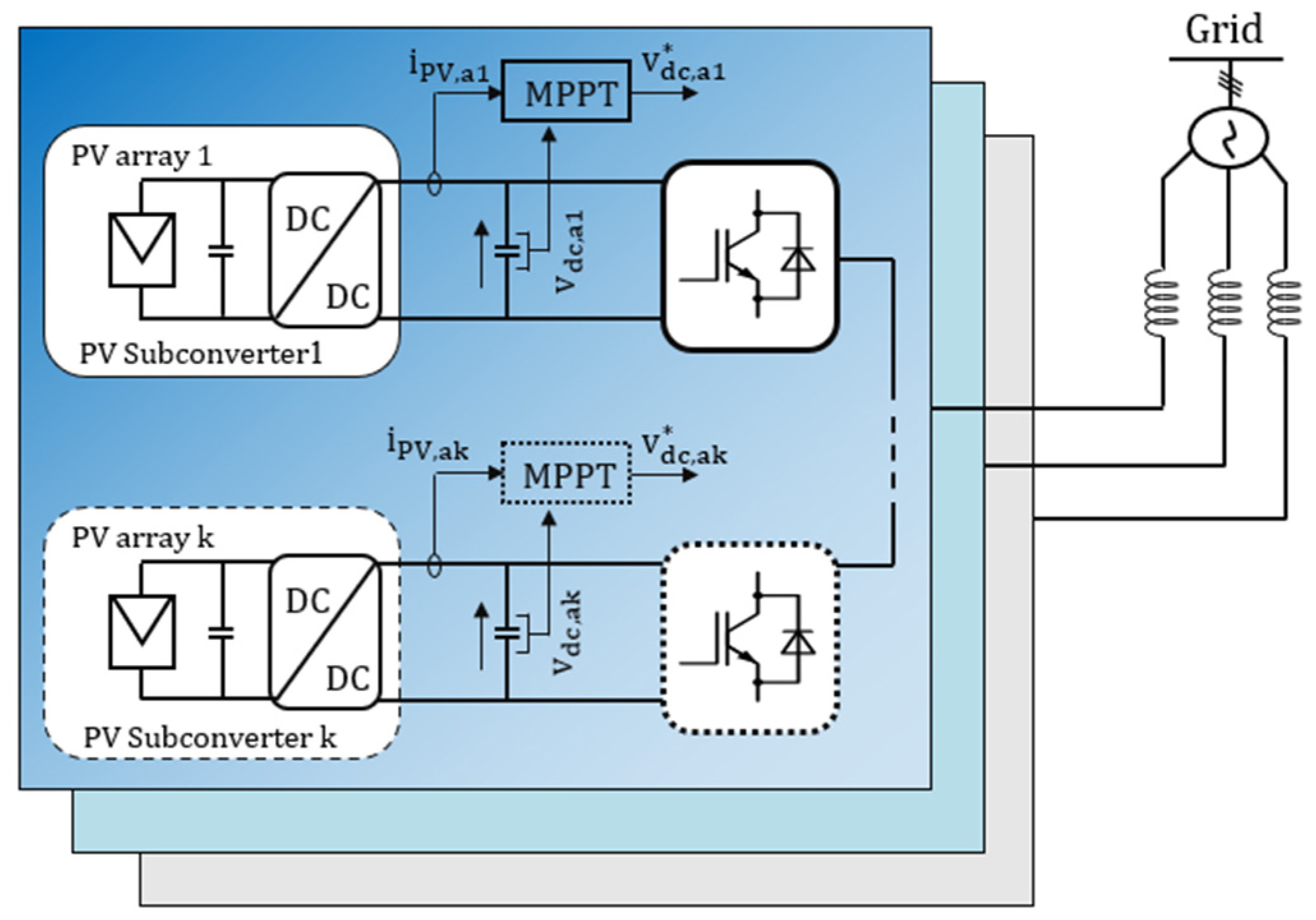
- Rivera, S.; Kouro, S.; Wu, B.; Leon, J.; Rodriguez, J.; Franquelo, L. Cascaded H-Bridge multilevel converter multistring topology for Large Scale Photovoltaic System. In Proceedings of the 2011 IEEE International Symposium on Industrial Electronics, Gdansk, Poland, 27–30 June 2011; pp. 1837–1844. [Google Scholar]
- Xiao, B.; Hang, L.; Mei, J.; Riley, C.; Tolbert, L.M.; Ozpineci, B. Modular cascaded h-bridge multilevel PV inverter with distributed MPPT for grid-connected applications. IEEE Trans. Ind. Appl. 2015, 51, 1722–1731. [Google Scholar] [CrossRef]
- Yu, Y.; Konstantinou, G.; Townsend, C.D. Power balance of cascaded H-bridge multilevel converters for large-scale photovoltaic integration. IEEE Trans. Power Electron. 2016, 1, 292–303. [Google Scholar] [CrossRef]
- Yu, Y.; Konstantinou, G.; Hredzak, B.; Agelidis, V.G. On extending the energy balancing limit of multilevel cascaded H-bridge converters for largescale photovoltaic farms. In Proceedings of the 2013 Australasian Universities Power Engineering Conference (AUPEC), Hobart, Australia, 29 September–3 October 2013; pp. 1–6. [Google Scholar]
- Yu, Y.; Konstantinou, G.; Hredzak, B.; Agelis, V. Operation of cascaded H-bridge multilevel converters for large-scale photovoltaic power plants under bridge failures. IEEE Trans. Ind. Electron. 2015, 62, 7228–7236. [Google Scholar] [CrossRef]
- Xue, H.; He, J. A Simplified Power Balance Strategy for Three-Phase Cascaded H-bridge Photovoltaic Inverter. In Proceedings of the 2022 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 9–13 October 2022; pp. 1–6. [Google Scholar]
- Yu, Y.; Konstantinou, G.; Townsend, C.D.; Agelis, V. Comparison of zero-sequence injection methods in cascaded h-bridge multilevel converters for large-scale photovoltaic integration. IET Renew. Power Gen. 2017, 11, 603–613. [Google Scholar] [CrossRef]
- Summers, T.J.; Betz, R.E.; Mirzaeva, G. Phase leg voltage balancing of a cascaded H-Bridge converter based STATCOM using zero sequence injection. In Proceedings of the 2009 13th European Conference on Power Electronics and Applications, Barcelona, Spain, 8–10 September 2009; pp. 1–10. [Google Scholar]
- Cho, Y.; Labella, T.; Lai, J.S.; Senesky, M.K. A Carrier-Based Neutral Voltage Modulation Strategy for Multilevel Cascaded Inverters under Unbalanced DC Sources. IEEE Trans. Ind. Electron. 2014, 61, 625–636. [Google Scholar] [CrossRef]
- Kim, J.; Cho, Y. Neutral Voltage Modulation for Maximizing the Linear Modulation Region and Limp-Home Mode Operation of Multilevel Cascaded Inverters under DC-Link Imbalance Conditions. IEEE Access 2022, 10, 13515–13524. [Google Scholar] [CrossRef]
- Ye, Z.B.; Wang, T.T. A PWM strategy based on state transition for cascaded H-bridge inverter under unbalanced DC sources. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 8, 1686–1700. [Google Scholar] [CrossRef]
- Xia, Z.; Wu, Q.; Ye, Z.; Yu, D.; Zhu, L.; Hu, W. A Novel DC-Power Control Method for Cascaded Converter under DC Voltage-Imbalance Condition. J. Electr. Comput. Eng. 2022, 37, 12301–12315. [Google Scholar] [CrossRef]
- Lezana, P.; Pou, J.; Meynard, T.A.; Rodriguez, J.; Ceballos, S.; Richardeau, F. Survey on fault operation on multilevel inverters. IEEE Trans. Ind. Electron. 2010, 57, 2207–2218. [Google Scholar] [CrossRef]
- Zhang, W.; Xu, D.; Enjeti, P.N.; Li, H.; Hawke, J.T.; Krishnamoorthy, H.S. Survey on fault-tolerant techniques for power electronic converters. IEEE Trans. Power Electron. 2014, 29, 6319–6331. [Google Scholar] [CrossRef]
- Rahimpour, S.; Husev, O.; Vinnikov, D.; Kurdkandi, N.V.; Tarzamni, H. Fault management techniques to enhance the reliability of power electronic converters: An overview. IEEE Access 2023, 11, 13432–13446. [Google Scholar] [CrossRef]
- Salimian, H.; Iman-Eini, H. Fault-tolerant operation of three-phase cascaded H-bridge converters using an auxiliary module. IEEE Trans. Ind. Electron. 2017, 64, 1018–1027. [Google Scholar] [CrossRef]
- Kang, J.-W.; Hyun, S.-W.; Ha, J.-O.; Won, C.-Y. Improved Neutral-Point Voltage-Shifting Strategy for Power Balancing in Cascaded NPC/H-Bridge Inverter. Electronics 2018, 7, 167. [Google Scholar] [CrossRef]
- Rodriguez, J.; Hammond, P.W.; Pontt, J.; Musalem, R.; Lezana, P.; Escobar, M.J. Operation of a medium-voltage drive under faulty conditions. IEEE Trans. Ind. Electron. 2005, 52, 1080–1085. [Google Scholar] [CrossRef]
- Kim, S.; Lee, J.; Lee, K. A modified level-shifted PWM strategy for fault-tolerant cascaded multilevel inverters with improved power distribution. IEEE Trans. Ind. Electron. 2016, 63, 7264–7274. [Google Scholar] [CrossRef]
- Lopez, O.; Komrska, T.; Alvarez, J.; Adam, J. Post-Fault Operation Strategy for Cascaded H-Bridge Inverters Driving a Multiphase Motor. IEEE Trans. Ind. Electron. 2024, 71, 4309–4319. [Google Scholar] [CrossRef]
- Carnielutti, F.; Pinheiro, H.; Rech, C. Generalized carrier-based modulation strategy for cascaded multilevel converters operating under fault conditions. IEEE Trans. Ind. Electron. 2012, 59, 679–689. [Google Scholar] [CrossRef]
- Aleenejad, M.; Mahmoudi, H.; Jafarishiadeh, S.; Ahmadi, R. Fault-tolerant space vector modulation for modular multilevel converters with bypassed faulty submodules. IEEE Trans. Ind. Electron. 2019, 66, 2463–2473. [Google Scholar] [CrossRef]
- Carnielutti, F.; Rech, C.; Pinheiro, H. Extending the operation of asymmetrical cascaded multilevel converters under fault conditions on the converter power cells. IEEE Trans. Ind. Electron. 2017, 64, 1853–1862. [Google Scholar] [CrossRef]
- Li, G.; Liu, C.; Wang, Y. Voltage Space Vector Equivalent Substitution Fault-Tolerance Control for Cascaded H-Bridge Multilevel Inverter with Current-Tracking. Electronics 2020, 9, 93. [Google Scholar] [CrossRef]
- Ipoum Ngome, P.G.; Mon-Nzongo, D.L.; Tang, J.; Jin, T.; Flesch, R.C.C.; Wang, M. Multiobjective Model-Free Predictive Control for Motor Drives and Grid-Connected Applications: Operating with Unbalanced Multilevel Cascaded H-Bridge Inverters. IEEE Trans. Ind. Electron. 2023, 38, 3014–3028. [Google Scholar] [CrossRef]
- Ipoum Ngome, P.G.; Mon-Nzongo, D.L.; Tang, J.; Jin, T.; Flesch, R.C.C.; Wang, M. Fast Data-Driven Predictive Controller for Multilevel Cascaded H-bridge Inverters with Faulty H-bridge cells. In Proceedings of the 2021 IEEE International Conference on Predictive Control of Electrical Drives and Power Electronics (PRECEDE), Jinan, China, 18–20 September 2021; pp. 709–714. [Google Scholar]
- Aguilera, R.P.; Acuna, P.; Yu, Y.; Konstantinou, G.; Townsend, C.D.; Wu, B.; Agelidis, V.G. Predictive control of cascaded H-bridge converters under unbalanced power generation. IEEE Trans. Ind. Electron. 2017, 64, 4–13. [Google Scholar] [CrossRef]
- Lingom, P.M.; Song-Manguelle, J.; Unruh, R.; Nyobe-Yome, J.M.; Doumbia, M.L. Performance Evaluation of Multi-Modulation Single-Carrier PWM Strategies for Motor drive applications. In Proceedings of the 14th IEEE International Conference on Power Electronics and Drive Systems (PEDS), Montreal, QC, Canada, 7–10 August 2023. [Google Scholar]
- Lingom, P.M.; Song-Manguelle, J.; Menye, J.; Unruh, R.; Doumbia, M.L.; Jin, T. Single-Carrier PWM method for Unifor Step Asymmetrical Multilevel Converters. In Proceedings of the 10th IEEE International Symposium on Power Electronics for Distributed Generation Systems, Xi’an, China, 3–6 June 2019. [Google Scholar]
- Lingom, P.M.; Song-Manguelle, J.; Flesch RC, C.; Jin, T. A generalized Single-Carrier PWM scheme for multilevel converters. IEEE Trans. Power Electron. 2021, 36, 12112–12126. [Google Scholar] [CrossRef]
- Bhanuchandar, A.K.; Murthy, B. A new generalized predictive current control algorithm with integration of fractional part function-based modulation technique: Single phase self-balanced type multilevel inverter topologies. Int. J. Circuit Theory Appl. 2023, 1–20. [Google Scholar] [CrossRef]
- IEEE Standard C37.2-2008; Electrical Power System Device Function Numbers, Acronyms, and Contact, Designations. IEEE: New York, NY, USA, 2008.
- Ounejjar, Y.; Al-Haddad, K. A novel high energetic efficiency multilevel topology with reduced impact on supply network. In Proceedings of the 34th IEEE Annual Conference of IEEE Industrial Electronics, Orlando, FL, USA, 10–13 November 2008; pp. 489–494. [Google Scholar] [CrossRef]
- Sharif Zadeh, M.; Al-Haddad, K. Packed E-Cell (PEC) converter topology operation and experimental validation. IEEE Access 2019, 7, 93049–93061. [Google Scholar] [CrossRef]
- Laib, A.; Krama, A.; Sahli, A.; Kihal, A.; Abu-Rub, H. Reconfigurable Model Predictive Control for Grid Connected PV Systems Using Thirteen-Level Packed E-Cell Inverter. IEEE Access 2022, 10, 102210–102222. [Google Scholar] [CrossRef]


| NPC | FC | CHB | |
|---|---|---|---|
| Number of components | ↑ ↑ | ↑ ↑ ↓ | ↓ ↓ Isolated DC sources |
| Modularity | Low | High | High |
| Control complexity | Medium | High | High |
| Control concerns | Voltage balancing | Voltage setup | Power sharing |
| Fault tolerance | Difficult | Easy | Easy |
| Concerns | CHBMI with unequal DC voltage sources | |
| Methods | ||
| Feed-forward control methods [12,43,44] |
| |
| Zero-sequence injection-based control methods [45,46,47,48,49,50] |
| |
| Concerns | CHBMI with failed cells | |
| Methods | ||
| Neutral-shift fault-tolerant control method [36,59,60] |
| |
| Peak-reduction fault-tolerant control method [61,62,63] |
| |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lingom, P.M.; Song-Manguelle, J.; Nyobe-Yome, J.M.; Doumbia, M.L. A Comprehensive Review of Compensation Control Techniques Suitable for Cascaded H-Bridge Multilevel Inverter Operation with Unequal DC Sources or Faulty Cells. Energies 2024, 17, 722. https://doi.org/10.3390/en17030722
Lingom PM, Song-Manguelle J, Nyobe-Yome JM, Doumbia ML. A Comprehensive Review of Compensation Control Techniques Suitable for Cascaded H-Bridge Multilevel Inverter Operation with Unequal DC Sources or Faulty Cells. Energies. 2024; 17(3):722. https://doi.org/10.3390/en17030722
Chicago/Turabian StyleLingom, Pascal M., Joseph Song-Manguelle, Jean Maurice Nyobe-Yome, and Mamadou L. Doumbia. 2024. "A Comprehensive Review of Compensation Control Techniques Suitable for Cascaded H-Bridge Multilevel Inverter Operation with Unequal DC Sources or Faulty Cells" Energies 17, no. 3: 722. https://doi.org/10.3390/en17030722
APA StyleLingom, P. M., Song-Manguelle, J., Nyobe-Yome, J. M., & Doumbia, M. L. (2024). A Comprehensive Review of Compensation Control Techniques Suitable for Cascaded H-Bridge Multilevel Inverter Operation with Unequal DC Sources or Faulty Cells. Energies, 17(3), 722. https://doi.org/10.3390/en17030722






