Rapid Breakdown Time in Positive Impulse Voltages through Spectroscopy Analysis
Abstract
1. Introduction
2. Experimental Methods
3. Results
3.1. Breakdown Voltage
3.2. Breakdown Time Determination
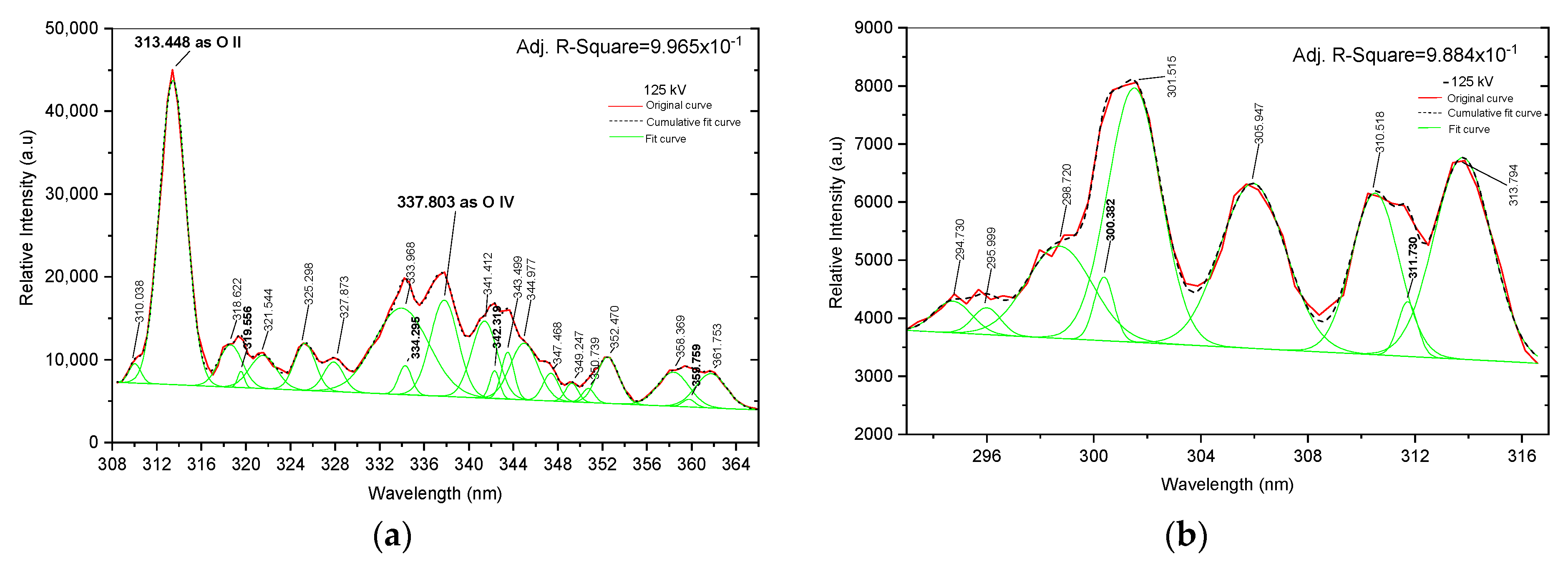
3.3. Spectral Line Identifications
4. Discussion
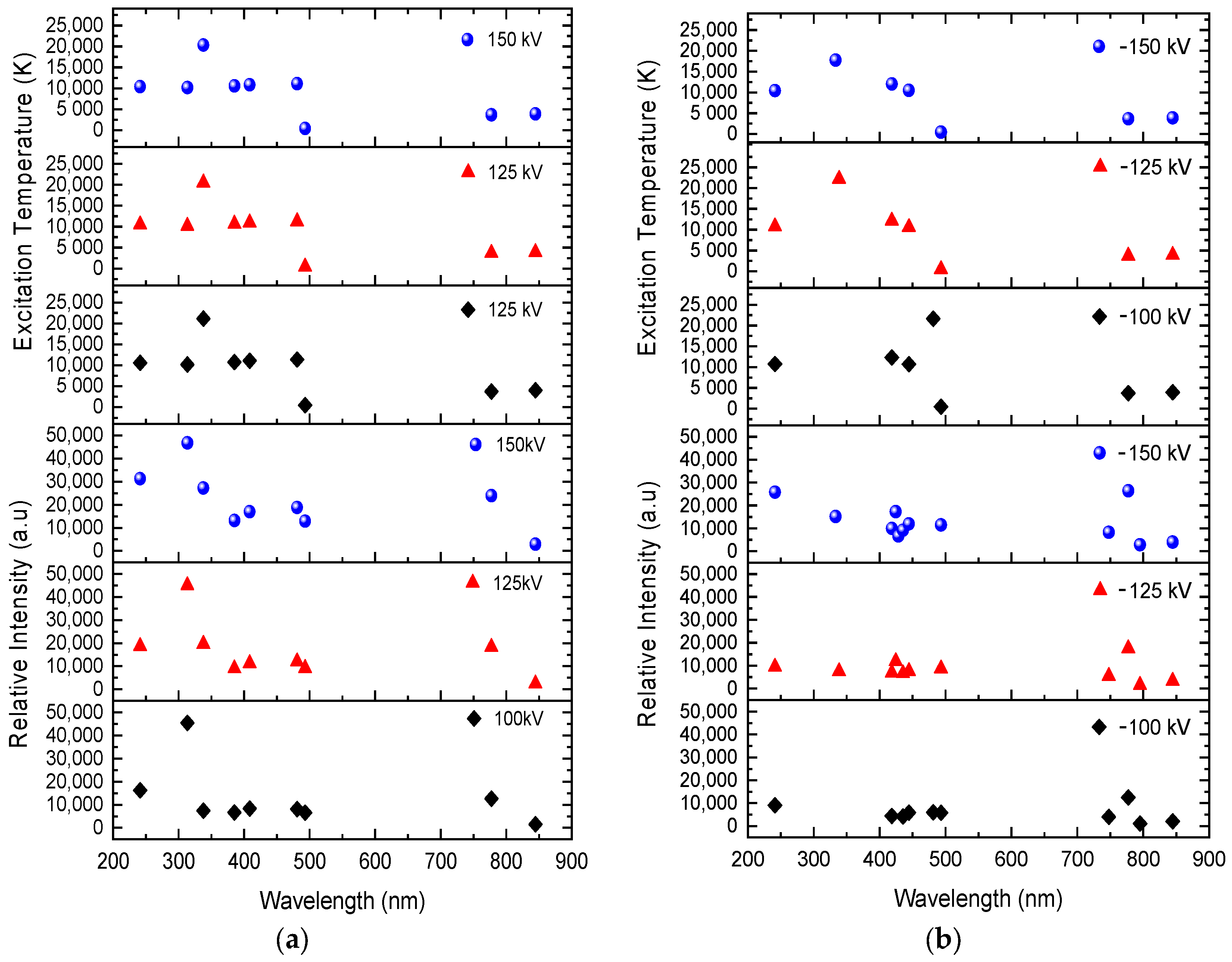
4.1. Atomic Distribution and Decay Time
4.2. Correlation between Excitation Temperature and Decay Time
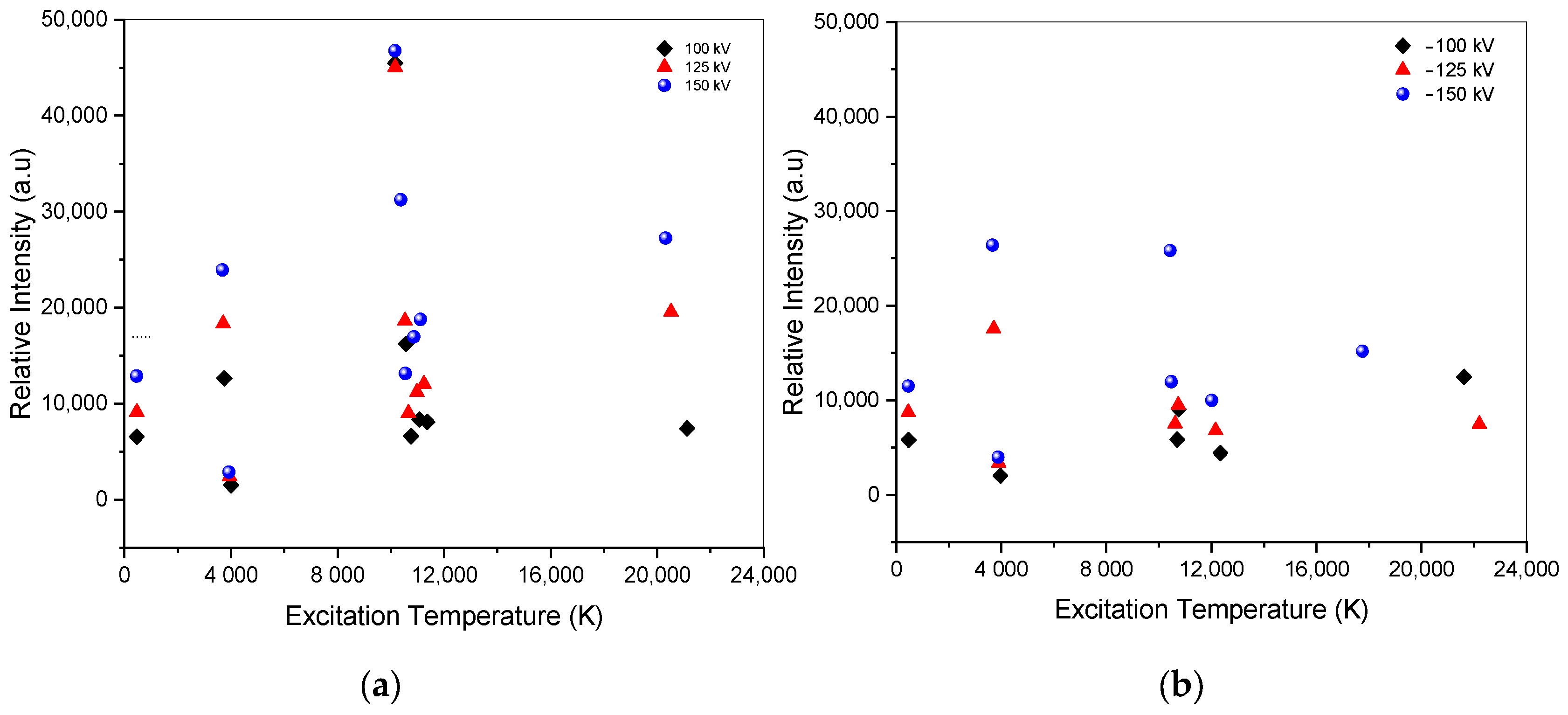
4.3. Correlation Decay Time, Excitation Temperature, and Intensity
5. Conclusions
- The higher intensities of energetic photons in the 200–400 nm bands, compared to the bands in the 400–900 nm range, are only observed in positive spectra at 241.162 nm, 313.421 nm, and 337.806 nm, with the greatest intensity observed at 313.421 nm. The distributions of oxygen atoms in the excited state are more intense in positive voltages than in negative voltages. The spectral line of O II at 313.421 nm was found to decay more quickly than other oxygen atoms in the wavelength range of 200 to 900 nm in positive voltage, falling to lower energy levels in a decay period of 1.302 × 10−8 s. Similar to this, the O II line at 418.545 nm has a decay negative voltage duration of 5.236 × 10−9 s. On the other hand, as is covered in Section 4.1, the recombination state of positive and negative voltages occurs at 2.71 × 10−8 s.
- Increased energy transitions of atomic excited states to lower energy states, which produce photons with a specific wavelength under both positive and negative voltages after their limited decay time occurs, are typically indicators of a higher temperature during the excitation process. As discussed in Section 4.2, the excitation temperatures of positive and negative voltages are different: positive voltages exceed 10,000 K in 1.302 × 10−8 s and negative voltages above 12,000 K in 5.236 × 10−9 s, respectively. Due to this, negative voltages breakdown more quickly than positive ones, requiring more heat to keep the channel stable and is not achieved until the recombination state happens in 2.710 × 10−8 s.
- Section 4.3 concludes that, for positive voltages, peak intensities are higher in the excited state than in the recombination state; however, for negative voltages, there are no higher peak intensities in comparison to the recombination state. Positive voltages tend to favor excitation temperatures between 10,000 and 22,000 K more than negative voltages in the excited state. In order to make conductive channels more practical for releasing Joule heating and allowing electrons to flow more readily in positives than in negatives, this helps maintain channels. Interestingly, at positive voltages, there are spectral lines at 241.162 nm, 313.421 nm, and 337.806 nm that are higher in the excited state than in the recombination state. These lines can help hit and accelerate electrons through processes like scattering, which is correlated with photon emission and leads to a rapid breakdown time in positive discharge.
- Positive voltages experience higher excitation temperatures than negative voltages, suggesting a more intense release of Joule heating and facilitating easier electron flows, resulting in a rapid breakdown time with higher peak intensity of O II at 313.421 and 241.162 nm, as well as OIV at 337.806 nm, than O I at 777.417 nm. Moreover, fast arc discharge is caused by these energetic photon emissions hitting and accelerating electrons.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kojima, H.; Hotta, K.; Kitamura, T.; Hayakawa, N.; Otake, A.; Kobayashi, K.; Kato, T.; Rokunohe, T.; Okubo, H. Classification of impulse breakdown mechanisms under non-uniform electric field in air. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 194–201. [Google Scholar] [CrossRef]
- Sigmond, R.S. The residual streamer channel: Return strokes and secondary streamers. J. Appl. Phys. 1984, 56, 1355–1370. [Google Scholar] [CrossRef]
- Kip, A.F. Positive-Point-to-Plane Discharge in Air at Atmospheric Pressure. Phys. Rev. 1938, 54, 139–146. [Google Scholar] [CrossRef]
- Ducasse, O.; Eichwald, O.; Merbahi, N.; Dubois, D.; Yousfi, M. Numerical simulation and comparison with experiment for a positive point to plane corona discharges in dry air. In Proceedings of the ICPIG 2007: XXVIII International Conference on Phenomena in Ionized Gases, Prague, Czech Republic, 15–20 July 2007. [Google Scholar]
- Naidis, G.V. Dynamics of streamer breakdown of short non uniform air gaps. J. Phys. D 2005, 38, 3889–3893. [Google Scholar] [CrossRef]
- Marode, E. The mechanism of spark breakdown in air at atmospheric pressure between a positive point and plane. II. Theoretical: Computer simulation of the streamer track. J. Appl. Phys. 1975, 46, 2016–2020. [Google Scholar] [CrossRef]
- Chowdhuri, P.; Mishra, A.K.; Martin, P.M.; McConnell, B.W. The effects of nonstandard lightning voltage waveshapes on the impulse strength of short air gaps. IEEE Trans. Power Deliv. 1994, 9, 1991–1999. [Google Scholar] [CrossRef]
- David, Z.P.; Deanna, A.L.; Christophe, O.L. Transitions between corona, glow, and spark regimes of nanosecond repetitively pulsed discharges in air at atmospheric pressure. J. Appl. Phys. 2010, 107, 093303. [Google Scholar]
- Schubert, U.; Shirvani, A.; Schmidt, U. Description of the Discharge Process in Long Air Gaps under Lightning Impulse Voltage. IEEE Trans. Power Deliv. 2023, 38, 2454–2463. [Google Scholar] [CrossRef]
- Gallimberti, I.; Bacchiega, G.; Anne, B.C.; Philippe, L. Fundamental processes in long air gap discharges. Comptes Rendus Phys. 2002, 3, 1335–1359. [Google Scholar] [CrossRef]
- NIST Atomic Spectra Spectra Database Lines Form. Available online: https://physics.nist.gov/PhysRefData/ASD/lines_form.html (accessed on 23 August 2023).
- Hansjoachim, B. Pulsed Power Systems Principles and Applications; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 2006; pp. 17–18. [Google Scholar]
- Janet, L.; Pralhad, R. Foundations of Pulsed Power Technology; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2017; pp. 158–162. [Google Scholar]
- Prueitt, M. The excitation temperature of lightning. J. Geophys. Res. 1963, 68, 803–811. [Google Scholar] [CrossRef]
- Sahin, N.; Tanish, M. Electron temperature estimation of helium plasma via line intensity ratio at atmospheric pressure. Eur. Phys. J. Plus 2020, 135, 653. [Google Scholar] [CrossRef]
- Bloch, J.; Cavalleri, A.; Galitski, V.; Hafezi, M.; Rubio, A. Strongly correlated electron–photon systems. Nature 2022, 606, 41–48. [Google Scholar] [CrossRef]
- Wang, J.S.; Peng, J.; Zhang, Z.Q.; Zhang, Y.M.; Zhu, T. Transport in electron-photon systems. Front. Phys. 2023, 18, 43602. [Google Scholar] [CrossRef]


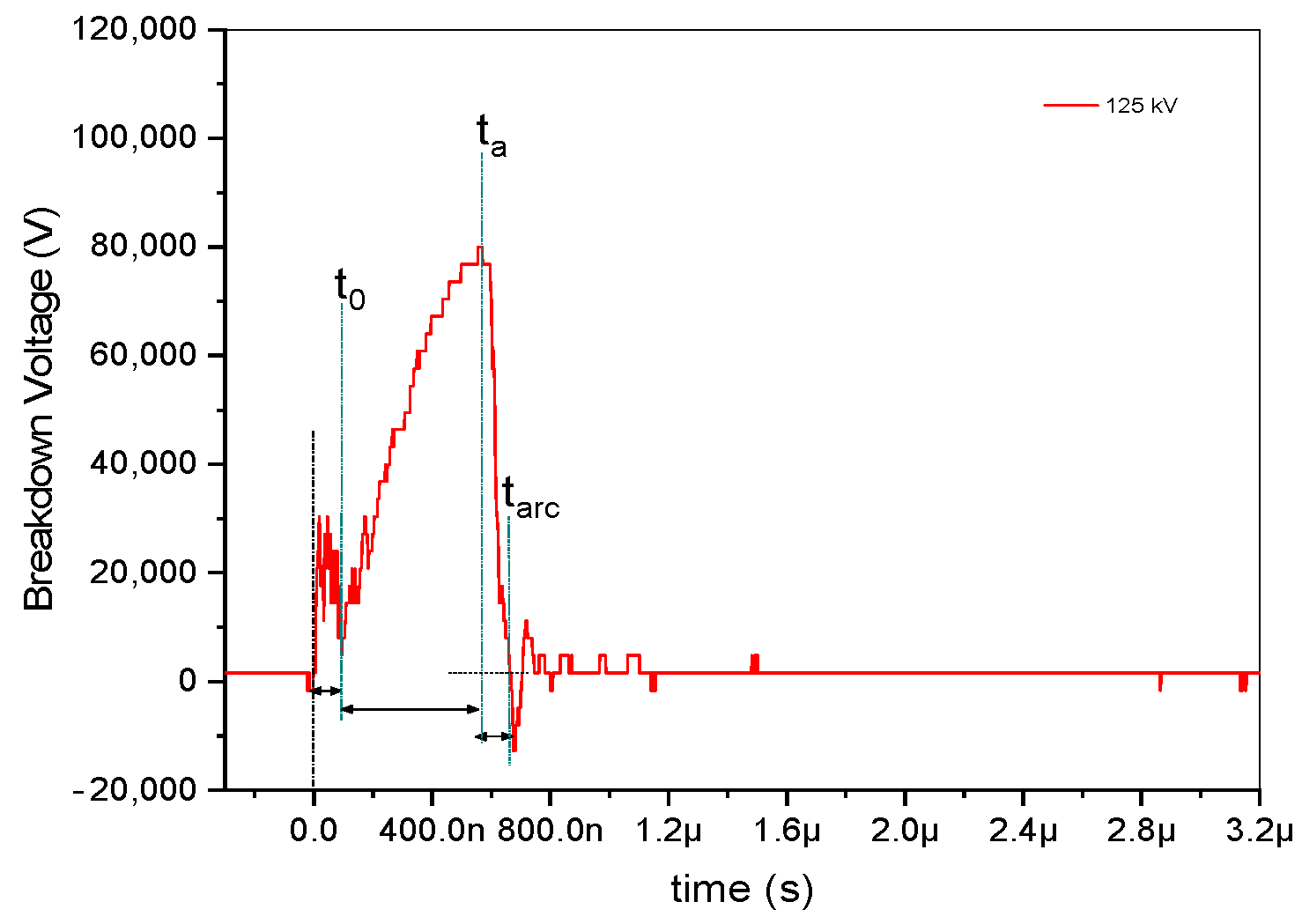


| Applied Voltage | Positive Impulse Voltages (tarc − ta) | Negative Impulse Voltages (tarc − ta) |
|---|---|---|
| ±100 kV | (0.689 − 0.587) µs = 0.101 µs = 101 ns | (2.993 − 2.772) µs = 0.221 µs = 221 ns |
| ±125 kV | (0.666 − 0.570) µs = 0.096 µs = 96 ns | (1.156 − 0.944) µs = 0.212 µs = 212 ns |
| ±150 kV | (0.570 − 0.497) µs = 0.073 µs = 73 ns | (0.914 − 0.643) µs = 0.271 µs = 271 ns |
| Peak Type | 125 kV | −125 kV | ||||
|---|---|---|---|---|---|---|
| Center Peak (nm) | Identified | Overlapped Center Peak of | Center Peak (nm) | Identified | Overlapped Center Peak of | |
| Gaussian | 310.038 | W II | - | 294.730 | W I | - |
| 313.448 | O II | - | 295.999 | W I | - | |
| 318.622 | Ar II | - | 298.720 | W II | - | |
| 319.556 | Ar II | 318.622 | 300.382 | W I | 301.515 | |
| 321.544 | W I | - | 301.515 | W II | - | |
| 325.298 | W I | - | 305.947 | W I | - | |
| 327.873 | N V | - | 310.518 | W I | - | |
| 333.968 | W II | - | 311.730 | W I | 310.518 | |
| 334.295 | W II | 333.968 | 313.794 | W I | - | |
| 337.803 | O IV | - | None | None | None | |
| 341.412 | W I | - | None | None | None | |
| 342.319 | W I | 341.412 | None | None | None | |
| 343.499 | W I | - | None | None | None | |
| 344.977 | W II | - | None | None | None | |
| 347.368 | W I | - | None | None | None | |
| 349.247 | W I | - | None | None | None | |
| 350.739 | W I | - | None | None | None | |
| 352.470 | W I | - | None | None | None | |
| 358.365 | W II | - | None | None | None | |
| 359.759 | W I | 361.753 and 358.365 | None | None | None | |
| 361.753 | W I | - | None | None | None | |
| Atoms | Wavelength (nm) | Positive Voltage: | Negative Voltage: | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Ek (eV) | ΔE (eV) | gk | Aki (s−1) | Ek (eV) | ΔE (eV) | gk | Aki (s−1) | ||
| (1) | (2) | (3) | (4) | (5) | (6) | (7) | (8) | (9) | (10) |
| O II | 241.162 | 30.801 | 5.140 | 2 | 2.05 × 107 | 30.801 | 5.140 | 2 | 2.05 × 107 |
| O II | 313.421 | 29.586 | 3.955 | 2 | 7.68 × 107 | - | - | - | No data |
| O III | 333.2406 | - | - | - | No data | 49.420 | 3.720 | 3 | 7.92 × 107 |
| O IV | 337.806 | 59.843 | 3.669 | 4 | 1.66 × 107 | - | - | - | No data |
| O IV | 338.552 | - | - | - | No data | 58.119 | 3.661 | 8 | 1.02 × 108 |
| O II | 385.238 | 31.727 | 3.217 | 6 | 2.98 × 106 | - | - | - | No data |
| O III | 408.426 | 36.893 | 3.035 | 3 | 9.02 × 104 | - | - | - | No data |
| O II | 418.545 | - | - | - | No data | 31.320 | 2.962 | 8 | 1.91 × 108 |
| O II | 424.477 | - | - | - | No data | 31.751 | 2.920 | 6 | No data |
| O II | 428.412 | - | - | - | No data | 31.750 | 2.930 | 4 | No data |
| O II | 435.359 | - | - | - | No data | 31.710 | 2.847 | 8 | No data |
| O II | 435.418 | 31.710 | 2.847 | 6 | No data | - | - | - | No data |
| O II | 444.352 | - | - | - | No data | 31.148 | 2.790 | 8 | 1.89 × 106 |
| O III | 480.978 | 36.435 | 2.577 | 3 | 4.52 × 105 | - | - | - | No data |
| O IV | 481.315 | - | - | - | No data | 61.973 | 2.616 | 6 | 8.65 × 106 |
| O III | 493.123 | 2.514 | 2.514 | 5 | 2.41 × 10−6 | 2.514 | 2.514 | 5 | 2.41 × 10−6 |
| O I | 747.724 | - | - | - | No data | 15.782 | 1.658 | 3 | No data |
| O I | 777.417 | 10.741 | 1.595 | 5 | 3.69 × 107 | 10.740 | 1.594 | 5 | 3.69 × 107 |
| O I | 795.08 | 14.100 | 1.559 | 7 | No data | 14.100 | 1.559 | 7 | No data |
| O I | 844.676 | 10.989 | 1.468 | 3 | 3.22 × 107 | 10.989 | 1.468 | 3 | 3.22 × 107 |
| Atoms | Wavelength (nm) | Intensity in the Applied Voltage of | Lifetime τk (s) | Intensity in the Applied Voltage of | ||||
|---|---|---|---|---|---|---|---|---|
| 100 kV | 125 kV | 150 kV | −100 kV | −125 kV | −150 kV | |||
| O II | 241.162 | 16,237 | 18,630 | 31,245 | 4.878 × 10−8 | 9072 | 9490 | 25,825 |
| O II | 313.421 | 45,457 | 45,041 | 46,765 | 1.302 × 10−8 | - | - | - |
| O III | 333.241 | - | - | - | 1.263 × 10−8 | - | - | 15,193 |
| O IV | 337.806 | 7430 | 19,561 | 27,246 | 6.024 × 10−8 | - | - | - |
| O IV | 338.552 | - | - | - | 9.804 × 10−9 | - | 7471 | - |
| O II | 385.238 | 6617 | 9030 | 13,130 | 3.356 × 10−7 | - | - | - |
| O III | 408.426 | 8340 | 11,200 | 16,956 | 1.109 × 10−5 | - | - | - |
| O II | 418.545 | - | - | - | 5.236 × 10−9 | 4410 | 6820 | 9991 |
| O II | 424.477 | - | - | - | No data | - | 11,888 | 17,258 |
| O II | 428.412 | - | - | - | No data | - | - | 6658 |
| O II | 435.359 | - | - | - | No data | 4161 | 6637 | 9219 |
| O II | 435.418 | 7022 | 9196 | 12,524 | No data | - | - | - |
| O II | 444.352 | - | - | - | 5.291 × 10−7 | 5851 | 7528 | 11,957 |
| O III | 480.978 | 8088 | 12,050 | 18,772 | 2.212 × 10−6 | - | - | - |
| O IV | 481.315 | - | - | - | 1.156 × 10−7 | 5978 | - | - |
| O III | 493.123 | 6548 | 9120 | 12,867 | 4.149 × 105 | 5797 | 8727 | 11,510 |
| O I | 747.724 | - | - | - | No data | 3979 | 5465 | 8258 |
| O I | 777.417 | 12,631 | 18,356 | 23,929 | 2.710 × 10−8 | 12,477 | 17,563 | 26,413 |
| O I | 795.08 | 1440 | 2112 | 2622 | No data | 1028 | 1569 | 2834 |
| O I | 844.676 | 1511 | 2406 | 2868 | 3.106 × 10−8 | 2022 | 3368 | 3986 |
| Atoms | Wavelength (nm) | Excitation Temperature (K) of | Excitation Temperature (K) of | Decay Time (s) | ||||
|---|---|---|---|---|---|---|---|---|
| 100 kV | 125 kV | 150 kV | −100 kV | −125 kV | −150 kV | |||
| O II | 241.162 | 10,572 | 10,529 | 10,371 | 10,757 | 10,743 | 10,429 | 4.878 × 10−8 |
| O II | 313.421 | 10,164 | 10,166 | 10,155 | - | - | - | 1.302 × 10−8 |
| O III | 333.241 | - | - | - | - | - | 17,750 | 1.263 × 10−8 |
| O IV | 337.806 | 21,119 | 20,515 | 20,316 | 6.024 × 10−8 | |||
| O IV | 338.552 | - | - | - | - | 22,199 | - | 9.804 × 10−9 |
| O II | 385.238 | 10,762 | 10,665 | 10,551 | - | - | - | 3.356 × 10−7 |
| O III | 408.426 | 11,065 | 10,982 | 10,866 | - | - | - | 1.109 × 10−5 |
| O II | 418.545 | - | - | - | 12,346 | 12,166 | 12,012 | 5.236 × 10−9 |
| O II | 444.352 | - | - | - | 10,698 | 10,620 | 10,480 | 5.291 × 10−7 |
| O III | 480.978 | 11,362 | 11,242 | 11,111 | - | - | - | 2.212 × 10−6 |
| O IV | 481.315 | - | - | - | 21,619 | - | - | 1.156 × 10−7 |
| O III | 493.123 | 467 | 464 | 462 | 468 | 465 | 463 | 4.149 × 105 |
| O I | 777.417 | 3752 | 3710 | 3681 | 3753 | 3714 | 3670 | 2.710 × 10−8 |
| O I | 844.676 | 4006 | 3948 | 3927 | 3970 | 3908 | 3888 | 3.106 × 10−8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ikhwanus, M.; Morimoto, T. Rapid Breakdown Time in Positive Impulse Voltages through Spectroscopy Analysis. Energies 2024, 17, 705. https://doi.org/10.3390/en17030705
Ikhwanus M, Morimoto T. Rapid Breakdown Time in Positive Impulse Voltages through Spectroscopy Analysis. Energies. 2024; 17(3):705. https://doi.org/10.3390/en17030705
Chicago/Turabian StyleIkhwanus, Muhammad, and Takeshi Morimoto. 2024. "Rapid Breakdown Time in Positive Impulse Voltages through Spectroscopy Analysis" Energies 17, no. 3: 705. https://doi.org/10.3390/en17030705
APA StyleIkhwanus, M., & Morimoto, T. (2024). Rapid Breakdown Time in Positive Impulse Voltages through Spectroscopy Analysis. Energies, 17(3), 705. https://doi.org/10.3390/en17030705






