Two-Dimensional Analysis of Absorber Plates in Solar Collectors with a Nonlinear Plate Temperature at the Tube Section
Abstract
1. Introduction
2. Mathematical Model
- Heat transfer in absorber plates satisfies two-dimensional flow under a steady state.
- For simplicity, the analysis omits the transparent covers.
- The solar insolation distributes uniformly over the plate and remains unchanged.
- The plate material’s thermal conductivity is invariable.
- There is no change in the surrounding temperature and overall loss coefficient.
- At the plate and tube junction, the plate temperature varies nonlinearly as a quadratic function.
2.1. Analytical Methodology
2.2. Design Parameters
2.3. Numerical Analysis
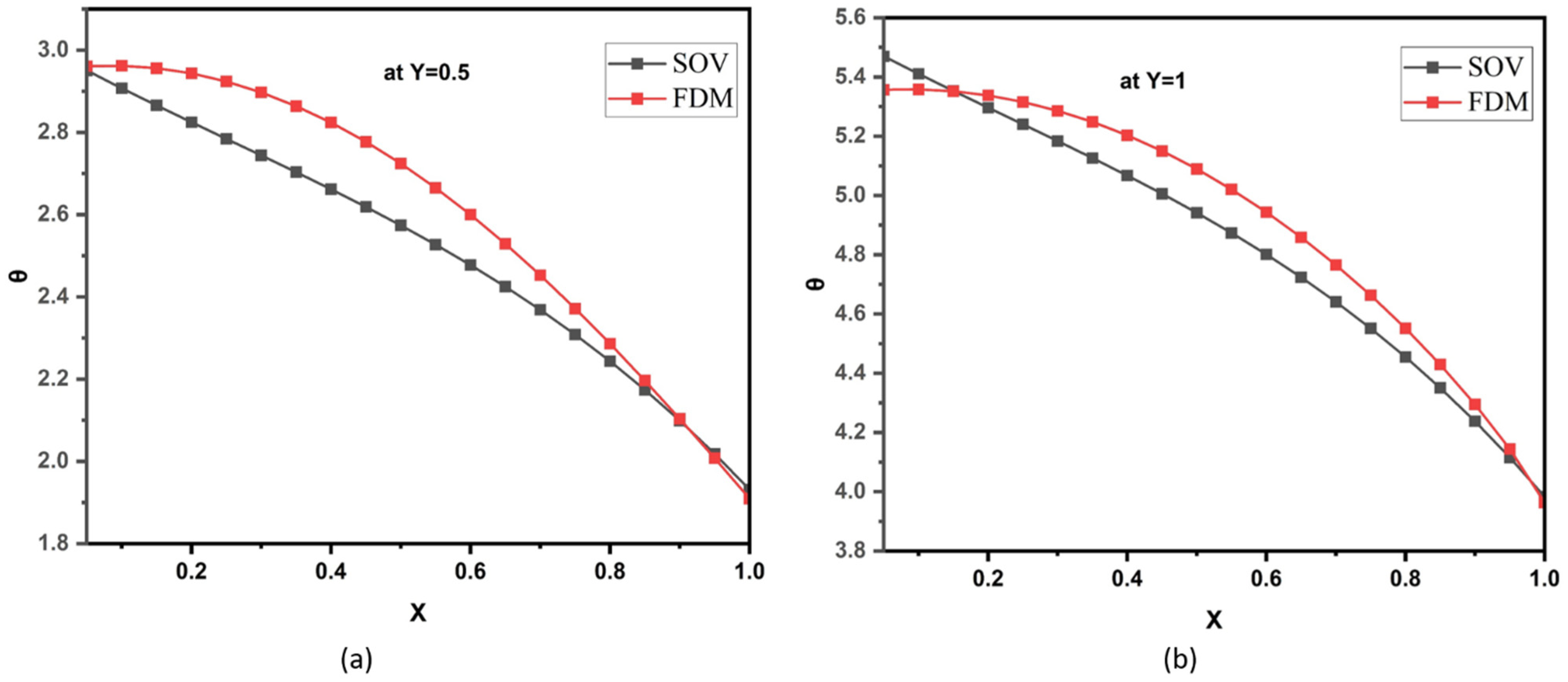
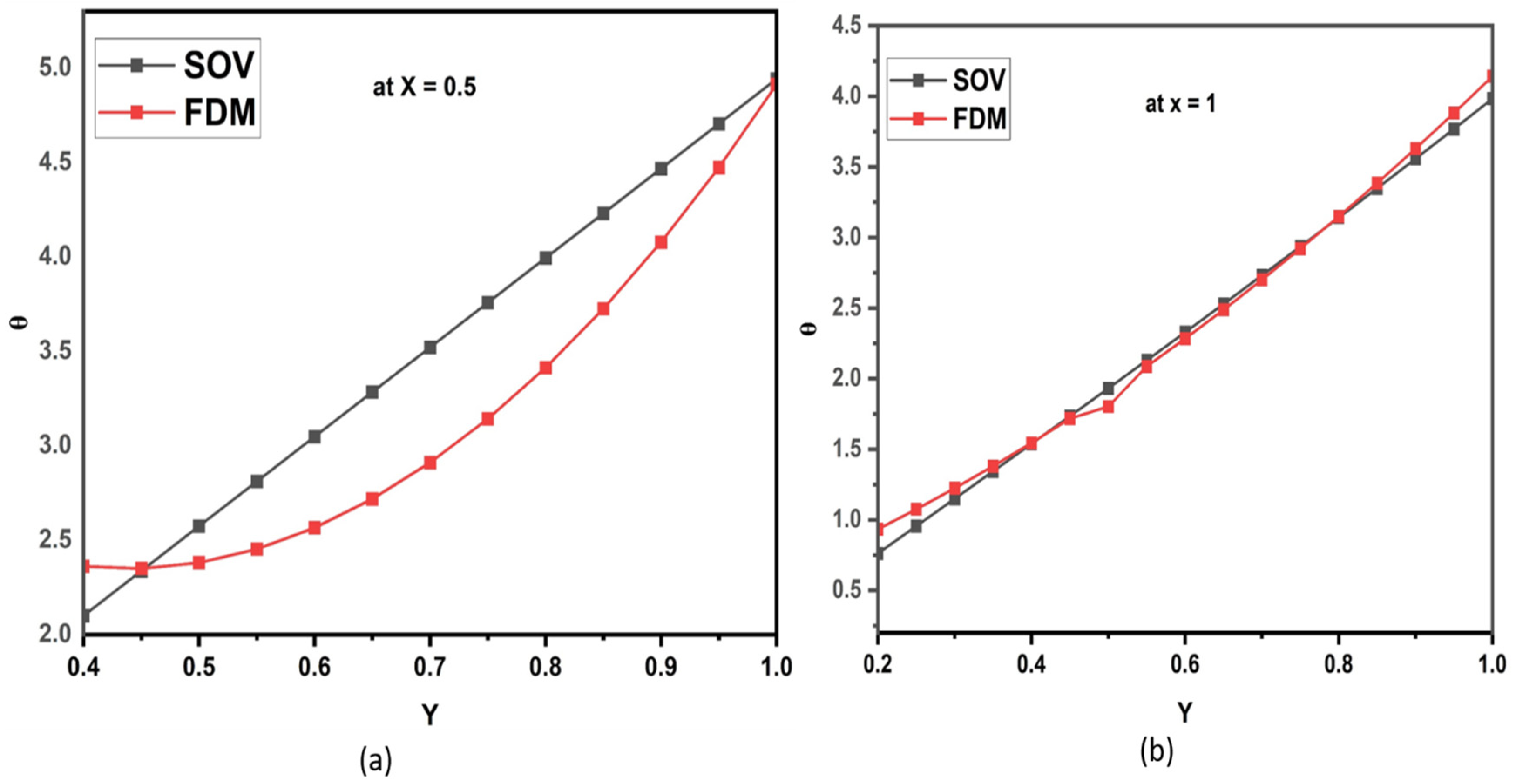
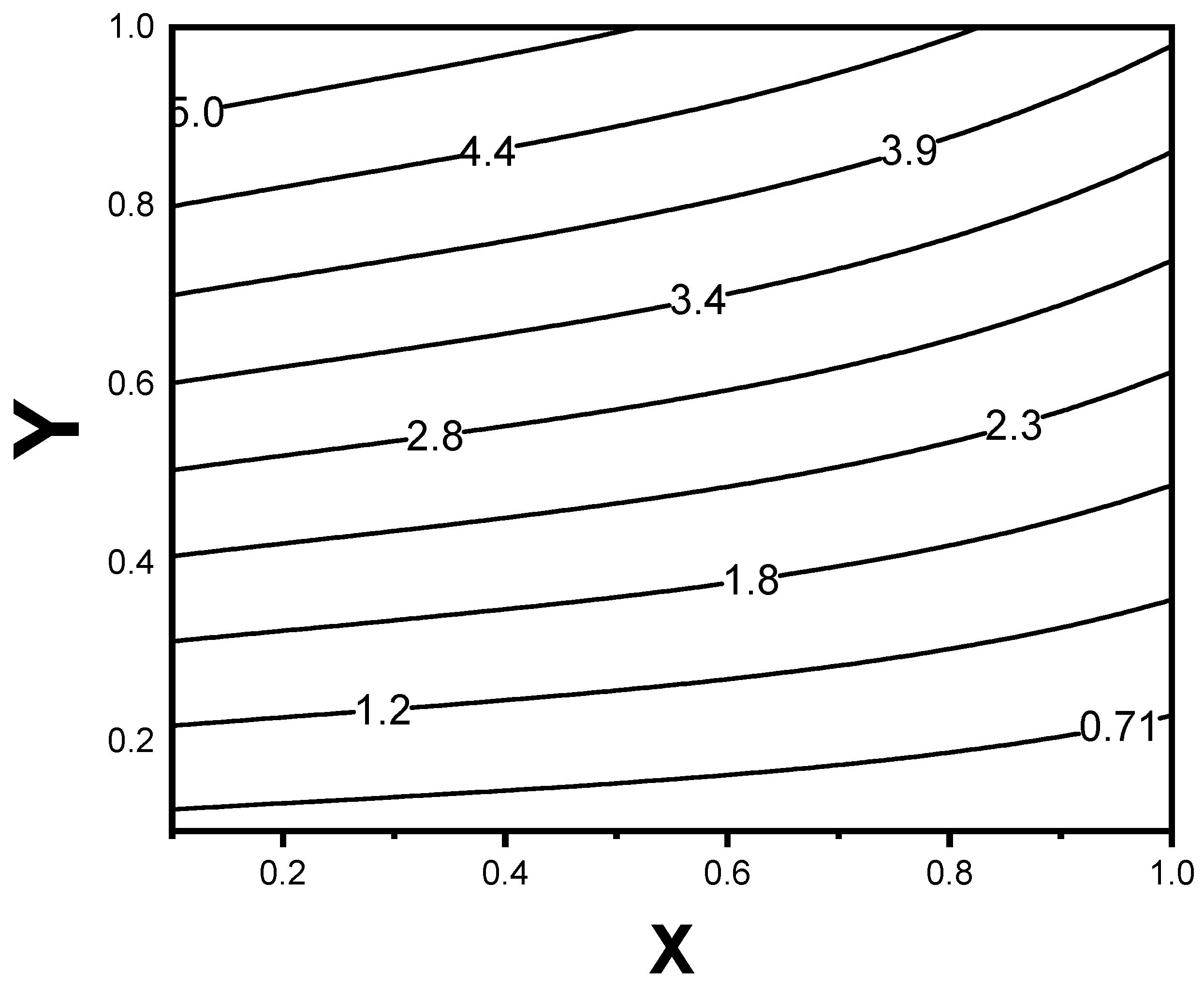
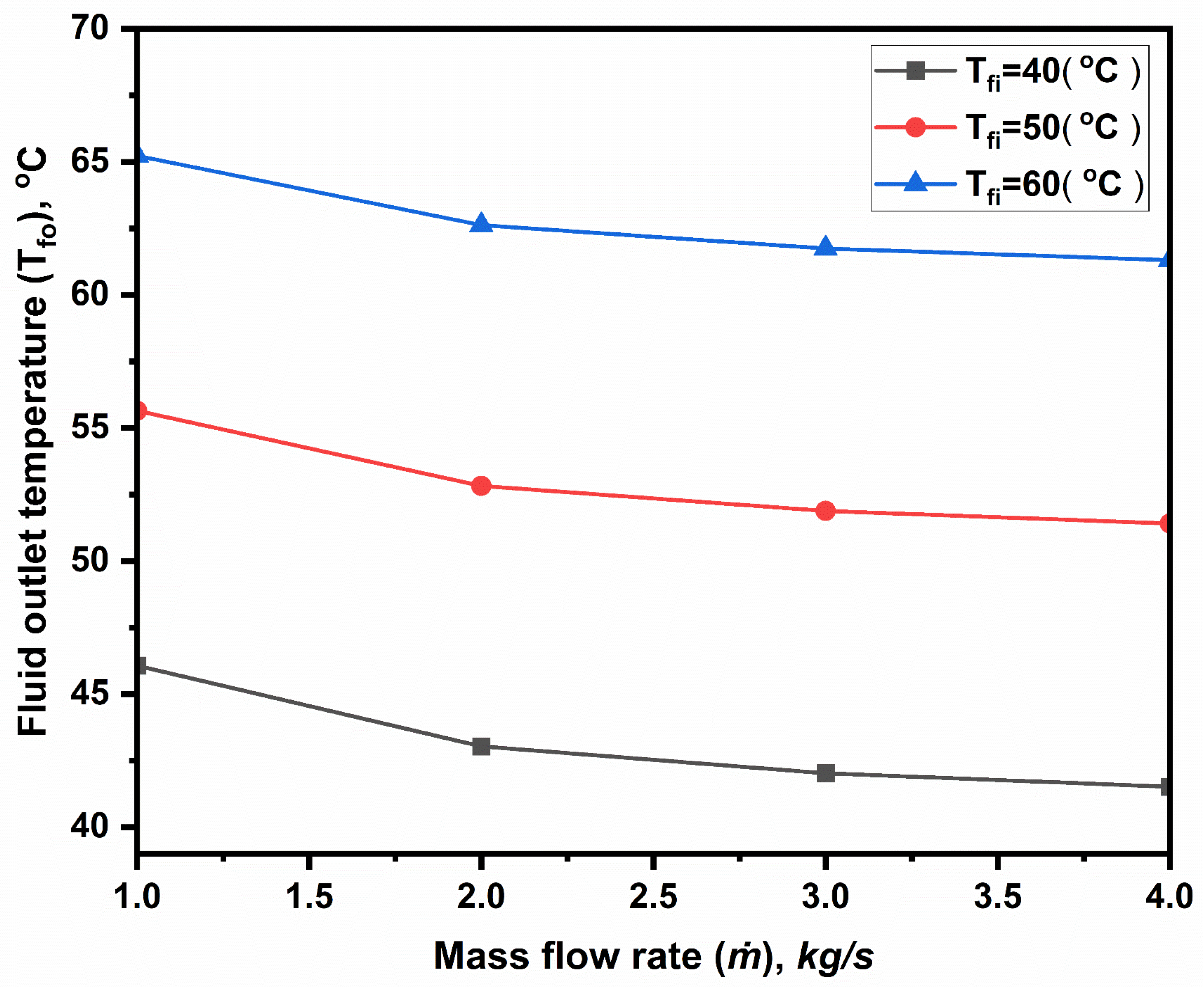
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| dimensionless constant | |
| dimensionless constant | |
| normalized constant | |
| fluid-carrying tube length (m) | |
| half-pitch length between two tubes (m) | |
| Tube outer diameter(m) | |
| Tube inner diameter(m) | |
| heat conductivity (W m−1 K−1) | |
| convective coefficient at tube inner surface | |
| Mass transfer rate in the tube [kg/s] | |
| Biot number | |
| normalized thermo-geometric parameter | |
| normalized flux | |
| absorbed sun energy flux (W m−2) | |
| absorber temperature (local) (°C) | |
| ambient temperature (°C) | |
| absorber thickness (m) | |
| overall energy loss coefficient (W m−2 K−1) | |
| normalized coordinate, | |
| cartesin coordinate (m) | |
| normalized coordinate, | |
| cartesian -coordinate (m) | |
| thermo-geometric parameter | |
| efficiency factor | |
| normalized heat removal parameter | |
| collector fluid inlet temperature (°C) | |
| collector fluid outlet temperature (°C) | |
| absorber plate area (m2) | |
| collector gross area (m2) | |
| collector fluid specific heat (W kg−1 K−1) | |
| useful energy gain (W) | |
| sun radiation intensity (W/m2) | |
| Greek Symbols | |
| Width to length ratio of absorber plate | |
| dimensionless temperature | |
| plate efficiency factor | |
| Eigen constant | |
| collector efficiency | |
| transmissivity | |
| absorber plate absorptivity | |
| Subscripts | |
| inlet condition | |
References
- Kumar, L.; Hasanuzzaman, M.; Rahim, N.A. Global advancement of solar thermal energy technologies for industrial process heat and its future prospects: A review. Energy Convers. Manag. 2019, 195, 885–908. [Google Scholar] [CrossRef]
- Estevão, J.; Lopes, J.D. SDG7 and renewable energy consumption: The influence of energy sources. Technol. Forecast. Soc. Chang. 2024, 198, 123004. [Google Scholar] [CrossRef]
- Mahakud, J.; Kundu, B. Trapezoidal Approach to Establish One-Dimensional Analysis of an Absorber Plate for Two-Dimensional Heat Flow. In Advances in Mechanical Engineering: Select Proceedings of ICRIDME 2018; Springer: Singapore, 2020; pp. 1361–1372. [Google Scholar]
- Kundu, B.; Mondal, P.K.; Datta, S.P.; Wongwises, S. Operating design conditions of a solar-powered vapor absorption cooling system with an absorber plate having different profiles: An analytical study. Int. Commun. Heat Mass Transf. 2010, 37, 1238–1245. [Google Scholar] [CrossRef]
- Sukhatme, S.P.; Nayak, J. Solar Energy Principles of Thermal Collection and Storage, 3rd ed.; McGraw-Hill Education: New York, NY, USA, 2010. [Google Scholar]
- Hottel, H.C.; Woertz, B.S. The performance of flat plate solar heat collectors. Trans. Am. Soc. Mech. Eng. 1942, 64, 91–104. [Google Scholar] [CrossRef]
- Hottel, H.C.; Whillier, A. Evaluation of Flat-Plate Collector Performance. In Transactions of the Conference on the Use of Solar Energy; University of Arizona Press: Tuscan, AZ, USA, 1958; Volume 2, p. 74. [Google Scholar]
- Bliss, R.W. The Derivations of Several Plate-Efficiency Factors Useful in the Design of Flat-Plate Solar Heat Collectors. Sol. Energy 1959, 3, 55–69. [Google Scholar] [CrossRef]
- Duffie, J.A.; Beckman, W.A. Solar Energy Thermal Processes; Wiley: New York, NY, USA, 1974. [Google Scholar]
- Kundu, B. Performance analysis and optimization of absorber plates of different geometry for a flat-plate solar collector: A comparative study. Appl. Therm. Eng. 2002, 22, 999–1012. [Google Scholar] [CrossRef]
- Kundu, B. The influence of collector fluid inlet temperature on the performance of a solar-assisted absorption system using step-finned flat-plate collector. Heat Transf. Eng. 2007, 28, 496–505. [Google Scholar] [CrossRef]
- Kundu, B. Performance and optimum design analysis of an absorber plate fin using recto-trapezoidal profile. Sol. Energy 2008, 82, 22–32. [Google Scholar] [CrossRef]
- Hollands, K.G.T.; Stedman, B.A. Optimization of an absorber plate fin having a step change in local thickness. Sol. Energy 1992, 49, 493–495. [Google Scholar] [CrossRef]
- Kundu, B. Analytic method for thermal performance and optimization of an absorber plate fin having variable thermal conductivity and overall loss coefficient. Appl. Energy 2010, 87, 2243–2255. [Google Scholar] [CrossRef]
- Nayak, J.K.; Amer, E.H. Experimental and theoretical evaluation of dynamic test procedures for solar flat-plate collectors. Sol. Energy 2000, 69, 377–401. [Google Scholar] [CrossRef]
- Kalogirou, S.A. Prediction of flat-plate collector performance parameters using artificial neural networks. Sol. Energy 2006, 80, 248–259. [Google Scholar] [CrossRef]
- Mausam, K.; Singh, S.; Ghosh, S.K.; Singh, R.P. Thermal performance modelling of solar flat plate parallel tube collector using ANN. Energy 2024, 303, 131940. [Google Scholar] [CrossRef]
- Mohseni-Gharyehsafa, B.; Esfahani, J.A.; Kim, K.C.; Ouerdane, H. Soft computing analysis of thermohydraulic enhancement using twisted tapes in a flat-plate solar collector: Sensitivity analysis and multi-objective optimization. J. Clean. Prod. 2021, 314, 127947. [Google Scholar] [CrossRef]
- Alawi, O.A.; Kamar, H.M.; Salih, S.Q.; Abba, S.I.; Ahmed, W.; Homod, R.Z.; Jamei, M.; Shafik, S.S.; Yaseen, Z.M. Development of optimized machine learning models for predicting flat plate solar collectors thermal efficiency associated with Al2O3-water nanofluids. Eng. Appl. Artif. Intell. 2024, 133, 108158. [Google Scholar] [CrossRef]
- Filipović, P.; Dović, D.; Horvat, I.; Ranilović, B. Evaluation of a novel polymer solar collector using numerical and experimental methods. Energy 2023, 284, 128558. [Google Scholar] [CrossRef]
- Bao, Y.; Chen, J.; Shao, S. Comparison on thermal and hydraulic performances of transverse mini-channel based on a novel comprehensive evaluation factor. Appl. Therm. Eng. 2024, 253, 123795. [Google Scholar] [CrossRef]
- Bharathiraja, R.; Ramkumar, T.; Karthick, L.; Mohanraj, M. Performance investigation on flat plate solar water collector using a hybrid nano-enhanced phase change material (PCM). J. Energy Storage 2024, 86, 111163. [Google Scholar] [CrossRef]
- Bhowmik, H.; Amin, R. Efficiency improvement of flat plate solar collector using reflector. Energy Rep. 2017, 3, 119–123. [Google Scholar] [CrossRef]
- Garcia, R.P.; del Rio Oliveira, S.; Scalon, V.L. Thermal efficiency experimental evaluation of solar flat plate collectors when introducing convective barriers. Sol. Energy 2019, 182, 278–285. [Google Scholar] [CrossRef]
- Zheng, J.; Febrer, R.; Castro, J.; Kizildag, D.; Rigola, J. A new high-performance flat plate solar collector. Numerical modelling and experimental validation. Appl. Energy 2024, 355, 122221. [Google Scholar] [CrossRef]
- He, Y.; Yu, H.; Duan, G.; Wang, Y.; Yang, Q.; Feng, L.; Zhang, J. Thermal performance and experimental analysis of stainless steel flat plate solar collector with full-flow channels. Heliyon 2024, 10, e28255. [Google Scholar] [CrossRef] [PubMed]
- Verma, S.K.; Tiwari, A.K. Progress of nanofluid application in solar collectors: A review. Energy Convers. Manag. 2015, 100, 324–346. [Google Scholar] [CrossRef]
- Elcioglu, E.B.; Genc, A.M.; Karadeniz, Z.H.; Ezan, M.A.; Turgut, A. Nanofluid figure-of-merits to assess thermal efficiency of a flat plate solar collector. Energy Convers. Manag. 2020, 204, 112292. [Google Scholar] [CrossRef]
- Akram, N.; Montazer, E.; Kazi, S.N.; Soudagar, M.E.M.; Ahmed, W.; Zubir, M.N.M.; Afzal, A.; Muhammad, M.R.; Ali, H.M.; Márquez, F.P.G.; et al. Experimental investigations of the performance of a flat-plate solar collector using carbon and metal oxides based nanofluids. Energy 2021, 227, 120452. [Google Scholar] [CrossRef]
- Ajeena, A.M.; Víg, P.; Farkas, I. A comprehensive analysis of nanofluids and their practical applications for flat plate solar collectors: Fundamentals, thermophysical properties, stability, and difficulties. Energy Rep. 2022, 8, 4461–4490. [Google Scholar] [CrossRef]
- Demirdelen, T.; Alıcı, H.; Esenboğa, B.; Güldürek, M. Performance and economic analysis of designed different solar tracking systems for Mediterranean climate. Energies 2023, 16, 4197. [Google Scholar] [CrossRef]
- Desisa, T.R. Experimental and numerical investigation of heat transfer characteristics in solar flat plate collector using nanofluids. Int. J. Thermofluids 2023, 18, 100325. [Google Scholar] [CrossRef]
- Zima, W.; Mika, Ł.; Sztekler, K. Numerical and Experimental Determination of Selected Performance Indicators of the Liquid Flat-Plate Solar Collector under Outdoor Conditions. Energies 2024, 17, 3454. [Google Scholar] [CrossRef]
- Kundu, B.; Lee, K.S. Fourier and non-Fourier heat conduction analysis in the absorber plates of a flat-plate solar collector. Sol. Energy 2012, 86, 3030–3039. [Google Scholar] [CrossRef]
- Kalogirou, S.A.; Karellas, S.; Braimakis, K.; Stanciu, C.; Badescu, V. Exergy analysis of solar thermal collectors and processes. Prog. Energy Combust. Sci. 2016, 56, 106–137. [Google Scholar] [CrossRef]
- Mostafizur, R.M.; Rasul, M.G.; Nabi, M.N. Energy and exergy analyses of a flat plate solar collector using various nanofluids: An analytical approach. Energies 2021, 14, 4305. [Google Scholar] [CrossRef]
- Wciślik, S.; Taler, D. Economic and Exergy Analysis of TiO2 + SiO2 Ethylene-Glycol-Based Hybrid Nanofluid in Plate Heat Exchange System of Solar Installation. Energies 2024, 17, 3107. [Google Scholar] [CrossRef]
- Rao, P.P.; Francis, J.E.; Love, T.J. Two-dimensional analysis of a flat-plate solar collector. J. Energy 1977, 1, 324–328. [Google Scholar] [CrossRef]
- Lund, K.O. General thermal analysis of parallel-flow flat-plate solar collector absorbers. Sol. Energy 1986, 5, 443. [Google Scholar] [CrossRef]
- Nag, A.; Misra, D.; De, K.E.; Bhattacharya, A.; Saha, S.K. Study of parallel flow flat plate solar collector using finite element method. In Numerical Methods in Thermal Problems, Proceedings of the 6th International Conference, Swansea, UK, 3–7 July 1989; The College, Swansea University: Swansea, UK, 1989. [Google Scholar]
- Kazeminejad, H. Numerical analysis of two dimensional parallel flow flat-plate solar collector. Renew. Energy 2002, 26, 2309–2323. [Google Scholar] [CrossRef]
- Cerón, J.F.; Pérez-García, J.; Solano, J.P.; García, A.; Herrero-Martín, R. A coupled numerical model for tube-on-sheet flat-plate solar liquid collectors. Analysis and validation of the heat transfer mechanisms. Appl. Energy 2015, 140, 275–287. [Google Scholar] [CrossRef]
- Quitiaquez, W.; Estupiñán-Campos, J.; Nieto-Londoño, C.; Quitiaquez, P. CFD Analysis of Heat Transfer Enhancement in a Flat-Plate Solar Collector/Evaporator with Different Geometric Variations in the Cross Section. Energies 2023, 16, 5755. [Google Scholar] [CrossRef]
- García-Guendulain, J.M.; Riesco-Avila, J.M.; Elizalde-Blancas, F.; Belman-Flores, J.M.; Serrano-Arellano, J. Numerical study on the effect of distribution plates in the manifolds on the flow distribution and thermal performance of a flat plate solar collector. Energies 2018, 11, 1077. [Google Scholar] [CrossRef]
- Kundu, B.; Das, P.K. Optimum dimensions of plate fins for fin-tube heat exchangers. Int. J. Heat Fluid Flow 1997, 18, 530–537. [Google Scholar] [CrossRef]
- Kundu, B.; Lee, K.S. A non-Fourier analysis for transmitting heat in fins with internal heat generation. Int. J. Heat Mass Transf. 2013, 64, 1153–1162. [Google Scholar] [CrossRef]
- Patankar, S.V. Numerical Heat Transfer and Fluid Flow; Hemisphere Publishing, Taylor and Francis Group: New York, NY, USA, 1980. [Google Scholar]


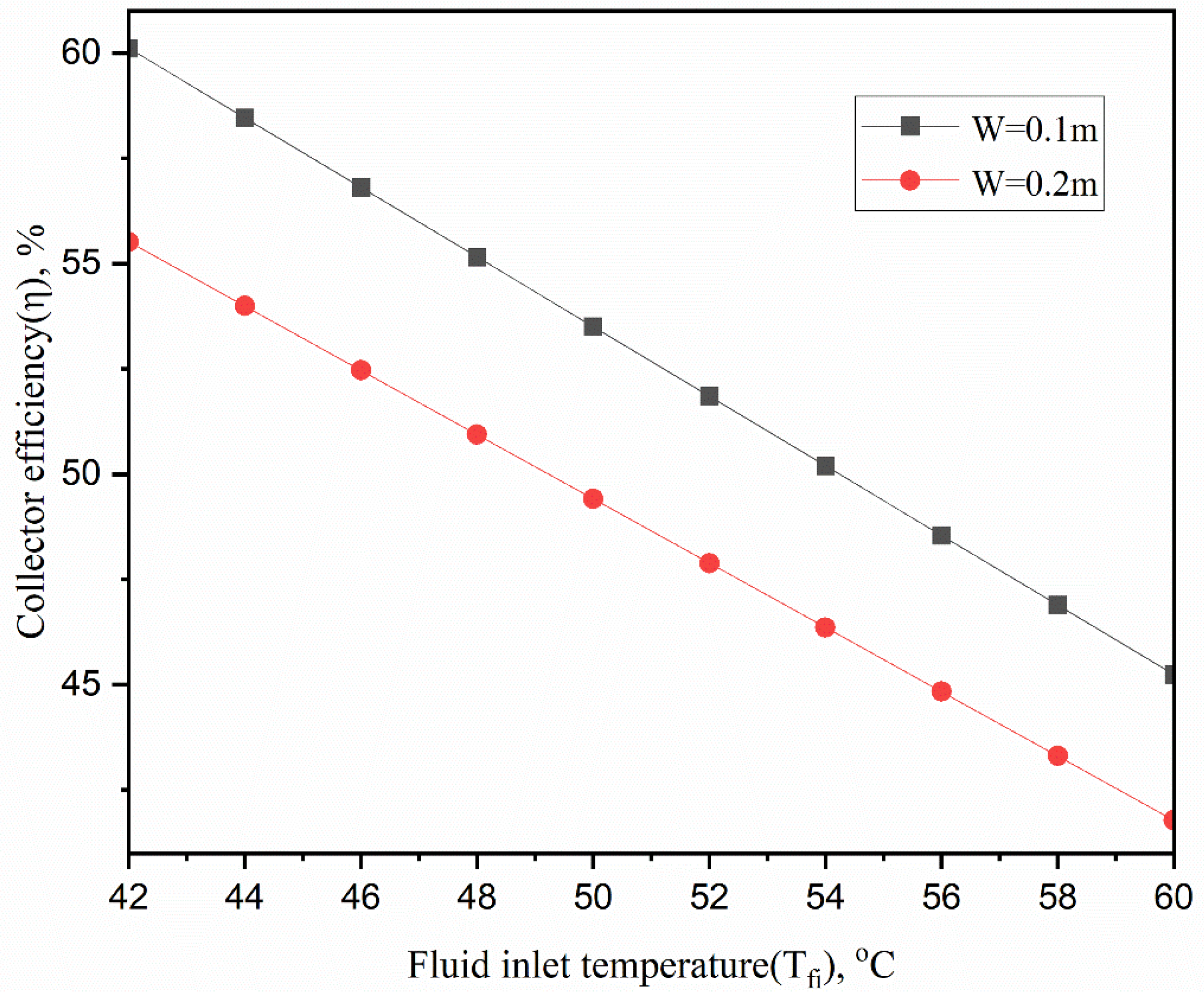
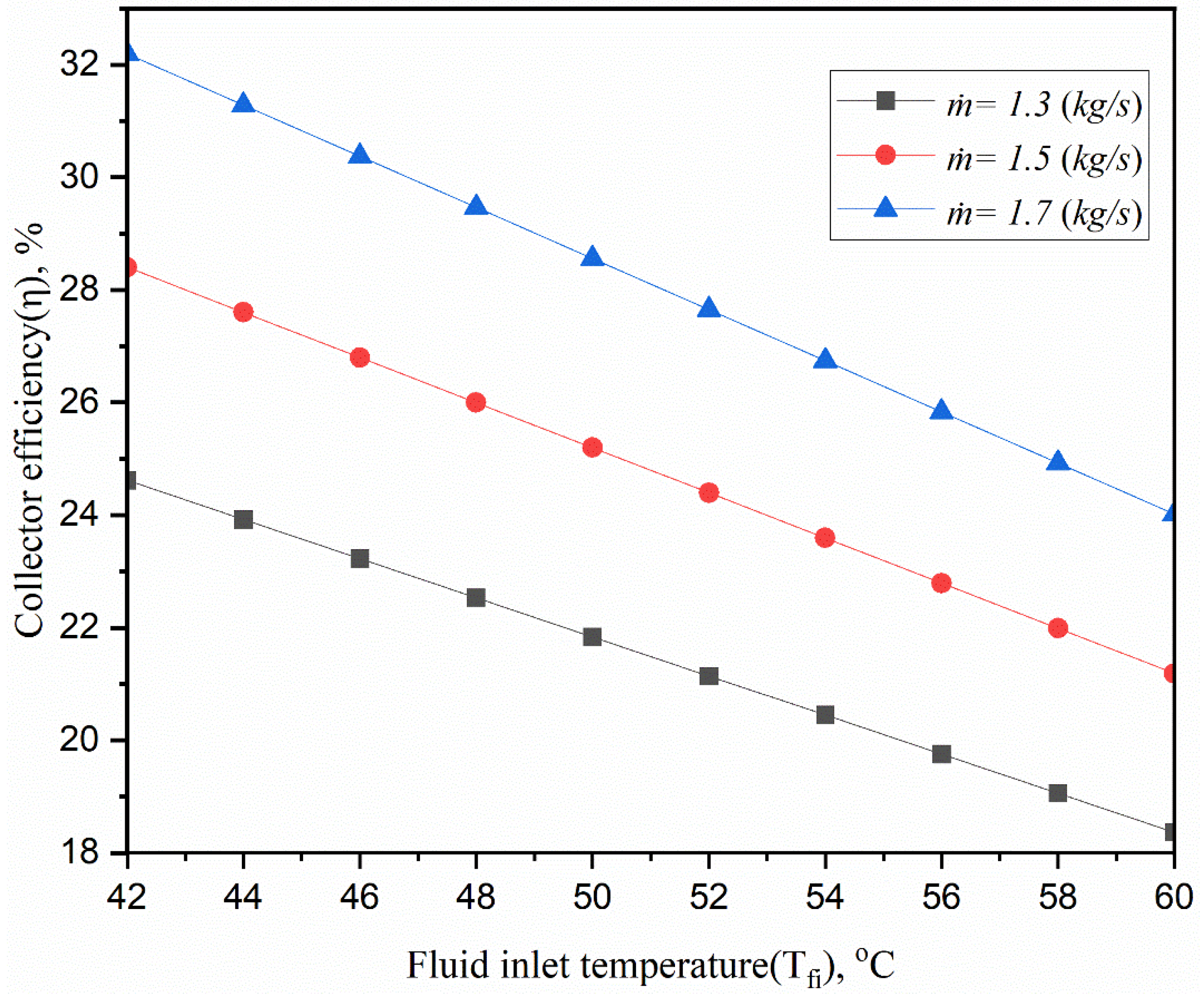
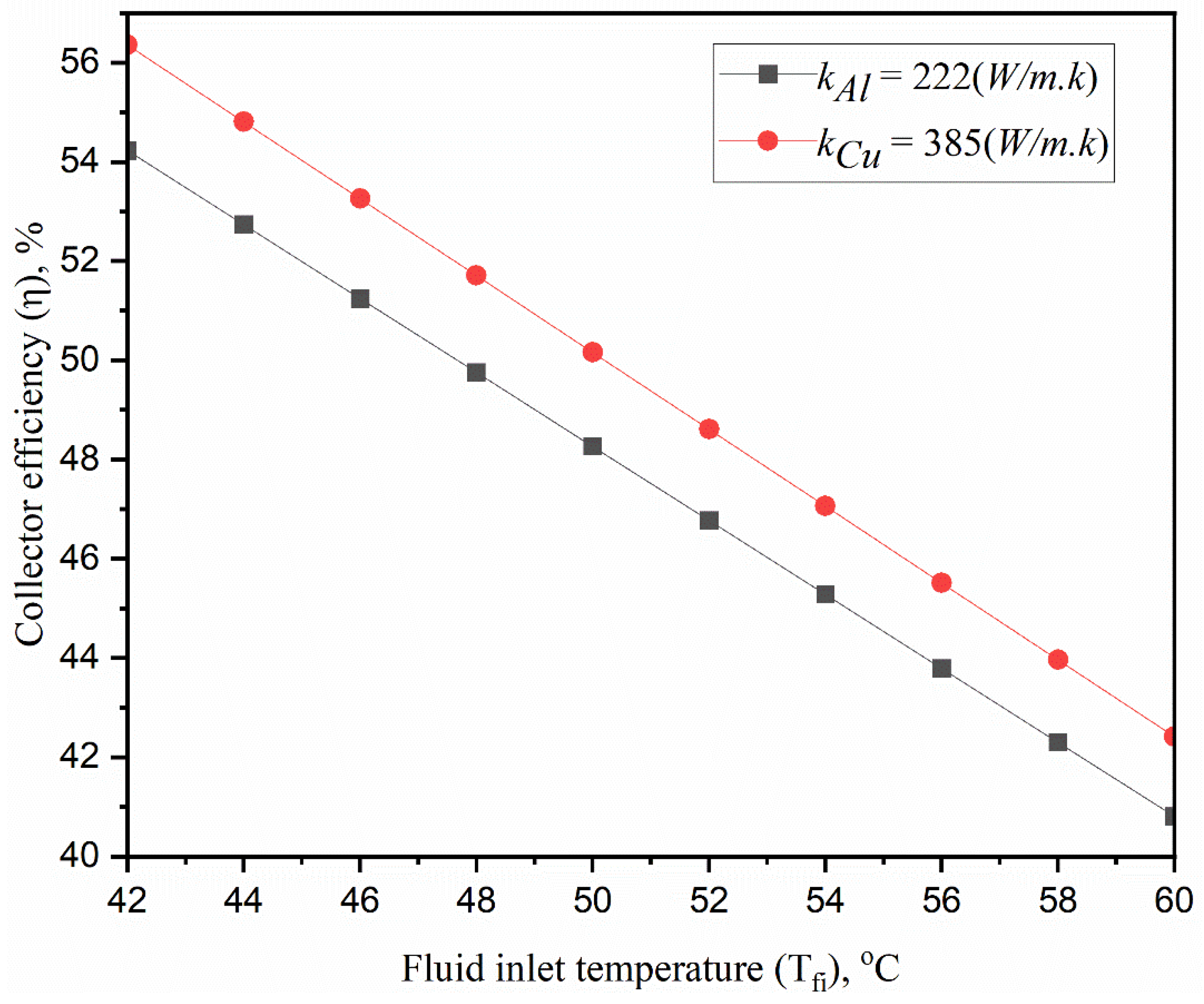
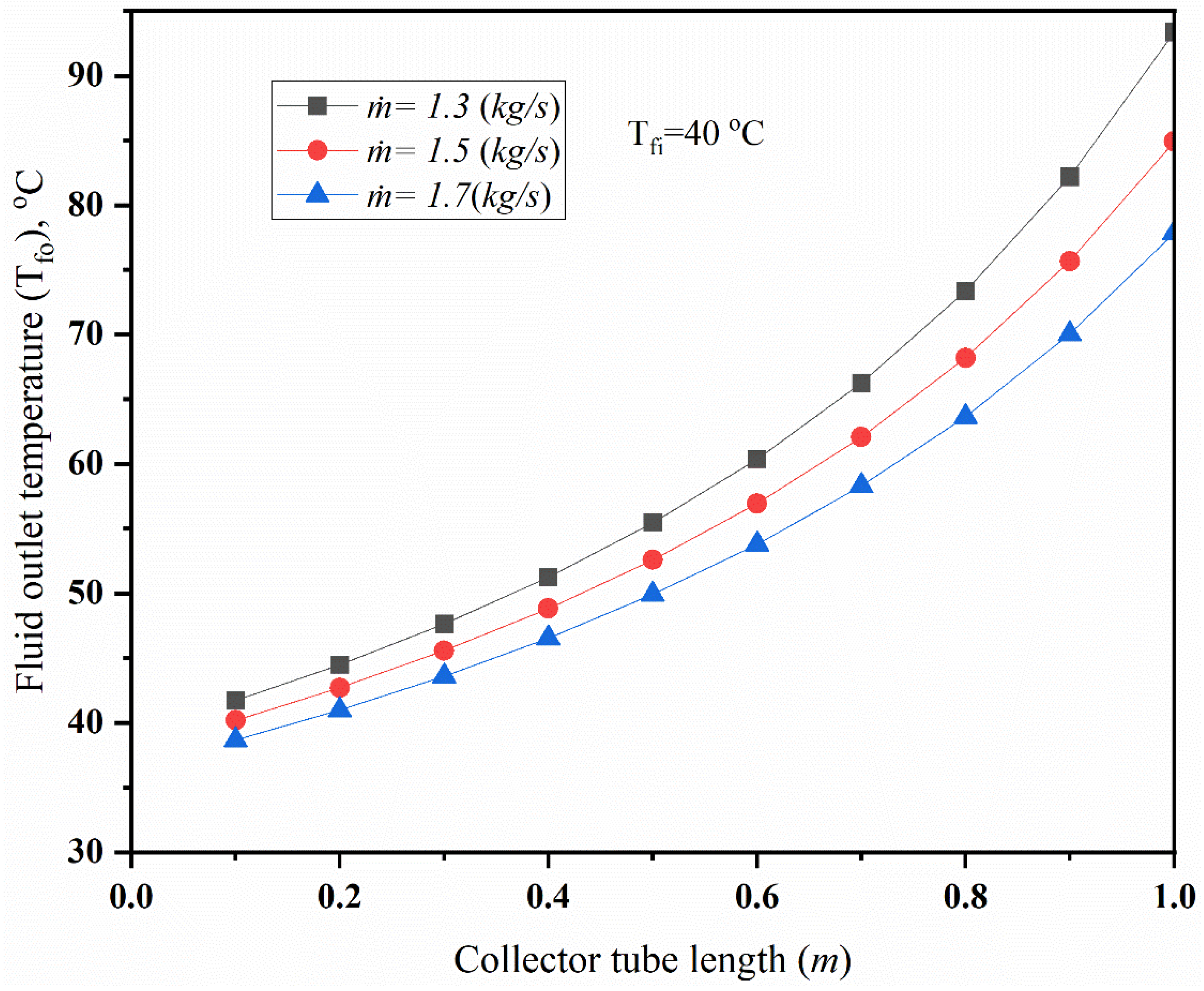
| Notation | Parameters | Values |
|---|---|---|
| Absorber plate length [m] | 2 m | |
| Plate width [m] | 0.1 m | |
| Solar irradiation absorbed by plate [w/m2] | 718 w/m2 | |
| Ambient temperature [°C] | 35 °C | |
| Thermal conductivity of plate material (Cu) [W/m·K] | 380 W/m·K | |
| Fluid inlet temperature [°C] | 40 °C | |
| Collector area [m2] | 1 m2 | |
| Fluid specific heat [kJ/kg·K] | 4.187 kJ/kg·K | |
| Convection heat transfer coefficient, tube to fluid [W/m2·K] | 205 W/m2·K | |
| Overall heat loss coefficient [W/m2·K] | 8 W/m2·K | |
| Tube internal diameter [m] | 0.01 m | |
| Tube external diameter [m] | 0.015 m |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mahakud, J.; Kundu, B. Two-Dimensional Analysis of Absorber Plates in Solar Collectors with a Nonlinear Plate Temperature at the Tube Section. Energies 2024, 17, 5979. https://doi.org/10.3390/en17235979
Mahakud J, Kundu B. Two-Dimensional Analysis of Absorber Plates in Solar Collectors with a Nonlinear Plate Temperature at the Tube Section. Energies. 2024; 17(23):5979. https://doi.org/10.3390/en17235979
Chicago/Turabian StyleMahakud, Jayanarayan, and Balaram Kundu. 2024. "Two-Dimensional Analysis of Absorber Plates in Solar Collectors with a Nonlinear Plate Temperature at the Tube Section" Energies 17, no. 23: 5979. https://doi.org/10.3390/en17235979
APA StyleMahakud, J., & Kundu, B. (2024). Two-Dimensional Analysis of Absorber Plates in Solar Collectors with a Nonlinear Plate Temperature at the Tube Section. Energies, 17(23), 5979. https://doi.org/10.3390/en17235979







