Active Support Pre-Synchronization Control and Stability Analysis Based on the Third-Order Model of Synchronous Machine
Abstract
1. Introduction
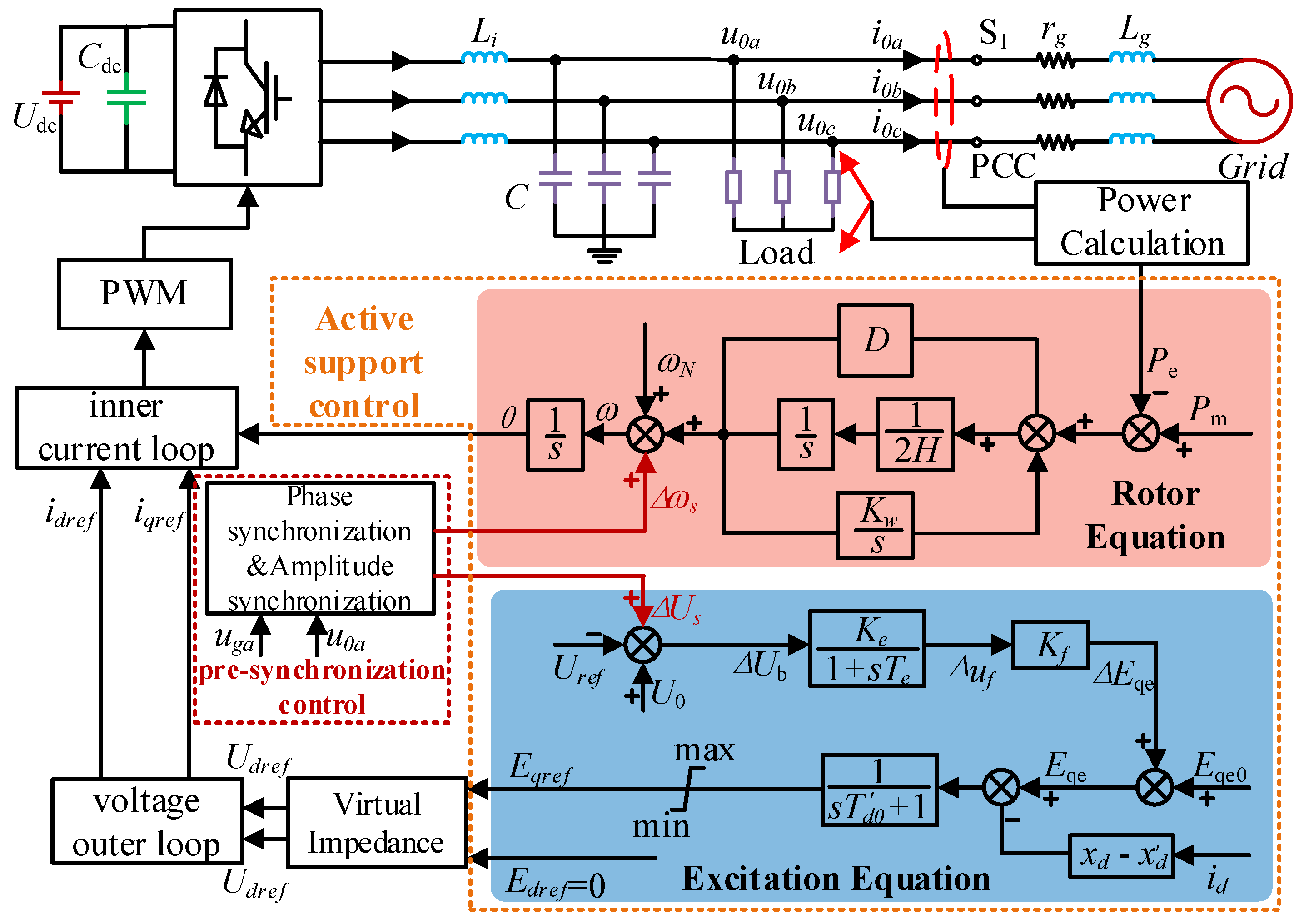
2. Mathematical Model and Control Strategy of Active Support
2.1. Rotor Motion Equations
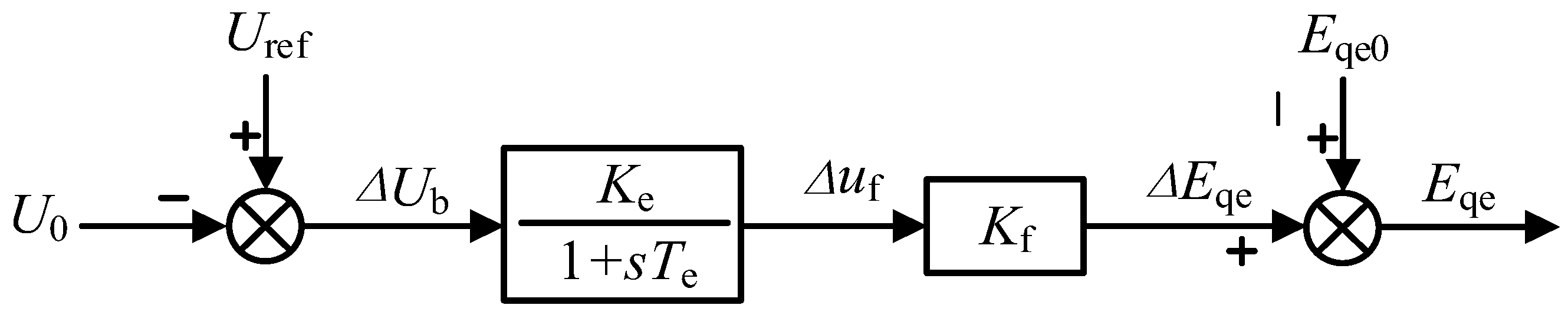
2.2. Excitation Controller
3. Analysis of Active Support Grid-Connected Mode
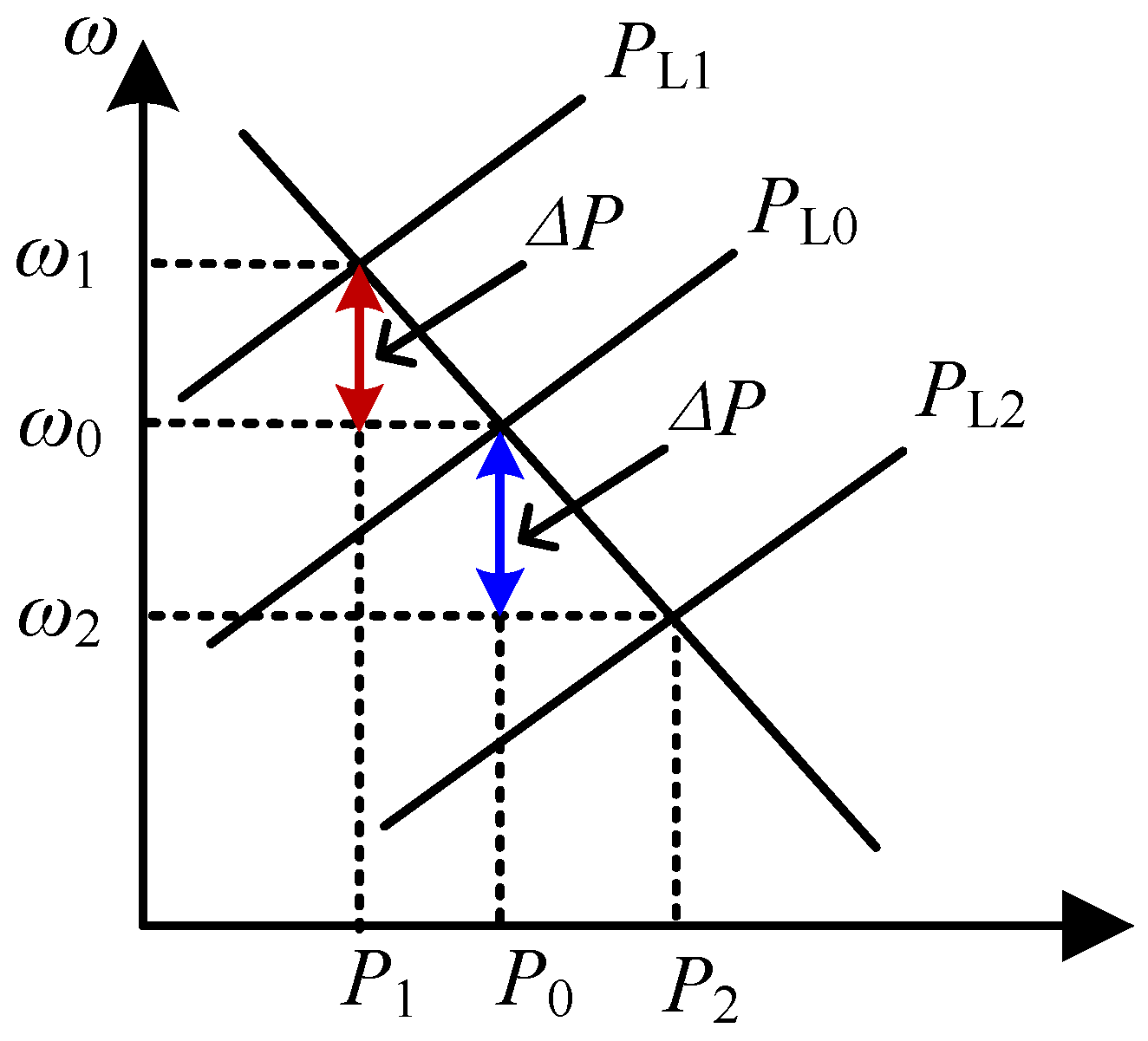
3.1. Off-Grid Mode Frequency Regulation
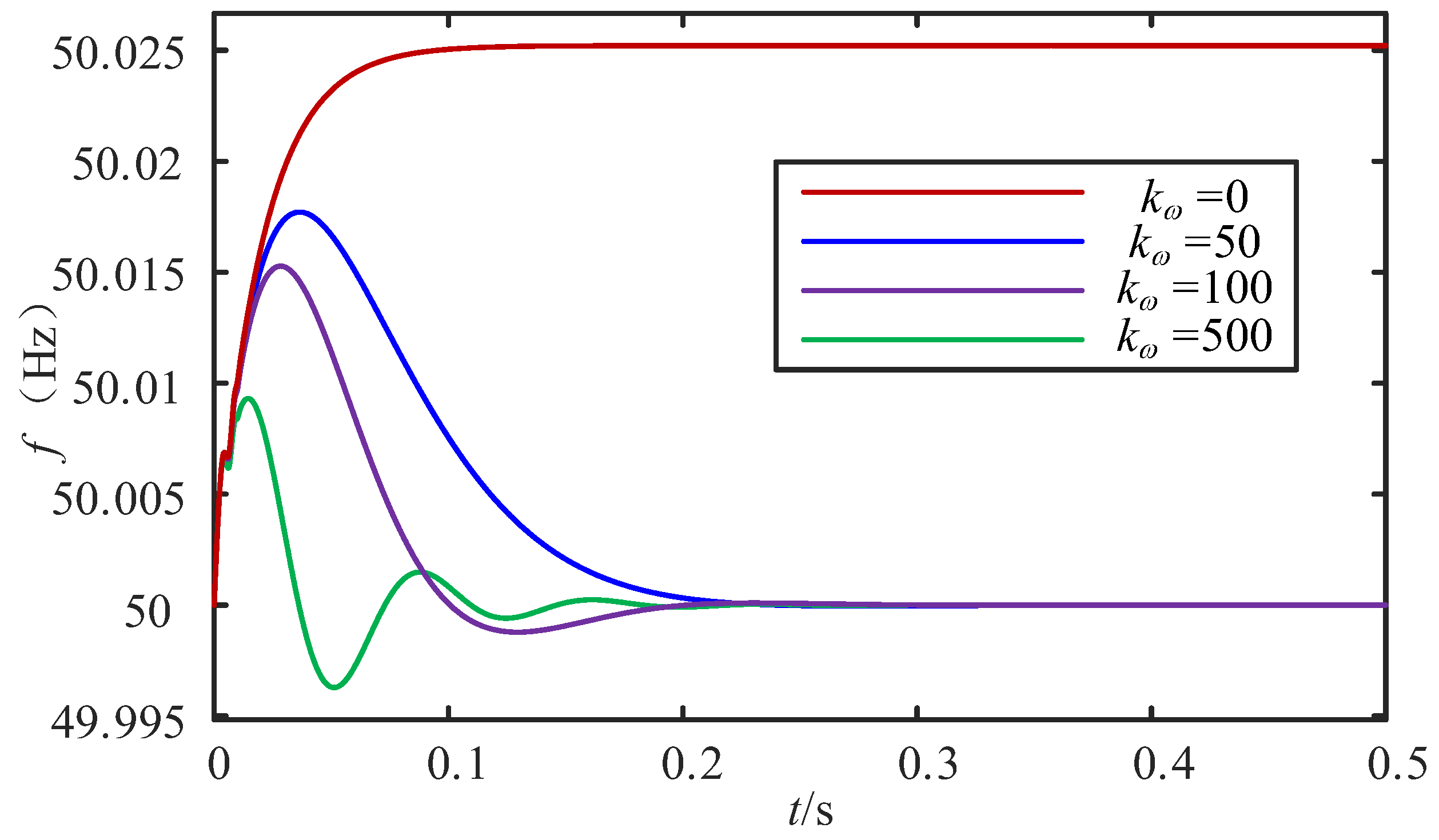
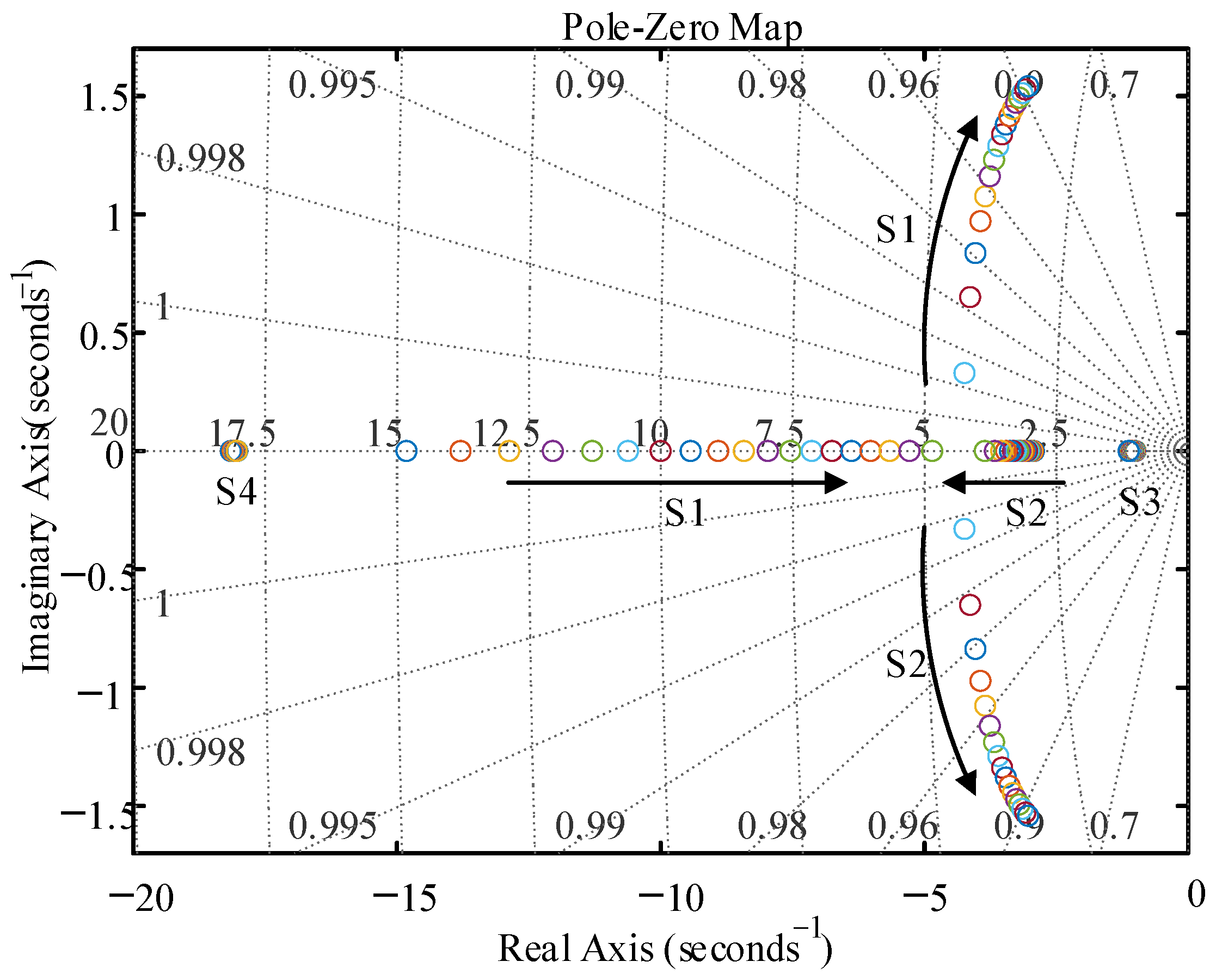
3.2. Small Signal Analysis of Active Support with Integral Link
4. Active Support Pre-Synchronization Grid-Connected Technology
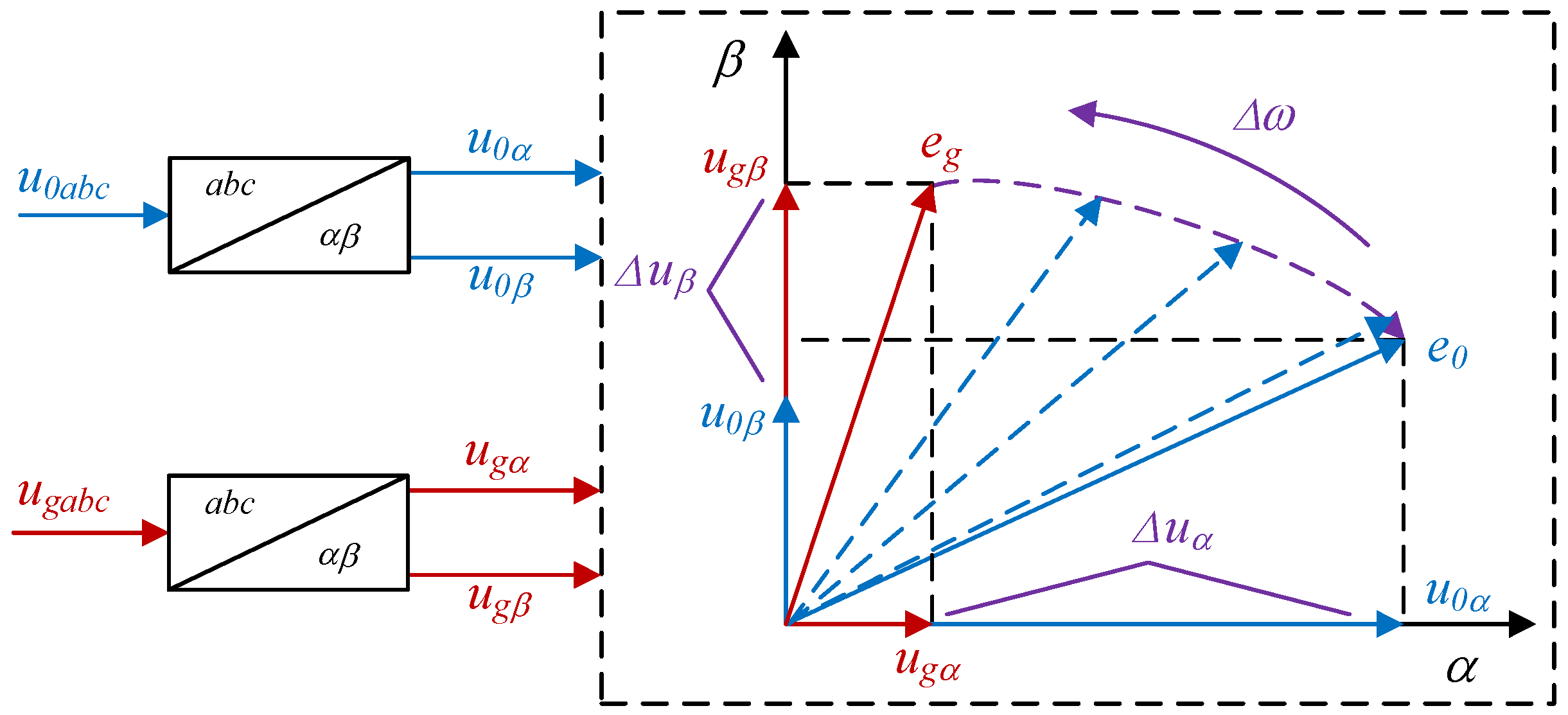
4.1. Phase Controller
4.2. Amplitude Controller
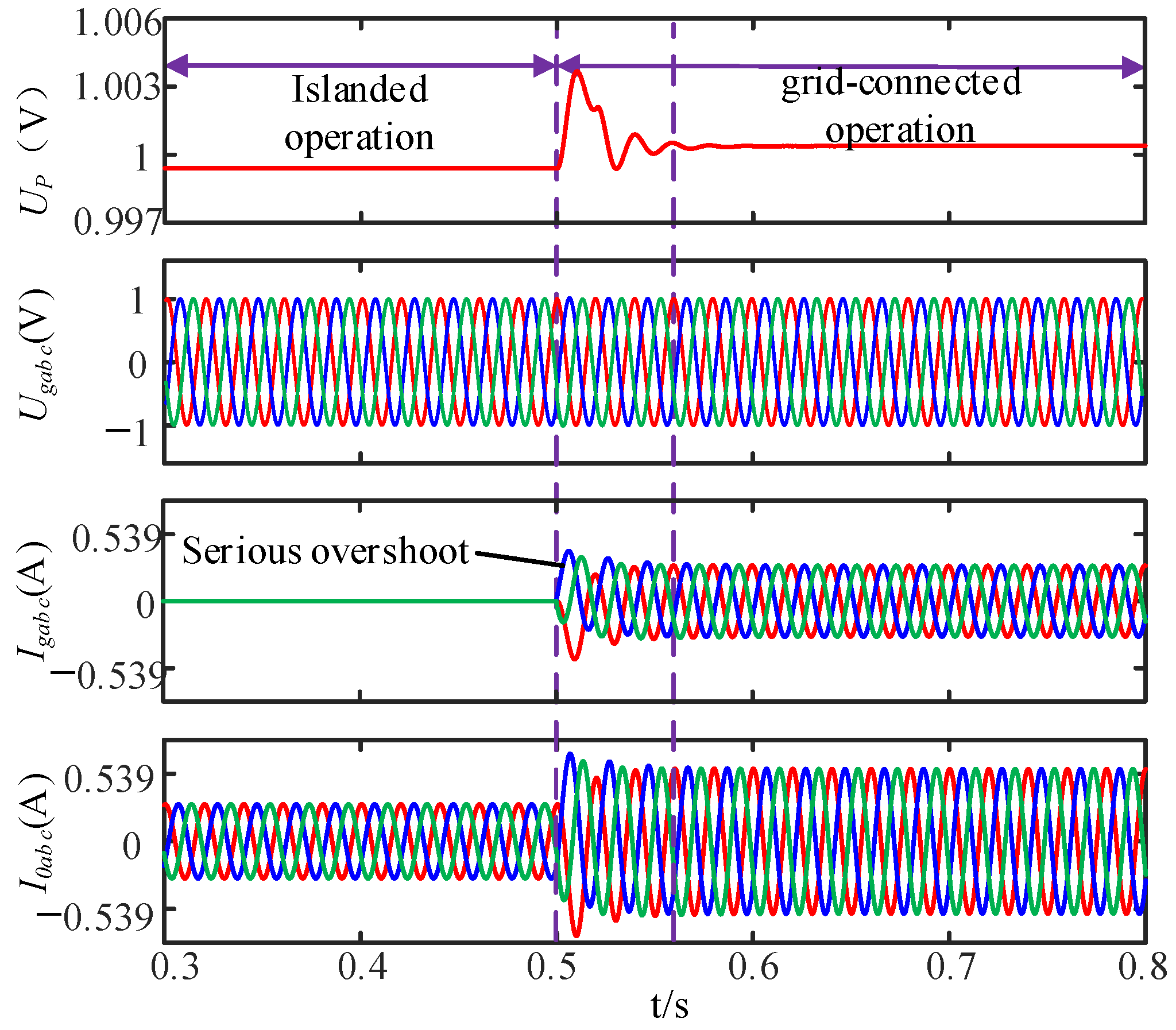
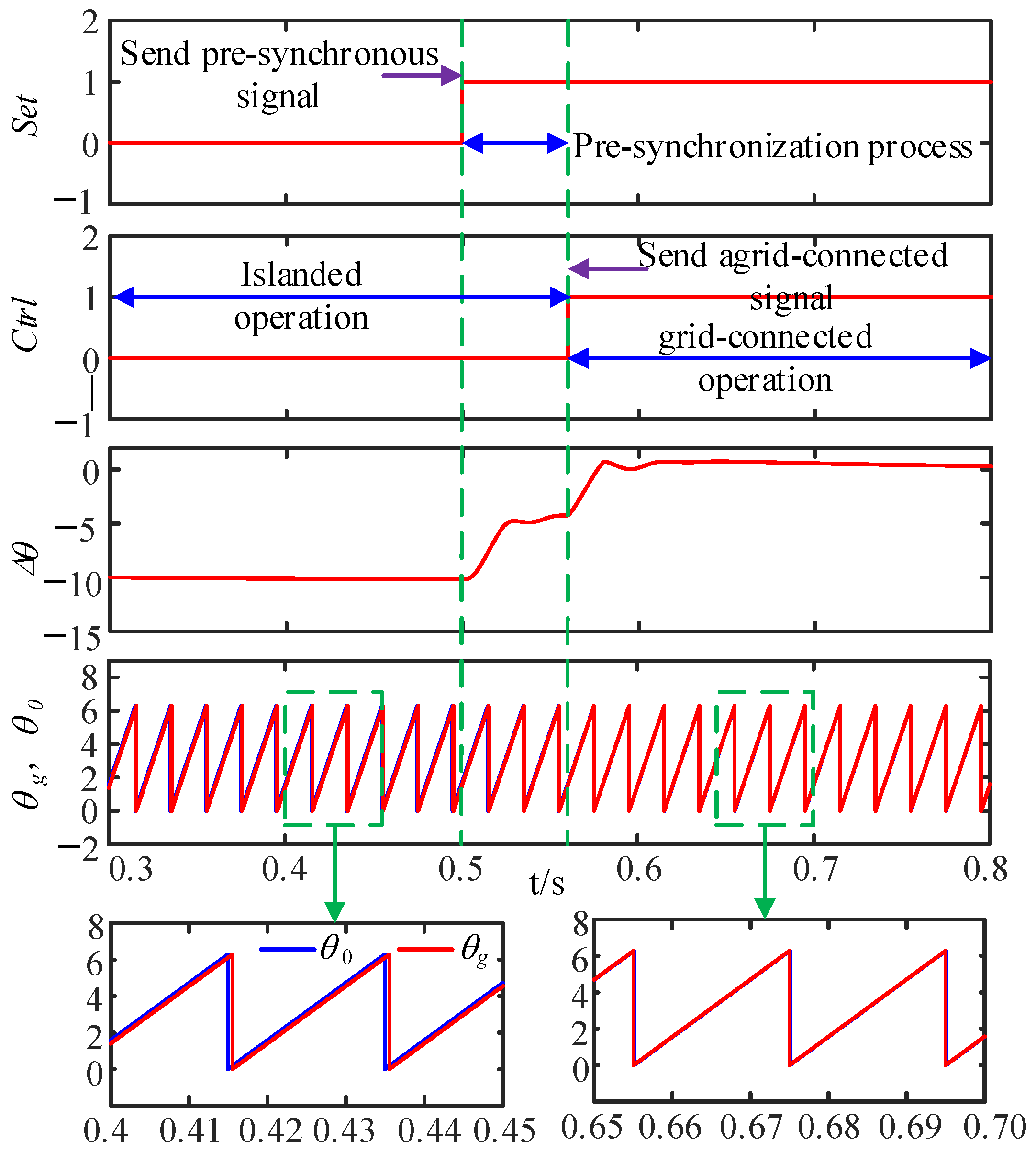
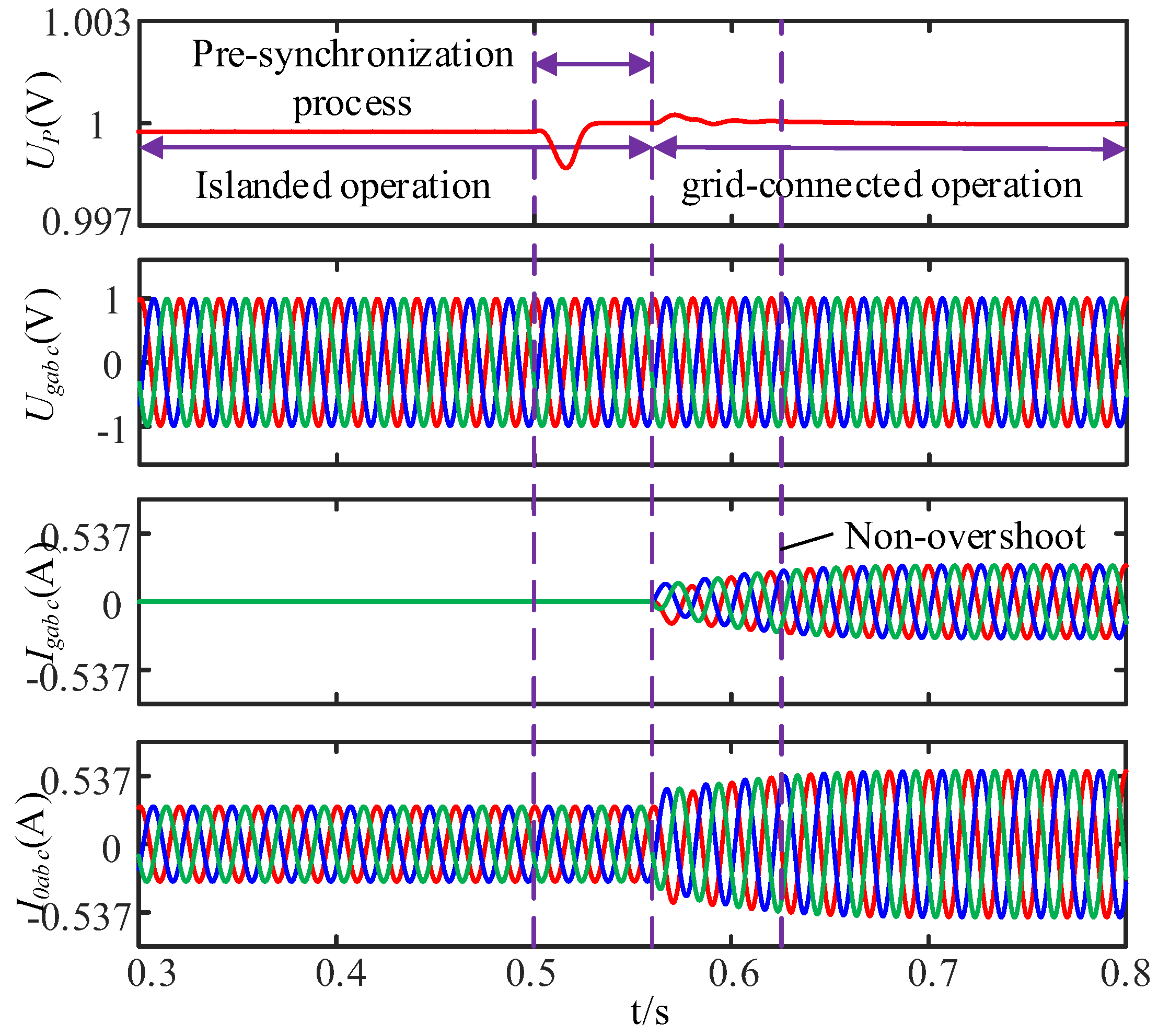
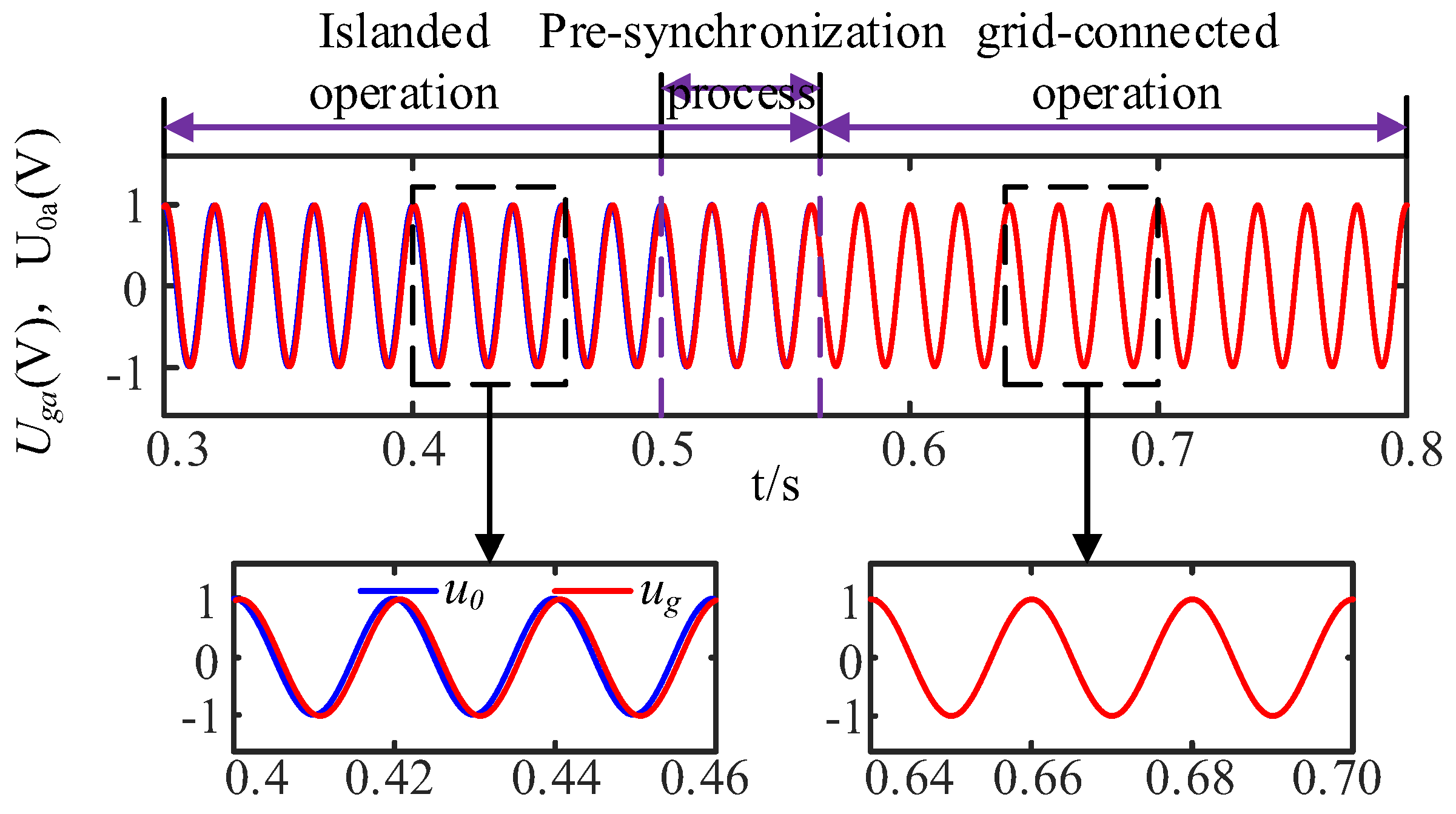
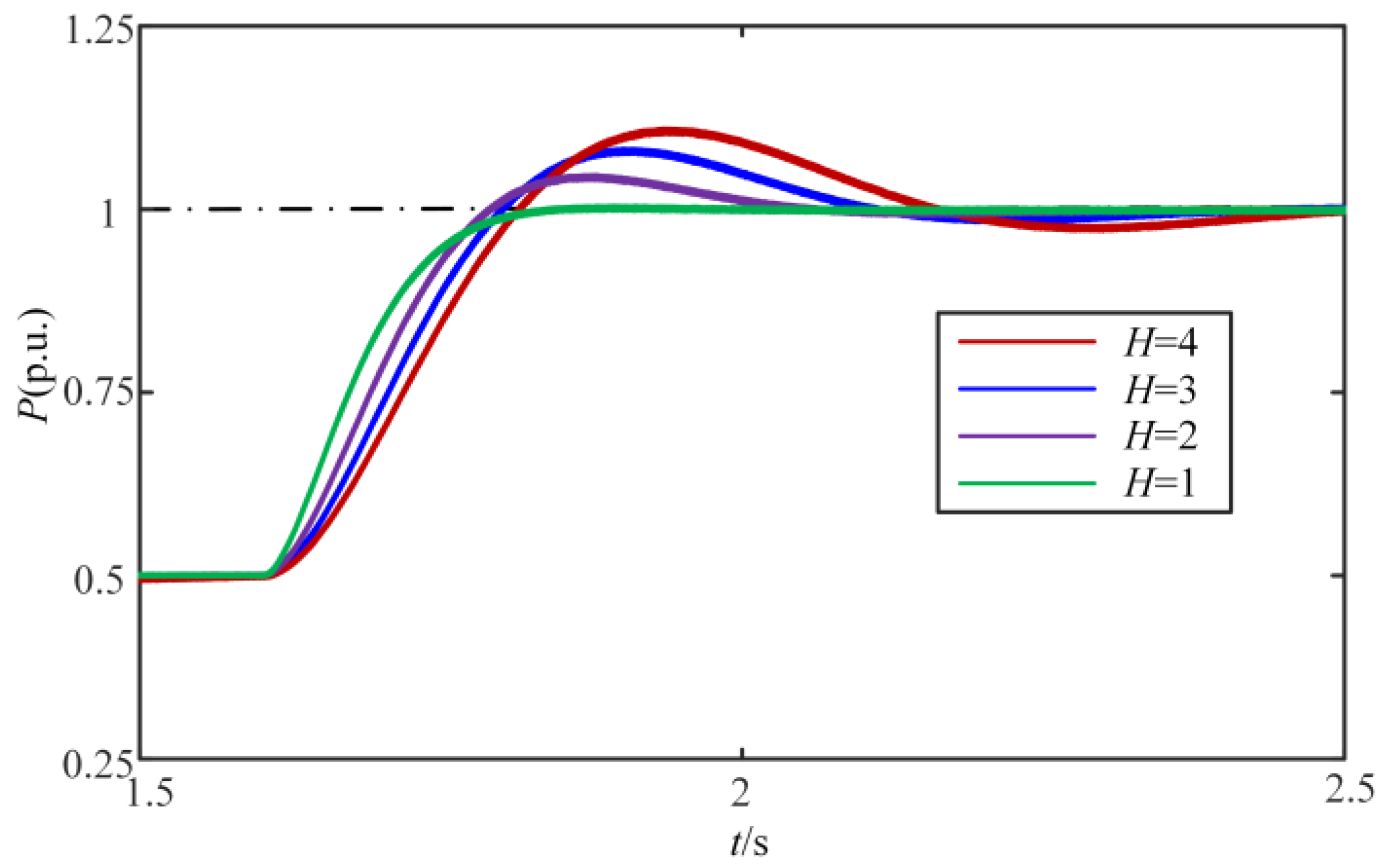
5. Simulation Verification
6. Conclusions
- (1)
- An active support model with a frequency integral feedback link is established in off-grid mode, which effectively improves the frequency stability of local load in off-grid mode.
- (2)
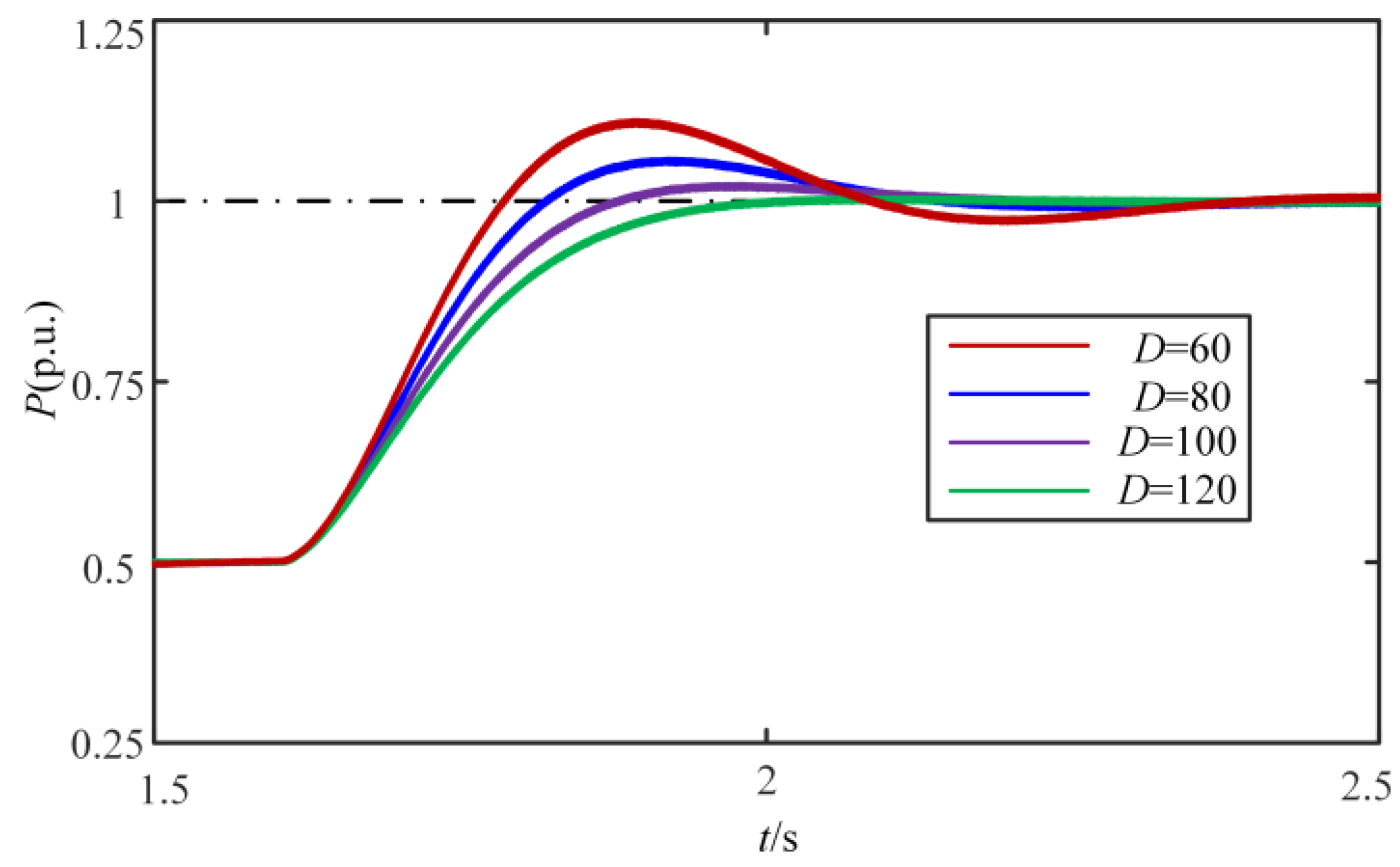
- By reasonably setting and adjusting the values of H and D, the overshoot and dynamic response speed of active support control can be balanced, and the stability of the system can be improved.
- (3)
- A pre-synchronization control model based on the third-order model of active support is established. The voltage on both sides of the PCC is used to compensate for the frequency and voltage of the active frequency link and the excitation voltage link, respectively, and the fast and smooth grid-connected mode of the active support converter is realized.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhuo, Z.; Zhang, N.; Xie, X.; Kang, C. Key Technologies and Development Challenges of High Ratio Renewable Energy Power Systems. Autom. Electr. Power Syst. 2021, 45, 171–191. [Google Scholar]
- Huang, L.; Xin, H.; Huang, W.; Yang, H.; Wang, Z. Quantitative Analysis Method for Frequency Response Characteristics of Power Systems with Virtual Inertia. Autom. Electr. Power Syst. 2018, 42, 31–38. [Google Scholar]
- Cheng, C.; Yang, H.; Zeng, Z.; Tang, S.; Zhao, R. Adaptive control method for rotor inertia of virtual synchronous generator. Autom. Electr. Power Syst. 2015, 39, 82–89. [Google Scholar]
- Wang, B.; Yang, D.; Cai, G. Summary of research on inertia related issues in power systems under high proportion of new energy integration. Power Syst. Technol. 2020, 44, 2998–3007. [Google Scholar]
- Zhu, F.; Peng, Z.; Hu, W.; Wang, H.; Zhang, C.; Zhao, Z. An Improved VSG control strategy for Microgrid. In Proceedings of the 2021 IEEE International Conference on Electrical Engineering and Mechatronics Technology (ICEEMT), Qingdao, China, 2–4 July 2021; pp. 338–342. [Google Scholar]
- Wen, T.; Zhu, D.; Zou, X.; Jiang, B.; Kang, Y. Power Coupling Mechanism Analysis and Improved Decoupling Control for Virtual Synchronous Generator. IEEE Trans. Power Electron. 2021, 36, 3028–3041. [Google Scholar] [CrossRef]
- Liu, B.; Liu, Z.; Liu, J.; An, R.; Zheng, H.; Shi, Y. An Adaptive Virtual Impedance Control Scheme Based on Small-AC-Signal Injection for Unbalanced and Harmonic Power Sharing in Islanded Microgrids. IEEE Trans. Power Electron. 2019, 34, 12333–12355. [Google Scholar] [CrossRef]
- Qi, J.; Li, Y.; Tong, H.; Zhou, D.; Zhang, Y. A Power Second Order Decoupling Strategy for Pre synchronous VSG Based on Dynamic Virtual Current Feedforward. Power Syst. Technol. 2020, 44, 3556–3565. [Google Scholar]
- Zhou, P.; Zhang, X.; Di, Q.; Yue, J.; Xing, C. Pre-synchronization and grid-connected strategy of doubly fed wind turbines based on virtual synchronous machine control. Autom. Electr. Power Syst. 2020, 44, 71–78. [Google Scholar]
- Li, X.; Liu, G.; Yang, R.; Chen, G. Control Strategy and Seamless Switching Method for Virtual Synchronous Generators with Transient Damping Characteristics. Power Syst. Technol. 2018, 42, 2081–2088. [Google Scholar]
- Dong, D.; Wen, B.; Boroyevich, D.; Mattavelli, P. Analysis of phase-locked loop low-frequency stability in three-phase grid-connected power converters considering impedance interactions. IEEE Trans. Ind. Electron. 2014, 62, 310–321. [Google Scholar] [CrossRef]
- Atmaca, Ö.; Karabacak, M. Frequency, Phase, and Magnitude Difference Locked-Loop Based Linear Synchronization Scheme for Islanded Inverters and Microgrids. IEEE Access 2023, 11, 61748–61772. [Google Scholar] [CrossRef]
- Wang, X.; Wang, Y.; Liu, Y.; Liu, C.; Wang, S. Low voltage ride through control method for actively supported new energy units based on virtual reactance. Power Syst. Technol. 2022, 46, 4435–4444. [Google Scholar]
- Zhang, Q.; Peng, C.; Chen, Y.; Jin, G.; Luo, A. A control strategy for parallel operation of multi-inverters in microgrid. Proc. CSEE 2012, 32, 126–132+18. [Google Scholar]
- Liu, C.; Sun, T.; Cai, G.; Ge, W.; Ge, Y.; Yan, Y.; Liu, Y. Active Support Control and Primary Frequency Modulation Contribution Analysis of Battery Energy Storage Power Station Based on Synchronous Machine Third Order Model. Proc. CSEE 2020, 40, 4854–4866. [Google Scholar]

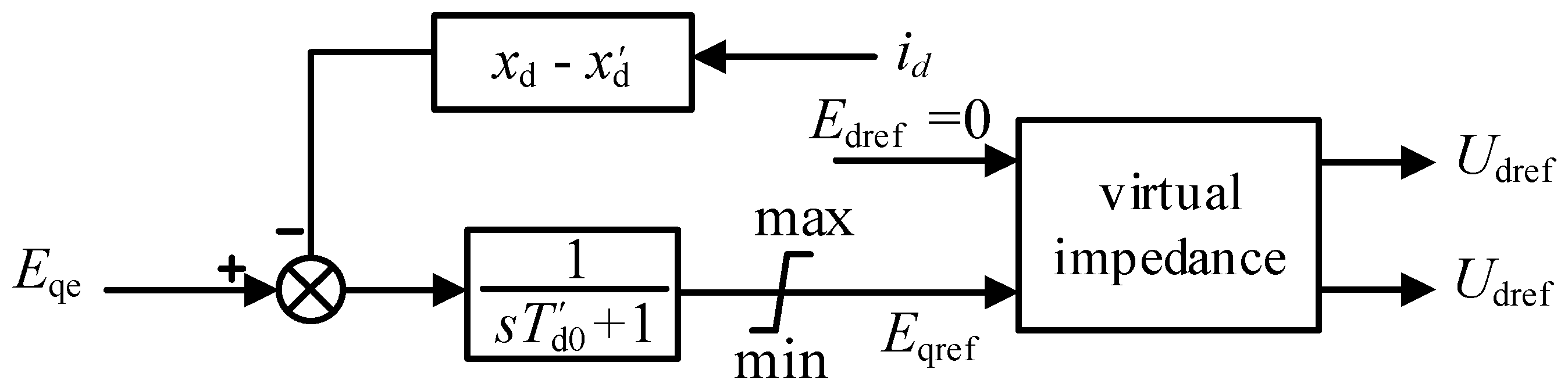




| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Td0′ | 5s | Xd | 1.18 |
| Xd′ | 1 | Xad | 0.295 |
| Xf | 1.13 | X | 5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, C.; Zhi, Y.; Su, Z.; Yang, Z.; Yin, L.; Man, J.; Yang, Y. Active Support Pre-Synchronization Control and Stability Analysis Based on the Third-Order Model of Synchronous Machine. Energies 2024, 17, 5072. https://doi.org/10.3390/en17205072
Liu C, Zhi Y, Su Z, Yang Z, Yin L, Man J, Yang Y. Active Support Pre-Synchronization Control and Stability Analysis Based on the Third-Order Model of Synchronous Machine. Energies. 2024; 17(20):5072. https://doi.org/10.3390/en17205072
Chicago/Turabian StyleLiu, Chuang, Yuemei Zhi, Zhida Su, Zedong Yang, Limin Yin, Jiawei Man, and Yuze Yang. 2024. "Active Support Pre-Synchronization Control and Stability Analysis Based on the Third-Order Model of Synchronous Machine" Energies 17, no. 20: 5072. https://doi.org/10.3390/en17205072
APA StyleLiu, C., Zhi, Y., Su, Z., Yang, Z., Yin, L., Man, J., & Yang, Y. (2024). Active Support Pre-Synchronization Control and Stability Analysis Based on the Third-Order Model of Synchronous Machine. Energies, 17(20), 5072. https://doi.org/10.3390/en17205072






