Improvement of Heat Transfer Correlation for Fluoroplastic Steel Heat Exchanger and Theoretical Analysis of Application Characteristics
Abstract
1. Introduction
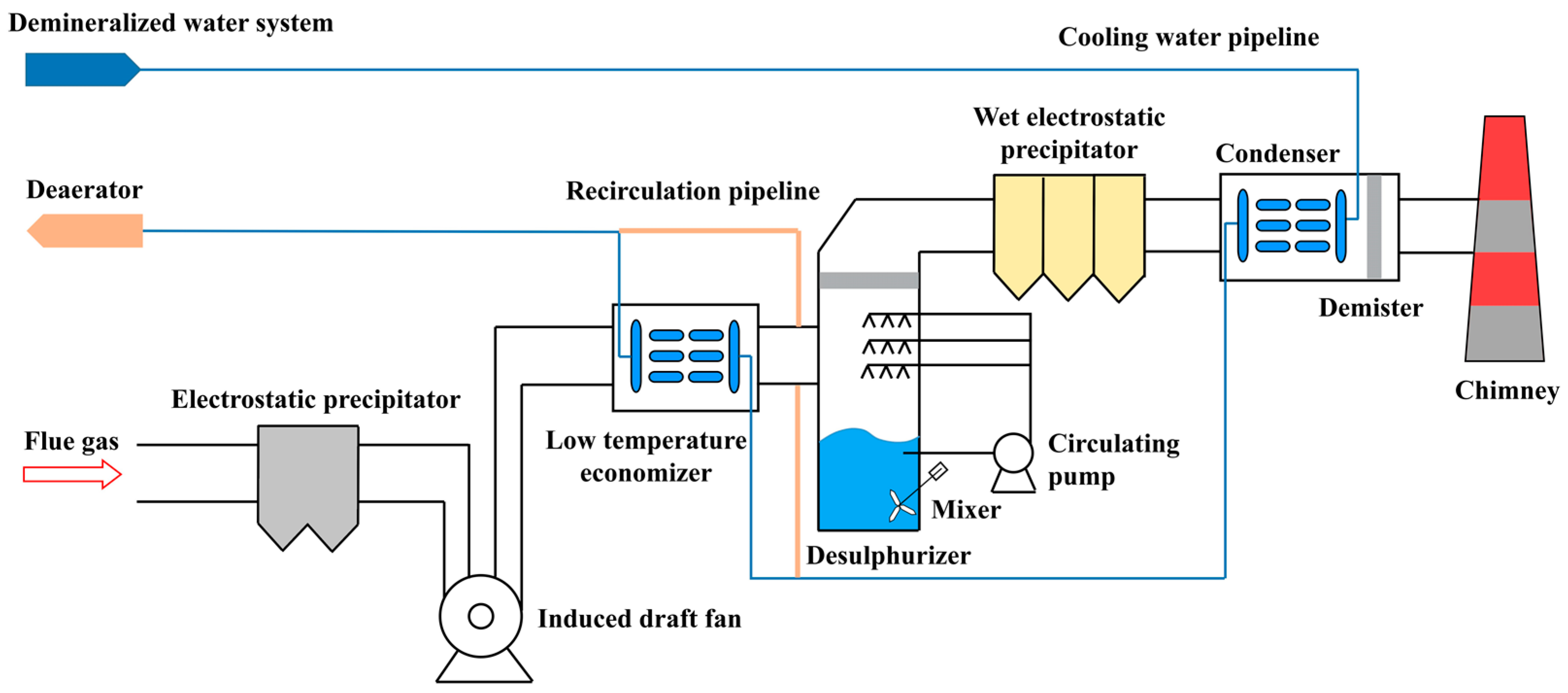
2. Testing System
2.1. Testing Setup
2.2. Fluoroplastic Steel Heat Exchanger
2.3. Experimental Data Processing
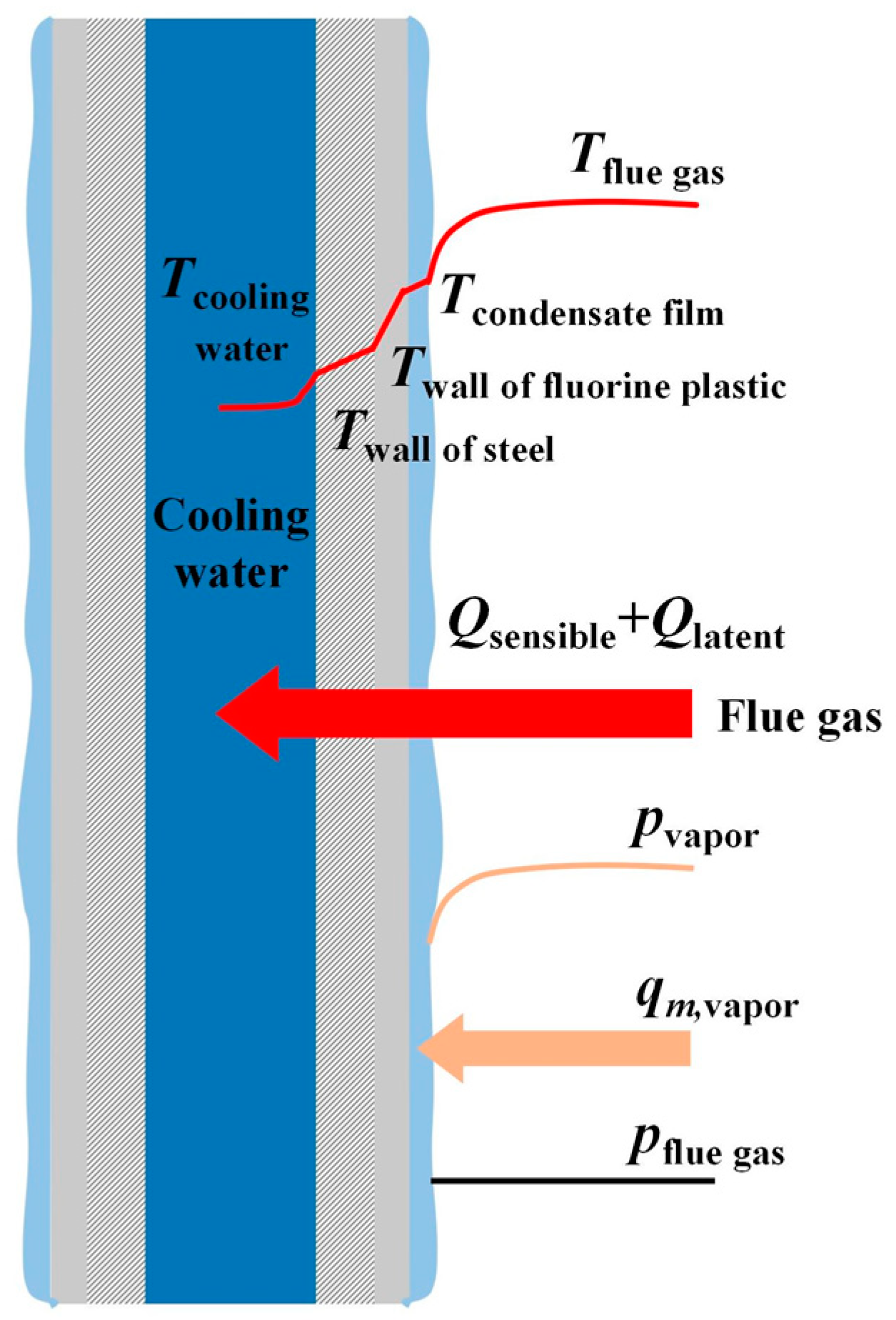
3. Heat Transfer Model
4. Results and Discussions
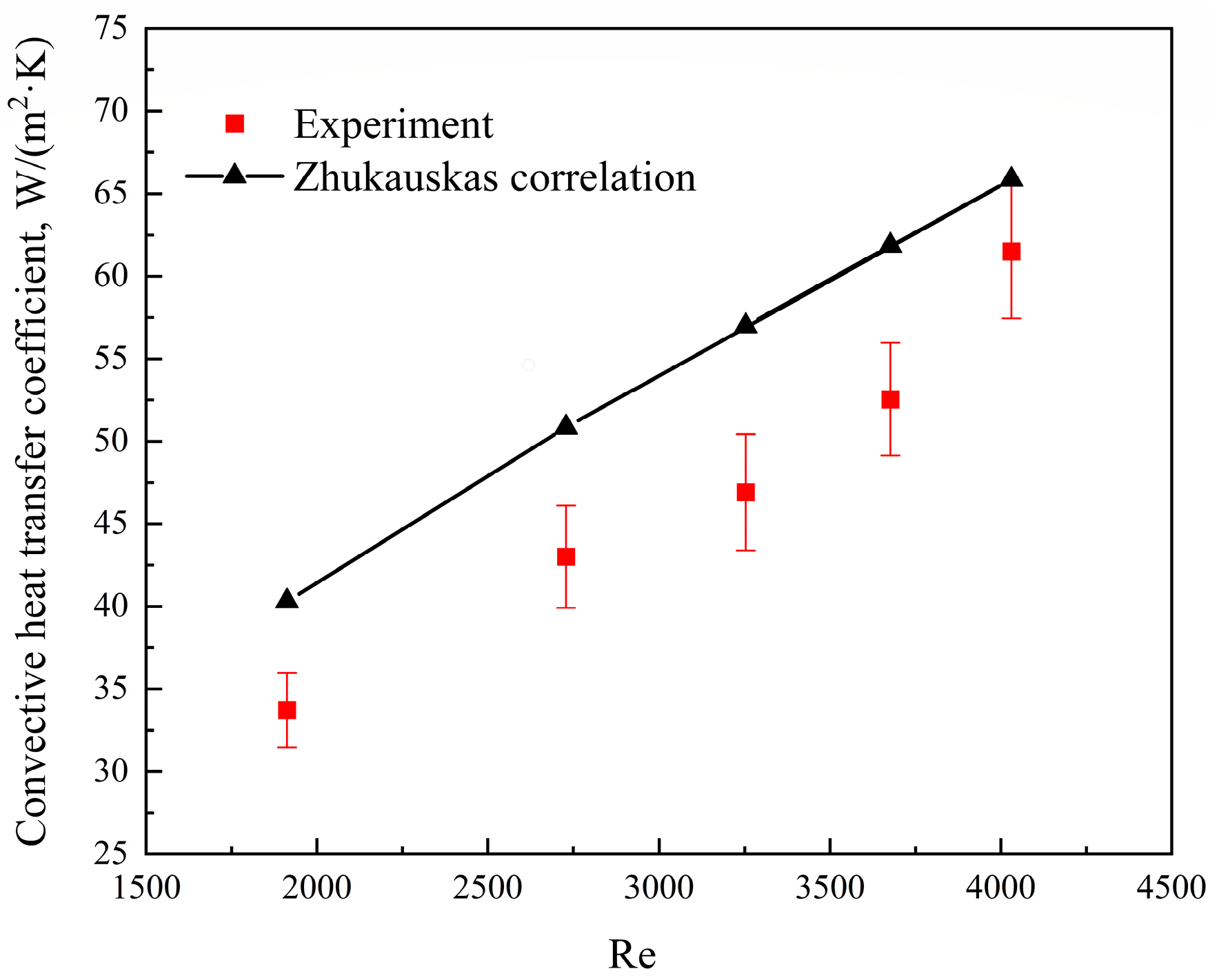
4.1. Heat Transfer Correlation Fitting and Verification
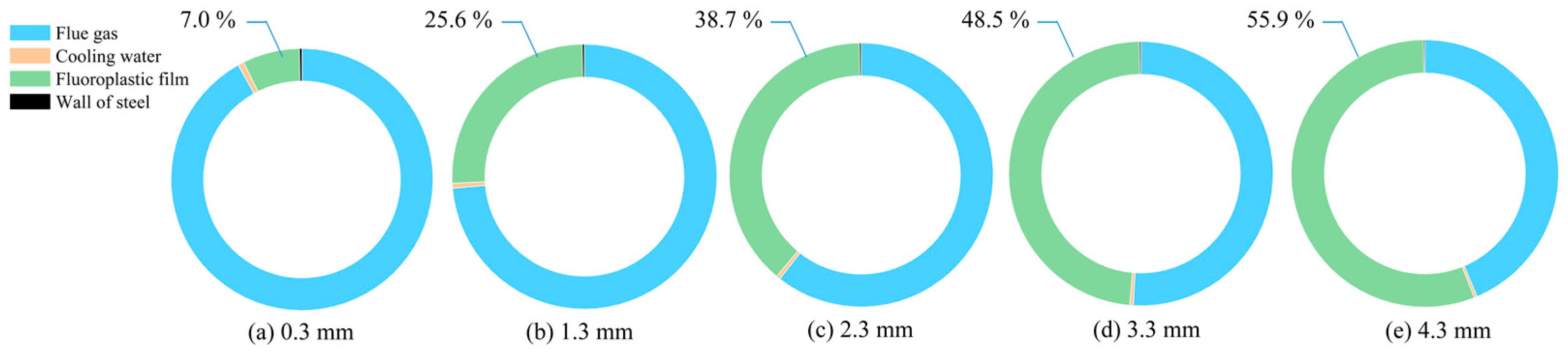
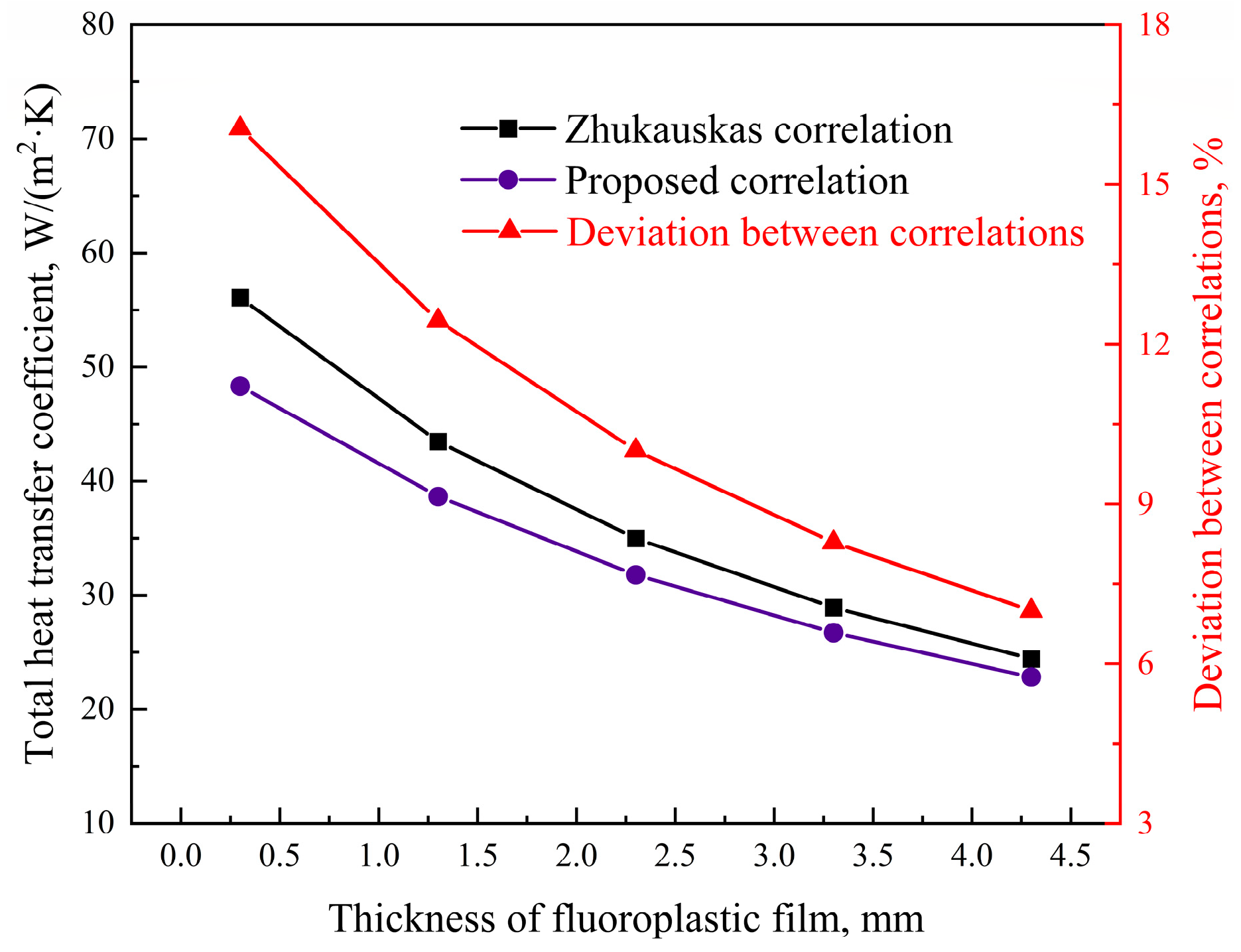
4.2. Influence of Fluoroplastic Film Thickness
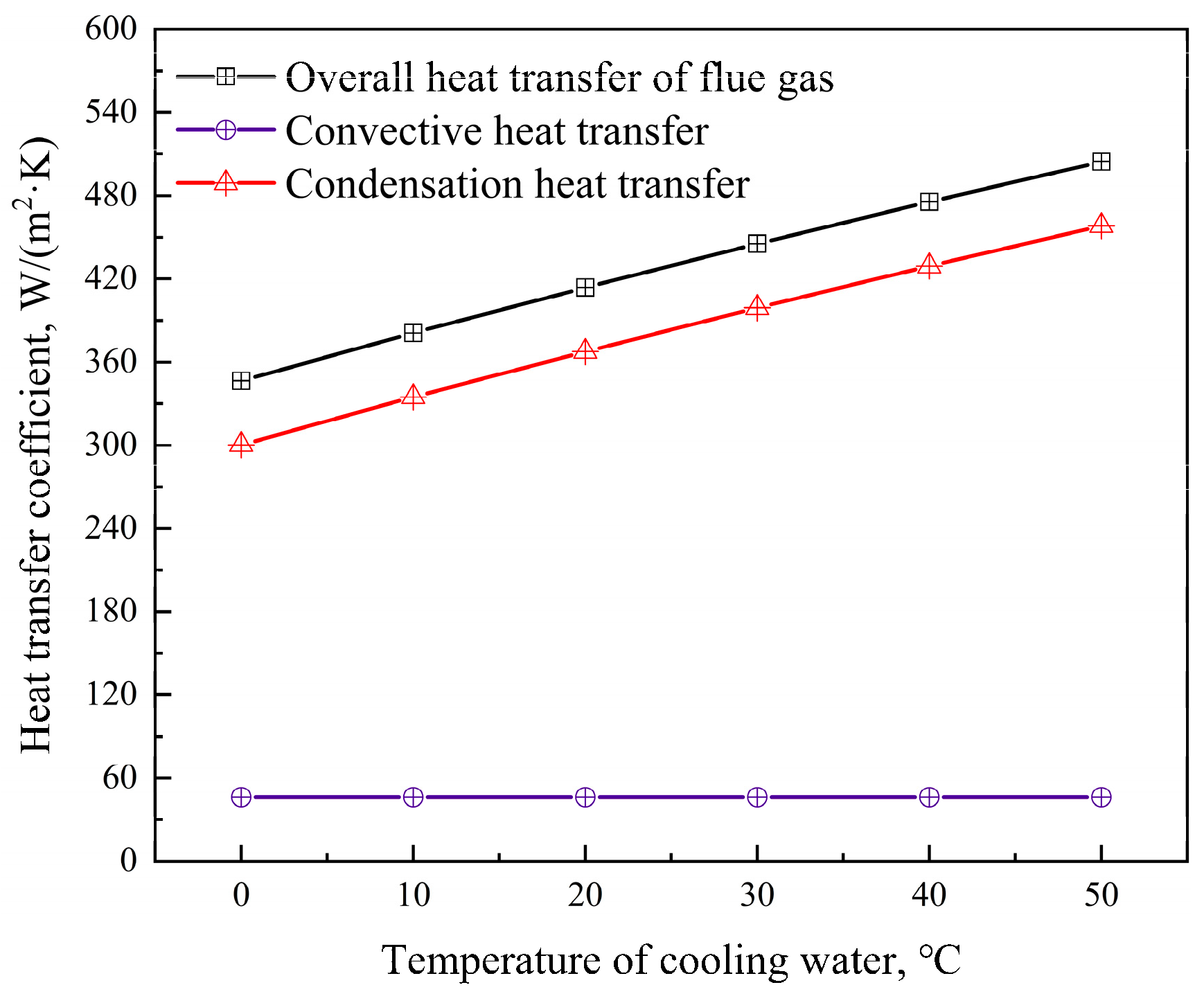
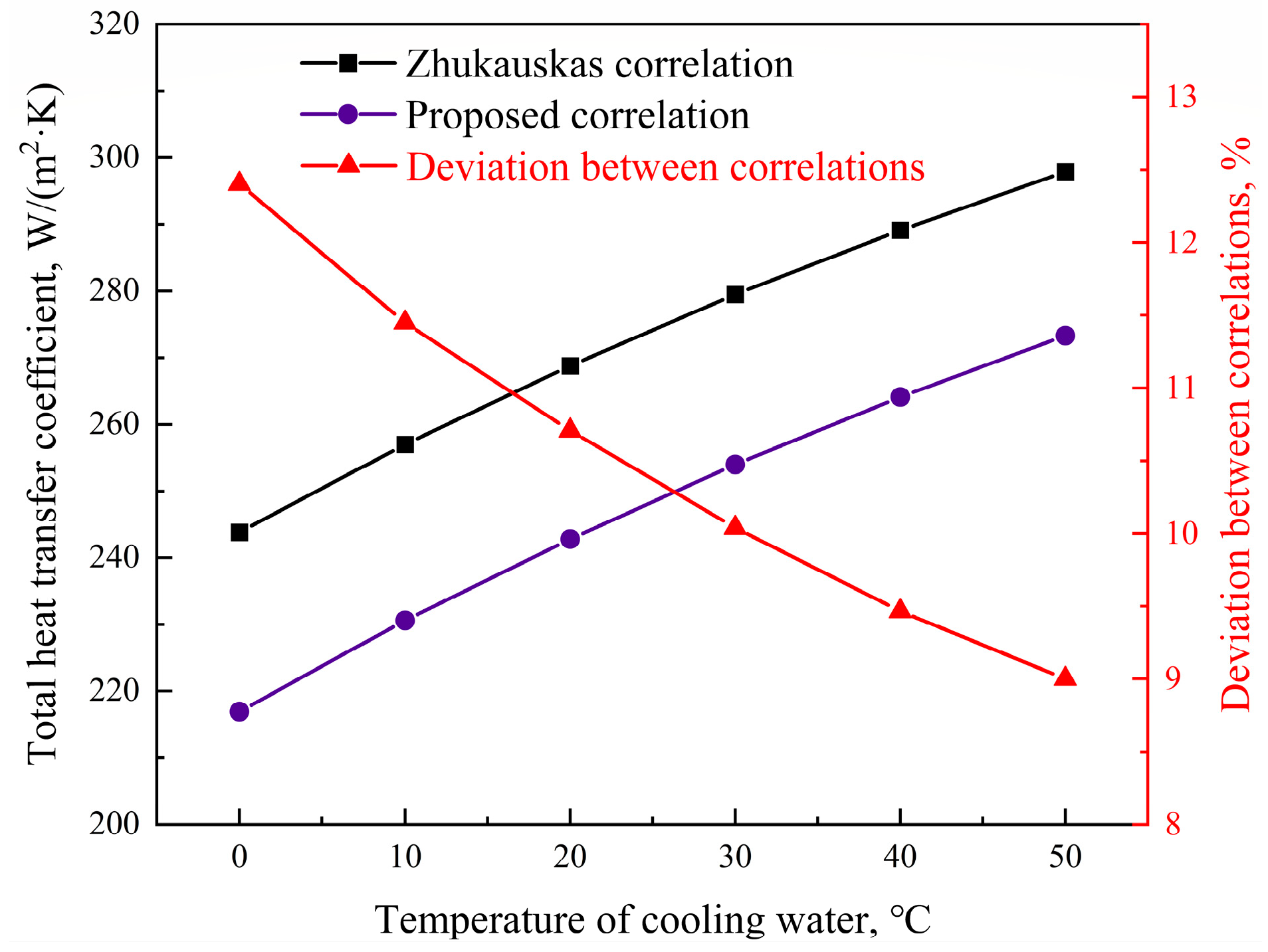
4.3. Influence of Condensation Intensity
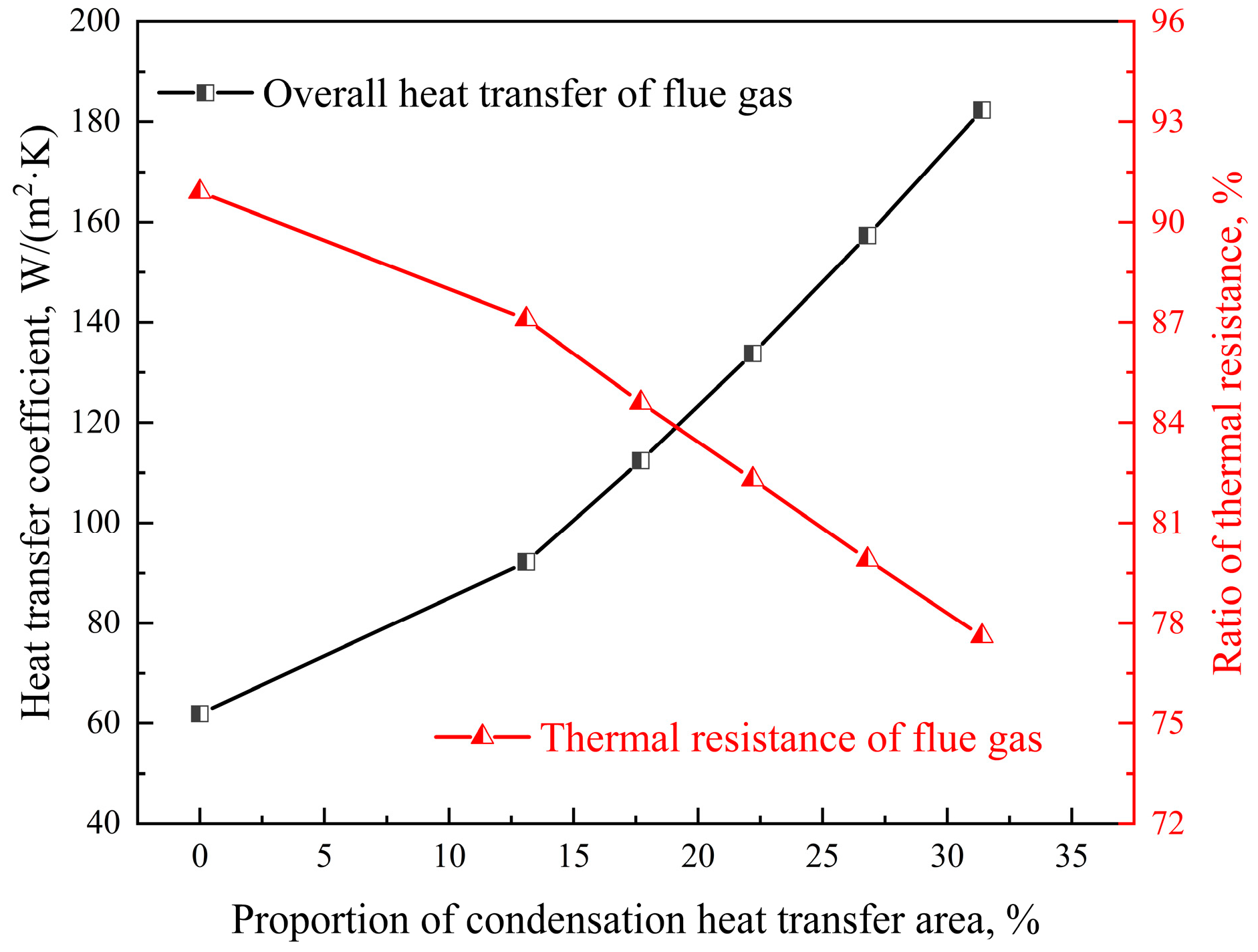
4.4. Influence of Condensation Heat Transfer Area Ratio
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- The State Council of the People’s Republic of China, Guiding Opinions of the State Council on Accelerating the Establishment and Improvement of a Green and Low-carbon Circular Development Economic System. 2021; (In Chinese). Available online: https://www.gov.cn/zhengce/content/2021-02/22/gcontent_5588274.htm (accessed on 19 November 2023).
- Li, X.; Zhou, T.; Chen, H.; Zhang, H. Study on heat transfer performance using ceramic membrane to recover moisture and waste heat from flue gas. Appl. Therm. Eng. 2023, 231, 120887. [Google Scholar] [CrossRef]
- Wang, C.; He, B.; Sun, S.; Wu, Y.; Yan, N.; Yan, L.; Pei, X. Application of a low pressure economizer for waste heat recovery from the exhaust flue gas in a 600 MW power plant. Energy 2012, 48, 196–202. [Google Scholar] [CrossRef]
- Terhan, M.; Comakli, K. Design and economic analysis of a flue gas condenser to recover latent heat from exhaust flue gas. Appl. Therm. Eng. 2016, 100, 1007–1015. [Google Scholar] [CrossRef]
- Xiong, Y.; Niu, Y.; Wang, X.; Tan, H. Pilot study on in-depth water saving and heat recovery from tail flue gas in lignite-fired power plant. Energy Procedia 2014, 61, 2558–2561. [Google Scholar] [CrossRef]
- Han, Y.; Xu, G.; Zheng, Q.; Xu, C.; Hu, Y.; Yang, Y.; Lei, J. New heat integration system with bypass flue based on the rational utilization of low-rade extraction steam in a coal–fired power plant. Appl. Therm. Eng. 2017, 113, 460–471. [Google Scholar] [CrossRef]
- Yan, M.; Zhang, L.; Shi, Y.; Zhang, L.; Li, Y.; Ma, C. A novel boiler cold-end optimisation system based on bypass flue in coal-fired power plants: Heat recovery from wet flue gas. Energy 2018, 152, 84–94. [Google Scholar] [CrossRef]
- Liu, J.; Zhu, F.; Ma, X. Industrial application of a deep purification technology for flue gas involving phase-transition agglomeration and dehumidification. Engineering 2018, 4, 416–420. [Google Scholar] [CrossRef]
- Li, K.; Wang, E.; Li, D.; Husnain, N.; Fareed, S. Numerical and experimental investigation on water vapor condensation in turbulent flue gas. Appl. Therm. Eng. 2019, 160, 114009. [Google Scholar] [CrossRef]
- Zhao, S.; Yan, S.; Wang, D.K.; Wei, Y.; Qi, H.; Wu, T.; Feron, P.H. Feron, Simultaneous heat and water recovery from flue gas by membrane condensation: Experimental investigation. Appl. Therm. Eng. 2017, 113, 843–850. [Google Scholar] [CrossRef]
- Klemes, J.J.; Arsenyeva, O.; Kapustenko, P.; Tovazhnyanskyy, L. Compact Heat Exchangers for Energy Transfer Intensifification: Low Grade Heat and Fouling Mitigation; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Ma, H.; Liang, N.; Liu, Y.; Luo, X.; Hou, C.; Wamg, G. Experimental study on novel waste heat recovery system for sulfide-containing flue gas. Energy 2021, 227, 120479. [Google Scholar] [CrossRef]
- Cao, R.; Tan, H.; Xiong, Y.; Mikulčić, H.; Vujanović, M.; Wang, X.; Duić, N. Improving the removal of particles and trace elements from coal-fired power plants by combining a wet phase transition agglomerator with wet electrostatic precipitator. J. Clean. Prod. 2017, 161, 1459–1465. [Google Scholar] [CrossRef]
- Xiong, Y.; Tan, H.; Wang, Y.; Xu, W.; Mikulčić, H.; Duić, N. Pilot-scale study on water and latent heat recovery from flue gas using fluorine plastic heat exchangers. J. Clean. Prod. 2017, 161, 1416–1422. [Google Scholar] [CrossRef]
- Yang, J.; Sun, Z.; Yuan, R.; Huang, H.; Hu, Y. Study of heat transfer and ash deposit characteristics of fluoroplastic steel air-preheater in power plant. J. Zhejiang Univ. (Eng. Sci.) 2018, 52, 577–583. (In Chinese) [Google Scholar]
- Li, K.; Zhang, P.; Gong, J.; Wu, B.; Tan, Q. Practice and analysis of flue gas waste heat recovery system in co-generation plant. J. Shanghai Univ. Electr. Power 2021, 67, 533–538. (In Chinese) [Google Scholar]
- Jin, Y.; Gao, N.; Zhu, T. Techno-economic analysis on a new conceptual design of waste heat recovery for boiler exhaust flue gas of coal-fired power plants. Energ. Convers. Manag. 2019, 200, 112097. [Google Scholar] [CrossRef]
- Xu, G.; Xu, C.; Yang, Y.; Fang, Y.; Li, Y.; Song, X. A novel flue gas waste heat recovery system for coal-fired ultra-supercritical power plants. Appl. Therm. Eng. 2014, 67, 240–249. [Google Scholar] [CrossRef]
- Tan, H.; Cao, R.; Wang, S.; Wang, Y.; Deng, S.; Duić, N. Proposal and techno-economic analysis of a novel system for waste heat recovery and water saving in coal-fired power plants: A case study. J. Clean. Prod. 2020, 281, 124372. [Google Scholar] [CrossRef]
- Xu, G.; Chen, Y.; Niu, C.; Man, X.; Wang, Y.; Ren, Y.; Xu, G. Optimization of heat transfer model and performance analysis of fluorine plastic heat exchangers. Proc. CSEE 2017, 37, 2297–2304. (In Chinese) [Google Scholar] [CrossRef]
- Jeong, K.; Kessen, M.J.; Bilirgen, H.; Levy, E.K. Analytical modeling of water condensation in condensing heat exchanger. Int. J. Heat Mass Tran. 2010, 53, 2361–2368. [Google Scholar] [CrossRef]
- Macháčková, A.; Kocich, R.; Bojko, M.; Kunčická, L.; Polko, K. Numerical and experimental investigation of flue gases heat recovery via condensing heat exchanger. Int. J. Heat Mass Tran. 2018, 124, 1321–1333. [Google Scholar] [CrossRef]
- Mohammadaliha, N.; Amani, M.; Bahrami, M. Thermal performance of heat and water recovery systems: Role of condensing heat exchanger material. Clean. Eng. Technol. 2020, 1, 100024. [Google Scholar] [CrossRef]
- Mohammadaliha, N.; Amani, M.; Bahrami, M. A Thermal-hydraulic assessment of condensing tube bank heat exchangers for heat and water recovery from flue gas. Appl. Therm. Eng. 2022, 215, 118976. [Google Scholar] [CrossRef]
- Yang, S.; Tao, W. Heat Transfer; Higher Education Press: Beijing, China, 2006; pp. 246–261. (In Chinese) [Google Scholar]
- Shen, D.W.; Jiang, Z.M.; Tong, J.G. Engineering Thermodynamics; Higher Education Press: Beijing, China, 2007; p. 379. (In Chinese) [Google Scholar]
- Moffat, R.J. Describing the uncertainties in experimental results. Exp. Therm. Fluid Sci. 1988, 1, 3–17. [Google Scholar] [CrossRef]
- Wang, E.; Li, K.; Mao, J.; Husnain, N.; Li, D.; Wu, W. Experimental study of flow and heat transfer in rotary air preheaters with honeycomb ceramics and metal corrugated plates. Appl. Therm. Eng. 2018, 130, 1549–1557. [Google Scholar] [CrossRef]
- Zschaeck, G.; Frank, T.; Burns, A.D. CFD modelling and validation of wall condensation in the presence of non-condensable gases. Nucl. Eng. Des. 2014, 279, 137–146. [Google Scholar] [CrossRef]
- Han, L.; Yang, K.; Yang, J.; Li, R.; Li, Y.; Deng, L.; Che, D. A thermal calculation model for tubular condensing heat exchanger. Appl. Therm. Eng. 2024, 244, 122701. [Google Scholar] [CrossRef]
- Colburn, A.P.; Hougen, O.A. Design of cooler condensers for mixtures of vapors with noncondensing gases. Ind. Eng. Chem. 1934, 26, 1178–1182. [Google Scholar] [CrossRef]
- Li, J.; Saraireh, M.; Thorpe, G. Condensation of vapor in the presence of noncondensable gas in condensers. Int. J. Heat Mass Tran. 2011, 54, 4078–4089. [Google Scholar] [CrossRef]
- Colburn, A.P. A method of correlating forced convection heat-transfer data and a comparison with fluid friction. Int. J. Heat Mass Tran. 1964, 7, 1359–1384. [Google Scholar] [CrossRef]
- Chilton, T.H.; Colburn, A.P. Mass transfer (absorption) coefficients prediction from data on heat transfer and fluid friction. Ind. Eng. Chem. 1934, 26, 1183–1187. [Google Scholar] [CrossRef]
- Jeong, K.; Levy, E.K. Theoretical prediction of sulfuric acid condensation rates in boiler flue gas. Int. J. Heat Mass Tran. 2012, 55, 8010–8019. [Google Scholar] [CrossRef]
- Lian, Z. Principle of Heat and Mass Exchange with Equipment; China Architecture & Building Press: Beijing, China, 2006; pp. 58–60. (In Chinese) [Google Scholar]
- Žukauskas, A. Heat Transfer from Tubes in Crossflow. Adv. Heat Transf. 1972, 8, 93–160. [Google Scholar]



| Parameters | Units | Values |
|---|---|---|
| Inlet flue gas temperature | °C | 101.3–109.6 |
| Inlet demineralized water temperature | °C | 57.3–61.1 |
| Outlet flue gas temperature | °C | 81.4–91.6 |
| Outlet demineralized water temperature | °C | 66–74.7 |
| Inlet glue gas velocity | m/s | 6.4–12.8 |
| Inlet demineralized water velocity | m/s | 1.32–1.52 |
| Flue gas pressure | Pa | 1490–2910 |
| Volume fraction of water vapor in flue gas | % | 7.6–10.4 |
| Measurement Parameters | Ranges | Errors |
|---|---|---|
| Demineralized water temperature | 0–300 °C | ±12 °C |
| Flue gas temperature | −20 to 1300 °C | ±6.6 °C |
| Flue gas Pressure | ±5 kPa | ±3.0% |
| CO2 | 0–21 vol.% | ±5.0% |
| O2 | 0–25 vol.% | ±5.0% |
| SO2 | 0–0.02 vol.% | ±5.0% |
| Humidity | 0–60% | ±1.5% |
| Demineralized water velocity | 0.5–3 m/s | ±2.5% |
| Flue gas velocity | 0.1–130 m/s | ±1.0% |
| Parameters | Units | Values |
|---|---|---|
| Height × Width × Length | m | 4 × 8 × 1.5 |
| Outer diameter | mm | 20.6 |
| Thickness of fluoroplastic film | mm | 0.3 |
| Thickness of the stainless steel | mm | 1 |
| Longitudinal pitches | mm | 43 |
| Transverse pitches | mm | 55 |
| Longitudinal rows | / | 24 |
| Transverse rows | / | 88 |
| Surface roughness of fluoroplastic | μm | 0.17 |
| Thermal conductivity of stainless steel | W/(m·K) | 16.27 |
| Thermal conductivity of fluoroplastic | W/(m·K) | 0.209 |
| Re | % | ||||
|---|---|---|---|---|---|
| 1913 | 0.337 | 0.553 | 0.445 | 2.107 | 6.7 |
| 2729 | 0.43 | 0.874 | 0.727 | 2.849 | 7.2 |
| 3253 | 0.469 | 0.996 | 0.828 | 2.718 | 7.5 |
| 3677 | 0.526 | 1.149 | 0.96 | 3.092 | 6.5 |
| 4031 | 0.615 | 1.368 | 1.153 | 3.092 | 6.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, K.; Meng, Z.; Feng, H.; Wang, Y.; Wang, Q.; Gao, X. Improvement of Heat Transfer Correlation for Fluoroplastic Steel Heat Exchanger and Theoretical Analysis of Application Characteristics. Energies 2024, 17, 5054. https://doi.org/10.3390/en17205054
Li K, Meng Z, Feng H, Wang Y, Wang Q, Gao X. Improvement of Heat Transfer Correlation for Fluoroplastic Steel Heat Exchanger and Theoretical Analysis of Application Characteristics. Energies. 2024; 17(20):5054. https://doi.org/10.3390/en17205054
Chicago/Turabian StyleLi, Kai, Zhihao Meng, Hong Feng, Yihong Wang, Qi Wang, and Xiang Gao. 2024. "Improvement of Heat Transfer Correlation for Fluoroplastic Steel Heat Exchanger and Theoretical Analysis of Application Characteristics" Energies 17, no. 20: 5054. https://doi.org/10.3390/en17205054
APA StyleLi, K., Meng, Z., Feng, H., Wang, Y., Wang, Q., & Gao, X. (2024). Improvement of Heat Transfer Correlation for Fluoroplastic Steel Heat Exchanger and Theoretical Analysis of Application Characteristics. Energies, 17(20), 5054. https://doi.org/10.3390/en17205054







