Experimental Investigation on the Performance of the Scroll Expander under Various Driving Cycles
Abstract
1. Introduction
2. Experimental Setup
3. Results and Discussion
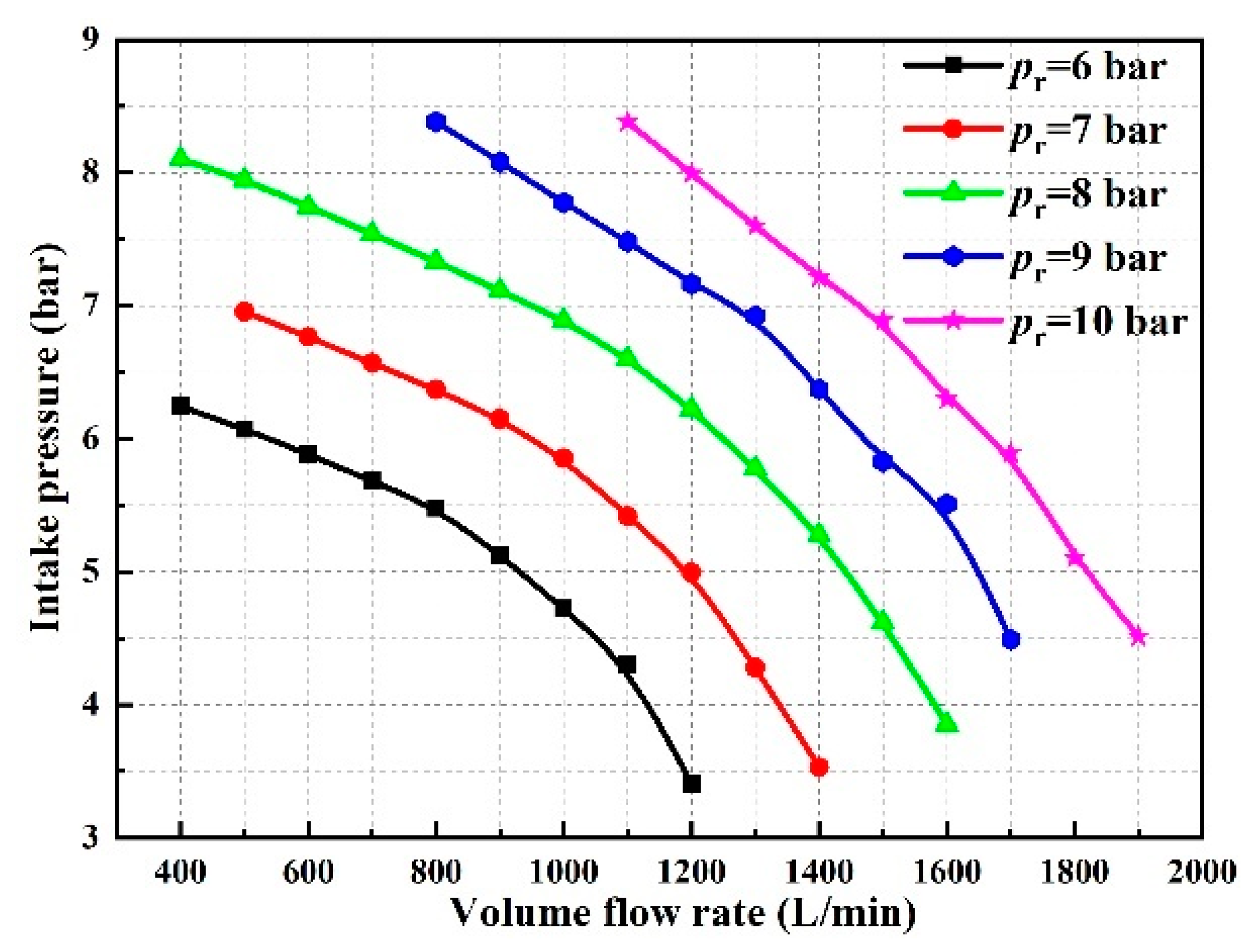
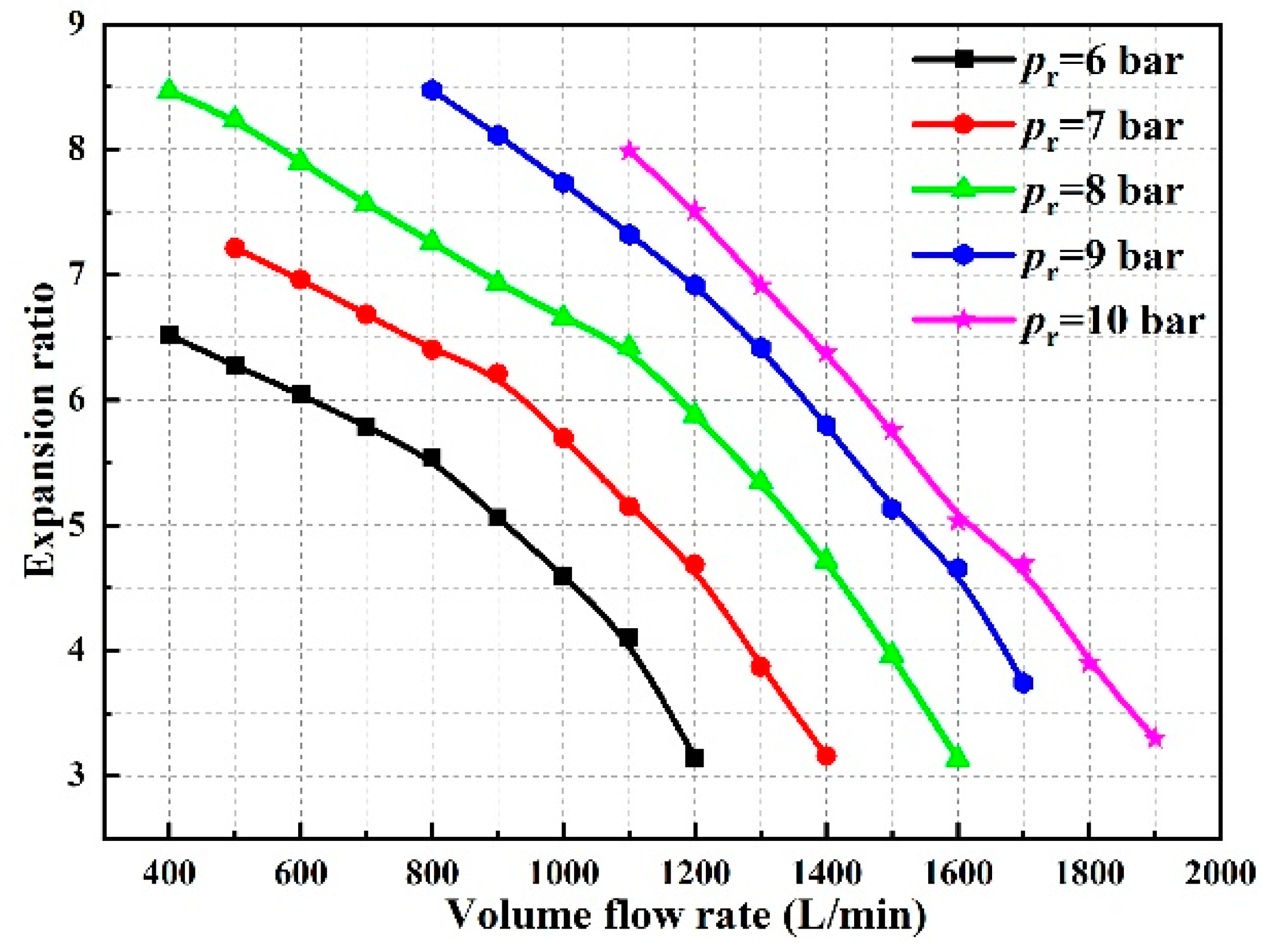
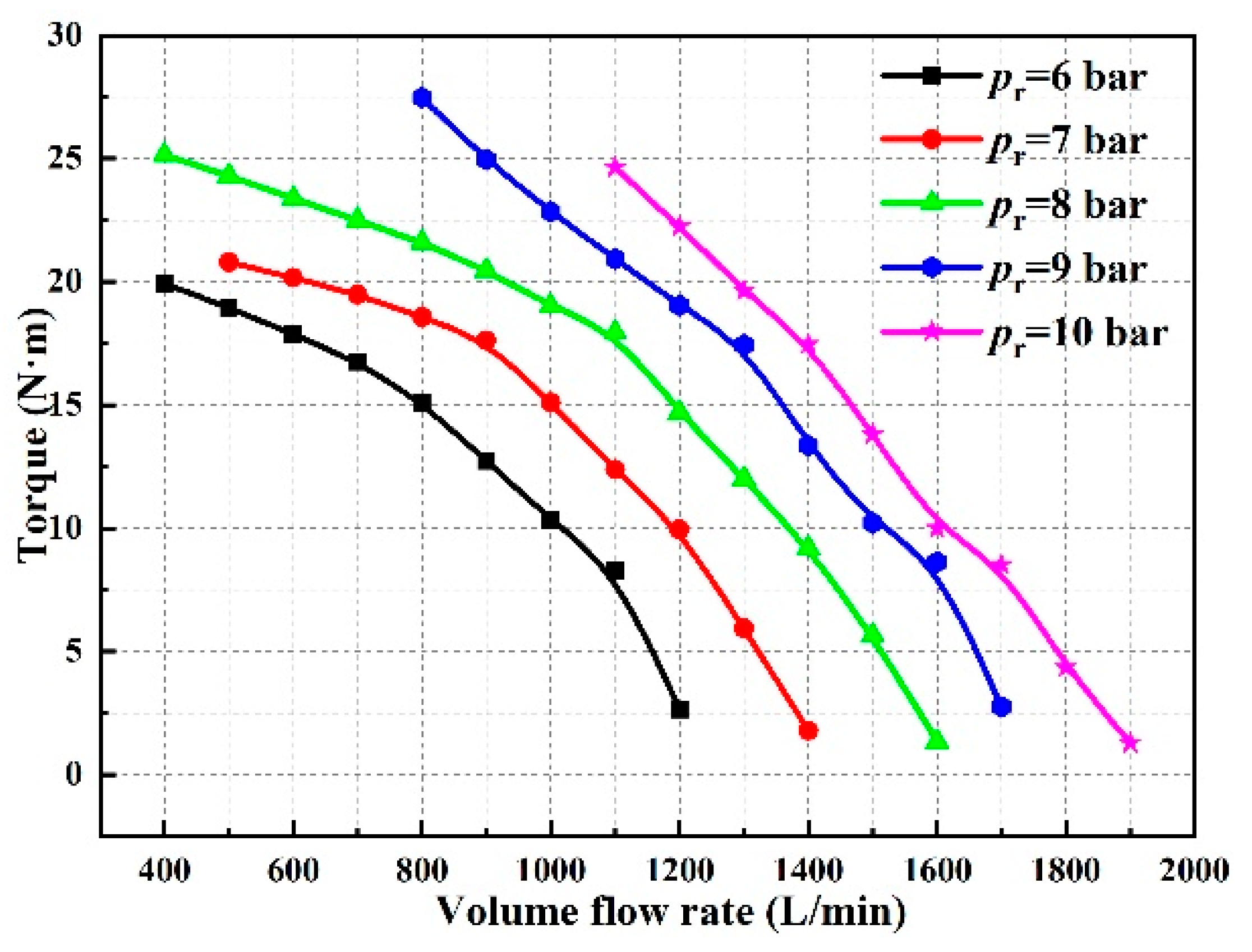
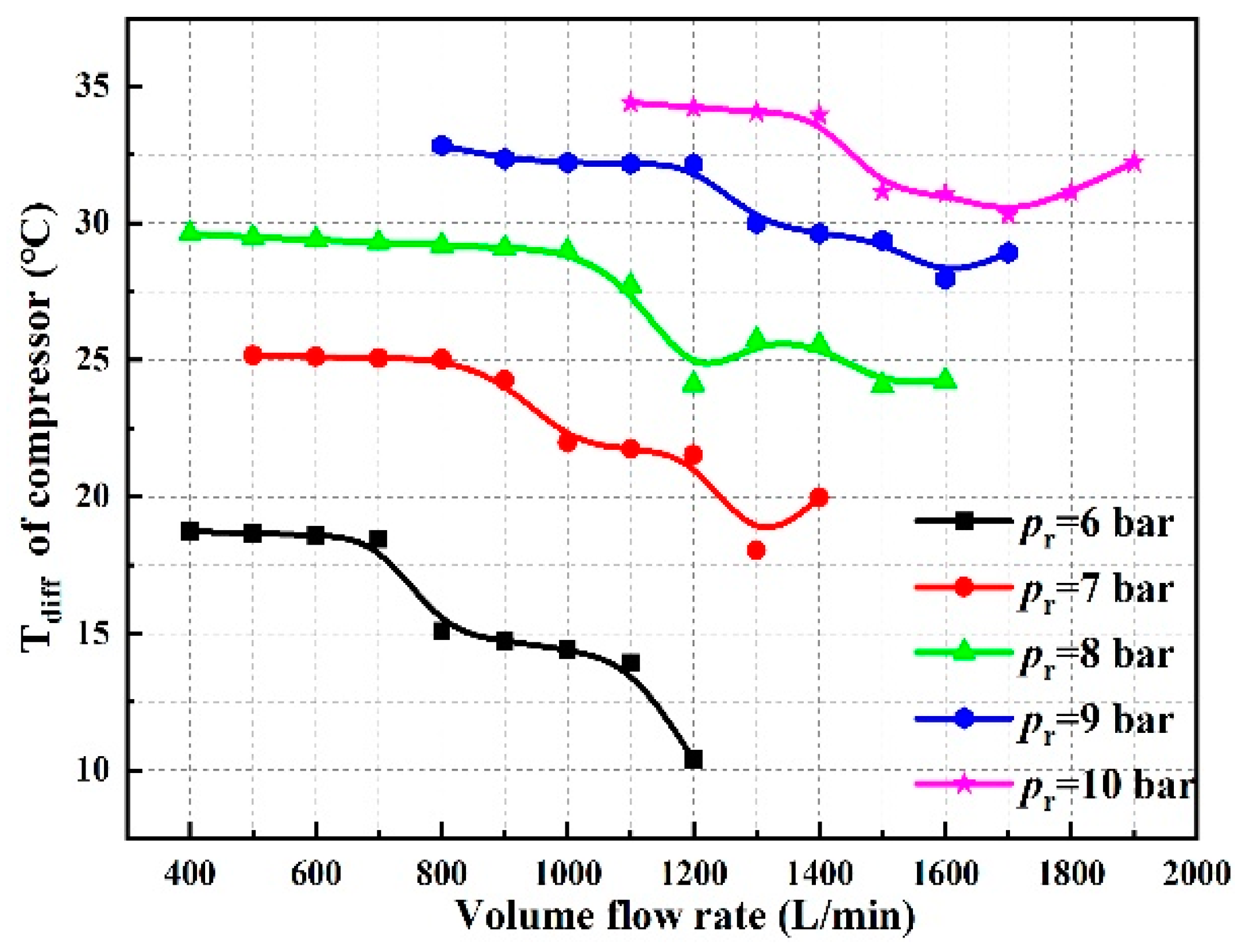
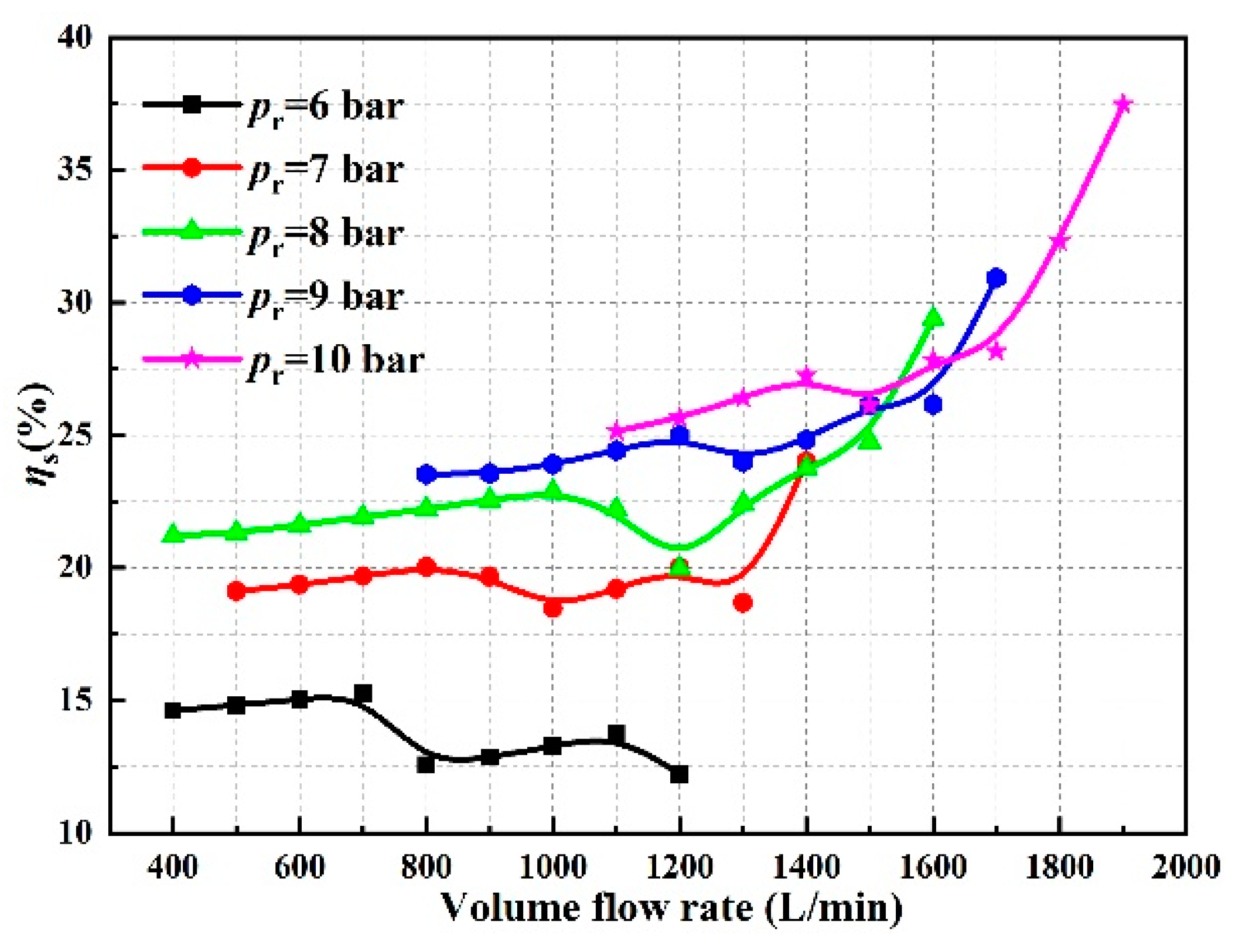
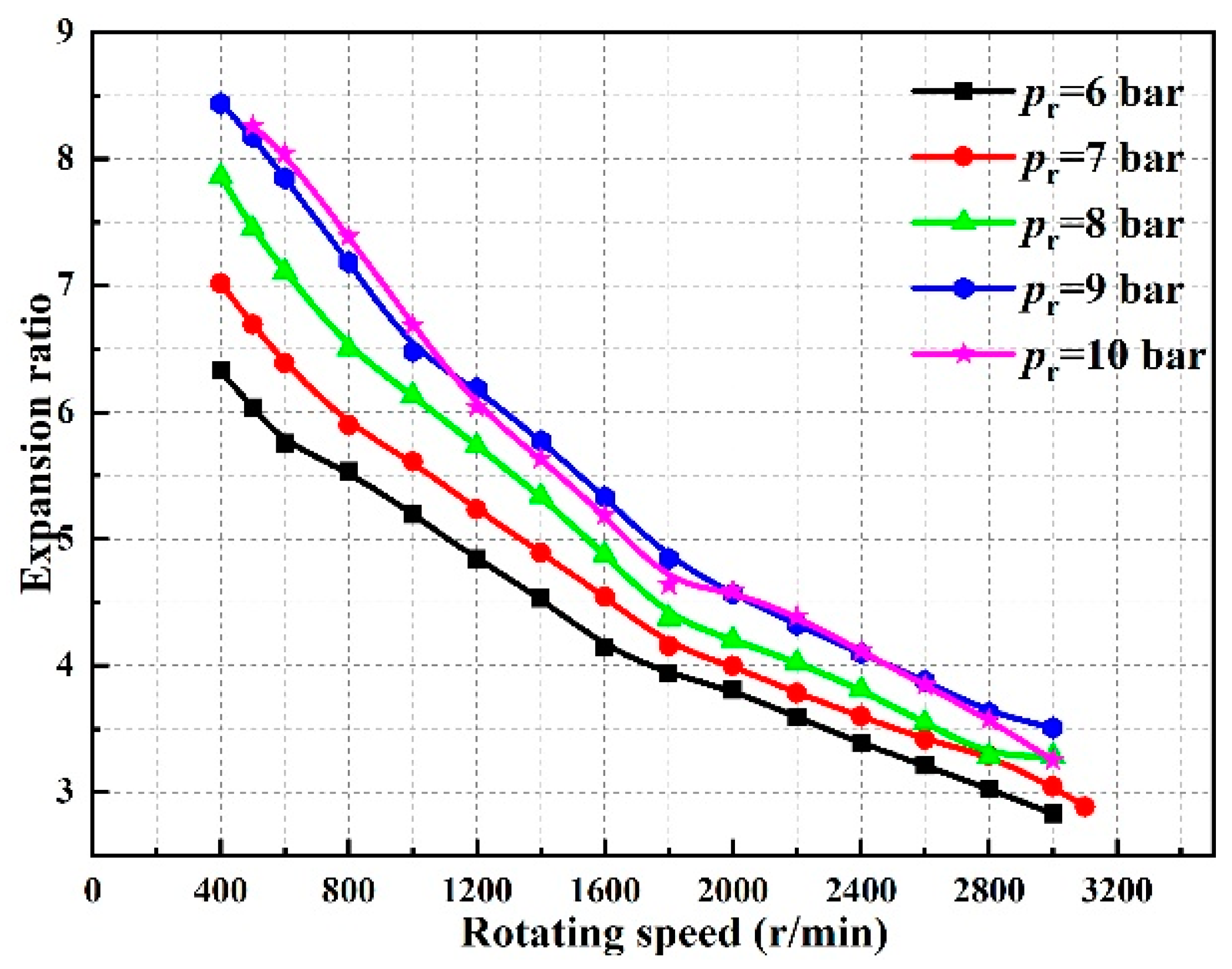
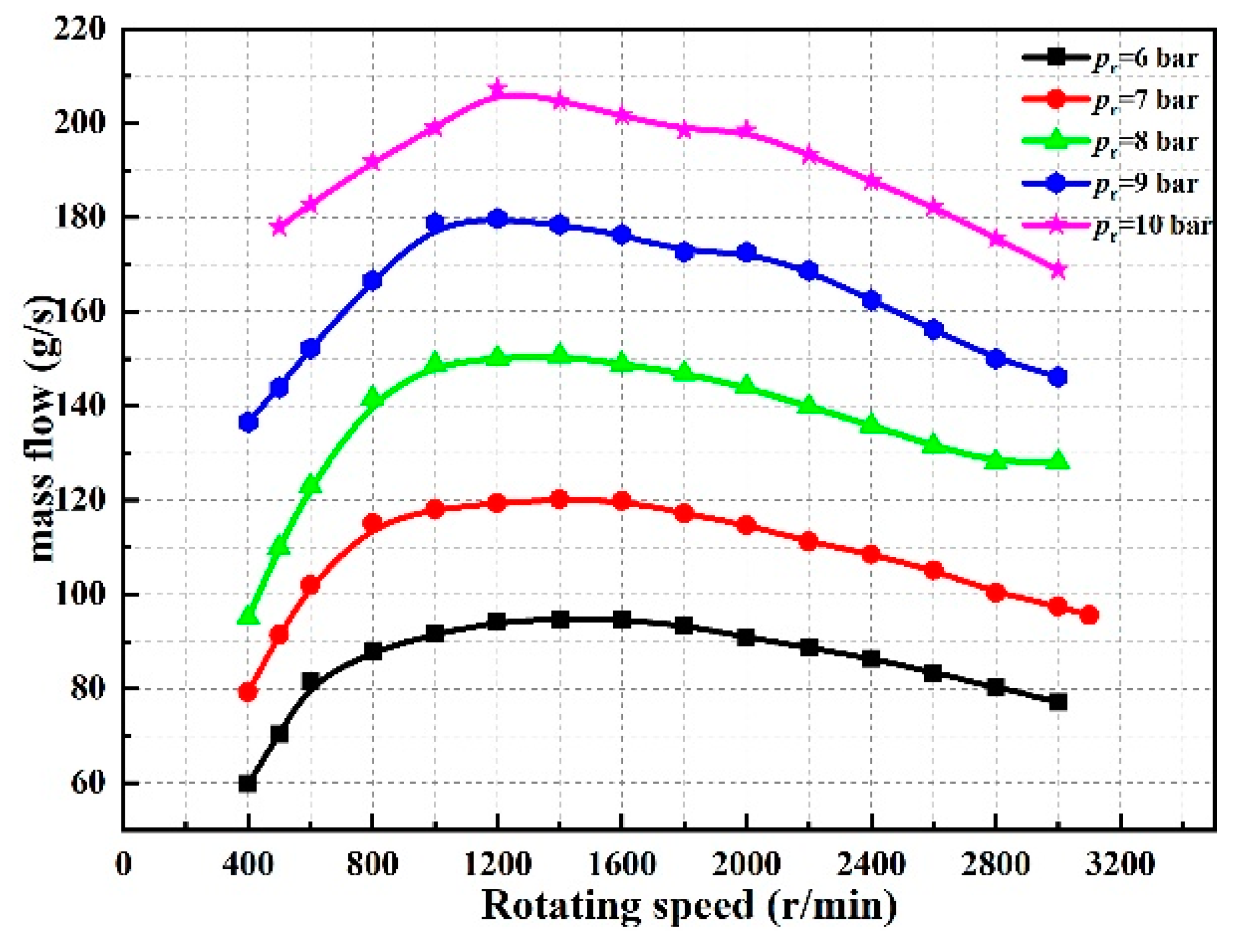
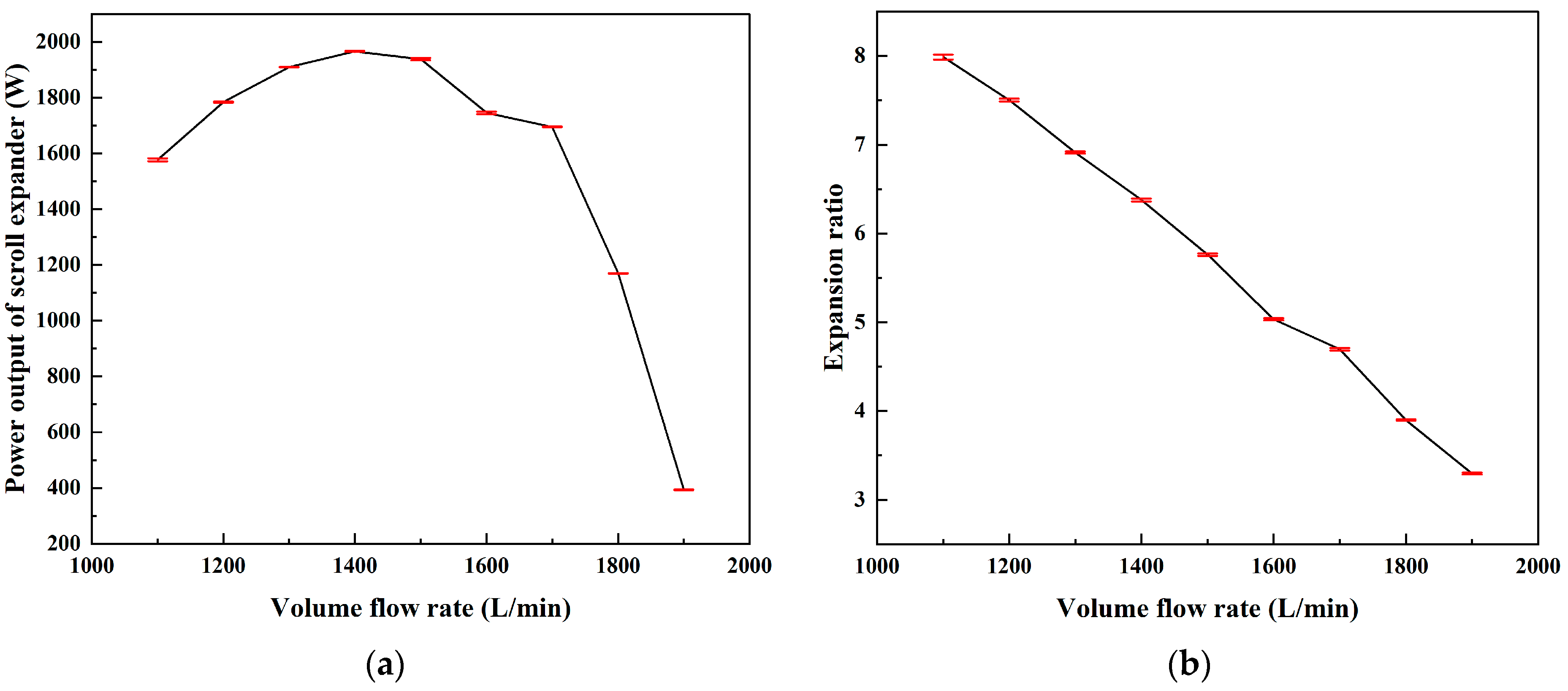
3.1. Influence of the Volume Flow Rate on the Performance of the Scroll Expander
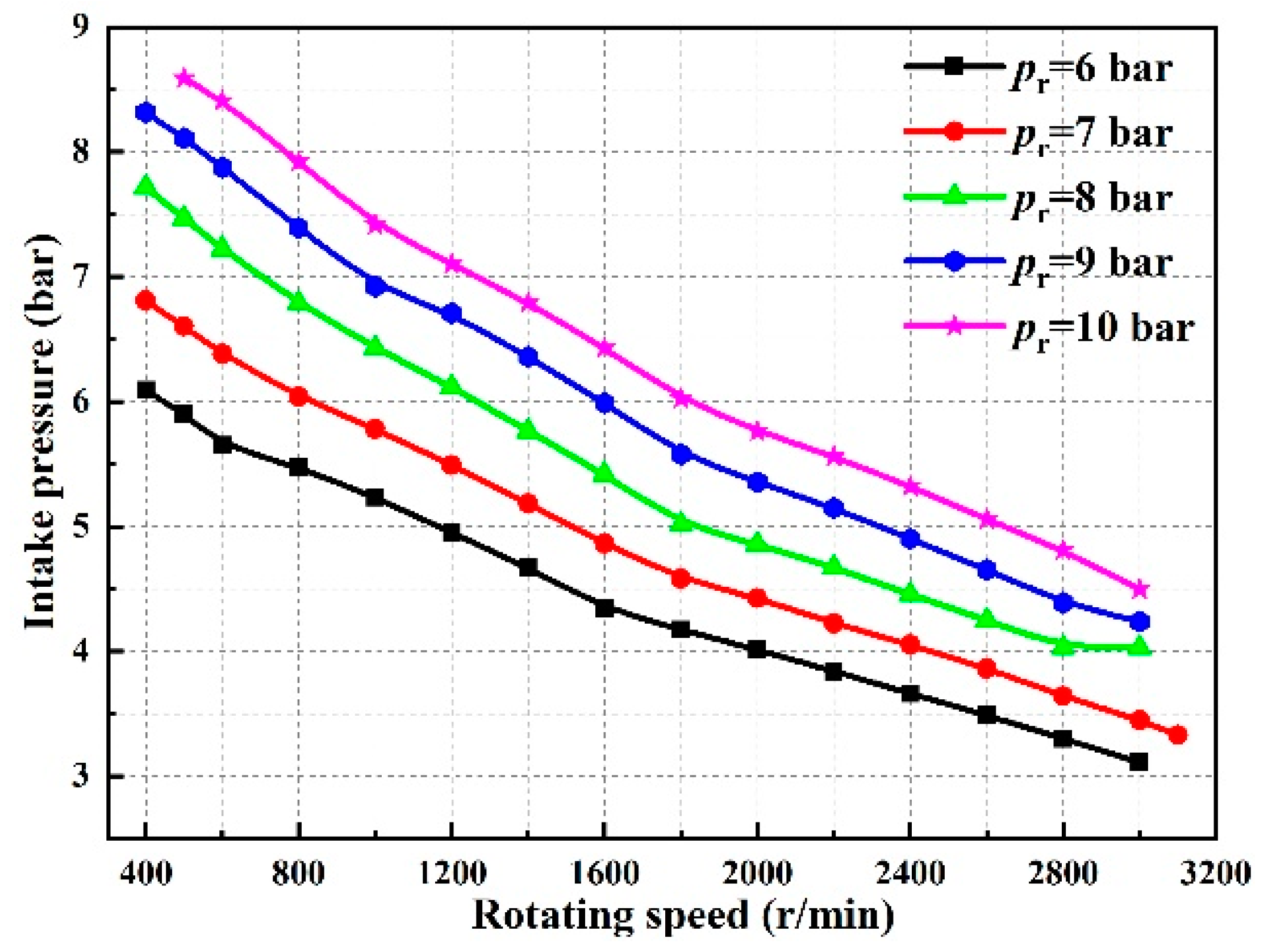
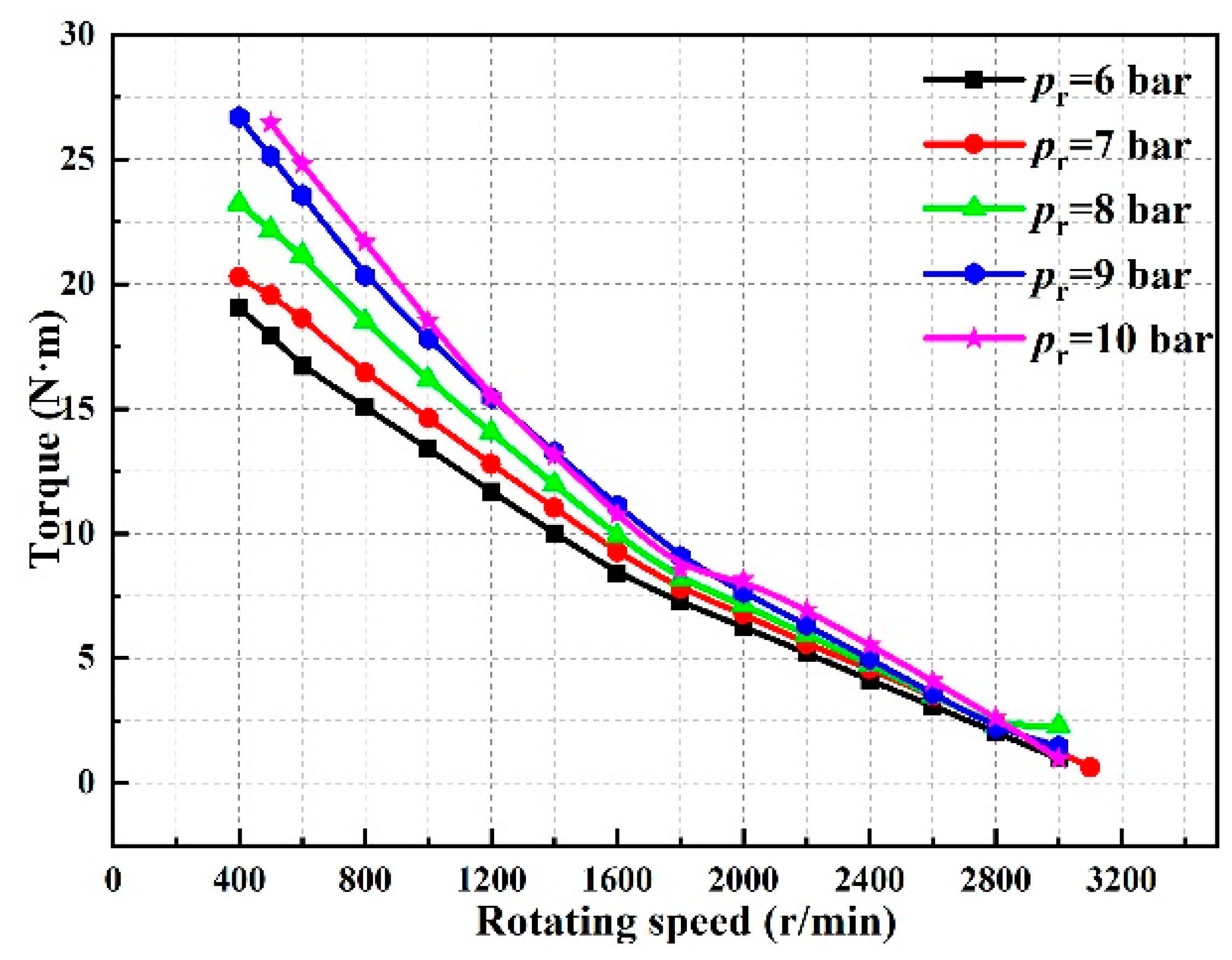
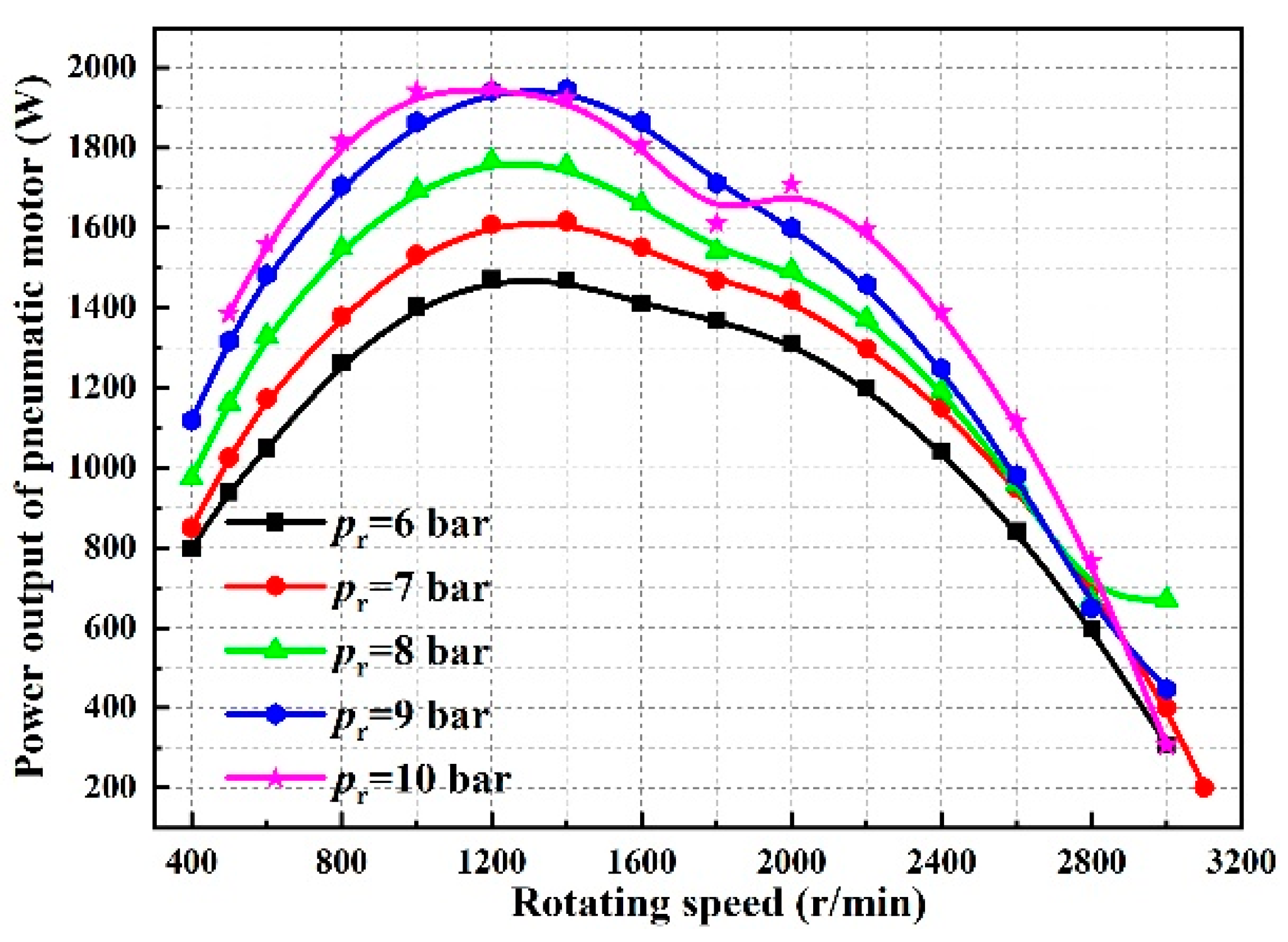
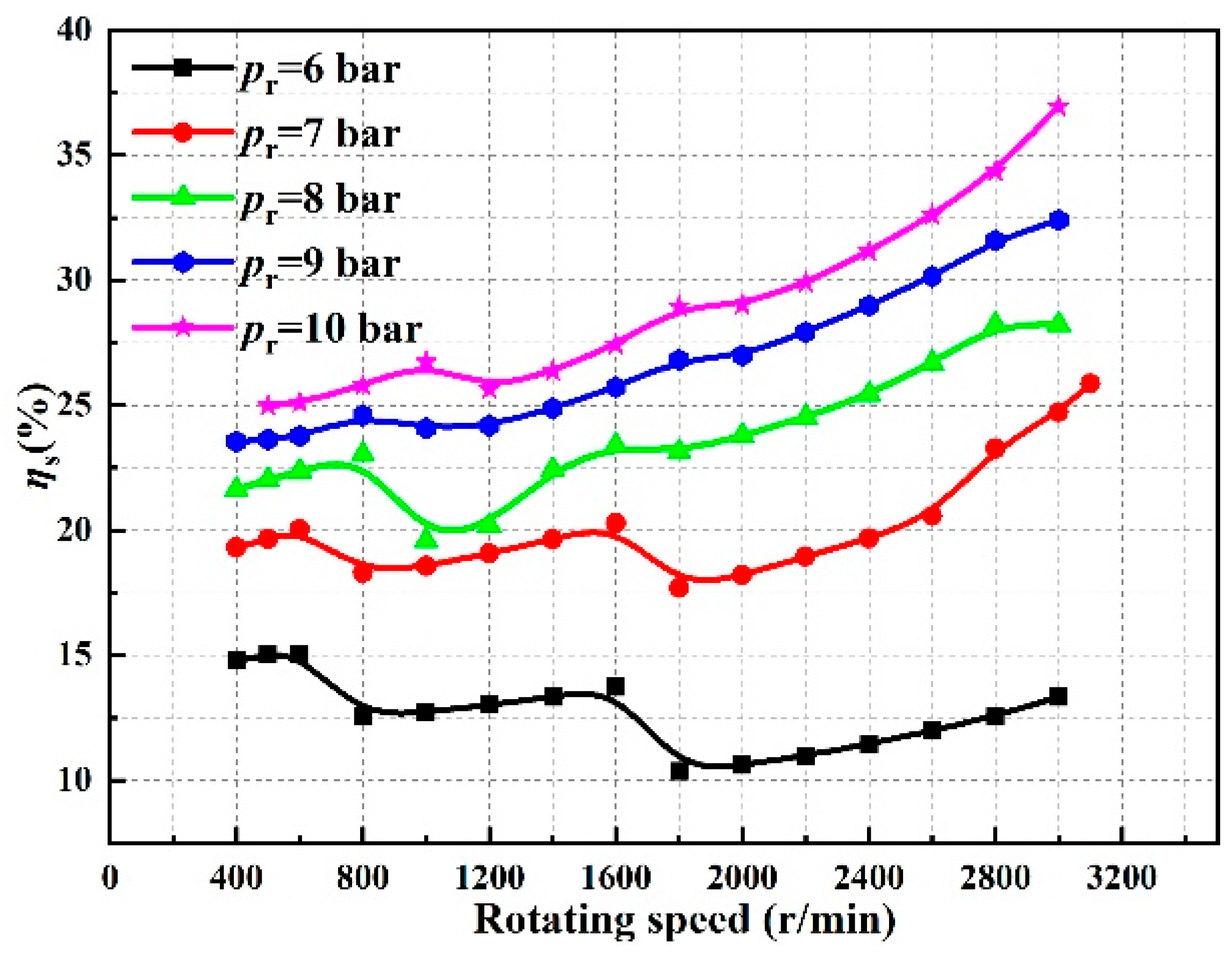
3.2. Influence of the Rotation Speed on the Performance of the Scroll Expander
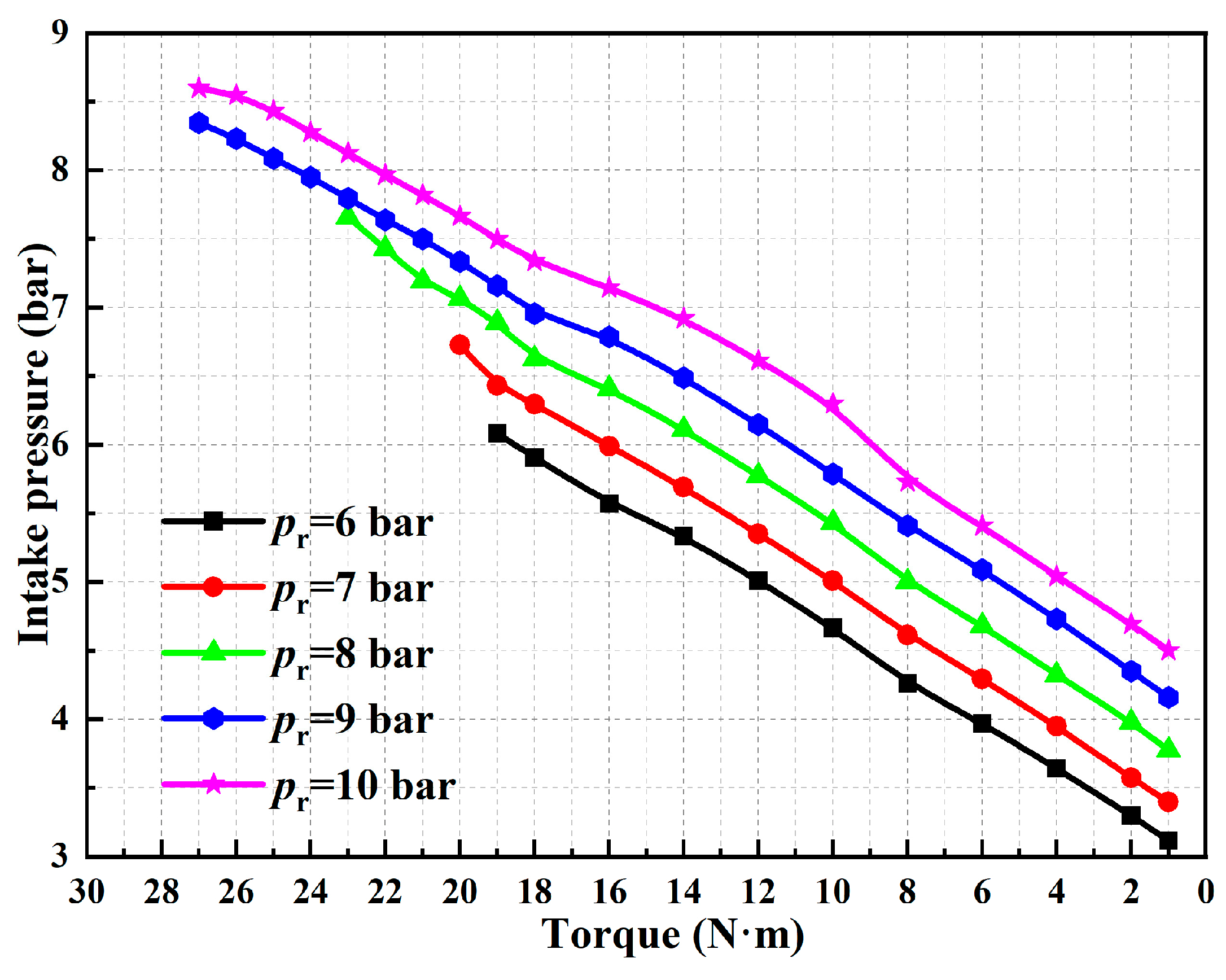
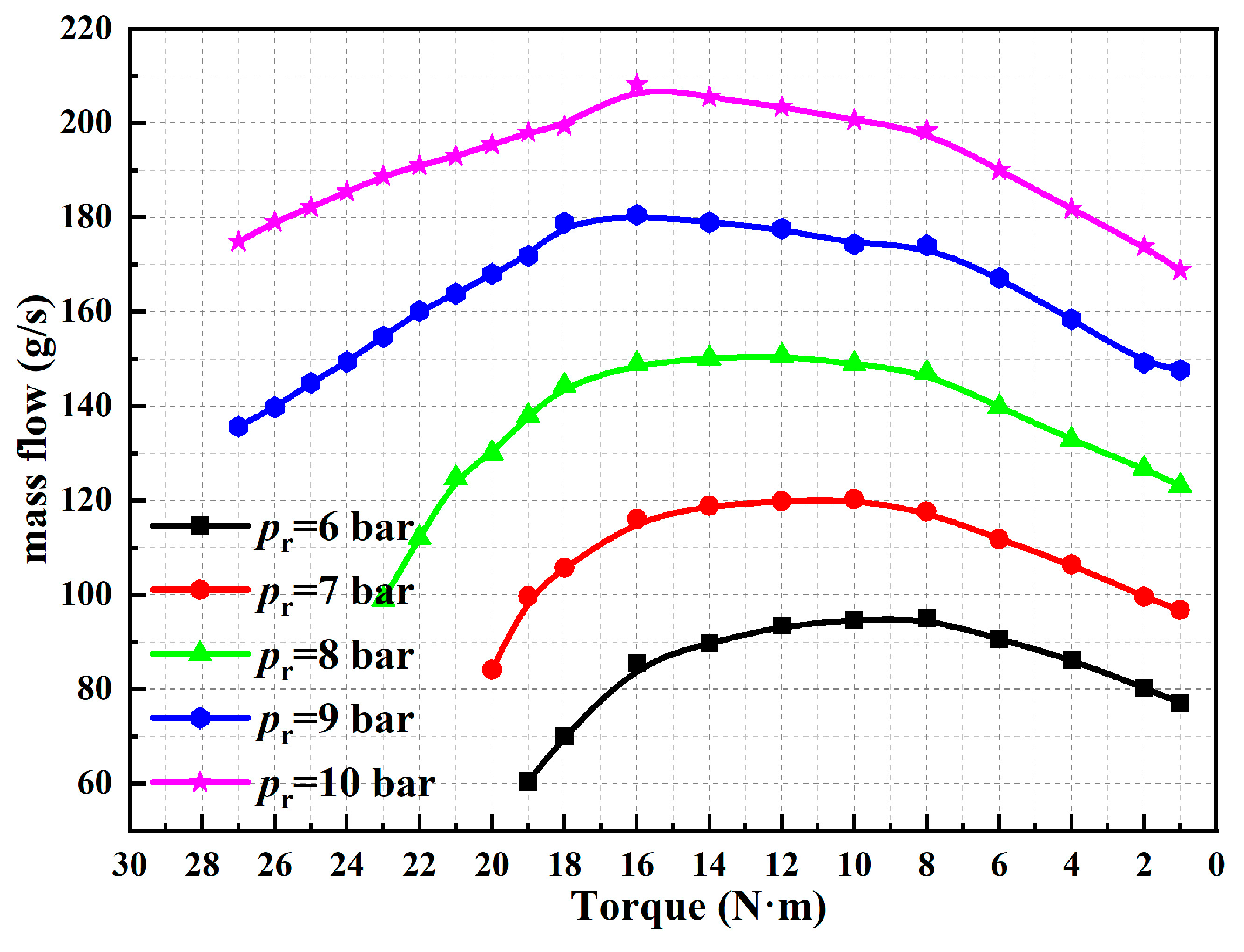
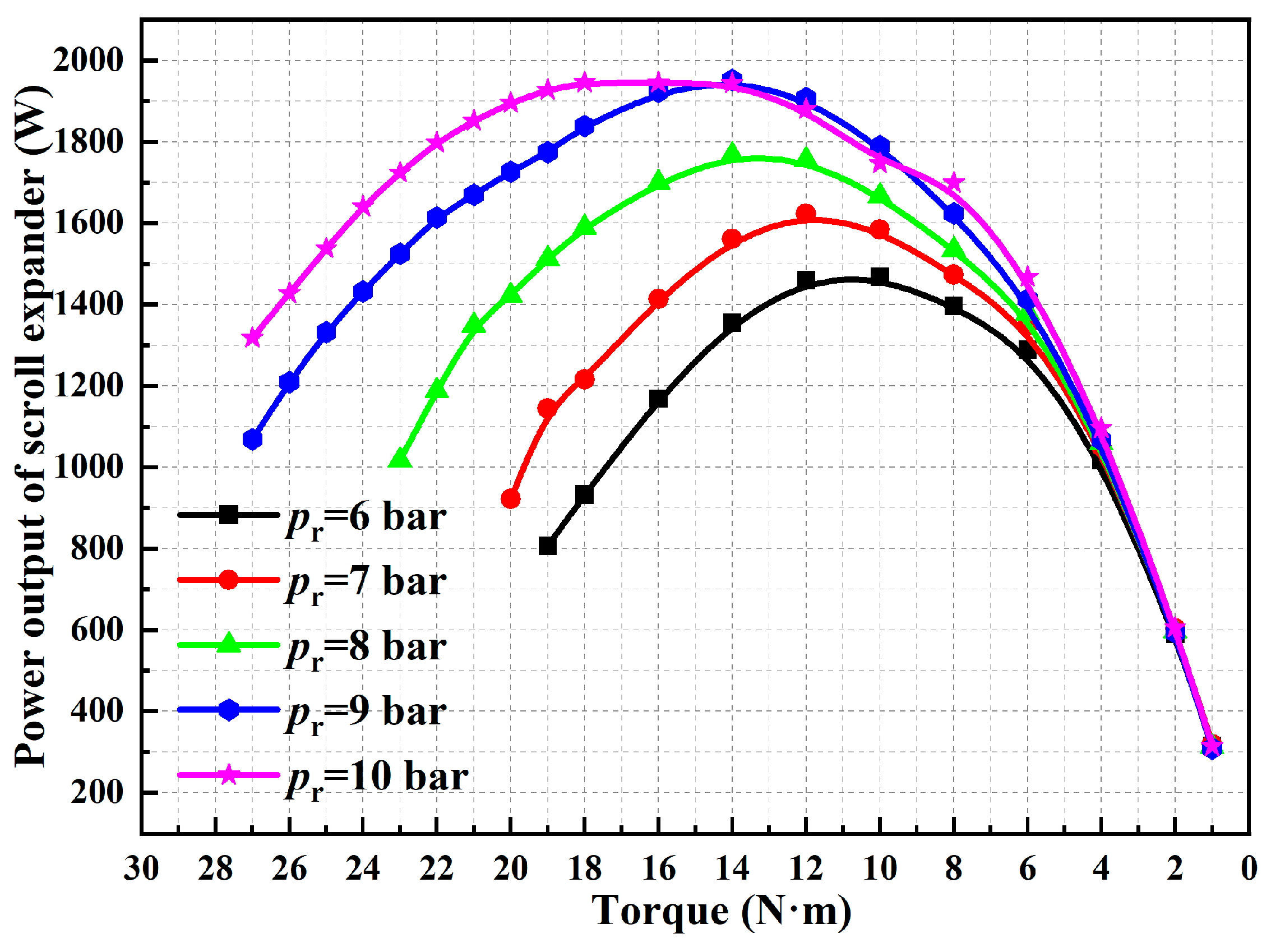
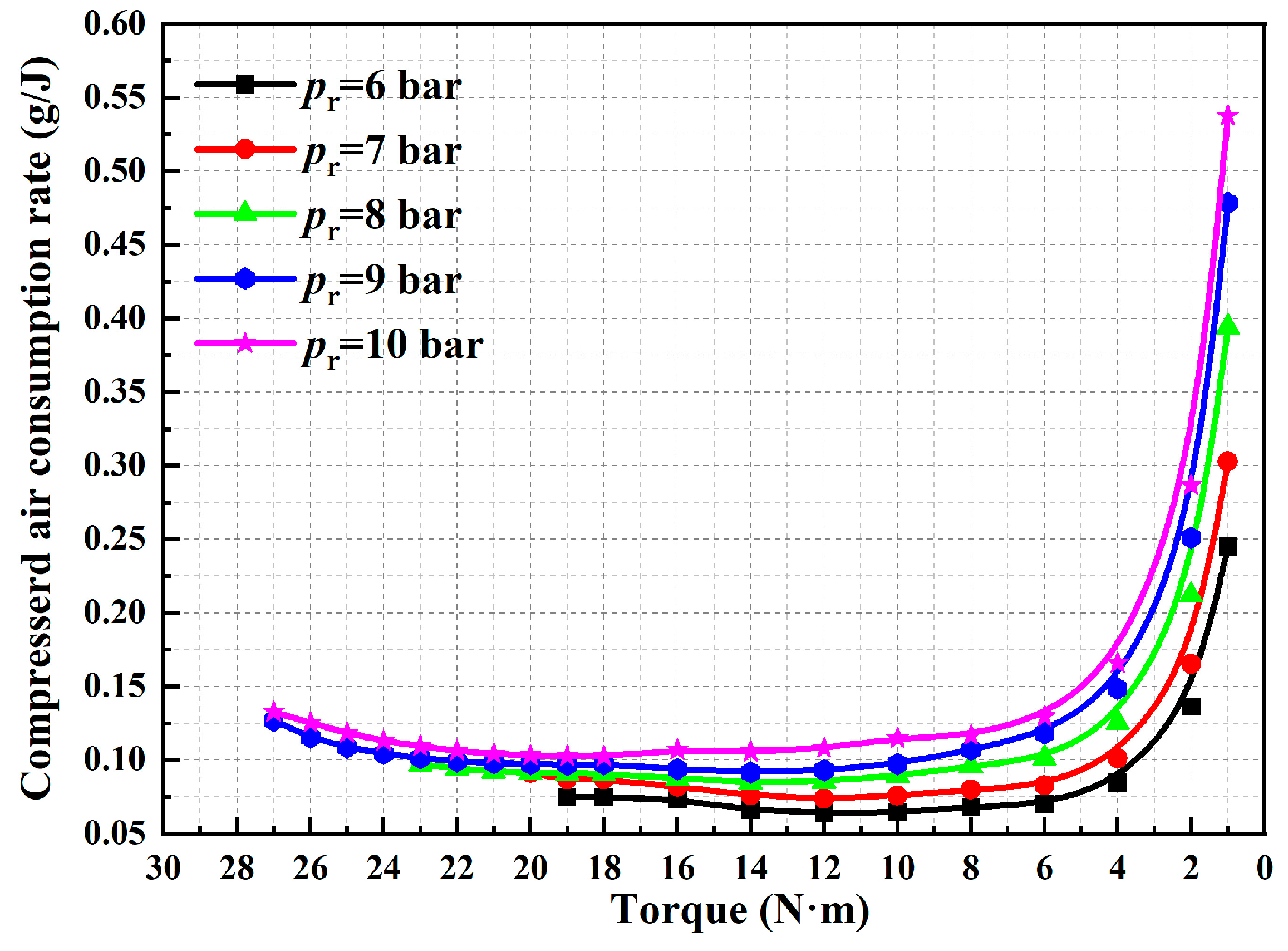
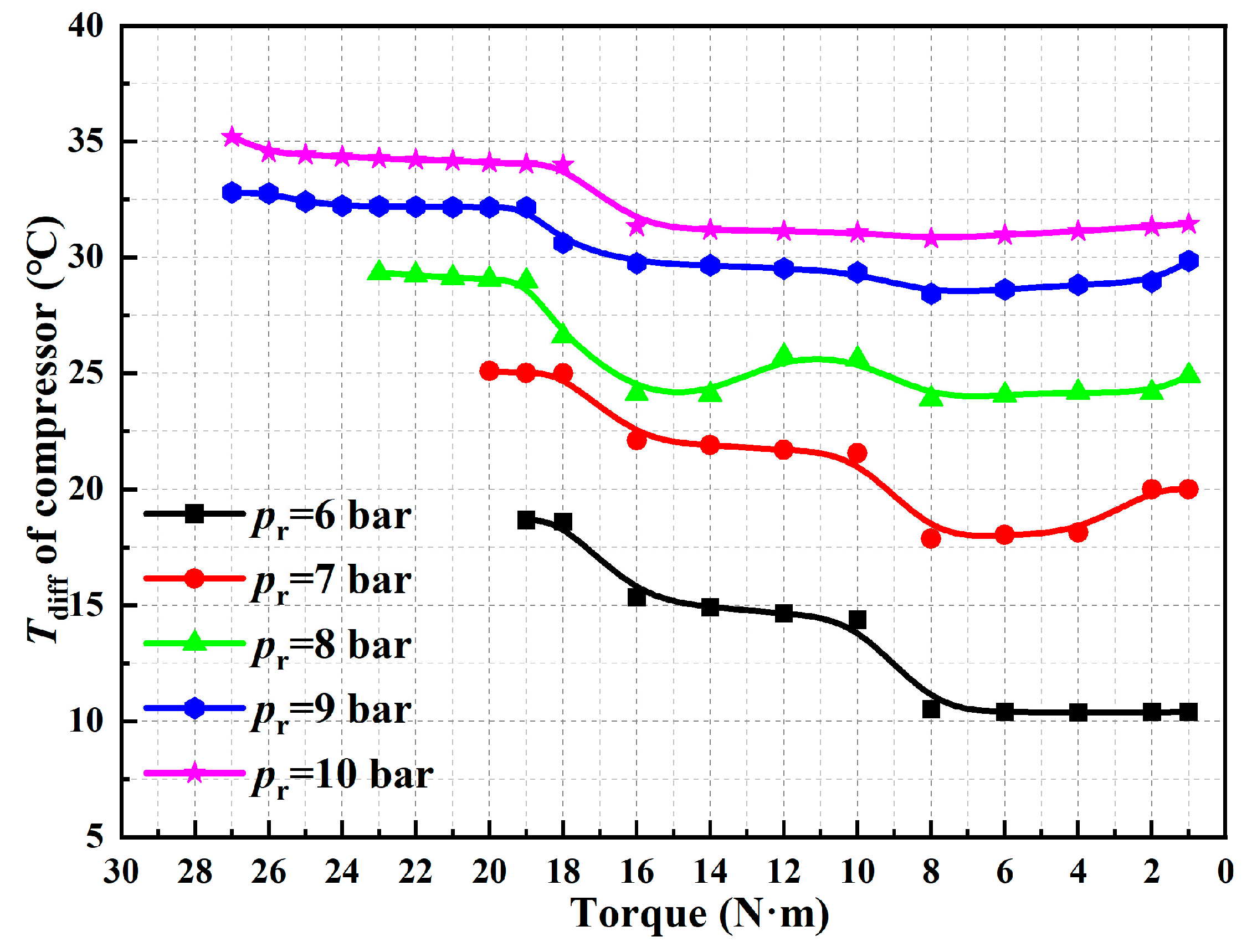
3.3. Influence of the Torque on the Performance of the Scroll Expander
3.4. Uncertainty Analysis
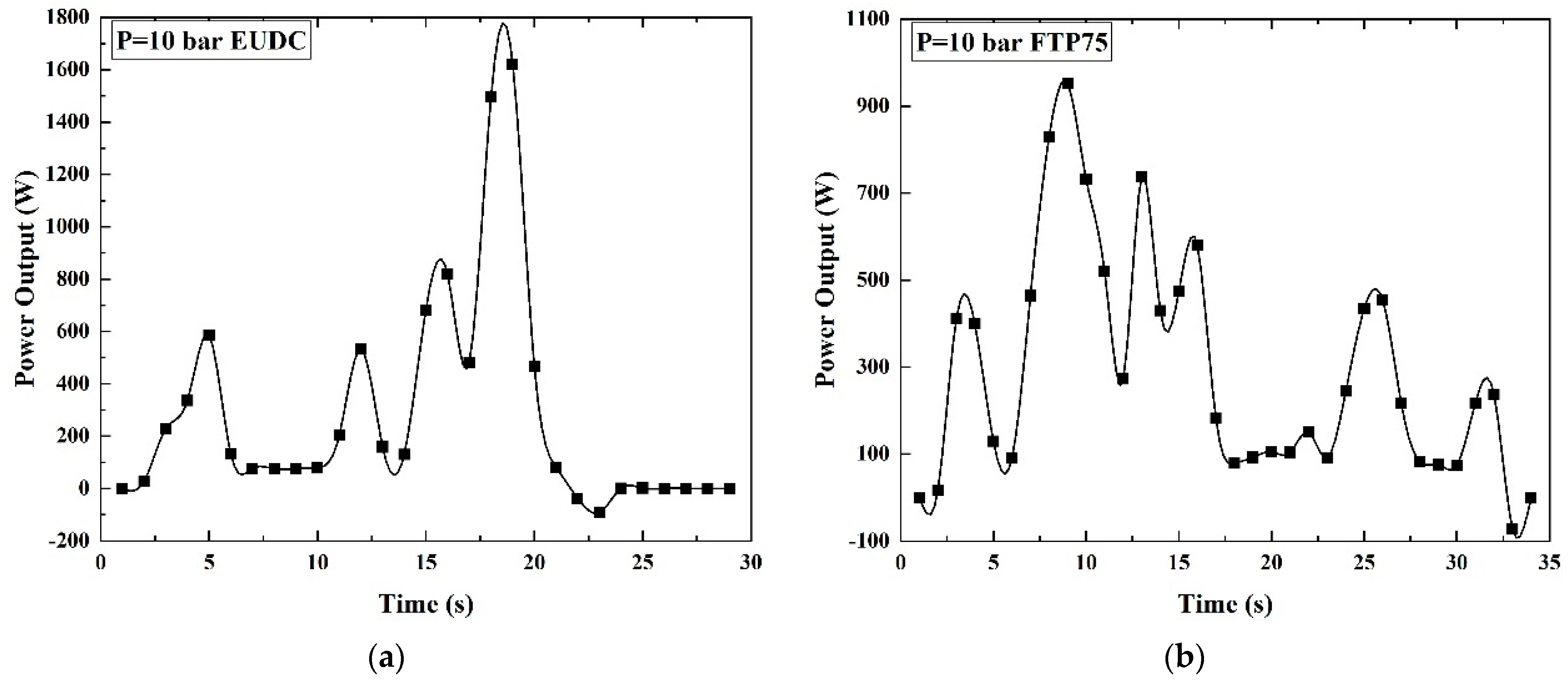
3.5. Performance of the CAES Unit under Various Driving Cycles
3.6. Economic Model
3.7. Discussion
4. Summary and Conclusions
- The larger inlet pressure results in a larger volume flow, speed, and torque. The inlet pressure decreases with the increase in the flow rate, decreases with the increase in the speed, and decreases with the decrease in the torque.
- Under different pressures of the regulator, the influence of the volume flow on the expansion ratio is obvious, and the influence of torque on the expansion ratio is the weakest.
- There is an obvious relationship between the maximum output power of the scroll expander and the pressure of the regulator. The maximum output power is 1965.23 W under the pressure of a 10 bar pressure regulator, and it is not the maximum value of the torque, speed, and volume flow.
- Larger regulator pressures can lead to a larger temperature drop as they expand, which can be used for vehicle cooling and as a vehicle air conditioner.
- When compressed air is used as vehicle power, the system cost needs to be considered, and the higher the peak power, the higher the cost. But higher inlet pressures also result in larger volumetric flows, which leads to the need for larger air reservoirs.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| intake pressure (bar) | |
| exhaust pressure (bar) | |
| rotation speed of the scroll expander (r/min) | |
| torque of the scroll expander (N·m) | |
| pressure (bar) | |
| power output of the scroll expander (W) | |
| volume (m3) | |
| mass (g) | |
| mass flow rate (g/s) | |
| CACR (g/J) | |
| enthalpy of air exiting (J/g) | |
| enthalpy of air entering (J/g) | |
| isentropic enthalpy of compressed air at the outlet (J/g) | |
| experimental data | |
| average of multiple experimental data | |
| S | parameter error |
| n | number of experimental repetitions |
| cost of the expander (USD) | |
| material factor of the expander | |
| base cost of the expander (USD) | |
| pressure factor of the expander | |
| a constant based on the type of expander | |
| a constant based on the type of expander | |
| a constant based on the type of expander | |
| power output of the scroll expander (kW) | |
| Greek letters | |
| expansion ratio | |
| isentropic efficiency | |
| Acronyms | |
| CAES | compressed air energy storage |
| PHES | pumped-hydro energy storage |
| WLTC | world-harmonized light-duty vehicle test cycle |
| NEDC | new European driving cycle |
| FTP75 | federal test procedure |
| EUDC | extra-urban driving cycle |
| CACR | compressed air consumption rate |
| CEPCI | chemical engineering plant cost index |
References
- Chang, J.W.; Li, Z.; Huang, Y.; Yu, X.N.; Jiang, R.C.; Huang, R.; Yu, X.L. Multi-objective optimization of a novel combined cooling, dehumidification and power system using improved M-PSO algorithm. Energy 2022, 239, 122487. [Google Scholar] [CrossRef]
- Li, P.; Hu, Q.Y.; Han, Z.H.; Wang, C.X.; Wang, R.X.; Han, X.; Wang, Y.Z. Thermodynamic analysis and multi-objective optimization of a trigenerative system based on compressed air energy storage under different working media and heating storage media. Energy 2022, 239, 122252. [Google Scholar] [CrossRef]
- Dib GHaberschill PRullière, R.; Perroit, Q.; Davies, S.; Revellin, R. Thermodynamic simulation of a micro advanced adiabatic compressed air energy storage for building application. Appl. Energy 2020, 260, 114248. [Google Scholar]
- Li, J.; Yang, F.B.; Zhang, H.G.; Wu, Z.; Hou, X.C.; Xu, Y.H.; Ren, J. Comparative analysis of different valve timing control methods for single-piston free piston expander-linear generator via an orthogonal experimental design. Energy 2020, 195, 116966. [Google Scholar] [CrossRef]
- Mahmoud, M.; Ramadan, M.; Olabi, A.G.; Pullen, K.; Naher, S. A review of mechanical energy storage systems combined with wind and solar applications. Energy Convers. Manag. 2020, 210, 112670. [Google Scholar] [CrossRef]
- Yu, X.L.; Jiang, R.C.; Li, Z.; Gao, Q.; Wang, B.Z.; Wang, L.; Huang, R. Synergistic improvement of melting rate and heat storage capacity by a rotation-based method for shell-and-tube latent thermal energy storage. Appl. Therm. Eng. 2023, 219, 119480. [Google Scholar] [CrossRef]
- Malozyomov, B.V.; Kukartsev, V.V.; Martyushev, N.V.; Kondratiev, V.V.; Klyuev, R.V.; Karlina, A.I. Improvement of hybrid electrode material synthesis for energy accumulators based on carbon nanotubes and porous structures. Micromachines 2023, 14, 1288. [Google Scholar] [CrossRef] [PubMed]
- Fang, J.; Wang, D.C.; Xu, H.M.; Sun, F.; Fan, Y.N.; Rong, C.; Liu, Q.B. Unleashing solar energy’s full potential: Synergetic thermo-photo catalysis for enhanced hydrogen production with metal-free carbon nitrides. Energy Convers. Manag. 2024, 300, 117995. [Google Scholar] [CrossRef]
- Koohi-Fayegh, S.; Rosen, M.A. A review of energy storage types, applications and recent developments. J. Energy Storage 2020, 27, 101047. [Google Scholar] [CrossRef]
- Alirahmi, S.M.; Mousavi, S.B.; Razmi, A.R.; Ahmadi, P. A comprehensive techno-economic analysis and multi-criteria optimization of a compressed air energy storage (CAES) hybridized with solar and desalination units. Energy Convers. Manag. 2021, 236, 114053. [Google Scholar] [CrossRef]
- He, W.; Wang, J. Optimal selection of air expansion machine in Compressed Air Energy Storage: A review. Renew. Sustain. Energy Rev. 2018, 87, 77–95. [Google Scholar] [CrossRef]
- Marvania, D.; Subudhi, S. A comprehensive review on compressed air powered engine. Renew. Sustain. Energy Rev. 2017, 70, 1119–1130. [Google Scholar] [CrossRef]
- Zahoor, A.; Mehr, F.; Mao, G.Z.; Yu, Y.J.; Sápi, A. The carbon neutrality feasibility of worldwide and in China’s transportation sector by E-car and renewable energy sources before 2060. J. Energy Storage 2023, 61, 10669. [Google Scholar] [CrossRef]
- Nayak, H.K.; Goswami, D.; Hablani, V. Technical review on study of compressed air vehicle (CAV). Int. J. Automob. Eng. 2013, 3, 81–90. [Google Scholar]
- Finkelstein, T.; Organ, A.J. Air Engines: The History, Science, and Reality of the Perfect Engine; ASME Press: New York, NY, USA, 2009. [Google Scholar]
- Thipse, S.S. Compressed air car. Tech Monit. 2008, 11, 33–37. [Google Scholar]
- Hodges, C.B. Compressed-Air Engine. U.S. Patent US953336, 29 March 1910. [Google Scholar]
- Hodges, C.B. Compressed-Air Engine. U.S. Patent US1024778, 30 April 1912. [Google Scholar]
- Verma, S.S. Latest developments of a compressed air. Glob. J. Res. Eng. 2013, 13, 1119–1130. [Google Scholar]
- Mousavi, S.; Kara, S.; Kornfeld, B. Energy efficiency of compressed air systems. Procedia CIRP 2014, 15, 313–318. [Google Scholar] [CrossRef]
- Higelin, P.; Charlet, A. Thermodynamic Cycles for a New Hybrid Pneumatic Combustion Engine Concept; SAE Technical Paper 2001-24-0033; SAE International: Warrendale, PA, USA, 2001. [Google Scholar]
- Higelin, P.; Charlet, A.; Chamaillard, Y. Thermodynamic simulation of a hybrid pneumatic-combustion engine concept. Appl. Thermodyn. 2002, 5, 1–11. [Google Scholar]
- Tai, C.; Tsao, T.C.; Levin, M.B.; Barta, G.; Schechter, M.M. Using camless valvetrain for air hybrid optimization. SAE Trans. 2003, 112, 196–210. [Google Scholar]
- Andersson, M.; Johansson, B.; Hultqvist, A. An Air Hybrid for High Power Absorption and Discharg; SAE Technical Paper 2005-01-2137; SAE International: Warrendale, PA, USA, 2005. [Google Scholar]
- Trajkovic, S.; Tunestål, P.; Johansson, B. Simulation of a Pneumatic Hybrid Powertrain with VVT in GT-Power and Comparison with Experimental Data; SAE Technical Paper 2009-01-1323; SAE International: Warrendale, PA, USA, 2009. [Google Scholar]
- Trajkovic, S.; Tunestål, P.; Johansson, B.; Carlson, U.; Höglund, A. Introductory Study of Variable Valve Actuation for Pneumatic Hybridization; SAE Technical Paper 2007-01-0288; SAE International: Warrendale, PA, USA, 2007. [Google Scholar]
- Trajkovic, S.; Tunestål, P.; Johansson, B. Investigation of different valve geometries and valve timing strategies and their effect on regenerative efficiency for a pneumatic hybrid with variable valve actuation. SAE Int. J. Fuels Lubr. 2008, 1, 1715. [Google Scholar] [CrossRef][Green Version]
- Brejaud, P.; Charlet, A.; Chamaillard, Y.; Ivanco, A.; Higelin, P. Pneumatic-combustion hybrid engine: A study of the effect of the valvetrain sophistication on pneumatic modes. Oil Gas Sci. Technol. Rev. d’IFP Energ. Nouv. 2009, 65, 27–37. [Google Scholar] [CrossRef]
- Brejaud, P.; Charlet, A.; Higelin, P. Improving the Overall Efficiency of a Pneumatic-Combustion Hybrid Engine by Adding an Intermediate Heated Tank; SAE Technical Paper 2013-24-0075; SAE International: Warrendale, PA, USA, 2013. [Google Scholar]
- Brejaud, P.; Higelin, P.; Charlet, A.; Colin, G.; Chamaillard, Y. One dimensional modeling and experimental validation of single cylinder pneumatic combustion hybrid engine. SAE Int. J. Engines 2011, 4, 2326–2337. [Google Scholar] [CrossRef]
- Fazeli, A.; Khajepour, A.; Devaud, C.; Azad, N.L. A New Air Hybrid Engine Using Throttle Control; SAE Technical Paper 2009-01-1319; SAE International: Warrendale, PA, USA, 2009. [Google Scholar]
- Bao, R.; Stobart, R. Using Pneumatic Hybrid Technology to Reduce Fuel Consumption and Eliminate Turbo-Lag; SAE Technical Paper 2013-01-1452; SAE International: Warrendale, PA, USA, 2013. [Google Scholar]
- Dimitrova, Z.; Lourdais, P.; Maréchal, F. Performance and economic optimization of an organic Rankine cycle for a gasoline hybrid pneumatic powertrain. Energy 2015, 86, 574–588. [Google Scholar] [CrossRef]
- Liu, G.B.; Zhao, Y.Y.; Li, L.S.; Shu, P.C. Simulation and experiment research on wide ranging working process of scroll expander driven by compressed air. Appl. Therm. Eng. 2010, 30, 2073–2079. [Google Scholar]
- Lemort, V.; Quoilin, S.; Cuevas, C.; Lebrun, J. Testing and modeling a scroll expander integrated into an Organic Rankine Cycle. Appl. Therm. Eng. 2009, 29, 3094–3102. [Google Scholar] [CrossRef]
- Song, P.P.; Wei, M.S.; Shi, L.; Danish, S.N.; Ma, C.C. A review of scroll expanders for organic Rankine cycle systems. Appl. Therm. Eng. 2015, 75, 54–64. [Google Scholar] [CrossRef]
- Chu, X.; Zhang, C.; Li, K.; Jing, Y. Dynamic modeling and efficiency analysis of compressed air energy storage system equipped with scroll compressor. Trans. China Electrotech. Soc. 2011, 26, 126–132. [Google Scholar]
- Clemente, S.; Micheli, D.; Reini, M.; Taccani, R. Energy efficiency analysis of organic Rankine cycles with scroll expanders for cogenerative applications. Appl. Energy 2012, 97, 792–801. [Google Scholar] [CrossRef]
- Qiu, G.Q.; Liu, H.; Riffat, S. Expanders for micro-CHP systems with organic Rankine cycle. Appl. Therm. Eng. 2011, 31, 3301–3307. [Google Scholar] [CrossRef]
- Declaye, S.; Quoilin, S.; Guillaume, L.; Lemort, V. Experimental study on an open drive scroll expander integrated into an ORC (organic Rankine cycle) system with R245fa as working fluid. Energy 2013, 55, 173–183. [Google Scholar] [CrossRef]
- Kim, H.J.; Ahn, J.M.; Cho, S.O.; Cho, K.R. Numerical simulation on scroll expander–compressor unit for CO2 trans-critical cycles. Appl. Therm. Eng. 2008, 28, 1654–1661. [Google Scholar] [CrossRef]
- Kohsokabe, H.; Koyama, M.; Tojo, K.; Matsunaga, M.; Nakayama, S. Performance characteristics of scroll expander for CO2 refrigeration cycles. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 2008; p. 1847. [Google Scholar]
- Clemente, S.; Micheli, D.; Reini, M.; Taccani, R. Bottoming organic Rankine cycle for a small scale gas turbine: A comparison of different solutions. Appl. Energy 2013, 106, 355–364. [Google Scholar] [CrossRef]
- Chen, Y.; Halm, N.P.; Groll, E.A.; Braun, J.E. Mathematical modeling of scroll Compressors-Part I: Compression process modeling. Int. J. Refrig. 2002, 25, 731–750. [Google Scholar] [CrossRef]
- Chen, Y.; Halm, N.P.; Braun, J.E.; Groll, E.A. Mathematical modeling of scroll Compressors-Part II: Overall scroll compressor modeling. Int. J. Refrig. 2002, 25, 751–764. [Google Scholar] [CrossRef]
- Wang, B.L.; Li, X.T.; Shi, W.X. A general geometrical model of scroll compressors based on discretional initial angles of involute. Int. J. Refrig. 2005, 28, 958–966. [Google Scholar] [CrossRef]
- Park, Y.C.; Kim, Y.C.; Cho, H.H. Thermodynamic analysis on the performance of a variable speed scroll compressor with refrigerant injection. Int. J. Refrig. 2002, 25, 1072–1082. [Google Scholar] [CrossRef]
- Li, X.M. Study on the Influence of Component Characteristics for Advanced Adiabatic Compressed Air Energy Storage System. Ph.D. Thesis, University of Chinese Academy of Sciences (Institute of Engineering Thermophysics), Beijing, China, 2015. [Google Scholar]
- Song, P.P.; Wei, M.S.; Liu, Z.; Zhao, B. Effects of suction port arrangements on a scroll expander for a small scale ORC system based on CFD approach. Appl. Energy 2015, 150, 274–285. [Google Scholar] [CrossRef]
- Li, J.; Peng, X.Y.; Yang, Z.; Hu, S.Z.; Duan, Y.Y. Design, improvements and applications of dual-pressure evaporation organic Rankine cycles: A review. Appl. Energy 2022, 311, 118609. [Google Scholar] [CrossRef]
- Yang, X.H. Performance Study of Scroll Compressor/Expander Composite Machine in Compressed Air Energy Storage. Ph.D. Thesis, East China University of Science and Technology, Shanghai, China, 2014. [Google Scholar]
- Wu, Z.; Yan, J.; Xie, F.B.; Zhu, T.; Gao, N.P. Research on the effect of suction port shape on a scroll expander pressure loss during the suction process. Compress. Technol. 2016, 1, 1–6. [Google Scholar]
- Ping, X.; Zhang, H.G.; Xing, C.D.; Pan, Y.C.; Zhang, W.J.; Wang, Y. Nonlinear modeling and multi-scale influence characteristics analysis of organic Rankine cycle (ORC) system considering variable driving cycle. Energy 2023, 265, 126311. [Google Scholar] [CrossRef]
- Xu, Y.H.; Wang, X.; Zhang, J.; Zhang, H.G.; Yang, F.B.; Yan, D.; Liang, J.; Yang, H.L.; Wang, Y. Experimental investigation and artificial neural network prediction of small-scale compressed air energy storage system based on pneumatic motor. Therm. Sci. Eng. Prog. 2024, 47, 102287. [Google Scholar] [CrossRef]
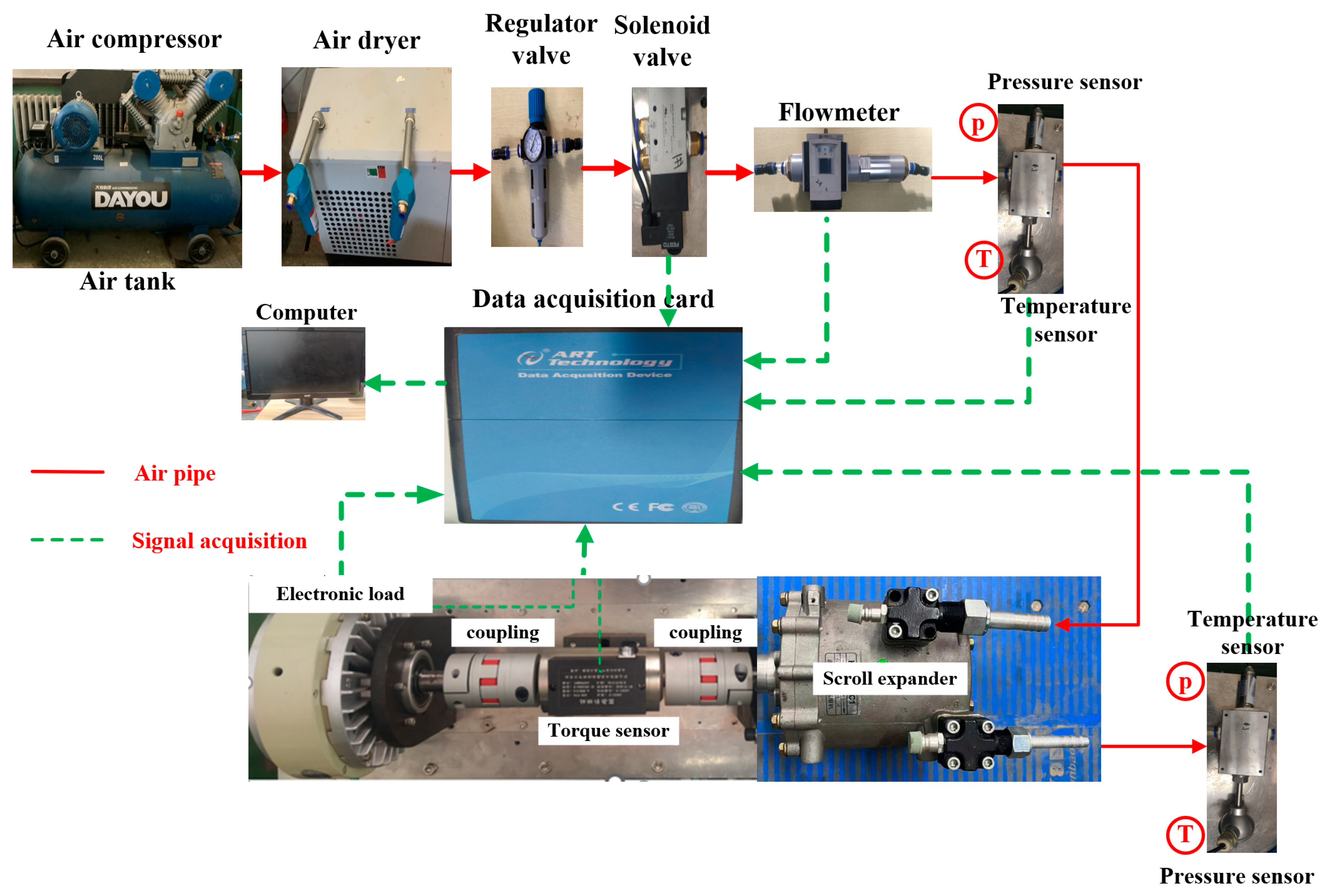
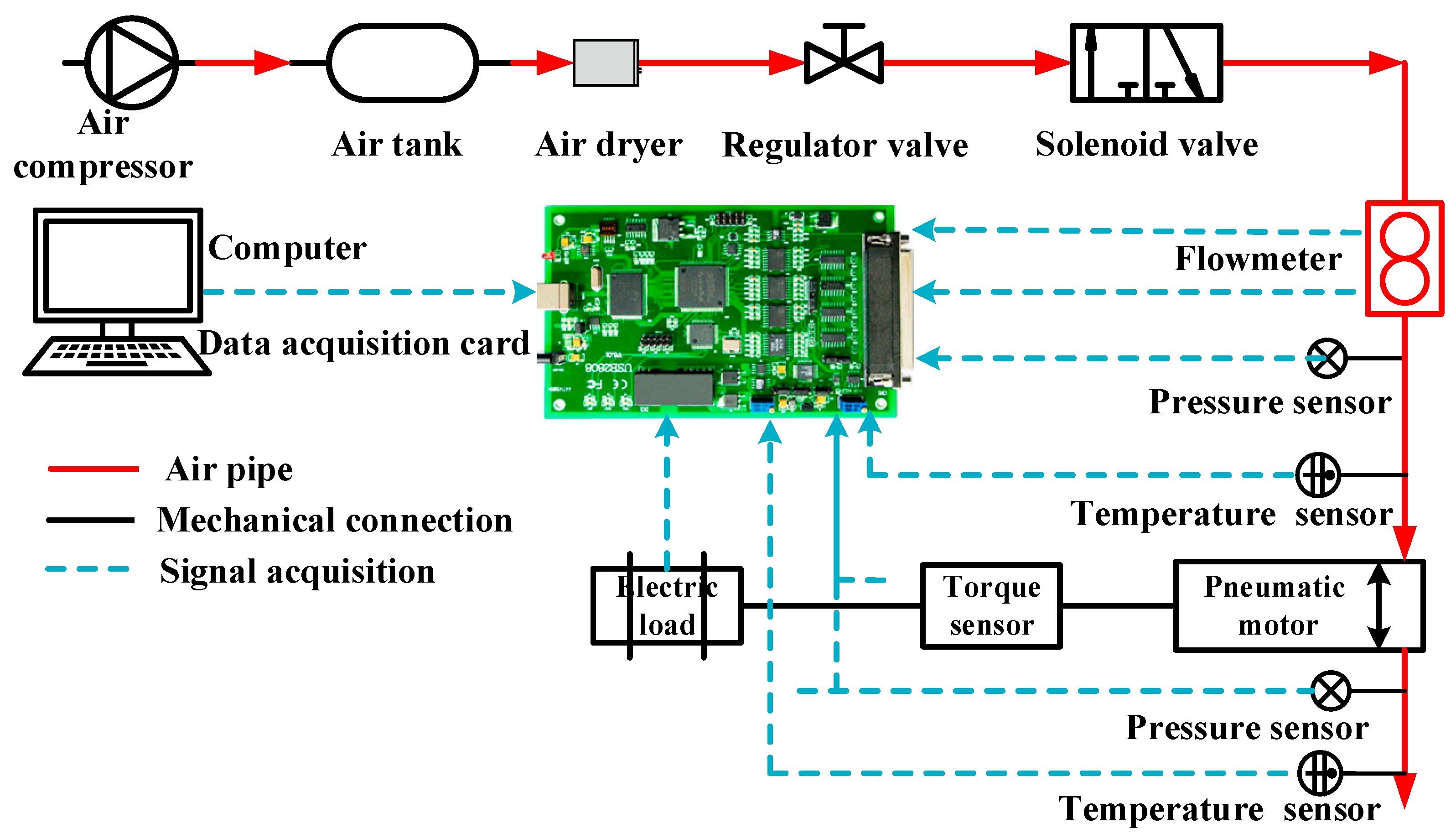











| Name | Type | Parameter |
|---|---|---|
| Scroll expander | KWX-40-C1 | |
| Rated rotation speed | 2000 r/min | |
| Rated power | 485 W | |
| Rated torque | 7.0 N·m | |
| Magnetic powder brake | FZ-50-D | |
| Rated voltage | 24 V | |
| Rated current | 2.0 A | |
| Rated torque | 50.0 N·m |
| Name | Measure Range | Tolerance |
|---|---|---|
| Pressure sensor | 0~15 bar | ±0.2% FS |
| Temperature sensor | −20~100 °C | ±0.5% FS |
| Torque sensor | 0~20 N·m | ±0.5% FS |
| Rotation speed sensor | 0~6000 r/min | ±0.5% FS |
| Flowmeter | 0~5000 L/min | ±0.5% FS |
| Name | Parameter |
|---|---|
| FS | 1.7 |
| FMP | 3.5 |
| K1,EXP | 2.2659 |
| K2,EXP | 1.4398 |
| K3,EXP | −0.1776 |
| CEPCI2023 | 813.0 |
| CEPCI2020 | 596.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, H.; Xu, Y.; Zhong, X.; Zeng, J.; Yang, F. Experimental Investigation on the Performance of the Scroll Expander under Various Driving Cycles. Energies 2024, 17, 433. https://doi.org/10.3390/en17020433
Yang H, Xu Y, Zhong X, Zeng J, Yang F. Experimental Investigation on the Performance of the Scroll Expander under Various Driving Cycles. Energies. 2024; 17(2):433. https://doi.org/10.3390/en17020433
Chicago/Turabian StyleYang, Hailong, Yonghong Xu, Xiaohui Zhong, Jiajun Zeng, and Fubin Yang. 2024. "Experimental Investigation on the Performance of the Scroll Expander under Various Driving Cycles" Energies 17, no. 2: 433. https://doi.org/10.3390/en17020433
APA StyleYang, H., Xu, Y., Zhong, X., Zeng, J., & Yang, F. (2024). Experimental Investigation on the Performance of the Scroll Expander under Various Driving Cycles. Energies, 17(2), 433. https://doi.org/10.3390/en17020433







