Simple Design Scheme for a 300 kW Modular NPC Inverter Using Thermal RC Network Analysis
Abstract
1. Introduction
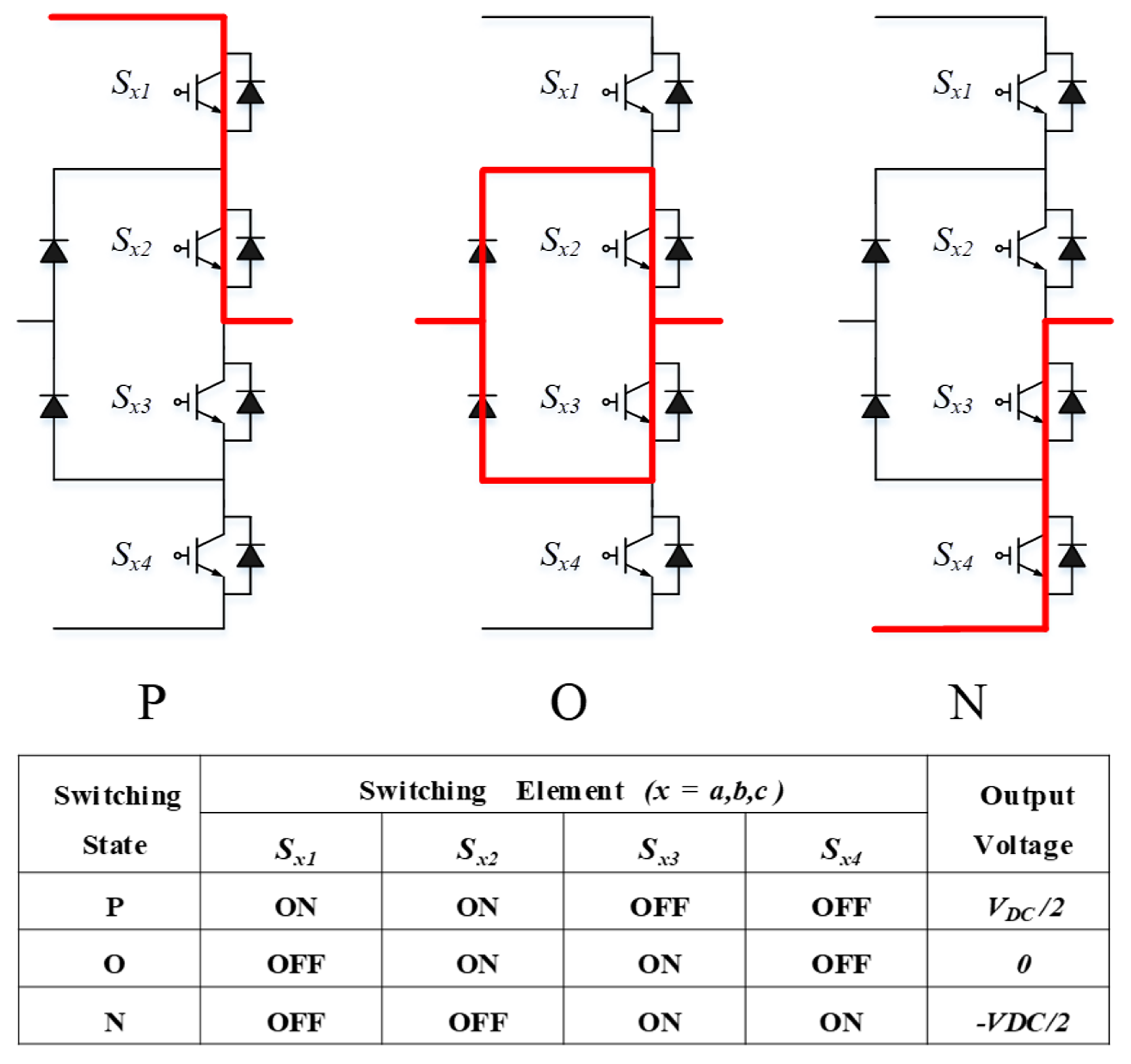
2. The 3-Level NPC Inverter
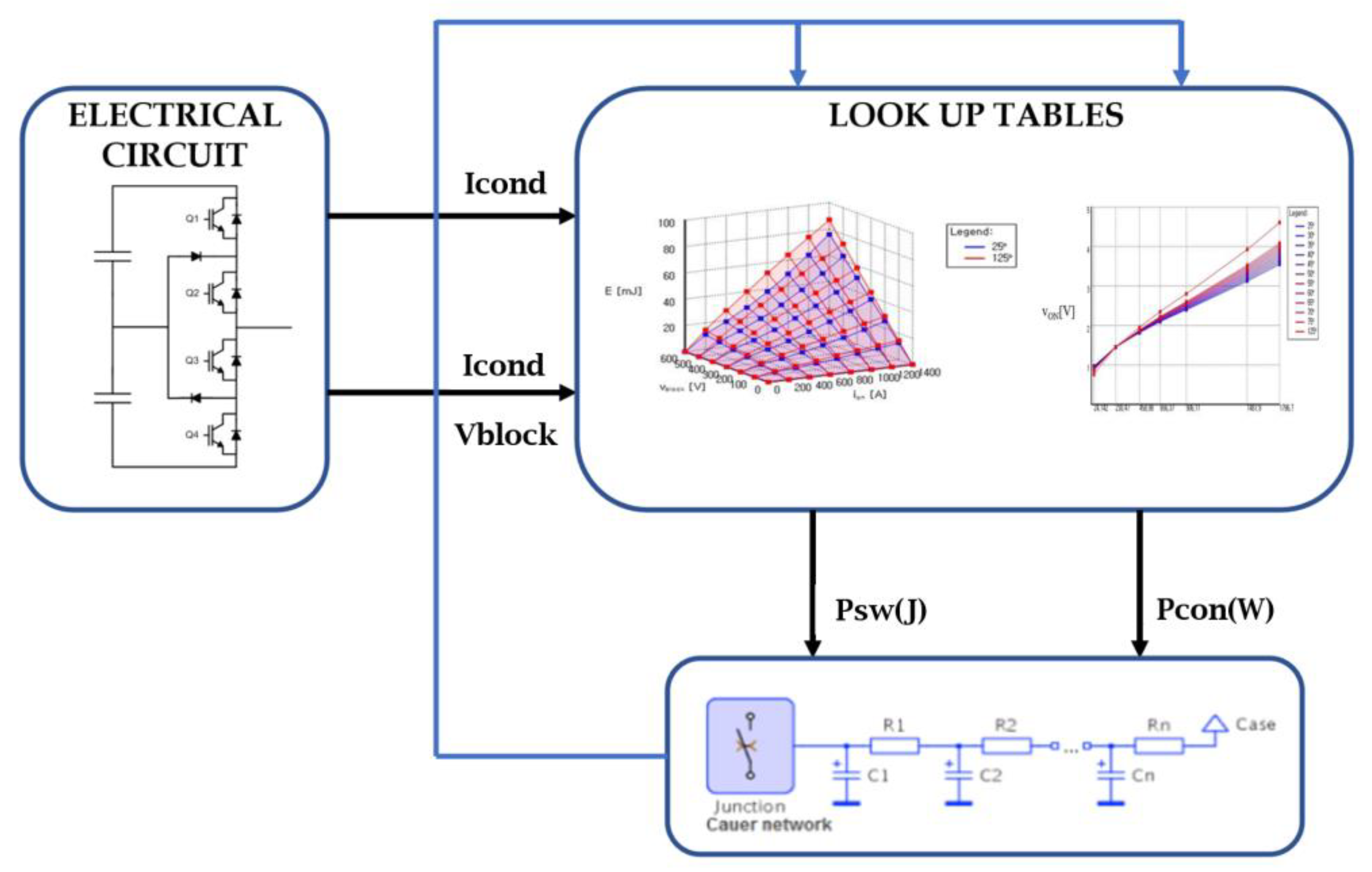
3. Inverter Heat Dissipation Design Model
3.1. Conduction Loss
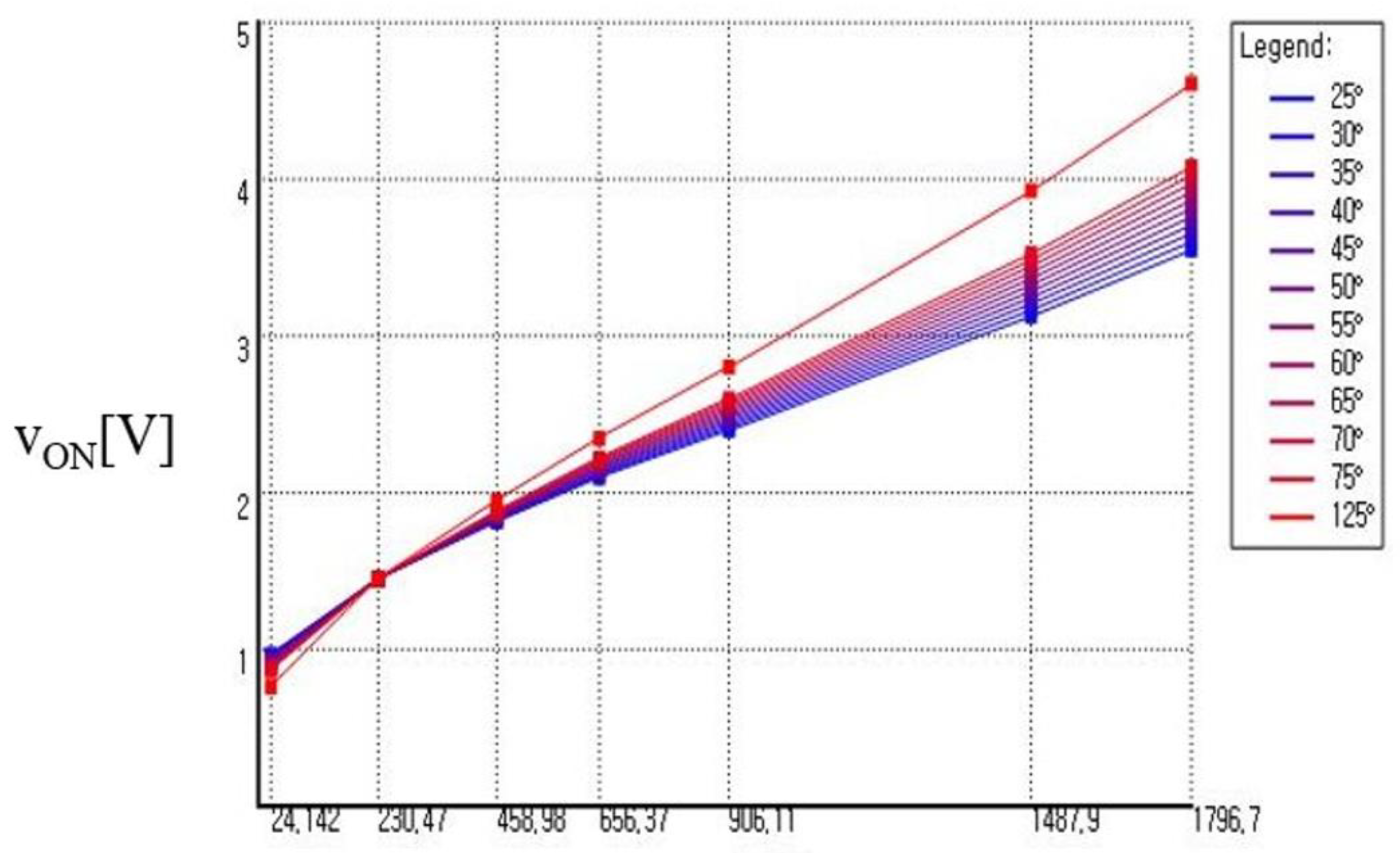
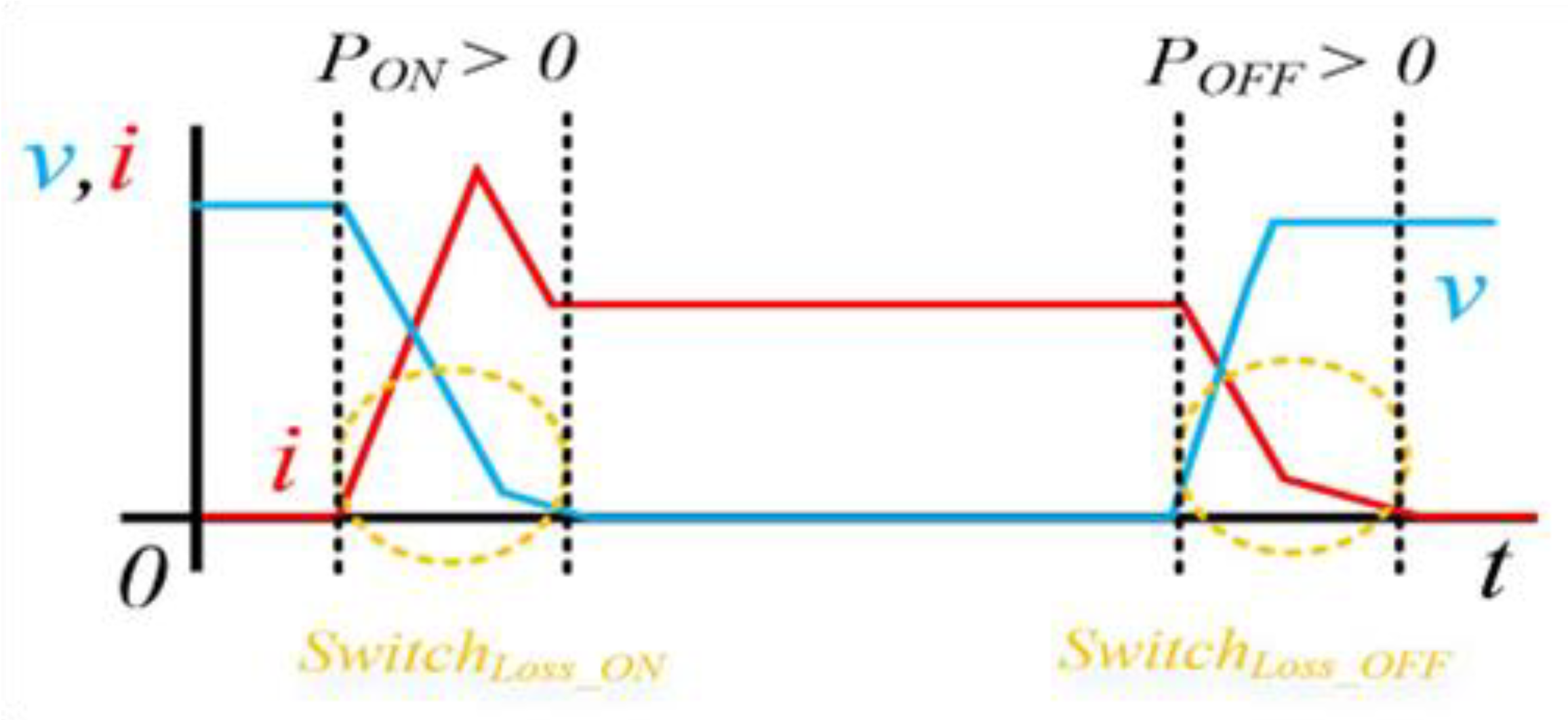
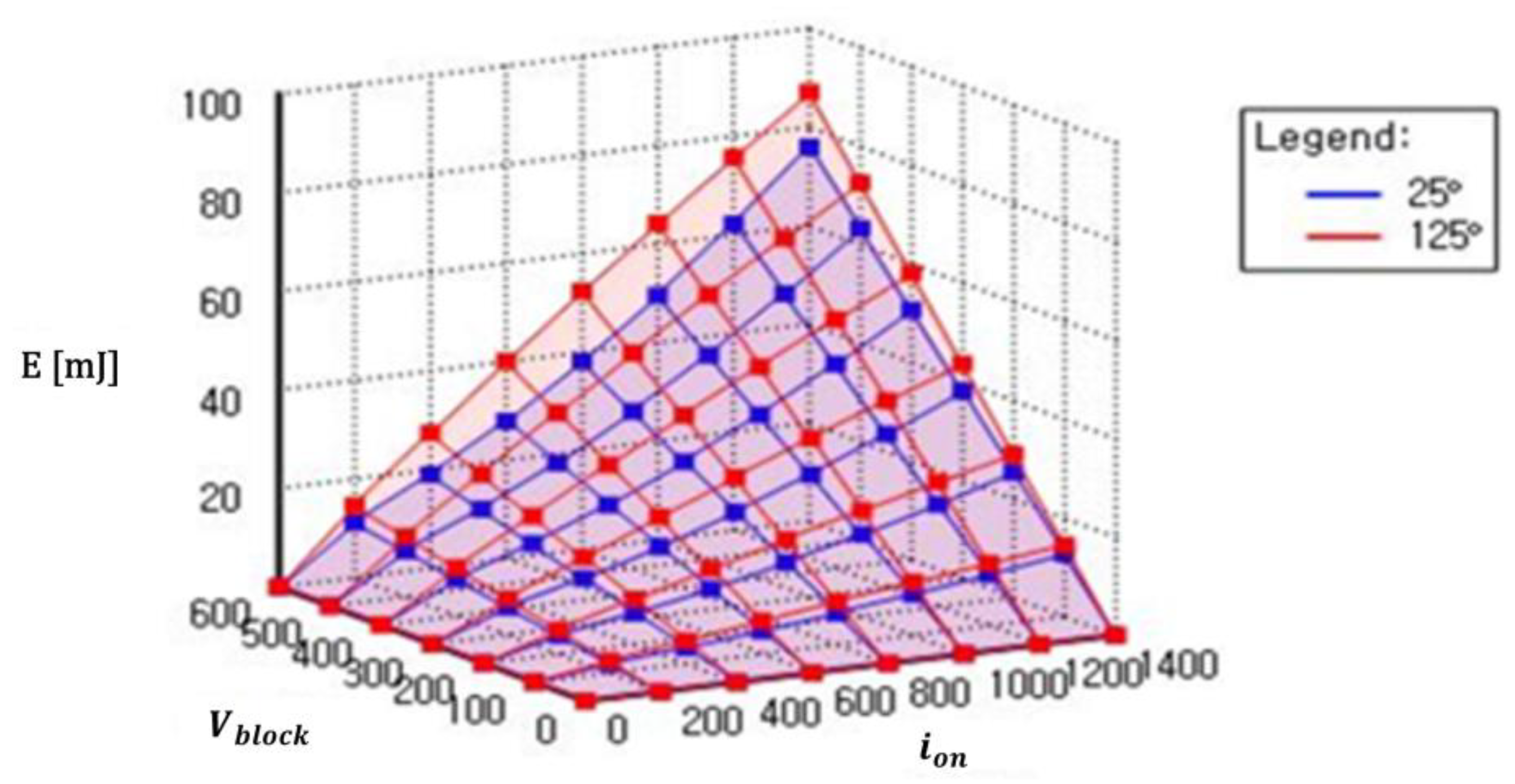
3.2. Switching Loss
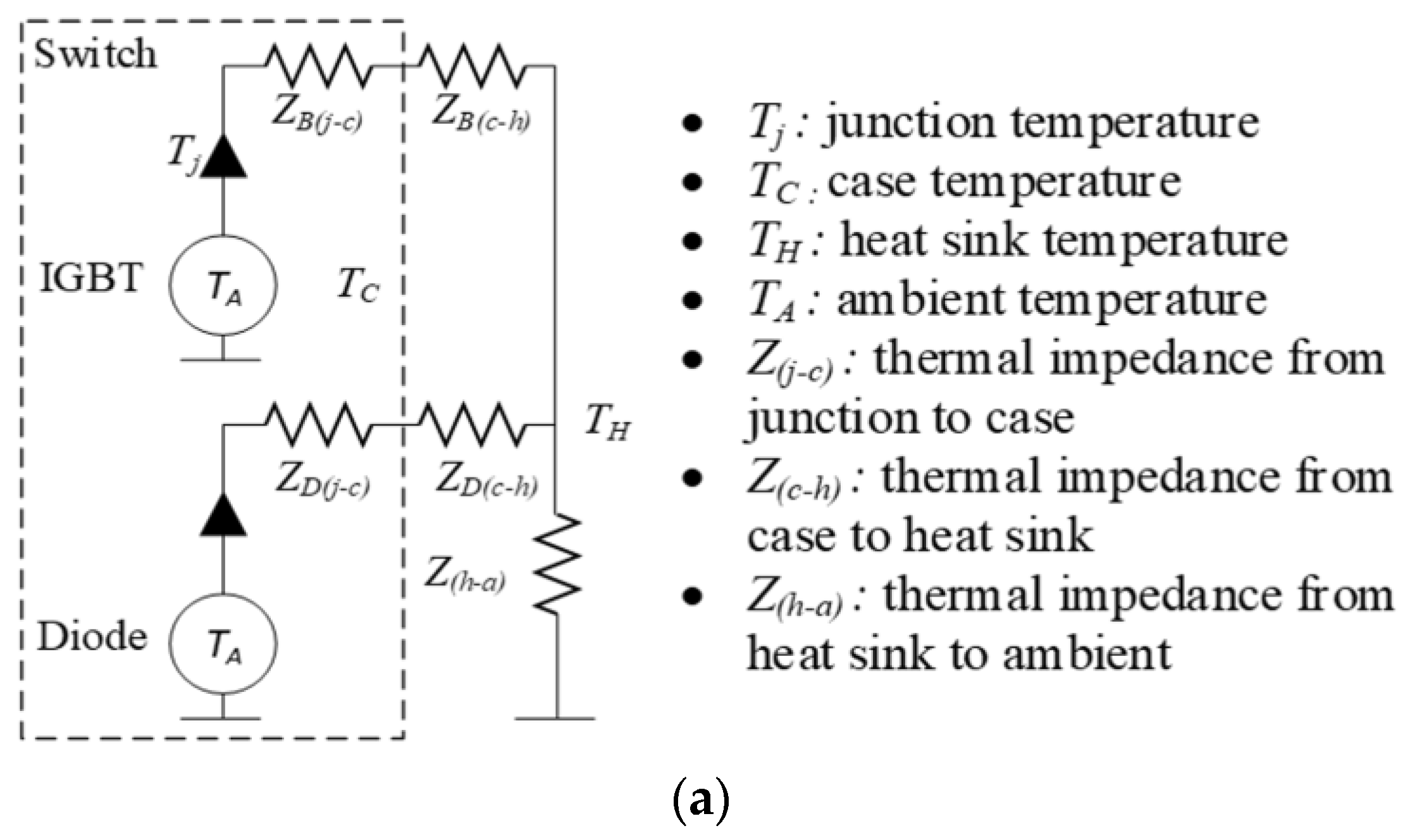
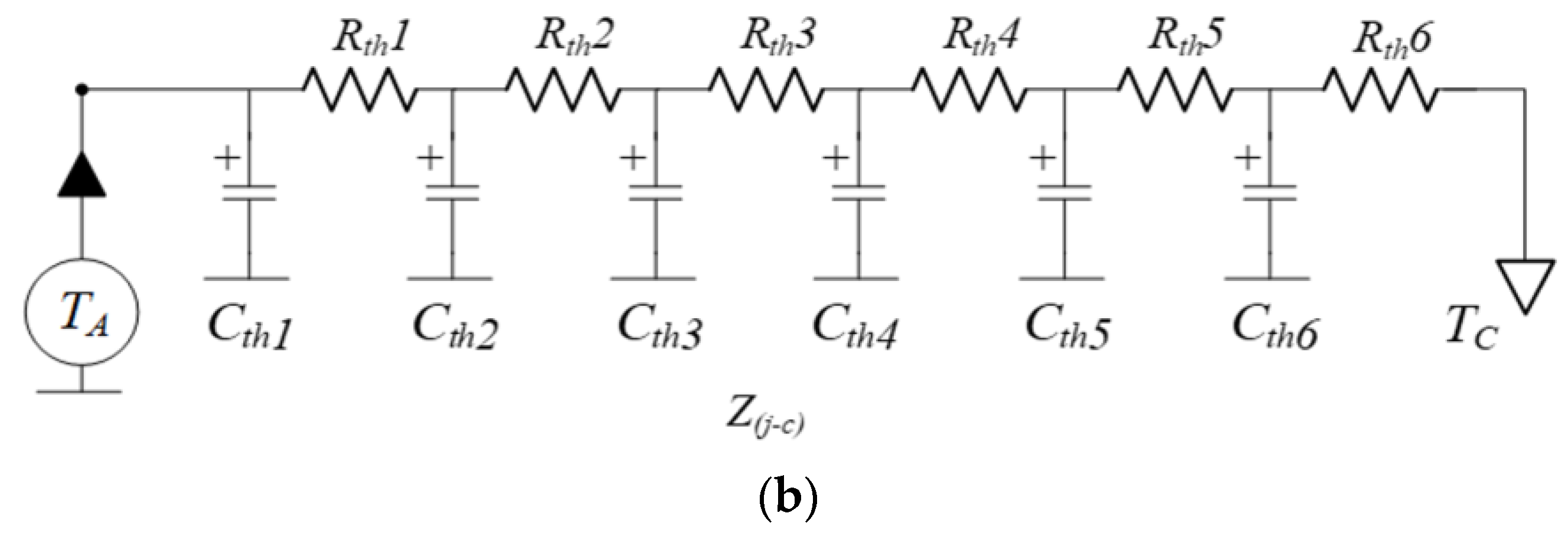
3.3. Heat Sink Design
- The values of current (I) and voltage (V) applied to the switch are provided to look up the table after the switching event;
- The calculated losses are added to the thermal circuit;
- Temperature is fed back to look up the table for the next event;
- Losses are calculated as functions of current, voltage, and temperature during switching;
- The loss calculation is based on interpolation.
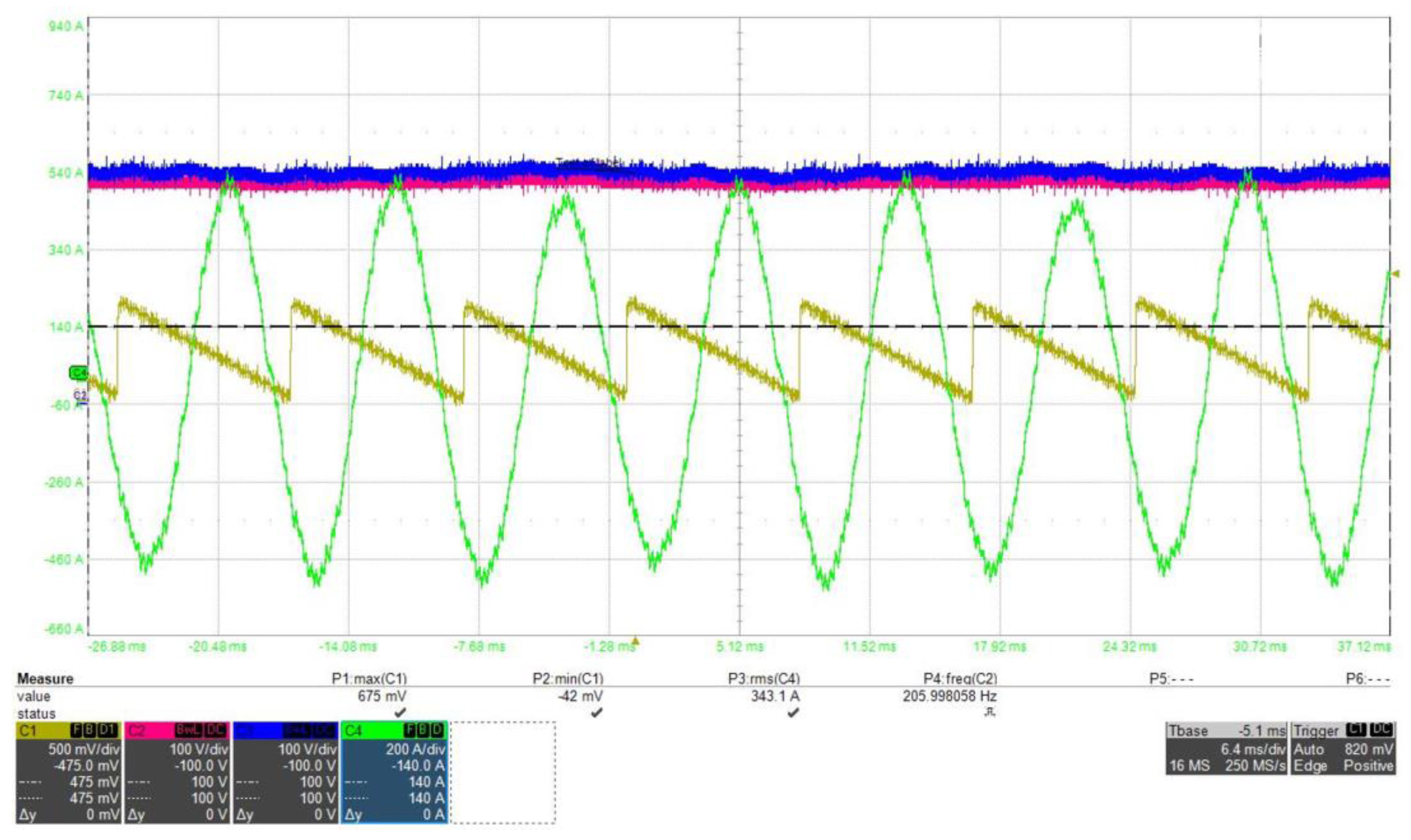
4. Simulation and Experiment Results
5. Discussion
5.1. Differentiating Factors from Existing Heat Dissipation Design Studies
5.2. Unique Contribution of Conduction Loss Analysis Model
5.3. Integration of Heat Dissipation Design with Drive Control System
5.4. Anticipated Societal and Economic Impact
5.5. Innovation in Various Applications of Large-Capacity Inverters
6. Conclusions
6.1. Validation of Heat Dissipation Design
- The study extensively analyzes heat dissipation for the design of a 300 kW modular neutral-point-clamped (NPC) inverter, with a specific focus on the heat sink design of the inverter module;
- Both the simulation model and practical implementation outcomes exhibit a close alignment, providing strong validation for the accuracy of the conducted heat loss analysis.
6.2. Future Application of the Loss Analysis Model
- The developed loss analysis model holds substantial potential for application in the systematic development of inverter systems across diverse operating conditions;
- Leveraging this model allows for the achievement of an optimized heat dissipation design, contingent upon the selected switching techniques.
6.3. Prospects for Cost Reduction and Accelerated Development
- The proposed approach offers promise for cost reduction and a shortened development timeline in practical system design;
- By integrating the loss analysis model, developers can efficiently design inverter systems with enhanced heat dissipation, addressing critical challenges in a more streamlined manner.
6.4. Foundational Insights for Megawatt-Class Inverters
- The comprehensive insights derived from the 300 kW NPC modular inverter design process, including production and performance evaluation results, serve as foundational data;
- Intended to advance Megawatt (MW)-class large-capacity inverters, these contributions offer a robust foundation for reference and further exploration in the design and implementation of high-capacity inverter systems.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kim, S.-G.; Dujic, D. Extending Protection Selectivity in DC Shipboard Power Systems by Means of Additional Bus Capacitance. IEEE Trans. Ind. Electron. 2020, 67, 3673–3683. [Google Scholar] [CrossRef]
- Kouro, S.; Malinowski, M.; Gopakumar, K.; Pou, J.; Franquelo, L.G.; Wu, B.; Rodriguez, J.; A Pérez, M.; Leon, I.J. Recent Advances and Industrial Applications of Multilevel Converters. IEEE Trans. Ind. Electron. 2010, 57, 2553–2580. [Google Scholar] [CrossRef]
- Javaid, U.; Freijedo, F.D.; Dujic, D.; van der Merwe, W. Dynamic Assessment of Source–Load Interactions in Marine MVDC Distribution. IEEE Trans. Ind. Electron. 2017, 64, 4372–4381. [Google Scholar] [CrossRef]
- Ghimire, P.; Park, D.; Zadeh, M.K.; Thorstensen, J.; Pedersen, E. Shipboard Electric Power Conversion: System Architecture, Applications, Control, and Challenges. IEEE Electrif. Mag. 2019, 7, 6–20. [Google Scholar] [CrossRef]
- Sadigh, A.K.; Dargahi, V.; Corzine, K.A. Analytical Determination of Conduction and Switching Power Losses in Flying-Capacitor-Based Active Neutral-Point-Clamped Multilevel Converter. IEEE Trans. Power Electron. 2016, 31, 5473–5494. [Google Scholar] [CrossRef]
- Barros, J.D. Optimal Predictive Control of Three-Phase NPC Multi level Converter for Power Quality Applications. IEEE Trans. Ind. Electron. 2008, 55, 3670–3681. [Google Scholar] [CrossRef]
- Raciti, A.; Cristaldi, D.; Greco, G.; Vinci, G.; Bazzano, G. Electrothermal PSpice Modeling and Simulation of Power Modules. IEEE Trans. Ind. Electron. 2015, 62, 6260–6271. [Google Scholar] [CrossRef]
- Gilson, G.M.; Pickering, S.J.; Hann, D.B.; Gerada, C. Piezoelectric Fan Cooling: A Novel High Reliability Electric Machine Thermal Management Solution. IEEE Trans. Ind. Electron. 2013, 60, 4841–4851. [Google Scholar] [CrossRef]
- Ma, M.; Guo, W.; Yan, X.; Yang, S.; Zhang, X.; Chen, W.; Cai, G. A Three-Dimensional Boundary-Dependent Compact Thermal Network Model for IGBT Modules in New Energy Vehicles. IEEE Trans. Ind. Electron. 2021, 68, 5248–5258. [Google Scholar] [CrossRef]
- Yang, X.; Heng, K.; Wu, X. Distributed Thermal Modeling for Power Devices and Modules With Equivalent Heat Flow Path Extraction. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 5863–5876. [Google Scholar] [CrossRef]
- Chen, H.; Yang, J.; Xu, S. Electrothermal-Based Junction Temperature Estimation Model for Converter of Switched Reluctance Motor Drive System. IEEE Trans. Ind. Electron. 2020, 67, 874–883. [Google Scholar] [CrossRef]
- Sodan, V.; Stoffels, S.; Oprins, H.; Decoutere, S.; Altmann, F.; Baelmans, M.; De Wolf, I. Fast and Distributed Thermal Model for Thermal Modeling of GaN Power Devices. IEEE Trans. Compon. 2018, 8, 1747–1755. [Google Scholar] [CrossRef]
- Shahjalal, M.; Ahmed, R.; Lu, H.; Bailey, C.; Forsyth, A.J. An Analysis of the Thermal Interaction Between Components in Power Converter Applications. IEEE Trans. Power Electron. 2020, 35, 9082–9094. [Google Scholar] [CrossRef]
- Boglietti, A.; Carpaneto, E.; Cossale, M.; Vaschetto, S. Stator-Winding Thermal Models for Short-Time Thermal Transients: Definition and Validation. IEEE Trans. Ind. Electron. 2016, 63, 2713–2721. [Google Scholar] [CrossRef]
- Deepak, K.; Tran, M.-T.; Tran, D.-D.; El Baghdadi, M.; Hegazy, O. Design Investigation of Liquid Cooled Heat Sink for GaN FET Dual-Three Phase Inverter. Int. Symp. Power Electron. 2022, 149–154. [Google Scholar] [CrossRef]
- Qiao, G.; Cao, W.; Hu, Y.; Li, J.; Sun, L.; Hu, C. Surrogate Model-Based Heat Sink Design for Energy Storage Converters. Energies 2023, 16, 1075. [Google Scholar] [CrossRef]
- Yu, Y.; Lee, T.-Y.T.; Chiriac, V.A. Compact Thermal Resistor-Capacitor-Network Approach to Predicting Transient Junction Temperatures of a Power Amplifier Module. IEEE Trans. Compon. 2012, 2, 1172–1181. [Google Scholar] [CrossRef]
- An, T.; Zhou, R.; Qin, F.; Dai, Y.; Gong, Y.; Chen, P. Comparative Study of the Parameter Acquisition Methods for the Cauer Thermal Network Model of an IGBT Module. Electronics 2023, 12, 1650. [Google Scholar] [CrossRef]
- Muller, N.; Kouro, S.; Malinowski, M.; Rojas, C.A.; Jasinski, M.; Estay, G. Medium-Voltage Power Converter Interface for Multigenerator Marine Energy Conversion Systems. IEEE Trans. Ind. Electron. 2017, 64, 1061–1070. [Google Scholar] [CrossRef]
- Lee, J.-S.; Yoo, S.; Lee, K.-B. Novel Discontinuous PWM Method of a Three-Level Inverter for Neutral-Point Voltage Ripple Reduction. IEEE Trans. Ind. Electron. 2016, 63, 3344–3354. [Google Scholar] [CrossRef]
- Choi, U.-M.; Blaabjerg, F.; Lee, K.-B. Study and Handling Methods of Power IGBT Module Failures in Power Electronic Converter Systems. IEEE Trans. Power Electron. 2015, 30, 2517–2533. [Google Scholar] [CrossRef]
- Oh, H.; Han, B.; McCluskey, P.; Han, C.; Youn, B.D. Physics-of-Failure, Condition Monitoring, and Prognostics of Insulated Gate Bipolar Transistor Modules: A Review. IEEE Trans. Power Electron. 2015, 30, 2413–2426. [Google Scholar] [CrossRef]
- Falck, J.; Felgemacher, C.; Rojko, A.; Liserre, M.; Zacharias, P. Reliability of Power Electronic Systems: An Industry Perspective. IEEE Ind. Electron. Mag. 2018, 12, 24–35. [Google Scholar] [CrossRef]
- Wang, H.; Zhou, D.; Blaabjerg, F. A reliability-oriented design method for power electronic converters. IEEE Appl. Power Electron. Conf. Expo. (APEC) 2013, 2921–2928. [Google Scholar] [CrossRef]
- Sadigh, A.K.; Dargahi, V.; Corzine, K.A. Investigation of Conduction and Switching Power Losses in Modified Stacked Multicell Converters. IEEE Trans. Ind. Electron. 2016, 63, 7780–7791. [Google Scholar] [CrossRef]
- Andresen, M.; Ma, K.; Buticchi, G.; Falck, J.; Blaabjerg, F.; Liserre, M. Junction Temperature Control for More Reliable Power Electronics. IEEE Trans. Power Electron. 2018, 33, 765–776. [Google Scholar] [CrossRef]
- Codecasa, L. Canonical forms of one-port Passive Distributed thermal networks. IEEE Trans. Compon. Packag. Technol. 2005, 28, 5–13. [Google Scholar] [CrossRef]




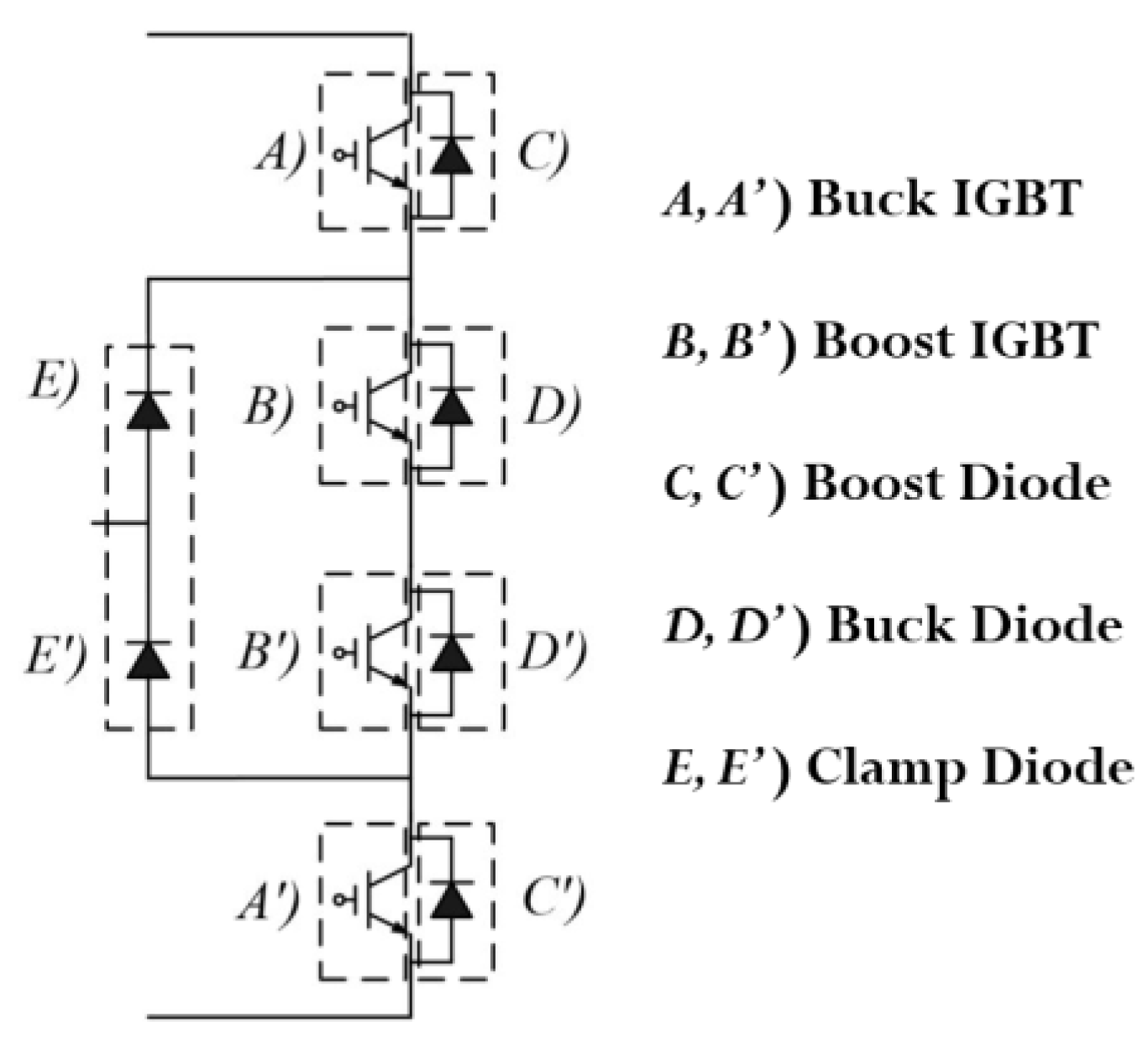



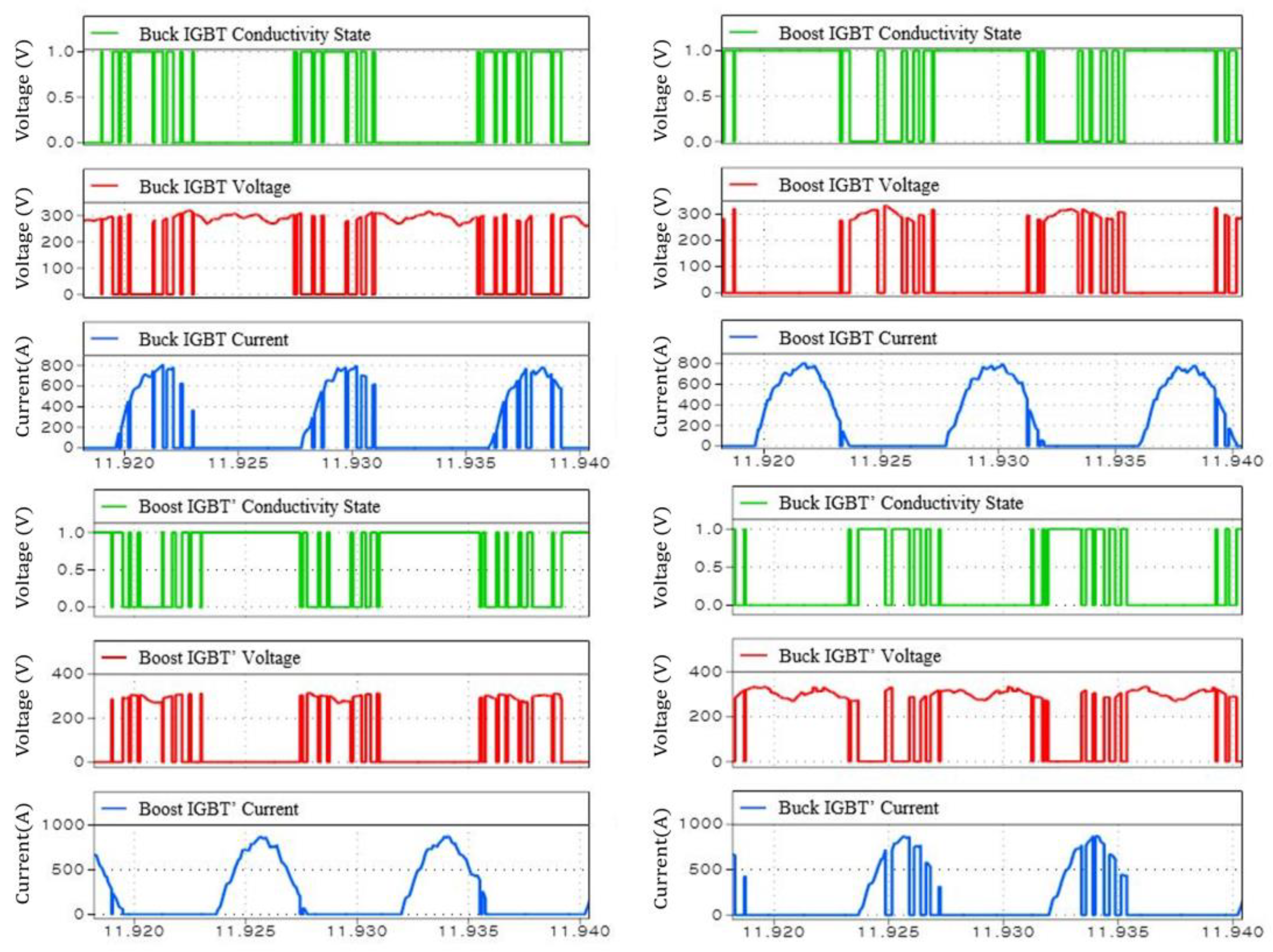
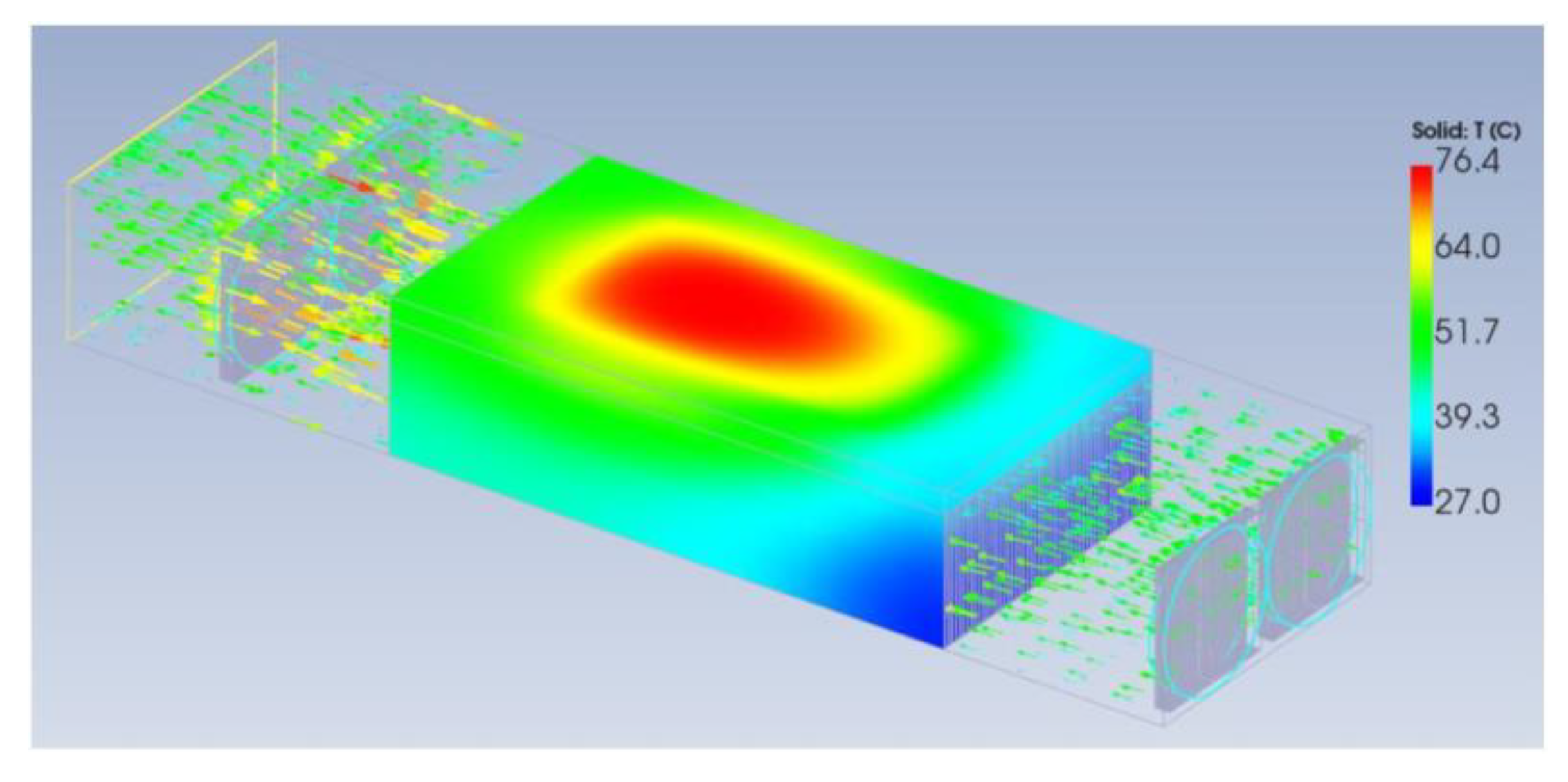
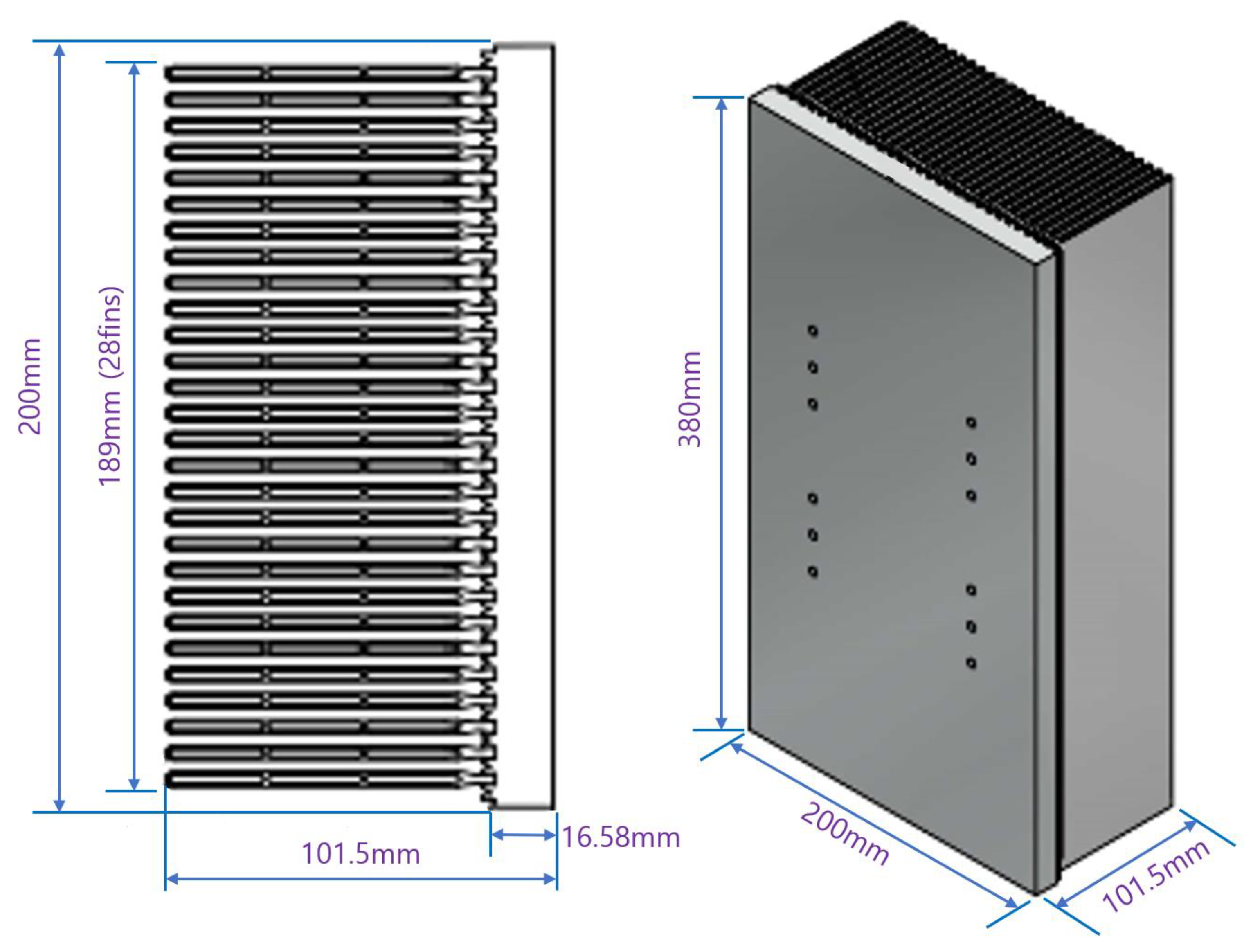


| Thermal Parameter | Unit | Electrical Parameter | Unit |
|---|---|---|---|
| Temperature | T in K | Voltage | V |
| Heat Flux | P in W | Current | A |
| Thermal Resistance | Rth in K/W | Resistance | Ω |
| Thermal Capacitance | Cth in J/K | Capacitance | F |
| Parameter | Value | Unit |
|---|---|---|
| Rated Power | 300 | kW |
| Line Frequency | 60 | Hz |
| Output Voltage | 380 | V |
| Output Current | 580 | A |
| DC link Voltage | 600 | V |
| DC link Capacitance | 3.1 | mH |
| Switching Frequency | 1000 | Hz |
| Thermal Impedance | Z(j−c) | |||||
|---|---|---|---|---|---|---|
| Sec1 | Sec2 | Sec3 | Sec4 | Sec5 | Sec6 | |
| Buck IGBT Resistance | 0.003 | 0.007 | 0.01 | 0.008 | 0.013 | 0.012 |
| Buck IGBT Capacitance | 0.017 | 1.198 | 2.022 | 18.09 | 44.46 | 196.2 |
| Boost IGBT Resistance | 0.005 | 0.011 | 0.011 | 0.015 | 0.012 | 0.002 |
| Boost IGBT Capacitance | 1.564 | 1.604 | 16.83 | 40.65 | 148.7 | 3687 |
| Buck Diode Resistance | 0.009 | 0.028 | 0.014 | 0.013 | 0.011 | 0.005 |
| Buck Diode Capacitance | 0.3 | 0.854 | 5.05 | 25.6 | 143.4 | 1619 |
| Boost Diode Resistance | 0.006 | 0.022 | 0.016 | 0.033 | 0.019 | 0.005 |
| Boost Diode Capacitance | 0.261 | 0.736 | 1.935 | 15.12 | 81.81 | 1473 |
| Clamp Diode Resistance | 0.005 | 0.01 | 0.011 | 0.015 | 0.012 | 0.003 |
| Clamp Diode Capacitance | 1.564 | 1.604 | 16.83 | 40.65 | 148.7 | 3687 |
| Buck IGBT | Boost IGBT | Boost IGBT’ | Buck IGBT’ | Total | |
|---|---|---|---|---|---|
| SWLoss (W) | 11 | 2.8 | 2.7 | 10.7 | 27.2 |
| CondLoss (W) | 388 | 490 | 491 | 390 | 1759 |
| Junction °C | 89.7 | 105.1 | 105.4 | 93.6 | - |
| Heatsink °C | 72 | 78 | 78 | 72 | - |
| Boost Diode | Buck Diode | Buck Diode’ | Boost Diode’ | ||
| SWLoss (W) | 0.45 | 0.002 | 0.0005 | 0.47 | 0.9225 |
| CondLoss (W) | 11.6 | 11.6 | 12.03 | 12.01 | 47.24 |
| Junction °C | 35 | 34 | 34 | 35 | - |
| Heatsink °C | 28.7 | 27.4 | 27.2 | 28.5 | - |
| Clamp Diode | Clamp Diode | ||||
| SWLoss (W) | 4.2 | 3.97 | 8.17 | ||
| CondLoss (W) | 165.1 | 164.8 | 329.9 | ||
| Junction °C | 65.3 | 65.6 | - | ||
| Heatsink °C | 55 | 55 | - | ||
| 2172.4 | |||||
| Flow Rate | Air Temperature | Thermal Impedance/Device Loss | Max Temperature | Pressure Drop |
|---|---|---|---|---|
| 4 Fans | 25 °C | datasheet | 76.4 °C | 75 Pa |
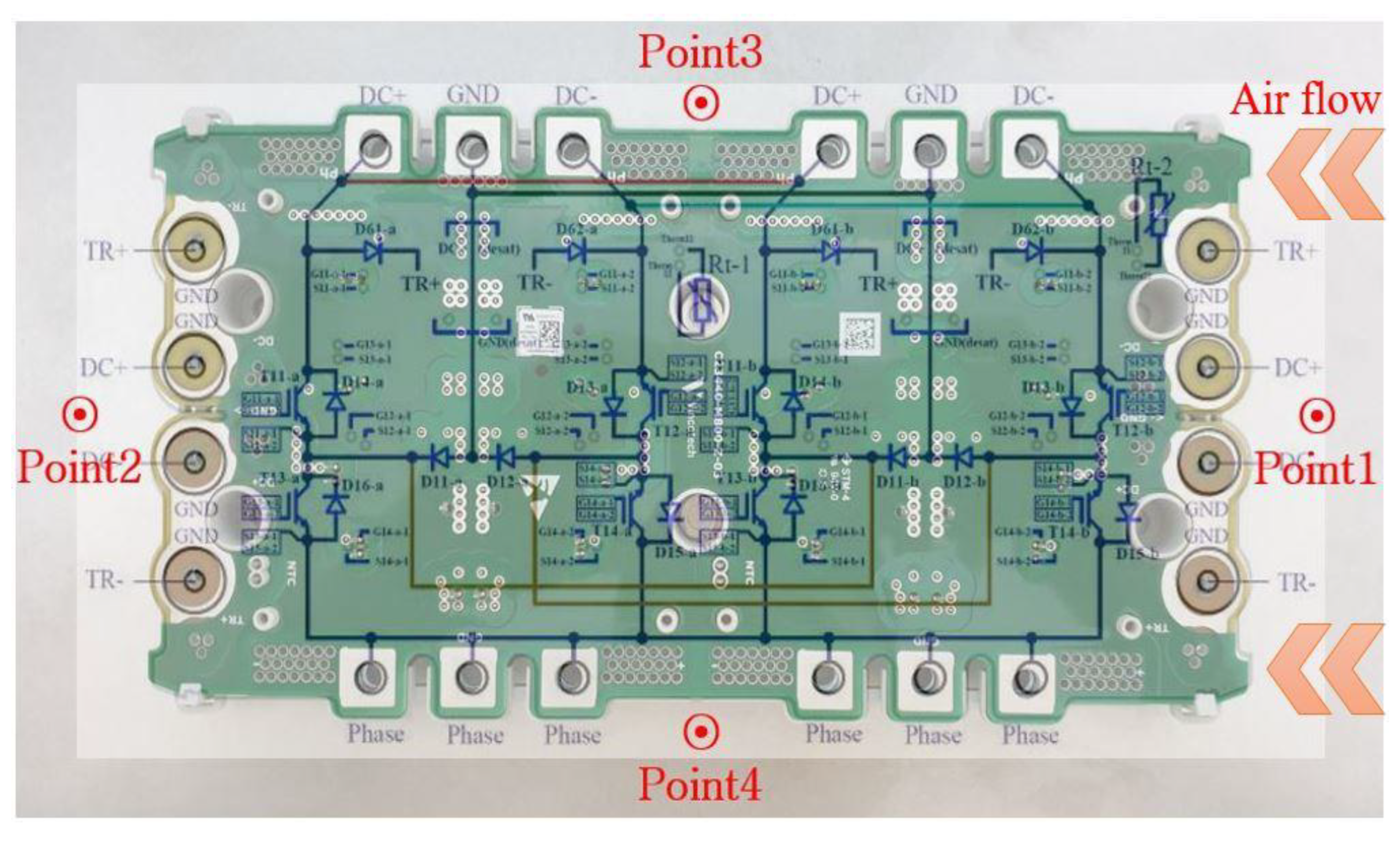
| Point1 | Point2 | Point3 | Point4 | |
|---|---|---|---|---|
| Saturated Heat Sink Temperature in experiment | 71.3 °C | 74.6 °C | 60.2 °C | 65.5 °C |
| Inverter Efficiency | Simulation | Experiment | ||
| 97.9% | 97.1% | |||
| Max Temperature | PLECS | CFD | Experiment | |
| 78 °C | 76.4 °C | 74 °C | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, J.-W.; Kim, J.-W.; Lee, C.-W.; Park, B.-G.; Lee, J.-K. Simple Design Scheme for a 300 kW Modular NPC Inverter Using Thermal RC Network Analysis. Energies 2024, 17, 393. https://doi.org/10.3390/en17020393
Lee J-W, Kim J-W, Lee C-W, Park B-G, Lee J-K. Simple Design Scheme for a 300 kW Modular NPC Inverter Using Thermal RC Network Analysis. Energies. 2024; 17(2):393. https://doi.org/10.3390/en17020393
Chicago/Turabian StyleLee, Jae-Woon, Ji-Won Kim, Chee-Woo Lee, Byoung-Gun Park, and Jin-Kyu Lee. 2024. "Simple Design Scheme for a 300 kW Modular NPC Inverter Using Thermal RC Network Analysis" Energies 17, no. 2: 393. https://doi.org/10.3390/en17020393
APA StyleLee, J.-W., Kim, J.-W., Lee, C.-W., Park, B.-G., & Lee, J.-K. (2024). Simple Design Scheme for a 300 kW Modular NPC Inverter Using Thermal RC Network Analysis. Energies, 17(2), 393. https://doi.org/10.3390/en17020393








