Numerical Investigations of the Thermal-Hydraulic Characteristics of Microchannel Heat Sinks Inspired by Leaf Veins
Abstract
1. Introduction
2. Design of Bioinspired MCHSs
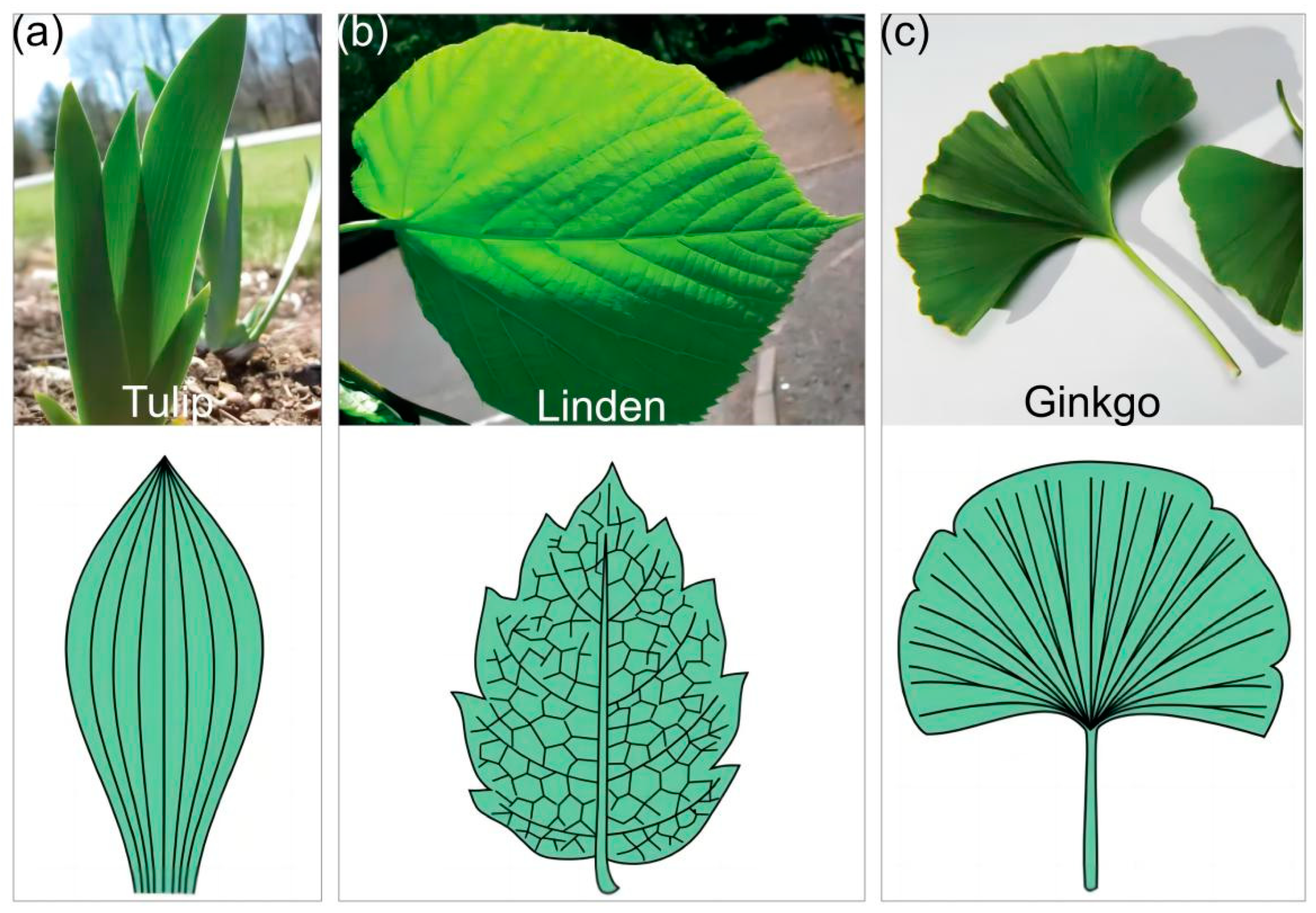
2.1. Natural Prototype
2.2. Physical Model of MCHSs
3. Numerical Method
3.1. Assumptions
3.2. Governing Equations
3.3. Boundary Conditions
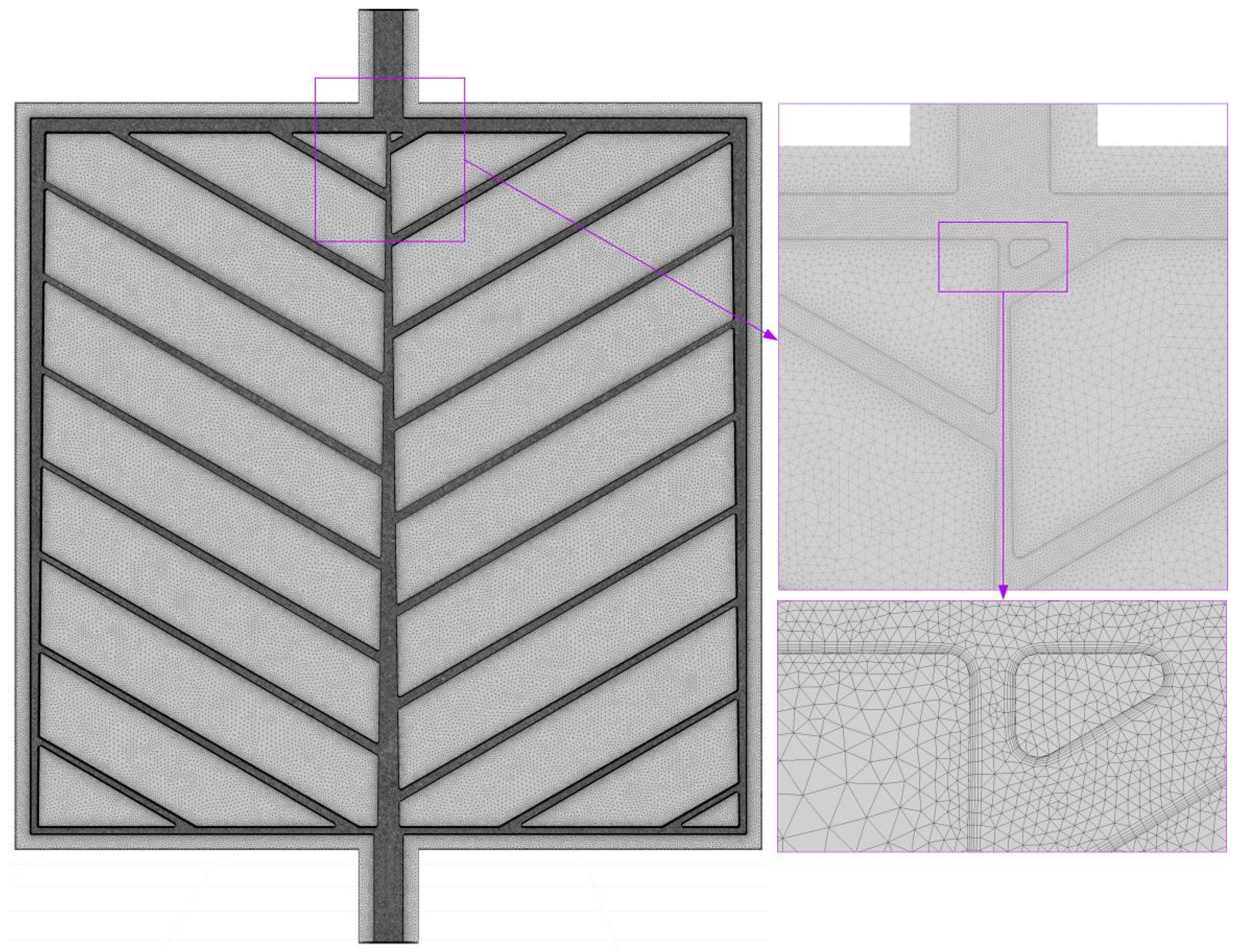
3.4. Meshing
3.5. Solution Strategy
3.6. Data Reduction
4. Results and Discussion
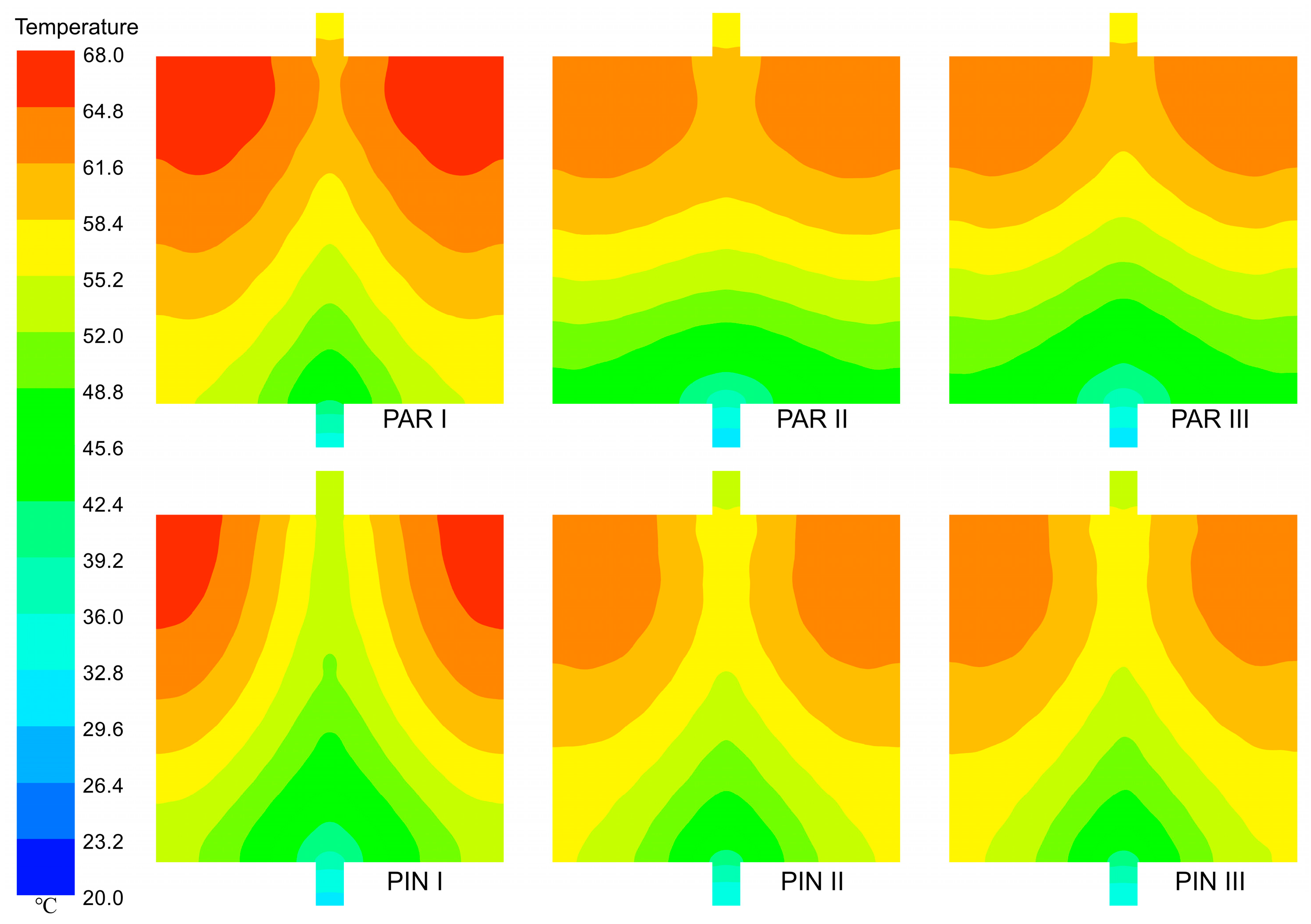
4.1. Effect of Leaf-Vein Structure
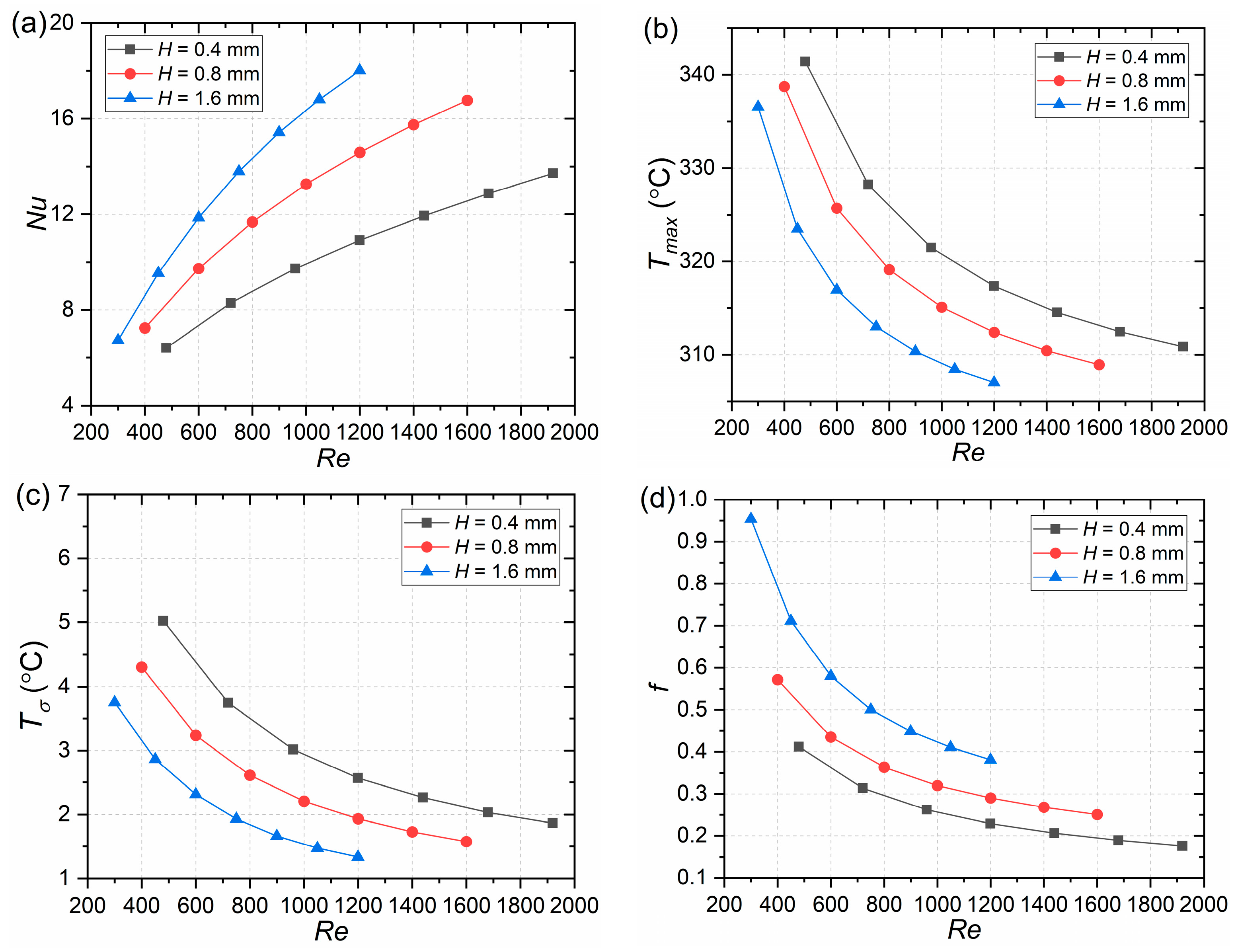
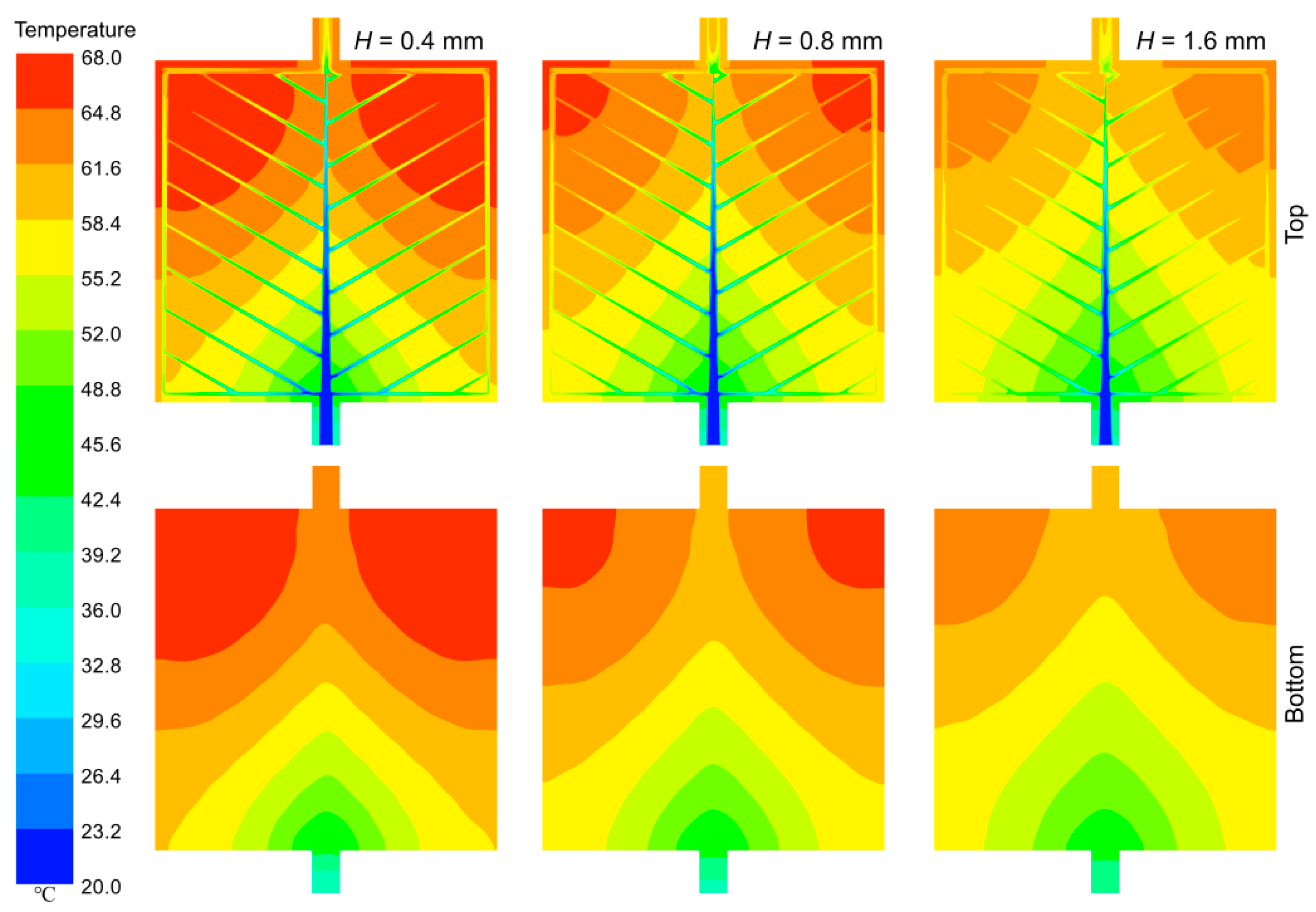
4.2. Effect of Channel Depth
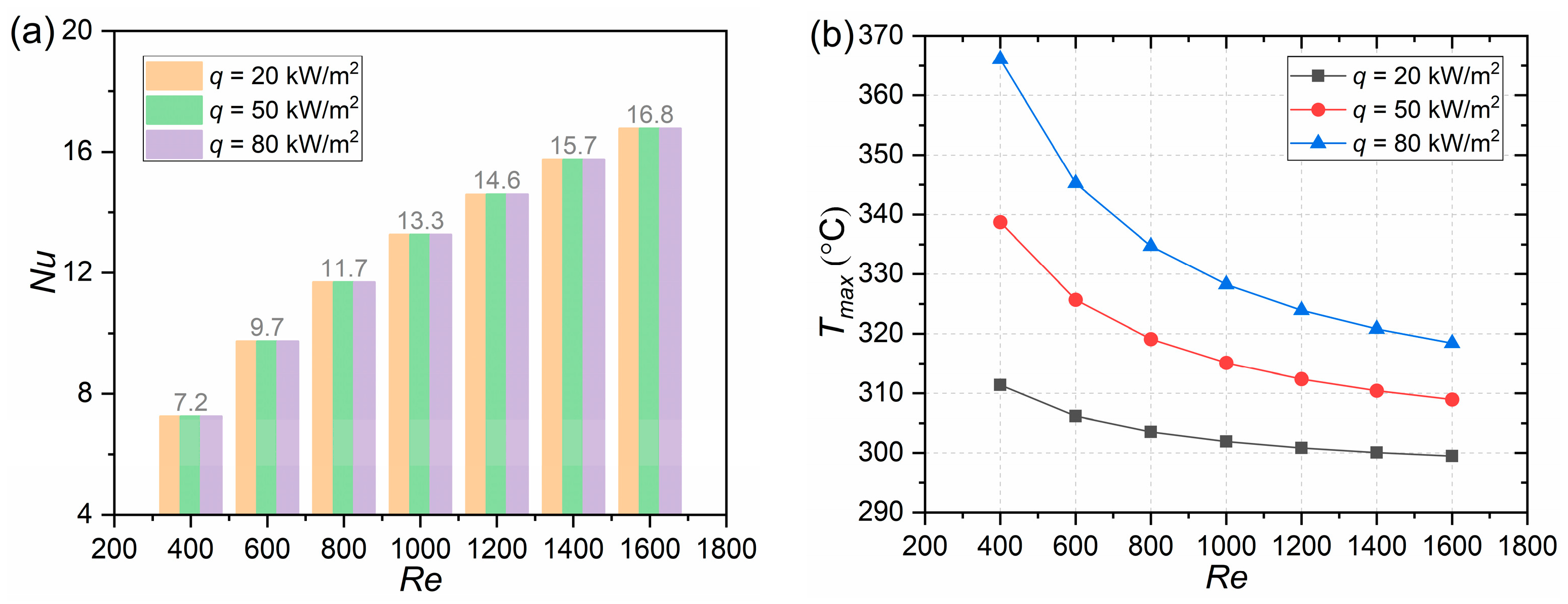
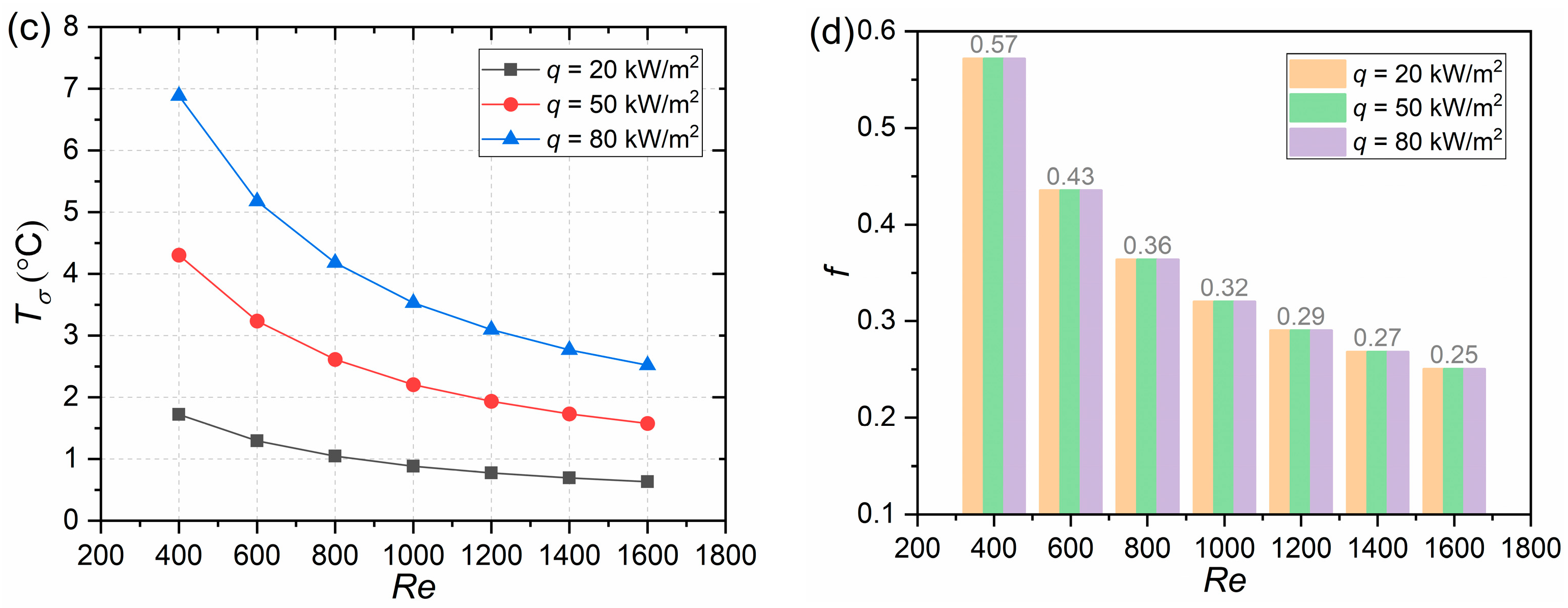
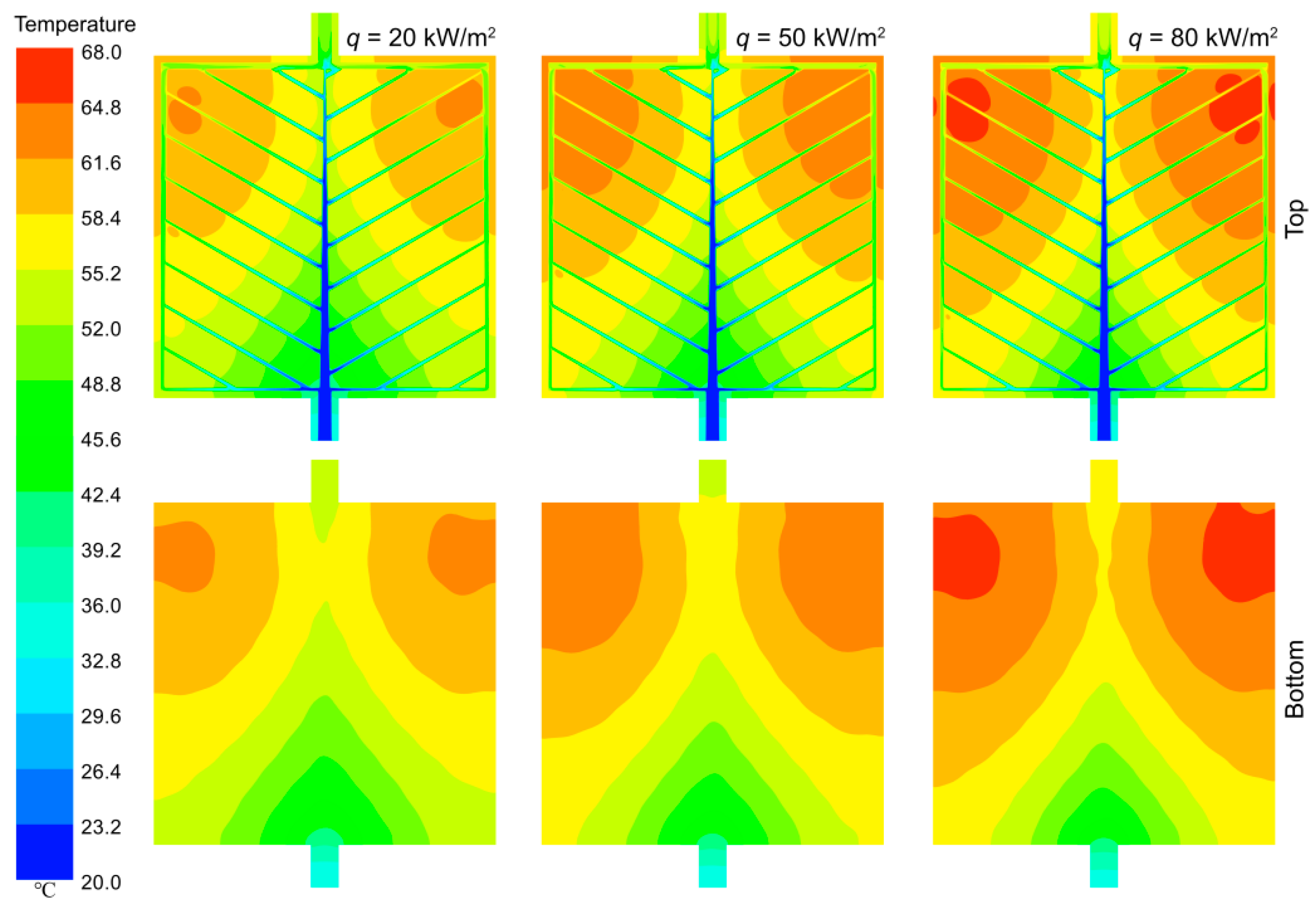
4.3. Effect of Heat Flux
5. Conclusions
- (1)
- Among the six vein configurations, PAR II and PIN III exhibited the highest Nu with values of 14.59 and 13.26 at q = 50 kW/m2, H = 0.8 mm, and Re = 1000, respectively, while PIN III and PAR II demonstrated the lowest Tmax with values of 315.09 and 315.11 K, respectively. Additionally, PIN III displayed the lowest Tσ with a value of 2.21 °C, whereas PAR II had the second lowest f with a value of 0.26. Consequently, a combination of PAR II and PIN III yielded superior overall performance.
- (2)
- An increase in channel depth results in an increase in Nu, a decrease in Tmax and Tσ, and an increase in f. Augmenting the channel depth can enhance heat transfer; however, this increase comes at the expense of increased flow resistance. Moreover, a higher heat flux led to an amplification of Tmax and Tσ without affecting Nu or f.
- (3)
- For MCHSs such as the squares used in this study with a single inlet–outlet configuration, addressing local overheating near right angles close to the outlet to prevent excessive Tmax is an important area for further investigation.
- (4)
- The present study offers valuable insights into the optimization of various MCHS designs for specific applications based on their thermal-hydraulic properties. By comprehending these factors, more efficient cooling solutions can be devised.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A0 | heating wall area [m2] |
| cp | specific heat [J/kg K] |
| Dh | hydraulic diameter [m] |
| f | Darcy–Weisbach friction factor [–] |
| h | heat transfer coefficient [W/m2 K] |
| L | length [m] |
| Nu | Nusselt number [–] |
| p | pressure [Pa] |
| q | heat flux [W/m2] |
| Re | Reynolds number [–] |
| T | temperature [°C] |
| u | velocity [m/s] |
| Δp | pressure drop [Pa] |
| Greek symbols | |
| ρ | density [kg/m3] |
| λ | thermal conductivity [W/m K] |
| μ | viscosity [Pa·s] |
| Subscripts | |
| avg | average |
| f | fluid |
| in | inlet |
| out | outlet |
| s | solid |
| w | wall |
References
- Deng, T.; Zhang, G.; Ran, Y.; Liu, P. Thermal performance of lithium ion battery pack by using cold plate. Appl. Therm. Eng. 2019, 160, 114088. [Google Scholar] [CrossRef]
- Akbarzadeh, M.; Jaguemont, J.; Kalogiannis, T.; Karimi, D.; He, J.; Jin, L.; Xie, P.; Van Mierlo, J.; Berecibar, M. A novel liquid cooling plate concept for thermal management of lithium-ion batteries in electric vehicles. Energy Convers. Manag. 2021, 231, 113862. [Google Scholar] [CrossRef]
- Sakanova, A.; Tong, C.F.; Nawawi, A.; Simanjorang, R.; Tseng, K.J.; Gupta, A.K. Investigation on weight consideration of liquid coolant system for power electronics converter in future aircraft. Appl. Therm. Eng. 2016, 104, 603–615. [Google Scholar] [CrossRef]
- Zilio, C.; Righetti, G.; Mancin, S.; Hodot, R.; Sarno, C.; Pomme, V.; Truffart, B. Active and passive cooling technologies for thermal management of avionics in helicopters: Loop heat pipes and mini-Vapor Cycle System. Therm. Sci. Eng. Prog. 2018, 5, 107–116. [Google Scholar] [CrossRef]
- Wang, J.; Liu, X.; Liu, F.; Liu, Y.; Wang, F.; Yang, N. Numerical optimization of the cooling effect of the bionic spider-web channel cold plate on a pouch lithium-ion battery. Case Stud. Therm. Eng. 2021, 26, 101124. [Google Scholar] [CrossRef]
- Neumann, H.; Gamisch, S.; Gschwander, S. Comparison of RC-model and FEM-model for a PCM-plate storage including free convection. Appl. Therm. Eng. 2021, 196, 124079. [Google Scholar] [CrossRef]
- Xu, Y.; Li, L.; Wang, J. Experimental and numerical investigations of the thermal–hydraulic characteristics of novel micropin-fin heat sinks. Int. J. Heat Mass Transf. 2023, 209, 124079. [Google Scholar] [CrossRef]
- Huang, Z.; Hwang, Y.; Radermacher, R. Review of nature-inspired heat exchanger technology. Int. J. Refrig. 2017, 78, 1–17. [Google Scholar] [CrossRef]
- Asadi, A.; Pourfattah, F. Effects of constructal theory on thermal management of a power electronic system. Sci. Rep. 2020, 10, 21436. [Google Scholar] [CrossRef] [PubMed]
- Peng, Y.; Yang, X.; Li, Z.; Li, S.; Cao, B. Numerical simulation of cooling performance of heat sink designed based on symmetric and asymmetric leaf veins. Int. J. Heat Mass Transf. 2021, 166, 120721. [Google Scholar] [CrossRef]
- Xia, L.; Yu, Z.; Xu, G.; Ji, S.; Sun, B. Design and optimization of a novel composite bionic flow field structure using three-dimensional multiphase computational fluid dynamic method for proton exchange membrane fuel cell. Energy Convers. Manag. 2021, 247, 114707. [Google Scholar] [CrossRef]
- Alnaqi, A.A. Numerical analysis of pressure drop and heat transfer of a Non-Newtonian nanofluids in a Li-ion battery thermal management system (BTMS) using bionic geometries. J. Energy Storage 2022, 45, 103670. [Google Scholar] [CrossRef]
- Deng, T.; Ran, Y.; Zhang, G.; Yin, Y. Novel leaf-like channels for cooling rectangular lithium ion batteries. Appl. Therm. Eng. 2019, 150, 1186–1196. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, C.; Shi, M.; Yang, Y. Thermal and hydrodynamic characteristics of constructal tree-shaped minichannel heat sink. AIChE J. 2009, 56, 2018–2029. [Google Scholar] [CrossRef]
- Wang, X.-Q.; Xu, P.; Mujumdar, A.S.; Yap, C. Flow and thermal characteristics of offset branching network. Int. J. Therm. Sci. 2010, 49, 272–280. [Google Scholar] [CrossRef]
- Tan, H.; Wu, L.; Wang, M.; Yang, Z.; Du, P. Heat transfer improvement in microchannel heat sink by topology design and optimization for high heat flux chip cooling. Int. J. Heat Mass Transf. 2019, 129, 681–689. [Google Scholar] [CrossRef]
- Yenigun, O.; Cetkin, E. Experimental and numerical investigation of constructal vascular channels for self-cooling: Parallel channels, tree-shaped and hybrid designs. Int. J. Heat Mass Transf. 2016, 103, 1155–1165. [Google Scholar] [CrossRef]
- Han, X.-h.; Liu, H.-l.; Xie, G.; Sang, L.; Zhou, J. Topology optimization for spider web heat sinks for electronic cooling. Appl. Therm. Eng. 2021, 195, 117154. [Google Scholar] [CrossRef]
- Tan, H.; Du, P.; Zong, K.; Meng, G.; Gao, X.; Li, Y. Investigation on the temperature distribution in the two-phase spider netted microchannel network heat sink with non-uniform heat flux. Int. J. Therm. Sci. 2021, 169, 107079. [Google Scholar] [CrossRef]
- Qasemi, E.; Mahdavinejad, M.; Aliabadi, M.; Zarkesh, A. Leaf venation patterns as a model for bioinspired fog harvesting. Colloids Surf. A Physicochem. Eng. Asp. 2020, 603, 125170. [Google Scholar] [CrossRef]
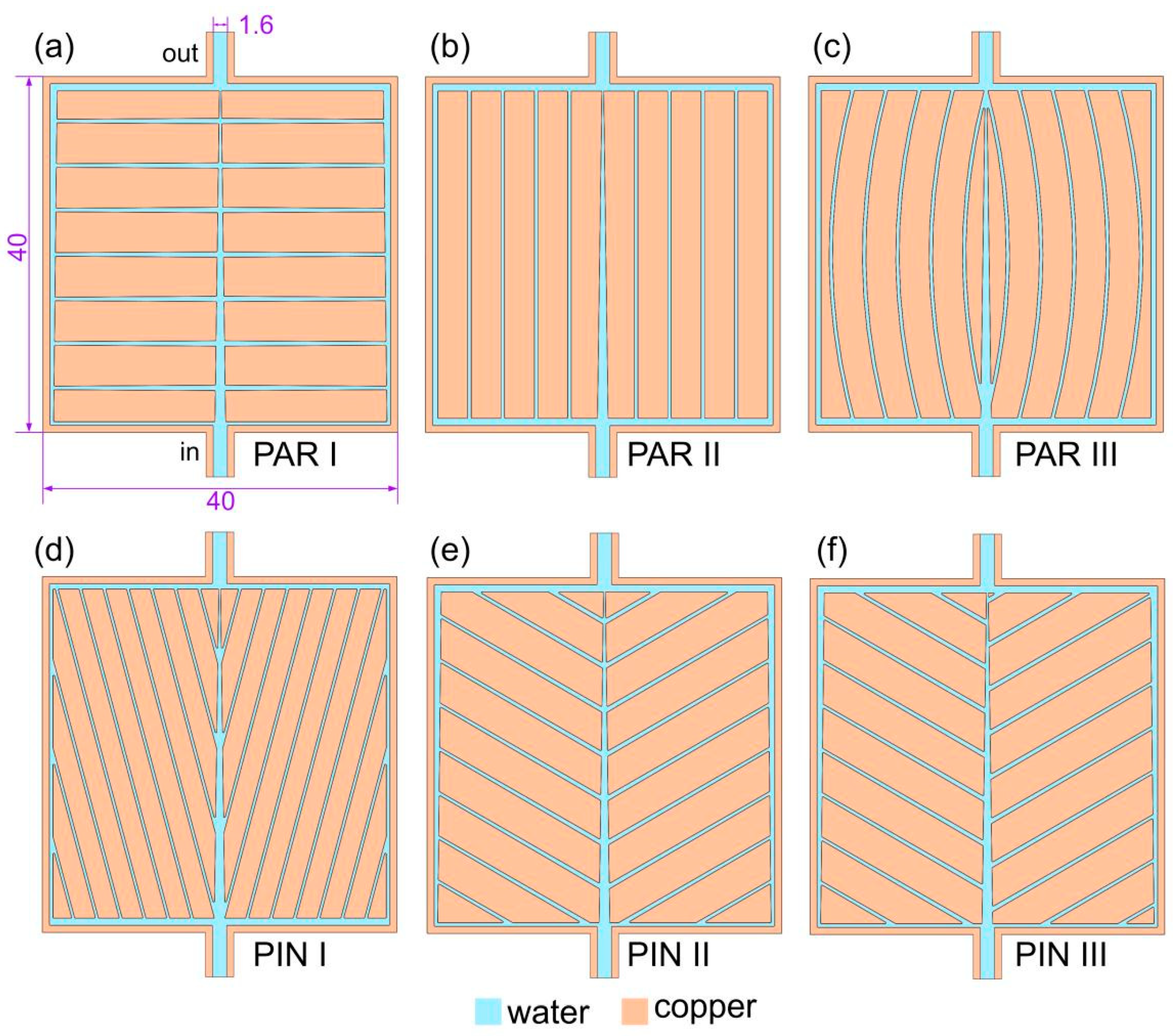


| MCHS | Liquid Area Ratio a | Solid–Liquid Contact Area (mm2) |
|---|---|---|
| PAR I | 14.47% | 942.99 |
| PAR II | 14.74% | 1015.09 |
| PAR III | 16.46% | 1148.48 |
| PIN I | 16.30% | 1386.75 |
| PIN II | 14.98% | 1032.30 |
| PIN III | 15.00% | 1033.15 |
| Mesh Quantity | Tmax (°C) | Tσ (°C) | Nu | f |
|---|---|---|---|---|
| 2.484 × 107 | 315.16 | 2.26 | 13.23 | 0.32 |
| 3.130 × 107 | 314.93 | 2.25 | 13.50 | 0.32 |
| 3.470 × 107 | 314.83 | 2.27 | 13.66 | 0.32 |
| 4.177 × 107 | 314.86 | 2.30 | 13.68 | 0.32 |
| MCHS | Nu | Tmax (K) | Tσ (°C) | f |
|---|---|---|---|---|
| PAR I | 13.24 | 316.44 | 2.45 | 0.40 |
| PAR II | 14.59 | 315.11 | 3.12 | 0.26 |
| PAR III | 13.08 | 315.51 | 3.22 | 0.20 |
| PIN I | 10.34 | 316.62 | 3.06 | 0.28 |
| PIN II | 13.23 | 315.16 | 2.26 | 0.32 |
| PIN III | 13.26 | 315.09 | 2.21 | 0.32 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Qi, S.; Xu, Y. Numerical Investigations of the Thermal-Hydraulic Characteristics of Microchannel Heat Sinks Inspired by Leaf Veins. Energies 2024, 17, 311. https://doi.org/10.3390/en17020311
Wang J, Qi S, Xu Y. Numerical Investigations of the Thermal-Hydraulic Characteristics of Microchannel Heat Sinks Inspired by Leaf Veins. Energies. 2024; 17(2):311. https://doi.org/10.3390/en17020311
Chicago/Turabian StyleWang, Jiale, Shaohuan Qi, and Yu Xu. 2024. "Numerical Investigations of the Thermal-Hydraulic Characteristics of Microchannel Heat Sinks Inspired by Leaf Veins" Energies 17, no. 2: 311. https://doi.org/10.3390/en17020311
APA StyleWang, J., Qi, S., & Xu, Y. (2024). Numerical Investigations of the Thermal-Hydraulic Characteristics of Microchannel Heat Sinks Inspired by Leaf Veins. Energies, 17(2), 311. https://doi.org/10.3390/en17020311







