Modeling and Characterization of Li-Ion 18650 Nickel–Cobalt–Alumina Battery Jellyroll Subjected to Static and Dynamic Compression Loading
Abstract
1. Introduction
2. Research Methodology
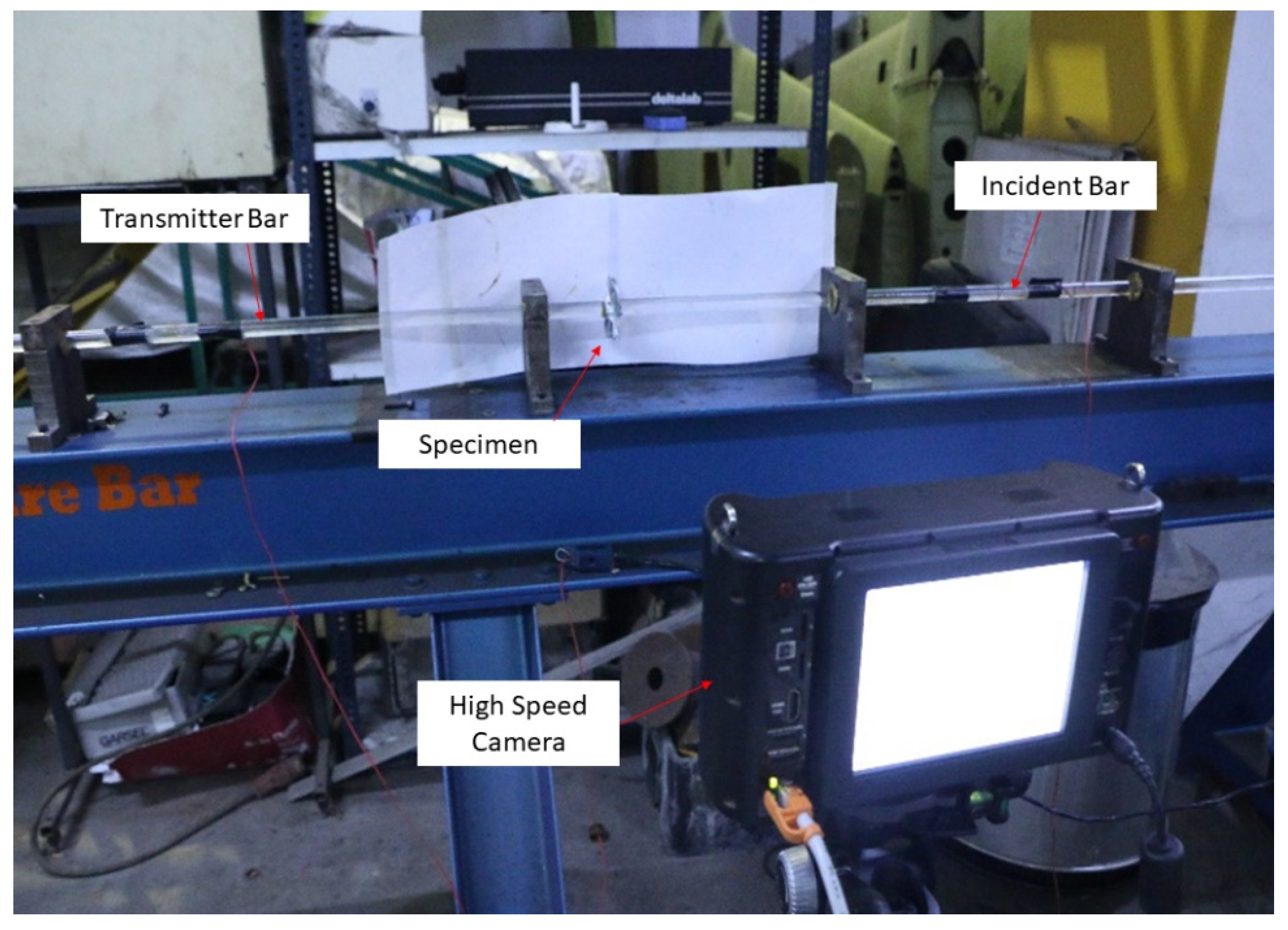
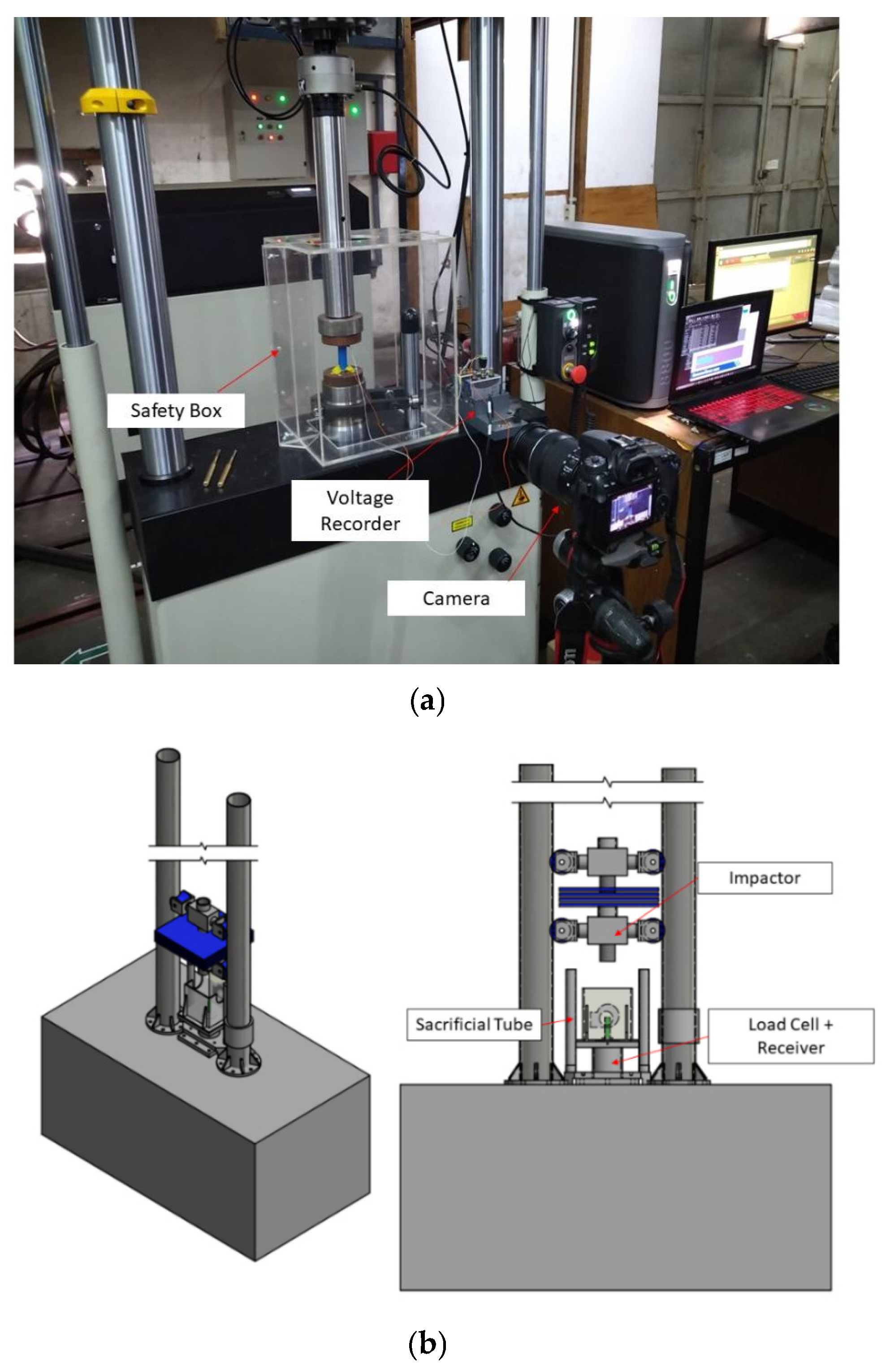
2.1. Experiment Setup
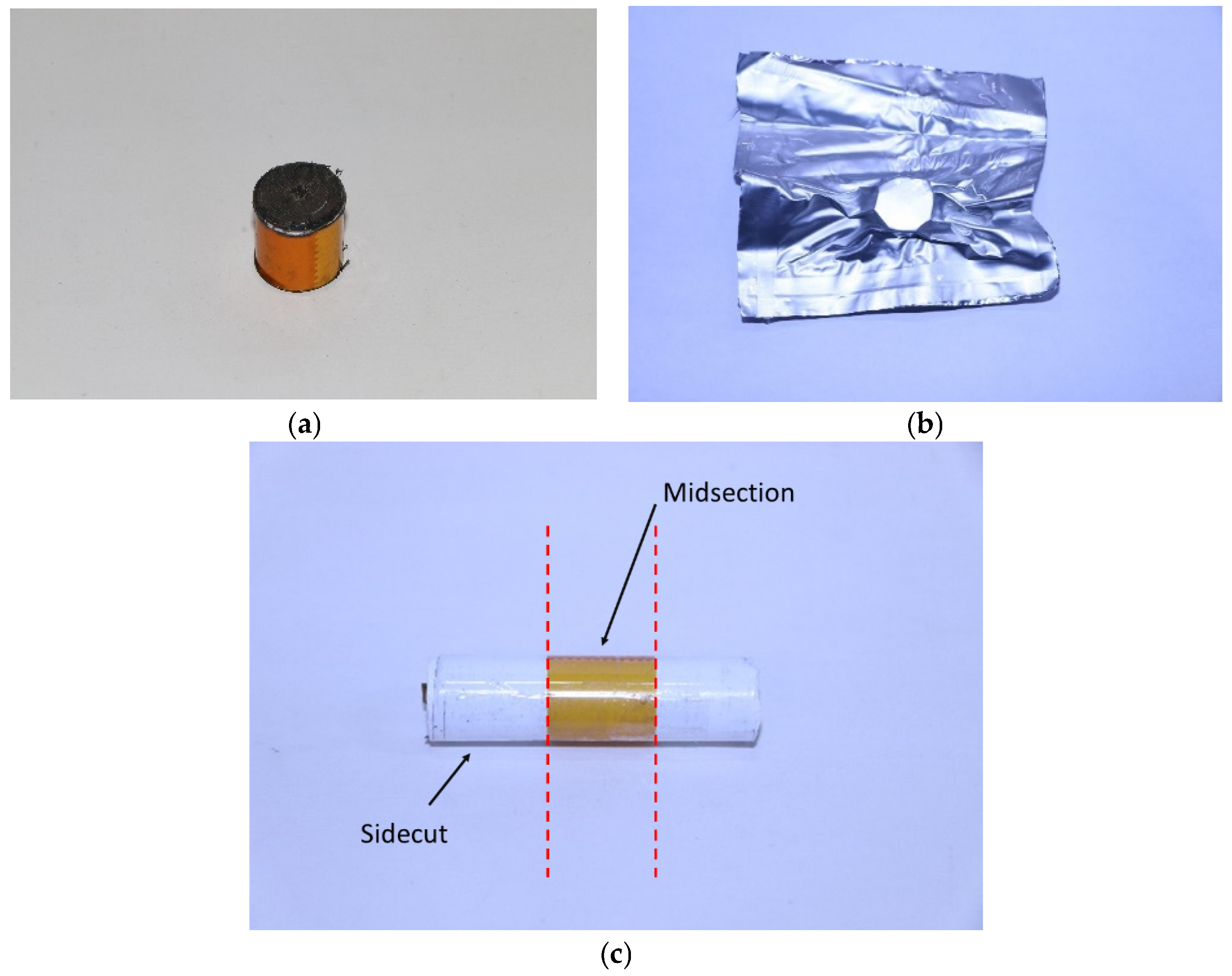
2.1.1. Jellyroll Compression
2.1.2. Battery Compression
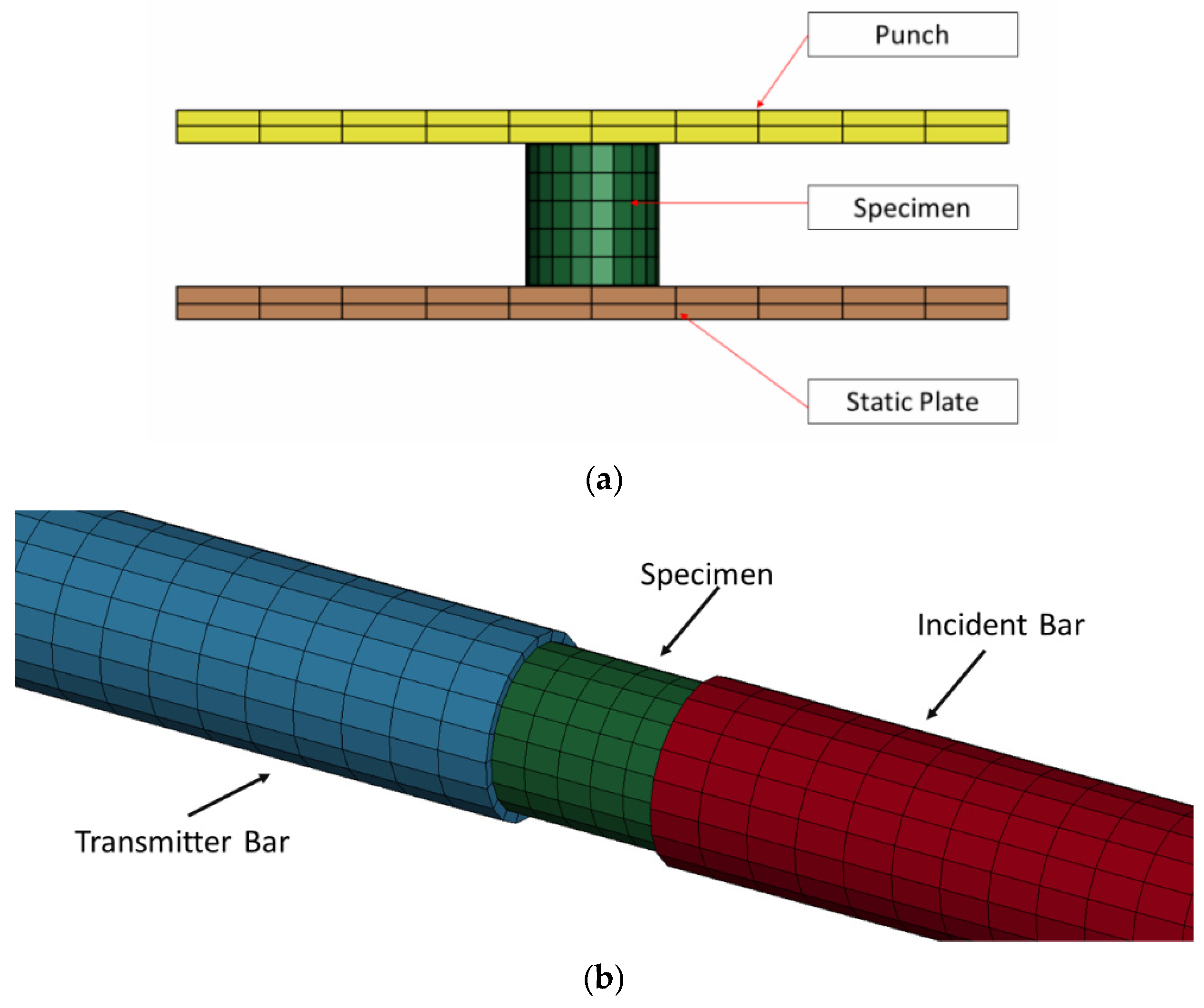
2.2. Numerical Model
3. Results
3.1. Experimental Result
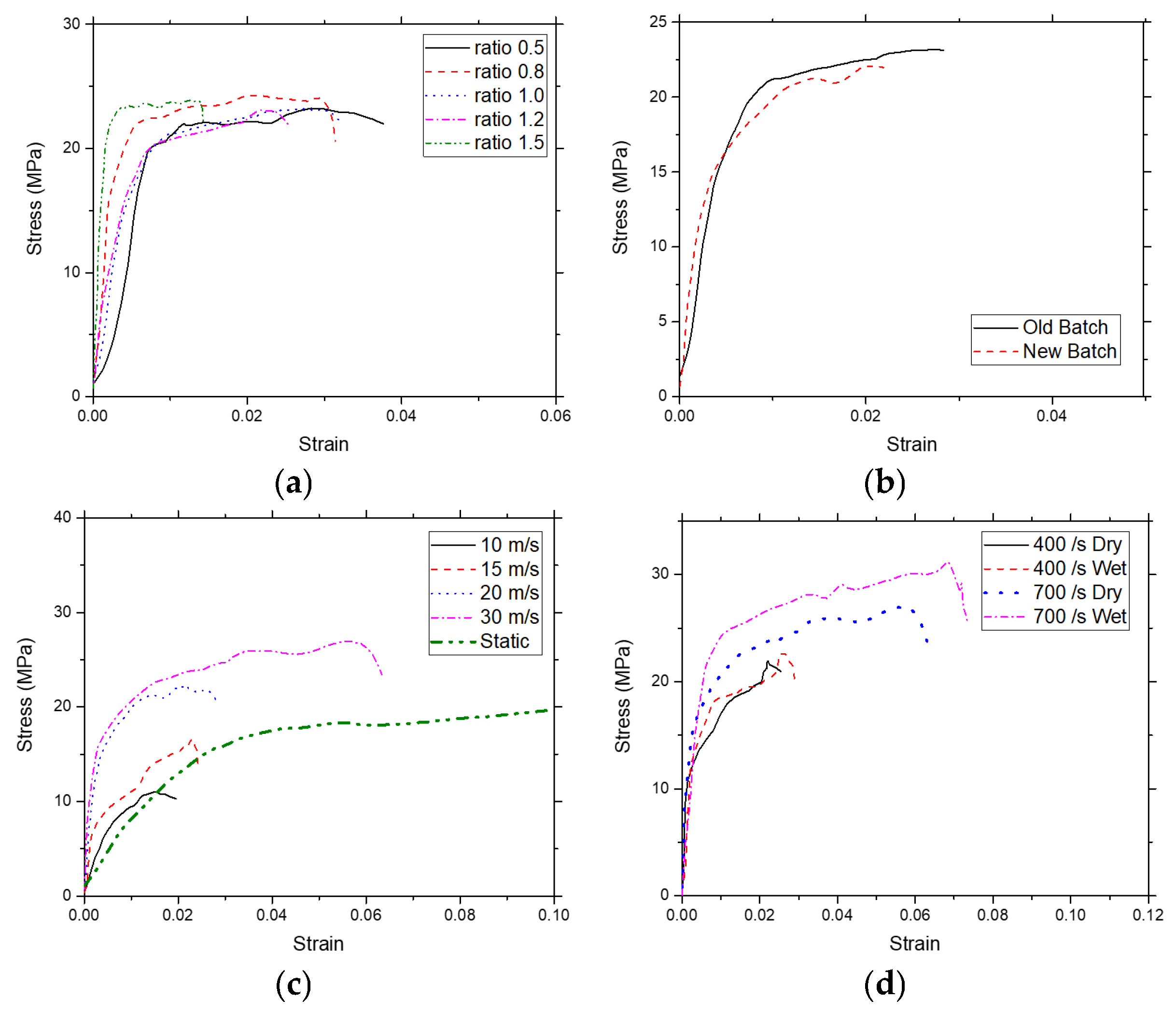
3.1.1. Static Jellyroll Compression Experiment
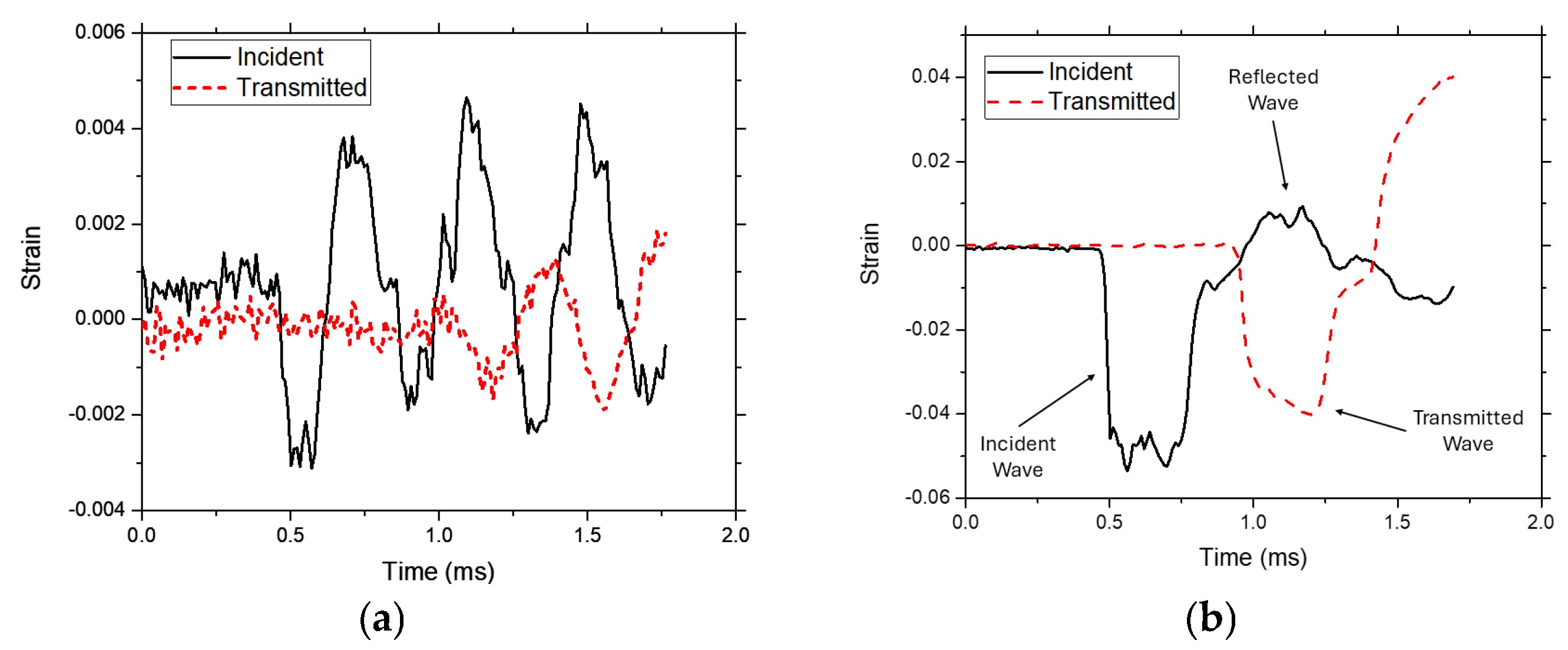
3.1.2. Dynamic Jellyroll Compression Experiment
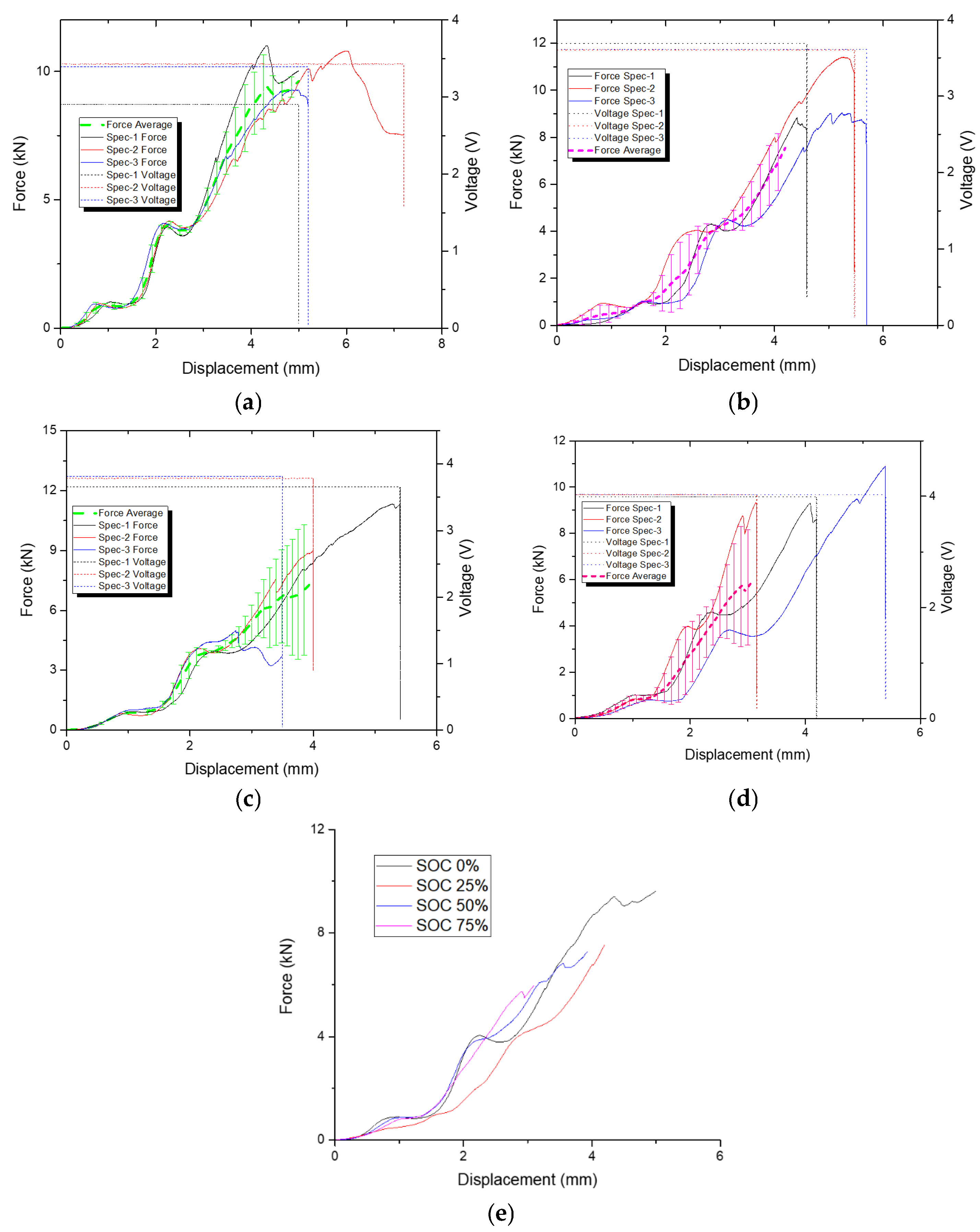
3.1.3. Static Battery Compression
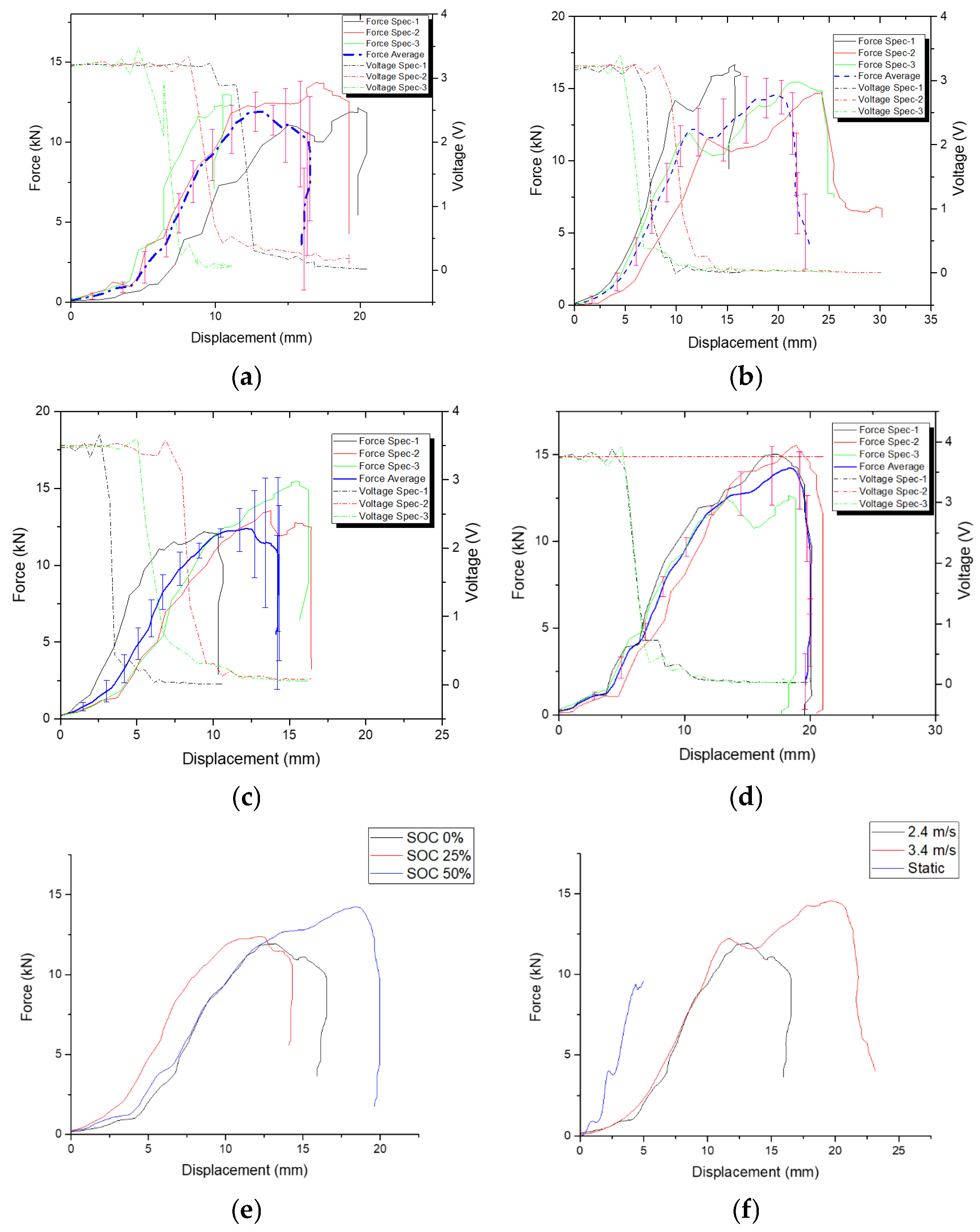
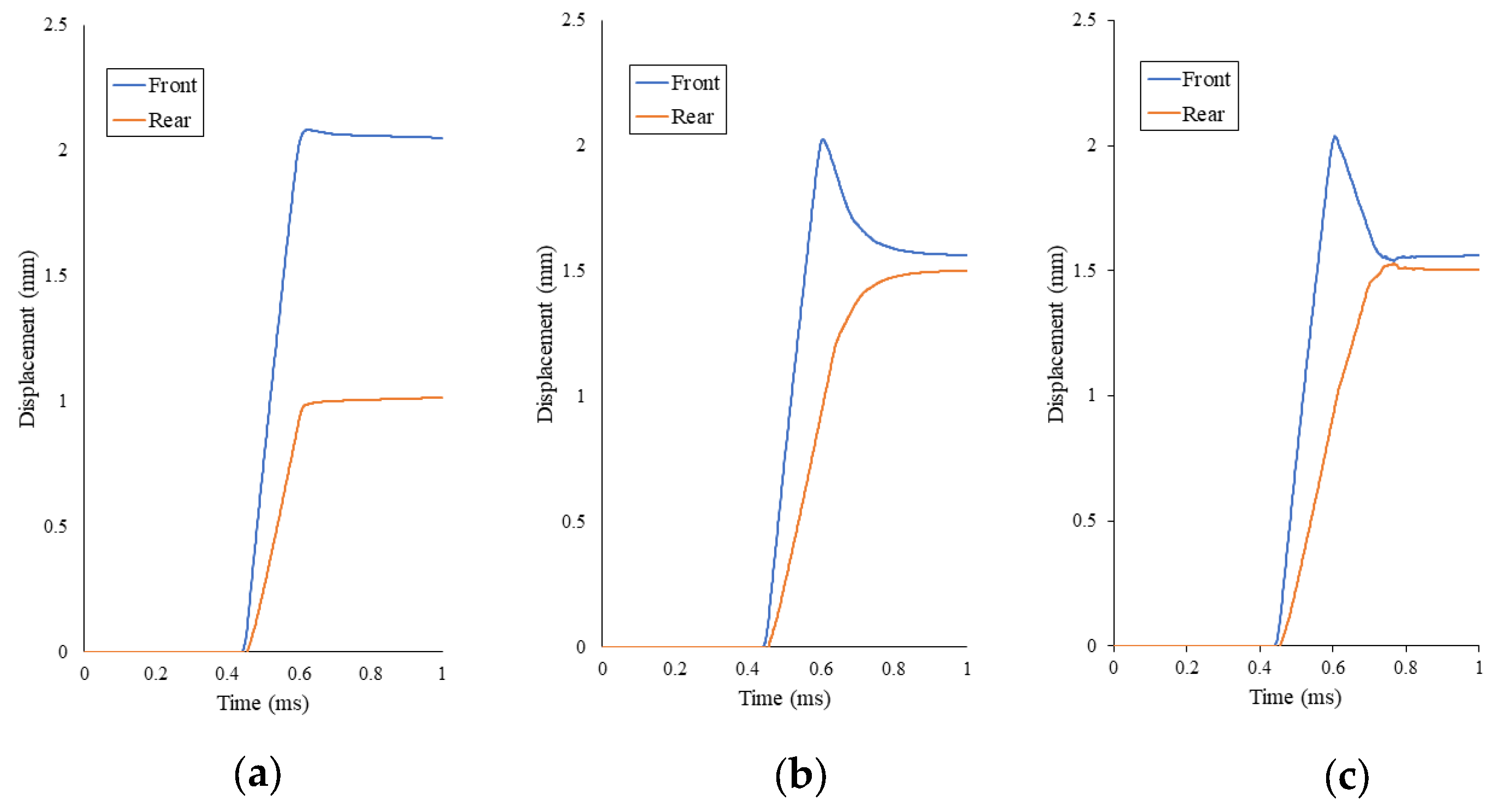
3.1.4. Dynamic Battery Compression
3.2. Numerical Results
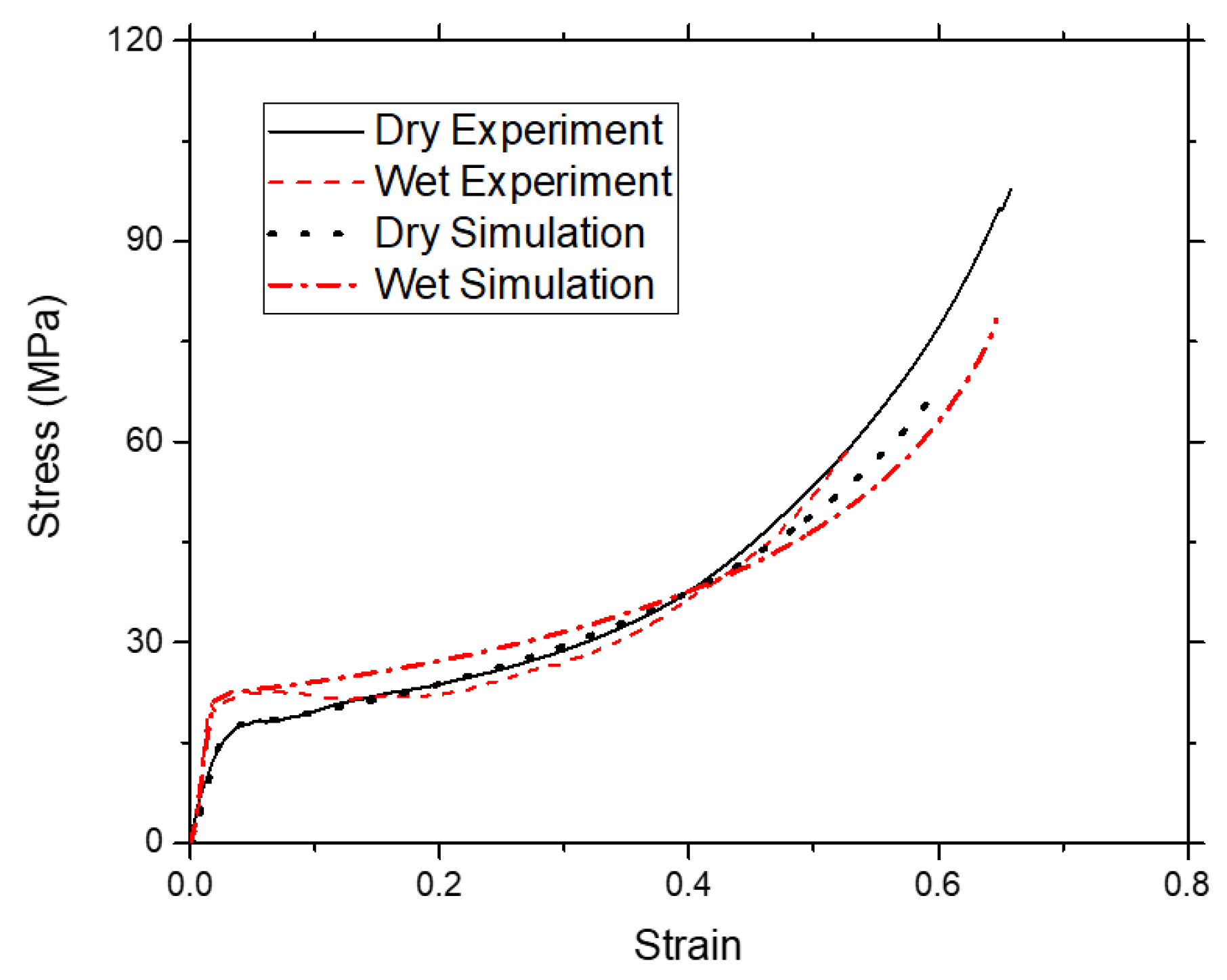
3.2.1. Static Experiment
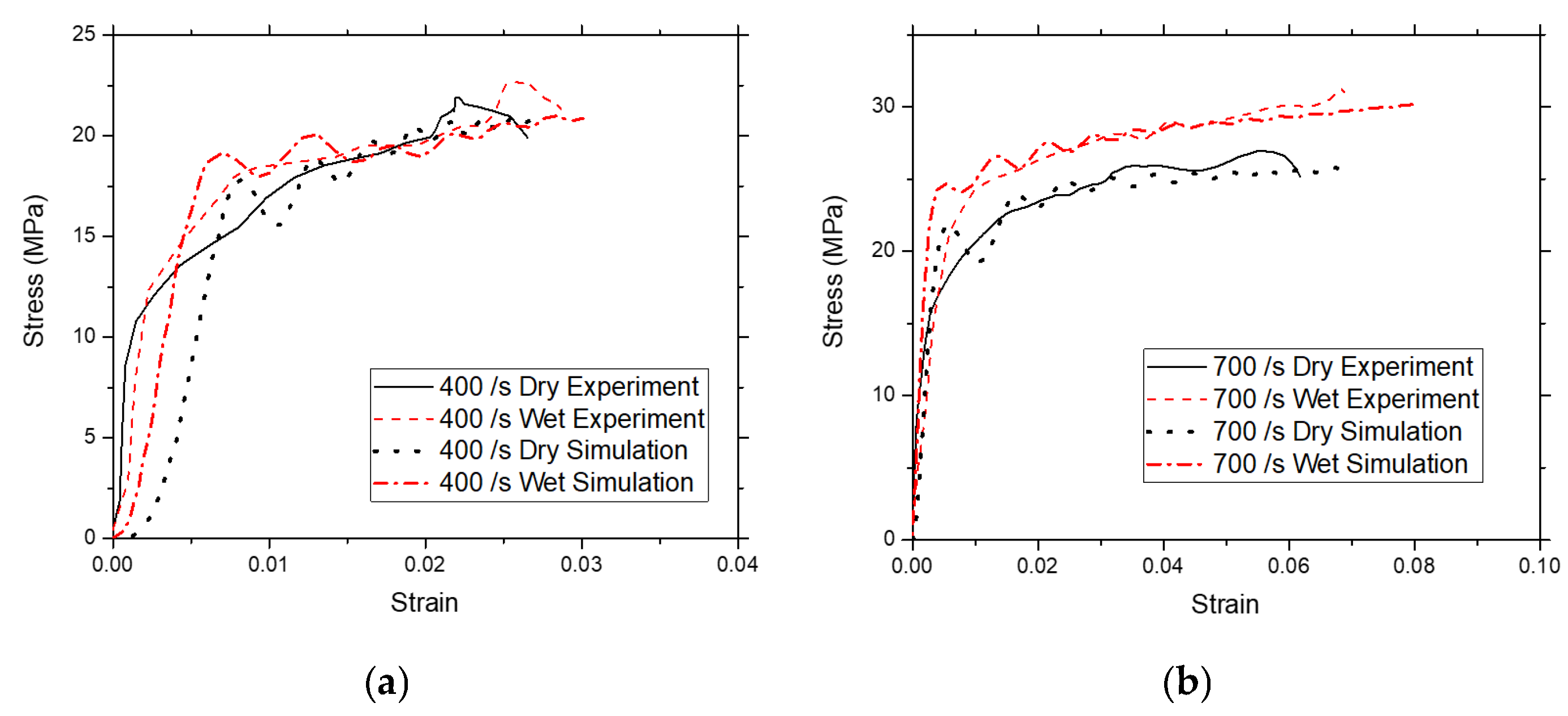
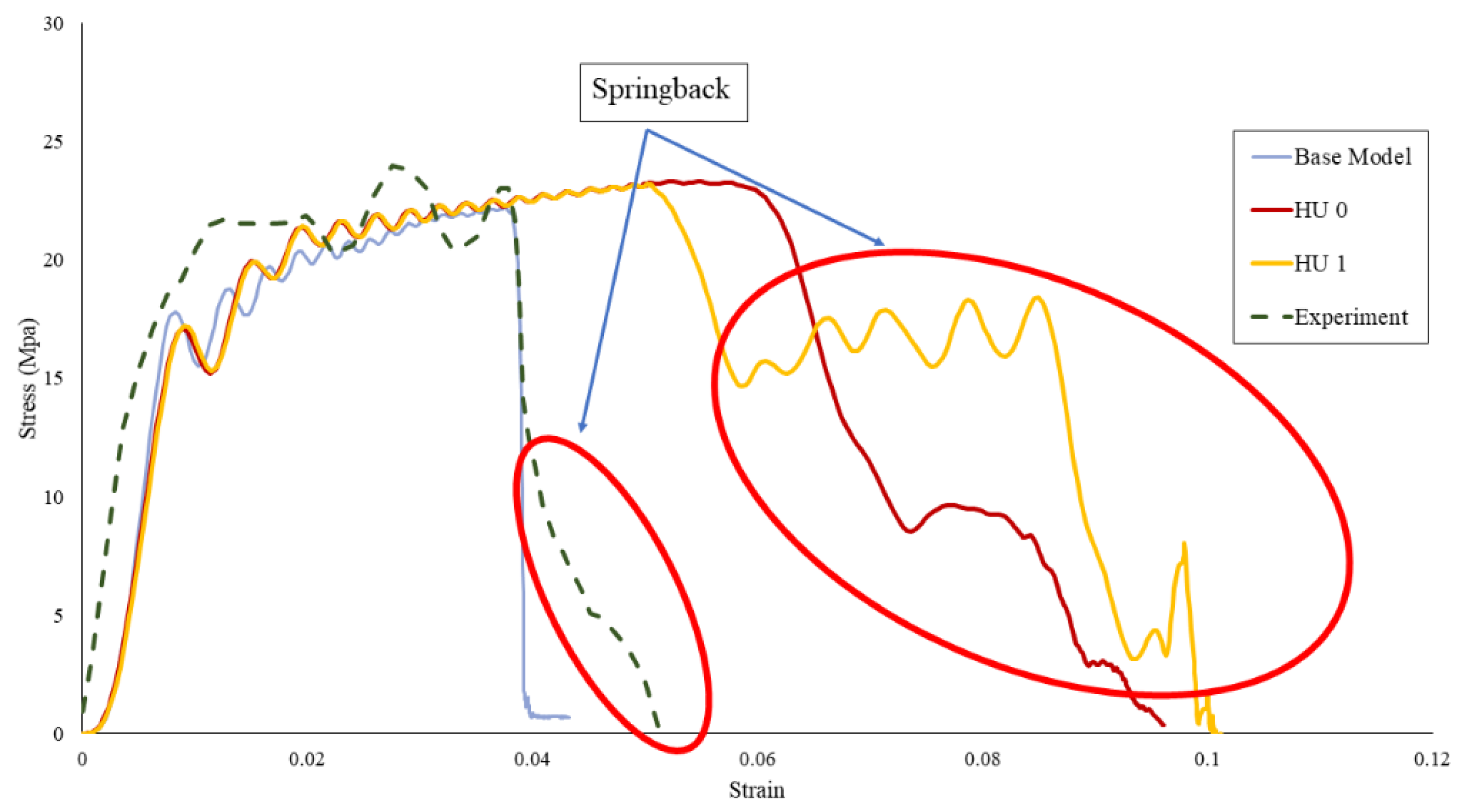
3.2.2. Dynamic Experiment
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- International Energy Agency. Global EV Outlook 2018; International Energy Agency: Paris, France, 2018; 143p. [Google Scholar]
- Jensen, C. Chevy Volt Fire Prompts Federal Investigation into Lithium-Ion Batteries. 2011. Available online: https://wheels.blogs.nytimes.com/2011/11/11/chevy-volt-fire-prompts-federal-investigation-into-lithium-ion-batteries/ (accessed on 20 February 2019).
- ABC7. Tesla Driver Killed in Fiery Crash on Highway 101 in Mountain View Identified; ABC7: San Francisco, CA, USA, 2018. [Google Scholar]
- Kuzin, V. The Details of the Tesla Electric Car Fire in Moscow Have Been Published. 2019. Available online: https://4pda.to/2019/08/12/360223/ (accessed on 21 February 2019).
- Mendoza, A. Tesla Slams into Lake Forest Garage, Severely Damaging It and Sparking a Fire. 2017. Available online: https://www.ocregister.com/2017/08/25/tesla-slams-into-lake-forest-garage-severely-damaging-it-and-sparking-a-fire/ (accessed on 20 February 2019).
- Sean, G. Everything We Know about the Chevy Bolt EV Fires. 2021. Available online: https://electrek.co/2021/07/28/everything-we-know-about-the-chevy-bolt-ev-fires/ (accessed on 20 February 2019).
- Fred, L. Tesla Vehicle Caught on Fire While Plugged in at Supercharger Station. 2019. Available online: https://electrek.co/2019/06/01/tesla-fire-supercharger/ (accessed on 20 February 2019).
- Porsche Catches Fire While Charging. 2018. Available online: https://www.bangkokpost.com/thailand/general/1429518/porsche-catches-fire-while-charging (accessed on 20 February 2019).
- Tesla Car Catches Fire in Hong Kong Parking Lot: Media. 2019. Available online: https://www.reuters.com/article/technology/tesla-car-catches-fire-in-hong-kong-parking-lot-media-idUSKCN1SK0EO/ (accessed on 20 February 2019).
- Lambert, F. Porsche Taycan Caught on Fire—Burning down a Garage in Florida. 2020. Available online: https://electrek.co/2020/02/17/porsche-taycan-fire-burning-garage-florida/ (accessed on 20 February 2019).
- Cori-Manocchio, V. Electric Car Catches Fire and Explodes in Île-Bizard Garage. 2019. Available online: https://www.cbc.ca/news/canada/montreal/electric-car-catches-fire-and-explodes-in-%C3%AEle-bizard-garage-1.5227665 (accessed on 22 February 2019).
- Sheidaei, A.; Xiao, X.; Huang, X.; Hitt, J. Mechanical Behavior of a Battery Separator in Electrolyte Solutions. J. Power Sources 2011, 196, 8728–8734. [Google Scholar] [CrossRef]
- Kalnaus, S.; Wang, Y.; Turner, J.A. Mechanical Behavior and Failure Mechanisms of Li-Ion Battery Separators. J. Power Sources 2017, 348, 255–263. [Google Scholar] [CrossRef]
- Sahraei, E.; Bosco, E.; Dixon, B.; Lai, B. Microscale Failure Mechanisms Leading to Internal Short Circuit in Li-Ion Batteries under Complex Loading Scenarios. J. Power Sources 2016, 319, 56–65. [Google Scholar] [CrossRef]
- Wang, L.; Yin, S.; Zhang, C.; Huan, Y.; Xu, J. Mechanical Characterization and Modeling for Anodes and Cathodes in Lithium-Ion Batteries. J. Power Sources 2018, 392, 265–273. [Google Scholar] [CrossRef]
- Zhang, C.; Xu, J.; Cao, L.; Wu, Z.; Santhanagopalan, S. Constitutive Behavior and Progressive Mechanical Failure of Electrodes in Lithium-Ion Batteries. J. Power Sources 2017, 357, 126–137. [Google Scholar] [CrossRef]
- Zhang, X.; Wierzbicki, T. Characterization of Plasticity and Fracture of Shell Casing of Lithium-Ion Cylindrical Battery. J. Power Sources 2015, 280, 47–56. [Google Scholar] [CrossRef]
- Sahraei, E.; Campbell, J.; Wierzbicki, T. Modeling and Short Circuit Detection of 18650 Li-Ion Cells under Mechanical Abuse Conditions. J. Power Sources 2012, 220, 360–372. [Google Scholar] [CrossRef]
- Zhu, J.; Zhang, X.; Sahraei, E.; Wierzbicki, T. Deformation and Failure Mechanisms of 18650 Battery Cells under Axial Compres-Sion. J. Power Sources 2016, 336, 332–340. [Google Scholar] [CrossRef]
- Kisters, T.; Gilaki, M.; Nau, S.; Sahraei, E. Modeling of Dynamic Mechanical Response of Li-Ion Cells with Homogenized Elec-Trolyte-Solid Interactions. J. Energy Storage 2022, 49, 104069. [Google Scholar] [CrossRef]
- Kisters, T.; Sahraei, E.; Wierzbicki, T. Dynamic Impact Tests on Lithium-Ion Cells. Int. J. Impact Eng. 2017, 108, 205–216. [Google Scholar] [CrossRef]
- Xia, Y.; Chen, G.; Zhou, Q.; Shi, X.; Shi, F. Failure Behaviours of 100% SOC Lithium-Ion Battery Modules under Different Impact Loading Conditions. Eng. Fail. Anal. 2017, 82, 149–160. [Google Scholar] [CrossRef]
- Xu, J.; Liu, B.; Wang, X.; Hu, D. Computational Model of 18650 Lithium-Ion Battery with Coupled Strain Rate and SOC Depend-Encies. Appl. Energy 2016, 172, 180–189. [Google Scholar] [CrossRef]
- Spielbauer, M.; Berg, P.; Ringat, M.; Bohlen, O.; Jossen, A. Experimental Study of the Impedance Behavior of 18650 Lithium-Ion Battery Cells under Deforming Mechanical Abuse. J. Energy Storage 2019, 26, 101039. [Google Scholar] [CrossRef]
- Afdhal. Development fof Split Hopkinson Pressure Bar to Measure Mechanical Properties of Material at High Strain Rates; Institut Teknologi Bandung: Bandung, Indonesia, 2015. [Google Scholar]
- Sahraei, E.; Hill, R.; Wierzbicki, T. Calibration and Finite Element Simulation of Pouch Lithium-Ion Batteries for Mechanical Integrity. J. Power Sources 2012, 201, 307–321. [Google Scholar] [CrossRef]
- Chen, W.W.; Song, B. Split Hopkinson (Kolsky) Bar: Design, Testing and Applications; Springer: New York, NY, USA, 2011. [Google Scholar]
- Pankow, M.; Attard, C.; Waas, A.M. Specimen Size and Shape Effect in Split Hopkinson Pressure Bar Testing. J. Strain Anal. Eng. Des. 2009, 44, 689–698. [Google Scholar] [CrossRef]
- Sheikh, M.; Elmarakbi, A.; Elkady, M. Thermal Runaway Detection of Cylindrical 18650 Lithium-Ion Battery under Quasi-Static Loading Conditions. J. Power Sources 2017, 370, 61–70. [Google Scholar] [CrossRef]
- Jia, Y.; Yin, S.; Liu, B.; Zhao, H.; Yu, H.; Li, J.; Xu, J. Unlocking the Coupling Mechanical-Electrochemical Behavior of Lithium-Ion Battery upon Dynamic Mechanical Loading. Energy 2019, 166, 951–960. [Google Scholar] [CrossRef]
- Zhu, J.; Luo, H.; Li, W.; Gao, T.; Xia, Y.; Wierzbicki, T. Mechanism of Strengthening of Battery Resistance under Dynamic Loading. Int. J. Impact Eng. 2019, 131, 78–84. [Google Scholar] [CrossRef]
- Fan, L.; Guo, X.; Li, W. Rational Design of Prussian Blue Analogue-Derived Manganese-Iron Oxides-Based Hybrids as High-Performance Li-Ion-Battery Anodes. Chin. Chem. Lett. 2023, 34, 107447. [Google Scholar] [CrossRef]
- Du, M.; Geng, P.; Jiang, X. High-Entropy Prussian Blue Analogues and Their Oxide Family as Sulfur Hosts for Lithium-Sulfur Batteries. Angew. Chem. Int. Ed. 2022, 61, e202209350. [Google Scholar] [CrossRef] [PubMed]



| Part | Length (mm) | Diameter (mm) |
|---|---|---|
| Impactor | 200 | 18 |
| Incident Bar | 1000 | 18 |
| Transmitter Bar | 1000 | 18 |
| Specimen | 17 | 17 |
| Material Model | Parameter | Value |
|---|---|---|
| Fu-Chang foam Dry | Density (kg/m3) | 1600 |
| Young’s modulus (GPa) | 20 | |
| HU | 0.01 | |
| SHAPE | 200 | |
| Fu-Chang foam Wet | Density (kg/m3) | 2000 |
| Young’s modulus (GPa) | 47.9 | |
| HU | 0.01 | |
| SHAPE | 200 |
| State-of-Charge (%) | Loading Condition | Specimen | Peak Load (kN) | Voltage Cutoff (mm) | Average Peak Load (kN) | Average Cutoff (mm) |
|---|---|---|---|---|---|---|
| 0 | Static | Specimen-1 | 11 | 5 | 10.36 | 6.45 |
| Specimen-2 | 10.8 | 7.2 | ||||
| Specimen-3 | 9.28 | 7.15 | ||||
| Drop weight 0.5 m | Specimen-1 | 12.18 | 9.63 | 12.96 | 7.48 | |
| Specimen-2 | 13.72 | 8.16 | ||||
| Specimen-3 | 12.97 | 4.66 | ||||
| Drop weight 1 m | Specimen-1 | 16.67 | 5.83 | 15.62 | 6.21 | |
| Specimen-2 | 14.71 | 8.28 | ||||
| Specimen-3 | 15.48 | 4.52 | ||||
| 25 | Static | Specimen-1 | 8.84 | 4.6 | 9.76 | 5.26 |
| Specimen-2 | 11.4 | 5.48 | ||||
| Specimen-3 | 9.04 | 5.7 | ||||
| Drop weight 0.5 m | Specimen-1 | 12.18 | 2.58 | 13.73 | 4.83 | |
| Specimen-2 | 13.55 | 6.88 | ||||
| Specimen-3 | 15.45 | 5.04 | ||||
| 50 | Static | Specimen-1 | 11.34 | 5.4 | 8.45 | 4.3 |
| Specimen-2 | 9 | 4 | ||||
| Specimen-3 | 5 | 3.5 | ||||
| Drop weight 0.5 m | Specimen-1 | 15.03 | 5.28 | 14.41 | 5.41 | |
| Specimen-2 | 15.56 | - | ||||
| Specimen-3 | 12.65 | 5.54 | ||||
| 75 | Static | Specimen-1 | 9.31 | 4.2 | 9.84 | 4.25 |
| Specimen-2 | 9.32 | 3.16 | ||||
| Specimen-3 | 10.9 | 5.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Santosa, S.P.; Fadillah, H. Modeling and Characterization of Li-Ion 18650 Nickel–Cobalt–Alumina Battery Jellyroll Subjected to Static and Dynamic Compression Loading. Energies 2024, 17, 4967. https://doi.org/10.3390/en17194967
Santosa SP, Fadillah H. Modeling and Characterization of Li-Ion 18650 Nickel–Cobalt–Alumina Battery Jellyroll Subjected to Static and Dynamic Compression Loading. Energies. 2024; 17(19):4967. https://doi.org/10.3390/en17194967
Chicago/Turabian StyleSantosa, Sigit Puji, and Hafiz Fadillah. 2024. "Modeling and Characterization of Li-Ion 18650 Nickel–Cobalt–Alumina Battery Jellyroll Subjected to Static and Dynamic Compression Loading" Energies 17, no. 19: 4967. https://doi.org/10.3390/en17194967
APA StyleSantosa, S. P., & Fadillah, H. (2024). Modeling and Characterization of Li-Ion 18650 Nickel–Cobalt–Alumina Battery Jellyroll Subjected to Static and Dynamic Compression Loading. Energies, 17(19), 4967. https://doi.org/10.3390/en17194967






