A Thermodynamic Comparison of the Exergy Production from Sugarcane and Photovoltaic Modules in Brazilian Energy Transition Context
Abstract
1. Introduction
2. Methods
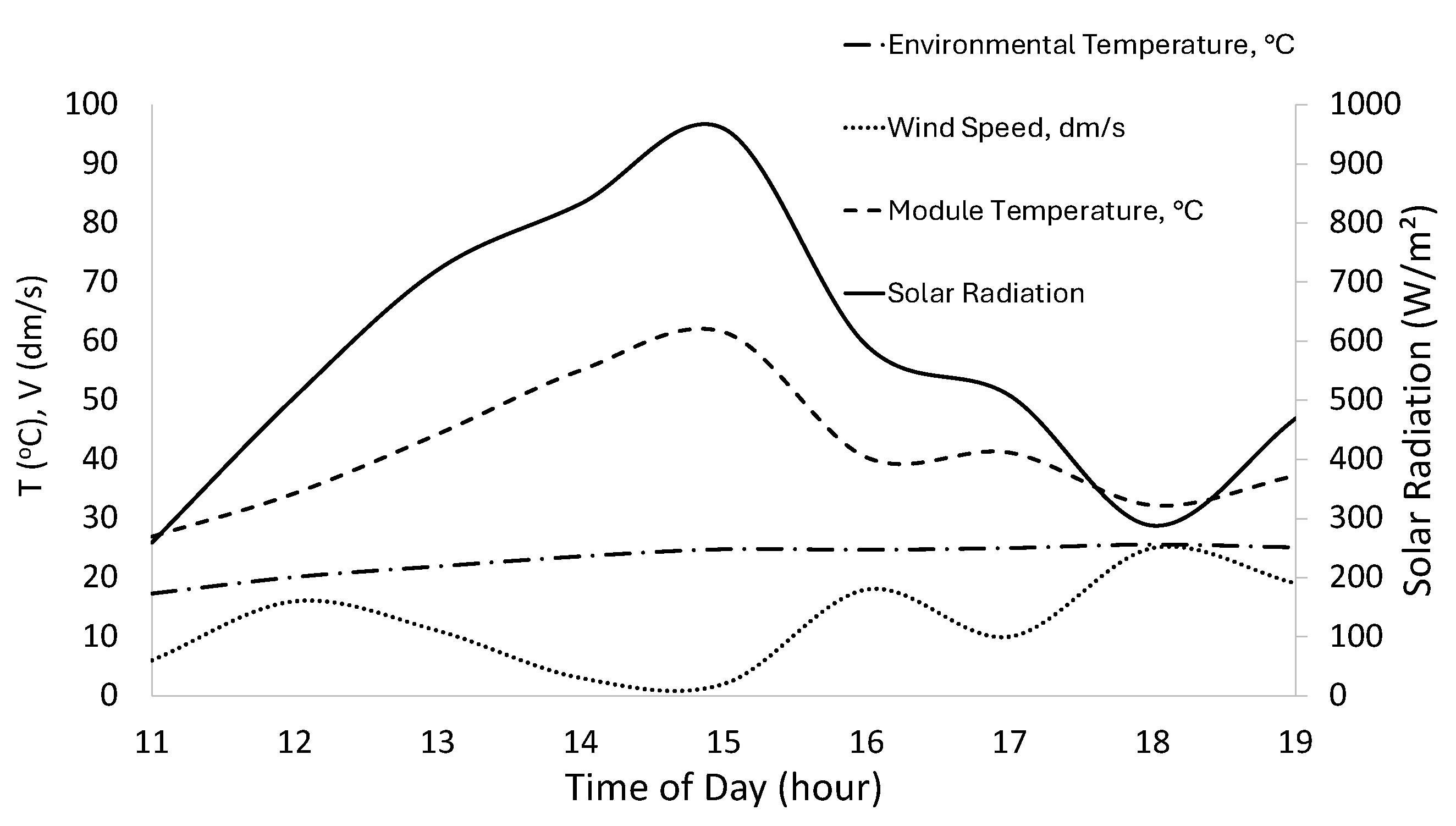
2.1. Solar Radiation
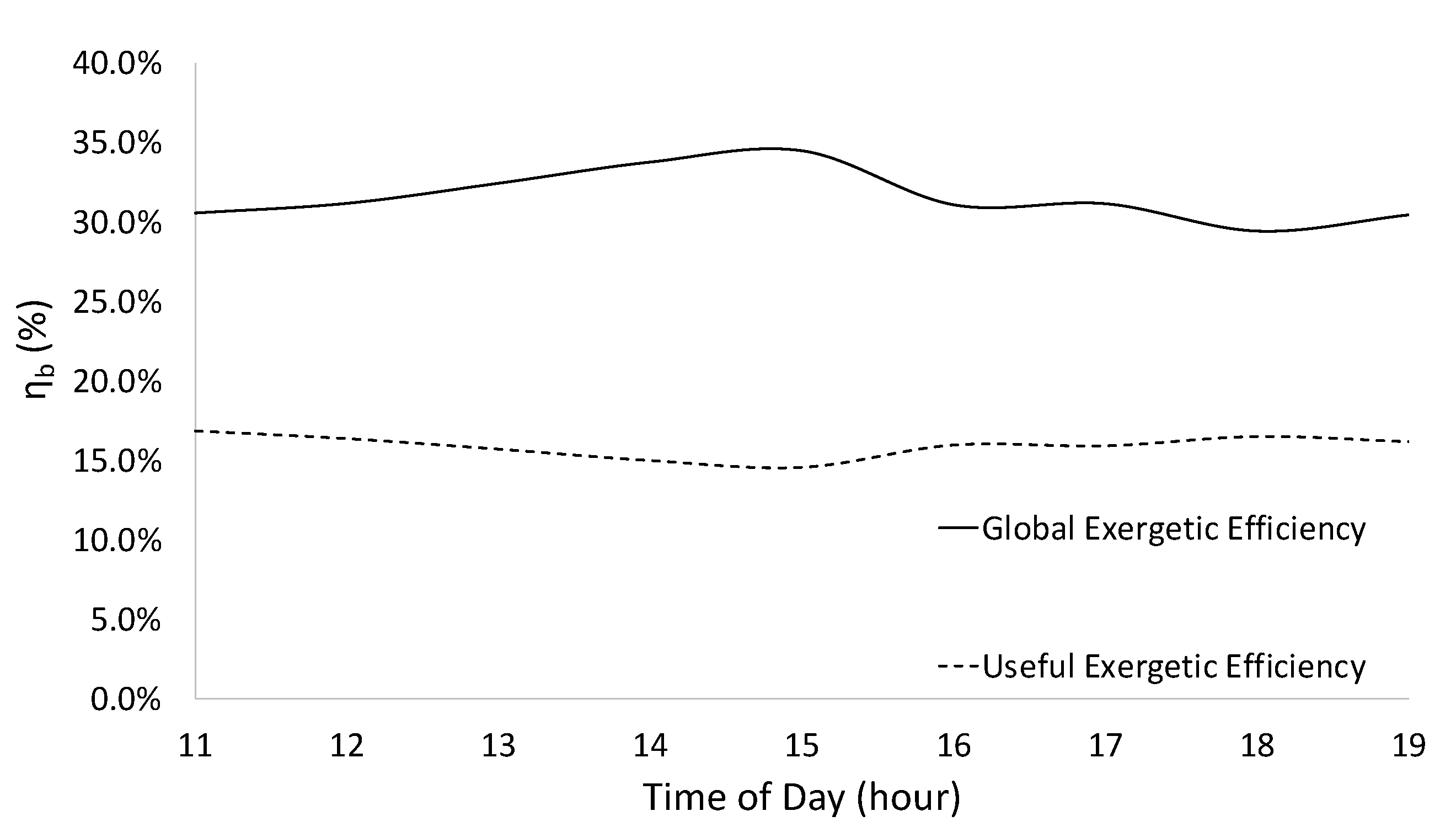
2.2. Exergy Analysis
2.3. Biorefinary
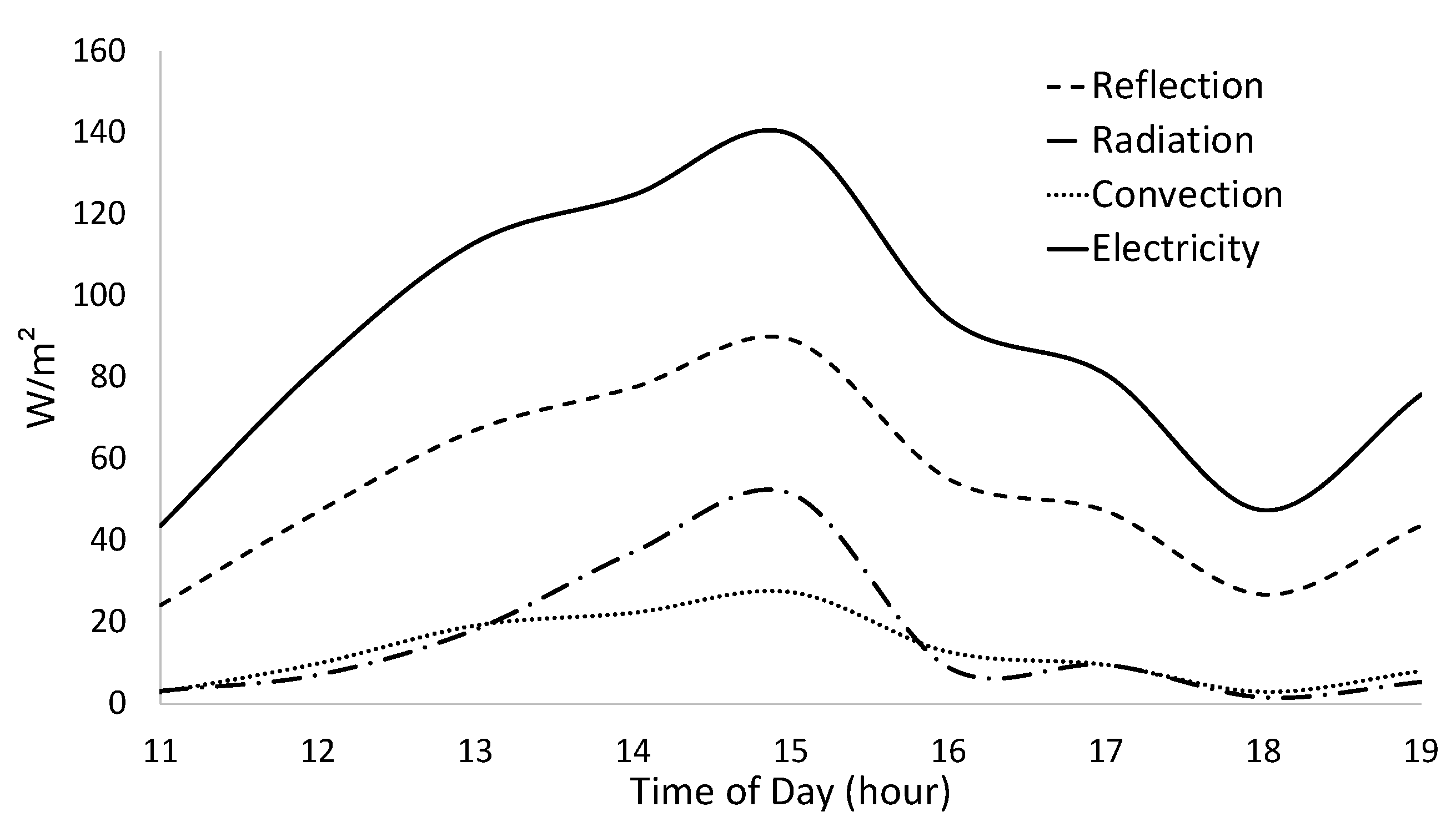
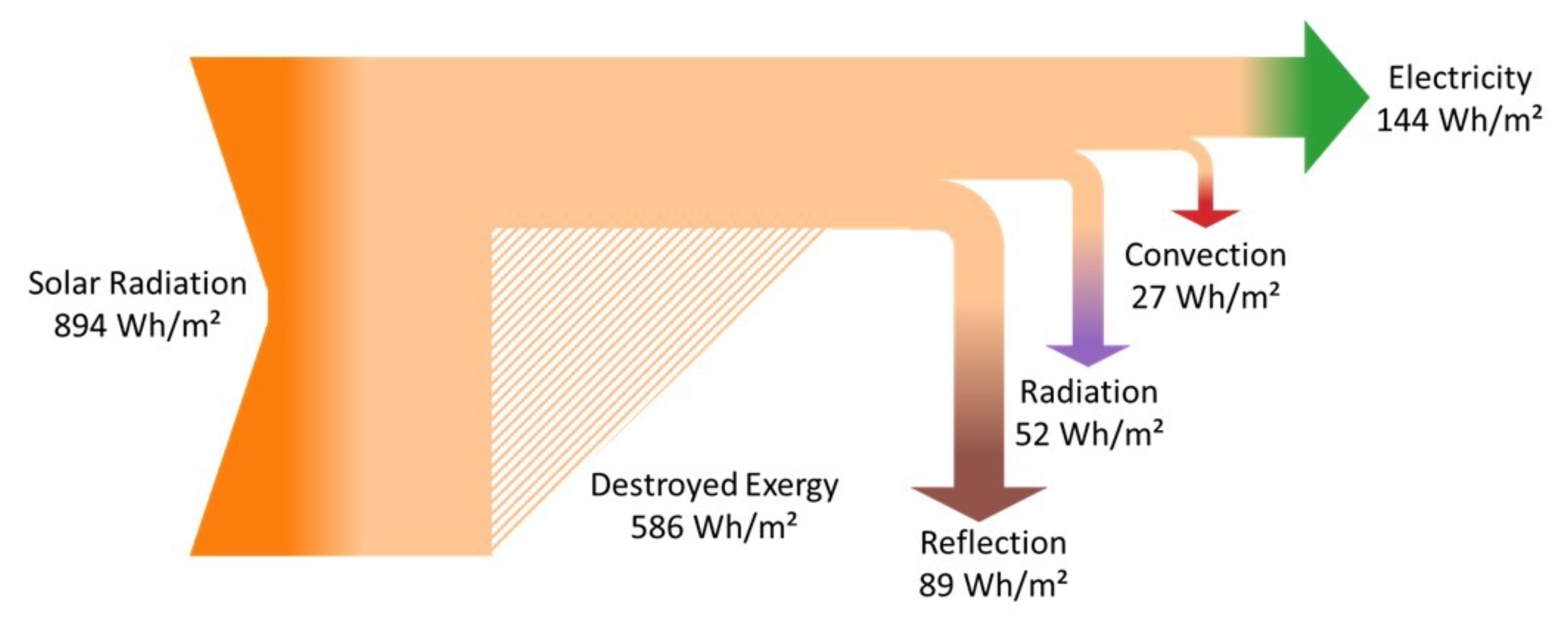
3. Photovoltaic Panels
4. Results and Discussions
5. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Angström–Prescott coefficient | |
| Angström–Prescott coefficient | |
| n | Actual insolation |
| N | Photoperiod |
| Solar irradiance [] | |
| Solar irradiance that reaches the ground [] | |
| V | Velocity [] |
| A | Surface area [] |
| Mass flow rate [kg/s] | |
| Exergy content of the control volume [kJ, kWh] | |
| Destroyed exergy rate [kW] | |
| T | Temperature [oC, K] |
| Temperature obtained from the energy balance [oC, K] | |
| Property at environmental conditions | |
| h | Specific enthalpy [kJ/kg], heat transfer coefficient [W/(m2K)] |
| s | Specific entropy [kJ/(kgK)] |
| b | Specific exergy [kJ/kg] |
| Heat transfer rate [kW] | |
| Heat transfer rate associated with radiation [kW] | |
| Heat transfer rate associated with convection [kW] | |
| Exergy rate [kW] | |
| Exergy rate associated with radiation [kW] | |
| Exergy rate associated with convection [kW] | |
| Exergy destroyed rate [kW] | |
| Exergy of the ethanol [kJ, kWh] | |
| Exergy of the biogas [kJ, kWh] | |
| Performed power [kW] | |
| STC | Standard test condition |
| NOCT | Nominal operating cell temperature [] |
| Thermal emissivity [-] | |
| Stefan–Boltzmann constant [] | |
| Latitude of the analyzed location | |
| Solar declination on the analyzed day | |
| Global exergy efficiency [-] | |
| Useful exergy efficiency [-] |
References
- Pereira, M.T.R.M.; Carvalho, M.; Mady, C.E.K. Adressing energy demand and climate change through the second law of thermodynamics and LCA towards a rational use of energy in Brazilian households. Entropy 2022, 24, 1524. [Google Scholar] [CrossRef] [PubMed]
- Rovai, F.F.; da Cal Seixas, S.R.; Mady, C.E.K. Regional energy policies for electrifying car fleets. Energy 2023, 278, 127908. [Google Scholar] [CrossRef]
- Righetto, F.G.; Mady, C.E.K. Exergy Analysis of a Sugarcane Crop: A Planting-to-Harvest Approach. Sustainability 2023, 15, 14686. [Google Scholar] [CrossRef]
- Barbieri, V. limatic Conditioning of the Potential Productivity of Sugarcane (Saccharum spp.): A mathematical-Physiological Estimation Model. Ph.D. Thesis, University of São Paulo, São Paulo, Brazil, 1993. [Google Scholar]
- Benvenutti, L.M.; Uriona-Maldonado, M.; Campos, L.M. The impact of CO2 mitigation policies on light vehicle fleet in Brazil. Energy Policy 2019, 126, 370–379. [Google Scholar] [CrossRef]
- Mosquim, R.F.; Mady, C.E.K. Performance and efficiency trade-offs in Brazilian passenger vehicle fleet. Energies 2022, 15, 5416. [Google Scholar] [CrossRef]
- Zhang, M.; Yuan, M.; Jiang, J. A Comprehensive Review of the Multiphase Motor Drive Topologies For High-Power Electric Vehicle: Current Status, Research Challenges, and Future Trends. IEEE Trans. Transp. Electrif. 2024. [Google Scholar] [CrossRef]
- Palacios-Bereche, R.; Mosqueira-Salazar, K.J.; Modesto, M.; Ensinas, A.V.; Nebra, S.A.; Serra, L.M.; Lozano, M.A. Exergetic analysis of the integrated first-and second-generation ethanol production from sugarcane. Energy 2013, 62, 46–61. [Google Scholar] [CrossRef]
- Tibúrcio Neto, L.; Peiter, F.; Chaves, T.; de Almeida, C.; de Amorim, E. Methane production from anaerobic co-digestion of vinasse and molasses: Effects of substrate proportion, COD and alkalizing agent. Int. J. Environ. Sci. Technol. 2024, 1–16. [Google Scholar] [CrossRef]
- Petersen, A.M.; Gorgens, J. Flowsheet analysis of bio-derived hydrogen as a surplus product at sugar mills and associated biorefineries from processing residues. Int. J. Hydrogen Energy 2024, 49, 225–237. [Google Scholar] [CrossRef]
- Kumar, J.; Vyas, S. Comprehensive review of biomass utilization and gasification for sustainable energy production. Environ. Dev. Sustain. 2024, 1–40. [Google Scholar] [CrossRef]
- Brazilian Energy Balance for 2023 Energy Research Office—EPE: Rio de Janeiro. 2023. Available online: https://www.epe.gov.br/pt/publicacoes-dados-abertos/publicacoes/balanco-energetico-nacional-2023 (accessed on 29 September 2024).
- Silva, T.C. Estudo da Implantação de uma Miniusina Solar Fotovoltaica na UNIFEI, Aspectos Técnicos, Econômicos e Exergéticos. Ph.D. Thesis, Federal University of Itajubá, Itajubá, Brazil, 2021. [Google Scholar]
- Rawat, P. Experimental Investigation of Effect of Environmental Variables on Performance of Solar Photovoltaic Module. Int. Res. J. Eng. Technol. (IRJET) 2017, 4, 13–18. [Google Scholar]
- Medeiros, F.J.d.; Silva, C.M.S.e.; Bezerra, B.G. Calibration of ångström-Prescott equation to estimate daily solar radiation on Rio Grande do Norte state, Brazil. Rev. Bras. Meteorol. 2017, 32, 409–416. [Google Scholar] [CrossRef][Green Version]
- de Oliveira Junior, S. Exergy: Production, Cost and Renewability; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Rodríguez, E.; Cardemil, J.M.; Starke, A.R.; Escobar, R. Modelling the exergy of solar radiation: A review. Energies 2022, 15, 1477. [Google Scholar] [CrossRef]
- CLIMATE-DATA. International Data Portal: Average Daily Temperature and Relative Humidity of Campinas. 2022. Available online: https://pt.climate-data.org/america-do-sul/brasil/sao-paulo/campinas-745/#climate-table (accessed on 29 September 2024).
- INMETRO. National Institute of Meteorology, Department of Agriculture, Livestock and Food Supply (MAPA). 2024. Available online: https://portal.inmet.gov.br/ (accessed on 29 September 2024).
- CRESESB. Brazlian Center for Reference in Solar and Eolic Energy Sérgio de S. Brito (CRESESB). 2024. Available online: https://cresesb.cepel.br/ (accessed on 29 September 2024).
- Salazar, K. Uso de Água e Análise Exergética na Produção Integrada de Etanol de Primeira e Segunda Geração a Partir da Cana-de-açúcar. Ph.D. Thesis, University of Campinas, Campinas, Brazil, 2012. [Google Scholar]
- Sun, V.; Asanakham, A.; Deethayat, T.; Kiatsiriroat, T. Evaluation of nominal operating cell temperature (NOCT) of glazed photovoltaic thermal module. Case Stud. Therm. Eng. 2021, 28, 101361. [Google Scholar] [CrossRef]
- Luque, A.; Hegedus, S. Handbook of Photovoltaic Science and Engineering; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Righetto, F.G. Avaliação da Qualidade dos Processos de Conversão de Energia na Cana-de-açúcar Visando o uso Racional da Energia na Sociedade. Master’s Thesis, School of Mechanical Engineering, University of Campinas, Campinas, Brazil, 2023. [Google Scholar]
- Silva, T.C.; Pinto, G.M.; de Souza, T.A.; Valerio, V.; Silvério, N.M.; Coronado, C.J.; Guardia, E.C. Technical and economical evaluation of the photovoltaic system in Brazilian public buildings: A case study for peak and off-peak hours. Energy 2020, 190, 116282. [Google Scholar] [CrossRef]
- Rawat, P. Exergy performance analysis of 300 W solar photovoltaic module. Int. J. Eng. Sci. Res. Technol. 2017, 6, 381–390. [Google Scholar]
- Watmuff, J.; Charters, W.; Proctor, D. Solar and wind induced external coefficients-solar collectors. Coop. Mediterr. Pour L’Energie Sol. 1977, 2, 56. [Google Scholar]
- Klein, S. Calculation of monthly average insolation on tilted surfaces. Sol. Energy 1977, 19, 325–329. [Google Scholar] [CrossRef]
- Martin, M.; Elnour, M.; Siñol, A.C. Environmental life cycle assessment of a large-scale commercial vertical farm. Sustain. Prod. Consum. 2023, 40, 182–193. [Google Scholar] [CrossRef]
- Xiang, X.; Zhang, Y.; Peng, L.; Lan, M.; Du, W.; Xue, S.; Jiang, T.; Jiang, X. Exploring the food-energy-water nexus in China’s national industries: Insights from network structure and production disturbances. Sustain. Prod. Consum. 2023, 43, 377–388. [Google Scholar] [CrossRef]
- Arcasi, A.; Mauro, A.; Napoli, G.; Tariello, F.; Vanoli, G. Energy and cost analysis for a crop production in a vertical farm. Appl. Therm. Eng. 2024, 239, 122129. [Google Scholar] [CrossRef]
- Restrepo-Flórez, J.M.; Cuello-Penaloza, P.; Canales, E.; Witkowski, D.; Rothamer, D.A.; Huber, G.W.; Maravelias, C.T. Ethanol to diesel: A sustainable alternative for the heavy-duty transportation sector. Sustain. Energy Fuels 2023, 7, 693–707. [Google Scholar] [CrossRef]
- Tiburcio, R.S.; de Macêdo, T.R.; Neto, A.M.P. Brazilian Biofuels Policy (RenovaBio): Overview and generation of decarbonization credits by biodiesel production facilities. Energy Sustain. Dev. 2023, 77, 101334. [Google Scholar] [CrossRef]
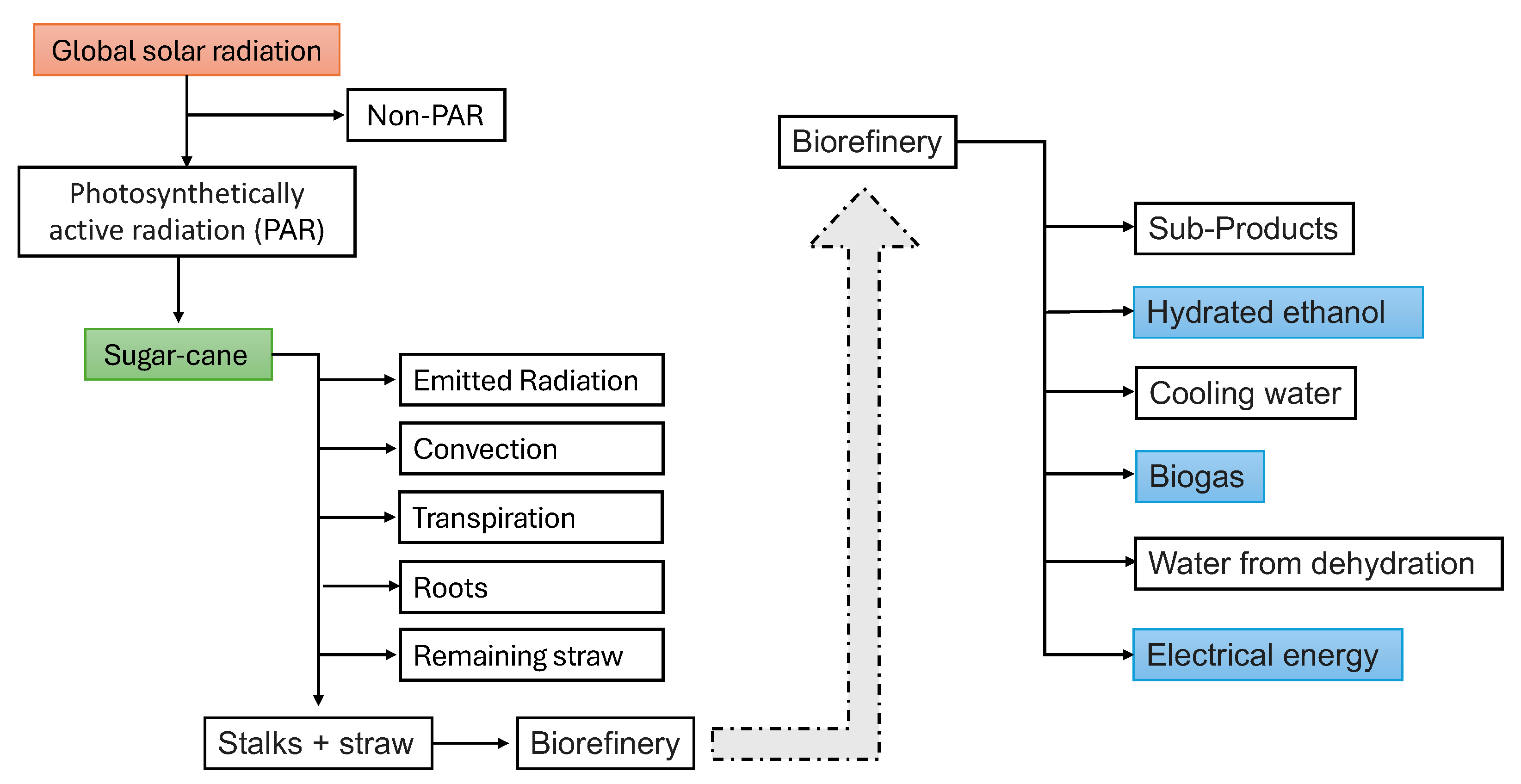
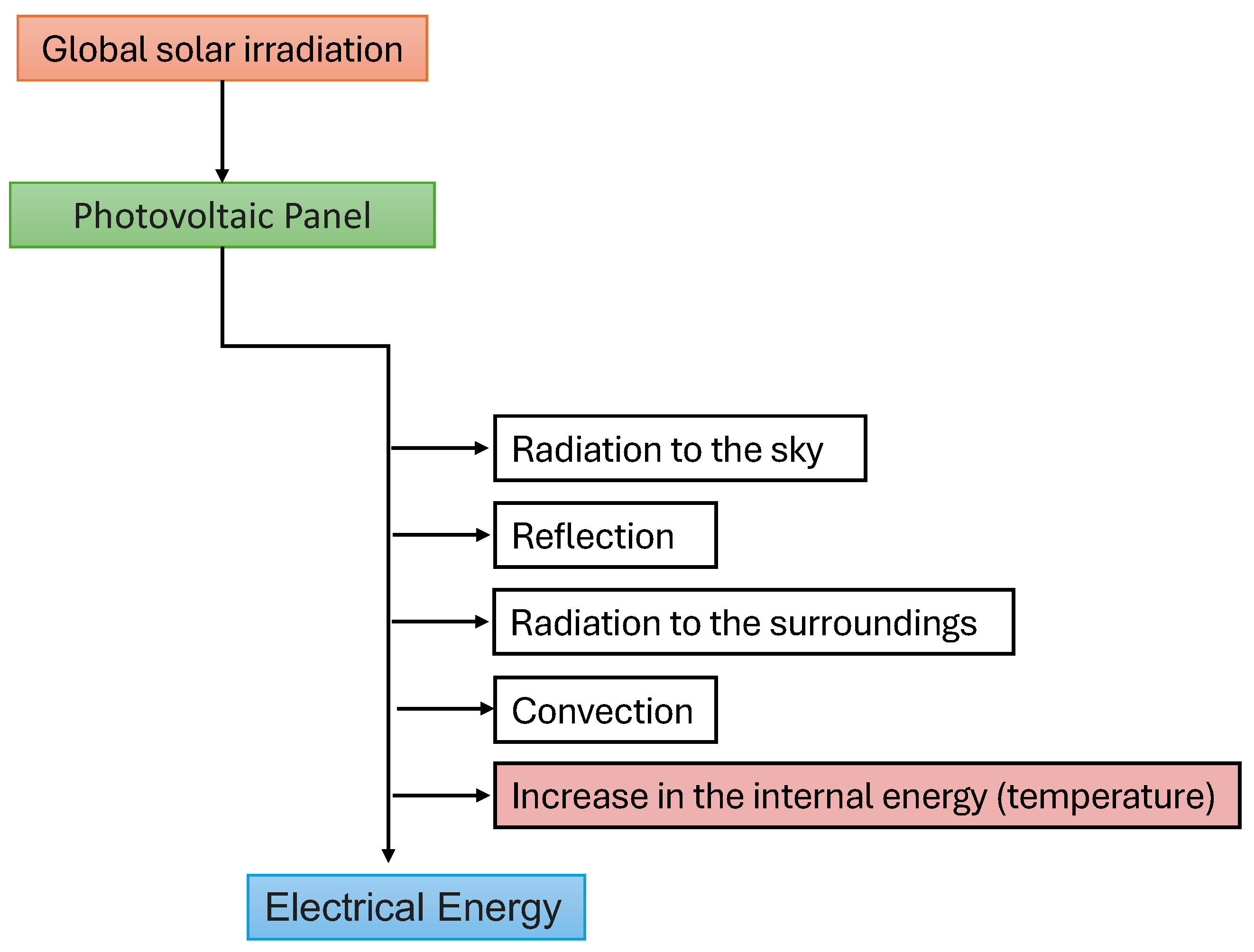
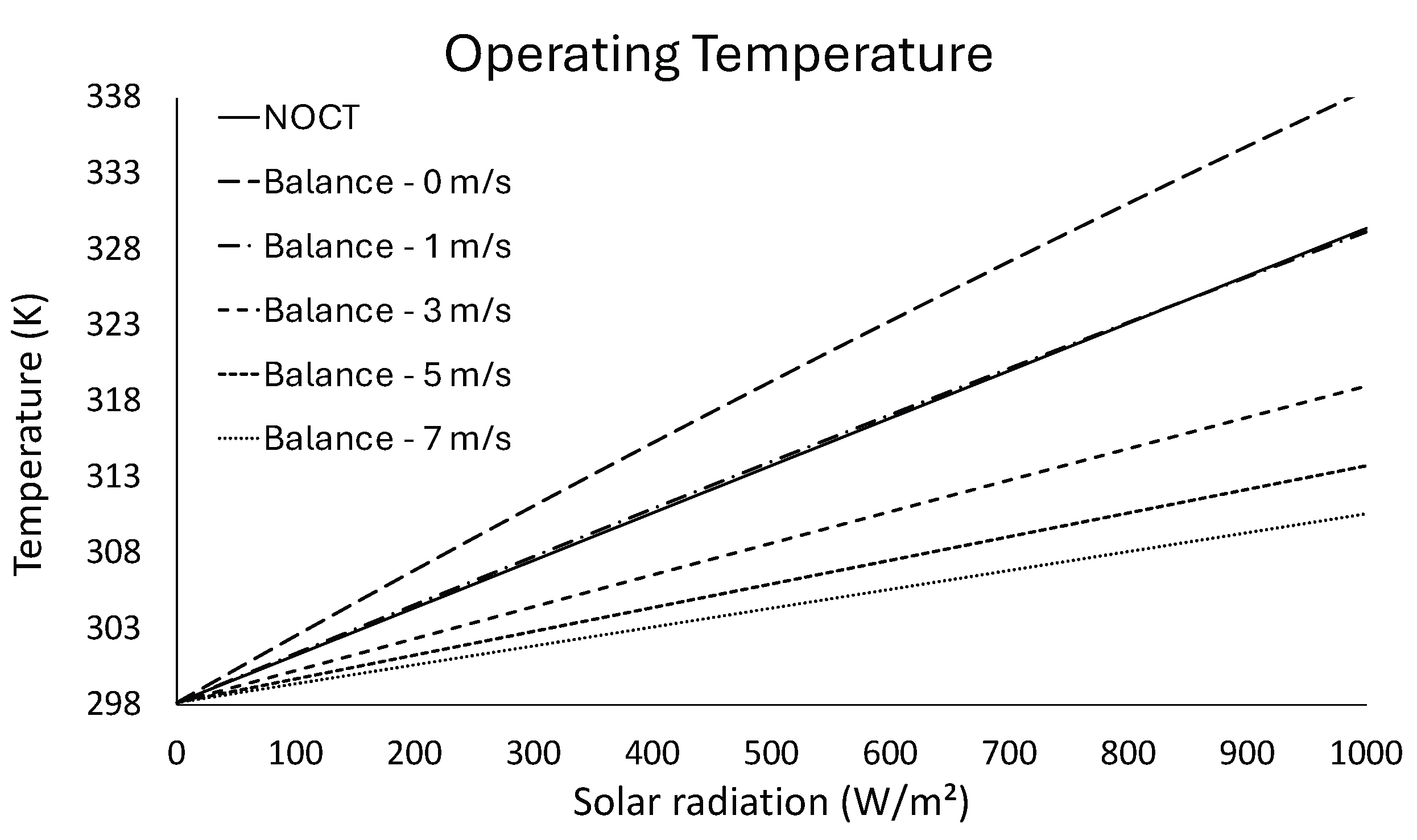
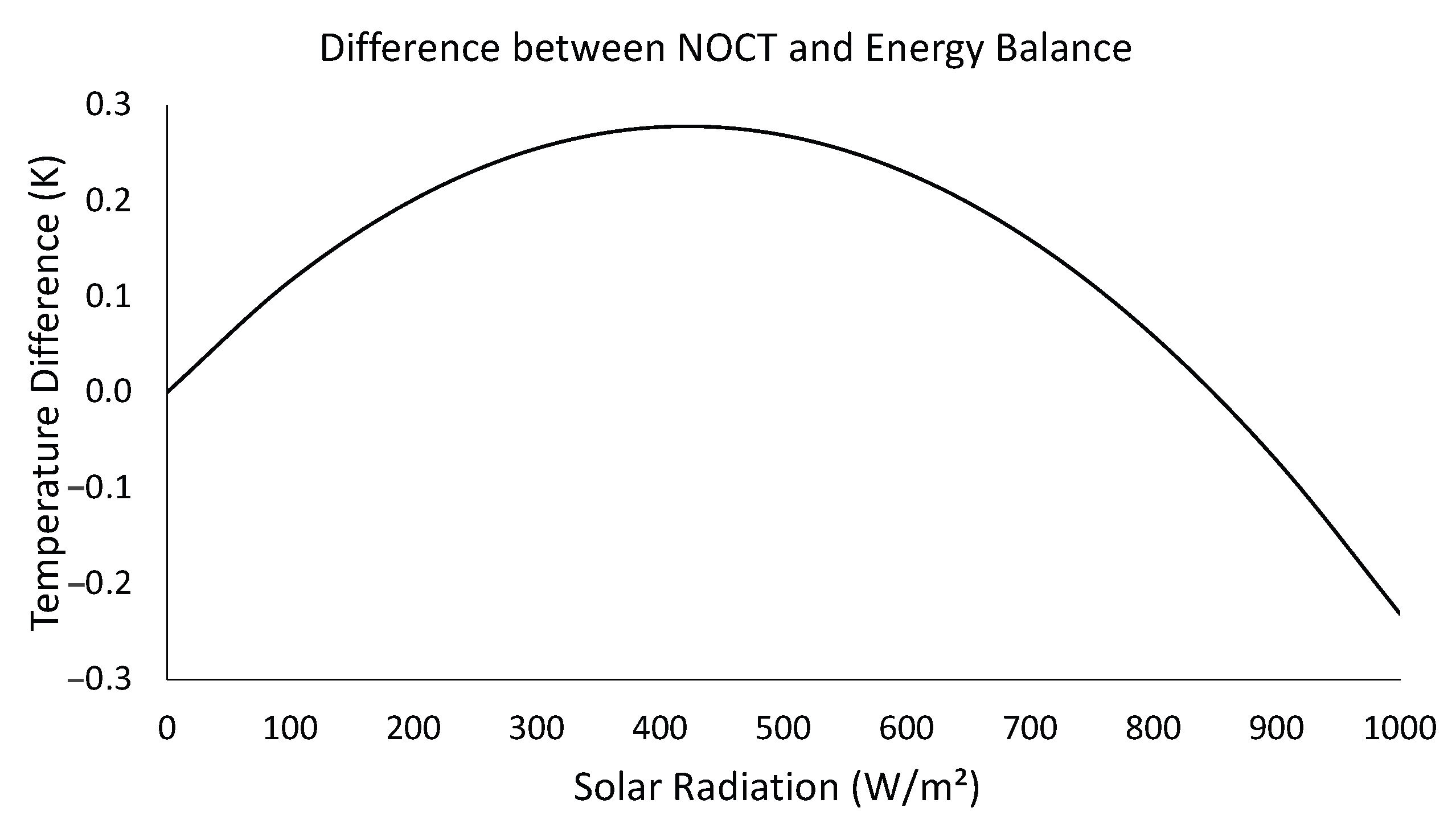
| Flow | Wh/m2 | |
|---|---|---|
| Input | Culms | 12,913.00 |
| Straw | 2767.00 | |
| Output | Byproducts | 150.00 |
| Hydrated Ethanol | 4504.00 | |
| Cooling Water | 84.00 | |
| Biogas | 451.00 | |
| Dehydration water | 153.00 | |
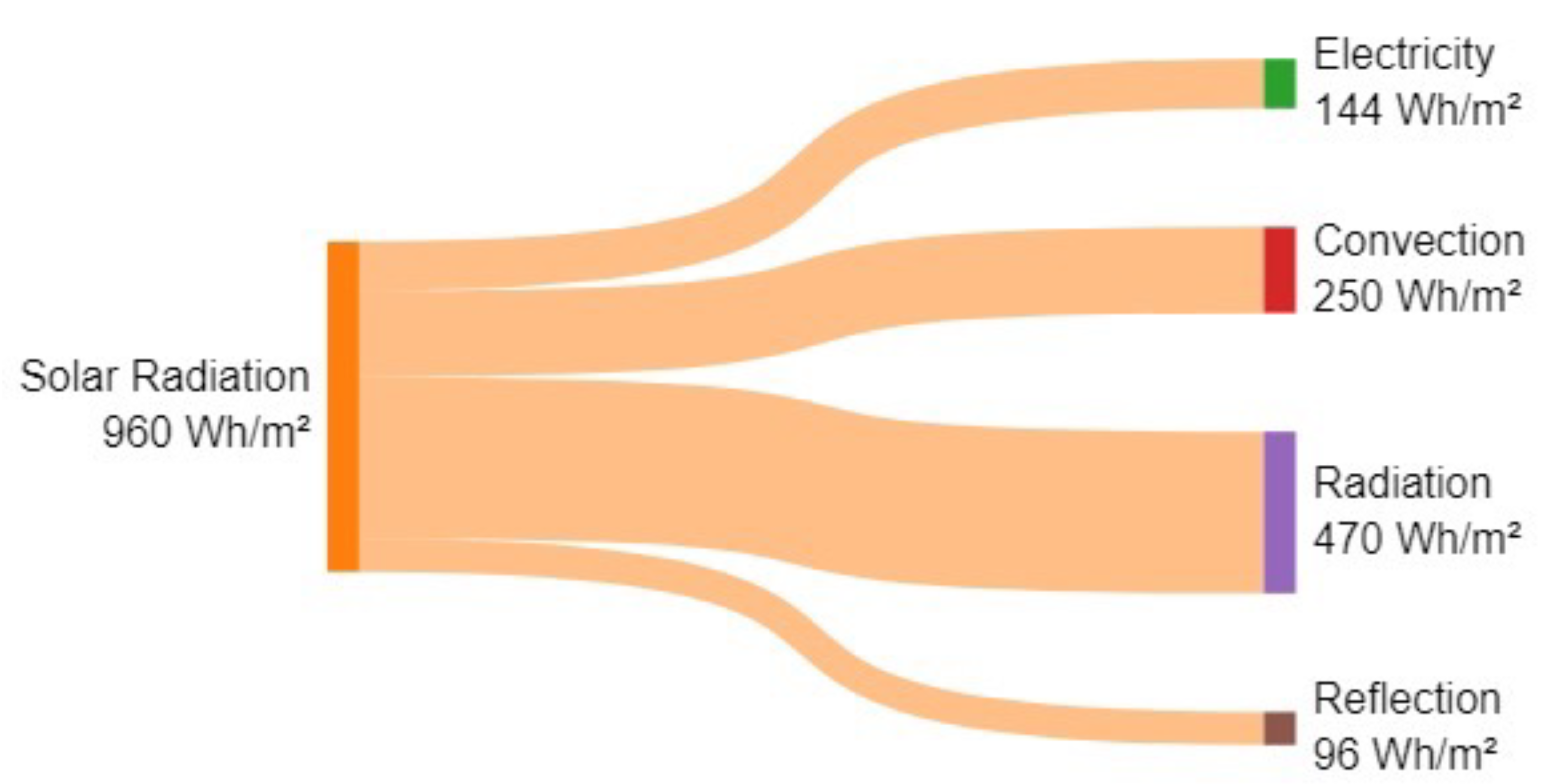
| Electricity | 628.00 |
| Flow | Wh/m2 | |
|---|---|---|
| Input | Ground radiation | 1,481,365.00 |
| Output | Remaining straw | 2173.00 |
| Roots | 2608 | |
| Heat by radiation | 2752.00 | |
| Heat by convection | 1057.00 | |
| Heat by transpiration | 22,422.00 | |
| Byproducts | 150.12 | |
| Hydrated ethanol | 4504.00 |
| Sugarcane | (Wh/m2) | Photovoltaic | (Wh/m2) | |
|---|---|---|---|---|
| Useful Product | 450.8 | 255,838.0 | ||
| 4503.80 | ||||
| 628.0 | ||||
| Total Useful | 5582.60 | 255,838.00 | ||
| Global Total | 36,981.00 | 468,004.00 | ||
| 1,481,365.00 | 1,481,365.00 | |||
| 2.50% | 31.60% | |||
| 0.38% | 17.30% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Righetto, F.G.; Mady, C.E.K. A Thermodynamic Comparison of the Exergy Production from Sugarcane and Photovoltaic Modules in Brazilian Energy Transition Context. Energies 2024, 17, 4940. https://doi.org/10.3390/en17194940
Righetto FG, Mady CEK. A Thermodynamic Comparison of the Exergy Production from Sugarcane and Photovoltaic Modules in Brazilian Energy Transition Context. Energies. 2024; 17(19):4940. https://doi.org/10.3390/en17194940
Chicago/Turabian StyleRighetto, Felipe Godoy, and Carlos Eduardo Keutenedjian Mady. 2024. "A Thermodynamic Comparison of the Exergy Production from Sugarcane and Photovoltaic Modules in Brazilian Energy Transition Context" Energies 17, no. 19: 4940. https://doi.org/10.3390/en17194940
APA StyleRighetto, F. G., & Mady, C. E. K. (2024). A Thermodynamic Comparison of the Exergy Production from Sugarcane and Photovoltaic Modules in Brazilian Energy Transition Context. Energies, 17(19), 4940. https://doi.org/10.3390/en17194940







