A Bi-Level Peak Regulation Optimization Model for Power Systems Considering Ramping Capability and Demand Response
Abstract
1. Introduction
2. Calculation Method for Ramping Demand
2.1. Deterministic Ramping Demand
2.2. Ramping Demand Considering Uncertainty
3. Bi-Level Peak Regulation Optimization Model
3.1. Upper-Level Model
- Constraints of the load adjustment amount
- 2.
- Constraints of price-driven demand response
- 3.
- Constraints of electricity usage satisfaction and electricity cost satisfaction
3.2. Lower-Level Model
- Power balance constraints
- 2.
- Power flow constraints
- 3.
- Voltage constraints
- 4.
- Thermal power unit ramping capability constraints
- 5.
- Stepwise ramping rate constraints of thermal power units
- 6.
- System reserve constraints
- 7.
- Generation output constraints of thermal power units
- 8.
- Logical constraints
- 9.
- Operation constraints of carbon capture units
- 10.
- Operation constraints of energy storage
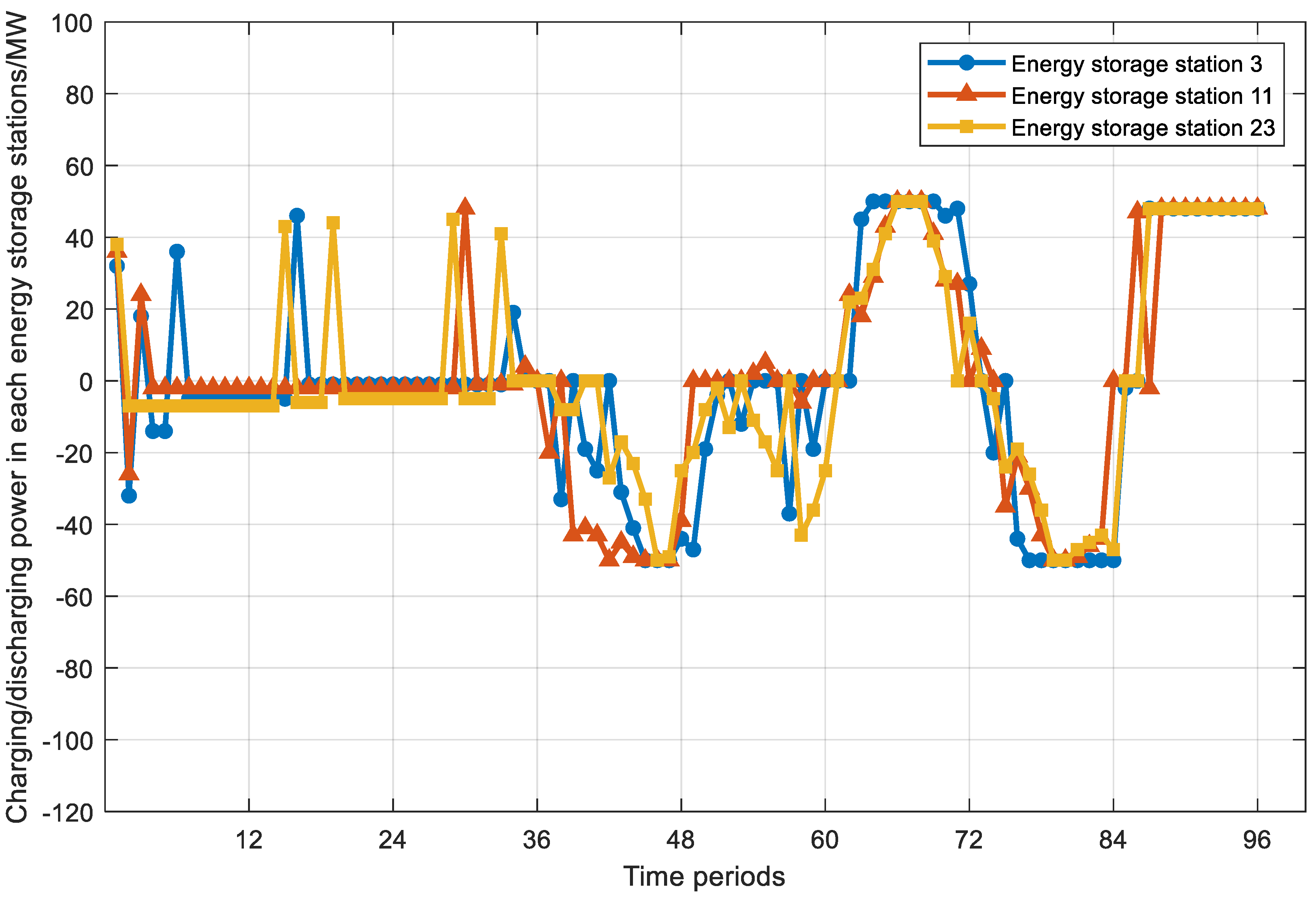
4. Case Study
4.1. Case Study Parameters
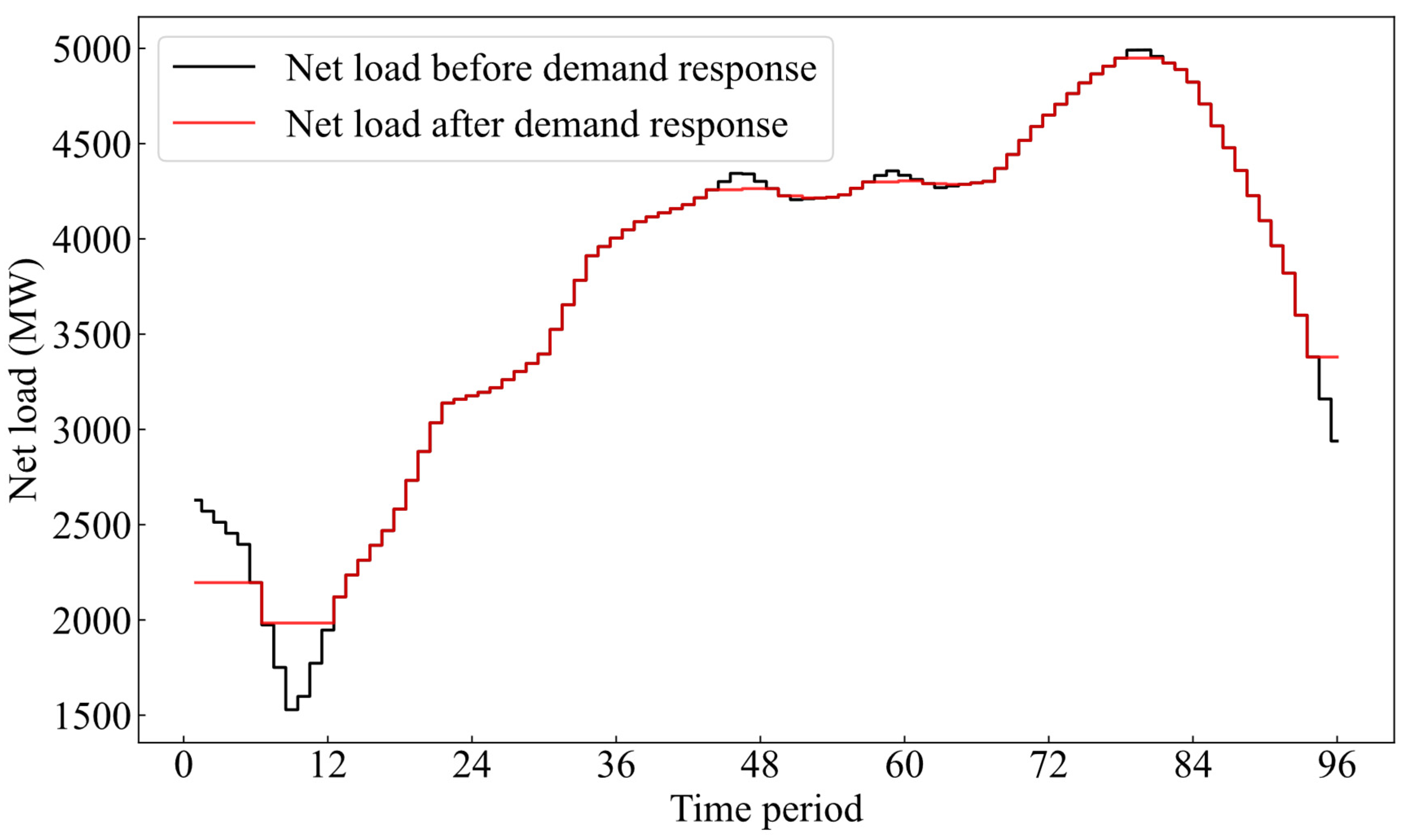
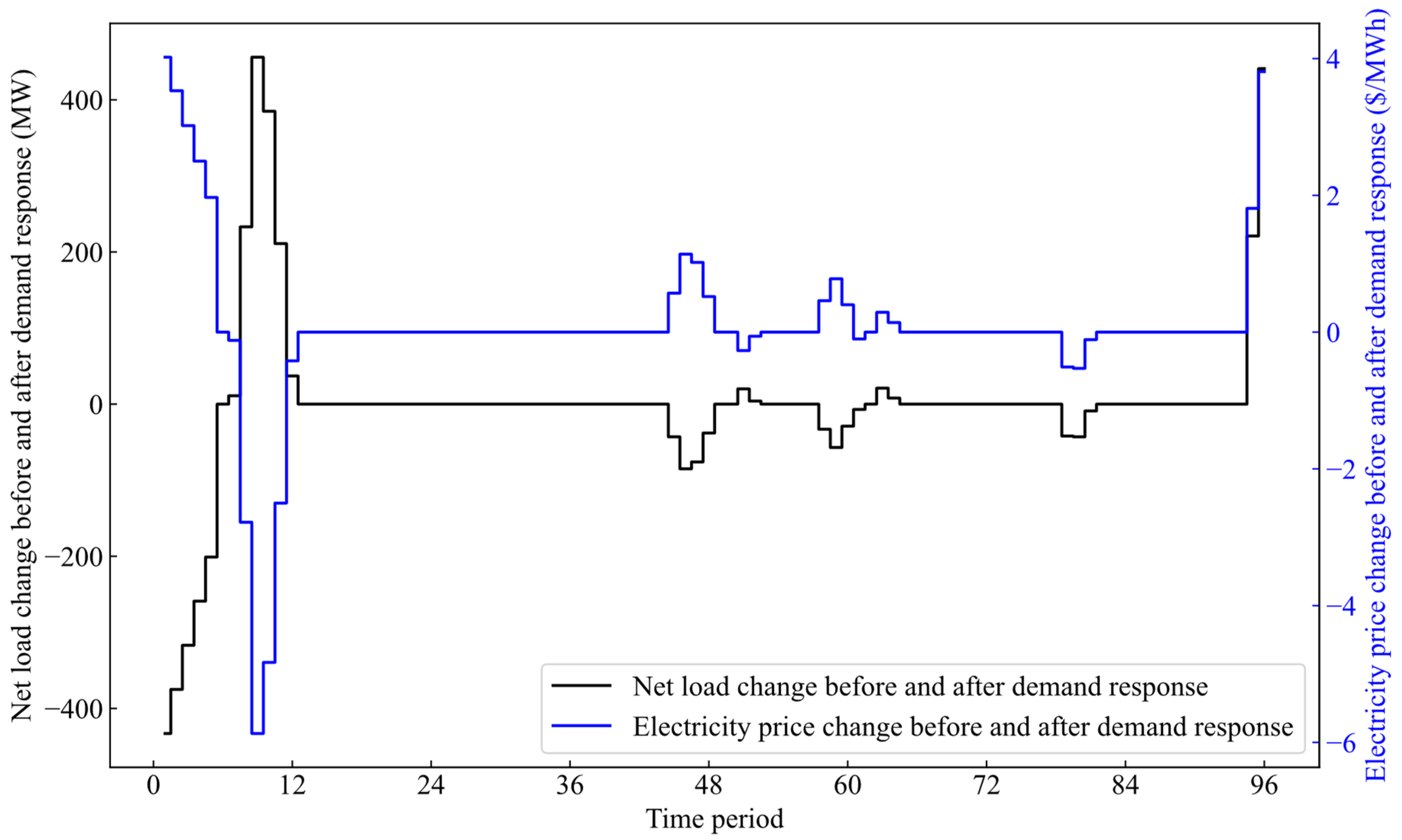
4.2. Optimization Results of Price-Driven Demand Response
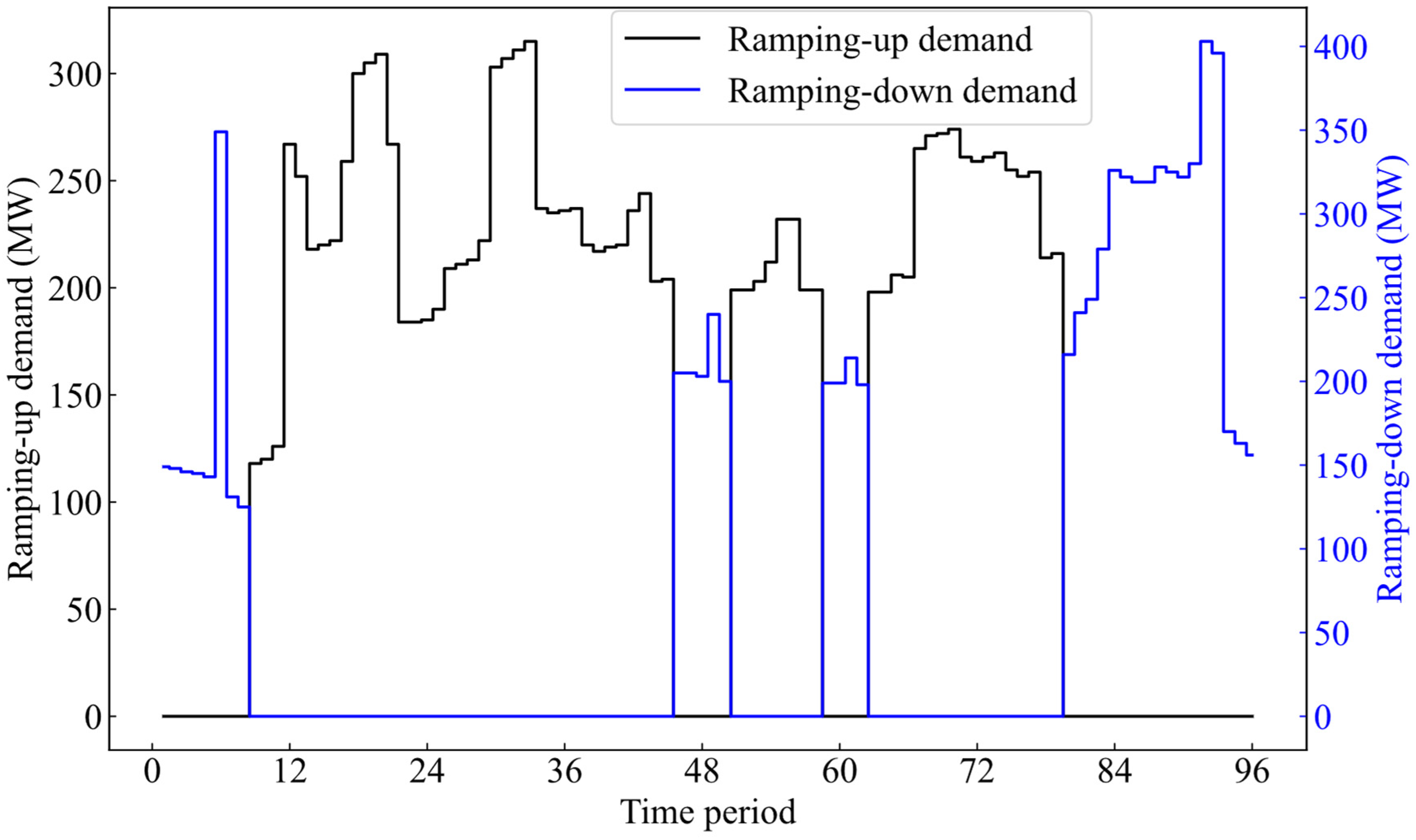
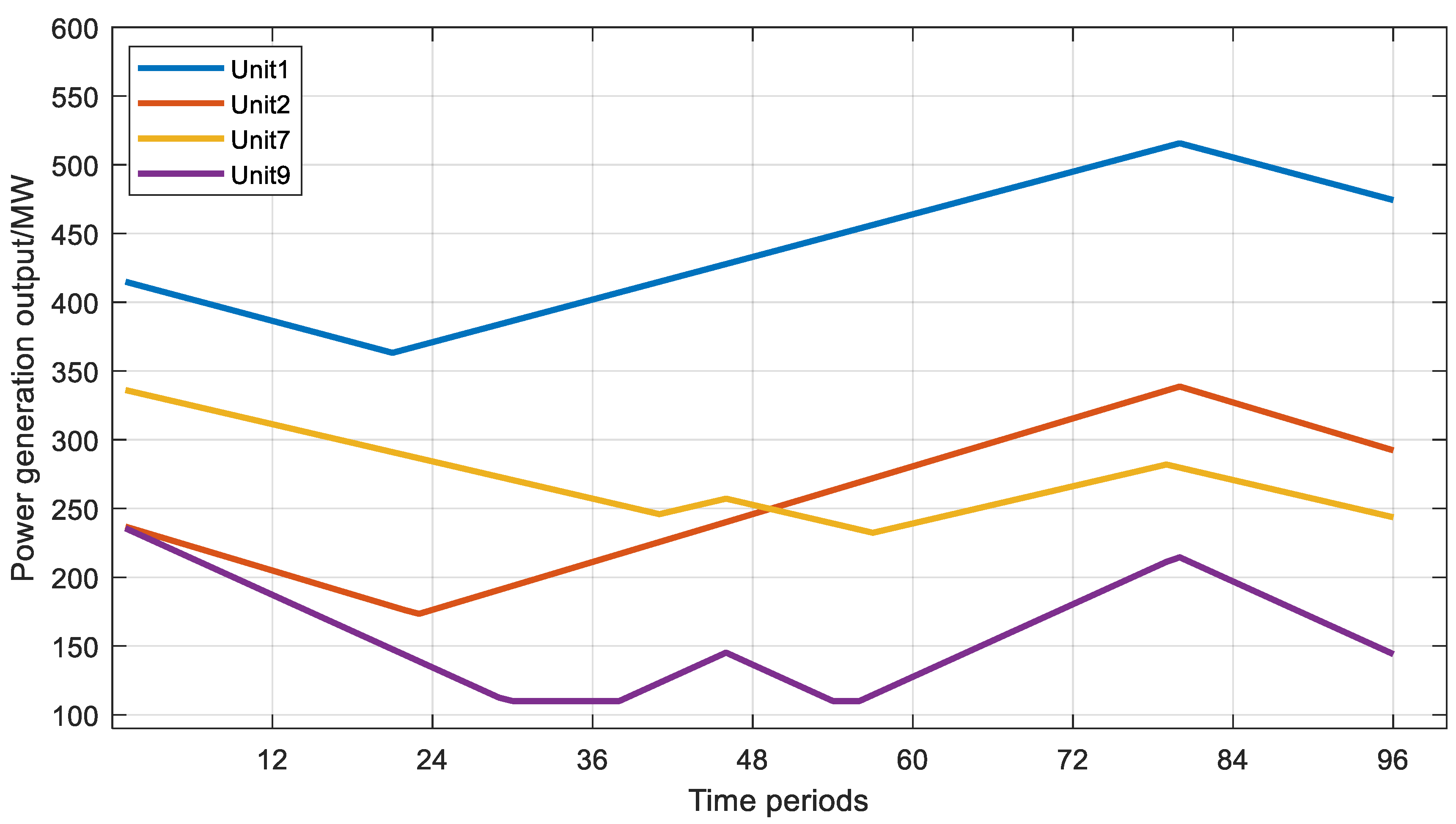
4.3. Ramping Demand and Peak Regulation Optimization Results
4.4. Sensitivity Analysis
4.4.1. Impact of Renewable Energy Generation Forecasts on Scheduling Results
4.4.2. Impact of Carbon Prices on Associated Costs
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Khatami, R.; Parvania, M.; Narayan, A. Flexibility Reserve in Power Systems: Definition and Stochastic Multi-Fidelity Optimization. IEEE Trans. Smart Grid 2020, 11, 644–654. [Google Scholar] [CrossRef]
- Li, J.; Pan, Y.; Mu, G.; Chen, G.; Zhu, X.; Yan, G.; Li, C.; Jia, C. A Hierarchical Demand Assessment Methodology of Peaking Resources in Multi-Areas Interconnected Systems with a High Percentage of Renewables. Appl. Energy 2024, 367, 123371. [Google Scholar]
- Yang, J.; Dong, Z.; Wen, F.; Chen, Q.; Liang, B. Spot Electricity Market Design for a Power System Characterized by High Penetration of Renewable Energy Generation. Energy Convers. Econom. 2021, 2, 67–78. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhao, H.; Li, B. Distributionally Robust Comprehensive Declaration Strategy of Virtual Power Plant Participating in the Power Market Considering Flexible Ramping Product and Uncertainties. Appl. Energy 2023, 343, 121133. [Google Scholar]
- Chen, H.; Huang, J.; Lin, Z.; Huang, F.; Li, M. Stochastic Economic Dispatch Based Optimal Market Clearing Strategy Considering Flexible Ramping Products under Wind Power Uncertainties. CSEE J. Power Energy Syst. 2024, 10, 1525–1535. [Google Scholar]
- Fang, X.; Sedzro, K.S.; Yuan, H.; Ye, H.; Hodge, B.-M. Deliverable Flexible Ramping Products Considering Spatiotemporal Correlation of Wind Generation and Demand Uncertainties. IEEE Trans. Power Syst. 2020, 35, 2561–2574. [Google Scholar] [CrossRef]
- Kwon, H.; Park, J.-K.; Kim, D.; Yi, J.; Park, H. A Flexible Ramping Capacity Model for Generation Scheduling with High Levels of Wind Energy Penetration. Energies 2016, 9, 1040. [Google Scholar] [CrossRef]
- Yang, L.; Zhou, N.; Zhou, G.; Chi, Y.; Chen, N.; Wang, L.; Wang, Q.; Chang, D. Day-Ahead Optimal Dispatch Model for Coupled System Considering Ladder-Type Ramping Rate and Flexible Spinning Reserve of Thermal Power Units. J. Mod. Power Syst. Clean Energy 2022, 10, 1482–1493. [Google Scholar] [CrossRef]
- Wei, W.; Ye, Z.; Wang, Y.; Dai, S.; Chen, L.; Liu, X. An Economic Optimization Method for Demand-Side Energy-Storage Accident Backup Assisted Deep Peaking of Thermal Power Units. Chin. J. Electr. Eng. 2022, 8, 62–74. [Google Scholar] [CrossRef]
- Yang, T.; Lou, S.; Zhang, M.; Li, Y.; Feng, W.; Liu, J. Research on a Two-Layer Optimal Dispatching Method Considering the Mutual Aid of Peak Regulating Resources among Regional Power Grids. Energies 2024, 17, 667. [Google Scholar] [CrossRef]
- Wu, W.; Zhu, J.; Chen, Y.; Luo, T.; Shi, P.; Guo, W.; Shi, P.; Jiang, C. Modified Shapley Value-Based Profit Allocation Method for Wind Power Accommodation and Deep Peak Regulation of Thermal Power. IEEE Trans. Ind. Applicat. 2023, 59, 276–288. [Google Scholar] [CrossRef]
- Yue, X.; Liao, S.; Xu, J.; Ke, D.; Wang, H.; Yang, J.; He, X. Collaborative Optimization of Renewable Energy Power Systems Integrating Electrolytic Aluminum Load Regulation and Thermal Power Deep Peak Shaving. Appl. Energy 2024, 373, 123869. [Google Scholar] [CrossRef]
- Yang, B.; Cao, X.; Cai, Z.; Yang, T.; Chen, D.; Gao, X.; Zhang, J. Unit Commitment Comprehensive Optimal Model Considering the Cost of Wind Power Curtailment and Deep Peak Regulation of Thermal Unit. IEEE Access 2020, 8, 71318–71325. [Google Scholar] [CrossRef]


| Units | Maximum Output/MW | Minimum Output/MW | Fuel Cost | Carbon Emission Intensity/(t/(MW·h)) | ||
|---|---|---|---|---|---|---|
| a/($/MW2) | b/($/MW) | c/$ | ||||
| 1 | 646 | 60 | 0.00198 | 16.7 | 900 | 0.9 |
| 2 | 725 | 70 | 0.00198 | 16.7 | 900 | 0.9 |
| 3 | 652 | 60 | 0.00712 | 22.3 | 370 | 0.98 |
| 4 | 508 | 50 | 0.0022 | 25.5 | 600 | 1.05 |
| 5 | 687 | 60 | 0.00198 | 16.7 | 900 | 1.05 |
| 6 | 580 | 50 | 0.00712 | 22.3 | 370 | 0.95 |
| 7 | 564 | 50 | 0.00712 | 22.3 | 370 | 0.98 |
| 8 | 865 | 80 | 0.00198 | 16.7 | 900 | 1.05 |
| 9 | 1100 | 100 | 0.00048 | 16.2 | 1000 | 1.05 |
| Parameters | Value |
|---|---|
| λ/(MW·h/t) | 0.269 |
| φ/(kg/t) | 1.5 |
| Ks/($/kg) | 8.2 |
| /($/t) | 100 |
| /(g/mol) | 44 |
| /(g/mol) | 61.07 |
| η | 0.9 |
| γ | 1.05 |
| θ/(mol) | 0.24 |
| ρv/% | 30 |
| δv/(g/mol) | 1.01 |
| Cost | Results in Scenario 1 | Results in Scenario 2 | Results in Scenario 3 | Results in Scenario 4 |
|---|---|---|---|---|
| Operation cost of thermal power units/$ | 770,515.1 | 754,421.8 | 769,391.3 | 752,702.7 |
| Carbon trading cost/$ | 160,608 | 99,801 | 156,379 | 96,535.9 |
| Total cost/$ | 939,791.4 | 862,888.7 | 934,416.9 | 857,963.4 |
| Variations in Renewable Energy Generation Output Forecasts/% | Ramping-Up Capability/MW | Ramping-Down Capability/MW | Total Cost of the Power System/$ |
|---|---|---|---|
| −20 | 11,500.3 | 7952.2 | 871,928.2 |
| −10 | 11,784.3 | 8015.5 | 864,755.4 |
| 0 | 12,053 | 8076.2 | 857,963.4 |
| 10 | 12,349.8 | 8132.9 | 851,623.6 |
| 20 | 12,677.4 | 8188.3 | 845,717.3 |
| Carbon Trading Price/($/t) | Operation cost of Thermal Power Units/$ | Carbon Trading Cost/$ | Total Cost of the Power System/$ |
|---|---|---|---|
| 50 | 752,619.8 | 48,058.7 | 809,343.5 |
| 75 | 752,691.4 | 72,042.8 | 833,383.1 |
| 100 | 752,702.7 | 96,535.9 | 857,963.4 |
| 125 | 752,704.3 | 120,059.0 | 881,417.8 |
| 150 | 752,721.7 | 144,034.0 | 905,428.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fang, L.; Peng, W.; Li, Y.; Yang, Z.; Sun, Y.; Liu, H.; Xu, L.; Sun, L.; Fang, W. A Bi-Level Peak Regulation Optimization Model for Power Systems Considering Ramping Capability and Demand Response. Energies 2024, 17, 4892. https://doi.org/10.3390/en17194892
Fang L, Peng W, Li Y, Yang Z, Sun Y, Liu H, Xu L, Sun L, Fang W. A Bi-Level Peak Regulation Optimization Model for Power Systems Considering Ramping Capability and Demand Response. Energies. 2024; 17(19):4892. https://doi.org/10.3390/en17194892
Chicago/Turabian StyleFang, Linbo, Wei Peng, Youliang Li, Zi Yang, Yi Sun, Hang Liu, Lei Xu, Lei Sun, and Weikang Fang. 2024. "A Bi-Level Peak Regulation Optimization Model for Power Systems Considering Ramping Capability and Demand Response" Energies 17, no. 19: 4892. https://doi.org/10.3390/en17194892
APA StyleFang, L., Peng, W., Li, Y., Yang, Z., Sun, Y., Liu, H., Xu, L., Sun, L., & Fang, W. (2024). A Bi-Level Peak Regulation Optimization Model for Power Systems Considering Ramping Capability and Demand Response. Energies, 17(19), 4892. https://doi.org/10.3390/en17194892






