Impact of Adding Fast Switching Fault Current Limiter (FSFCL) to the Neutral Point of 220 kV Transformer

Abstract

In recent years, as the power grid continues to expand, the issue of asymmetrical short-circuit currents exceeding limits on the 220 kV medium-voltage side has become increasingly severe, and traditional current-limiting methods have certain limitations. Therefore, this paper explores the potential benefits and feasibility of installing a Fast Switching Fault Current Limiter (FSFCL) at the neutral point of a 220 kV transformer to effectively limit asymmetrical short-circuit currents on the medium-voltage side. The paper first analyzes the current-limiting performance of the FSFCL under different installation configurations, transformer operating conditions, and fault conditions through theoretical calculations. Subsequently, through simulation studies, the impact of different limiting reactance values on the overvoltage effect at the neutral point is discussed. The results show that the installation of the FSFCL has a significant effect on suppressing the asymmetrical short-circuit current on the medium-voltage side of the transformer, but this measure has also led to an increase in the voltage at the grounded neutral point. Finally, taking the No. 2 main transformer of a certain 220 kV substation as an example, to achieve the expected current-limiting effect, the limiting reactance value of the FSFCL needs to be at least 4 ohms. At this reactance value, the overvoltage level at the neutral point remains well below the withstand limit of its insulating material. Additionally, given the existing overvoltage protection devices at the neutral point, no further overvoltage protection measures are required.

1. Introduction

In China, the ongoing expansion of the power grid and the increase in installed capacity have led to a rise in short-circuit current levels across various voltage levels, posing an increasingly serious challenge to effectively limiting system short-circuit currents [1]. Particularly for the main transformers at 220 kV substations, when an asymmetrical short-circuit fault occurs on the medium-voltage side, the unbalanced current caused by unbalanced loads, coupled with the relatively low short-circuit impedance of the windings, can lead to a significant short-circuit current impact on the medium-voltage side neutral grounding line [2,3]. This situation can easily cause overheating, deformation, or even damage to transformers that are already in operation and initially designed with insufficient short-circuit current resistance [4].

However, due to the unique location and high voltage level of the neutral point on the medium-voltage side of three-winding transformers, there is still a lack of effective methods to limit short-circuit currents. Traditional current-limiting measures primarily include adjusting the grid structure, changing operational modes, and adding current-limiting devices, but each of these approaches has its limitations [5]. For instance, adjusting the grid structure or changing operating modes may extend the construction period and increase costs, potentially affecting the stability and reliability of the power grid. Installing series current-limiting reactors on lines to limit current will increase reactive power loss and affect the system’s power flow distribution [6]. Installing current-limiting reactors at the transformer neutral point, although reducing losses and costs compared to line installation, still incurs some losses due to the frequent flow of unbalanced current at the neutral point [7], and this method may also affect the sensitivity of related relay protection, necessitating a re-examination and adjustment of related equipment parameters.

Fault current limiters (FCLs), due to their unique working characteristics, provide an effective current-limiting solution with minimal loss and minimal impact on the original power grid [8,9,10]. Among the types of FCLs, the fast switching fault current limiter (FSFCL) is particularly suitable for large-scale promotion and application within the power grid due to its economic and reliable nature [11].

Currently, global research on FCLs mainly focuses on the design optimization of the equipment itself and its application on lines. For example, Xia Shengguo from Huazhong University of Science and Technology conducted research on the current-limiting characteristics of FCLs using high-coupling split reactors on transmission lines based on electromagnetic transient simulation [12]. Reference [13] designed an SCTC energy-dissipating FCL and verified its current-limiting effect in high-voltage AC transmission systems and the adjustment effect on line RC parameters through finite element simulation. Reference [14] proposed a new FCL topology suitable for DC transmission systems, effectively controlling the peak and rate of rise in short-circuit currents by reducing equivalent inductance, quickly putting in energy-dissipating resistors, and cooperating with DC circuit breakers, achieving rapid clearance of short-circuit fault currents on the DC side. Although the existing literature demonstrates the application of FCLs on transmission lines, there is still a lack of exploration regarding the application methods and feasibility of FCLs at transformer neutral points.

Reference [15] indicates that installing current-limiting devices at the transformer’s neutral point is more effective for limiting asymmetric short-circuit currents than installing them on transmission lines. Additionally, since only one device is needed at the neutral point compared to three devices on the lines, the cost of current limiting at the neutral point is lower. Furthermore, because the current flowing through the neutral grounding wire is minimal under normal conditions but increases significantly during a fault [16], fault identification and short-circuit point prediction based on neutral point current are simpler. Korean scholar I.G. Im, through simulation research, analyzed the current-limiting characteristics of superconducting FCLs installed at the transformer neutral point under different fault conditions, affirming the development prospects of neutral point FCLs [17]. However, the above studies only focused on the current-limiting characteristics of FCLs installed at the neutral point and did not explore their impact on the system. Ali Sahebi proposed that, compared with the method of directly increasing the current-limiting reactor at the neutral point, installing FCLs has a smaller impact on the sensitivity of transformer differential protection [18], but his research only considered the case of installing FCLs at the high-voltage side neutral point of double-winding transformers and did not involve the potential impact of installing FCLs on other relay protections. In summary, there is still a lack of in-depth analysis of the impact of installing FCLs at the medium-voltage side neutral point of transformers on the transformer system in existing research.

To explore the potential benefits and feasibility of installing FSFCL at the neutral point of the medium-voltage side of transformers to limit asymmetrical short-circuit currents, this paper uses a combination of theoretical calculations and simulation analysis to assess the current-limiting effect of FSFCL and its impact on system performance. The study first analyzed and calculated the current-limiting performance of the FSFCL at different installation positions under the same current-limiting reactance value. Then, it examined the current-limiting characteristics of the FSFCL under different fault types. Finally, an electromagnetic transient simulation model was constructed to analyze the impact of the FSFCL on the insulation state of the neutral point.

2. Materials and Methods

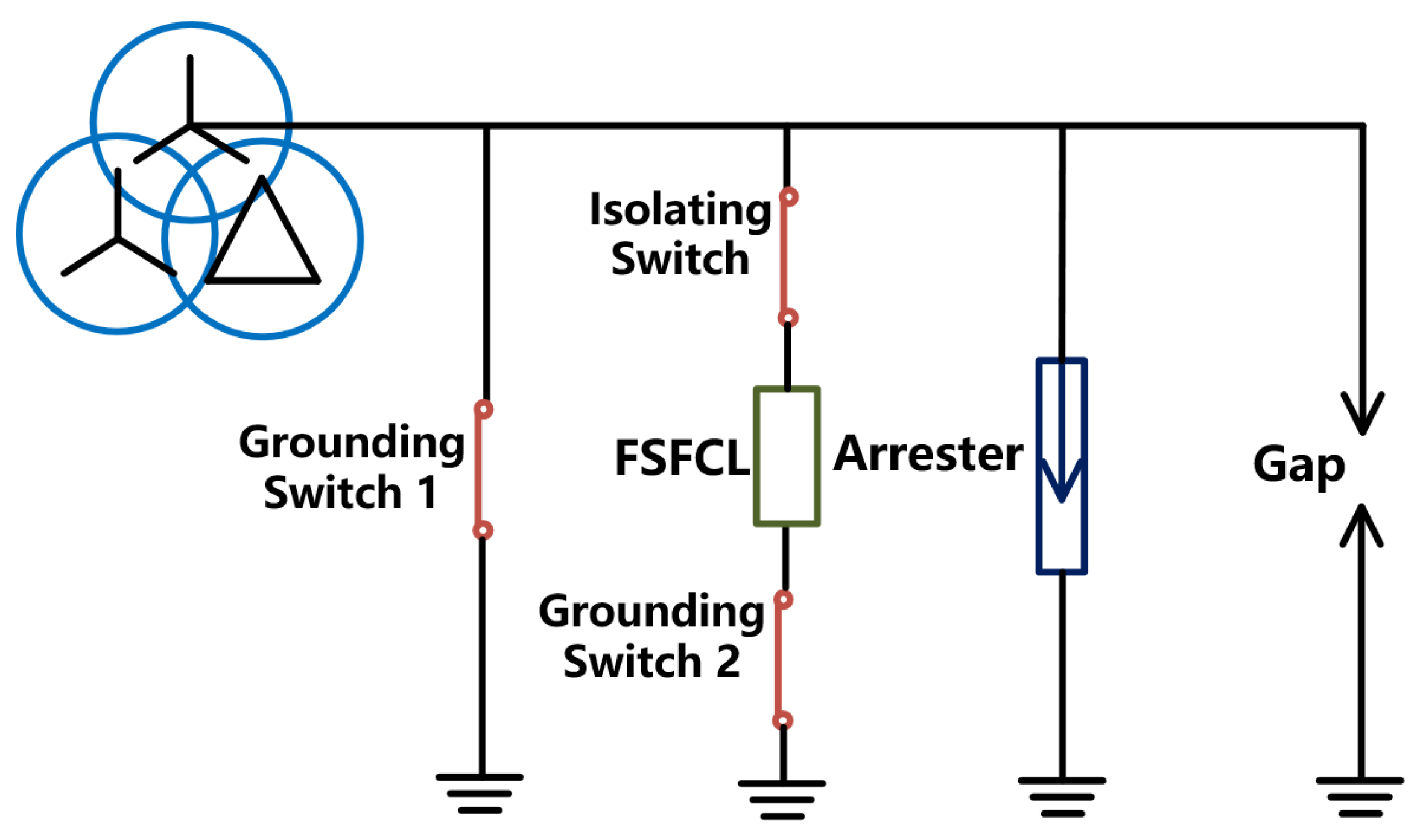
2.1. Working Principle of FSFCL

As shown in Figure 1, the FSFCL mainly consists of a current-limiting reactor, a fast switch, a controller, and current transformers [19]. Under normal operating conditions, the fast switch remains closed with extremely low device loss, having no negative impact on the power grid operation. When the current transformer detects a short-circuit current, the fast switch quickly opens and the current-limiting reactor is engaged, effectively controlling the fault current within the safe bearing capacity of the transformer. After the fault is cleared, the fast switch closes again to ensure the stable operation of the power grid.

Based on the characteristics of the FSFCL, as shown in Figure 2, the control process can be summarized as follows: At system startup, the fast switch is closed, and the current-limiting reactor is in a short-circuited state. The controller collects current signals using current transformers to detect faults and predict the current zero-crossing point. Upon detecting a short circuit, the controller triggers the fast switch to open at the current zero-crossing, engaging the current-limiting reactor. After approximately 2 s of current limitation, the controller reassesses the current status. If the current returns to normal, indicating a transient fault, the fast switch will close, the current-limiting reactor will be short-circuited again, and the circuit breaker will attempt to reclose. If the current remains abnormal, the controller determines it to be a permanent fault, and the circuit breaker will remain open.

Installing an FSFCL at the neutral point also requires consideration of its integration with existing neutral point equipment. To ensure the proper operation and maintenance of the FSFCL, this paper proposes a neutral point installation scheme for the FSFCL: as shown in Figure 3, one end of the FSFCL is connected to the grounding switch, and the other end is connected to the transformer neutral point via a series isolation switch, while the other end of the grounding switch is grounded. The FSFCL branch is connected in parallel with the existing neutral point equipment, such as the grounding switch, surge arrester, and spark gap. This configuration allows for flexible adjustment of the transformer’s neutral point grounding state, enabling switching among non-direct grounding, direct grounding, or grounding through the limiter, which facilitates changes in the transformer’s operating mode and neutral point maintenance. Additionally, if the FSFCL experiences a fault, it can be quickly isolated and repaired, ensuring continuous system operation and reliability.

2.2. Short-Circuit Current Calculation Method

To study the current-limiting characteristics of the FSFCL, it is first necessary to calculate the maximum asymmetrical short-circuit current that may occur on the neutral point and the windings on each side of the transformer. To achieve this, this paper employs the symmetrical component method for short-circuit current calculation. This approach decomposes the asymmetrical state of the power system into three independent symmetrical systems: positive-sequence, negative-sequence, and zero-sequence systems. First, each independent system is analyzed; secondly, a composite-sequence network is constructed according to the type of fault, and the sequence components are calculated, with the results of each combined to obtain the current and voltage values at the fault point; finally, the currents in the transformer windings and at the neutral point are obtained based on the impedance distribution. Taking the short-circuit current at the fault point during a single-phase ground fault as an example, the mathematical expression is as follows:

$$\left(\begin{array}{c}\stackrel{•}{I_A}\\ \stackrel{•}{I_B}\\ \stackrel{•}{I_C}\end{array}\right)=\left(\begin{array}{ccc}1& 1& 1\\ a^2& a& 1\\ a& a^2& 1\end{array}\right)\left(\begin{array}{c}\stackrel{•}{I_{f(1)}}\\ \stackrel{•}{I_{f(2)}}\\ \stackrel{•}{I_{f(0)}}\end{array}\right)$$

(1)

In the formula, $\stackrel{•}{I_{\mathrm{A}}}$, $\stackrel{•}{I_B}$, and $\stackrel{•}{I_C}$ represent the three-phase currents at the fault point; $\stackrel{•}{I_{f(1)}}$, $\stackrel{•}{I_{f(2)}}$, and $\stackrel{•}{I_{f(3)}}$ represent the positive, negative, and zero-sequence current components at the fault point; and a is the three-phase phase shift operator, a = e^j120.

Taking the No. 2 main transformer in a 220 kV substation as an example, this paper analyzes the asymmetric short circuit on the medium-voltage side of the transformer. The high-voltage side of the transformer supplies power separately, and the high-voltage side and medium-voltage side operate side by side with another transformer with basically the same parameters, while the low-voltage side operates independently.

The basic parameters of the transformer are as follows: the rated voltage is 220/121/10.5 kV; the rated capacity is 120/120/60 MVA; the short-circuit impedance is 14.52%, 23.98%, and 7.40%; the connection group is YNyn0D11; and the transformer core is a three-phase five-column type.

To accurately assess the severe short-circuit faults that the medium-voltage side may encounter under the most unfavorable conditions, this paper assumes that the transformer and its various side systems are all operating at maximum capacity, and the fault location is set at the near end of the medium-voltage side of the transformer. The simplified system topology is shown in Figure 4.

The equivalent impedance standard values of the transformer and its sides are shown in

Table 1. Impedance standard values of transformers and systems on each side.

Impedance Name	Transformer (p.u.)		System (p.u.)
	Positive Sequence	Zero Sequence	Maximum Operating Mode		Minimum Operating Mode
			Positive Sequence	Zero Sequence	Positive Sequence	Zero Sequence
High Voltage	0.1555	0.1423	0.0108	0.0252	0.0480	0.0900
Medium Voltage	−0.0103	−0.0093	0.0600	0.0360	0.2160	0.0960
Low Voltage	0.0843	0.0768	/	/	/	/

.

When a single-phase ground fault occurs at the near end of the medium-voltage side, the sequence network diagrams for each sequence are shown in Figure 5.

In the diagram above, X₁₁, X₁₂, and X₁₀ represent the positive-sequence, negative-sequence, and zero-sequence impedances of the high-voltage side winding, respectively. Similarly, X₂₁, X₂₂, and X₂₀, and X₃₁, X₃₂, and X₃₀ correspond to the positive-sequence, negative-sequence, and zero-sequence impedances of the medium-voltage and low-voltage side windings, respectively; X_g1, X_g2, and X_g0 represent the positive-sequence, negative-sequence, and zero-sequence impedances of the high-voltage side system.

Based on the sequence network diagrams, the total impedance for each sequence is calculated as follows:

$$\left\{\begin{array}{c}{X}_{\Sigma 1}=\left(X_{11}+X_{21}\right)/3+X_{g1}=0.0834\\ X_{\Sigma 2}=\left(X_{12}+X_{22}\right)/3+X_{g2}=0.0834\\ X_{\Sigma 0}=X_{20}+\left(X_{10}+X_{g0}\right)//X_{30}=0.0434\end{array}\right.$$

(2)

The sequence short-circuit currents at the fault point are as follows:

$$I_{f(1)}=I_{f(2)}=I_{f(0)}={\displaystyle \frac{1}{X_{\Sigma 1}+X_{\Sigma 2}+X_{\Sigma 0}}}=4.7584$$

(3)

Upon analyzing the neutral point on the medium-voltage side of the transformer, the current flowing through the neutral point is determined to be:

$$\stackrel{•}{I_0}=\stackrel{•}{I_A}+\stackrel{•}{I_B}+\stackrel{•}{I_C}=\stackrel{•}{I_A}=3\stackrel{•}{I_{f(0)}}=14.2751$$

(4)

By analyzing the equivalent circuits for each sequence, the fault phase currents on the high-, medium-, and low-voltage windings of the transformer are found to be as follows:

$$\left\{\begin{array}{l}{\stackrel{•}{I}}_{\mathrm{gA}}={\stackrel{•}{I}}_{f\left(1\right)}+{\stackrel{•}{I}}_{f\left(2\right)}+{\stackrel{•}{I}}_{f\left(0\right)}{\displaystyle \frac{X_{30}}{X_{10}+X_{30}+X_{g0}}}=6.2542\\ {\stackrel{•}{I}}_{\mathrm{zA}}={\stackrel{•}{I}}_{f\left(1\right)}+{\stackrel{•}{I}}_{f\left(2\right)}+{\stackrel{•}{I}}_{f\left(0\right)}=9.5167\\ {\stackrel{•}{I}}_{\mathrm{dA}}={\stackrel{•}{I}}_{f\left(0\right)}{\displaystyle \frac{X_{10}+X_{g0}}{X_{10}+X_{30}+X_{g0}}}=3.2625\end{array}\right.$$

(5)

The rated values of the fault phase currents for the high-, medium-, and low-voltage windings are as follows: I_gA = 1969 A, I_zA = 5449 A, and I_dA = 12,428 A; the rated value of the fault current passing through the neutral point is I₀ = 8174 A.

3. Results and Discussion

3.1. Analysis of the Neutral Point Fault Current Limiter Connection Method

Typically, 220 kV transformers use the YNyn0D11 connection method, which allows for the addition of limiting reactors at both the high-voltage and medium-voltage neutral points. To clarify which is more advantageous, adding a limiting reactor at the medium- or high-voltage neutral point, an analysis and comparison of these two situations will be conducted.

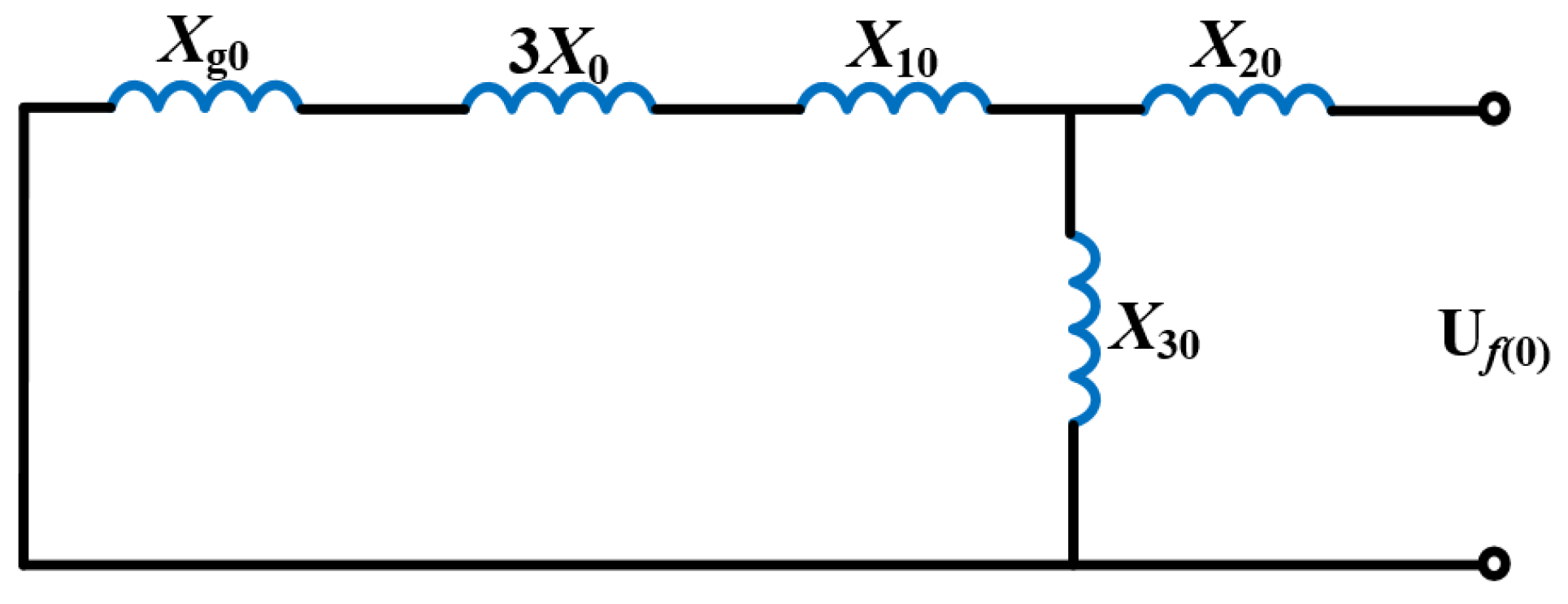
3.1.1. Analysis of the Current-Limiting Reactor Installation at the High-Voltage Neutral Point

The zero-sequence network diagram after installing a current-limiting reactor at the high-voltage side neutral point of the main transformer is shown in Figure 6.

The total zero-sequence impedance at this point is as follows:

$$X_{\Sigma 0}=X_{20}+\left(X_{10}+X_{g0}+3X_0\right)//X_{30}$$

(6)

where X₀ is the value of the current-limiting reactance.

Based on the modified zero-sequence impedance and equivalent circuit, recalculate the short-circuit current according to the method in Section 2.2. Analyze the situation where a single-phase ground fault occurs near the exit of the high-voltage side of the transformer, and a 2-ohms limiting reactor is installed on the neutral point of the high-voltage side. The calculation results are shown in

Table 2. Calculation results of current-limiting reactance at neutral point on high-voltage side.

Limiting Reactor (Ω)	Fault Point Zero Sequence		High-Voltage Side Winding Current (kA)	Medium-Voltage Side Winding Current (kA)	Low-Voltage Side Winding Current (kA)
	Impedance (p.u.)	Current (kA)
0	0.0434	2.72	1.97	5.45	12.43
2	0.0496	2.65	1.79	5.29	13.50

.

From Table 2, it can be seen that, at this time, the zero-sequence impedance has increased by 14.29%, the zero-sequence current at the fault point has only decreased by 2.57%, the current in the medium-voltage side winding has decreased by 2.94%, and the current in the high-voltage side winding has decreased by 9.14%, while the current in the low-voltage side winding has increased by 8.61%. The results indicate that installing a limiting reactor on the neutral point of the high-voltage side is not sufficient to significantly increase the zero-sequence impedance at the fault point and its limiting effect is not ideal. At the same time, due to the increased impedance on the high-voltage side, the zero-sequence fault current flowing to the low-voltage side winding actually rises during asymmetrical ground faults, which may introduce new safety hazards.

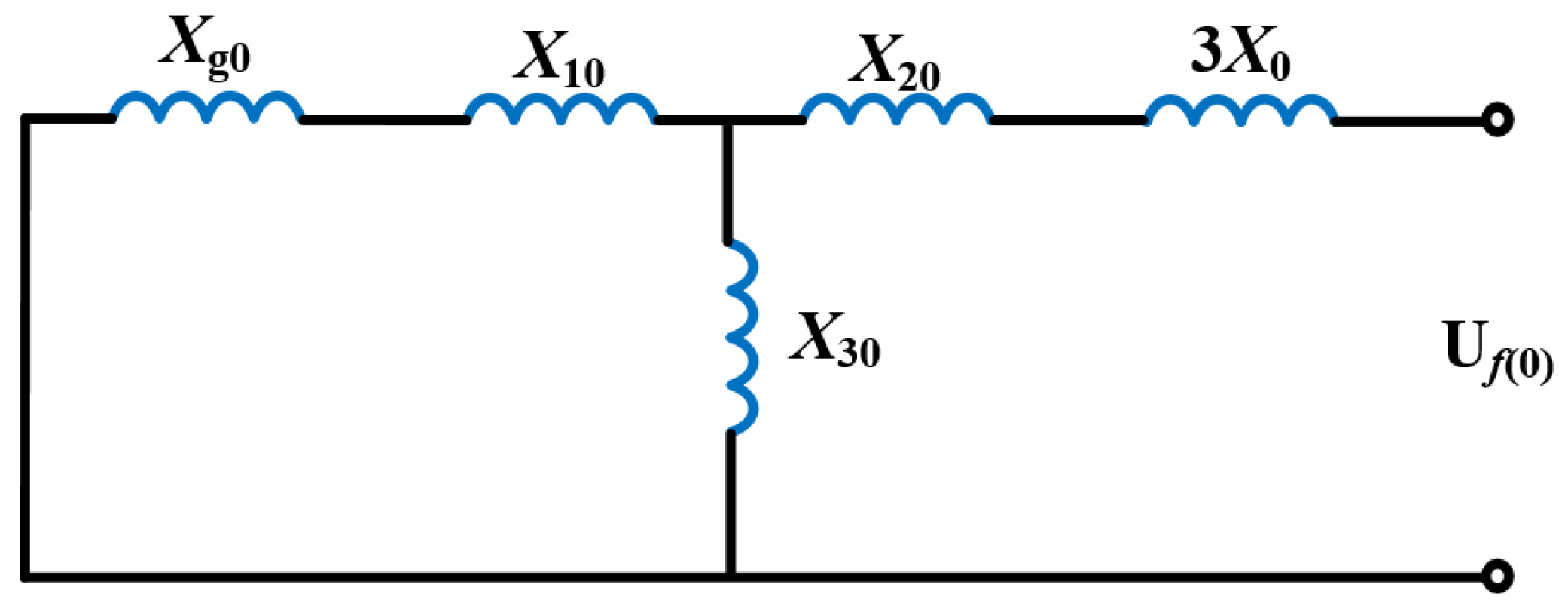
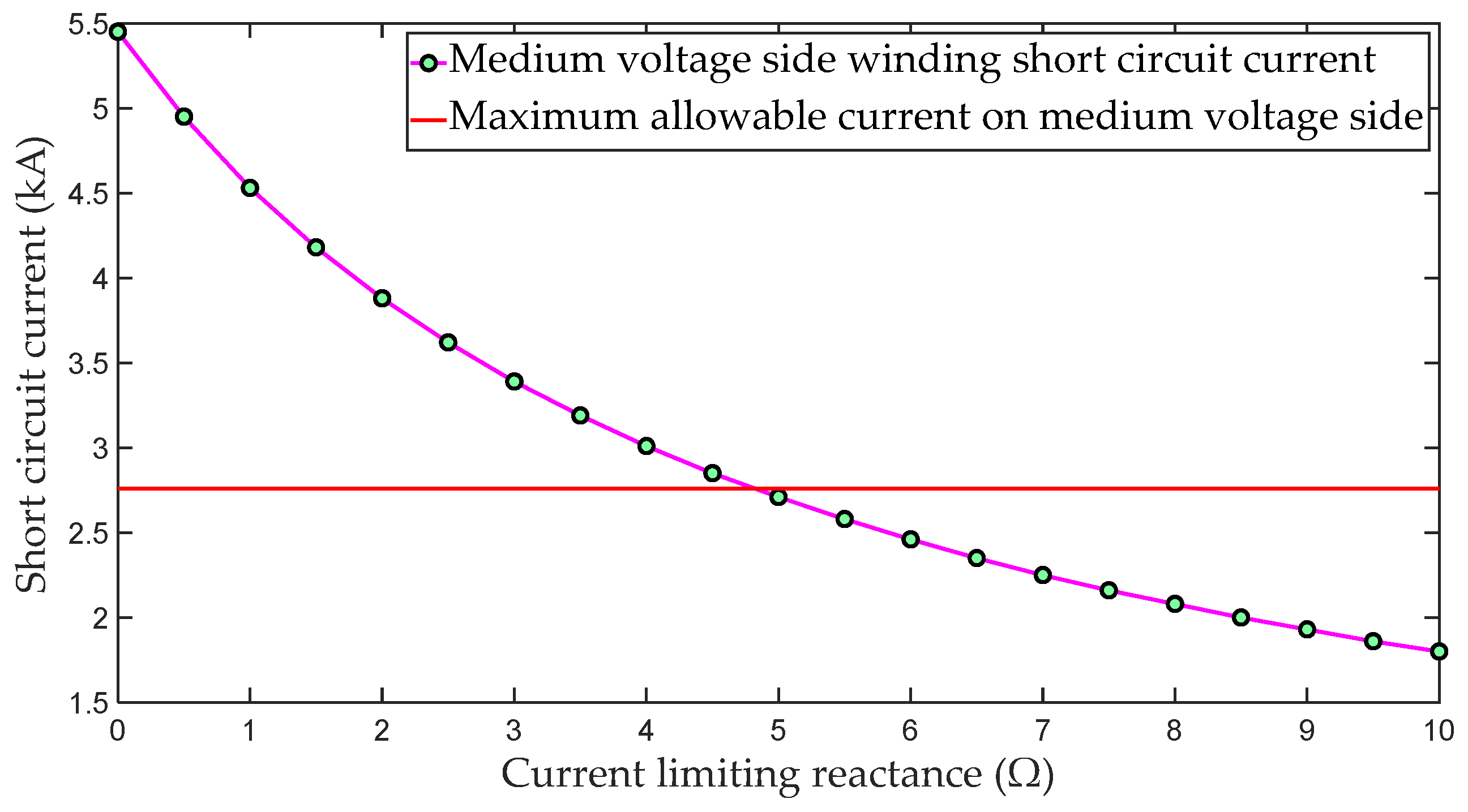
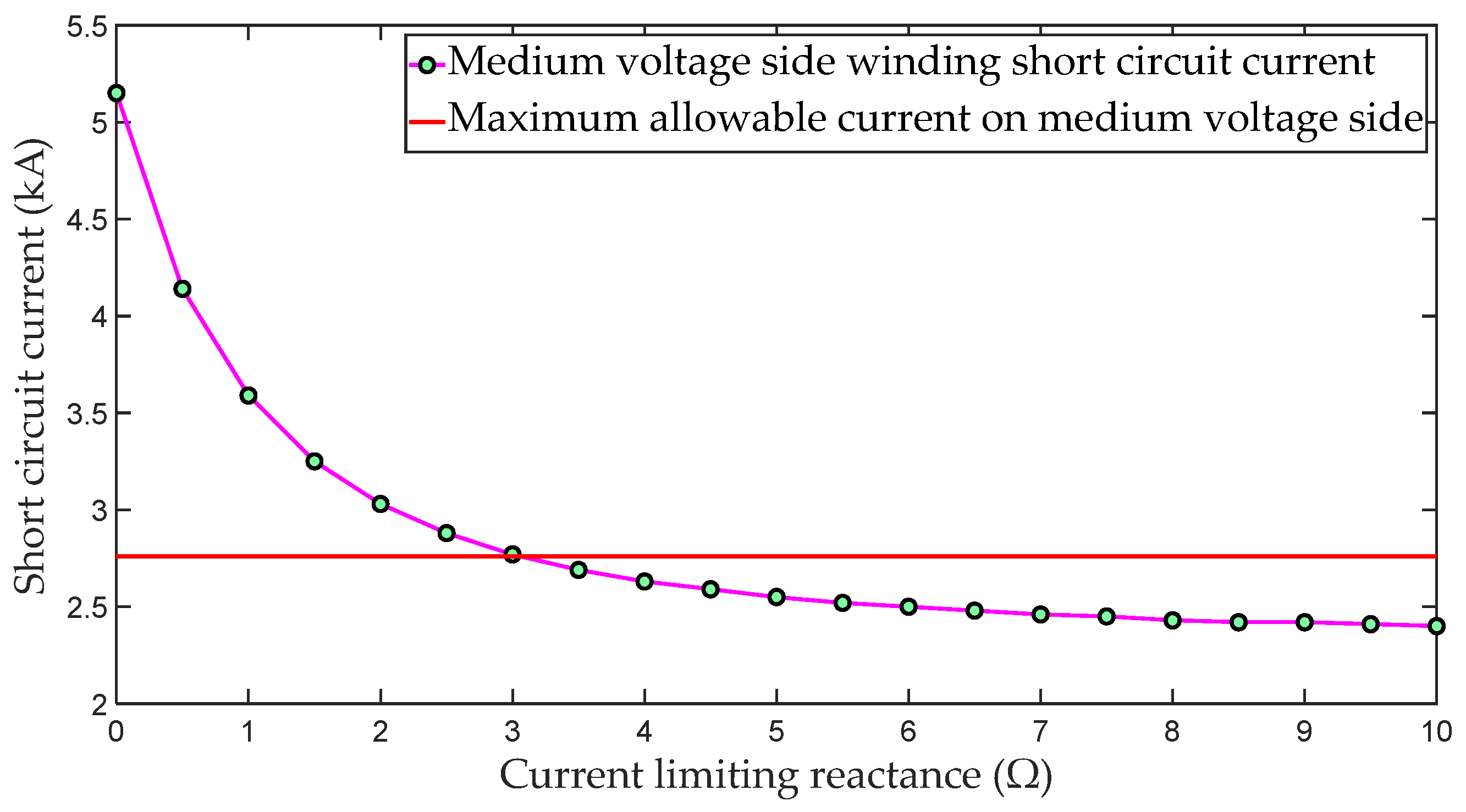
3.1.2. Adding a Limiting Reactor to the Neutral Point on the Medium-Voltage Side

The zero-sequence network diagram after adding a limiting reactor to the neutral point on the medium-voltage side of the main transformer is shown in Figure 7.

The total zero-sequence impedance at this time is as follows:

$$X_{\Sigma 0}=X_{20}+3X_0+\left(X_{10}+X_{g0}\right)//X_{30}$$

(7)

Similarly, based on the modified zero-sequence impedance and equivalent circuit, the situation of adding a 2-ohms limiting reactor on the medium-voltage side was analyzed, and the calculation results are shown in

Table 3. Calculation results of current-limiting reactance at neutral point on medium-voltage side.

Limiting Reactor (Ω)	Fault Point Zero Sequence		High-Voltage Side Winding Current (kA)	Medium-Voltage Side Winding Current (kA)	Low-Voltage Side Winding Current (kA)
	Impedance (p.u.)	Current (kA)
0	0.0434	2.72	1.97	5.45	12.43
2	0.1286	1.94	1.40	3.88	8.84

.

As can be seen from Table 3, at this time, the zero-sequence impedance increased by 196.31%, the zero-sequence current at the fault point decreased by 28.68%, the current in the high-voltage side winding of the transformer decreased by 28.93%, the current in the medium-voltage side winding decreased by 28.81%, and the current in the low-voltage side winding decreased by 28.88%. These results indicate that adding a current-limiting reactor at the medium-voltage side neutral point is more effective in increasing the zero-sequence impedance at the fault point compared to adding it on the high-voltage side, thereby effectively reducing the fault current and zero-sequence current.

In addition to straightforward data comparisons, a theoretical analysis can be performed by examining the zero-sequence total impedance formulas. When the FCL is installed at the neutral point on the high-voltage side, the current-limiting reactance X₀ is in parallel with X₃₀, causing changes in X₀ to be constrained by X₃₀, which limits the variation in zero-sequence total impedance. Conversely, when the FCL is installed at the neutral point on the medium-voltage side, the current-limiting reactance X₀ can more effectively enhance the zero-sequence total impedance.

Based on this, subsequent analyses and calculations will only consider the situation of adding a current-limiting reactor at the medium-voltage side neutral point.

3.2. Effect of Different Current-Limiting Reactance Values on Fault Current under Various Fault Conditions

To specifically analyze the current-limiting effect of FSFCL when installed at the medium-voltage side neutral point, this work conducted calculations to evaluate the impact of different current-limiting reactance values on the current-limiting effect under various short-circuit fault conditions.

3.2.1. Evaluation of the Limiting Effect during Single-Phase Ground Faults

According to Section 2.2, calculate the currents in the windings on each side of the No. 2 main transformer when a single-phase ground fault occurs near the medium-voltage side, for different limiting reactance values. The specific results are shown in

Table 4. Calculation results of single-phase ground fault of 110 kV busbar.

Limiting Reactor (Ω)	Zero-Sequence Current (kA)			High-Voltage Winding Current (kA)	Medium-Voltage Winding Current (kA)	Low-Voltage Winding Current (kA)
	High-Voltage Winding	Medium-Voltage Winding	Low-Voltage Winding
0	0.47	2.72	12.42	1.97	5.45	12.42
0.5	0.43	2.47	11.28	1.79	4.95	11.28
1	0.39	2.26	10.33	1.64	4.53	10.33
1.5	0.36	2.09	9.53	1.51	4.18	9.53
2	0.34	1.94	8.84	1.40	3.88	8.84
2.5	0.31	1.81	8.25	1.31	3.62	8.25
3	0.29	1.69	7.73	1.22	3.39	7.73
3.5	0.28	1.59	7.27	1.15	3.19	7.27
4	0.26	1.50	6.86	1.09	3.01	6.86
4.5	0.25	1.42	6.50	1.03	2.85	6.50
5	0.23	1.35	6.17	0.98	2.71	6.17
5.5	0.22	1.29	5.88	0.93	2.58	5.88
6	0.21	1.23	5.61	0.89	2.46	5.61
6.5	0.20	1.18	5.36	0.85	2.35	5.36
7	0.19	1.13	5.14	0.81	2.25	5.14
7.5	0.19	1.08	4.93	0.78	2.16	4.93
8	0.18	1.04	4.74	0.75	2.08	4.74
8.5	0.17	1.00	4.56	0.72	2.00	4.56
9	0.17	0.96	4.40	0.70	1.93	4.40
9.5	0.16	0.93	4.25	0.67	1.86	4.25
10	0.16	0.90	4.11	0.65	1.80	4.11

.

The allowable maximum short-circuit currents on each side of the No. 2 main transformer at the substation are shown in

Table 5. Maximum allowable short-circuit current of windings on each side of main transformer.

	High Voltage	Medium Voltage	Low Voltage
Maximum allowable current (kA)	2.00	2.76	13.40

. To ensure the safe operation of the transformer, after the installation of FSFCL, the maximum short-circuit current of all windings should not exceed their respective maximum allowable short-circuit currents. Table 4 shows the maximum allowable short-circuit currents for the windings on each side of the No. 2 main transformer at the substation.

From the above two tables, it can be seen that, under the maximum operating mode, the short-circuit current on the medium-voltage side of the No. 2 main transformer during a single-phase ground fault exceeded the maximum allowable short-circuit current for that winding. After installing the limiting reactor, it is possible to effectively reduce the short-circuit current on each side. The trend of the short-circuit current with the change in the limiting reactance is shown in Figure 8.

In Figure 8, as the value of the limiting reactance increases, its limiting effect tends to saturate. Furthermore, when a single-phase ground fault occurs near the medium-voltage side, to achieve the limiting objective, the neutral point limiting reactance value must not be lower than 5 ohms.

3.2.2. Evaluation of the Limiting Effect during Two-Phase Ground Faults

When analyzing the two-phase ground fault near the medium-voltage side of the No. 2 main transformer at the 220 kV substation, the composite-sequence network structure should be transformed into a parallel-sequence network of the three-sequence system.

Similarly, under the maximum operating mode, when a two-phase ground fault occurs at the near end of the medium-voltage side of the No. 2 main transformer, the short-circuit current on the medium-voltage side significantly exceeds the predetermined current-limiting target. Therefore, the current-limiting measures are primarily focused on the medium-voltage side, and the variation in the short-circuit current with the current-limiting reactance is shown in Figure 9.

From the above figure, it can be deduced that when a two-phase ground fault occurs near the medium-voltage side, to achieve the limiting target, the neutral point limiting reactance value should be no less than 3.5 ohms.

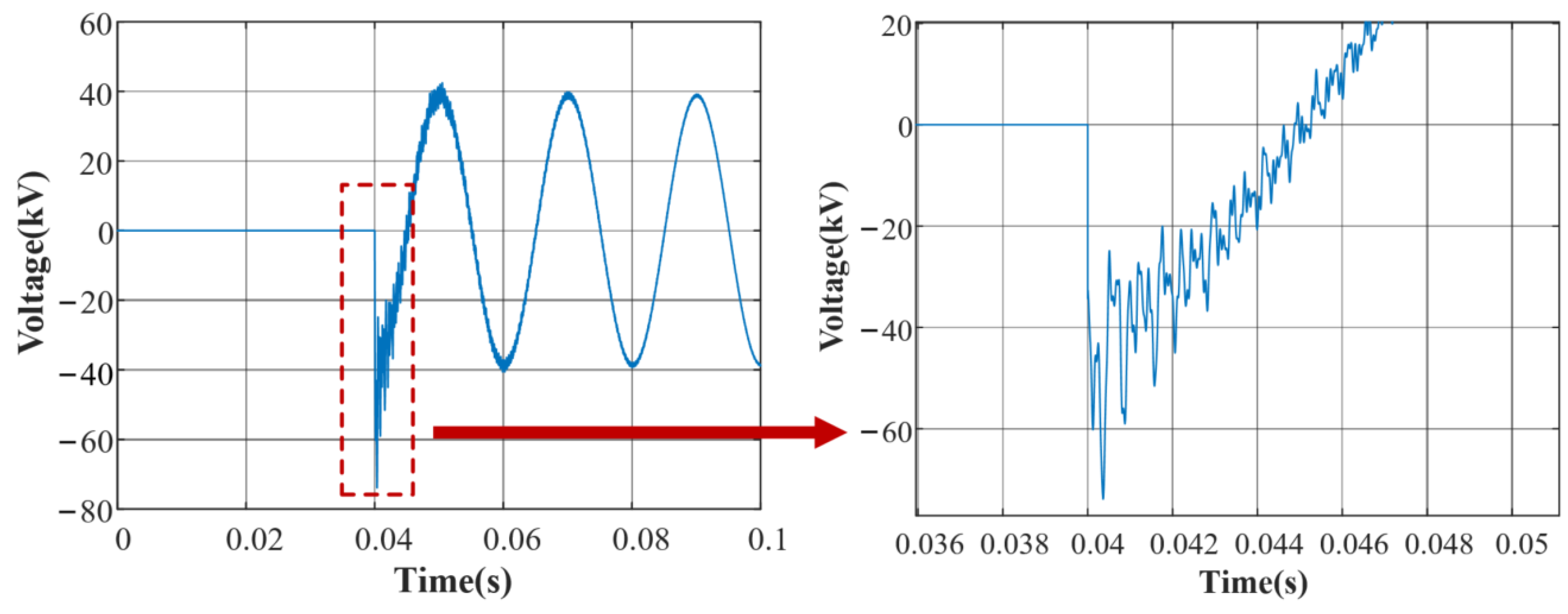
3.3. Overvoltage Simulation Analysis

The neutral point FCL (Fixed Current Limiter) only affects the zero-sequence circuit and does not affect voltage or power flow during normal system operation. However, during asymmetric short-circuit faults, if the zero-sequence current enters the ground through the neutral point limiting reactor, it will cause the neutral point voltage to rise [20].

To avoid overvoltage at the transformer neutral point caused by the connection of the limiting reactor, which could exceed the insulation level and potentially lead to serious accidents, it is essential to conduct an overvoltage check based on the system configuration and transformer parameters before installing the FCL.

3.3.1. Simulation Model Construction

In this study, the ATP-EMTP simulation software (The version number is GNU-Mingw32 ATP) was utilized to model the power frequency overvoltage and transient overvoltage conditions at the neutral point of the No. 2 main transformer after the integration of a current-limiting reactor. During the construction of the simulation model, the power grid was subject to simplified equivalent processing, adhering to the principle of maintaining constant power flow and node voltage to ensure the precision of the simulation data [21].

Based on the FSFCL installation scheme proposed in Section 2.1, we observed that the FSFCL branch was connected in parallel with each neutral point device. When the voltage across the relevant neutral point devices (such as the neutral point surge arrester or spark gap) did not reach its discharge voltage, these devices could be considered in an open circuit state and, thus, did not affect the operation of the FSFCL. The insulation level of these neutral point devices is typically the same as that of the neutral point itself. Therefore, we can initially assume that the neutral point voltage did not reach the insulation level and temporarily ignore the neutral point devices in the simulation. If subsequent simulations reveal that the neutral point overvoltage exceeds the insulation level, it will be necessary to include the neutral point devices in the model and perform the simulation again.

The simulation model, as depicted in Figure 10, consisted of the following six main components:

(1)

The 220 kV high-voltage side power grid utilized a three-phase ideal voltage source and three symmetrical parallel resistors for equivalent representation, with a phase voltage peak value of 179.6 kV.

(2)

Transformers were modeled using the BCT (Bipolar Charge Transformer) model, with a capacity of 120 MVA and rated voltages of 220/121/10.5 kV. Two transformers operated in parallel on the high- and medium-voltage sides, while the low-voltage side operated independently, and only one transformer was grounded. The excitation and short-circuit losses were set based on actual values.

(3)

The fault current limiter was simplified to a current-limiting reactor.

(4)

Asymmetrical faults were simulated at the medium-voltage side exit of the transformer with two scenarios: one involved phase A grounding through a 0.01 Ω small resistor, and the other involved phases B and C grounding through a 0.01 Ω small resistor.

(5)

The 110 kV medium-voltage side power grid comprised three transmission lines, all using the LCC (Line Commutated Converter) model, with lengths of 23.33 km, 5.73 km, and 21.53 km, respectively. The ends of these lines were equivalently loaded with three-phase symmetrical Y-connected grounding resistors.

(6)

The 10.5 kV low-voltage side power grid was equivalently represented by three-phase symmetrical Y-connected grounding resistors.

3.3.2. Simulation Results Analysis

In the system, an asymmetrical short-circuit fault occurred near the medium-voltage side exit at 0.04 s. With the fault type a single-phase grounding fault and the limiting reactance value of 4 ohms, the overvoltage waveform at the neutral point is shown in Figure 11.

From the above figure, it can be seen that the steady-state overvoltage peak at the neutral point was 39.1 kV, and the transient overvoltage peak was 73.5 kV. According to GB 311.1-2012 “Insulation Coordination Part 1: Definitions, Principles, and Rules” [22], the operating voltage of the transformer’s 110 kV side neutral point equipment was 72.5 kV, with a rated lightning impulse withstand voltage of 325 kV and a rated short-duration power frequency withstand voltage of 140 kV. Therefore, when exactly meeting the limiting requirements, the overvoltage at the neutral point was far below the insulation level of the neutral point.

To further clarify the impact of the neutral point overvoltage by the limiting reactance value and fault type and to ensure that it does not have too much impact on relay protection, this work simulated the neutral point overvoltage under different fault types with limiting reactance values ranging from 5 to 10 ohms, and the results are shown in

Table 6. Neutral overvoltage data sheet with different current-limiting reactance values.

Limiting Reactor (Ω)	Single-Phase Ground Fault		Two-Phase Ground Fault
	Steady State (kV)	Transient (kV)	Steady State (kV)	Transient (kV)
5	39.1	73.5	28.9	37.0
5.5	41.1	77.0	30.0	38.2
6	43.2	80.7	30.9	39.3
6.5	45.1	83.6	31.7	40.3
7	46.8	86.3	32.5	41.1
7.5	48.5	88.8	33.1	41.9
8	50.0	91.1	33.8	42.7
8.5	51.4	93.2	34.4	43.3
9	52.7	95.1	34.9	43.9
9.5	54.0	96.6	35.4	44.5
10	55.2	97.9	35.8	45.0

.

The data in Table 6 show that, as the limiting reactance value increased, the neutral point overvoltage also gradually increased. However, when the limiting reactance value was within the range of 5 to 10 ohms, the maximum neutral point overvoltage was only 97.9 kV, which is far below the insulation withstand voltage level of the neutral point. Therefore, the addition of a Fixed Current Limiter (FCL) will not cause the neutral point overvoltage to exceed insulation safety limits. Moreover, with the existing overvoltage protection devices in place, no additional protection measures are necessary.

4. Conclusions

This paper investigated the benefits and feasibility of installing an FSFCL at the neutral point of a 220 kV transformer to limit the asymmetrical short-circuit current on the medium-voltage side. Results demonstrated that the FSFCL can significantly limit the asymmetrical short-circuit current in the medium- and low-voltage windings of the transformer, and the current-limiting effect increases with the reactance value. However, the results also indicate that installing the FSFCL may induce an increase in neutral point overvoltage during short-circuit faults. Then, the reactance value was optimized to ensure the overvoltage stays within the insulation tolerance range, eliminating the need for additional overvoltage protection. This paper provides theoretical guidance for the installation of fault current limiters at the neutral point and the references for the selection of FSFCL parameters.