1. Introduction
Various storable hypergolic propellants, such as monomethylhydrazine (MMH) and dinitrogen tetroxide (N
2O
4), have been extensively applied in space rocket engines for attitude and orbit control due to their fast ignition, variable thrust capabilities, and high specific impulse. However, little research has been conducted to explore the mechanisms of the chemical reaction and the characteristics of spray combustion in MMH/N
2O
4 bipropellants [
1,
2,
3]. To a large extent, this is attributed to their extreme toxicity, corrosivity, and explosive reactivity. As space exploration advances, space propulsion systems face increasingly harsh operational environments to accomplish the missions. Therefore, it is imperative to develop high-performance space engines through a systematic investigation into the spray, combustion, and heat transfer of MMH/N
2O
4.
The development of accurate chemical kinetic models plays a crucial role in both experimental and computational studies of the combustion process. However, there are still few specific mechanisms proposed for MMH/N
2O
4. Catoire et al. [
4] developed a kinetic model involving 403 elementary reactions and 82 species to describe the process of hypergolic ignition for MMH/N
2O
4 gas mixtures. On this basis, Anderson [
5] proposed a mechanism that involves 82 species and 513 reaction steps for MMH and red fuming nitric acid. These mechanisms are considered reliable as they integrate the reaction pathways and theoretical data provided in previous studies. However, they are highly complex, which renders them unsuited to CFD applications. In order to address this issue, Hu et al. [
6] developed a chemical reaction kinetic model by slightly simplifying the mechanism proposed by Anderson for MMH/N
2O
4, which involves 72 species and 406 elementary reactions. Additionally, Hou et al. [
7] established a simplified mechanism with 23 species and 20 steps by taking into account the different reaction characteristics of the MMH/N
2O
4 combustion process at various stages. Although these models have been validated, the scope of their practical application is still limited due to insufficient model details. To reduce computational costs, plenty of numerical studies have been conducted on the spray combustion of MMH/N
2O
4, but they require the use of global reaction models that involve one or more steps [
8,
9]. Notably, these models often ignore the most intermediate reactions, which affects their applicability and accuracy.
In bipropellant thrusters, liquid propellants usually undergo a series of physical and chemical processes, including injection, atomization, evaporation, mixing, turbulent combustion, and conjugate heat transfer. Despite the variation in temporal and spatial scales between these processes, they are closely correlated with each other. For the accurate prediction of these multiphase combustion processes, the numerical modeling of the gas–liquid flow is indispensable [
10,
11]. Typically, there are two approaches adopted to serve this purpose: the Euler–Euler and Euler–Lagrange methods. Each of them has both advantages and disadvantages, depending on the specific application. Under the Euler–Lagrange framework, the liquid phase is represented by the discrete particles tracked using the Lagrangian approach, while the gas phase is solved using the Eulerian approach. However, this leads to a notable limitation that the primary atomization process cannot be resolved, which involves the formation, breakup, and atomization of the liquid sheet. To address this issue, Wei et al. [
12] proposed a doublet injector model under the Euler–Lagrange framework to describe the impinging atomization of liquid propellant. This model has been applied to simulate the process of spray combustion in a 2 kN thruster for accurate prediction of propellant distributions at the impingement points. Comparatively, the Euler–Euler framework, where both the liquid and gas phases are treated as continua, is superior in predicting the atomization process by relying on its ability to capture the moving interfaces. Previous studies have demonstrated that the VOF model, a widely used subset of Eulerian models, is particularly well-suited for simulating primary breakup processes [
13,
14,
15]. Jacobsohn et al. [
16] applied the VOF model to conduct a series of simulations of impinging jet sprays given high Weber numbers, whose results are highly consistent with the experimental results. However, Eulerian models, which require the interface to be fully resolved, are heavily dependent on the grid. For the flows where the dispersed phase exhibits steep gradients and a large void, the computational cost of Eulerian models could be prohibitively high. The VOF to DPM was proposed to be addressed.
Currently, liquid film cooling is commonly practiced in small bipropellant thrusters for a compact design, with the fuel partially injected toward the combustor wall for the formation of a protective cooling film. Due to computational limits, most prior studies of rocket engine combustors focus only on the fluid domain, for which the thermal effects of the solid domain are ignored [
17]. For example, Lee et al. [
18] numerically investigated the effects of impingement jet cooling on combustion flow, efficiency, and wall temperature. The thermal effect in the solid region was ignored in their work. Similarly, Fu et al. [
19] explored the formation and development of cooling liquid films but without considering internal heat conduction within the solid. However, the steep temperature gradients within the solid region, resulting from the presence of the liquid film, means that the accurate modeling of the heat flux and the wall temperature requires the conjugate heat transfer between the high-temperature gas and the solid region [
20]. Differently, Ohminami et al. [
21] considered the effects of the solid region in their study of film-cooled bipropellant thrusters based on a reduced chemical reaction mechanism. In spite of this, wall temperatures were still significantly overestimated, with an error of about 20%.
In this paper, a simplified chemical reaction mechanism suitable for MMH/N2O4 was constructed first for the purpose of three-dimensional combustion. Then, this mechanism was applied to model the jet impingement atomization, turbulent combustion, and conjugate heat transfer occurring in a small bipropellant thruster. Furthermore, the spray combustion characteristics were systematically analyzed, and the performance of different mechanisms was evaluated according to the simulation results.
3. Reduced Mechanisms for MMH/N2O4 Combustion
As previously discussed, a global or multi-step chemical kinetic model has been adopted in most of the existing numerical studies on the MMH/N
2O
4 combustion system. The combustion of MMH/N
2O
4 is a highly complex process involving multiple stages, such as the cold reaction, thermal decomposition, hypergolic ignition, and high-temperature combustion. This poses a challenge to accurately predicting the entire combustion process using a multi-step reaction mechanism. However, it is often costly to perform 3D combustion simulations using a detailed mechanism. To address this issue, the detailed chemical reaction mechanism for MMH/N
2O
4 developed by Anderson et al. [
5] was simplified in this paper through the sensitivity analysis method [
34]. The sensitivity coefficients of elementary reactions, denoted as
, were calculated under the conditions of variable pressure (0.05–0.4 MPa), initial temperature (300–650 K), and molar ratio (0.25–4), as described in Equation (17).
where
Y represents the combustion characteristic, which is defined as the ignition time herein, and
indicates the model parameter of the
ith reaction. By excluding the reactions with sensitivity coefficients below the established threshold, a simplified mechanism involving 31 species and 58 reactions was established.
Table 1 shows a comparison between the proposed reaction mechanism and the existing chemical kinetic models, including the detailed mechanisms proposed by Catoire et al. [
4] and Anderson et al. [
5], as well as the reduced mechanisms presented by Hou et al. [
7] and Wei et al. [
12]. To validate the reaction mechanisms, a series of MMH/N
2O
4 ignition simulations in a zero-dimensional closed homogeneous reactor were performed using Chemkin-Pro software (version 2022 R2).
Figure 1 shows the results that demonstrate the instantaneous temperature changes under different mechanisms. Two distinct cases were analyzed, of which one involved a mixture of MMH/N
2O
4/NO
2 at T = 298 K and P = 0.242 bar, while the other involved MMH/NO
2 at T = 1000 K and P = 1 bar. The temperature changes predicted through the mechanisms proposed by Catoire et al. [
4] and Anderson et al. [
5] are highly consistent, indicating the reliability of these detailed mechanisms. In the absence of experimental data, the results obtained from these detailed mechanisms provide a reference for this paper. Through comparison, it is revealed that the reduced mechanism proposed by Hou et al. [
7] shows a significant discrepancy, with a delayed prediction of the combustion process and an underestimation of the equilibrium temperature. The equilibrium temperatures predicted using the mechanism proposed by Wei et al. [
12] are basically consistent with the reference values, despite some differences in temporal changes. Overall, the results obtained from the proposed reaction mechanism are consistent with those obtained from the detailed mechanisms, demonstrating a higher accuracy compared to the existing reduced mechanisms.
Figure 2 illustrates the changes in equilibrium temperature and ignition delay as a function of the mixture ratio, as predicted using different mechanisms. The mixture ratio, denoted as
Mr, is defined as the molar ratio of N
2O
4 to MMH. Four sets of cases with varying initial pressures (ranging from 1 to 30 atm) and temperatures (from 400 to 600 K) are included. Whether under low or high pressures, the prediction made using the mechanism proposed by Hou et al. [
7] exhibits significant discrepancies relative to the detailed mechanisms. These discrepancies become more pronounced as the mixture ratio varies. In contrast, the mechanism proposed by Wei et al. leads to equilibrium temperature predictions highly consistent with those based on the detailed mechanisms. However, it exhibits notable discrepancies in ignition time, particularly at larger mixture ratios. Furthermore, the mechanism proposed by Wei et al. [
12] shows larger errors at lower mixture ratios, as depicted in
Figure 3.
Figure 3 presents the results of ignition obtained for an MMH/N
2O
4 mixture at T = 400 K, P = 30 bar, and
Mr = 0.15. The combustion process predicted using the mechanism proposed by Wei et al. [
12] exhibits a noticeable delay, as evidenced by the variations in temperature and MMH concentration. In such fuel-rich conditions, the reaction pathways from MMH to CO play a significant role, which results in the substantial production of CO and NH
3, as illustrated in
Figure 4. For the fuel-rich case depicted in
Figure 3, the pathways related to HCN contribute to over 90% of CO production at the peak CO production rate. However, the mechanism proposed by Wei et al. [
12] excludes these pathways, with only the conversion of MMH to HCN retained. Therefore, as shown in
Figure 5, the mechanism proposed by Wei et al. [
12] is used to predict a significant accumulation of HCN with minimal CO production, which results in a significant delay to the combustion process.
As revealed by the above comparisons, the results obtained using the proposed mechanism are more consistent with the reference values obtained from detailed mechanisms under various conditions. The proposed mechanism shows enhanced accuracy and stability compared to the existing models, which makes it more suitable for the modeling of three-dimensional combustion in the following sections.
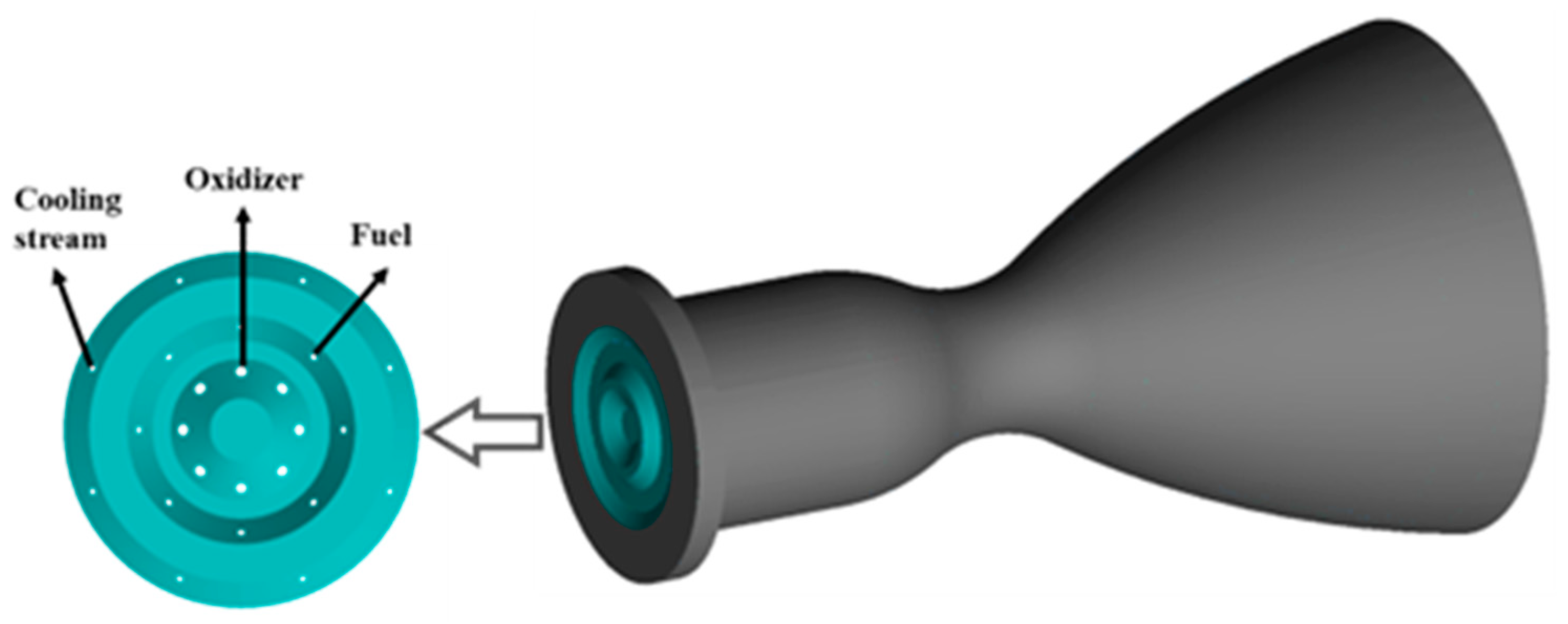
4. Modeling of Atomization Dynamics of Unlike-Doublet Impinging Jets
Doublet impinging jet injectors are frequently used in small bipropellant rocket engines due to their ease of fabrication and high atomization performance. In such systems, the liquid jets of MMH and N2O4 exhibit excellent physical properties, velocities, injection angles, and nozzle sizes, which leads to an asymmetric liquid sheet upon impingement. This asymmetry adds complexity compared to symmetric configurations. In this section, the VOF model is used to explore the spray characteristics of unlike-doublet jets in practical propulsion systems through the transient simulation of the atomization process.
To simplify the computational model, only half of the combustor was included in the computational domain, with the impinging injectors positioned in the mid-plane, as depicted in
Figure 6. The nozzles for MMH and N
2O
4, whose diameters were 0.48 mm and 0.39 mm, respectively, were used to produce jets at the injection angles of 15 and 20 degrees. The mass flux ratio of the two jets was set to 1:1.65. The computational domain was first discretized using a non-uniform grid consisting of 3.5 million hexahedral cells. Then, an automated adaptive refinement method was applied to capture the phase interfaces. Mesh refinement was controlled by the gradients in the liquid phase volume fraction, with constraints imposed on the smallest cell size (10
−18 m
3) and a maximum refinement level of 6. After the spray reached a steady state, the final mesh that contained over 11 million cells was obtained.
Figure 7 illustrates the evolution of the spray generated by the impinging jets of MMH and N
2O
4, as described by the iso-surface of the liquid-phase volume fraction. Initially, a fan-shaped liquid sheet develops at the point of impingement and expands downstream. With the development of unsteady waves induced by hydrodynamic instability and the interactions between the gas and liquid phases, the liquid sheet decomposes progressively into arc-shaped ligaments, large clumps, and, ultimately, finer droplets. This model is effective at capturing both the primary and secondary processes of atomization. When the spray reached a steady state by 0.5 ms, it was characterized by the periodic and consistent shedding of liquid ligaments. The steady-state spray distribution is further illustrated in
Figure 8. Notably, the radial momentum is not fully neutralized due to the larger nozzle diameter and higher mass flux of the N
2O
4 jet compared to the MMH jet. This results in the liquid sheet moving slightly upwards, which is particularly evident in the side regions. Thus, arc-shaped distribution is exhibited in the y–z cross-section. The MMH jet remains more concentrated in the central region of the fan-shaped liquid sheet, with a smaller spray angle and larger breakup length compared to the N
2O
4 jet.
In the subsequent simulation of spray combustion, the Euler–Lagrange method was used. Within this framework, the continuous liquid sheet was represented by discrete droplets, with their initial distributions determined using the flat-fan injection model [
35]. This model requires the use of various parameters, such as the impinging point position, spray half-angle, normal vector, and orifice width. In this study, a statistical analysis of the calculated spray distributions was conducted to determine the key characteristics, such as the spatial location, spray angle, breakup length, and droplet diameter. Then, these characteristics were analyzed to improve the initial droplet settings in the flat-fan model.
Figure 9 shows the discrete droplets generated by the flat-fan model in conjunction with the original liquid sheet distributions. The droplet diameters range from 15 to 125
, conforming to the Rosin–Rammler size distribution, as determined by the spray characteristics. For better visualization, the droplets in the figure are enlarged, with some droplets omitted. According to the results of the comparative analysis, the discrete droplets accurately reflect the overall distribution of the liquid sheet formed by the impinging jets, making them suitable for use as initial propellant distributions in the subsequent simulations of spray combustion.
5. Modeling of Spray Combustion and Heat Transfer in a Small Bipropellant Thruster
In this section, the spray combustion and heat transfer in a small bipropellant thruster are modeled through the proposed mechanism, along with two established mechanisms developed by Wei and Hou. The configuration of the thruster, as depicted in
Figure 10, consists of the injection plane, a combustor, a nozzle-convergent section, and a nozzle-divergent section. The thruster carries a set of unlike impinging injectors, with MMH and N
2O
4 introduced via the inner and outer orifices, respectively. Additionally, an outermost orifice ring is put in place to direct some MMH fuel towards the wall for enhanced impingement cooling. Each ring is equipped with eight uniformly distributed orifices, with the injection parameters detailed in
Section 4. The exhaust back pressure was set to 100 Pa, and the initial distributions of liquid propellant droplets were determined using the flat-fan injection model. Moreover, the parameters were determined according to the spray characteristics described in
Section 4.
To reduce computational costs, only one-quarter of the symmetric cylindrical thruster was included in the computational domain. This domain was discretized using a non-uniform grid comprising 0.58 million hexahedral cells, with specific refinements in the throat and shear layer regions, as illustrated in
Figure 11. The accuracy of the present model was verified by comparing numerical results with the experimental data regarding the wall temperature, chamber pressure, and thrust, as shown in
Table 2. Additionally, to evaluate the grid for its sensitivity, the results obtained with the present mechanism using a refined mesh of 0.75 million cells were compared. The experimental data, including temperature, pressure, and flow sensor measurements, were collected through the hot firing tests conducted in vacuum [
36]. The differences between the computational results obtained from the two mesh resolutions were found to be less than 1%, indicating the reliability of the computational mesh.
Compared to the experimental results, the proposed mechanism can be used to predict the chamber pressure, thrust, and wall temperature with an error of less than 5%. Although the throat wall temperature is slightly overestimated by about 70 K, these discrepancies are still acceptable given the complex interactions between high-temperature gas, liquid film, and solid regions. In contrast, the mechanism proposed by Wei et al. [
12] can also be used to accurately predict the chamber pressure and thrust, but the throat temperature is significantly underestimated by 15%. The mechanism proposed by Hou et al. [
7] leads to a notable underestimation of the chamber pressure, temperature, and thrust, which is consistent with the analysis of the chemical kinetic model presented in
Section 2.
Figure 12 shows the comparisons of temperature distributions calculated using the three mechanisms. The presence of low-temperature regions is predominantly observed around the impingement points of the injected propellants, where intense mixing and vaporization occur. As the propellants move downstream, the temperature increases dramatically due to combustion reactions, with the chamber filled by high-temperature gas products. As shown in
Figure 12, the temperature distribution predicted using the mechanism proposed by Hou et al. [
7] differs significantly from those predicted by Wei et al. [
12] and in the present study. The maximum flame temperature calculated by the mechanism of Hou et al. [
7] is significantly underestimated, with their predicted value being approximately 2600 K. This error is primarily due to the incomplete combustion caused by oversimplified reaction routes.
In contrast, the temperature distributions predicted by Wei et al. [
12] and the present mechanism are more comparable. The main difference lies in the temperature changes at the corner of the injection plane and the side wall. As shown in
Figure 12, the mechanism proposed by Wei et al. [
12] is used to predict a lower temperature at this corner compared to the present mechanism. In this area, the liquid MMH partially impacts the side wall, while the remainder spreads to form a liquid film around the inner chamber wall, which gives rise to a fuel-rich region. The discrepancies in the prediction of Wei et al. [
12] can be attributed to their simplified reaction pathways from MMH to CO. Because their model fails to accurately represent CO production under fuel-rich conditions, excess HCN accumulates, as depicted in
Figure 13 and
Figure 14. These observations are consistent with the zero-dimensional reactor calculations described in
Section 2. Consequently, the wall temperature is underestimated, as detailed in
Table 2, largely due to the incomplete combustion of MMH. The proposed mechanism, however, is accurate in capturing the conversion of HCN to CO in the fuel-rich region, which improves the accuracy of wall temperature prediction.
As shown in
Figure 12, the liquid film forms a thin, low-temperature layer around the inner wall, resulting in a sharp radial temperature gradient. Protected by the liquid film and its vaporized gas, the chamber wall maintains a significantly lower temperature than that of the combustion gas.
Figure 15 shows the distribution of temperatures on the inner and outer wall surfaces calculated using the present mechanism. Although the maximum temperature of the chamber gas exceeds 3000 K, the maximum wall temperature remains around 1700 K, highlighting the protective effect of the impinging cooling jets.
Figure 16 illustrates the distribution of the height of the liquid film around the inner wall. Obviously, this height peaks below the impact point, as indicated by the black circles, before spreading both axially and radially. During this process, the film continuously absorbs heat from the gas side and ultimately evaporates near the contraction section. According to the correlation observed between
Figure 15 and
Figure 16, the liquid film distribution along the inner wall falls within the sawtooth-shaped low-temperature regions, which is consistent with experimental observations [
36].
Figure 17 shows the axial variations in wall temperature and heat flux. Due to the presence of liquid film, a negative heat flux develops on the front side of the chamber wall, with the initial inner wall temperature being lower than that of the outer wall. As the axial distance increases, the protective effect of the liquid film diminishes gradually, resulting in a rise in the inner wall temperature. Near the convergent section, the liquid film disappears, causing a rapid increase in the wall heat flux. Due to the significant decrease in gas temperature and pressure in the divergent region, the wall heat flux and temperature are observed to peak around the throat. It is indicated that the liquid film acting as a thermal barrier reduces the convective heat transfer from the high-temperature gas to the inner chamber wall, which indirectly mitigates the temperature rise in the throat area. Therefore, the reasonable design of the impinging cooling jets is crucial for the high performance of the engine.