Heat Transfer Enhancement in a 3D-Printed Compact Heat Exchanger
Abstract
1. Introduction
1.1. Additive Manufacturing
1.2. Heat Exchange in Complex Structures
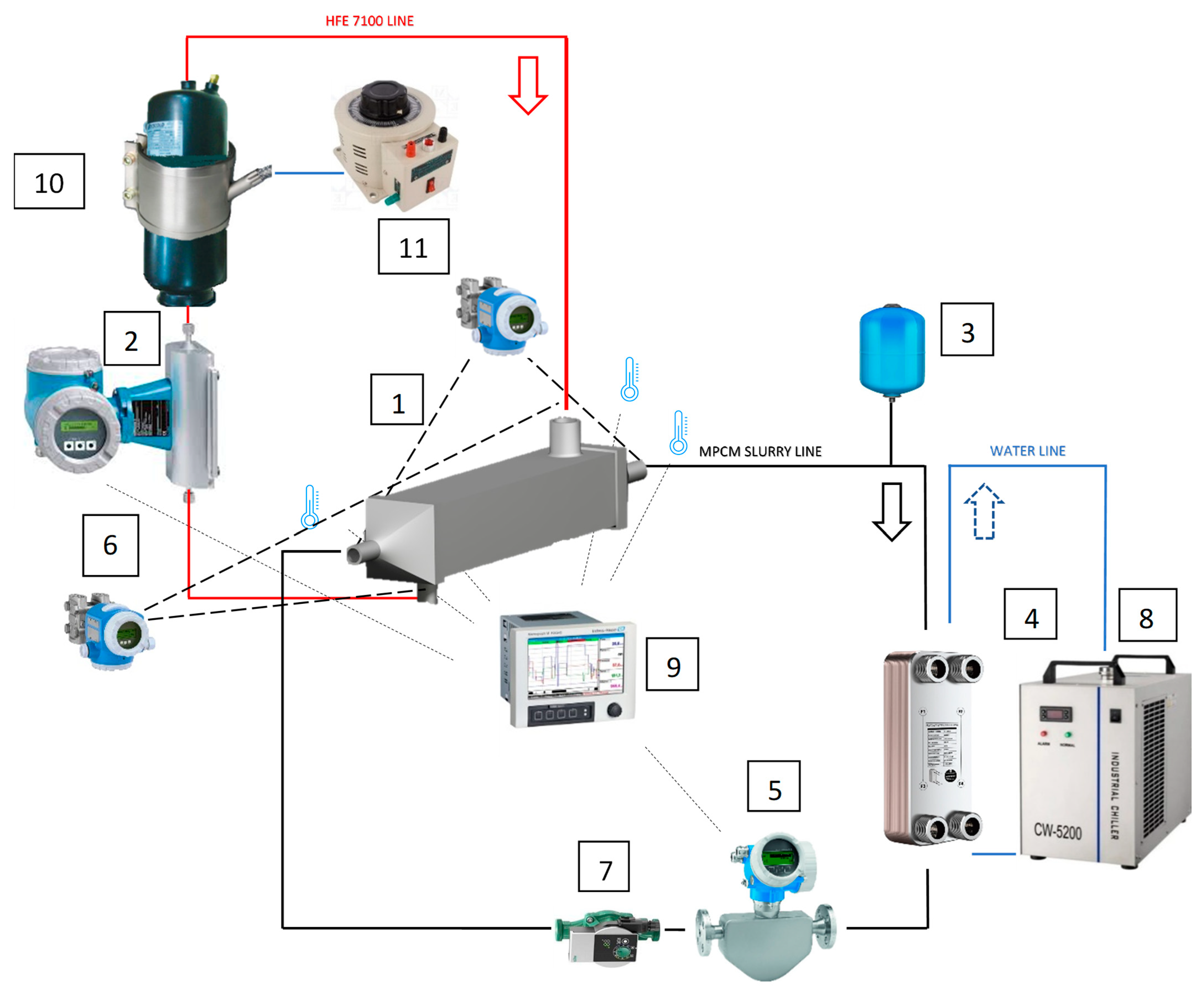
2. The Experimental Facility
3. Experimental Data
4. Conclusions
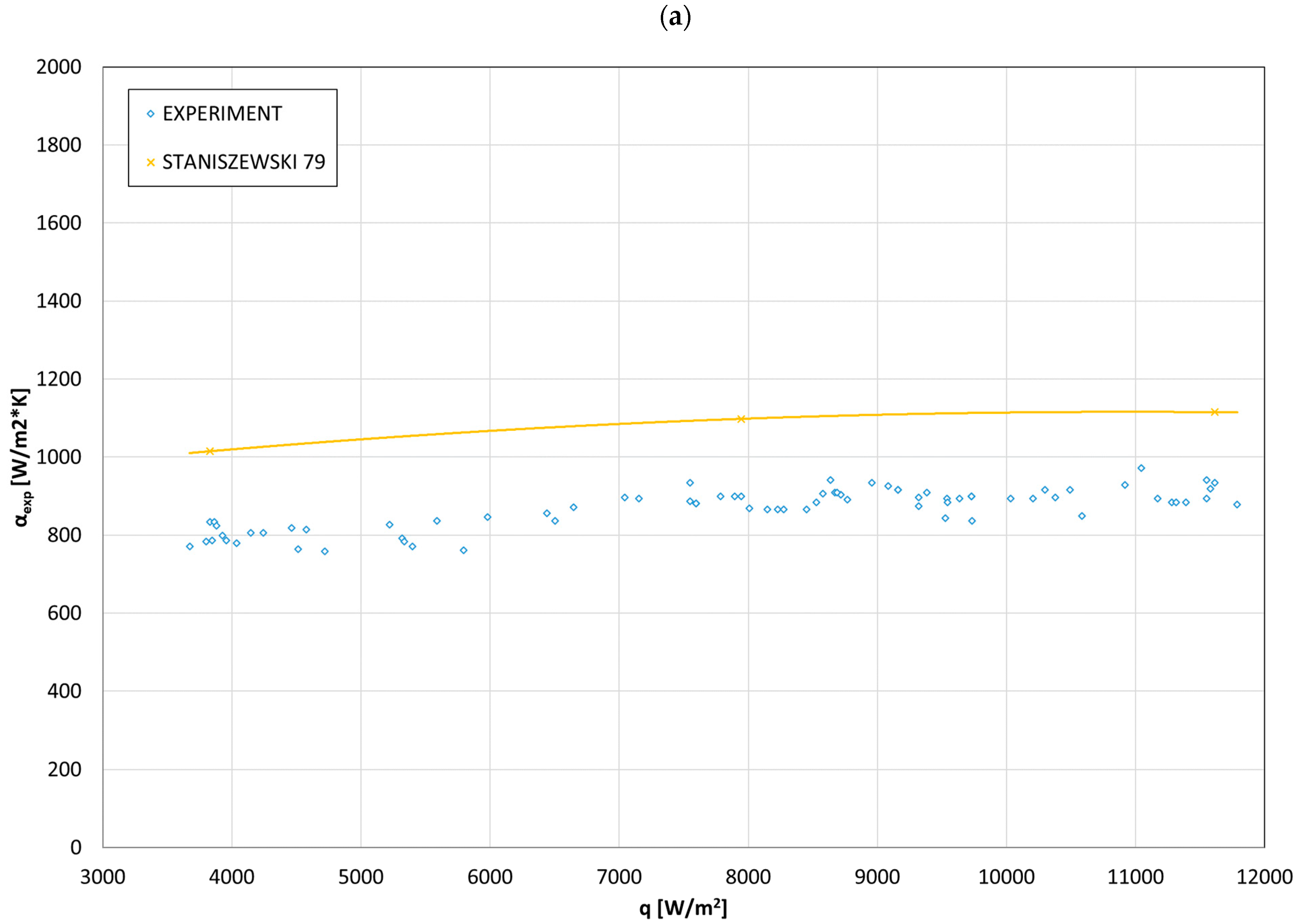
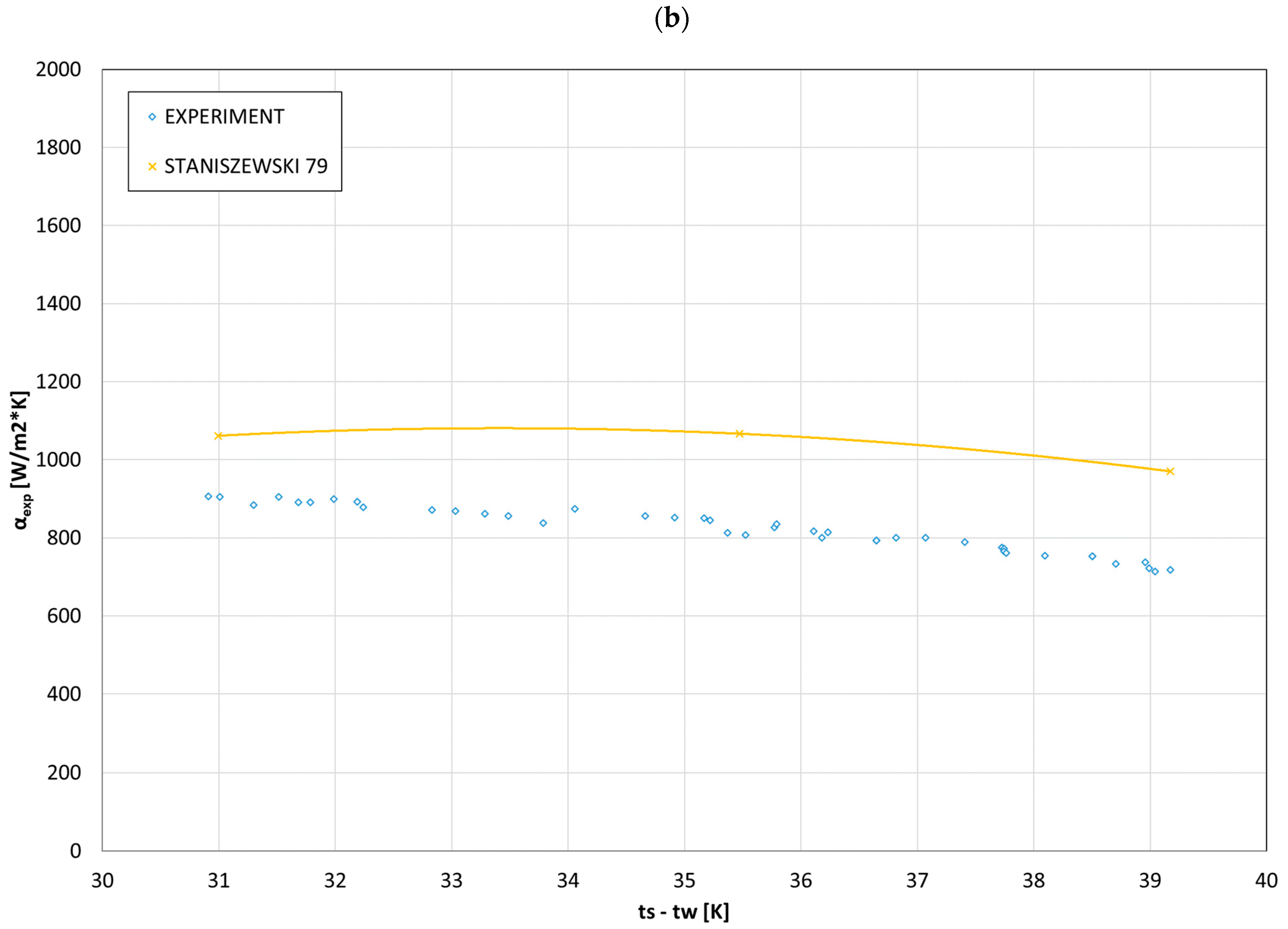
- The test results confirmed that the HTC value has a slight dependence on the heat flux density during refrigerant condensation. The heat exchange coefficient gradually increases with the heat flux density. However, a four-fold increase in q causes only a 10% increase in the value of the α on the cooled surface.
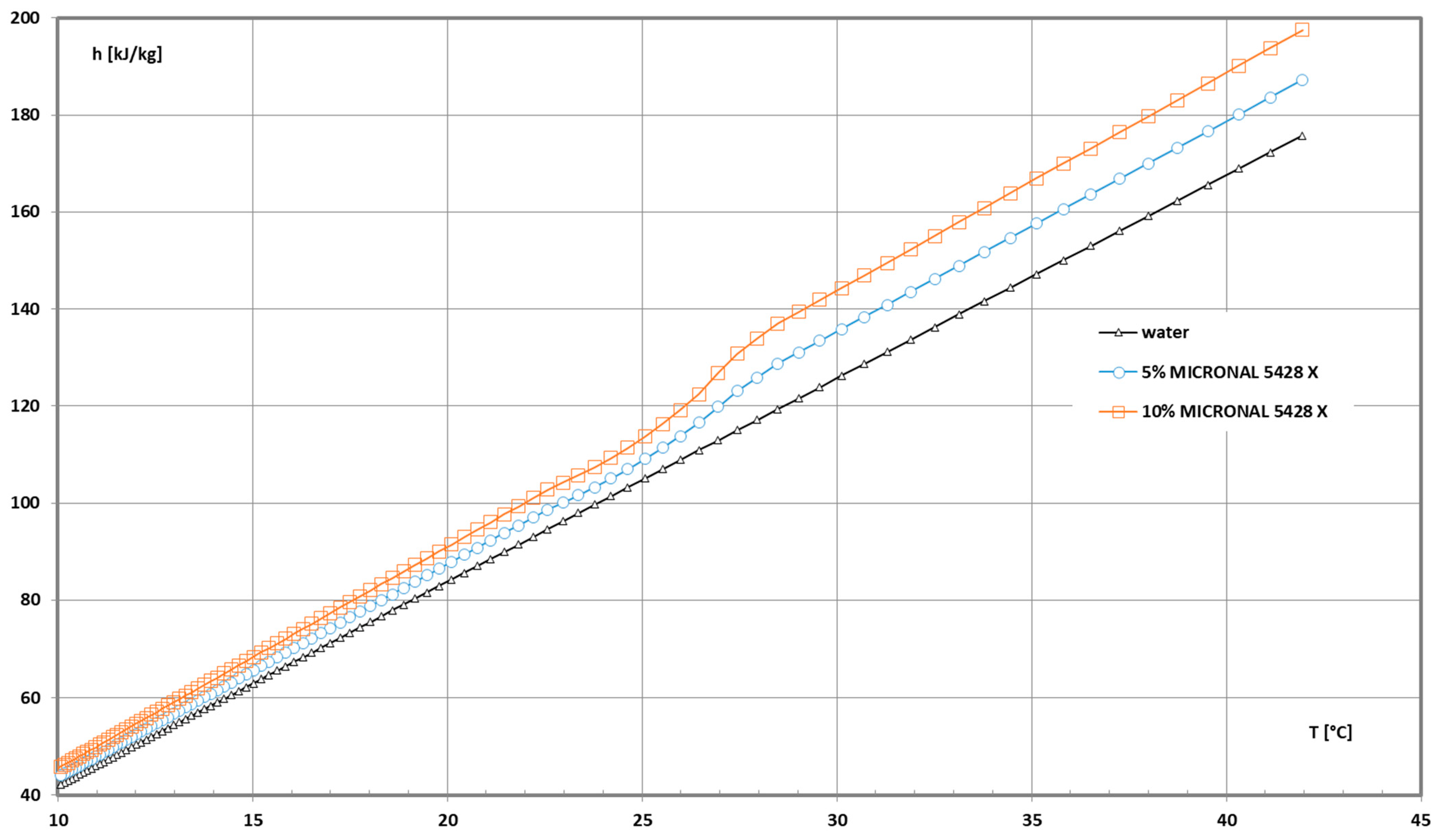
- The thermal power strongly depends on the type of coolant. The highest values were observed during cooling with a 10% mPCM slurry. The lowest heat flux values were noted for water cooling. It was also found that the heat flux increases during the PCM phase transition. The influence of mass share on the value of heat flux was also observed. The higher the mass fraction of mPCM in the mixture, the greater the heat flux.
- The OHTC also depends on the type of coolant. The lowest values were observed during water cooling. The highest overall heat exchange coefficient values were noted during the 10% mPCM slurry cooling. It was also found that the overall heat exchange coefficient increased during the PCM phase transition. For the 10% mPCM, there was an over 11% increase in the overall heat exchange coefficient. The influence of mass share on the value of heat flux was also observed. The higher the mass fraction of mPCM in the mixture, the greater the heat flux.
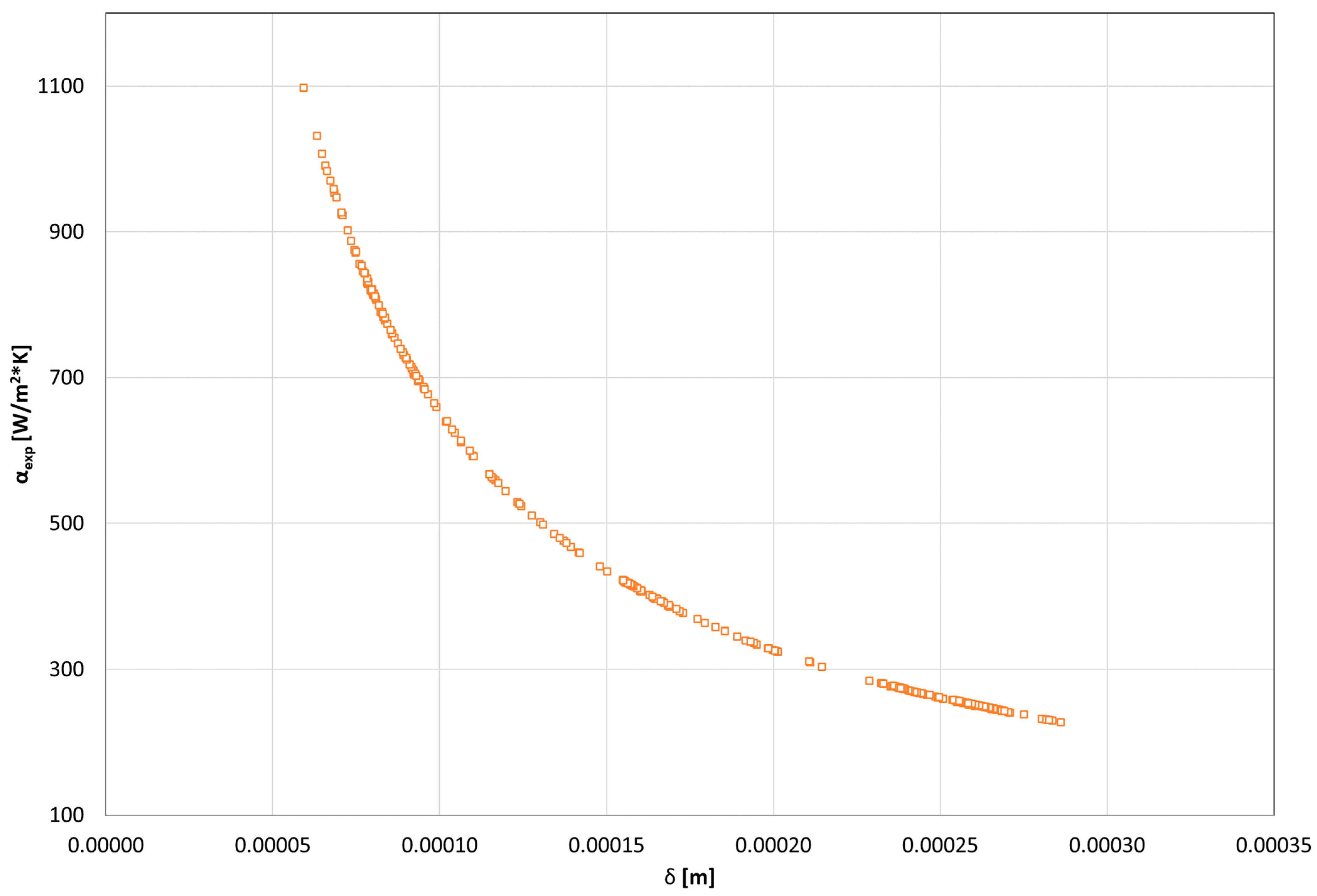
- The refrigerant’s HTC values depend on the thickness of the condensate film. The HTC values decrease significantly between 0.00003 and 0.001 m of the thickness of the condensate film.
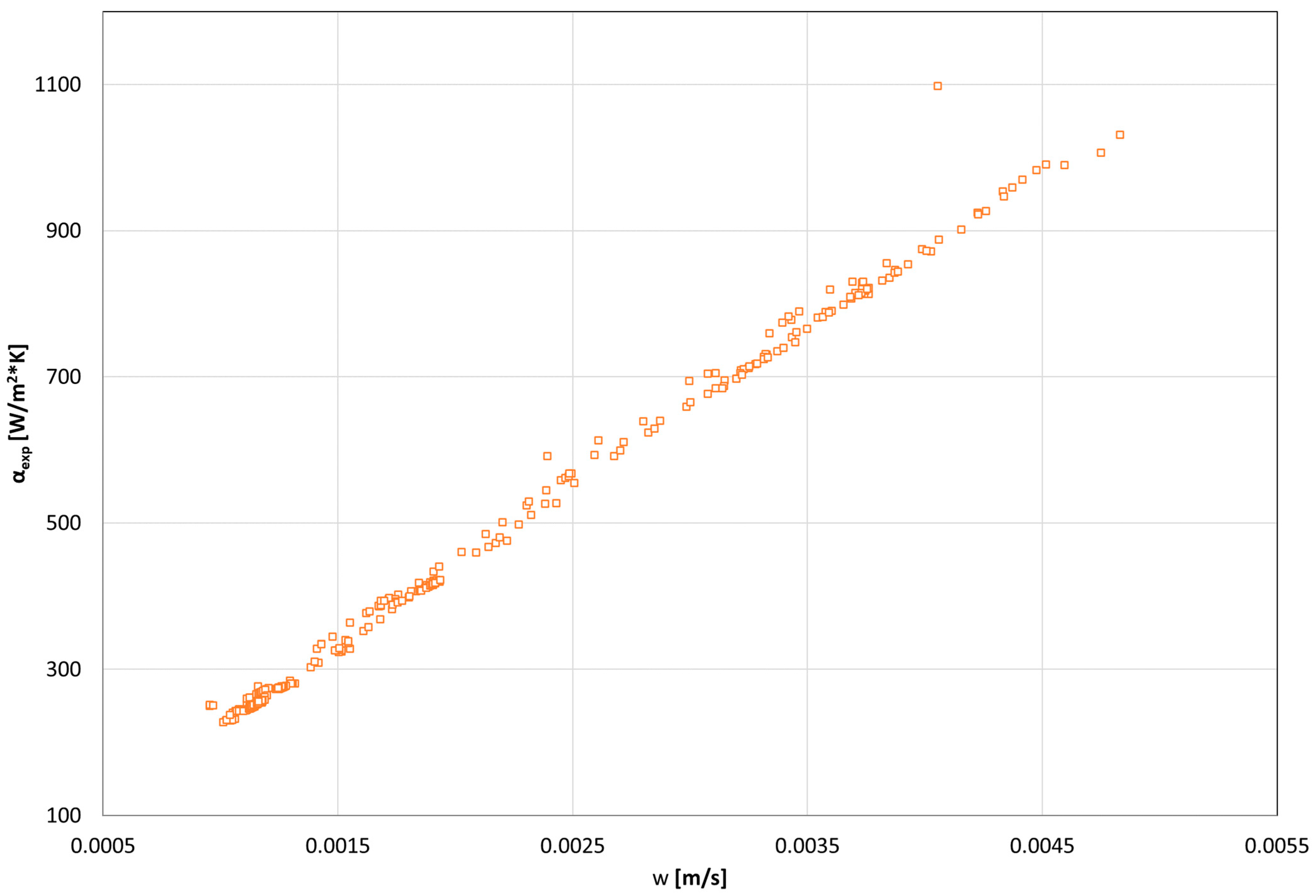
- The value of the HTC is proportional to the increase in the velocity of the condensate, which results from the laminar nature of the condensate flow. An increase in the condensate velocity reduces the thickness of the condensate layer, which reduces the value of thermal resistance and increases the value of the HTC.
- The authors demonstrated the possibility of using Equation (9) to determine the value of the HTC during condensation of the refrigerant on the surface of a smooth pipe bundle inside a compact heat exchanger made by 3D printing. This equation can be used in compact heat exchanger projects.
- The research results indicate the need to conduct further experimental research on the heat exchange enhancement regarding the impact of process parameters such as Δtlog, or the mass fraction of the mPCM in the cooling liquid, and the internal geometry of 3D-printed mini heat exchangers.
- The future direction of the experimental research is to determine the effect of the state of matter of the phase change material mixture on the flow resistance in compact heat exchangers, and the impact of deposits of cooling mixtures on the operating parameters of heat exchangers.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | area (m2) |
| d | diameter (m) |
| G | mass flux density (kg·m−2·s−1); |
| h | enthalpy (kJ·kg−1) |
| L | length (m) |
| ṁ | mass flow rate (kg·h−1) |
| Nu | Nusselt number |
| q | heat flux density (W·m−2) |
| Q | heat flux (W) |
| r | heat of condensation/evaporation (J·k−1) |
| Re | Reynolds number |
| t | temperature (°C) |
| T | temperature (K) |
| Index | |
| c | condensation, coolant |
| exp | experiment |
| e | external |
| f | fluid |
| h | hydraulic |
| i | internal |
| l | liquid |
| r | heat of phase change |
| th | theoretical |
| w | wall, water |
| Greek symbols | |
| α | heat exchange coefficient (W·m−2∙K−1) |
| Δ | difference |
| λ | thermal conductivity (W·m−1·K−1) |
| ν | kinematic viscosity (m−2 s−1) |
| Acronyms | |
| HE | heat exchanger |
| HTC | heat exchange coefficient |
| OHTC | Overall heat exchange coefficient |
| mPCM | microencapsulated phase change material |
References
- Tang, W.; Zou, C.; Zhou, H.; Zhang, L.; Zeng, Y.; Sun, L.; Zhao, Y.; Yan, M.; Fu, J.; Hu, J.; et al. A novel convective heat transfer enhancement method based on precise control of Gyroid-type TPMS lattice structure. Appl. Therm. Eng. 2023, 230, 120797. [Google Scholar] [CrossRef]
- Reynolds, B.W.; Fee, C.J.; Morison, K.R.; Holland, D.J. Characterisation of Heat Transfer within 3D Printed TPMS Heat Exchangers. Int. J. Heat Mass Transf. 2023, 212, 124264. [Google Scholar] [CrossRef]
- Wu, Y.; Zhi, C.; Wang, Z.; Chen, Y.; Wang, C.; Chen, Q.; Tan, G.; Ming, T. Enhanced thermal and mechanical performance of 3D architected micro-channel heat exchangers. Heliyon 2023, 9, e13902. [Google Scholar] [CrossRef]
- Haertel, J.H.K.; Nellis, G.F. A fully developed flow thermofluid model for topology optimization of 3D-printed air-cooled heat exchangers. Appl. Therm. Eng. 2017, 119, 3–24. [Google Scholar] [CrossRef]
- Ahmadi, B.; Cesarano, J.; Nawaz, K.; Ninos, N.; Bigham, S. A high-performance lung-inspired ceramic 3D-printed heat exchanger for high-temperature energy-efficient systems. Appl. Therm. Eng. 2023, 219, 119378. [Google Scholar] [CrossRef]
- Puttur, U.; Ahmadi, M.; Ahmadi, B.; Bigham, S. A novel lung-inspired 3D-printed desiccant-coated heat exchanger for high-performance humidity management in buildings. Energy Convers. Manag. 2022, 252, 115074. [Google Scholar] [CrossRef]
- Kus, K.; Wójcik, M.; Malecha, Z.; Rogala, Z. Numerical and experimental investigation of the gyroid heat exchanger. Int. J. Heat Mass Transf. 2024, 231, 125882, ISSN 0017-9310. [Google Scholar] [CrossRef]
- Pracht, S.; Will, J.; Klöppel, S.; Funke, T.; Quack, H.; Haberstroh, C. Experimental and numerical study of a 3D-printed aluminium cryogenic heat exchanger for compact Brayton refrigerators. Cryogenics 2022, 123, 103418. [Google Scholar] [CrossRef]
- Dixit, T.; Al-Hajri, E.; Paul, M.C.; Nithiarasu, P.; Kumar, S. High performance, microarchitected, compact heat exchanger enabled by 3D printing. Appl. Therm. Eng. 2022, 210, 118339. [Google Scholar] [CrossRef]
- Shin, D.; Jeon, J.; Kim, T.; Park, J.H.; Kim, S.J. Development of the thermal performance model using temperature gradient analysis for optimized design of steam surface condenser. Int. J. Heat Mass Transf. 2020, 163, 120411. [Google Scholar] [CrossRef]
- Li, Z.; Xing, W.; Sun, J.; Feng, X. Multiscale structural characteristics and Heat–Moisture properties of 3D printed building Walls: A review. Constr. Build. Mater. 2023, 365, 130102. [Google Scholar] [CrossRef]
- Ahmadi, B.; Bigham, S. Performance Evaluation of hi-k Lung-inspired 3D-printed Polymer Heat Exchangers. Appl. Therm. Eng. 2022, 204, 117993. [Google Scholar] [CrossRef]
- Wang, C.S.; Wang, E.S.; Huang, Y.J.; Liou, T.M. PIV and IRT measurements of hydrothermal performance in a U-shaped heat exchanger with 3D printed detached curved ribs. Int. J. Heat Mass Transf. 2023, 201, 123562. [Google Scholar] [CrossRef]
- Khan, H.H.; Aneesh, A.M.; Sharma, A.; Srivastava, A.; Chaudhuri, P. Thermal-hydraulic characteristics and performance of 3D wavy channel based printed circuit heat exchanger. Appl. Therm. Eng. 2015, 87, 3–528. [Google Scholar] [CrossRef]
- Aneesh, A.M.; Sharma, A.; Srivastava, A.; Vyas, K.N.; Chaudhuri, P. Thermal-hydraulic characteristics and performance of 3D straight channel based printed circuit heat exchanger. Appl. Therm. Eng. 2016, 98, 3–482. [Google Scholar] [CrossRef]
- Lowrey, S.; Hughes, C.; Sun, Z. Thermal-hydraulic performance investigation of an aluminium plate heat exchanger and a 3D-printed polymer plate heat exchanger. Appl. Therm. Eng. 2021, 194, 117060. [Google Scholar] [CrossRef]
- Bayaniahangar, R.; Okoh, I.; Nawaz, K.; Cesarano, J.; Bigham, S. Toward extreme high-temperature supercritical CO2 power cycles: Leakage characterization of ceramic 3D-printed heat exchangers. Addit. Manuf. 2022, 54, 102783. [Google Scholar] [CrossRef]
- Zhao, B.; Zhang, J.; Lian, W. Numerical Modeling of Heat Exchanger Filled with Octahedral Lattice Frame Porous Material. Aerospace 2022, 9, 238. [Google Scholar] [CrossRef]
- Kim, S.; Nam, S.H.; Kim, S.; Cho, Y.T.; Fang, N.X. Low Heat Capacity 3D Hollow Microarchitected Reactors for Thermal and Fluid Applications. Energies 2022, 15, 4073. [Google Scholar] [CrossRef]
- Dutkowski, K.; Kruzel, M.; Rokosz, K. Review of the State-of-the-Art Uses of Minimal Surfaces in Heat Transfer. Energies 2022, 15, 7994. [Google Scholar] [CrossRef]
- Zhu, Z.; Li, J.; Peng, H.; Liu, D. Nature-inspired structures applied in heat transfer enhancement and drag reduction. Micromachines 2021, 12, 656. [Google Scholar] [CrossRef] [PubMed]
- Pelanconi, M.; Zavattoni, S.; Cornolti, L.; Puragliesi, R.; Arrivabeni, E.; Ferrari, L.; Gianella, S.; Barbato, M.; Ortona, A. Application of ceramic lattice structures to design compact, high temperature heat exchangers: Material and architecture selection. Materials 2021, 14, 3225. [Google Scholar] [CrossRef] [PubMed]
- Kelava, L.; Ivić, I.; Pakai, E.; Fekete, K.; Maroti, P.; Told, R.; Ujfalusi, Z.; Garami, A. Stereolithography 3D Printing of a Heat Exchanger for Advanced Temperature Control in Wire Myography. Polymers 2022, 14, 471. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Xu, P.; Li, L.; Li, Z.; Qi, H.; Wang, C.; Zhang, Y.; Xie, Y.; Tan, D. Multiphase dynamic interfaces and abrasive transport dynamics for abrasive flow machining in shear thickening transition states. Powder Technol. 2024, 446, 120150. [Google Scholar] [CrossRef]
- Mehendale, S.; Jacobi, A.M.; Shah, M.M. Fluid flow and heat transfer at micro- and meso-scales with application to heat exchanger design. Appl. Mech. Rev. 2000, 53, 175–193. [Google Scholar] [CrossRef]
- Kandlikar, S.G.; Grande, W.J. Evolution of Microchannel Flow Passages--Thermohydraulic Performance and Fabrication Technology. Heat Transf. Eng. 2003, 24, 3–17. [Google Scholar] [CrossRef]
- Dutkowski, K.; Kruzel, M.; Zajączkowski, B.; Białko, B. The experimental investigation of mPCM slurries density at phase change temperature. Int. J. Heat Mass Transf. 2020, 159, 3. [Google Scholar] [CrossRef]
- Dutkowski, K.; Kruzel, M.; Zajączkowski, B. Determining the Heat of Fusion and Specific Heat of Microencapsulated Phase Change Material Slurry by Thermal Delay Method. Energies 2020, 14, 3. [Google Scholar] [CrossRef]
- Dutkowski, K.; Kruzel, M. Microencapsulated PCM slurries’ dynamic viscosity experimental investigation and temperature-dependent prediction model. Int. J. Heat Mass Transf. 2019, 145, 118741. [Google Scholar] [CrossRef]
- Kruzel, M.; Bohdal, T.; Dutkowski, K.; Radchenko, M. The Effect of Microencapsulated PCM Slurry Coolant on the Efficiency of a Shell and Tube Heat Exchanger. Energies 2022, 15, 5142. [Google Scholar] [CrossRef]
- Kruzel, M.; Bohdal, T.; Dutkowski, K. External condensation of hfe 7000 and hfe 7100 refrigerants in shell and tube heat exchangers. Materials 2021, 14, 6825. [Google Scholar] [CrossRef] [PubMed]
- Dutkowski, K.; Kruzel, M.; Bohdal, T. Experimental studies of the influence of microencapsulated phase change material on thermal parameters of a flat liquid solar collector. Energies 2021, 14, 5135. [Google Scholar] [CrossRef]
- Staniszewski, B. Heat Eexchange. Theoretical Fundamentals; PWN: Warsaw, Poland, 1979. [Google Scholar]
- Madejski, J. Theory of Heat Transfer; Scientific Publishing House of the Szczecin University of Technology: Szczecin, Poland, 1998; ISBN 83-87423-47-5. (In Polish) [Google Scholar]
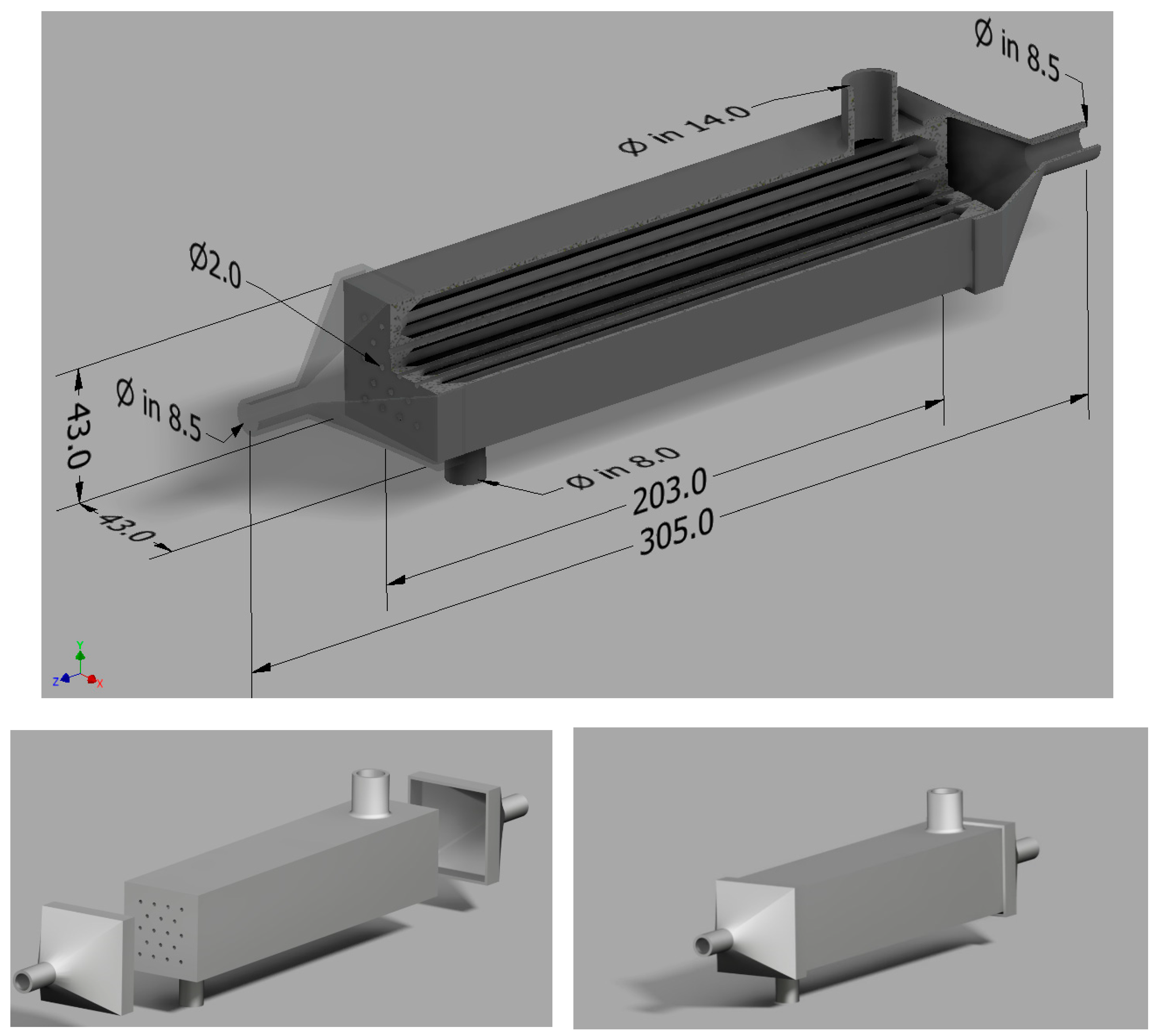
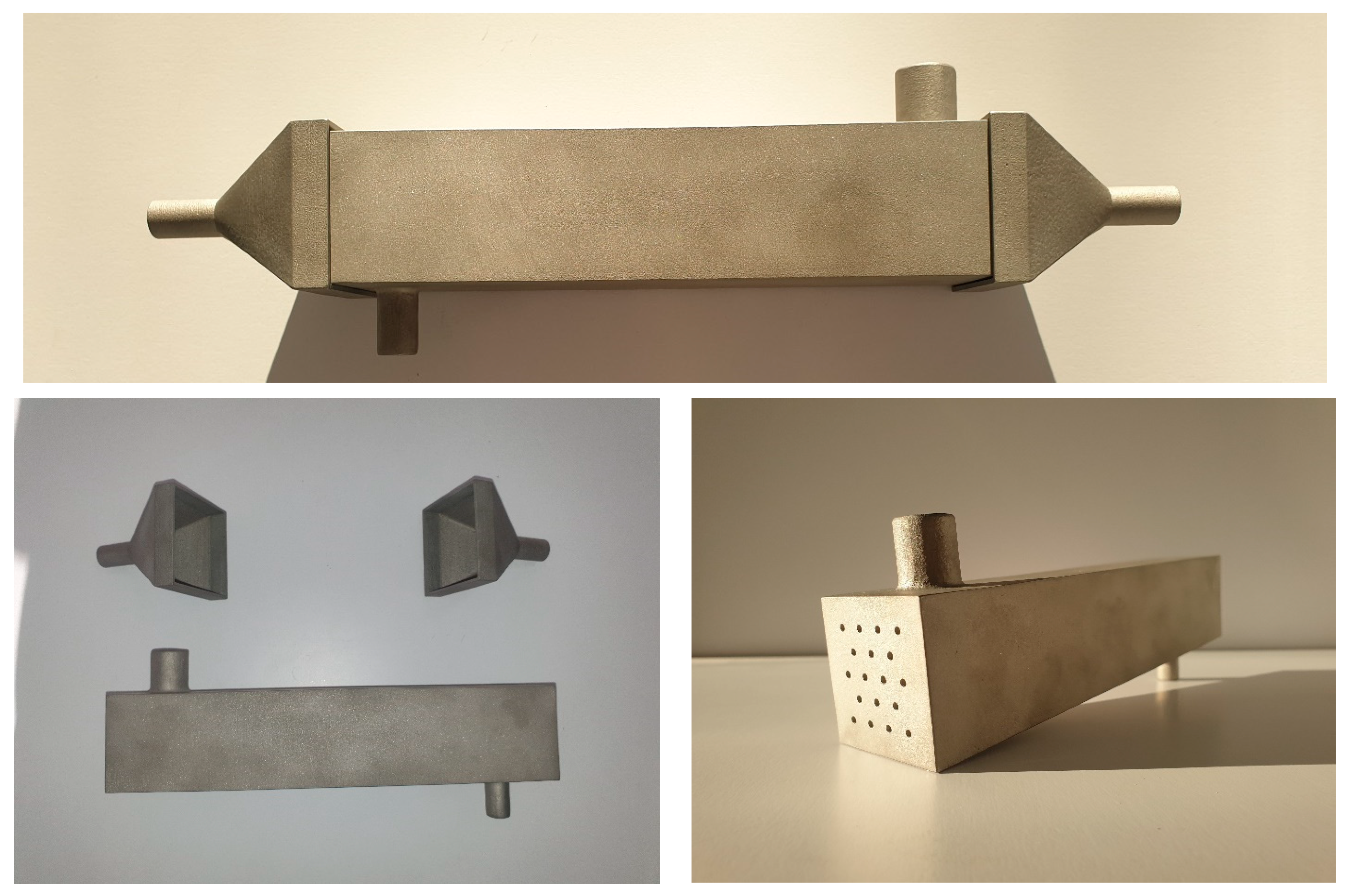



| Parameter | Value |
|---|---|
| internal diameter dw | 2 mm |
| volume | 3.754 × 10−4 m3 |
| material | Steel alloy SS316L |
| number of channels | 18 |
| roughness Ra | 11.9 μm |
| roughness Rz | 62.2 μm |
| roughness Rmax | 74.8 μm |
| Temperature [°C] | Density [kg/m3] | Heat Conductivity Coefficient [W/mK] | Specific Enthalpy [kJ/kg] | |||
|---|---|---|---|---|---|---|
| Concentration | 5% | 10% | 5% | 10% | 5% | 10% |
| 20 | 994.97 | 991.77 | 0.5887 | 0.579 | 86.0 | 87.84 |
| 22 | 994.67 | 991.59 | 0.5910 | 0.581 | 93.4 | 95.37 |
| 24 | 994.26 | 991.26 | 0.6032 | 0.601 | 103.2 | 105.07 |
| 26 | 993.85 | 990.89 | 0.6607 | 0.713 | 111.3 | 113.80 |
| 28 | 993.25 | 990.26 | 0.6157 | 0.619 | 121.5 | 125.86 |
| 30 | 992.38 | 989.16 | 0.6165 | 0.618 | 131.0 | 135.83 |
| Value | Equipment | Range | Uncertainty |
|---|---|---|---|
| Mass flow rate | Mass flow meters | 0–450 kg·h−1 | ±0.15% |
| Pressure | Pressure sensor | 0–2500 kPa | ±0.05% |
| Differential pressure sensor | 0–50 kPa | ±0.075% | |
| Temperature | Thermocouples TP-201K-1B-100 | −40−+475 °C | ±0.2 K |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kruzel, M.; Bohdal, T.; Dutkowski, K. Heat Transfer Enhancement in a 3D-Printed Compact Heat Exchanger. Energies 2024, 17, 4754. https://doi.org/10.3390/en17184754
Kruzel M, Bohdal T, Dutkowski K. Heat Transfer Enhancement in a 3D-Printed Compact Heat Exchanger. Energies. 2024; 17(18):4754. https://doi.org/10.3390/en17184754
Chicago/Turabian StyleKruzel, Marcin, Tadeusz Bohdal, and Krzysztof Dutkowski. 2024. "Heat Transfer Enhancement in a 3D-Printed Compact Heat Exchanger" Energies 17, no. 18: 4754. https://doi.org/10.3390/en17184754
APA StyleKruzel, M., Bohdal, T., & Dutkowski, K. (2024). Heat Transfer Enhancement in a 3D-Printed Compact Heat Exchanger. Energies, 17(18), 4754. https://doi.org/10.3390/en17184754








