Dielectric Constant Predictions for Jet-Range Hydrocarbons: Evaluating the Clausius–Mossotti Relation and Correcting for Molecular Dipole Moments
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Dipole Moment Calculation
3.2. Model Performance
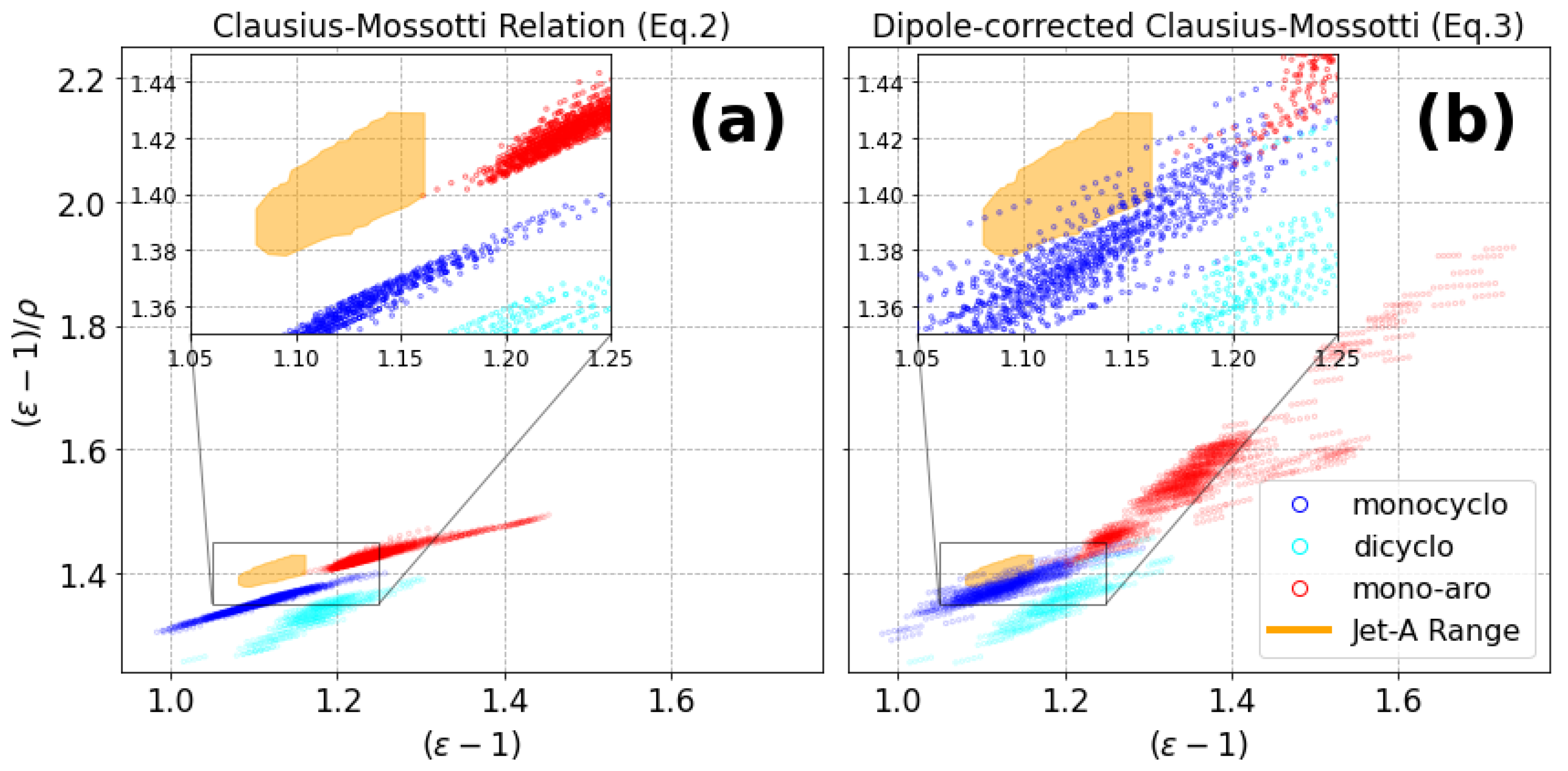
3.3. Impacts on Model Application
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| α | Molecular polarizability. |
| ASTM | ASTM International. |
| C-M (or CM) | Clausius–Mossotti relation. |
| CPK | Cycloparaffinic kerosene. |
| D | Debye. |
| ε | Dielectric constant. |
| FQIS | Fuel quantity indicator system. |
| μ | Permanent dipole moment. |
| Na | Avogadro’s number. |
| NIST | National Institute of Standards and Technology. |
| QSPR/QSAR | Quantitative structure property/activity relationship. |
| R2 | Coefficient of determination. |
| SAF | Sustainable aviation fuel. |
| SPK | Synthetic paraffinic kerosene |
| Vm | Molar volume. |
References
- Air Transport Action Group. Waypoint 2050: Balancing Growth in Connectivity with a Comprehensive Global Air Transport Response to the Climate Emergency: A Vision of Net-Zero Aviation by Mid-Century; Air Transport Action Group: Geneva, Switzerland, 2021; Available online: https://aviationbenefits.org/environmental-efficiency/climate-action/waypoint-2050/ (accessed on 16 June 2024).
- SAF Grand Challenge Roadmap Flight Plan for Sustainable Aviation Fuel; US Department of Energy: Washington, DC, USA, 2022. Available online: https://www.energy.gov/sites/default/files/2022-09/beto-saf-gc-roadmap-report-sept-2022.pdf (accessed on 25 January 2023).
- ASTM D7566-22a; Standard Specification for Aviation Turbine Fuel Containing Synthesized Hydrocarbons 1. ASTM: West Conshohocken, PA, USA, 2023. [CrossRef]
- Connors, M. A Systems Design Approach to Fuel Measurement in Hybrid-Electric Aircraft; Vertical Flight Society: Fairfax, VA, USA, 2020. [Google Scholar]
- Brasfield, R.G. Patent: Fuel Gaging System. WO1984001428A1, 12 April 1984. [Google Scholar]
- ASTM D924; JetDC 88500-0 Measurement of Dielectric Constant of Aviation Turbine Fuel IP PM-FC/21. ASTM: West Conshohocken, PA, USA. Available online: https://www.stanhope-seta.co.uk/ (accessed on 22 May 2024).
- Yang, Z.; Bell, D.C.; Boehm, R.C.; Fischer Marques, P.; Boze, J.A.; Kosilkin, I.V.; Heyne, J.S. Assessing the Effect of Composition on Dielectric Constant of Sustainable Aviation Fuel. Available online: https://ssrn.com/abstract=4700499 (accessed on 22 May 2024).
- ASTM D7566-23b; Standard Specification for Aviation Turbine Fuel Containing Synthesized Hydrocarbons 1. ASTM: West Conshohocken, PA, USA, 2024. [CrossRef]
- Kosir, S.; Landsaw, A.; Steinecker, W.H.; West, Z.J.; Zabarnick, S. Synthetic Blend Component Study: The Effects of Hydrocarbon Composition on Aviation Fuel Dielectric Constant. Energy Fuels 2024, 38, 16451–16457. [Google Scholar] [CrossRef]
- Boehm, R.C.; Faulhaber, C.; Behnke, L.; Heyne, J. The Effect of Theoretical SAF Composition on Calculated Engine and Aircraft Efficiency. Fuel 2024, 371, 132049. [Google Scholar] [CrossRef]
- Faulhaber, C.; Borland, C.; Boehm, R.; Heyne, J. Measurements of Nitrile Rubber Absorption of Hydrocarbons: Trends for Sustainable Aviation Fuel Compatibility. Energy Fuels 2023, 37, 9207–9219. [Google Scholar] [CrossRef]
- Yang, Z.; Xu, Z.; Feng, M.; Cort, J.R.; Gieleciak, R.; Heyne, J.; Yang, B. Lignin-Based Jet Fuel and Its Blending Effect with Conventional Jet Fuel. Fuel 2022, 321, 124040. [Google Scholar] [CrossRef]
- Muldoon, J.A.; Harvey, B.G. Bio-Based Cycloalkanes: The Missing Link to High-Performance Sustainable Jet Fuels. ChemSusChem 2020, 13, 5777–5807. [Google Scholar] [CrossRef]
- Wang, J.; Xie, X.Q.; Hou, T.; Xu, X. Fast Approaches for Molecular Polarizability Calculations. J. Phys. Chem. A 2007, 111, 4443–4448. [Google Scholar] [CrossRef]
- Van Rysselberghe, P. Remarks Concerning the Clausius-Mossotti Law. J. Phys. Chem. 1932, 36, 1152–1155. [Google Scholar] [CrossRef]
- Talebian, E.; Talebian, M. A General Review on the Derivation of Clausius-Mossotti Relation. Optik 2013, 124, 2324–2326. [Google Scholar] [CrossRef]
- Kosir, S.; Stachler, R.; Heyne, J.; Hauck, F. High-Performance Jet Fuel Optimization and Uncertainty Analysis. Fuel 2020, 281, 118718. [Google Scholar] [CrossRef]
- Kroyan, Y.; Wojcieszyk, M.; Kaario, O.; Larmi, M. Modeling the Impact of Sustainable Aviation Fuel Properties on End-Use Performance and Emissions in Aircraft Jet Engines. Energy 2022, 255, 124470. [Google Scholar] [CrossRef]
- Hadaller, O.; Johnson, J. World Fuel Sampling Program; Coordinating Research Council: Alpharetta, GA, USA, 2006. [Google Scholar]
- ARINC611-1; ARINC Report 611-1 Guidance for the Design and Installation of Fuel Quantity Systems. ARINC: Annapolis, MD, USA, 1999.
- Kroenlein, K.; Muzny, C.; Kazakov, A.; Diky, V.; Chirico, R.; Magee, J.; Abdulagatov, I.; Frenkel, M. NIST Standard Reference 203: TRC Web Thermo Tables (WTT) Version 2-2012-1 Professional; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2012. [Google Scholar]
- Bosque, R.; Sales, J. Polarizabilities of Solvents from the Chemical Composition. J. Chem. Inf. Comput. Sci. 2002, 42, 1154–1163. [Google Scholar] [CrossRef] [PubMed]
- Szabo, A.; Ostlund, N. Modern Quantum Chemistry: Introduction to Advanced Electronic Structure Theory; Dover Publications: Mineola, NY, USA, 1996. [Google Scholar]
- Smith, D.G.A.; Burns, L.A.; Simmonett, A.C.; Parrish, R.M.; Schieber, M.C.; Galvelis, R.; Kraus, P.; Kruse, H.; Di Remigio, R.; Alenaizan, A.; et al. 1.4: Open-Source Software for High-Throughput Quantum Chemistry. J. Chem. Phys. 2020, 152, 184108. [Google Scholar] [CrossRef] [PubMed]
- O’Boyle, N.M.; Banck, M.; James, C.A.; Morley, C.; Vandermeersch, T.; Hutchison, G.R. Open Babel: An Open Chemical Toolbox. J. Cheminform. 2011, 3, 33. [Google Scholar] [CrossRef] [PubMed]
- Weininger, D. SMILES, a Chemical Language and Information System. 1. Introduction to Methodology and Encoding Rules. J. Chem. Inf. Comput. Sci. 1988, 28, 31–36. [Google Scholar] [CrossRef]
- Landrum, G. RDKit: Open-Source Cheminformatics. Q2 2010. Version 2023.09.05. Available online: https://www.rdkit.org (accessed on 23 January 2024).
- Hariharan, P.C.; Pople, J.A. The Influence of Polarization Functions on Molecular Orbital Hydrogenation Energies. Theor. Chim. Acta 1973, 28, 213–222. [Google Scholar] [CrossRef]
- Francl, M.M.; Pietro, W.J.; Hehre, W.J.; Binkley, J.S.; Gordon, M.S.; DeFrees, D.J.; Pople, J.A. Self-Consistent Molecular Orbital Methods. XXIII. A Polarization-Type Basis Set for Second-Row Elements. J. Chem. Phys. 1982, 77, 3654–3665. [Google Scholar] [CrossRef]
- Hait, D.; Head-Gordon, M. How Accurate Is Density Functional Theory at Predicting Dipole Moments? An Assessment Using a New Database of 200 Benchmark Values. J. Chem. Theory Comput. 2018, 14, 1969–1981. [Google Scholar] [CrossRef]
- Zapata, J.C.; McKemmish, L.K. On the Computation of Dipole Moments: A Recommendation on the Choice of the Basis Set and the Level of Theory. J. Phys. Chem. 2020, 124, 7538–7548. [Google Scholar] [CrossRef]
- Caleman, C.; Van Maaren, P.J.; Hong, M.; Hub, J.S.; Costa, L.T.; Van Der Spoel, D. Force Field Benchmark of Organic Liquids: Density, Enthalpy of Vaporization, Heat Capacities, Surface Tension, Isothermal Compressibility, Volumetric Expansion Coefficient, and Dielectric Constant. J. Chem. Theory Comput. 2012, 8, 61–74. [Google Scholar] [CrossRef]
- ASTM D1319-20a; Standard Test Method for Hydrocarbon Types in Liquid Petroleum Products by Fluorescent Indicator Adsorption 1. ASTM: West Conshohocken, PA, USA, 2020. [CrossRef]
- CAAFI. Fuel Readiness Level (FRL); CAAFI: Bristol, UK, 2010; Available online: https://www.caafi.org/information/pdf/FRL_CAAFI_Jan_2010_V16.pdf (accessed on 13 June 2024).
- Sharipov, A.S.; Loukhovitski, B.I.; Starik, A.M. The Influence of Vibrations of Polyatomic Molecules on Dipole Moment and Static Dipole Polarizability: Theoretical Study. J. Phys. B At. Mol. Opt. Phys. 2017, 50, 165101. [Google Scholar] [CrossRef]
- Miller, T.M.; Bederson, B. Atomic and Molecular Polarizabilities—A Review of Recent Advances. Adv. At. Mol. Phys. 1978, 13, 1–55. [Google Scholar] [CrossRef]
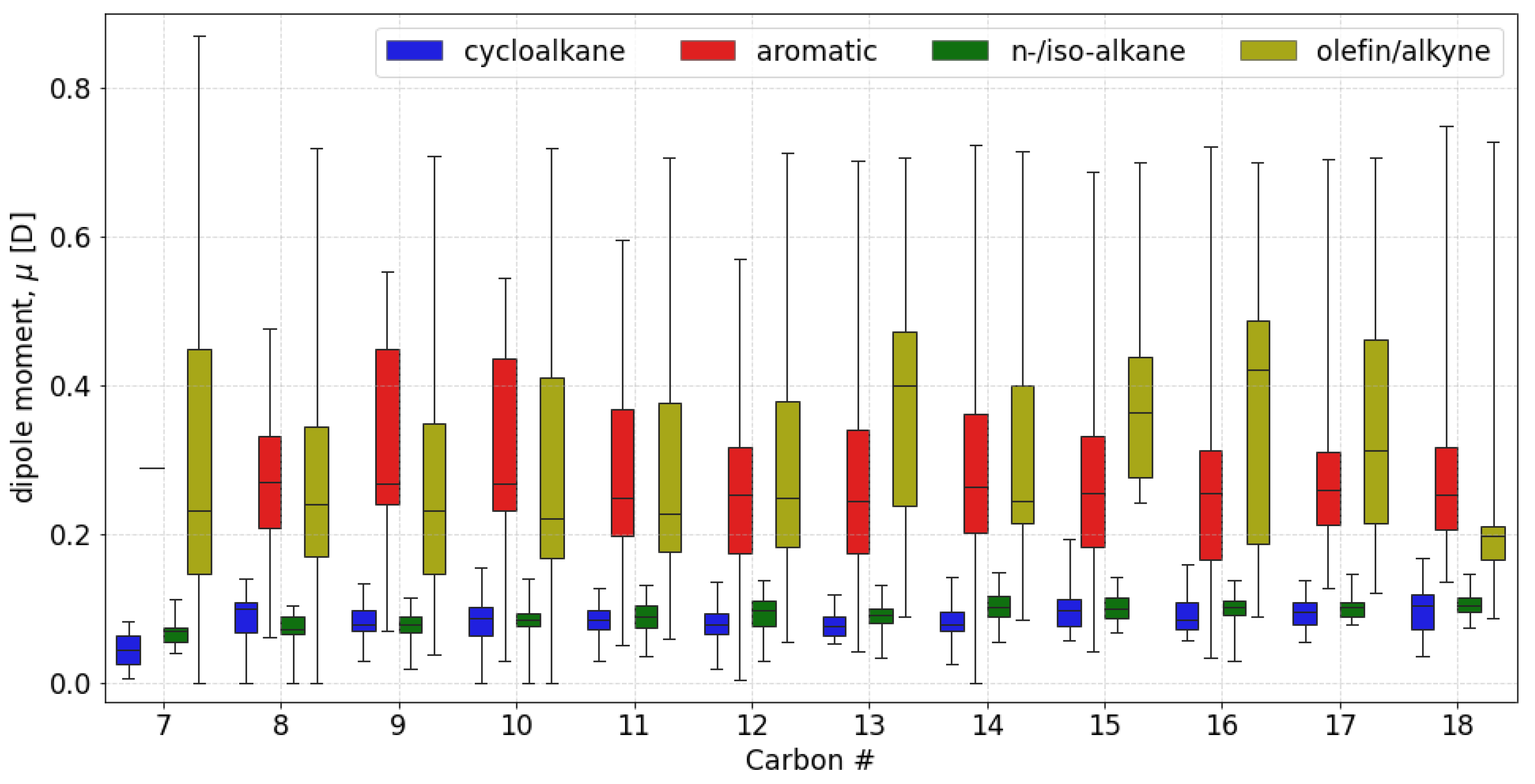
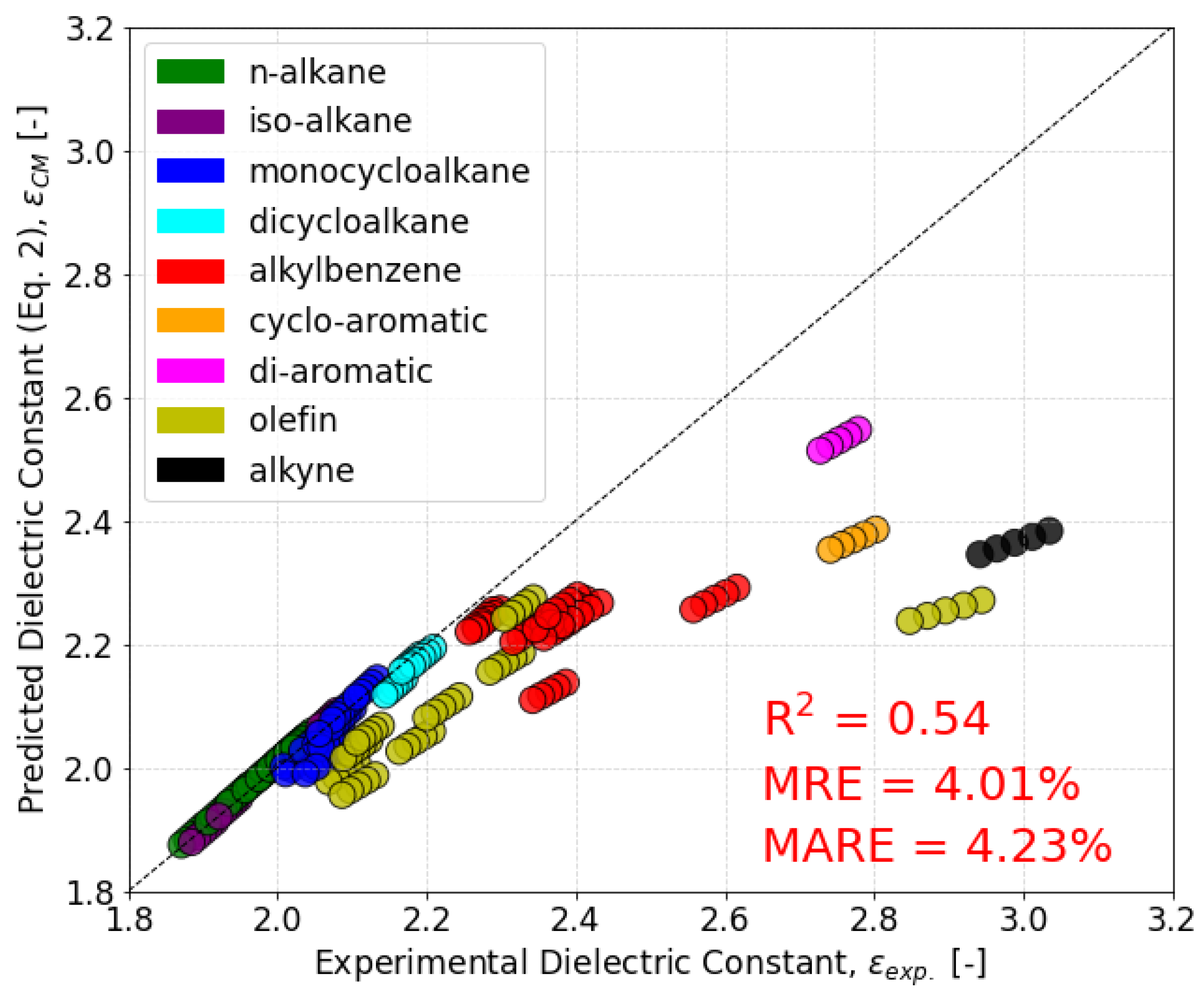
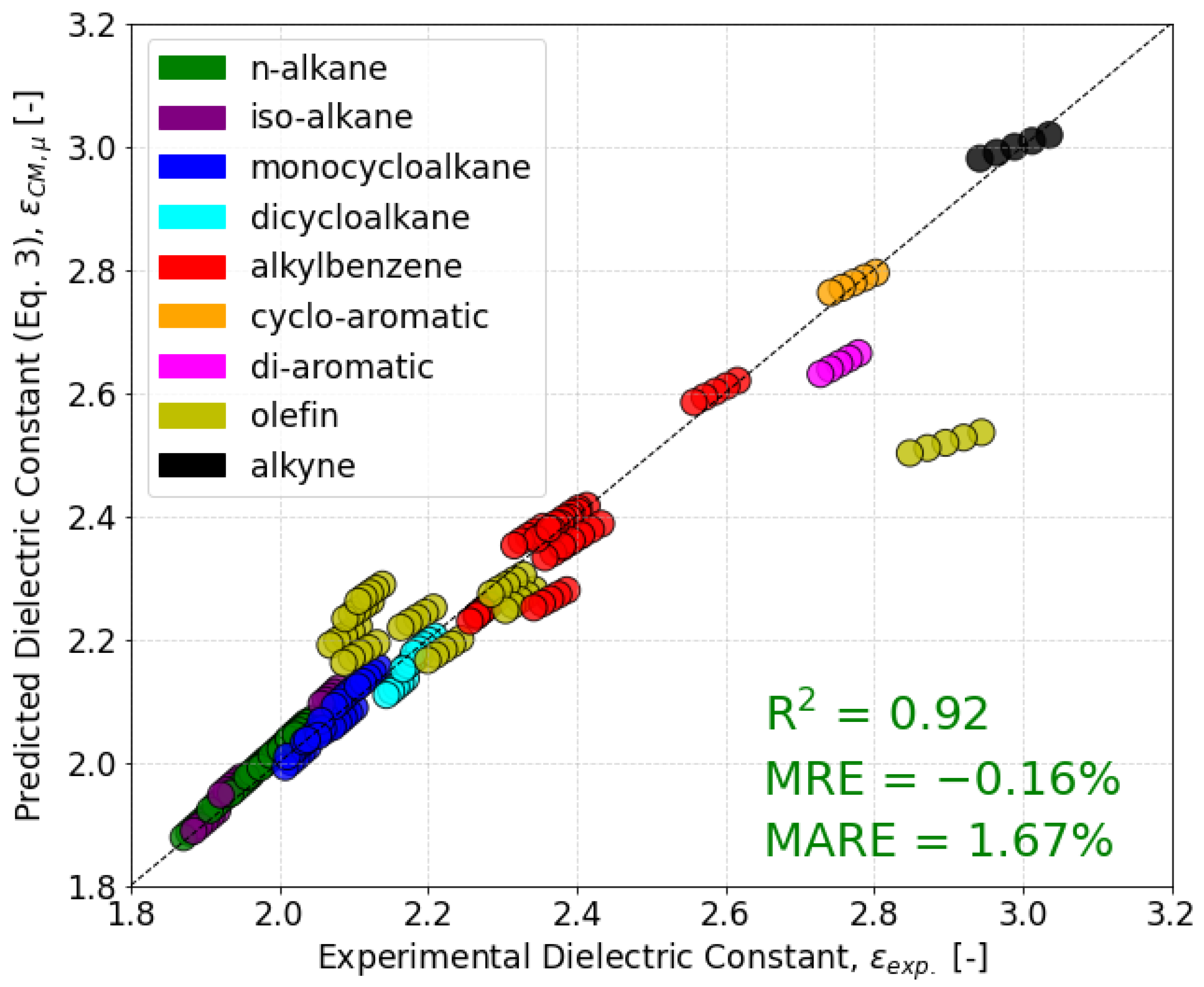


| Clausius–Mossotti (Figure 2) | Dipole-Corrected Model (Figure 3) | |||
|---|---|---|---|---|
| HC Class | MRE [%] | MARE [%] | MRE [%] | MARE [%] |
| N-alkane | −0.45 | 0.45 | −0.97 | 0.98 |
| Iso-alkane | −0.08 | 0.27 | −1.16 | 1.16 |
| Monocycloalkane | 0.73 | 0.85 | −0.02 | 0.43 |
| Dicyclocycloalkane | 0.78 | 0.78 | 0.82 | 0.82 |
| Alkene | 6.76 | 6.76 | −0.77 | 4.80 |
| Cyclo-aromatic | 14.53 | 14.53 | −0.24 | 0.35 |
| Alkyne | 20.89 | 20.89 | −0.36 | 0.63 |
| Di-aromatic | 8.11 | 8.11 | 3.86 | 3.86 |
| Alkylbenzene | 5.98 | 5.98 | 0.61 | 1.27 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Faulhaber, C.; Bell, D.C.; Boehm, R.; Heyne, J. Dielectric Constant Predictions for Jet-Range Hydrocarbons: Evaluating the Clausius–Mossotti Relation and Correcting for Molecular Dipole Moments. Energies 2024, 17, 4700. https://doi.org/10.3390/en17184700
Faulhaber C, Bell DC, Boehm R, Heyne J. Dielectric Constant Predictions for Jet-Range Hydrocarbons: Evaluating the Clausius–Mossotti Relation and Correcting for Molecular Dipole Moments. Energies. 2024; 17(18):4700. https://doi.org/10.3390/en17184700
Chicago/Turabian StyleFaulhaber, Conor, David C. Bell, Randall Boehm, and Joshua Heyne. 2024. "Dielectric Constant Predictions for Jet-Range Hydrocarbons: Evaluating the Clausius–Mossotti Relation and Correcting for Molecular Dipole Moments" Energies 17, no. 18: 4700. https://doi.org/10.3390/en17184700
APA StyleFaulhaber, C., Bell, D. C., Boehm, R., & Heyne, J. (2024). Dielectric Constant Predictions for Jet-Range Hydrocarbons: Evaluating the Clausius–Mossotti Relation and Correcting for Molecular Dipole Moments. Energies, 17(18), 4700. https://doi.org/10.3390/en17184700






