Experimental Investigation on Heat Transfer in Two-Phase Closed Thermosyphon Containing Non-Condensable Gas
Abstract
1. Introduction
2. Experimental Setup
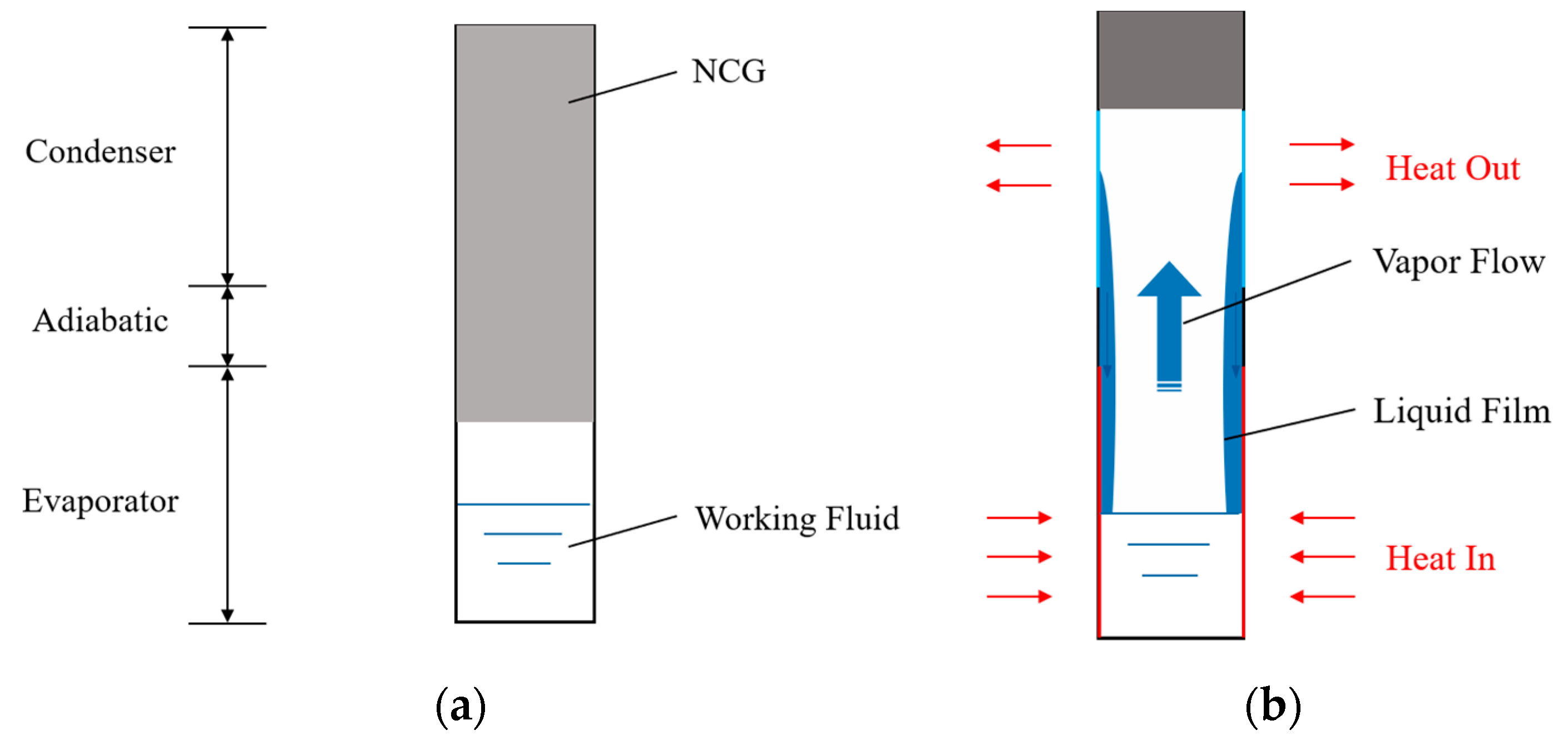
2.1. Working Principle
2.2. Experimental Equipment
2.3. Experimental Uncertainty Assessment
3. Experimental Method and Calculation
3.1. Experimental Condition
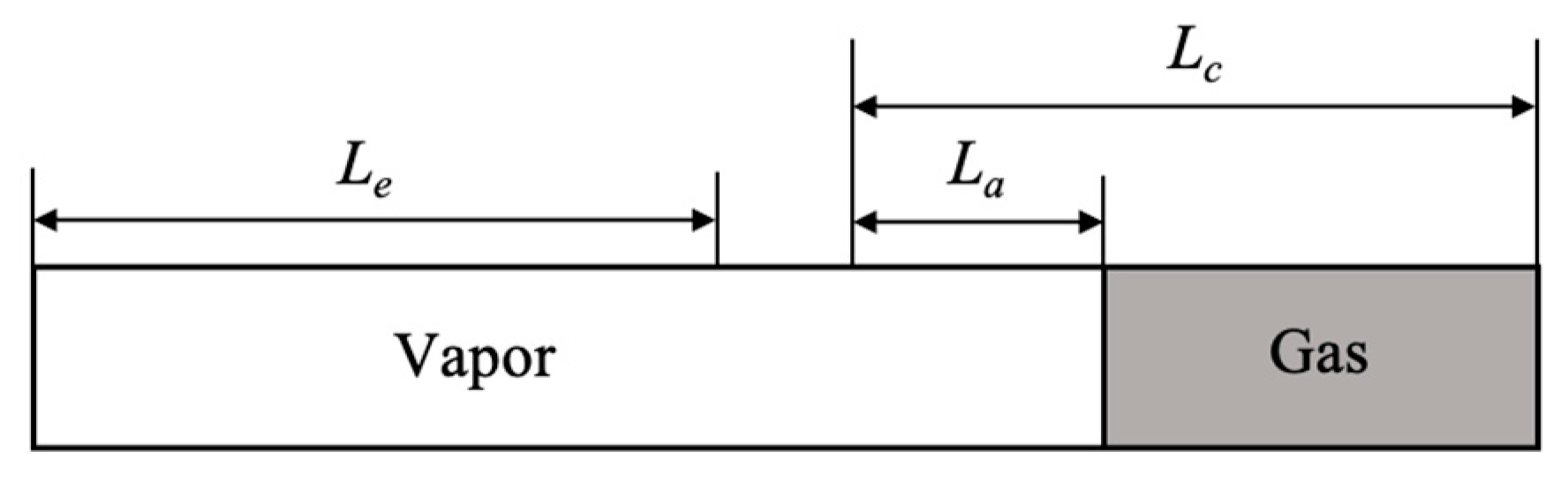
3.2. Heat Transfer Analysis
- The heat pipe operates in a steady state.
- A discrete interface is observed between the NCG and the vapor, which effectively demarcates the two phases.
- The NCG and vaporous substances are in accordance with the ideal gas law.
- The axial heat conduction along the tube wall is disregarded, and the total axial pressure is assumed to be equal.
4. Results and Discussions
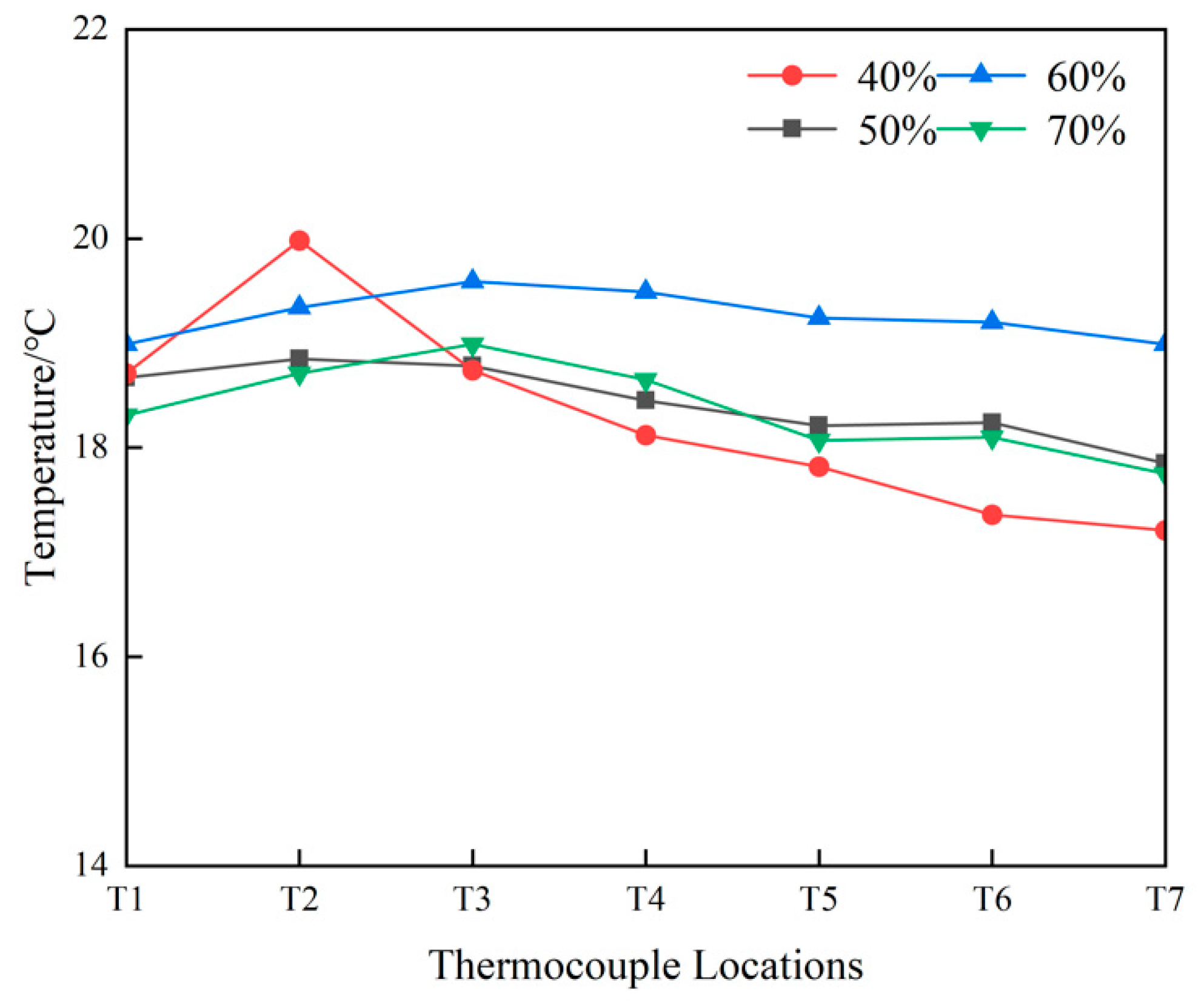
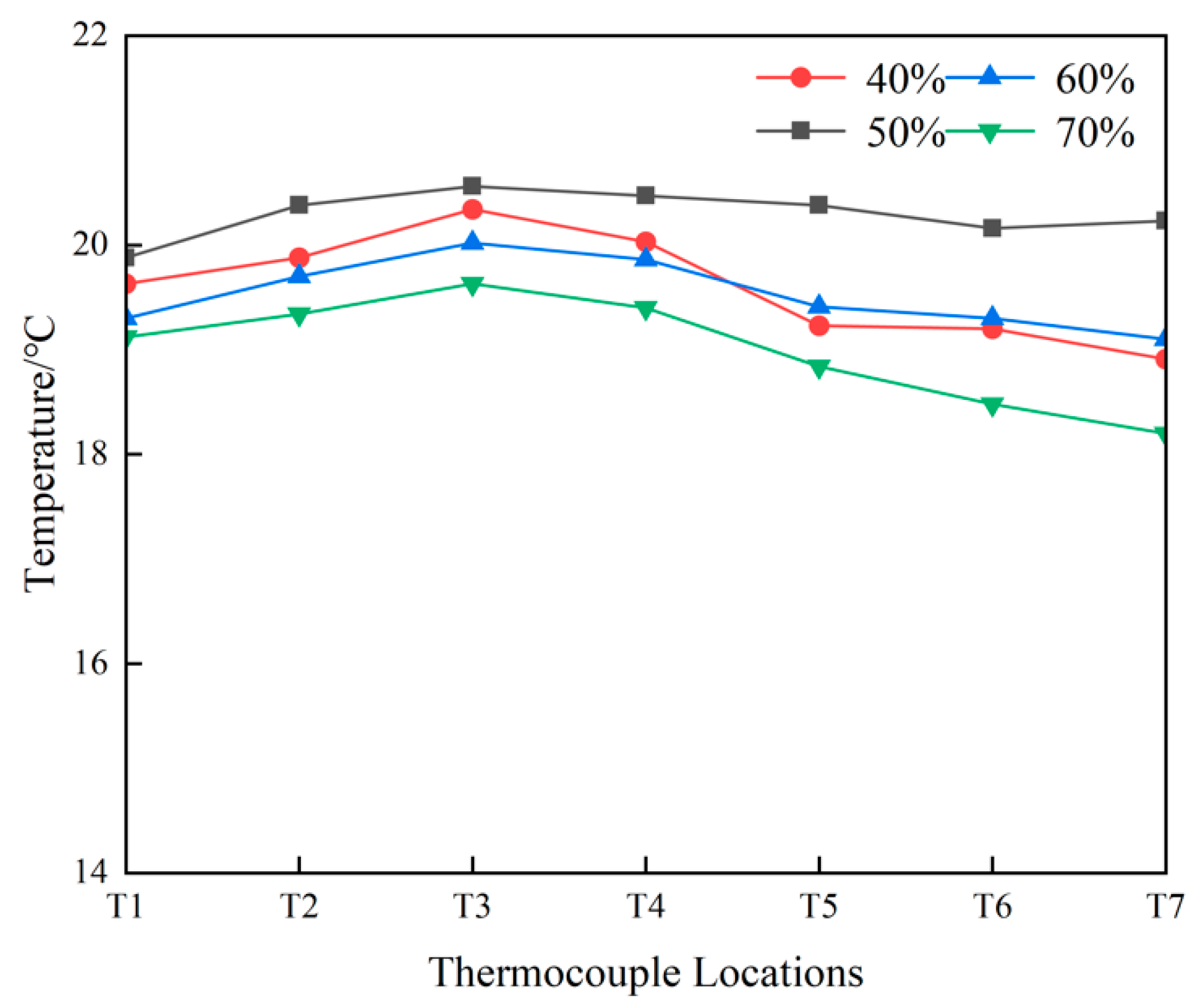
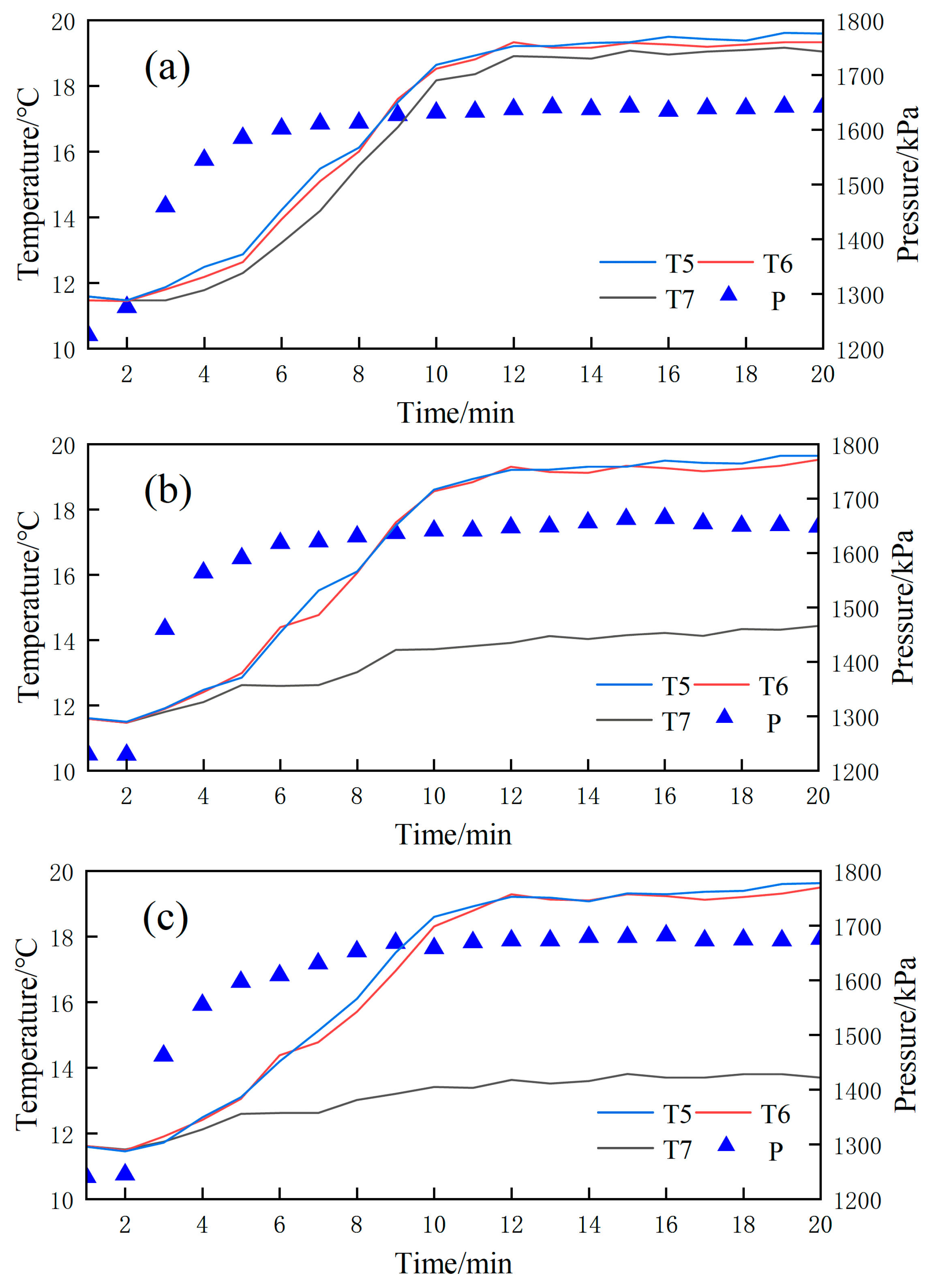
4.1. TPCT at Different Fluid-Filling Ratios
4.2. TPCT at Different NCG Mass
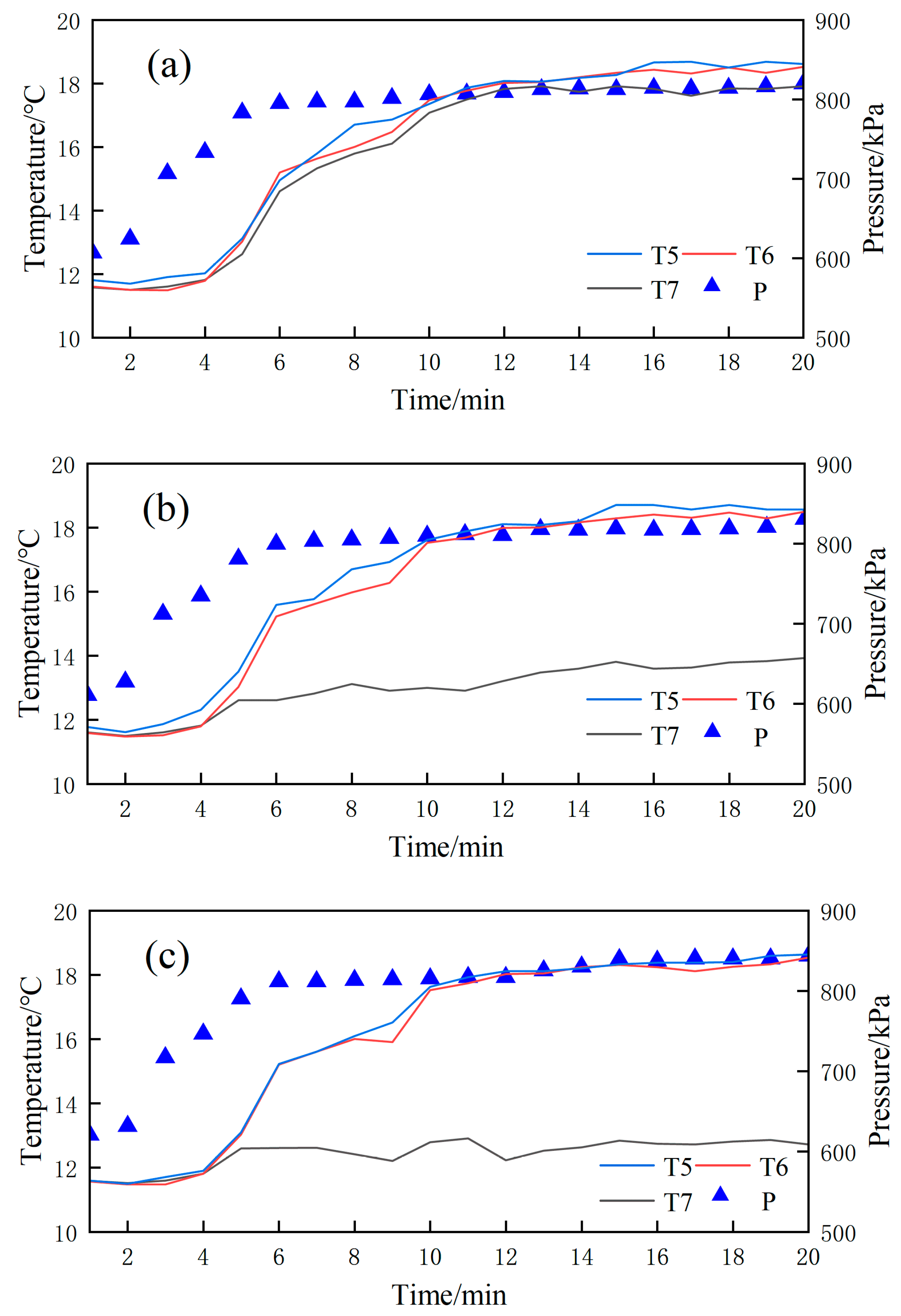
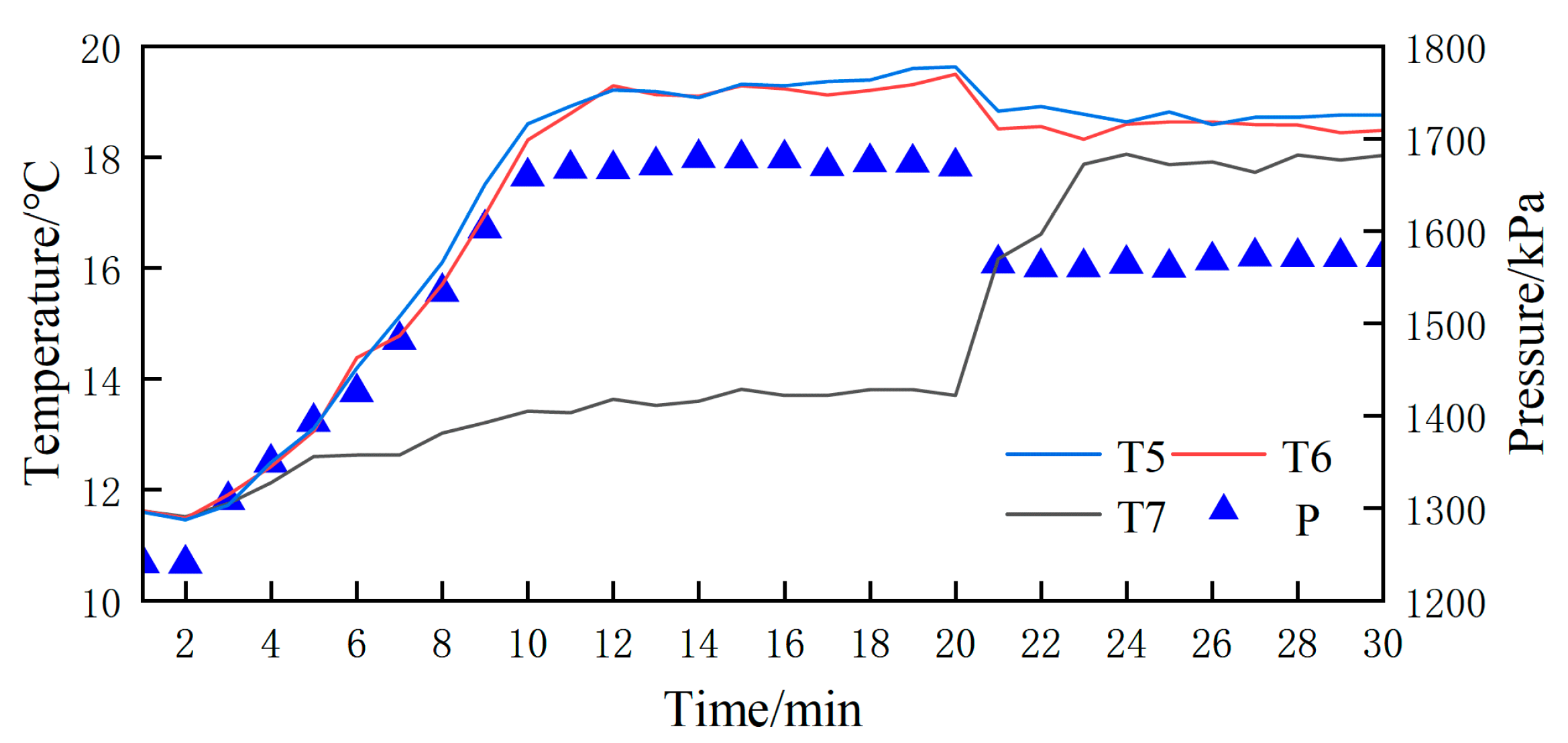
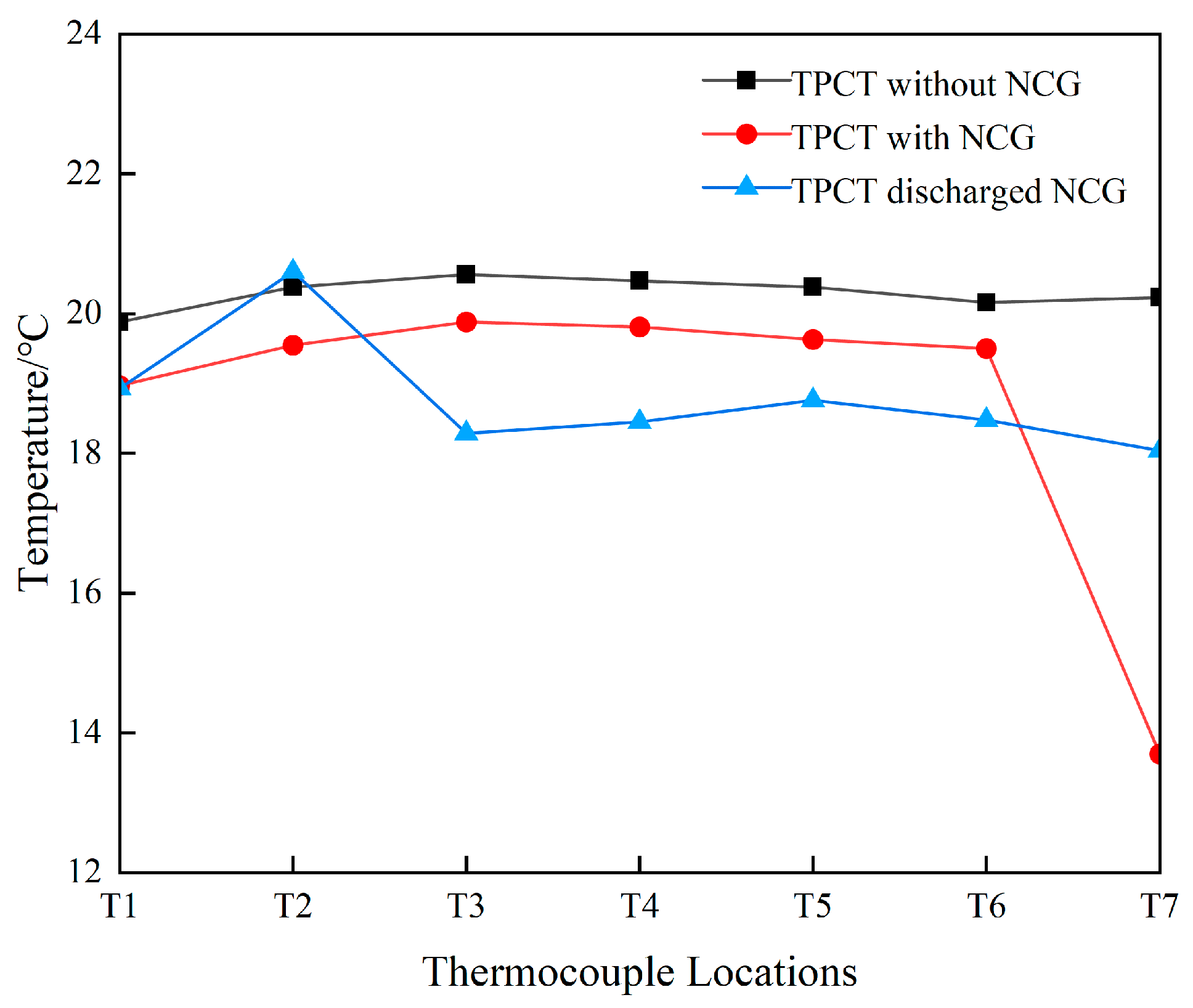
4.3. TPCT Discharged NCG by Check Valve
5. Conclusions
- (1)
- The R22 TPCT with a fluid-filling ratio of 60% and the R410a TPCT with a fluid-filling ratio of 50% demonstrate favorable isothermal and heat transfer characteristics. At this juncture, the heat flux within the TPCT increases, the rate of bubble generation is high, the rising resistance is reduced, and the heat transfer coefficient of the evaporator section of the TPCT is enhanced. The liquid film and the evaporator section are continuous, thereby preventing dry burning. In addition, compared with the R22 TPCT with a fluid-filling ratio of 60%, the mean temperature of the R410a TPCT with a fluid-filling ratio of 50% is 1 °C higher and the temperature variance is lower. This suggests that the thermal performance of the working fluid R410a is superior to that of the working fluid R22.
- (2)
- The existence of NCG has a negative effect on the condensation heat transfer process of vapor. The accumulation of NCG at the upper portion of the TPCT condenser section results in a reduction in the average temperature Tca and a deterioration in the isotherm of the TPCT. Furthermore, it was observed that as the mass of NCG increased, the effective length La of the condenser section and the effective heat transfer rate κ also decreased when the TPCT containing NCG operated stably. It was observed that an increase in the mass of NCG from 0.0097 to 0.0197 mol resulted in a reduction in the effective length of the condenser section and effective heat transfer rate of R22 TPCT, with a decrease of 75.1 mm and 15.02%, respectively. Moreover, at the identical NCG mass, the effective heat transfer rate of R410a TPCT is demonstrably superior to that of R22 TPCT.
- (3)
- It is possible to discharge the NCG in TPCT by means of a check valve. Furthermore, the condenser section exhibits favorable isothermal properties after NCG is removed. Nevertheless, it should be noted that this process will result in the discharge of vapor with NCG, which will lead to a reduction in the fluid-filling ratio of TPCT. After the NCG is exhausted, a sudden change in temperature is observed within the evaporator section of the TPCT. It indicates that the current working liquid in TPCT is insufficient. The temperature of the R410a TPCT containing 0.0197 mol NCG with a fluid-filling ratio of 50% is comparable to that observed in a fluid-filling ratio of 40% after the NCG is exhausted.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Faghri, A. Heat pipes: Review, opportunities and challenges. Front. Heat Pipes 2014, 5. [Google Scholar] [CrossRef]
- Jafari, D.; Franco, A.; Filippeschi, S.; Di Marco, P. Two-phase closed thermosyphons: A review of studies and solar applications. Renew. Sustain. Energy Rev. 2016, 53, 575–593. [Google Scholar] [CrossRef]
- Sabry, M.; Lashin, A. Performance of a heat-pipe cooled concentrated photovoltaic/thermoelectric hybrid system. Energies 2023, 16, 1438. [Google Scholar] [CrossRef]
- Wang, G.; Bi, J.; Fan, Y.; Zhu, L.; Zhang, F.; Feng, D. Settlement characteristic of warm permafrost embankment with two-phase closed thermosyphons in Daxing’anling Mountains region. Sustainability 2022, 14, 12272. [Google Scholar] [CrossRef]
- Faghri, A. Heat Pipe Science and Technology; Global Digital Press: Kanpur, India, 1995. [Google Scholar]
- Czerwiński, G.; Wołoszyn, J. Numerical Study of a Cooling System Using Phase Change of a Refrigerant in a Thermosyphon. Energies 2021, 14, 3634. [Google Scholar] [CrossRef]
- Faraji, H.; Teggar, M.; Arshad, A.; Arıcı, M.; Berra, E.M.; Choukairy, K. Lattice Boltzmann simulation of natural convection heat transfer phenomenon for thermal management of multiple electronic components. Therm. Sci. Eng. Prog. 2023, 45, 102126. [Google Scholar] [CrossRef]
- Faraji, H.; Faraji, M.; El Alami, M.; Hariti, Y.; Arshad, A.; Hader, A.; Benkaddour, A. Cooling of recent microprocessors by the fusion of nano-enhanced phase change materials. Mater. Today Proc. 2020, 30, 865–869. [Google Scholar] [CrossRef]
- Chandran, M.; Elavarasan, R.M.; Neelakandan, R.B.; Subramaniam, U.; Pugazhendhi, R. Influence of Geometrical Changes in an Adiabatic Portion on the Heat Transfer Performance of a Two-Phase Closed Thermosiphon System. Energies 2021, 14, 3070. [Google Scholar] [CrossRef]
- Wu, Y.; Zhang, Z.; Li, W.; Xu, D. Effect of the inclination angle on the steady-state heat transfer performance of a thermosyphon. Appl. Sci. 2019, 9, 3324. [Google Scholar] [CrossRef]
- Kim, Y.; Shin, D.H.; Kim, J.S.; You, S.M.; Lee, J. Boiling and condensation heat transfer of inclined two-phase closed thermosyphon with various filling ratios. Appl. Therm. Eng. 2018, 145, 328–342. [Google Scholar] [CrossRef]
- Noie, S.H. Heat transfer characteristics of a two-phase closed thermosyphon. Appl. Therm. Eng. 2005, 25, 495–506. [Google Scholar] [CrossRef]
- Fadhl, B.; Wrobel, L.C.; Jouhara, H. CFD modelling of a two-phase closed thermosyphon charged with R134a and R404a. Appl. Therm. Eng. 2015, 78, 482–490. [Google Scholar] [CrossRef]
- Jiao, B.; Qiu, L.; Zhang, X.; Zhang, Y. Investigation on the effect of filling ratio on the steady-state heat transfer performance of a vertical two-phase closed thermosyphon. Appl. Therm. Eng. 2008, 28, 1417–1426. [Google Scholar] [CrossRef]
- Naresh, Y.; Balaji, C. Thermal performance of an internally finned two phase closed thermosyphon with refrigerant R134a: A combined experimental and numerical study. Int. J. Therm. Sci. 2018, 126, 281–293. [Google Scholar] [CrossRef]
- Yuan, J.; Wang, C.; Zhang, J.; Ling, J.; Zhou, Z.; Gao, F. Effect of non-condensable gas on thermal characteristics in two-phase closed thermosyphon. Appl. Therm. Eng. 2020, 173, 115233. [Google Scholar] [CrossRef]
- Gedik, E. Experimental investigation of the thermal performance of a two-phase closed thermosyphon at different operating conditions. Energy Build. 2016, 127, 1096–1107. [Google Scholar] [CrossRef]
- Lataoui, Z.; Jemni, A. Experimental investigation of a stainless steel two-phase closed thermosyphon. Appl. Therm. Eng. 2017, 121, 721–727. [Google Scholar] [CrossRef]
- Jafari, D.; Di Marco, P.; Filippeschi, S.; Franco, A. An experimental investigation on the evaporation and condensation heat transfer of two-phase closed thermosyphons. Exp. Therm. Fluid Sci. 2017, 88, 111–123. [Google Scholar] [CrossRef]
- Lin, T.; Quan, X.; Cheng, P. Experimental investigation of superlong two-phase closed thermosyphons for geothermal utilization. Int. J. Therm. Sci. 2022, 171, 107199. [Google Scholar] [CrossRef]
- Ozbas, E.; Selimli, S.; Ozkaymak, M.; Frej, A.S.S. Evaluation of internal structure modifications effect of two-phase closed thermosyphon on performance: An experimental study. Sol. Energy 2021, 224, 1326–1332. [Google Scholar] [CrossRef]
- Alammar, A.A.; Al-Mousawi, F.N.; Al-Dadah, R.K.; Mahmoud, S.M.; Hood, R. Enhancing thermal performance of a two-phase closed thermosyphon with an internal surface roughness. J. Clean. Prod. 2018, 185, 128–136. [Google Scholar] [CrossRef]
- Huang, J.; Zhang, J.; Wang, L. Review of vapor condensation heat and mass transfer in the presence of non-condensable gas. Appl. Therm. Eng. 2015, 89, 469–484. [Google Scholar] [CrossRef]
- Kumar, G.V.; Cammiade, L.M.F.; Kelm, S.; Prakash, K.A.; Groß, E.M.; Allelein, H.J.; Kneer, R.; Rohlfs, W. Implementation of a CFD model for wall condensation in the presence of non-condensable gas mixtures. Appl. Therm. Eng. 2021, 187, 116546. [Google Scholar] [CrossRef]
- Zhang, W.; Wang, S.; Lianbo, M. Analytical modeling for vapor condensation in the presence of noncondensable gas and experimental validation. J. Heat Transf. 2021, 143, 011601. [Google Scholar] [CrossRef]
- Bérut, E.; Lips, S.; Lefèvre, F.; Sartre, V. Effect of non-condensable gas on heat transfer within the hollow fin condenser of metallic and polymer vapor chambers. Appl. Therm. Eng. 2021, 190, 116777. [Google Scholar] [CrossRef]
- He, J.; Lin, G.; Bai, L.; Miao, J.; Zhang, H. Effect of non-condensable gas on the operation of a loop heat pipe. Int. J. Heat Mass Transf. 2014, 70, 449–462. [Google Scholar] [CrossRef]
- Guo, Y.; Liu, C.; Pan, H.; Lyu, W.; Zhang, H.; Lin, G.; Miao, J. Experimental investigation and multiparameter analysis of variable conductance heat pipes. Appl. Therm. Eng. 2022, 202, 117589. [Google Scholar] [CrossRef]
- Lemmon, E.W.; Huber, M.L.; McLinden, M.O. NIST reference fluid thermodynamic and transport properties–REFPROP. NIST Stand. Ref. Database 2002, 23, v7. [Google Scholar]
- Marcus, B.D. Theory and Design of Variable Conductance Heat Pipes; NASA: Washington, DC, USA, 1972. [Google Scholar]
- Huang, C.-N.; Lee, K.-L.; Tarau, C.; Kamotani, Y.; Kharangate, C.R. Computational fluid dynamics model for a variable conductance thermosyphon. Case Stud. Therm. Eng. 2021, 25, 100960. [Google Scholar] [CrossRef]
- Shi, C.; Wang, Y.; Liao, Q.; Yang, Y. Analysis and application of variable conductance heat pipe air preheater. J. Therm. Sci. 2011, 20, 248–253. [Google Scholar] [CrossRef]
- Lee, K.-L.; Kadambi, J.R.; Kamotani, Y. The influence of non-condensable gas on an integral planar heat pipe radiators for space applications. Int. J. Heat Mass Transf. 2017, 110, 496–505. [Google Scholar] [CrossRef]
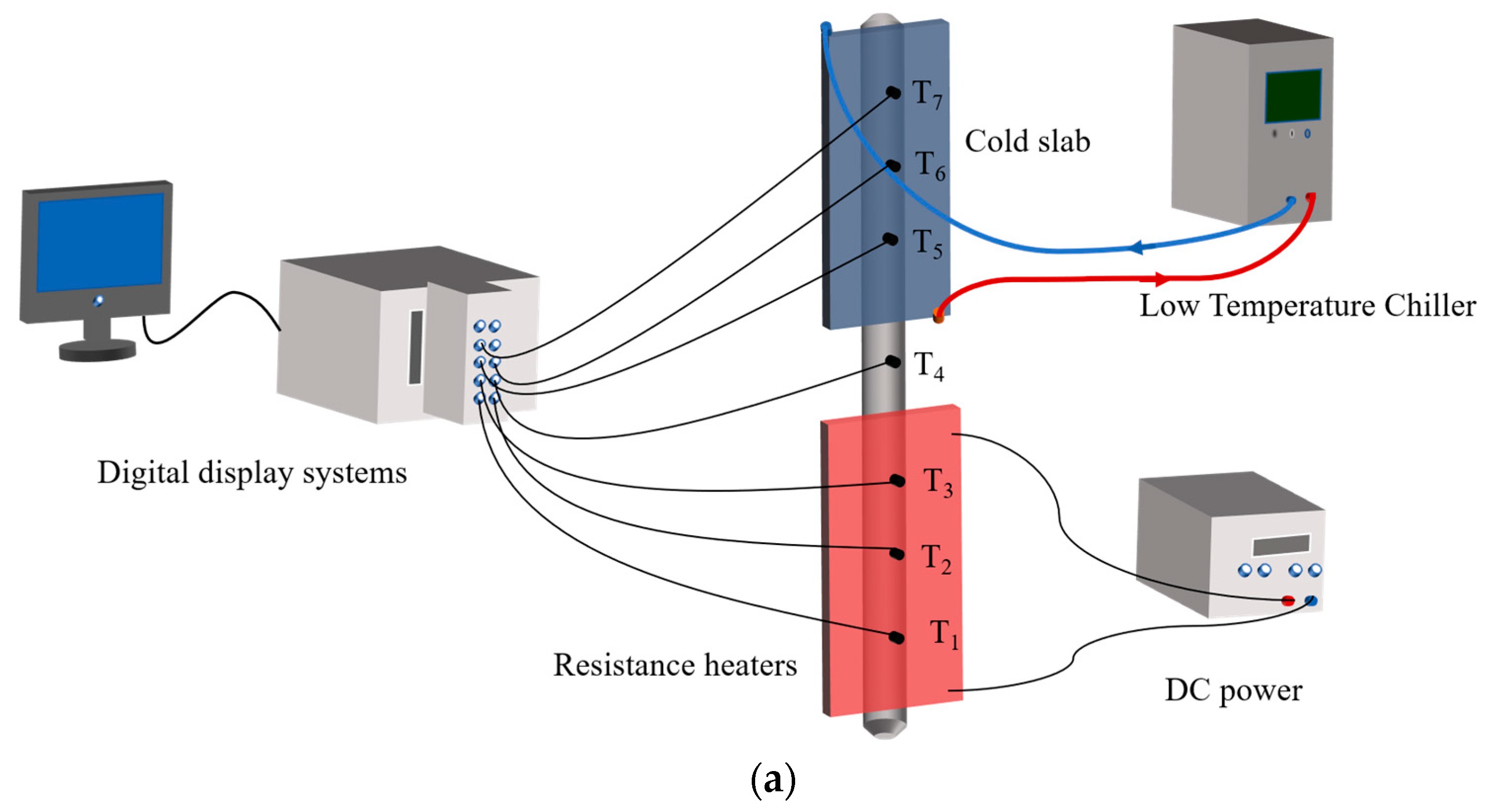
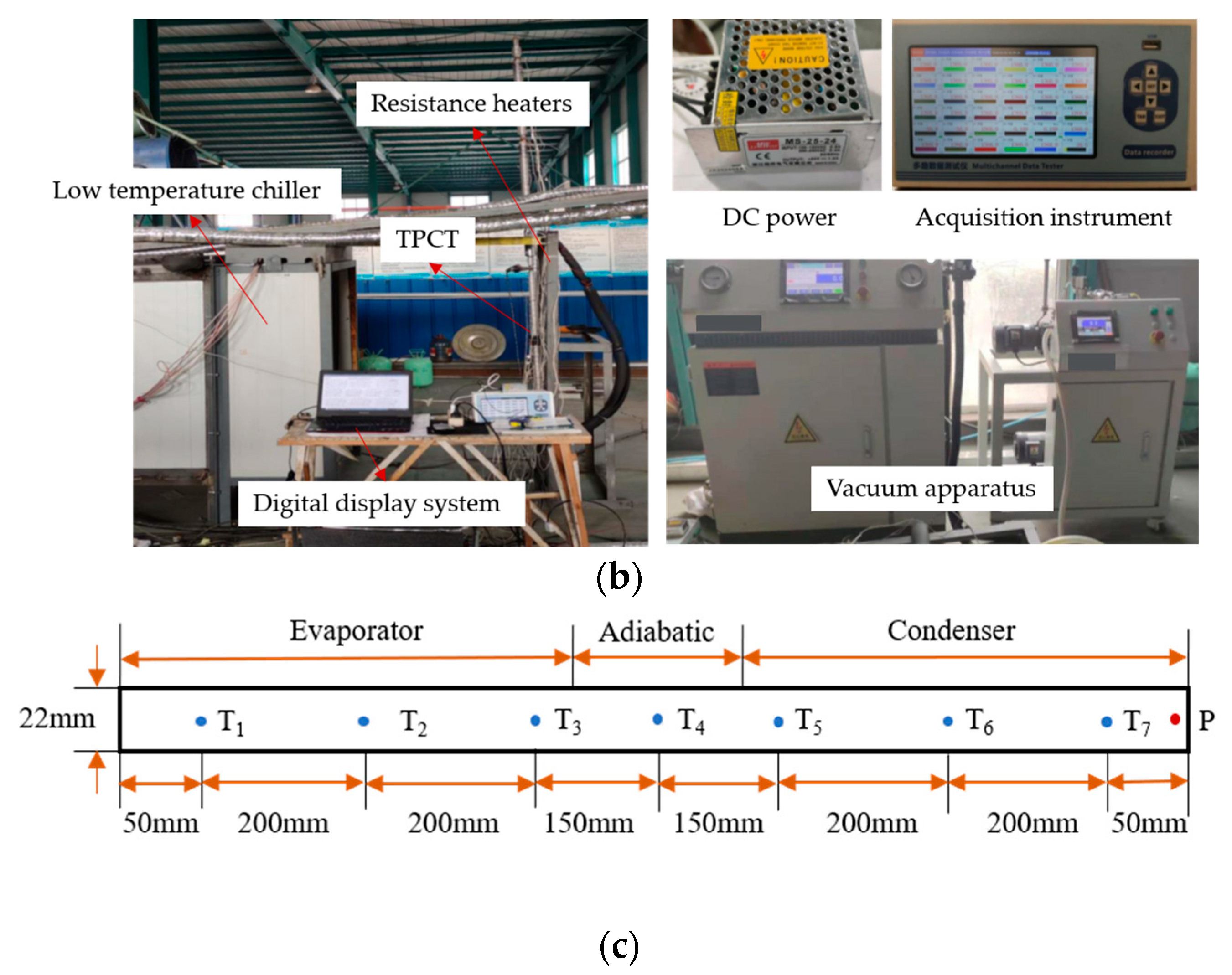
| Experimental Equipment | Range | Precision |
|---|---|---|
| Low-temperature chiller | 5~35 °C | ±0.1 °C |
| DC power | 0~36 V, 0~10 A | ±0.5% |
| Vacuum apparatus | 0.001~101 kPa | ±1% |
| Pressure sensor | 0~4 MPa | ±0.2% |
| Thermocouple | −200~350 °C | ±0.1 °C |
| Properties | R22 | R410a |
|---|---|---|
| Molecular weight (g/mol) | 86.47 | 72.59 |
| Boiling point at 1 atm (°C) | −40.81 | −51.53 |
| Critical temperature (°C) | 96.16 | 70.46 |
| Critical pressure (kPa) | 4990 | 4770 |
| Latent heat of vaporization at boiling point (kJ/kg) | 191.3 | 204.7 |
| Heat capacity of liquid (kJ/kg K) | 1.22 | 1.62 |
| Heat capacity of vapor at 1 atm (kJ/kg K) | 0.82 | 1.32 |
| Thermal conductivity of liquid at 16 °C (W/mK) | 0.0875 | 0.0942 |
| Thermal conductivity of vapor at 1 atm (W/mK) | 0.0106 | 0.0142 |
| Density of liquid at 16 °C (kg/m3) | 1224.89 | 1101.72 |
| Density of vapor at 1 atm (kg/m3) | 34.36 | 49.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, B.; Meng, G.; Huang, W.; Wang, A.; Cheng, X.; Yang, J. Experimental Investigation on Heat Transfer in Two-Phase Closed Thermosyphon Containing Non-Condensable Gas. Energies 2024, 17, 4693. https://doi.org/10.3390/en17184693
Song B, Meng G, Huang W, Wang A, Cheng X, Yang J. Experimental Investigation on Heat Transfer in Two-Phase Closed Thermosyphon Containing Non-Condensable Gas. Energies. 2024; 17(18):4693. https://doi.org/10.3390/en17184693
Chicago/Turabian StyleSong, Binglin, Guoying Meng, Wei Huang, Aiming Wang, Xiaohan Cheng, and Jie Yang. 2024. "Experimental Investigation on Heat Transfer in Two-Phase Closed Thermosyphon Containing Non-Condensable Gas" Energies 17, no. 18: 4693. https://doi.org/10.3390/en17184693
APA StyleSong, B., Meng, G., Huang, W., Wang, A., Cheng, X., & Yang, J. (2024). Experimental Investigation on Heat Transfer in Two-Phase Closed Thermosyphon Containing Non-Condensable Gas. Energies, 17(18), 4693. https://doi.org/10.3390/en17184693






