Influence of Volute Casing Design Methods and Changes in Geometric Parameters on Pump Operation
Abstract
1. Introduction
2. Research Methodology
2.1. Object of Research
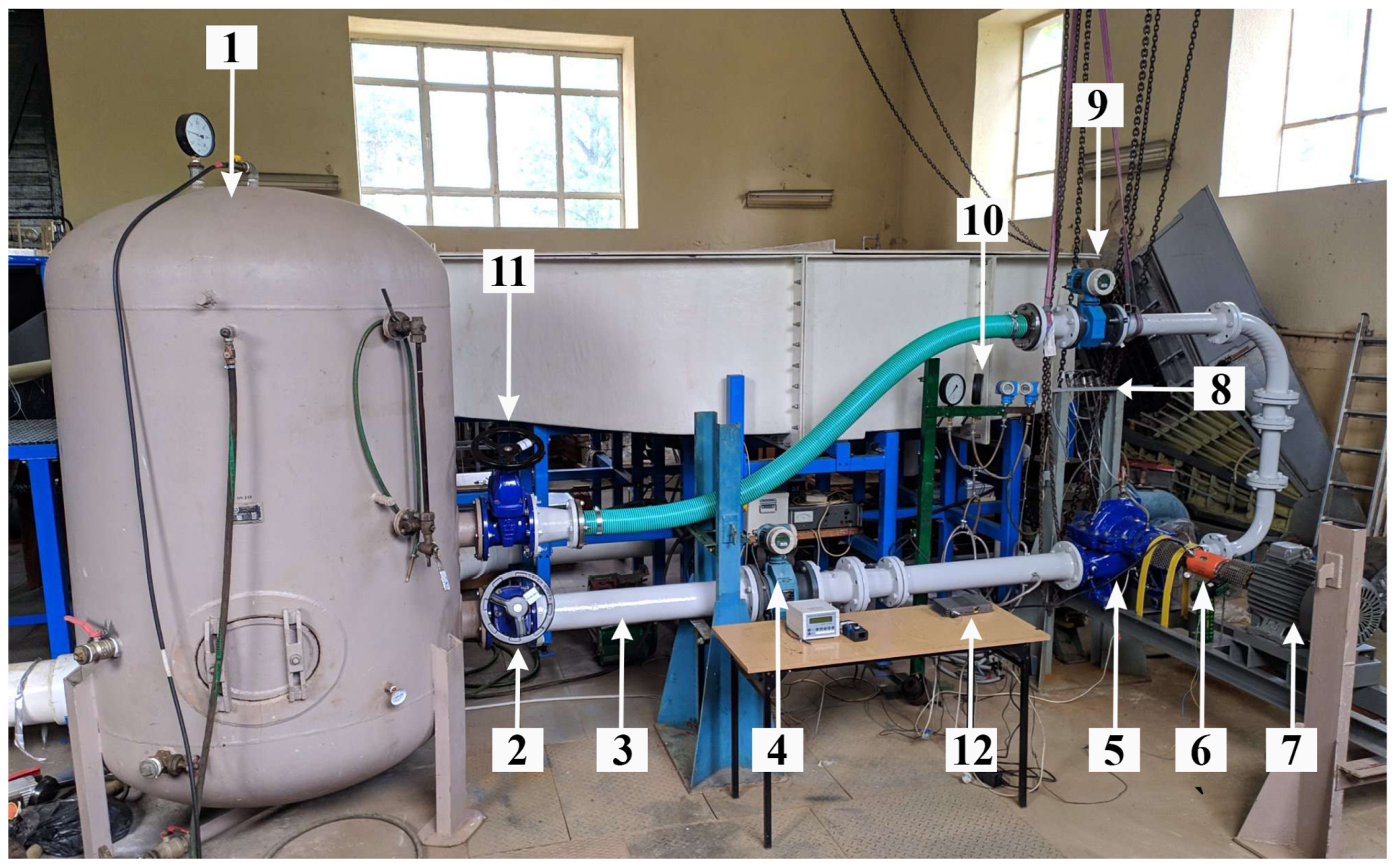
2.2. Experimental Research
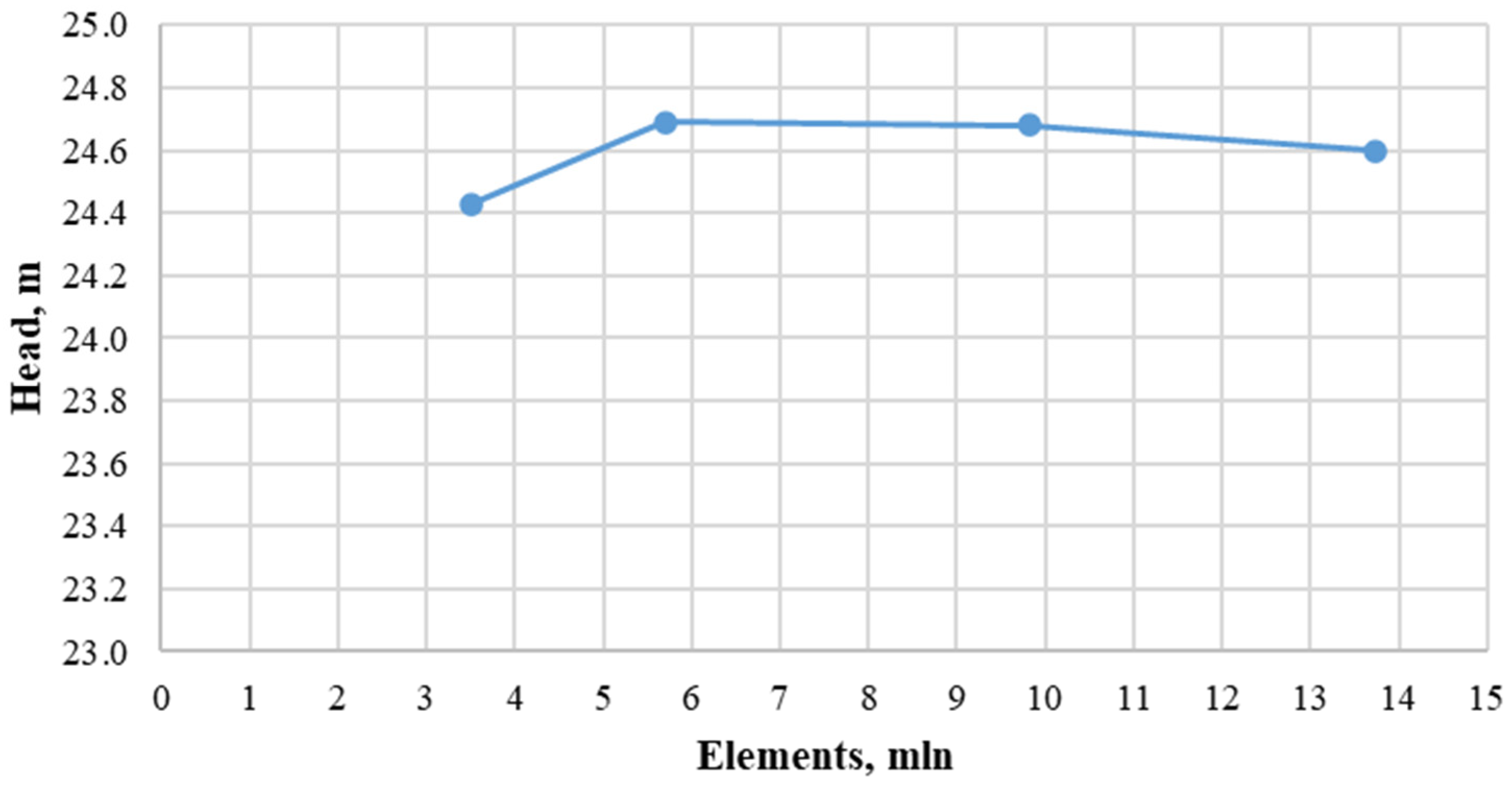
2.3. Numerical Studies
3. Research Results and Their Analysis
3.1. Influence of the Volute Casing Design Method
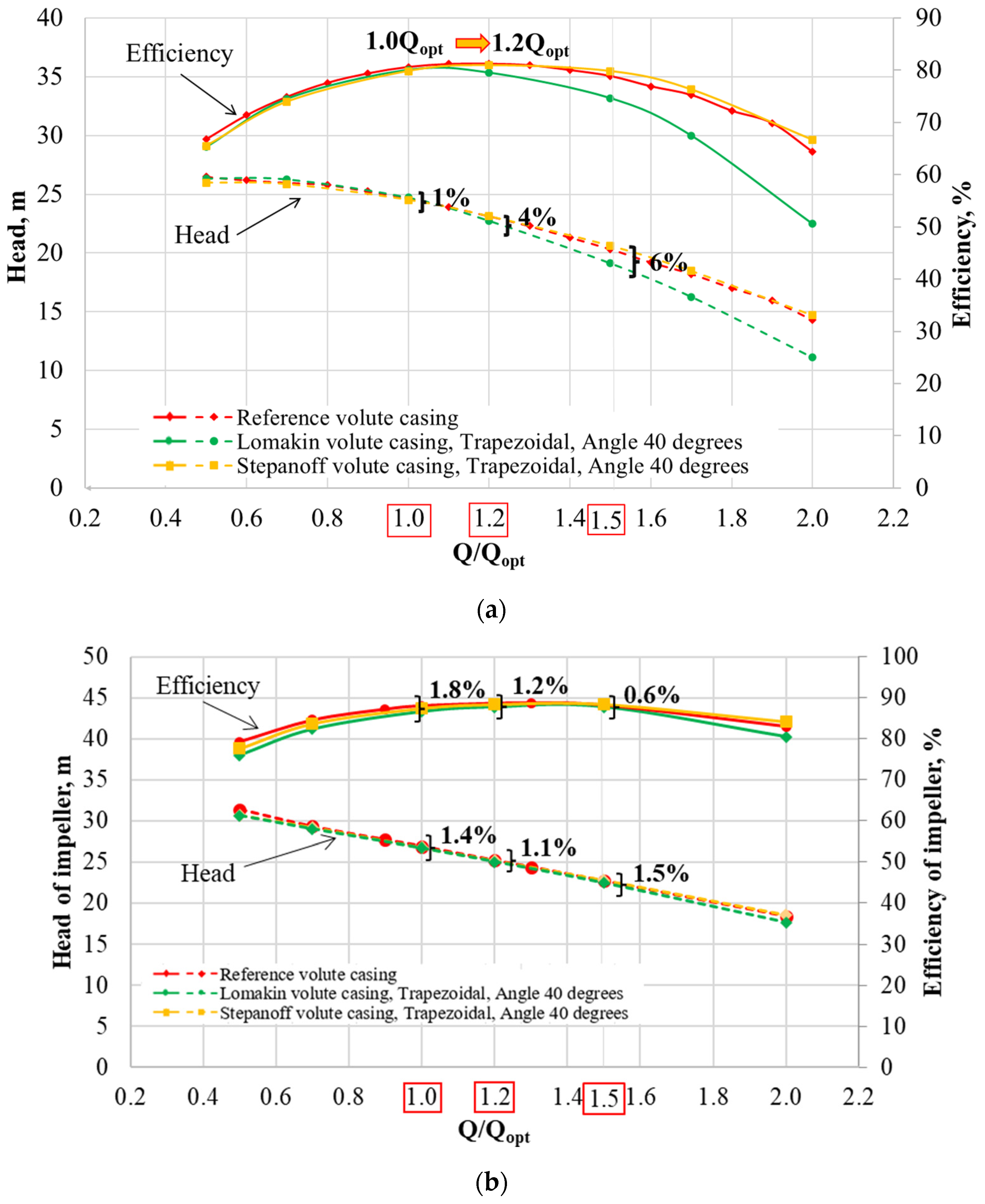
3.1.1. Impact on Pump and Impeller Efficiency
3.1.2. Influence on the Pump and Impeller Head
3.1.3. Cross-Sectional Area of the Volute Casing
3.1.4. Volute Casing and Impeller Losses
3.1.5. Summary of the Influence of the Volute Casing Design Method
3.2. Influence of the Geometric Parameters of the Volute Casing
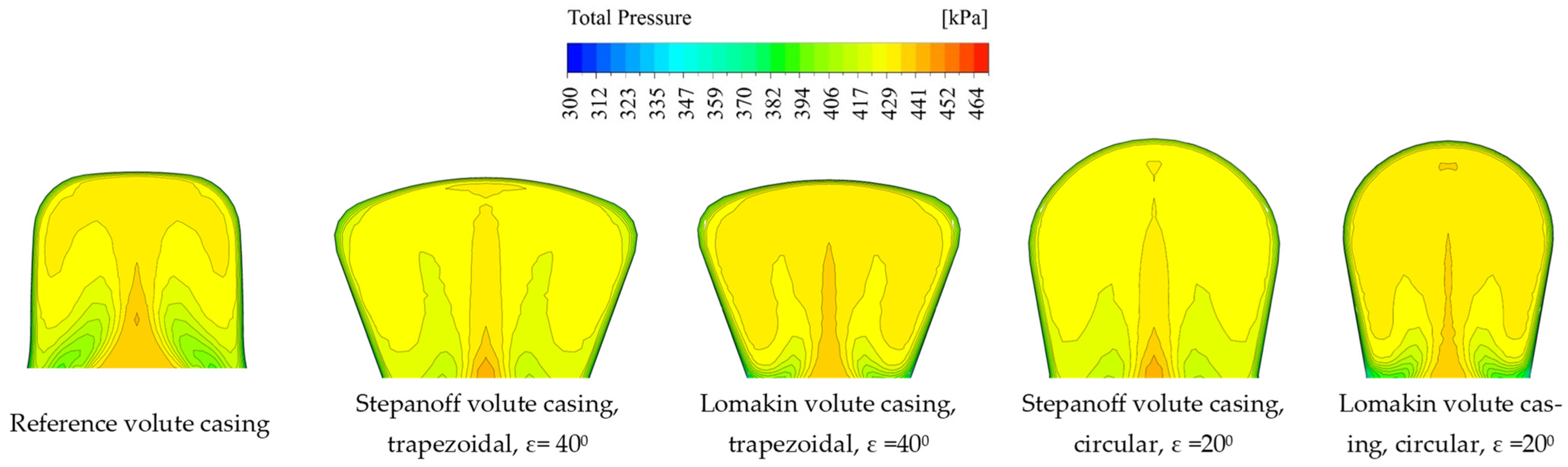
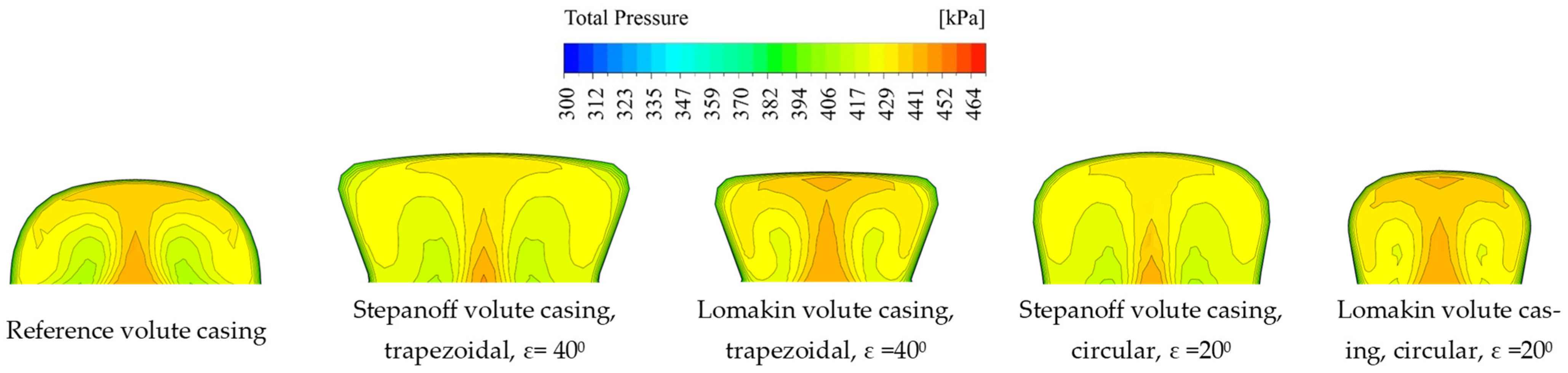
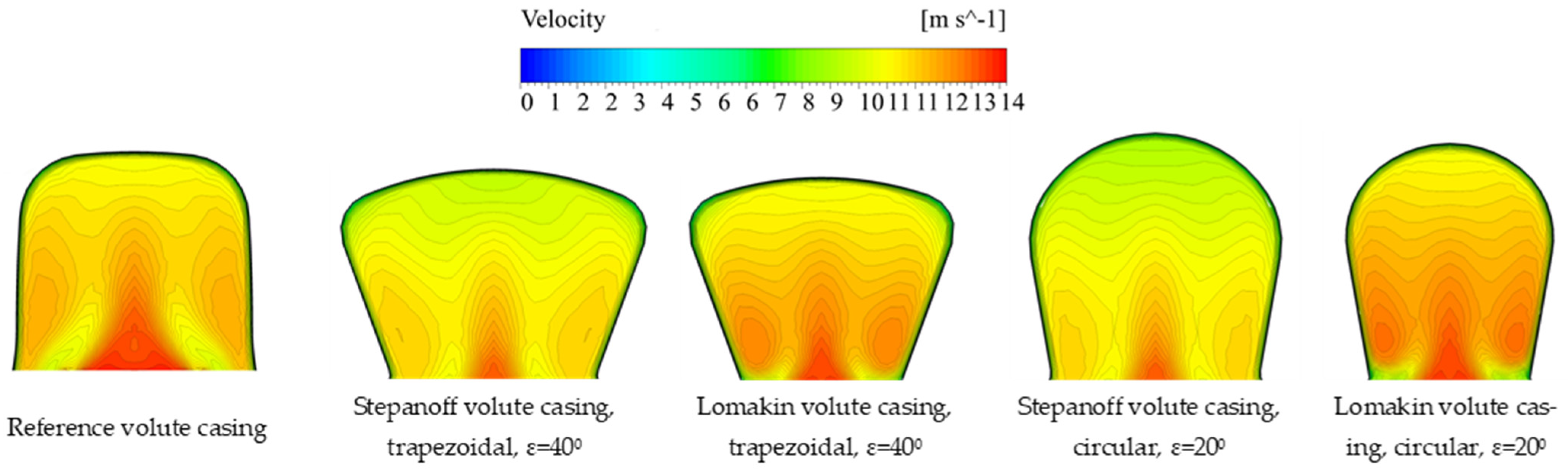
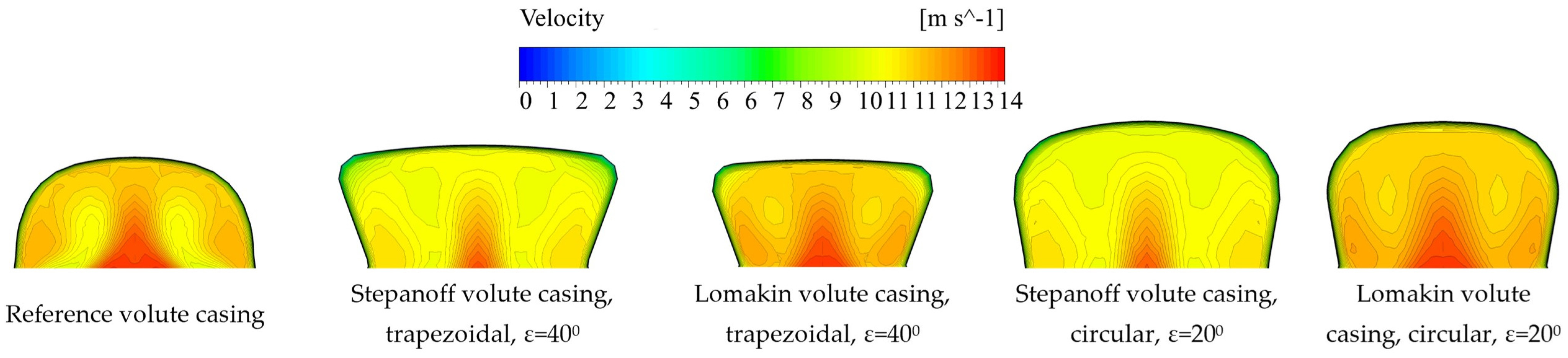
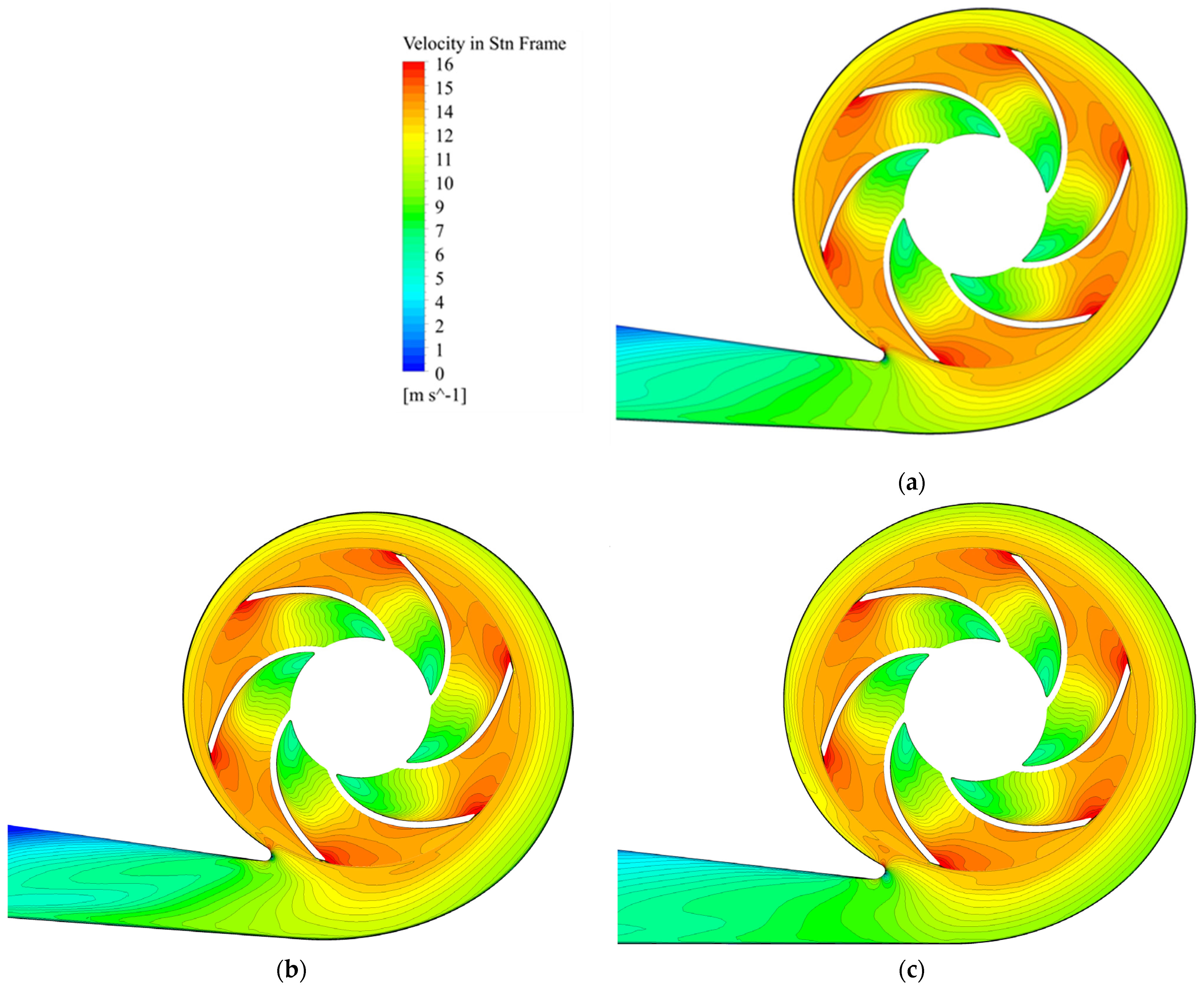
3.3. Flow Structure
4. Conclusions
- The constant velocity method (Stepanoff volute casing) gives a flatter head–capacity curve shape of the pump characteristics than the method of conservation of angular momentum of the flow (Lomakin volute casing). The pump with the Lomakin volute casing has a steep Q-H curve and efficiency curve.
- The pump efficiency difference between volute casings is less than 1% at 1.0Qopt. The BEP for the pump with Lomakin volute casings is at the design point. In the case of Stepanoff volute casings, the BEP is shifted towards a higher flow rate (1.2Qopt). There is also a larger pump operating range with the highest efficiency (from 1.0Qopt to 1.5Qopt) than in the case with Lomakin volute casings (from 1.0Qopt to 1.2Qopt).
- The design method of the volute casing has the greatest impact on hydraulic losses. The difference in losses between the Stepanoff and Lomakin volute casings at 1.0Qopt is 15%. The smaller cross-sectional area of the Lomakin volute casings causes smaller hydraulic losses at 1.0Qopt than the Stepanoff volute casings, while from 1.2Qopt, the losses are greater. In the case of Stepanoff volute casings, the reference point of least losses (1.63 m) is shifted to the right about the optimal flow rate.
- 4.
- Design methods have no significant effect on the head in the recommended operation range. The difference between the pumps with various volute casings is less than 1% at 1.0Qopt. The difference increases with the increase in flow rate and reaches 6% at point 1.5Qopt (Table 3).
- 5.
- Changing the geometric parameters such as the cross-sectional shape and wall opening angle did not have a significant impact on the pump’s operating parameters.
- 6.
- The fluid flow presented in the longitudinal cross-section does not show significant differences in the flow structure for volute casings. Small differences are observed in the area of the tongue and in the diffuser. They depend on the different heights of the cross-sections of the volute.
- 7.
- For the researched double-entry centrifugal pump, the volute casing designed according to the method of constant velocity works most efficiently. A pump with such a volute casing has a wider range of work with high efficiency, which is needed in the process of operation.
- 8.
- The investigated change in the volute casing parameters has practically no effect on the impeller for the investigated pump.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gülich, J.F. Centrifugal Pumps; Springer: Berlin/Heidelberg, Germany, 2014; ISBN 978-3-642-40113-8. [Google Scholar]
- Lomakin, A. Tsentrobezhnyye i Osevyye Nasosy; Mashinostroyeniye: Saint Petersburg, Russia, 1965. [Google Scholar]
- Stepanoff, A.J. Centrifugal and Axial Flow Pumps: Theory, Design and Application; Wiley: Hoboken, NJ, USA, 1957; ISBN 978-0-471-82137-3. [Google Scholar]
- Aizenstein, M. Tsentrobezhnyye Nasosy Dlya Neftyanoy Promyshlennosti; Gostoptekhizdat: Moscow, Russia, 1957. [Google Scholar]
- Lobanoff, V.S.; Ross, R.R. Centrifugal Pumps: Design and Application, 2nd ed.; Gulf Publishing Company: Houston, TX, USA, 1992; ISBN 0-87201-200-X. [Google Scholar]
- Pfleiderer, C.; Petermann, H. Strömungsmaschinen, 7th ed.; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Polotskiy, I.; Bognitskaya, F.; Agulnik, R. Raschet Otvodyashchikh Ustroystv Tsentrobezhnogo Nasosa; Rudnev, S., Ed.; PMB CINTIhimneftemash: Moscow, Russia, 1967. [Google Scholar]
- Mashin, A. Raschet i Proyektirovaniye Spiral’nogo Otvoda; Moskovskiy Energeticheskiy Institut: Moscow, Russia, 1980. [Google Scholar]
- Spiridonov, E.; Prokhasko, L. Raschet i Proyektirovaniye Tsentrobezhnykh Nasosov; YUUrGU: Chelyabinsk, Russia, 2004; ISBN 5-696-02887-X. [Google Scholar]
- Mihajlow, A.; Maliushenko, V. Konstruktsiya i Raschet Tsenrobezhnikh Ustroystv Vysokogo Davleniya; Maszinostroienie: Moscow, Russia, 1971. [Google Scholar]
- Son, C.H.; Jin, H.B.; Kim, M.J.; Chung, W.J. Spiral Casing of a Volute Centrifugal Pump. J. Mech. Sci. Technol. 2014, 28, 2697–2706. [Google Scholar] [CrossRef]
- Tao, Y.; Yuan, S.; Liu, J.; Zhang, F. Influence of Cross-Sectional Flow Area of Annular Volute Casing on Transient Characteristics of Ceramic Centrifugal Pump. Chin. J. Mech. Eng. 2019, 32, 4. [Google Scholar] [CrossRef]
- Baloni, B.D.; Channiwala, S.A.; Mayavanshi, V.K. Pressure Recovery and Loss Coefficient Variations in the Two Different Centrifugal Blower Volute Designs. Appl. Energy 2012, 90, 335–343. [Google Scholar] [CrossRef]
- Gou, Q.; Xiao, S.; Wang, T.; Yan, J. Analysis and Comparison of Two Kinds of Design Approaches for Volutes of Centrifugal Pump. Energies 2023, 16, 6128. [Google Scholar] [CrossRef]
- Wang, T.; Yu, H.; Fang, Y.; Xiang, R.; Kan, N.; Yan, J. A New Design for Energy-Saving Volutes in Centrifugal Pumps. Phys. Fluids 2022, 34, 115119. [Google Scholar] [CrossRef]
- Khan, M.F.; Gjernes, T.; Guenther, N.; Hickey, J.-P. Impact of Volute Throat Area and Gap Width on the Hydraulic Performance of Low-Specific-Speed Centrifugal Pump. Modelling 2024, 5, 659–672. [Google Scholar] [CrossRef]
- KIM, J.-H.; Oh, K.; Pyun, K.; Kim, C.-K.; Choi, Y.-S.; Yoon, J.-Y. Design Optimization of a Centrifugal Pump Impeller and Volute Using Computational Fluid Dynamics. IOP Conf. Ser. Earth Environ. Sci. 2012, 15, 2025. [Google Scholar] [CrossRef]
- Yang, S.; Kong, F.; Chen, B. Research on Pump Volute Design Method Using CFD. Int. J. Rotating Mach. 2011, 2011, 137860. [Google Scholar] [CrossRef]
- Ji, C.; Wang, Y.; Yao, L. Numerical Analysis and Optimization of the Volute in a Centrifugal Compressor. In Proceedings of the Challenges of Power Engineering and Environment; Cen, K., Chi, Y., Wang, F., Eds.; Springer: Berlin/Heidelberg, Germany, 2007; pp. 1352–1356. [Google Scholar]
- Ji, C.; Wang, Y. The Design and Flow Simulation of the Volute Casing of a Mixed Flow Pump. In Proceedings of the 2011 International Conference on Electronic & Mechanical Engineering and Information Technology, Harbin, China, 12–14 August 2011; Volume 2, pp. 820–823. [Google Scholar]
- Chen, Z.; Nguyen, V.T.T.; Tran, N.T. Optimum Design of the Volute Tongue Shape of a Low Specific Speed Centrifugal Pump. J. Electr. Electron Syst. 2017, 6, 2332-0796. [Google Scholar] [CrossRef]
- Golbabaei Asl, M.; Torabi, R.; Nourbakhsh, S.A. Experimental and FEM Failure Analysis and Optimization of a Centrifugal-Pump Volute Casing. Eng. Fail. Anal. 2009, 16, 1996–2003. [Google Scholar] [CrossRef]
- Zhang, Y.-L.; Li, J.-F.; Wang, T.; Xiao, J.-J.; Jia, X.-Q.; Zhang, L. Pressure Distribution on the Inner Wall of the Volute Casing of a Centrifugal Pump. Sci. Technol. Nucl. Install. 2022, 2022, 3563459. [Google Scholar] [CrossRef]
- Jia, X.; Li, Y.; Zhang, J.; Yan, C.; Lin, Z.; Zhu, Z. Research on the Effects of Volute Area Ratios on Centrifugal Pump Internal Flow and Noise. Phys. Fluids 2024, 36, 075111. [Google Scholar] [CrossRef]
- Han, Z.; Yang, S.; Chen, J.; Wang, W. Research on the Effect of Various Tongue Types on Large Volute Pumps. J. Phys. Conf. Ser. 2024, 2707, 012031. [Google Scholar] [CrossRef]
- Dong, R.; Chu, S.; Katz, J. Effect of Modification to Tongue and Impeller Geometry on Unsteady Flow, Pressure Fluctuations, and Noise in a Centrifugal Pump. J. Turbomach. 1997, 119, 506–515. [Google Scholar] [CrossRef]
- Baun, D.O.; Köstner, L.; Flack, R.D. Effect of Relative Impeller-to-Volute Position on Hydraulic Efficiency and Static Radial Force Distribution in a Circular Volute Centrifugal Pump. J. Fluids Eng. 2000, 122, 598–605. [Google Scholar] [CrossRef]
- Li, X.; Zhu, Z.; Li, Y.; Chen, X. Experimental and Numerical Investigations of Head-Flow Curve Instability of a Single-Stage Centrifugal Pump with Volute Casing. Proc. Inst. Mech. Eng. Part A J. Power Energy 2016, 230, 633–647. [Google Scholar] [CrossRef]
- Tan, L.; Zhang, D.; Shi, W.; Zhou, L.; Cai, X. Influence of Volute Basic Circle Diameter on the Pressure Fluctuations and Flow Noise of a Low Specific Speed Sewage Pump. J. Vibroeng. 2017, 19, 3779–3796. [Google Scholar] [CrossRef][Green Version]
- Chernobrova, A.; Sotnyk, M.; Moloshnyi, O.; Antonenko, S.; Boiko, V. Influence of Different Volute Casings Theoretical Methods Design on Pump Working Processes. J. Phys. Conf. Ser. 2021, 1741, 12014. [Google Scholar] [CrossRef]
- Rong, L.; Böhle, M.; Yandong, G. Improving the Hydraulic Performance of a High-Speed Submersible Axial Flow Pump Based on CFD Technology. Int. J. Fluid Eng. 2024, 1, 013902. [Google Scholar] [CrossRef]
- Chen, B.; Li, X.; Zhu, Z. Investigations of Energy Distribution and Loss Characterization in a Centrifugal Impeller through PIV Experiment. Ocean Eng. 2022, 247, 110773. [Google Scholar] [CrossRef]
- International Organization for Standardization. ISO 9906 Rotodynamic Pumps—Hydraulic Performance Acceptance Tests—Grades 1, 2 and 3, 2nd ed.; ISO: Geneva, Switzerland, 2012. [Google Scholar]
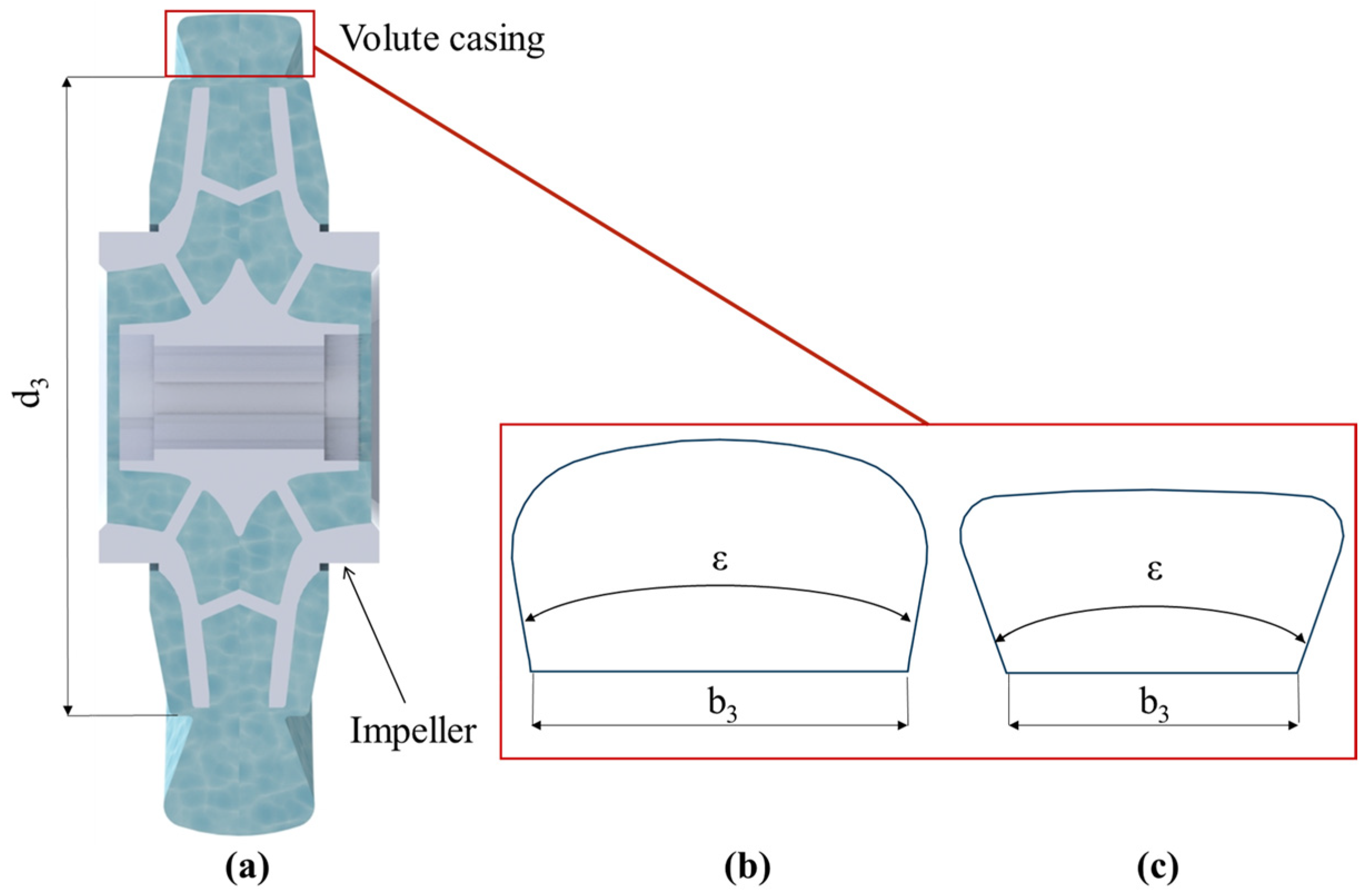





| Parameter | Reference Volute Casing | Lomakin Volute Casing | Stepanoff Volute Casing |
|---|---|---|---|
| Method | Does not correspond to any method | Conservation of angular momentum of the flow | Constant velocity |
| Inlet width (b3) | 60 mm | 40 mm | 52 mm |
| Inlet diameter (d3) | 276 mm | 281 mm | 297 mm |
| Cross-sectional shape | Rectangular | Trapezoidal and circular | Trapezoidal and circular |
| Opening angle of the walls (ε) | 0 | 20°, 30°, 40° | 20°, 30°, 40° |
| Sensor Type | Measured Quantity | Range | Accuracy |
|---|---|---|---|
| Electromagnetic flowmeter | Flow rate | 8.5–283 | ±0.5% |
| Electromagnetic flowmeter | Flow rate | 19–636 | ±0.5% |
| Electronic pressure transmitters with display | Pressure | 0–1 MPa | ±0.05% |
| Electronic pressure transmitters with display | Pressure | 0–0.2 MPa | ±0.05% |
| Thermometer | Temperature | 0–60 °C | ±1% |
| Torque meter | Torque on the shaft | 0–100 Nm | ±0.5% |
| Photo/contact tachometer | Speed rotation | 5–100,000 rpm | ±0.05% |
| Frequency converter with wattmeter | Electrical power | 0–100 A, 380–460 V | ±3.0% |
| Shape | ε | Efficiency, % | Head, m | Losses, m | Shape | ε | Efficiency, % | Head, m | Losses, m |
|---|---|---|---|---|---|---|---|---|---|
| Lomakin volute casing | Stepanoff volute casing | ||||||||
| Circular | 20° | 80.15 | 24.64 | 1.60 | Circular | 20° | 80.08 | 24.70 | 2.00 |
| Trapez. | 20° | 80.05 | 24.64 | 1.60 | Trapez. | 20° | 80.07 | 24.71 | 2.05 |
| Circular | 30° | 79.97 | 24.67 | 1.62 | Circular | 30° | 80.12 | 24.60 | 1.96 |
| Trapez. | 30° | 79.85 | 24.60 | 1.65 | Trapez. | 30° | 79.93 | 24.54 | 2.04 |
| Circular | 40° | 80.14 | 24.72 | 1.58 | Circular | 40° | 80.02 | 24.56 | 1.95 |
| Trapez. | 40° | 80.03 | 24.68 | 1.65 | Trapez. | 40° | 79.85 | 24.52 | 2.02 |
| Parameter | Reference Volute Casing | Stepanoff Volute Casing | Lomakin Volute Casing |
|---|---|---|---|
| Pump efficiency at 1.0Qopt | 80.5% | 80% | 80% |
| The point of highest efficiency at appropriate flow rate | 81% at 1.2Qopt | 81% at 1.2Qopt | 80% at 1.0Qopt |
| Pump operating range with the highest efficiency | 1.0Qopt–1.4Qopt | 1.0Qopt–1.5Qopt | 1.0Qopt–1.2Qopt |
| Hydraulic losses in the volute casing at 1.0Qopt | 1.94 m | 2.0 m | 1.7 m |
| Value of least loss at appropriate flow rate | 1.63 m at 1.3Qopt | 1.63 m at 1.5Qopt | 1.7 m at 1.0Qopt |
| Hydraulic losses in the impeller working with different volute casings at 1.0Qopt | 3.66 m | 3.87 m | 4.12 m |
| Value of least loss at appropriate flow rate | 3.0 m at 1.45Qopt | 3.0 m at 1.45Qopt | 3.1 m at 1.45Qopt |
| Head at 1.0Qopt | 24.68 m | 24.70 m | 24.50 m |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chernobrova, A.; Moloshnyi, O.; Szulc, P. Influence of Volute Casing Design Methods and Changes in Geometric Parameters on Pump Operation. Energies 2024, 17, 4590. https://doi.org/10.3390/en17184590
Chernobrova A, Moloshnyi O, Szulc P. Influence of Volute Casing Design Methods and Changes in Geometric Parameters on Pump Operation. Energies. 2024; 17(18):4590. https://doi.org/10.3390/en17184590
Chicago/Turabian StyleChernobrova, Anna, Oleksandr Moloshnyi, and Piotr Szulc. 2024. "Influence of Volute Casing Design Methods and Changes in Geometric Parameters on Pump Operation" Energies 17, no. 18: 4590. https://doi.org/10.3390/en17184590
APA StyleChernobrova, A., Moloshnyi, O., & Szulc, P. (2024). Influence of Volute Casing Design Methods and Changes in Geometric Parameters on Pump Operation. Energies, 17(18), 4590. https://doi.org/10.3390/en17184590







