Sherwood (Sh) Number in Chemical Engineering Applications—A Brief Review

Abstract

This paper reviews a series of cases for which the correct determination of the mass transfer coefficient is decisive for an appropriate design of the system and its operating conditions. The cases are of interest for applications in the energy sector, such as the thermoconversion of a fuel particle, processes in pipes, packed and fluidised beds, and corollary unit operations, such as extraction, absorption, and adsorption. The analysis is carried out by examining the expressions for the determination of the Sherwood number (which contains the mass transfer coefficient), and, when possible, generalised relationships (also in graphic form) have been provided, to offer a useful tool to cognoscenti.

1. Background

Chemical engineering applications involving mass transfer phenomena (such as, for example, those at the interface between fluids or between a fluid and a solid, with or without a chemical reaction) require an accurate prediction of the mass transfer coefficient to correctly define the design and operating conditions of the system [1]. This is of particular interest both in production processes (related to energy and materials) and in those of environmental relevance (for instance, adsorption and absorption for the purification of effluents). Moreover, the question is relevant for specific applications concerning phenomena occurring at interfaces, such as those involving organic material-based thermal switch in electronic devices [2] and tribovoltaic effects and electron transfer at liquid-semiconductor interfaces [3].

In general, we define $k_m$ as the mass transfer coefficient (readers can refer to the Nomenclature section). In its traditional dimensions, length per time, $k_m$, can therefore be conceived as a mass transfer rate.

The mass transfer coefficient can be known through the dimensionless Sherwood number ($Sh$) (Named after Thomas Kilgore Sherwood (1903–1976), U.S. chemical engineer, Professor at Massachusetts Institute of Technology and at University of California, Berkeley. Among publications, we can recall Sherwood et al. [4], while, in the years after his departure, the literature [5,6] has sketched out further details on the scientist), to which this review is dedicated. $Sh$, whose insights are present in the renowned text by Bird et al. [7], an illuminated review of which was provided by Astarita and Ottino [8], generally represents the ratio of the total mass transfer rate (i.e., by convection and diffusion) to the diffusive mass transfer rate

$$Sh={\displaystyle \frac{k_{m}L}{\mathfrak{D}}}$$

(1)

where, to keep the generality, $L$ is the characteristic length of the system under investigation, and $\mathfrak{D}$ is a diffusion coefficient.

The Sherwood number is usually represented as a function

$$Sh\left(Re,Sc\right)$$

(2)

where the Reynolds number (Named after Osborne Reynolds (1842–1912), British (Irish-born) scientist, Fellow of the Royal Society) normally characterises a measure of the ratio between inertial and viscous forces

$$Re={\displaystyle \frac{\rho uL}{\mu }},$$

(3)

with $\rho$ as the fluid density, $\mu$ as the dynamic viscosity of the fluid, and $u$ as a characteristic velocity, while the Schmidt number (Named after Ernst H.W. Schmidt (1892–1975), German scientist, Professor at Technical University of Munich) of a fluid is defined as the ratio of momentum diffusivity (i.e., the kinematic viscosity = $\mu /\rho$) and mass diffusivity, as follows:

$$Sc={\displaystyle \frac{\mu }{\rho \mathfrak{D}}}.$$

(4)

It is observed that $Sc$, unlike $Sh$ and $Re$, is an intrinsic fluid property (once conditions of, e.g., temperature and pressure are set). In fact, all the quantities determining $Sc$ in Equation (4) are intrinsic fluid properties that can be obtained by corresponding state principles (see, e.g., Scalabrin et al. [9] for $\rho$, Al-Syabi et al. [10] for $\mu$, and Yu and Gao [11] for $\mathfrak{D}$). All these quantities are independent of the system geometry (L) and fluid-dynamics (L, u).

Scientific literature has devoted much interest to the analysis of the Sherwood number for many different systems. In the development of this review, pertinent articles will be referred to. On the other hand, the literature lacks work that present in a concise and synoptical manner this specific topic. The purpose of this brief review is to analyse a series of reference cases for which the literature has provided relationships of the type in Equation (2) or similar. The cases range from fluid–particle to fluid–fluid applications (e.g., reacting particles, mass transfer in packed and fluidised beds, in pipes, extraction, and ab(d)sorption), and generalised relationships/figures are given, when possible.

2. Mass Transfer between a Solid Object and a Fluid

First, take the example of an isolated object in a fluid under forced convection, where mass transfer occurs. We can generalise Equation (2) into

$$Sh=\alpha +\beta {Re}^{\lambda }{Sc}^{\sigma }.$$

(5)

Table 1. $Sh\left(Re,Sc\right)$ relations, as in Equation (5), for mass transfer between a solid object and a fluid.

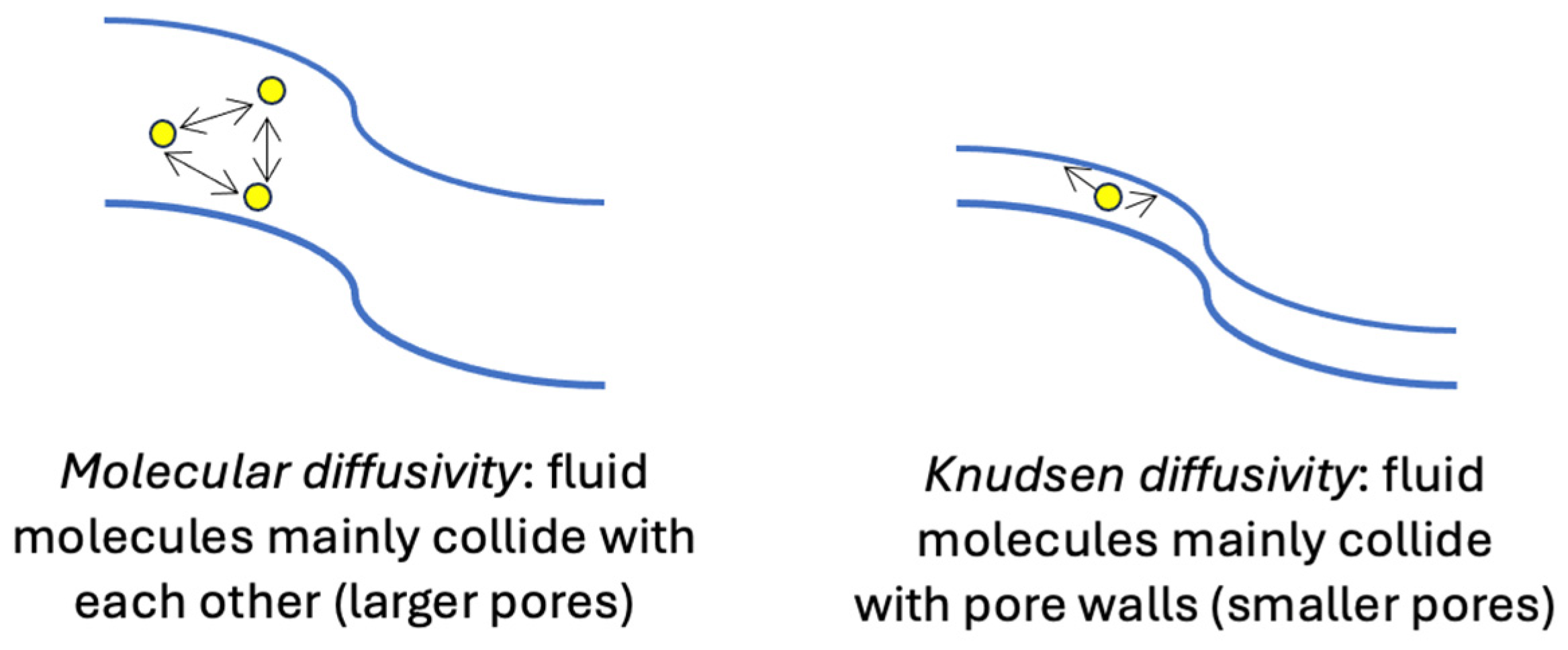
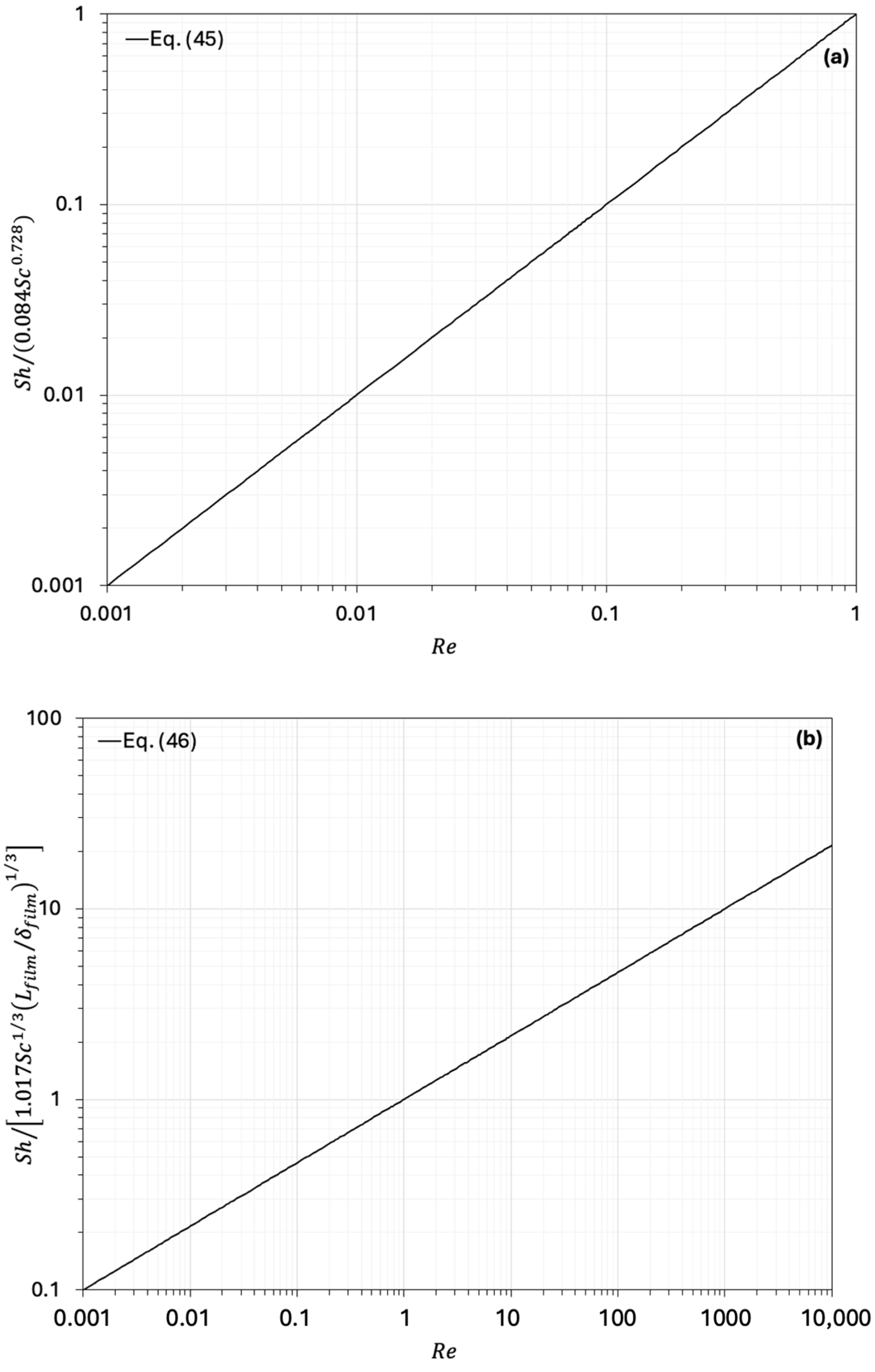
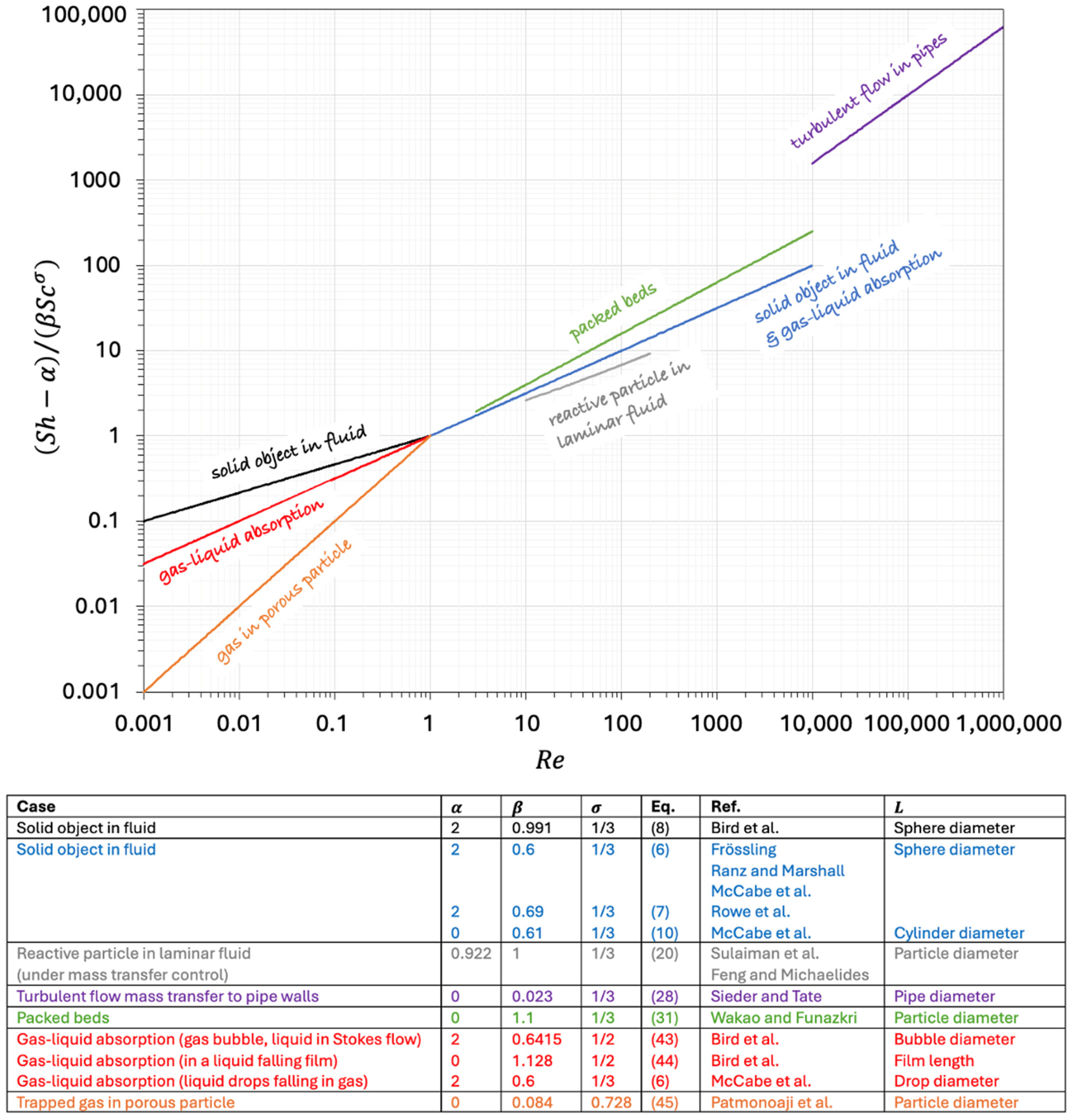
Equation	$\mathit{\alpha }$	$\mathit{\beta }$	$\mathit{\lambda }$	$\mathit{\sigma }$	Ref.	Fields of Validity
Isolated sphere ($L$ = sphere diameter; $u$ = fluid terminal velocity), forced convection
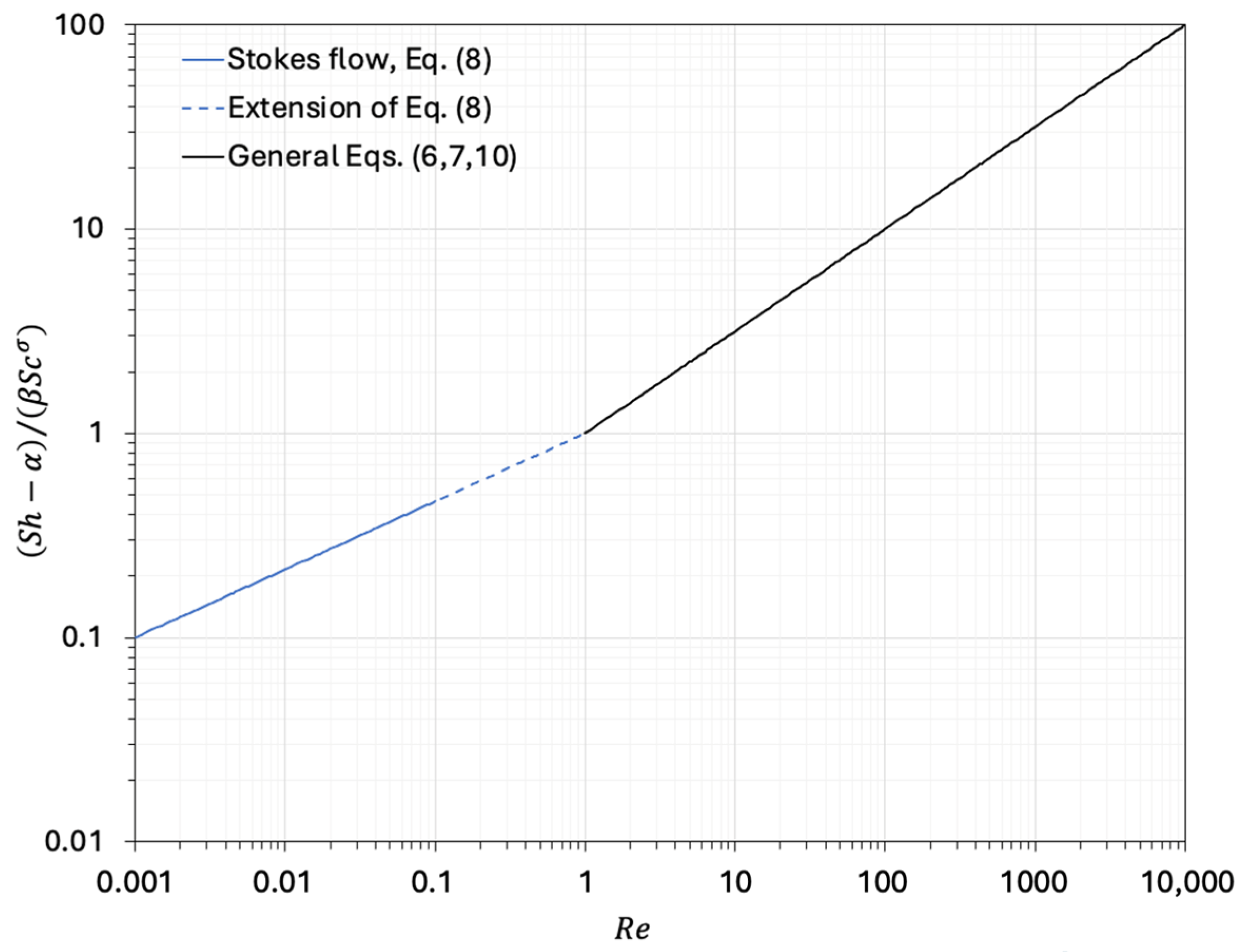
(6)	2	0.6	1/2	1/3	Frössling [12] Ranz and Marshall [13,14] McCabe et al. [15]	$Re$ ≤ 150; 0.5 ≤ $Sc$ ≤ 2 McCabe et al. [15] report that Equation (6) is fairly accurate also for $Re$ up to 1000
(7)	2	0.69	1/2	1/3	Rowe et al. [16]	20 ≤ $Re$ ≤ 2000
(8)	2	0.991	1/3	1/3	Bird et al. [7]	$Re$ ≤ 0.1
(9)	1	0.724	0.48	1/3	Clift et al. [17]	100 ≤ $Re$ ≤ 2000; $Sc$ ≥ 200
Isolated cylinder ($L$ = cylinder diameter; $u$ = fluid terminal velocity), forced convection
(10)	0	0.61	1/2	1/3	McCabe et al. [15]	10 ≤ $Re$ ≤ 104

illustrates the cases under investigation, although it has an introductory role and does not cover all possible circumstances. In this framework, when considering the Reynolds number (Equation (3)), $L$ is referred to the object (the diameter of a spherical particle or of a cylindrical object, for example), while $u$ is the terminal velocity established between the fluid and the object.

In Table 1, the following equations have been introduced:

$$Sh=2+0.6{Re}^{1/2}{Sc}^{1/3}$$

(6)

$$Sh=2+0.69{Re}^{1/2}{Sc}^{1/3}$$

(7)

$$Sh=2+0.991{Re}^{1/3}{Sc}^{1/3}$$

(8)

$$Sh=1+0.724{Re}^{0.48}{Sc}^{1/3}$$

(9)

$$Sh=0.61{Re}^{1/2}{Sc}^{1/3}.$$

(10)

For a sphere, adopting the traditional modelling according to the stagnant film theory (that suggests the exponent of 1/2 and 1/3 for $Re$ and $Sc$, respectively), we end up into the classical Equation (6), often named the equation by Frössling in 1938 [12] or by Ranz and Marshall who, in 1952 [13,14], modified the 1938 equation (that reported $\beta$ = 0.522 instead of 0.6). In Table 1, when possible, we also give indications of the fields of validity in terms of Reynolds and Schmidt numbers. Equation (6) has been further reviewed by Rowe et al. [16], with a slight modification for $\beta$, Equation (7). If we consider the particular case of Stokes (creeping) flow, at very low $Re$, we end up in Equation (8) as given by Bird et al. [7], where both $\beta$ and $\lambda$ change vs. the traditional equation by Ranz and Marshall. Equations (6)–(8) all agree in predicting $Sh$ = 2 for $Re$ = 0, i.e., for stagnant situations where the continuous phase velocity is zero. This gives the lower limit for the mass transfer coefficient (any fluid motion will increase it). In the attempt to find expressions for Equation (2) that vary as $Re$ varies, Clift et al. [17] reported

$$\left\{\begin{array}{c}Sh=1+{\left(1+ReSc\right)}^{1/3}\\ Sh=1+{\left(1+ReSc\right)}^{1/3}{Re}^{0.077}\\ Sh=1+0.724{Re}^{0.48}{Sc}^{1/3}\end{array}\right.\left\{\begin{array}{c}Re\le 1\\ 1\le Re\le 400\\ 100\le Re\le 2000\end{array}\right.\left\{\begin{array}{c}0.24\le Sc\le 100\\ 0.24\le Sc\le 100\\ Sc\ge 200\end{array}\right.$$

(11)

where the third formulation of Equation (11) corresponds to Equation (9) in Table 1, and it is written in the form of Equation (5) with $\lambda$ very close to the classical formulations (while $\alpha$ and $\beta$ vary). If we consider mass transfer around a cylindrical object, McCabe et al. [15] refer to Equation (10), which is very similar to Equation (6) but with $\alpha$ = 0.

Once given the Schmidt number for the fluid under consideration, $Sh$ can be seen as a function of $Re$ by reworking Equation (5) into

$${\displaystyle \frac{Sh-\alpha }{\beta {Sc}^{\sigma }}}={Re}^{\lambda }.$$

(12)

This allows for a synoptical graphical view of data given in Table 1, as illustrated in Figure 1. We have reported, for creeping flow, the expression in the BSL mass transfer book [7], Equation (8), which has been further extended for $Re$ up to 1, i.e., when the left-hand side of Equation (12) values 1 for any $\lambda$. Starting from this point, we adopt the generalised form of Equations (6), (7), and (10) for $Re$ up to 10,000. Of course, once chosen the expression to use from Table 1, (i) the application of the appropriate values for $\alpha$ and $\beta$ (while $\sigma$ = 1/3), (ii) the evaluation of $Sc$, and (iii) the check of the fields of validity, will allow for the determination of $Sh$. As the reader has observed, data in Figure 1 are illustrated on a log–log basis to better emphasise the power–law relationship (linear relationship on a log–log plot, with slope = $\lambda$) in Equation (12). From a physical point of view (this applies also to other relationships presented in this communication), the value of $\lambda$ gives an indication of the dependency of the mass transfer coefficient (contained in $Sh$) on the ratio between inertial and viscous forces (given by $Re$).

3. Particle–Fluid Mass Transfer with Chemical Reaction

In the case of a particle that reacts in a fluid phase, three controlling mechanisms can be envisaged [18]: (1) fluid diffusion over the particle external film; (2) fluid diffusion through the pores of the particle; (3) chemical reaction. In general, the chemical reaction is the limiting stage, when the temperature is not high. As the temperature increases, the rate of the chemical reaction increases much more quickly (due to the exponential dependence of the kinetic constant—Arrhenius’ law) than the rate of diffusive phenomena (regulated by external/internal diffusivity, usually a function of temperature through milder power laws). Thus, at increasing temperature, the system is oriented towards diffusion-controlled regimes.

If the controlling regime is that of external diffusion (i.e., with internal diffusion and chemical reaction much faster than external diffusion), the knowledge of $k_m$ (the mass transfer coefficient in the external film) is important as it appears in the design equation (at the particle and the reactor scale) relating particle residence time and degree of conversion [18].

Simple approaches consider the application of Equation (5) for very fine particles, where $Re$ becomes negligibly small, and $Sh\cong \alpha$. For $\alpha$ = 2

$$Sh={\displaystyle \frac{k_{m}L}{\mathfrak{D}}}=2⟹k_m={\displaystyle \frac{\mathfrak{D}}{\frac{L}{2}}},$$

(13)

i.e., the mass transfer coefficient is the ratio between the diffusion coefficient (in the external film) and the particle radius (supposing a spherical particle). A more elaborated approach has been developed in an article by El-Genk and Tournier [19], where the authors have considered the case-study of the gasification of a graphite particle at high temperature (under external diffusion control) and have expressed the Sherwood number as a function of a laminar and a turbulent contribution.

If, on the other hand, the kinetic control begins to be relevant, we should consider the following approach. When the fluid reactant arrives on the surface of the reacting particle (be it an external or an internal surface), the chemical reaction does not occur only on this surface but also inside the pores. In other terms, we have at the same time the intra-particle diffusion of the fluid molecule, and the chemical reaction when this molecule encounters the pores wall made of reacting solid material. These aspects are taken into account by the Thiele modulus (Named after Ernest W. Thiele (1895–1993), U.S. chemical engineer, Professor at University of Notre Dame), $Th$. For a first-order irreversible chemical reaction, a possible expression (others can be found in the literature) is

$$Th=L\sqrt{{\displaystyle \frac{k_{kin}}{{\mathfrak{D}}_{eff}}}},$$

(14)

where we recall that $L$ is the particle diameter. In Equation (14), $k_{kin}$ is a kinetic constant ruling the first-order irreversible reaction, while ${\mathfrak{D}}_{eff}$ represents the effective diffusion coefficient in the particle pores (vide infra for more details on ${\mathfrak{D}}_{eff}$). Equation (14) can be rewritten as

$$Th=\sqrt{{\displaystyle \frac{L^2{k}_{kin}}{{\mathfrak{D}}_{eff}}}}=\sqrt{{\displaystyle \frac{\frac{L^2}{{\mathfrak{D}}_{eff}}}{\frac{1}{k_{kin}}}}}.$$

(15)

The characteristic diffusion and reaction time can be expressed as order of magnitude, respectively, as

$$t_D={\displaystyle \frac{L^2}{{\mathfrak{D}}_{eff}}}$$

(16)

$$t_R={\displaystyle \frac{1}{k_{kin}}},$$

(17)

meaning that the number of Thiele represents the ratio between the two

$$Th=\sqrt{{\displaystyle \frac{t_D}{t_R}}}.$$

(18)

Therefore, when $Th$ is sufficiently low, chemical reaction is the controlling stage (fine particles, high diffusivity, and low kinetic constant). In contrast, at high Thiele numbers (coarse particles, low diffusivity, and high kinetic constant), a diffusion controlling regime is established.

The expression in Equation (14) is the one adopted by Sulaiman et al. [20] (further extensions are given in [21]). They considered the case of mass transfer towards a reactive particle in a fluid laminar flow (first-order irreversible chemical reaction), and proposed, based on the concept of the summation of resistances to transfer (on the right-hand side, the first addend represents a “kinetic resistance” and the second one a “purely diffusive resistance”),

$${\displaystyle \frac{1}{Sh}}={\displaystyle \frac{1}{2\frac{{\mathfrak{D}}_{eff}}{\mathfrak{D}}}}\left[{\displaystyle \frac{\mathrm{t}\mathrm{a}\mathrm{n}\mathrm{h}\left(Th/2\right)}{Th/2-\mathrm{t}\mathrm{a}\mathrm{n}\mathrm{h}\left(Th/2\right)}}-{\displaystyle \frac{12}{{Th}^2}}\right]+{\displaystyle \frac{1}{{Sh}_0}},$$

(19)

where $\mathfrak{D}$ is the diffusion coefficient in the fluid phase, while ${Sh}_0$ represents the Sherwood number for a purely mass transfer-controlled system (i.e., without chemical reaction), with an expression deriving from [22]

$${Sh}_0=0.922+{Re}^{1/3}{Sc}^{1/3}+0.1{Re}^{2/3}{Sc}^{1/3}=0.922+{Pe}^{1/3}+0.1{Re}^{1/3}{Pe}^{1/3}$$

(20)

where it introduced the Péclet number (Named after Jean Claude E. Péclet (1793–1857), French physicist, Professor at Central School of Paris),

$$Pe=ReSc={\displaystyle \frac{uL}{\mathfrak{D}}}.$$

(21)

Equation (20) has been reported to be valid for $Pe$ and $Re$ both larger than 10, and it is plotted in Figure 2 for the Reynolds number up to 200.

Back to Equation (19), for an extremely fast reaction rate (diffusion controlling regime, $Th\to \infty$), the kinetic resistance (first addend) disappears and $Sh\to {Sh}_0$. For an extremely slow reaction rate (kinetic controlling regime, $Th\to 0$), $\mathrm{t}\mathrm{a}\mathrm{n}\mathrm{h}\left(Th/2\right)\to Th/2$, therefore the kinetic resistance $\to \infty$ and $Sh\to 0$. The observation that the hyperbolic tangent of an argument $x$ tends to the argument itself if $x$ is close to zero can be demonstrated by considering the definition

$$\mathrm{t}\mathrm{a}\mathrm{n}\mathrm{h}\left(x\right)={\displaystyle \frac{e^x-e^{-x}}{e^x+e^{-x}}}$$

(22)

and by applying the Taylor expansion formula up to the first derivative

$$\mathrm{t}\mathrm{a}\mathrm{n}\mathrm{h}\left(x\right){|}_{x=0}\cong \mathrm{t}\mathrm{a}\mathrm{n}\mathrm{h}\left(x=0\right)+{\displaystyle \frac{\mathrm{d}\left[\mathrm{t}\mathrm{a}\mathrm{n}\mathrm{h}\left(x\right)\right]}{\mathrm{d}x}}{|}_{x=0}(x-0)=0+{\left.{\displaystyle \frac{{\left(e^x+e^{-x}\right)}^2-{\left(e^x-e^{-x}\right)}^2}{{\left(e^x+e^{-x}\right)}^2}}\right|}_{x=0}x=x.$$

(23)

Both Equations (14) and (19) rely on ${\mathfrak{D}}_{eff}$. It depends on [18] (i) the molecular diffusivity (${\mathfrak{D}}_{mol}$), which regulates the fluid transport in larger pores, i.e., where the fluid molecules prevailingly collide with each other (their mean free path is smaller than the pore mean size), and (ii) the Knudsen diffusivity (${\mathfrak{D}}_{Kn}$), which rules the fluid transport in smaller pores, i.e., where the fluid molecules mainly collide with the pore walls (their mean free path is greater than the mean pore size), Figure 3. It is here recalled the effect that the mean free path, in its broadest sense, has on mass transfer, as discussed in the relevant literature (e.g., [23,24]).

For ${\mathcal{D}}_{mol}$, it can be used

$${\mathcal{D}}_{mol}\left(T\right)={\mathcal{D}}_{mol}\left(T_0\right){\left({\displaystyle \frac{T}{T_0}}\right)}^{1.75}$$

(24)

where $T$ is the temperature and once a value for ${\mathcal{D}}_{mol}$ at a reference temperature $T_0$ is known. For ${\mathcal{D}}_{Kn}$, a useful expression is

$${\mathcal{D}}_{Kn}=48.5d_{pore}\sqrt{{\displaystyle \frac{T}{\mathcal{M}}}},$$

(25)

where $d_{pore}$ and $\mathcal{M}$ are the mean pore diameter [m] and the molecular mass of the diffusing species, respectively; $T$ is in [K]. The formula gives ${\mathcal{D}}_{Kn}$ in [m²/s]. The diffusivity is an increasing function of temperature, and, for smaller pores, increases when the molecular mass of the diffusing species decreases.

Furthermore, ${\mathfrak{D}}_{eff}$ is proportional to the porosity $\epsilon$ of the particle and inversely proportional to the pores’ tortuosity $\varsigma$ (the proposed symbol comes from the Greek ancient word streblós = tortuous). The tortuosity can be seen as the ratio between the path that the fluid molecule has to travel inside the pore ($L_{eff}$) and the minimum path that can be travelled ($L_{min}$), i.e., for a straight pore (Figure 4). It is observed that $\varsigma$ is equal to 1 for non-tortuous pores; otherwise it is >1. For particles that are virtually empty ($\epsilon$ = 1), the tortuosity would be unitary. For increasingly fuller particles ($\epsilon$ will decrease), the tortuosity increases, and for particles virtually full ($\epsilon$ = 0), the tortuosity would be infinite. Thus, one can approximate

$$\varsigma ={\displaystyle \frac{1}{\epsilon }}$$

(26)

Considering the two processes (molecular- and Knudsen-diffusivity) in series, and adopting the classic formulation for resistances in series, it is

$${\mathcal{D}}_{eff}={\displaystyle \frac{\epsilon }{\varsigma }}\left({\displaystyle \frac{1}{\frac{1}{{\mathcal{D}}_{mol}}+\frac{1}{{\mathcal{D}}_{Kn}}}}\right)\cong {\epsilon }^2{\mathcal{D}}_{Kn},$$

(27)

which is further shortened by considering Equation (26) and approximating ${\mathcal{D}}_{mol}\gg {\mathcal{D}}_{Kn}$ (the controlling diffusion mechanism is that in the smallest pores, and the resistance due to molecular diffusion is of minor relevance).

4. Mass Transfer in Chemical Reactors/Plants

4.1. Pipes, Packed Beds, and Monolith Channels

The general Equation (5) has been also applied to other cases, as listed in

Table 2. $Sh\left(Re,Sc\right)$ relations, Equation (5), for mass transfer in chemical reactors/plants.

Equation	$\mathit{\alpha }$	$\mathit{\beta }$	$\mathit{\lambda }$	$\mathit{\sigma }$	Ref.	Fields of Validity
Pipe turbulent flow ($L$ = pipe diameter)
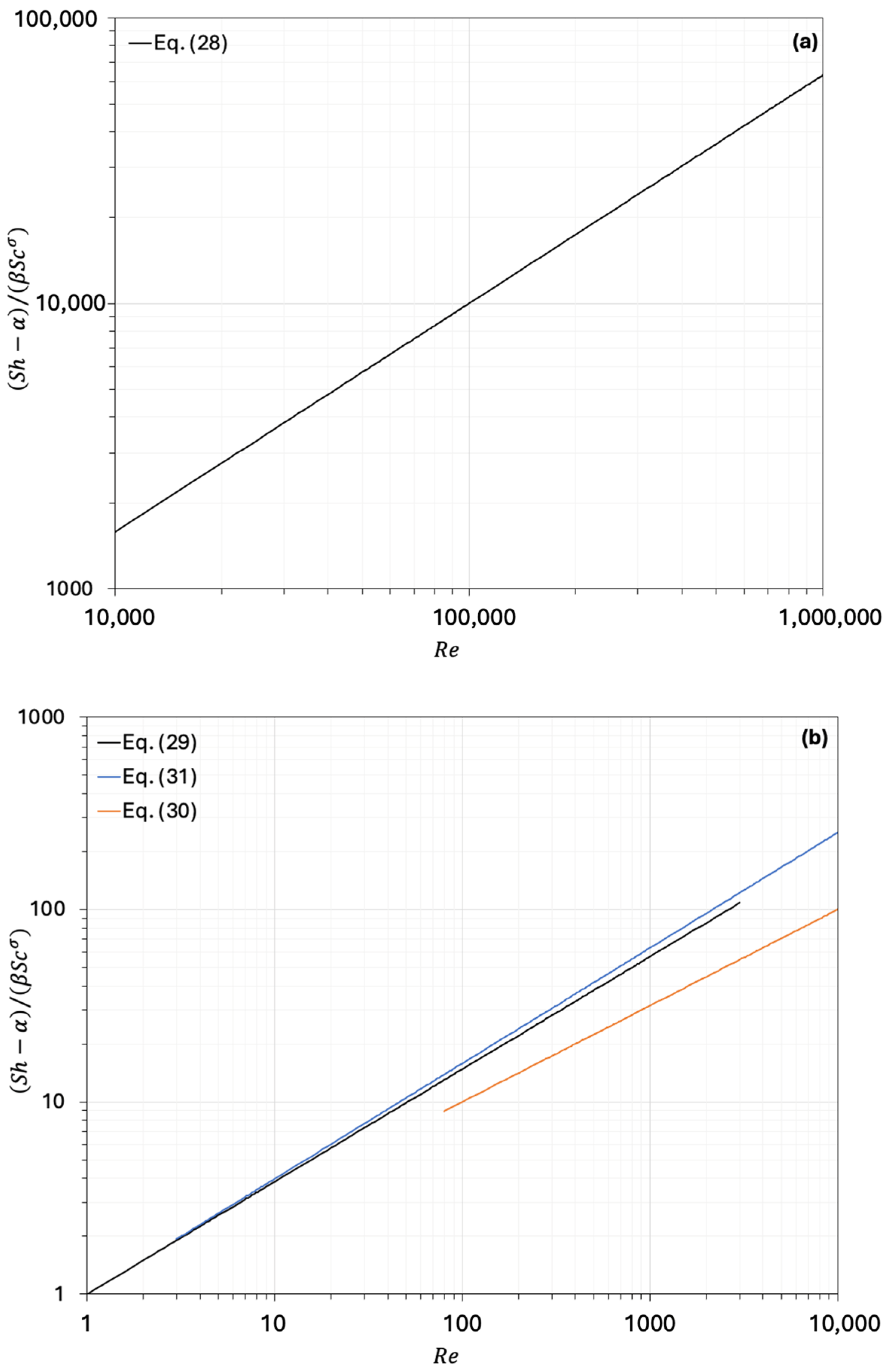
(28)	0	0.023	0.8	1/3	Sieder and Tate [25]	$Re\ge {10}^4$
Fluid and a packed bed of particles ($L$ = particle diameter)
(29)	0	1.17	0.585	1/3	Sherwood et al. [4]	$1\le Re\le 3000$; $ϵ$ = 0.4–0.45
(30)	2	1.8	1/2	1/3	Ranz [26]	$Re\ge 80$
(31)	0	1.1	0.6	1/3	Wakao and Funazkri [27]	3 ≤ $Re$ ≤ ${10}^4$

.

The following equations have been introduced:

$$Sh=0.023{Re}^{0.8}{Sc}^{1/3}$$

(28)

$$Sh=1.17{Re}^{0.585}{Sc}^{1/3}$$

(29)

$$Sh=2+1.8{Re}^{1/2}{Sc}^{1/3}$$

(30)

$$Sh=1.1{Re}^{0.6}{Sc}^{1/3}.$$

(31)

For the well-developed turbulent flow mass transfer to pipe walls, we can borrow the analogous Sieder and Tate [25] equation, valid for heat transfer, ending up in Equation (28) if the variations in the dynamic viscosity between core fluid and wall temperature is neglected. Otherwise, it is

$$Sh=0.023{Re}^{0.80}{Sc}^{1/3}{\varphi }^{0.14},$$

(32)

where (with ${\mu }_w$ as the fluid viscosity at wall temperature)

$$\varphi ={\displaystyle \frac{\mu }{{\mu }_w}}.$$

(33)

Starting from Equation (28) or Equation (32), further modifications have been reported, e.g., in Sherwood et al. [4] and McCabe et al. [15].

For mass transfer involving a fluid phase and a packed bed of particles, Sherwood et al. [4] proposed Equation (29) (if the bed presents a void percentage, $ϵ$, of 40–45%; for higher values, see [28]), with further considerations given in McCabe et al. [15]. Two other widely used correlations for fluid passing through a packed bed of particles are those by Ranz [26] for Reynolds number larger than 80, Equation (30), and by Wakao and Funazkri [27], Equation (31), which is not much different from Equation (29).

By considering again Equation (12), we plot (Figure 5a,b) the key equations listed in Table 2. The similarity between the expressions by Sherwood et al. [4] and by Wakao and Funazkri [27] can be observed (the discrepancy in the ordinate value is 1.66% at $Re$ = 3, and 12.8% at $Re$ = 3000; although, for the evaluation of $Sh$, differences in $\beta$ must be also taken into account). Equation (30) has an inherently different plot, as in this case: $\alpha \ne 0$.

For a monolith channel with length $L_{channel}$ and a diameter of $D$, Hawthorn [29] proposed the following:

$$Sh=3.66{\left[1+0.095{\displaystyle \frac{D}{L_{channel}}}ReSc\right]}^{0.45},$$

(34)

with further elaboration given by Hayes and Kolaczkowski [30].

4.2. Extraction in Columns

Departure from the more traditional approaches can be seen when treating the case of liquid–liquid extraction in a structured packed column [31]. Here the characteristic length appearing in the Sherwood number is $L$ = $d_{drop}$, the (Sauter) average drop is diameter, and $Re$ is defined accordingly as

$$Re={\displaystyle \frac{{\rho }_c{v}_{slip}{d}_{drop}}{{\mu }_c}},$$

(35)

where ${\rho }_c$ and ${\mu }_c$ are the density and viscosity of the continuous phase, respectively, and $v_{slip}$ is the single drop slip velocity. Moreover, the number of Eötvös (Named after Loránd Eötvös de Vásárosnamény (1848–1919), Hungarian physicist, Professor at University of Budapest) appears (it can be seen as the ratio of buoyancy forces to surface tension), that includes ${\rho }_d$ (density of the dispersed phase), $g$ (gravitational acceleration), and $\gamma$ (interfacial tension)

$$Eö={\displaystyle \frac{\left({\rho }_c-{\rho }_d\right)g{d}_{drop}^2}{\gamma }}.$$

(36)

The proposed relation is

$$Sh=7.264{Re}^{0.046}{Eö}^{0.017}{\left({\displaystyle \frac{h}{D}}\right)}^{-0.091},$$

(37)

where $h$ is the height of sampling (from the top of the nozzle), and $D$ the system (column) diameter. Further information is also given by Kumar and Hartland [32].

4.3. Fluidised Beds

Switching to the case of mass transfer in fluidised beds, we can generalise rewriting Equations (5) and (12) as

$$Sh=\alpha ϵ+\beta {\left({\displaystyle \frac{Re}{{ϵ}^{\iota }}}\right)}^{\lambda }{Sc}^{\sigma }$$

(38)

$${\displaystyle \frac{Sh-\alpha ϵ}{\beta {Sc}^{\sigma }}}={\left({\displaystyle \frac{Re}{{ϵ}^{\iota }}}\right)}^{\lambda },$$

(39)

i.e., considering the bed voidage degree $ϵ$, as in

Table 3. $Sh\left(Re,Sc\right)$ relations, Equation (38), for mass transfer in fluidised beds.

Equation	$\mathit{\alpha }$	$\mathit{\beta }$	$\mathit{\iota }$	$\mathit{\lambda }$	$\mathit{\sigma }$	Ref.	$\mathit{ϵ}$ Is
(40)	2	0.61	0	0.48	1/3	Hayhurst and Parmar [33]	${ϵ}_{mf}$
(41)	2	0.69	1	1/2	1/3	LaNauze and Jung [34]	$ϵ$ or ${ϵ}_{mf}$
(42)	2	0.84	1	1/2	1/3	Molignano et al. [35]	${ϵ}_e$

.

The following equations have been introduced:

$$Sh=2ϵ+0.61{Re}^{0.48}{Sc}^{1/3}$$

(40)

$$Sh=2ϵ+0.69{\left({\displaystyle \frac{Re}{ϵ}}\right)}^{1/2}{Sc}^{1/3}$$

(41)

$$Sh=2ϵ+0.84{\left({\displaystyle \frac{Re}{ϵ}}\right)}^{1/2}{Sc}^{1/3}.$$

(42)

Hayhurst and Parmar [33] investigated the case of carbon spheres burning in a bubbling fluidised bed under external mass transfer control (i.e., using sufficiently coarse particles). They proposed Equation (40), where the bed voidage degree is calculated at minimum fluidisation conditions (${ϵ}_{mf}$). The velocity appearing in $Re$ is that of the gas in the fluidised bed dense phase; the diffusion coefficient appearing in both $Sh$ and $Sc$ is that in the fluid phase, and $L$ refers to the particle. Earlier, a similar equation (Equation (41)) appeared in LaNauze and Jung [34]. Further developments have been given by Dennis et al. [36], while Equation (41) has also been confirmed in the work by Scala [37]. The same author [38] has further addressed the case of particle–fluid mass transfer in multiparticle systems at low Reynolds numbers. In a recent work by Molignano et al. [35], Equation (41) has been re-worked into Equation (42), where ${ϵ}_e$ is the bed voidage in the fluidised bed emulsion (dense) phase. We have plotted Equation (39) in Figure 6, where only small discrepancies among various cases can be observed (again, the exact value for $Sh$ will also depend on the other parameters, $\beta$, $\iota$, and $\lambda$). Moreover, it is recognised that mass transfer in fluidised beds is attracting increasing importance for new applications, such as solid–gas reactions for thermochemical energy storage [39,40,41], for which these considerations may be applied.

4.4. Adsorption and Absorption Systems

In sorption operations and with reference to spherical particles [7,42], a value for the overall $Sh$ number of 10 has been reported that can be used for counter-current adsorption in plug flow and fluidised bed columns.

For gas–liquid absorption, take again the general Equations (5) and (12). Details are given in

Table 4. $Sh\left(Re,Sc\right)$ relations, cf. Equation (5), for gas–liquid absorption.

Equation	$\mathit{\alpha }$	$\mathit{\beta }$	$\mathit{\lambda }$	$\mathit{\sigma }$	Ref.	Valid for
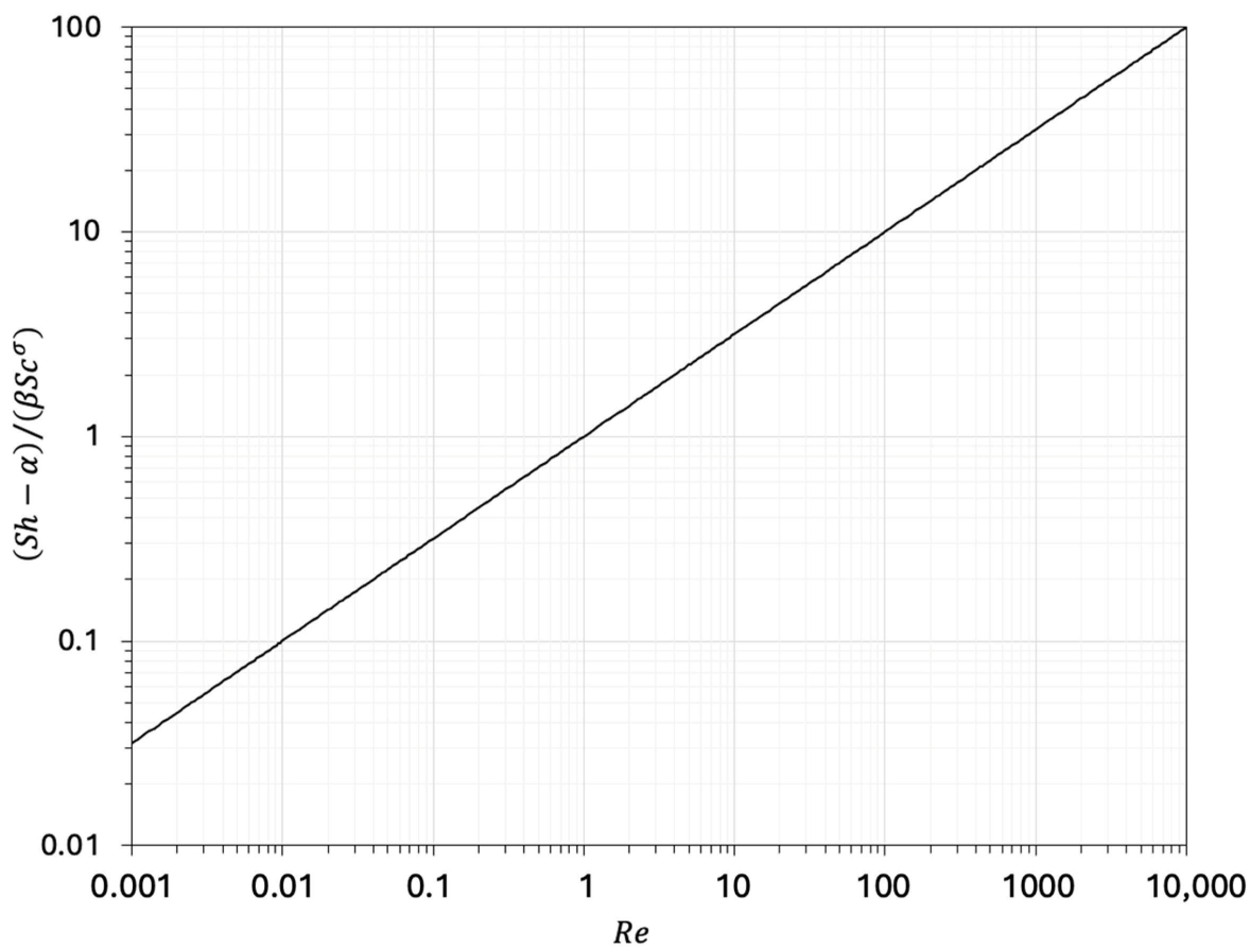
(43)	2	0.6415	1/2	1/2	Bird et al. [7]	Gaseous bubble (diameter $L)$ liquid in Stokes flow
(44)	0	1.128	1/2	1/2	Bird et al. [7]	Gas absorbed into a liquid falling film (length $L$)
(6)	2	0.6	1/2	1/3	McCabe et al. [15]	Small spherical liquid drops (diameter $L)$ falling in gas

, where the following equations have been introduced:

$$Sh=2+0.6415{Re}^{1/2}{Sc}^{1/2}$$

(43)

$$Sh=1.128{Re}^{1/2}{Sc}^{1/2}.$$

(44)

In liquid Stokes flow, Bird et al. [7] report Equation (43) ($L$ is referred to the bubble), converted into Equation (44) for gas absorption into a falling film of pure liquid. For Equation (44), we should indeed remember that the characteristic length $L$ is now the film length and that the velocity (also appearing in $Re$) is the maximum velocity in the falling film. In the book by McCabe et al. [15], we find the Ranz and Marshall Equation (6) also applied to small spherical drops of liquid falling through a gas. However, if we have a system in which (low-viscous) drops fall through a viscous liquid, the authors proposed Equation (44).

Results in the form of Equation (12) have been plotted in Figure 7. In this case, the equations in Table 4 collapse into one plot, as the exponent $\lambda$ for $Re$ is the same; however, careful attention must be paid to the correct definition of $L$ and $u$ appearing in Equation (3).

5. Other Cases

Patmonoaji et al. [43] reported the case of trapped gases in porous media, obtaining a correlation valid for $Re\le 1;200\le Sc\le 2000$ (for $Re$ referring to the particle)

$$Sh=0.084Re{Sc}^{0.728}.$$

(45)

If we have the dissolution of a solid from the wall into a falling film (with length and thickness $L_{film}$ and ${\delta }_{film}$, respectively) of pure liquid, Bird et al. [7] proposed the following:

$$Sh=1.017{Re}^{1/3}{Sc}^{1/3}{\left({\displaystyle \frac{L_{film}}{{\delta }_{film}}}\right)}^{1/3}.$$

(46)

As for Equation (44), the Reynolds number is defined with respect to the film length, and the velocity is the maximum in the film. Figure 8a,b plot Equations (45) and (46), respectively.

Chemical engineering applications involving mass transfer phenomena are much more extensive than this brief review can accommodate. Some, among those not covered here, are recognised in

Table 5. List of some works treating topics related to the Sherwood number, and not covered in this communication.

Ref.	Topic
Miyauchi [44]	Dilute sphere-packed beds
Suzuki [45]	Multiparticle systems with stagnant fluid
Isaacson and Sonin [46]	Electrodialysis
Burganos et al. [47]	Spheroidal (but not spherical) adsorbing particles
Jacobs and Verhoef [48]	Evaporation from soil
Glatzer and Doraiswamy [49] Coltrin and Kee [50] Asadollahzadeh et al. [51]	Rotating disk flow
Bird et al. [7]	Free convection
Lee et al. [52] Banerjee and De [53] Murmura et al. [54]	Membrane systems
Martín et al. [55]	Oscillating bubbles in gas–liquid contactors
Vennela et al. [56]	Electro-osmosis
Prajongkan et al. [57] Vepsäläinen et al. [58]	Computational fluid dynamics applied to fluidised beds
Brereton and Mehravaran [59]	Submicron-particle mass transfer in laminar wall-bounded flow
Schrive et al. [60]	Liquid food pasteurisation by pulsed electric fields
Wan et al. [61]	Vertical plate channels with falling film evaporation
Pigeonneau et al. [62]	Rising bubble in liquid with chemical reaction
Dani et al. [63]	Gas–liquid mass transfer involving contaminated bubbles
Nugraha et al. [64]	Sherwood number correction due to Stefan flow around spheres
Albrand and Lalanne [65]	Flow in capillary channels

, to which the interested reader is redirected for further information.

6. Conclusions: Looking for a Synoptical Graph

The evaluation of the Sherwood number in chemical engineering applications passes through the correct definition of the system under examination. For example, attention must be paid to the quantities that appear in the Reynolds number, in the Sherwood and Schmidt numbers, and to the fields of validity of the relations proposed in the literature, starting from the oldest works (in the 1930s) up to the present day.

However, in this review, it has been noticed that many cases can be described by the general equation ${\displaystyle \frac{Sh-\alpha }{\beta {Sc}^{\sigma }}}={Re}^{\lambda }$ (i.e., Equation (12)), where the value of $\lambda$ gives an indication of the dependency of the mass transfer coefficient on the ratio between inertial and viscous forces.

The graph that is now proposed, shown in Figure 9, shows (with the aid of an illustrative legend) in a synoptical key the relationships for the cases describable using Equation (12).

While apologising to readers for any oversights, the author hopes that this tool can be of help for the determination, in applications that require it, of the mass transfer coefficient to correctly define the design and operating conditions of the system.

7. Future Directions

Detailed aspects concerning the relation between the Sherwood number and, e.g., scaling, mass transfer efficiency, and analogies with other dimensionless numbers have been dealt with in textbooks and papers referred to in this work, and they are outside the scope of this brief review. Nonetheless, some particular aspects representing potential future directions are listed below.

In general, the validity of the spherical particle assumption should be checked (otherwise, particle shape effects should be considered).

The development of models and equations able to take into account, when relevant, thermal effects (e.g., non-isothermal systems) is suggested; in general, it appears important to consider the role of the simultaneous build-up of boundary layers in terms of momentum, heat, and mass transfer in cases where this cannot be neglected.

In fluidised beds, the changes in the effective fluid-dynamics (when switching from bench to pilot and industrial scale) should be carefully considered for checking the validity of the proposed relations.

Expressions for absorption in the presence of a chemical reaction should be more widely developed.

In the case of trapped gases in porous media, the possibility that the approach can be valid also in presence of a liquid, the inclusion of the effect of neighbouring particles (when the solids concentration is high), and the role of the reaction kinetics and network assume relevant interest for practical applications.