Electric-Thermal Analysis of Power Supply Module in Graphitization Furnace
Abstract
1. Introduction
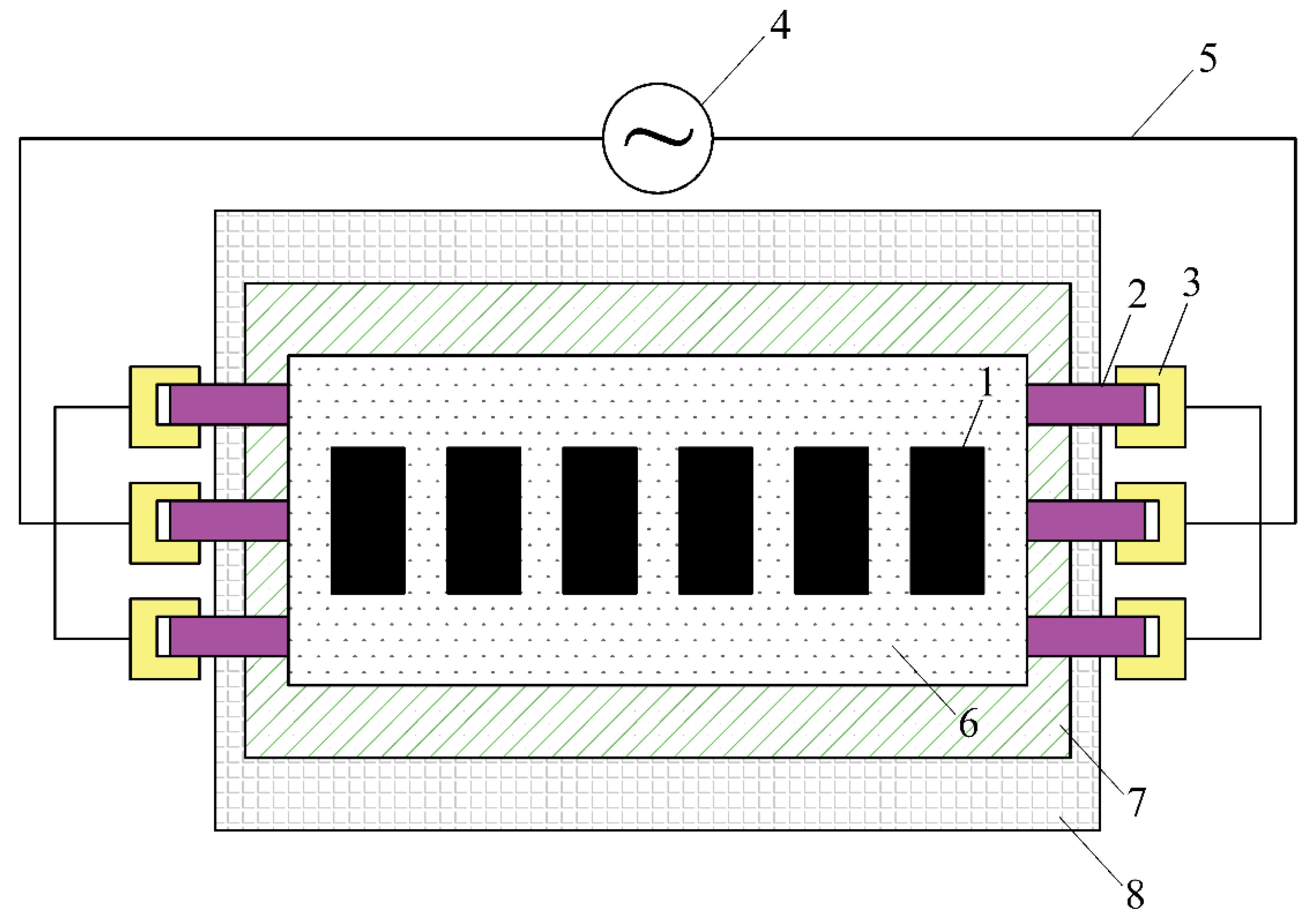
2. Research Subject and Mathematical Model
2.1. Research Subject
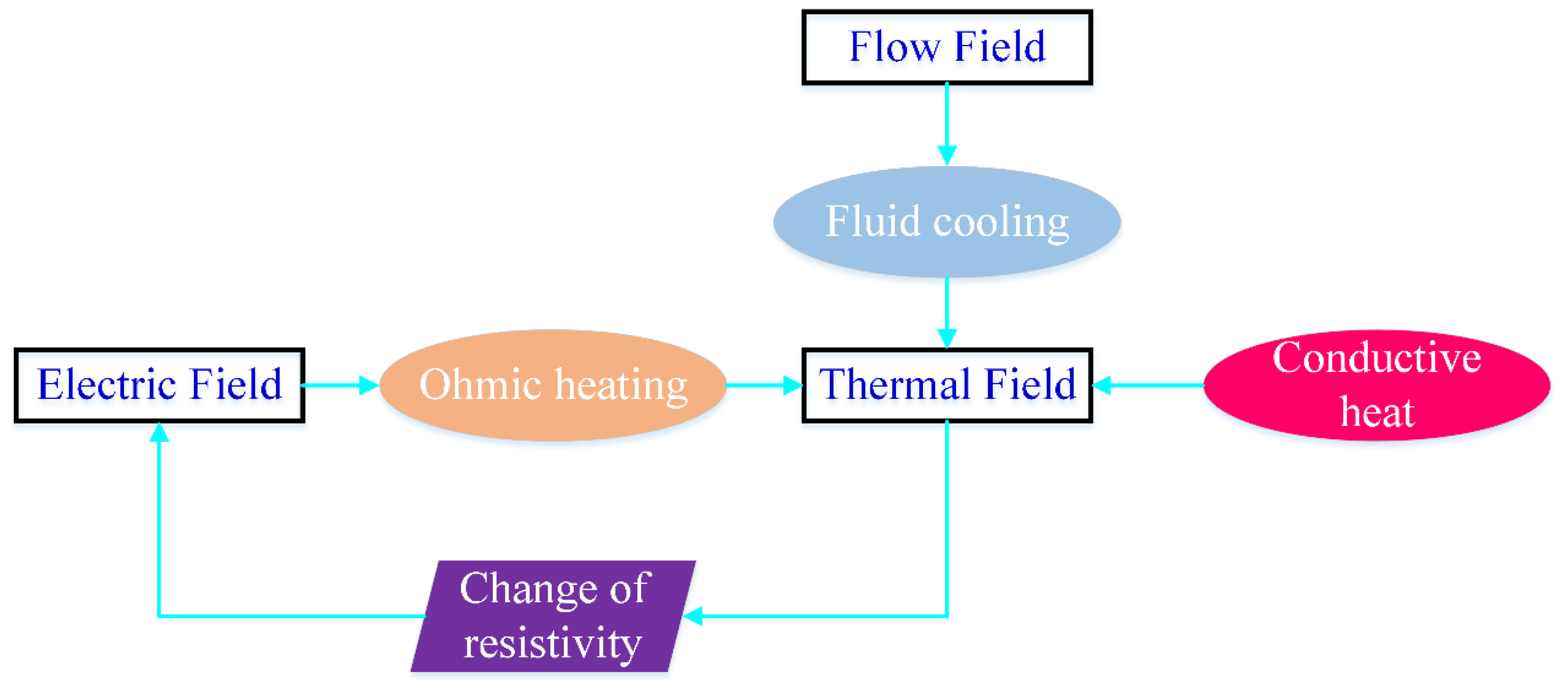
2.2. Electric-Thermal-Fluid Coupling Process
2.3. Mathematical Model
- (1)
- Electric Field Governing Equation
- (2)
- Fluid Field Governing Equation
- (3)
- Thermal Field Governing Equation
3. Numerical Simulation and Validation
3.1. Mesh and Material Properties
3.2. Model Setup and Solving
3.2.1. Initial Conditions
- (1)
- Ambient temperature: The entire computational domain is initialized at an ambient temperature of 20 °C.
- (2)
- Fluid flow: The fluid domain is initialized with a velocity of 0 m/s and a gauge pressure of 0 Pa.
- (3)
- Electric potential: The entire solid domain is initialized with an electrical potential of 0 V.
3.2.2. Boundary Conditions
- (1)
- Thermal boundary conditions
- (2)
- Fluid Flow Boundary Conditions
- (3)
- Electrical Boundary Conditions
3.2.3. Contact Conditions
3.2.4. Solving Setup
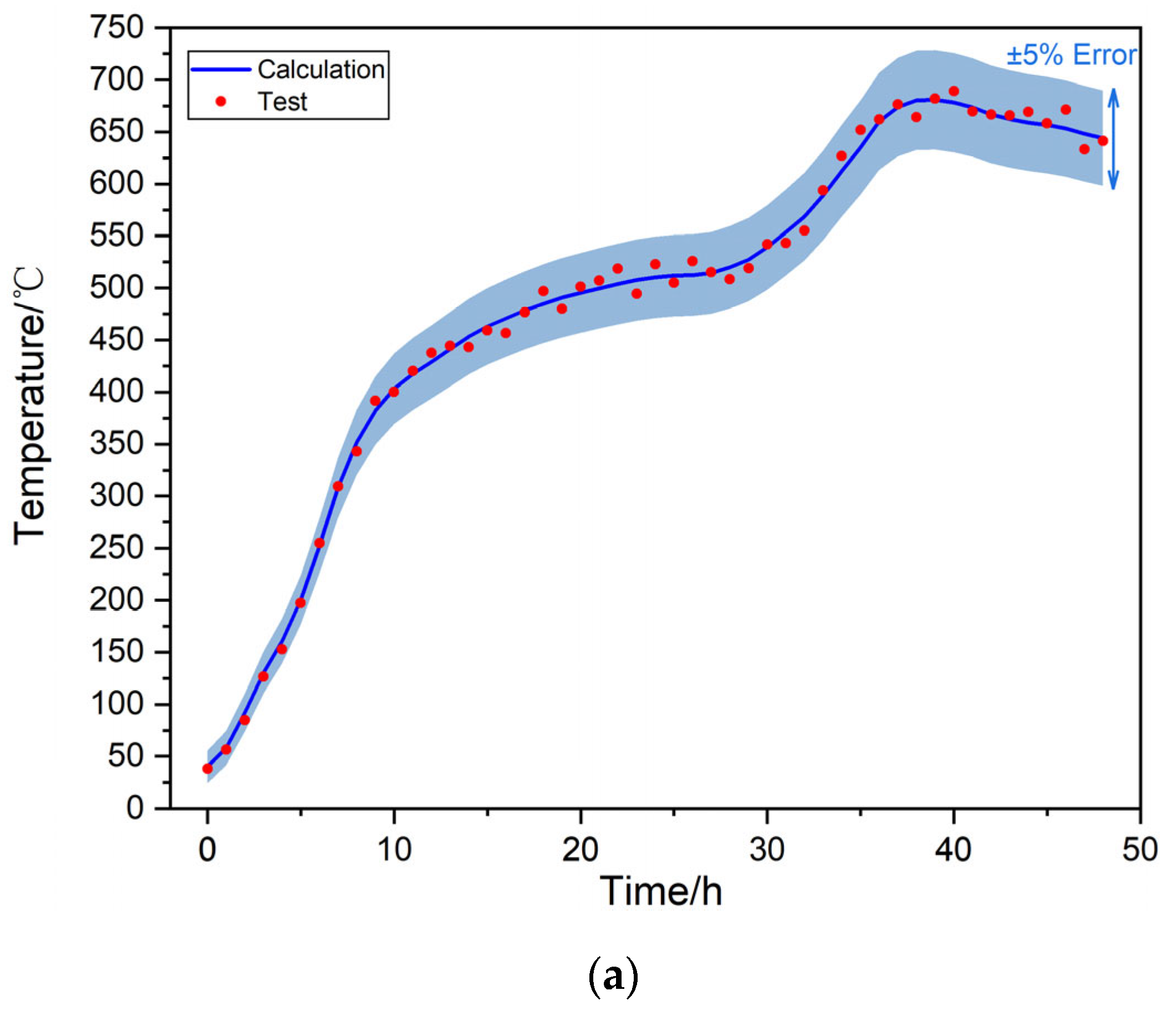
3.3. Test Validation
4. Results and Discussion
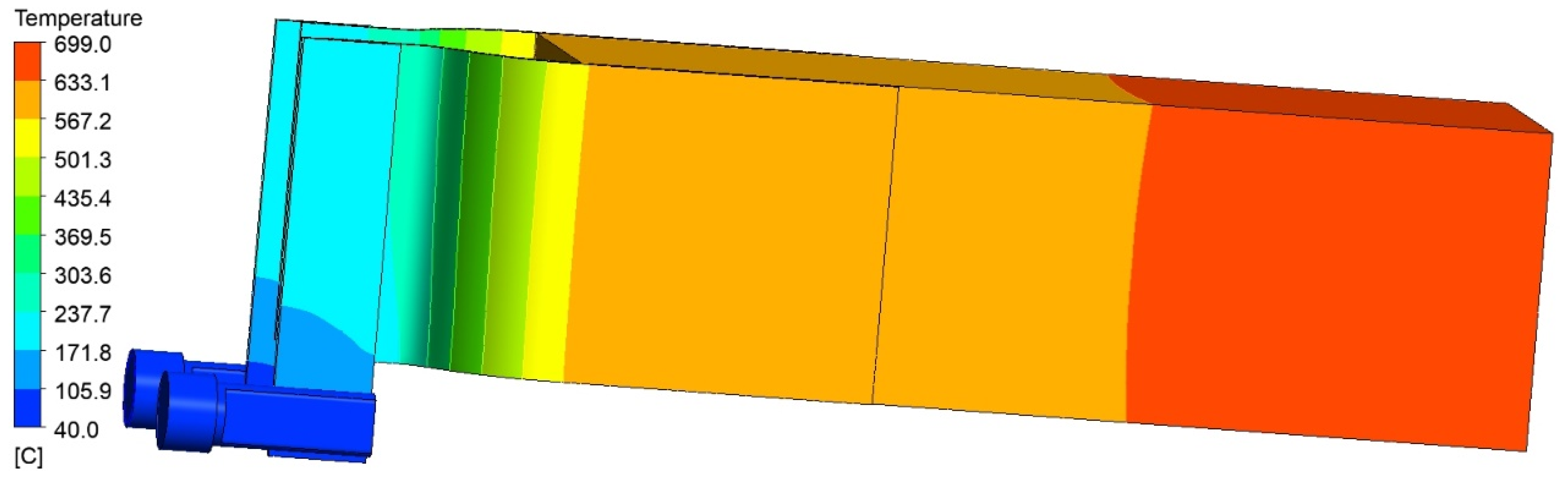
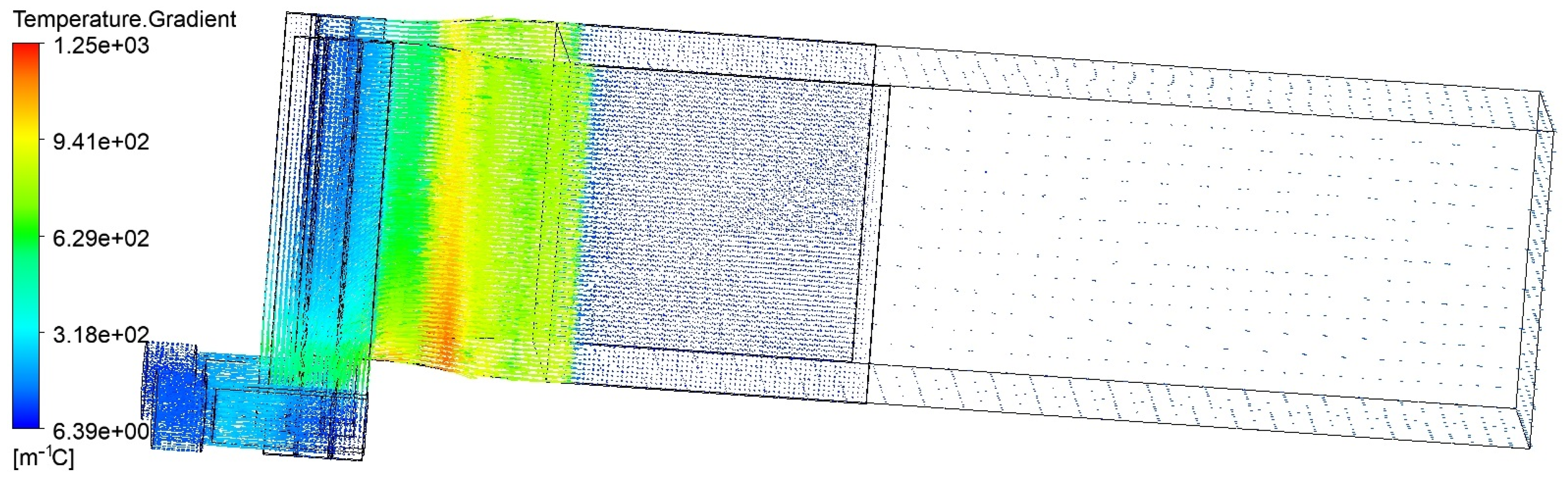
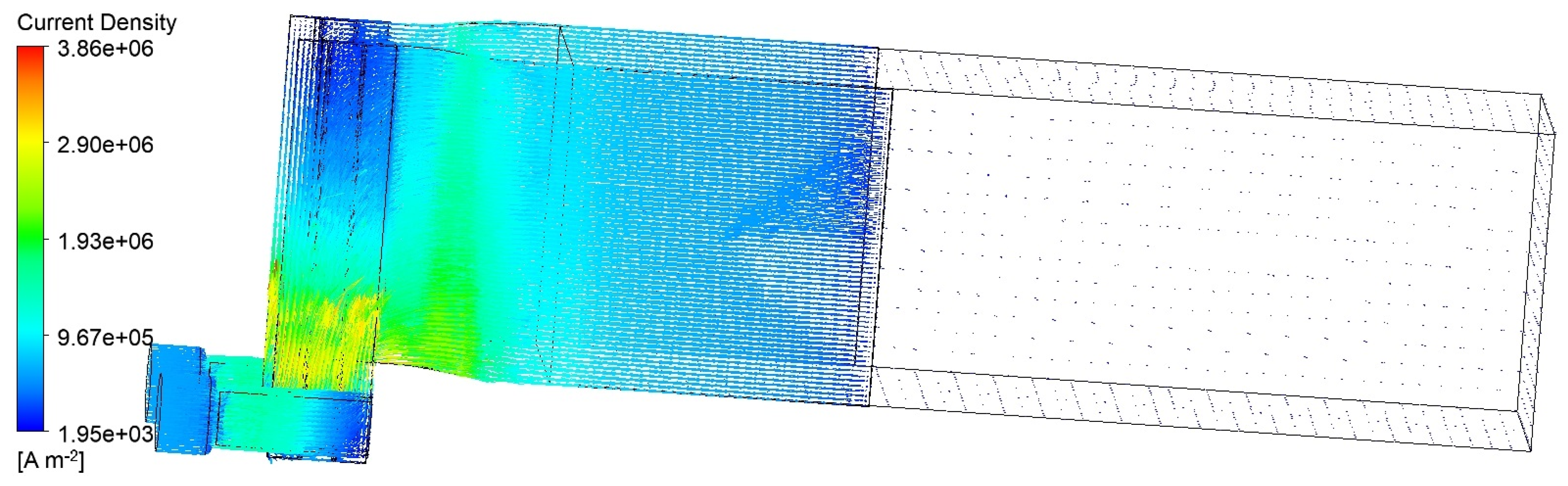
4.1. End-Time Distribution
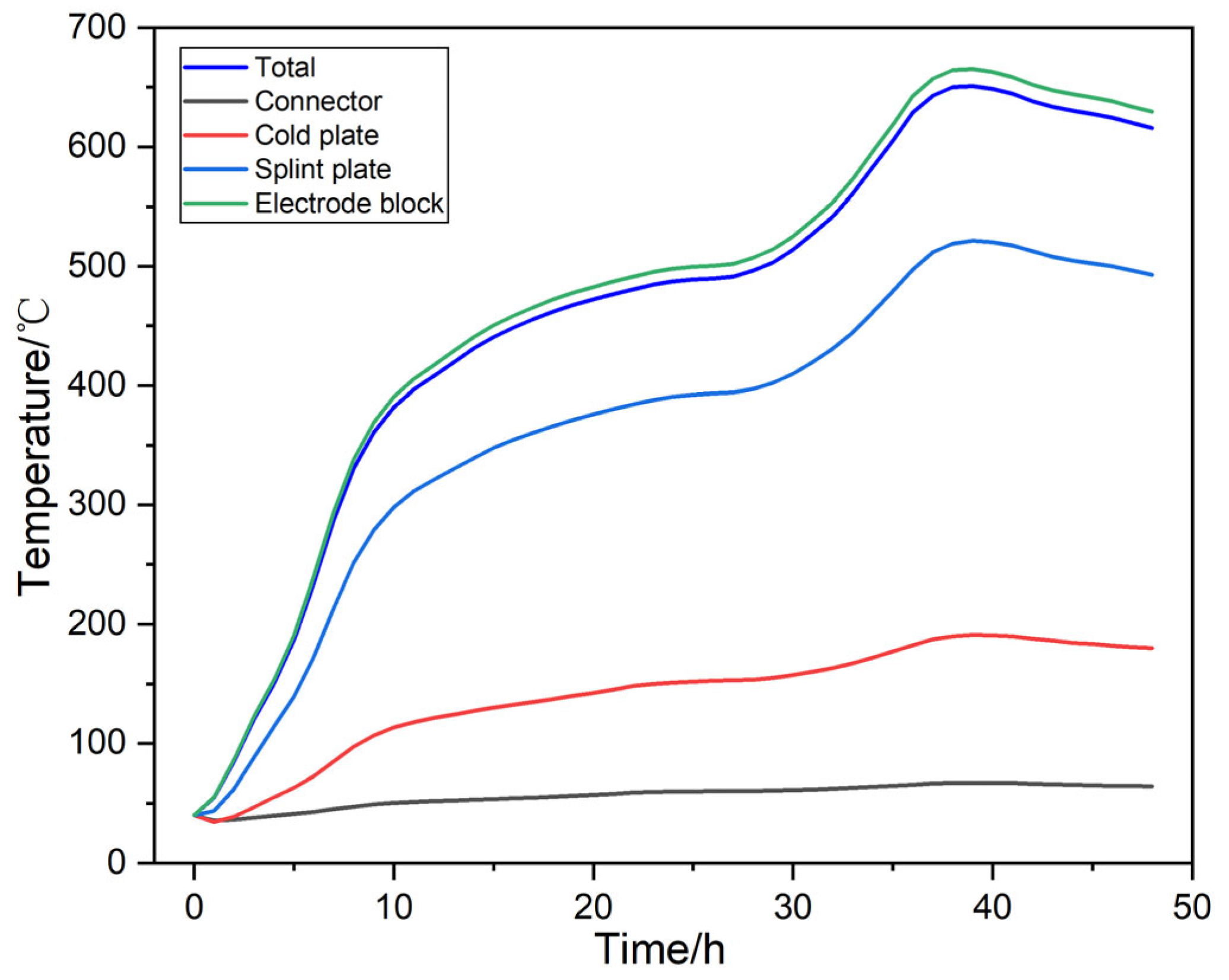
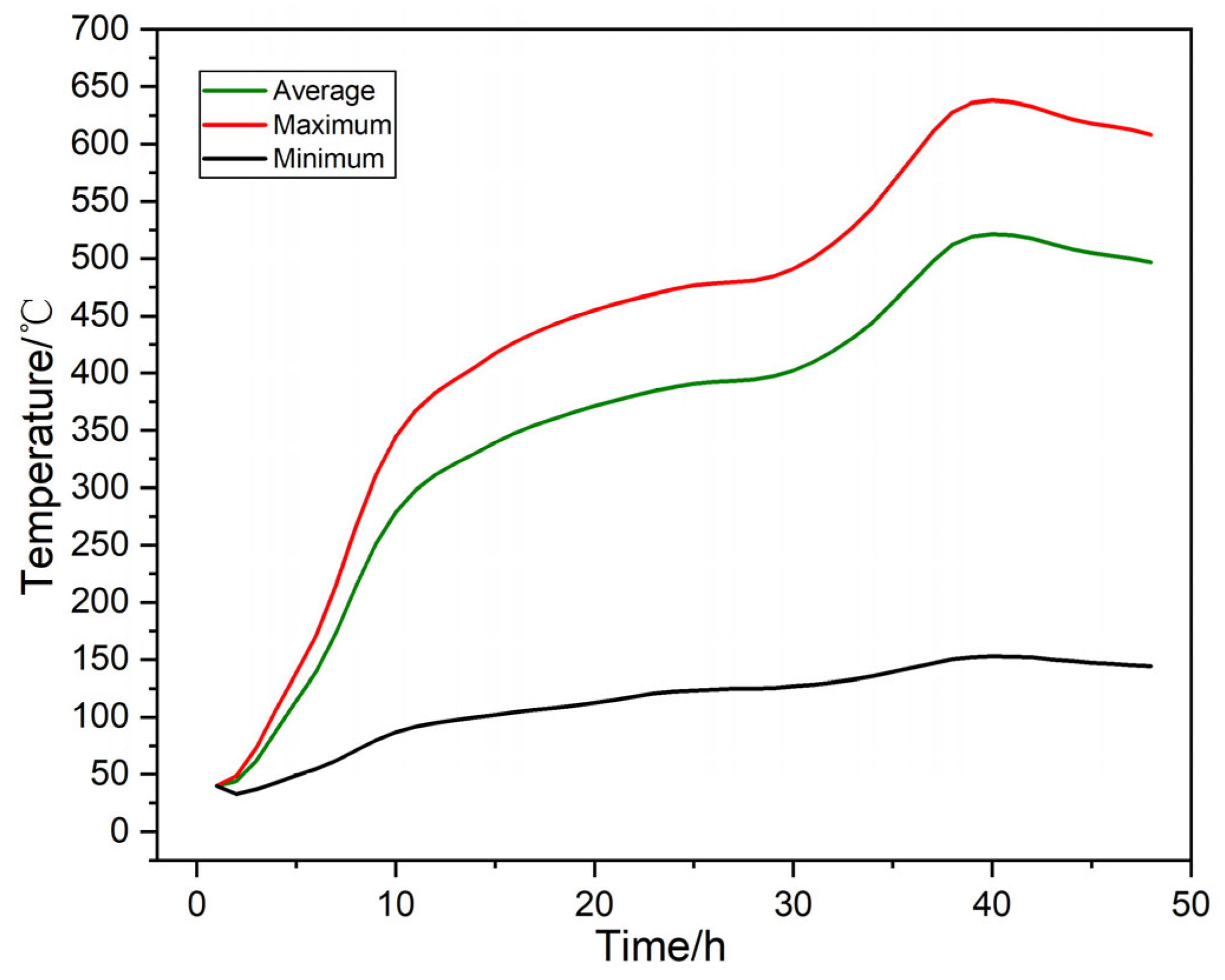
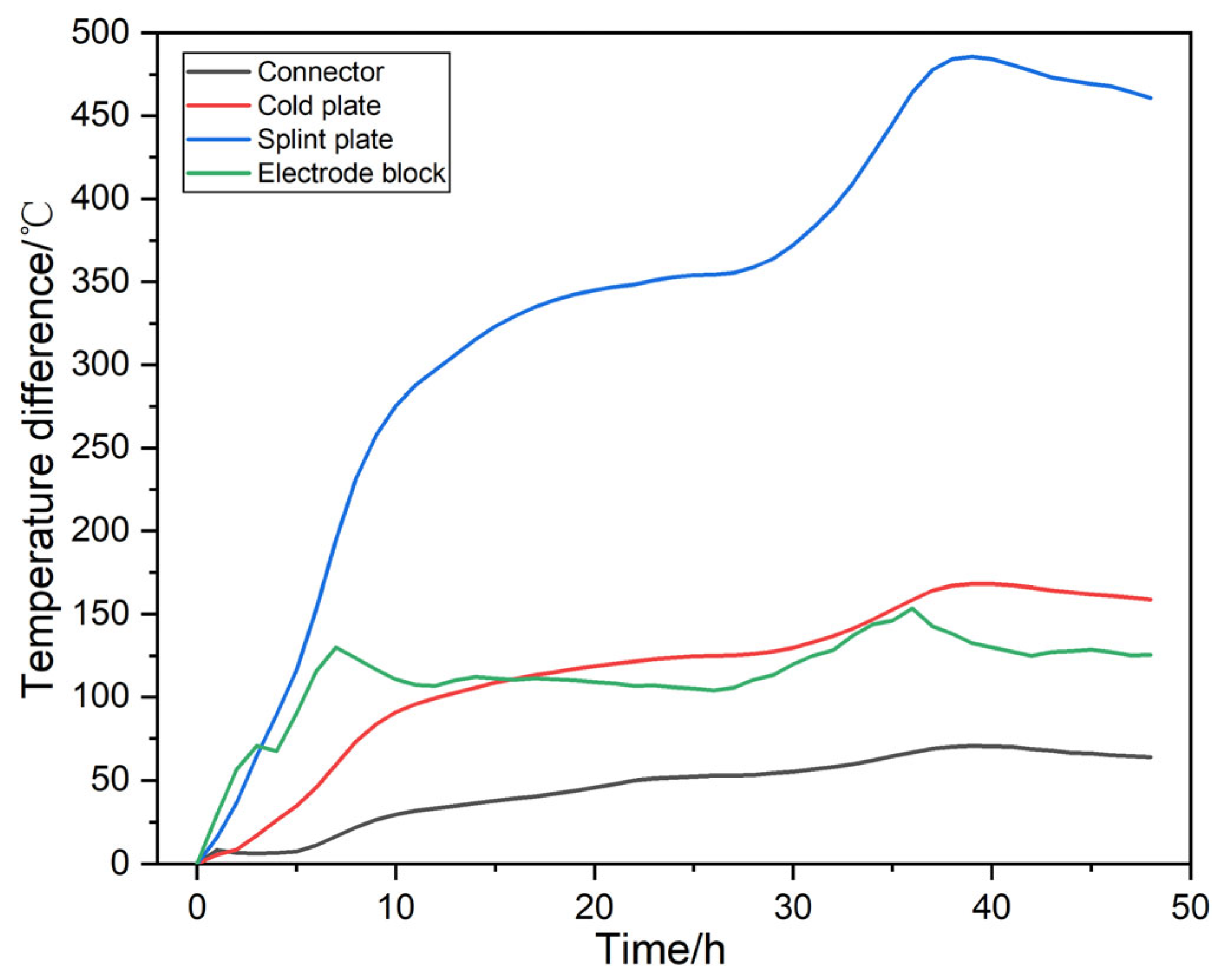
4.2. Temporal Variation of Temperature
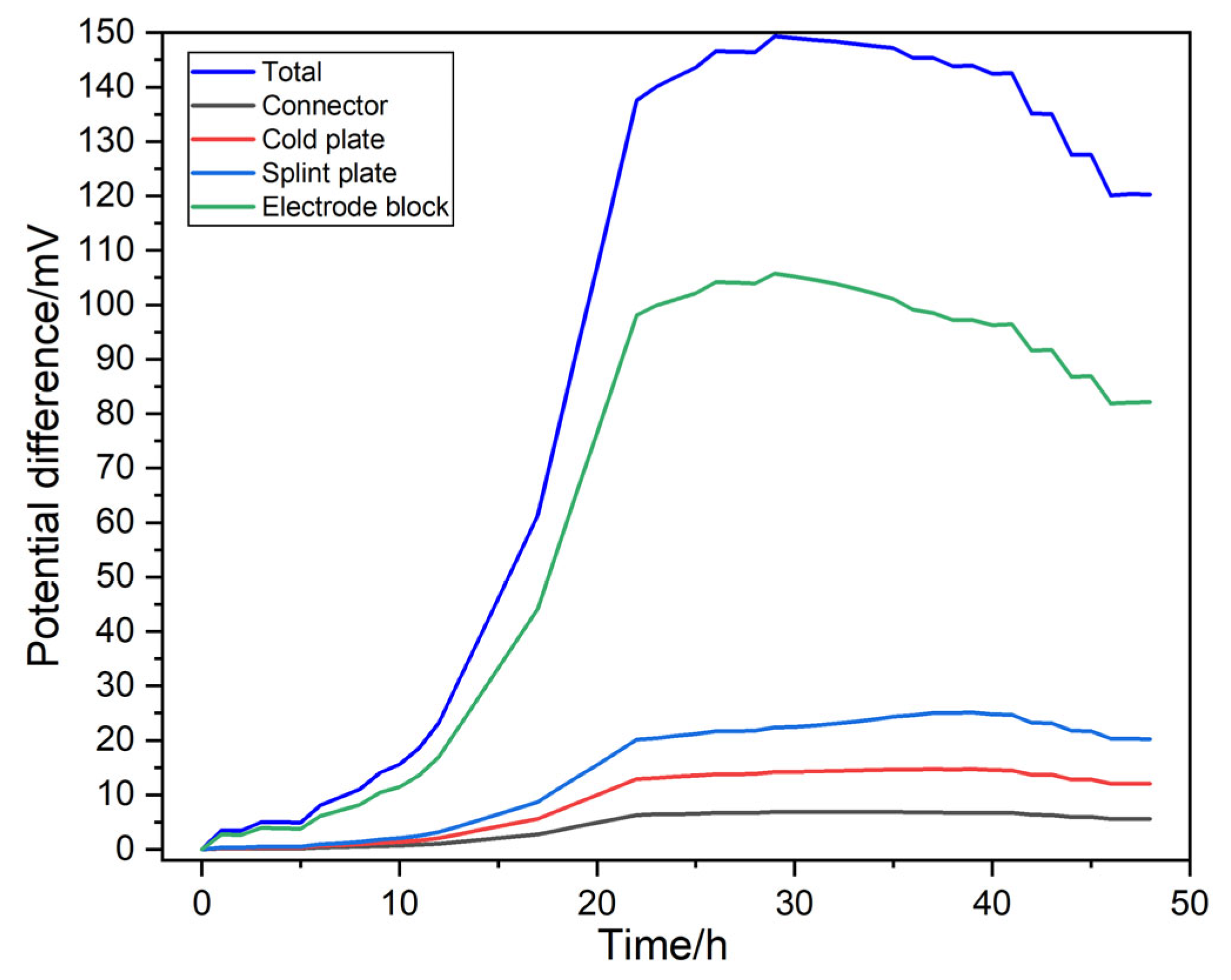
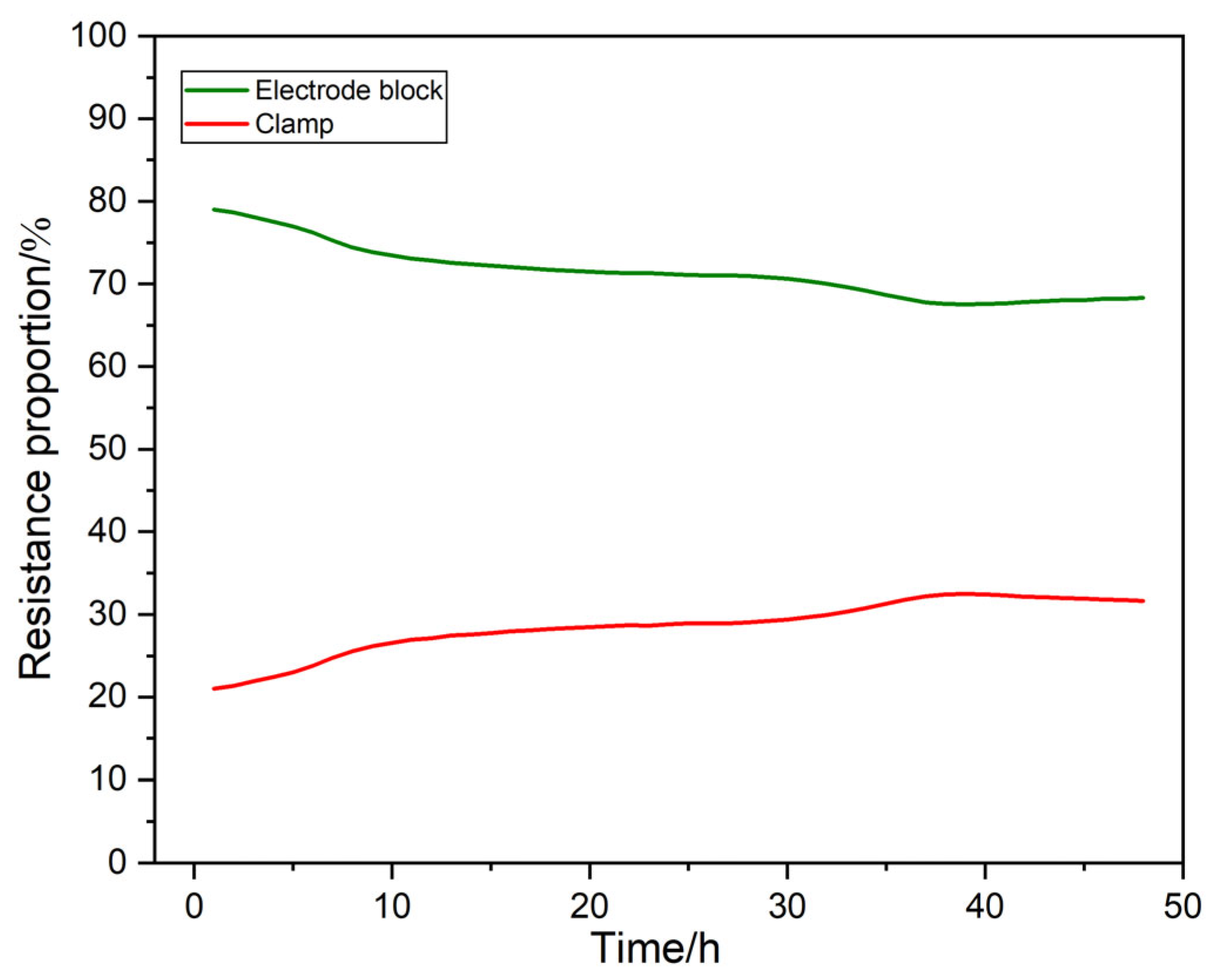
4.3. Temporal Variation of Potential Differences
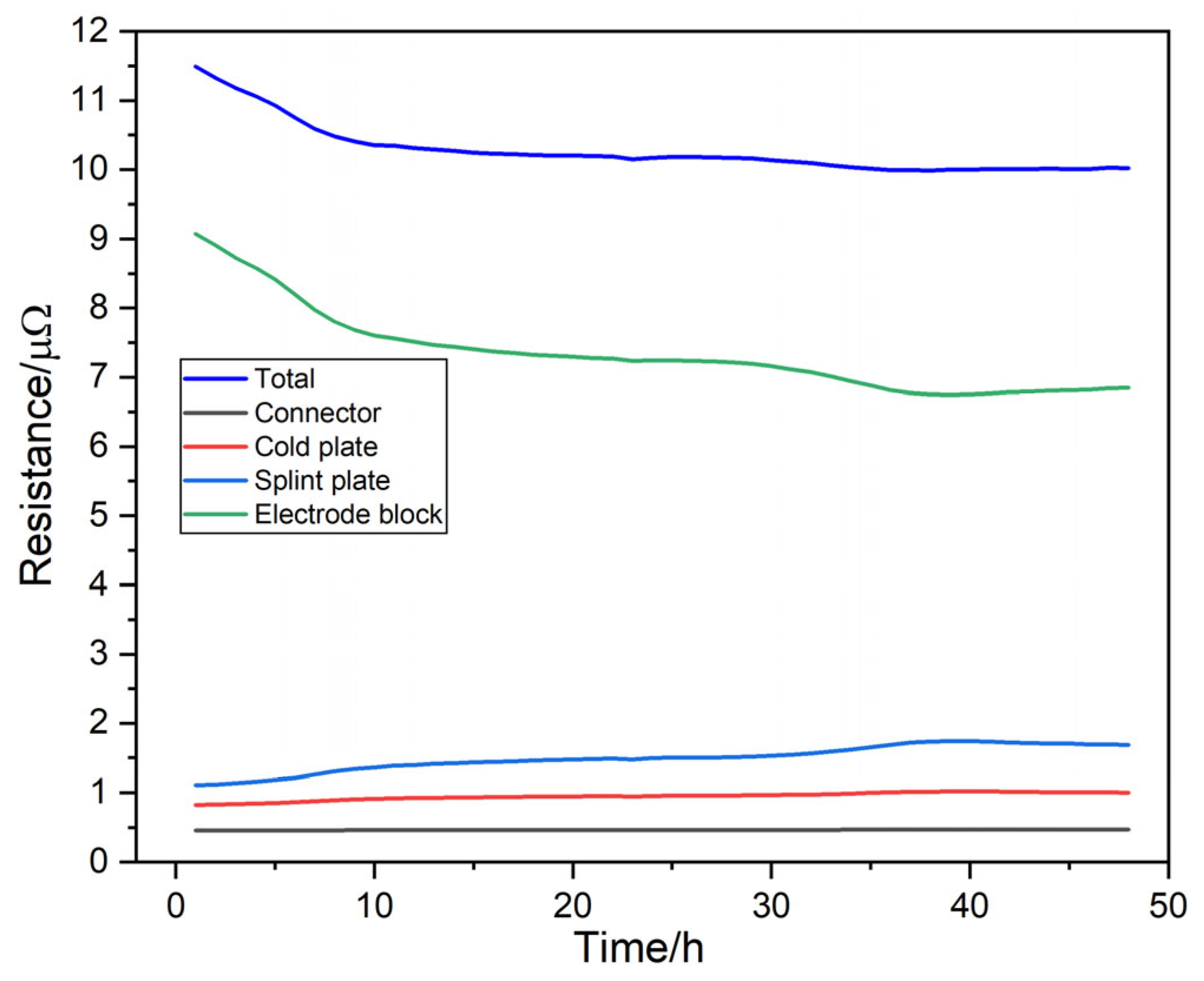
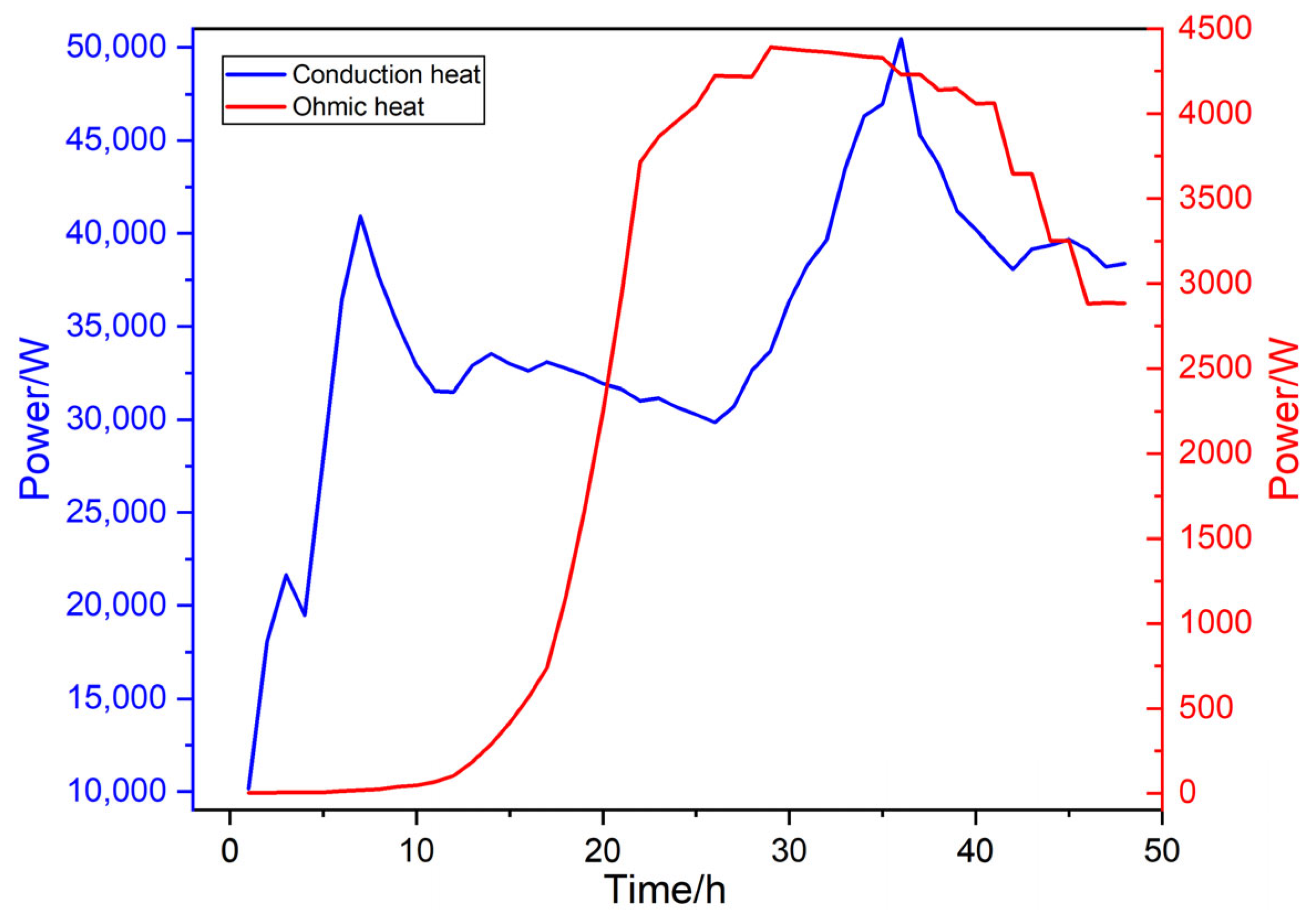
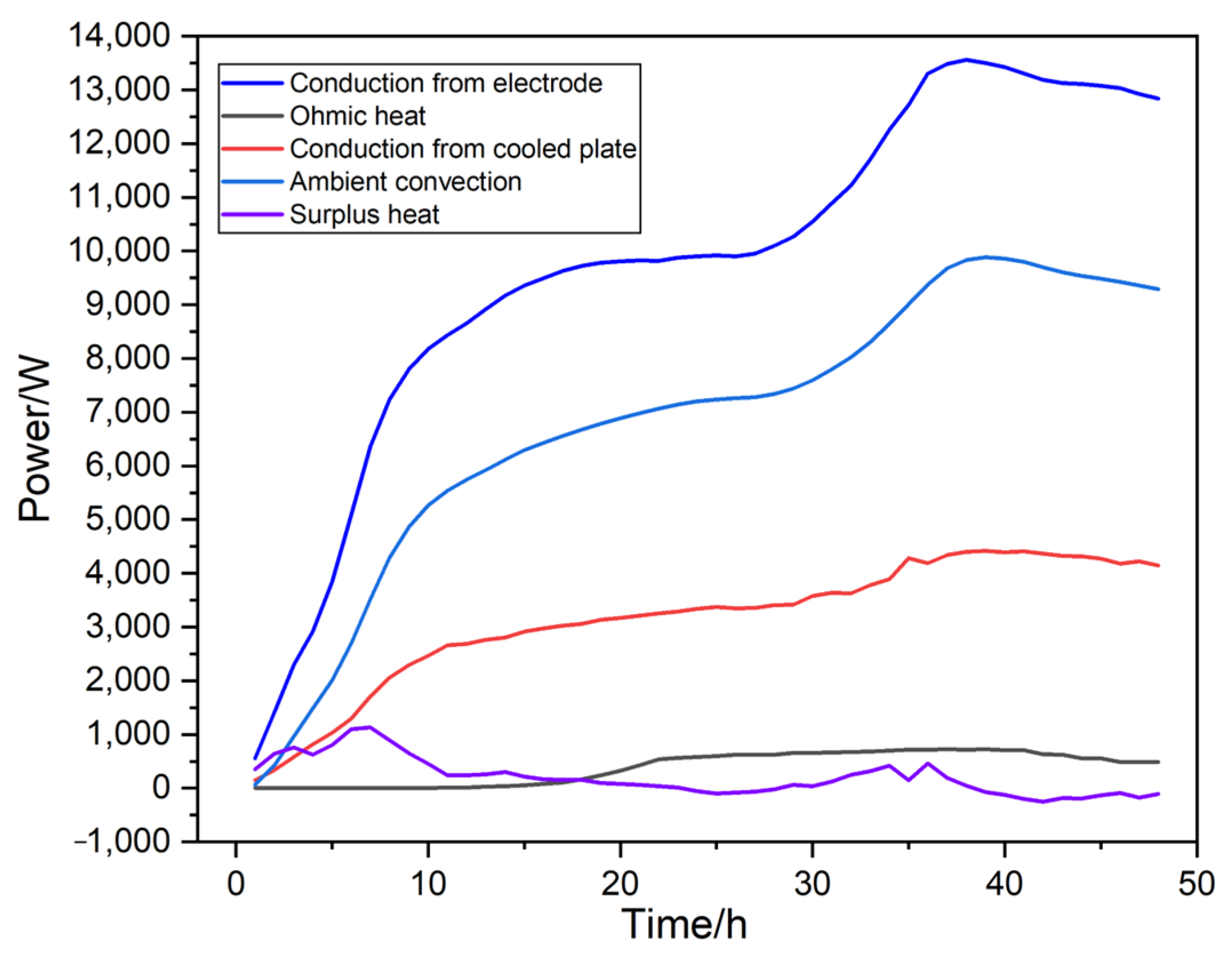
4.4. Heat Budget Analysis
4.5. Comparison with Previous Work
5. Conclusions and Future Work
5.1. Conclusions
- (1)
- Significant temperature differences and potential failure risks: The power transmission module exhibits notable temperature differences, with the highest temperature observed at the electrode block end face proximal to the furnace core and the lowest at the connector end face. Temperature gradient and current density analyses reveal elevated thermal stresses and ohmic heating at the bends of the splint plate, posing potential failure risks. Additionally, the temperature differences within the splint during graphitization are also pronounced, necessitating close monitoring to prevent thermal damage.
- (2)
- Resistance dominance and power savings: The electrode block accounts for a substantial portion of the total resistance within the power transmission module. Thus, maintaining relatively high temperatures, without compromising the splint plate, aids in keeping the total potential difference across the module at a relatively low level, thereby contributing to operational power savings.
- (3)
- Heat budget analysis and efficiency enhancement: The primary heat source for the power transmission module is thermal conduction from the furnace core through the electrode. Natural convection and forced convection via liquid cooling are the primary heat dissipation pathways. Optimizing the design of the cold plate and its liquid cooling channels, coupled with appropriate coolant flow rates and temperatures, is crucial for enhancing the energy efficiency of the graphitization process and ensuring stable operation of the power transmission clamp.
5.2. Future Work
6. Patent
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| J | [A/m3] | Current density |
| Qj | [A/m3] | Current source |
| σ | [S/m] | Electrical conductivity |
| E | [V/m] | Electric field intensity |
| φ | [V] | Electric potential |
| V | [m/s] | Velocity vector |
| ρl | [kg/m3] | Fluid density |
| p | [Pa] | Pressure |
| μ | [Pa·s] | Viscosity |
| g | [m/s2] | Gravitational acceleration |
| ρ | [kg/m3] | Density |
| c | [J/(kg·K)] | Specific heat capacity |
| T | [K] | Temperature |
| t | [s] | Time |
| λ | [W/(m·K)] | Thermal conductivity |
| Sφ | [W/m3] | Heat source |
| q | [W/m2] | Heat flux density |
| h | [W/(m2·K)] | Convection heat transfer coefficient |
| ΔT | [K] | Temperature difference |
| L(T) | [W/(m·K)] or [S/m] | Thermal conductivity (or electrical conductivity) at temperature T |
| T0 | [K] | Reference temperature |
| L0 | [W/(m·K)] or [S/m] | Thermal conductivity (or electrical conductivity) at the reference temperature |
| α | [K−1] | Temperature coefficient |
References
- Goodenough, J.B. How we made the Li-ion rechargeable battery. Nat. Electron. 2018, 1, 204. [Google Scholar] [CrossRef]
- Kumar, S.; Rao, S.K.; Singh, A.R.; Naidoo, R. Switched-resistor passive balancing of li-ion battery pack and estimation of power limits for battery management system. Int. J. Energy Res. 2023, 2, 1–21. [Google Scholar] [CrossRef]
- Das Goswami, B.R.; Mashayek, F.; Yurkiv, V.; Mastrogiorgio, M.; Ragone, M.; Jabbari, V.; Shahbazian-Yassar, R. A combined multiphysics modeling and deep learning framework to predict thermal runaway in cylindrical Li-ion batteries. J. Power Sources 2024, 595, 234065. [Google Scholar] [CrossRef]
- Williams, D.; Copley, R.; Bugryniec, P.; Dwyer-Joyce, R.; Brownb, S. A review of ultrasonic monitoring: Assessing current approaches to Li-ion battery monitoring and their relevance to thermal runaway. J. Power Sources 2024, 590, 233777. [Google Scholar] [CrossRef]
- Gomez, W.; Wang, F.X.; Chou, J.H. Li-ion battery capacity prediction using improved temporal fusion transformer model. Energy 2024, 296, 131114. [Google Scholar] [CrossRef]
- Ly, S.; Sadeghi, M.A.; Misaghian, N.; Fathiannasab, H.; Gostick, J. Rapid prediction of particle-scale state-of-lithiation in Li-ion battery microstructures using convolutional neural networks. Appl. Energy 2024, 360, 122803. [Google Scholar] [CrossRef]
- Jordan, S.; Schreiber, C.; Parhizi, M.; Shah, K. A new multiphysics modeling framework to simulate coupled electrochemical-thermal-electrical phenomena in Li-ion battery packs. Appl. Energy 2024, 360, 122746. [Google Scholar] [CrossRef]
- Nzereogu, P.; Omah, A.D.; Ezema, F.I.; Iwuoha, E.I.; Nwanya, A.C. Anode materials for lithium-ion batteries: A review. Appl. Surf. Sci. Adv. 2022, 9, 100233. [Google Scholar] [CrossRef]
- Zhang, H.; Yang, Y.; Ren, D.; Wang, L.; He, X. Graphite as anode materials: Fundamental mechanism, recent progress and advances. Energy Storage Mater. 2021, 36, 147–170. [Google Scholar] [CrossRef]
- Hossain, M.H.; Chowdhury, M.A.; Hossain, N.; Islam, M.A.; Mobarak, M.H. Advances of lithium-ion batteries anode materials—A review. Chem. Eng. J. Adv. 2023, 16, 100569. [Google Scholar] [CrossRef]
- Asenbauer, J.; Eisenmann, T.; Kuenzel, M.; Kazzazi, A.; Chen, Z.; Bresser, D. The success story of graphite as a lithium-ion anode material–fundamentals, remaining challenges, and recent developments including silicon (oxide) composites. Sustain. Energy Fuels 2020, 4, 5387–5416. [Google Scholar] [CrossRef]
- Duan, S.; Wu, X.; Wang, Y.; Feng, J.; Hou, S.; Huang, Z.; Shen, K.; Chen, Y.; Liu, H.; Kang, F. Recent progress in the research and development of natural graphite for use in thermal management, battery electrodes and the nuclear industry. New Carbon Mater. 2023, 38, 73–91. [Google Scholar] [CrossRef]
- Wang, X.; Li, H.; Yao, H.; Chen, Z.; Guan, Q. Network feature and influence factors of global nature graphite trade competition. Resour. Policy 2019, 60, 153–161. [Google Scholar] [CrossRef]
- Sun, C.; Shen, S.; Wang, W.; Yuan, G.; Huang, Z.; Zhang, Y. High quality development of graphite resource and graphite material industry. Strateg. Study CAE 2022, 24, 29–39. [Google Scholar] [CrossRef]
- Qiu, T.; Yu, Z.Y.; Xie, W.N.; He, Y.Q.; Wang, H.F.; Zhang, T. Preparation of Onion-like Synthetic Graphite with a Hierarchical Pore Structure from Anthracite and Its Electrochemical Properties as the Anode Material of Lithium-Ion Batteries. Energy Fuels 2022, 36, 8256–8266. [Google Scholar] [CrossRef]
- Favreau, H.J.; Miroshnichenko, K.; Solberg, P.C.; Tsukrov, I.; Van Citters, D.W. Shear enhancement of mechanical and microstructural properties of synthetic graphite and ultra-high molecular weight polyethylene carbon composites. J. Appl. Polym. Sci. 2022, 139, 52175. [Google Scholar] [CrossRef]
- Belenchenko, V.M. Title of simulation of the physicochemical processes of high-temperature treatment of carbon products in Acheson furnaces. Soild Fuel Chem. 2009, 43, 43–49. [Google Scholar] [CrossRef]
- Bahl, O.P.; Chauhan, B.S. Anomalous behaviour of a small laboratory acheson graphitization furnace. Carbon 1974, 12, 214–216. [Google Scholar] [CrossRef]
- Li, R.; Zhang, Y.Y.; Chu, X.D.; Gan, L.; Li, J.; Li, B.H.; Du, H.D. Design and Numerical Study of Induction-Heating Graphitization Furnace Based on Graphene Coils. App. Sci. 2024, 14, 2528. [Google Scholar] [CrossRef]
- Zhang, X.J. Reasons analysis and measure for crack waste products in acheson furnace during graphitization. CarbonTech 2008, 27, 45–47. [Google Scholar]
- Gun, L.Y.; Wen, Z.; Dou, R.F.; Yang, P.P. Acheson graphitization furnace and the simulation of temperature distribution. Energy Metall. Ind. 2012, 31, 28–32+38. [Google Scholar]
- Yao, J. On Energy Efficiency Saving of Acheson Graphitization Furnace. Gansu Metall. 2013, 35, 42–47. [Google Scholar]
- Shang, T.; Zhan, H.; Gong, Q.; Zeng, T.; Li, P.; Zeng, Z. Insights into the thermal and electric field distribution and the structural optimization in the graphitization furnace. Energy 2024, 297, 131269. [Google Scholar] [CrossRef]
- Matizamhuka, W.R. Gas transport mechanisms and the behaviour of impurities in the Acheson furnace for the production of silicon carbide. Heliyon 2019, 5, e01535. [Google Scholar] [CrossRef] [PubMed]
- Kutuzov, S.V.; Buryak, V.V.; Derkach, V.V.; Panov, E.N.; Karvatskii, A.Y.; Vasil’chenko, G.N.; Leleka, S.V.; Chirka, T.V.; Lazarev, T.V. Making the Heat-Insulating Charge of Acheson Graphitization Furnaces More Efficient. Refract. Ind. Ceram 2014, 55, 15–16. [Google Scholar] [CrossRef]
- Ding, W.P.; Hao, Y. Effect of the transmission curve on the graphitization process. CarbonTech 2010, 6, 34–37. [Google Scholar]
- Gao, X.; Meng, Z.Q.; Zhou, H.A.; Shao, W. Research on the automatic control system of power distribution power of Acheson graphitization furnace. J. Guangxi Norm. Univ. 2017, 42, 547–552. [Google Scholar]
- Shen, C.; Zhang, M.; Li, X.T. Numerical study on the heat recovery and cooling effect by built-in pipes in a graphitization furnace. Appl. Therm. Eng. 2015, 90, 1021–1031. [Google Scholar] [CrossRef]
- Shen, C.; Zhang, M.; Li, X.T. Experimental investigation on the thermal performance of cooling pipes embedded in a graphitization furnace. Energy 2017, 121, 55–65. [Google Scholar] [CrossRef]
- Lan, Y.; Zhao, X.; Zhang, W.; Mu, L.; Wang, S. Investigation of the waste heat recovery and pollutant emission reduction potential in graphitization furnace. Energy 2022, 245, 123292. [Google Scholar] [CrossRef]
- Xia, X.B.; Xia, F.Q. Water-Cooled Clamp for Furnace Head Electrode. CN Patent 202320508912, 10 July 2024. [Google Scholar]
- Panton, R.L. Incompressible Flow, 4th ed.; Wiley: Hoboken, NJ, USA, 2013. [Google Scholar]
- Kulkarni, M.R.; Brady, R.P. A model of global thermal conductivity in laminated carbon/carbon composites. Compos. Sci. Technol. 1997, 57, 277–285. [Google Scholar] [CrossRef]
- Lavin, J.G.; Boyington, D.R.; Lahijani, J.; Nysten, B.; Issi, J.P. The Correlation of Thermal-Conductivity with Electric-Resistivity in Mesophase Pitch-Based Carbon-Fiber. Carbon 1993, 31, 1001–1002. [Google Scholar] [CrossRef]
- ANSYS, Inc. ANSYS Help System; ANSYS, Inc.: Canonsburg, PA, USA, 2023. [Google Scholar]

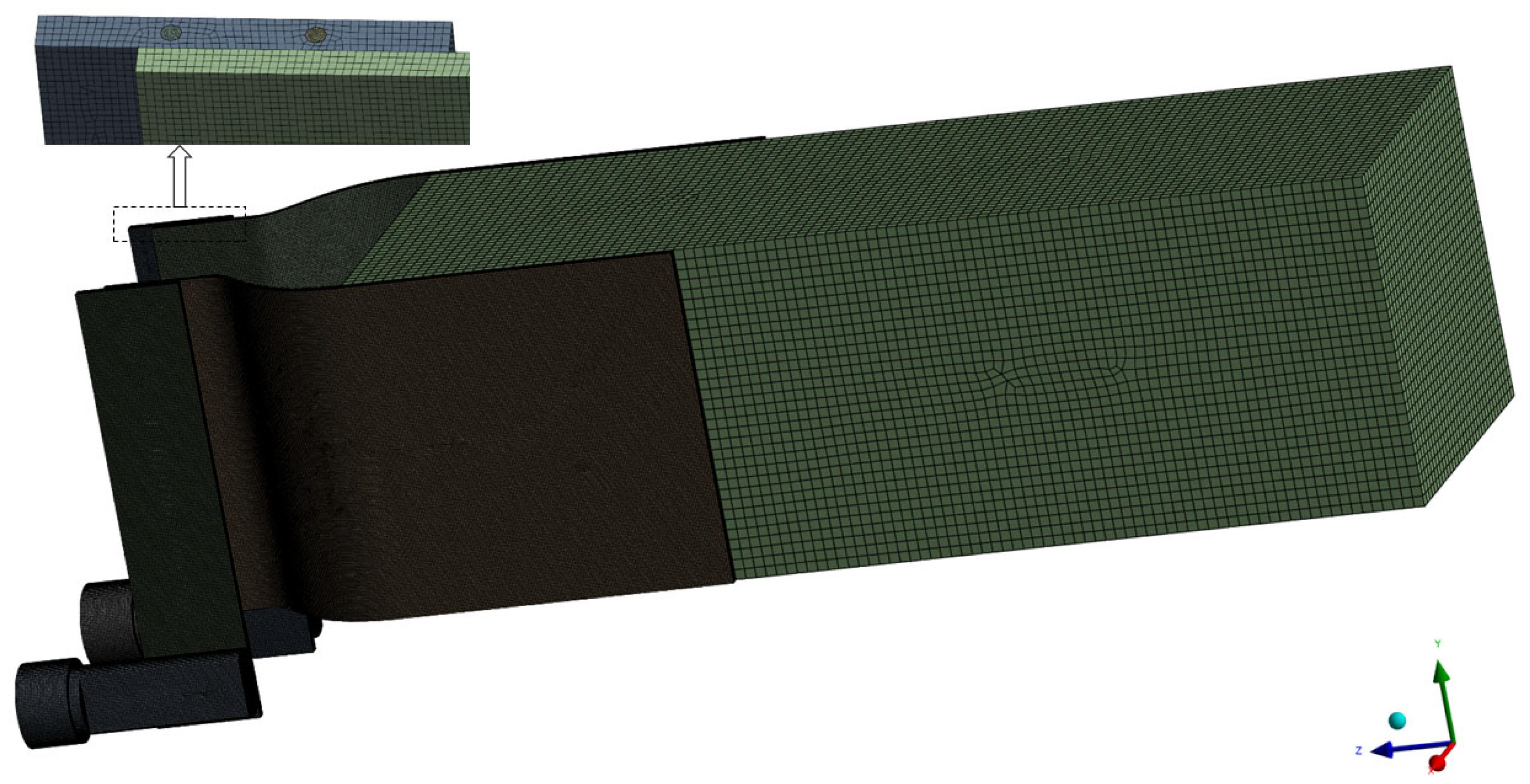






| Node Number | Element Number | Element Quality | Skewness | Orthogonal Quality | |||
|---|---|---|---|---|---|---|---|
| Average | Standard Deviation | Average | Standard Deviation | Average | Standard Deviation | ||
| 1,529,537 | 1,546,593 | 0.877 | 0.186 | 0.209 | 0.082 | 0.934 | 0.191 |
| Material | Copper Alloy | Graphite | |
|---|---|---|---|
| Density (kg·m−3) | 8933 | 2300 | |
| Specific heat capacity (J·kg−1·K−1) | 385 | 710 | |
| Thermal conductivity | Reference value (W·m−1·K−1) | 390 | 680 |
| Temperature coefficient (K−1) | −2.02 × 10−4 | 6.12 × 10−4 | |
| Electric conductivity | Reference value (S·m−1) | 5.96 × 107 | 7.53 × 105 |
| Temperature coefficient (K−1) | −8.10 × 10−4 | 6.12 × 10−4 | |
| Average Temperature | Total Current | End-Face Temperature of Electrode Block |
|---|---|---|
| Total | 0.848 | 0.999 |
| Connector | 0.914 | 0.978 |
| Cold plate | 0.883 | 0.991 |
| Splint plate | 0.856 | 0.996 |
| Electrode block | 0.847 | 0.999 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xia, X.; Li, S.; Luo, D.; Chen, S.; Liu, J.; Yao, J.; Wu, L.; Zhang, X. Electric-Thermal Analysis of Power Supply Module in Graphitization Furnace. Energies 2024, 17, 4251. https://doi.org/10.3390/en17174251
Xia X, Li S, Luo D, Chen S, Liu J, Yao J, Wu L, Zhang X. Electric-Thermal Analysis of Power Supply Module in Graphitization Furnace. Energies. 2024; 17(17):4251. https://doi.org/10.3390/en17174251
Chicago/Turabian StyleXia, Xiangbin, Shijun Li, Derong Luo, Sen Chen, Jing Liu, Jiacheng Yao, Liren Wu, and Ximing Zhang. 2024. "Electric-Thermal Analysis of Power Supply Module in Graphitization Furnace" Energies 17, no. 17: 4251. https://doi.org/10.3390/en17174251
APA StyleXia, X., Li, S., Luo, D., Chen, S., Liu, J., Yao, J., Wu, L., & Zhang, X. (2024). Electric-Thermal Analysis of Power Supply Module in Graphitization Furnace. Energies, 17(17), 4251. https://doi.org/10.3390/en17174251





