Resource-Saving Overcurrent Protection

Abstract

Construction of relay protections of electrical installations without the use of bulky metal- and insulation-intensive measuring current transformers (CTs) was repeatedly discussed at Conferences of the International Council on Large Electric Systems (CIGRE) as a challenge for the electric power industry. In the article, the authors present resource-saving reed switch current protection for 6–10-kV electrical installations connected to switchgear cells, which is an alternative to traditional protections. The scientific novelty of the work lies in (a) reed switch protection design on the basis of measuring the magnetic induction in different modes and at different points inside a cell, and we prove that inductance values are sufficient to detect phase short-circuits in electrical installations fed from this cell and derive the dependence of the induction on the position of an inductance coil inside the cell; (b) proven possibility of using the simplest formula of the Biot–Savart law for the calculations if the experimentally obtained coefficients are introduced into it; (c) development of a technique for calculating the parameters of the overcurrent protection based on reed switches. Experimental results are presented in graphical form and clearly show the points of EMS maxima and minima. Settings for resource-saving current protection are selected and a feasibility study of the effectiveness of resource-saving overcurrent protection is carried out. A technical and economical assessment of the efficiency of the suggested overcurrent protection is conducted.

1. Introduction

As is known microprocessor relay protection devices are widely used in the energy industry. The reliability of these protections is not higher than that of electromechanical or semiconductor protection systems [1,2,3,4]. The issue of resource-saving in the electric power industry has been repeatedly raised by the International Council on Large Electric Systems (CIGRE). It remains relevant for relay protection of electrical installations because of the common use of expensive, heavy and large and metal-intensive measuring current transformers (CTs) with metal cores [5,6,7,8]. Abandoning the use of traditional measuring current transformers with metal cores contributes to significant savings (conservation) of electrical and thermal energy required for their production, and this contributes to the resource-saving of the materials used. The creation of resource-saving relay protection devices against short circuits in electrical installations without the use of measuring current transformers (CT) was started in the 1960s [9,10,11,12,13,14,15,16,17,18,19] and remains a topical problem today. In addition, abandoning the use of measuring current transformers improves the reliability of relay protection systems. As an alternative to traditional relay protection systems, new devices are created on different elemental bases, for example, magnetic field sensors, such as magnetotransistors [20], magnetodiodes [20], magnetoresistors [21,22], Hall sensors [22], Rogowski coils [23,24] and reed switches [25]. Reed switches have several advantages over other magnetosensitive elements [26,27,28].

Principles of construction of reed switch current protections have been already developed. However, these principles cannot always be used without additional research because of the variety of arrangements of, for example, current-carrying busbars in switchgear cells supplying electrical installations to be protected [29,30,31]. Since reed switches are fixed inside a cell and respond to the sum of magnetic fields produced by currents in one of its current-carrying busbars opposite which it is mounted and in the current-carrying busbars of neighboring phases of this and neighboring cells, which are sources of interference, there is a need to study magnetic fields at the points where reed switches are located.

In this work, an attempt is made to solve these problems. We experimentally study three-phase, two-phase and single-phase short circuits in switchgear cells. To detect magnetic fields from current-carrying busbars, we measure the coil values of an intermediate MKU-48 type relay [32] without an internal metal core; they act as magnetic field sensors. Current protection is designed on the basis of reed switches as an alternative to traditional protections for 6–10-kV electrical installations connected to switchgear cells. Resource saving of this protection is provided by cost and weight and size parameters of reed switches, since these elements are an order of magnitude cheaper, lighter and smaller than current transformers [8,27]. The selection of settings for the suggested protection can be performed in the same way as for traditional protections.

The aim of this experimental research is the development of a resource-saving current protection based on inductive coils and reed switches without the use of traditional measuring current transformers with metal cores for protecting electrical installations connected to switchgear cells of 6–10 kV voltage. The choice in favor of such protections is because traditional protections use expensive, heavy, and metal-intensive measuring current transformers. To achieve this aim, it is necessary to perform laboratory experiments with a switchgear cell. The efficiency of the presented resource-saving protection is confirmed by the calculation of protection trip settings, checking its sensitivity coefficient, and the comparison of this protection with traditional protections.

The work includes the following sections: the Materials and Methods, which presents the means used to achieve the aim; the Experimental Section, which describes the performed experiments; the Results, which considers the results of all performed experiments including the graphical form, calculation of the field damping coefficient, correction factor, and phase coefficient necessary to select the protection settings, the use of reed switches in the relay protection, and an example of a Resource-Saving Reed Switch Current Protection; the Conclusions; the Patents (developed); Appendix A, which provides an example of the selection of settings for the proposed overcurrent protection; Appendix B, with the technical and economical confirmation of the efficiency of the resource-saving overcurrent protection; and the References, with the list of sources used.

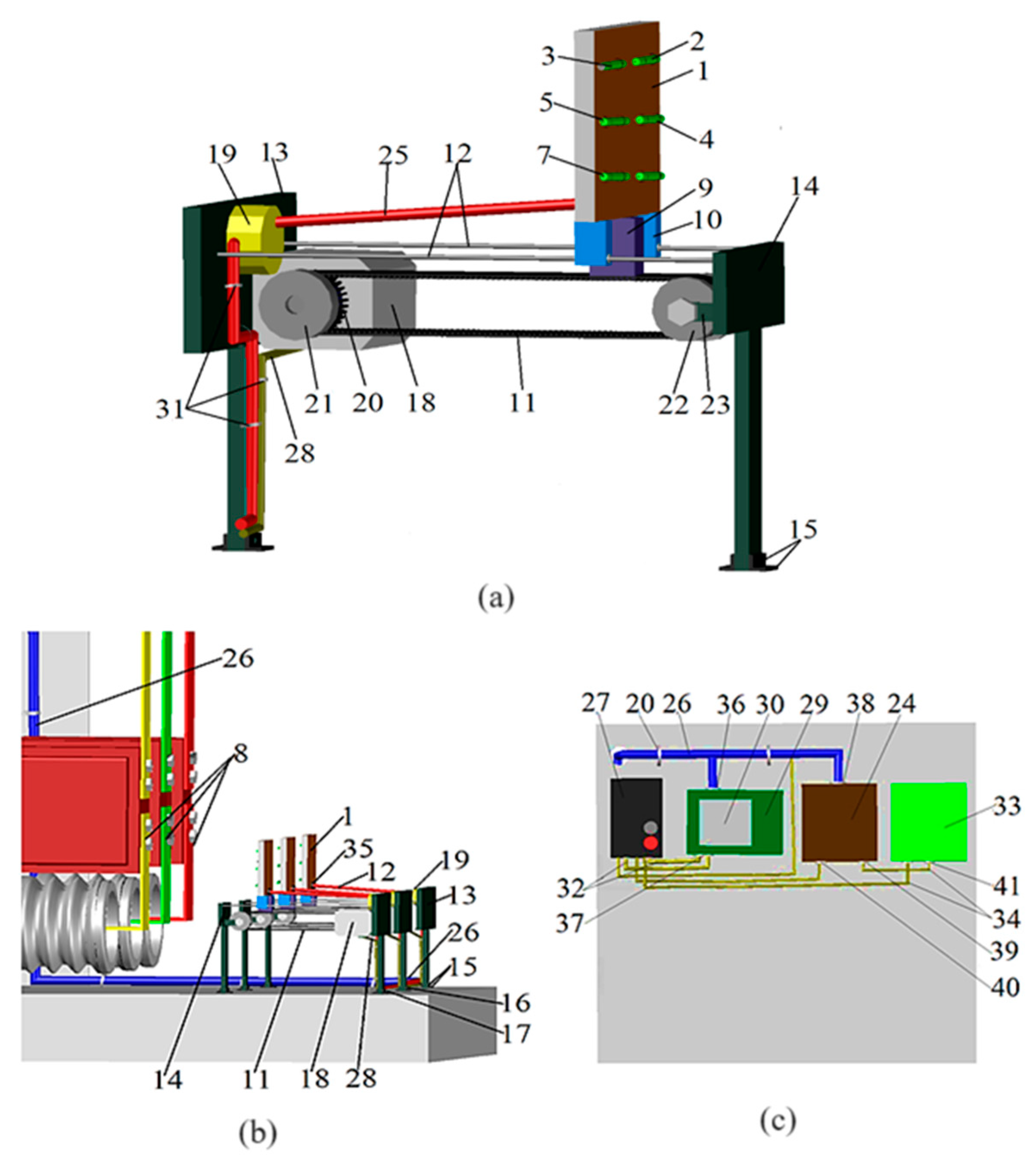
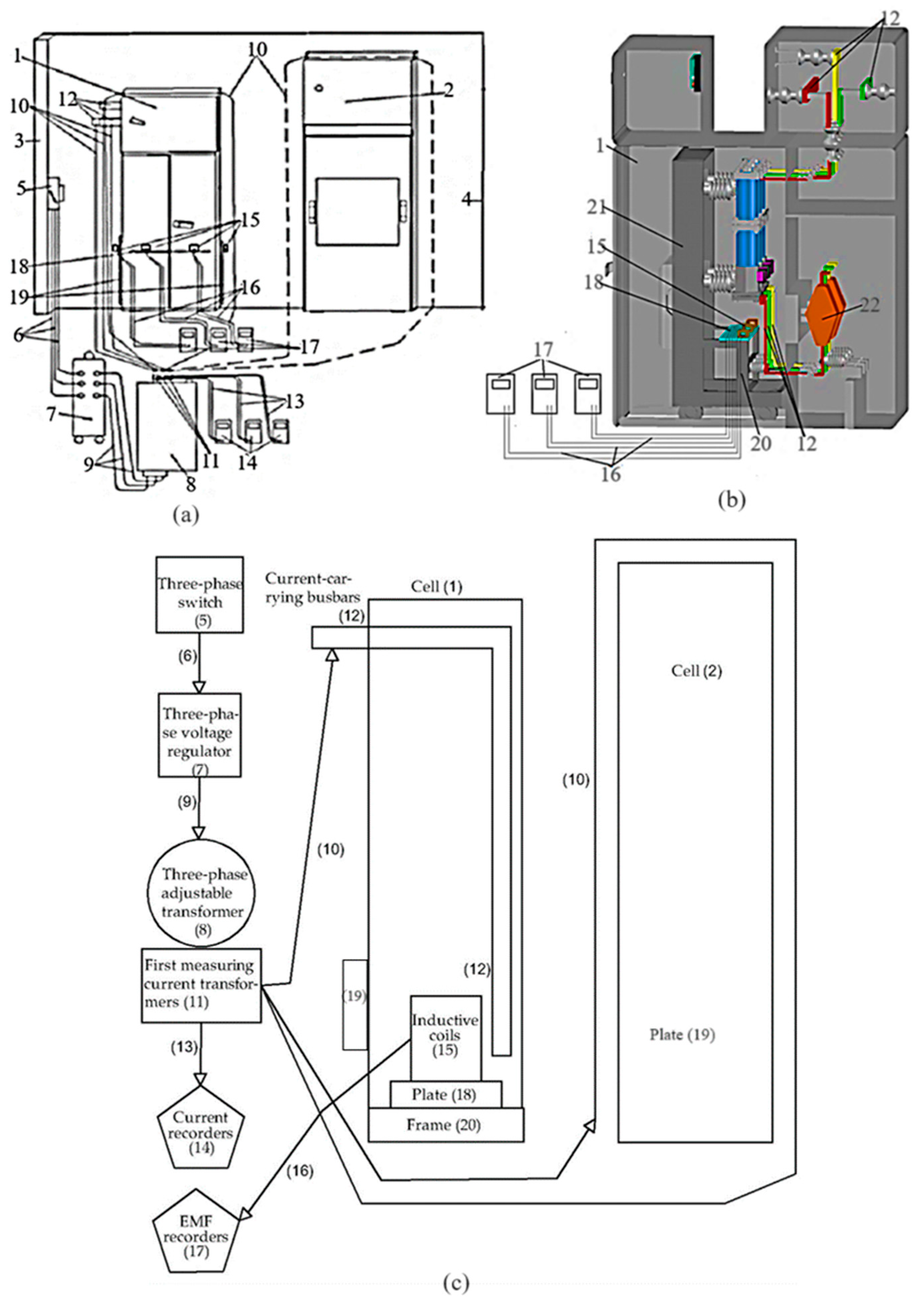
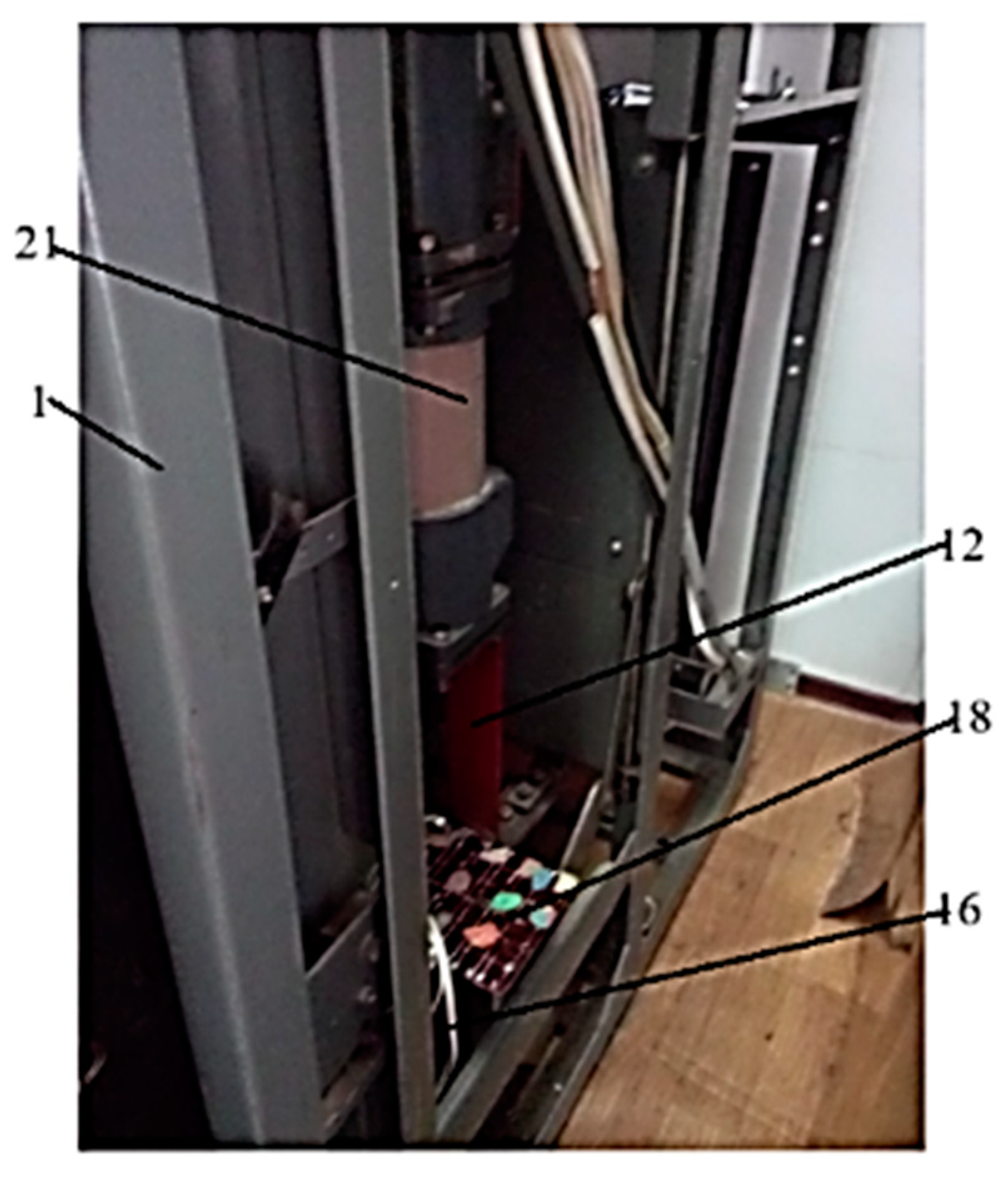
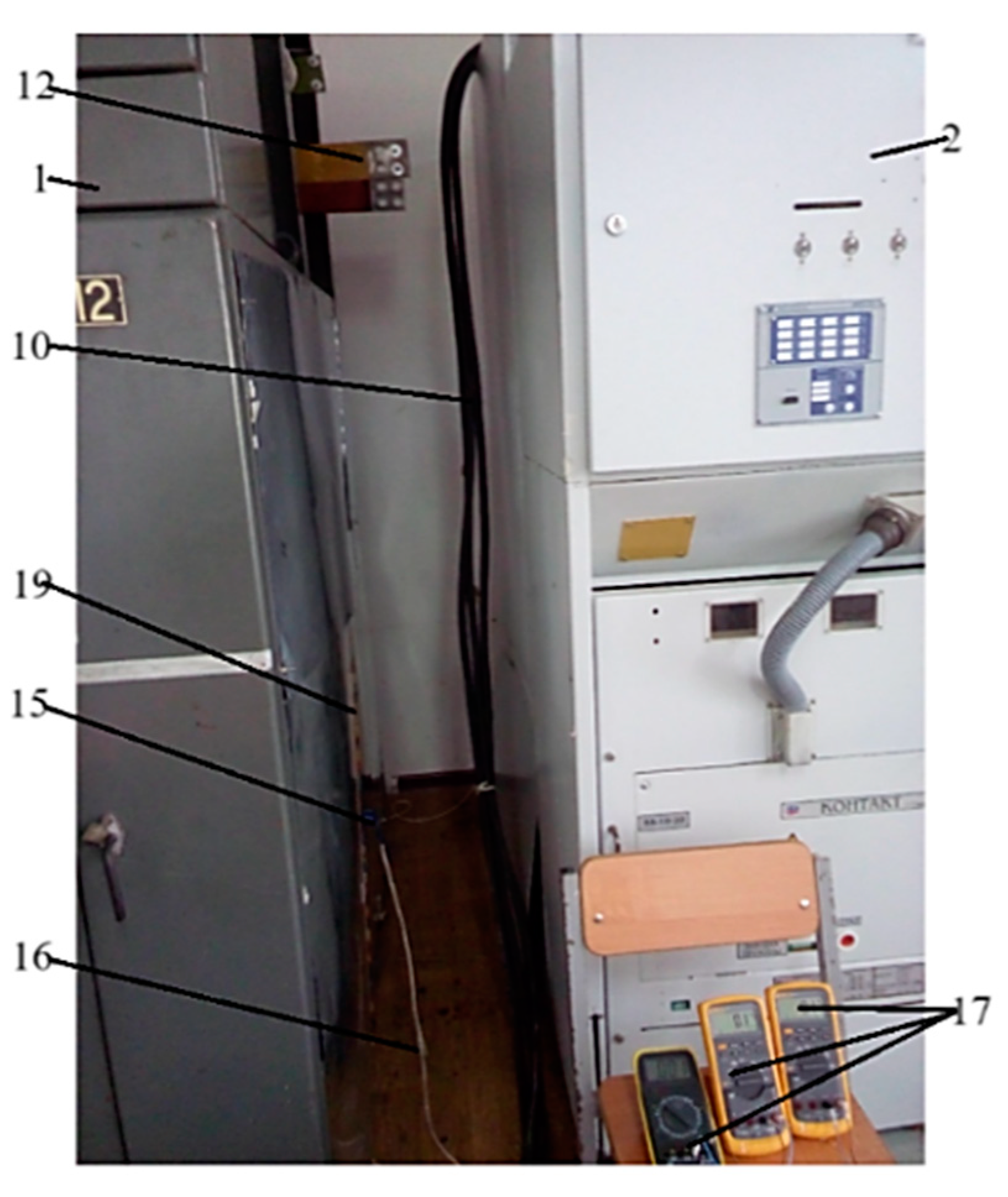
2. Materials and Methods

In the suggested reed switch overcurrent protection, a reed switch simultaneously acts as a current sensor and a measuring unit and responds to the magnetic field produced by currents in the phases of a protected electrical installation. For example, a reed switch actuates (closes its previously open contacts) if the magnetic induction acting on its contacts attains an actuating value, i.e., the so-called magnetomotive force of the reed switch arises, which is estimated at the factory with the use of a control winding of a certain length wound on the reed switch. To respond to the magnetic field, a reed switch is mounted close to the current-carrying busbars at a minimum permissible distance [27,33,34]. To attain the aim of the work, fundamental principles of the theoretical foundations of electrical engineering and relay protection, fundamentals of the theory of electromagnetic transient processes and the design of mechanisms and machines, and laboratory experiments were used. The work was carried out in accordance with the scientific directions of research committee B5 “Relay Protection and Automation” of the International Council on Large Electric Systems (CIGRE), which unites scientists and specialists in the field of electrical power systems throughout European and CIS countries.

3. Experimental Section

To estimate the effects of magnetic fields and interference, an experimental setup was assembled and experiments were performed inside KRU-2 cell (1) [35]; K-63 cell (2) [36] was placed to the right of cell 1 at a distance of 44 cm. For a better understanding of the composition of the experimental setup, Figure 1c shows its structural diagram. Cells 1 and 2 were placed in a laboratory room with a concrete floor (Figure 1 and Figure 2). Bearing wall (3) was on the left side of cell 1, and brick partition (4) was behind this cell at a distance of 35 cm. The width of cell 1 was 90 cm and the wall thickness was 0.3 cm. The experimental setup (Figure 1a) included the following: three-phase switch (5) with the rated current I = 100 A and alternating voltage U = 380 V; three-phase voltage regulator (7), which is connected to switch (5) by first cable (6).

The primary winding of a three-phase load transformer (8), which supplied three-phase sinusoidal alternating current, was connected by cable (9) to the three-phase voltage stabilizer (7), which was required to supply three-phase sinusoidal alternating voltage. The secondary winding of the three-phase load transformer (8) was connected to three current-carrying busbars (12) of cell 1 by second cables (10) passed through the windows of current transformers (11). Current-carrying busbars (12) were centered at points 68 cm (phase A), 45 cm (phase B), and 22 cm (phase C) relative to the right wall of cell 1. A three-phase load transformer (8) was mounted opposite the front wall of cell 1, and second cables (10) were laid on both the left and right sides of cell 1 and around cell 2 (shown by the dashed line in Figure 1). This connection enables the detection of magnetic fields inside cell 1 and their effect on the measured values of magnetic induction inside cell 1. Current recorders (14) were connected to the secondary windings of current transformers (11) using wires (13).

To estimate the magnetic field values inside cell 1, three identical inductive coils (ICs) (15) without a metal core were used. They were windings of an intermediate MKU-48 relay and functioned as magnetic field sensors (actuators of the resource-saving current protection). Inductive coils (15) were connected by wires (16) to EMF recorders (17) [32]. Fluke 87V multimeters were used as current and EMF recorders. To move and fix ICs (15) inside cell 1, the first textolite plate (18) of 90 × 18 × 0.5 cm in size with 1 cm graduation was used. The second plate (19) was fixed on the outside of cell 1. Inductive coils (15) were mounted on the first plate (18) in the plane of the busbars (12) and in three rows spaced 12, 18, and 24 cm apart. The EMF values were measured at 21 points on the first plate (18) and then converted into magnetic induction values.

Plates (18 and 19) with ICs (15) mounted on them were arranged as follows: plate (18) was horizontally fixed on the frame (20) of the circuit breaker (21) inside cell 1 in three vertical positions: 0, 6, and 12 cm (Figure 1 and Figure 2). Plate 19 was flat against the outer sides of the left and right walls of cell 1. The distance and, accordingly, the points where ICs (15) were fixed on the first plate (18) were measured from the right to the left wall of the cell and upward from the plane of current-carrying busbars (12) on a vertically arranged second plate (19).

Inputs L1 of the primary winding of TPL-10-300 22 measuring (Sverdlovsk Plant of Current Transformers, Sverdlovsk, Russia) current transformers [8] were connected to current-carrying busbars (12). Terminals L2 of this primary winding were three-phase short-circuited. The secondary winding of current transformers (22) was also short-circuited during the experiments, because if it is non-shorted (connected), then the supplied current can induce high EMF in it and burn it (since the insulation is insufficient for high EMF values), thus damaging the current transformer. During experiments, a three-phase voltage regulator (7), a load transformer (8), and the housings of cells 1 and 2 were grounded for safety.

To estimate EMF values inside cell 1 during all experiments and for all short circuit types, a three-phase switch (5) was first turned on (see Figure 1a) and an alternating current was supplied to the busbars (12) from the three-phase load transformer (8) using the three-phase voltage regulator (7); the current strength in the busbars (12) was controlled by readings of current recorders (14). This current induced the EMF at the terminals of ICs (15), which was measured and recorded by EMF recorders (17). All experiments were performed in laboratory conditions.

The first experiment: AC flows through three busbars. In this experiment, EMF values are measured at terminals of ICs (15) fixed on a plate (19) at a distance of 12 cm from the plane of busbars (12) starting from the current I = 100 A and up to I = 600 A and recorded by EMF recorders (17). Inductive coils (15) are moved from point 0 cm to point 90 cm while permanently recording EMF values. An alternating current is simultaneously supplied from three phases of the load transformer (7) to three busbars (12) of cell 1. The current is supplied to the input of the busbars (12) and flows through them and through TPL-10-300 current transformers (22) connected to the ends of the busbars (12). The ends of the primary windings of the TPL-10-300 current transformers are three-phase short-circuited to simulate a three-phase SC. The secondary windings of current transformers (22) are also short-circuited to prevent damage. The current is increased by 100 A up to 600 A at each subsequent IC point and magnetic field parameters are recorded at each current value. An alternating current of 600 A is the maximal output of the load transformer (8). After measuring the EMF of the magnetic field from the outer sides of cell 1, ICs (15) are fixed at a distance of 18 and then 24 cm from the plane of the busbars (12) on the plate (19) (see Figure 1a), and EMF values are again measured and recorded. The procedure for measuring and recording the EMF is the same for points spaced 12, 18, and 24 cm apart from the plane of current-carrying busbars (12) and for the first, second, and third positions of the first plate (18).

The second experiment: AC flows through two busbars. In this experiment, a two-phase SC is simulated with the use of the same setup, equipment, instruments, ICs, and technique as in the previous experiment (see Figure 1 and Figure 2). Alternating current is simultaneously and alternately supplied from two phases of load transformer (8) to two current-carrying busbars (12) of cell 1. Alternating current is supplied to the input of these busbars (12) and flows through them and through TPL-10-300 current transformers (22) connected to the ends of busbars (12). The ends of the primary windings of the TPL-10-300 current transformers are two-phase short-circuited to simulate a two-phase short circuit. The secondary windings of these current transformers are also short-circuited to prevent damage.

The third experiment: AC flows through one busbar. In this experiment, a single-phase SC is simulated with the use of the same setup, equipment, instruments, ICs, and technique as in the previous experiments (see Figure 1 and Figure 2). Alternating current is supplied from one of the phases of the load transformer (8) to each current-carrying busbar (12) of cell 1 in series. The current is supplied to the input of busbars (12) and flows through them and the TPL-10-300 current transformer (22) connected to the end of the busbar (12). The secondary windings of current transformers (22) are also short-circuited to prevent their internal damage. The end of the power cable coming from the load transformer (8) is connected to the input of the busbar (12). One end of the second power cable is connected to the output of the primary winding of the current transformer (22), and the other end of the cable is connected to the same phase of the load transformer (8).

4. Results

The results of all the performed experiments are shown in the figures. The results of three experiments on detecting points of maximal EMF inside cell 1, where reed switches (trip relays) of the suggested overcurrent protection are to be fixed, are presented in the form of plots.

Figure 3, Figure 4, Figure 5 and Figure 6 show the EMF as a function of the magnitude of alternating current supplied by a three-phase load transformer (8) to the busbars (12) and the points where the ICs (15) are fixed on the plate (18) during a three-phase short circuit for different positions (horizontal and vertical) of the plate (18) and three rows of the ICs with and without the right wall of cell 1. In these figures, the length of the plate (18) is plotted along the abscissa. As can be seen, the EMF values are maximum when the ICs (15) are in the first row on the plate (18), since they are closer to the current-carrying busbars (12) in this case. The EMF values are lower when the ICs (15) are fixed in the second row and are minimal when the ICs are in the third row because of the greater distance from the busbars (12) (18 and 24 cm, respectively).

EMF during a two-phase short circuit inside cell 1 is plotted in Figure 7a–c versus the supplied alternating current and the points of ICs (15) in the first row and a horizontal position of the first plate (18) in the presence of the right wall of cell 1. The EMF values are maximal at the following points: 45 and 68 cm in the case of a short circuit between phases A and B; 24 and 68 cm in the case of a short circuit between phases A and C; 22 and 47 cm in the case of a short circuit between phases B and C at a distance of 12 cm from the two corresponding busbars. Analysis of EMF values for three two-phase SC types (between phases A and B, A and C, and B and C) shows the EMF to be maximal in the case of a short circuit between phases A and C.

Figure 8 shows the measured EMF values in the case of a ground fault in phases A, B, and C in the presence of the right wall of cell 1 and when plate (18) is in a horizontal position and ICs (15) are in the first row. Knowing the EMF in the case of a single-phase short circuit inside cell 1, it was plotted as a function of the supplied alternating current and the points of ICs (15). The EMF values are maximal at the following points: 68 and 86 cm in the case of a ground fault in phase A; 47 and 86 cm in the case of a ground fault in phase B; 22 and 86 cm in the case of a ground fault in phase C. These values were recorded when ICs (15) were in the first row on the plate (18) at a distance of 12 cm from each corresponding busbar (12).

In these figures, the length of the plate (18), in cm, is plotted along the abscissa, and the EMF values, in mV, are plotted along the ordinate.

Figure 3. EMF as a function of the supplied current in the case of a three-phase short circuit in the presence of the right wall of cell 1: (a) the first, (b) the second, and (c) the third row on the plate (18) which is in the horizontal position.

Figure 3. EMF as a function of the supplied current in the case of a three-phase short circuit in the presence of the right wall of cell 1: (a) the first, (b) the second, and (c) the third row on the plate (18) which is in the horizontal position.

Figure 3 shows the dependence of EMF on the supplied current and the points of ICs (15) during a three-phase short circuit when plate (18) is at the first position inside cell 1 and the ICs are in the (a) first, (b) second, and (c) third row on the plate (18). The EMF curve is wave-like with maxima and minima. The EMF values are maximal when the ICs (15) are in the first row on the plate (18). This is explained by the fact that the ICs (15) being fixed in this position are closer to the current-carrying busbars (12). When the ICs (15) are in the other two rows on the plate (18), the EMF values are lower because the ICs (15) are further from the busbars (12), that is, at a distance of 18 and 24 cm from them. The figure shows the wave-like (three main waves) behavior of EMF, with maxima and minima.

Figure 4. EMF as a function of the supplied current in the case of a three-phase short circuit in the absence of the right wall of cell 1: (a) the first, (b) the second and (c) the third row on the plate (18) which is in the horizontal position.

Figure 4. EMF as a function of the supplied current in the case of a three-phase short circuit in the absence of the right wall of cell 1: (a) the first, (b) the second and (c) the third row on the plate (18) which is in the horizontal position.

Figure 4 shows the wave-like character of variations in the EMF versus the supplied current and the points of the ICs (15). The EMF is maximal when the ICs (15) are in the first row on the plate (18), that is, at a distance of 12 cm from current-carrying busbars (12), because the ICs are closer to the busbars (12) in this row than in the other two rows, at distances of 18 and 24 cm from busbars (12). The EMF values in Figure 4 are lower than in Figure 3. This is explained by the fact that the total value of the EMF inside cell 1 is lower in the absence of the right wall of cell 1 than with this wall.

Figure 5. EMF as a function of the supplied current in the case of a three-phase short circuit in the presence of the right wall of cell 1: (a) the first, (b) the second and (c) the third row on the plate (18) which is in vertical position 60 mm.

Figure 5. EMF as a function of the supplied current in the case of a three-phase short circuit in the presence of the right wall of cell 1: (a) the first, (b) the second and (c) the third row on the plate (18) which is in vertical position 60 mm.

Figure 5 shows the sawtooth (several waves) dependence of the EMF on the supplied current and the points of the ICs (15), with maxima and minima. The EMF is maximal when the ICs (15) are in the first row on the plate (18), that is, at a distance of 12 cm from the current-carrying busbars (12). This is explained by the fact that the ICs are closer to the current-carrying busbars in this case than in the other two rows, at distances of 18 and 24 cm from the busbars (12). In comparison with Figure 3 and Figure 4, EMF maxima are similar at some points and higher at others. This is because at an altitude of 6 cm, the ICs are located closer to the internal metal parts of cell 1, which creates additional (magnetic field) interference detected by the ICs (15).

Figure 6. EMF as a function of the supplied current in the case of a three-phase short circuit in the presence of the right wall of cell 1: (a) the first, (b) the second and (c) the third row on the plate (18) which is in vertical position 120 mm.

Figure 6. EMF as a function of the supplied current in the case of a three-phase short circuit in the presence of the right wall of cell 1: (a) the first, (b) the second and (c) the third row on the plate (18) which is in vertical position 120 mm.

The variation in EMF in Figure 6 has a sawtooth shape, with maxima and minima, like in Figure 5. The EMF is also maximal when the ICs (15) are in the first row on the plate (18), that is, at a distance of 12 cm from the current-carrying busbars (12), for the same reasons as in Figure 5. There are more EMF maxima in Figure 6 than in Figure 6, especially in the first row on plate (18). This is because at an altitude of 12 cm, the ICs are even closer to the internal metal parts of cell 1, which creates additional magnetic (magnetic fields) interference detected by ICs (15).

Figure 7. EMF as a function of the supplied current in the case of a two-phase short circuit between two phases: (a) A and B; (b) A and C; (c) B and C in the presence of the right wall of cell 1 when the plate (18) is in the horizontal position and the ICs (15) are fixed in the first row.

Figure 7. EMF as a function of the supplied current in the case of a two-phase short circuit between two phases: (a) A and B; (b) A and C; (c) B and C in the presence of the right wall of cell 1 when the plate (18) is in the horizontal position and the ICs (15) are fixed in the first row.

Figure 7 shows the wave-like (two large waves) dependence of the EMF, with maxima and minima. The EMF values are maximal in the case of a two-phase short circuit between phases A and C. This is due to the fact that the radius of propagation of magnetic waves from buses A and C includes magnetic fields not only from the current-carrying busbars but also reflected from the walls of the casing of cell 1. These buses are located on two sides, that is, closer to the side walls of cell 1 than bus B. Bus B is located in the very middle of the casing of cell 1.

Figure 8. EMF as a function of the supplied current in the case of a ground fault in (a) phase A, (b) phase B and (c) phase C in the presence of the right wall at cell 1 when the plate (18) is in the horizontal position and the ICs (15) are fixed in the first row.

Figure 8. EMF as a function of the supplied current in the case of a ground fault in (a) phase A, (b) phase B and (c) phase C in the presence of the right wall at cell 1 when the plate (18) is in the horizontal position and the ICs (15) are fixed in the first row.

Figure 8 shows the measured EMF values in the case of ground faults in phases A, B, and C, when ICs (15) are fixed in the first row on plate (18). The EMF is maximal at the following points: 68 and 86 cm in the case of a ground fault in phase A, 47 and 86 cm in the case of a ground fault in phase B, and 22 and 86 cm in the case of a ground fault in phase C. The EMF variation is wave-like (one large wave) in character, with maxima and minima. The EMF values in the case of ground fault are the highest in comparison with other short circuit types (three- and two-phase). This is explained by the absence of mutual damping of the magnetic fields from nearby current-carrying busbars and by the internal distribution of the magnetic field inside cell 1.

The determination of the field damping coefficient ${\mathrm{K}}_{\mathrm{f}\mathrm{d}}$, correction factor ${\mathrm{K}}_{\mathrm{C}}$ and phase coefficient ${\mathrm{K}}_{\mathrm{P}\mathrm{h}}$ for the calculation of the selection of resource-saving protection settings is presented below.

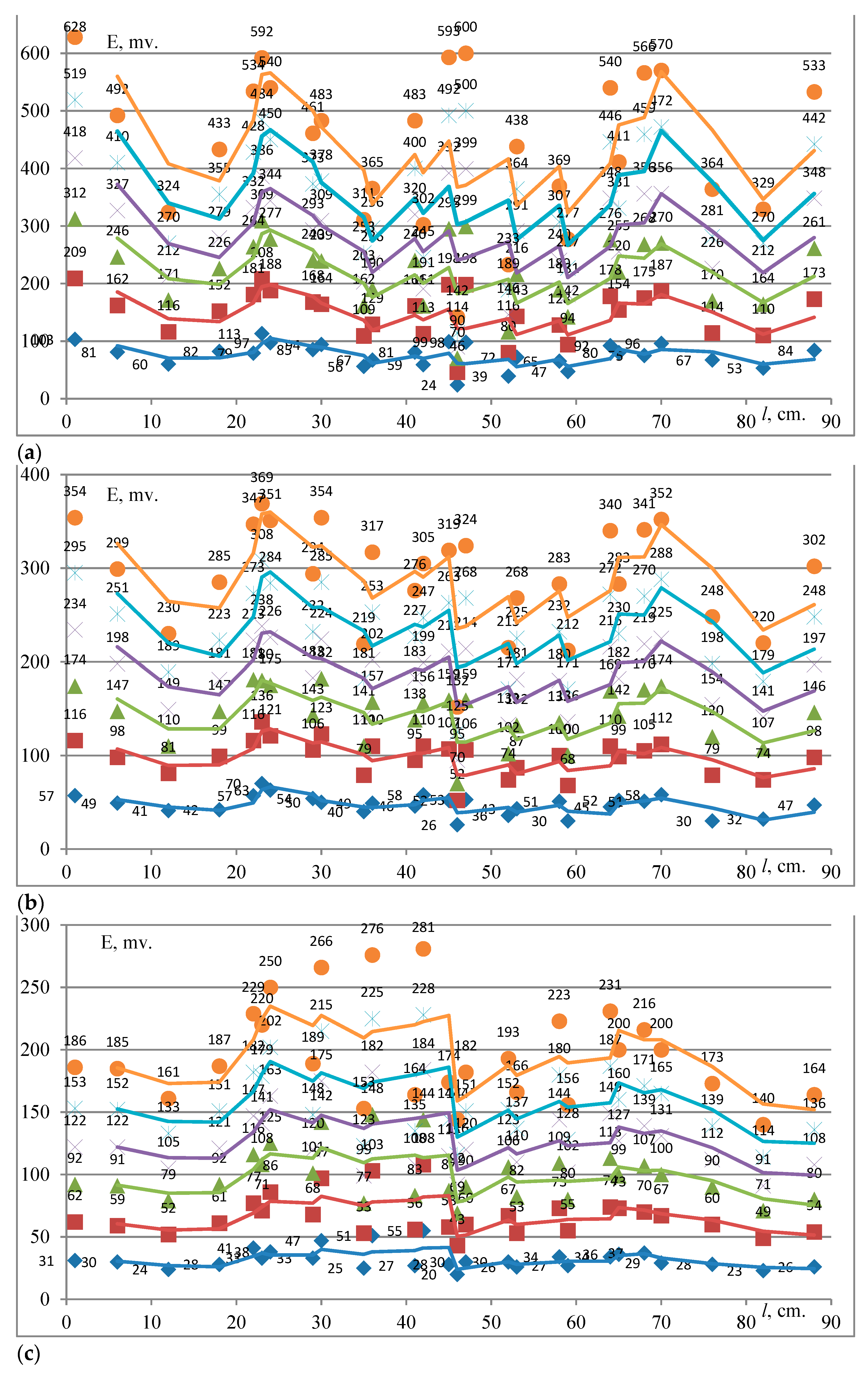
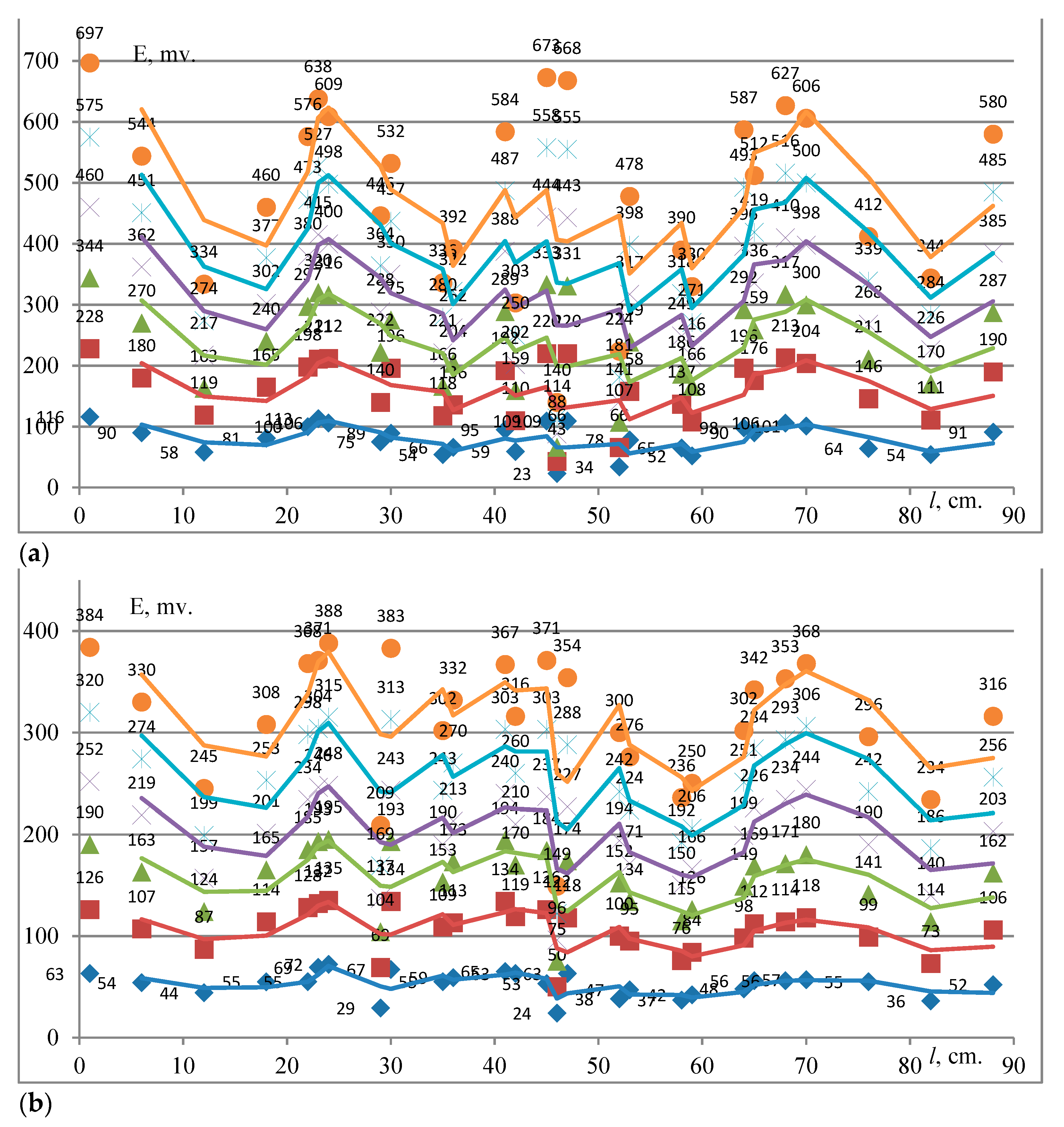
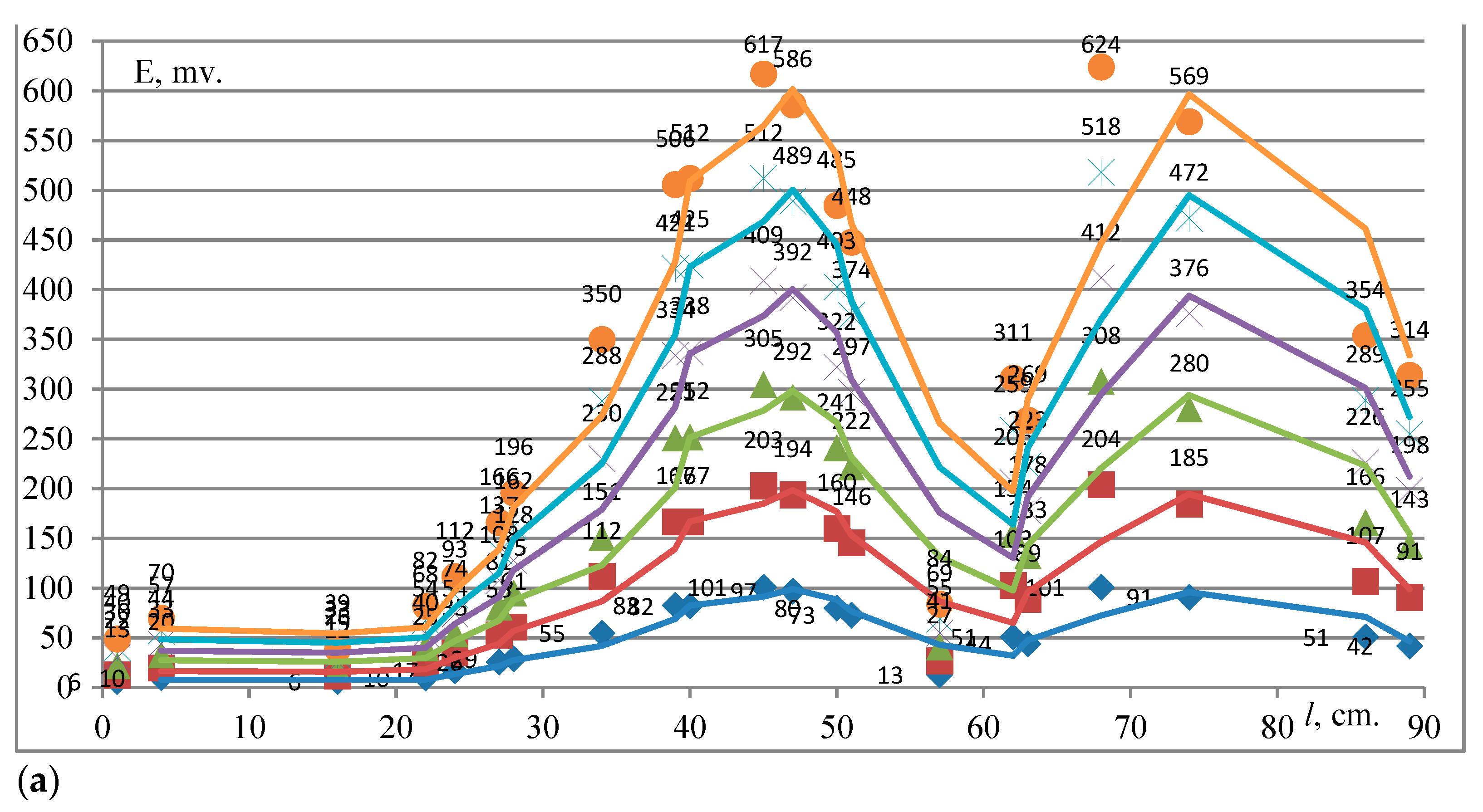
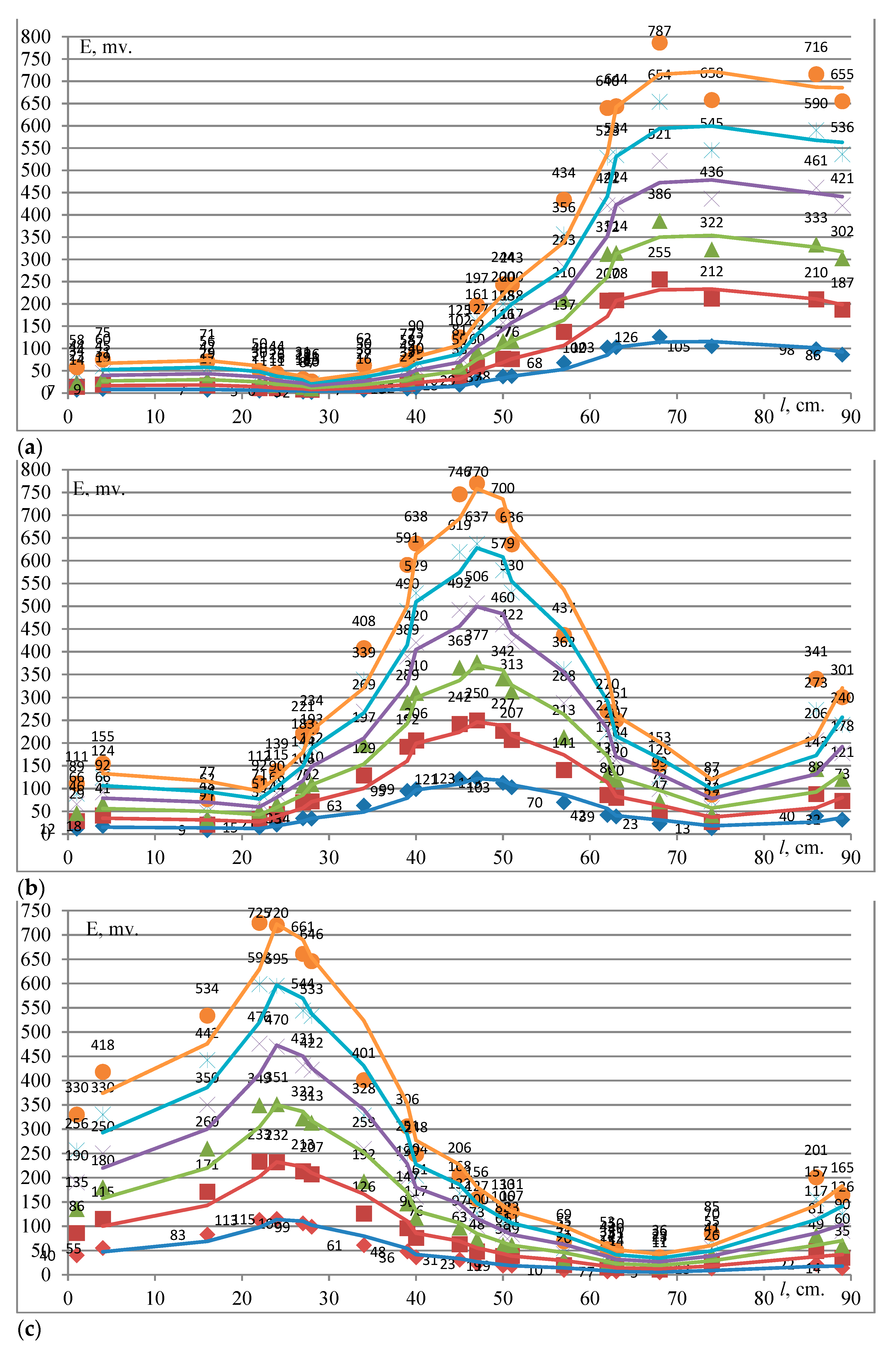
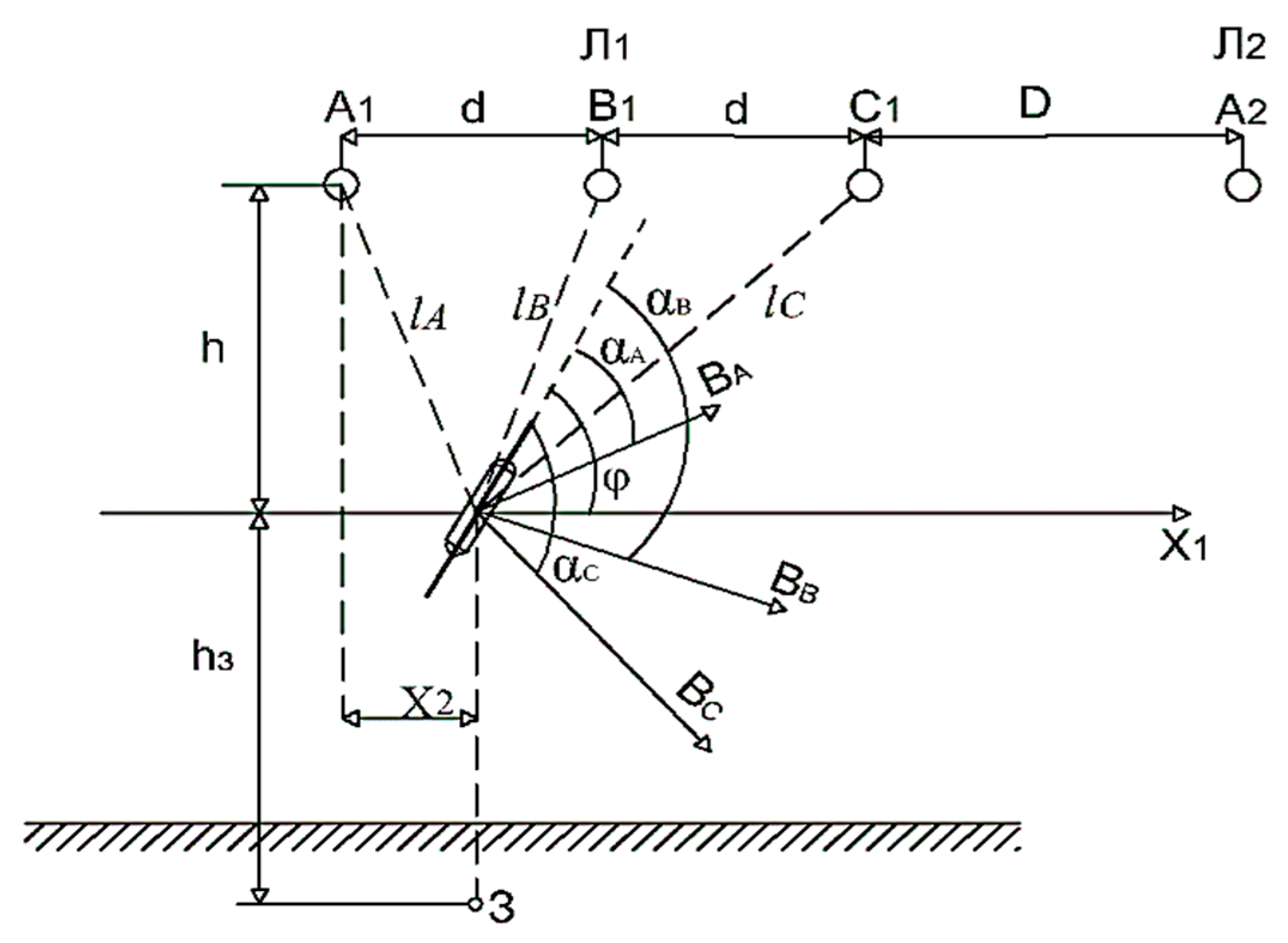
4.1. Field Damping Coefficient $K_{fd}$

By means of this coefficient, we have studied how the right or left wall of the body of cell 1 decreases the magnetic induction (measuring the EMF and recalculating it into magnetic induction) on the inner and outer sides of the wall under the maximal current (600 A) produced by the load transformer. Figure 1a and Figure 9 show the layout of cable 10 routing around cell 2, which simulates an external short circuit current in cell 1 from an electrical installation connected to cell 2. In this case, two ICs (15) are mounted on the first plate (18) at its first position and at a distance of 12 cm from the plane of the current-carrying busbars (12) inside cell 1, in its two endpoints 1 and 90 cm, which are maximally close to the neighboring cells. The third IC is fixed on the second plate (19) and is mounted on the outside of the right and left walls of cell 1 at a distance of 12 cm from the plane of the current-carrying busbars (12) of cell 1. All three ICs (15) are placed at the same level relative to the floor.

The induction values measured inside cell 1 are compared with the magnetic field induction values at the outer sides of this cell. On the basis of the results, the coefficients of field damping by the left (${\mathrm{K}}_{\mathrm{f}\mathrm{d}1}$) and right walls (${\mathrm{K}}_{\mathrm{f}\mathrm{d}2}$) of cell 1 are calculated as:

$${\mathrm{K}}_{\mathrm{f}\mathrm{d}}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{i}\mathrm{n}\mathrm{s}\mathrm{i}\mathrm{d}\mathrm{e}}}{{\mathrm{B}}_{\mathrm{o}\mathrm{u}\mathrm{t}\mathrm{s}\mathrm{i}\mathrm{d}\mathrm{e}}}},$$

(1)

where ${\mathrm{B}}_{\mathrm{i}\mathrm{n}\mathrm{s}\mathrm{i}\mathrm{d}\mathrm{e}}$ in and ${\mathrm{B}}_{\mathrm{o}\mathrm{u}\mathrm{t}\mathrm{s}\mathrm{i}\mathrm{d}\mathrm{e}}$ are the values of magnetic induction, T, measured at points 1 and 90 cm on the inner (${\mathrm{B}}_{\mathrm{i}\mathrm{n}\mathrm{s}\mathrm{i}\mathrm{d}\mathrm{e}}$) and at points −0.3 and 90.3 cm on the outer (${\mathrm{B}}_{\mathrm{o}\mathrm{u}\mathrm{t}\mathrm{s}\mathrm{i}\mathrm{d}\mathrm{e}}$) sides of the right and left walls of cell 1:

$$\begin{gathered} {\mathrm{K}}_{\mathrm{f}\mathrm{d}1}={\displaystyle \frac{{\mathrm{B}}_{90}}{{\mathrm{B}}_{0.3}}}={\displaystyle \frac{317}{134}}=2.36 \\ {\mathrm{K}}_{\mathrm{f}\mathrm{d}2}={\displaystyle \frac{{\mathrm{B}}_{-0.3}}{{\mathrm{B}}_1}}={\displaystyle \frac{146}{92}}=1.58 \end{gathered}$$

4.2. Correction Factor $K_C$

The correction factor enables taking into account the errors caused by alternating current flowing through busbars and by magnetic field distortion. For this purpose, we use the formula of the Biot–Savart law. According to this law, a reed switch actuates at the magnetic induction defined as [6,37]:

$${\mathrm{B}}_{\mathrm{C}}={\displaystyle \frac{{\mathrm{I}}_{\mathrm{C}}{\mathsf{\mu }}_0}{2\mathsf{\pi }\mathrm{h}}}={\mathrm{I}}_{\mathrm{C}}{\displaystyle \frac{{\mathsf{\omega }}_{\mathrm{C}}{\mathsf{\mu }}_0}{l_C}}={\displaystyle \frac{{\mathrm{F}}_{\mathrm{C}}{\mathsf{\mu }}_0}{l_C}}={\mathrm{H}}_{\mathrm{C}}{\mathsf{\mu }}_0,$$

(2)

where ${\mathrm{I}}_{\mathrm{C}}$, ${\mathrm{H}}_{\mathrm{C}}$ and ${\mathrm{B}}_{\mathrm{C}}$ are the minima of the current in a current-carrying busbar, magnetic field strength and induction, at which a reed switch actuates; ${\mathsf{\mu }}_0$ is the permeability of air.

The experiment was performed with first plate (18) in the first position. To find the correction factor, the induction ${\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}$ measured at all measuring points on plate (18) is compared with the magnetic field inductions ${\mathrm{B}}_{\mathrm{c}\mathrm{a}\mathrm{l}\mathrm{c}\mathrm{u}\mathrm{l}\mathrm{a}\mathrm{t}\mathrm{e}\mathrm{d}}$ calculated by the Biot–Savart law at the same points:

$${\mathrm{K}}_{\mathrm{C}}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{c}\mathrm{a}\mathrm{l}\mathrm{c}}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}}}.$$

(3)

The magnetic field induction is calculated by the formula

$${\mathrm{B}}_{\mathrm{c}\mathrm{a}\mathrm{l}\mathrm{c}}={\displaystyle \frac{\mathrm{I}\times {\mathsf{\mu }}_0}{2\times \mathsf{\pi }\times \mathrm{h}}}, \mathrm{mT},$$

(4)

where I is the current supplied by the load transformer to current-carrying busbars, A; ${\mathsf{\mu }}_0$ is the permeability of air; h is the distance between an inductance coil and the current-carrying busbars, cm.

The magnetic field induction is experimentally determined by the formula [37]:

$${\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}={\displaystyle \frac{{\mathrm{E}}_{\mathrm{e}\mathrm{x}\mathrm{p}}}{2\mathsf{\pi }\times \mathsf{\omega }\times \mathrm{S}\times \mathrm{f}}}, \mathrm{mT},$$

(5)

where ${\mathrm{E}}_{\mathrm{e}\mathrm{x}\mathrm{p}}$ is the EMF output from IC 15 during the experiments; ω and S are the number of turns and cross-section area of the inductance coil; f is the industrial frequency.

Taking into account the maximal magnetic inductions at the centers of axes of the current-carrying busbars of cell 1 in the case where the current I = 200, 400 and 600 A flows through a current-carrying busbar of phase A (point 68 cm), the correction factor is:

$${\mathrm{K}}_{\mathrm{C}200}={\displaystyle \frac{334{10}^{-6}}{132{10}^{-6}}}=2.5; {\mathrm{K}}_{\mathrm{C}400}={\displaystyle \frac{670{10}^{-6}}{270{10}^{-6}}}=2.48 \approx 2.5; {\mathrm{K}}_{\mathrm{C}600}={\displaystyle \frac{1005{10}^{-6}}{408{10}^{-6}}}= 2.46\approx 2.5$$

The correction factor ${\mathrm{K}}_{\mathrm{C}}$ is calculated in a similar way in the cases where the current flows through three and two busbars of cell 1.

4.3. Phase Coefficient $K_{Ph}$

This coefficient is introduced because each reed switch mounted opposite a proper phase is affected by magnetic inductions from both this phase and the other two phases when the current is flowing through three, two and one phases. In this case, the EMF values experimentally determined by the ICs (15) are also converted into magnetic induction values by Equation (1).

The purpose is to estimate how many times the induction ${\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}$ of the current flowing in one busbar differs from the inductions ${\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{//}$, ${\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{///}$ when the current flows through the two and three other busbars of cell 1.

Based on the induction values at different values of current through three, two and one busbars (phase A, point 68 cm), the phase coefficient ${\mathrm{K}}_{\mathrm{P}\mathrm{h}}$ is calculated by the formula

$${\mathrm{K}}_{\mathrm{P}\mathrm{h}1}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{//}}},$$

(6)

$${\mathrm{K}}_{\mathrm{P}\mathrm{h}2}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{///}}}.$$

(7)

When the current I = 200, 400 and 600 A flows through one (A) and two (AB; AC) busbars of cell 1, the phase coefficients are

$${\mathrm{K}}_{\mathrm{P}\mathrm{h}1.\mathrm{A}\mathrm{B}200}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}{10}^{-6}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}\mathrm{A}\mathrm{B}}^{//}{10}^{-6}}}={\displaystyle \frac{334}{105}}=3.1;\hspace{1em}\hspace{1em}\hspace{1em}{\mathrm{K}}_{\mathrm{P}\mathrm{h}1.\mathrm{A}\mathrm{C}200}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}{10}^{-6}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}\mathrm{A}\mathrm{C}}^{//}{10}^{-6}}}={\displaystyle \frac{334}{125}}=2.6;$$

$${\mathrm{K}}_{\mathrm{P}\mathrm{h}1.\mathrm{A}\mathrm{B}400}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}{10}^{-6}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}\mathrm{A}\mathrm{B}}^{//}{10}^{-6}}}={\displaystyle \frac{670}{213}}=3.1;\hspace{1em}\hspace{1em}\hspace{1em}{\mathrm{K}}_{\mathrm{P}\mathrm{h}1.\mathrm{A}\mathrm{C}400}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}{10}^{-6}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}\mathrm{A}\mathrm{C}}^{//}{10}^{-6}}}={\displaystyle \frac{670}{256}}=2.6$$

$${\mathrm{K}}_{\mathrm{P}\mathrm{h}1.\mathrm{A}\mathrm{B}600}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}{10}^{-6}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}\mathrm{A}\mathrm{B}}^{//}{10}^{-6}}}={\displaystyle \frac{1005}{323}}=3.1;\hspace{1em}\hspace{1em}\hspace{1em}{\mathrm{K}}_{\mathrm{P}\mathrm{h}1.\mathrm{A}\mathrm{C}200}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}{10}^{-6}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}\mathrm{A}\mathrm{C}}^{//}{10}^{-6}}}={\displaystyle \frac{1005}{390}}=2.6.$$

When the current I = 200, 400 and 600 A flows through one (A) and three (ABC) busbars,

$${\mathrm{K}}_{\mathrm{P}\mathrm{h}2.200}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}{10}^{-6}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{///}{10}^{-6}}}={\displaystyle \frac{334}{95}}=3.5;\hspace{1em}\hspace{1em}\hspace{1em}{\mathrm{K}}_{\mathrm{P}\mathrm{h}2.400}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}{10}^{-6}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{///}{10}^{-6}}}={\displaystyle \frac{670}{177}}=3.78;\hspace{1em}\hspace{1em}\hspace{1em}{\mathrm{K}}_{\mathrm{P}\mathrm{h}2.600}={\displaystyle \frac{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{/}{10}^{-6}}{{\mathrm{B}}_{\mathrm{e}\mathrm{x}\mathrm{p}}^{///}{10}^{-6}}}={\displaystyle \frac{1005}{268}}=3.75.$$

The phase coefficient is calculated in a similar way when current flows through three, two, and two single B and C busbars. When the current flows through one busbar, the phase coefficient.

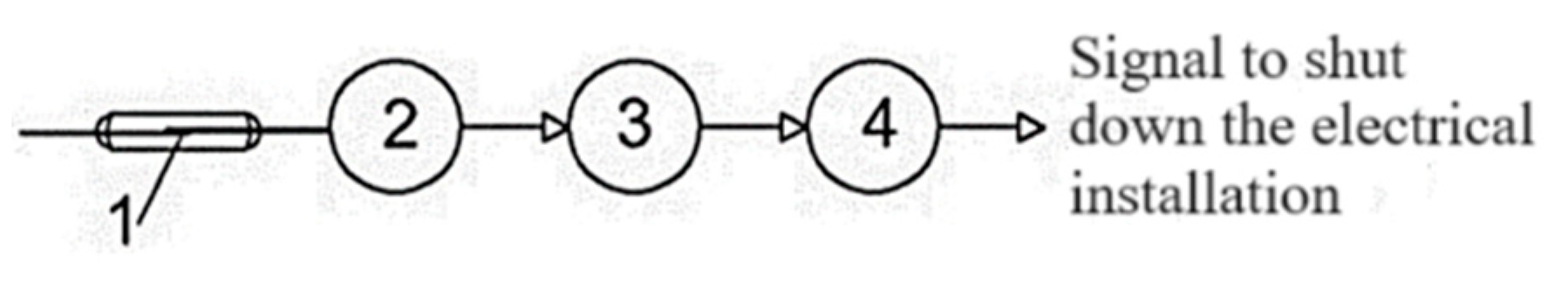
4.4. Use of Reed Switches in Relay Protection

Measuring current transformers have been used as the main transducers for relay protection and measurement devices for many years. They have such disadvantages as high errors, voltages that appear when the winding in the secondary circuit breaks, size, weight, and expensive materials. Due to these disadvantages, the International Council on Large Energy Systems (CIGRE) has repeatedly raised the question, for example, about a need to use or develop sensors that could become an alternative to traditional measuring current transformers.

Reed switches can help to solve this problem. They are commonly used in technology, are promising [27], and have important advantages for relay protection. Thus, reed switches can simultaneously function as a measuring current transformer, an actuator, and an analog-to-digital converter; they do not require amplifiers to transmit a signal, and the transmission is carried out through control, but not measuring circuits; and there is no need in devices which reduce the temperature effect.

Reed switches are mounted at specified points at a safe [33] distance h from the current-carrying busbar of the electrical installation and actuate (close or switch contacts 1 (Figure 10), fixed in glass tube 2 0.7 to 5 cm long) for 0.1–3 ms under the action of a magnetic field produced by the current in the busbar.

A reed switch current protection consists of simple accessible elements and is easily assembled. It consists (Figure 11) of a reed switch (1), pulse stretcher (2), time relay (3), and actuator (4) [6].

When a protection is based on reed switches, the scheme coefficient $k_{SK}$ and transformation coefficient $n_U$ are not used, but the protection operation current I_prot.oper and return current I_return remain [6]. Timing characteristics and sensitivity coefficient are applied in the same way as for overcurrent protections based on measuring current transformers with metal cores. The arrangement of a reed switch at a safe distance h from, for example, phase A in the plane of its cross section is shown in Figure 12 [6]

An example of selecting settings for the suggested overcurrent protection is described in Appendix A. Appendix B technically and economically substantiates the efficiency of the resource-saving overcurrent protection.

As an example of the implementation of resource-saving reed current protection, the following is an example of “Resource saving Motor Current Protection with Remote Setpoint Selection”.

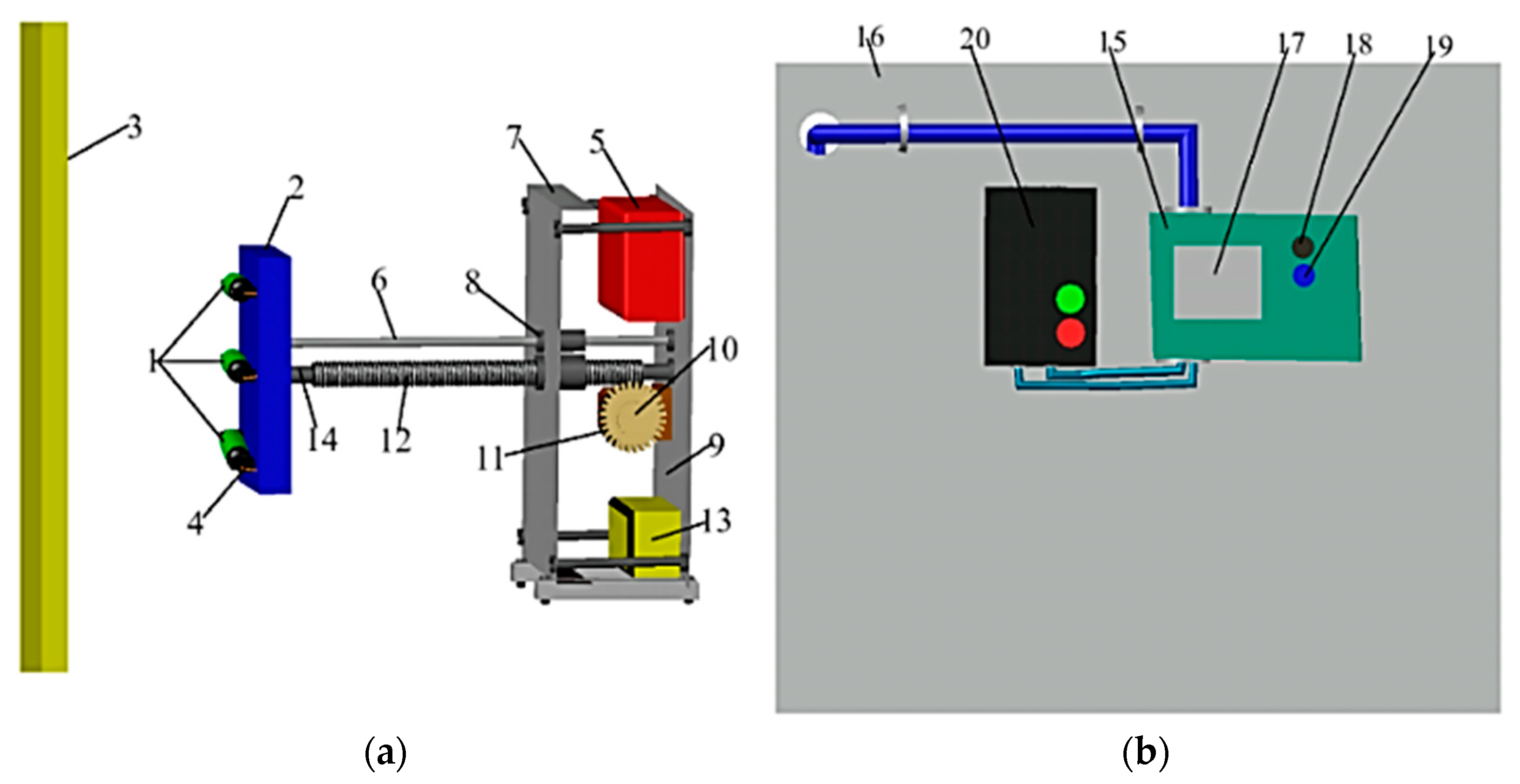
5. Resource Saving Electric Motor Current Protection with Remote Setpoint Selection

Current protection with remote setpoint selection, presented in the form of a device in its composition consists of reed switches (1) (Figure 13a), which are mounted on the plate (2) and are located at different angles to the plane of the cross section of the current-carrying busbar (3) of any cell of the complete switchgear. The leads of the contacts (4) of reed switches (1) are connected to the time-zapper (5). The plate (2) is fixed to the first end of the support post (6) and is located opposite the current-carrying busbar (3) at a safe distance from it. The other end of the support stand (6) is fixed inside the container (7) with the help of the sleeve (8) fixed to the wall of the container (7). Inside the container (7) on the platform (9), the motor reducer (10) is fixed, on which the worm wheel (11) is mounted, as well as the worm shaft (12), the time setter (5), as which uses a time relay and the actuator (13) as which uses an intermediate relay, are installed and fastened. On the rear wall of the container (7), the platform (9), the time-holder (5) and the actuator (13) are mounted. The plate (2) is connected to the first end of the worm shaft (12) by means of the hollow cylinder (14), and the second end of the worm shaft (12) is attached to the back wall of the container (7). The motor reducer (10) is attached to the platform (9). The penstock (7) is fixed in the switchgear panel. The number of these peninsulas (7) is three, each located opposite its current-carrying busbar (3) (Figure 13a).

The gear motor (10) is powered by the control unit (15) installed in the protective cabinet (16). On the front panel of the control unit (15), there is a display (17) for controlling the operation, as well as the “forward–backward” control buttons (18; 19) for the gear motor (10). The control unit (15) is powered by the circuit breaker (20) (Figure 13b).

Regulation of triggering parameters of current protections from short-circuit currents is carried out by approaching the current-carrying busbar (3), plate (2) and reed switches (1). At the same time, one protection uses one of the three reed switches (1). The plate (2) is moved by means of a geared motor (10) by pressing buttons (18; 19). The required distance from the plate (2) to the current-carrying bus (3) in a cubicle switchgear is defined by turning the geared motor (10) implemented by a microcontroller mounted on the control unit (15).

Before installing, the device in the switchgear cubicle calculates the necessary distance from the current-carrying bus (3) to the reed switches (1) and the angle at which the reed switch (1) must be in relation to the lines of force of the magnetic field created by the current in the current-carrying bus (3); according to the table, we take the reed switch (1) with a given magnetomotive force of operation.

The current protection works as follows. In the bus compartment of the cell at a safe distance equal to 0.12 m (the minimum allowable distance according to Electrical Installation Regulations from live parts of electrical installations with voltage U = 10 kV) from the live busbars (3), we install three penalized enclosures (7). In the bay protection cabinet (16), we place and connect the control unit (15) and circuit breaker (20). Then, we turn on the circuit breaker (20) supplying power to all elements of the current protection device (Figure 13b).

As an example, consider the setting of protection for phase A made with one of the three reed switches (1) (the first) located on the plate (2) relative to the plane of the cross-section of the current-carrying busbar (3) at an angle of 0⁰. The remaining two reed switches (1), also located on the plate (2), are designed to provide a more accurate choice of settings, and will be used in case the reed switch (1) (the first) cannot be selected with the necessary magnetomotive force. At the very beginning, the display (17) of the control unit (15) shows the distance to the current-carrying busbars (3) at which the plate (2) with the reed switches (1) is currently located, e.g., 0.15 m. Then, by pressing the “Forward” button (18), the geared motor (10) is started which moves the plate (2) together with the reed switches (1) closer to the current-carrying busbars (3). After the display (17) shows the value 0.12 m, the release button (18) and thus the reed switch (1) are smoothly set at the necessary distance from the current-carrying busbar (3) of phase A. Similarly, the current protection (the other two hangers (7)) is set for the other two phases B and C.

When a short circuit in the protected electric motor, the current flowing on the current-carrying bus (3) of the switchgear panel exceeds the current operation of protection, at which point, one of the reed switches (1) triggers (closes contacts), sending a signal to the time setter (6), which, after a specified time delay, sends a signal to the executive (13), which in turn sends a signal to the circuit breaker of the electric motor.

In nominal load modes, the electric motor current does not exceed its maximum value, and in this regard, the reed switches (1) are not triggered. The motor reducer (10) makes it possible to remotely adjust the distance from the reed switches (1) to the current-carrying busbar (3) of the switchgear panel, thus ensuring the selection of the required setting of the electric motor current protection operation and the absence of the use of expensive and bulky current transformer ferromagnetic cores (compared with reed switches), providing significant savings in material resources, which together makes it possible to use this current protection in any series of switchgear cells to implement current protections of electric motors of any type and voltage class.

6. Conclusions

All the numerical parameters and dependencies derived from the experiments are presented in the figures. The EMF is higher when the plate (18) is at the second and, especially, the third positions than when it is in the first position. This is explained by the fact that ICs (15) in this position are as close as possible to the metal structures of cell 1, which produce additional magnetic fields (interference).

The analysis of the experiments we performed has shown that when the first plate (18) is at the first position, the EMF is maximal at the following points:

-

At 22, 47 and 68 cm at a distance of 12 cm from the current carrying busbars;

-

At 22, 47 and 74 cm at a distance of 18 cm;

-

At 16, 47 and 74 cm at a distance of 24 cm from busbars (12).

When the first plate (18) is at the second and third positions, the EMF is maximal at the following points:

-

At 1, 23, 45 and 68 cm at a distance of 12 cm;

-

At 1, 24, 45 and 70 cm at a distance of 18 cm;

-

At 1, 24, 47 and 64 cm at a distance of 24 cm from the busbars (12), respectively.

Taking into account the field experiments for all short circuit types, which enable identifying points where the EMF is maximal, we have ascertained that actuators of the suggested protection, which are reed switches, are advisable to mount at the first position of the first plate (18), opposite the center of the axes (because the magnetic induction maximum is concentrated there) of the current-carrying busbars (12) of cell 1 at a distance of 12 cm from them, where the EMF is higher than at the other two distances of 18 and 24 cm. Considering the difficulty associated with the detuning of the overcurrent protection, it is also not desirable to mount the reed switches at the second and third positions of the first plate (18) because of the strong effect of the metal structures of cell 1, which produced additional interference.

Finally, we recommend the following. The suggested resource-saving protection can be used as an overcurrent protection for electrical installations connected to 6–10-kV switchgear cells. Our experiments with cells 1 and 2 have shown a possibility of creating resource-saving overcurrent protection using magnetic field sensors based on reed switches. This protection meets the requirements for relay protections and can be an alternative to traditional current protection. All the above is confirmed by the selection of settings for resource-saving overcurrent protection and verification of its sensitivity coefficient. The suggested overcurrent protection can be used for different electrical installations with any class of rated voltage, for example, 35, 110, 220 kV and so on.

The application of the developed protections will ensure the following:

-

The refusal of the use of measuring current transformers is in accordance with the strategically important task set by the international committee on large power systems of CIGRE. The resource-saving effect consists of a reduction in expenses for the realization of current protection, which in the end leads to an increase in material income of the world power industry and the world community as a whole by an amount greater than the initial expenses for the arrangement of this protection;

-

The expected results of the development of the main scientific direction (relay protection and automation) and related fields of science and technology have an impact on the development of resource-saving relay protection without the use of traditional current transformers.

The scientific and technical effect is that the developed protections are not inferior to the existing traditional protections made with the use of measuring current transformers.

The economic effects are as follows:

-

Developed protections perform the functions of an analog-discrete and measuring converter and the same protection body, and are resource-saving, consisting of minimizing initial material costs and subsequently minimum annual costs during operation;

-

Development of competitive, resource-saving relay protection for the future, and a reduction in production costs of such protection, namely that produced at stations, and electricity consumed at substations of enterprises, as well as a growth in labor productivity due to a reduction in time when producing such protection devices in comparison with the time spent on traditional production.

The environmental effect consists of saving both the extraction of non-ferrous and ferrous metals from the earth’s surface and their further processing at metallurgical plants. As a result, the load on power generation by power plants and on non-ferrous and ferrous metal consumption in the production of traditional measurements of current transformers is simultaneously reduced. The tasks of environmental protection and reduction in harmful emissions of production are solved; there is a reduced or even complete absence of heat and power consumption during the production of traditional current transformers due to their uselessness for power supply of relay protection devices. At the same time, the emission of harmful substances into the atmosphere of the planet decreases, and as a result, various human pathologies and diseases related to ecology are reduced. At the same time, the healthy and able-bodied population increases, which is undoubtedly a powerful personnel and social effect.

The conducted experiments, their results, and selected protection settings confirm the efficiency of the suggested resource-saving overcurrent protection as an alternative to traditional current protections. The results of this work significantly contribute to the further scientific development of power engineering and, in particular, relay protection (automation). The high efficiency of the presented overcurrent protection is also confirmed by the graphically represented experimental results with their detailed analysis, which clearly show the maximum and minimum points of EMF values, the selection of settings for resource-saving current protection, and technical and economical assessments.

7. Patents

(1)

Dauren Jambulovich Issabekov, KZ Patent “Design for preventing an overhead crane from crossing the maximum permissible positions on reed switches”, № 35293, Bulletin № 38, 24.09.2021, https://gosreestr.kazpatent.kz/Invention/Details?docNumber=330114, accessed on 27 September 2021;

(2)

Dauren Jambulovich Issabekov, KZ Patent “Device for maximum current protection of electrical installations on magnetically controlled elements”, № 35387, Bulletin № 47, 26.11.2021, https://gosreestr.kazpatent.kz/Invention/Details?docNumber=330086, accessed on 27 October 2021

Positive Aspects of the Research

The conducted experiments aimed at further development of current protections for different electrical installations connected to switchgear cells suggest a way of saving significant amounts of copper, steel and high-voltage insulation due to the complete refusal of the use of traditional measuring current transformers with metal cores. In the future, field experiments on the development of alternative resource-saving current protections for different 6-10 kV electrical installations will be continued, including for other voltage classes and other relay protection types, such as differential and gas protections for electrical installations both connected to switchgear cells and closed switchgears and for separate electrical installations, for example, gas protections for oil-filled arc-fault-reactive reactors. The results of experimental research can be used by the scientific community, industrial enterprises, power stations and substations of plants.