A Bi-Level Reactive Power Optimization for Wind Clusters Integrating the Power Grid While Considering the Reactive Capability
Abstract
1. Introduction
- A bi-level model is proposed to optimize reactive power and address the integration of large-scale wind power clusters into the power system. To jointly solve the upper and lower layers, a cross-iteration method is employed.
- The reactive power capacity of the wind power cluster is assessed through meticulous analysis, taking into full consideration the maximum reactive power margin of the wind farm in the optimization strategy to ensure the efficient operation of the power system.
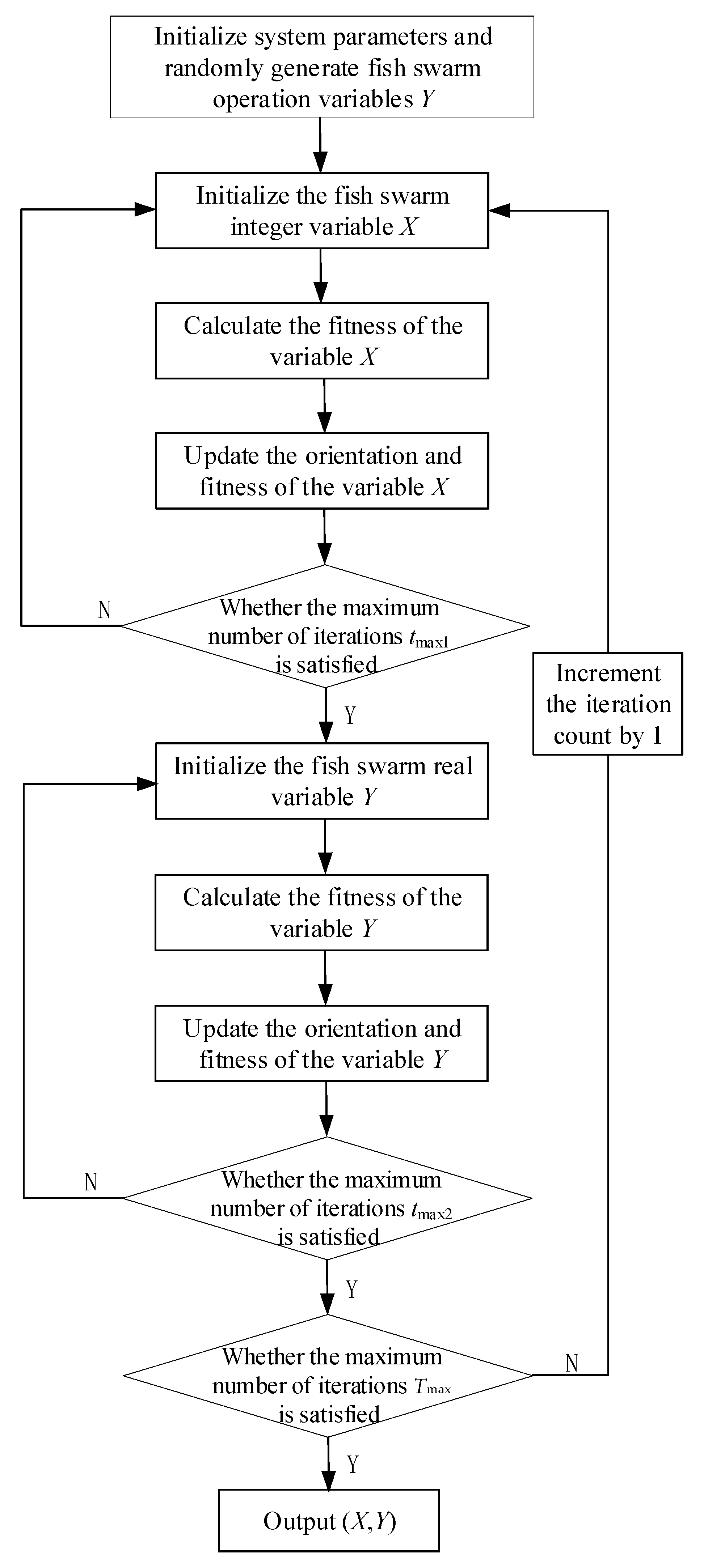
- An improved artificial fish swarm algorithm is proposed, which decouples real variables and integer variables, reduces the dimensions of variables, enhances the optimization ability of the algorithm, and solves the problem where the algorithm is susceptible to the influence of the local optimum.
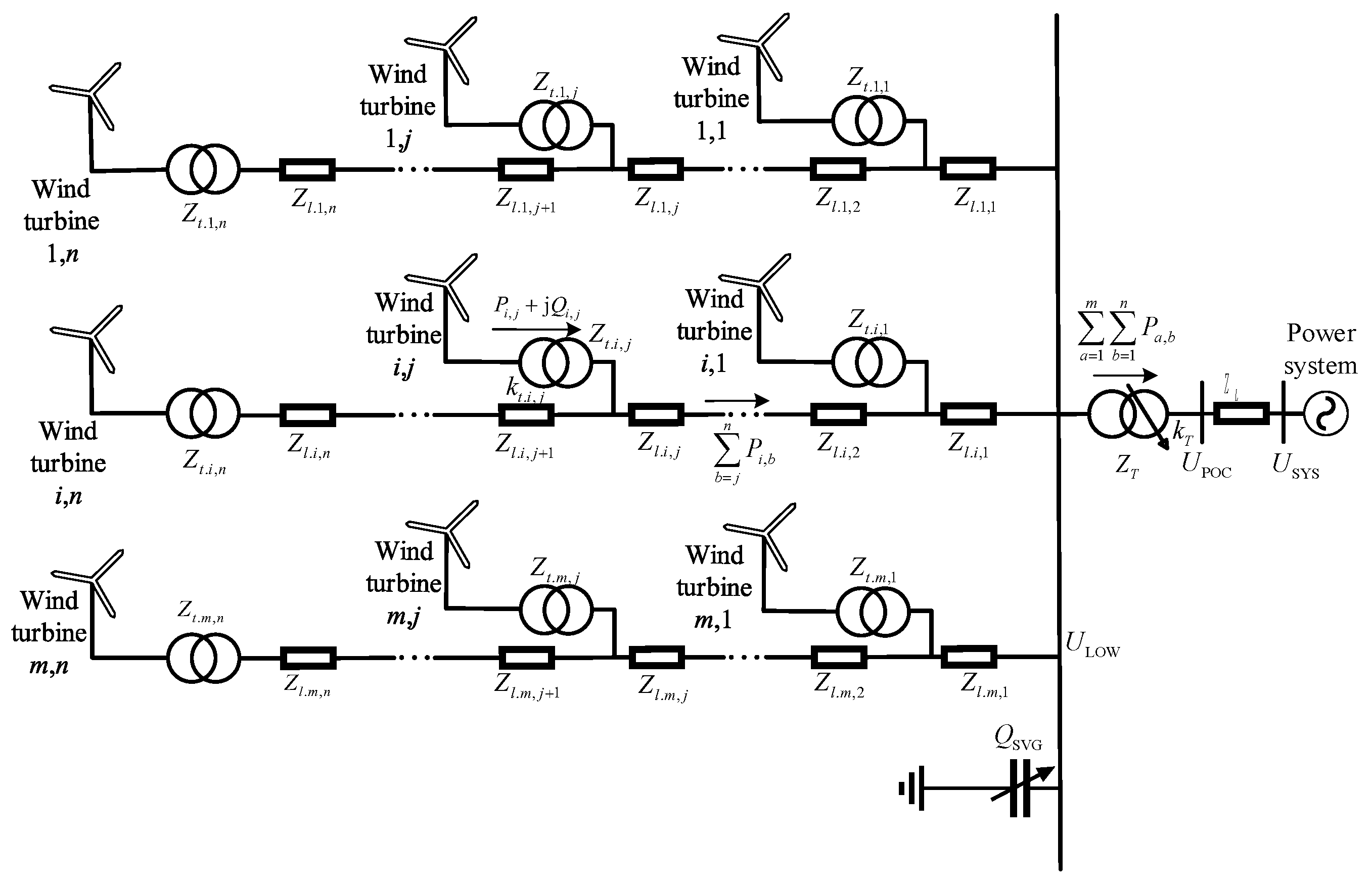
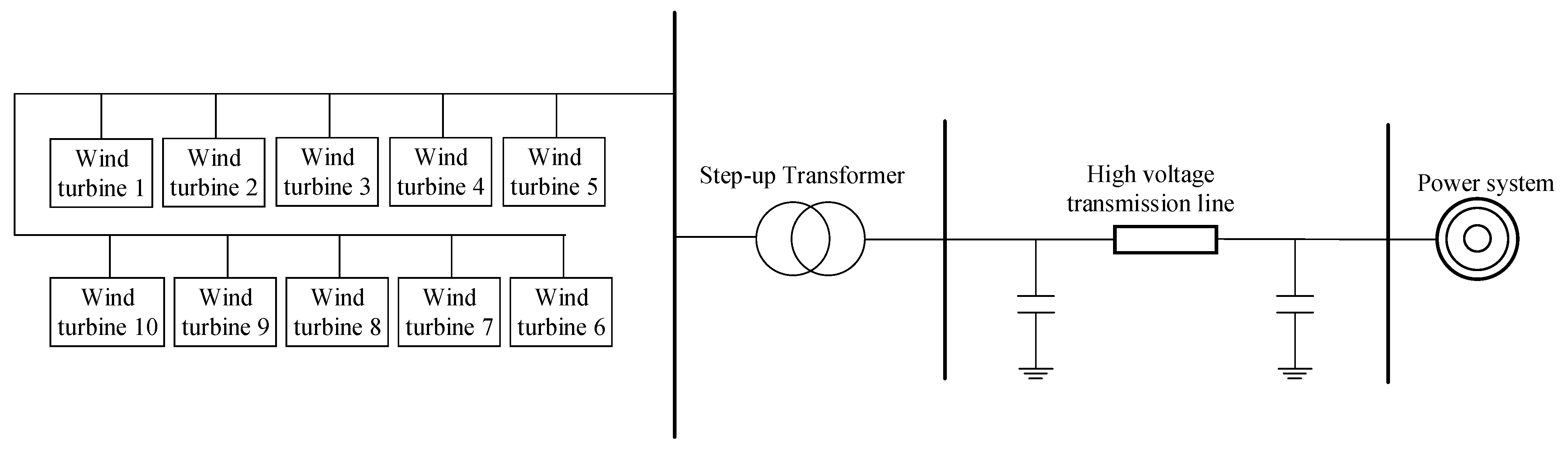
2. Wind Power Cluster Topology and Reactive Power Analysis
3. Wind Cluster Reactive Power Capacity Refinement Analysis
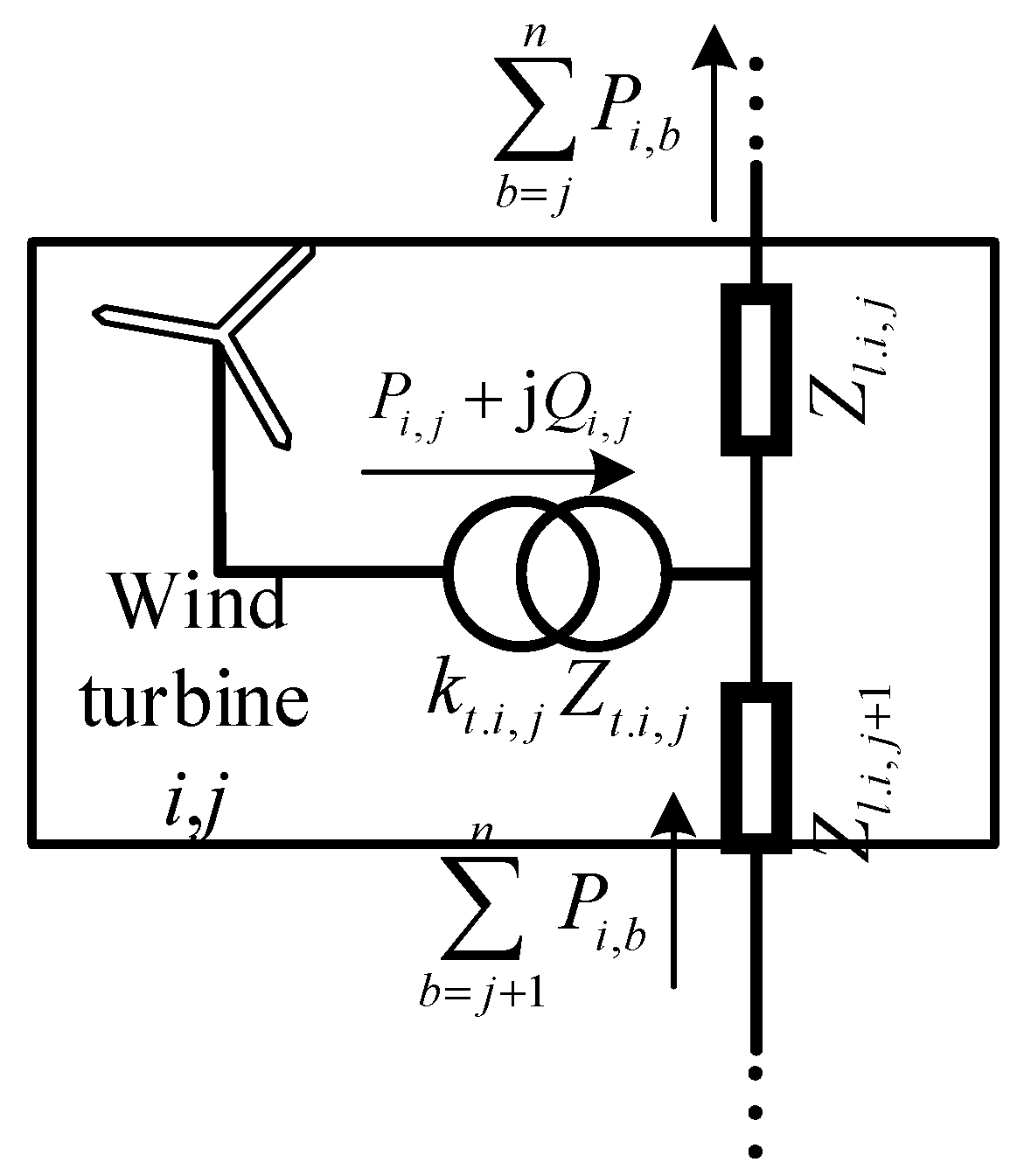
3.1. Analysis of Wind Turbine Reactive Power Loss
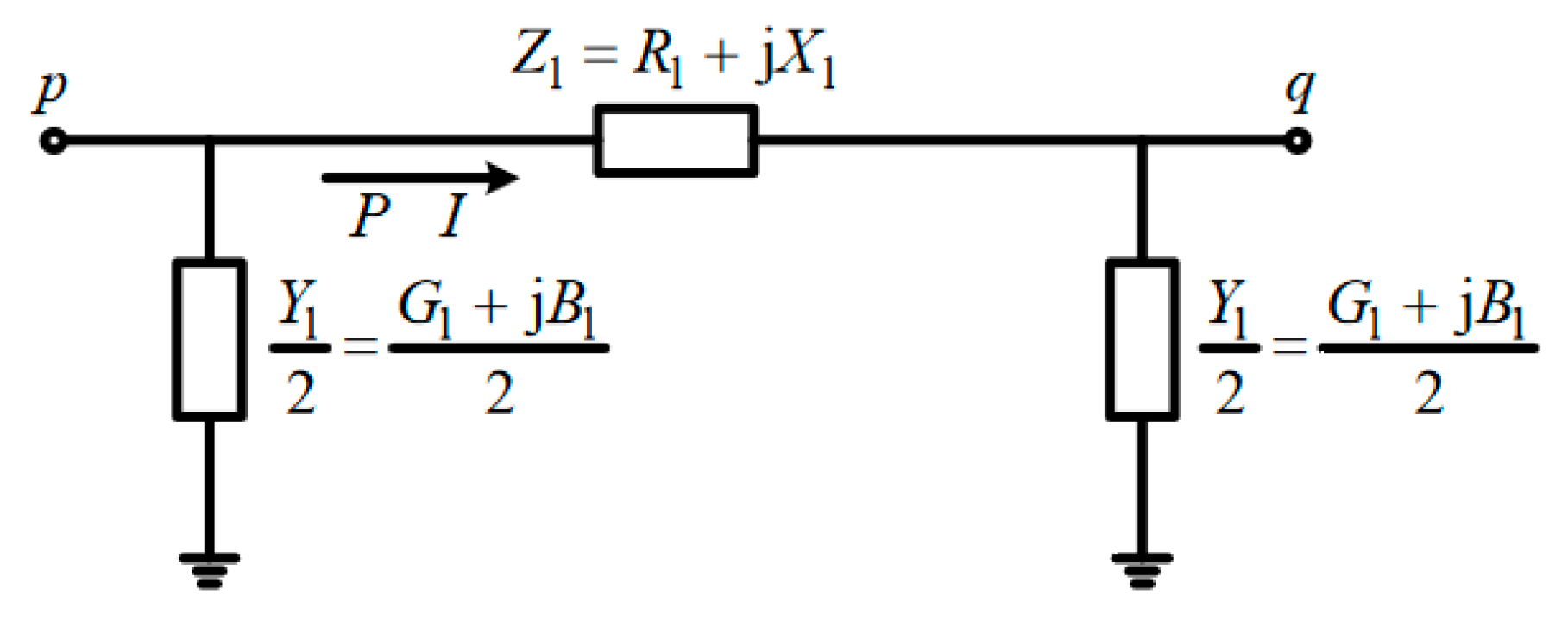
3.2. Analysis of Reactive Power Losses on Converging Lines
3.3. Total Reactive Power Capacity of Wind Farms
4. Bi-Level Reactive Power Optimization Model
4.1. Upper-Layer Optimization Model
- (1)
- Objective function
- (2)
- Constraints
4.2. Lower-Layer Optimization Model
- (1)
- Objective function
- (2)
- Constraints
4.3. Improved Artificial Fish Schooling Algorithm
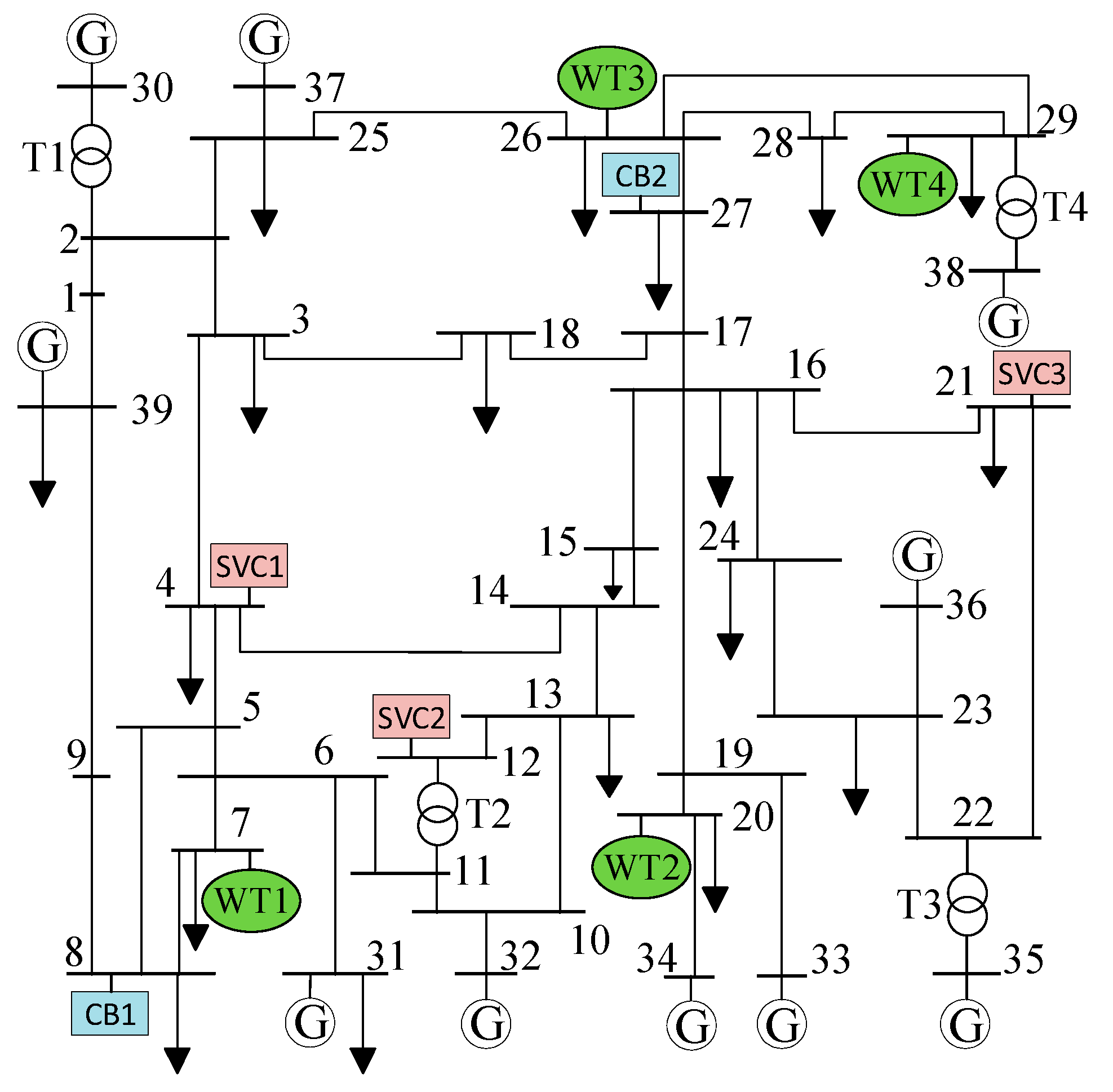
5. Case Study
5.1. Algorithm Parameter Settings
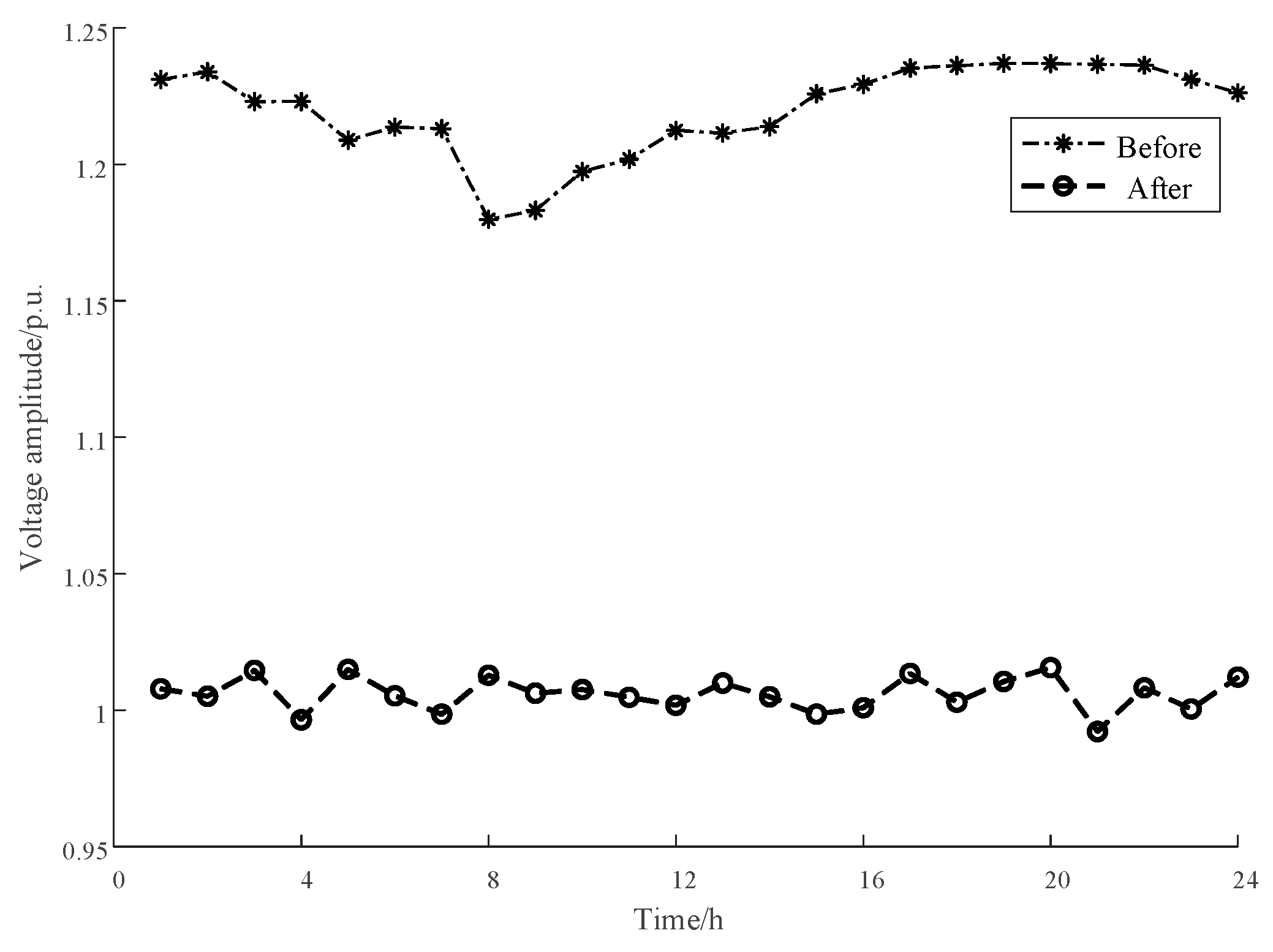
5.2. Model Verification
5.3. Comparative Analysis of Scenarios
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhuo, Z.; Zhang, N.; Hou, Q.; Du, E.; Kang, C. Backcasting Technical and Policy Targets for Constructing Low-Carbon Power Systems. IEEE Trans. Power Syst. 2022, 37, 4896–4911. [Google Scholar] [CrossRef]
- Jin, H.; Guo, J.; Tang, L.; Du, P. Long-Term Electricity Demand Forecasting under Low-Carbon Energy Transition: Based on the Bidirectional Feedback between Power Demand and Generation Mix. Energy 2024, 286, 129435. [Google Scholar] [CrossRef]
- Guţu-Chetruşca, C.; Braga, D. Energy Crises—Energy Transition Driving Force. In Proceedings of the 2023 International Conference on Electromechanical and Energy Systems (SIELMEN), Craiova, Romania, 11–13 October 2023; pp. 1–6. [Google Scholar]
- Ouyang, J.; Li, M.; Diao, Y.; Tang, T.; Xie, Q. Active Control Method of Large-Scale Wind Integrated Power System with Enhanced Reactive Power Support for Wind Speed Fluctuation. IET Gener. Transm. Distrib. 2018, 12, 5664–5671. [Google Scholar] [CrossRef]
- Zeng, L.; Chiang, H.-D. Online and Look-Ahead Determination of the Renewable Admissible Region for Managing the Uncertainty of Renewables: Theory and Some Applications. IEEE Trans. Power Syst. 2024, 39, 5609–5619. [Google Scholar] [CrossRef]
- Ibrahim, T.; Rubira, T.T.D.; Rosso, A.D.; Patel, M.; Guggilam, S.; Mohamed, A.A. Alternating Optimization Approach for Voltage-Secure Multi-Period Optimal Reactive Power Dispatch. IEEE Trans. Power Syst. 2022, 37, 3805–3816. [Google Scholar] [CrossRef]
- Yin, S.; Wu, L.; Song, W.; Wang, X. Multi-Objective Reactive Power Optimisation Approach for the Isolated Grid of New Energy Clusters Connected to VSC-HVDC. In Proceedings of the 6th International Conference on Renewable Power Generation, Wuhan, China, 19–20 October 2017; Institution of Engineering and Technology: Beijing, China, 2017; Volume 2017, pp. 1024–1028. [Google Scholar]
- Yang, M.; Liu, Y.; Guo, L.; Wang, Z.; Zhu, J.; Zhang, Y.; Cheng, B.; Wang, C. Hierarchical Distributed Chance-Constrained Voltage Control for HV and MV DNs Based on Nonlinearity-Adaptive Data-Driven Method. IEEE Trans. Power Syst. 2024, 1–14. [Google Scholar] [CrossRef]
- Lopez, J.; Gubia, E.; Olea, E.; Ruiz, J.; Marroyo, L. Ride Through of Wind Turbines With Doubly Fed Induction Generator Under Symmetrical Voltage Dips. IEEE Trans. Ind. Electron. 2009, 56, 4246–4254. [Google Scholar] [CrossRef]
- Zou, X.; Zhu, D.; Hu, J.; Zhou, S.; Kang, Y. Mechanism Analysis of the Required Rotor Current and Voltage for DFIG-Based WTs to Ride-Through Severe Symmetrical Grid Faults. IEEE Trans. Power Electron. 2018, 33, 7300–7304. [Google Scholar] [CrossRef]
- Shen, Y.; Zu, W.; Liang, L.; Zhang, B.; Li, Y.; Peng, Y. Comprehensive Evaluation of the Reactive Power and Voltage Control Capability of a Wind Farm Based on a Combined Weighting Method. Dianli Xitong Baohu Yu Kongzhi/Power Syst. Prot. Control 2020, 48, 18–24. [Google Scholar] [CrossRef]
- Li, Y.; Xu, Z.; Zhang, J.; Meng, K. Variable Droop Voltage Control For Wind FarmVariable Droop Voltage Control For Wind Farm. IEEE Trans. Sustain. Energy 2018, 9, 491–493. [Google Scholar] [CrossRef]
- Zhang, B.; Hu, W.; Hou, P.; Tan, J.; Soltani, M.; Chen, Z. Review of Reactive Power Dispatch Strategies for Loss Minimization in a DFIG-Based Wind Farm. Energies 2017, 10, 856. [Google Scholar] [CrossRef]
- Xu, Y.; Dong, Z.Y.; Zhang, R.; Hill, D.J. Multi-Timescale Coordinated Voltage/Var Control of High Renewable-Penetrated Distribution Systems. IEEE Trans. Power Syst. 2017, 32, 4398–4408. [Google Scholar] [CrossRef]
- von Appen, J.; Stetz, T.; Braun, M.; Schmiegel, A. Local Voltage Control Strategies for PV Storage Systems in Distribution Grids. IEEE Trans. Smart Grid 2014, 5, 1002–1009. [Google Scholar] [CrossRef]
- Agalgaonkar, Y.P.; Pal, B.C.; Jabr, R.A. Distribution Voltage Control Considering the Impact of PV Generation on Tap Changers and Autonomous Regulators. IEEE Trans. Power Syst. 2014, 29, 182–192. [Google Scholar] [CrossRef]
- Wang, P.; Wu, Q.; Huang, S.; Li, C.; Zhou, B. ADMM-Based Distributed Active and Reactive Power Control for Regional AC Power Grid with Wind Farms. J. Mod. Power Syst. Clean Energy 2022, 10, 588–596. [Google Scholar] [CrossRef]
- Kim, J.; Seok, J.-K.; Muljadi, E.; Kang, Y.C. Adaptive Q–V Scheme for the Voltage Control of a DFIG-Based Wind Power Plant. IEEE Trans. Power Electron. 2016, 31, 3586–3599. [Google Scholar] [CrossRef]
- Kim, J.; Muljadi, E.; Park, J.-W.; Kang, Y.C. Flexible IQ–V Scheme of a DFIG for Rapid Voltage Regulation of a Wind Power Plant. IEEE Trans. Ind. Electron. 2017, 64, 8832–8842. [Google Scholar] [CrossRef]
- Ren, X.; Wang, H.; Wang, Z.; Wang, Q.; Li, B.; Wu, H.; Yang, J.; Wang, W. Reactive Voltage Control of Wind Farm Based on Tabu Algorithm. Front. Energy Res. 2022, 10, 902623. [Google Scholar] [CrossRef]
- Deb, K.; Pratap, A.; Agarwal, S.; Meyarivan, T. A Fast and Elitist Multiobjective Genetic Algorithm: NSGA-II. IEEE Trans. Evol. Comput. 2002, 6, 182–197. [Google Scholar] [CrossRef]
- Yan, W.; Liu, F.; Chung, C.Y.; Wong, K.P. A Hybrid Genetic Algorithm–Interior Point Method for Optimal Reactive Power Flow. IEEE Trans. Power Syst. 2006, 21, 1163–1169. [Google Scholar] [CrossRef]
- Jayabarathi, T.; Jayaprakash, K.; Jeyakumar, D.N.; Raghunathan, T. Evolutionary Programming Techniques for Different Kinds of Economic Dispatch Problems. Electr. Power Syst. Res. 2005, 73, 169–176. [Google Scholar] [CrossRef]
- Ghasemi, M.; Aghaei, J.; Akbari, E.; Ghavidel, S.; Li, L. A Differential Evolution Particle Swarm Optimizer for Various Types of Multi-Area Economic Dispatch Problems. Energy 2016, 107, 182–195. [Google Scholar] [CrossRef]
- Li, R.; Yang, Y. Multi-Objective Capacity Optimization of a Hybrid Energy System in Two-Stage Stochastic Programming Framework. Energy Rep. 2021, 7, 1837–1846. [Google Scholar] [CrossRef]
- Koad, R.B.A.; Zobaa, A.F.; El-Shahat, A. A Novel MPPT Algorithm Based on Particle Swarm Optimization for Photovoltaic Systems. IEEE Trans. Sustain. Energy 2017, 8, 468–476. [Google Scholar] [CrossRef]
- Hou, P.; Chen, Z. Optimized Placement of Wind Turbines in Large-Scale Offshore Wind Farm Using Particle Swarm Optimization Algorithm. IEEE Trans. Sustain. Energy 2015, 6, 1272–1282. [Google Scholar] [CrossRef]
- Ahmed, I.; Alvi, U.-E.-H.; Basit, A.; Rehan, M.; Hong, K.-S. Multi-Objective Whale Optimization Approach for Cost and Emissions Scheduling of Thermal Plants in Energy Hubs. Energy Rep. 2022, 8, 9158–9174. [Google Scholar] [CrossRef]

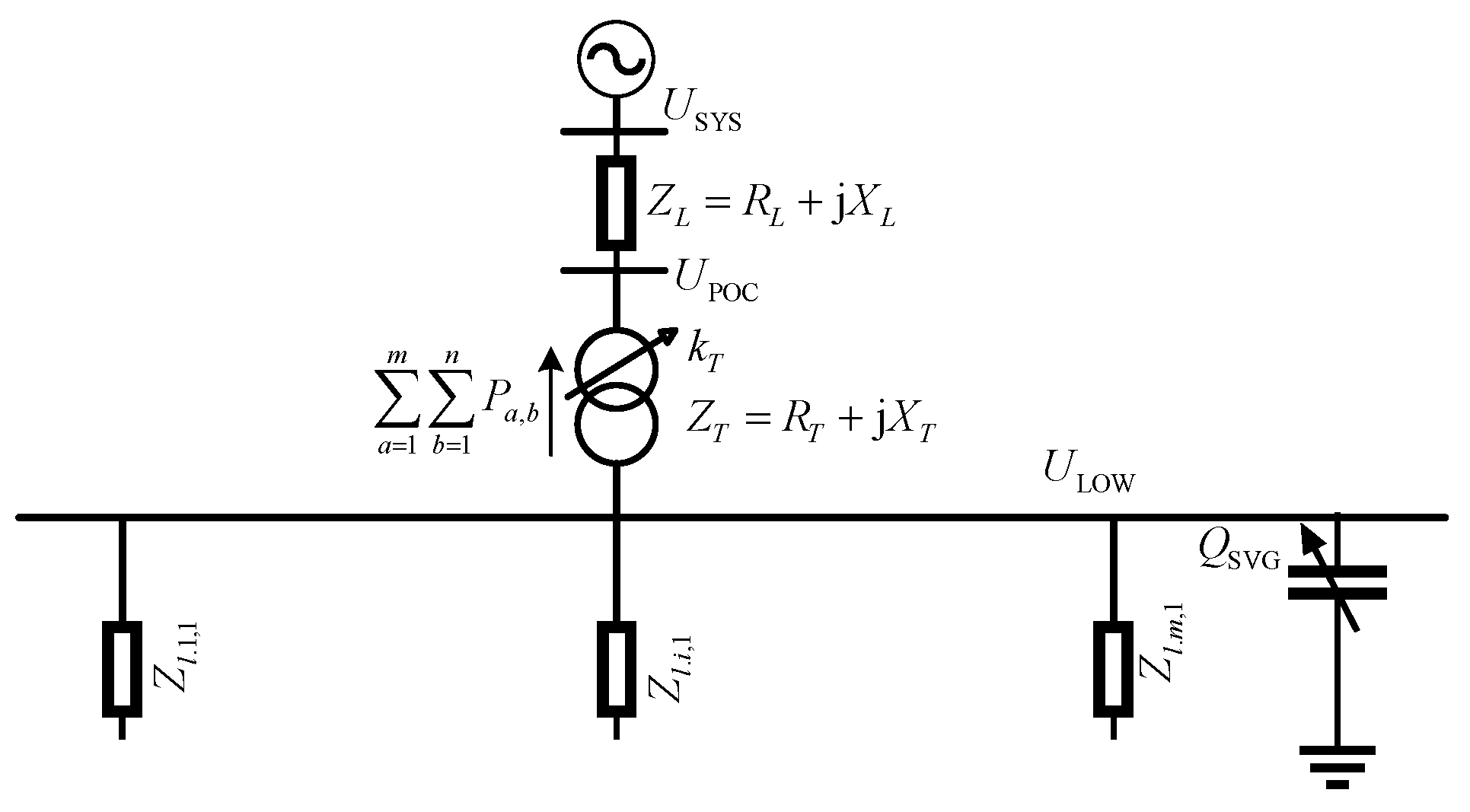

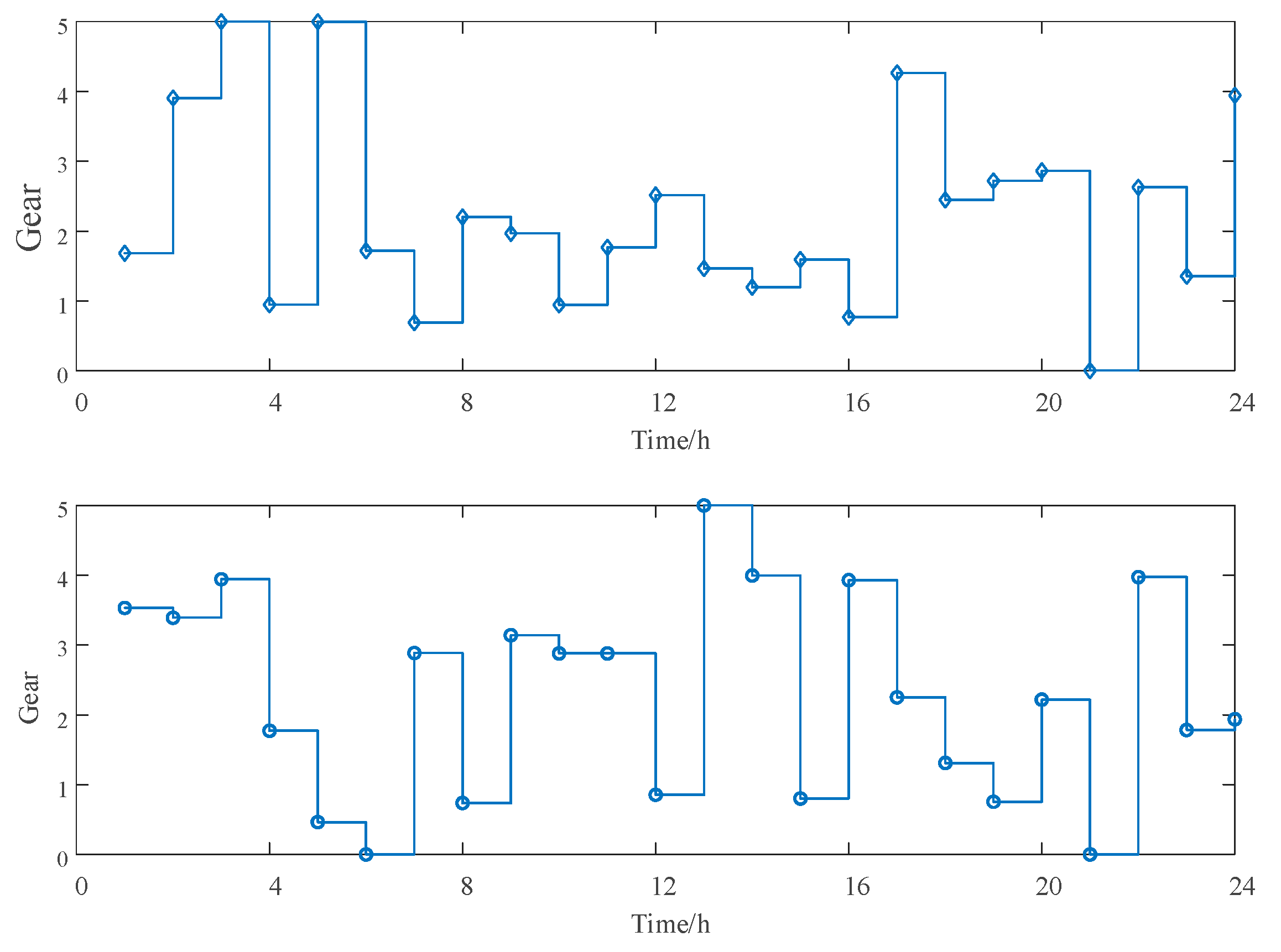
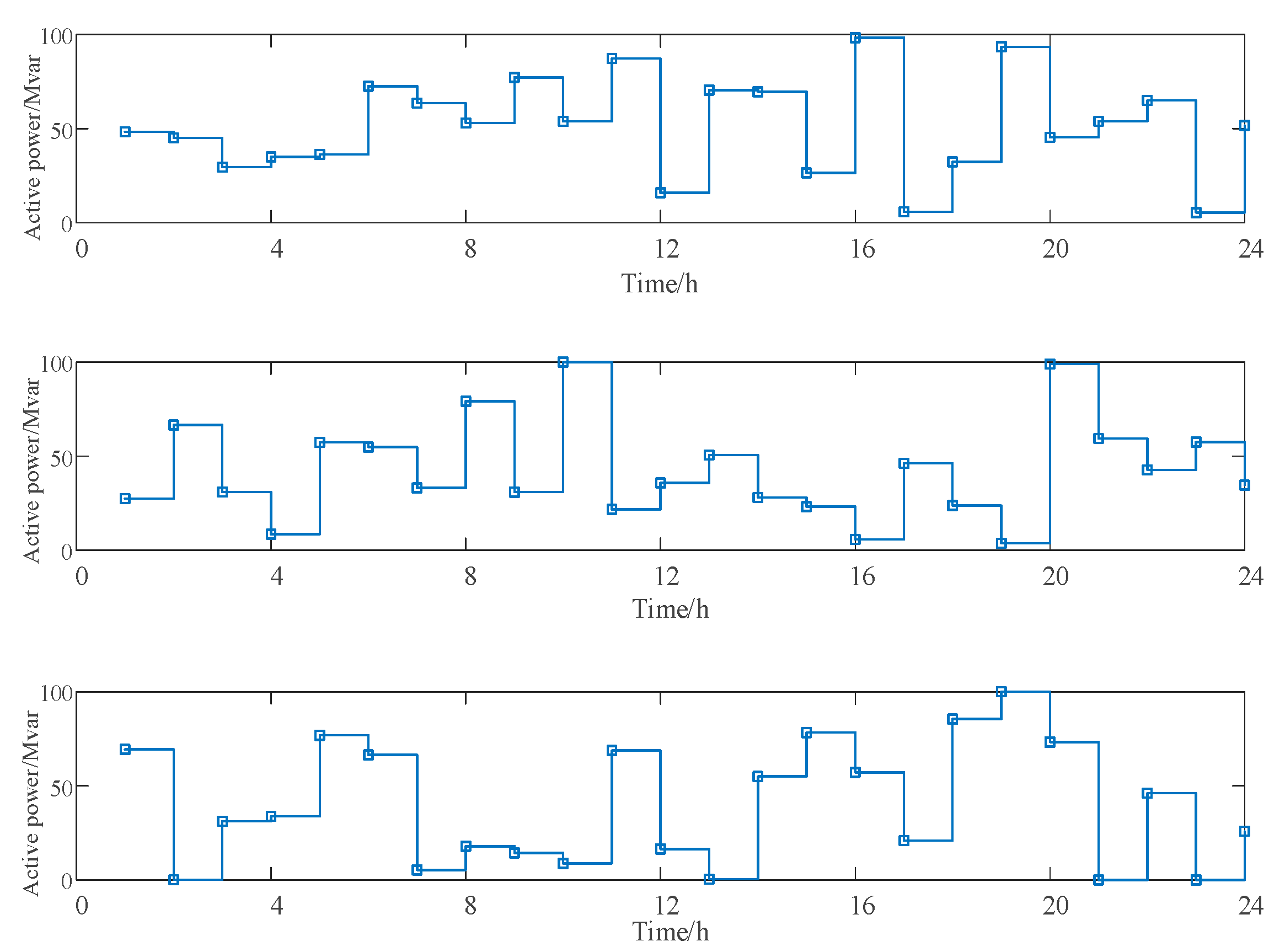
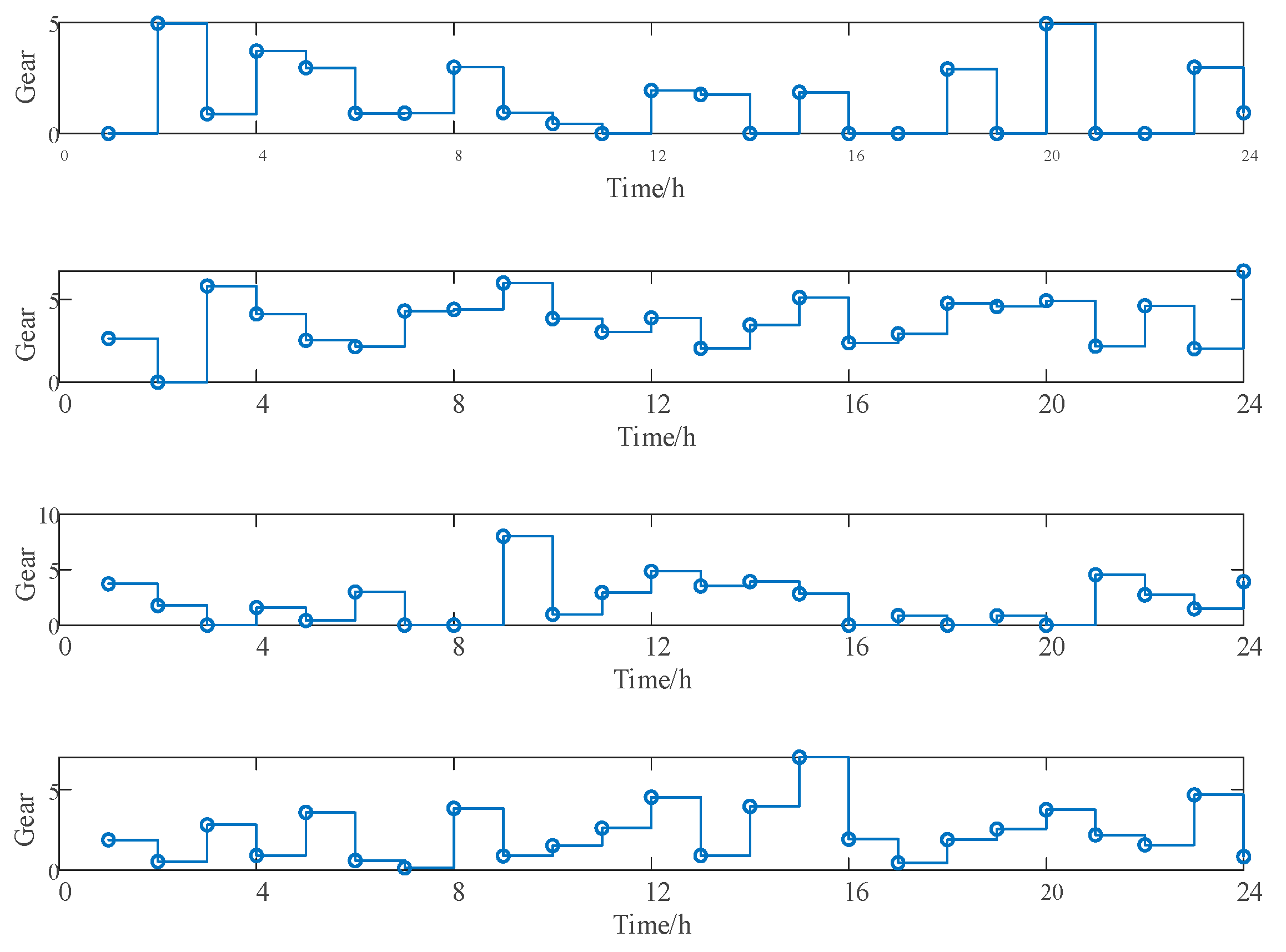
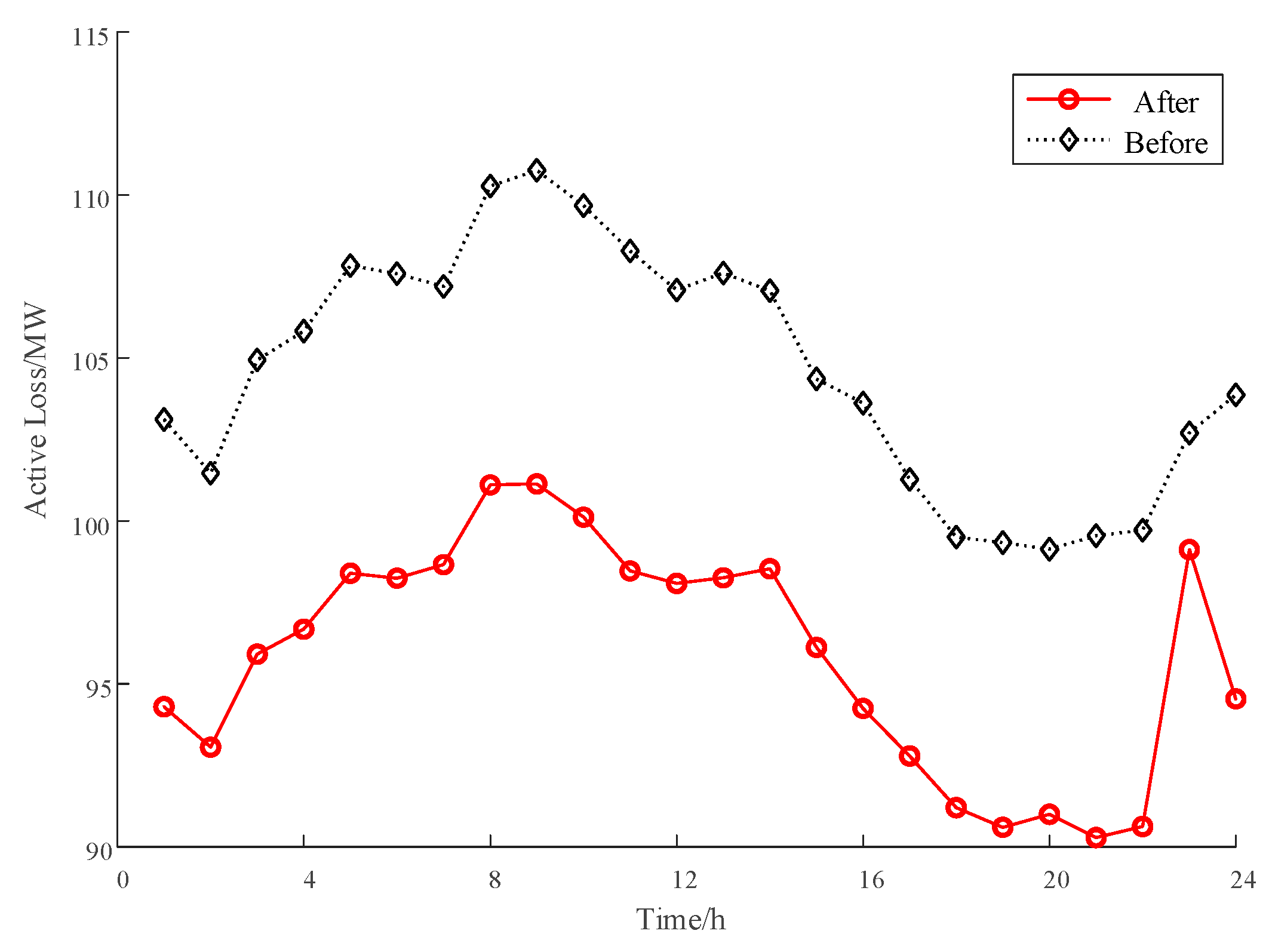
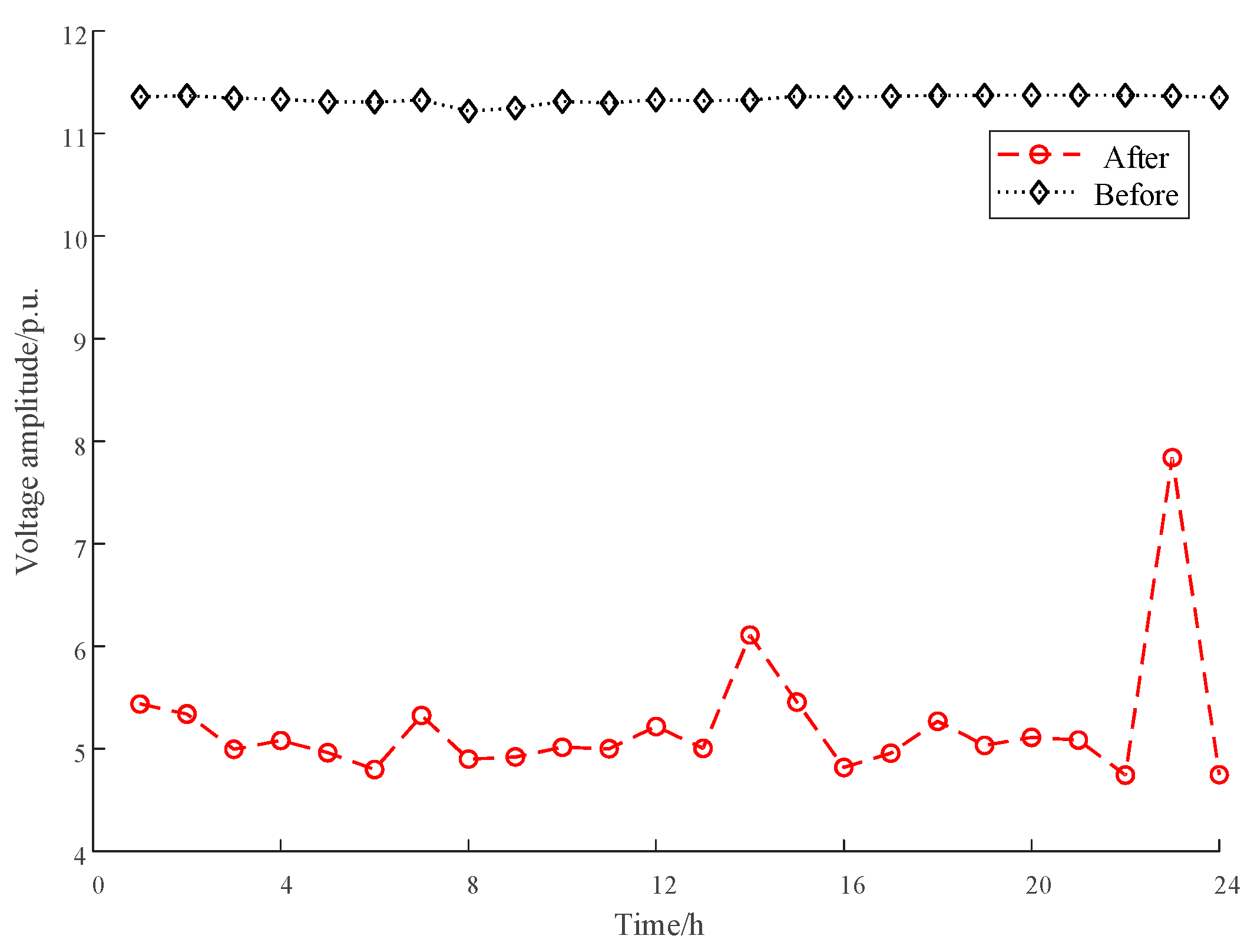

| Controllable Variable | Maximum Values | Minimum Value |
|---|---|---|
| Tk/p.u. | 1.1 | 0.9 |
| QCB/group | 5 | 0 |
| QSVC/Mvar | 100 | 0 |
| α/° | 18 | 8 |
| γ/° | 25 | 15 |
| Scenario | Active Power Loss/MW |
|---|---|
| Scenario 1 | 107.4 |
| Scenario 2 | 104.9 |
| Scenario 3 | 95.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, X.; Zhen, W.; Xu, R.; Dong, X.; Li, Y. A Bi-Level Reactive Power Optimization for Wind Clusters Integrating the Power Grid While Considering the Reactive Capability. Energies 2024, 17, 3910. https://doi.org/10.3390/en17163910
Ma X, Zhen W, Xu R, Dong X, Li Y. A Bi-Level Reactive Power Optimization for Wind Clusters Integrating the Power Grid While Considering the Reactive Capability. Energies. 2024; 17(16):3910. https://doi.org/10.3390/en17163910
Chicago/Turabian StyleMa, Xiping, Wenxi Zhen, Rui Xu, Xiaoyang Dong, and Yaxin Li. 2024. "A Bi-Level Reactive Power Optimization for Wind Clusters Integrating the Power Grid While Considering the Reactive Capability" Energies 17, no. 16: 3910. https://doi.org/10.3390/en17163910
APA StyleMa, X., Zhen, W., Xu, R., Dong, X., & Li, Y. (2024). A Bi-Level Reactive Power Optimization for Wind Clusters Integrating the Power Grid While Considering the Reactive Capability. Energies, 17(16), 3910. https://doi.org/10.3390/en17163910





