Harmonic Sequence Component Model-Based Small-Signal Stability Analysis in Synchronous Machines during Asymmetrical Faults
Abstract
1. Introduction
2. Sequence Component Model of the Synchronous Machine
- Calculation of the product mean: the mth phasor or the mean product of two signals and , as shown in [2], can be calculated as follows:
2.1. Dynamic Phasors in Polyphase Systems
2.2. Sequence Component Model of the Synchronous Machine in the Rotor Reference Frame
- For voltages:
- For magnetic fluxes:
- For currents:
- For the electrical torque:
- For the oscillation equation:
- For the rotor angle:
- ForExpanding the terms on the right-hand side of the equality for , the following definitions can be determined:where can be any of the currents of the damping windings or rotor field. It can be observed in (30) the need to identify the harmonic component with the greatest negative impact on rotor angle oscillations when the system is subjected to a large disturbance.
- For
- For
3. State Equation of the Synchronous Machine in the Harmonic Sequence Component Model
4. Sensitivity Analysis of the Eigenvalues
5. Participation Factors
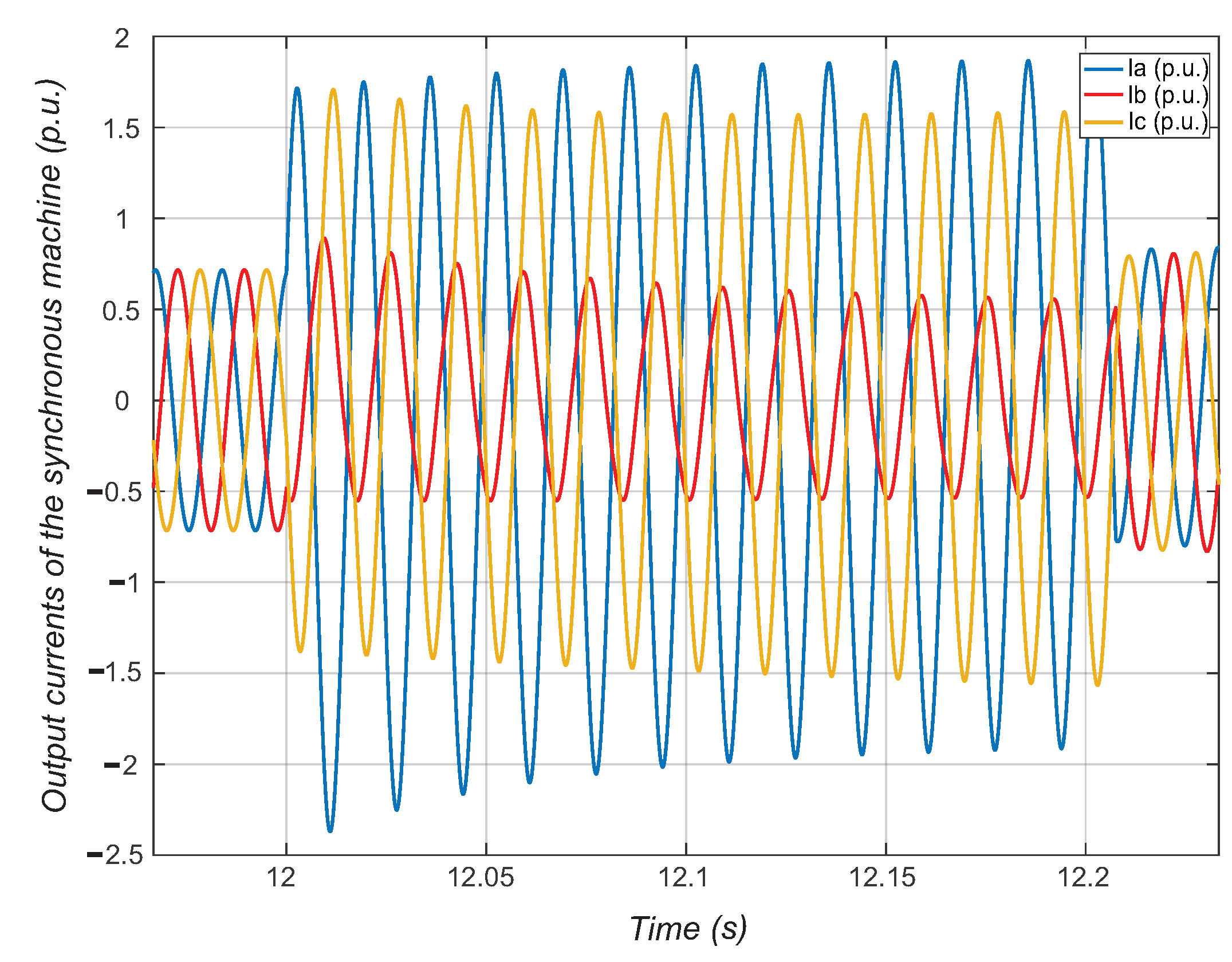
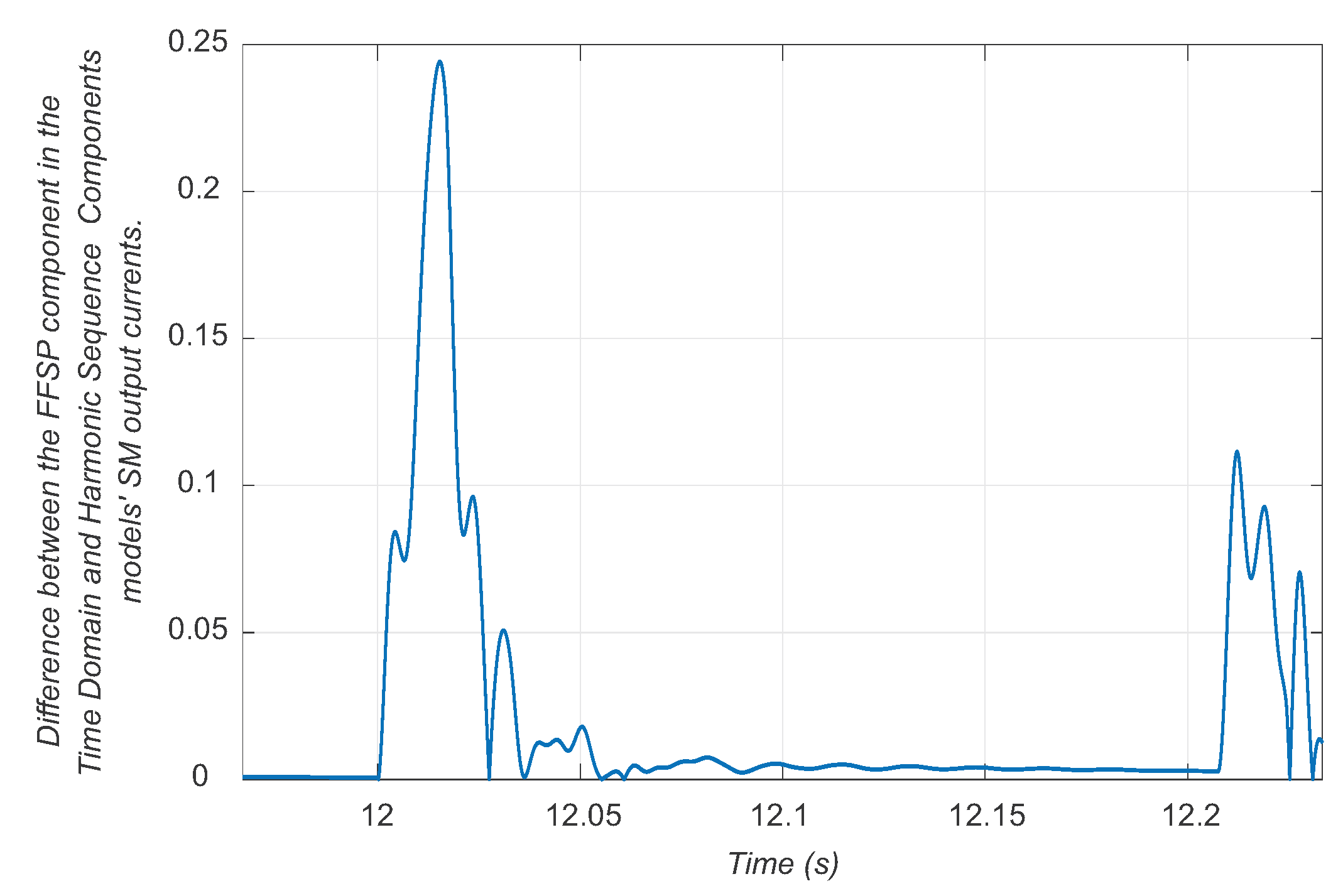
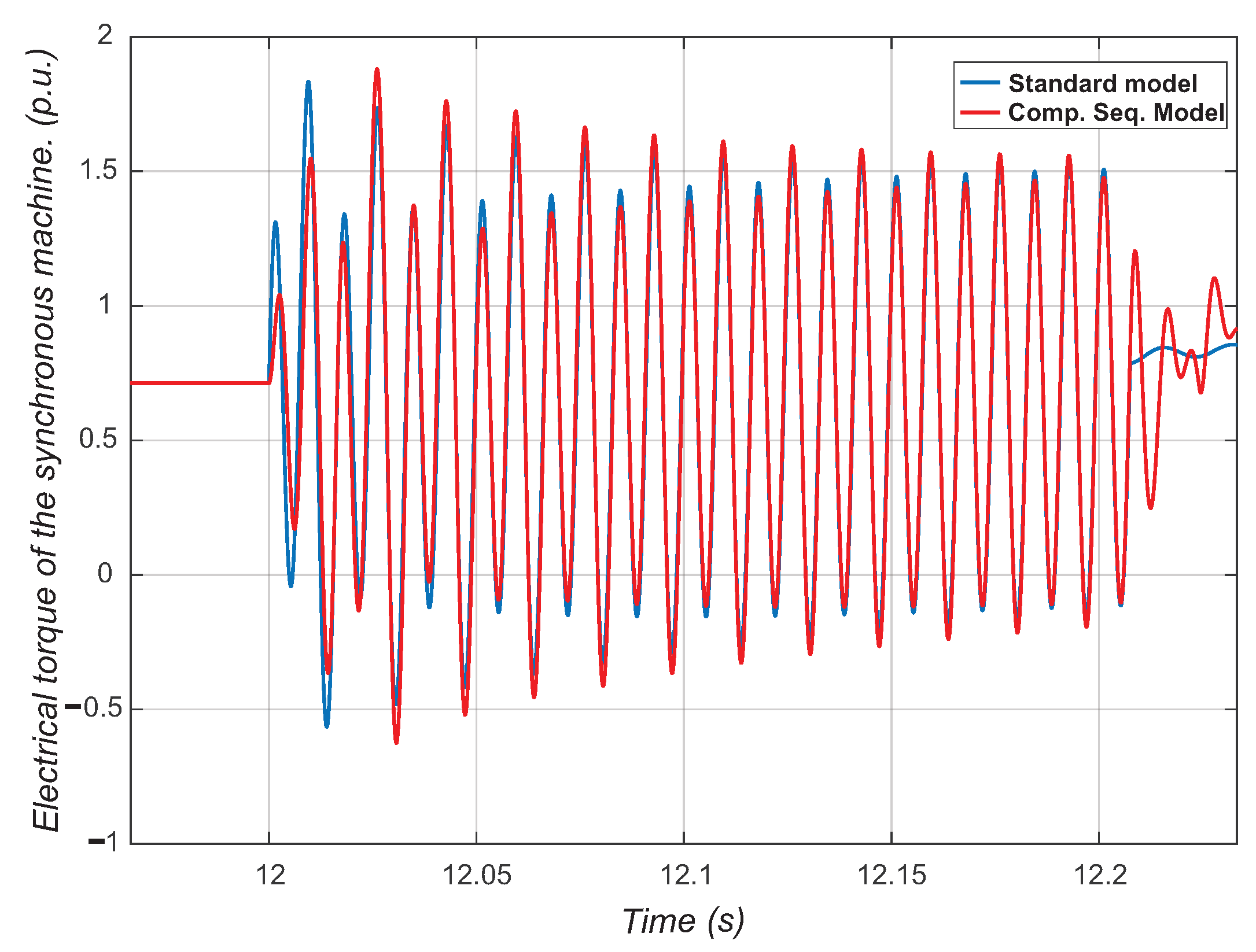
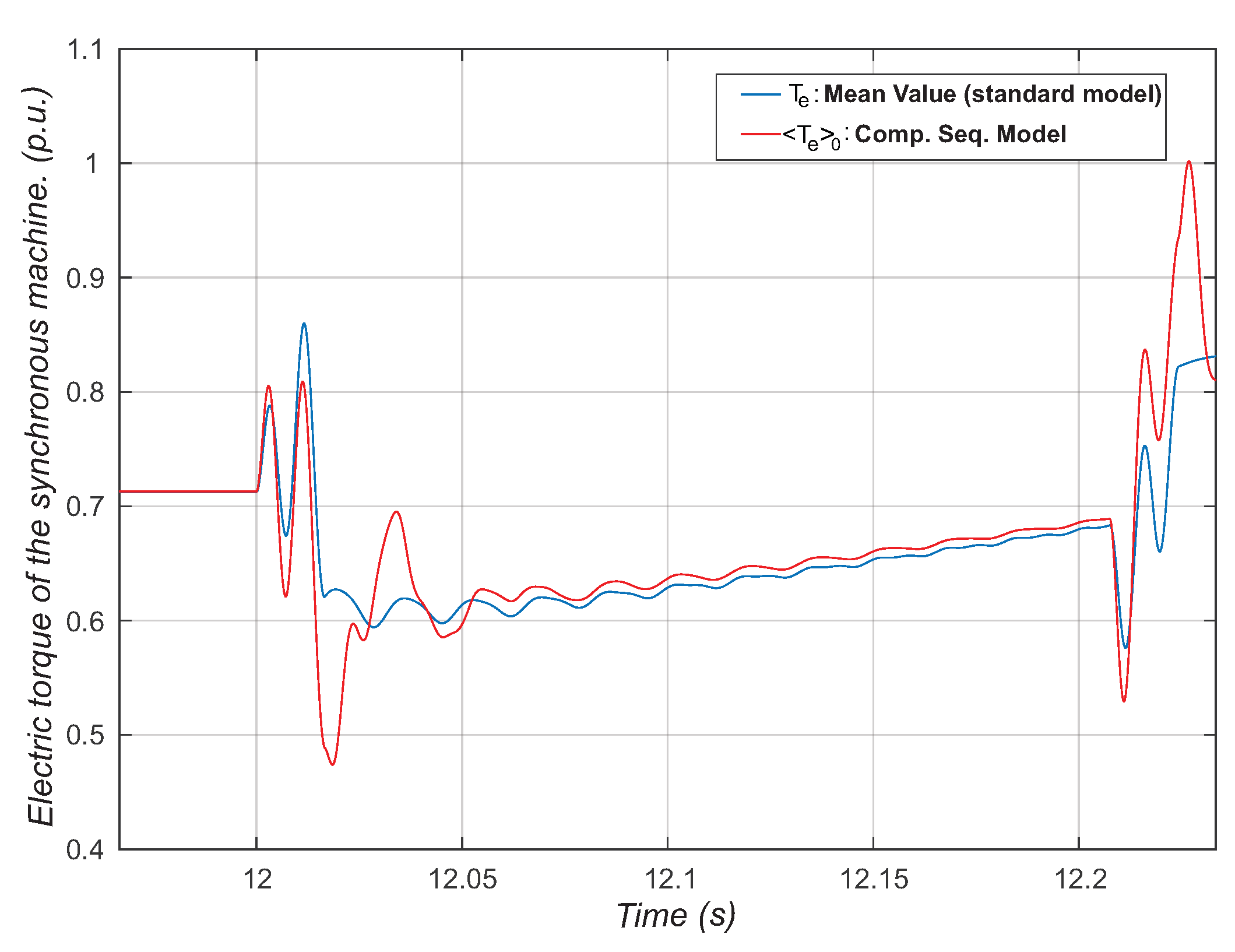
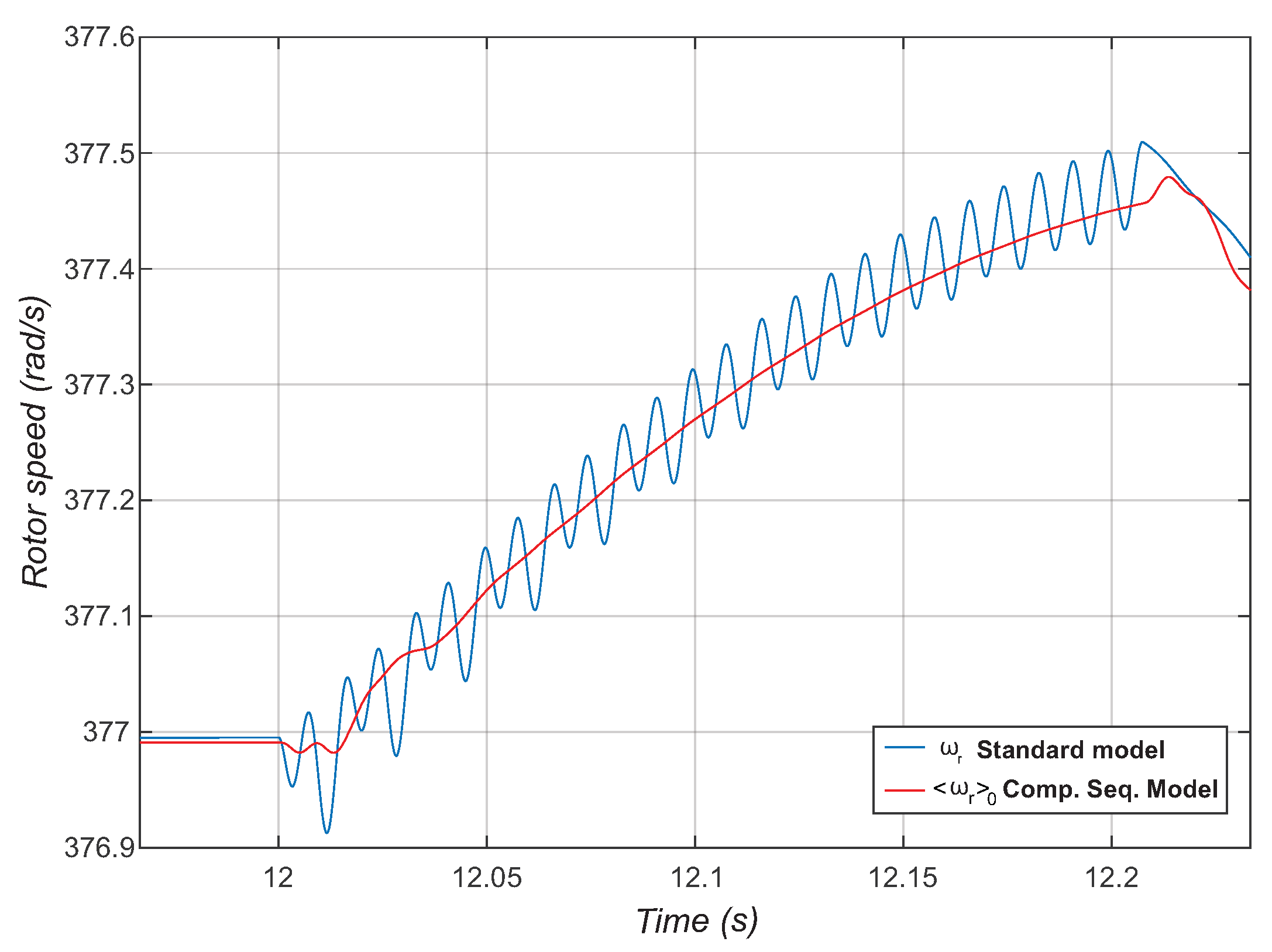
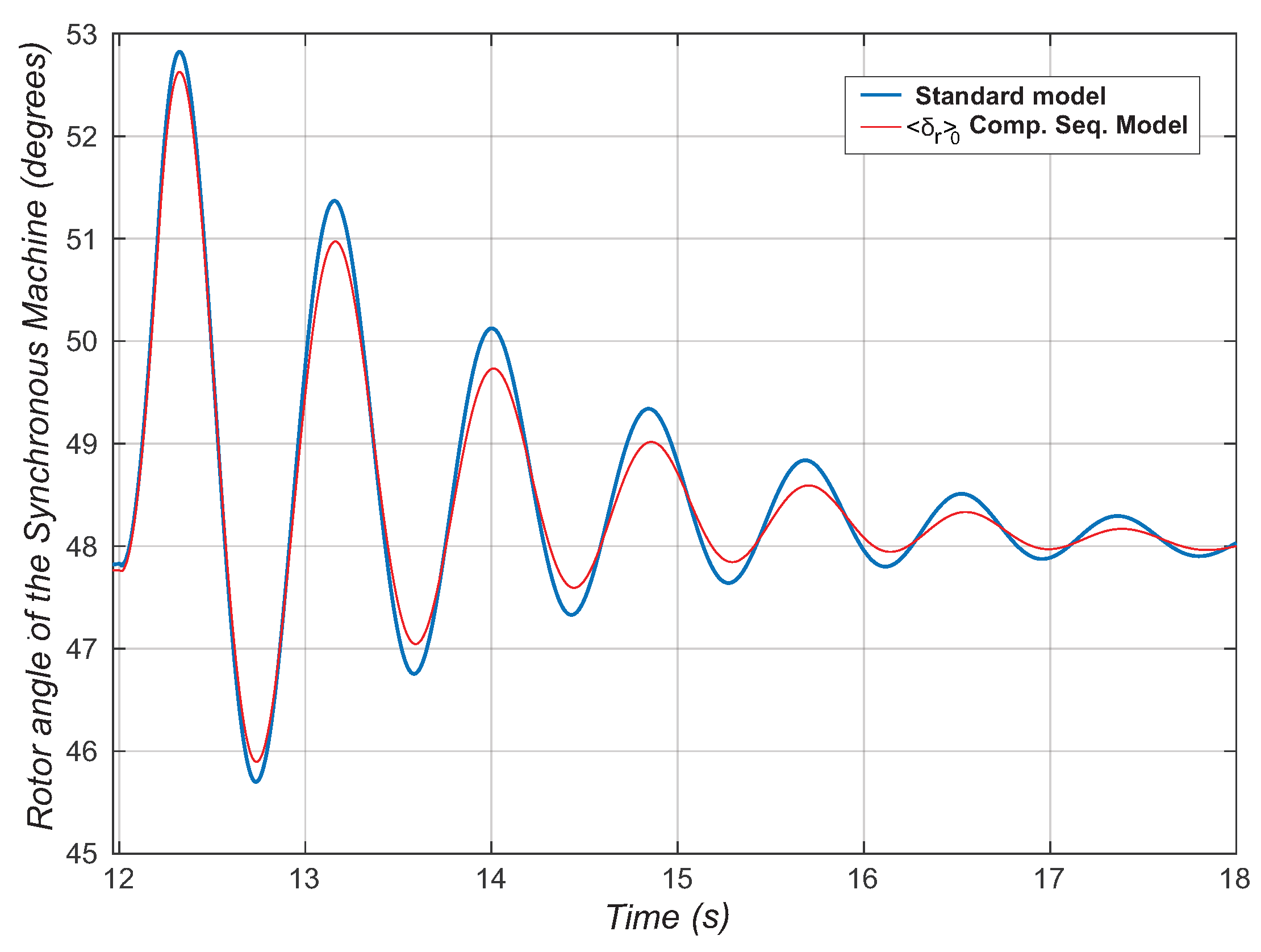
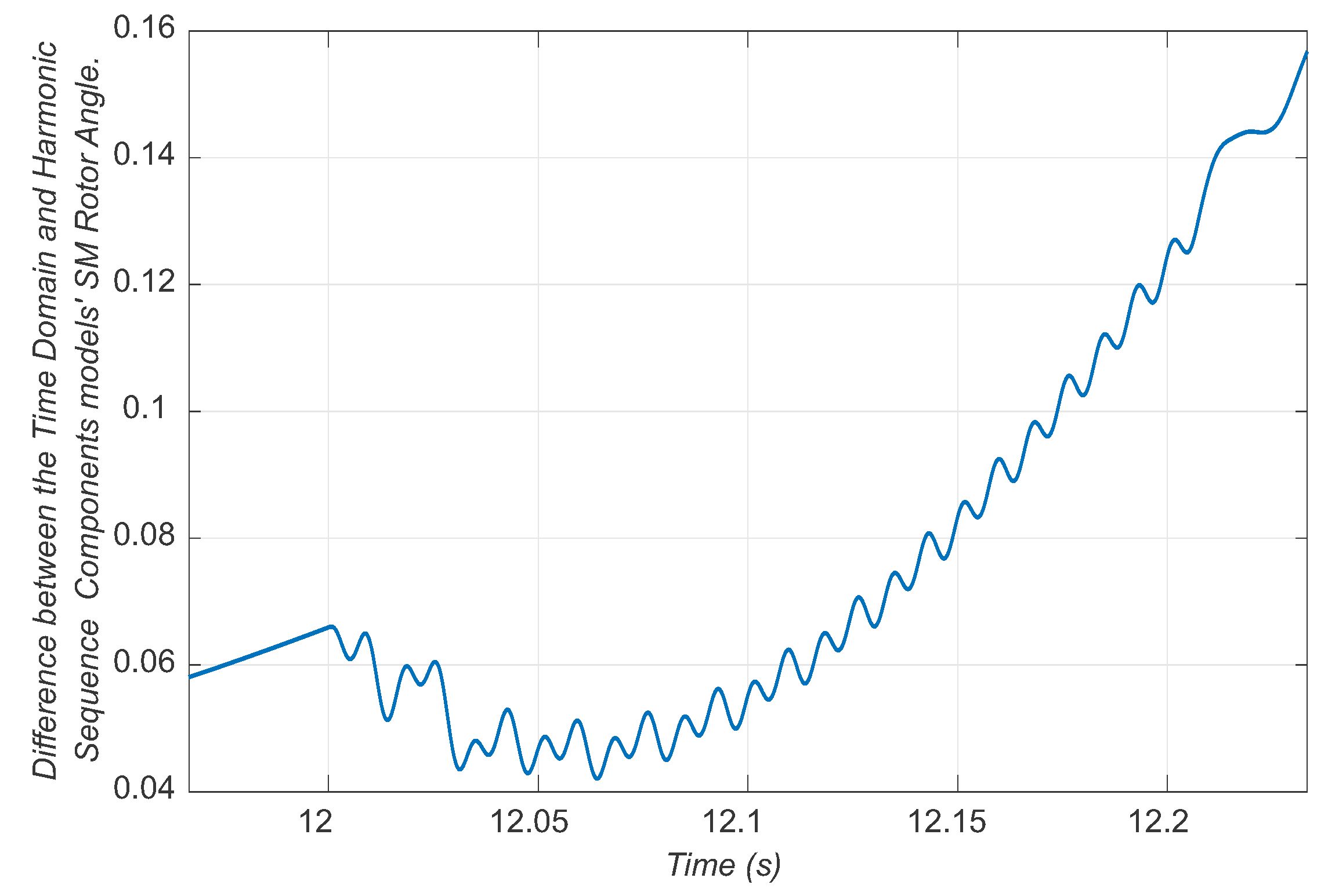
6. Comparison between Synchronous Machine Models in the Time Domain and Sequence Components
7. Sensitivity Analysis of the Test System
- a represents in dynamic phasors,
- b represents in dynamic phasors,
- c represents in dynamic phasors,
- d represents in dynamic phasors,
- e represents in dynamic phasors,
- f represents in dynamic phasors.
8. Conclusions
- We developed the state equation of a synchronous machine using the harmonic sequence component model;
- We used the state equation to perform a sensitivity analysis of the eigenvalues. The objective was to evaluate the effect of asymmetrical faults on the synchronous machine by determining critical modes through a sensitivity analysis of the rotor angular velocity and rotor angle;
- A participation factor analysis identified harmonic components with significant participation in the critical modes;
- While this paper focused on analyzing the impact of asymmetric faults on the dynamic behavior of a synchronous machine, the developed mathematical model can be applied to various operating conditions, provided that a sensitivity analysis is conducted on the variables directly affected by the contingency under consideration.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Complex Fourier coefficient of any electrical variable | |
| Complex Fourier coefficient in the stationary reference frame of any electrical variable | |
| Stator voltage referenced to the rotor in the rotating reference frame | |
| First rotor q- damping coil voltage | |
| Second rotor q- damping coil voltage | |
| Rotor d- field coil voltage | |
| Rotor d- damping coil voltage | |
| Stator current referenced to the rotor in the rotating reference frame | |
| First rotor q- damping coil current | |
| Second rotor q- damping coil current | |
| Rotor d- field coil current | |
| Rotor d- damping coil current | |
| Stator flux referenced to the rotor in the rotating reference frame | |
| First damping coil rotor flux in the q- reference frame | |
| Second damping coil rotor flux in the q- reference frame | |
| Field coil rotor flux in the d- reference frame | |
| Damping coil rotor flux in the d- reference frame | |
| Stator resistance | |
| First q- damping resistance | |
| Second q- damping resistance | |
| d- field resistance | |
| d- damping resistance | |
| d- reactance | |
| d- mutual reactance | |
| q- mutual reactance | |
| q- reactance | |
| First q- damping reactance | |
| Second q- damping reactance | |
| d- field reactance | |
| d- damping reactance | |
| Electrical torque | |
| Mechanical torque | |
| H | Inertia constant |
| Synchronous angular velocity | |
| Rotor angular velocity | |
| h | Harmonic order in the stationary reference frame |
| k | Harmonic order in the rotating reference frame |
| Complex Fourier coefficient or dynamic phasor of any electrical variable in the rotor reference frame | |
| Dynamic phasor of the conjugate spatial vector of any electrical variable in the rotor reference frame | |
| A | State matrix |
| Roots of the characteristic equation or eigenvalues | |
| Right eigenvector of the state matrix A | |
| Left eigenvector of the state matrix A | |
| P | Participation factors’ matrix |
References
- Krause, P.C.; Wasynczuk, O.; Sudhoff, S.D.; Pekarek, S. Analysis of Electric Machinery and Drive Systems; Wiley Online Library: Hoboken, NJ, USA, 2002; Volume 2. [Google Scholar]
- Caliskan, V.A.; Verghese, O.; Stankovic, A.M. Multifrequency averaging of DC/DC converters. IEEE Trans. Power Electron. 1999, 14, 124–133. [Google Scholar] [CrossRef]
- Sanders, S.R. On limit cycles and the describing function method in periodically switched circuits. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 1993, 40, 564–572. [Google Scholar] [CrossRef]
- Stankovic, A.M.; Sanders, S.R.; Aydin, T. Dynamic phasors in modeling and analysis of unbalanced polyphase AC machines. IEEE Trans. Energy Convers. 2002, 17, 107–113. [Google Scholar] [CrossRef]
- De Rua, P.; Sakinci, Ö.C.; Beerten, J. Comparative study of dynamic phasor and harmonic state-space modeling for small-signal stability analysis. Electr. Power Syst. Res. 2020, 189, 106626. [Google Scholar] [CrossRef]
- Sakinci, Ö.C.; Beerten, J. Generalized dynamic phasor modeling of the MMC for small-signal stability analysis. IEEE Trans. Power Deliv. 2019, 34, 991–1000. [Google Scholar] [CrossRef]
- Wang, X.; Blaabjerg, F. Harmonic stability in power electronic-based power systems: Concept, modeling, and analysis. IEEE Trans. Smart Grid 2018, 10, 2858–2870. [Google Scholar] [CrossRef]
- Lyu, J.; Zhang, X.; Cai, X.; Molinas, M. Harmonic state-space based small-signal impedance modeling of a modular multilevel converter with consideration of internal harmonic dynamics. IEEE Trans. Power Electron. 2018, 34, 2134–2148. [Google Scholar] [CrossRef]
- Yue, X.; Wang, X.; Blaabjerg, F. Review of small-signal modeling methods including frequency-coupling dynamics of power converters. IEEE Trans. Power Electron. 2018, 34, 3313–3328. [Google Scholar] [CrossRef]
- Yang, T.; Bozhko, S.; Asher, G. Assessment of dynamic phasors modelling technique for accelerated electric power system simulations. In Proceedings of the 2011 14th European Conference on Power Electronics and Applications, Birmingham, UK, 30 August–1 September 2011; pp. 1–9. [Google Scholar]
- Chudasama, M.C.; Kulkarni, A.M. Dynamic phasor analysis of SSR mitigation schemes based on passive phase imbalance. IEEE Trans. Power Syst. 2010, 26, 1668–1676. [Google Scholar] [CrossRef]
- Demiray, T.; Andersson, G.; Busarello, L. Evaluation study for the simulation of power system transients using dynamic phasor models. In Proceedings of the 2008 IEEE/PES Transmission and Distribution Conference and Exposition: Latin America, Bogota, Colombia, 13–15 August 2008; pp. 1–6. [Google Scholar]
- Bai, X.; Jiang, T.; Guo, Z.; Yan, Z.; Ni, Y. A unified approach for processing unbalanced conditions in transient stability calculations. IEEE Trans. Power Syst. 2006, 21, 85–90. [Google Scholar] [CrossRef]
- Kotian, S.M.; Shubhanga, K. Dynamic phasor modelling and simulation. In Proceedings of the 2015 Annual IEEE India Conference (INDICON), New Delhi, India, 17–20 December 2015; pp. 1–6. [Google Scholar]
- Meersman, B.; Degroote, L.; Renders, B.; Vandevelde, L. Simulating transients in electrical power systems using dynamic phasors. In Proceedings of the Proc. IEEE Benelux Young Researchers Symposium in Electrical Power Engineering (YRS’08), Eindhoven, The Netherlands, 7–8 February 2008. [Google Scholar]
- Stankovic, A.M.; Aydin, T. Analysis of asymmetrical faults in power systems using dynamic phasors. IEEE Trans. Power Syst. 2000, 15, 1062–1068. [Google Scholar] [CrossRef]
- Huang, S.; Song, R.; Zhou, X. Analysis of balanced and unbalanced faults in power systems using dynamic phasors. In Proceedings of the International Conference on Power System Technology, Kunming, China, 13–17 October 2002; Volume 3, pp. 1550–1557. [Google Scholar]
- Novotny, D.W.; Lipo, T.A. Vector Control and Dynamics of AC Drives; Oxford University Press: Oxford, UK, 1996; Volume 41. [Google Scholar]
- Kundur, P.S.; Balu, N.J.; Lauby, M.G. Power system dynamics and stability. Power Syst. Stab. Control 2017, 3, 700–701. [Google Scholar]
- Nolan, P.J.; Sinha, N.K.; Alden, R.T. Eigenvalue sensitivities of power systems including network and shaft dynamics. IEEE Trans. Power Appar. Syst. 1976, 95, 1318–1324. [Google Scholar] [CrossRef]

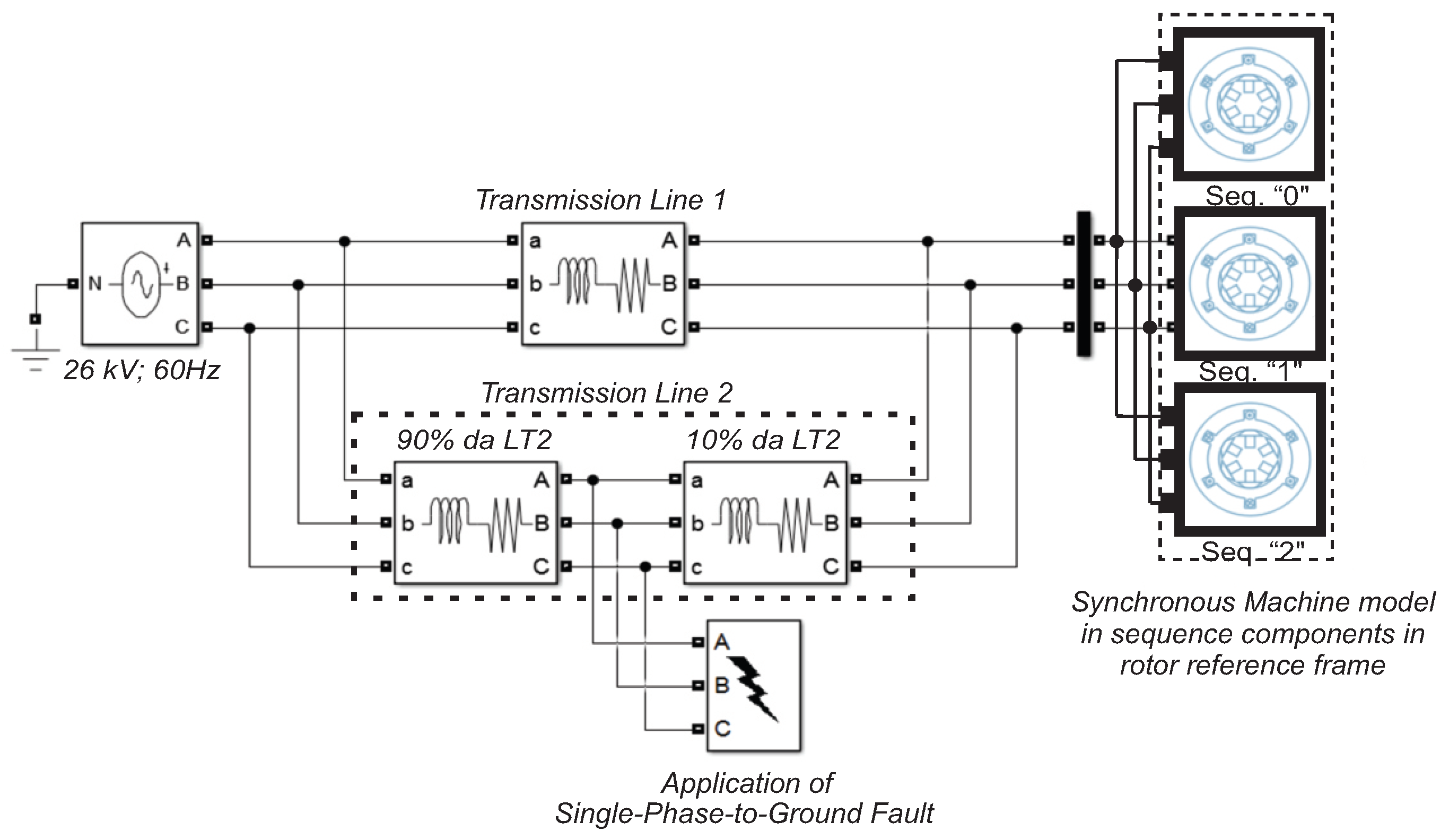



| MVA | V = 26 kV |
| 0.85 | |
| J·s2, H = 5.6 s | = 3600 rpm |
| , 0.9 p.u. | , 0.003 p.u. |
| , 1.8 p.u. | , 1.8 p.u. |
| , 0.00178 p.u. | , 0.000929 p.u. |
| , 0.8125 p.u. | , 0.1414 p.u. |
| , 0.00841 p.u. | , 0.01334 p.u. |
| , 0.0939 p.u. | , 0.08125 p.u. |
| Real Part of | Freq. (Hz) | Sensitivity | Sensitivity | Dominant Variable | |
|---|---|---|---|---|---|
| 1–2 | −5.5 | −0.3 | 0.6 | - | |
| 3 | −65.1 | 0 | −3.7 | −3.3 | |
| 4 | −27.2 | 0 | −1.6 | 2.0 | |
| 5 | 0 | 0 | 0 | 0 | - |
| 6–7 | −3 | 2.9 | −0.1 | ||
| 8 | −0.3 | 0 | −0.001 | 0.5 | - |
| 9–10 | −5.4 | −0.2 | 0 | - | |
| 11–12 | −5.4 | −0.2 | 0 | - | |
| 13–14 | −69.5 | 0.3 | 0 | - | |
| 15–16 | −28.6 | 0.02 | 0 | - | |
| 17–18 | −0.7 | 0 | 0 | - | |
| 19–20 | 0 | 0 | 0 | - | |
| 21–22 | −5.44 | −0.2 | 0 | - | |
| 23–24 | −5.44 | −0.2 | 0 | - | |
| 25–26 | −69.5 | 0.3 | 0 | - | |
| 27–28 | −28.6 | 0.02 | 0 | - | |
| 29–30 | −0.7 | 0 | 0 | - | |
| 31–32 | 0 | 0 | 0 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zevallos, O.C.; Gallego Landera, Y.A.; León Viltre, L.; Rohten Carrasco, J.A. Harmonic Sequence Component Model-Based Small-Signal Stability Analysis in Synchronous Machines during Asymmetrical Faults. Energies 2024, 17, 3634. https://doi.org/10.3390/en17153634
Zevallos OC, Gallego Landera YA, León Viltre L, Rohten Carrasco JA. Harmonic Sequence Component Model-Based Small-Signal Stability Analysis in Synchronous Machines during Asymmetrical Faults. Energies. 2024; 17(15):3634. https://doi.org/10.3390/en17153634
Chicago/Turabian StyleZevallos, Oscar C., Yandi A. Gallego Landera, Lesyani León Viltre, and Jaime Addin Rohten Carrasco. 2024. "Harmonic Sequence Component Model-Based Small-Signal Stability Analysis in Synchronous Machines during Asymmetrical Faults" Energies 17, no. 15: 3634. https://doi.org/10.3390/en17153634
APA StyleZevallos, O. C., Gallego Landera, Y. A., León Viltre, L., & Rohten Carrasco, J. A. (2024). Harmonic Sequence Component Model-Based Small-Signal Stability Analysis in Synchronous Machines during Asymmetrical Faults. Energies, 17(15), 3634. https://doi.org/10.3390/en17153634







