Cold Storage and Release Characteristics of Phase Change Cold Storage Plate with Non-Uniform Fins
Abstract
1. Introduction
2. Mathematical Models
2.1. Control Equations
- (1)
- The heat transfer equation for ice (water) inside the ISP is:
- (2)
- The heat transfer equation for the wall of the ISP is:
- (3)
- Air model
- (4)
- Initial conditions
- (5)
- Boundary conditions of box wall surface
- (6)
- Thermo-physical properties
2.2. Solution Methods
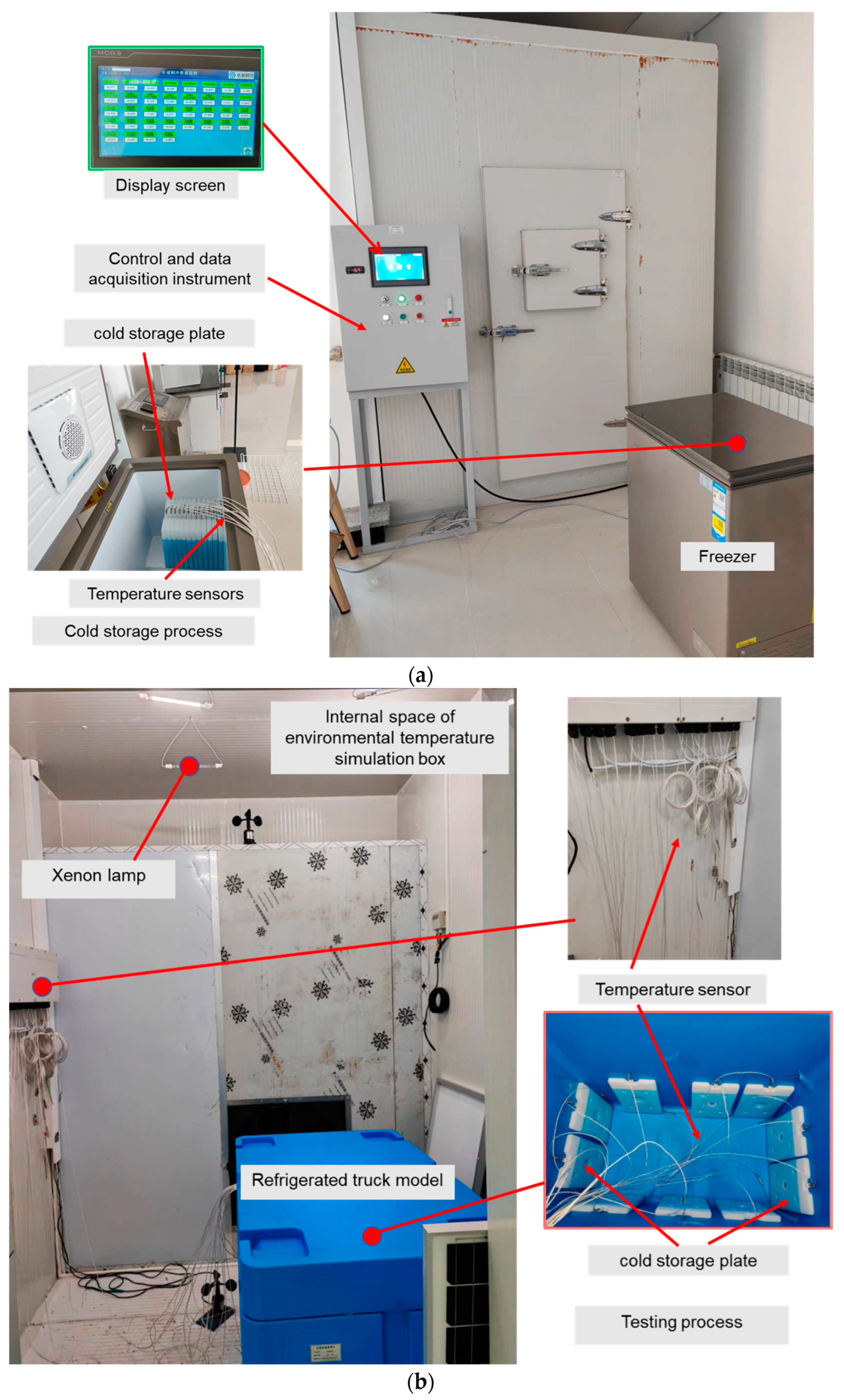
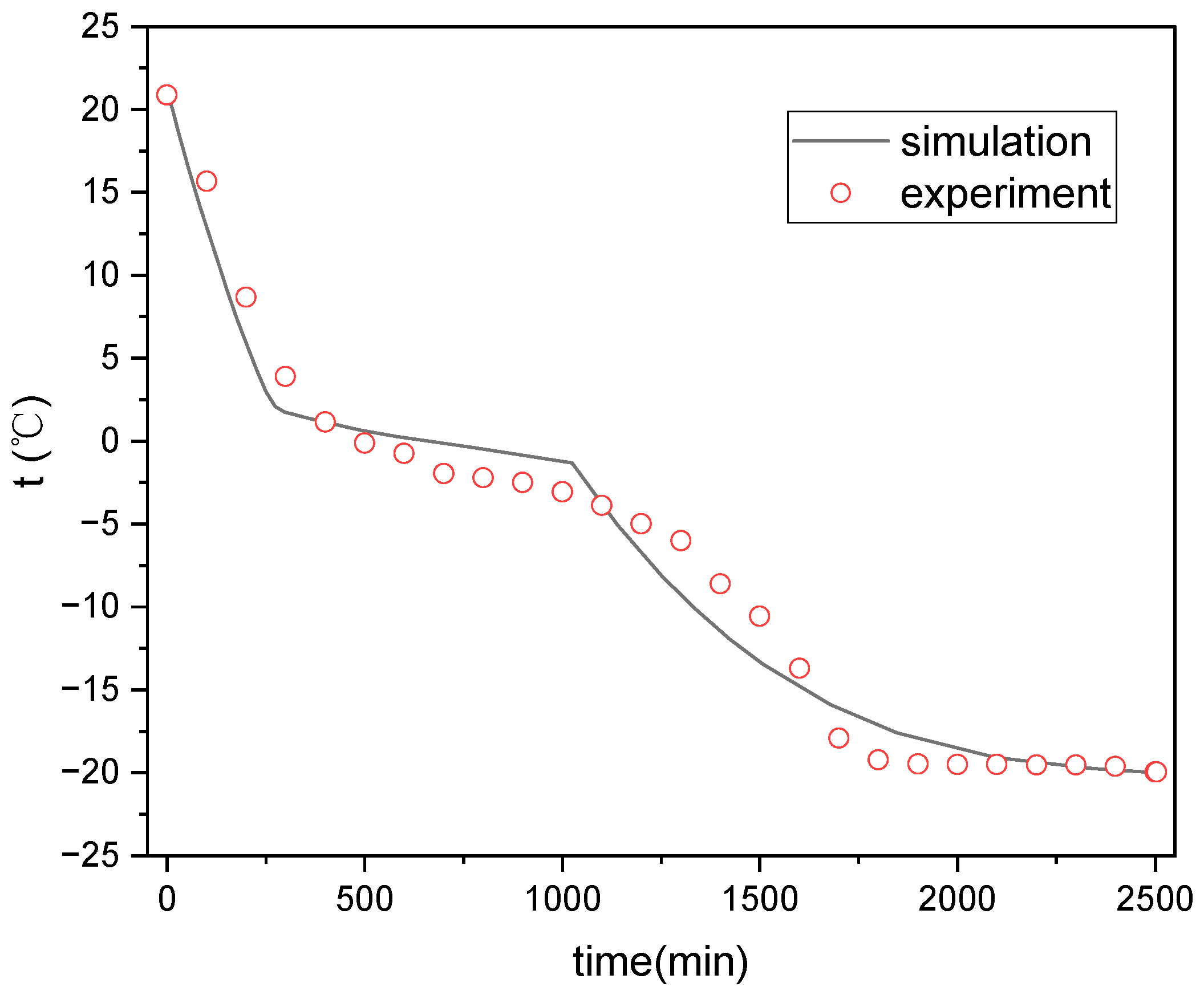
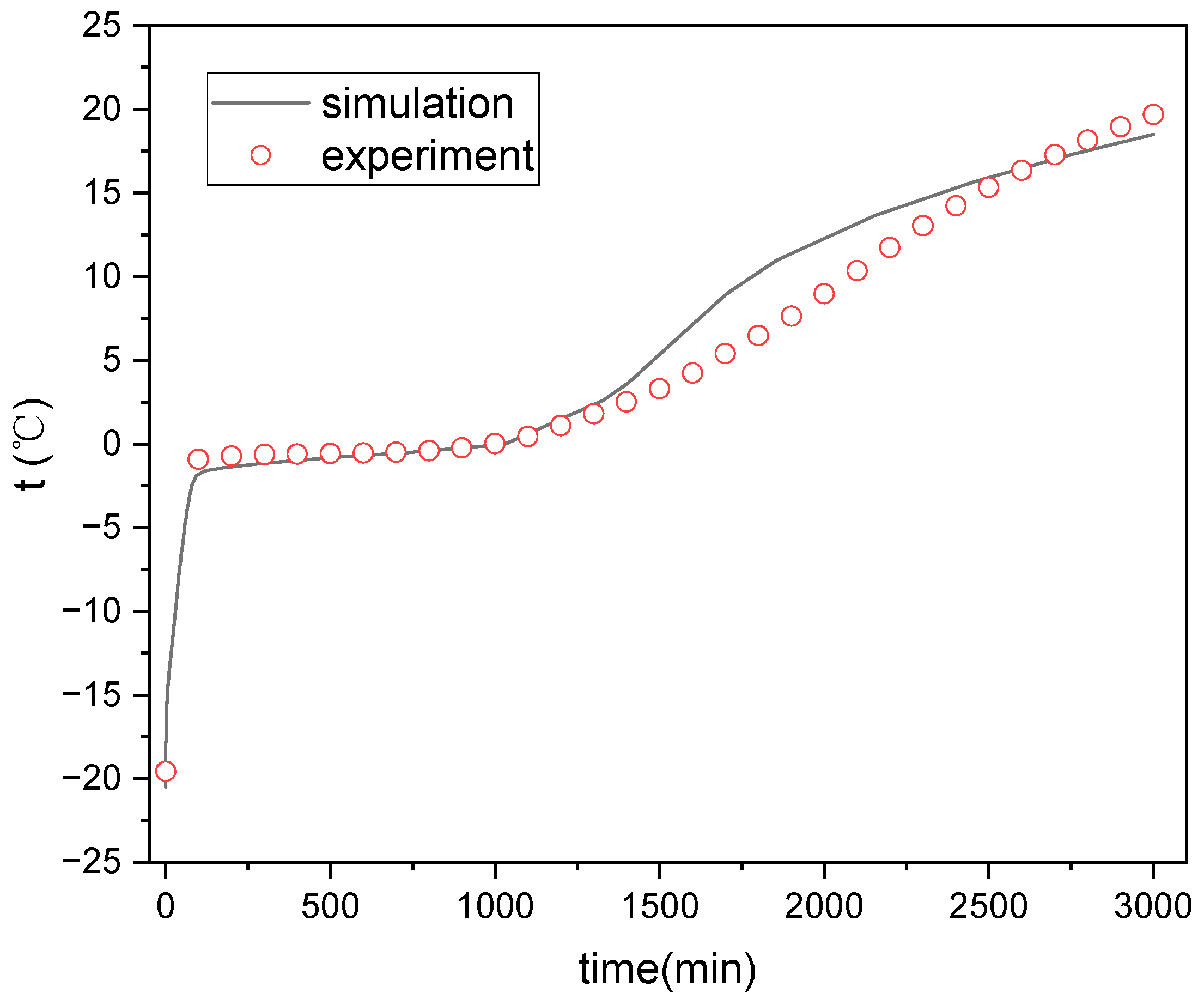
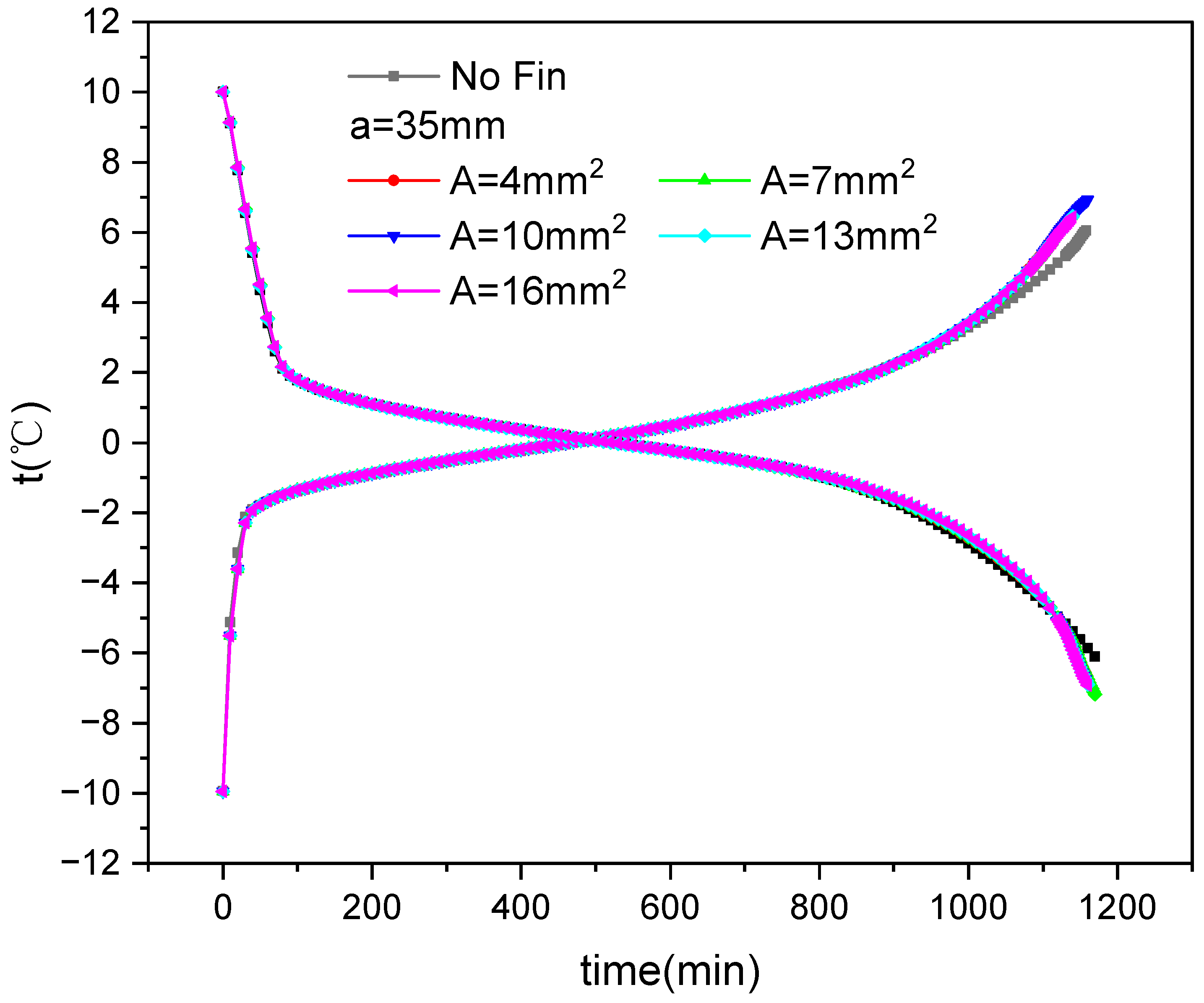
2.3. Model Verification
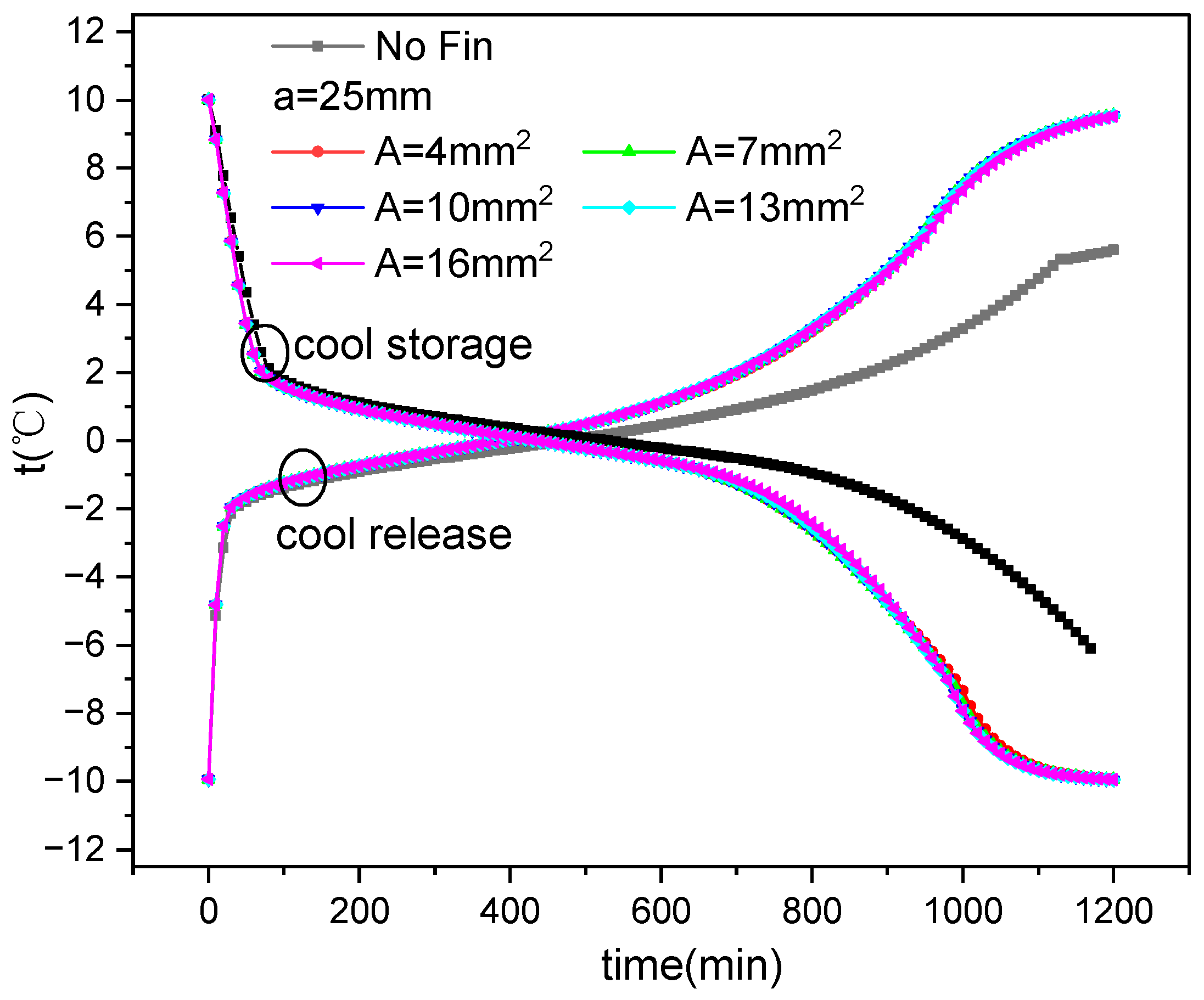
3. Analysis and Discussion
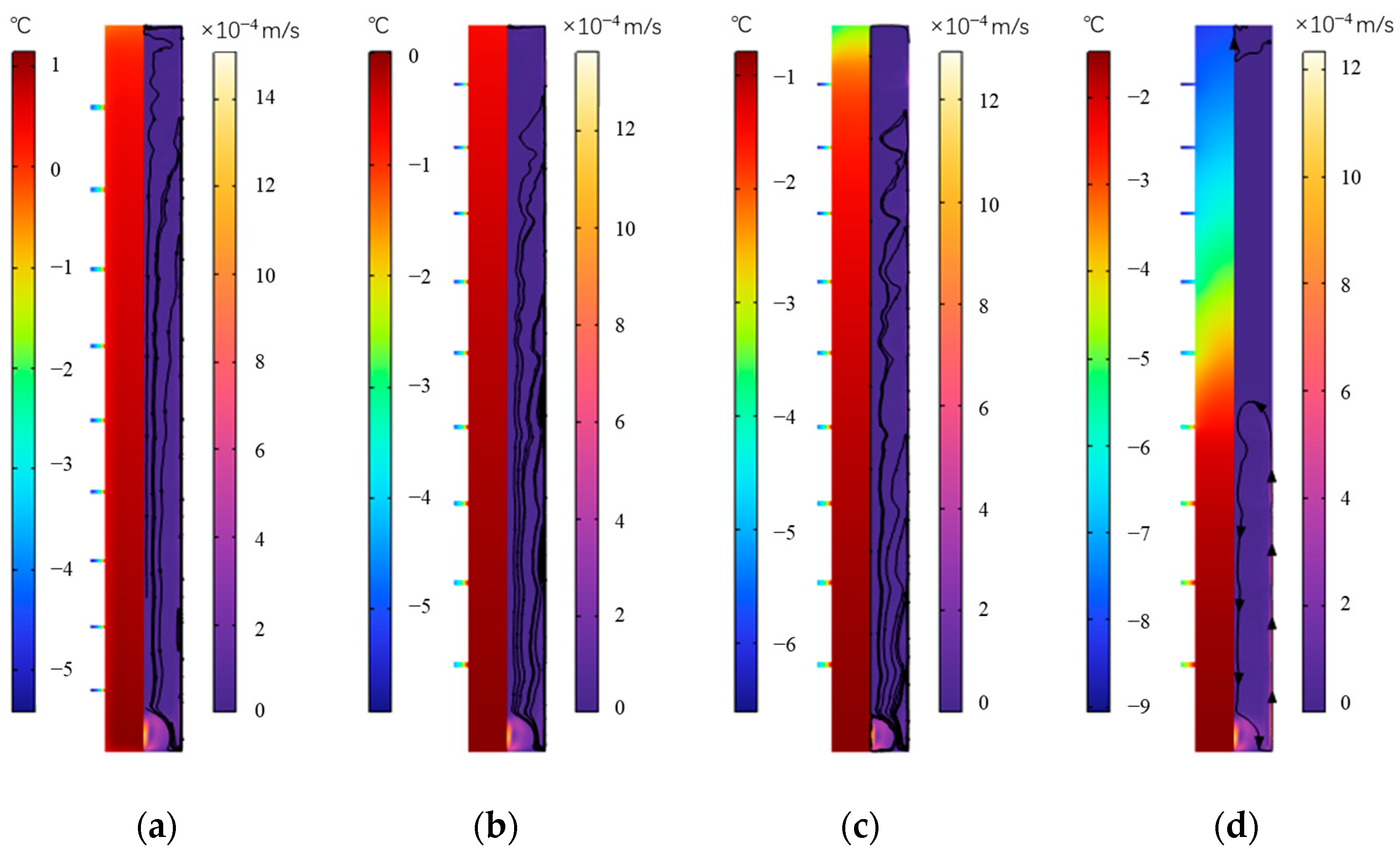
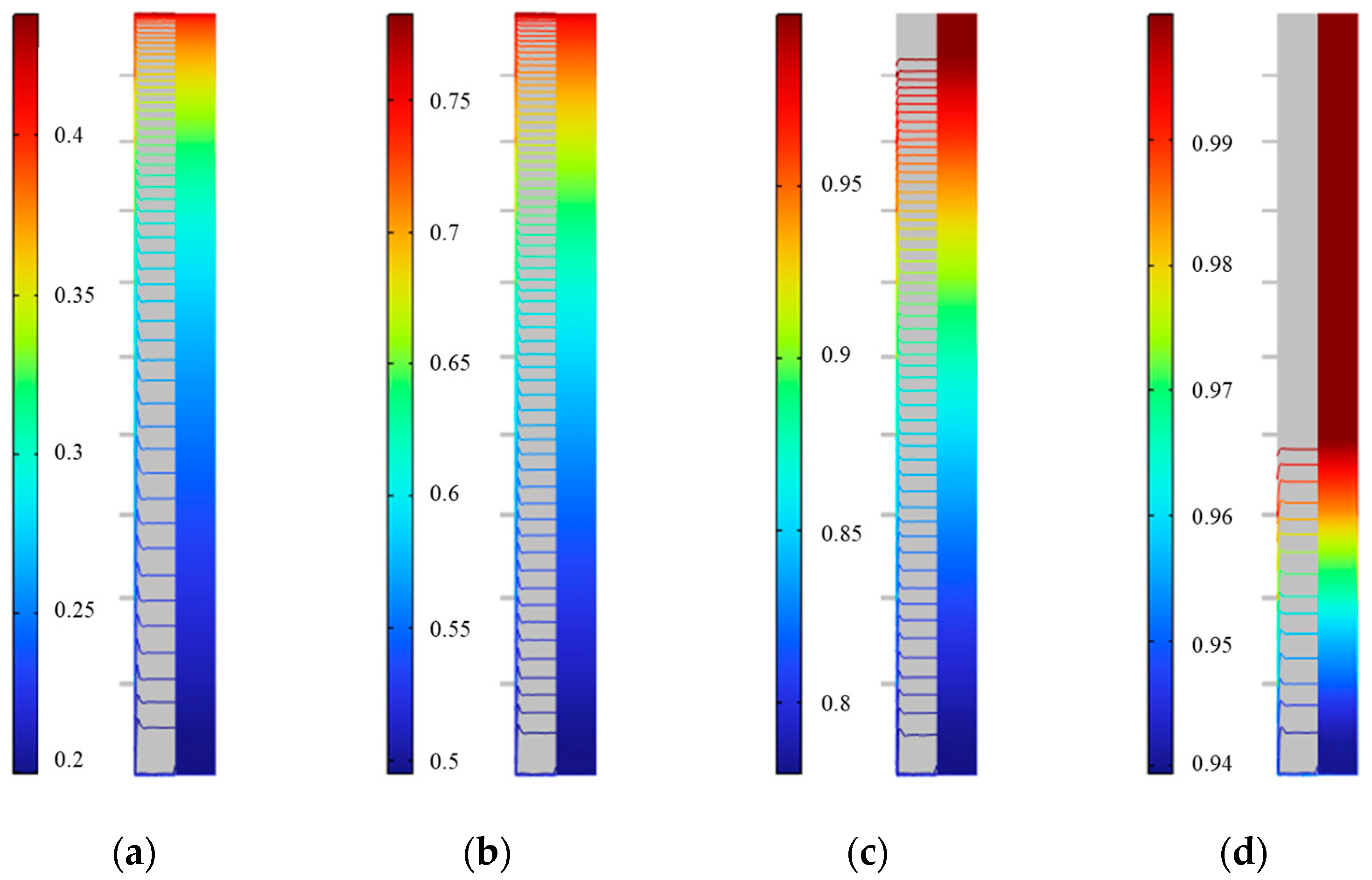
3.1. Internal Fin Ice Plate Cold Storage and Release Characteristics
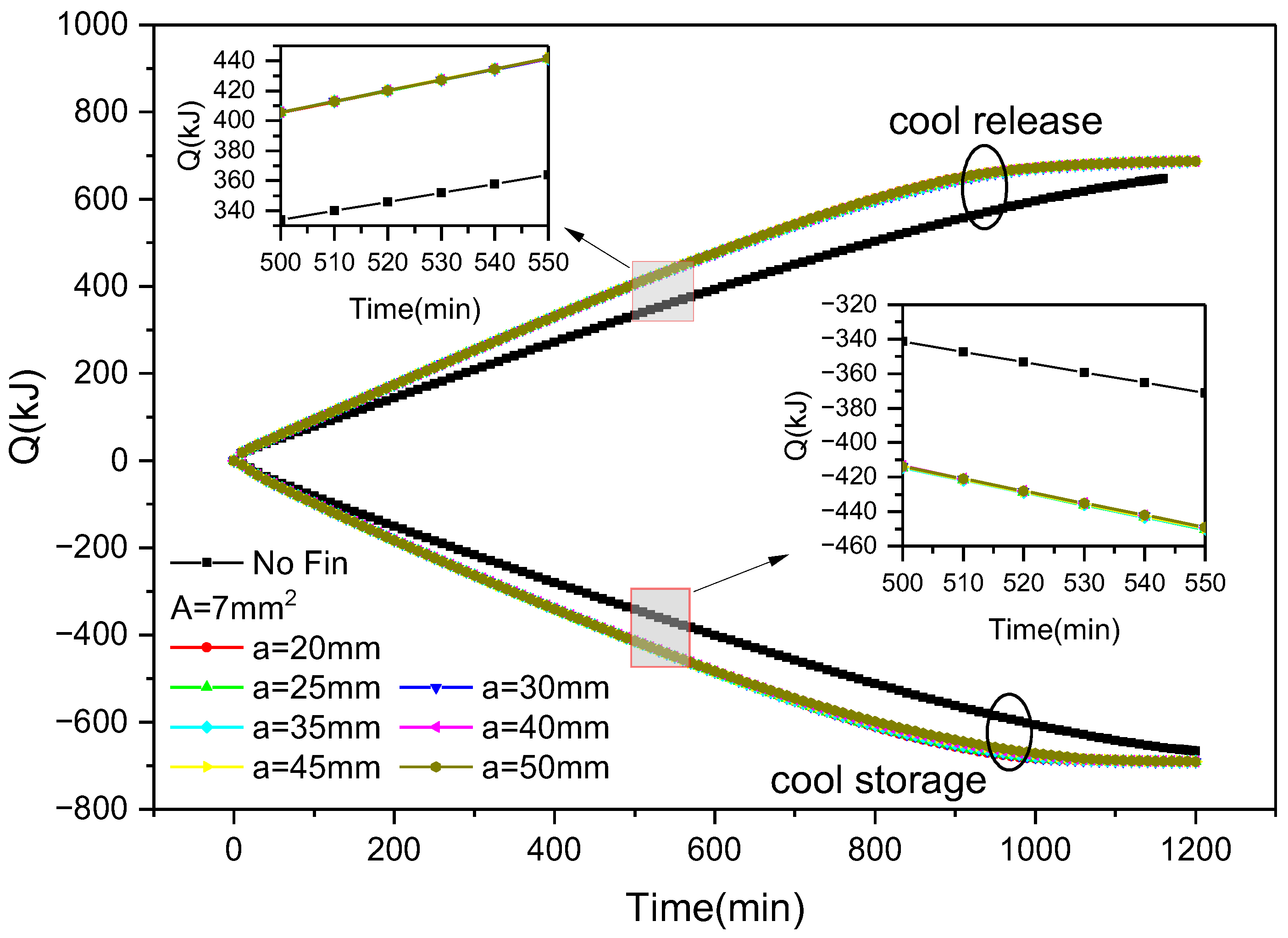
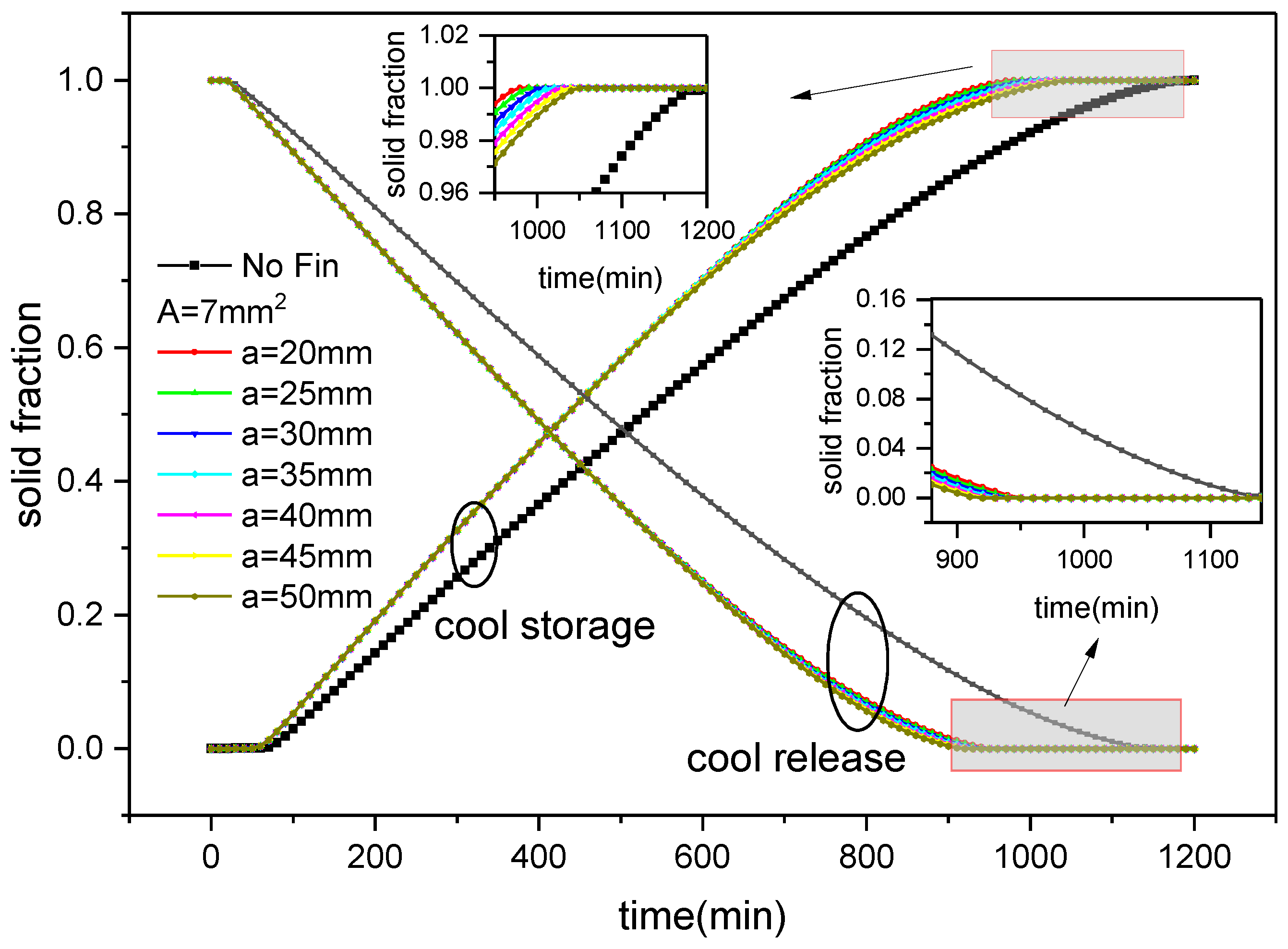
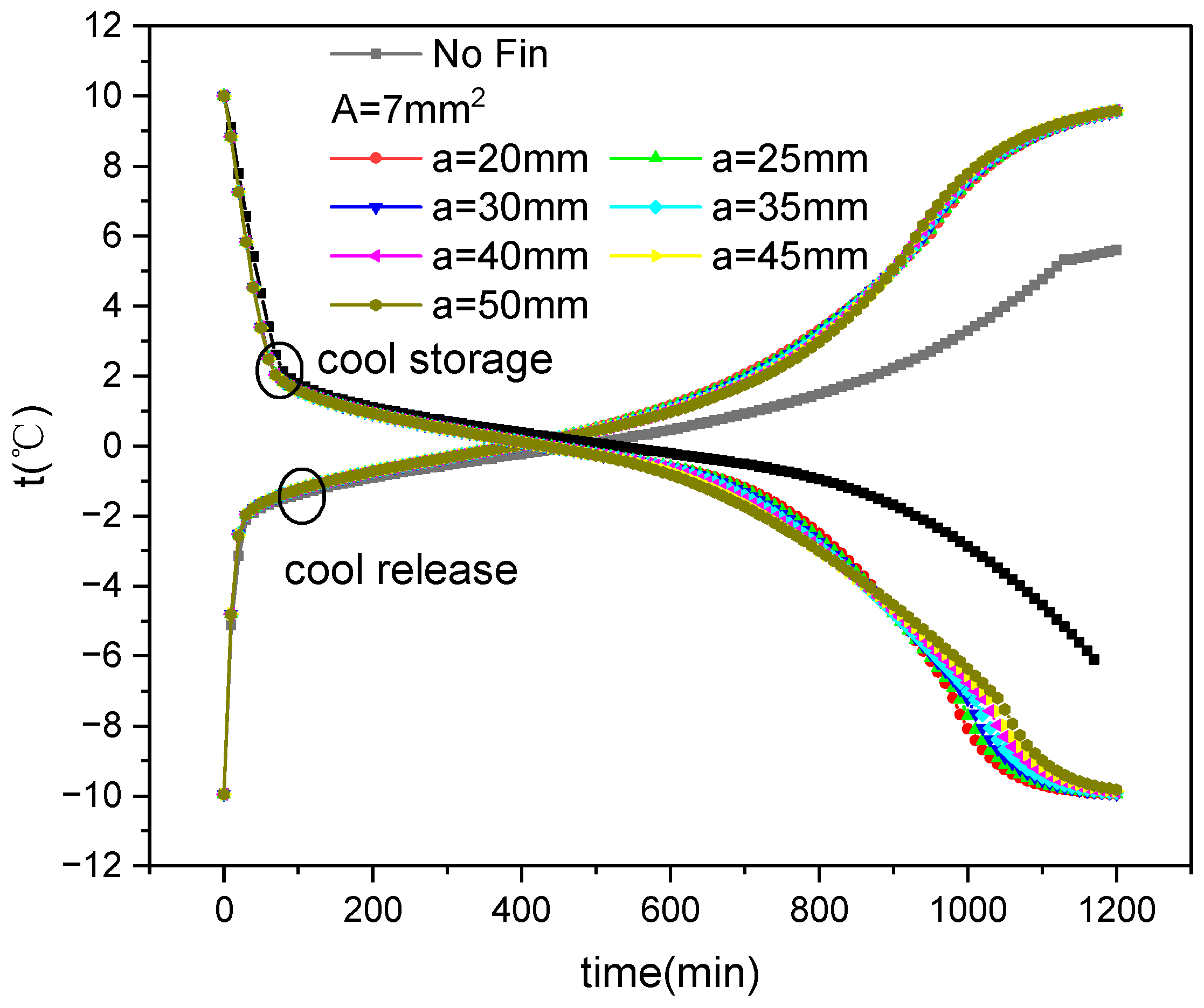
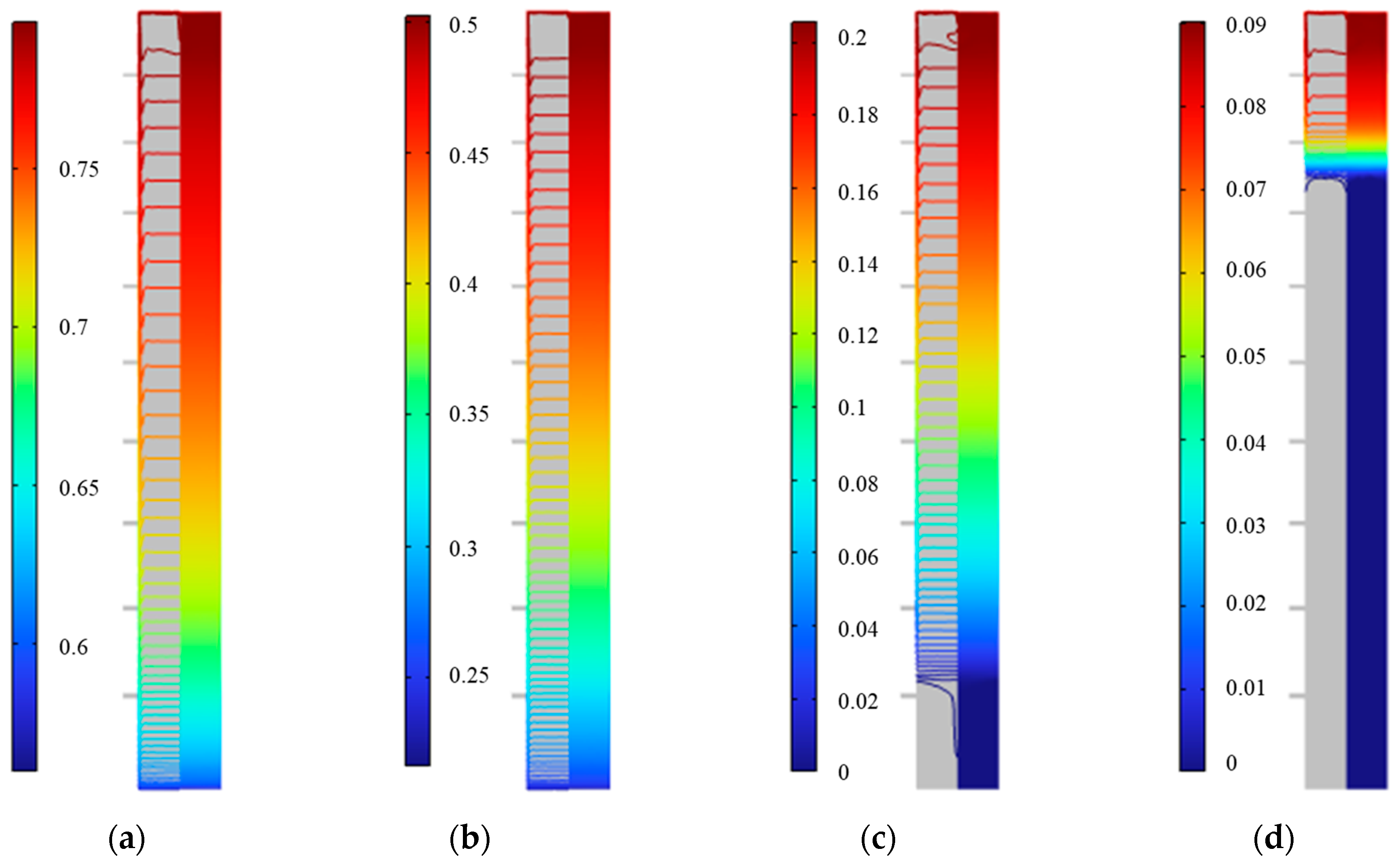
3.2. External Fin Ice Plate Cold Storage and Release Characteristics
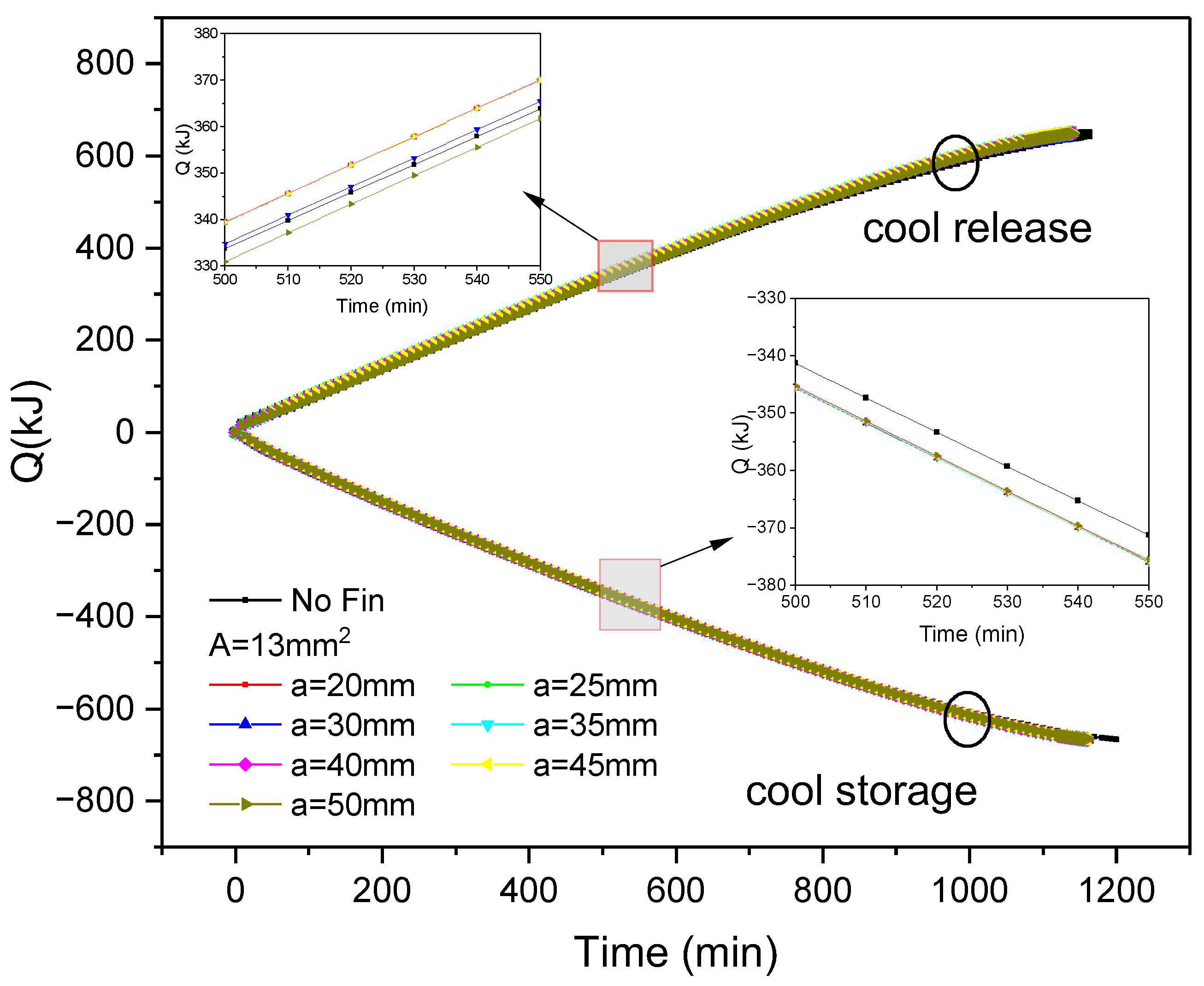
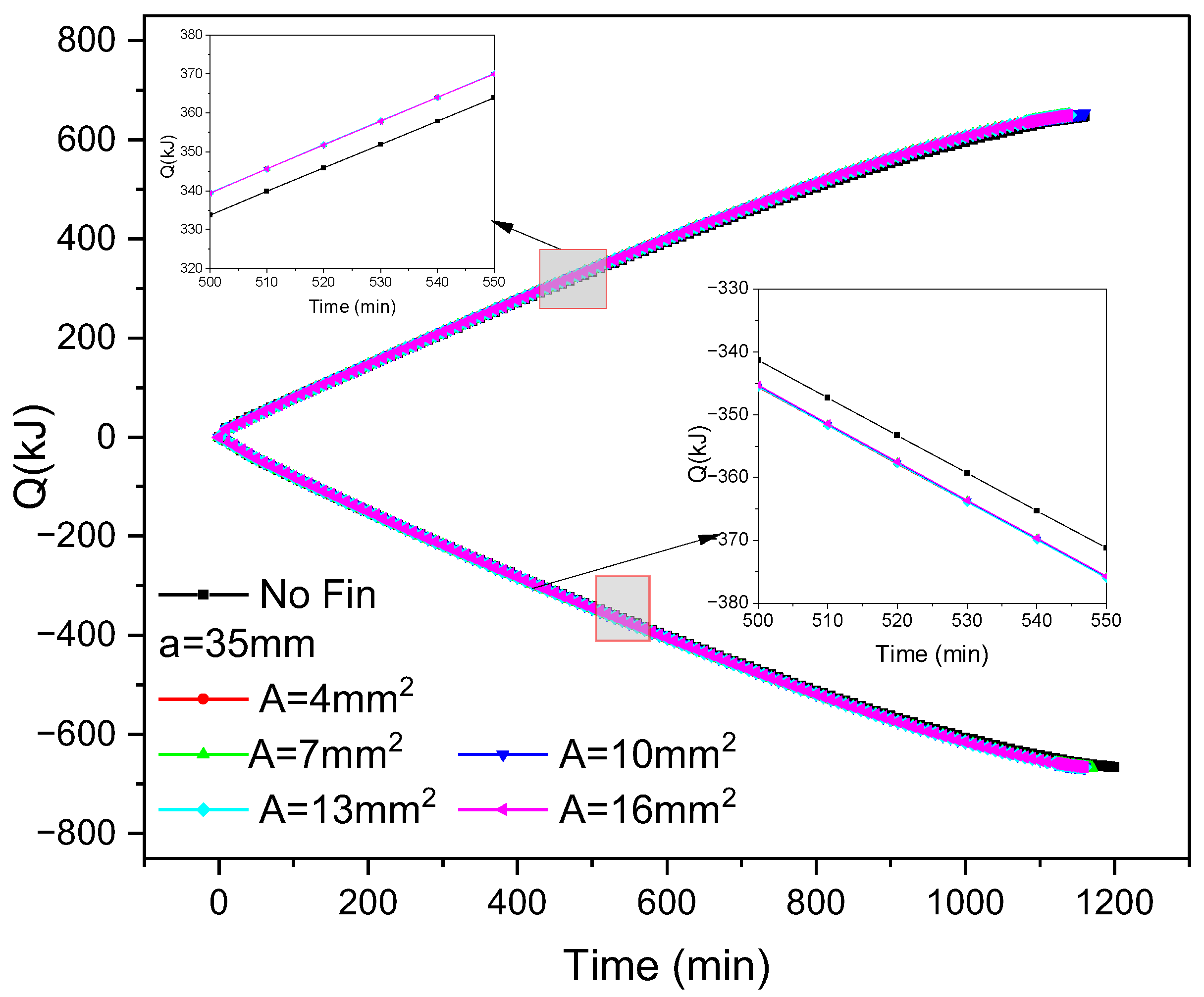
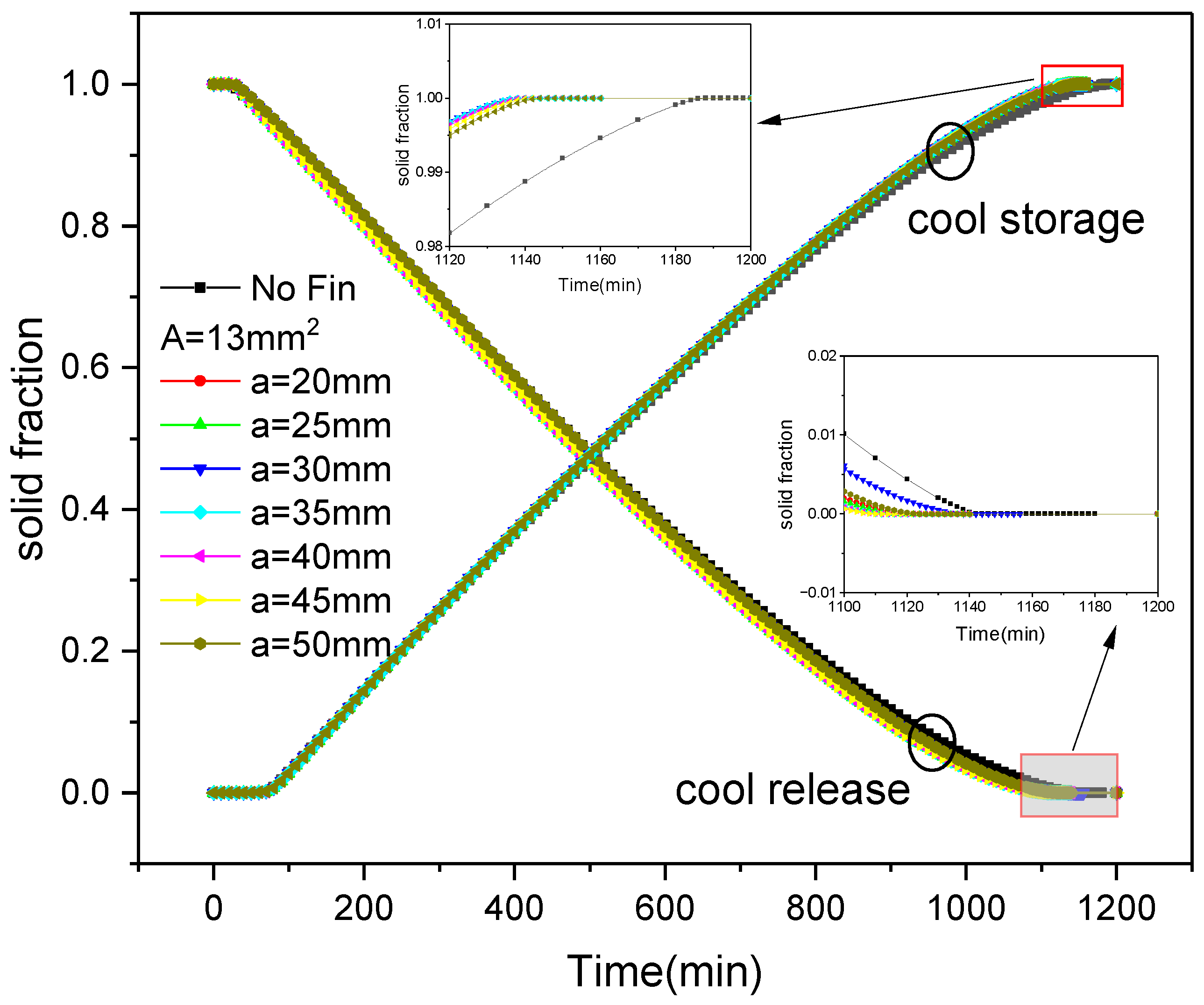
3.3. Finned Ice Plate Cold Storage and Release Time
4. Discussion
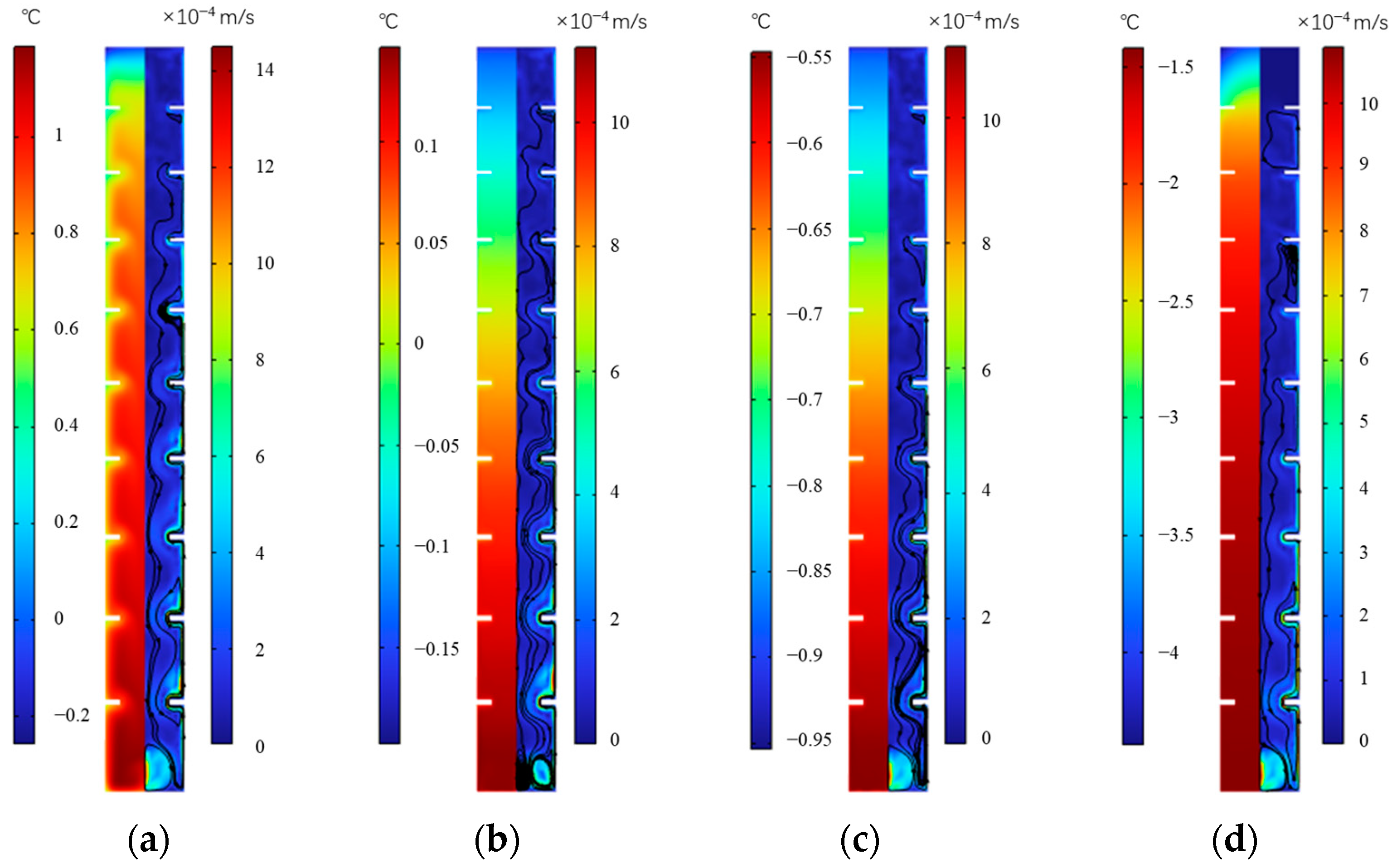
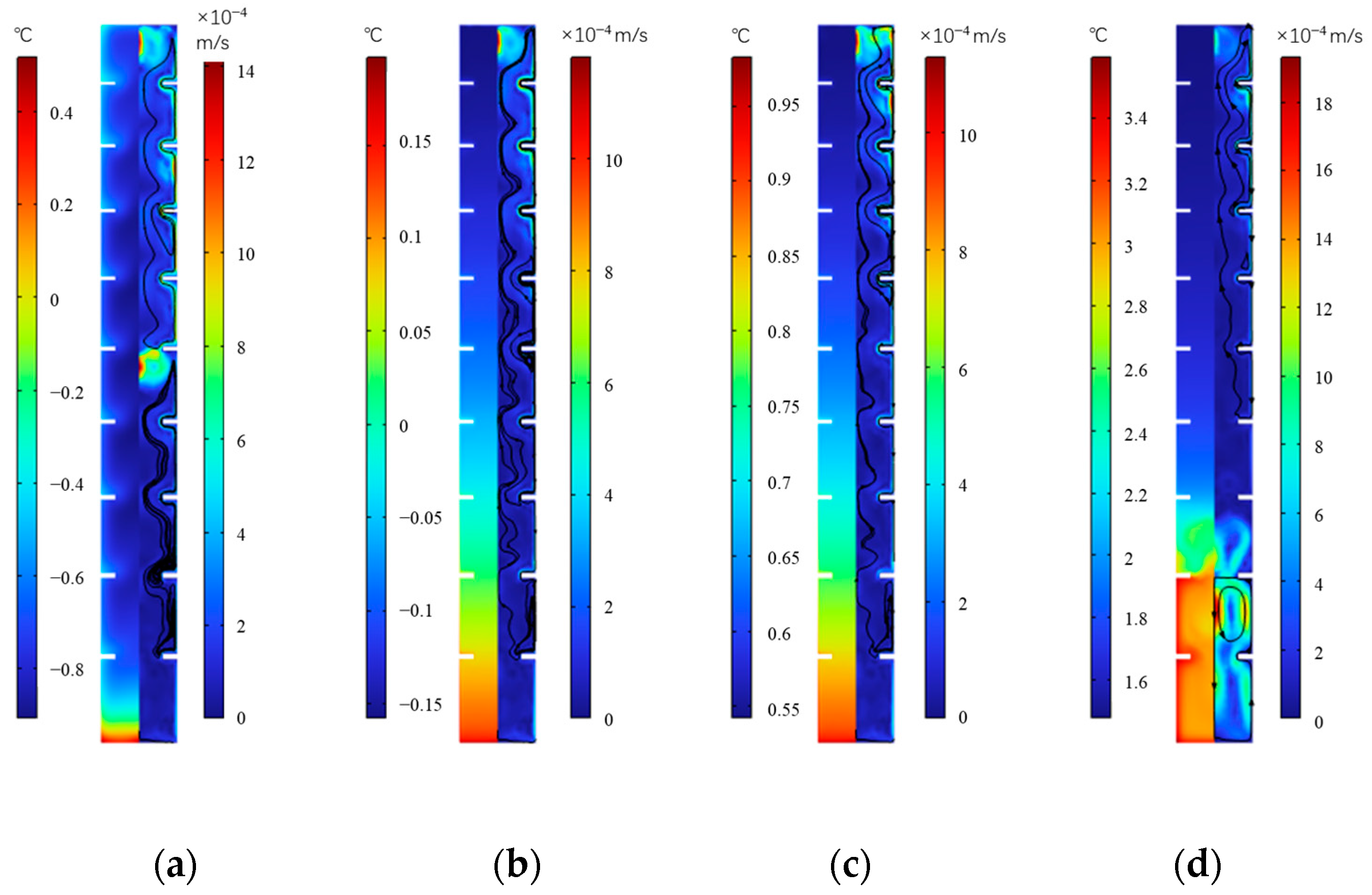
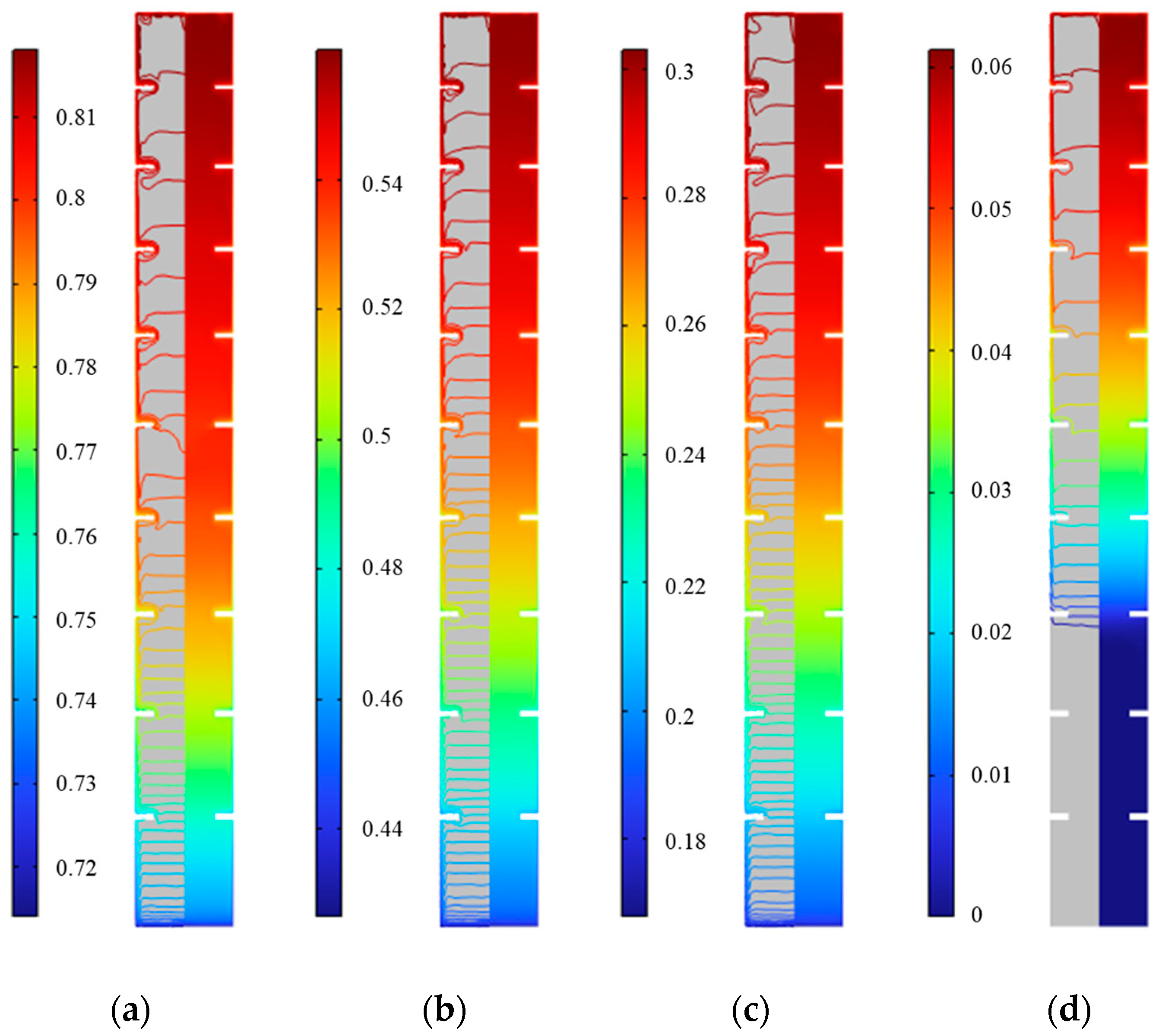
4.1. Internal Parameter Field of Inner Finned Plate
4.2. Internal Parameter Field of External Fin Ice Plate
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| ρ | Density, kg/m3 |
| time, s | |
| λ | thermal conductivity, W/m/K |
| dynamic viscosity, Pa∙s | |
| c | heat capacity, J/kg/K |
| h | convective heat transfer coefficient, W/m2/K |
| H | specific enthalpy, kJ/kg |
| p | pressure, Pa |
| t | temperature, °C |
| u | velocity, m/s |
| Subscripts | |
| b | plate wall |
| c | ice |
| g | dry air |
| l | denotes water |
| w | plate wall surface |
References
- Sha, Y.S.; Hua, W.S.; Cao, H.F.; Zhang, X.L. Properties and encapsulation forms of phase change material and various types of cold storage box for cold chain logistics: A review. J. Energy Storage 2022, 55, 105426. [Google Scholar] [CrossRef]
- Zhang, B.; Cao, H.-J.; Wei, W.-Y.; Ying, X.G. Influence of temperature fluctuations on growth and recrystallization of ice crystals in frozen peeled shrimp (Litopenaeus vannamei) pre-soaked with carrageenan oligosaccharide and xylooligosaccharide. Food Chem. 2020, 306, 125641. [Google Scholar] [CrossRef] [PubMed]
- Mercier, S.; Villeneuve, S.; Mondor, M.; Uysal, I. Time-temperature management along the food cold chain: A review of recent developments. Compr. Rev. Food Sci. Food Saf. 2017, 16, 647–667. [Google Scholar] [CrossRef] [PubMed]
- Khademi, A.; Shank, K.; Mehrjardi, S.A.A.; Tiari, S.; Sorrentino, G.; Said, Z.; Chamkha, A.J.; Ushak, S. A brief review on different hybrid methods of enhancement within latent heat storage systems. J. Energy Storage 2022, 54, 105362. [Google Scholar] [CrossRef]
- Mousazade, A.; Rafee, R.; Valipour, M.S. Thermal performance of cold panels with phase change materials in a refrigerated truck. Int. J. Refrig. 2020, 120, 119–126. [Google Scholar] [CrossRef]
- Liu, M.; Saman, W.; Bruno, F. Development of a novel refrigeration system for refrigerated trucks incorporating phase change material. Appl. Energy 2012, 92, 336–342. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, X.; Ji, J.; Zhang, C. Cold chain transportation energy conservation and emission reduction based on phase change materials under dual-carbon background: A review. J. Energy Storage 2024, 86, 111258. [Google Scholar] [CrossRef]
- Li, C.; Peng, M.; Xie, B.; Li, Y.; Li, M. Novel phase change cold energy storage materials for refrigerated transportation of fruits. Renew. Energy 2024, 220, 119583. [Google Scholar] [CrossRef]
- Comodi, G.; Carducci, F.; Nagarajan, B.; Romagnoli, A. Application of cold thermal energy storage (CTES) for building demand management in hot climates. Appl. Therm. Eng. 2016, 103, 1186–1195. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, X.; Xu, X.; Zhang, S. Development of composite phase change cold storage material and its application in vaccine cold storage equipment. J. Energy Storage 2020, 30, 101455. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, X.; Xu, X.; Zhang, S. Research progress of phase change cold storage materials used in cold chain transportation and their different cold storage packaging structures. J. Mol. Liq. 2020, 319, 114360. [Google Scholar] [CrossRef]
- Wang, Y.; Zadeh, P.G.; Duong, X.Q.; Chung, J.D. Optimizing fin design for enhanced melting performance in latent heat thermal energy storage systems. J. Energy Storage 2023, 73, 109108. [Google Scholar] [CrossRef]
- Qasem, N.A.A.; Belazreg, A.; Khetib, Y.; Abderrahmane, A.; Homod, R.Z.; Younis, O.; Rawa, M. Effect of novel fin distribution on the melting process of thermal storage units. Appl. Therm. Eng. 2024, 243, 122547. [Google Scholar] [CrossRef]
- He, Z.; Ma, H.; Lu, S. Design and experimental investigation of topology-optimized fin structures for enhanced heat transfer in latent heat thermal energy storage units. J. Energy Storage 2024, 80, 110272. [Google Scholar] [CrossRef]
- Yang, X.; Guo, J.; Yang, B.; Cheng, H.; Wei, P.; He, Y. Design of non-uniformly distributed annular fins for a shell-and-tube thermal energy storage unit. Appl. Energy 2020, 279, 115772. [Google Scholar] [CrossRef]
- Nie, C.; Liu, X.; Rao, Z.; Liu, J. Discharging performance evaluation and optimization of a latent heat thermal energy storage unit with helm-shaped fin. Appl. Therm. Eng. 2024, 236, 121595. [Google Scholar] [CrossRef]
- Mahdi, J.M.; Lohrasbi, S.; Ganji, D.D.; Nsofor, E.C. Accelerated melting of PCM in energy storage systems via novel configuration of fins in the triplex-tube heat exchanger. Int. J. Heat Mass Transf. 2018, 124, 663–676. [Google Scholar] [CrossRef]
- Joybari, M.M.; Haghighat, F.; Al-Abidi, A.A. Heat transfer enhancement of phase change materials by fins under simultaneous charging and discharging. Energy Convers. Manag. 2017, 152, 136–156. [Google Scholar] [CrossRef]
- Yang, X.; Wang, X.; Liu, Z.; Luo, X.; Yan, J. Effect of fin number on the melting phase change in a horizontal finned shell-and-tube thermal energy storage unit. Sol. Energy Mater. Sol. Cells 2022, 236, 111527. [Google Scholar] [CrossRef]
- Karami, R.; Kamkari, B. Investigation of the effect of inclination angle on the melting enhancement of phase change material in finned latent heat thermal storage units. Appl. Therm. Eng. 2019, 146, 45–60. [Google Scholar] [CrossRef]
- Yazici, M.Y.; Avci, M.; Aydin, O. Combined effects of inclination angle and fin number on thermal performance of a PCM-based heat sink. Appl. Therm. Eng. 2019, 159, 113956. [Google Scholar] [CrossRef]
- Ji, C.; Qin, Z.; Low, Z.H.; Dubey, S.; Choo, F.H.; Duan, F. Non-uniform heat transfer suppression to enhance PCM melting by angled fins. Appl. Therm. Eng. 2018, 129, 269–279. [Google Scholar] [CrossRef]
- Tong, S.; Nie, B.; Li, Z.; Li, C.; Zou, B.; Jiang, L.; Jin, Y.; Ding, Y. A phase change material (PCM) based passively cooled container for integrated road-rail cold chain transportation—An experimental study. Appl. Therm. Eng. 2021, 195, 117204. [Google Scholar] [CrossRef]
- Zheng, H.; Zhang, Z.; Sun, J. Study on performance of carbon nanotube composite phase change cold storage sphere with annular fins. J. Energy Storage 2024, 78, 110074. [Google Scholar] [CrossRef]
- Zhai, X.Q.; Cheng, X.W.; Wang, C.; Wang, R.Z. Experimental investigation and performance analysis of a fin tube phase change cold storage unit for high temperature cooling application. Energy Build. 2015, 89, 9–17. [Google Scholar] [CrossRef]
- Lee, Y.-T.; Chien, L.-H.; Cheung, F.-B.; Yang, A.-S. Numerical and experimental investigations on melting heat transfer performance of PCM in finned cold thermal energy storage. Int. J. Heat Mass Transf. 2023, 210, 124199. [Google Scholar] [CrossRef]
- Voller, V.R.; Prakash, C. A fixed grid numerical modeling methodology for convection-diffusion mushy region phase-change problems. Int. J. Heat Mass Transf. 1987, 30, 1709–1719. [Google Scholar] [CrossRef]
- Wu, F.; Fan, Y.B.; Zhang, X.J.; Zhang, H.; Wang, Z.L.; Wang, Z.W.; Jiang, L. Performance prediction on ice melting process for cold energy utilization: Effect of natural convection. J. Energy Storage 2022, 55, 105638. [Google Scholar] [CrossRef]

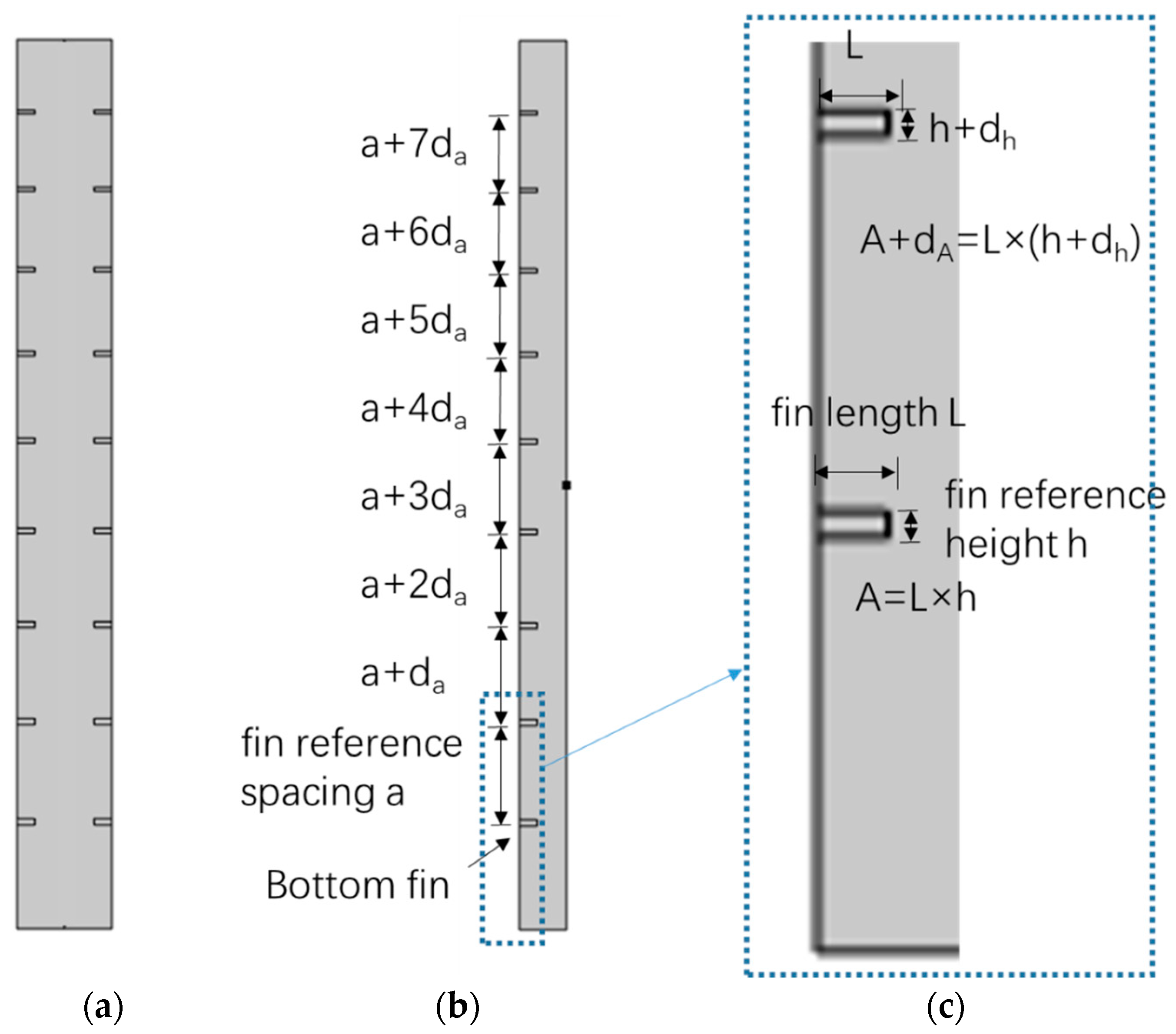
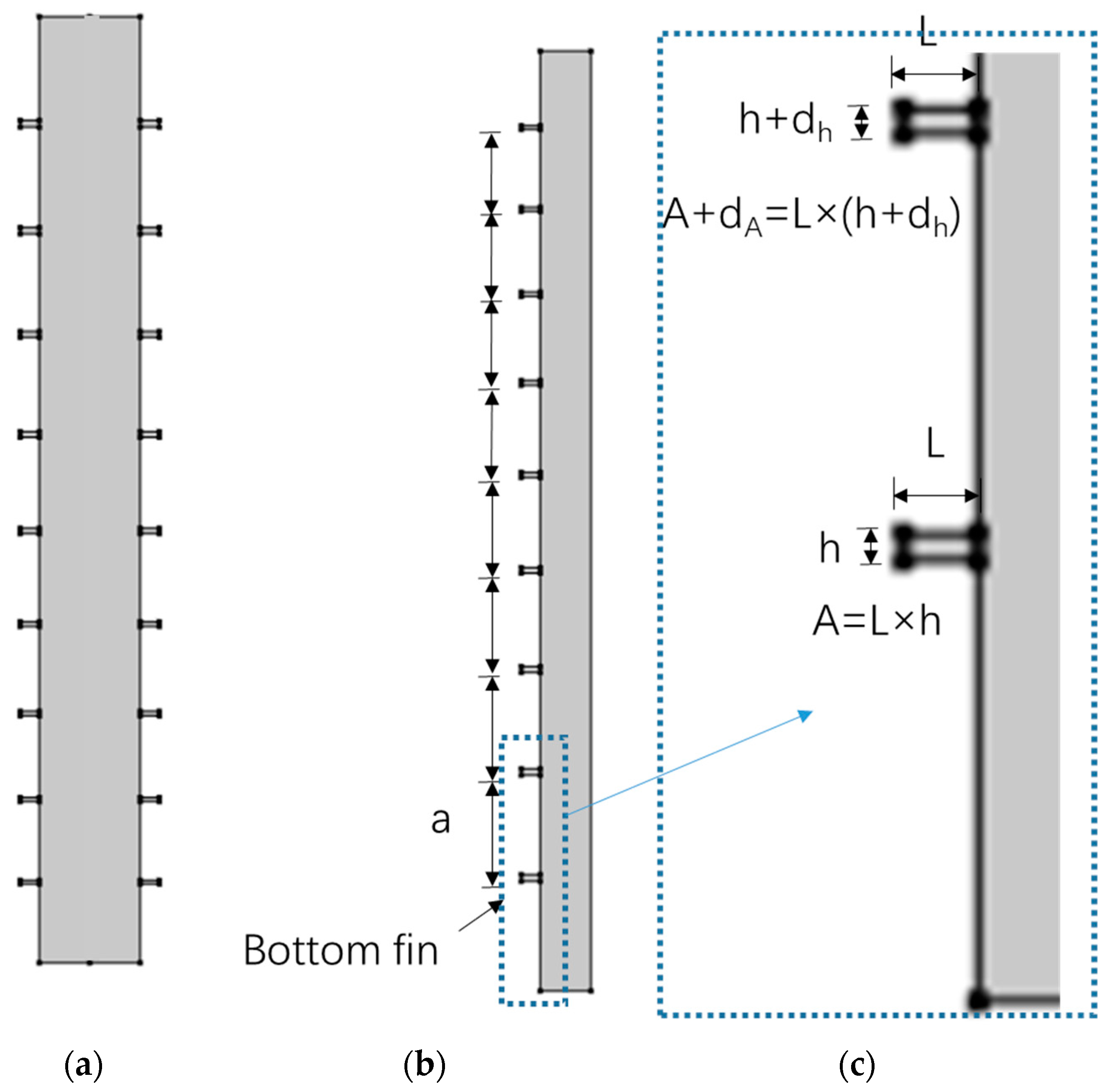







| Element Size | Mesh Number | Calculation Time (s) | Max. Temp. Deviation (°C) |
|---|---|---|---|
| extremely coarse | 8275 | 102 | 0.13 |
| extra coarse | 12,155 | 117 | 0.12 |
| coarser | 19,595 | 179 | 0.09 |
| coarse | 35,467 | 386 | 0.05 |
| normal | 53,405 | 599 | 0 |
| fine | 92,120 | 891 | 0.03 |
| finer | 195,486 | 2732 | 0.02 |
| Material | Parameter | Value |
|---|---|---|
| PVC | 1350 kg/m3 | |
| 1080 J/kg/K | ||
| 0.18 W/m/K | ||
| PCM | Latent heat | 333 kJ/kg |
| Total Volume of Fins (mm3) | Number of Fins | Bottom Fin Reference Spacing a (mm) | Common Difference of Fin Spacing da (mm) | Bottom Fin Reference Height h (mm) | Common Difference of Fin Height dh (mm) | Bottom Fin Reference Section Area A (mm2) | Common Difference of Fin Section Area dA (mm2) |
|---|---|---|---|---|---|---|---|
| No Fin | |||||||
| 10,800 | 9 | 20 | 2.2 | 0.67 | 0.25 | 4 | 1.5 |
| 25 | 1.1 | 1.17 | 0.125 | 7 | 0.75 | ||
| 30 | 0 | 1.67 | 0 | 10 | 0 | ||
| 35 | −1.1 | 2.17 | −0.125 | 13 | −0.75 | ||
| 40 | −2.2 | 2.67 | −0.25 | 16 | −1.5 | ||
| 45 | −3.3 | ||||||
| 50 | −4.4 | ||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, K.; Zhao, H.; Wang, Y. Cold Storage and Release Characteristics of Phase Change Cold Storage Plate with Non-Uniform Fins. Energies 2024, 17, 3610. https://doi.org/10.3390/en17153610
Wu K, Zhao H, Wang Y. Cold Storage and Release Characteristics of Phase Change Cold Storage Plate with Non-Uniform Fins. Energies. 2024; 17(15):3610. https://doi.org/10.3390/en17153610
Chicago/Turabian StyleWu, Kun, Haibo Zhao, and Ye Wang. 2024. "Cold Storage and Release Characteristics of Phase Change Cold Storage Plate with Non-Uniform Fins" Energies 17, no. 15: 3610. https://doi.org/10.3390/en17153610
APA StyleWu, K., Zhao, H., & Wang, Y. (2024). Cold Storage and Release Characteristics of Phase Change Cold Storage Plate with Non-Uniform Fins. Energies, 17(15), 3610. https://doi.org/10.3390/en17153610





