The Behavior of Terminal Voltage and Frequency of Wind-Driven Single-Phase Induction Generators under Variations in Excitation Capacitances for Different Operating Conditions
Abstract
1. Introduction
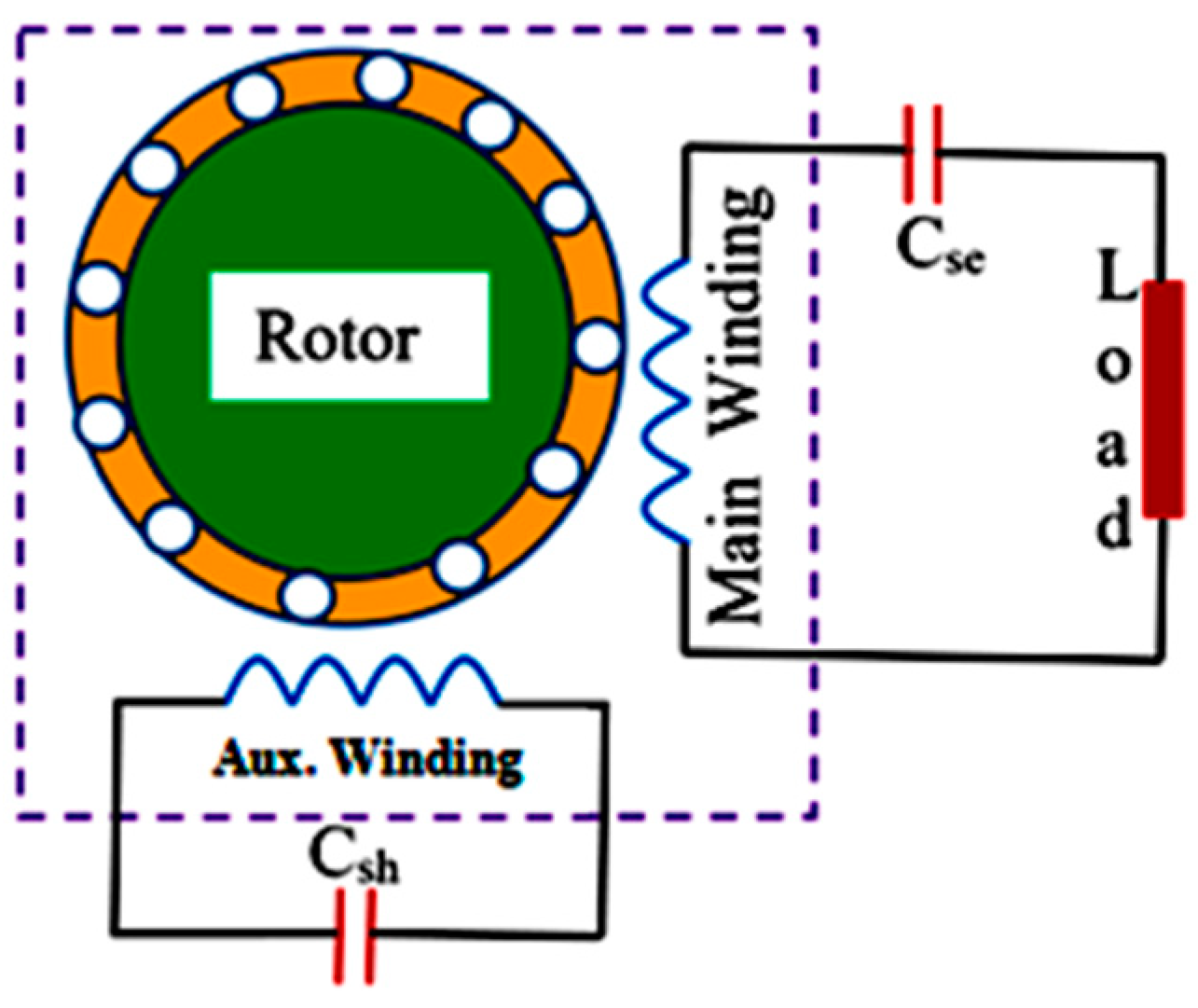
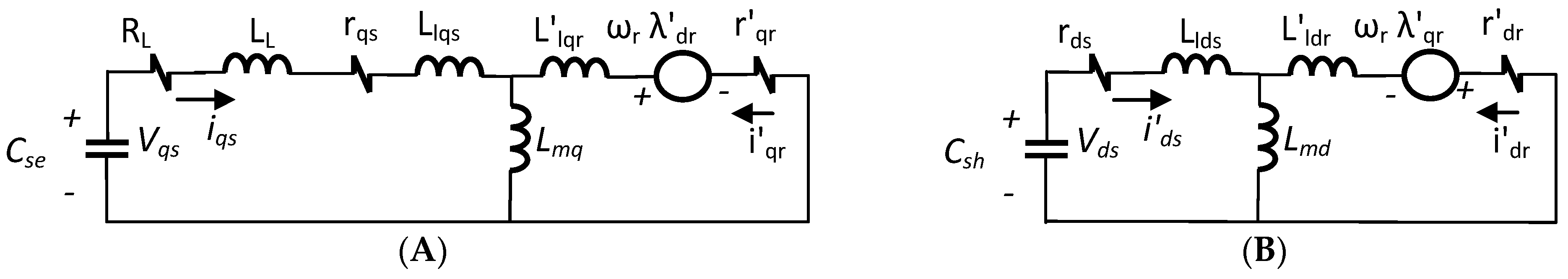
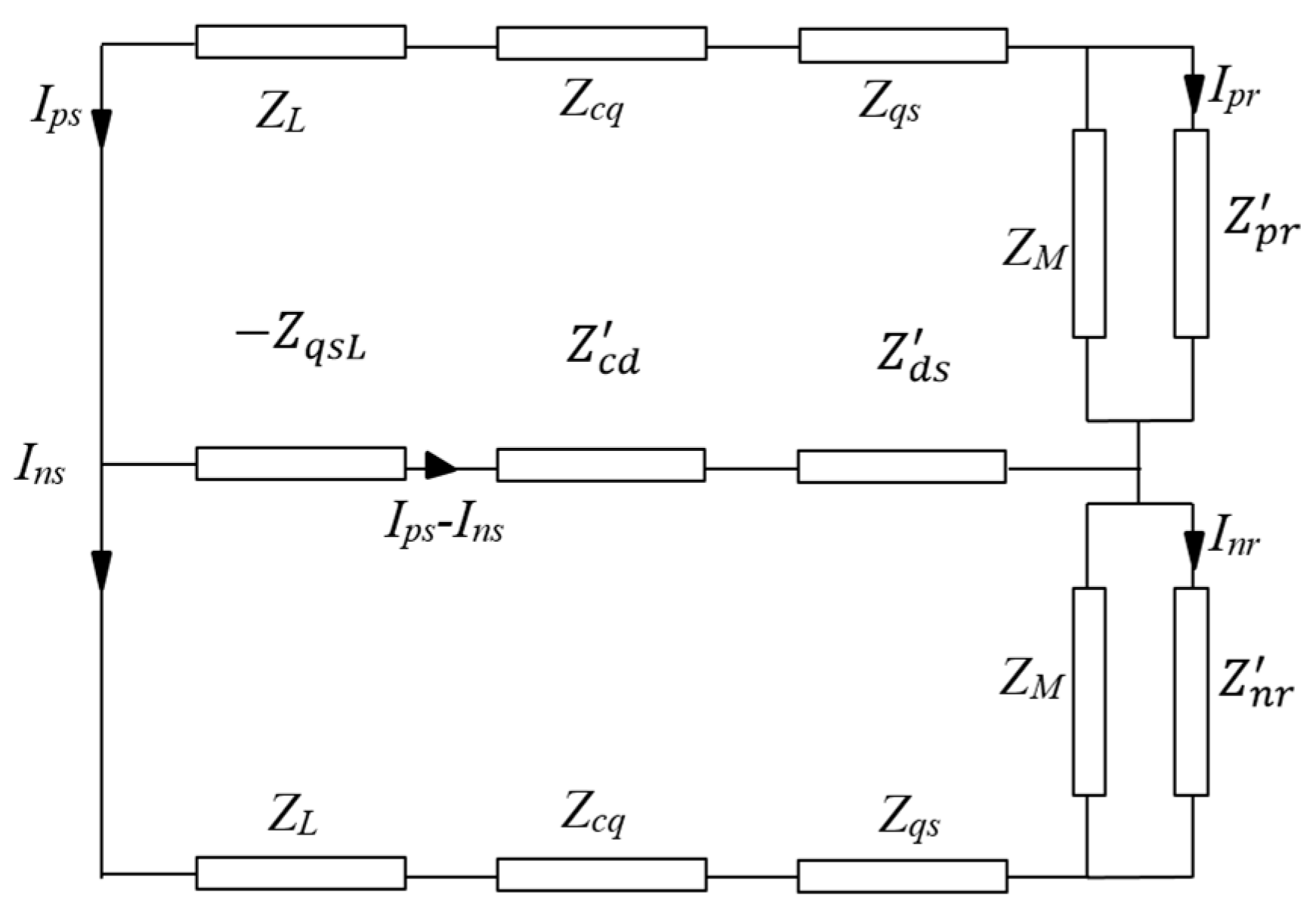
2. Modeling of Single-Phase Self-Excited Induction Generator
3. Results and Discussion
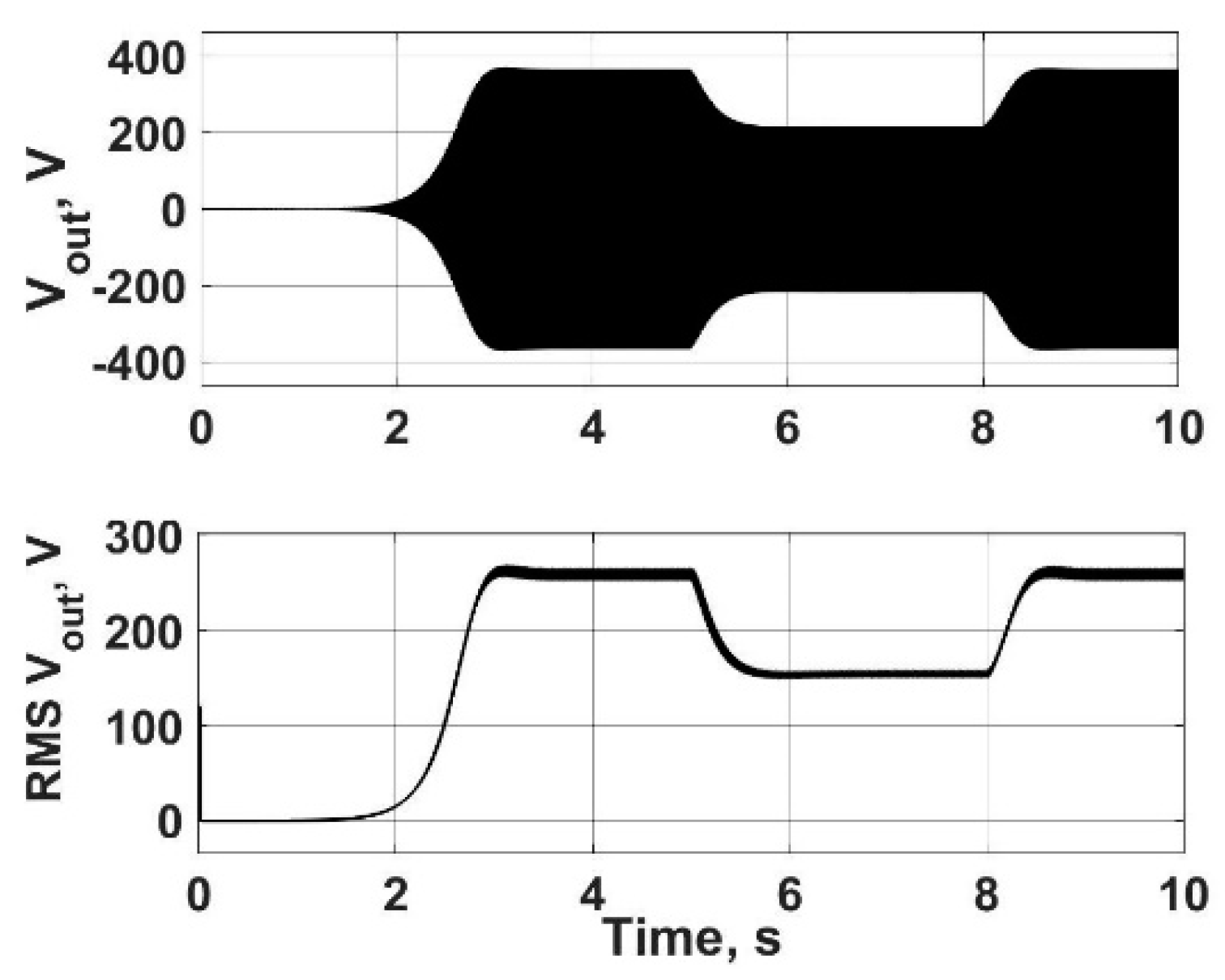
- (a)
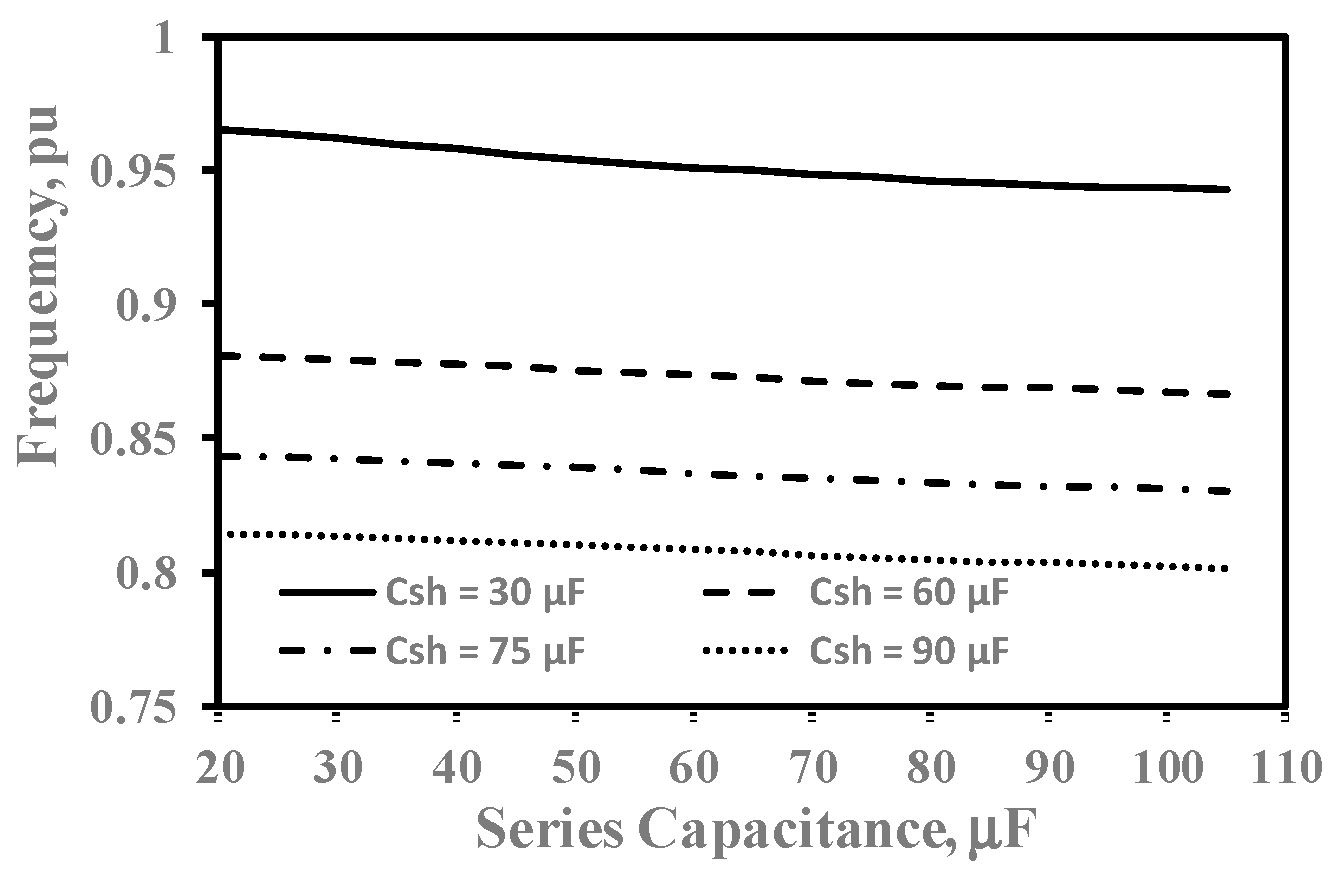
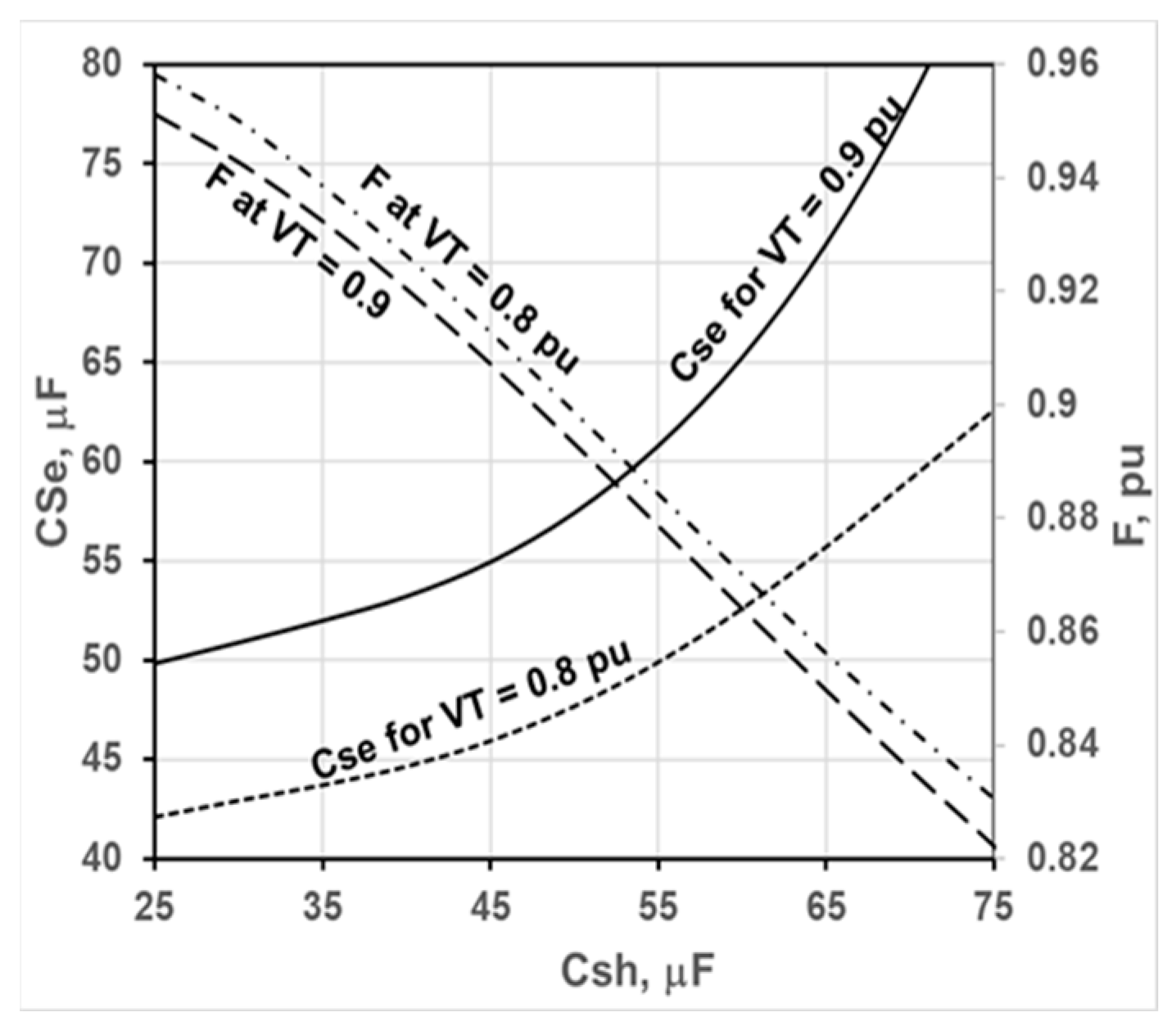
- Changes in the shunt excitation capacitance under fixed series excitation capacitance lead to noticeable effects on both terminal voltage and frequency;
- (b)
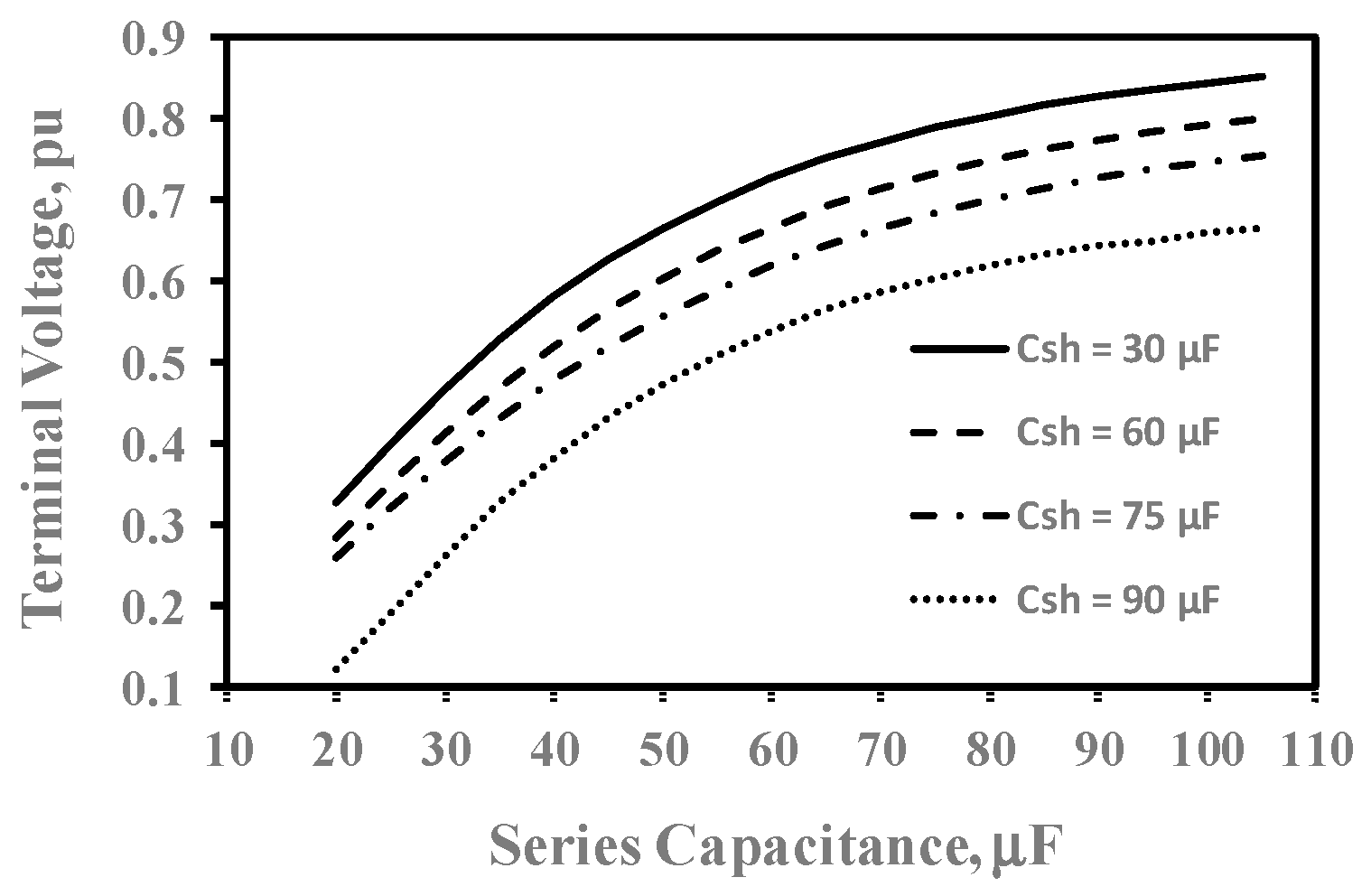
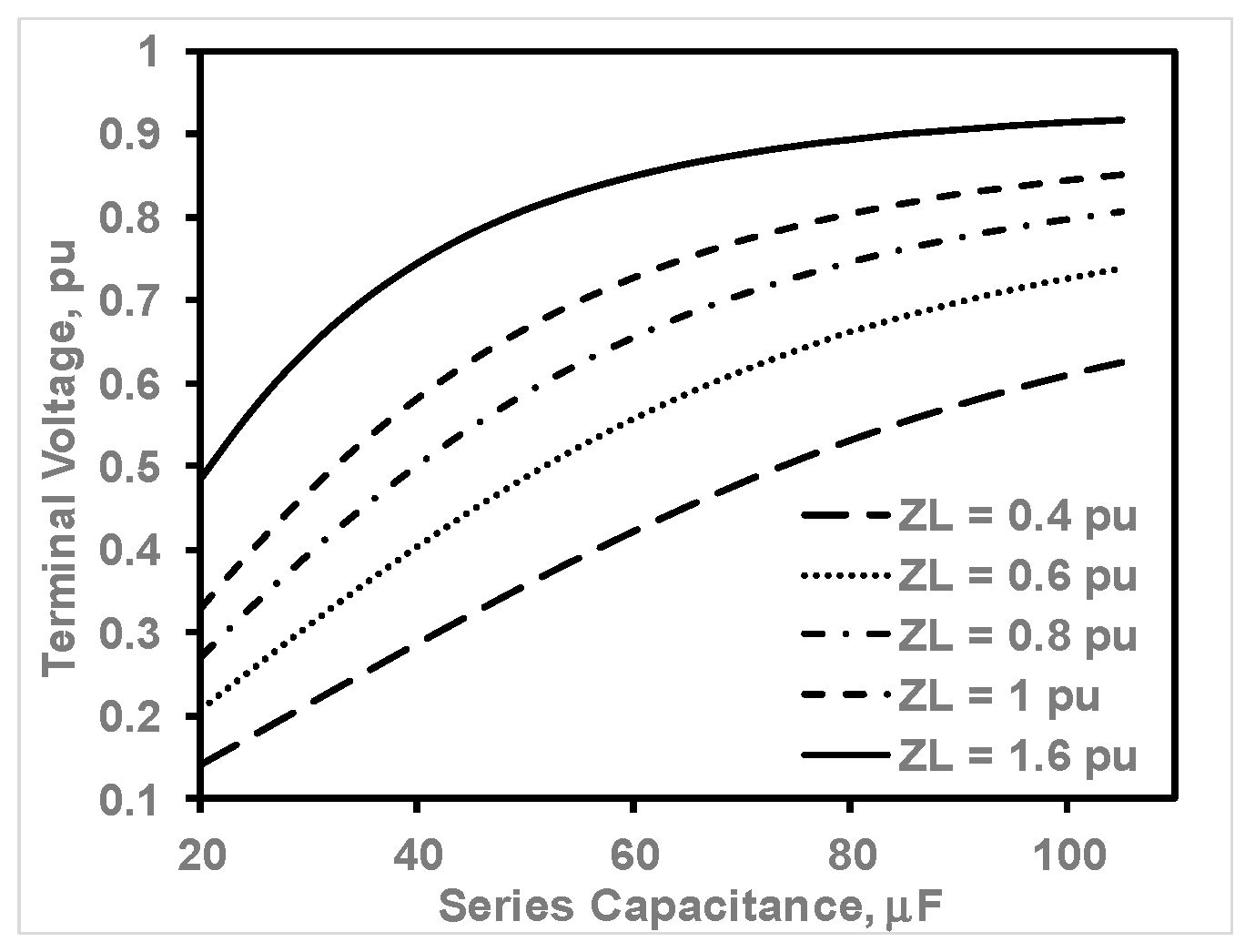
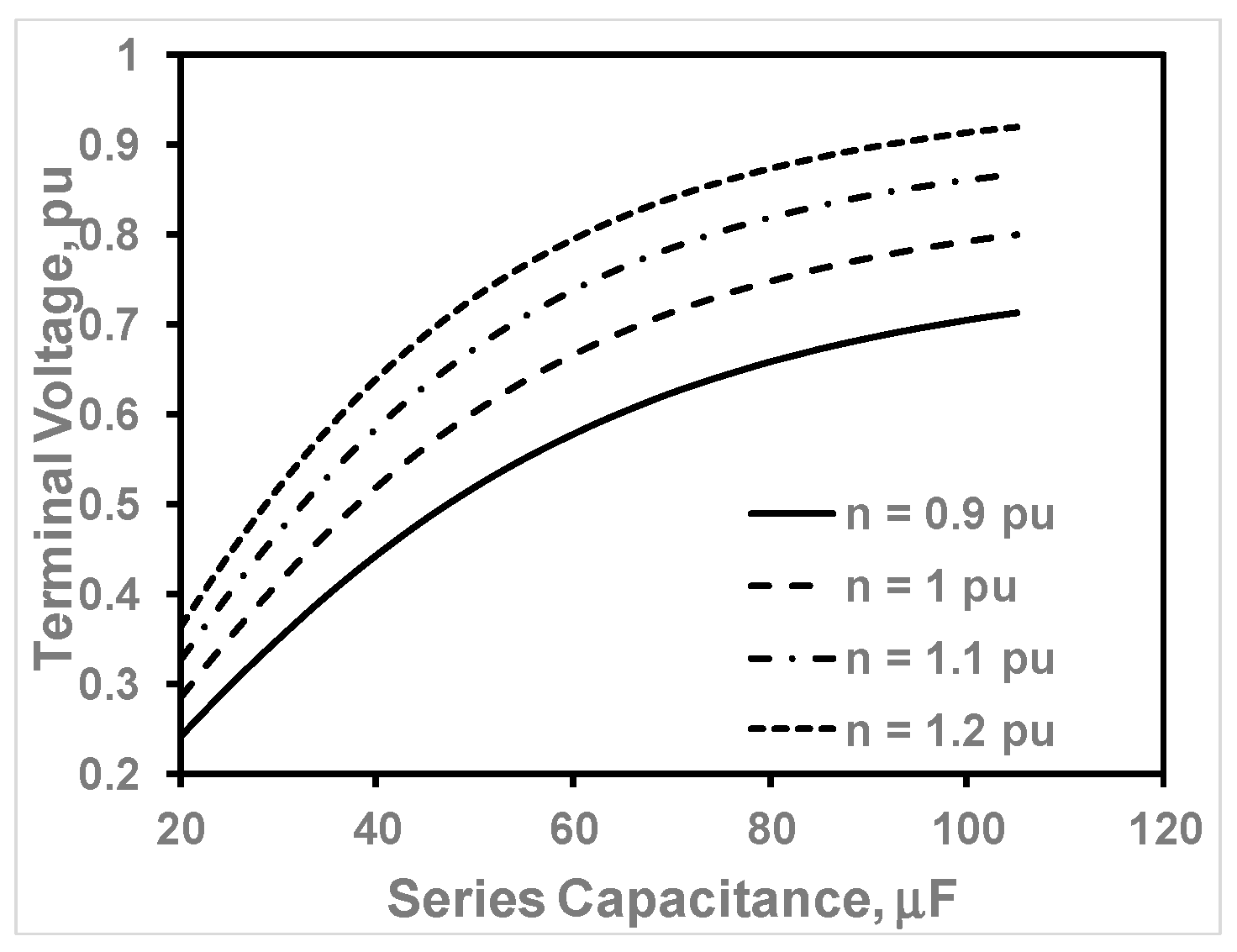
- Output terminal voltage is highly affected by changes to the series capacitance with fixed shunt capacitance under different loads and speeds. For example, the variation in terminal voltage when changing the series capacitance between 20 μF and 105 μF, with a shunt capacitance value of 60 μF, under full load and rated speed conditions is between 0.2851 and 0.7998 pu, respectively;
- (c)
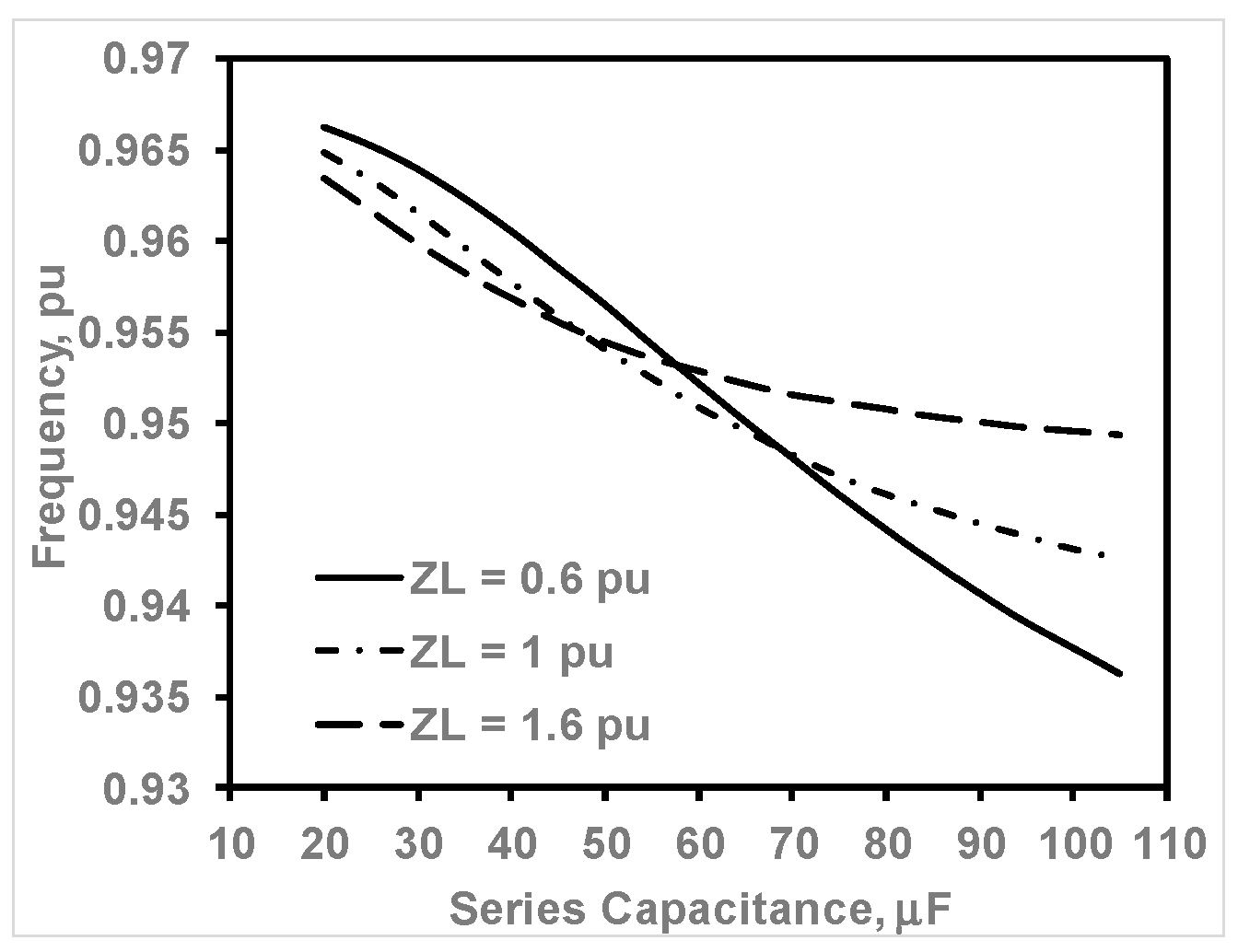
- The variation in output frequency is acceptable under different operating conditions with series excitation capacitance but fixed shunt capacitance. For example, the changes in output frequency for the same operating condition provided in the previous point are 0.8808 and 0.8667 pu, respectively;
- (d)
- A simply controlled electronic power circuit can be used to achieve fixed terminal voltage and approximately fixed frequency under different operating conditions by selecting the appropriate shunt excitation capacitance value and adjusting the series capacitance.
4. Conclusions
- ▪ The increase in Cse has a significant effect on increasing terminal voltage and a minor effect on decreasing output frequency;
- ▪ Increasing Csh decreases both terminal voltage and frequency;
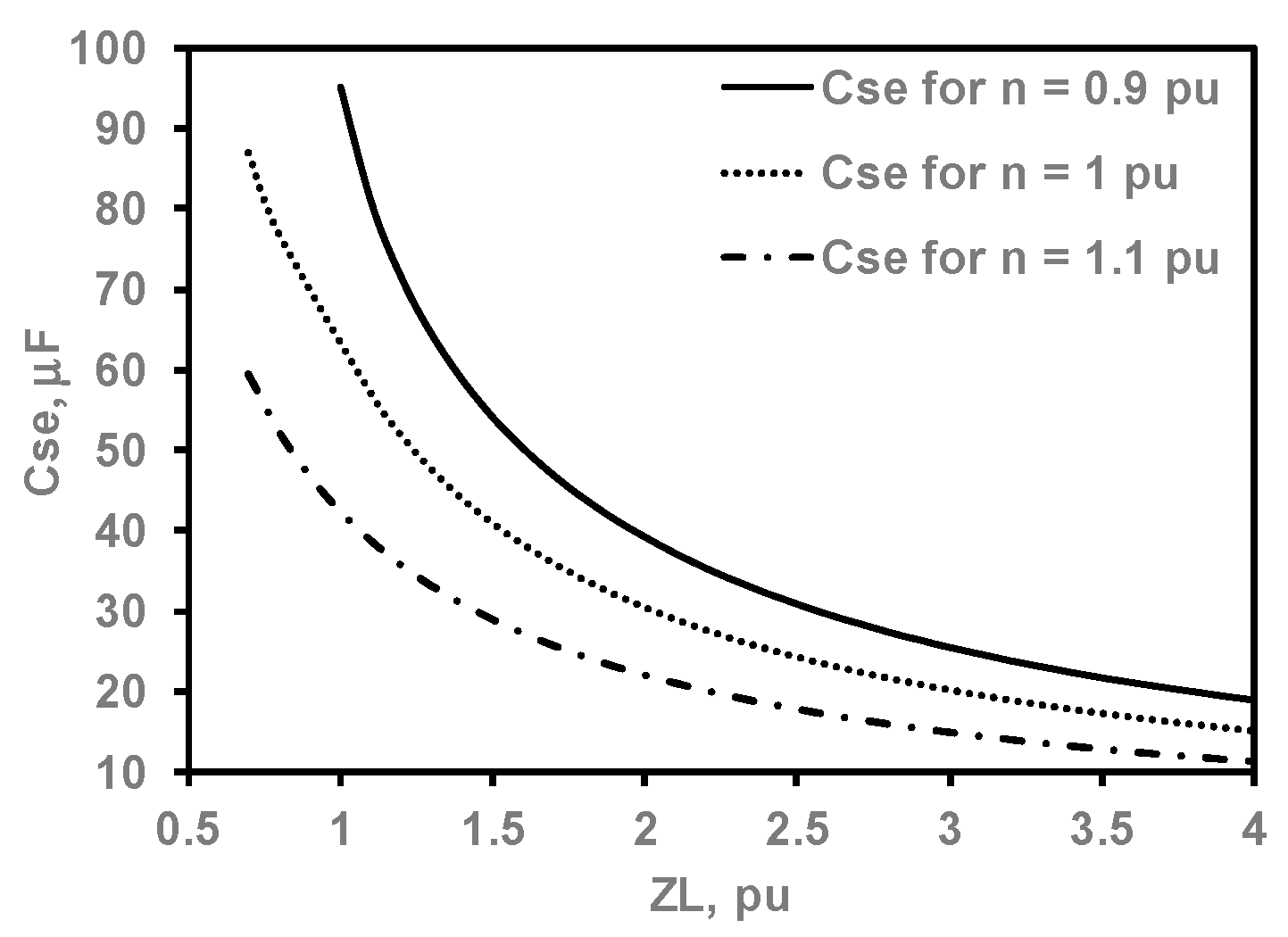
- ▪ For the same Csh and Cse values, terminal voltage increases by increasing load impedance or speed. The frequency also increases with speed, and is slightly affected by load impedance changes;
- ▪ The optimum Cse for fixed terminal voltage operations under constant load and speed increases with Csh;
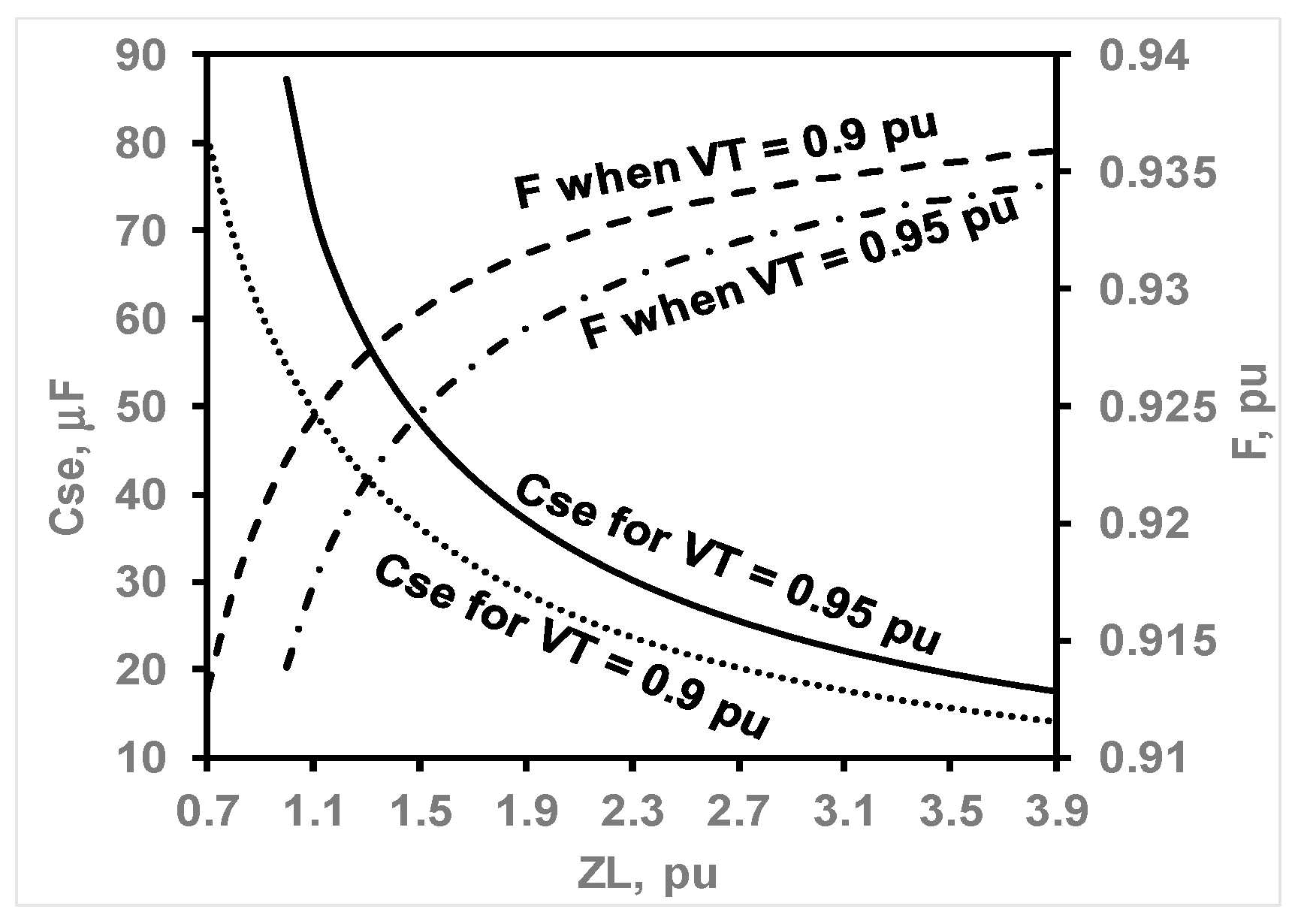
- ▪ The optimum Cse for fixed terminal voltage operation decreases with load impedance and increases with speed;
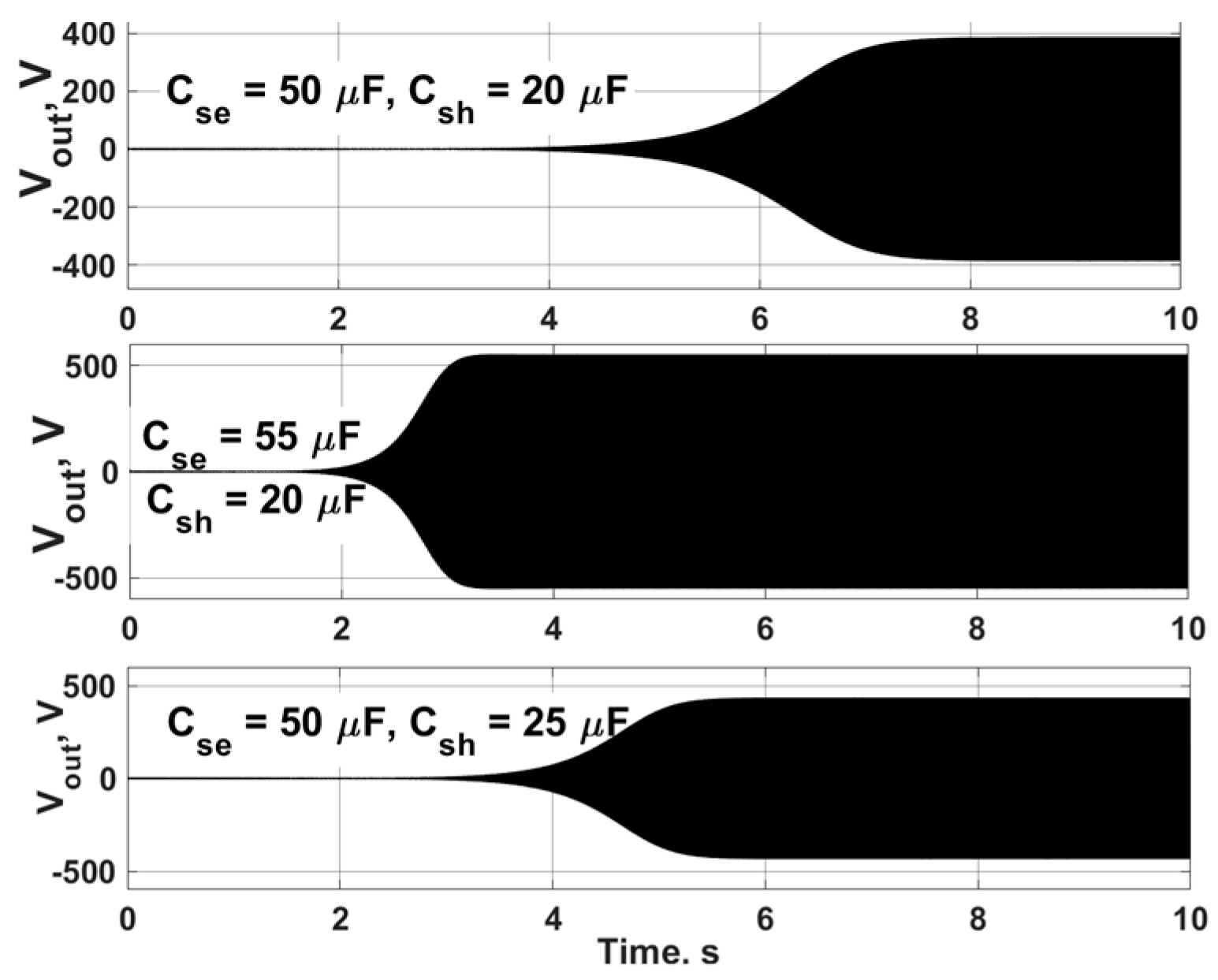
- ▪ During transient operations, a small increase in Cse shortens voltage buildup time and increases the voltage amplitude;
- ▪ Finally, a simple controller for adjusting the series excitation capacitance can successfully regulate the generator’s voltage with acceptable variation in the output frequency.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
List of Symbols
| Symbol | Description | Symbol | Description |
| main winding resistance | stator self-inductance | ||
| auxiliary winding resistance | rotor self-inductance | ||
| rotor winding resistance | stator leakage inductance | ||
| load resistance | rotor leakage inductance | ||
| load inductance | magnetizing inductance | ||
| synchronous speed | q-axis stator current | ||
| rotor speed | q-axis rotor current | ||
| series excitation capacitance | d-axis stator current | ||
| shunt excitation capacitor | d-axis rotor current | ||
| q-axis stator voltage | d-axis stator voltage | ||
| q-axis rotor voltage | d-axis rotor voltage | ||
| positive sequence of stator voltages | negative sequence of stator voltages | ||
| f | operating frequency in Hz | fb | base frequency in Hz |
| F | per-unit frequency | ωb | base frequency in rad/s |
| positive sequence of stator currents | negative sequence of stator currents | ||
| positive sequence of rotor currents | negative sequence of rotor currents | ||
| n | per-unit speed |
Appendix A
References
- Yolcan, O.O. World energy outlook and state of renewable energy: 10-Year evaluation. Innov. Green Dev. 2023, 2, 1–6. [Google Scholar] [CrossRef]
- Krishna, V.B.M.; Sandeep, V.; Murthy, S.S.; Yadlapati, K. Experimental investigation on performance comparison of self excited induction generator and permanent magnet synchronous generator for small scale renewable energy applications. Renew. Energy 2022, 195, 431–441. [Google Scholar] [CrossRef]
- Alex, Z.; Kimber, H.M.; Komp, R. Renewable Energy Village Power Systems for Remote and Impoverished Himalayan Villages in Nepal. In Proceedings of the International Conference on Renewable Energy for Developing Countries, Vienna, Austria, 14–16 March 2006. [Google Scholar]
- Benbouhenni, H.; Ionescu, L.-M.; Mazare, A.-G.; Zellouma, D.; Colak, I.; Bizon, N. Active and reactive power vector control using neural-synergetic-super twisting controllers of induction generators for variable-speed contra-rotating wind turbine systems. Meas. Control 2024, 57, 1–30. [Google Scholar] [CrossRef]
- Duvvuri, S.S.R.S.; Sandeep, V.; Yadlapati, K.; Krishna, V.B.M. Research on induction generators for isolated rural applications: State of art and experimental demonstration. Meas. Sens. 2022, 24, 1–7. [Google Scholar] [CrossRef]
- Apostoaia, C. MATLAB-Simulink Model of a Stand-Alone Induction Generator. In Proceedings of the 9th International Conference OPTIM’04, Brasov, Romania, 20–24 May 2004; Transylvania University of Brasov: Brasov, Romania, 2004; Volume 2, pp. 1–8. [Google Scholar] [CrossRef]
- Krishna, V.B.M.; Sandeep, V.; Narendra, B.K.; Prasad, K.R.K.V. Experimental study on self-excited induction generator for small-scale isolated rural electricity applications. Results Eng. 2023, 18, 2–8. [Google Scholar] [CrossRef]
- Chakraborty, S.; Samanta, J.; Pudur, R. Experimental analysis of a modified scheme for supplying single-phase remote loads from micro-hydro based three-phase self-excited induction generator. Meas. Sens. 2024, 33, 2–11. [Google Scholar] [CrossRef]
- Athamnah, I.; Anagreh, Y.; Anagreh, A. Optimization algorithms for steady state analysis of self excited induction generator. Int. J. Electr. Comput. Eng. (IJECE) 2023, 13, 6047–6057. [Google Scholar] [CrossRef]
- Anagreh, Y.N.; Al-Kofahi, I.S. Genetic Algorithm-Based Performance Analysis of Self-Excited Induction Generator. Int. J. Model. Simul. 2006, 26, 175–179. [Google Scholar] [CrossRef]
- Domínguez-García, J.L.; Gomis-Bellmunt, O.; Trilla-Romero, L.; Junyent-Ferré, A. Indirect vector control of a squirrel cage induction generator wind turbine. Comput. Math. Appl. 2012, 64, 102–114. [Google Scholar] [CrossRef]
- Makowski, K.; Leicht, A. Performance Characteristics of Single-Phase Self-Excited Induction Generators with an Iron Core of Various Non-Grain Oriented Electrical Sheets. Energies 2020, 13, 3166. [Google Scholar] [CrossRef]
- Ion, X.P. A Comprehensive Overview of Single–Phase Self-Excited Induction Generators. IEEE Access 2020, 8, 197420–197430. [Google Scholar] [CrossRef]
- Leicht, A.; Makowski, K. A single-phase induction motor operating as a self-excited induction generator. Arch. Electr. Eng. 2013, 62, 361–373. [Google Scholar] [CrossRef]
- Anagreh, Y.N. Matlab-Based Steady-State Analysis of Single-Phase Self-Excited Induction Generator. Int. J. Model. Simul. 2006, 26, 271–275. [Google Scholar] [CrossRef]
- Sanusi, K.A.; Olatomiwa, L.; Mohammed, A.D.; Sodiq, K.A. Capacitively-Excited Single-phase Asynchronous Generator for Autonomous Applications. Niger. J. Technol. Dev. 2022, 19, 128–135. [Google Scholar] [CrossRef]
- Arya, S.R.; Maurya, R.; Giri, A.K.; Qureshi, A.; Baladhanautham, C.B. Power quality solutions for effective utilization of single-phase induction generator using voltage source converter. Energy Sources Part A Recovery Util. Environ. Eff. 2020, 1–20. [Google Scholar] [CrossRef]
- Palwalia, D.K.; Singh, S.P. New Load Controller for Single-phase Self-excited Induction Generator. Electr. Power Compon. Syst. 2014, 57, 1455–1464. [Google Scholar] [CrossRef]
- Mossad, M.I.; Banakhr, F.A.; Ghoneim, S.S.M.; AbdulFattah, T.A.; Samy, M.M. Self-Regulated Single-phase Induction Generator for Variable Speed Stand-alone WECS. Intell. Autom. Soft Comput. (IASC) 2021, 28, 715–727. [Google Scholar] [CrossRef]
- Ahshan, R.; Iqbal, M.T. Voltage Controller of a Single Phase Self-excited Induction Generator. Open Renew. Energy J. 2009, 2, 84–90. [Google Scholar] [CrossRef][Green Version]
- Ojo, O.; Omozusi, O.; Gonoh, A.G.B. The Operation of a Stand-Alone, Single-Phase Induction Generator Using a Single-Phase, Pulse-Width Modulated Inverter with a Battery Supply. IEEE Trans. Energy Convers. 1999, 14, 526–531. [Google Scholar] [CrossRef]
- Krause, P.C.; Wasynczuk, O.; Sudhoff, S.D. Analysis of Electric Machinery and Drive Systems, 2nd ed.; Wiley-IEEE Press: Hoboken, NJ, USA, 2002. [Google Scholar]
- Ojo, O. The Transient and Qualitative Performance of a Self-Excited Single-Phase Induction Generator. IEEE Trans. Energy Convers. 1995, 10, 493–501. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anagreh, Y.; Al-Quraan, A. The Behavior of Terminal Voltage and Frequency of Wind-Driven Single-Phase Induction Generators under Variations in Excitation Capacitances for Different Operating Conditions. Energies 2024, 17, 3604. https://doi.org/10.3390/en17153604
Anagreh Y, Al-Quraan A. The Behavior of Terminal Voltage and Frequency of Wind-Driven Single-Phase Induction Generators under Variations in Excitation Capacitances for Different Operating Conditions. Energies. 2024; 17(15):3604. https://doi.org/10.3390/en17153604
Chicago/Turabian StyleAnagreh, Yaser, and Ayman Al-Quraan. 2024. "The Behavior of Terminal Voltage and Frequency of Wind-Driven Single-Phase Induction Generators under Variations in Excitation Capacitances for Different Operating Conditions" Energies 17, no. 15: 3604. https://doi.org/10.3390/en17153604
APA StyleAnagreh, Y., & Al-Quraan, A. (2024). The Behavior of Terminal Voltage and Frequency of Wind-Driven Single-Phase Induction Generators under Variations in Excitation Capacitances for Different Operating Conditions. Energies, 17(15), 3604. https://doi.org/10.3390/en17153604






