Improved Amott Method to Determine Oil Recovery Dynamics from Water-Wet Limestone Using GEV Statistics
Abstract
1. Introduction
2. Materials and Methods
2.1. Core Samples and Solutions
2.2. Core Plug Saturation with Mineral Oil
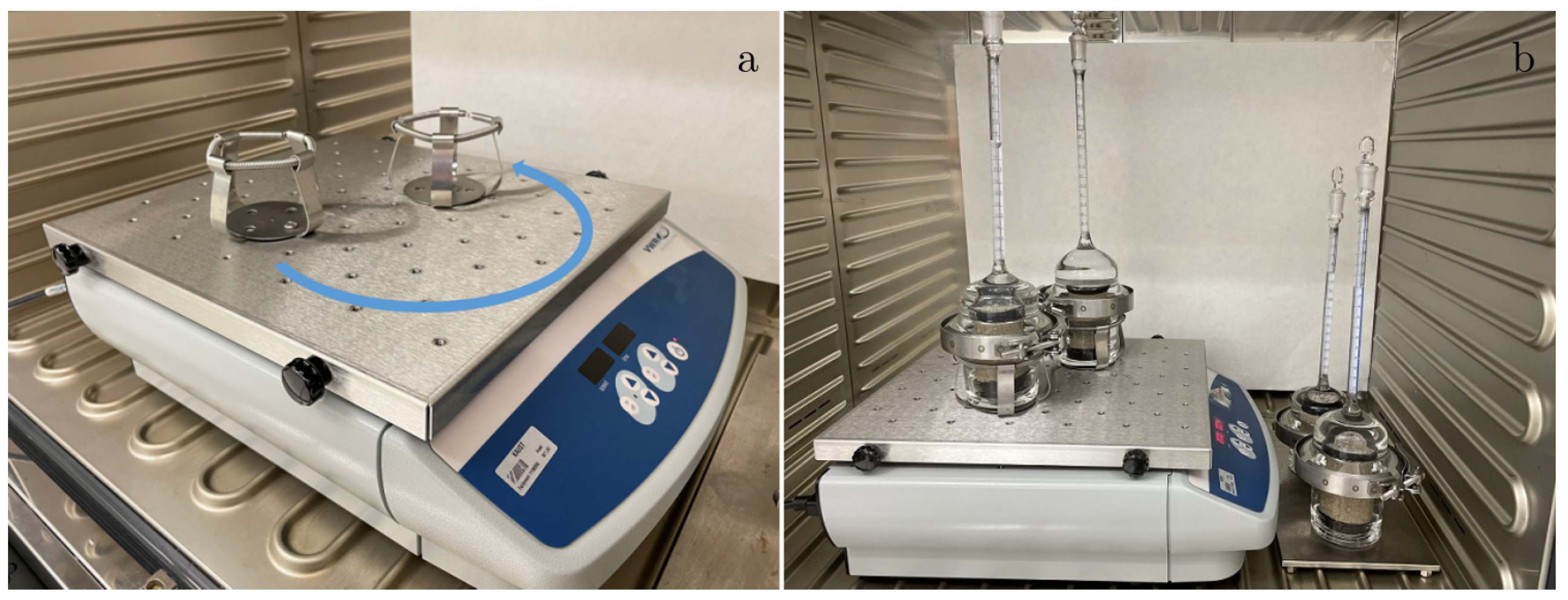
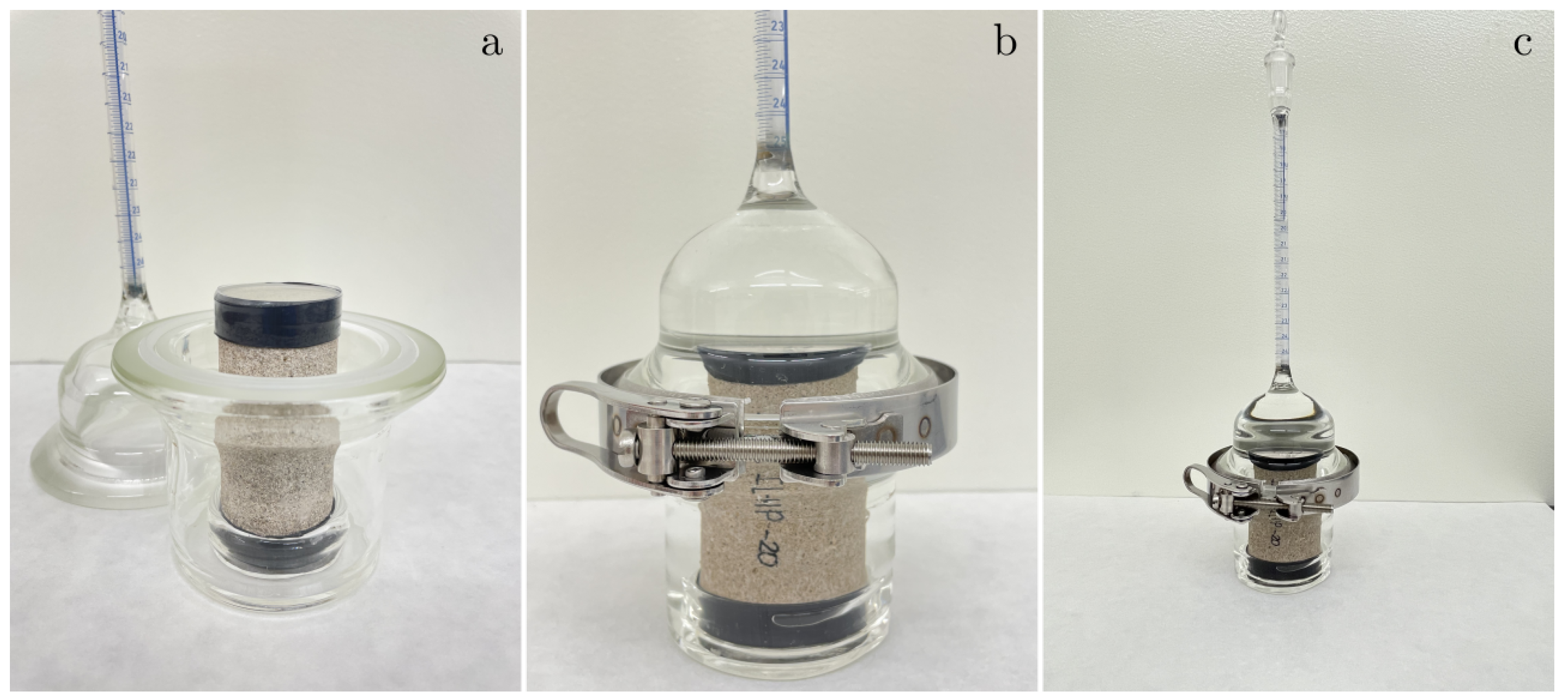
2.3. Modified Amott-Cell Experiment
2.4. GEV Scaling of Cumulative Oil Recovery
2.4.1. GEV Distribution
2.4.2. Finding from a Nonlinear Least Squares Minimization Problem
2.4.3. Analysis of Oil Production Dynamics
3. Results and Discussion
3.1. Oil Holdup in Amott Experiments
3.2. Results of Imbibition Experiments and GEV Modeling
3.2.1. High–Viscosity Oil
3.2.2. Low-Viscosity Oil
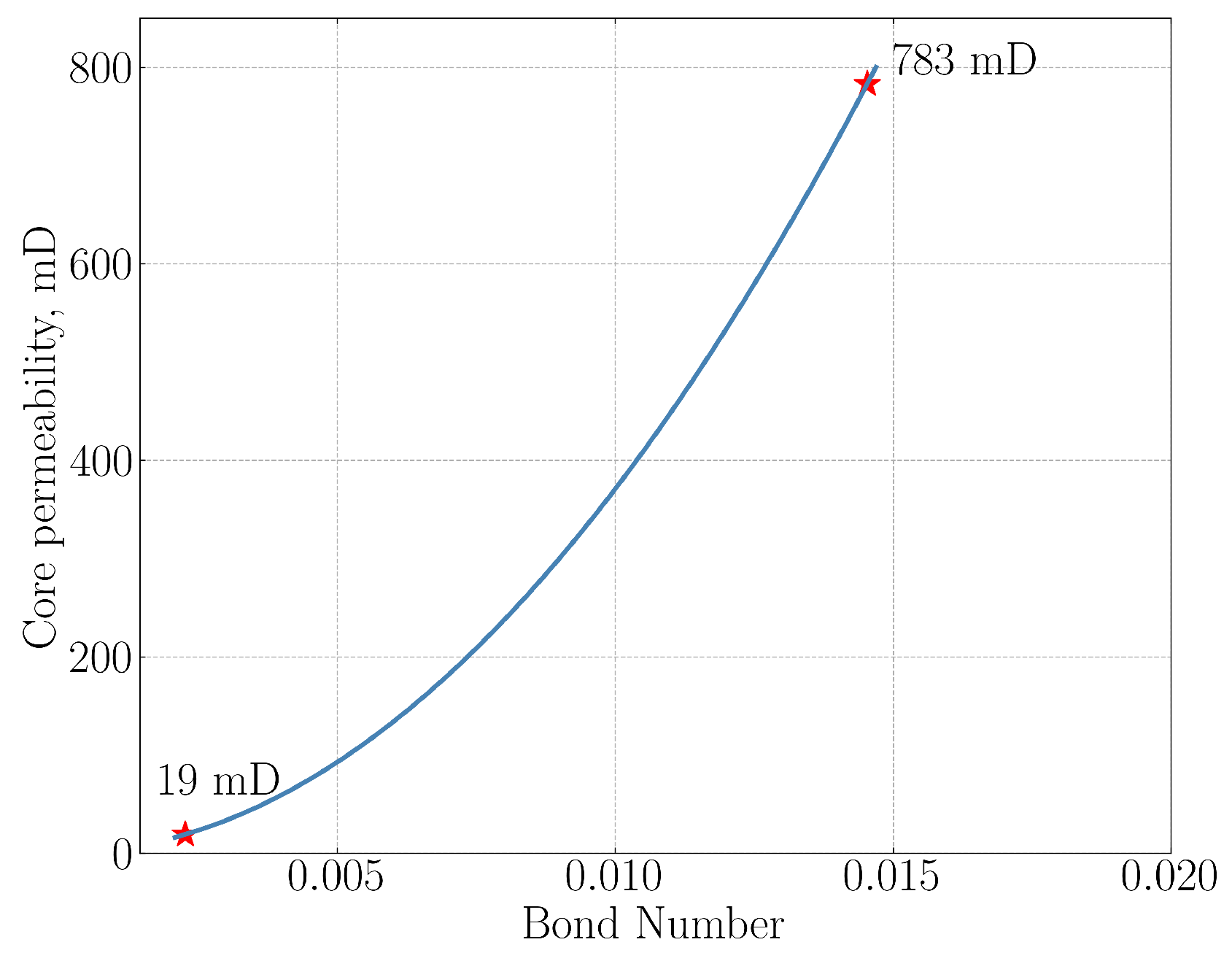
3.2.3. High-Permeability Core
3.2.4. Summary
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B
References
- Pooladi-Darvish, M.; Firoozabadi, A. Experiments and Modelling of Water Injection in Water-wet Fractured Porous Media. J. Can. Pet. Technol. 2000, 39, PETSOC-00-03-02. [Google Scholar] [CrossRef]
- Morrow, N.R.; Mason, G. Recovery of Oil by Spontaneous Imbibition. Curr. Opin. Colloid Interface Sci. 2001, 6, 321–337. [Google Scholar] [CrossRef]
- Patzek, T.W.; Saad, A.M.; Hassan, A. Multimodal Carbonates: Distribution of Oil Saturation in the Microporous Regions of Arab Formations. Energies 2022, 15, 1243. [Google Scholar] [CrossRef]
- Lenormand, R.; Zarcone, C.; Sarr, A. Mechanisms of the Displacement of One Fluid by Another in a Network of Capillary Ducts. J. Fluid Mech. 1983, 135, 337–353. [Google Scholar] [CrossRef]
- Zhao, B.; MacMinn, C.W.; Juanes, R. Wettability Control on Multiphase Flow in Patterned Microfluidics. Proc. Natl. Acad. Sci. USA 2016, 113, 10251–10256. [Google Scholar] [CrossRef] [PubMed]
- Amott, E. Observations Relating to the Wettability of Porous Rock. Pet. Trans. AIME 1959, 216, 156–162. [Google Scholar] [CrossRef]
- Alaamri, J.; Chandra, V.; Addassi, M.; Hoteit, H. Experimental and Numerical Investigation of Spontaneous Imbibition in Multilayered Porous Systems. Energy Fuels 2023, 37, 11694–11706. [Google Scholar] [CrossRef]
- Ma, S.; Zhang, X.; Morrow, N.; Zhou, X. Characterization of Wettability From Spontaneous Imbibition Measurements. J. Can. Pet. Technol. 1999, 38, PETSOC-99-13-49. [Google Scholar] [CrossRef]
- Fernø, M.; Haugen, Å.; Wickramathilaka, S.; Howard, J.; Graue, A.; Mason, G.; Morrow, N. Magnetic Resonance Imaging of the Development of Fronts During Spontaneous Imbibition. J. Pet. Sci. Eng. 2013, 101, 1–11. [Google Scholar] [CrossRef]
- Fernø, M.; Haugen, A.; Brattekas, B.; Morrow, N.; Mason, G. Spontaneous Imbibition Revisited—A New Method to Determine Kr and Pc by Inclusion of the Capillary Backpressure. In Proceedings of the IOR 2015—18th European Symposium on Improved Oil Recovery, Dresden, Germany, 14–16 April 2015; EAGE Publications BV: Utrecht, The Netherlands, 2015. [Google Scholar] [CrossRef]
- Graue, A.; Viksund, B.G.; Eilertsen, T.; Moe, R. Systematic Wettability Alteration by Aging Sandstone and Carbonate Rock in Crude Oil. J. Pet. Sci. Eng. 1999, 24, 85–97. [Google Scholar] [CrossRef]
- Cobos, J.E.; Sandnes, M.; Steinsbo, M.; Brattekas, B.; Sogaard, E.G.; Graue, A. Evaluation of Wettability Alteration in Heterogeneous Limestone at Microscopic and Macroscopic Levels. J. Pet. Sci. Eng. 2021, 202, 108534. [Google Scholar] [CrossRef]
- Zhou, X.; Morrow, N.; Ma, S. Interrelationship of Wettability, Initial Water Saturation, Aging Time, and Oil Recovery by Spontaneous Imbibition and Waterflooding. SPE J. 2000, 5, 199–207. [Google Scholar] [CrossRef]
- Ghedan, S.G.; Canbaz, C.H.; Mtawaa, B. Effect of Shape Factor, IFT and Amott Method Derived Wettability on the Imbibition Process. In Proceedings of the International Petroleum Technology Conference, Doha, Qatar, 7–9 December 2009. [Google Scholar] [CrossRef]
- Clerke, E.; Funk, J.; Shtepani, E. Spontaneous Imbibition of Water into Oil Saturated M_1 Bimodal Limestone. In Proceedings of the International Petroleum Technology Conference, Beijing, China, 26–28 March 2013. [Google Scholar] [CrossRef]
- Tang, G.Q.; Firoozabadi, A. Effect of Pressure Gradient and Initial Water Saturation on Water Injection in Water-Wet and Mixed-Wet Fractured Porous Media. SPE Reserv. Eval. Eng. 2001, 4, 516–524. [Google Scholar] [CrossRef]
- Fernø, M.A.; Torsvik, M.; Haugland, S.; Graue, A. Dynamic Laboratory Wettability Alteration. Energy Fuels 2010, 24, 3950–3958. [Google Scholar] [CrossRef]
- Akin, S.; Schembre, J.; Bhat, S.; Kovscek, A. Spontaneous imbibition characteristics of diatomite. J. Pet. Sci. Eng. 2000, 25, 149–165. [Google Scholar] [CrossRef]
- Salathiel, R. Oil Recovery by Surface Film Drainage In Mixed-Wettability Rocks. J. Pet. Technol. 1973, 25, 1216–1224. [Google Scholar] [CrossRef]
- Patzek, T.W. Verification of a Complete Pore Network Simulator of Drainage and Imbibition. SPE J. 2001, 6, 144–156. [Google Scholar] [CrossRef]
- Kovscek, A.R.; Wong, H.; Radke, C.J. A Pore-Level Scenario for the Development of Mixed Wettability in Oil Reservoirs. AIChE J. 1993, 39, 1072–1085. [Google Scholar] [CrossRef]
- Schmatz, J.; Klaver, J.; Jiang, M.; Urai, J.L. Nanoscale Morphology of Brine-Oil-Mineral Contacts in Connected Pores of Carbonate Reservoirs: Insights on Wettability from Cryo-BIB-SEM. SPE J. 2017, 22, 1374–1384. [Google Scholar] [CrossRef]
- Freer, E.; Svitova, T.; Radke, C. The role of interfacial rheology in reservoir mixed wettability. J. Pet. Sci. Eng. 2003, 39, 137–158. [Google Scholar] [CrossRef]
- Hu, X.; Yutkin, M.P.; Hassan, S.; Wu, J.; Prausnitz, J.M.; Radke, C.J. Asphaltene Adsorption from Toluene onto Silica through Thin Water Layers. Langmuir 2018, 35, 428–434. [Google Scholar] [CrossRef] [PubMed]
- Schechter, D.; Zhou, D.; Orr, F. Low IFT Drainage and Imbibition. J. Pet. Sci. Eng. 1994, 11, 283–300. [Google Scholar] [CrossRef]
- Kashchiev, D.; Firoozabadi, A. Analytical Solutions for 1-D Countercurrent Imbibition in Water-Wet Media. In Proceedings of the SPE/DOE Improved Oil Recovery Symposium, Tulsa, OK, USA, 13–17 April 2002. [Google Scholar] [CrossRef]
- Babadagli, T. Dynamics of Capillary Imbibition When Surfactant, Polymer, and Hot Water Are Used as Aqueous Phase for Oil Recovery. J. Colloid Interface Sci. 2002, 246, 203–213. [Google Scholar] [CrossRef] [PubMed]
- Xie, X.; Morrow, N.R. Oil Recovery by Spontaneous Imbibition from Weakly Water-Wet Rocks. Petrophysics 2001, 42, 313–322. [Google Scholar]
- Sukee, A.; Nunta, T.; Haruna, M.A.; Kalantariasl, A.; Tangparitkul, S. Influence of Sequential Changes in the Crude Oil-Water Interfacial Tension on Spontaneous Imbibition in Oil-Wet Sandstone. J. Pet. Sci. Eng. 2022, 210, 110032. [Google Scholar] [CrossRef]
- Standnes, D.C. Experimental Study of the Impact of Boundary Conditions on Oil Recovery by Co-Current and Counter-Current Spontaneous Imbibition. Energy Fuels 2003, 18, 271–282. [Google Scholar] [CrossRef]
- Standnes, D.C. Spontaneous imbibition of water into cylindrical cores with high aspect ratio: Numerical and experimental results. J. Pet. Sci. Eng. 2006, 50, 151–160. [Google Scholar] [CrossRef]
- Richards, L.A. The Usefulness of Capillary Potential to Soil Moisture and Plant Investigations. J. Agric. Res. 1928, 37, 719–742. [Google Scholar]
- Richards, L.A. Capillary Conduction of Liquids Through Porous Mediums. Physics 1931, 1, 318–333. [Google Scholar] [CrossRef]
- Muskat, M. The Flow of Homogeneous Fluids through Porous Media; McGraw-Hill Book Company, Inc.: New York, NY, USA, 1937. [Google Scholar]
- Rapoport, L.; Leas, W. Properties of Linear Waterfloods. J. Pet. Technol. 1953, 5, 139–148. [Google Scholar] [CrossRef]
- Kashchiev, D.; Firoozabadi, A. Analytical Solutions for 1D Countercurrent Imbibition in Water-Wet Media. SPE J. 2003, 8, 401–408. [Google Scholar] [CrossRef]
- Schmid, K.S.; Geiger, S. Universal scaling of spontaneous imbibition for water-wet systems. Water Resour. Res. 2012, 48, r3507. [Google Scholar] [CrossRef]
- Schmid, K.; Geiger, S. Universal scaling of spontaneous imbibition for arbitrary petrophysical properties: Water-wet and mixed-wet states and Handy’s conjecture. J. Pet. Sci. Eng. 2013, 101, 44–61. [Google Scholar] [CrossRef]
- Schmid, K.S.; Alyafei, N.; Geiger, S.; Blunt, M.J. Analytical Solutions for Spontaneous Imbibition: Fractional-Flow Theory and Experimental Analysis. SPE J. 2016, 21, 2308–2316. [Google Scholar] [CrossRef]
- Barenblatt, G.I.; Patzek, T.W.; Silin, D.B. The Mathematical Model of Nonequilibrium Effects in Water-Oil Displacement. SPE J. 2003, 8, 409–416. [Google Scholar] [CrossRef]
- Mason, G.; Fischer, H.; Morrow, N.; Ruth, D. Correlation for the effect of fluid viscosities on counter-current spontaneous imbibition. J. Pet. Sci. Eng. 2010, 72, 195–205. [Google Scholar] [CrossRef]
- Mirzaei-Paiaman, A.; Masihi, M. Scaling Equations for Oil/Gas Recovery from Fractured Porous Media by Counter-Current Spontaneous Imbibition: From Development to Application. Energy Fuels 2013, 27, 4662–4676. [Google Scholar] [CrossRef]
- Bassir, S.M.; Shokrollahzadeh Behbahani, H.; Shahbazi, K.; Kord, S.; Mirzaei-Paiaman, A. Towards prediction of oil recovery by spontaneous imbibition of modified salinity brine into limestone rocks: A scaling study. J. Pet. Explor. Prod. Technol. 2022, 13, 79–99. [Google Scholar] [CrossRef]
- Silin, D.; Patzek, T. On Barenblatt’s Model of Spontaneous Countercurrent Imbibition. Transp. Porous Media 2004, 54, 297–322. [Google Scholar] [CrossRef]
- Gumbel, E.J. Statistics of Extremes; Columbia University Press: New York, NY, USA, 1958. [Google Scholar] [CrossRef]
- Patzek, T.W.; Male, F.; Marder, M. Gas production in the Barnett Shale obeys a simple scaling theory. Proc. Natl. Acad. Sci. USA 2013, 110, 19731–19736. [Google Scholar] [CrossRef]
- Patzek, T.W.; Saputra, W.; Kirati, W.; Marder, M. Generalized Extreme Value Statistics, Physical Scaling, and Forecasts of Gas Production in the Barnett Shale. Energy Fuels 2019, 33, 12154–12169. [Google Scholar] [CrossRef]
- Saputra, W.; Kirati, W.; Patzek, T. Generalized Extreme Value Statistics, Physical Scaling and Forecasts of Oil Production in the Bakken Shale. Energies 2019, 12, 3641. [Google Scholar] [CrossRef]
- Saputra, W.; Kirati, W.; Patzek, T. Physical Scaling of Oil Production Rates and Ultimate Recovery from All Horizontal Wells in the Bakken Shale. Energies 2020, 13, 2052. [Google Scholar] [CrossRef]
- Saputra, W.; Kirati, W.; Patzek, T.W. Generalized extreme value statistics, physical scaling and forecasts of gas production in the Haynesville shale. J. Nat. Gas Sci. Eng. 2021, 94, 104041. [Google Scholar] [CrossRef]
- Saputra, W.; Kirati, W.; Patzek, T. Generalized Extreme Value Statistics, Physical Scaling and Forecasts of Oil Production from All Vertical Wells in the Permian Basin. Energies 2022, 15, 904. [Google Scholar] [CrossRef]
- Saputra, W.; Kirati, W.; Patzek, T. Forecast of Economic Tight Oil and Gas Production in Permian Basin. Energies 2022, 15, 43. [Google Scholar] [CrossRef]
- Saputra, W.; Patzek, T.; Torres-Verdín, C. URTEC-3858983-MS: Physics-Based and Data-Driven Production Forecasting in the Eagle Ford Shale. In Proceedings of the SPE/AAPG/SEG Unconventional Resources Technology Conference, Denver, CO, USA, 13–15 June 2023. [Google Scholar] [CrossRef]
- Haider, S.; Saputra, W.; Patzek, T. The Key Factors That Determine the Economically Viable, Horizontal Hydrofractured Gas Wells in Mudrocks. Energies 2020, 13, 2348. [Google Scholar] [CrossRef]
- Kaprielova, K.; Yutkin, M.; Gmira, A.; Ayirala, S.; Radke, C.; Patzek, T. Improved Amott Cell Procedure for Predictive Modeling of Oil Recovery Dynamics from Mixed-Wet Carbonates. In Proceedings of the SPE Improved Oil Recovery Conference, Virtual, 25–29 April 2022. [Google Scholar] [CrossRef]
- Nelder, J.A.; Mead, R. A Simplex Method for Function Minimization. Comput. J. 1965, 7, 308–313. [Google Scholar] [CrossRef]


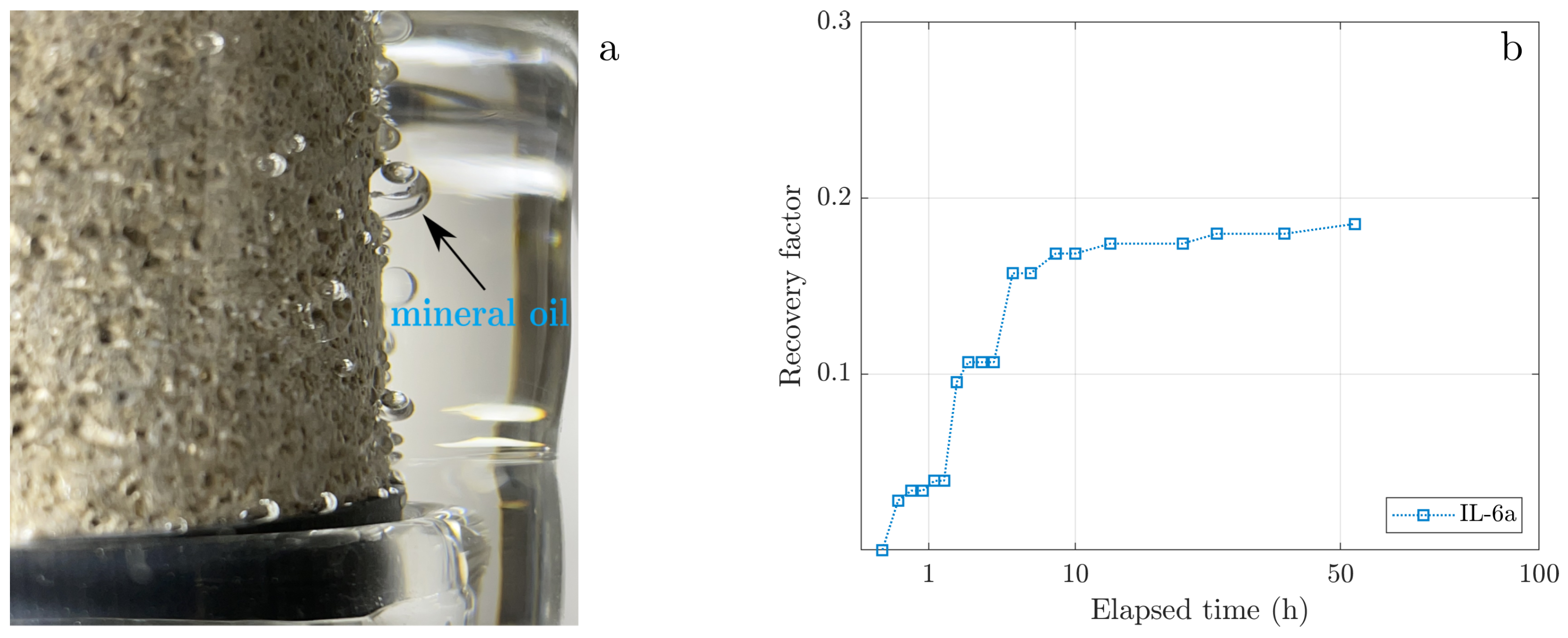
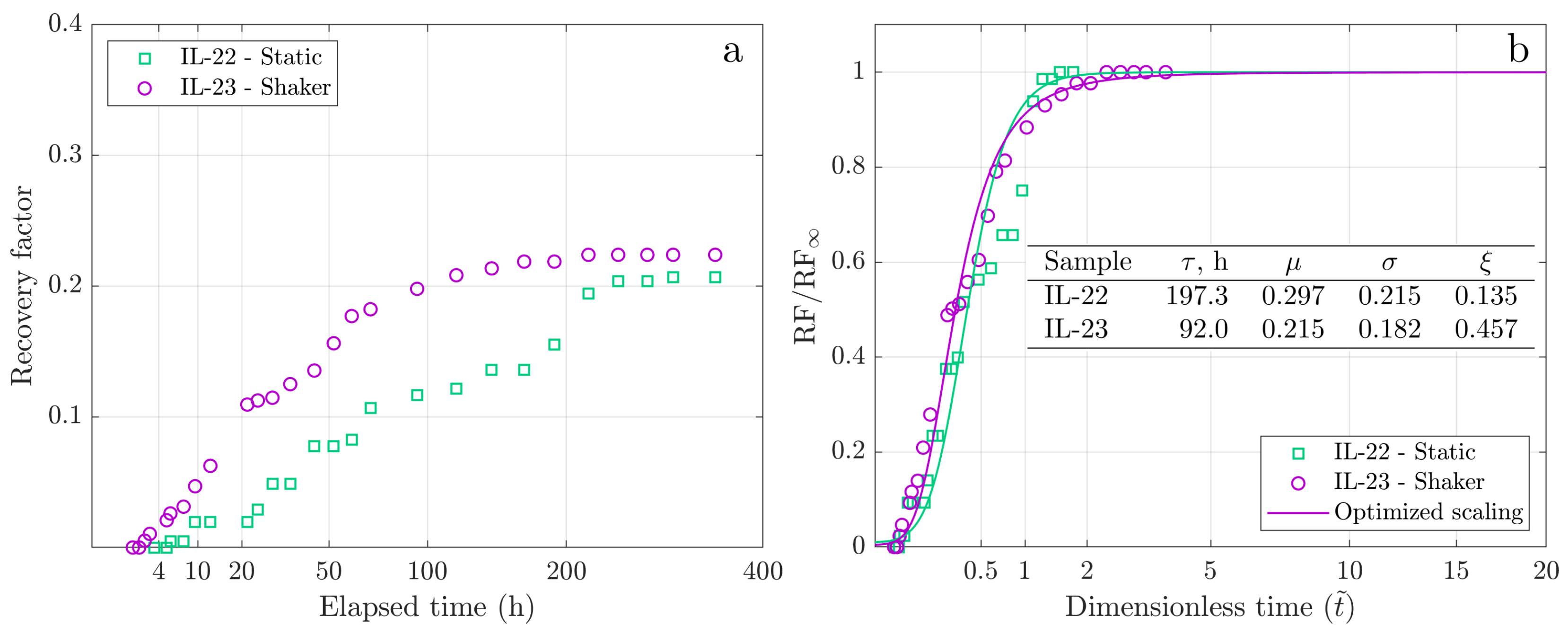
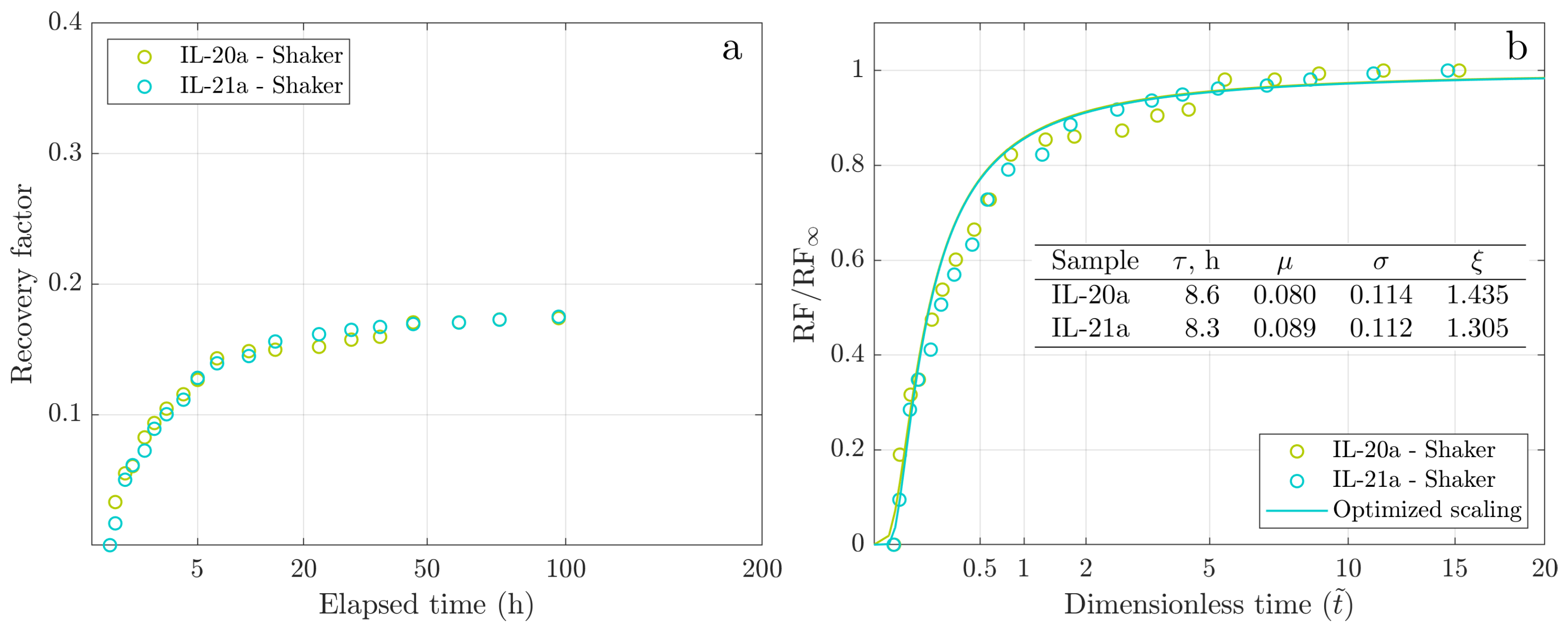



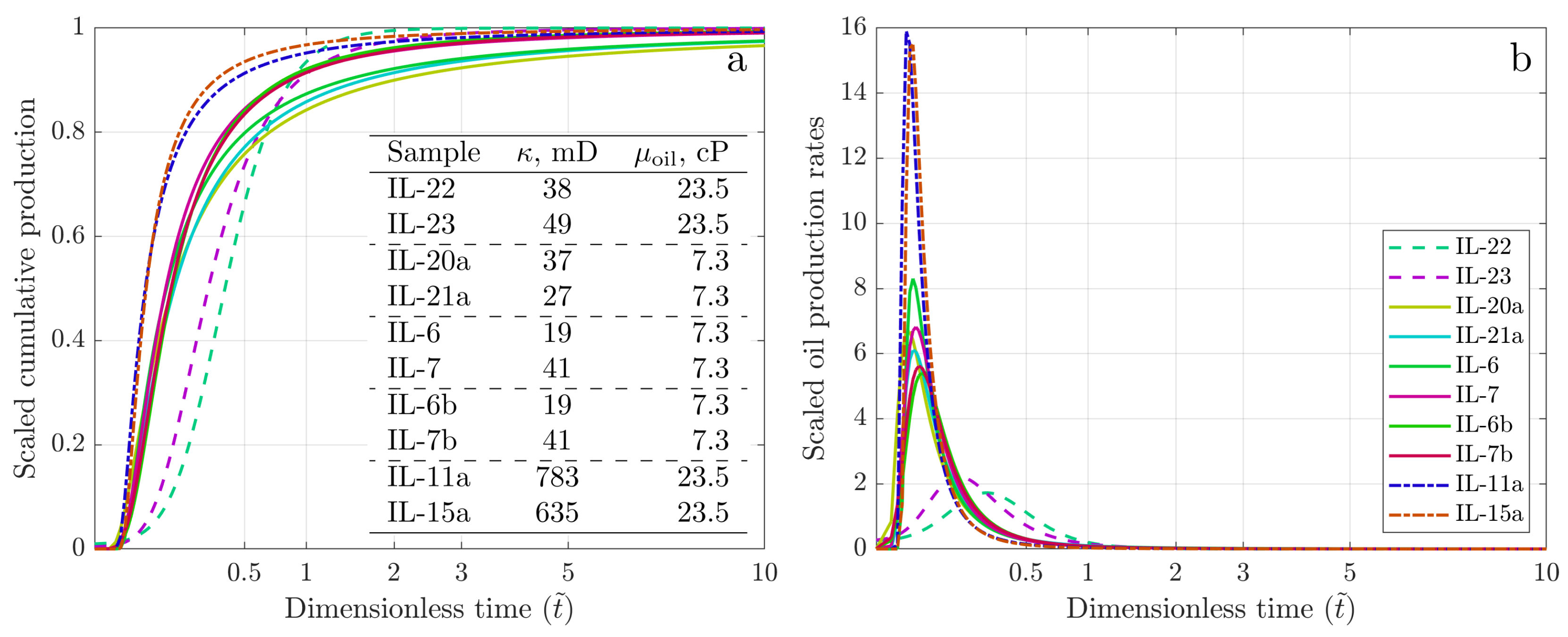
| Sample code | , % | , mD | Oil code | , cP at 60 °C | , fraction | RF fraction | Imbibition regime | GEV , h | GEV | GEV | GEV |
|---|---|---|---|---|---|---|---|---|---|---|---|
| IL-22 | 15.5 | 38 | Oil-2 * | 23.5 | 0.81 | 0.207 | static | 197.3 | 0.297 | 0.215 | 0.135 |
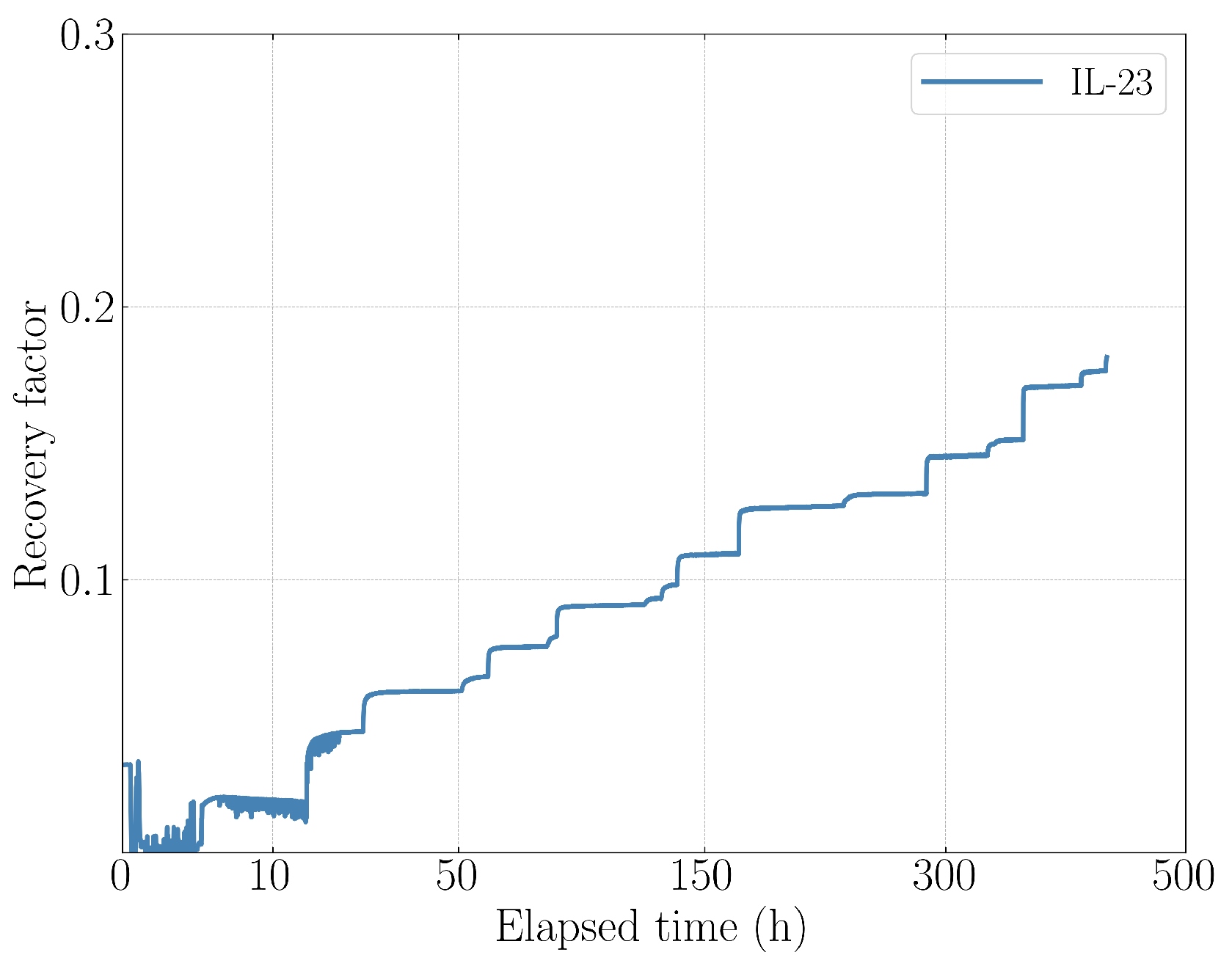
| IL-23 | 16.0 | 49 | Oil-2 | 23.5 | 0.72 | 0.224 | shaking | 92.0 | 0.215 | 0.182 | 0.457 |
| IL-20a | 16.0 | 37 | Oil-1 | 7.3 | 0.73 | 0.174 | shaking | 8.6 | 0.080 | 0.114 | 1.435 |
| IL-21a | 15.1 | 27 | Oil-1 | 7.3 | 0.75 | 0.176 | shaking | 8.3 | 0.089 | 0.112 | 1.305 |
| IL-6 | 14.8 | 19 | Oil-1 | 7.3 | 0.69 | 0.222 | shaking | 6.1 | 0.074 | 0.087 | 1.371 |
| IL-7 | 15.1 | 41 | Oil-1 | 7.3 | 0.70 | 0.215 | static | 6.2 | 0.076 | 0.082 | 1.045 |
| IL-6b | 14.8 | 19 | Oil-1 | 7.3 | 0.73 | 0.209 | shaking | 6.3 | 0.092 | 0.094 | 0.910 |
| IL-7b | 15.1 | 41 | Oil-1 | 7.3 | 0.70 | 0.208 | shaking | 7.2 | 0.088 | 0.094 | 0.968 |
| IL-11a | 15.9 | 783 | Oil-2 | 23.5 | 0.65 | 0.227 | shaking | 5.1 | 0.040 | 0.037 | 1.131 |
| IL-15a | 16.5 | 635 | Oil-2 | 23.5 | 0.70 | 0.179 | shaking | 6.6 | 0.044 | 0.034 | 0.981 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kaprielova, K.M.; Yutkin, M.P.; Mowafi, M.; Gmira, A.; Ayirala, S.; Yousef, A.; Radke, C.J.; Patzek, T.W. Improved Amott Method to Determine Oil Recovery Dynamics from Water-Wet Limestone Using GEV Statistics. Energies 2024, 17, 3599. https://doi.org/10.3390/en17143599
Kaprielova KM, Yutkin MP, Mowafi M, Gmira A, Ayirala S, Yousef A, Radke CJ, Patzek TW. Improved Amott Method to Determine Oil Recovery Dynamics from Water-Wet Limestone Using GEV Statistics. Energies. 2024; 17(14):3599. https://doi.org/10.3390/en17143599
Chicago/Turabian StyleKaprielova, Ksenia M., Maxim P. Yutkin, Mahmoud Mowafi, Ahmed Gmira, Subhash Ayirala, Ali Yousef, Clayton J. Radke, and Tadeusz W. Patzek. 2024. "Improved Amott Method to Determine Oil Recovery Dynamics from Water-Wet Limestone Using GEV Statistics" Energies 17, no. 14: 3599. https://doi.org/10.3390/en17143599
APA StyleKaprielova, K. M., Yutkin, M. P., Mowafi, M., Gmira, A., Ayirala, S., Yousef, A., Radke, C. J., & Patzek, T. W. (2024). Improved Amott Method to Determine Oil Recovery Dynamics from Water-Wet Limestone Using GEV Statistics. Energies, 17(14), 3599. https://doi.org/10.3390/en17143599