Research on Variable Speed Variable Displacement Power Unit with High Efficiency and High Dynamic Optimized Matching
Abstract
1. Introduction
2. Dynamic Characteristic Analysis of the VSVDPU
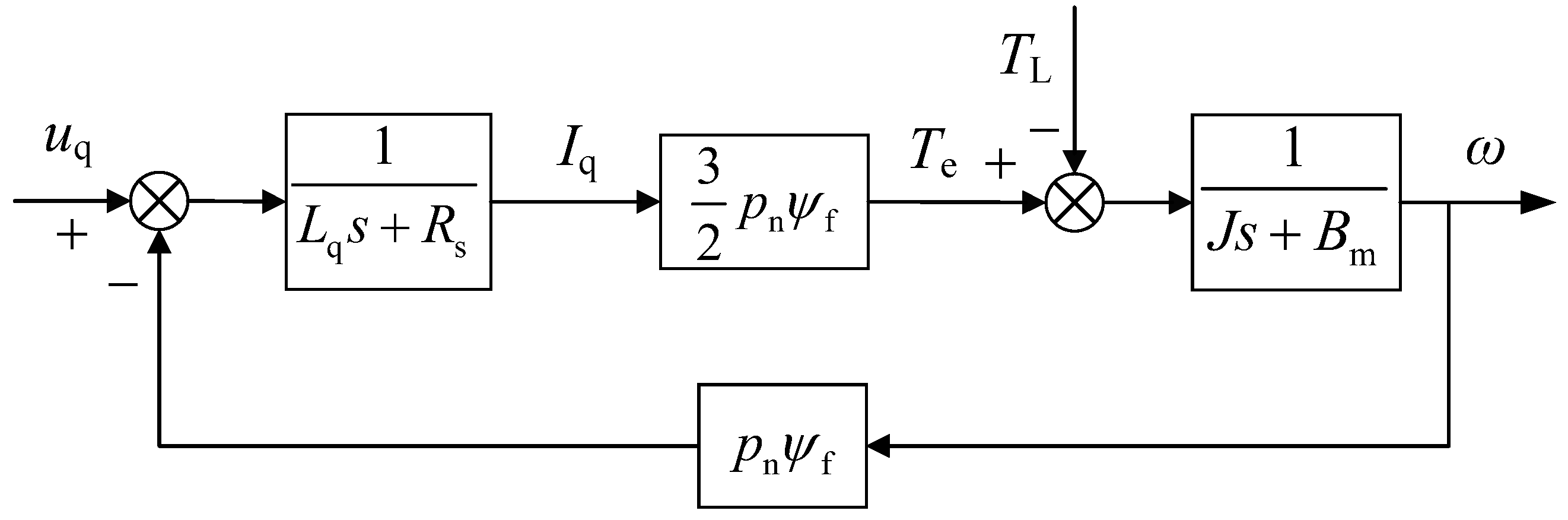
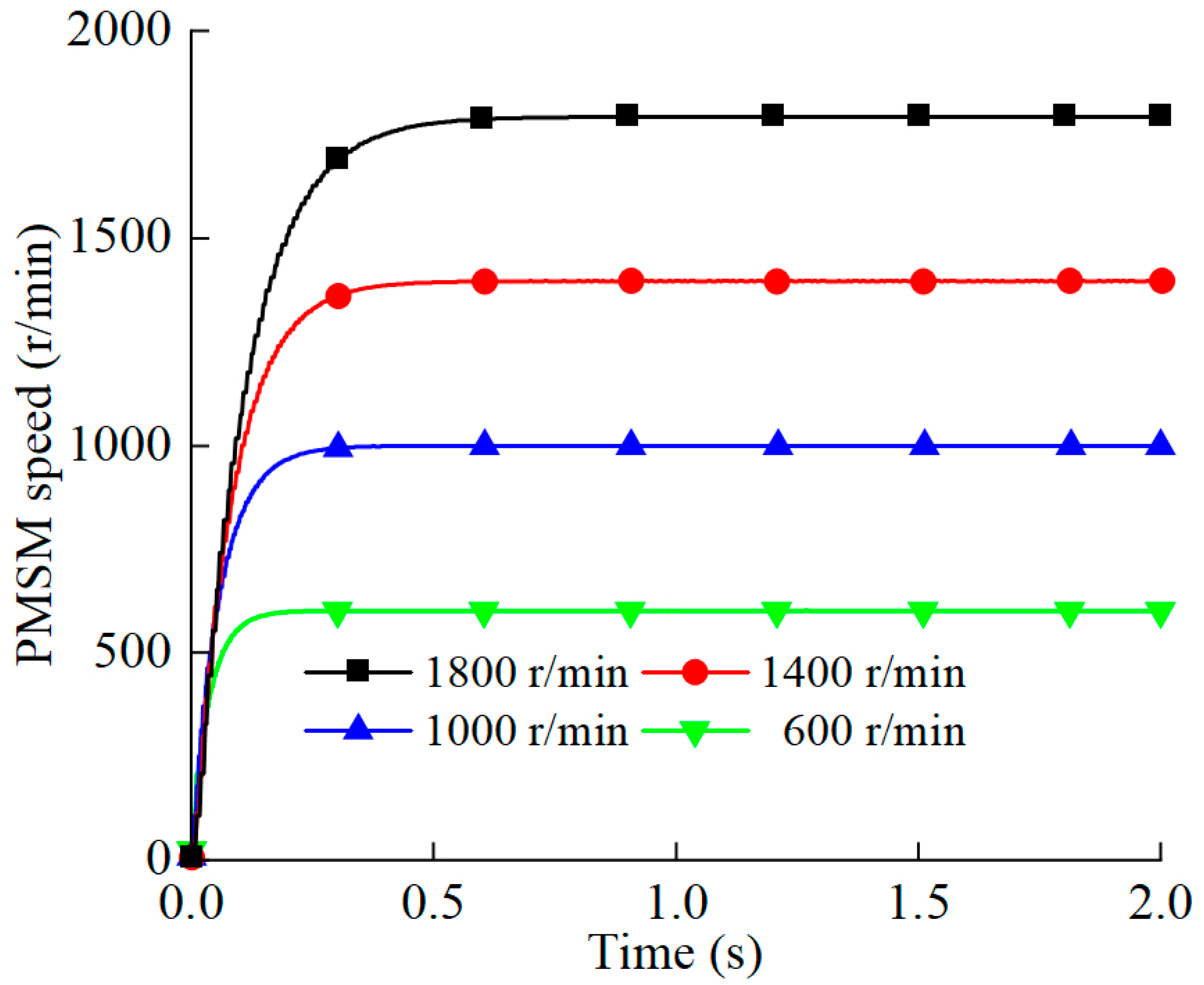
2.1. Dynamic Characteristics Analysis of the PMSM
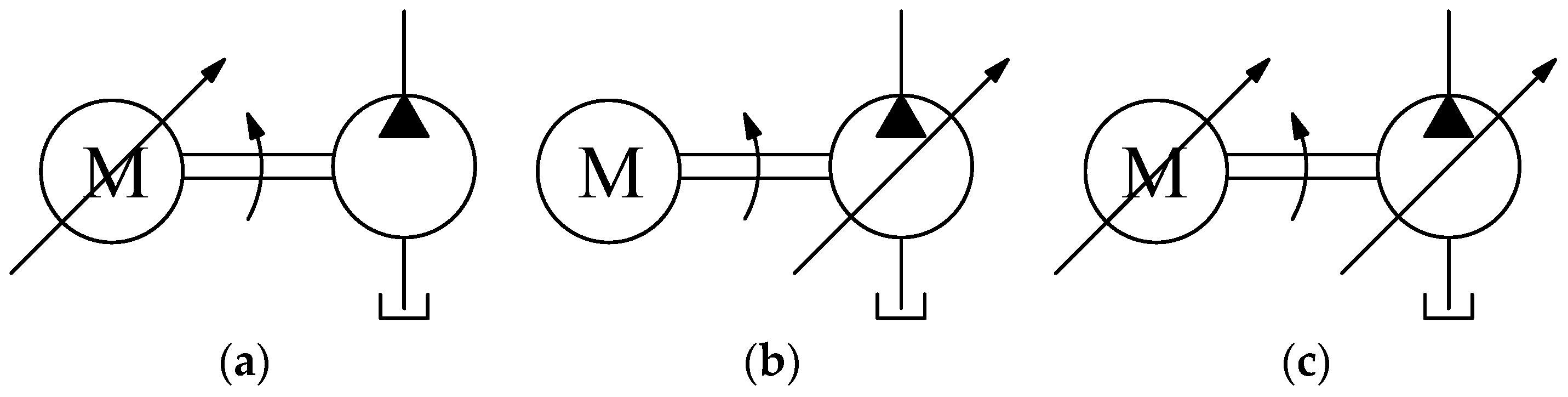
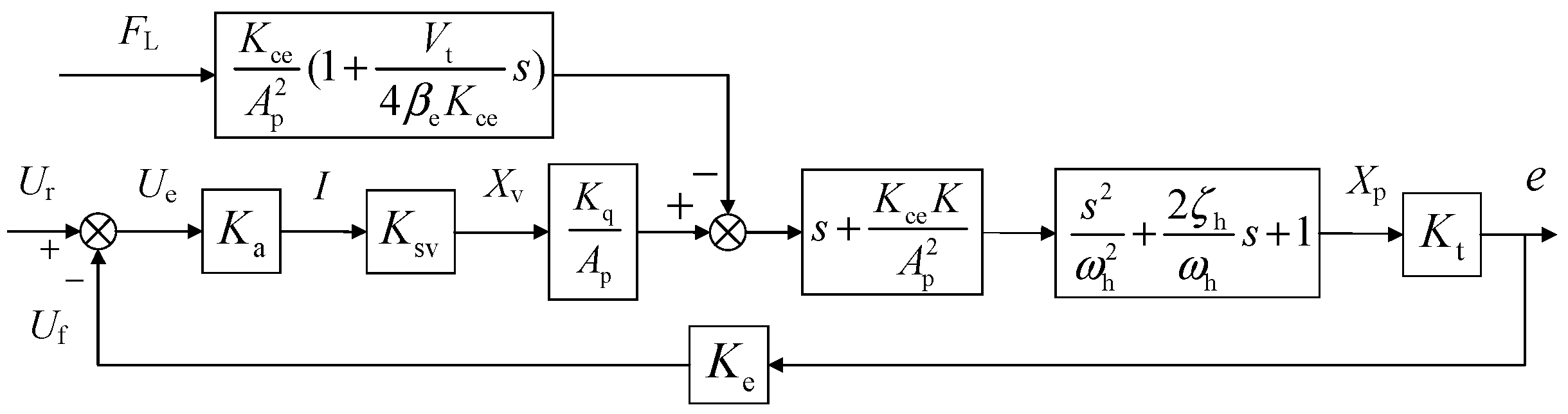
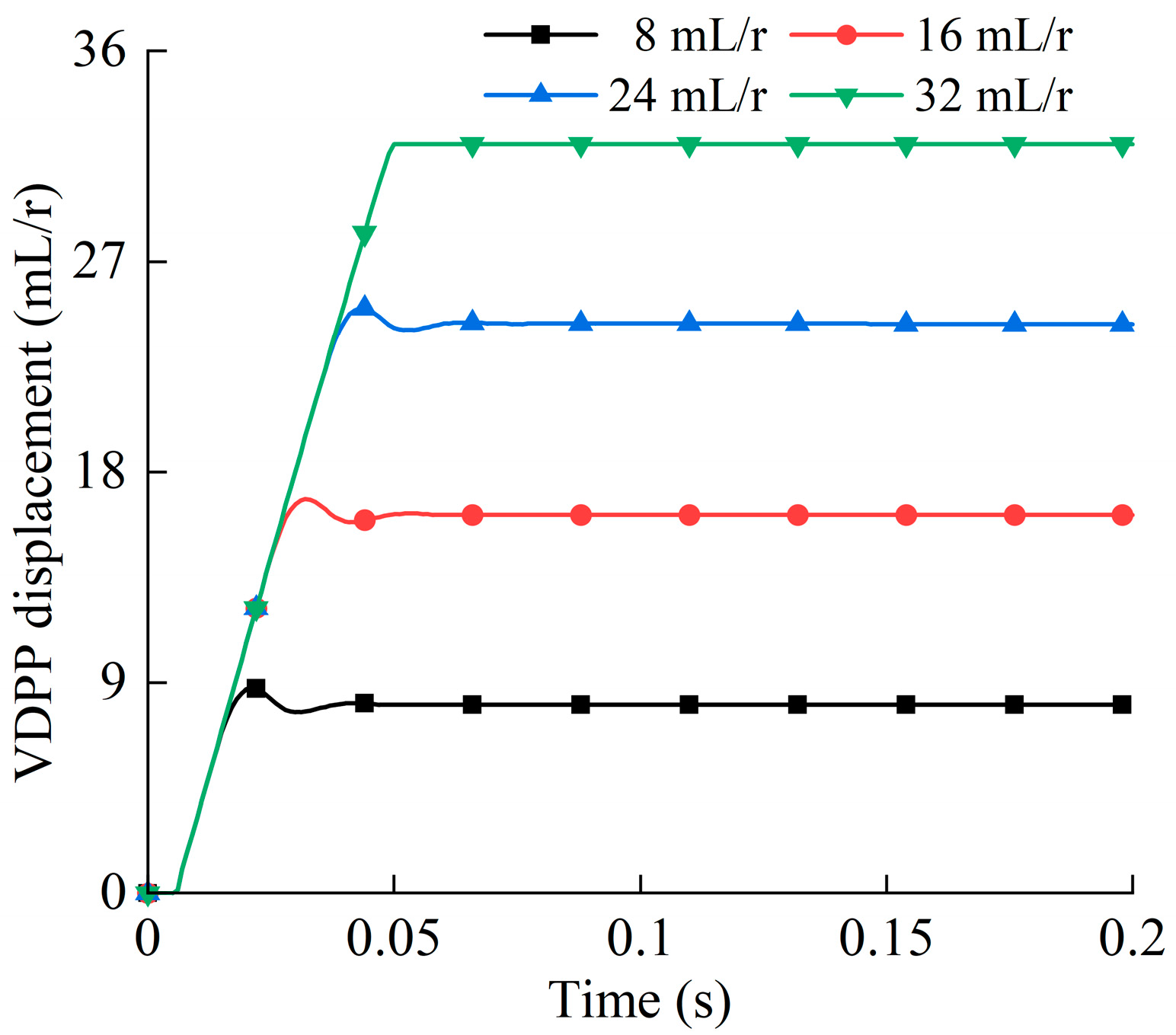
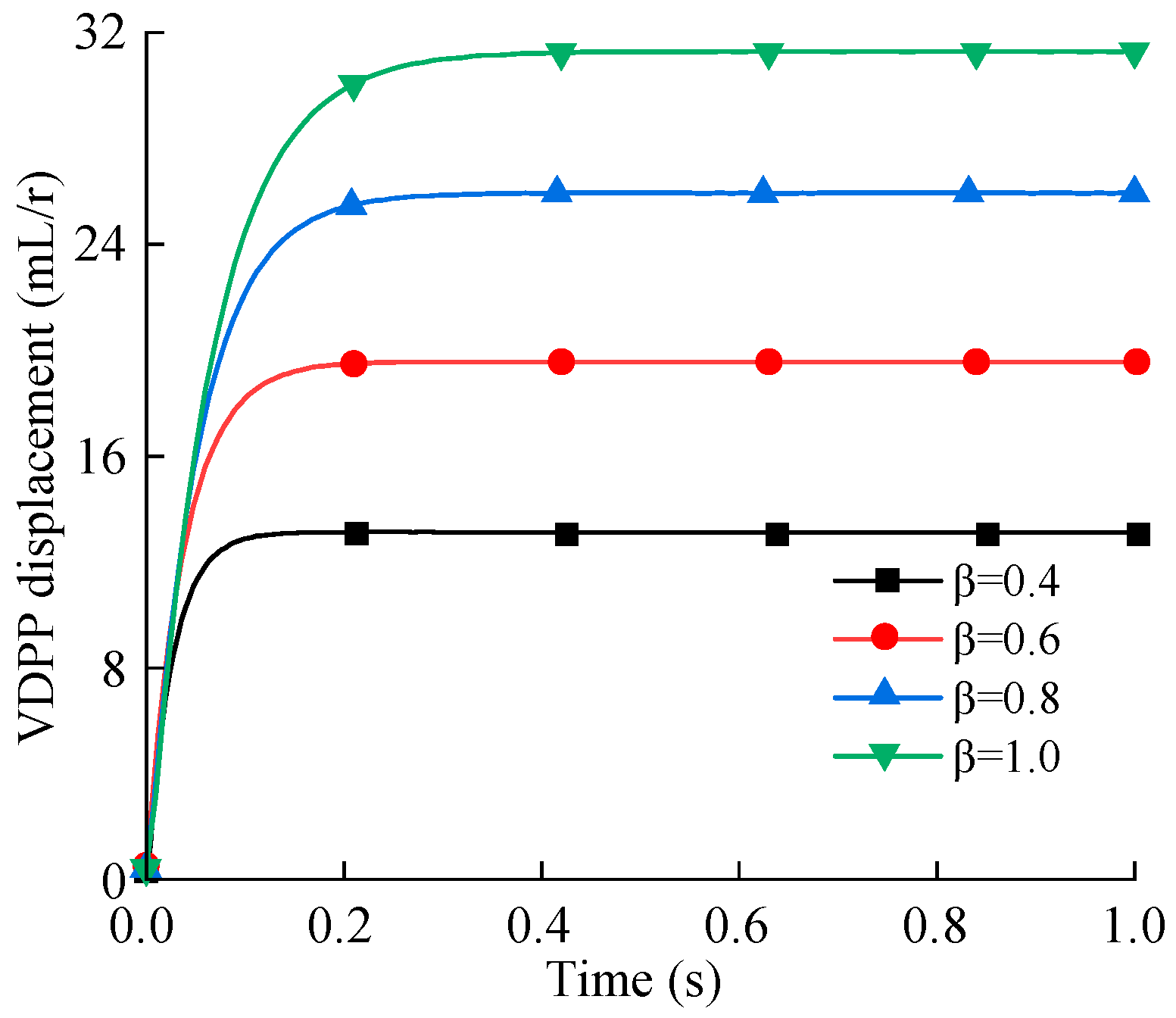
2.2. Dynamic Characteristics Analysis of the VDPP
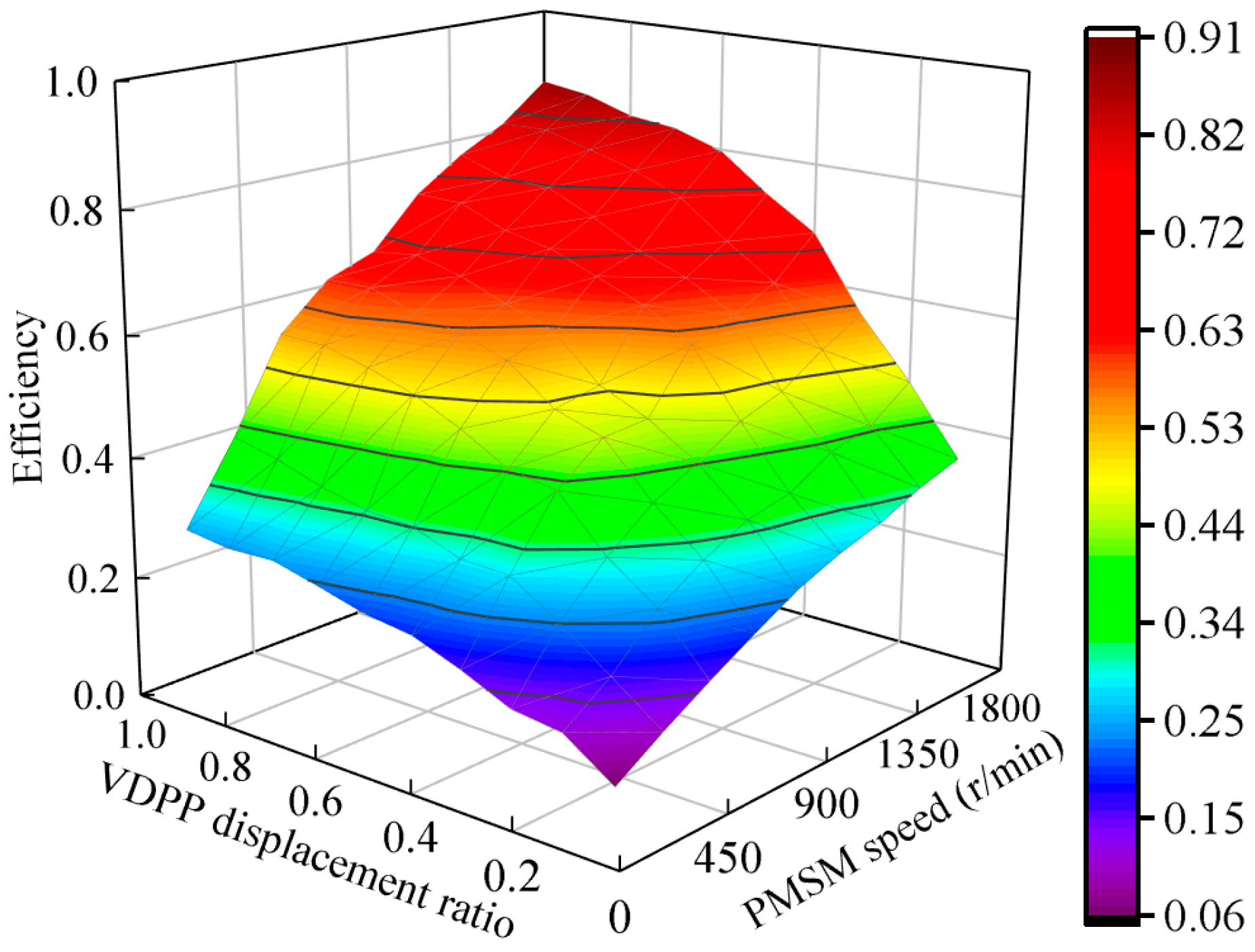
3. Energy Efficiency Characteristic Analysis of the VSVDPU
3.1. Energy Efficiency Characteristics Analysis of the PMSM
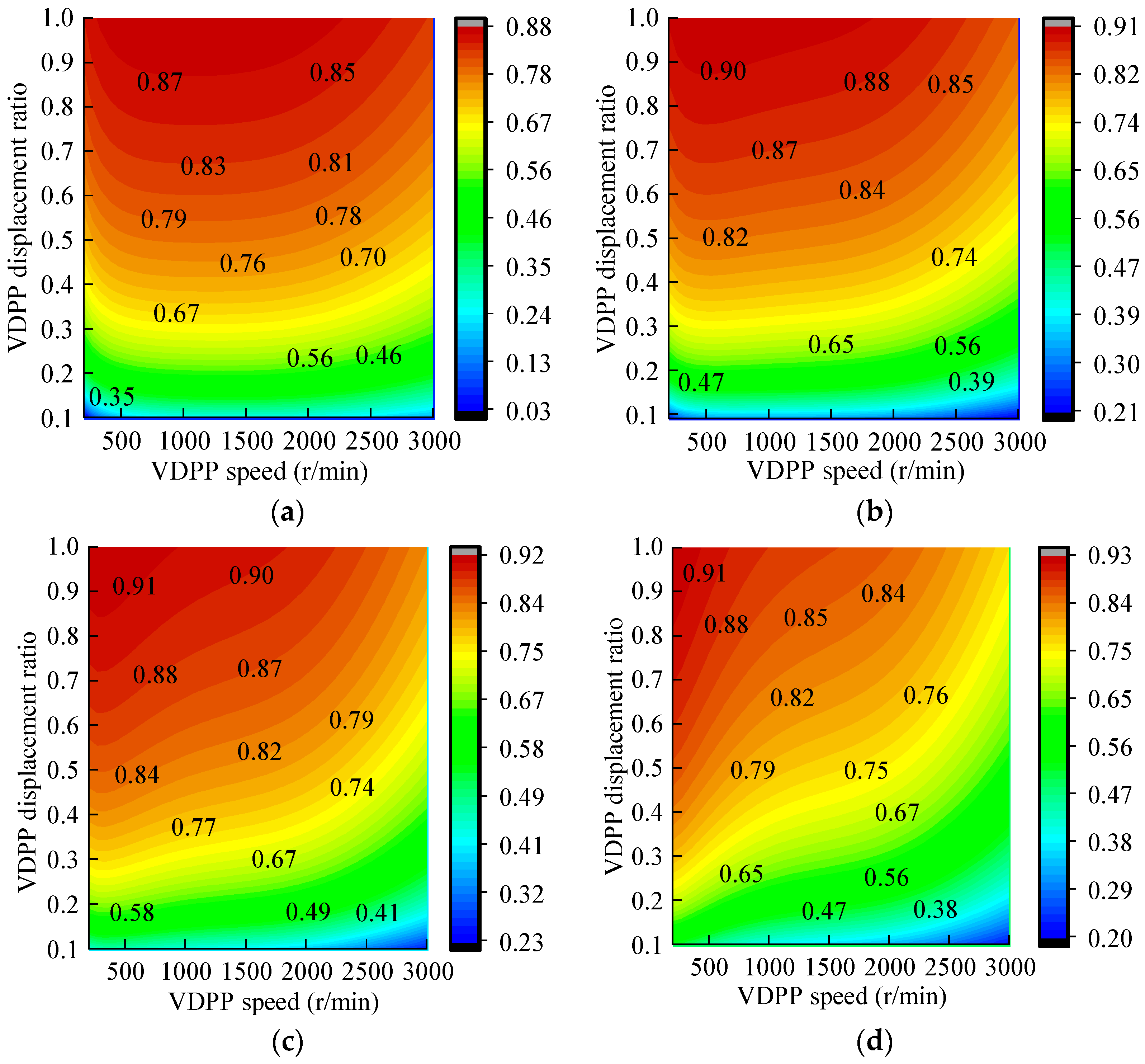
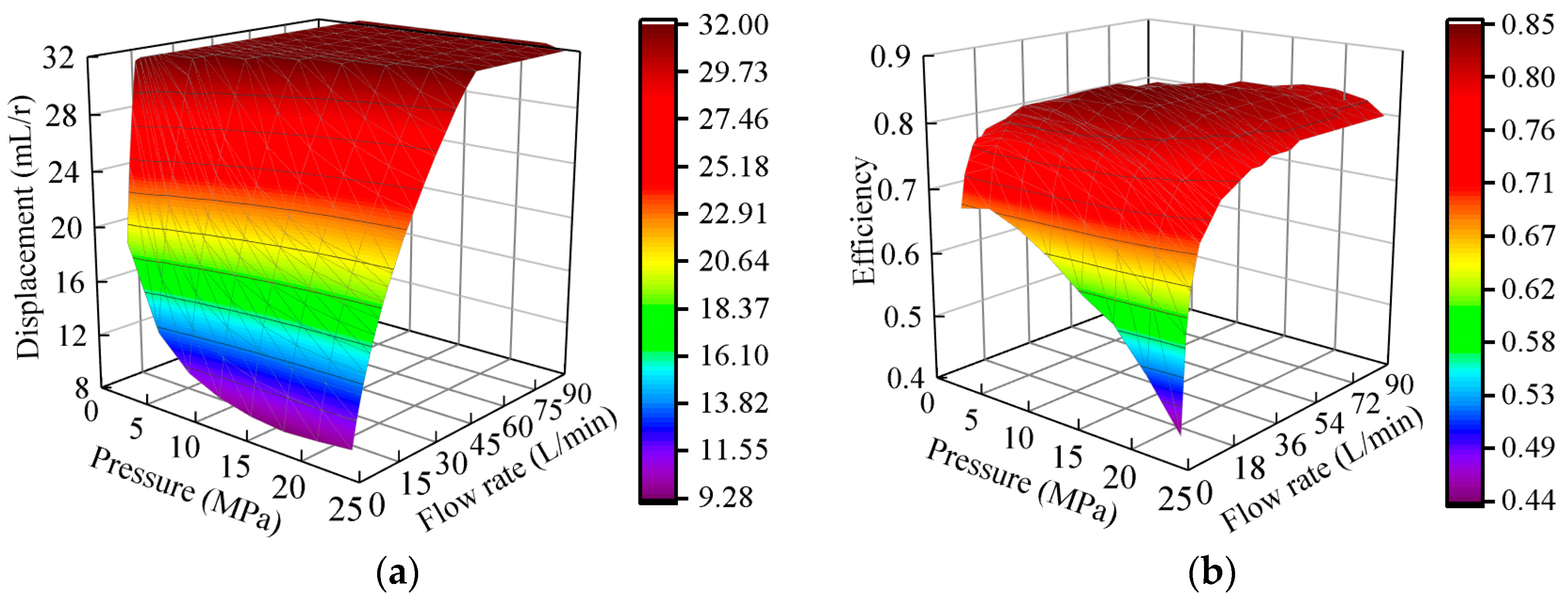
3.2. Energy Efficiency Characteristics Analysis of the VDPP
4. Optimized Matching Control of the VSVDPU Speed and Displacement
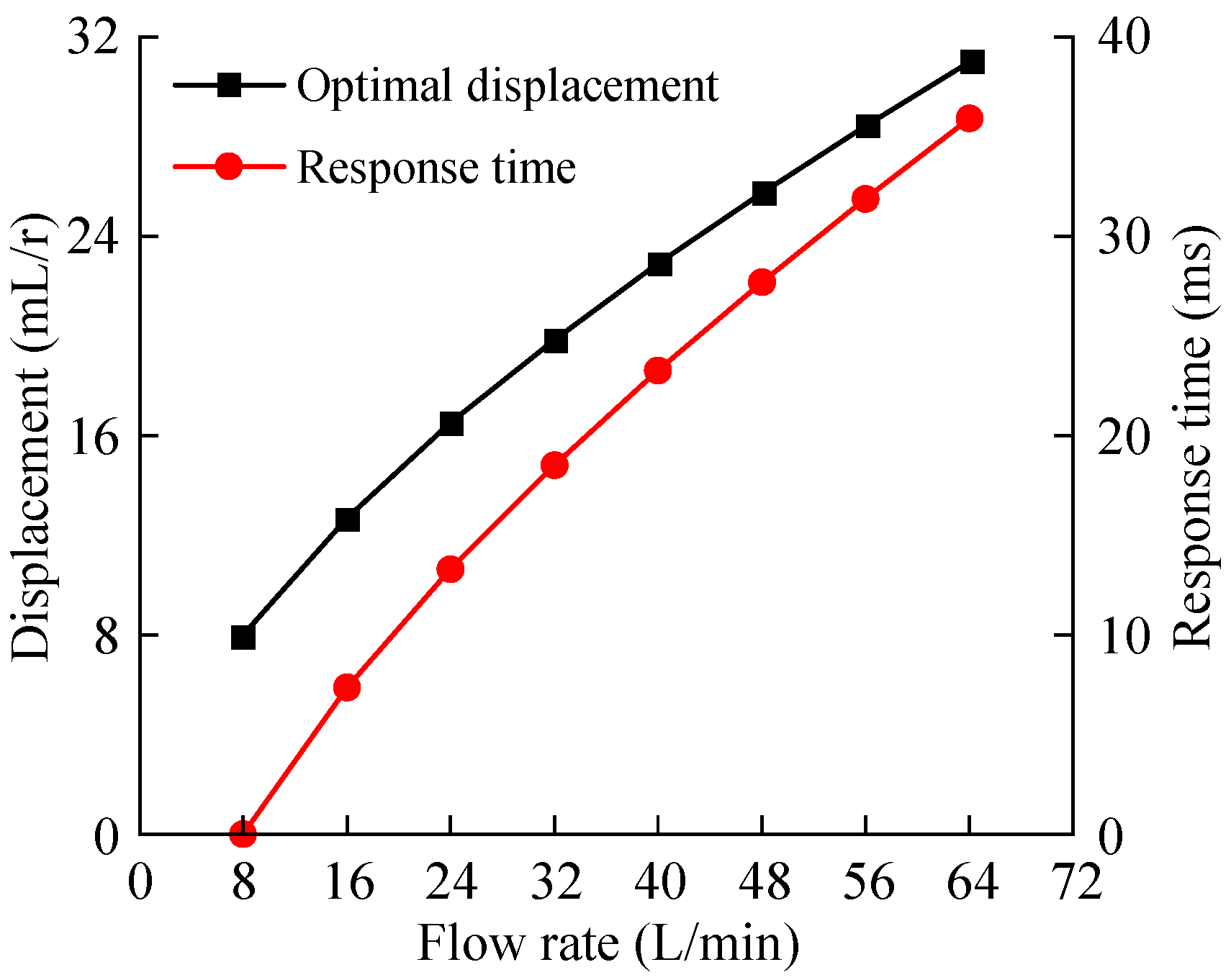
4.1. High Dynamic Optimization Matching
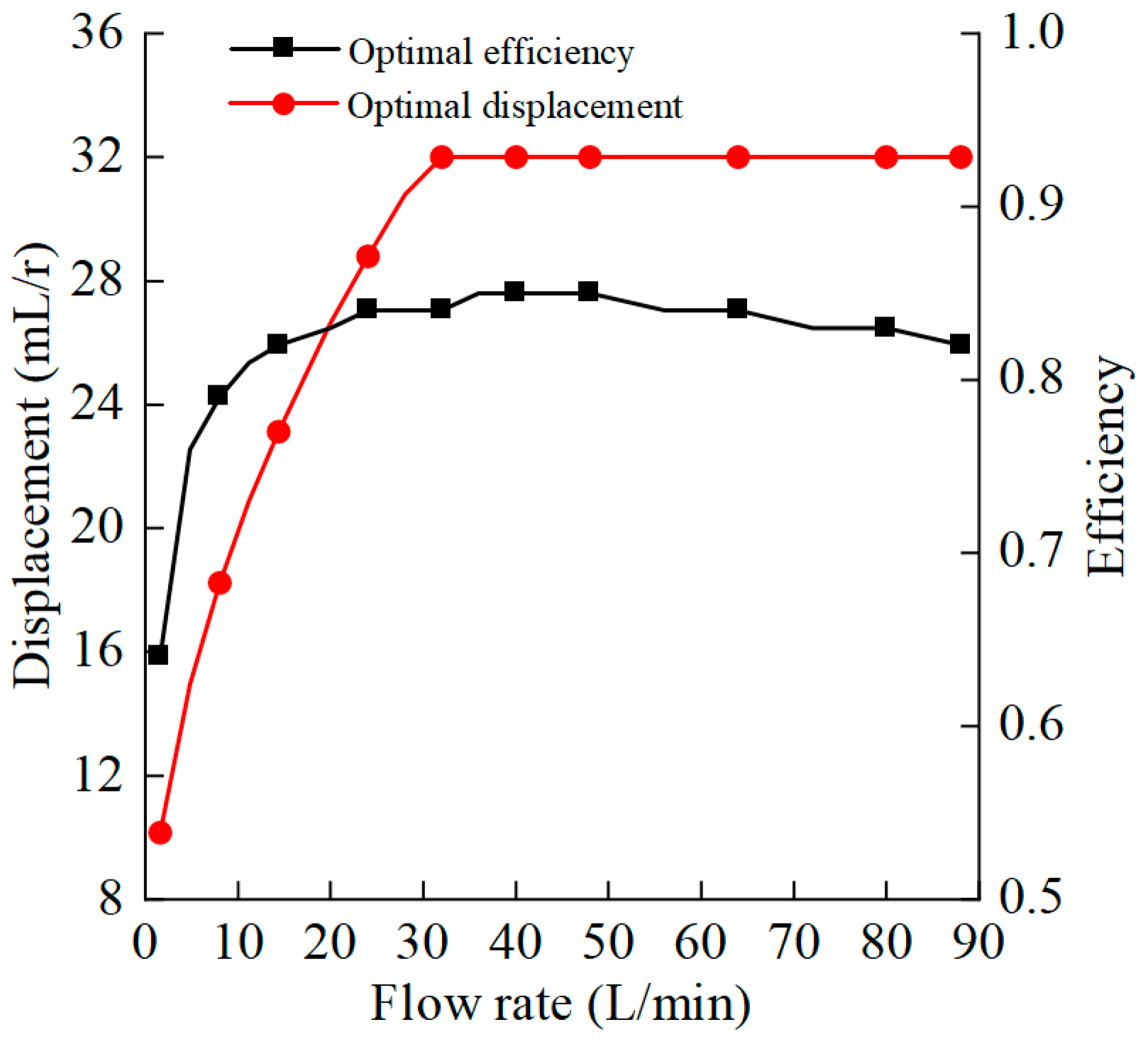
4.2. High Efficiency Optimization Matching
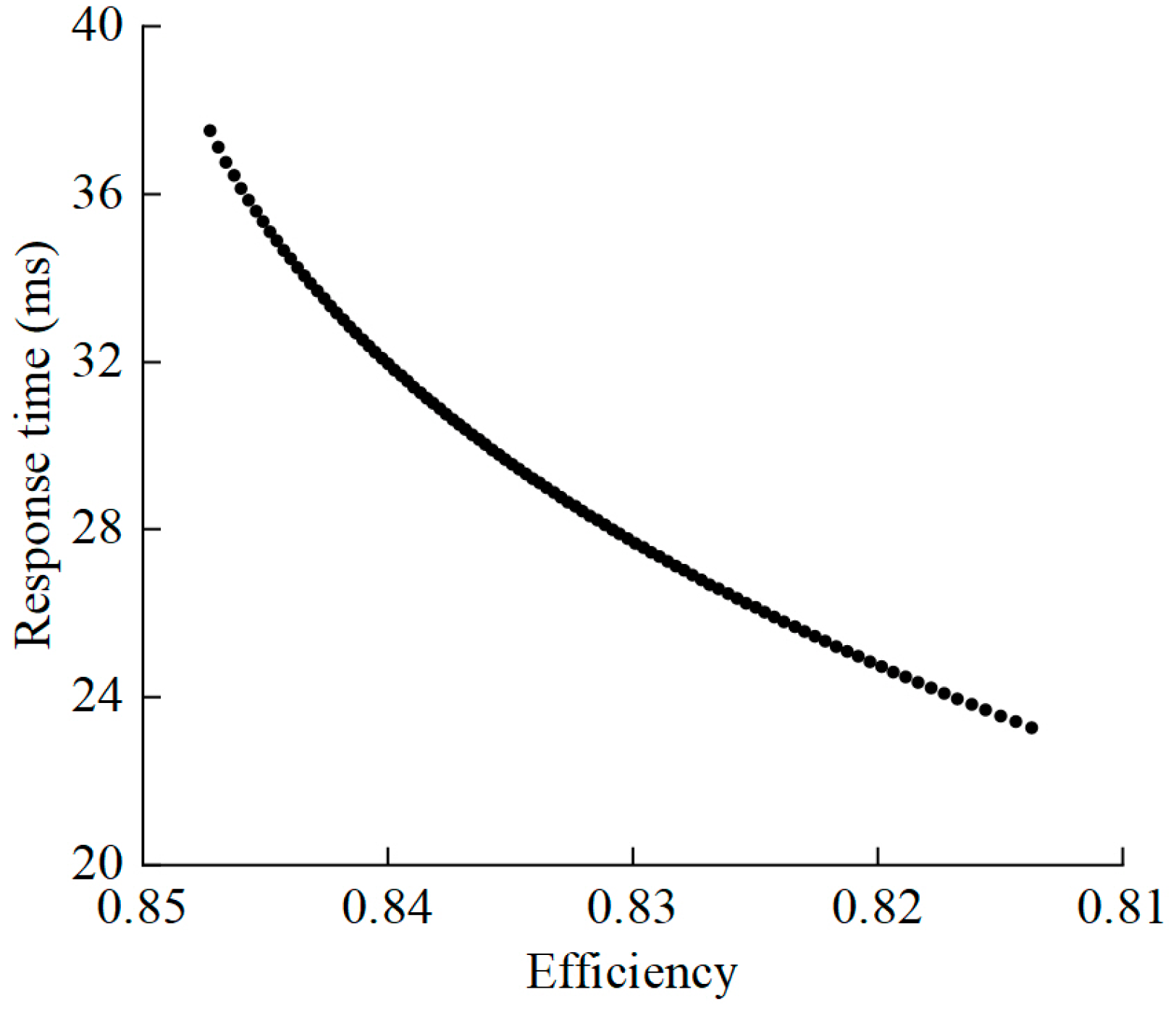
4.3. High Efficiency and Dynamic Multi-Objective Optimization Matching
5. Experimental Study of the VSVDPU
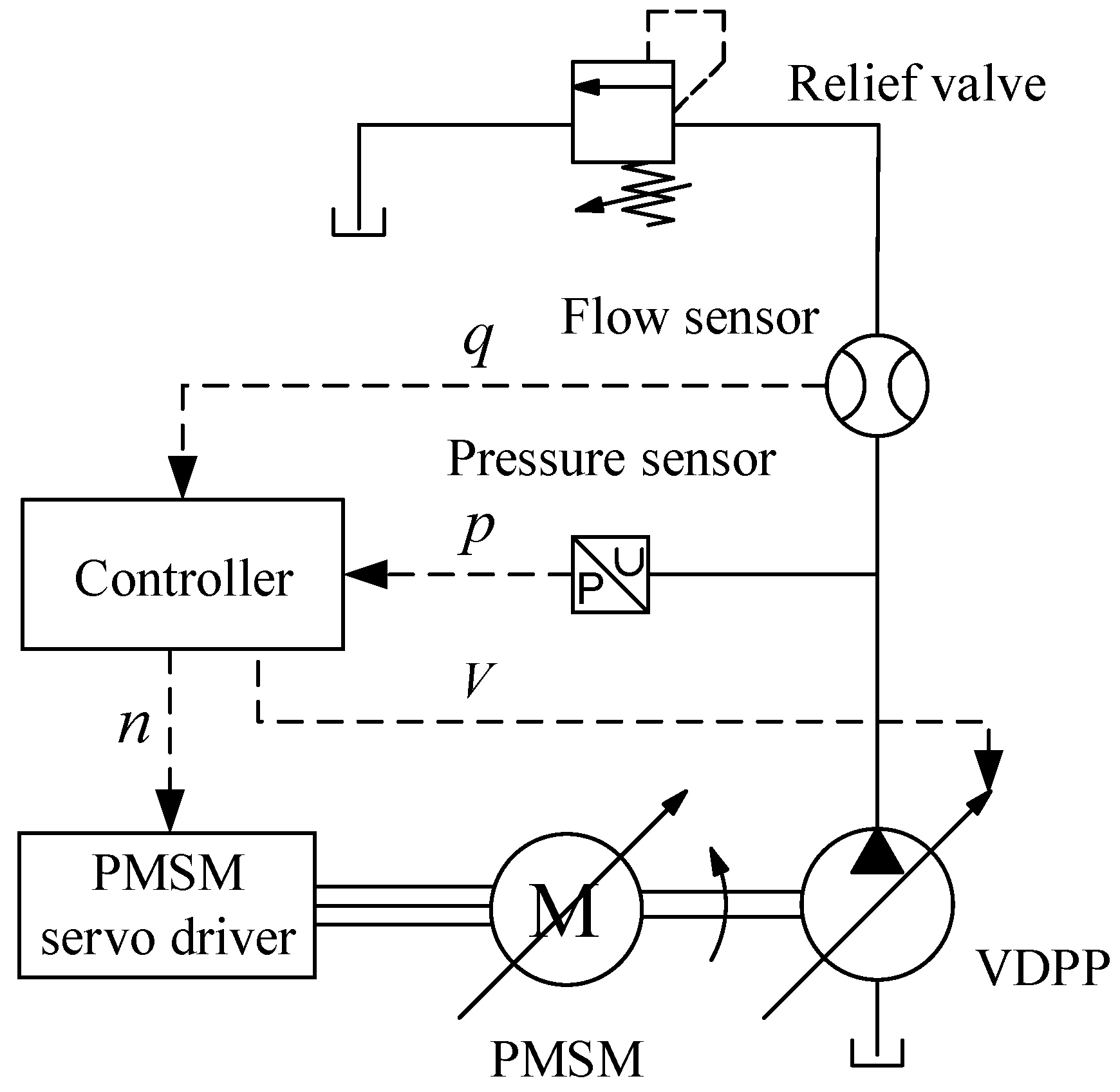
5.1. Construction of the VSVDPU Experimental Platform
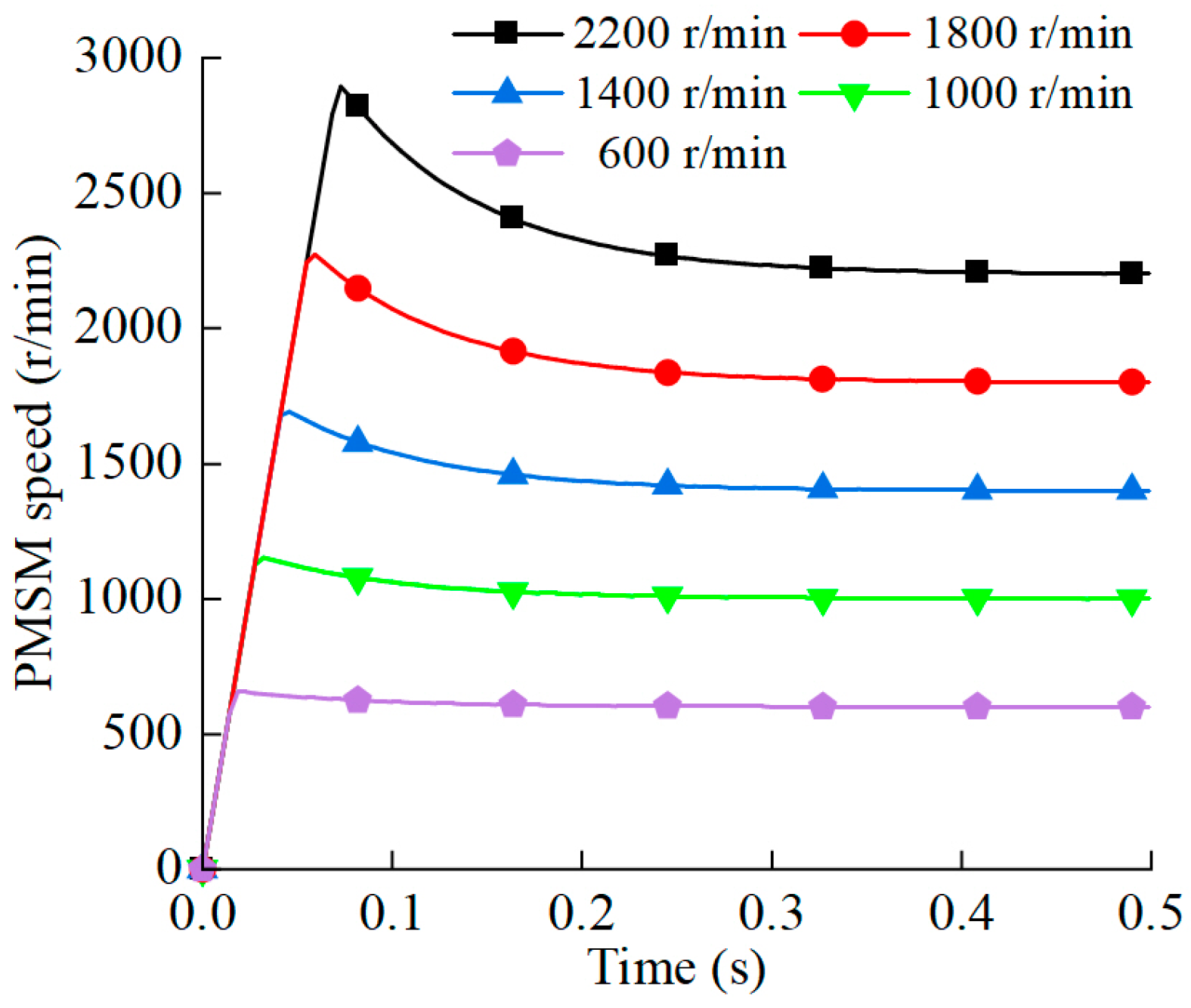
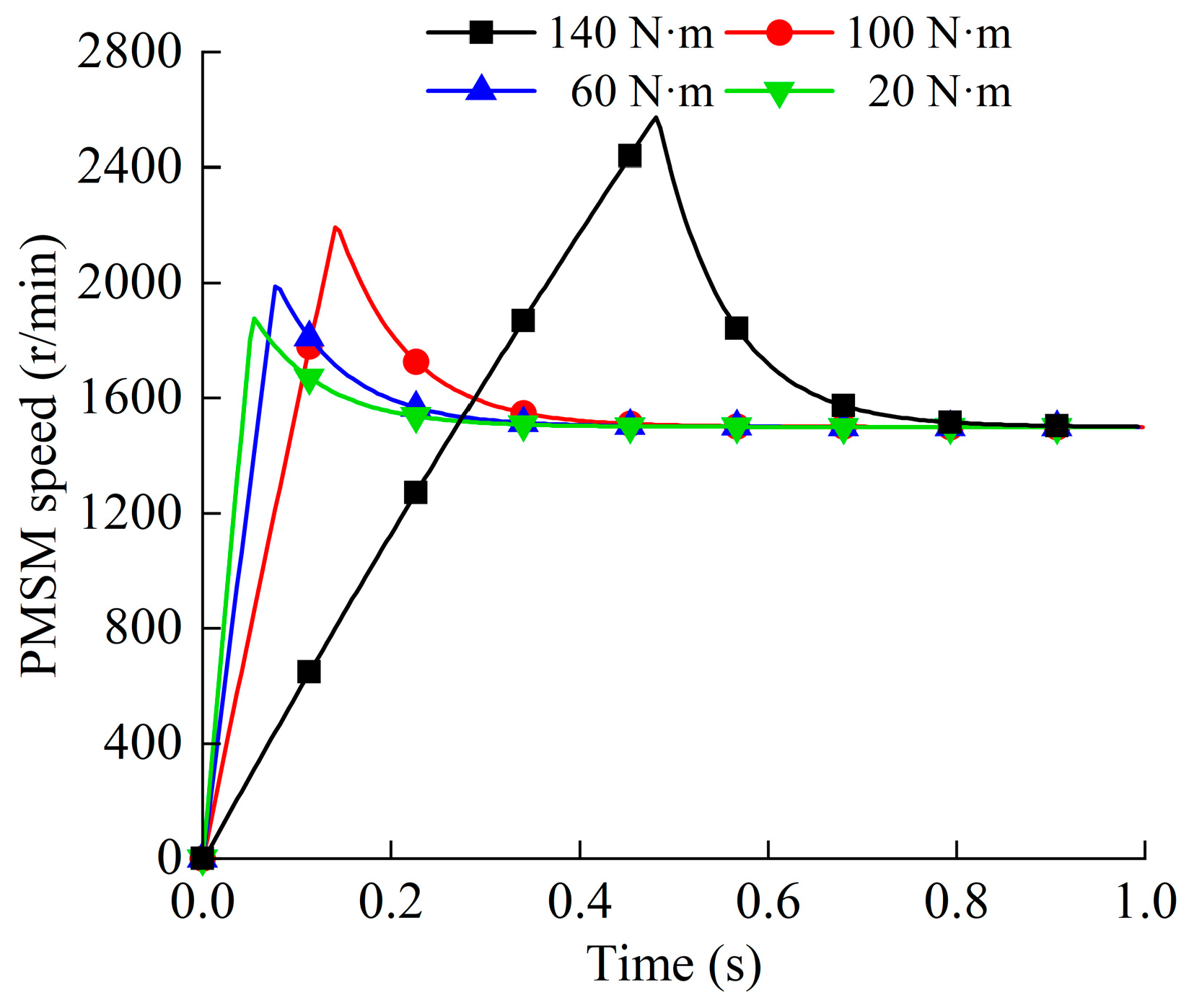
5.2. Analysis of the VSVDPU Experimental Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yan, G.; Jin, Z.; Yang, M.; Yao, B. The thermal balance temperature field of the electro-hydraulic servo pump control system. Energies 2021, 14, 1364. [Google Scholar] [CrossRef]
- Vacca, A. Energy efficiency and controllability of fluid power systems. Energies 2018, 11, 1169. [Google Scholar] [CrossRef]
- Li, Z.; Shang, Y.; Jiao, Z.; Lin, Y.; Wu, S.; Li, X. Analysis of the dynamic performance of an electro-hydrostatic actuator and improvement methods. Chin. J. Aeronaut. 2018, 31, 2312–2320. [Google Scholar] [CrossRef]
- Yu, B.; Wu, S.; Jiao, Z.; Shang, Y. Multi-Objective Optimization Design of an Electrohydrostatic Actuator Based on a Particle Swarm Optimization Algorithm and an Analytic Hierarchy Process. Energies 2018, 11, 2426. [Google Scholar] [CrossRef]
- Yan, G.; Jin, Z.; Zhang, T.; Zhao, P. Position control study on pump-controlled servomotor for steam control valve. Processes 2021, 9, 221. [Google Scholar] [CrossRef]
- Nguyen, M.T.; Dang, T.D.; Ahn, K.K. Application of Electro-Hydraulic Actuator System to Control Continuously Variable Transmission in Wind Energy Converter. Energies 2019, 12, 2499. [Google Scholar] [CrossRef]
- Quan, Z.; Quan, L.; Zhang, J. Review of energy efficient direct pump controlled cylinder electro-hydraulic technology. Renew. Sustain. Energy Rev. 2014, 35, 336–346. [Google Scholar] [CrossRef]
- Qu, S.; Fassbender, D.; Vacca, A.; Busquets, E. A high-efficient solution for electro-hydraulic actuators with energy regeneration capability. Energy 2021, 216, 119291. [Google Scholar] [CrossRef]
- Zhang, X.; Huang, W.; Ge, L.; Quan, L. Research on response characteristics and energy efficiency of power unit used for electric driving mobile machine. IEEE Access 2019, 7, 125747–125753. [Google Scholar] [CrossRef]
- Lovrec, D.; Tic, V.; Tasner, T. Simulation-aided determination of an efficiency field as a basis for maximum efficiency-controller design. Int. J. Simul. Model. 2015, 14, 669–682. [Google Scholar] [CrossRef]
- Schmidt, L.; Hansen, K.V. Electro-Hydraulic Variable-Speed Drive Networks—Idea, Perspectives, and Energy Saving Potentials. Energies 2022, 15, 1228. [Google Scholar] [CrossRef]
- Brecher, C.; Jasper, D.; Fey, M. Analysis of new, energy-efficient hydraulic unit for machine tools. Int. J. Precis. Eng. Manuf.-Green Technol. 2017, 4, 5–11. [Google Scholar] [CrossRef]
- Huang, L.; Yu, T.; Jiao, Z.; Li, Y. Research on power matching and energy optimal control of active load-sensitive electro-hydrostatic actuator. IEEE Access 2020, 9, 51121–51133. [Google Scholar] [CrossRef]
- Huang, J.; Yan, Z.; Quan, L.; Lan, Y.; Gao, Y. Characteristics of delivery pressure in the axial piston pump with combination of variable displacement and variable speed. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2015, 229, 599–613. [Google Scholar] [CrossRef]
- Han, X.; Zhang, P.; Minav, T.; Fu, Y.; Fu, J. A Modeling and Simulation Method for Preliminary Design of an Electro-Variable Displacement Pump. J. Vis. Exp. 2022, 184, e63593. [Google Scholar]
- Cecchin, L.; Frey, J.; Gering, S.; Manderla, M.; Trachte, A.; Diehl, M. Nonlinear Model Predictive Control for Efficient Control of Variable Speed Variable Displacement Pumps. IFAC-PapersOnLine 2023, 56, 331–336. [Google Scholar] [CrossRef]
- Willkomm, J.; Wahler, M.; Weber, J. Process-adapted control to maximize dynamics of speed-and displacement-variable pumps. In Proceedings of the ASME/BATH 2014 Symposium on Fluid Power and Motion Control, Bath, UK, 10–12 September 2014; p. V001T01A015. [Google Scholar]
- Jin, R.; Huang, H.; Li, L.; Zuo, H.; Gan, L.; GE, S.; Liu, Z. Artificial Intelligence Enabled Energy-Saving Drive Unit with Speed and Displacement Variable Pumps for Electro-Hydraulic Systems. IEEE Trans. Autom. Sci. Eng. 2023, 99, 1–12. [Google Scholar] [CrossRef]
- Zhang, Y.; Fu, Y.; Zhou, W. Optimal control for EHA-VPVM system based on feedback linearization theory. In Proceedings of the 2010 11th International Conference on Control Automation Robotics & Vision, Singapore, 7–10 December 2010; pp. 744–749. [Google Scholar]
- Malrait, F.; Ejjabraoui, K. Power conversion optimization for hydraulic systems controlled by variable speed drives. J. Process Control 2019, 74, 133–146. [Google Scholar] [CrossRef]
- Kim, H.; Yoo, S.; Cho, S.; Yi, K. Hybrid control algorithm for fuel consumption of a compound hybrid excavator. Automation in Construction 2016, 68, 1–10. [Google Scholar] [CrossRef]
- Assaf, H.; Sarode, S.; Vacca, A.; Sudhoff, S.D. Electric machine sizing consideration for ePumps in mobile hydraulics. Energy Sci. Eng. 2024, 12, 793–809. [Google Scholar] [CrossRef]
- Brecher, C.; Fey, M.; Brockmann, B.; Chavan, P. Multivariable control of active vibration compensation modules of a portal milling machine. J. Vib. Control 2018, 24, 3–17. [Google Scholar] [CrossRef]
- Huang, H.; Jin, R.; Li, L.; Liu, Z. Improving the energy efficiency of a hydraulic press via variable-speed variable-displacement pump unit. J. Dyn. Syst. Meas. Control 2018, 140, 111006. [Google Scholar] [CrossRef]
- Yan, Z.; Ge, L.; Quan, L. Energy-Efficient Electro-Hydraulic Power Source Driven by Variable-Speed Motor. Energies 2022, 15, 4804. [Google Scholar] [CrossRef]






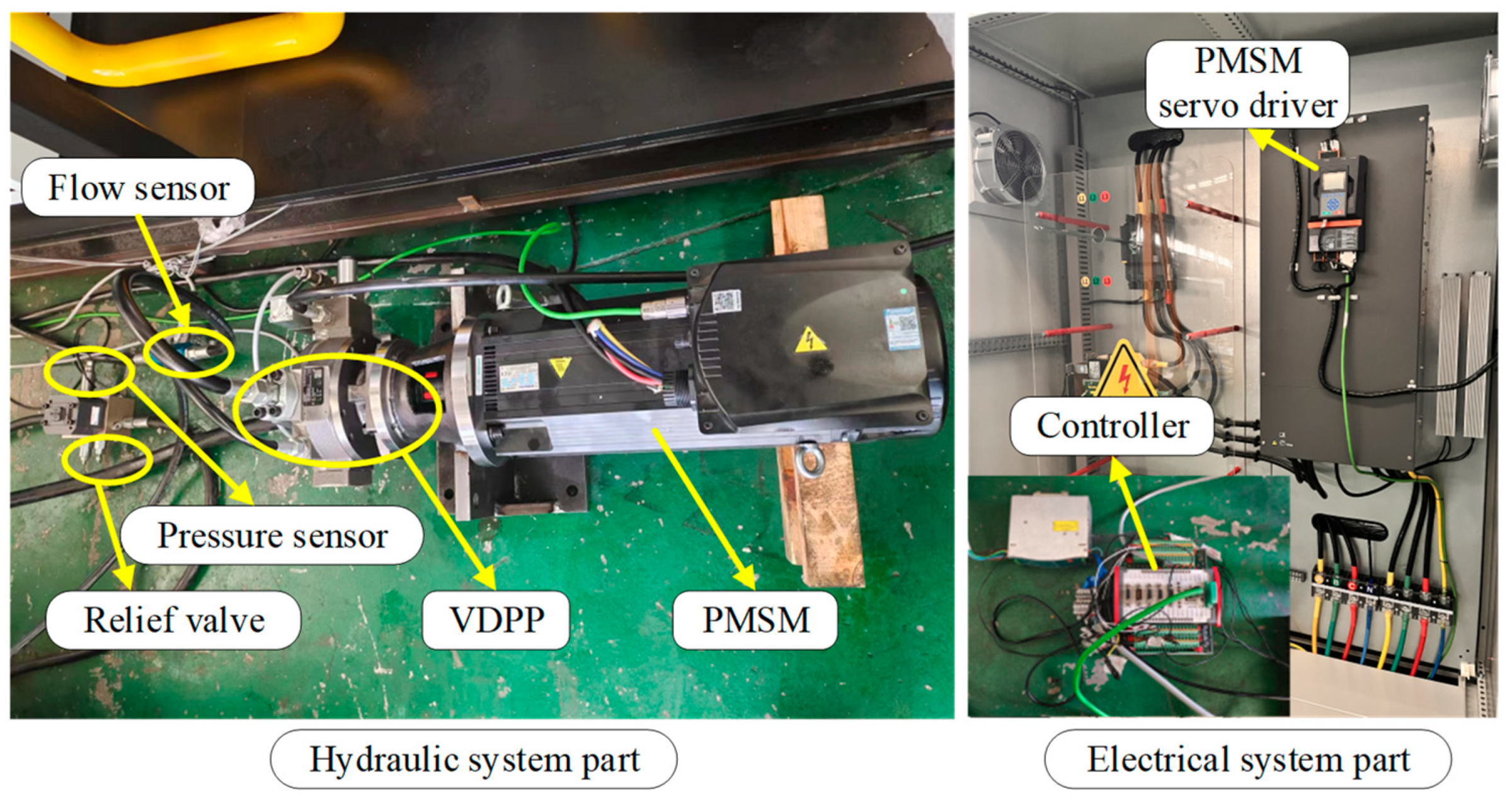


| Physical Parameter | Value of Number | Physical Unit |
| Nominal voltage | 380 | V |
| The d-axis inductance | 0.84 | mH |
| The q-axis inductance | 2.72 | mH |
| Stator phase resistance | 0.1098 | Ω |
| The number of poles of the PMSM | 4 | \ |
| The permanent magnet flux linkage | 0.185 | Wb |
| The rotor moment of inertia | 0.0375 | kg∙m2 |
| The damping coefficient | 0.015 | N/(m/s) |
| The gas density | 1.29 | kg/m3 |
| The rotor radius | 0.0345 | m |
| The rotor axial length | 0.34 | m |
| Physical Parameter | Value of Number | Physical Unit |
| The VDPP maximum displacement | 32 | mL/r |
| Plunger diameter | 1.2 × 10−2 | m |
| The number of plungers | 9 | \ |
| The oil bulk elastic modulus | 1.5 × 10−9 | N/m2 |
| The oil dynamic viscosity | 2.61 × 10−2 | Pa∙s |
| The variable mechanism input control voltage | ±10 | V |
| The effective area of the hydraulic cylinder piston | 2.2 × 10−4 | m2 |
| The control chamber volume | 1.5 × 10−5 | m3 |
| Physical Parameter | Value of Number | Physical Unit |
|---|---|---|
| The PMSM Rated speed | 1800 | r/min |
| The PMSM rated torque | 220.5 | N·m |
| The PMSM rated power | 41.6 | kW |
| The VDPP maximum displacement | 32 | mL/r |
| The VDPP maximum pressure | 35 | MPa |
| The VDPP maximum speed | 1800 | r/min |
| Pressure sensor range | 0–40 | MPa |
| Flow sensor range | 2–75 | L/min |
| Pressure regulation range of relief valve | 0–35 | MPa |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, M.; Liu, X.; Yan, G.; Ai, C.; Yu, C. Research on Variable Speed Variable Displacement Power Unit with High Efficiency and High Dynamic Optimized Matching. Energies 2024, 17, 3322. https://doi.org/10.3390/en17133322
Yang M, Liu X, Yan G, Ai C, Yu C. Research on Variable Speed Variable Displacement Power Unit with High Efficiency and High Dynamic Optimized Matching. Energies. 2024; 17(13):3322. https://doi.org/10.3390/en17133322
Chicago/Turabian StyleYang, Mingkun, Xianhang Liu, Guishan Yan, Chao Ai, and Cong Yu. 2024. "Research on Variable Speed Variable Displacement Power Unit with High Efficiency and High Dynamic Optimized Matching" Energies 17, no. 13: 3322. https://doi.org/10.3390/en17133322
APA StyleYang, M., Liu, X., Yan, G., Ai, C., & Yu, C. (2024). Research on Variable Speed Variable Displacement Power Unit with High Efficiency and High Dynamic Optimized Matching. Energies, 17(13), 3322. https://doi.org/10.3390/en17133322






