1. Introduction
In Germany, fossil fuels (e.g., natural gas, crude oil) are mainly used to heat buildings and industry. According to the Renewable Energies Heat Act [
1], the switch to renewable energy sources is mandatory for the installation of new heating systems from 1 January 2024, with the proportion of renewable energy sources exceeding 65 percent. By 2045, buildings should no longer use fossil fuels in order to achieve carbon neutrality. Besides, the latest version of Germany’s Renewable Energy Act [
2] stipulates that at least 80% of Germany’s electricity consumption will be covered by renewable energy sources by 2030 and will be free of greenhouse gases by 2035. However, fossil fuels are currently the main source of heat for around 41 million households, i.e., for more than half of existing households (
www.bmwk.de, accessed on 23 March 2024).
Given Germany’s current energy policy and the available renewable energy technologies, geothermal energy is recognized as an important clean energy alternative to fossil energy. Depending on the depth of exploitation, geothermal energy can be categorized as shallow geothermal energy (0–400 m), medium deep geothermal energy (400 m–1 km), and deep geothermal energy (>1 km). Distributed shallow geothermal systems are mostly used for small residential and commercial buildings (e.g., hospitals, banks, shopping malls, etc.). Shallow geothermal systems exist in various forms: shallow thermal collectors (i.e., horizontal ground heat exchangers), geothermal baskets, borehole exchangers, and so on. The heat exchangers can be of various shapes, e.g., the pipes can be distributed in U-shaped [
3], spiral, slinky, and stacked arrangements [
4,
5,
6]. In the past, the shape and arrangement of heat exchangers have been intensively studied for their thermal performance and economic efficiency [
7,
8,
9]. At the same time, novel backfill materials [
10,
11] and circulation fluids [
12] have been developed to improve the efficiency of the entire geothermal system. In addition, the efficiency of heat pumps is continuously being improved in recent years. Moreover, the size of heat pumps is being further reduced, increasing the possibility of decentralization of district heating networks.
In addition to geothermal energy, the installation of solar panels has exploded in Germany over the past decade due to sufficient capacity and relatively low costs. Whether distributed photovoltaic (PV) systems or solar parks, they provide electricity as well as heat. In the period 2013–2018, the average capacity of installed PV systems was only 1.9 GWp per year, whereas in 2023 it reached approximately 14 GWp, with a cumulative capacity of 81 GWp (source: Fraunhofer ISE). The annual generation of electricity by PV in 2023 was about 61.1 TWh, covering ca. 12% of Germany’s electricity consumption. However, the use of solar energy in Germany is severely limited by the local climate, especially in the northern part. The most abundant solar energy is concentrated in the summer months.
Another increasing renewable energy source is wind power. The share of wind energy in Germany’s renewable energy mix is increasing every year, from 9.5 TWh in 2000 to 139.8 TWh in 2023 (source: Fraunhofer ISE). By the end of 2023, the cumulative installed capacity of onshore wind turbines across Germany reached 61.01 GW, while the installed capacity of offshore wind turbines reached 8.5 GW. State Schleswig-Holstein leads the country in terms of installed capacity because it has a considerably larger wind potential than the other states in Germany (source:
globalwindatlas.info, accessed on 23 March 2024). Similarly, wind energy has the same problem of instability as solar energy and fluctuates with different locations and seasons, as well as the height of the building and its surrounding conditions.
Because of seasonal and daily fluctuations of solar and wind energy, for small- to large-scale applications, electricity generated by solar radiation or wind power is often combined with a battery bank or converted to other forms of energy for storage (e.g., hot water, compressed air [
13,
14,
15]). Specifically, hot water is stored in the form of borehole or aquifer thermal energy storage (BTES [
16,
17,
18] or ATES [
19,
20]). The main principle is that the excess solar or wind energy generated during the high production time is stored underground in the form of heat, which is then extracted for further use during the low production time. The scale of storage depends on the installed capacity of the solar panels or wind turbines, the amount of surplus energy, and the purpose and frequency of the energy use. For example, the size, mode, and time scale of storage for generating electricity versus providing thermal energy can be significantly different.
Combining these three different renewable energies to supply electricity and heat is a trend in Europe as well as in Germany. The German government encourages the use of renewable energies and decentralization of energy systems for economic and environmental reasons. Starting in 2023, many small and medium-sized energy transition projects in German cities and communities mainly focus on the feasibility study and planning of decentralization and the use of different clean energy sources on-site at different scales. The focus of the analysis and planning is on generating a specific energy concept to guide the building renovation and energy transition based on information about the building’s energy consumption and the local renewable energy potential. In our experience, the generation of this energy concept relies heavily on empirical calculations, scenario-based analysis, and field research data, which can vary from place to place and evolve over time. Currently, there is a lack of a mature and comprehensive model that complements and calibrates the energy concept over time, especially in the context of local economies and policies, as well as the changing climate. An integrated economic model that can be adjusted, updated, and optimized in the long term is of great interest to end-users, energy companies, and energy management and decision-making bodies.
In this study, an economic model of renewable energy for detached houses combining solar, wind and geothermal energy is developed. In
Section 2, the materials and methods used in this paper are shown. In
Section 2.1 and
Section 2.2, the heat and electricity demand of two detached houses in two northern German cities, Hamburg and Sylt, respectively are assumed, and the use of solar, wind, and geothermal energy is proposed to maximize the fulfilment of these energy demands. In
Section 2.3, the annual electricity potential and geothermal potential of the house site from local meteorological and soil data are calculated, as well as the dimensioning of the solar panel, wind turbine, and ground source heat pump (GSHP) is calculated. The electricity generated by the PV and wind turbines will be used for heat pumps and other house appliances, thus reducing the dependence of the house on the local grid. In
Section 2.4, an economic model is generated to automatically calculate the installation and long-term operating costs of the different options, taking into account the price and inflation rates in Germany as well as the current subsidy policy.
Section 3 shows the results of the design of a hybrid energy system for two houses and compares the installation and long-term operating costs and the payback period under different energy supply scenarios. In
Section 4 and
Section 5, the findings and limitations of this study are discussed, and its potential application scenarios and suggestions for future study directions are given.
2. Materials and Methods
2.1. Energy Demand of Two Detached Houses
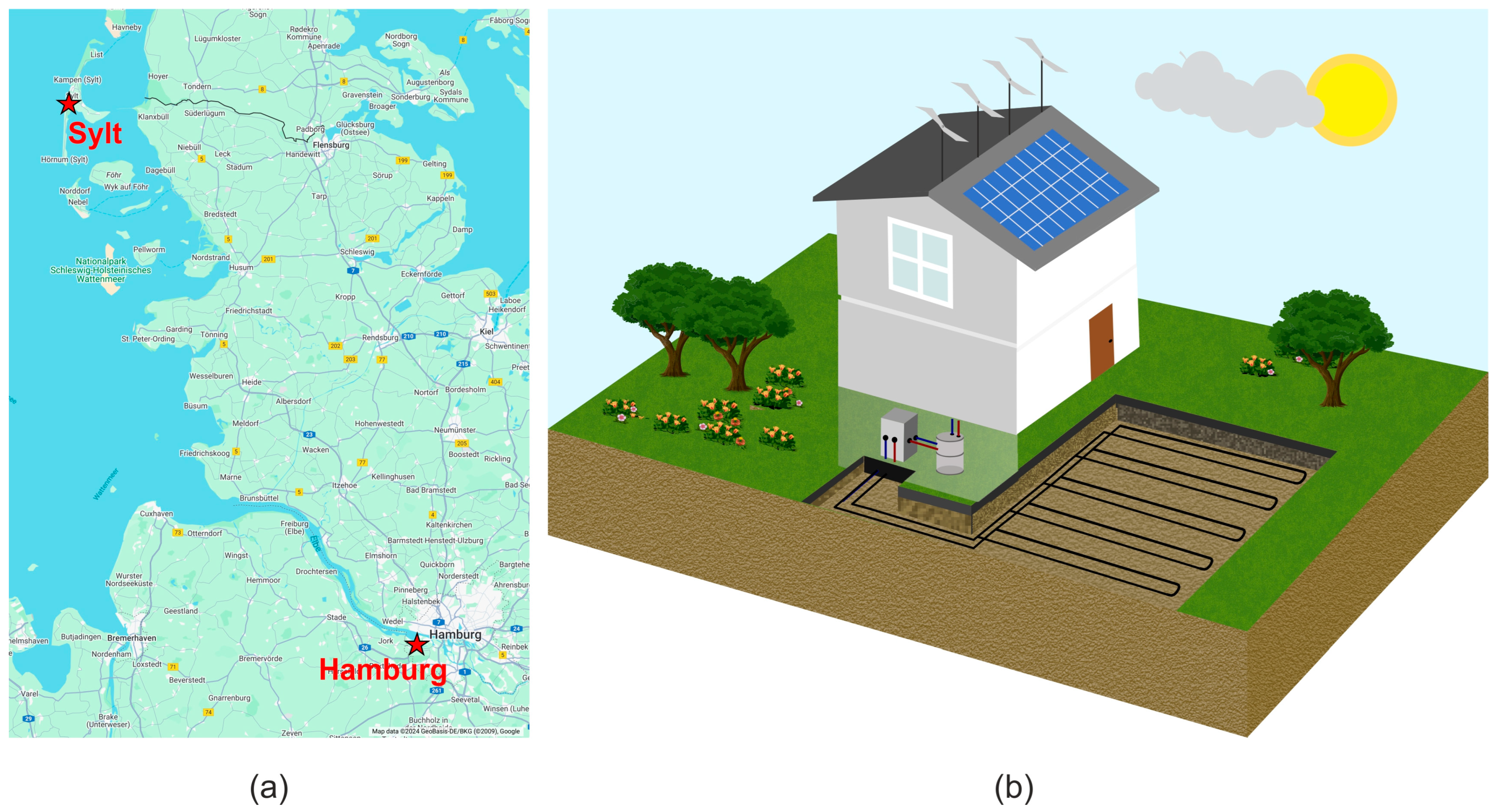
In the case study of this paper, it is assumed that two detached houses located in Hamburg and Sylt in northern Germany (
Figure 1a) have the same structure and energy class. Hamburg is the largest city in northern Germany, and it is representative of study houses in the Hamburg area. Whereas Sylt is an island in the north of Germany, and its meteorological data, especially local wind speed, may differ considerably from that of the urban area. The houses consist of two living floors and a basement (
Figure 1b). The length and width of each floor is 10 m × 9 m. Thus, the usable area of the house is 180 m
2. The roof area is about 110 m
2 (assuming a 30-degree roof slope). Both houses require heating throughout the winter and partly in spring and fall. In addition, the heating system provides domestic hot water. Assuming an energy efficiency class E for the houses according to the German energy efficiency classes (approximately 150 kWh/m
2/a), the heat demand (including floor heating and hot water) for a two-story house would be approximately 27,000 kWh/a. Of this amount, 21,006 kWh would be used for heating the house and 5994 kWh for domestic hot water.
Figure 2 and
Table 1 show the total space heating and domestic hot water demand for each month. The percentage of heat demand per month comes from the assumptions stated in VDI 4640, Part 2 [
21]. It is assumed that the domestic hot water usage is equal to 449.5 kWh per month. The three months with the highest demand are December, January and February, with heat demand of 3024, 3240 and 3105 kWh, respectively. All other months have a monthly heat demand of less than 2700 kWh. In the summertime, from June to August, heat is used only for domestic hot water. In addition, the electricity consumption of electrical appliances per household is 4300 kWh/a, assuming a household size of three or more persons (
www.destatis.de, accessed on 23 March 2024). The average monthly consumption of electricity is then about 390.9 kWh.
2.2. Solar-Wind Geothermal Coupled Renewable Energy System
The two houses now need to be designed with an energy system that will be powered by a variety of renewable energy sources. Heating is currently achieved by a gas boiler, which is planned to be replaced by a GSHP. Here, it is assumed that the heat pump achieves space heating through floor heating, where the heat-carrying fluid is at a lower temperature than ordinary radiators. The GSHP is installed in the basement and connected to the underfloor heating pipes. The other end of the heat pump is connected to an underground heat exchanger, which can take various forms. Here, three common forms are considered, including borehole heat exchangers (BHE), horizontal geothermal collectors, and geothermal baskets. On the roof of the house, solar panels are installed on the south side and some small wind turbines are fixed on the top of the roof. The entire configuration can be referred to in
Figure 1b.
The heat pump is assumed to be at a constant load. Heat extraction or discharge runs continuously until daily demand is met. The extracted heat is then stored in a storage tank, from which the heat is released when it is needed in the house and delivered to the building through the floor piping. It is assumed that the seasonal coefficient of performance (SCOP) of the heat pump for low-temperature floor heating will be 4.3, 3.9 and 4.1 for BHE-, collector- and basket-systems, respectively. For high-temperature domestic water, the SCOP of the heat pump will be 2.5. Therefore, the electricity consumption of the heat pump can be calculated based on the heat demand and the SCOP. The electricity used to drive the heat pump, circulation pump, and other household appliances will be supplied by PV and/or wind turbines installed on the roof of the house. During the low production season, electricity will be supplied from the local grid. This indicates that the house has a control panel that automatically switches between electricity sources. The capacity of the solar panels and wind turbines depends on the available area of the roof of the house.
2.3. Local Climate and Geological Data Used for Evaluating the Electricity and Geothermal Potential
To achieve the available electricity generated by PV and wind turbines, the peak sunshine hours and the wind speed for both sites are required, which are available online from the German Weather Service (
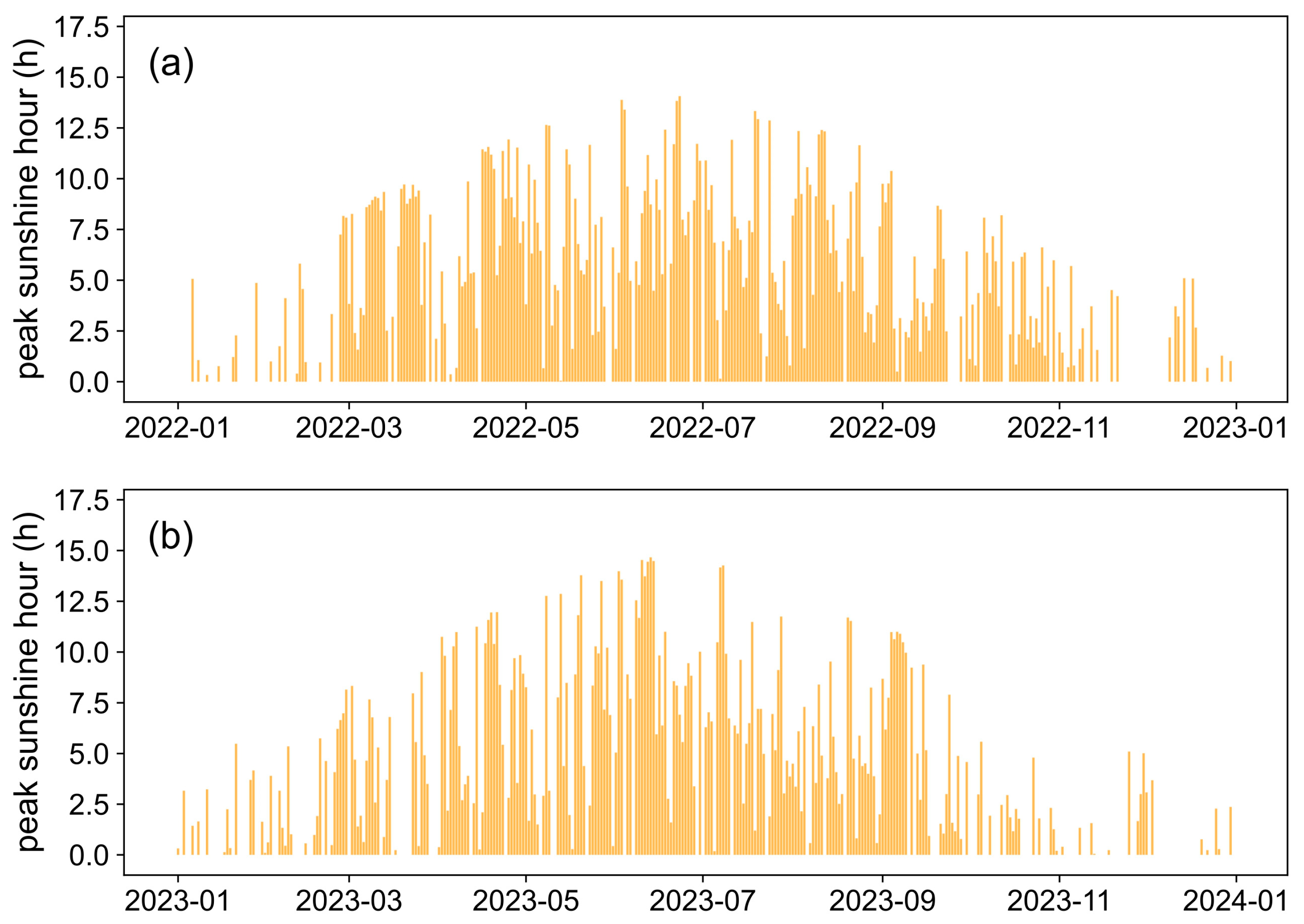
www.dwd.de, accessed on 23 March 2024). The meteorological data for the whole year from two weather stations, Hamburg-Fuhlsbüttel and Sylt, are used to estimate the potential of solar or wind energy to be converted into electricity. For data completion reasons, the meteorological data for the Hamburg-Fuhlsbüttel area are for the year 2022, while the meteorological data for Sylt are for the year 2023.
Raw data on sunshine hours and wind speeds at 10 m above ground level (m bgl) are given in hours. For simplicity, sunshine hours were averaged into daily data and then peak sunshine hours were calculated.
Figure 3 and
Figure 4 present the daily peak sunshine hours and hourly wind speeds. The sunny days and the high number of peak sunshine hours in both locations are mainly from April to September, and the peak daily sunshine hours are less than 15 h in both locations. Overall, Hamburg-Fuhlsbüttel has more sunny days than Sylt. The maximum peak sunshine hours in Hamburg and Sylt are 14.1 and 14.7, respectively. On the contrary, wind speeds do not vary much throughout the year. However, wind speeds are much higher in Sylt than in Hamburg-Fuhlsbüttel because Sylt is an island, whereas Hamburg-Fuhlsbüttel is in an urban area. The maximum annual wind speed in Sylt is as high as 24.1 m/s, while the maximum wind speed in Hamburg-Fuhlsbüttel is 16.3 m/s.
The generated electricity
Esp by the PV can be calculated by:
where
Qsp is the load of the solar panel per m
2 (in kWp/m
2), which equals to about 0.2 kWp/m
2. The area of the solar panel modules
Asp is assumed to be 30% of the roof area (
Asp = 33 m
2), and they face the south to have a best performance.
hpeak is the daily peak sunshine hours, and
εsp is the efficiency of the entire system. Here
εsp is assumed to be 80% considering the wiring losses and inverter efficiency [
22]. In the model, we assume that the energy supply efficiency of the energy equipment is constant, whereas in real scenarios the efficiency may decrease with lifetime. On the other hand, as technology improves, the efficiency of new replacement energy devices may be improved, and the price may decline as manufacturing costs decrease. Thus, losses due to reduced equipment efficiency may be compensated for in the long run. The extent of compensation may require a degree of forecasting of technological developments for each type of equipment and is therefore beyond the scope of this study.
The wind turbines used in this study is the Skywind NG (
www.myskywind.com, accesssed on 23 March 2024), and its potential electricity production
Ew can be calculated by the following equation:
where
vw is the wind speed in m/s, and
Ew is the electricity in kWh. The wind turbine has a start-up speed of 4 m/s. If
vw is less than 4 m/s, the wind turbine will not produce any electricity.
According to the German Climatic Zoning Standard [
23], the houses in Hamburg and Sylt are located in climate zones 3 and 1, respectively. Due to data protection principles, the exact addresses of the houses are not provided in this paper. Information on soil types, textures and their properties can usually be found in several European or German open databases. The shallow soil type below the house in Hamburg is clay (
https://geoportal-hamburg.de/, accessed on 23 March 2024) with a thermal conductivity ranging from 0.83 to 1.07 W/(m·K) (
www.thermomap.eu, accessed on 23 March 2024). Down to 100 m, the thermal conductivity is ca. 2.46 W/(m·K). The shallow soil type below the house in Sylt is coarse sand (
https://umweltportal.schleswig-holstein.de/, accessed on 23 March 2024) with a thermal conductivity ranging from 0.89 to 1.21 W/(m·K) (
www.thermomap.eu, accessed on 23 March 2024). The thermal conductivity is ca. 2.34 W/(m·K) down to 100 m. It is worth noting that in this study, higher resolution data were not used to estimate the geothermal potential, although detailed geologic profiles may exist in these open databases in the vicinity of the house location. Groundwater flow and recharge were also not considered, although they can positively affect geothermal performance [
24,
25].
2.4. Economic Model
Based on the above design, an economic model can be further generated based on the German market and policies (e.g., subsidies from certain state agencies or individual states and municipalities). In this study, the prices for materials, installation and operation are based on the average values of the German market, while subsidies follow the current policies of Hamburg or Schleswig-Holstein. At the same time, an average inflation rate (IR) of 2.5% was included in the model based on past records in Germany. The total service life N of the energy system is up to 100 years. In calculating and comparing the total costs, two main scenarios were considered:
Scenario I: Heating demand is realized by a gas boiler, and electricity is supplied from the local grid. The total cost of energy consumption at
ith year (TC
gb,i) for the house, including gas boiler heating and electricity purchased from the grid, can be expressed as follows:
where IC
gb is the installation and equipment cost of the gas boiler and its accessories. OMC
gb,i is the operation and maintenance cost at
ith year.
Eea,i is the annual electricity consumption all appliances, and
Pegrid is the amount of electricity paid by the household to the local grid. Concretely, OMC
gb,i can be calculated through:
where
Egas,i is the annual consumption of natural gas. Considering the efficiency of the gas boiler is 90% [
26] and the heating consumption of the house, the gas consumption can be 30,000 kWh/a.
Pgas is the unit price of natural gas, which is assumed to increase with inflation in the following years due to the shortage of natural gas in Germany. When
i reaches the service life of the gas boiler (LT
gb), the gas boiler will be replaced with a new one, considering that the investment will also increase due to inflation. The annual maintenance cost of a gas boiler MC
gb is about 1.3% of the initial cost IC
gb, a value that is also affected by inflation.
Scenario II: Heating and electricity demands are supplied by a solar-wind geothermal coupled system. The total costs of this renewable energy system at
ith year (TC
swg,i) can be estimated by the following equation:
The first two terms on the right-hand-side of Equation (5) represent the installation and maintenance costs of solar panels, wind turbines, a heat pump (abbreviation—hp), heat exchanger (abbreviation—hex), and a circulation pump (abbreviation—wp), respectively. In addition, household appliances may be used at night, when a battery bank (abbreviation in bb) may be needed for short-term electricity storage (up to several days without sunshine). Based on the average daily electricity consumption of appliances, the storage capacity of the battery pack should not be less than 11.8 kWh. If standard lithium batteries (12.8 V 10 Ah) are utilized, at least 10 of these batteries (with a charging and discharging efficiency of at least 90%) are needed.
OC
ea,i is the annual operating cost of the appliances, assuming that only the cost of electricity consumption is considered:
Households can receive the cost compensation for selling excess electricity generated by PV and wind turbines back to the grid. It is worth noting that under the current policy, the price of electricity sold back is generally much lower than the price of electricity purchased. The feed-in tariff
Rfd,i can be calculated through Equation (7):
Δ
Ei represents the difference between the electricity generated by PV and wind turbines (
Esp and
Ewt) and the electricity demand by the electrical appliances (
Ehp,
Ewp, and
Eea):
It is noted that the feed-in tariff price
Pefd,i will increase every 20 years in the inflation rate IR:
In Hamburg and Sylt, the initial feed-in tariff price PEfd,i is much less than the local purchase price.
The service life of the heat exchanger is expected to be unlimited. Detailed calculations for each IC and OMC in Equation (5) can be found in Equations (10)–(22):
The cost of the heat pump can be subsidized through a few local incentives, such as a subsidy for the installation of heat pumps. Some regions in Germany also subsidize the installation of solar panels and BHE. In this model, only subsidies for heat pumps and for each heat pump replacement are included. In Equation (14), Rhp equals to 40% according to the present policies.
Table 2 shows the German market prices (including purchase and installation costs) and operating costs for the components of the energy system.
3. Results
3.1. Dimensioning and Installation Cost of Geothermal System
According to VDI 4640 Part 2 [
21], it is possible to roughly estimate the dimensions of three different heat exchangers, as shown in
Table 3. Pipes (32 mm × 3 mm) used for all three heat exchangers are made of robust polymer material, PE-100 RC. The heat transfer medium is an antifreeze, e.g., 30% water-propylene-glycol mixture, to lower the outlet temperature of the heat pump (≥−5 °C) so that more heat can be extracted (temperature spread at the compressor is assumed to be 5 K).
It is assumed that two BHEs are used to satisfy the heat demand of the house, and the pipes of the BHEs are in the form of a double-U, which is commonly used in Germany. Theoretically, more BHEs with short lengths could also be used if the size and geology of the site near the house allow it. According to the regulations of Hamburg and Schleswig-Holstein, each BHE should have a diameter of not less than 150 mm and the grouting material should be a cement with a high thermal conductivity (more than 2 W/m·K). The distance between the two BHEs is greater than 6 m to minimize interference between them. Based on the full load hours per year, the heating demand of the house, and the SCOP, the BHE load is calculated to be 11 kW. As both sites have a similar average thermal conductivity at 0–100 m, the length of each BHE is close, at about 122 m and 126 m, respectively, based on the heat extraction rate and the load of the heat exchanger (44.7 W/m in Hamburg and 43.5 W/m in Sylt). BHE installation costs include drilling, grout, and material costs (incl. double-U heat exchanger and cement). The initial investment cost of the two locations amounted to approximately EUR 31,350 and EUR 32,260, respectively.
Horizontal collectors and geothermal baskets have a slightly larger load because they operate 150 h less per year than the BHE system. Horizontal heat collectors use the simplest linear arrangement. The distance between the linear loops depends on the climatic zone in which the house is located. In Hamburg, a pipe spacing of 0.45 to 0.55 m is recommended, which is almost twice that of Sylt. This results in much longer pipes being used in Sylt. The heat extraction rate of the collectors also depends on the climatic zone in which they are located and the type of soil (i.e., Hamburg: clay, Sylt: sand). Because the heat extraction rate is higher in Hamburg, it will require less land area and excavation volume. To ensure that the soil temperature in the vicinity of the heat exchanger is not too low and to ensure that sufficient heat regeneration is obtained, the geothermal heat exchanger is buried at 1.5 m below the surface. Combining these factors, unlike the BHE system, the initial investment cost of Sylt is approximately 1.5 times that of Hamburg for a linear-collector system.
A geothermal basket is a special type of shallow geothermal collector. In principle, it occupies less space than a horizontal collector as it is buried in the vertical direction and has better thermal efficiency. Here, a smaller basket with a dimension of 1.3 m (average diameter) × 1.3 m (height) is used for the design. The maximum and minimum diameters of the basket are 1.7 m and 0.9 m, respectively. The burial depth and the distance between the baskets are both 4 m, according to the recommendations of VDI 4640. A basket has a pipe length of about 32.5 m and an excavation volume (assuming its volume is 1.8 m × 1.8 m × 4 m) of about 13 m3. In Hamburg, 21 baskets are needed to meet the heat demand of the house, while in Sylt, as many as 36 are needed. By calculating the total volume to be excavated as well as the length of pipes needed, it was found that the initial cost in Sylt is about 1.7 times that of Hamburg.
3.2. Electricity Balance and Potential
An ideal case is considered for these two houses where the maximum area of solar panels that can be installed on the house is 30% of the roof area, and at the same time, up to four wind turbines can be placed on the roof.
Table 4 shows the breakdown of the annual electricity balance, where the green numbers indicate the electricity generated by the housing facilities, the red numbers indicate the electricity consumed by the houses, the gray numbers indicate the electricity that needs to be purchased from the grid, and the blue numbers indicate the electricity that can be fed in back to the grid.
As demonstrated in the previous section, houses in Hamburg have more days of sunshine per year than in Sylt, so the same area of solar panels can produce more electricity in Hamburg, about 1152 kWh/a more than in Sylt, whereas Sylt has more wind-converted electricity, with a total of 4323.5 kWh/a, compared to 16% of that in Hamburg. The electricity required for the heat pump and circulation pump is calculated from the SCOP and the heat demand of the house. Since the piping of the two near-surface heat exchangers is much larger than the BHE, their circulation pumps are more powerful and consume more electricity.
Figure 5 illustrates the electricity difference calculated according to Equations (1), (2) and (8). Overall, the seasons when electricity needs to be purchased from the grid are mainly spread over the winter months, roughly from November to February. During the summer months (May to September), due to the abundant sunshine in northern Germany, a lot of excess electricity is generated that can be sold back to the grid. Because of the seasonal fluctuations in solar and wind energy, solar panels and wind turbines do not produce enough electricity to fully satisfy heat and circulation pumps, as well as household appliances. As electricity is cheaper for the heat pump, the electricity needs of heat pumps are prioritized to be fulfilled. Calculations show that for the three different heat exchangers, the house in Hamburg would need to purchase 3332.7 kWh, 3693.1 kWh, and 3503.9 kWh of electricity per year from the grid for the heat pump, and the house in Sylt would need to purchase 2274.2 kWh, 2578.7 kWh, and 2417.3 kWh of electricity per year for the heat pump. The circulation pump and household appliances also need to purchase a certain amount of electricity from the grid at normal electricity prices to meet their use during the low electricity production period.
Figure 5a,b show the total amount of electricity that needs to be purchased and can be fed back to the grid each day for the Hamburg and Sylt houses. A comparison between these data in
Table 4 as well as
Figure 5a,b indicates that the house in Sylt needs to buy less electricity per year from the grid than in Hamburg since the local wind speed in Sylt is greater than in Hamburg. Correspondingly, it is able to deliver more electricity to the grid and thus receives some more compensation in the form of rebates. In this exemplary renewable energy system, the Sylt house spends less on electricity than the Hamburg house.
3.3. Costs of Different Energy Systems during Long Operation Times
Based on Equations (9)–(22) and the price list (
Table 2), the total costs for different energy systems could be calculated, including initial investment, replacement costs of materials, as well as operating and maintenance costs.
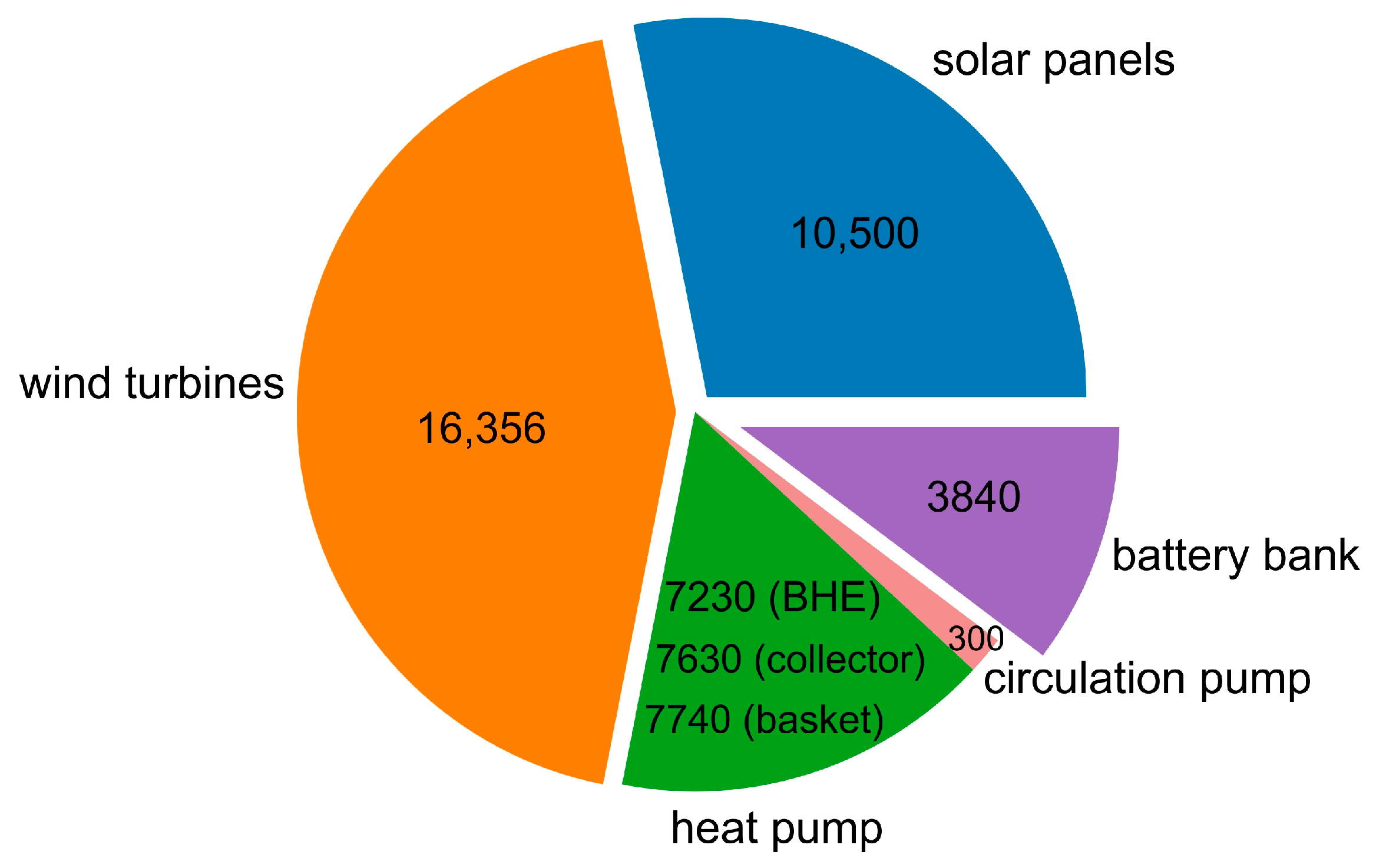
Figure 6 illustrates the initial investment cost of the different facilities of the two houses. Since both houses are the same size and have the same energy consumption, the cost of the different equipment is equal. Solar panels, wind turbines, and battery banks cost EUR 10,500, EUR 16,356 and EUR 3840 euros to purchase and install, respectively. Due to the policy of subsidizing the heat pump, it costs considerably less than solar panels as well as wind turbines.
Figure 7 shows the total cost of the different energy systems of the house when they have been operating for
N years (
N = 0, 1, …, 100). The abrupt jump (marked in triangles) above the bars in the graph indicates that at this time point certain equipment is reaching the end of its service life and needs to be replaced.
From
Figure 7a–l, the gas system is compared to a pure geothermal system sequentially, as well as to different hybrid systems. If the system runs for 100 years, the total cost of the gas system can reach EUR 2,126,728. Overall, if the renewable systems are operating for at least 20 years, the total cost will be less than a gas-boiler system. The renewable system with BHEs consumes less annual electricity. Therefore, even if it has a much higher initial investment, after a long operating period, the total cost will be similar to the other two; at some point, it will be slightly less. For instance,
Figure 7a–c indicate that, after 100 years, the total cost of the pure BHE system (EUR 664,110) is lower than the linear collector (EUR 667,328) and basket systems (EUR 674,074). In addition, a comparison of the three pure geothermal systems also shows that the Sylt house costs more to run in the long term than the Hamburg house if it is equipped with linear-collector or geothermal-basket systems due to the fact that the shallow soil in Hamburg has a higher heat extraction rate.
The renewable energy systems with wind turbines (
Figure 7i–l) have little difference in the cost over the first 40 years than other clean energy systems without wind turbines. However, due to the current high purchase and replacement costs of wind turbines and the low conversion rates (wind turbines not being high enough, too many local shelters, and other possible factors), and considering continuous inflation, the costs of wind-turbine-equipped systems will increase significantly over the longer term and greatly exceed the costs of other non-wind-turbine-equipped systems.
Due to the increased number of devices included in the hybrid systems and the different service lives of each device, there are several significant jumps in the cost of the renewable energy systems in
Figure 7c–l, especially when multiple devices need to be replaced at the same time in a given year (e.g., in the 100th year). Of these hybrid systems, the system that uses solar panels in combination with geothermal energy is the least expensive. The long-term cost of this system is similar to that of a pure geothermal system. Since Hamburg has a much larger potential PV capacity,
Figure 7d–i show that Sylt house spends more than in the Hamburg house in the long term. The opposite is observed when a wind turbine is included in the system, and since Sylt produces more electricity through the wind, the Sylt house spends less on electricity than the Hamburg house.
3.4. Payback Period
Assuming both houses are already running a natural gas heating system, the energy systems now need to be upgraded and retrofitted. The payback periods (
tpayback) required for the different energy retrofit and upgrade options can be calculated by Equation (23):
where
Msaving,i is the energy savings costs at
ith year. Because the long-term operational economic model demonstrated in that study does not grow linearly, annual energy savings are calculated by averaging the total within the operation time
N (
N = 10, 11, 12, …, 100).
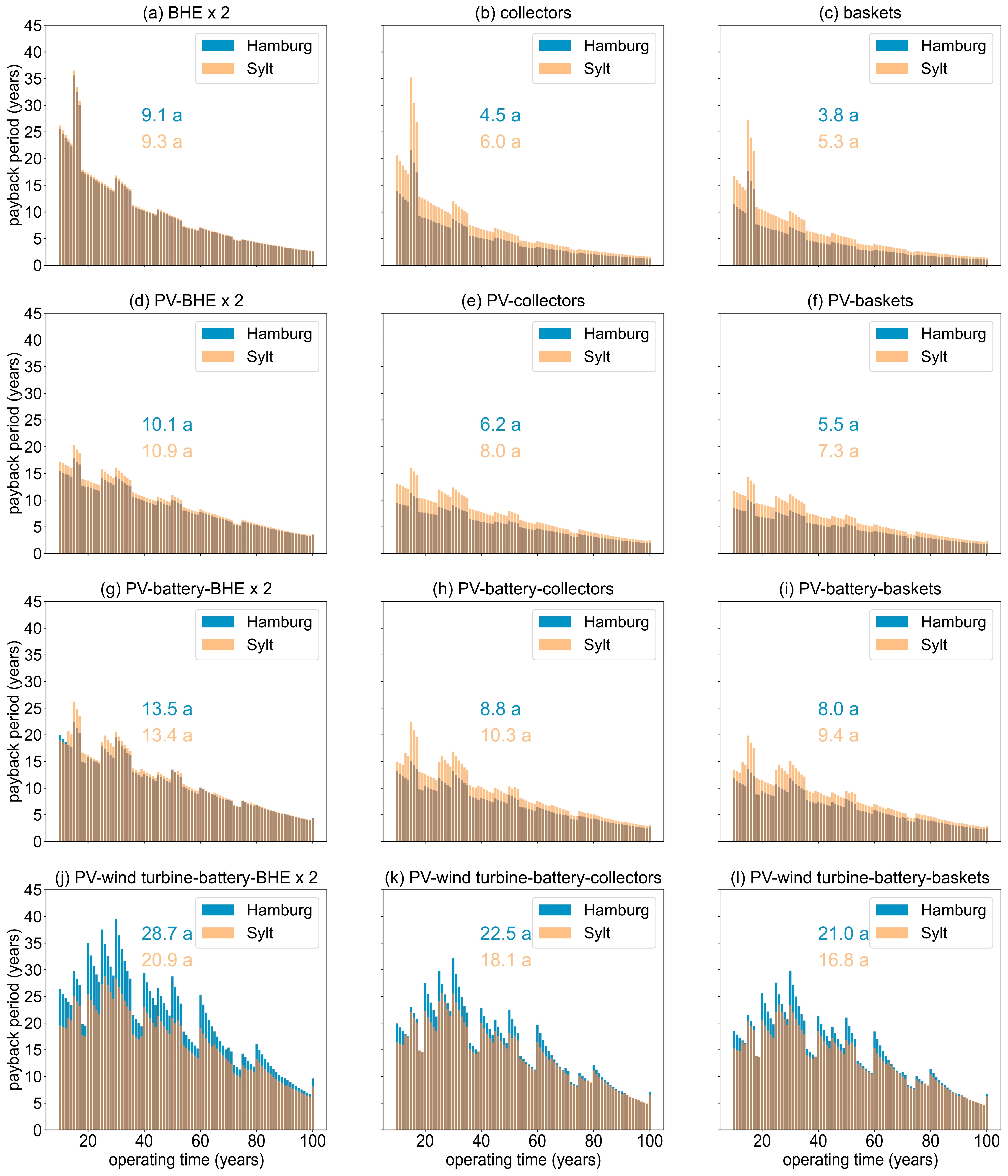
Figure 8 shows the payback period when the renewable systems run for different time periods. If the payback period is longer than the operating time, it means that the investment is at a loss. All subplots in
Figure 8 indicate that the longer the system is in operation, the shorter the payback period. The numbers in the plots are the payback periods when the entire system runs for 50 years. Looking only at
Figure 8a–f, a geothermal system alone or a combination of geothermal plus PV systems gives a good payback period. The payback period for the Hamburg house is shorter if the installation of wind turbines is not taken into consideration. The payback for the Hamburg house is faster due to the better thermal conductivity of the near-surface subsoil, and the similar payback for the Sylt house for a BHE system is due to the similar thermal conductivity of the deeper formation (
Figure 8a–i). Most of the payback periods for collectors and baskets are less than 10 years because they cost considerably less to install than BHE systems in Germany. When on-roof wind turbines are added, the payback period increases by a factor of 3–5 due to the more expensive purchase cost (
Figure 8j–l). Also, due to the more abundant wind energy in Sylt, payback is faster than in Hamburg with the additional wind turbines.
4. Discussion
Overall, near-surface heat exchangers are much cheaper than BHEs because drilling in Germany is relatively expensive. Moreover, BHEs also require additional approval procedures. On the other hand, boreholes require a smaller land area and cause little damage to the landscape of the surface, thus providing an advantage where building clusters are crowded. Near-surface heat exchangers are simple to construct, and the systems are easy to maintain and refurbish. They are more susceptible to thermal regeneration than BHEs due to the influence of solar radiation. The main disadvantage of linear collectors is that they are more land- and space-intensive. However, some recent studies have shown that linear exchangers can be staggered in layers to take up less land space, while ensuring sufficient heat exchange efficiency. Without considering the manufacturing costs of geothermal baskets, it is possible that geothermal baskets are cheaper than linear exchangers because they may require less excavation costs. Larger geothermal baskets may be able to have better heat exchange and further reduce the initial cost, which will not be analyzed more in this article. In addition to the geometry of the heat exchanger, geothermal potential depends on the land use, local soil, and rock types, as well as the climate. Although not revealed by this study, other studies have found that certain climatic phenomena, e.g., precipitation, i.e., snowing (soil freezing and thawing), could change the saturation of the soil and thus influence thermal performance. Also, groundwater flow and the heterogeneity of the stratigraphy are key factors that affect the thermal performance, especially the BHEs.
Both PV and wind energy can generate electricity. Since solar panels are cheaper today, installing solar panels on the roof of a house and using the power generated by the panels to drive a heat pump allows the house to use clean energy as a source of electricity at a lower cost, especially if the excess electricity can be sold back to the grid. Conversely, because of the higher selling price of wind turbines, it may not be a very economical option for detached houses. However, for tall buildings and houses in open rural areas, choosing a suitable wind turbine or small windmill can significantly increase the amount of electricity converted from wind power, thus better compensating the energy supply, and improving the economic efficiency of the whole hybrid system. On the other hand, the house in this study is assumed to have an energy class of E. If the house has a higher energy class (e.g., above C) and is accompanied by stronger wind energy (e.g., taller buildings, open countryside), it is possible that, with the appropriate design, the system can be independent from dependence on the grid.
Considering the possibility of continued rising natural gas prices, it is foreseeable that the cost of a natural gas heating system will be higher than a geothermal-only system or hybrid renewable energy systems for these two houses over the next 100 years. Therefore, it makes economic sense to replace the gas heating system with a clean energy system. By comparing the total cost and payback period of the different energy systems, the system without wind turbines is more economical. Integrating solar panels into a geothermal system does not significantly increase the cost, and the payback period is only 1 to 2 years longer when the system operates for 50 years. Due to the higher initial cost of wind turbines, the total cost of a hybrid system with one type of wind turbine may be significantly higher than other systems after several equipment replacements, even doubling the payback period.
In Germany, since most existing buildings have a long history, they usually only require normal maintenance and renovation. Furthermore, in this study, the economic model includes the lifetime of the equipment, which in our code can be flexibly changed according to market changes. Certainly, the mix of different energy sources and associated equipment may also change over time when new technologies become available. According to the regulations of most German states, geothermal systems (especially BHE) should operate for at least 30 years. House owners or building management authorities, as well as real estate companies, can calculate the payback period based on the economic model presented in this paper for different portfolios and ensure that the payback period is less than the system’s lifetime.