Experimental Study on Performance of a Wave Energy Converter Rotor with a Moving Platform
Abstract
1. Introduction
2. Experimental Set-Up
2.1. Experimental Model
2.2. Characteristics of Experimental Rotor Model
2.2.1. Without PTO Damping
2.2.2. With PTO Damping
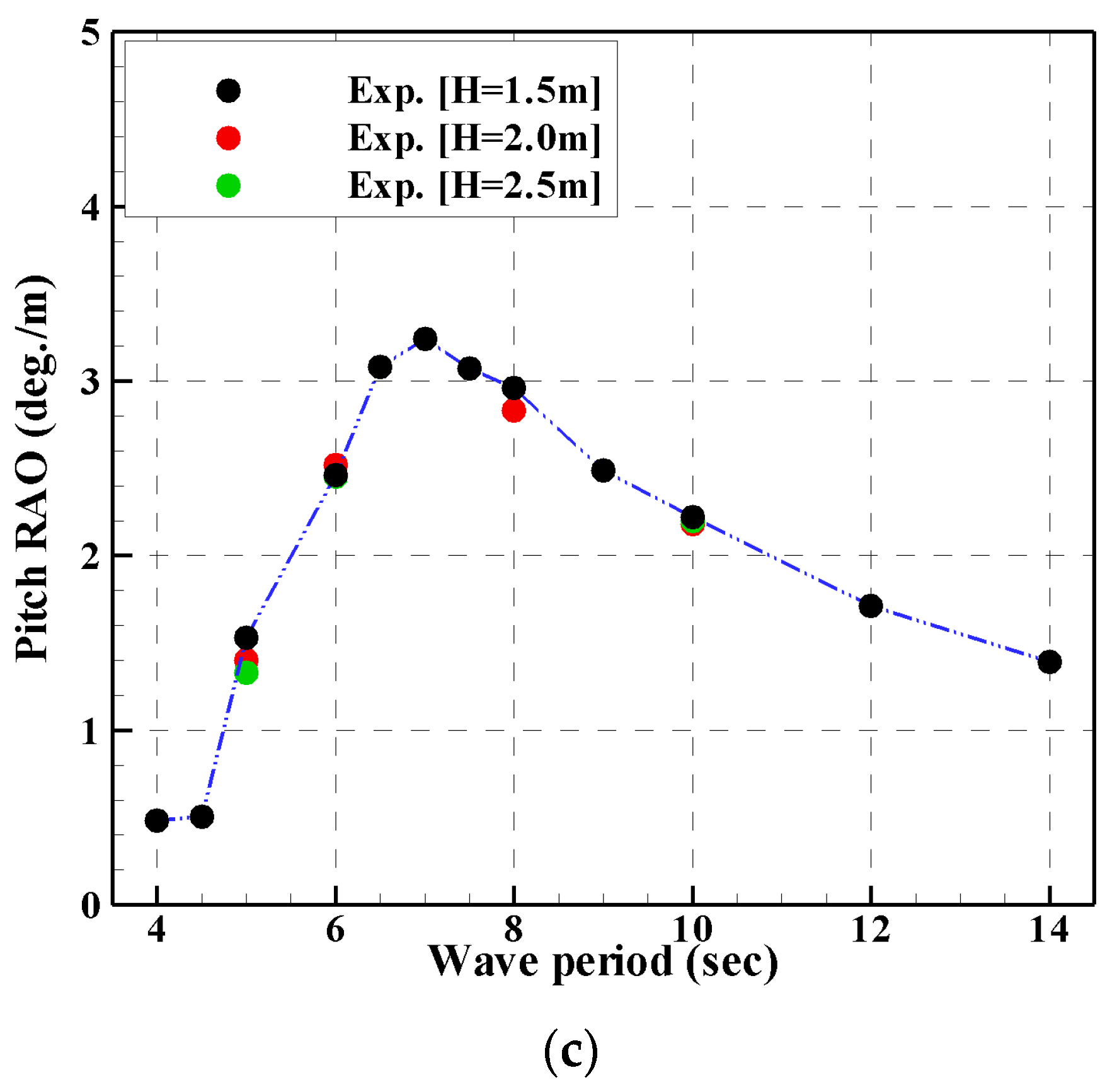
2.3. Wave Conditions
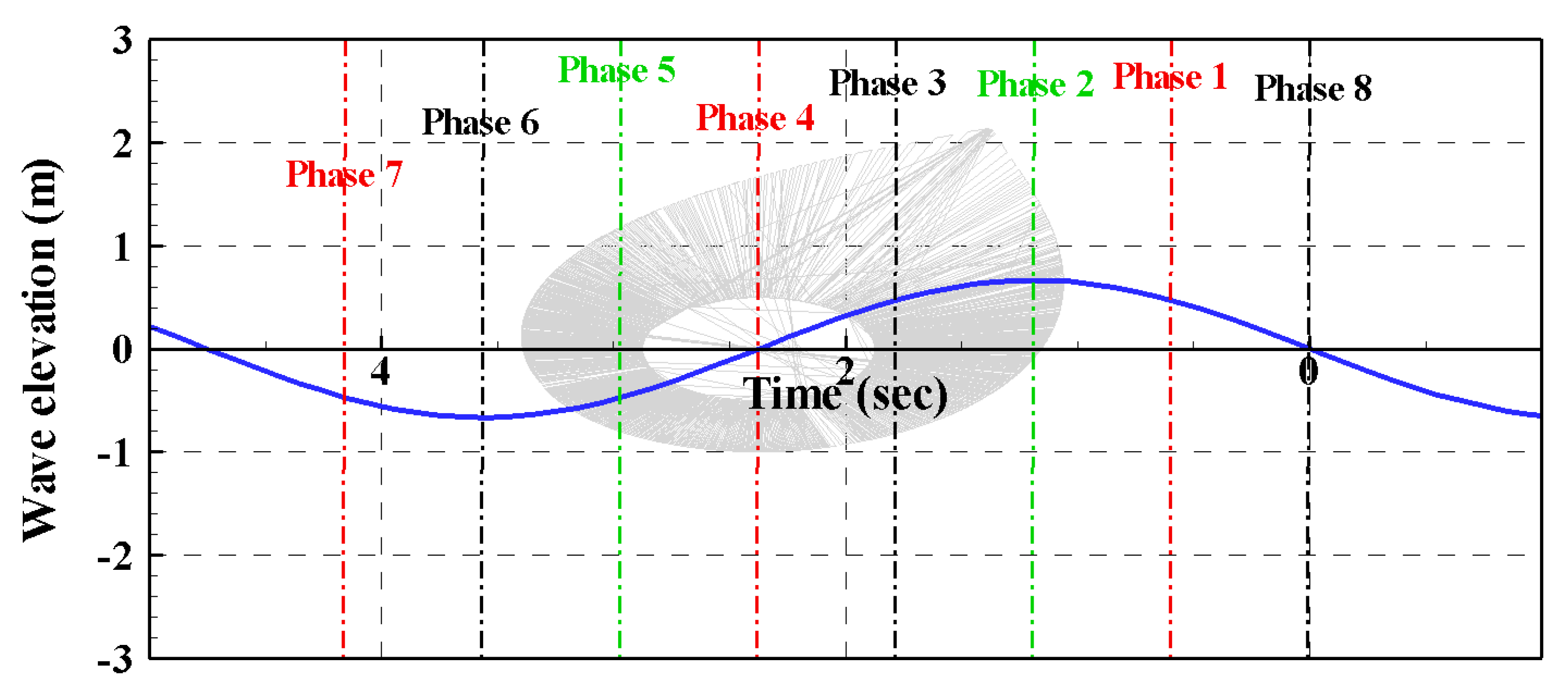
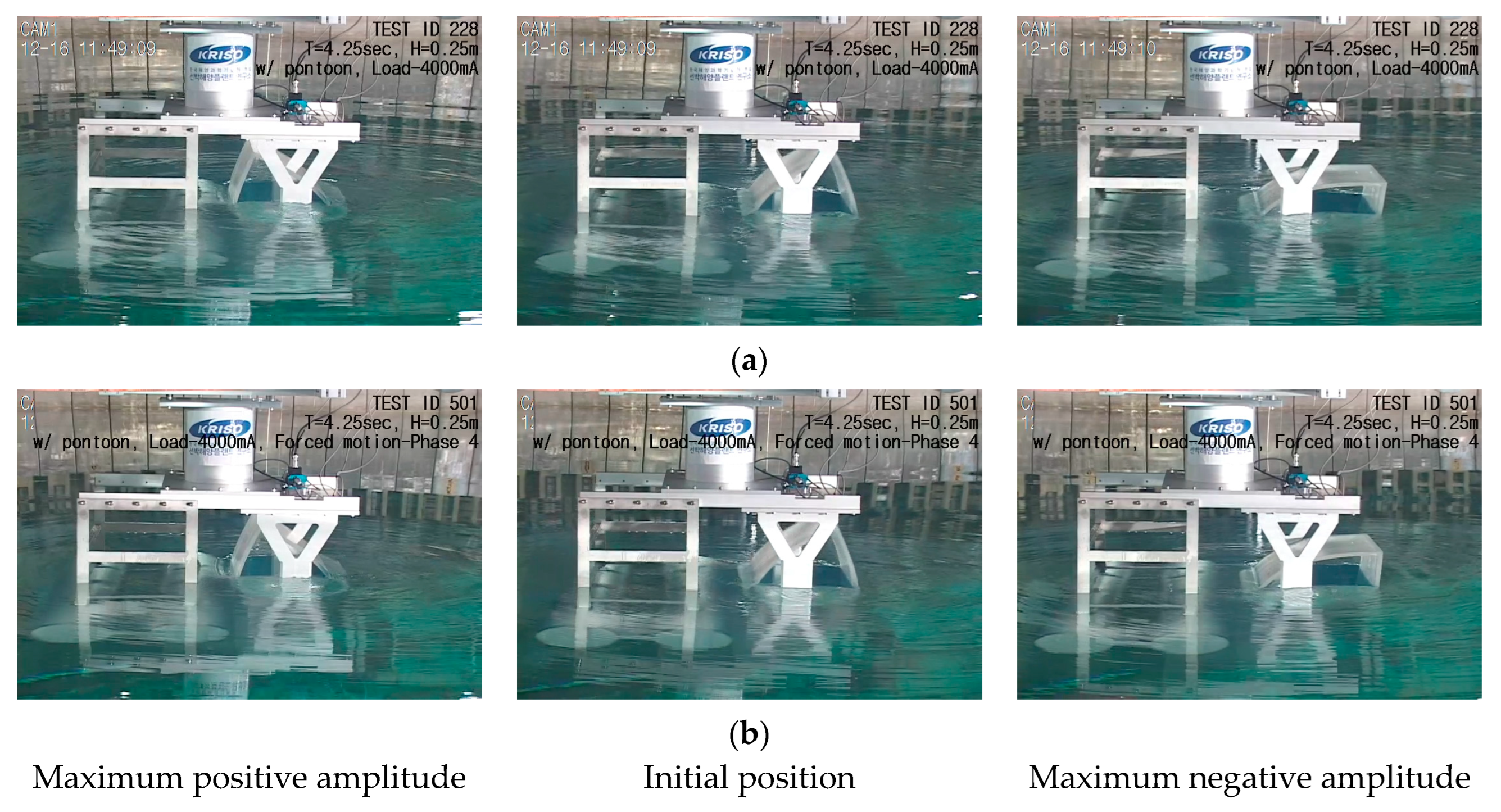
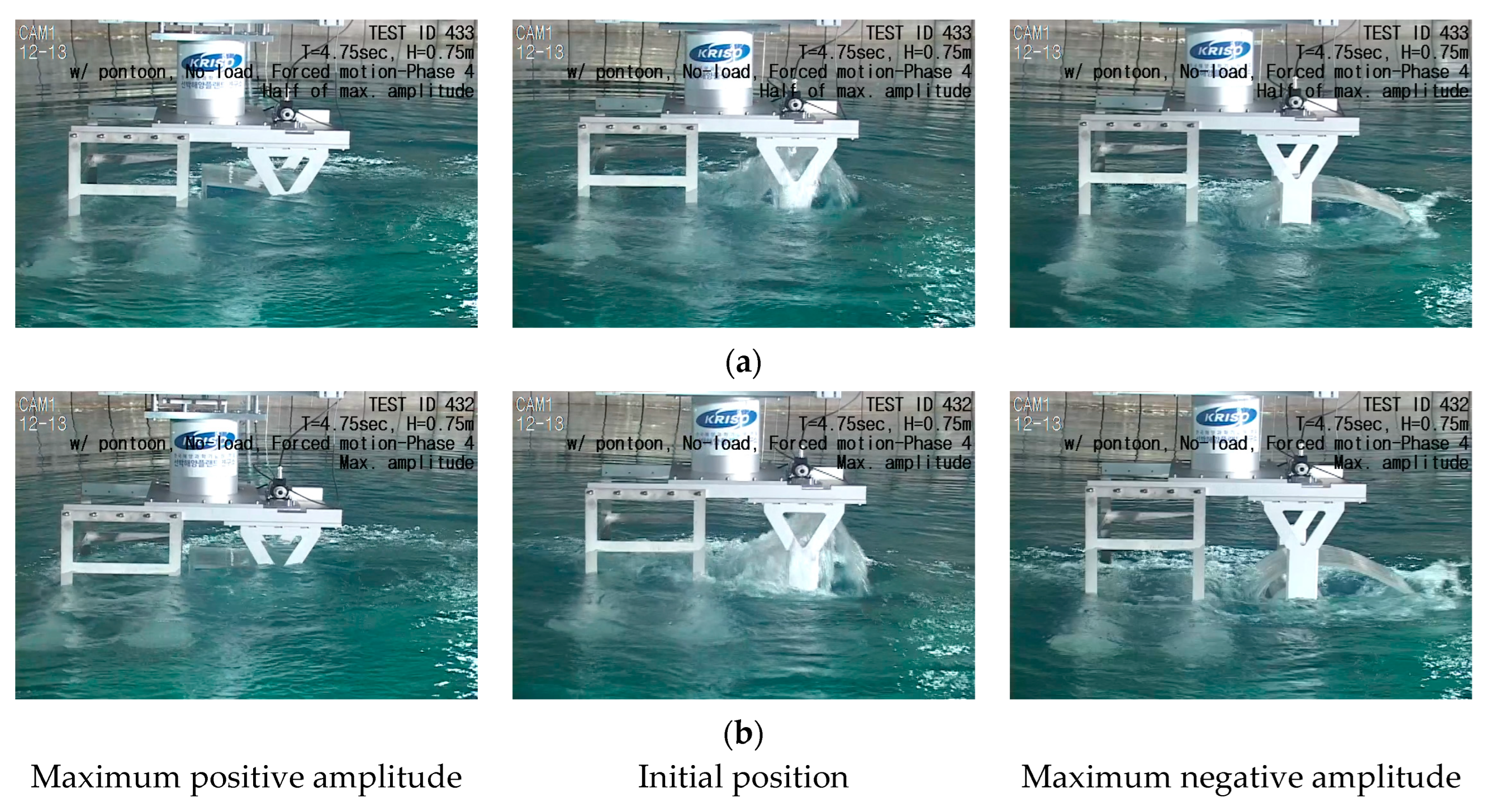
2.4. Definition of Phase
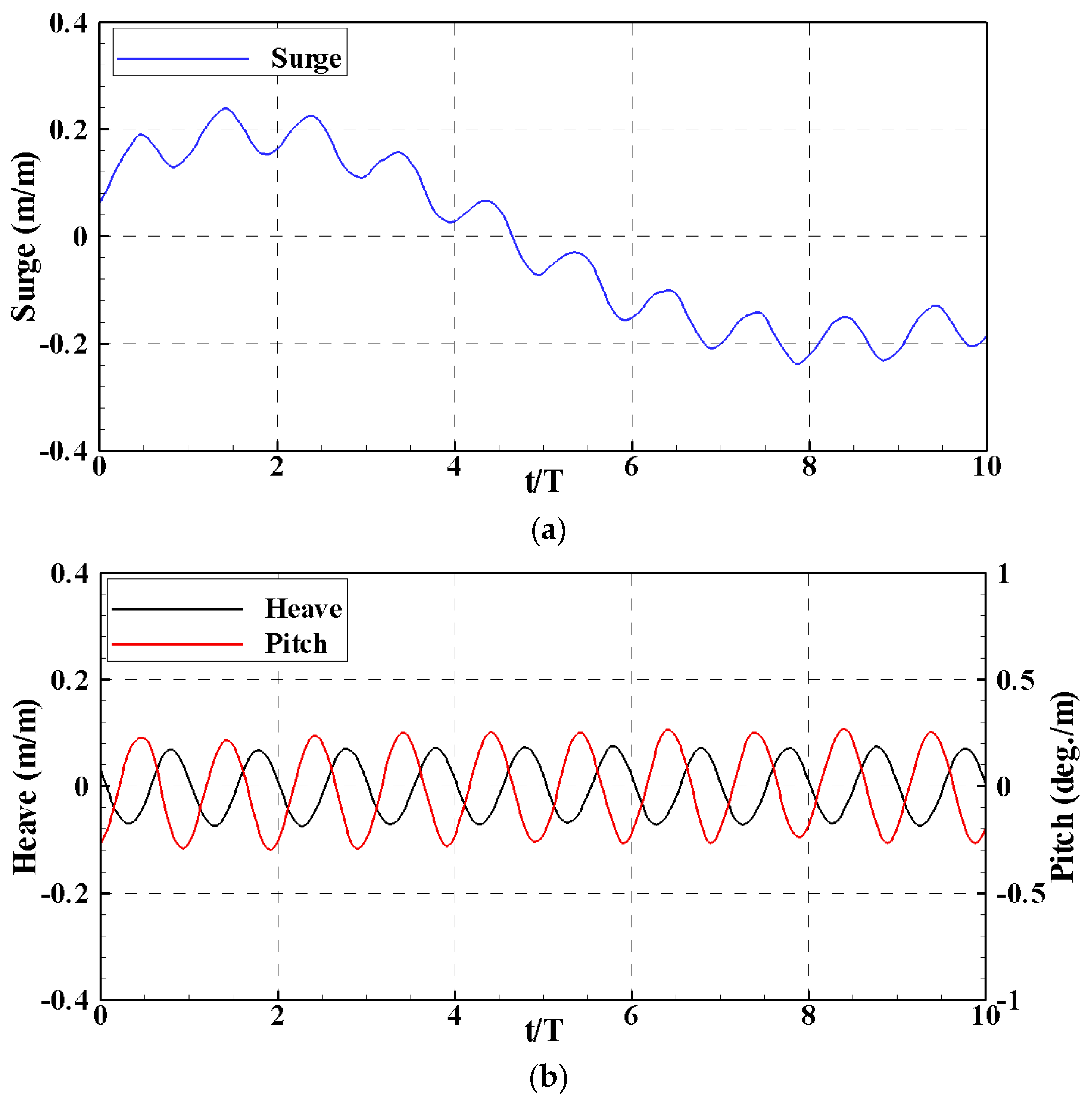
2.5. Forced Motion Conditions
3. Experimental Results
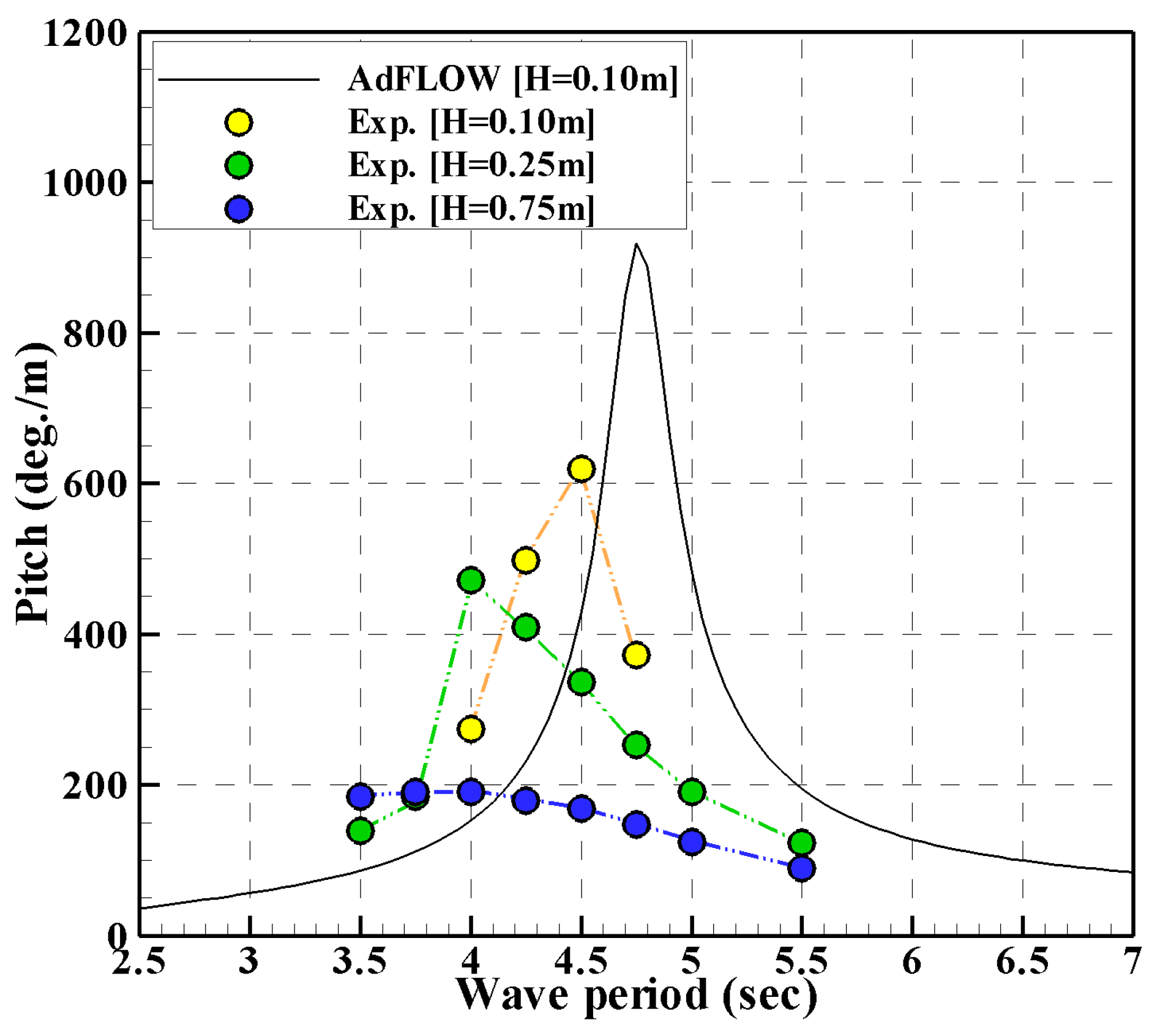
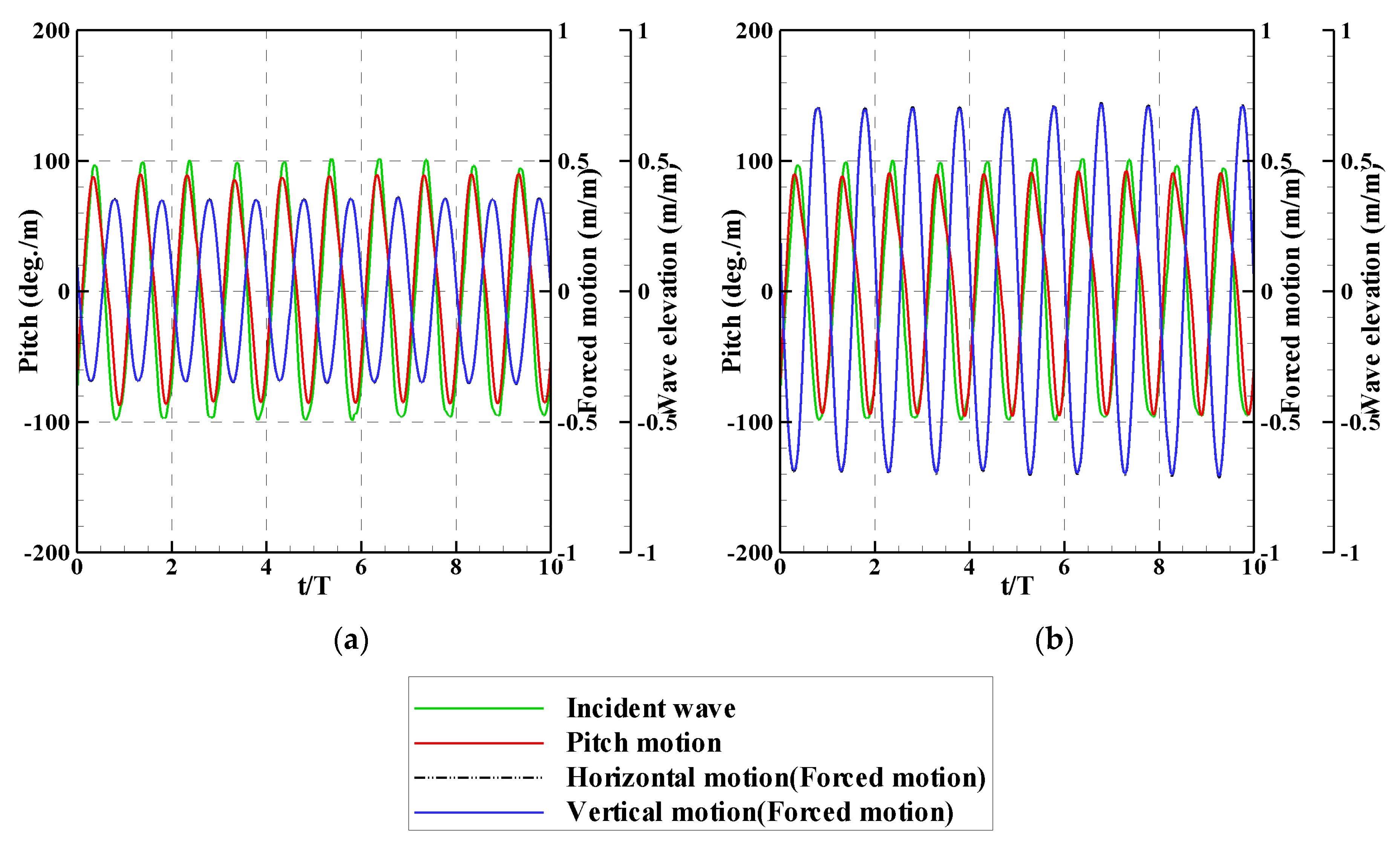
3.1. Without PTO Damping
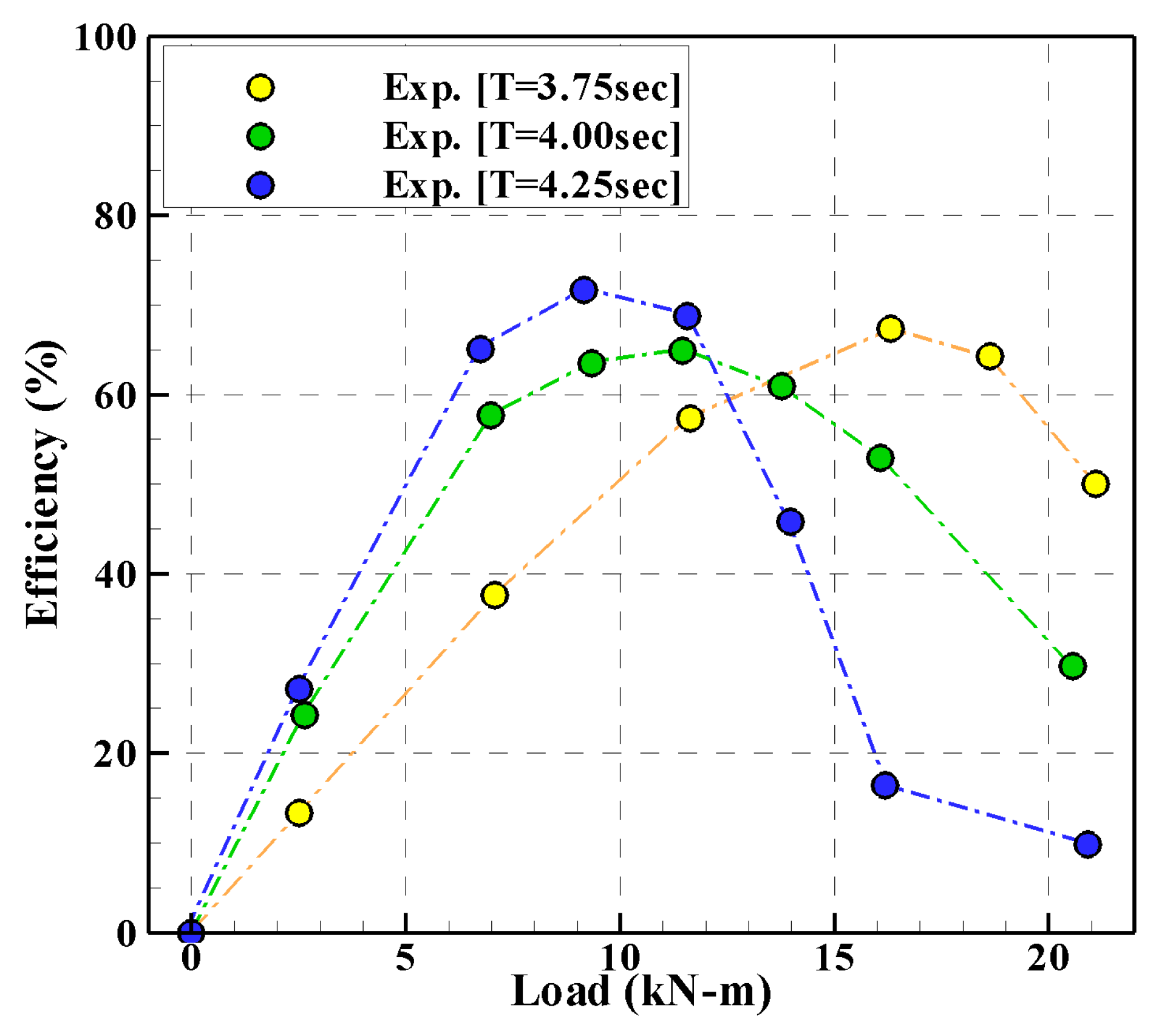
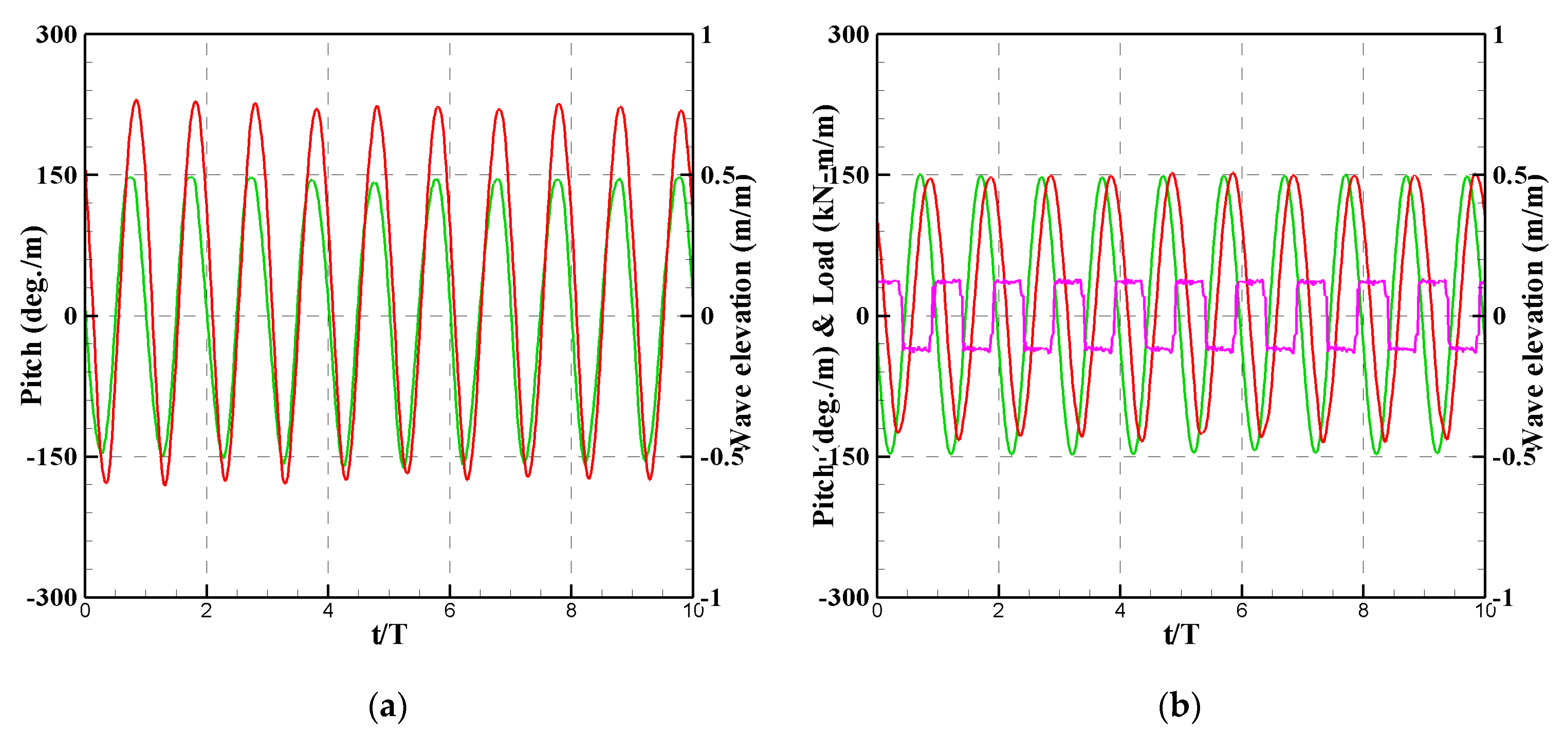
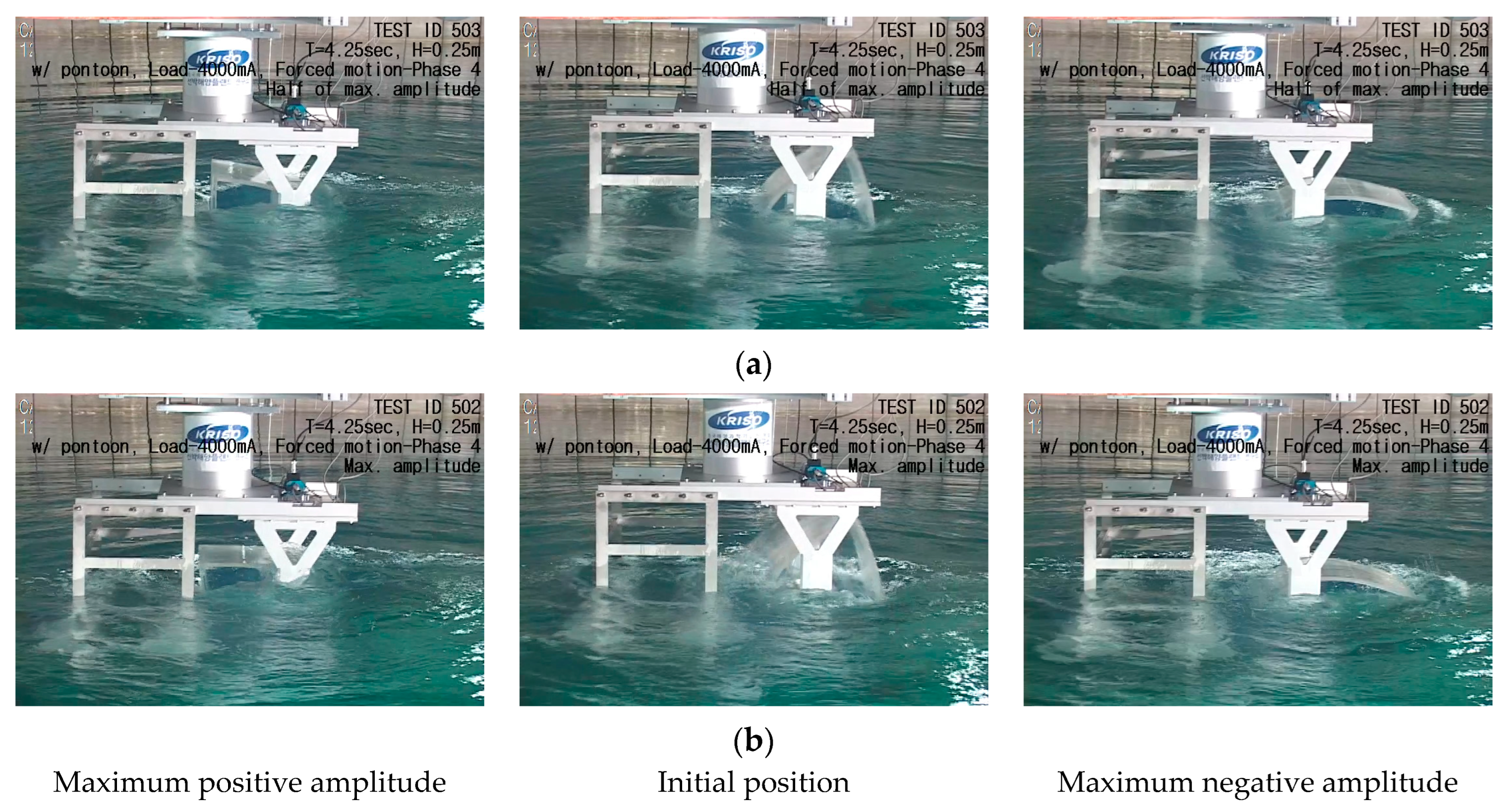
3.2. With PTO Damping
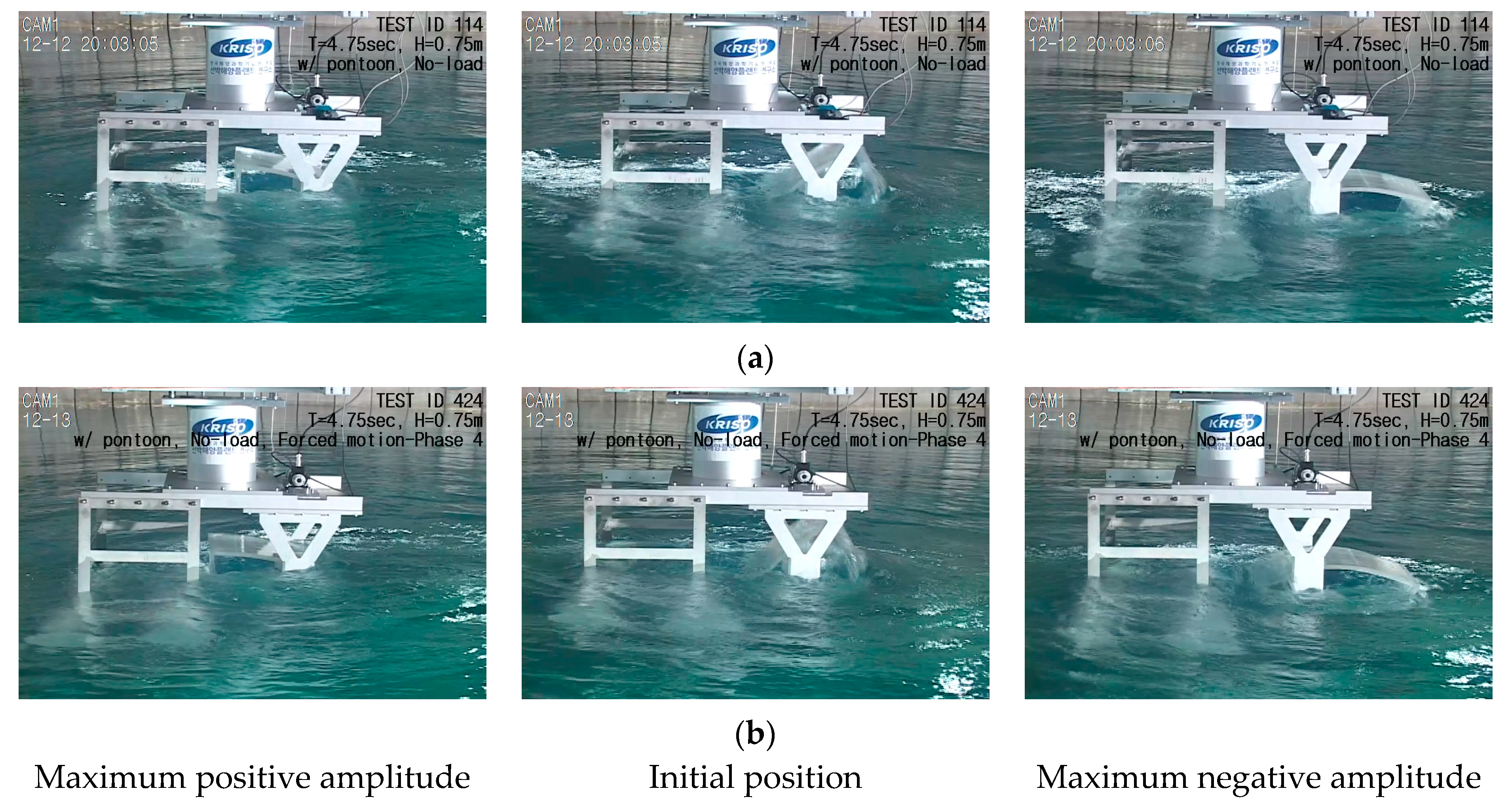
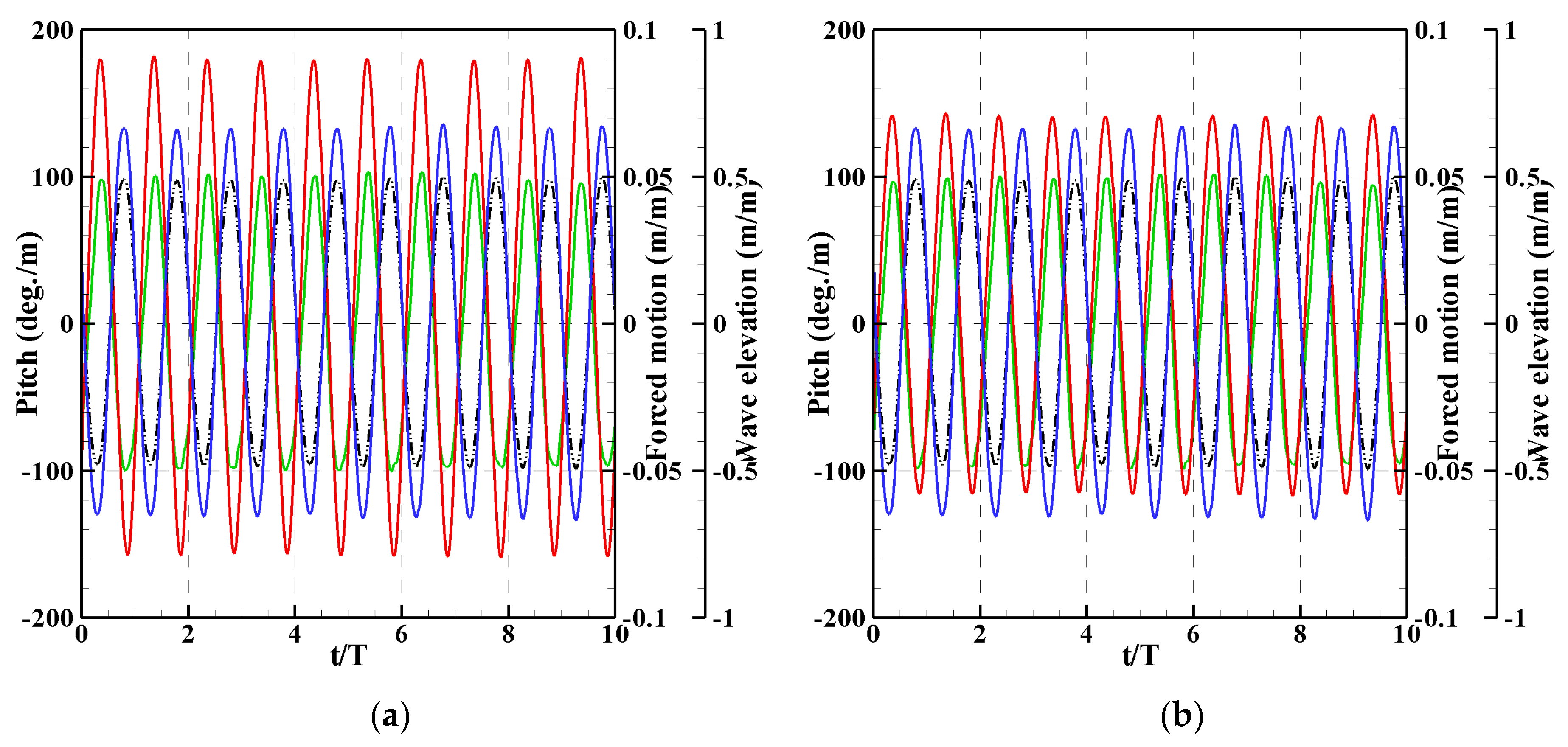
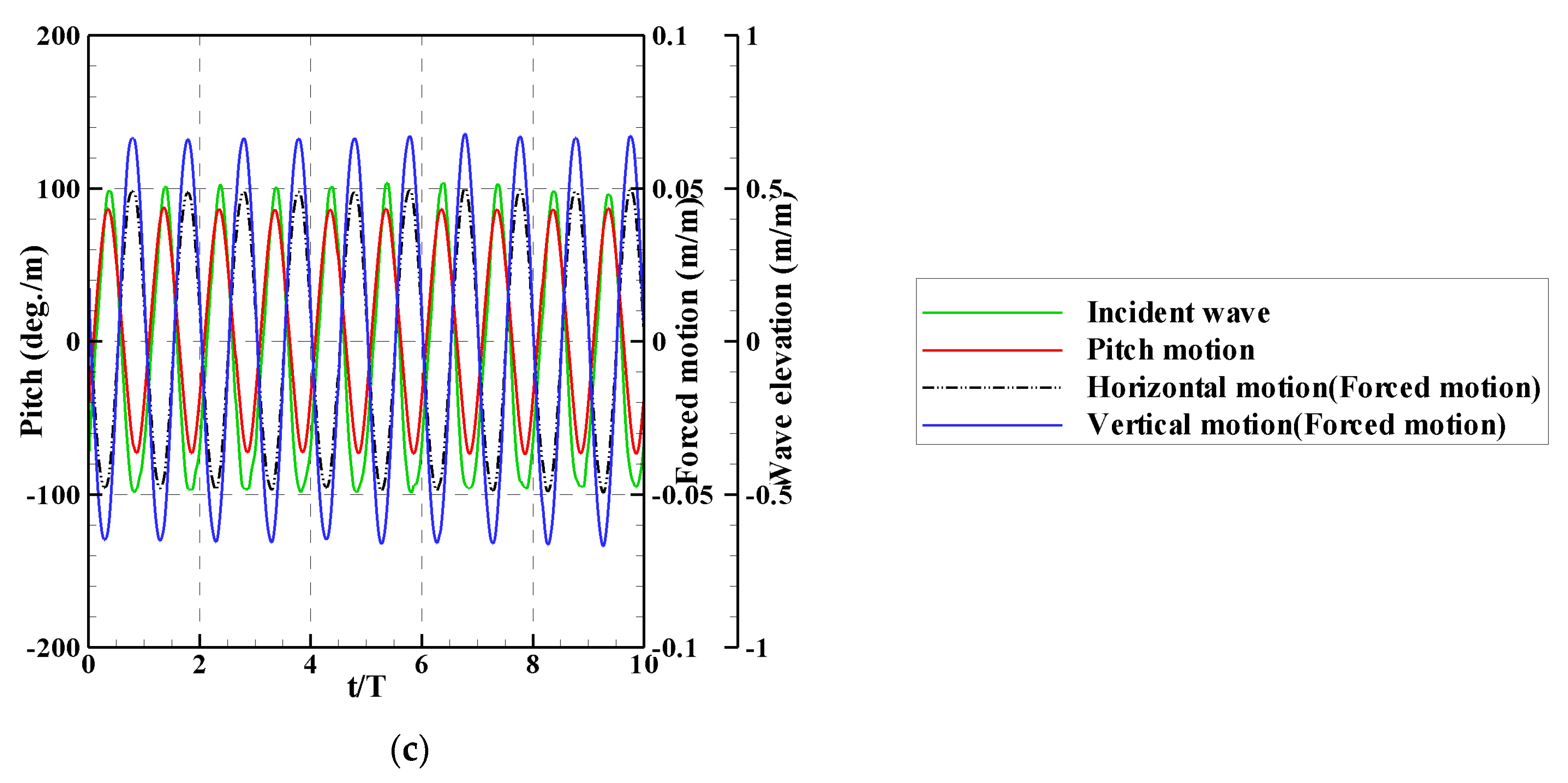
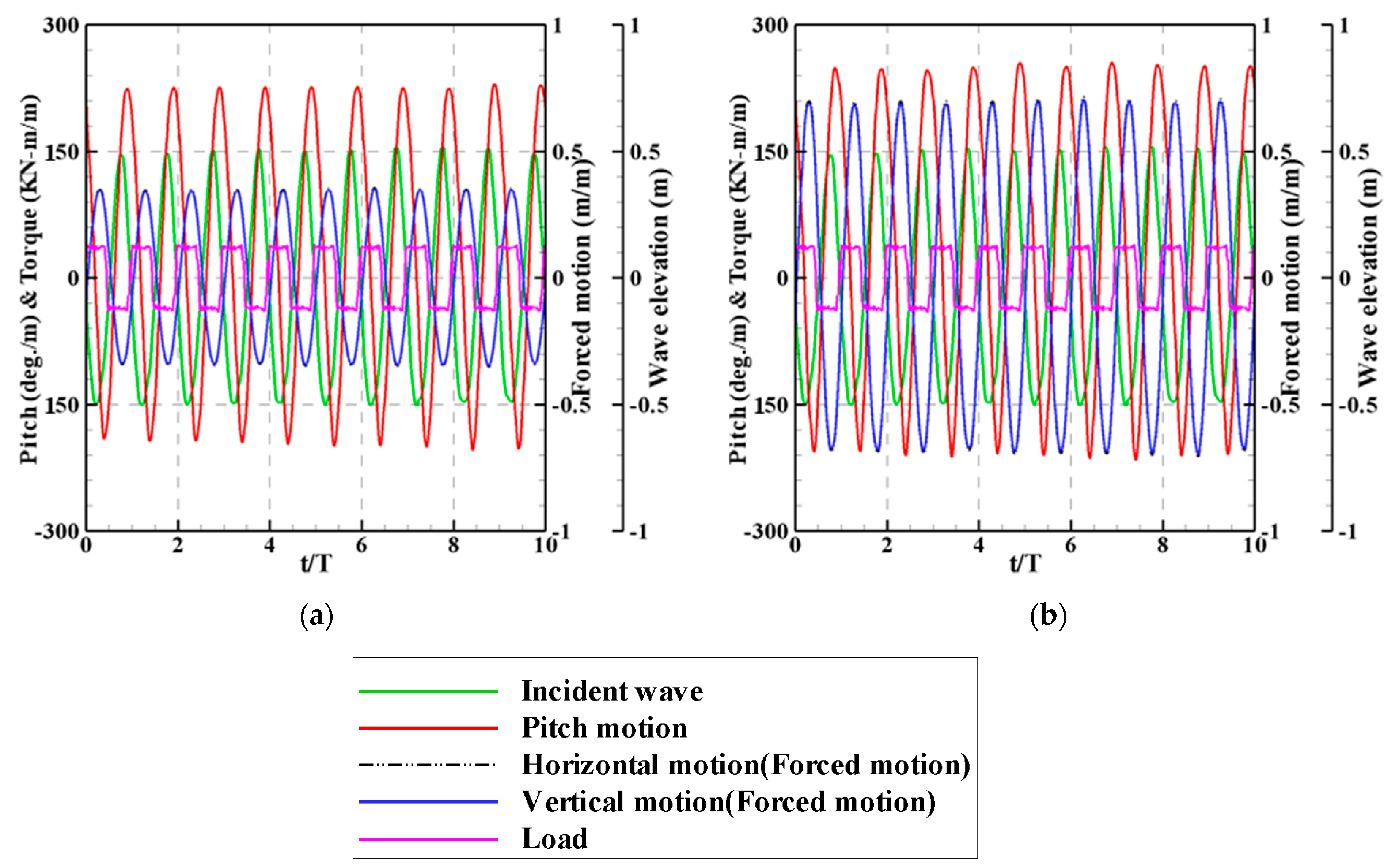
3.3. Additional Experiment on Large Motion of Platform
4. Conclusions
- (1)
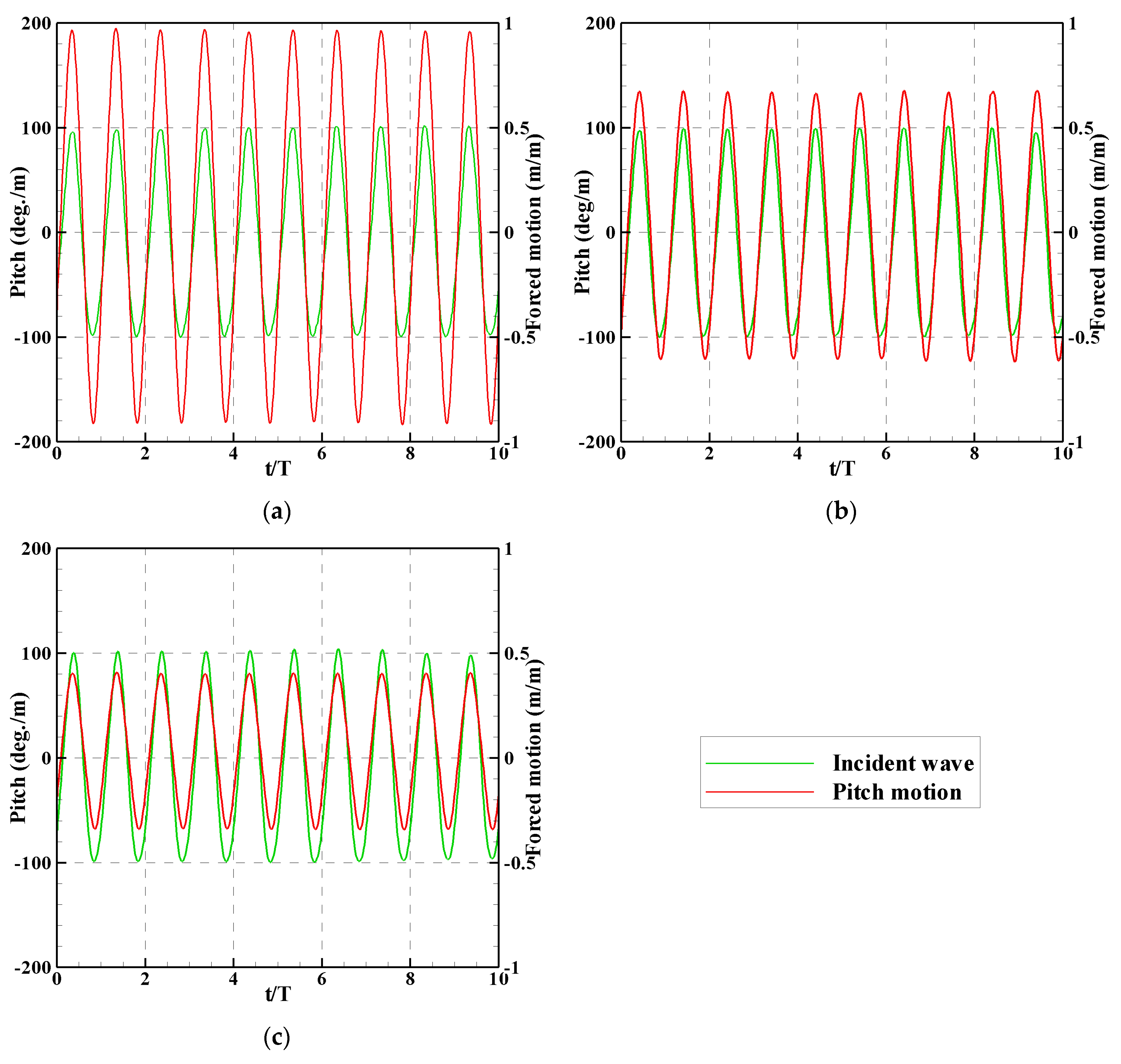
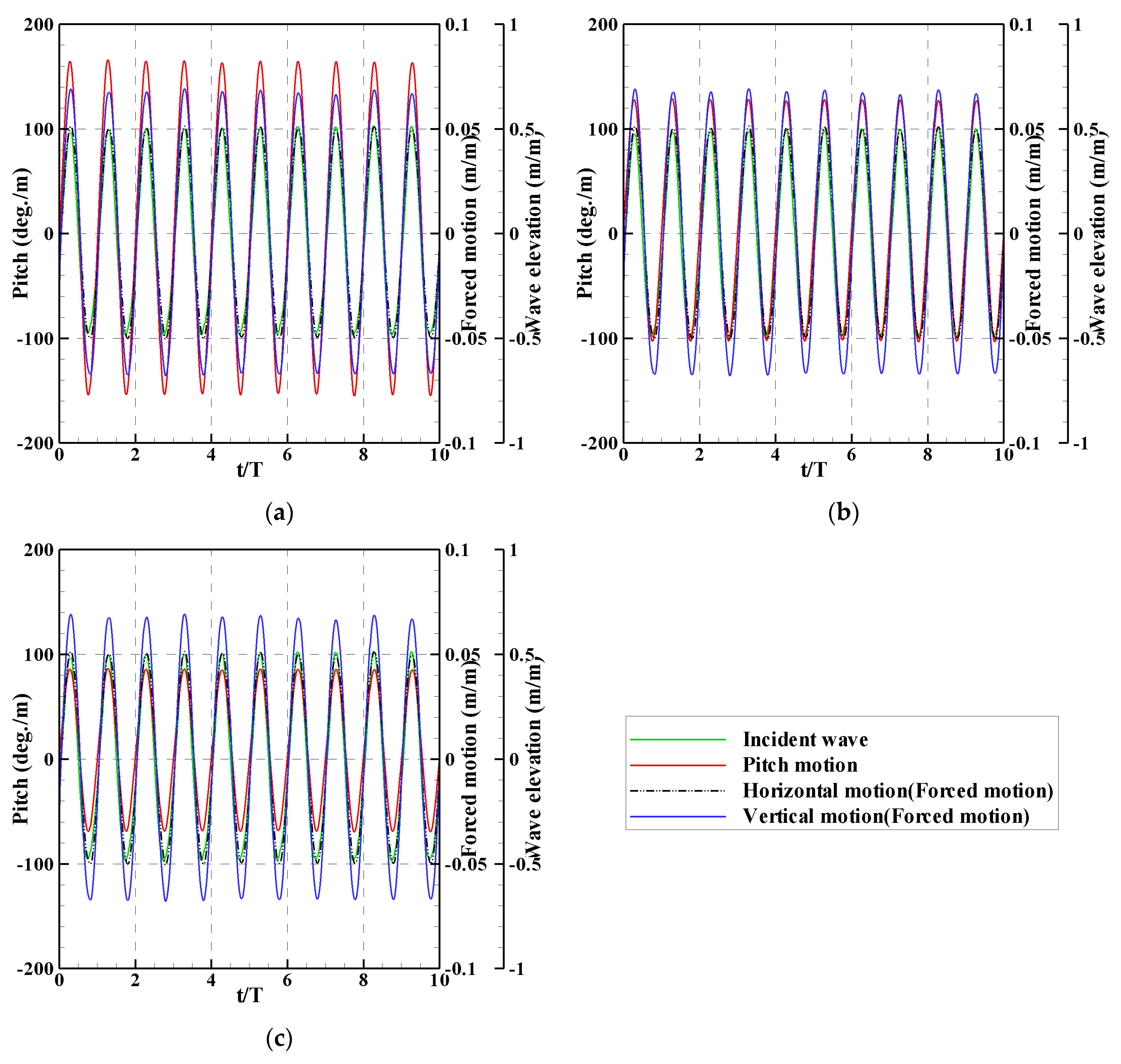
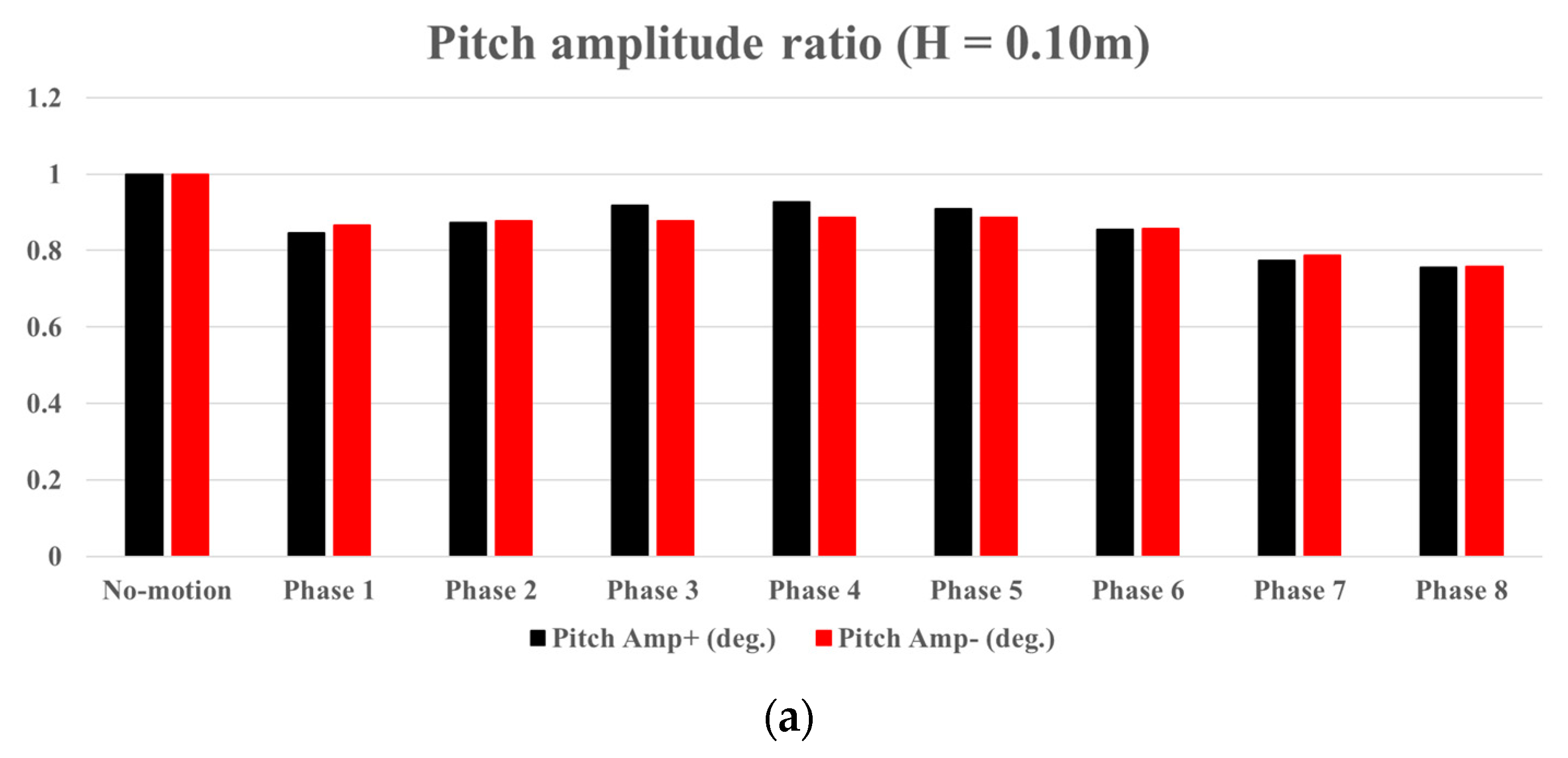
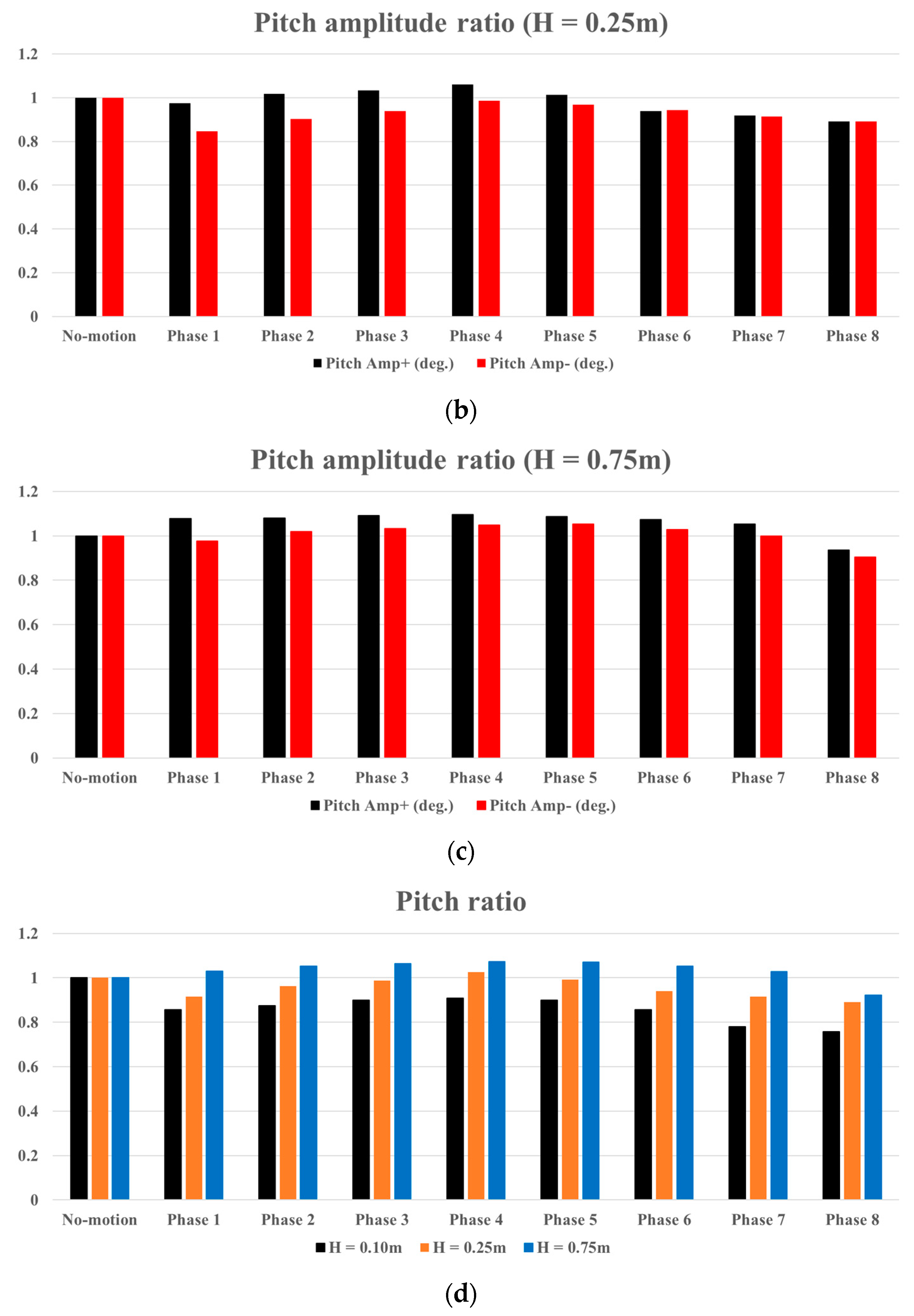
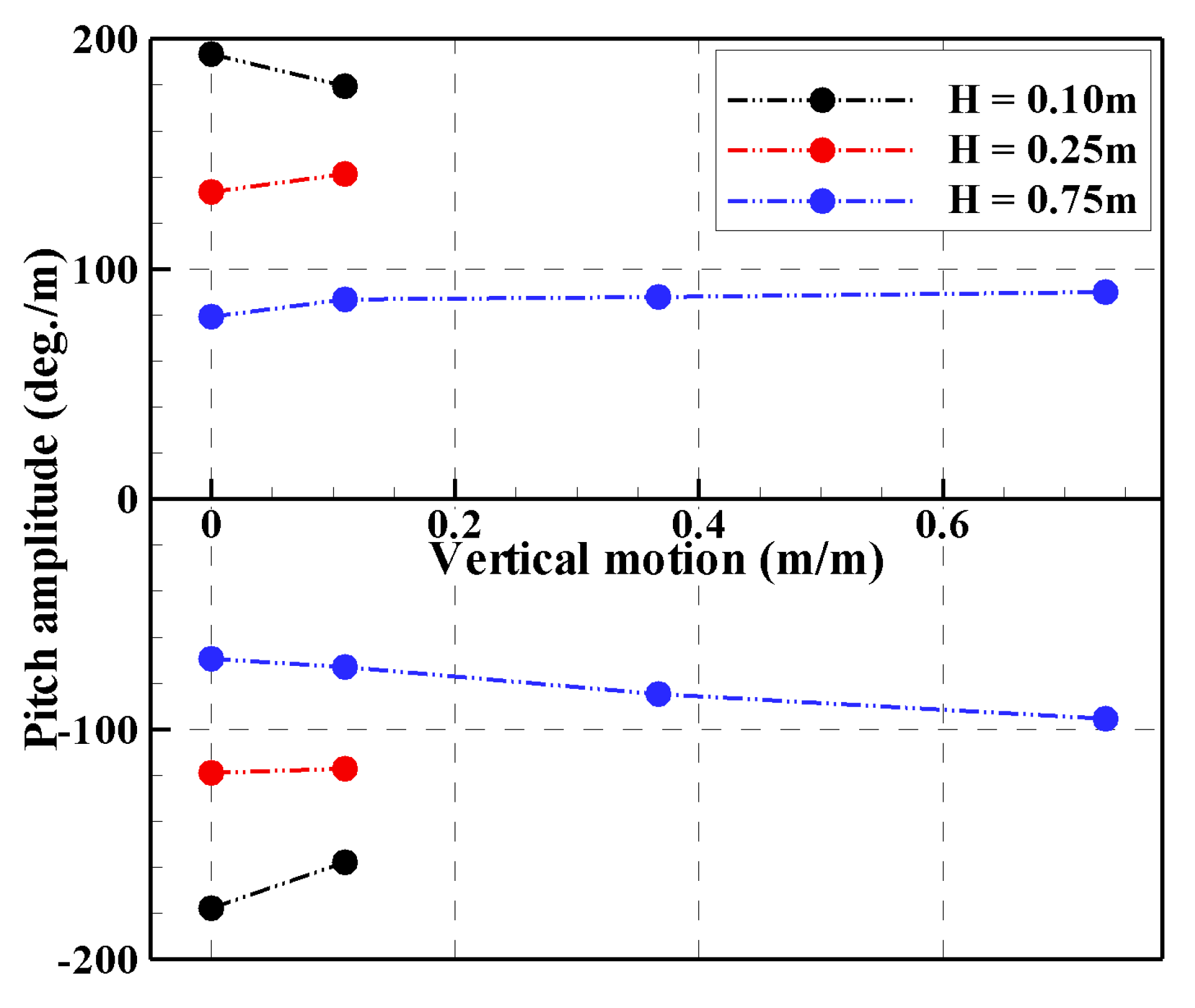
- In the absence of a PTO system, the largest pitch motion of the rotor with platform motion occurred during the Phase 4 stage across all wave heights. However, with the smallest wave height, the pitch motion of the rotor with platform motion was relatively small compared to that of the rotor without platform motion, while for other wave heights, the pitch motions of the rotor with platform motion increased compared to those without platform motion. The resulting variation in pitch motions could be attributed to changes in the added moment or GM of the rotor across different wave heights. This finding is consistent with [21].
- (2)
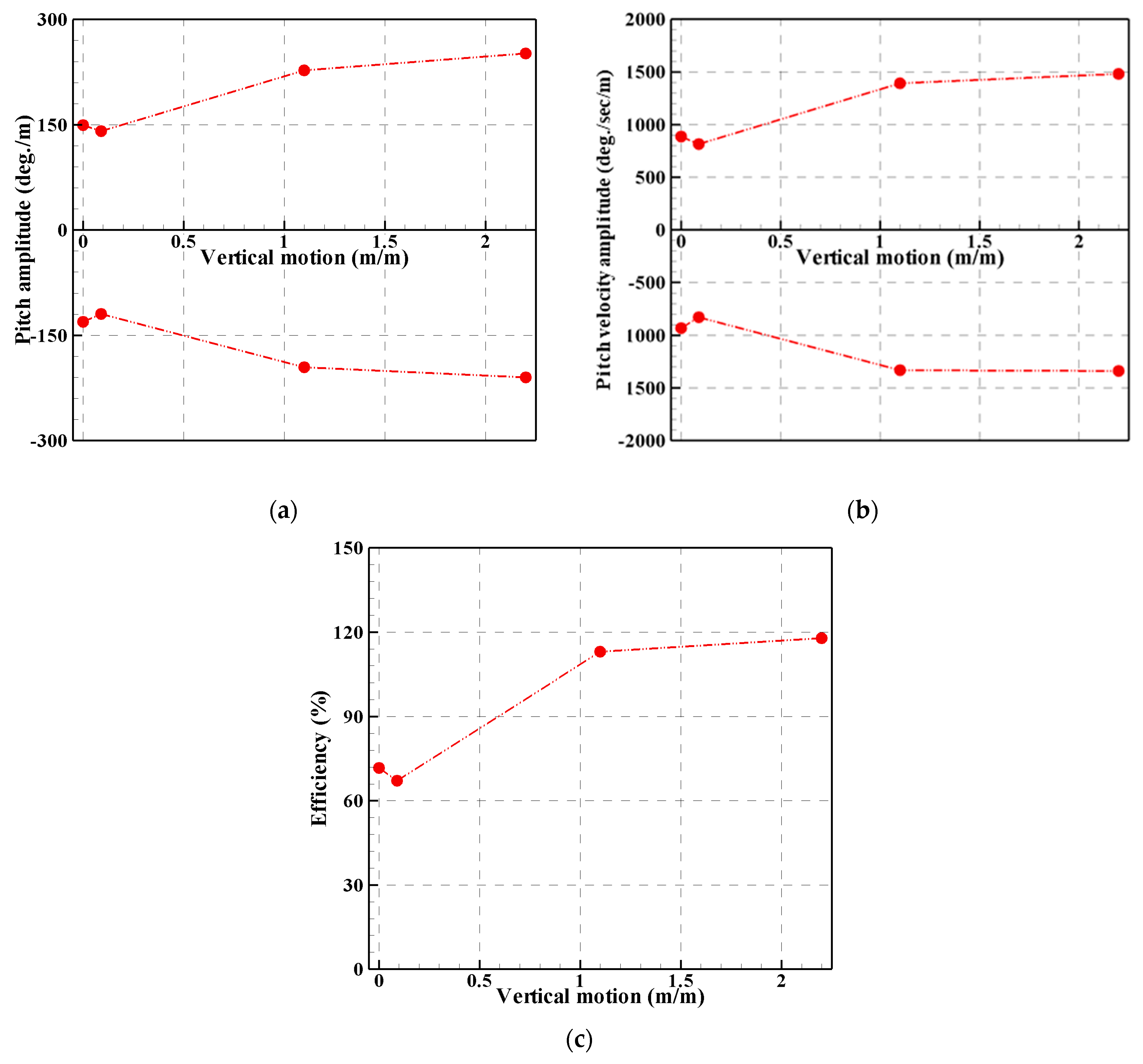
- In relation to the pitch motion of the rotor under significant platform motion, the trend of the rotor’s pitch motion at the wave height of 0.75 m seems to converge with increasing vertical motions of the platform. This convergence may be attributed to the limitation of the rotor’s rotating angle in both positive and negative directions, imposed by its buoyancy.
- (3)
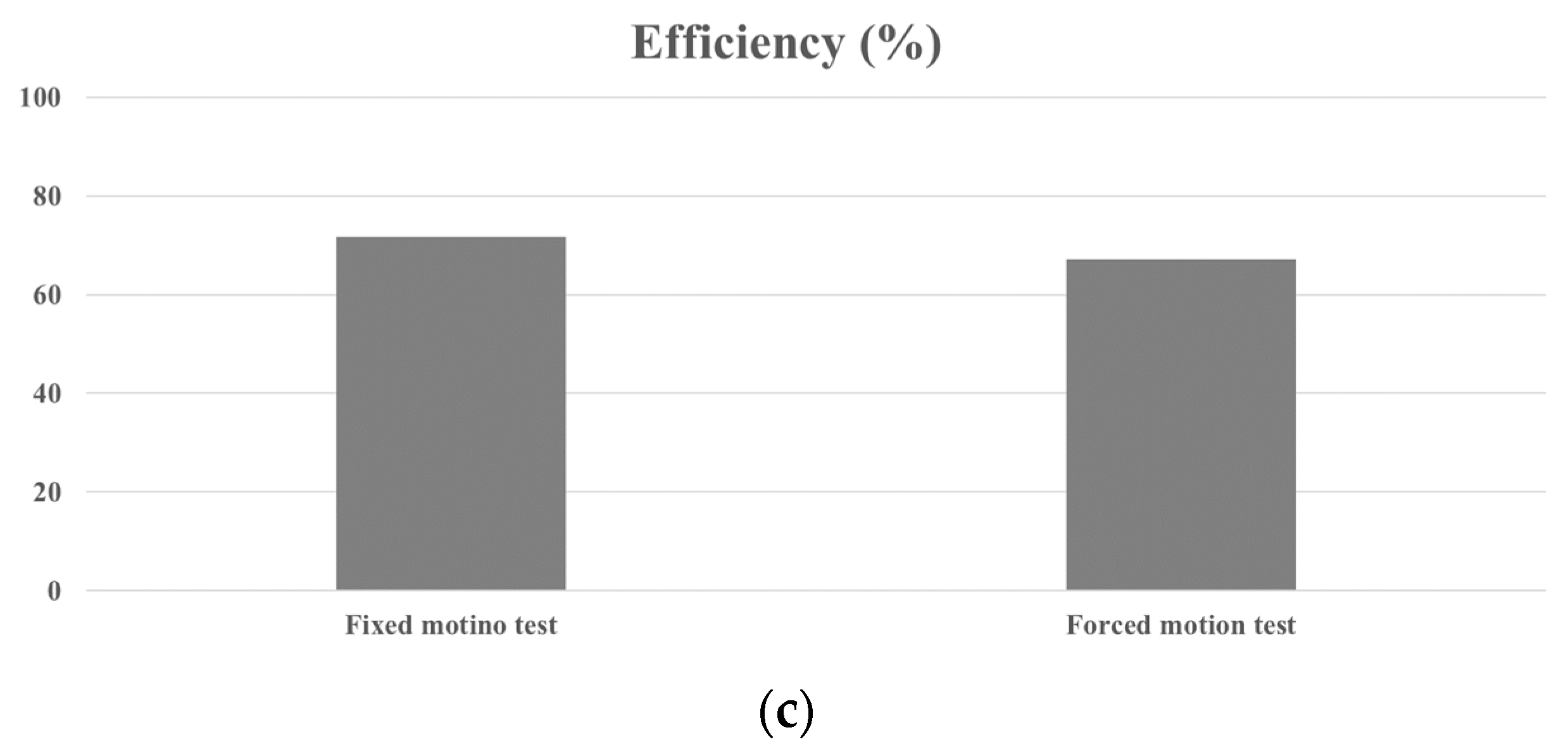
- In the presence of a PTO system, the efficiency of the rotor decreased with platform motion compared to the experiments without platform motion. The movement of the platform could alter the magnitude of the rotor’s pitch motion due to variations in the added moment or GM of the rotor. Consequently, the optimal PTO damping for a moving platform may differ from that for a stationary platform.
- (4)
- In the case of significant platform motion, the rotor’s efficiency increased due to the rotor’s inertia. Therefore, increasing the platform’s motion could be a viable method to enhance the absorbed power of the rotor during the design phase of a WEC rotor. Particularly, for the maximum platform motion, the rotor’s efficiency showed a slight increase compared to that at half the maximum platform motion.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yeung, R.W. Added Mass and Damping of a Vertical Cylinder in Finite-depth Waters. Appl. Ocean Res. 1981, 3, 119–133. [Google Scholar] [CrossRef]
- Evans, D.V.; Mciver, P. Added Mass and Damping of a Sphere Section in Heave. Appl. Ocean Res. 1984, 6, 45–53. [Google Scholar] [CrossRef]
- Budal, K.; Falnes, J. Wave Power Conversion by Point Absorbers: A Norwegian Project. Int. J. Ambient Energy 1982, 3, 59–67. [Google Scholar] [CrossRef]
- Cummins, W.E. The Impulse Response Function and Ship Motions. Schiffstechnik 1962, 47, 101–109. [Google Scholar]
- Falcão, A.F.O. Modelling and Control of Oscillating-body Wave Energy Converters with Hydraulic Power Take-off and Gas Accumulator. Ocean Eng. 2007, 34, 2021–2032. [Google Scholar] [CrossRef]
- Zurkinden, A.S.; Ferri, F.; Beatty, S.; Kofoed, J.P.; Kramer, M.M. Non-linear Numerical Modeling and Experimental Testing of a Point Absorber Wave Energy Converter. Ocean Eng. 2014, 78, 11–21. [Google Scholar] [CrossRef]
- Do, H.T.; Dinh, Q.T.; Nguyen, M.T.; Phan, C.B.; Dang, T.D.; Lee, S.; Park, H.G.; Ahn, K.K. Effects of Non-vertical Linear Motions of a Hemispherical-float Wave Energy Converter. Ocean Eng. 2015, 109, 430–438. [Google Scholar] [CrossRef]
- Gao, H.; Liang, R. Performance Analysis of Axisymmetric Floating Energy Harvesters and Influences of Parameters and Shape Variation. Int. J. Energy Res. 2018, 43, 2057–2074. [Google Scholar] [CrossRef]
- Salter, S.H. Wave Power. Nature 1974, 249, 720–724. [Google Scholar] [CrossRef]
- Mynett, A.E.; Serman, D.D.; Mei, C.C. Characteristics of Salter’s Cam for Extracting Energy from Ocean Waves. Appl. Ocean Res. 1979, 1, 13–20. [Google Scholar] [CrossRef]
- Rapuc, S. Numerical Study of the WEPTOS Single Rotor. Master Thesis, Aalborg University, Aalborg, Denmark, 2012. [Google Scholar]
- Pecher, A.; Kofoed, J.P.; Marchalot, T. Experimental Study on a Rotor for WEPTOS; Aalborg University DCE Contract Report No. 110; Aalborg University: Aalborg, Denmark, 2011. [Google Scholar]
- Poguluri, S.K.; Bae, Y.H. A Study on Performance Assessment of WEC Rotor in the Jeju Western Waters. Ocean Syst. Eng. 2018, 8, 361–380. [Google Scholar]
- Ko, H.S.; Kim, D.E.; Cho, I.H.; Bae, Y.H. Numerical and Experimental Study for Nonlinear Dynamic Behavior of an Asymmetric Wave Energy Converter. In Proceedings of the International Society of Offshore and Polar Engineering, Honolulu, HI, USA, 16–21 June 2019. [Google Scholar]
- Ha, Y.J.; Park, J.Y.; Shin, S.H.; Bae, Y.H. Evaluation of Generating Power in Low Wave Height for Asymmetric Rotor using Frequency Domain Analysis. J. Korean Soc. Mar. Environ. Energy 2019, 22, 253–261. [Google Scholar] [CrossRef]
- Margheritini, L.; Kofoed, J.P. WEPTOS Wave Energy Converters to Cover the Energy Needs of a Small Island. Energies 2019, 12, 423. [Google Scholar] [CrossRef]
- Yazdi, H.; Ghafari, H.R.; Ghassemi, H.; He, G.; Karimirad, M. Wave Power Extraction by Multi-Salter’s Duck WECs Arrayed on the Floating Offshore Wind Turbine Platform. Energy 2023, 278, 127930. [Google Scholar] [CrossRef]
- Park, J.Y.; Nam, B.W.; Shin, S.H.; Park, I.B.; Won, Y.U.; Oh, Y.J.; Roh, C.; Cho, S.J. An Experimental Study on the Wave Response of Arrayed-buoy Wave Energy Converter Changing Platform Included Angle. In Proceedings of the Autumn Meetings of Korean Society for Marine Environmental and Energy, Yeosu, Republic of Korea, 22 November 2018. [Google Scholar]
- Ha, Y.J.; Park, J.Y.; SHIN, S.H. Numerical Study on Non-linear Dynamic Behavior of an Asymmetric Rotor for Wave Energy Converter in Regular Waves. Processes 2021, 9, 1477. [Google Scholar] [CrossRef]
- Hong, K.Y.; Ryu, H.J.; Shin, S.H.; Hong, S.W. Wave Energy Distribution at Jeju Sea and Investigation of Optimal Sites for Wave Power Generation. J. Ocean Eng. Technol. 2004, 18, 8–15. [Google Scholar]
- Ha, Y.J.; Park, J.Y.; Rho, C.; Shin, S.H. An Experimental Study for the Non-linear Motion Characteristics of an Asymmetric Rotor by Pontoons. J. Korean Soc. Mar. Environ. Energy 2020, 23, 192–202. [Google Scholar] [CrossRef]

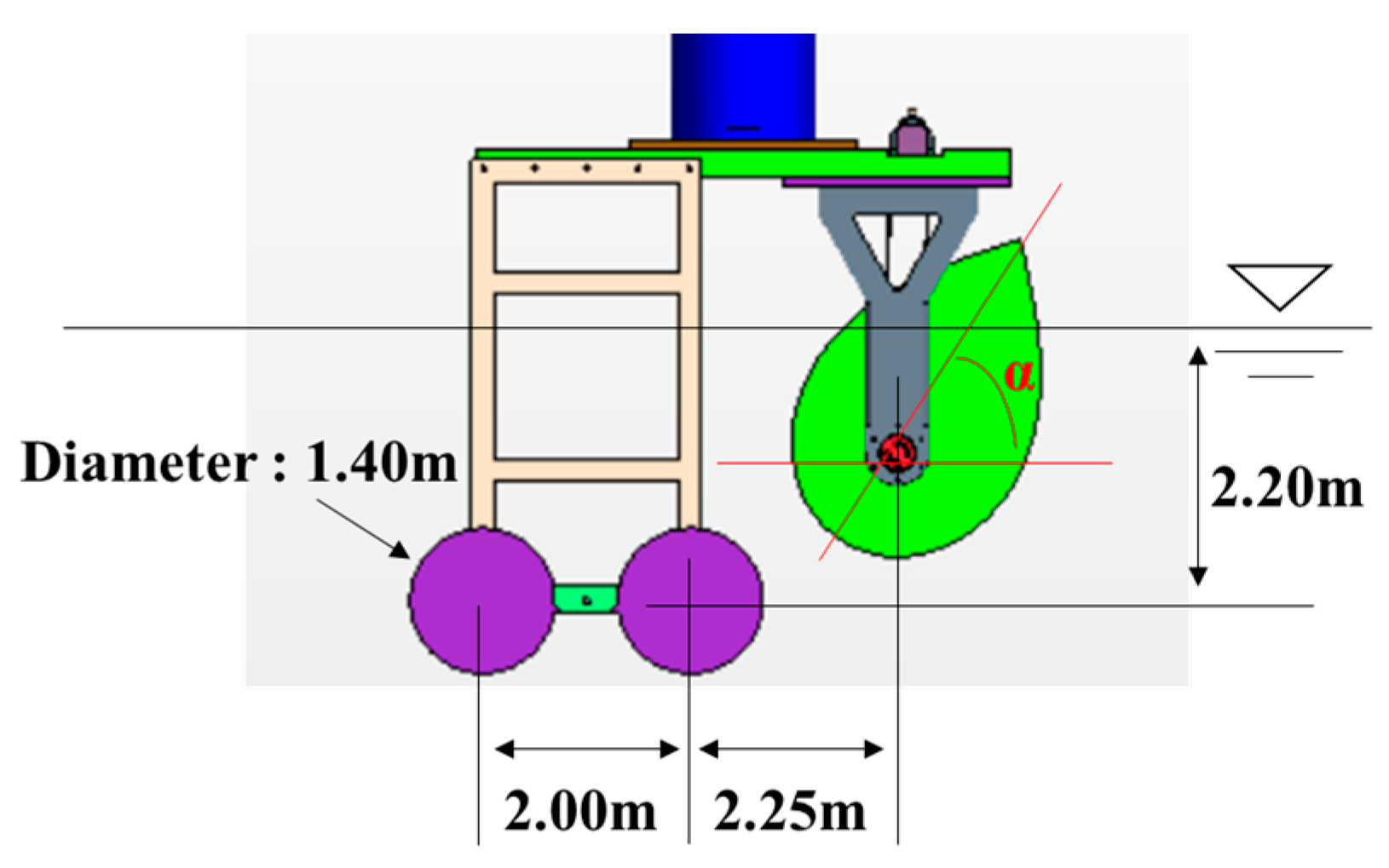





| Unit | ||
|---|---|---|
| Beak angle (α) | [deg.] | 65 |
| Radius of stern | [m] | 1.0 |
| Draft (Rotating center) | [m] | 0.8 |
| Width | [m] | 2.5 |
| Mass | [kg] | abt. 7000 |
| CGx | [m] | −0.1388 |
| CGz | [m] | 0.1623 |
| Ixx | [kg·m2] | 9710 |
| Iyy | [kg·m2] | 6791 |
| Izz | [kg·m2] | 7227 |
| Condition | Wave Period [s] | Wave Height [m] | Wave Heading [deg.] |
|---|---|---|---|
| Without PTO damping | 4.75 | 0.10, 0.25, 0.75 | 180 |
| With PTO damping | 4.25 | 0.25 |
| Condition | Wave Period [s] | Horizontal Motion Height [m/m] | Vertical Motion Height [m/m] |
|---|---|---|---|
| Without PTO damping | 4.75 | 0.11 | 0.14 |
| With PTO damping | 4.25 | 0.19 | 0.09 |
| Wave Height [m] | Phase Stage | Positive Amplitude [%] | Negative Amplitude [%] | Magnitude [%] |
|---|---|---|---|---|
| 0.10 | 1 | 84.6 | 86.7 | 85.6 |
| 2 | 87.3 | 87.7 | 87.5 | |
| 3 | 91.9 | 87.8 | 89.9 | |
| 4 | 92.8 | 88.7 | 90.8 | |
| 5 | 91.0 | 88.7 | 89.9 | |
| 6 | 85.5 | 85.7 | 85.6 | |
| 7 | 77.4 | 78.8 | 78.1 | |
| 8 | 75.5 | 75.8 | 75.7 | |
| 0.25 | 1 | 97.6 | 84.7 | 91.5 |
| 2 | 101.8 | 90.2 | 96.3 | |
| 3 | 103.4 | 93.8 | 98.9 | |
| 4 | 106.0 | 98.5 | 102.5 | |
| 5 | 101.3 | 96.7 | 99.1 | |
| 6 | 93.9 | 94.4 | 94.1 | |
| 7 | 91.8 | 91.4 | 91.6 | |
| 8 | 89.1 | 89.1 | 89.1 | |
| 0.75 | 1 | 107.7 | 97.6 | 103.0 |
| 2 | 108.1 | 101.9 | 105.2 | |
| 3 | 109.2 | 103.3 | 106.4 | |
| 4 | 109.5 | 105.0 | 107.4 | |
| 5 | 108.6 | 105.3 | 107.1 | |
| 6 | 107.4 | 102.9 | 105.3 | |
| 7 | 105.3 | 99.9 | 102.8 | |
| 8 | 93.5 | 90.5 | 92.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ha, Y.-J.; Lim, C.-H.; Shin, S.-H.; Park, J.-Y. Experimental Study on Performance of a Wave Energy Converter Rotor with a Moving Platform. Energies 2024, 17, 2736. https://doi.org/10.3390/en17112736
Ha Y-J, Lim C-H, Shin S-H, Park J-Y. Experimental Study on Performance of a Wave Energy Converter Rotor with a Moving Platform. Energies. 2024; 17(11):2736. https://doi.org/10.3390/en17112736
Chicago/Turabian StyleHa, Yoon-Jin, Chang-Hyuck Lim, Seung-Ho Shin, and Ji-Yong Park. 2024. "Experimental Study on Performance of a Wave Energy Converter Rotor with a Moving Platform" Energies 17, no. 11: 2736. https://doi.org/10.3390/en17112736
APA StyleHa, Y.-J., Lim, C.-H., Shin, S.-H., & Park, J.-Y. (2024). Experimental Study on Performance of a Wave Energy Converter Rotor with a Moving Platform. Energies, 17(11), 2736. https://doi.org/10.3390/en17112736






