Multi-Physical Field Analysis and Optimization Design of the High-Speed Motor of an Air Compressor for Hydrogen Oxygen Fuel Cells
Abstract
1. Introduction
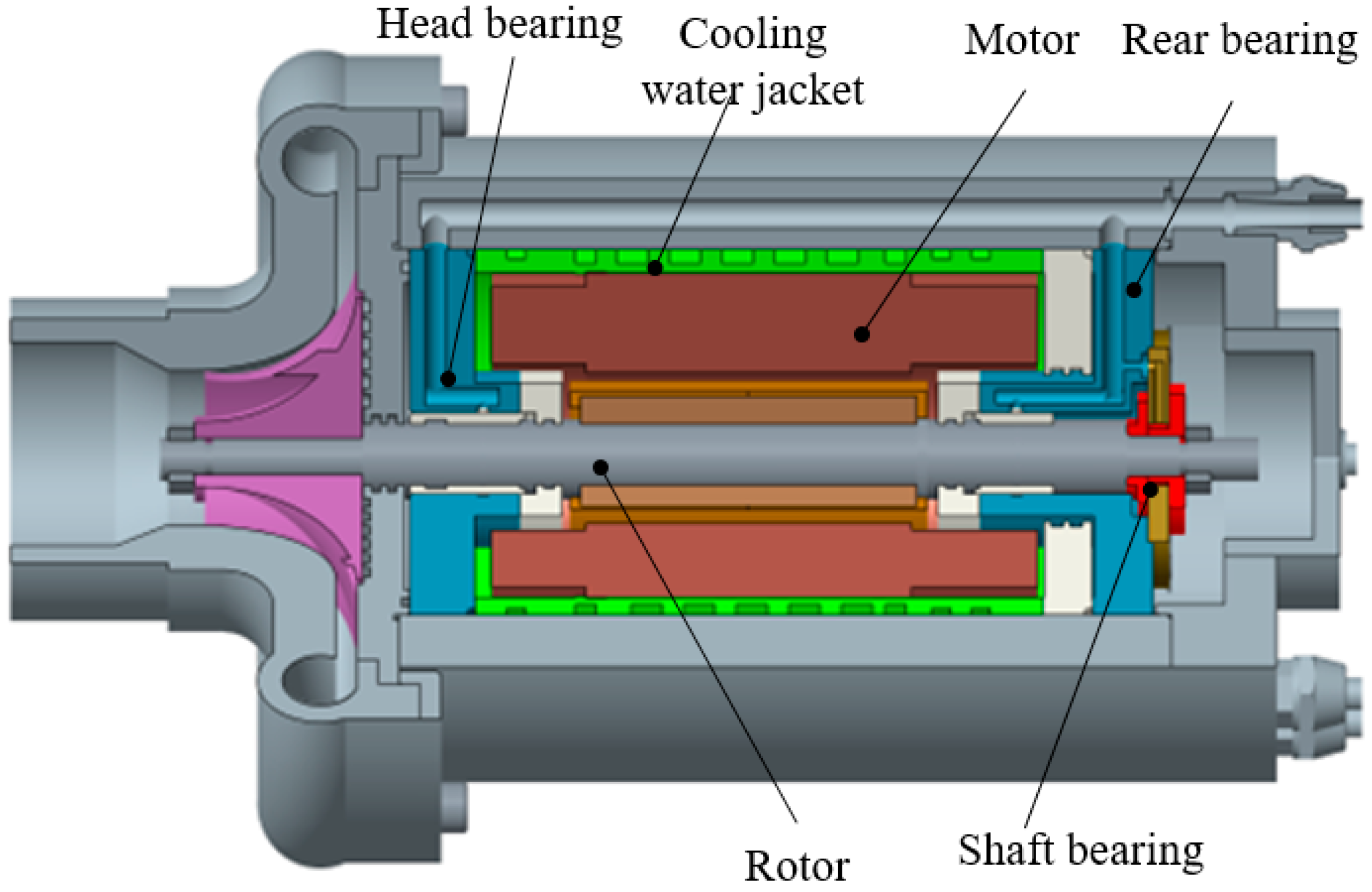
2. Motor Structure of Air Compressor
3. Optimization of Motor Performance
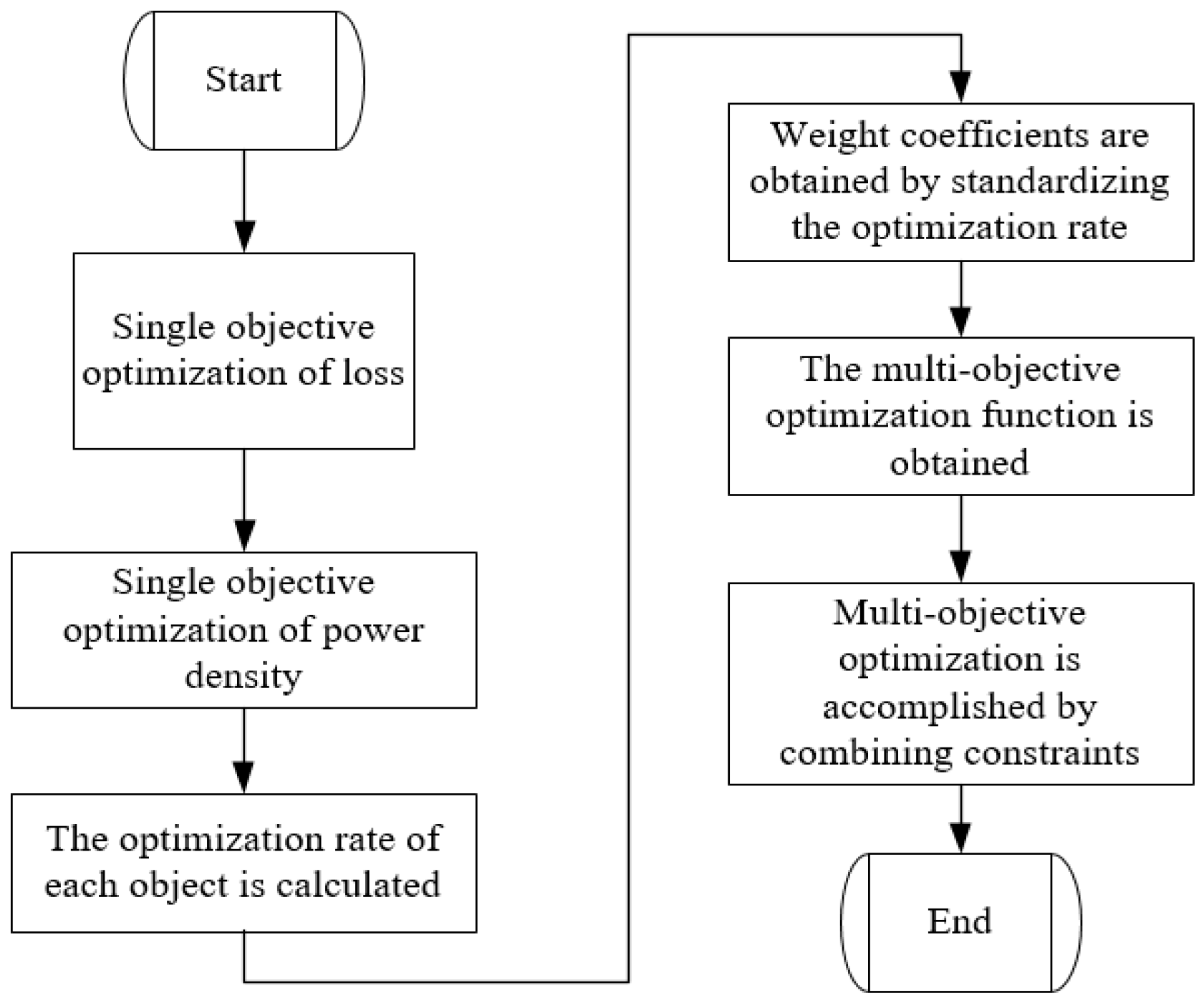
3.1. Optimization Algorithm
3.2. Optimization Objectives and Design Variables
3.2.1. Design Variables
3.2.2. Optimization Objectives
- (1)
- Output power constraints
- (2)
- Current constraints
- (3)
- Stiffness constraint
- (4)
- Strength constraint
3.3. Optimization Results
4. Multi Physical Field Analysis of Motor
4.1. Calculation of Loss
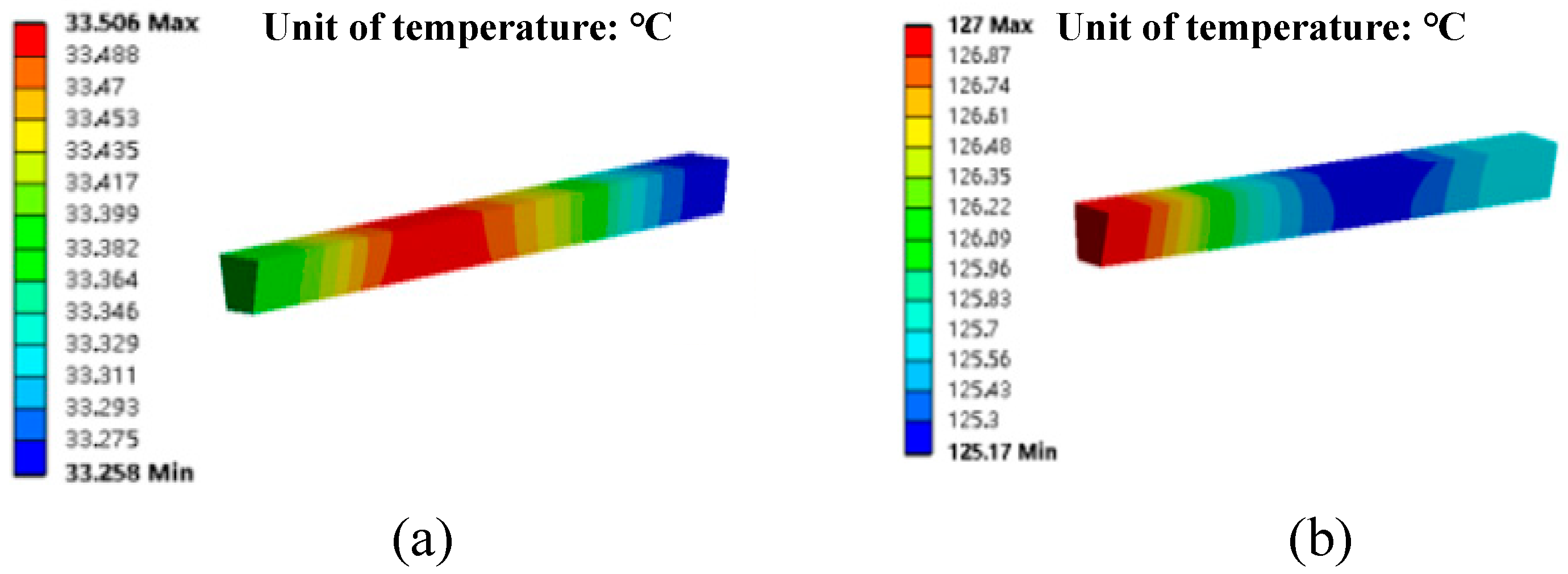
4.2. Stress Analysis of Rotor
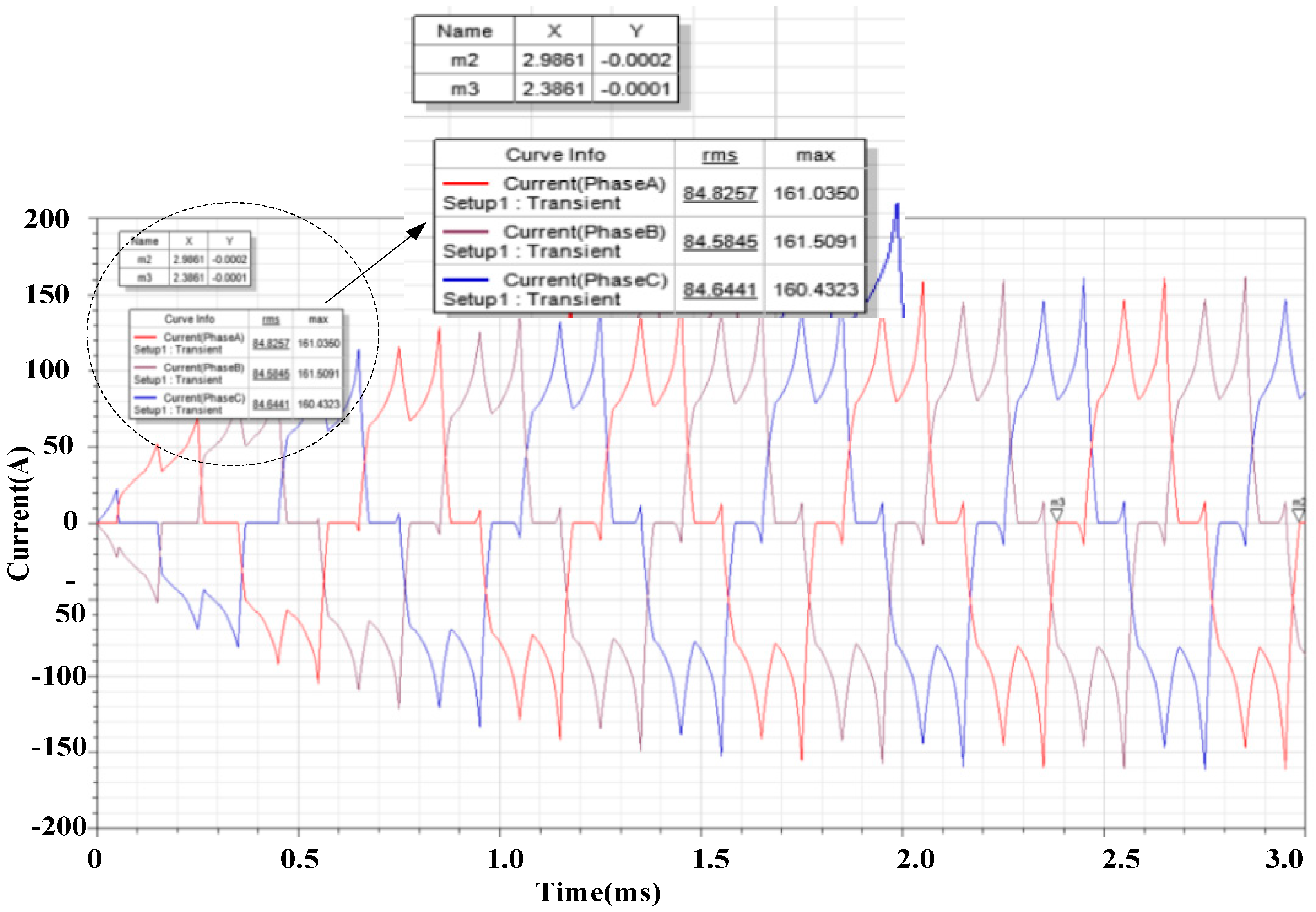
4.3. Magnetic Field Analysis
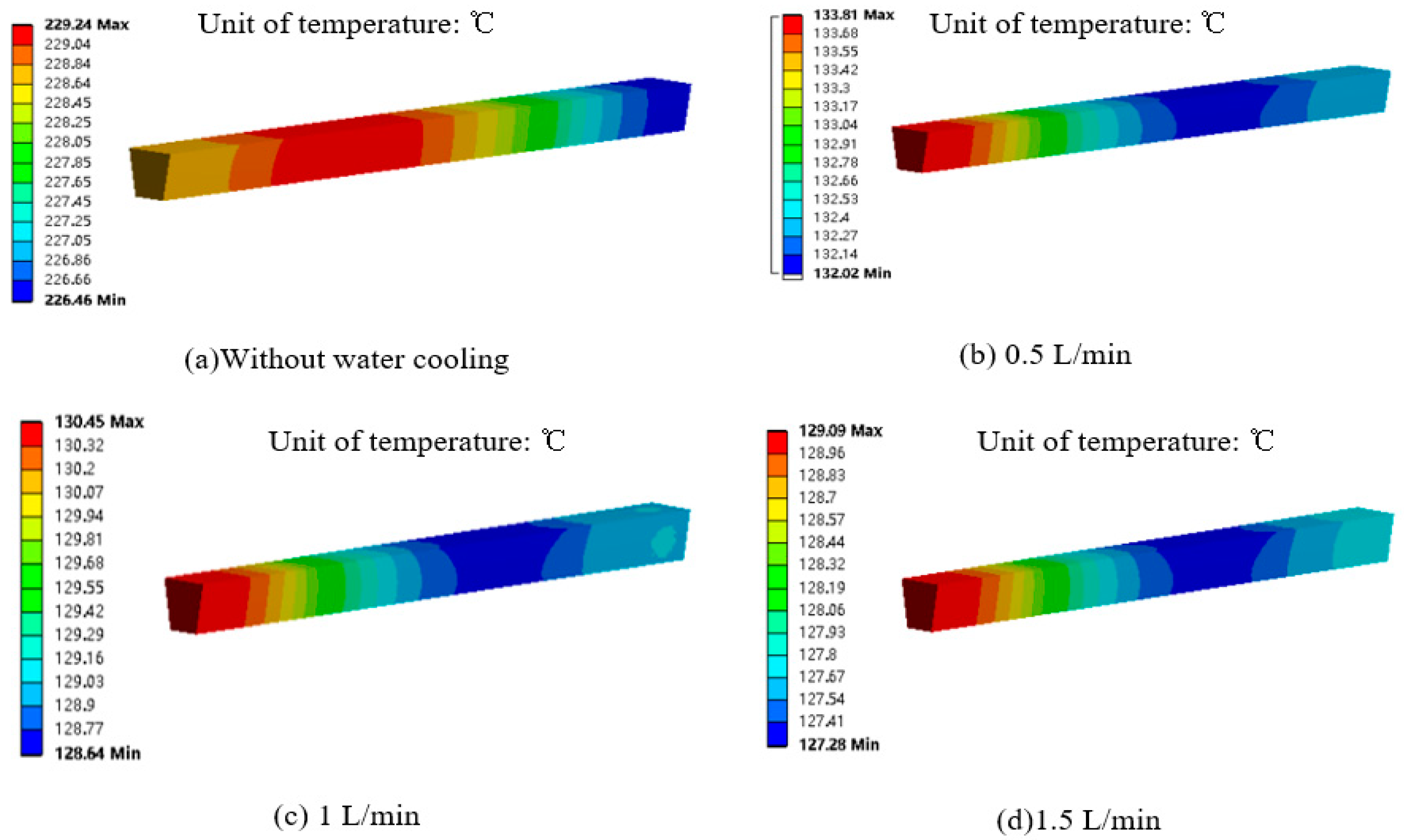
5. Temperature Field and Water-Cooling Analysis
5.1. Temperature Field Analysis
- (1)
- Determination of the heat transfer coefficient of the outer surface of the shell and the end cover. There are two kinds of heat transfer in the fluid: convection and conduction, and the heat exchange caused by these two kinds of heat transfer is called convective heat transfer. In the heating process of the motor, there is convective heat transfer between the casing and the outside air, the sheath and the air gap, and the end cover and the outside air, so it is necessary to determine the convective heat transfer coefficient of the heat exchange surface.
- (2)
- Determination of the heat transfer coefficient of the outer surface of the rotor
- (3)
- Determination of thermal conductivity
5.2. Effect of Cooling Water on Motor Temperature
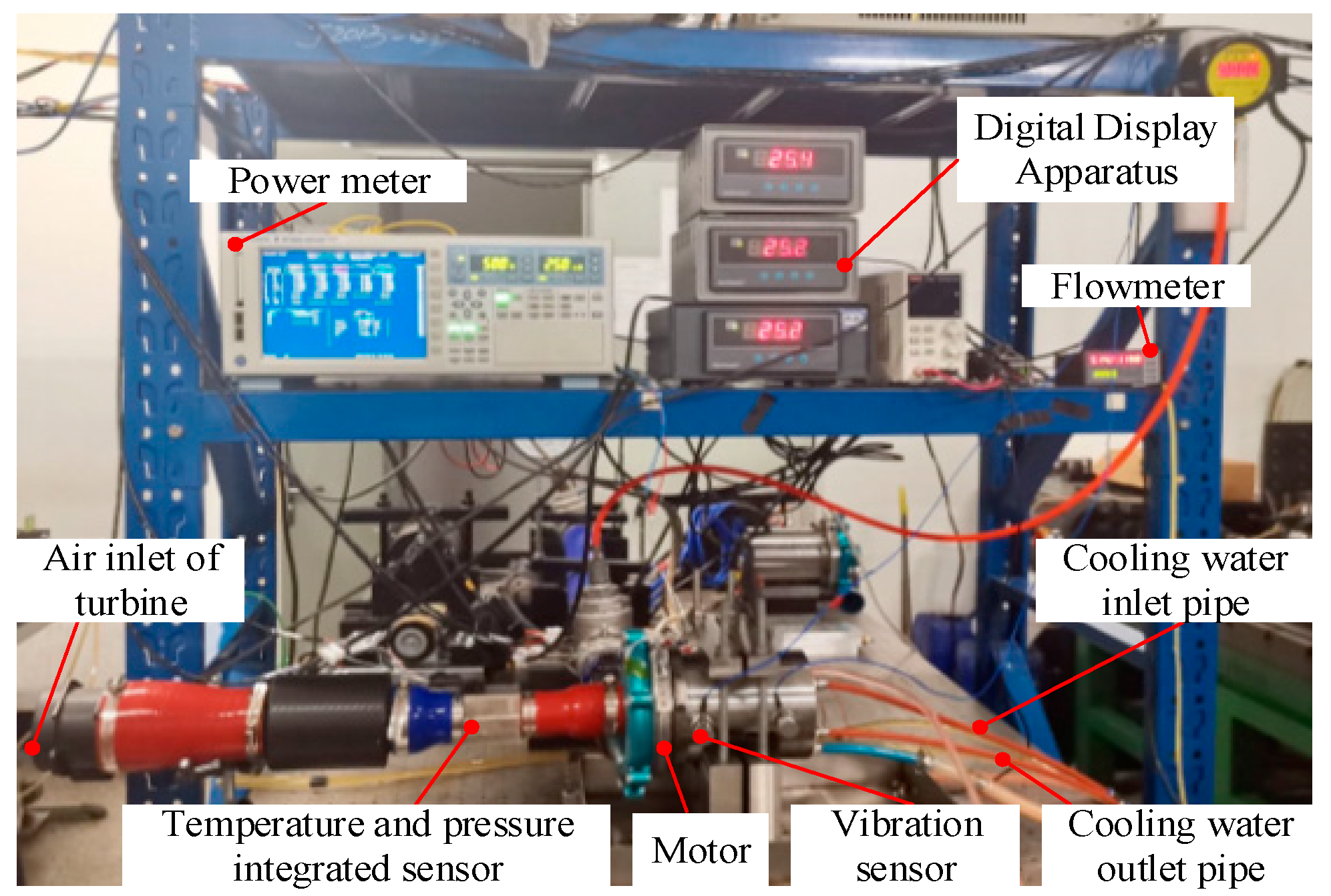
6. Experiments
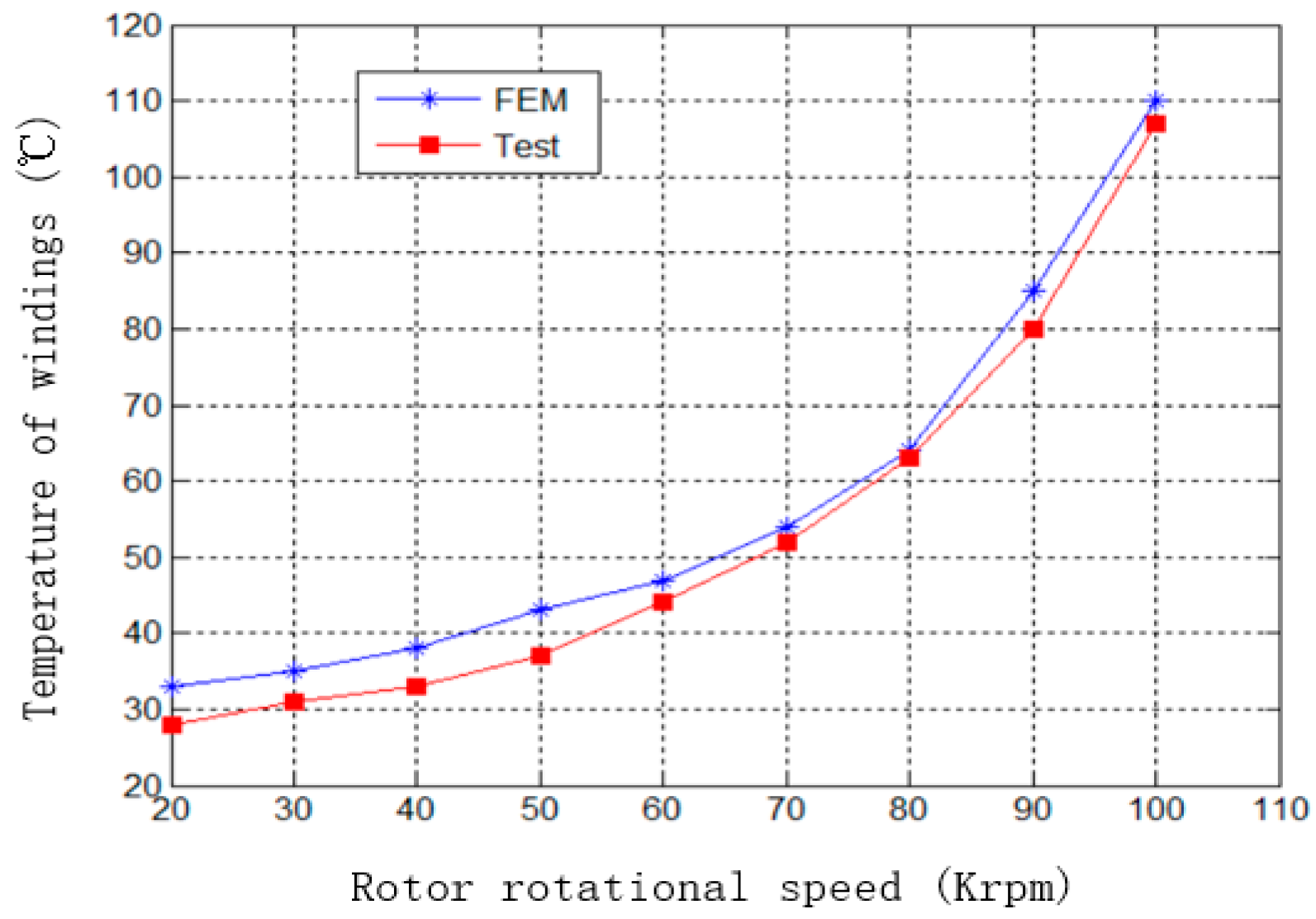
6.1. Test of Temperature Rising
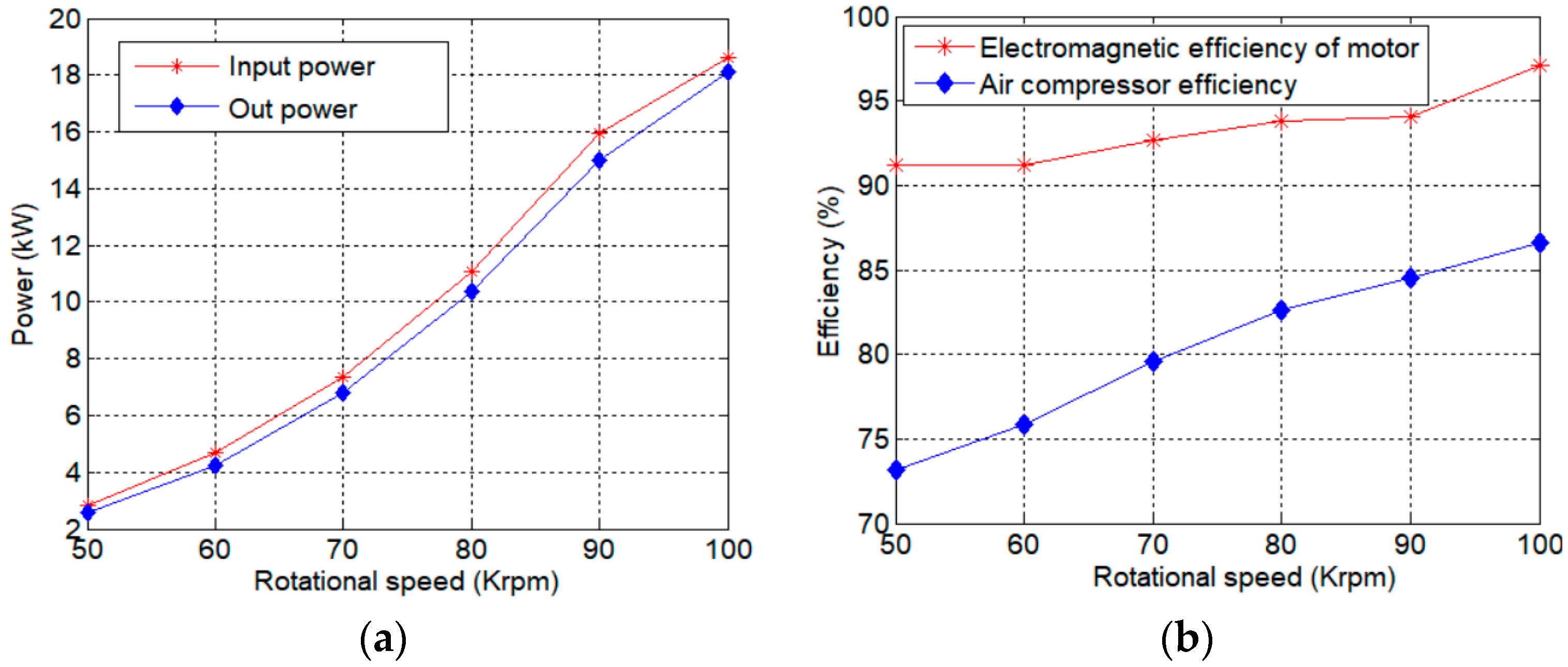
6.2. Test of Motor Efficiency
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, Y.; Zhu, C.; Wu, L.; Zheng, Y. Multi-Objective Optimal Design of High-Speed Surface-Mounted Permanent Magnet Synchronous Motor for Magnetically Levitated Flywheel Energy Storage System. IEEE Trans. Magn. 2019, 55, 8202708. [Google Scholar] [CrossRef]
- Zhao, J.; Gu, Z.; Li, B.; Liu, X.; Li, X.; Chen, Z. Research on the torque and back EMF performance of a high speed PMSM used for flywheel energy storage. Energies 2015, 8, 2867–2888. [Google Scholar] [CrossRef]
- Hong, D.; Hwang, H. Design, Analysis, and Experimental Validation of a Permanent Magnet Synchronous Motor for Articulated Robot Applications. IEEE Trans. Magn. 2018, 54, 8201304. [Google Scholar] [CrossRef]
- Kim, J.H.; Kim, D.M.; Jung, Y.-H.; Lim, M.-S. Design of Ultra-High-Speed Motor for FCEV Air Compressor Considering Mechanical Properties of Rotor Materials. IEEE Trans. Energy Convers. 2021, 36, 2850–2860. [Google Scholar] [CrossRef]
- Chasiotis, I.D.; Karnavas, Y.L. A Generic Multi-Criteria Design Approach Toward High Power Density and Fault-Tolerant Low-Speed PMSM for Pod Applications. IEEE Trans. Transp. Electr. 2019, 5, 356–370. [Google Scholar] [CrossRef]
- Chen, Y.; Ding, Y.; Zhuang, J.; Zhu, X. Multi-Objective Optimization Design and Multi-Physics Analysis a Double-Stator Permanent-Magnet Doubly Salient Machine. Energies 2018, 11, 2130. [Google Scholar] [CrossRef]
- Zhang, Y.; McLoone, S.; Cao, W. High speed permanent magnet motor design and power loss analysis. In Proceedings of the 2017 IEEE Transportation Electrification Conference and Expo, Asia-Pacific (ITEC Asia-Pacific), Harbin, China, 7–10 August 2017; pp. 1–6. [Google Scholar]
- Park, S.H.; Lee, E.-C.; Park, J.-C.; Hwang, S.-W.; Lim, M.-S. Prediction of Mechanical Loss for High-Power-Density PMSM Considering Eddy Current Loss of PMs and Conductors. IEEE Trans. Magn. 2021, 57, 6300205. [Google Scholar] [CrossRef]
- Sun, Y.; Shi, S.; Li, Y.; Wang, Q. Development of quantitative structure-activity relationship models to predict potential nephrotoxic ingredients in traditional Chinese medicines. Food Chem. Toxicol. 2019, 128, 163–170. [Google Scholar] [CrossRef]
- Tang, X.; Zhang, Y.; Xu, S. Temperature sensitivity characteristics of PEM fuel cell and output performance improvement based on optimal active temperature control. Int. J. Heat Mass Transf. 2023, 206, 123966. [Google Scholar] [CrossRef]
- Milewski, J.; Ryś, P.; Krztoń-Maziopa, A.; Żukowska, G.; Majewska, K.; Zybert, M.; Kowalczyk, J.; Siekierski, M. Structural Investigation of Orthoborate-Based Electrolytic Materials for Fuel Cell Applications. Energies 2024, 17, 2097. [Google Scholar] [CrossRef]
- Lu, Z.; Yan, Y. Temperature Control of Fuel Cell Based on PEI-DDPG. Energies 2024, 17, 1728. [Google Scholar] [CrossRef]
- Mun, J.; Choi, K.K.; Kim, D.H. System-Level Robust Design Optimization of a Permanent Magnet Motor Under Design Parameter Uncertainties. In Proceedings of the 2022 IEEE 20th Biennial Conference on Electromagnetic Field Computation (CEFC), Denver, CO, USA, 24–26 October 2022; pp. 1–2. [Google Scholar]
- Zhang, Y.; Xu, J.; Han, Z.; Wu, Z.; Huang, C.; Li, M. System-Level Optimization Design of Tubular Permanent-Magnet Linear Synchronous Motor for Electromagnetic Emission. In Proceedings of the 2021 13th International Symposium on Linear Drives for Industry Applications (LDIA), Wuhan, China, 1–3 July 2021; pp. 1–4. [Google Scholar] [CrossRef]
- Liu, Y.X.; Li, L.Y. The Optimization Design of Short-Term High-Overload Permanent Magnet Motors Considering the Nonlinear Saturation. Energies 2018, 11, 3272. [Google Scholar] [CrossRef]
- Wu, J.; Zhu, X.; Xiang, Z.; Fan, D.; Quan, L.; Xu, L. Robust Optimization of a Rare-Earth-Reduced High-Torque-Density PM Motor for Electric Vehicles Based on Parameter Sensitivity Region. IEEE Trans. Veh. Technol. 2022, 71, 10269–10279. [Google Scholar] [CrossRef]
- Lei, G.; Wang, T.; Zhu, J.; Guo, Y.; Wang, S. System-Level Design Optimization Method for Electrical Drive Systems—Robust Approach. IEEE Trans. Ind. Electron. 2015, 62, 4702–4713. [Google Scholar] [CrossRef]
- Neto, M.G.; da Silva, F.F.; Branco, P.J.d.C. Operational Analysis of an Axial and Solid Double-Pole Configuration in a Permanent Magnet Flux-Switching Generator. Energies 2024, 17, 1698. [Google Scholar] [CrossRef]
- Shuhua, F.; Huan, L. High Power Density PMSM with Lightweight Structure and High-Performance Soft Magnetic Alloy Core. IEEE Trans. Appl. Supercond. 2019, 29, 0602805. [Google Scholar]
- Huang, Z.; Fang, J.; Liu, X.; Han, B. Loss Calculation and Thermal Analysis of Rotors Supported by Active Magnetic Bearings for High-Speed Permanent-Magnet Electrical Machines. IEEE Trans. Ind. Electron. 2016, 63, 2027–2035. [Google Scholar] [CrossRef]
- Wang, W.; Tang, Q.; Xu, X.; Gong, S. Finite element analysis of normal contact stiffness between real rough surfaces based on ANSYS. In Proceedings of the 2012 Fourth International Conference on Computational and Information Sciences (ICCIS), Chongqing, China, 17–19 August 2012; pp. 127–130. [Google Scholar]
- Ng, K.; Zhu, Z.Q.; Howe, D. Open-circuit field distribution in a brushless motor with diametrically magnetised PM rotor, accounting for slotting and eddy current effects. IEEE Trans. Magn. 1996, 32, 5070–5072. [Google Scholar] [CrossRef]
- Aubry, J.; Ahmed, H.B.; Multon, B. Sizing Optimization Methodology of a Surface Permanent Magnet Machine-Converter System Over a Torque-Speed Operating Profile: Application to a Wave Energy Converter. IEEE Trans. Ind. Electron. 2012, 59, 2116–2125. [Google Scholar] [CrossRef]
- Jędryczka, C.; Knypiński, Ł.; Demenko, A.; Sykulski, J.K. Methodology for Cage Shape Optimization of a Permanent Magnet Synchronous Motor Under Line Start Conditions. IEEE Trans. Magn. 2018, 54, 3. [Google Scholar] [CrossRef]
- Mohammad, H.M.; Rodrigo, C.P. Finding Optimal Performance Indices of Synchronous AC Motors. IEEE Trans. Magn. 2017, 53, 8104004. [Google Scholar] [CrossRef]
- Han, B.; Xu, Q.; Yuan, Q. Multiobjective Optimization of a Combined Radial-Axial Magnetic Bearing for Magnetically Suspended Compressor. IEEE Trans. Ind. Electron. 2015, 63, 2284–2293. [Google Scholar] [CrossRef]
- Tong, W.; Sun, R.; Zhang, C.; Wu, S.; Tang, R. Loss and Thermal Analysis of a High-Speed Surface-Mounted PMSM With Amorphous Metal Stator Core and Titanium Alloy Rotor Sleeve. IEEE Trans. Magn. 2019, 55, 8102104. [Google Scholar] [CrossRef]
- Zhang, C.; Chen, L.; Wang, X.; Tang, R. Loss Calculation and Thermal Analysis for High-Speed Permanent Magnet Synchronous Machines. IEEE Access 2020, 8, 92627–92636. [Google Scholar] [CrossRef]
- Dong, B.; Wang, K.; Han, B.; Zheng, S. Thermal Analysis and Experimental Validation of a 30 kW 60,000 r/min High-Speed Permanent Magnet Motor with Magnetic Bearings. IEEE Access 2019, 7, 92184–92192. [Google Scholar] [CrossRef]






| Name | Parameters | Value Range |
|---|---|---|
| d0 | Outer diameter of PM, mm | 28 ≤ d0 ≤ 32 |
| d1 | Inner diameter of PM, mm | 20 ≤ d1 ≤ 30 |
| d2 | Outer diameter of stator, mm | 80 ≤ d2 ≤ 94 |
| lsle | Thickness of rotor sleeve, mm | 2 ≤ lsle ≤ 5 |
| lgap | Length of air gap, mm | 1 ≤ lgap ≤ 4 |
| lax | Axial length of motor, mm | 6 ≤ lax ≤ 10 |
| Name | Value | Name | Value |
|---|---|---|---|
| Rotor topology | Surface- mounted PM | Connection configuration of Windings | Double layer/star connection |
| Outer diameter of stator | 94 mm | Core length | 8 mm |
| Inner diameter of stator | 45 mm | Pitch | 5 |
| Outer diameter of magnetic steel | 32 mm | Number of parallel branches | 2 |
| Length of air gap | 4 mm | Number of conductors per slot | 10 |
| Axial length of motor | 8 mm | Inner diameter of magnetic steel | 20 mm |
| Outer diameter of sheath | 37 mm | slot filling rate | 0.8 |
| Sleeve material | TC11 | Number of stator slots | 12 |
| Number of parallel branches | 2 | Number of pole pairs | 1 |
| Nominal diameter of wire | 0.63 mm | Magnet material | NdFeB |
| Heat Source | Loss (W) | Volume (m3) | Heat Generation Rate (W/m3) |
|---|---|---|---|
| Windings | 255 | ||
| Stator core | 152 | ||
| Magnetic steel | 143 |
| Component | Density (kg/m3) | Heat Capacity C () | Thermal Conductivity λ (W/mK) |
|---|---|---|---|
| Equivalent insulation in slots | 780 | ||
| Windings (copper) | 8933 | ||
| Waterproof jacket (PEEK) | 1300 | ||
| Sleeve (TC11) | 4480 | 600 | 6.3 |
| Stator (10JNEX900) | 7650 | ||
| Magnetic steel | 7400 | ||
| Support elements (4Cr13) | 7750 | ||
| Water | 997 | ||
| Air | 1.185 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, X.; Feng, M.; Liu, J.; Du, R. Multi-Physical Field Analysis and Optimization Design of the High-Speed Motor of an Air Compressor for Hydrogen Oxygen Fuel Cells. Energies 2024, 17, 2722. https://doi.org/10.3390/en17112722
Ren X, Feng M, Liu J, Du R. Multi-Physical Field Analysis and Optimization Design of the High-Speed Motor of an Air Compressor for Hydrogen Oxygen Fuel Cells. Energies. 2024; 17(11):2722. https://doi.org/10.3390/en17112722
Chicago/Turabian StyleRen, Xiaojun, Ming Feng, Jinliang Liu, and Rui Du. 2024. "Multi-Physical Field Analysis and Optimization Design of the High-Speed Motor of an Air Compressor for Hydrogen Oxygen Fuel Cells" Energies 17, no. 11: 2722. https://doi.org/10.3390/en17112722
APA StyleRen, X., Feng, M., Liu, J., & Du, R. (2024). Multi-Physical Field Analysis and Optimization Design of the High-Speed Motor of an Air Compressor for Hydrogen Oxygen Fuel Cells. Energies, 17(11), 2722. https://doi.org/10.3390/en17112722






