Power Components Mean Values Determination Using New I_p-I_q Method for Transients

Abstract

This paper deals with the quasi-instantaneous determination (in a single-step response time) of apparent, active, and reactive (i.e., blind and distortion) power mean values including the total power factor, total harmonic distortion, and phase shift of fundamentals of a power electronic and electrical system (PEES) using the ip-iq method, which is the main contribution of the paper. The power components’ mean values are investigated during the transient and steady states. The power components’ mean values can be determined directly from phase current and voltage quantities, using an integral calculus over one period within the next calculation step and using moving average and moving rms techniques (or digital filtering). Consequently, the power factor can be evaluated with known values of a phase shift of fundamentals (using a Fourier analysis). The results of this study show how a distortion power component during transients is generated even under a harmonic supply and linear resistive–inductive load. The paper contains a theoretical base, modeling, and simulation for the three and single phases of the transients in power electronic systems. The worked-out results can be used to determine and size any PES. The presented approach brings a detailed time waveform verified by simulations in Matlab/Simulink 2022a and the Real-time HW Simulator Plecs RT Box 1.

1. Introduction

It is known that in power system theory, by now the instantaneous reactive power theory (IRP) raised by Akagi, Kanazawa, and Nabae, and Czarnecki’s currents’ physical component theory (CPC) based on the Budeanu method, are the most widely used [1,2,3]. Both line disturbances and the features of the connected appliances have an impact on the quality of the electrical energy that is extracted from the network. We can list a variety of power quality indices and events among the first ones, such as flicker, sags, transients, and slot harmonics. Reactive power compensation devices, particularly active power filters, have effectively utilized their application. Appliances, on the other hand, have the ability to overload the network with a variety of waveforms, non-linearities, and higher harmonics. For instance, the third harmonic current produced by switched-mode power supplies, which are often used in television sets, personal computers, etc., is almost the same size (80–90%) as the fundamental frequency component. In power systems, the range of harmonic sources can be represented by these load types taken together. Note that even seemingly small adjustments to control strategies and parameter settings can have a big influence on the creation of a harmonic current. Only the active power component is physically significant in an AC power network; the other components, apparent, active, blind, and distortion, make up the electric power features [4,5]. The apparent power is the sum of the active, blind, and distortion powers. Its size needs to be considered in the design because it is transferred from the source to the load. The measurement of blind and/or distortion power is related with certain challenges and cannot be accomplished using a conventional ’sinusoidal´ wattmeter (power meter). However, the active power may be determined and measured rather easily. The active power can be represented by the scalar product of the voltage and current phasors, and the blind power by the vector product of them, when rotating phasors of instantaneous time waveforms of voltages and currents are substituted in complex plains [6,7]. It is necessary to know the phase displacement between phasors in order to use this calculus. Determining the performance power factor’s value is likewise related to this. Although both approaches have been modified, the majority of methods for an instantaneous computation of power components as a function of time in steady states are described in the previously mentioned literature [3]. However, in transient conditions, it is frequently necessary to ascertain the average value of the apparent, active, blind, and distortion power components. As a result, the study in this paper deals with the calculation of the mean value of each power component in a single step in the transient states of PEES, but it does not use Akagi’s p-q theory as in [8]. Numerous studies [9,10,11,12] explain transient phenomena and Fourier analysis, particularly in power electrical and electronic systems. The Fourier transform is a helpful technique for transient solutions centered on harmonic analysis, as is made evident in [8]. However, during transient situations, power component mean values are not solved in any of ref. [1,2,3,4,5,6,7].

This paper introduces the advanced power theory of electrical systems with non-sinusoidal voltages and currents, based on the concept of current physical components (CPCs), and compares it to the instantaneous reactive power theory (IRP) based on the Clarke transform [13]. CPCs includes single- and three-phase systems with linear, time-invariant (LTI), and harmonic generating loads. Work [14] brings a new physical interpretation of the reactive power, while work [15] describes physical phenomena that affect the effectiveness of the power components’ transfer. A present point of view on the instantaneous reactive power theory is given in [16]. Unlike the id-iq method [17,18] that works in a rotary coordinate system, the ip-iq method works with a stationary coordinate system.

Unfortunately, no one from the mentioned works [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18] solves the problem of an average value of apparent, active, blind, and distortion power components in transient states.

In the article, there are the following four sections (besides the introduction), including:

-

The ip-iq method for the determination of the apparent, active, blind, and distortion powers’ mean values during transients under the harmonic supply and linear LTI load;

-

The determination of the power components’ mean values during transients under the harmonic supply and non-linear load and under transient conditions caused by a step change in the load;

-

The determination of the power components’ mean values during transients under the nonharmonic supply and LTI load and under transient conditions caused by a switch-over decreased load;

-

The application of the ip-iq method for a three-phase power system under steady and transient states in Matlab/Simulink;

-

A modeling and real-time (RT) simulation for single- and three-phase supply systems under different steady and transient conditions using a HIL Simulator Plecs RT Box [19];

-

A discussion of each mode of operation, also to time the waveform of each power component during transient, and a conclusion.

2. Ip-Iq Method Used for Apparent, Active, Blind, and Distortion Power Components

The aim is to determine individual components of power as we know them from the classical power theory [2,4]. So

• $S_{av}—$proportional to $U_{rms}\times I_{rms}$,
• $P_{av}—$proportional to $U_{rms}\times I_{p,rms}$,
• $Q_{av}—$proportional to ($U_{rms}\times I_{q,rms}$),
• $D_{av}—$proportional to ($U_{rms}\times I_{\sum ,rms}$),
• and consequently
• $PF—$as ratio of $P_{av}$/$S_{av}$,
• THD—as ratio of $D_{av}$/$S_{av}$,

and those as quasi-instantaneous quantities in any k-steps.

So, the first goal is the determination of the $P_{av} \mathrm{a}\mathrm{n}\mathrm{d} Q_{av}$ components using ip-iq currents. Based on definitional relationships, the instantaneous power is given as [4]

$$s\left(t\right)=u\left(t\right)i\left(t\right)$$

(1)

where $u\left(t\right)=U_{max}\mathrm{s}\mathrm{i}\mathrm{n}\left(\omega t\right)$ and $i\left(t\right)$ is the calculated current course.

And for its mean value

$$S_{av}=U_{rms}{I}_{rms}.$$

(2)

Each harmonic waveform can be decomposed into a sine and cosine component. So, for the harmonic supply and linear RL load

$$i\left(t\right)=I_{max}\mathrm{s}\mathrm{i}\mathrm{n}\left(\omega t-\phi \right)=i_p\left(t\right)+i_q\left(t\right)=I_p\mathrm{s}\mathrm{i}\mathrm{n}\left(\omega t\right)+I_q\mathrm{c}\mathrm{o}\mathrm{s}\left(\omega t\right)$$

(3)

where

$$I_{max}=\frac{U_{max}}{\left|Z\right|}; \left|Z\right|=\sqrt{R^2+{\left(\omega L\right)}^2} ;I_p=I_{max}\mathrm{s}\mathrm{i}\mathrm{n}\left(\frac{\pi }{2}-\phi \right) \mathrm{a}\mathrm{n}\mathrm{d} I_q=I_{max}\mathrm{s}\mathrm{i}\mathrm{n}\left(0-\phi \right).$$

(4)

Similarly, for the power components

$$s\left(t\right)=u\left(t\right)\left[i_p\left(t\right)+i_q\left(t\right)\right]=p\left(t\right)+q\left(t\right).$$

(5)

A graphic presentation is shown in Figure 1.

Thus, similarly as $S_{av}$ in (2)

$$P_{av}=U_{rms}{I}_{p,rms}$$

(6a)

and

$$Q_{av}=U_{rms}{I}_{q,rms}.$$

(6b)

Then the distortion power mean value (if exists) will be

$$D_{av}=\sqrt{S_{av}^2-P_{av}^2-Q_{av}^2}.$$

(7)

Although the $P_{av}, Q_{av}, D_{av}$ power components are significant electrical quantities in PEES systems, determining their instantaneous size during transients is not so simple, as we can see in the next sections.

During Transients

Since the input voltage is shifted by an angle $\alpha$

$$u\left(t\right)=U_m\mathrm{s}\mathrm{i}\mathrm{n}\left({\omega }_{1}t+\alpha \right)$$

(8)

then

$$i_{RL}\left(t\right)=I_m\left[\mathrm{c}\mathrm{o}\mathrm{s}\left({\omega }_{1}t+\alpha -{\phi }_1\right)-\mathrm{c}\mathrm{o}\mathrm{s}\left(\alpha -{\phi }_1\right)e^{-t/{\tau }_1}\right]+I_0{e}^{-t/{\tau }_1}.$$

(9a)

$$i_{RL}\left(t\right)=I_m\left[\mathrm{s}\mathrm{i}\mathrm{n}\left({\omega }_{1}t+\alpha -{\phi }_1\right)-\mathrm{s}\mathrm{i}\mathrm{n}\left(\alpha -{\phi }_1\right)e^{-t/{\tau }_1}\right]+I_0{e}^{-t/{\tau }_1}.$$

(9b)

where $I_m=\frac{U_m}{Z}$ and $\alpha$ is a voltage shift and $I_0$ is the initial value of the current.

Transient components of $i_{RL}\left(t\right)$ are

$${I_m\mathrm{c}\mathrm{o}\mathrm{s}\left(\alpha -{\phi }_1\right)e}^{-t/{\tau }_1}+I_0{e}^{-t/{\tau }_1} .$$

(9c)

$$I_{p,rms}\left(k\right)\iff \sqrt{\frac{1}{T}\underset{0}{\overset{T}{\int }}{i}_p\left(t\right)\mathrm{d}t} \mathrm{a}\mathrm{n}\mathrm{d} I_{q,rms}\left(k\right)=\sqrt{\frac{1}{T}\underset{0}{\overset{T}{\int }}{i}_q\left(t\right)\mathrm{d}t},$$

(10)

Using the $movRMS$ function, we can calculate the RMS value of the currents both during the transient and steady state, Figure 2.

In generally, in the case of non-harmonic current,

$$I_{rms}=\sqrt{I_{1,rms}^2+\sum _{n=2}^{\infty }{I}_{n,rms}^2}$$

(11)

But $I_{1,rms}\left(k\right)$ can be determined by [8], where there are more ways to do it:

-

Using continuous-time filter;

-

Using digital filtering;

-

Using integral calculus;

-

Using a discrete Fourier transform;

-

Using artificial neural networks [20].

The use of the continuous-time filter seems to be the fastest way to process a signal, but it requires the use of auxiliary hardware for conversion and processing (DAC-ADC converter, multiplier, integrator, etc.). So, the time of calculation also depends on the settling time, time of conversion of DAC converter, and soon. The last two items are not directly bound to the ip-iq method.

By this, we can use Fourier coefficients for the maximal value calculation of ip-iq currents and their time waveforms.

The first term in Equation (11)

$$I_{rms}\left(t\right)=\sqrt{\frac{1}{T}\underset{0}{\overset{T}{\int }}{i}^2\left(t\right)\mathrm{d}t} \iff \mathrm{u}\mathrm{s}\mathrm{i}\mathrm{n}\mathrm{g} movRMS\iff I_{rms}\left(k\right)$$

(12a)

or calculating the sliding window using the square or (more exactly) meander rule

$$I_{rms}\left(k\right)=\sqrt{\frac{1}{N}\left[\frac{i_0^2+i_N^2}{2}\sum _{k=1}^{N-1}{i}^2\left(k\right)\right]}.$$

(12b)

And the first term under sqrt in (11)

$$I_{1,rms}\left(t\right)=\sqrt{\frac{1}{T}\underset{0}{\overset{T}{\int }}{i}_1^2\left(t\right)\mathrm{d}t} \iff \mathrm{u}\mathrm{s}\mathrm{i}\mathrm{n}\mathrm{g} movRMS\iff I_{1,rms}\left(k\right).$$

(12c)

So, for single power components, we can write:

For active power

$$\begin{gathered} P_{av}\left(t\right)=\frac{1}{T}\underset{0}{\overset{T}{\int }}u\left(t\right)i_p\left(t\right)\mathrm{d}t=\sqrt{\frac{1}{T}\underset{0}{\overset{T}{\int }}{u}^2\left(t\right)i_p^2\left(t\right)\mathrm{d}t}\iff \mathrm{u}\mathrm{s}\mathrm{i}\mathrm{n}\mathrm{g} movRMS\iff \begin{array}{c}{P}_{av}\left(k\right)\end{array} \\ =U_{rms}\left(k\right)I_{p,rms}\left(k\right). \end{gathered}$$

(13)

For other power components analogically:

For blind reactive power

$$Q_{av}\left(k\right)=U_{rms}\left(k\right)I_{q,rms}\left(k\right)$$

(14a)

although

$$Q_{\left(1\right)av}\left(t\right)\iff \frac{1}{T}\underset{0}{\overset{T}{\int }}{U_{max}\mathrm{s}\mathrm{i}\mathrm{n}\left(\omega t\right)I}_{1q,max}\mathrm{c}\mathrm{o}\mathrm{s}\left(\omega t\right)=0!$$

(14b)

For apparent power

$$S_{av}\left(k\right)=U_{rms}\left(k\right)I_{rms}\left(k\right)$$

(15a)

It can be also calculated as

$${\tilde{S}}_{av}\left(k\right)=\sqrt{P_{av}^2\left(k\right)+Q_{av}^2\left(k\right)}$$

(15b)

which is, in steady state, the same.

Since the distortion reactive power is, in steady state, equal to zero, distortion power in the transient can be determined as

$$D_{av}\left(k\right)=U_{rms}\left(k\right)I_{d,rms}\left(k\right)=U_{rms}\left(k\right)\sqrt{I_{rms}^2\left(k\right)-I_{1,rms}^2\left(k\right)}$$

(16a)

And it can also be calculated as

$$D_{av}\left(k\right)=\sqrt{S_{av}^2\left(k\right)-P_{av}^2\left(k\right)-Q_{av}^2\left(k\right)}$$

(16b)

In practical simulation or implementation, the distortion power component $D_{av}\left(k\right)$, however, is not zero due to the different calculated time of $I_{rms}\left(k\right)$ and $I_{p,rms}\left(k\right)$ or $I_{q,rms}\left(k\right)$, respectively. The principal time waveforms of the power components S, P, Q, D (k) by using the movRMS method are shown in both transient states’ start-up (a) and recovery (b), Figure 3.

As can be seen from the figure, the apparent power (black) is not equal to the square of the sum of the active and reactive ones (blue dashes). Let us name the difference between these power components the fictitious distortion component (purple).

Let us note, in this regard, using the Fourier transform on Equation (9a) for the total transient current waveform

$$i_{RL}\left(t\right)=\frac{U_m}{Z}\left[\mathrm{s}\mathrm{i}\mathrm{n}\left({\omega }_{1}t+\alpha -{\phi }_1\right)-{\mathrm{s}\mathrm{i}\mathrm{n}\left(\alpha -{\phi }_1\right)e}^{-t/{\tau }_1}\right]+I_0{e}^{-t/{\tau }_1}$$

which consists of steady and transient components (two-times $e^{-t/{\tau }_1}$). Since the equation consists of steady- and transient-state components, we can write

$$\begin{gathered} F_{i_{RL}}\left(j\omega \right)=F_{st-st}\left(j\omega \right)+F_{trans}\left(j\omega \right) \\ =\frac{U_m}{Z}\underset{0}{\overset{\infty }{\int }}\mathrm{c}\mathrm{o}\mathrm{s}\left({\omega }_{1}t-{\phi }_1\right)e^{-j\omega t}\mathrm{d}t+\frac{U_m}{Z}\underset{0}{\overset{\infty }{\int }}\mathrm{c}\mathrm{o}\mathrm{s}\left(-{\phi }_1\right)e^{-t/{\tau }_1}{e}^{-j\omega t}\mathrm{d}t. \end{gathered}$$

(17)

Taking into account Tabs in [8], the Fourier transform of the $i_{RL}\left(t\right)$ function can be derived (a simplified approach [8]), namely, using the time-shift rule

$$f\left(t\pm t_1\right) \leftrightarrow e^{j\omega t_1}\mathcal{F}\left\{f\left(t\right)\right\}$$

(18)

where $t_1=\frac{{\phi }_1}{{\omega }_1}$.

Then, for the steady-state component

$$\begin{gathered} F_{st-st}\left(j\omega \right)=e^{j{\phi }_1}{\mathcal{F}}_{st-st}\left\{u\left(t\right)\mathrm{c}\mathrm{o}\mathrm{s}{\omega }_{1}t\right\}=\frac{U_m}{Z}{e}^{j{\phi }_1}{\mathcal{F}}_{st-st}\left\{\frac{1}{2}\left[e^{j{\omega }_{1}t}+e^{-j{\omega }_{1}t}\right]\right\}=\frac{U_m}{Z}\frac{1}{2}{e}^{j{\phi }_1}\left\{F_u\left[j\left(\omega -{\omega }_1\right)\right]+F_u\left[j\left(\omega +{\omega }_1\right)\right]\right\} \\ =\frac{U_m}{Z}\frac{1}{2}{e}^{j\omega \frac{{\phi }_1}{{\omega }_1}}\left[\pi \delta \left(\omega -{\omega }_1\right)+\frac{1}{j\left(\omega -{\omega }_1\right)}+\pi \delta \left(\omega +{\omega }_1\right)+\frac{1}{j\left(\omega +{\omega }_1\right)}\right] \\ =\frac{U_m}{Z}\left\{\frac{\pi }{2}\left[\delta \left(\omega -{\omega }_1\right)+\delta \left(\omega +{\omega }_1\right)\right]e^{j{\phi }_1}+\frac{j\omega }{\left({{\omega }_1}^2{-\omega }^2\right)}{e}^{j{\phi }_1}\right\}. \end{gathered}$$

(19)

And, for the transient component

$$F_{trans}\left(j\omega \right)={\mathcal{F}}_{trans}\left\{\frac{U_m}{Z}\mathrm{c}\mathrm{o}\mathrm{s}\left(-{\phi }_1\right)e^{-t/{\tau }_1}\right\}=\frac{U_m}{Z}\cos {\phi }_1\frac{1}{j\omega +\frac{1}{{\tau }_1}}.$$

(20)

This equation shows the distortion component is not quite fictitious.

(a)

In the case of harmonic supply and non-harmonic current:

The total non-harmonic current can be decomposed

$$I_{rms}=\sqrt{I_{1,rms}^2+\sum _{k=2}^{\infty }{I}_{k,rms}^2}$$

(21)

We need to know $I_{rms}$, $I_{1,rms}$ to determine the rms value of the sum of higher harmonics

$$\sum _{k=2}^{\infty }{I}_{k,rms}^2=I_{\sum ,rms}=\sqrt{{{I^2}_{rms}-I}_{1,rms}^2}$$

(22)

By this, we can use Fourier coefficients for the maximal value calculation of $ip-iq$ currents and their time waveforms

$$I_{1p,max}\equiv b_1=\frac{2}{T}\underset{0}{\overset{T}{\int }}i\left(t\right)\mathrm{s}\mathrm{i}\mathrm{n}\left(\omega t\right)\mathrm{d}t \to i_{1p}\left(t\right)=I_{1p,max}\mathrm{s}\mathrm{i}\mathrm{n}\left(\omega t\right)$$

(23)

The time waveforms of decomposed current components are shown in Figure 4.

And

$$I_{1q,max}\equiv a_1=\frac{2}{T}\underset{0}{\overset{T}{\int }}i\left(t\right)\mathrm{c}\mathrm{o}\mathrm{s}\left(\omega t\right)\mathrm{d}t \to i_{1q}\left(t\right)=I_{1q,max}\mathrm{c}\mathrm{o}\mathrm{s}\left(\omega t\right)$$

(24)

Using Equations (13)–(16), we obtain the active and blind reactive power components $S_{av}\left(k\right)$ $P_{\left(1\right)av}\left(t\right)$, $Q_{\left(1\right)av}\left(t\right)$, and $D_{av}\left(k\right)$.

• (b) In the case of non-harmonic supply and quasi-harmonic current:

A classical non-harmonic rectangular time waveform causes under R-L load a non-harmonic current, Figure 5.

The voltage and the current can be decomposed into p- and q- components.

Since the supply voltage is non-harmonic

$$U_{rms}=\sqrt{U_{1,rms}^2+\sum _{k=2}^{\infty }{U}_{k,rms}^2}$$

(25)

We need to know $U_{rms}$, $U_{1,rms}$ to determine the rms value of the sum of higher harmonics

$$\sum _{k=2}^{\infty }{U}_{k,rms}^2=U_{\sum ,rms}\sqrt{{{U^2}_{rms}-U}_{1,rms}^2}.$$

(26)

The first term under sqrt

$$U_{rms}\left(k\right)\iff \sqrt{\frac{1}{T}\underset{0}{\overset{T}{\int }}u\left(t\right)\mathrm{d}t},\mathrm{u}\mathrm{s}\mathrm{i}\mathrm{n}\mathrm{g} movRMS$$

(27)

and

$$U_{1,rms}\left(k\right)\iff \sqrt{\frac{1}{T}\underset{0}{\overset{T}{\int }}{u}_1\left(t\right)\mathrm{d}t},\mathrm{u}\mathrm{s}\mathrm{i}\mathrm{n}\mathrm{g} movRMS$$

(28)

By this, we can use Fourier coefficients for the maximal value calculation of the $up, uq$ voltages and their time waveforms

$$U_{1p,max}\equiv b_1=\frac{2}{T}\underset{0}{\overset{T}{\int }}u\left(t\right)\mathrm{s}\mathrm{i}\mathrm{n}\left(\omega t\right)\mathrm{d}t \to u_{1p}(t)=U_{1p,max}\mathrm{s}\mathrm{i}\mathrm{n}\left(\omega t\right)$$

(29)

$$U_{1q,max}\equiv b_1=\frac{2}{T}\underset{0}{\overset{T}{\int }}u\left(t\right)\mathrm{c}\mathrm{o}\mathrm{s}\left(\omega t\right)\mathrm{d}t \to u_{1q}(t)=U_{1q,max}\mathrm{c}\mathrm{o}\mathrm{s}\left(\omega t\right)$$

(30)

The time waveforms of the decomposed current components are shown in Figure 6.

Figure 6 shows non-harmonic input voltage (inverter voltage) u(t), its fundamental harmonic, and its decomposition into u1p, u1q components (a), and also the decomposition of the total current fundamental harmonic into i1p, i1q components, respectively. Decomposition (a) follows Equations (29)–(30) and decomposition (b) follows Equations (23) and (24), in which the ip-iq method is included. So, again using Equations (13)–(16), we obtain the active and blind reactive power components $S_{av}\left(k\right)$ $P_{\left(1\right)av}\left(t\right)$, $Q_{\left(1\right)av}\left(t\right)$, and $D_{av}\left(k\right)$.

The calculated power components $S_{av}\left(k\right)$, $P_{av}\left(k\right)$, $Q_{av}\left(k\right),$ and $D_{av}\left(k\right)$ are applied in the next application section.

While for a non-symmetric system, the inequality holds

$$x_a\left(t\right)+x_b\left(t\right)+x_c\left(t\right)\ne 0,$$

(31)

because of including a zero-phase sequence component $x_0\left(t\right)$ into the Clarke transform [13].

$$\left[\begin{array}{c}{x}_{\alpha ,\beta }\left(t\right)\\ x_0\left(t\right)\end{array}\right]=C_t \left[\begin{array}{ccc}1& a& a^2\\ \frac{1}{2}& \frac{1}{2}& \frac{1}{2}\end{array}\right]\left[\begin{array}{c}{x}_a\left(t\right)\\ x_b\left(t\right)\\ x_c\left(t\right)\end{array}\right];\mathrm{o}\mathrm{r} \left[\begin{array}{c}{x}_{\alpha }\left(t\right)\\ x_{\beta }\left(t\right)\\ x_0\left(t\right)\end{array}\right]=\frac{1}{3}\left[\begin{array}{ccc}2& -1& -1\\ 0& \sqrt{3}& -\sqrt{3}\\ 1& 1& 1\end{array}\right]\left[\begin{array}{c}{x}_a\left(t\right)\\ x_b\left(t\right)\\ x_c\left(t\right)\end{array}\right].$$

(32)

where $\mathit{a}=$−1/2 +$\sqrt{3}/2$ and $C_t$ is the transformation constant.

We can also use the method of symmetrical components $x_{\mathrm{1,2},0}\left(t\right)$, [7,11].

$$\left[\begin{array}{c}{x}_1\left(t\right)\\ x_2\left(t\right)\\ x_0\left(t\right)\end{array}\right]=\frac{1}{3}\left[\begin{array}{ccc}1& a& a^2\\ 1& a^2& a\\ 1& 1& 1\end{array}\right]\left[\begin{array}{c}{x}_a\left(t\right)\\ x_b\left(t\right)\\ x_c\left(t\right)\end{array}\right].$$

(33)

The inverse transformation into the $a,b,c$ system can be obtained using the inverse transformation matrix. Consequently, the power in a non-symmetrical system also features an instantaneous zero-sequence component $p_0\left(t\right)$.

$$\left[\begin{array}{c}\begin{array}{c}{p}_{\alpha ,\beta }\left(t\right)\\ q_{\alpha ,\beta }\left(t\right)\end{array}\\ p_0\left(t\right)\end{array}\right]=\frac{3}{2}\left[\begin{array}{ccc}{u}_{\alpha }\left(t\right)& u_{\beta }\left(t\right)& 0\\ {-u}_{\beta }\left(t\right)& u_{\alpha }\left(t\right)& 0\\ 0& 0& \frac{u_0\left(t\right)}{3}\end{array}\right]\left[\begin{array}{c}{i}_{\alpha }\left(t\right)\\ i_{\beta }\left(t\right)\\ i_0\left(t\right)\end{array}\right].$$

(34)

The phase power components $p_{a,b,c}\left(t\right)$ can be expressed by the inverse transform of Equation (34).

3. Application of ip-iq Theory on Three-Phase Network and Linear Time-Invariant Load—Simulation Using Matlab/Simulink

The three-phase network and symmetric linear time-invariant R-L load can be connected between each other:

-

Directly, without any means of interconnection;

-

Employing a rectifier (controlled, uncontrolled);

-

Employing an inverter (direct, indirect, or cycloconverter).

By the simulation of some of them, we can confirm the theoretical derivation performed in the previous section.

3.1. Simulation Using Matlab/Simulink

There will be simulated transient states during the start-up and during a change in the load of the chosen connection of the systems. As investigated, the quantities are time courses of the power components’ mean values $S_{av}\left(k\right)$, $P_{av}\left(k\right)$, $Q_{av}\left(k\right),$ and $D_{av}\left(k\right)$.

A. 

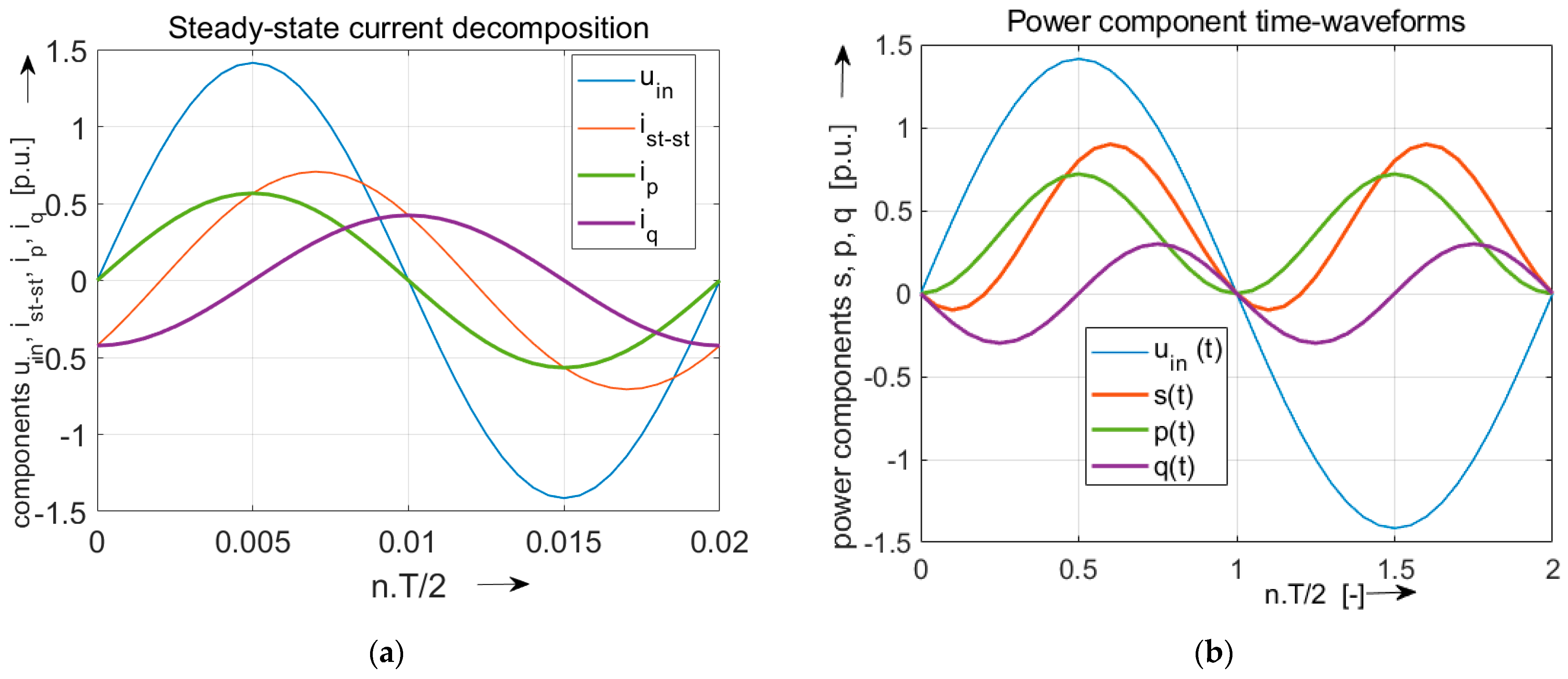
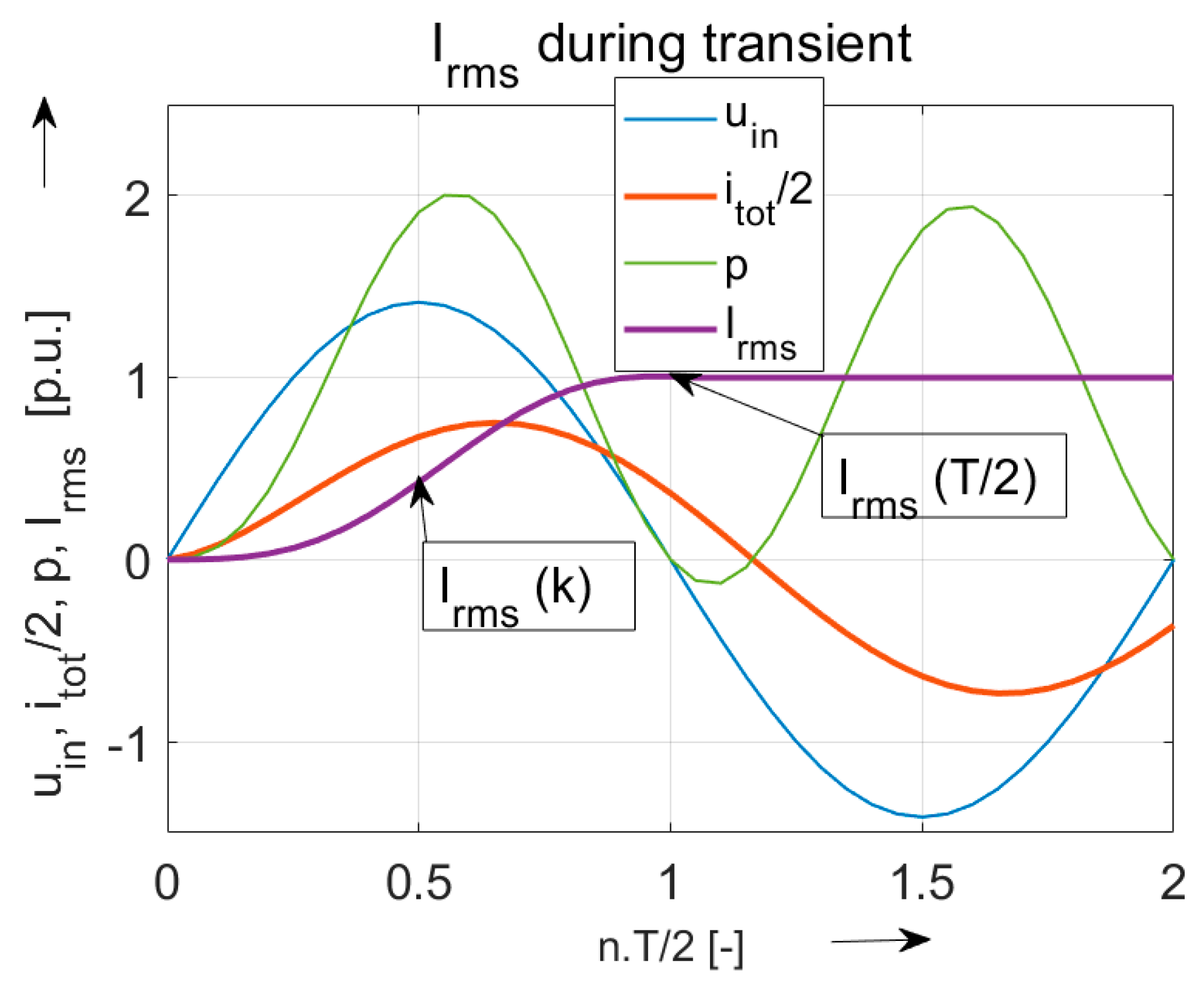
Direct connection

Parameters of the system for steady states at $t\in <0.0;0.1>$ and at $t\in <0.1;0.16>$ are given in

Table 1. Parameters of RL load for steady states before and after load change in direct connection.

Load	$\mathit{R} [\Omega$]	$\mathit{L} [\mathbf{m}\mathbf{H}$]	$\mathit{Z}\left[\Omega \right]$	$\mathit{\tau }$$[\mathbf{m}\mathbf{s}$]	$\mathit{\phi }$$[°\mathbf{e}\mathbf{l}.$]	$\mathbf{c}\mathbf{o}\mathbf{s}\mathit{\phi }$$\left[-\right]$	$\mathbf{s}\mathbf{i}\mathbf{n}\mathit{\phi }[-]$
before	$18.4$	43.94	$23.09$	$2.38$	$36.87$	$0.8$	$0.6$
after	$36.8$	$43.94$	$39.30$	$1.19$	$20.56$	$0.94$	$0.35$

$U_{rms}=230 V, f=50 \mathrm{H}\mathrm{z},\mathrm{t}\mathrm{h}\mathrm{r}\mathrm{e}\mathrm{e}-\mathrm{p}\mathrm{h}\mathrm{a}\mathrm{s}\mathrm{e} \mathrm{s}\mathrm{y}\mathrm{s}\mathrm{t}\mathrm{e}\mathrm{m}$.

.

The start-up and load change simulation have been performed using Matlab/Simulink. The time courses of the average power components are shown in Figure 7.

The graphic interpretations of the Fourier transform amplitude spectra of both the steady-state and transient components of the current are shown in Figure 8 in the absolute units.

Since the time constant ${\tau }_1$ is rather small (cca 2.4 ms), i.e., smaller than one-fourth of the time period (Table 1), the predominant component is the steady-state one.

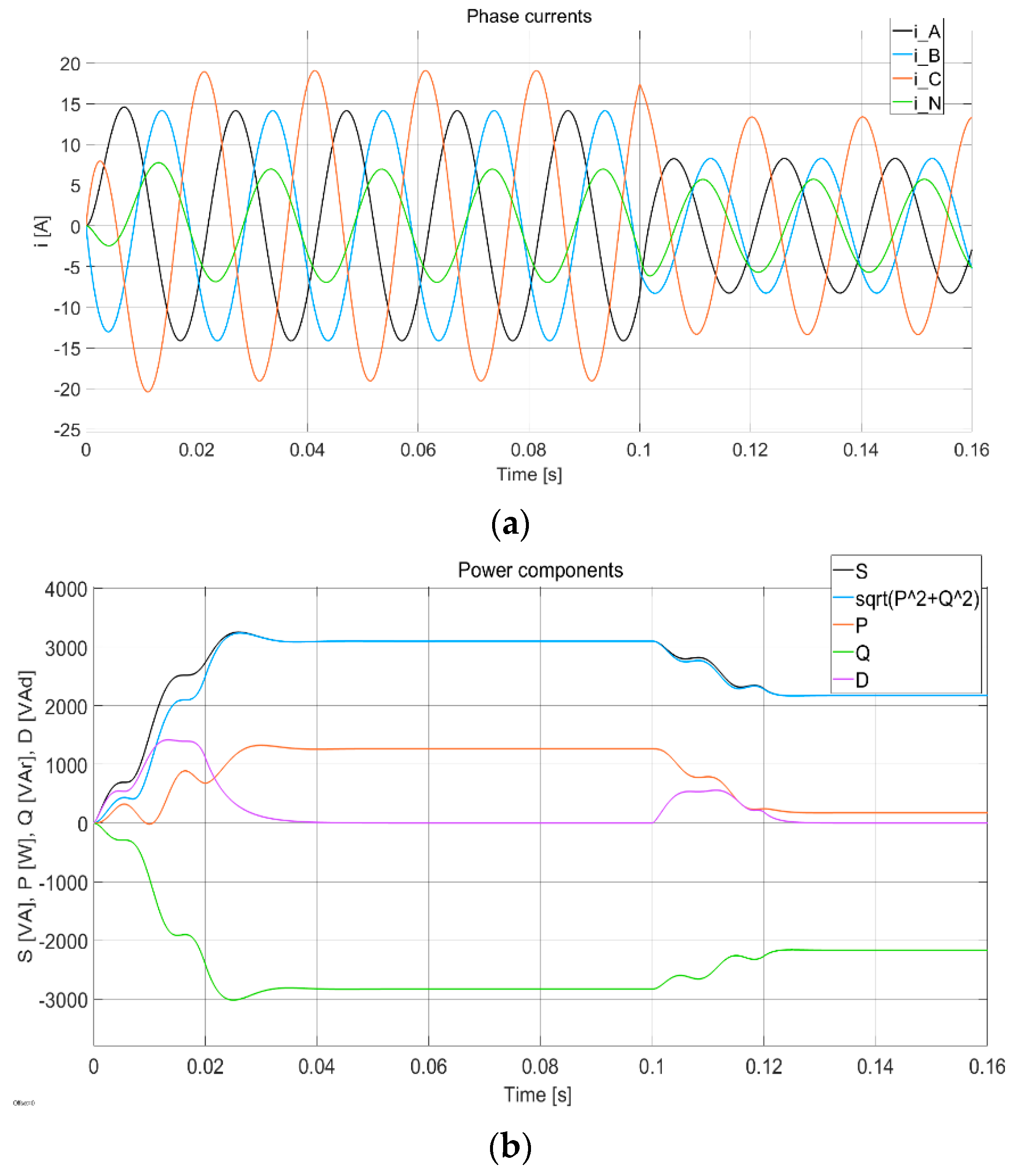
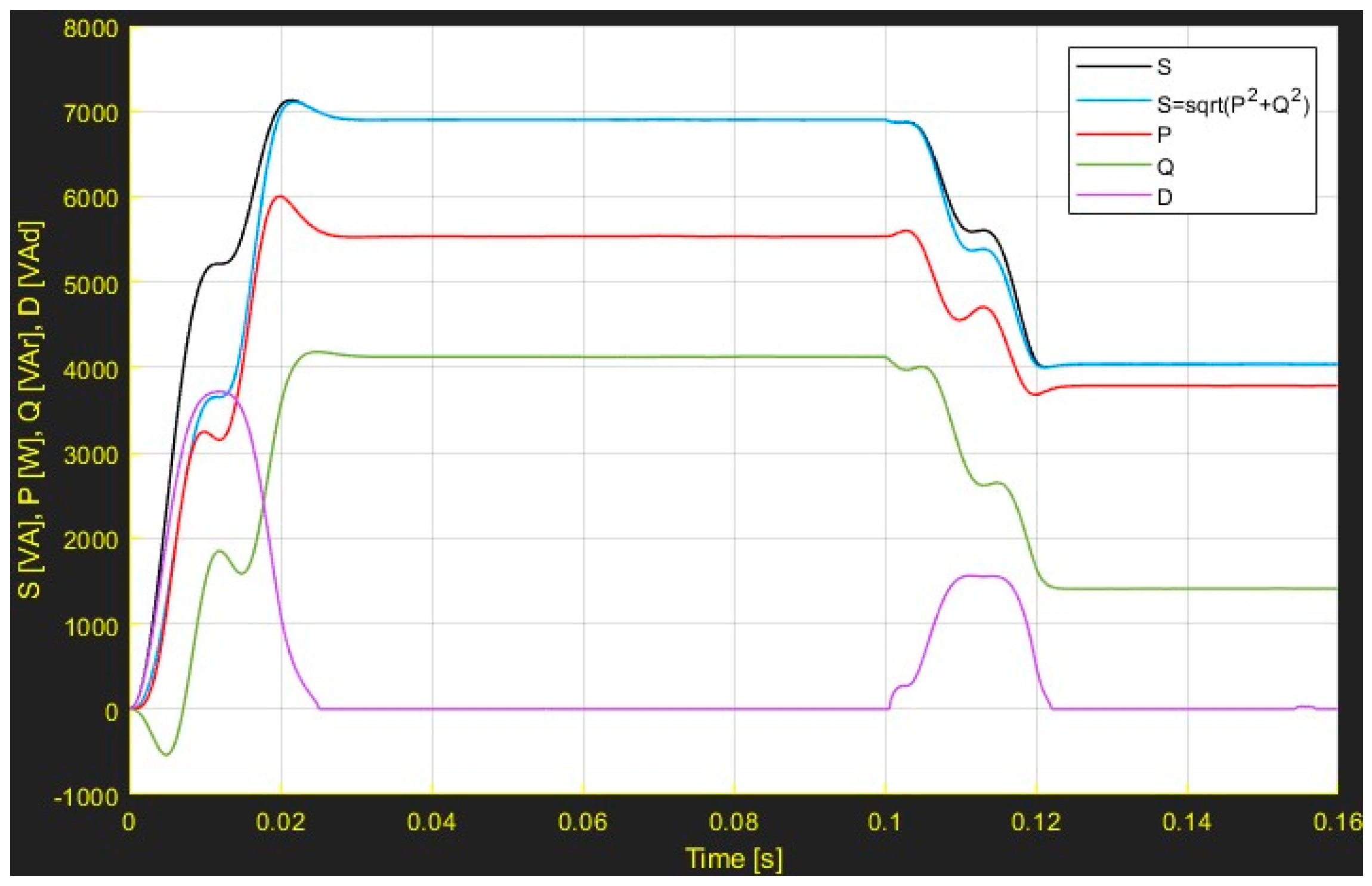
3.2. Case of Non-Symmetrical Load

The load asymmetry has been provided by a different value of resistor in one phase. The resistors of the asymmetrical phase c are $R_c=10 \mathsf{\Omega }$ and 20 $\mathsf{\Omega }$. Parameters for both steady states are given in

Table 2. Load parameters for steady states before and after load change in case of non-symmetrical load.

Load	${\mathit{R}}_{\mathit{a}}$$[\Omega$]	$L [\mathbf{m}\mathbf{H}$]	$\mathit{Z}\left[\Omega \right]$	${\mathit{\tau }}_{\mathit{a}}$$[\mathbf{m}\mathbf{s}$]	${\mathit{\phi }}_{\mathit{a}}$$[°\mathbf{e}\mathbf{l}.$]	$\mathbf{c}\mathbf{o}\mathbf{s}{\mathit{\phi }}_{\mathit{a}}$$\left[-\right]$	$\mathbf{s}\mathbf{i}\mathbf{n}{\mathit{\phi }}_{\mathit{a}}[-]$
before	$10$	$43.93$	$17.04$	$4.39$	$54.07$	$0.59$	$0.81$
after	$20$	$43.93$	$24.29$	$2.19$	$34.61$	0.82	$0.57$
Simulation results before and after change (at steady states)
Time	${\mathit{S}}_{\mathit{a}\mathit{v}}\left[\mathbf{V}\mathbf{A}\right]$	${\mathit{P}}_{\mathit{a}\mathit{v}}\left[\mathbf{W}\right]$	${\mathit{Q}}_{\mathit{a}\mathit{v}}\left[\mathbf{V}\mathbf{A}\mathbf{r}\right]$	${\mathit{D}}_{\mathit{a}\mathit{v}}\left[\mathbf{V}\mathbf{A}\mathbf{d}\right\}$	$\mathit{P}\mathit{F}[-]$	${\mathit{P}}_{1\mathit{a}\mathit{v}}\left[\mathbf{W}\right]$	${\mathit{P}}_{0\mathit{a}\mathit{v}}\left[\mathbf{W}\right]$
$\mathrm{t}=0.1 \mathrm{s}$	$3098$	$1264$	$2828$	$0$	$0.408$	$1264$	$0$
$\mathrm{t}=0.16 \mathrm{s}$	$2173$	$174.9$	$2166$	$0$	$0.08$	$174.9$	$0$

$U_{rms}=230 V, f=50 \mathrm{H}\mathrm{z}, \mathrm{t}\mathrm{h}\mathrm{r}\mathrm{e}\mathrm{e}-\mathrm{p}\mathrm{h}\mathrm{a}\mathrm{s}\mathrm{e} \mathrm{s}\mathrm{y}\mathrm{s}\mathrm{t}\mathrm{e}\mathrm{m}$.

.

Power component average values, computed in Matlab/Simulink, are given in Table 2.

Network voltages and currents in $a,b,c,N$ coordinate system at steady states are shown in Figure 9a,b.

In this case, the network neutral is connected to the load neutral. Therefore, current I_N flows through the neutral wire despite the voltage zero-sequence being zero. It is interesting that active power in the steady state after the transient is smaller than before, Figure 9b, although load resistance in phase “c” is changed to double. But the phase current i_c and the current of neutral I_N are in antiphase (shifted by 180 °el.), Figure 9a. So, the power loss (Joule loss) in the neutral wire acts negatively on the load active power. During the transient state, waveforms feature oscillating characters, and the power component $Po$ is zero, due to the zero-sequence component $Uo$ equal to zero.

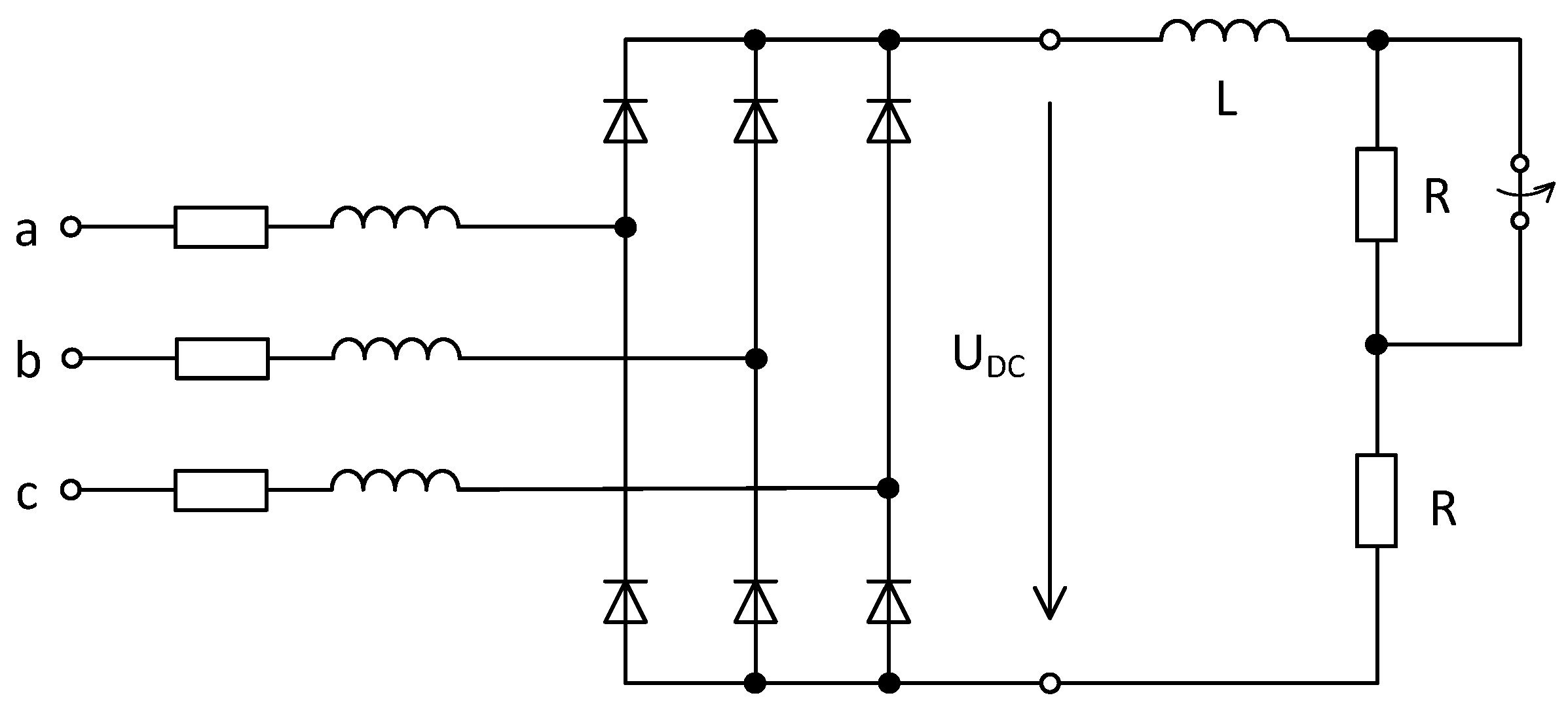
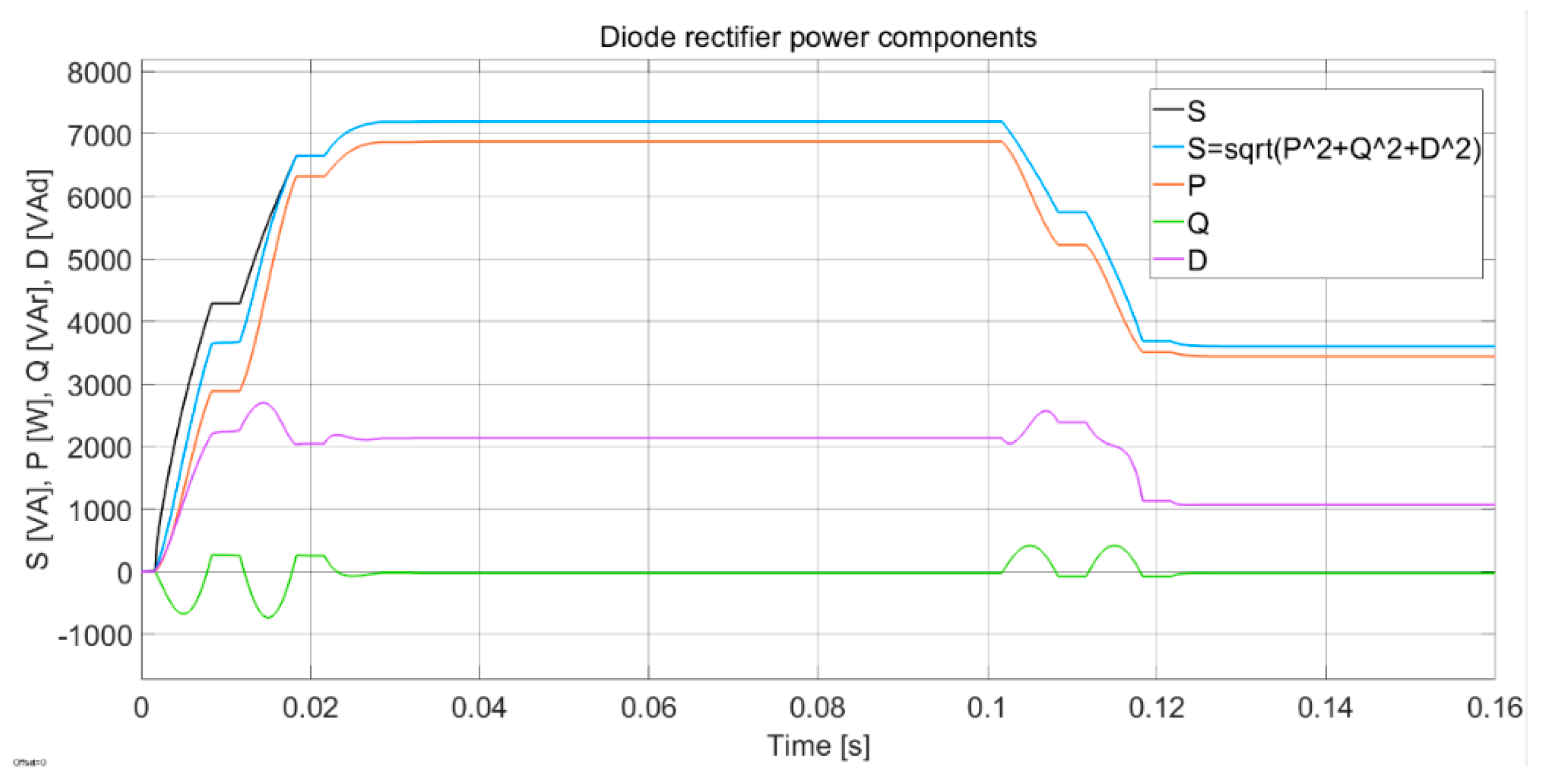
B. 

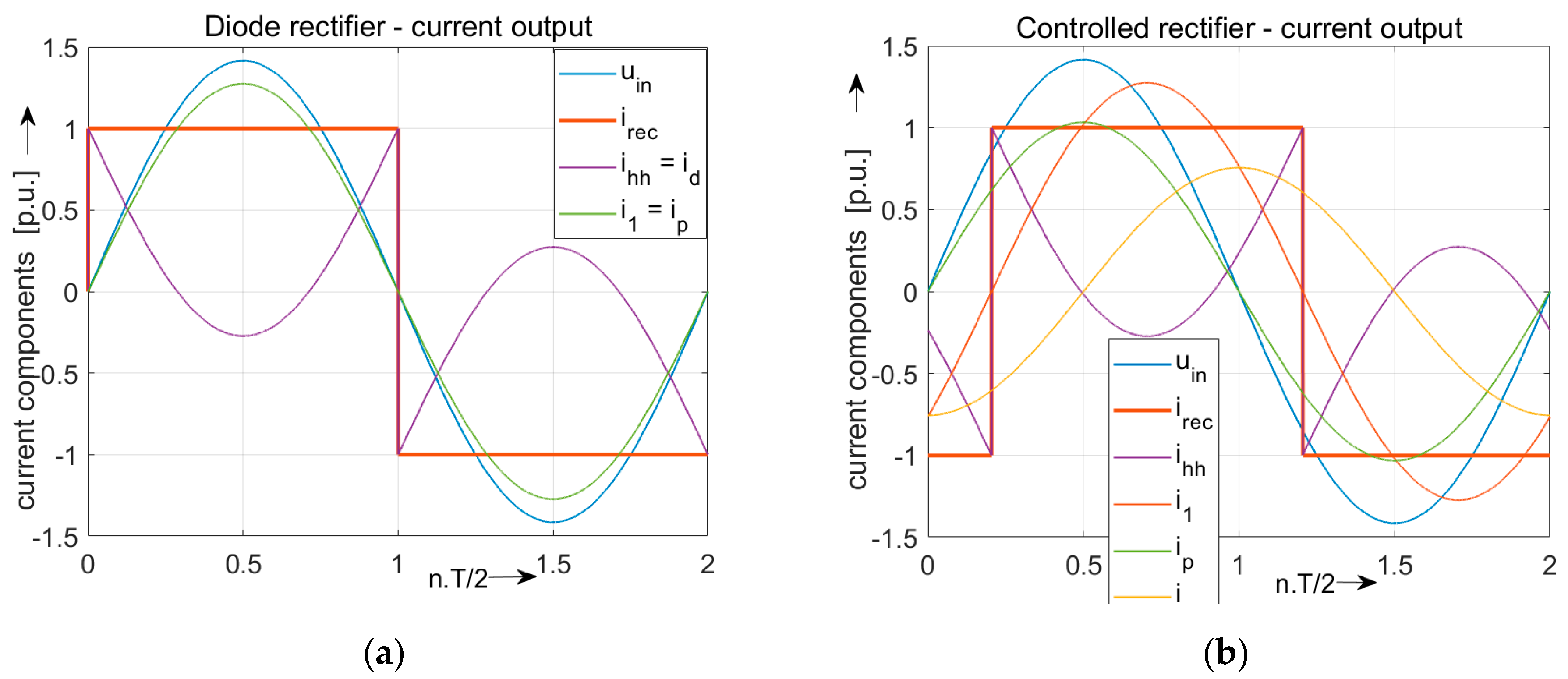
System with the connection of a diode rectifier

Let us consider systems supplied from a harmonic network and equipped with a three-phase diode rectifier with a linear resistive R-L load, Figure 10.

Parameters for steady states at $t\in <0.0;0.1>$ and at $t\in <0.1;0.16>$ are given in

Table 3. Load parameters for steady states before and after load change in diode connection.

Load	$\mathit{R} [\Omega$]	$\mathit{L} [\mathbf{m}\mathbf{H}$]	α [deg}
before	41.91	100	0
after	83.82	100	0

$U_{rms}=230 V, f=50 \mathrm{H}\mathrm{z}, \mathrm{t}\mathrm{h}\mathrm{r}\mathrm{e}\mathrm{e}-\mathrm{p}\mathrm{h}\mathrm{a}\mathrm{s}\mathrm{e} \mathrm{s}\mathrm{u}\mathrm{p}\mathrm{p}\mathrm{l}\mathrm{y} \mathrm{s}\mathrm{y}\mathrm{s}\mathrm{t}\mathrm{e}\mathrm{m}$.

.

The start-up and load change simulation have been performed using Matlab/Simulink. The time courses of average power components are shown in Figure 11.

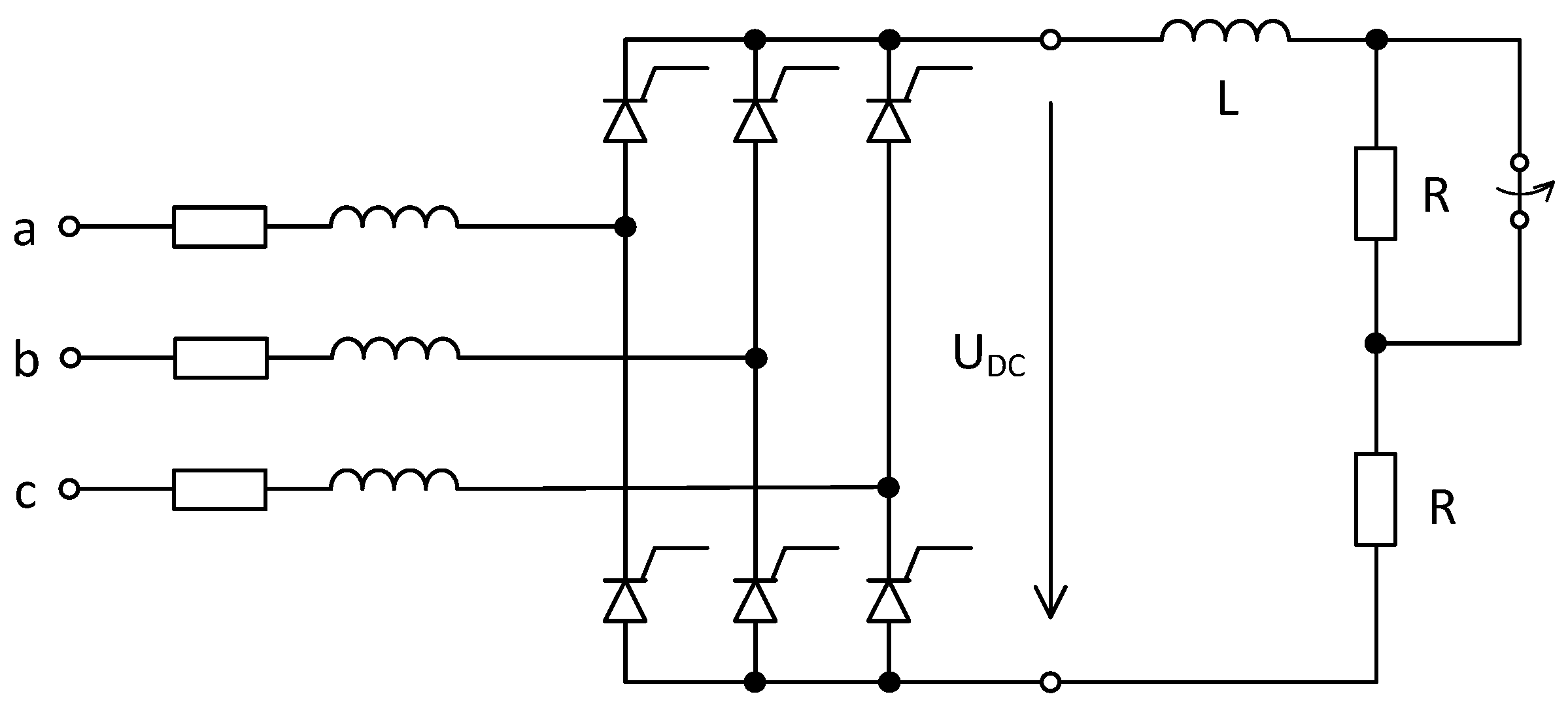
C. 

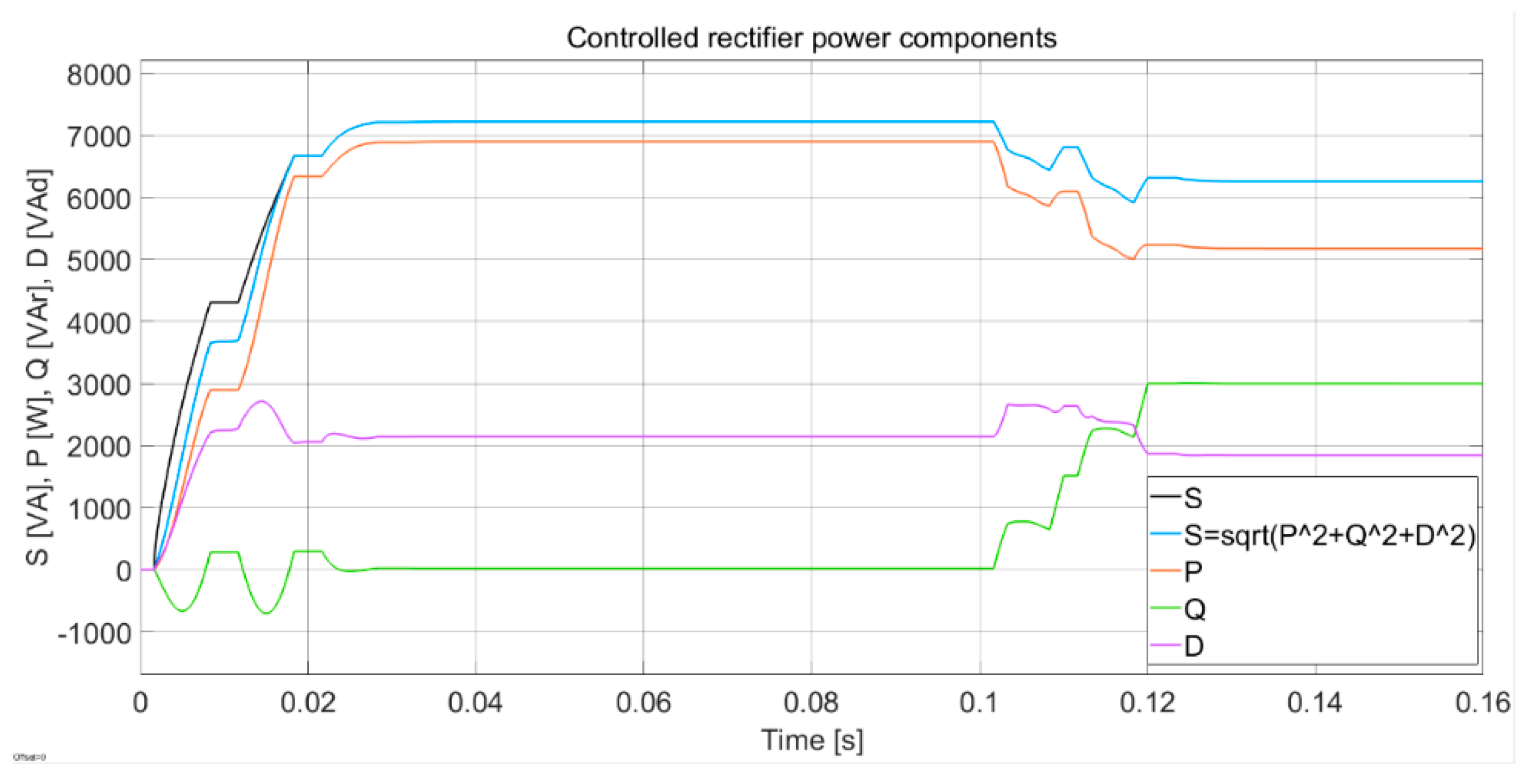
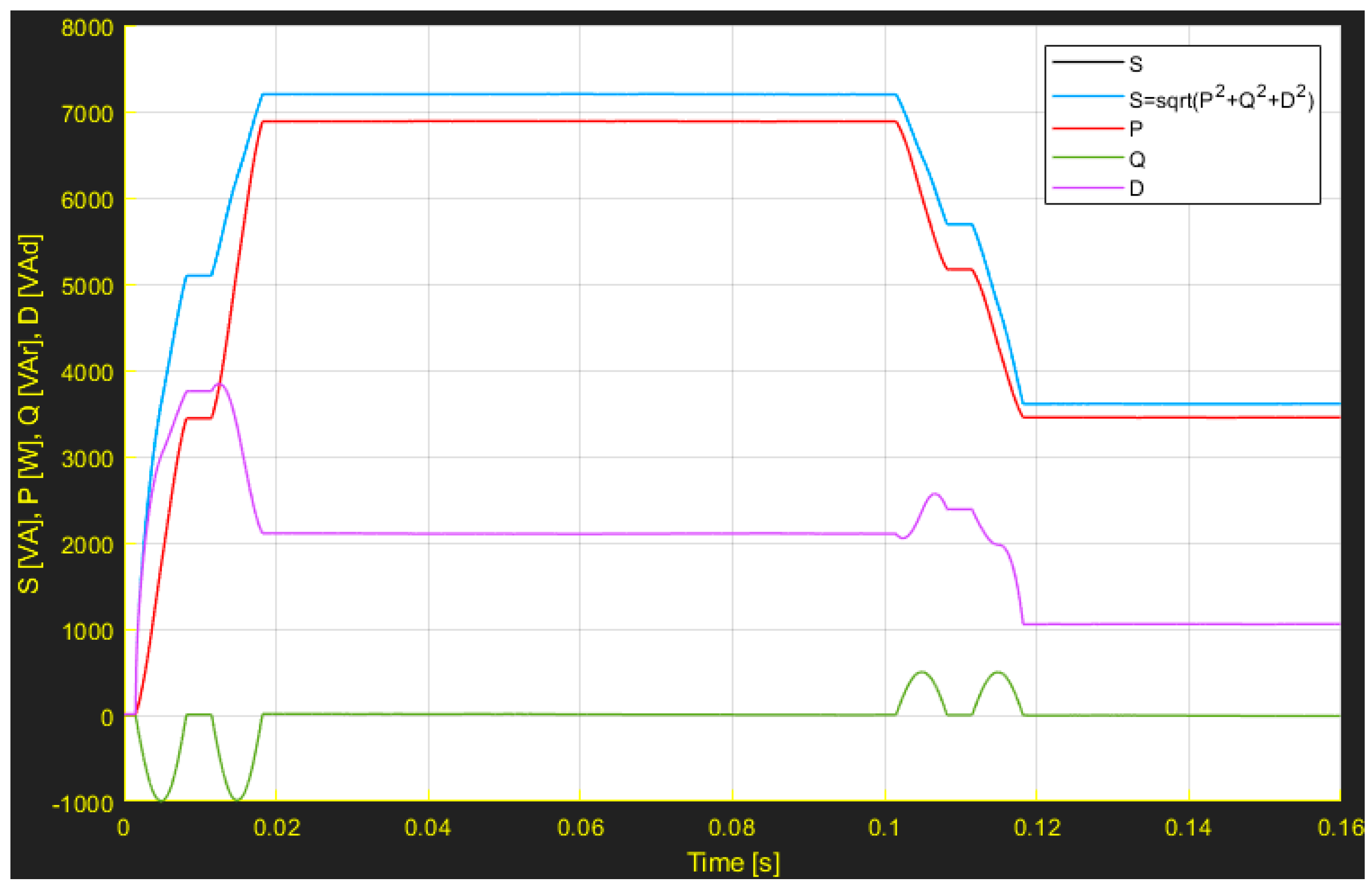
System with the connection of a controlled rectifier

Let us consider systems supplied from a harmonic network and equipped with a three-phase controlled rectifier and a linear resistive R-L load, Figure 12.

Parameters for steady states at $t\in <0.0;0.1>$ and at $t\in <0.1;0.16>$ are given in

Table 4. Load parameters for steady states before and after load change in controlled rectifier connection.

Load	$\mathit{R} [\Omega$]	$\mathit{L} [\mathbf{m}\mathbf{H}$]	α [deg}
before	41.91	100	0
after	41.91	100	30

$U_{rms}=230 V, f=50 \mathrm{H}\mathrm{z}, \mathrm{t}\mathrm{h}\mathrm{r}\mathrm{e}\mathrm{e}-\mathrm{p}\mathrm{h}\mathrm{a}\mathrm{s}\mathrm{e} \mathrm{s}\mathrm{u}\mathrm{p}\mathrm{p}\mathrm{l}\mathrm{y} \mathrm{s}\mathrm{y}\mathrm{s}\mathrm{t}\mathrm{e}\mathrm{m}$.

.

The start-up and load change simulation have been performed using Matlab/Simulink. The time courses of average power components are shown in Figure 13.

D. 

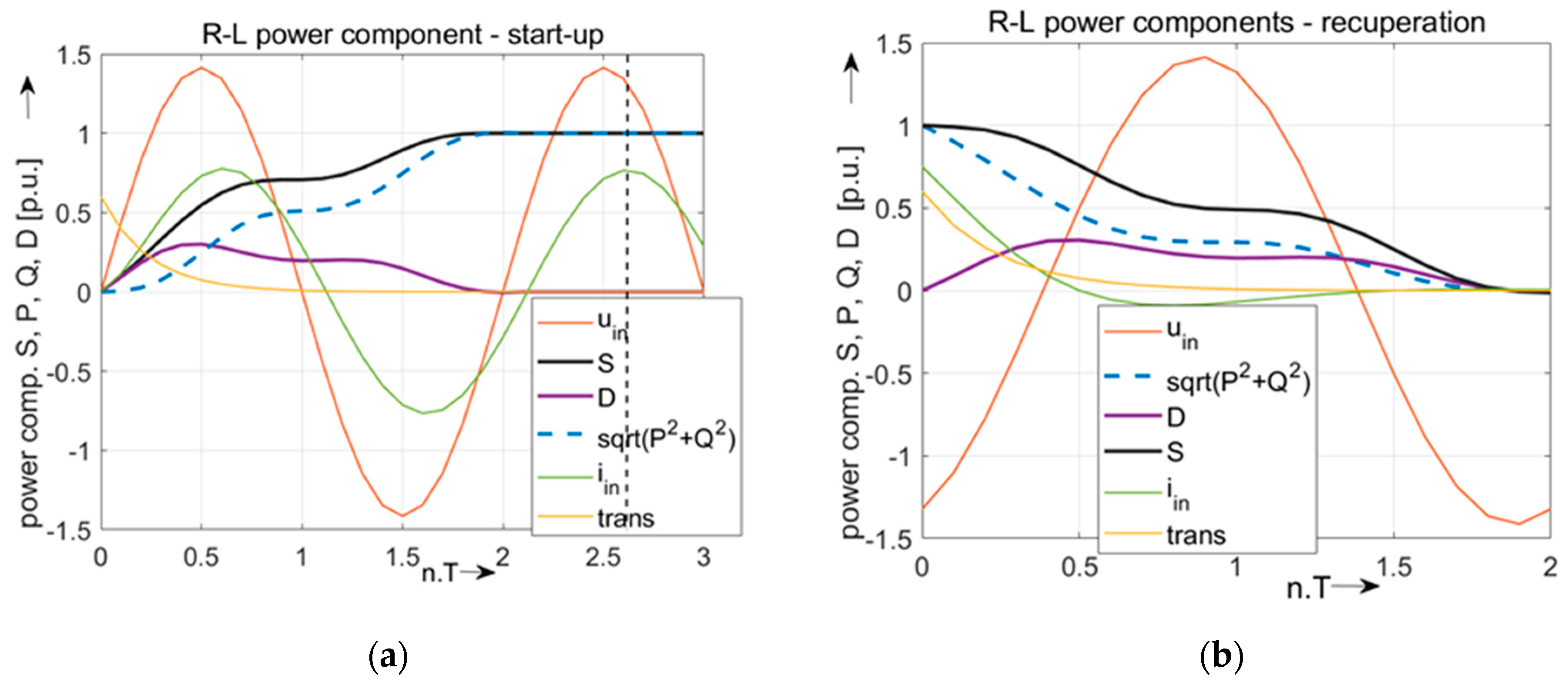
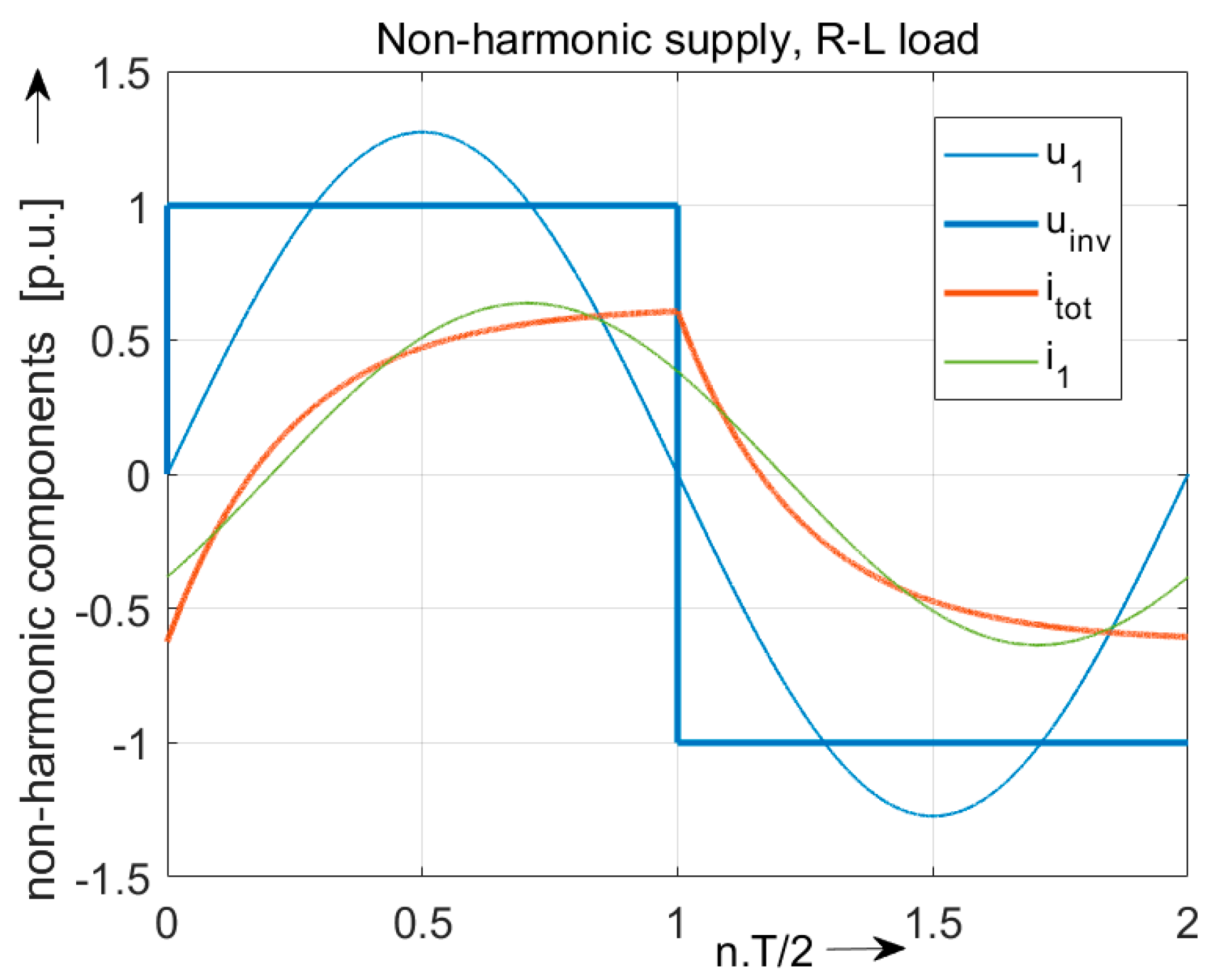
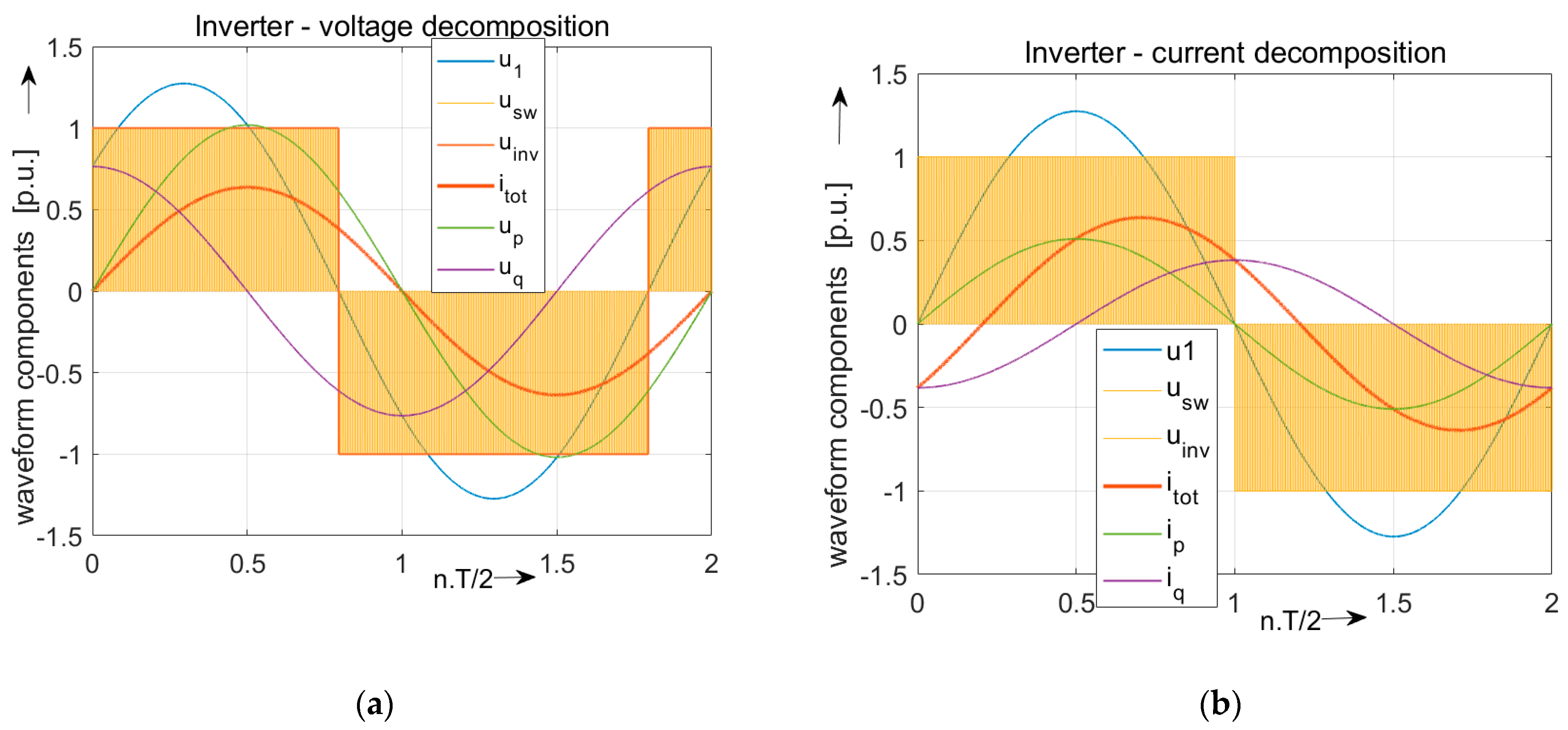
Three-phase inverter type of VSI and linear RL load

Let us consider systems supplied from a harmonic network and equipped with a three-phase VSI inverter and a linear resistive R-L load, Figure 14.

Parameters for steady states at $t\in <0.0;0.1>$ and at $t\in <0.1;0.2>$ are given in

Table 5. Load parameters for steady states before and after load change.

Load	$\mathit{R} [\Omega$]	$\mathit{L} [\mathbf{m}\mathbf{H}$]	$\mathit{Z}\left[\Omega \right]$	$\mathit{\tau }$$[\mathbf{m}\mathbf{s}$]	$\mathit{\phi }$$[°\mathbf{e}\mathbf{l}.$]	$\mathbf{c}\mathbf{o}\mathbf{s}\mathit{\phi }$$\left[-\right]$	$\mathbf{s}\mathbf{i}\mathbf{n}\mathit{\phi }[-]$
before	$9.2$	$43.93$	16.58	4.775	56.31	0.550	0.835
after	$18.4$	$43.93$	23.00	2.387	37.87	0.797	0.604

$U_{rms}=230 V, f=50 \mathrm{H}\mathrm{z}, \mathrm{t}\mathrm{h}\mathrm{r}\mathrm{e}\mathrm{e}-\mathrm{p}\mathrm{h}\mathrm{a}\mathrm{s}\mathrm{e} \mathrm{s}\mathrm{y}\mathrm{s}\mathrm{t}\mathrm{e}\mathrm{m}$.

.

The start-up and load change simulation have been performed using Matlab/Simulink. The time courses of average power components are shown in Figure 15.

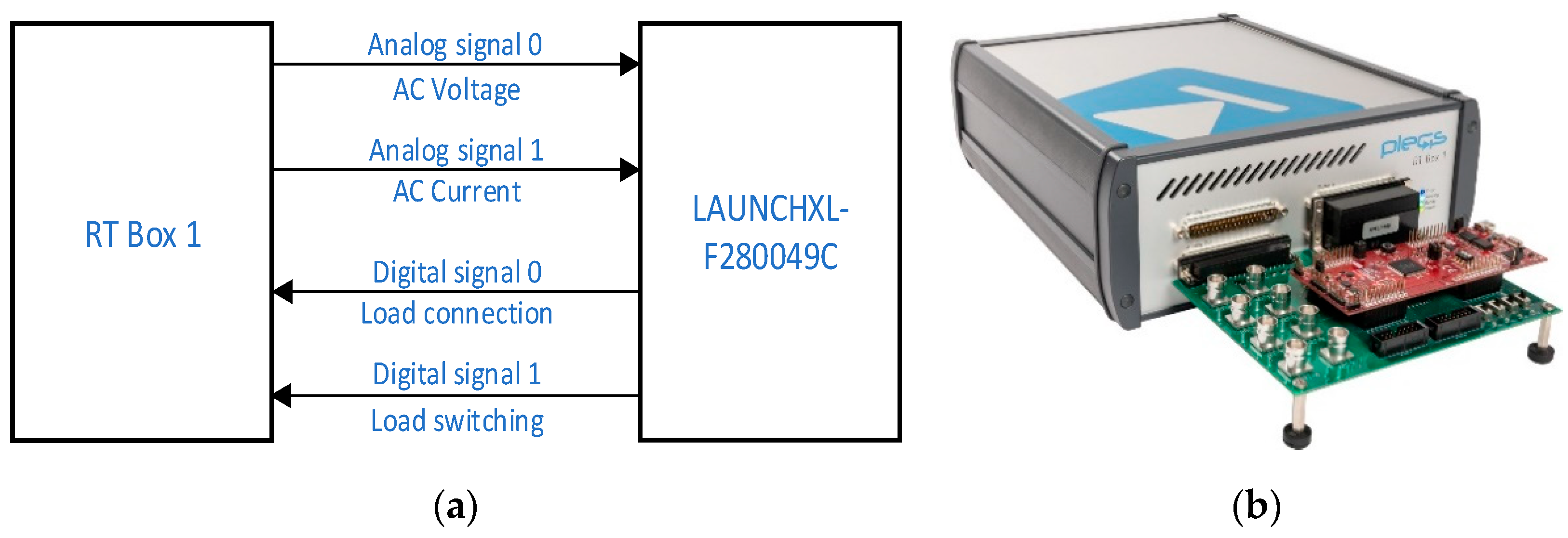
4. Verification of ip-iq Theory by a HIL Simulation (in Real Time)

A HIL simulator presented by the Plexim RT Box 1 with CPU cores 2 x ARM Cortex-A9, 1 GHz, is suitable for HIL simulations of power electronic circuits of moderate complexity and for single-tasking control prototyping [19]. The block scheme for interconnection of the microprocessor board with the RT Box and its assembly is shown in Figure 16.

There are simulated transient states during the start-up and during the changes in the load as they have been chosen in Section 2. As investigated, the quantities are time courses of the power components’ mean values $S_{av}\left(k\right)$, $P_{av}\left(k\right)$, $Q_{av}\left(k\right),$ and $D_{av}\left(k\right)$.

A. 

Direct connection of RL load to the network

Parameters of the system for steady states at $t\in <0.0;0.1>$ and at $t\in <0.1;0.16>$ are given in Table 1 above.

The start-up and load change simulation have been performed using Matlab/Simulink. The time courses of average power components are shown in Figure 17.

B. 

System with the connection of a diode rectifier

Let us consider systems supplied from a harmonic network and employing a three-phase diode rectifier with a linear resistive R-L load (Figure 9 above). Parameters for steady states at $t\in <0.0;0.1>$ and at $t\in <0.1;0.16>$ are given in Table 3 (in Section 2 above).

The start-up and load change simulation have been performed using Matlab/Simulink. The time courses of average power components are shown in Figure 18.

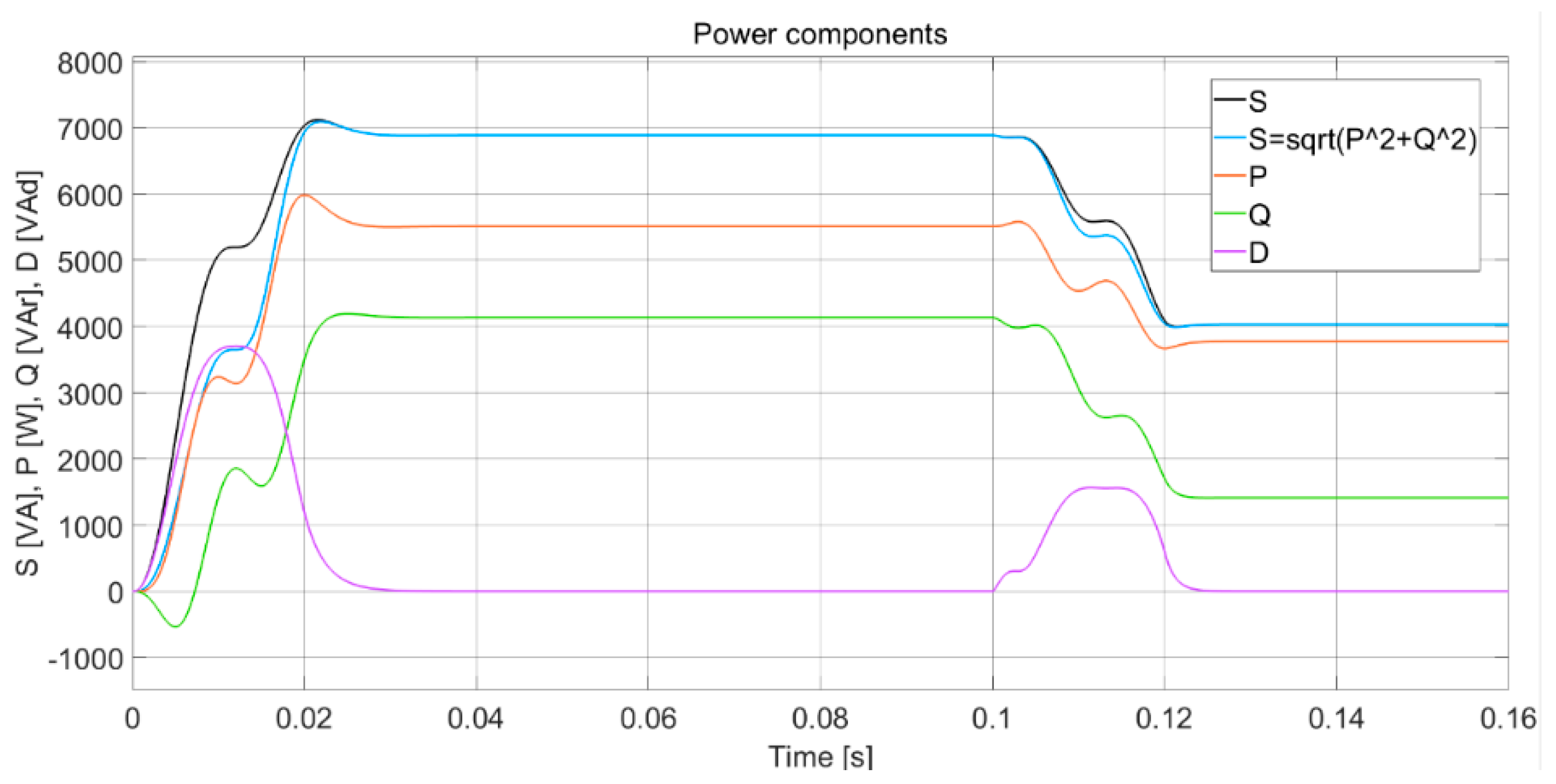
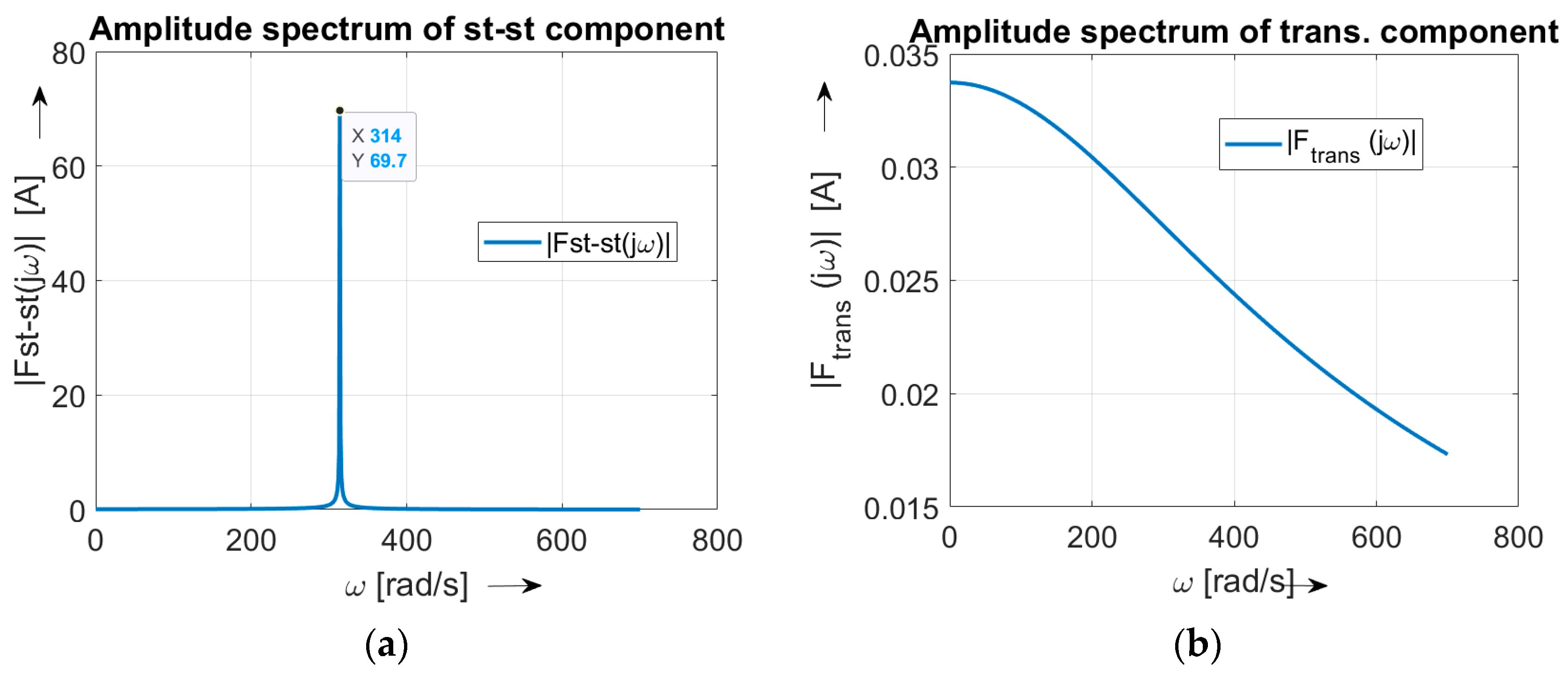
5. Discussion and Conclusions

This paper shows the behavior of power electronic systems, as well as power systems, under transient states presented by a step change of the load or another quantity, using the instantaneous reactive power ip-iq method. The simulation was performed under different types of loads and supply voltages (linear and non-linear load, sinusoidal and non-sinusoidal voltage). The simulation results are shown with the quasi-instantaneous determination of power components’ mean values, including the phase shift of fundamentals (resp. cos φ₁) and total power factor PF. The waveforms of apparent, active, blind, and distorted power components are displayed in the timeline. It has been shown that the distortion power components are generated during the transient under harmonic supply conditions and linear load.

The moving average and moving rms methods have been used to determine a power component’s mean values in the next calculation step, directly from measurable phase current and voltage quantities.

The results are comparable with those obtained using the p-q method (IRP), published by the authors in paper [8].

A comparison of the working-out results of the HIL and Matlab/Simulink has shown that these are nearly identical. The essential difference is in the time of the computation. In the case of HIL, the quantities sensing and computation of power components, including the moving RMS, takes about 5 microseconds, which is practically real-time, unlike the Matlab/Simulink simulation.

Finally, while the p-q method does not allow a direct application to a single-phase system or an unbalanced load, the ip-iq method using decomposition into Fourier coefficients yields results like the p-q method without the mentioned disadvantages. Moreover, neither the IRP method nor CPC method investigates the instantaneous power components in transient states which is the main contribution of this paper.