Research on the Material Characteristics and Loss Calculation Method of Cryogenic Permanent Magnet Motor Stator for LNG Pump
Abstract
1. Introduction
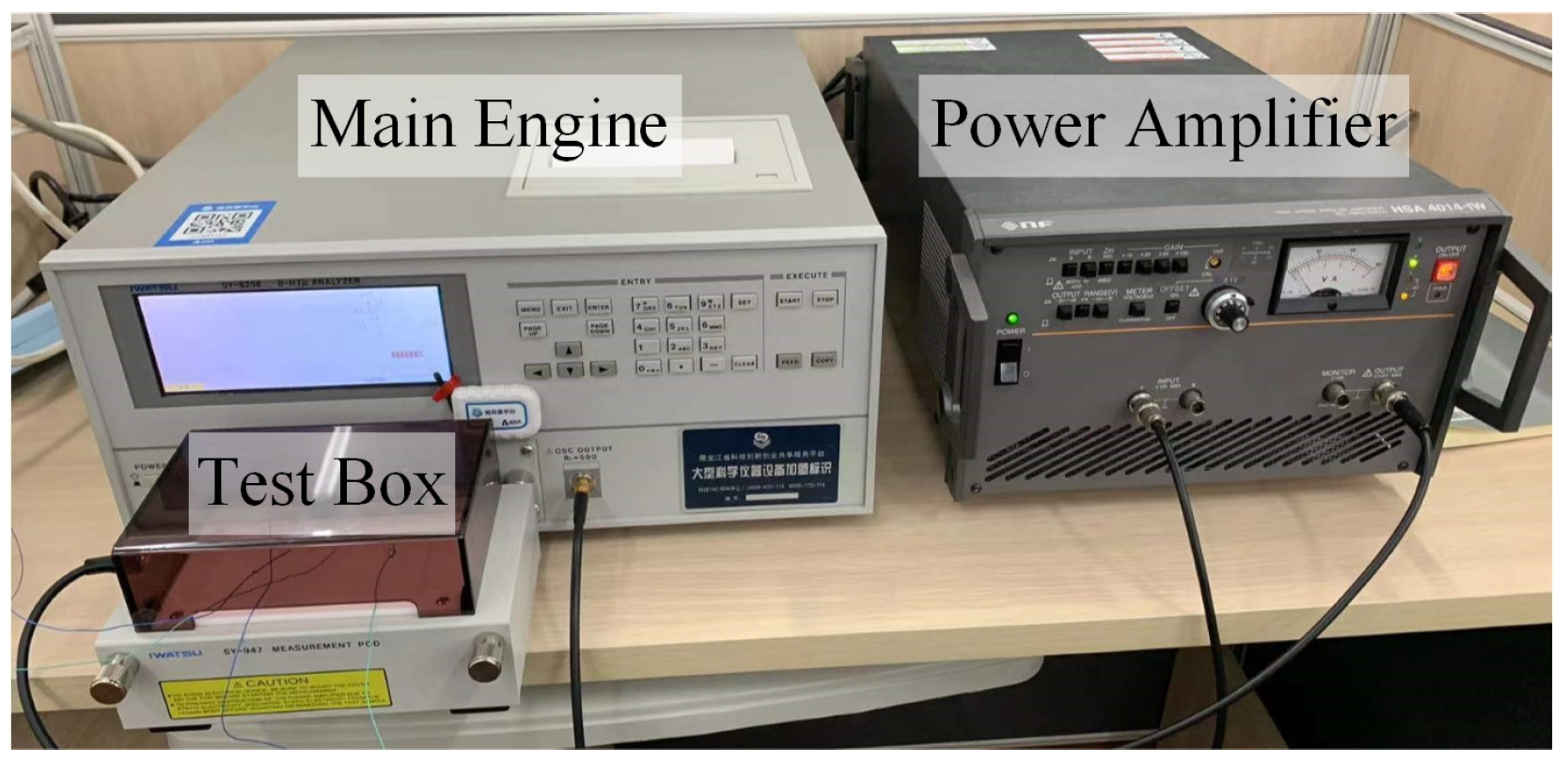
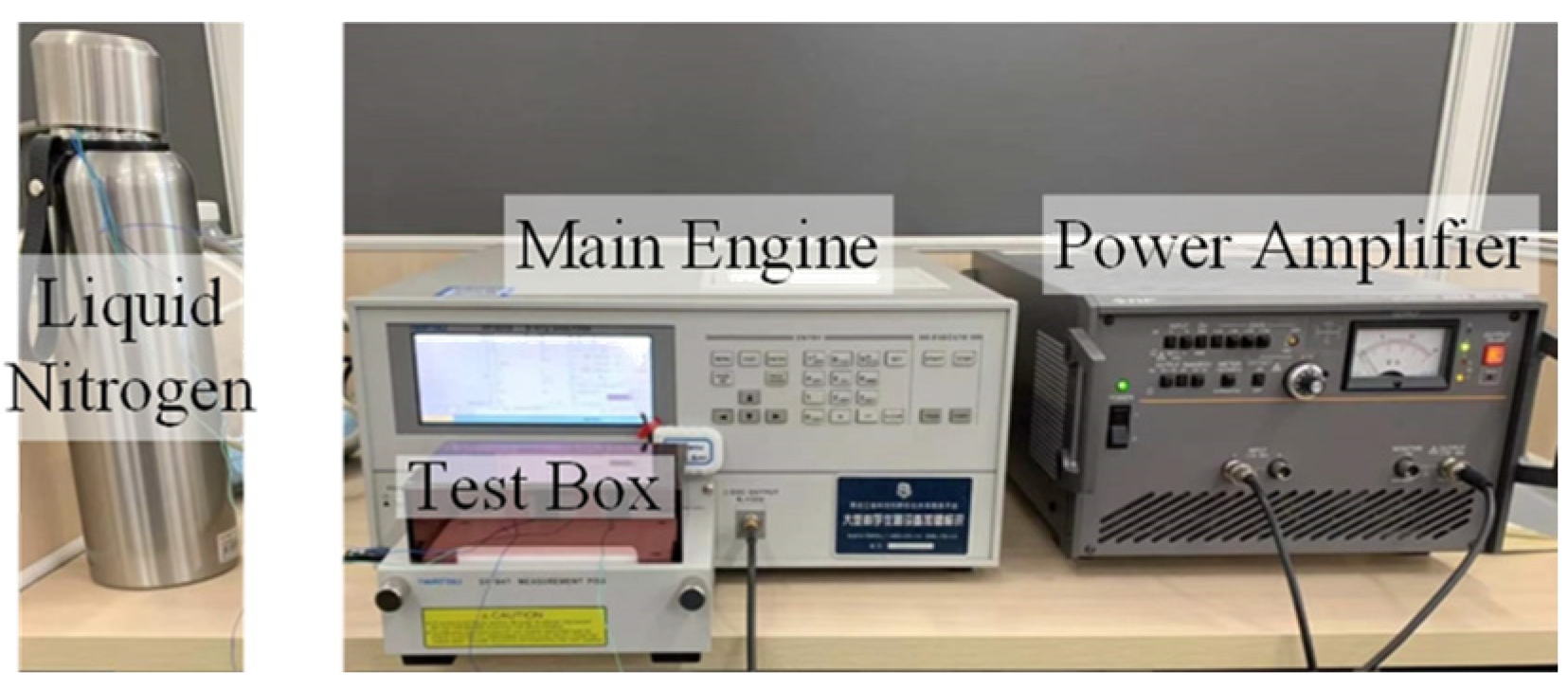
2. Ultra-Low-Temperature Experiment of Motor Stator Material
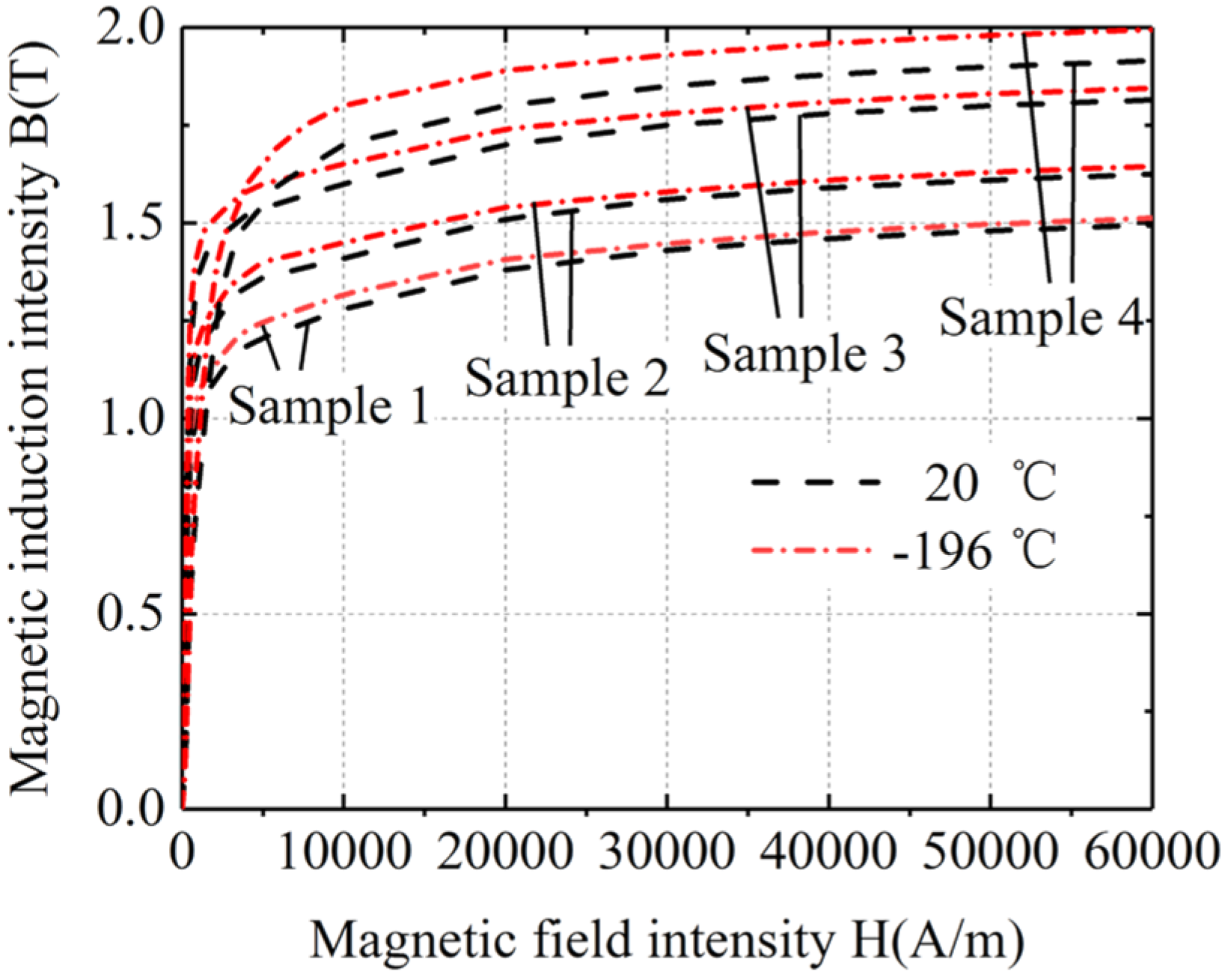
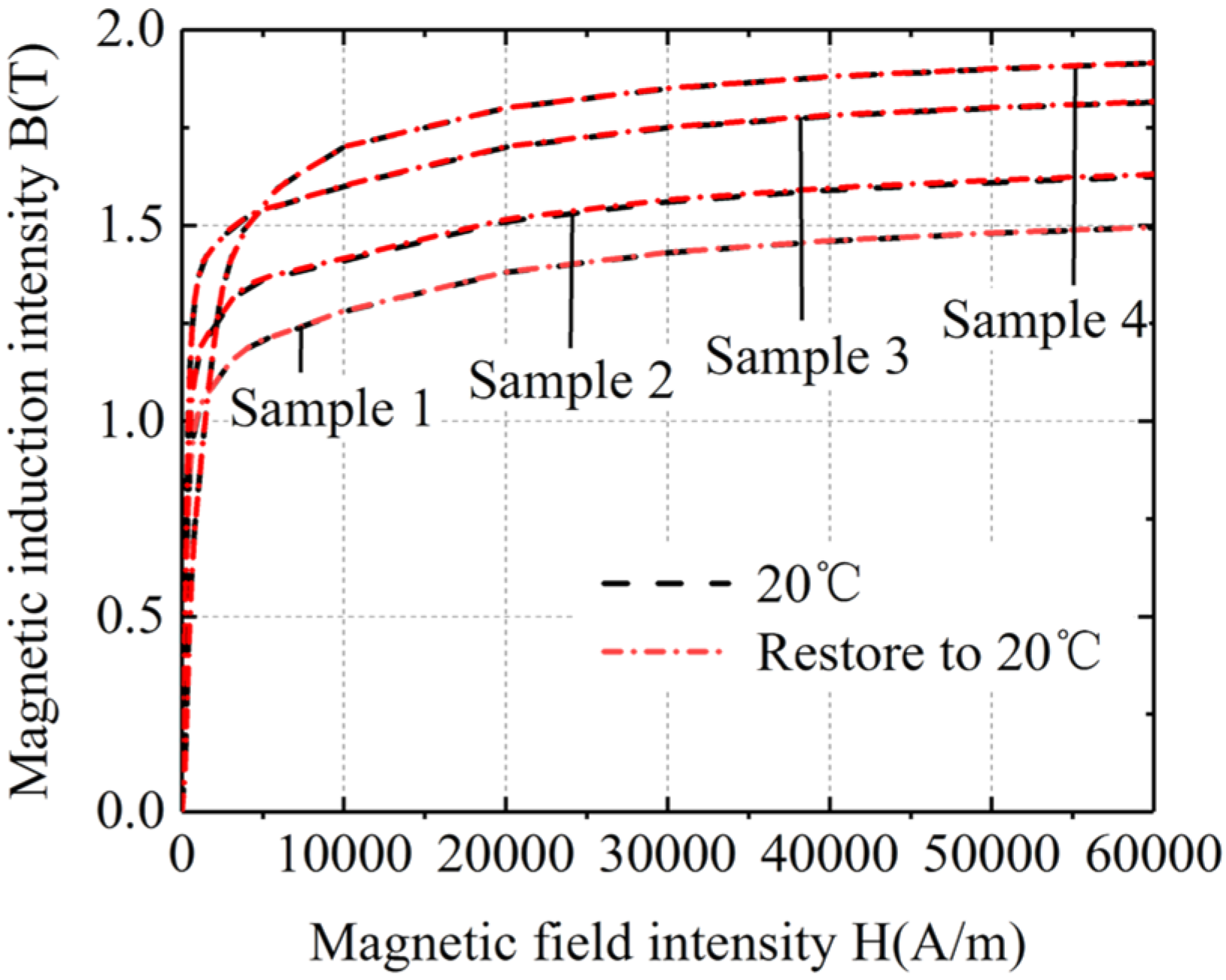
2.1. Magnetization Experiment of Silicon Steel Sheets
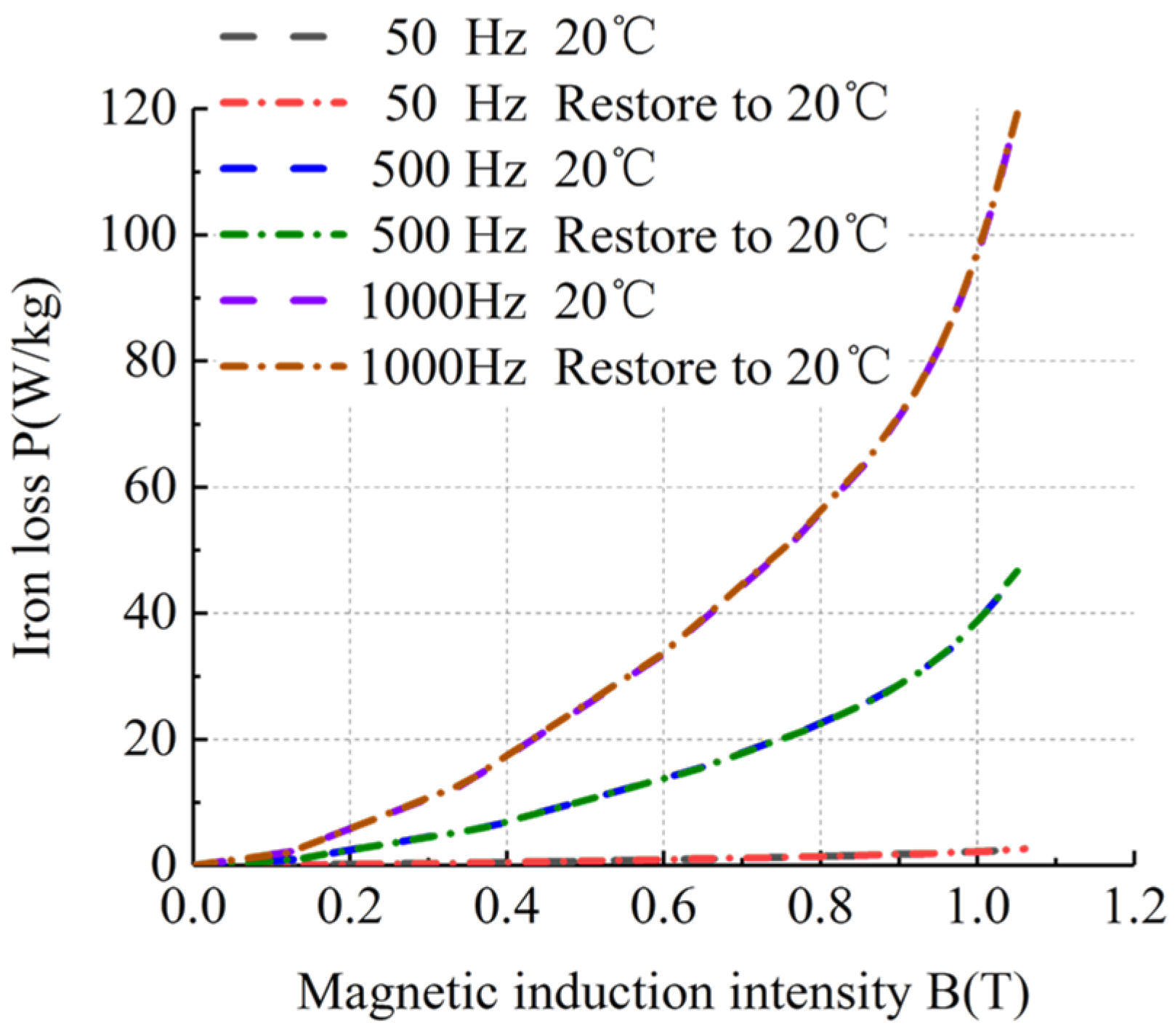
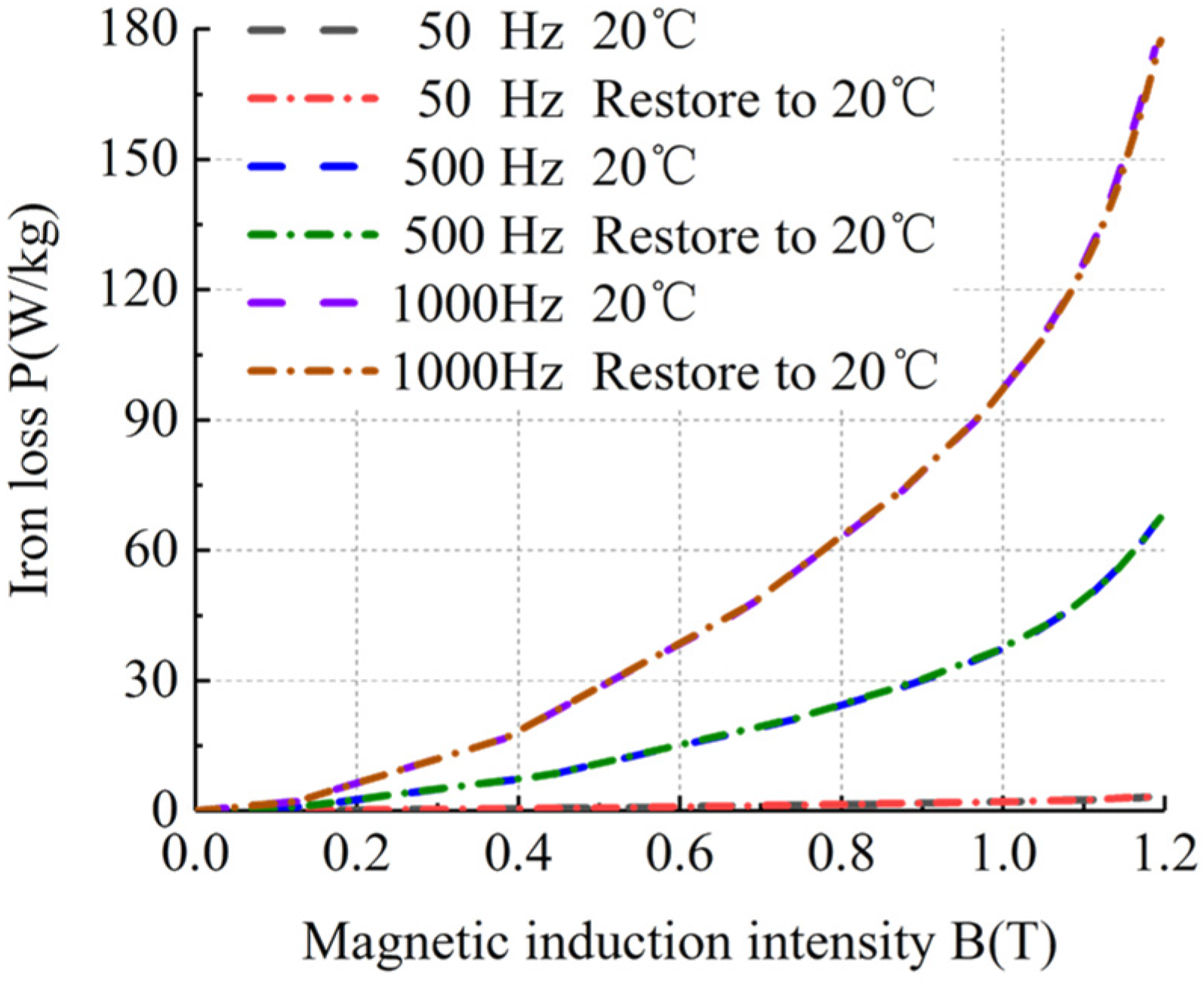
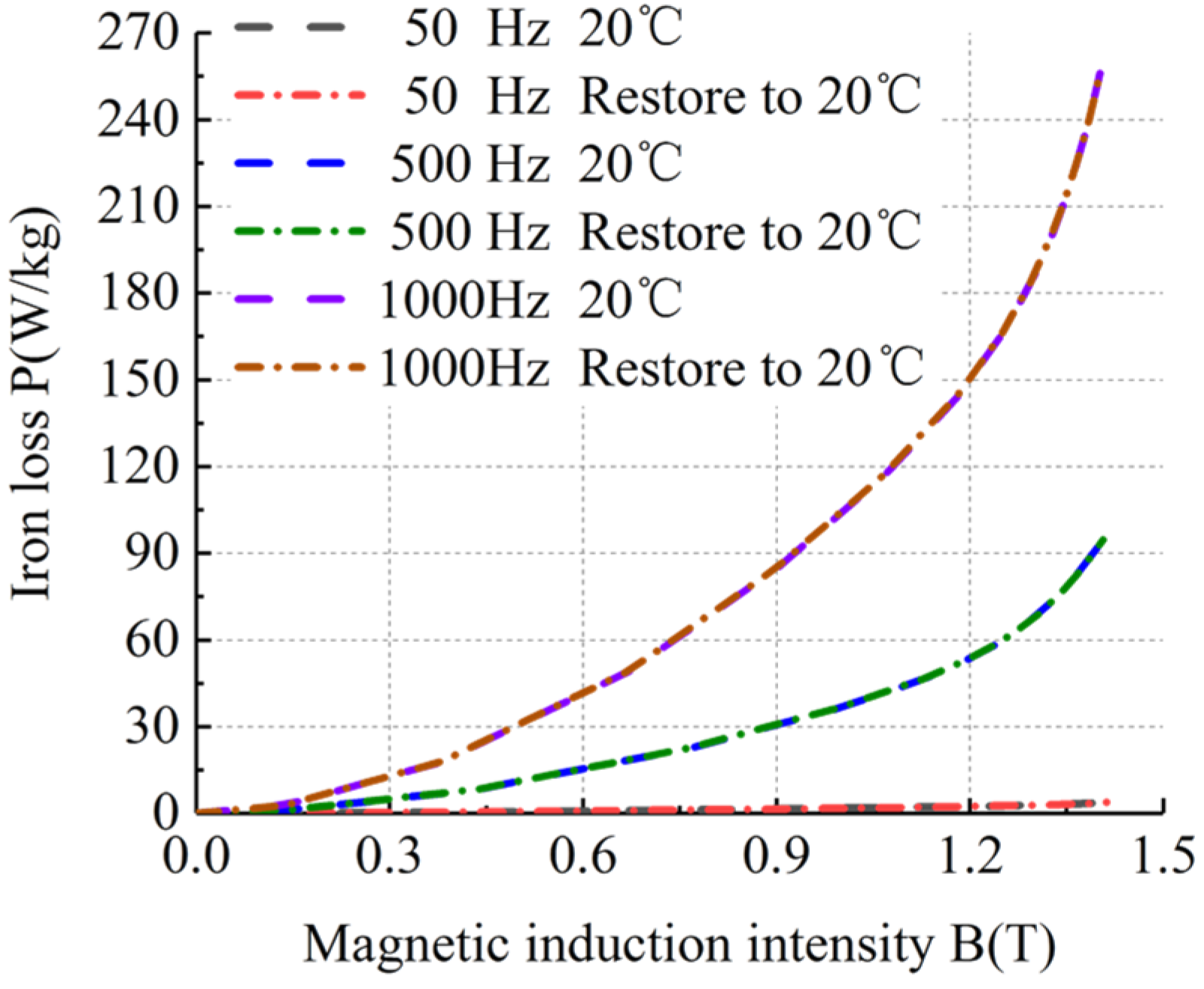
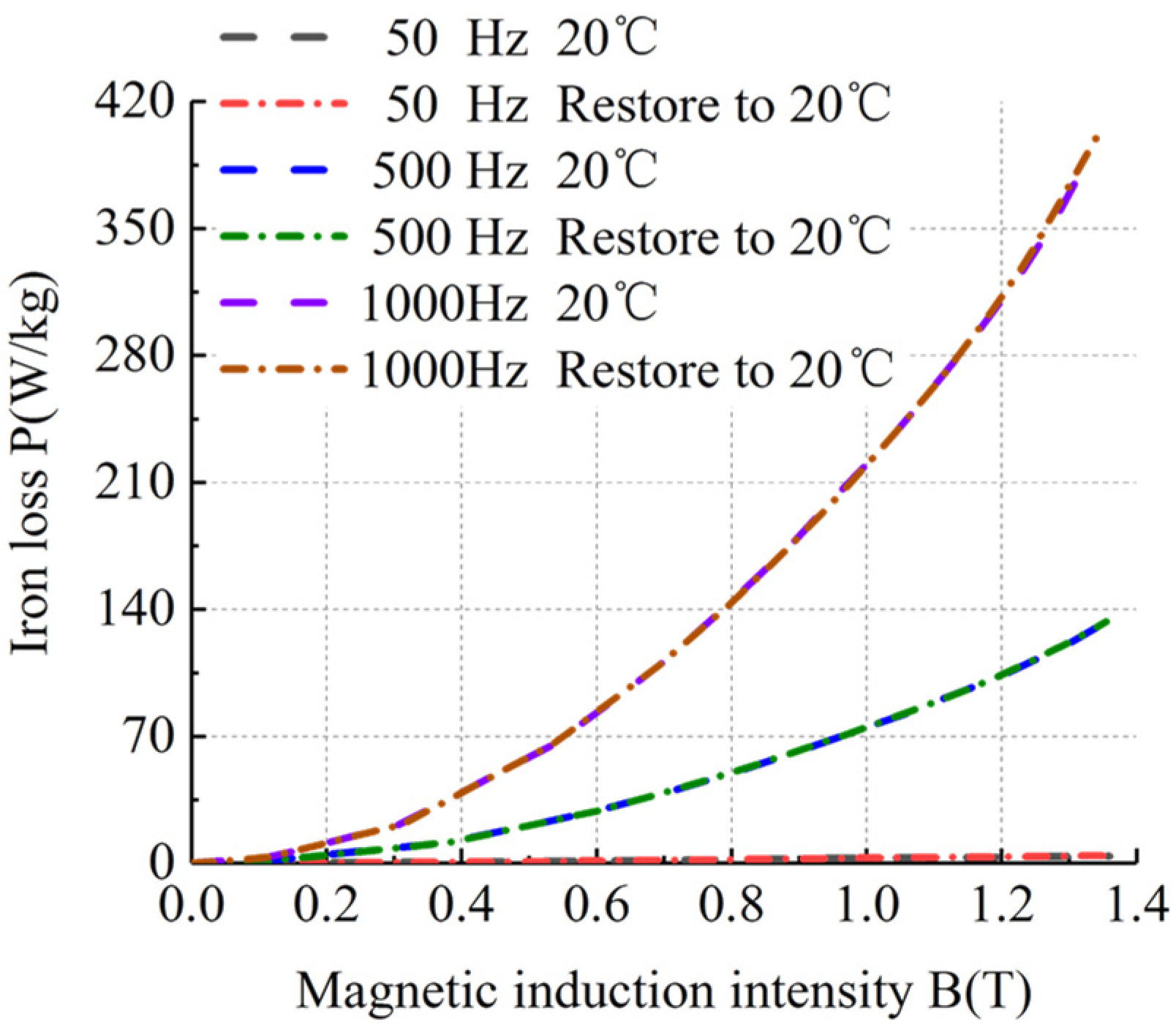
2.2. Loss Experiment of Silicon Steel Sheets
3. Research on Basic Iron Loss of Silicon Steel Sheets
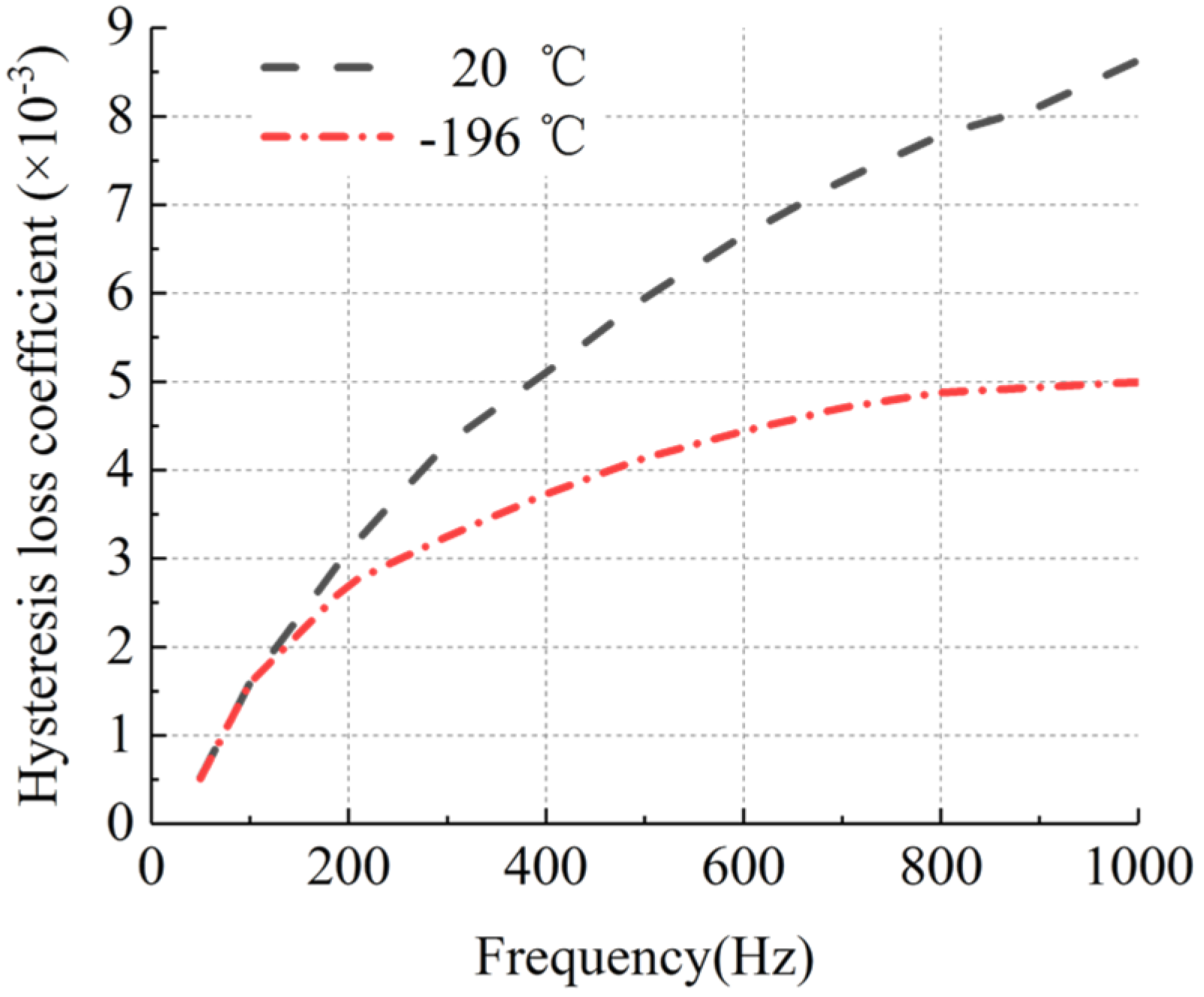
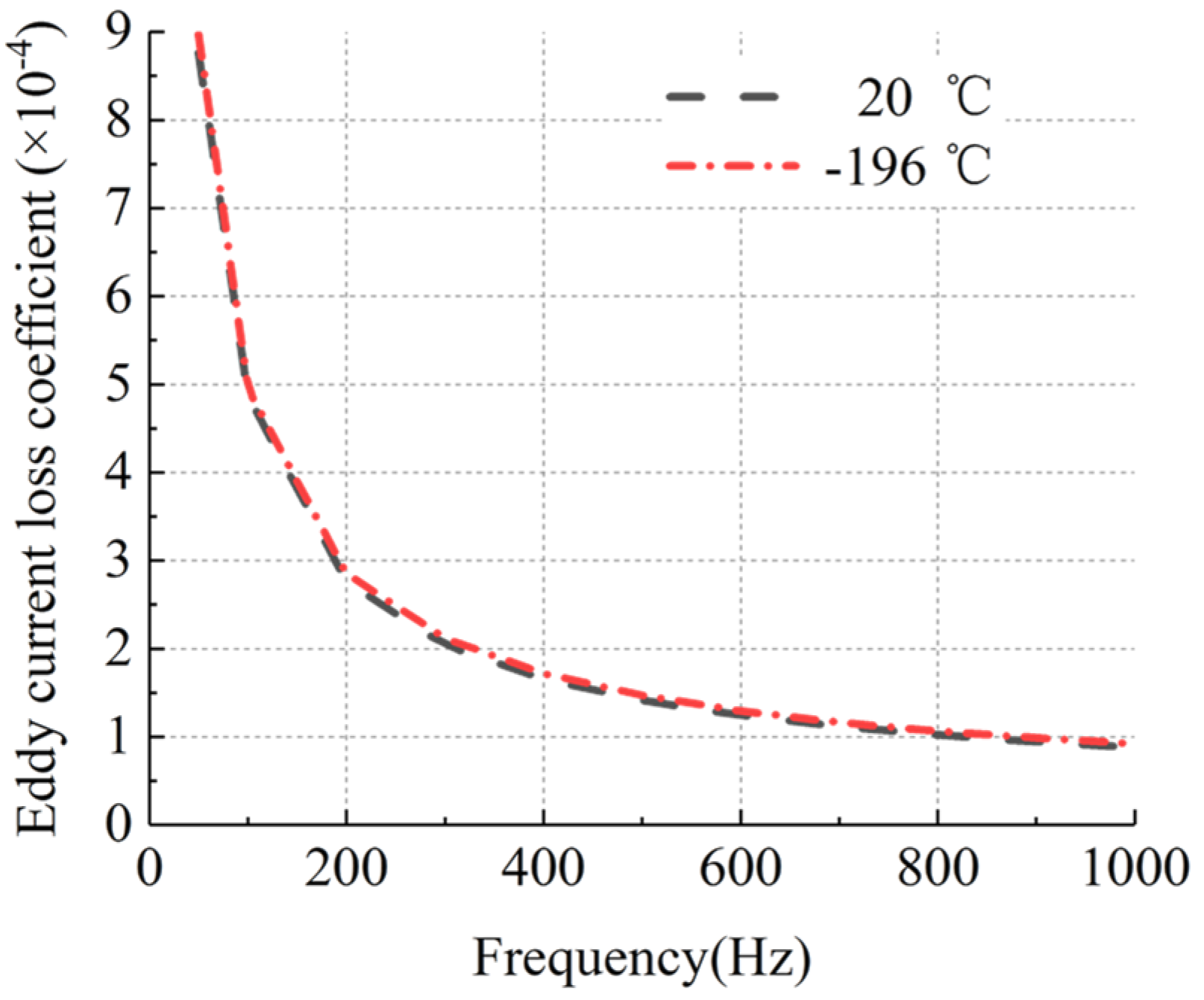
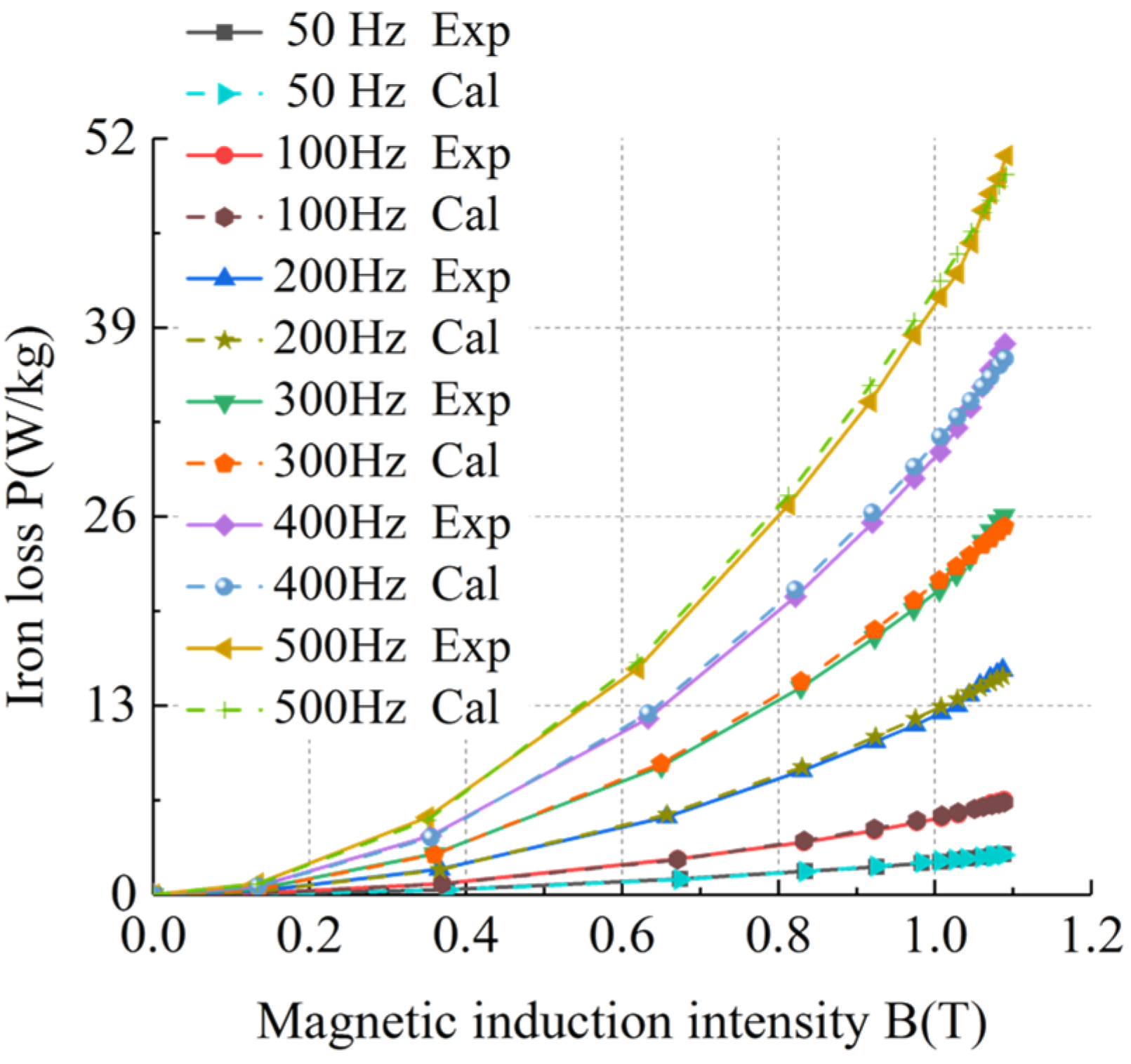
3.1. Basic Iron Loss Model of Silicon Steel Sheets
3.2. Feasibility Verification of the Improved Basic Iron Loss Model of Silicon Steel Sheets
4. Research on the Basic Iron Loss for Motors
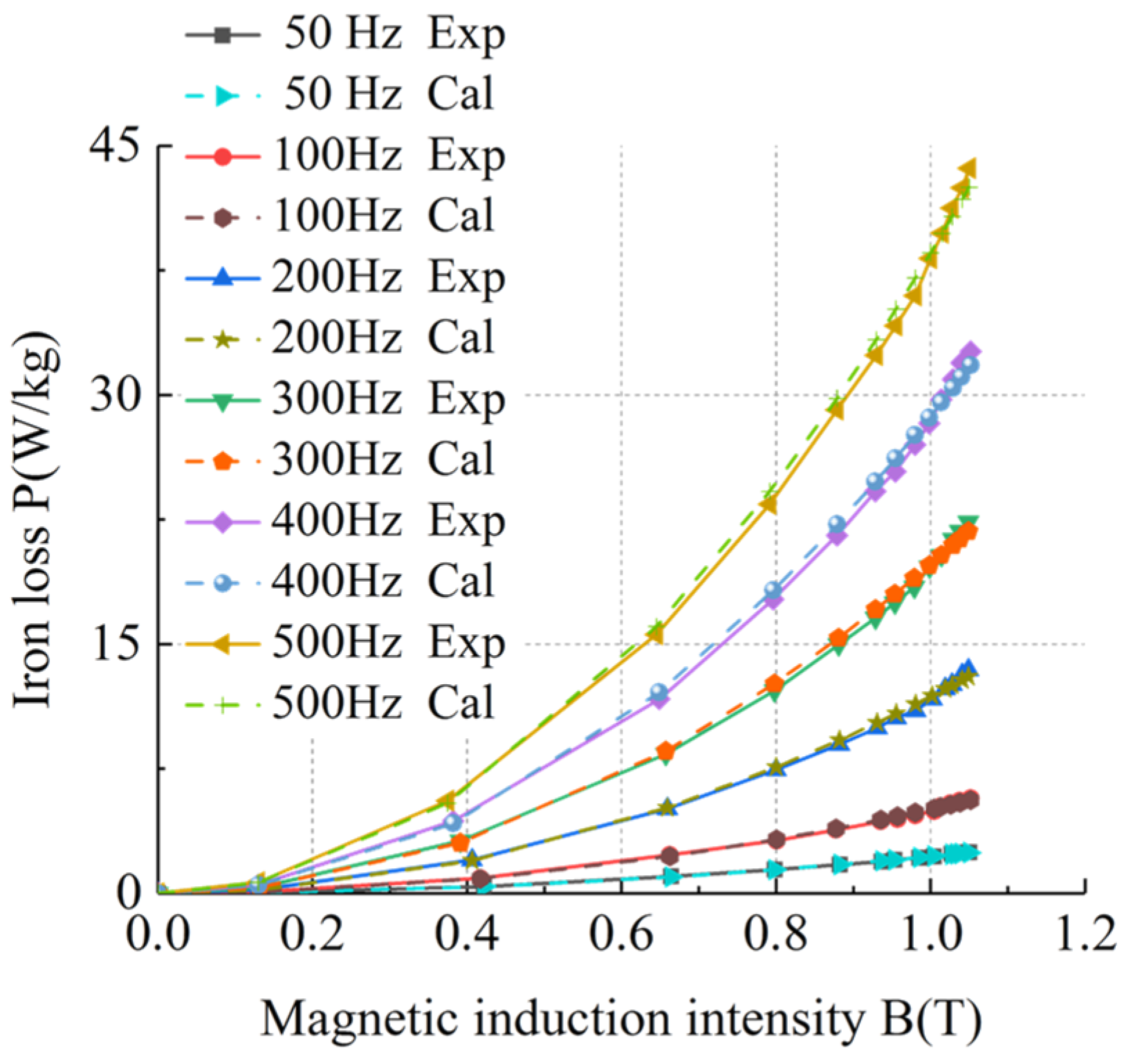
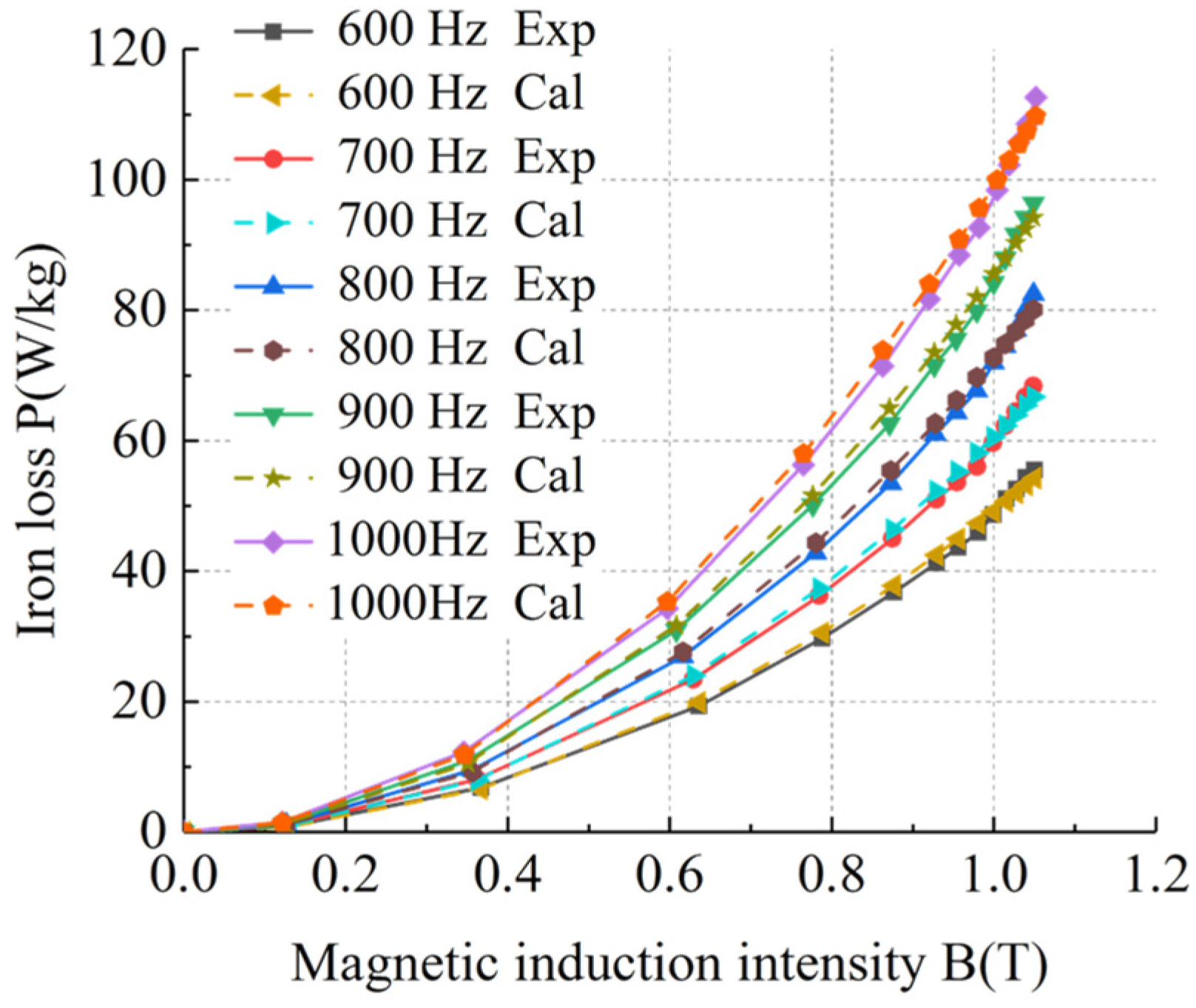
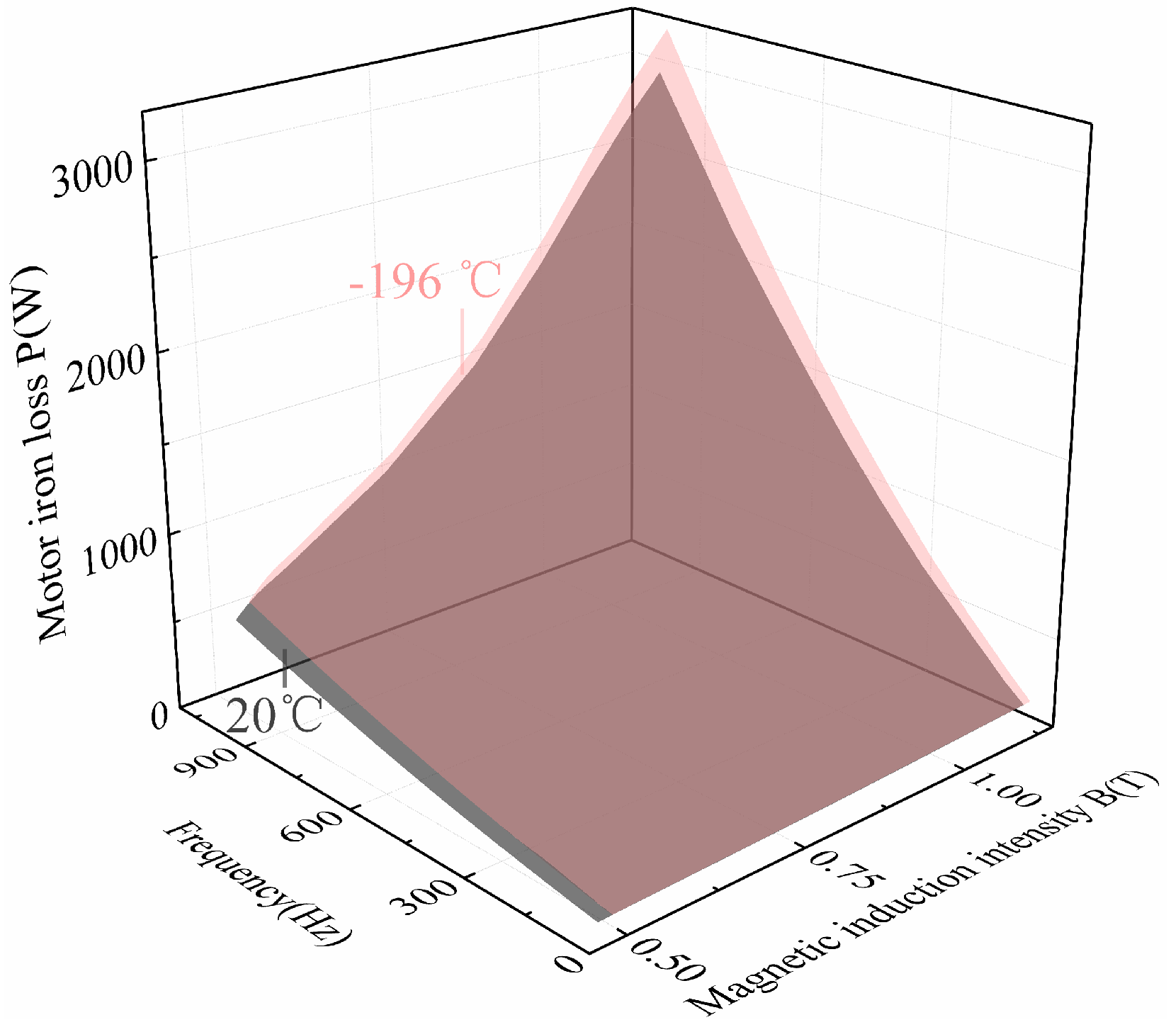
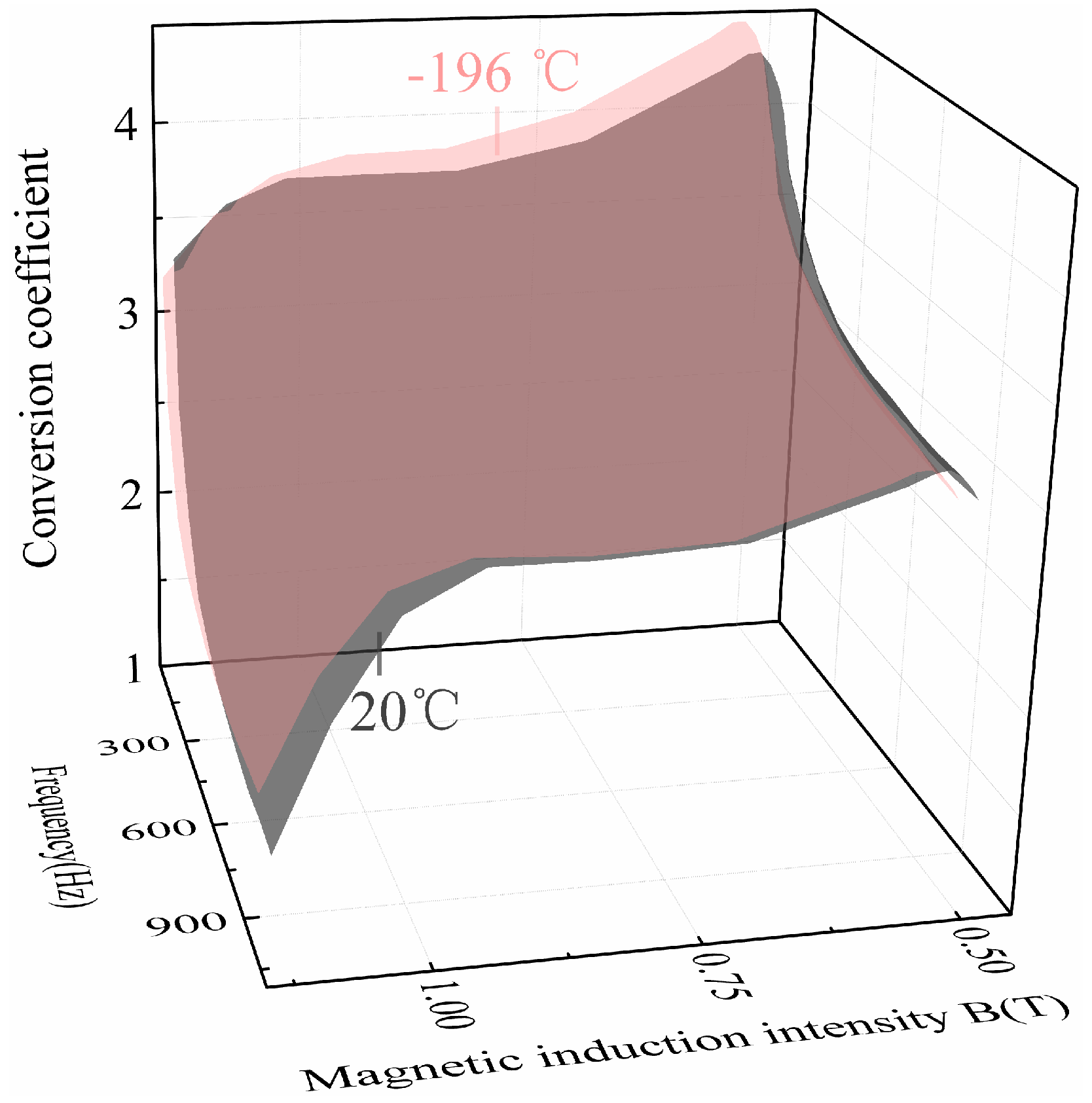
4.1. Basic Iron Loss Model of Motors
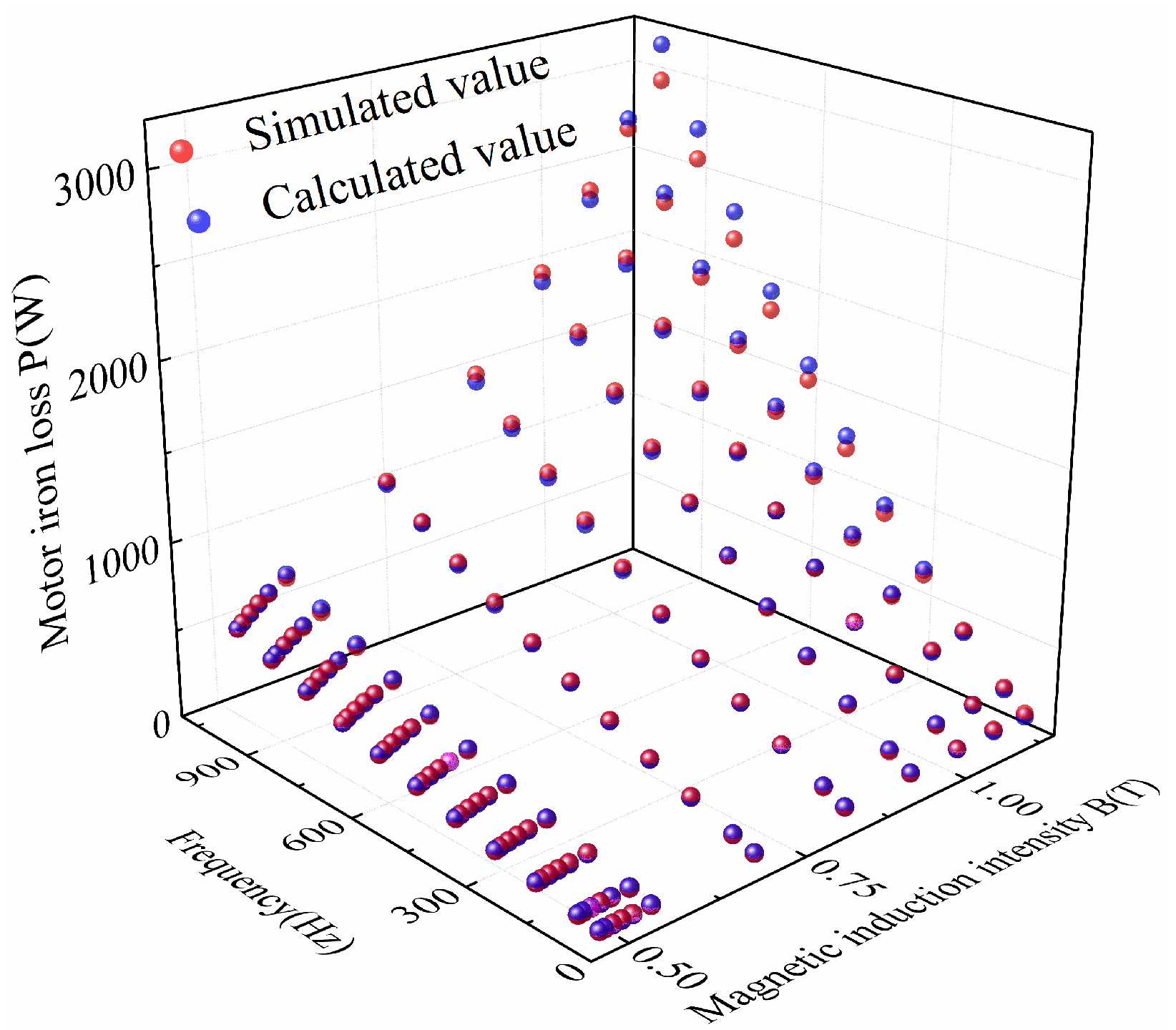
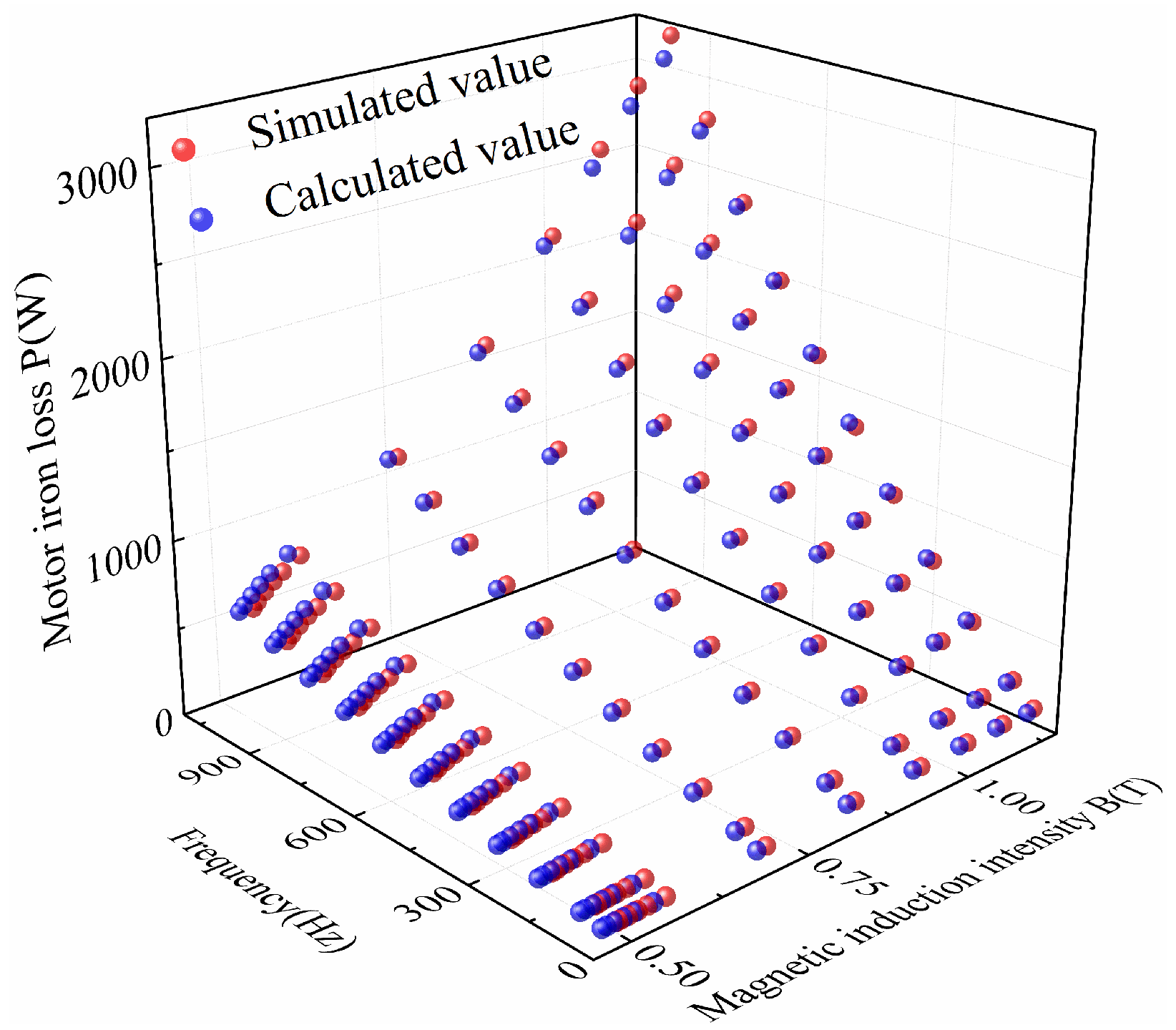
4.2. Feasibility Verification of the Basic Iron Loss Model of Motors
5. Conclusions
- Under the same magnetic field intensity, the magnetic induction intensity of the silicon steel sheets at ultra-low temperature was higher than that at room temperature.
- Under the same frequency and the same magnetic induction intensity, the iron loss of silicon steel sheets at ultra-low temperature was always higher than the iron loss at room temperature.
- The magnetization curve of the silicon steel sheets after being restored to room temperature from ultra-low temperature basically coincided with the magnetization curves at initial room temperature, without any loss of magnetization characteristics. Therefore, the tested silicon steel sheets can repeatedly work at ultra-low temperatures and room temperature.
- Based on the basic iron loss model of silicon steel sheets, the improved models of basic iron loss of silicon steel sheets were obtained by fitting the hysteresis loss coefficient and eddy current loss coefficient using the least squares method at room temperature and ultra-low temperature. The accuracy and feasibility of the improved basic iron loss models of silicon steel sheets were verified.
- Based on the improved basic iron loss model of silicon steel sheets, the basic iron loss models for motors were obtained by finite element simulation and comparative analysis at room temperature and ultra-low temperature. The accuracy and feasibility of the basic iron loss models for motors were verified.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mei, Q.; Hu, Q.; Liu, X.; Zhao, R.; Yang, C.; Wang, P.; Qi, Y.; Yang, Y.; Yuan, Q. YUAN Qirui, Research on the Evolution of Global LNG Maritime Transportation Network and Trade Condition of China. J. Geo-Inf. Sci. 2022, 24, 1701–1716. [Google Scholar]
- Huang, H. Characteristics and development trends of the global LNG market in 2021. Int. Pet. Econ. 2022, 30, 79–91. [Google Scholar]
- Zhou, S.W.; Zhu, J.; Shan, T.; Fu, Q.; Zhang, D.; Wang, J. Development status and outlook of natural gas and LNG industry in China. China Offshore Oil Gas 2022, 34, 1–8. [Google Scholar]
- Hou, Z.; Luo, J.; Cao, C.; Ding, G. Development and Contribution of Natural Gas Industry Under the Goal of Carbon of Carbon Neutrality in Chuna. Adv. Eng. Sci. 2023, 55, 243–252. [Google Scholar]
- Lyu, Y. Opportunities and Challenges of China’s natural gas development under the background of carbon peaking and carbon neutrality goals. Ecol. Environ. 2022, 114, 91–93. [Google Scholar]
- Zhao, G.; Kong, D.; Gao, X. Performance Difference Study on Permanent Magnet Synchronous Motor Based on Soft Magnetic Composite Material and Silicon Steel Sheet. Trans. China Electrotech. Soc. 2018, 33, 75–81. [Google Scholar]
- Zhang, D.; Zhao, H.; Wang, Y.; Xu, G.; Liu, X. A Piecewise Variable Coefficient Model for Precise Analysis on Iron Losses of Electrical Machines. Trans. China Electrotech. Soc. 2016, 31, 16–24. [Google Scholar]
- He, Z.; Zhu, L.; Wang, Z.; Koh, C.-S. Anomalous Loss and Hysteresis Loop in Electrical Steel Sheet. IEEE Trans. Magn. 2021, 57, 6300804. [Google Scholar] [CrossRef]
- Miyagi, D.; Otome, D.; Nakano, M.; Takahashi, N. Measurement of Magnetic Properties of Nonoriented Electrical Steel Sheet at Liquid Nitrogen Temperature Using Single Sheet Tester. IEEE Trans. Magn. 2010, 46, 314–317. [Google Scholar]
- Breining, P.; Veige, M.; Doppelbauer, M.; Liu, Y.; Noe, M. Iron loss measurement of nonoriented silicon and cobalt iron electrical steel sheets at liquid nitrogen temperature using ring specimen. In Proceedings of the 2017 IEEE International Electric Machines and Drives Conference (IEMDC), Miami, FL, USA, 21–24 May 2017. [Google Scholar]
- Luo, Y.; Zhao, H.; Yao, B.; Chen, W. Engineering Computation Methods Analysis of Iron Losses of AC Electric Machines. Electr. Mach. Control Appl. 2010, 37, 1–10. [Google Scholar]
- Li, M.; Wang, W. Correction and Analysis for the Iron Loss Coefficient of Permanent Magnet Motor. Micromotors 2016, 49, 33–36. [Google Scholar]
- Zhu, L.F.; Tong, W.M.; Han, X.Y.; Zhu, J.G. Iron loss research of amorphous alloy motor by considering the influences of solidifying and annealing on stator core. COMPEL-Int. J. Comput. Math. Electr. Electron. Eng. 2017, 36, 1612–1622. [Google Scholar] [CrossRef]
- Meng, R.; Kuang, J. The Application of the Method of Least Squares Parameter Fitting of Core Loss. Mod. Sci. Instrum. 2013, 147, 91–96. [Google Scholar]
- Zhang, J.; Su, J.; Fu, R.; Bian, C. Calculation of Stator Iron Losses in a High-speed AC Permanent Magnet Generator. Micromotors 2014, 47, 10–14. [Google Scholar]

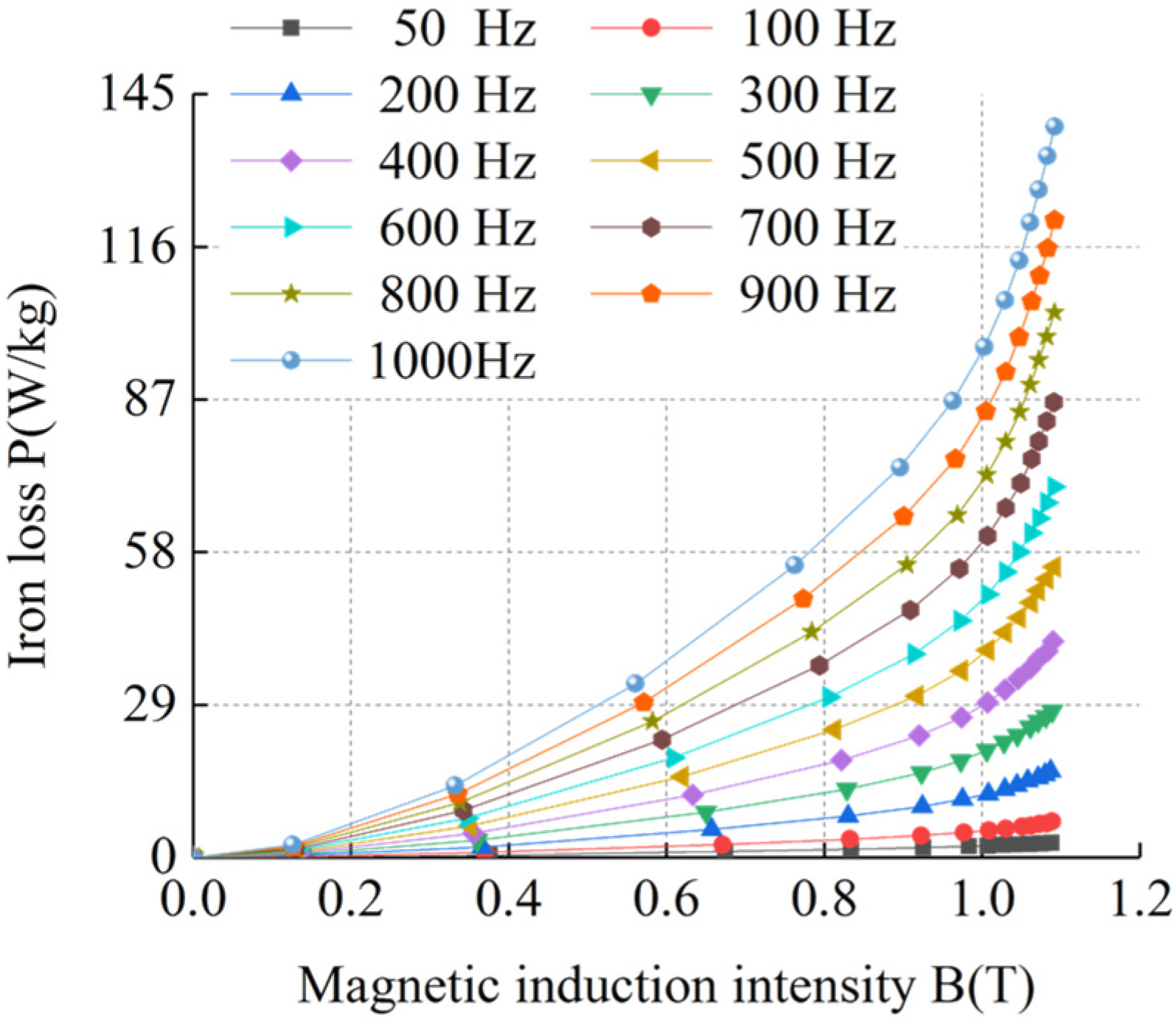



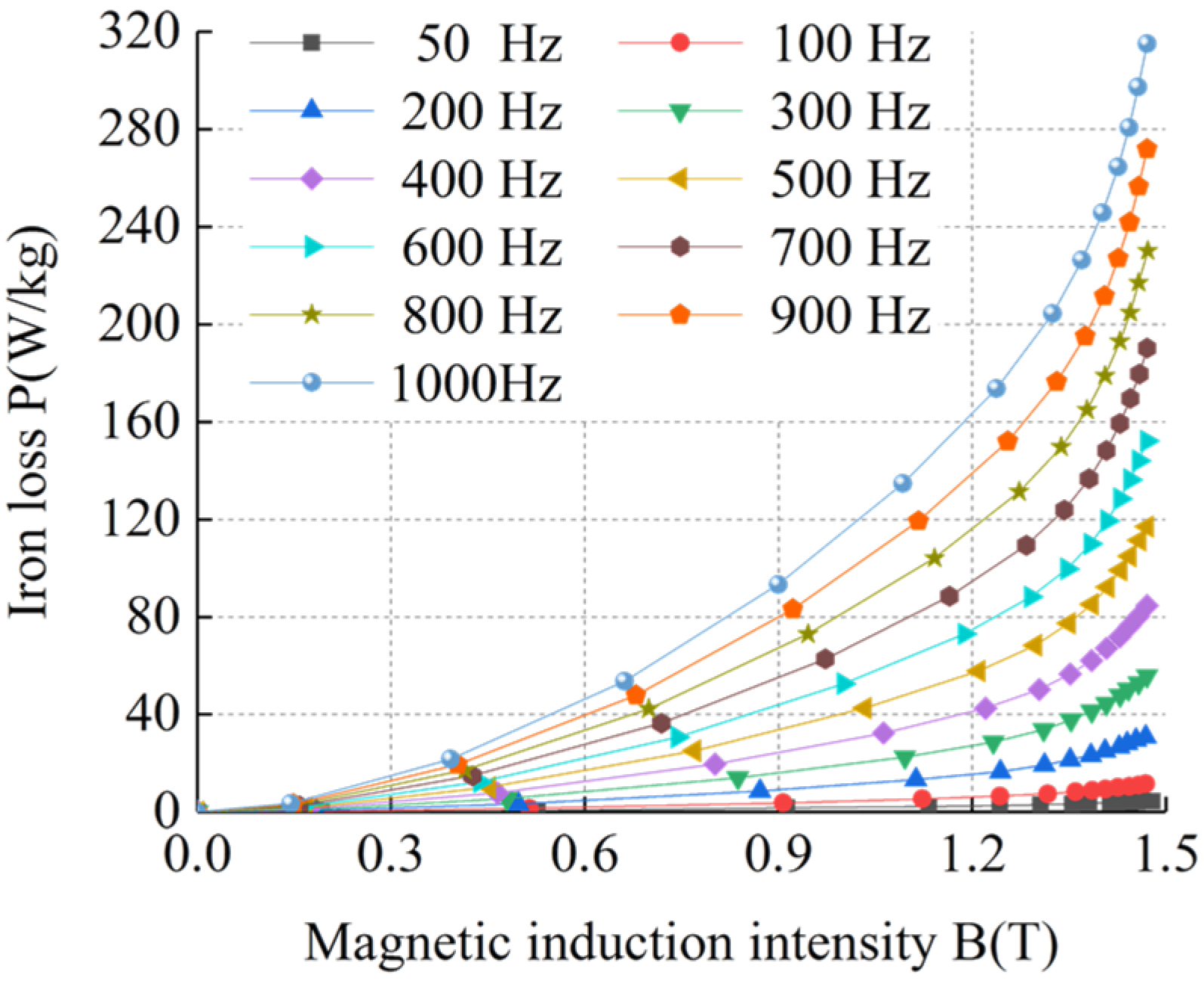
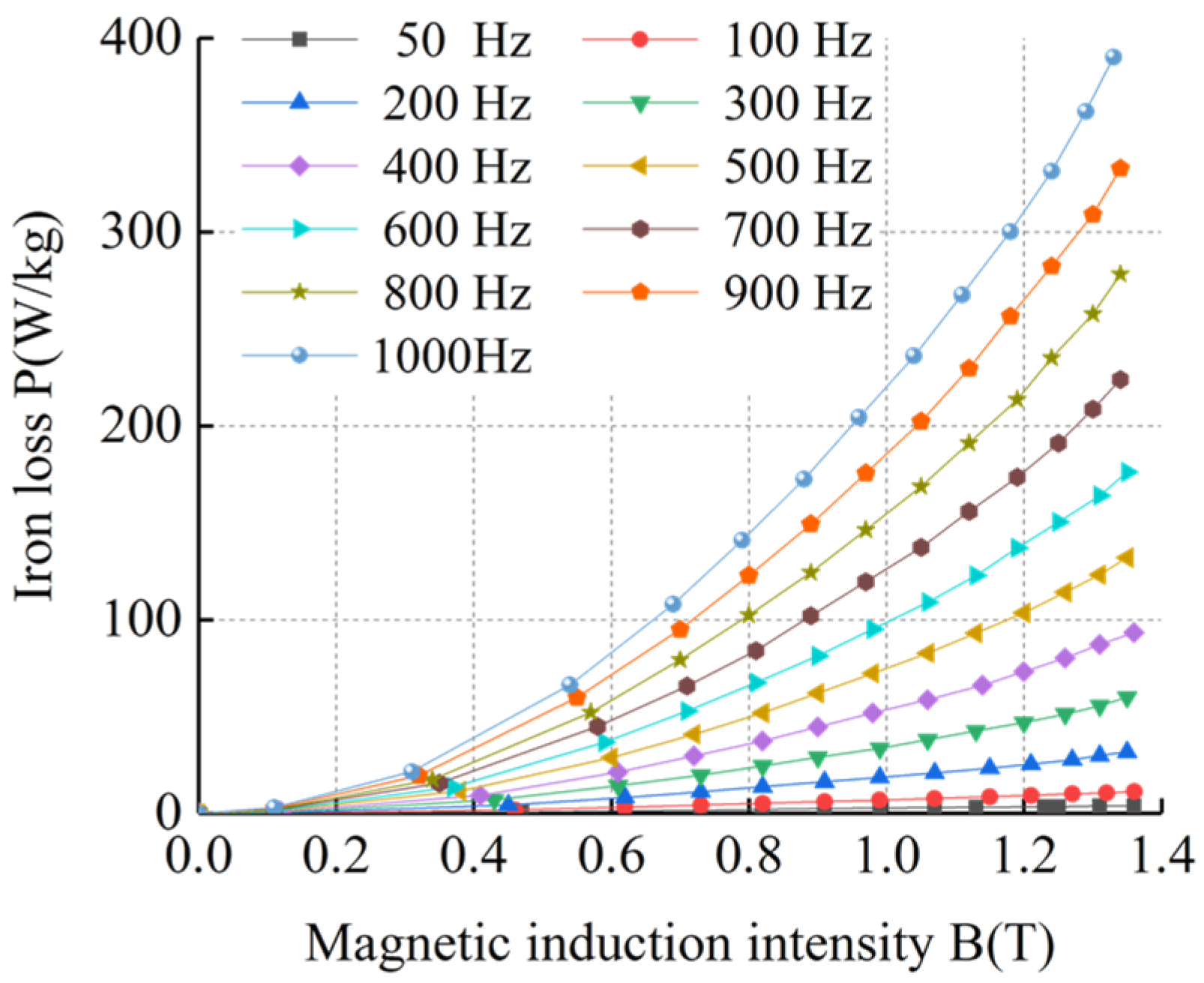
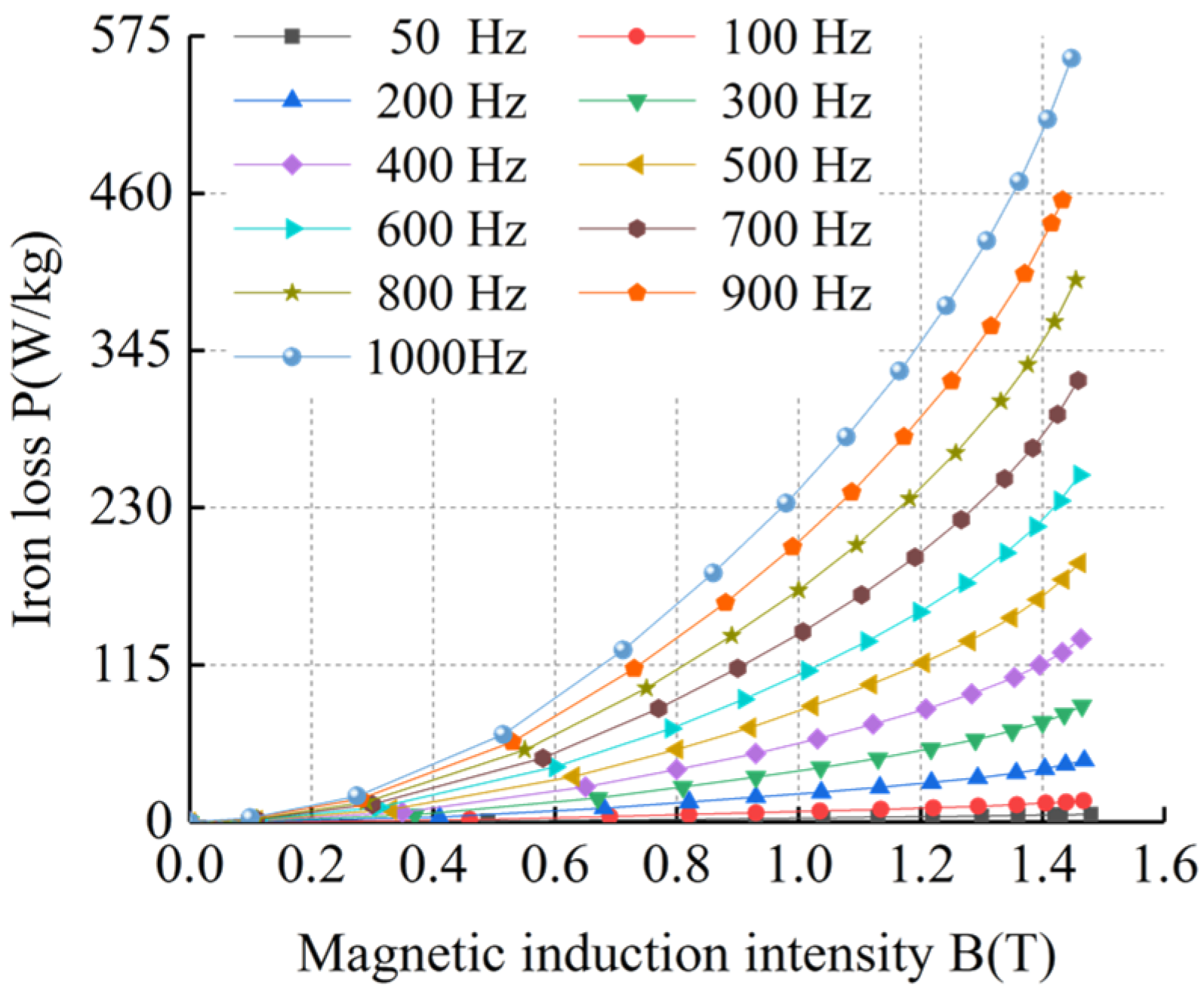




| Frequency (Hz) | (×10−3) | (×10−4) | |
|---|---|---|---|
| 50 | 0.520 | 8.764 | 20 |
| 100 | 1.600 | 4.872 | 20 |
| 200 | 3.094 | 2.759 | 20 |
| 300 | 4.308 | 2.041 | 20 |
| 400 | 5.100 | 1.656 | 20 |
| 500 | 5.946 | 1.414 | 20 |
| 600 | 6.651 | 1.246 | 20 |
| 700 | 7.273 | 1.119 | 20 |
| 800 | 7.785 | 1.021 | 20 |
| 900 | 8.114 | 0.944 | 20 |
| 1000 | 8.634 | 0.876 | 20 |
| Frequency (Hz) | (×10−3) | (×10−4) | |
|---|---|---|---|
| 50 | 0.513 | 8.973 | 20 |
| 100 | 1.593 | 4.947 | 20 |
| 200 | 2.717 | 2.852 | 20 |
| 300 | 3.253 | 2.118 | 20 |
| 400 | 3.729 | 1.719 | 20 |
| 500 | 4.139 | 1.469 | 20 |
| 600 | 4.439 | 1.294 | 20 |
| 700 | 4.707 | 1.164 | 20 |
| 800 | 4.878 | 1.065 | 20 |
| 900 | 4.935 | 0.987 | 20 |
| 1000 | 4.993 | 0.923 | 20 |
| Frequency (Hz) | Fitted Value (×10−3) (20 °C) | Fitting Error (20 °C) | Fitted Value (×10−3) (−196 °C) | Fitting Error (−196 °C) |
|---|---|---|---|---|
| 50 | 0.518 | 0.333% | 0.510 | 0.542% |
| 100 | 1.595 | 0.292% | 1.608 | 0.925% |
| 200 | 3.115 | 0.680% | 2.656 | 2.257% |
| 300 | 4.239 | 1.598% | 3.298 | 1.375% |
| 400 | 5.164 | 1.252% | 3.777 | 1.297% |
| 500 | 5.954 | 0.139% | 4.146 | 0.167% |
| 600 | 6.639 | 0.187% | 4.430 | 0.200% |
| 700 | 7.233 | 0.544% | 4.649 | 1.223% |
| 800 | 7.751 | 0.436% | 4.819 | 1.218% |
| 900 | 8.202 | 1.082% | 4.949 | 0.286% |
| 1000 | 8.594 | 0.460% | 5.050 | 1.139% |
| Frequency (Hz) | Fitted Value (×10−4) (20 °C) | Fitting Error (20 °C) | Fitted Value (×10−4) (−196 °C) | Fitting Error (−196 °C) |
|---|---|---|---|---|
| 50 | 8.758 | 0.068% | 8.960 | 0.150% |
| 100 | 4.877 | 0.095% | 4.953 | 0.131% |
| 200 | 2.734 | 0.920% | 2.827 | 0.864% |
| 300 | 2.065 | 1.158% | 2.144 | 1.248% |
| 400 | 1.676 | 1.234% | 1.739 | 1.143% |
| 500 | 1.412 | 0.118% | 1.464 | 0.358% |
| 600 | 1.228 | 1.433% | 1.274 | 1.531% |
| 700 | 1.099 | 1.766% | 1.143 | 1.786% |
| 800 | 1.009 | 1.177% | 1.053 | 1.158% |
| 900 | 0.946 | 0.241% | 0.990 | 0.334% |
| 1000 | 0.902 | 2.889% | 0.947 | 2.560% |
| Frequency (Hz) | Average Error (20 °C) | Average Error (−196 °C) |
|---|---|---|
| 50 | 1.690% | 2.086% |
| 100 | 2.589% | 2.535% |
| 200 | 2.122% | 2.699% |
| 300 | 2.364% | 2.480% |
| 400 | 2.528% | 2.372% |
| 500 | 2.688% | 2.387% |
| 600 | 2.386% | 2.326% |
| 700 | 2.268% | 2.212% |
| 800 | 2.426% | 2.402% |
| 900 | 2.437% | 2.478% |
| 1000 | 2.398% | 2.576% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, S.; Ge, B.; Wang, L.; Wang, Y. Research on the Material Characteristics and Loss Calculation Method of Cryogenic Permanent Magnet Motor Stator for LNG Pump. Energies 2024, 17, 2641. https://doi.org/10.3390/en17112641
Liu S, Ge B, Wang L, Wang Y. Research on the Material Characteristics and Loss Calculation Method of Cryogenic Permanent Magnet Motor Stator for LNG Pump. Energies. 2024; 17(11):2641. https://doi.org/10.3390/en17112641
Chicago/Turabian StyleLiu, Shuqi, Baojun Ge, Likun Wang, and Yue Wang. 2024. "Research on the Material Characteristics and Loss Calculation Method of Cryogenic Permanent Magnet Motor Stator for LNG Pump" Energies 17, no. 11: 2641. https://doi.org/10.3390/en17112641
APA StyleLiu, S., Ge, B., Wang, L., & Wang, Y. (2024). Research on the Material Characteristics and Loss Calculation Method of Cryogenic Permanent Magnet Motor Stator for LNG Pump. Energies, 17(11), 2641. https://doi.org/10.3390/en17112641





