Inner Flow Analysis of Kaplan Turbine under Off-Cam Conditions
Abstract
1. Introduction
2. Researched Kaplan Turbine Unit
2.1. Basic Parameters
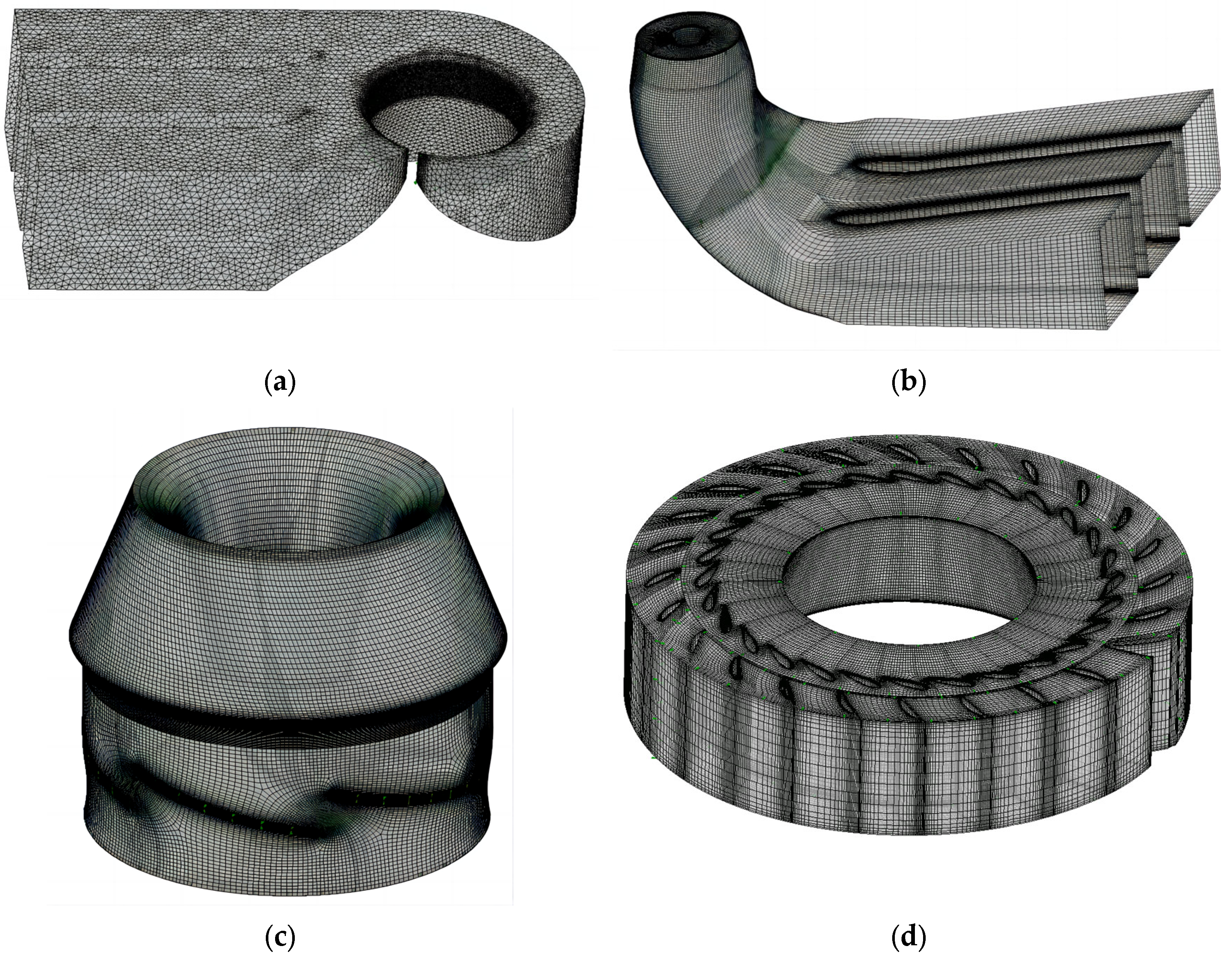
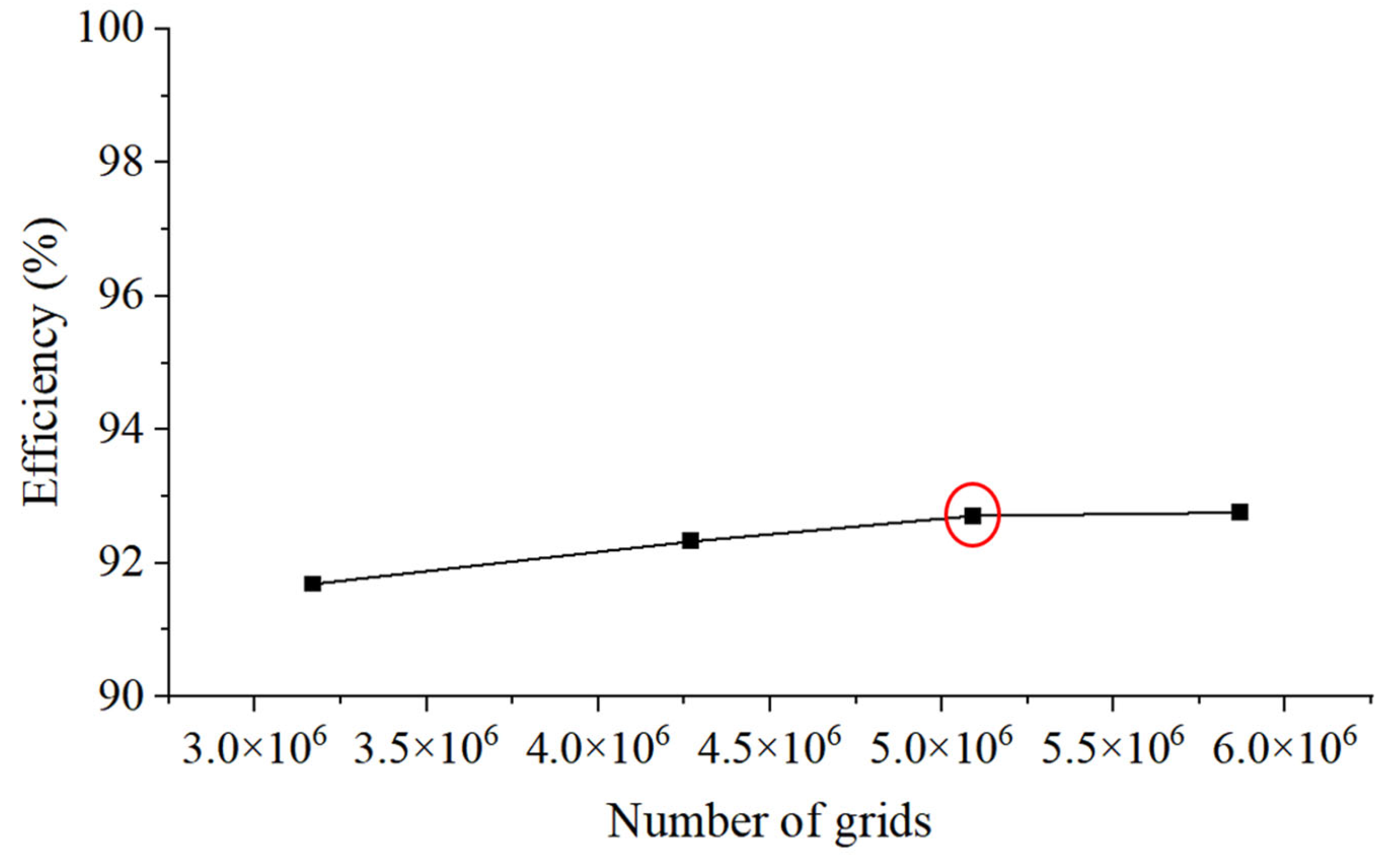
2.2. Model and Mesh
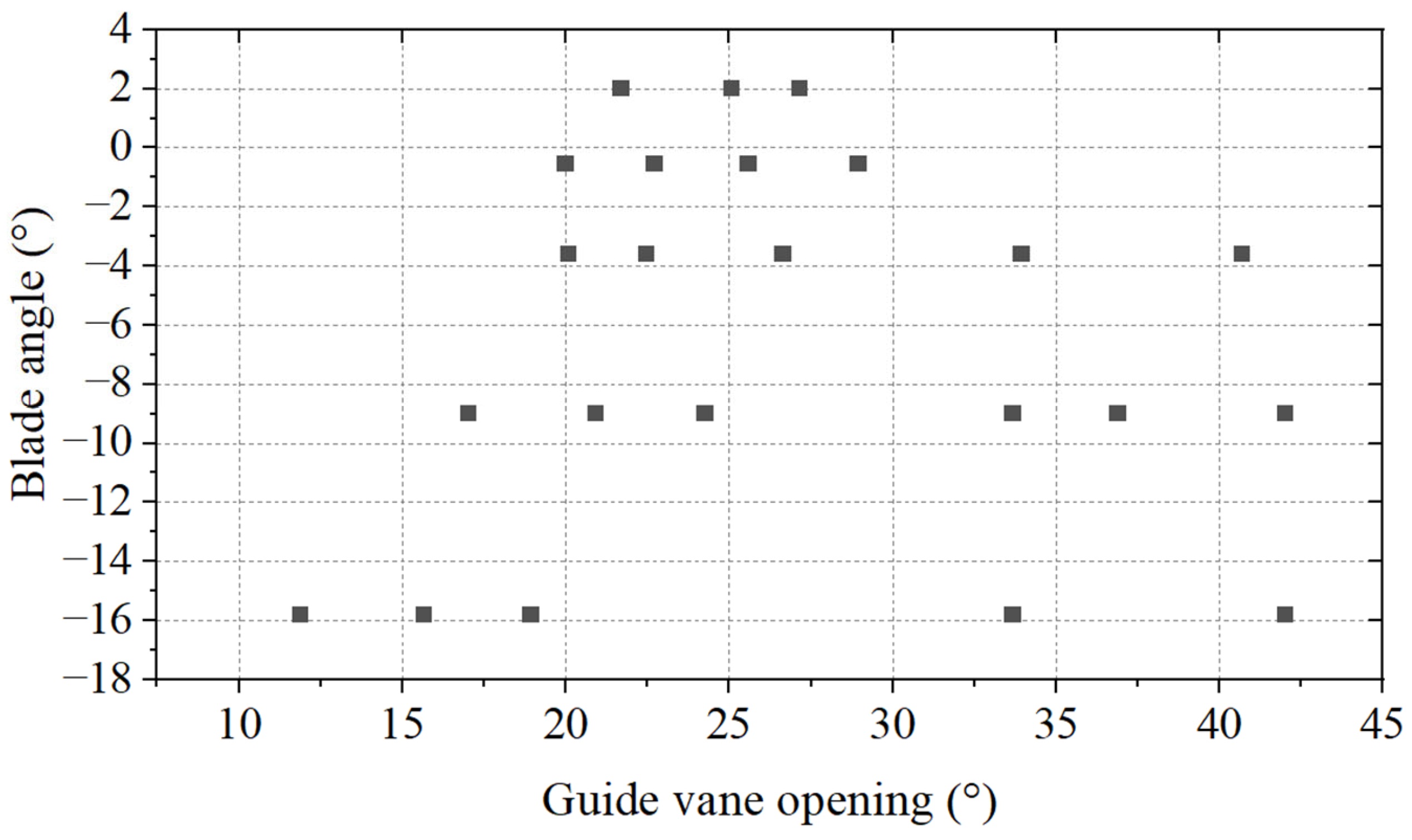
2.3. Boundary Conditions, Selection of Operating Points
3. Comparative Analysis of Different Working Conditions
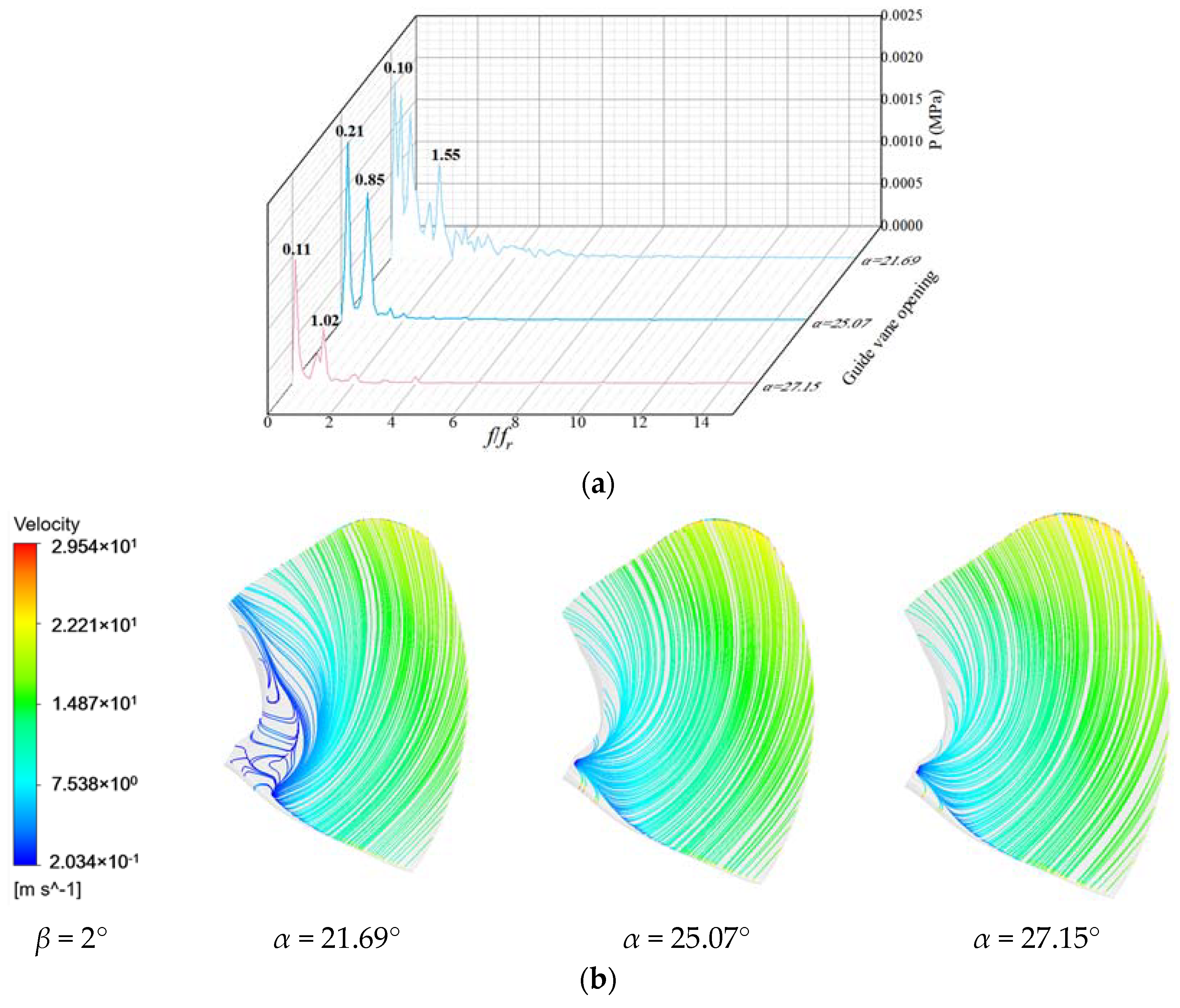
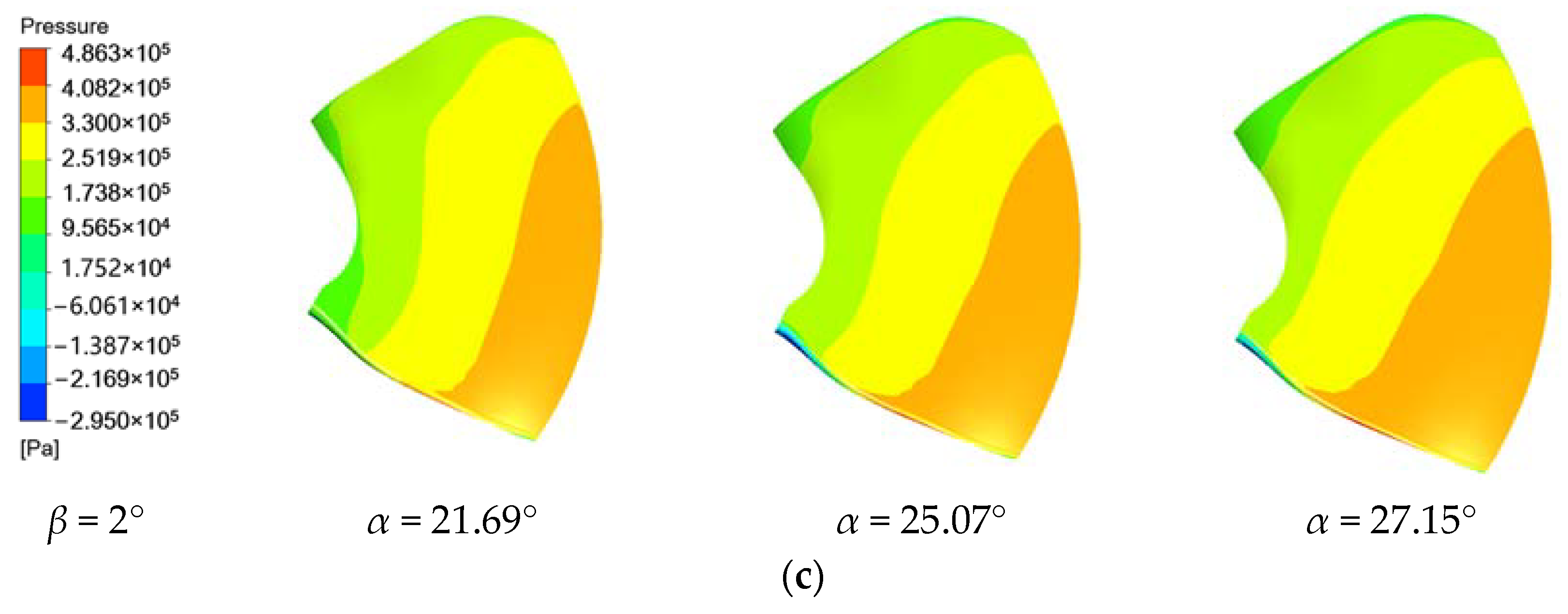
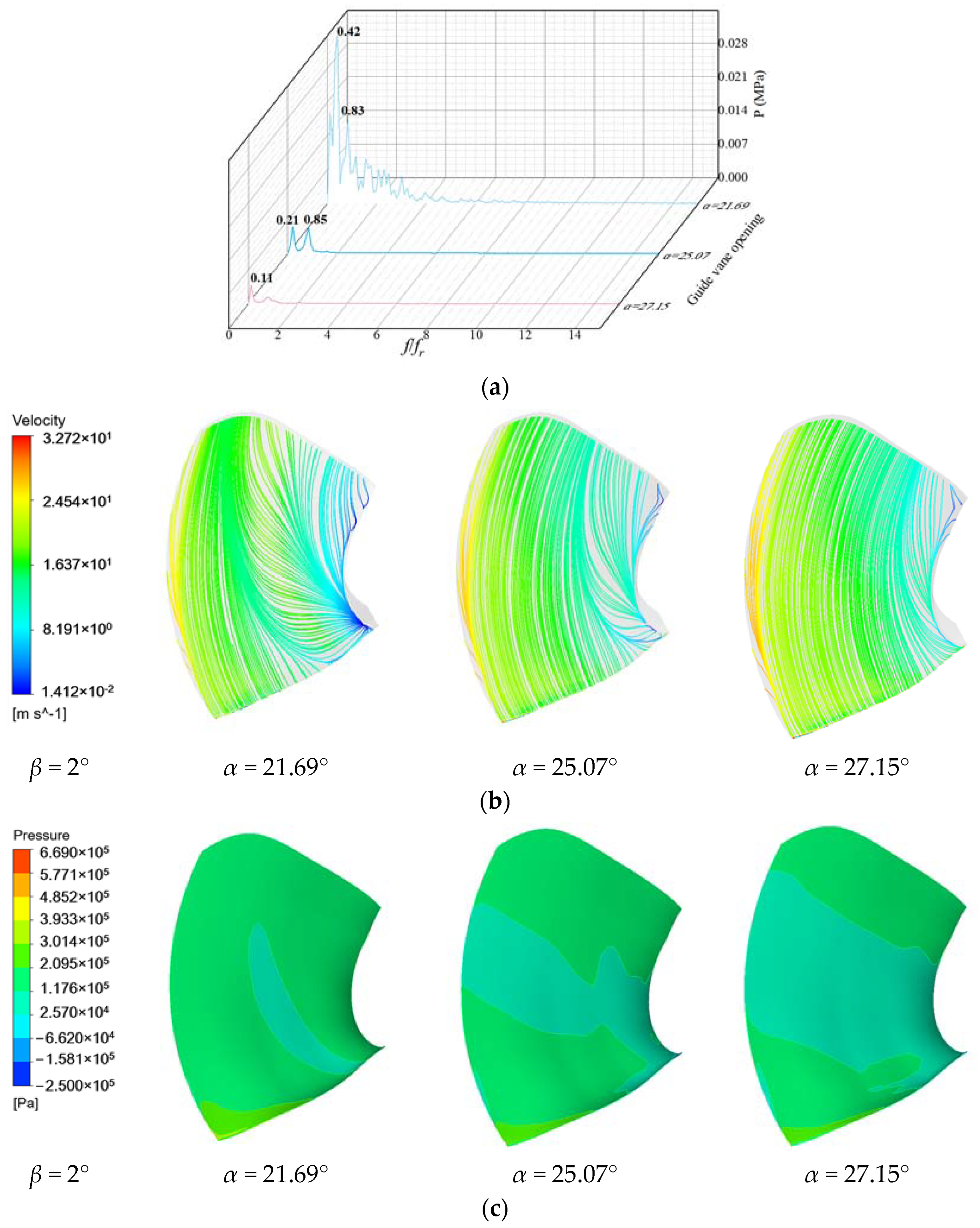
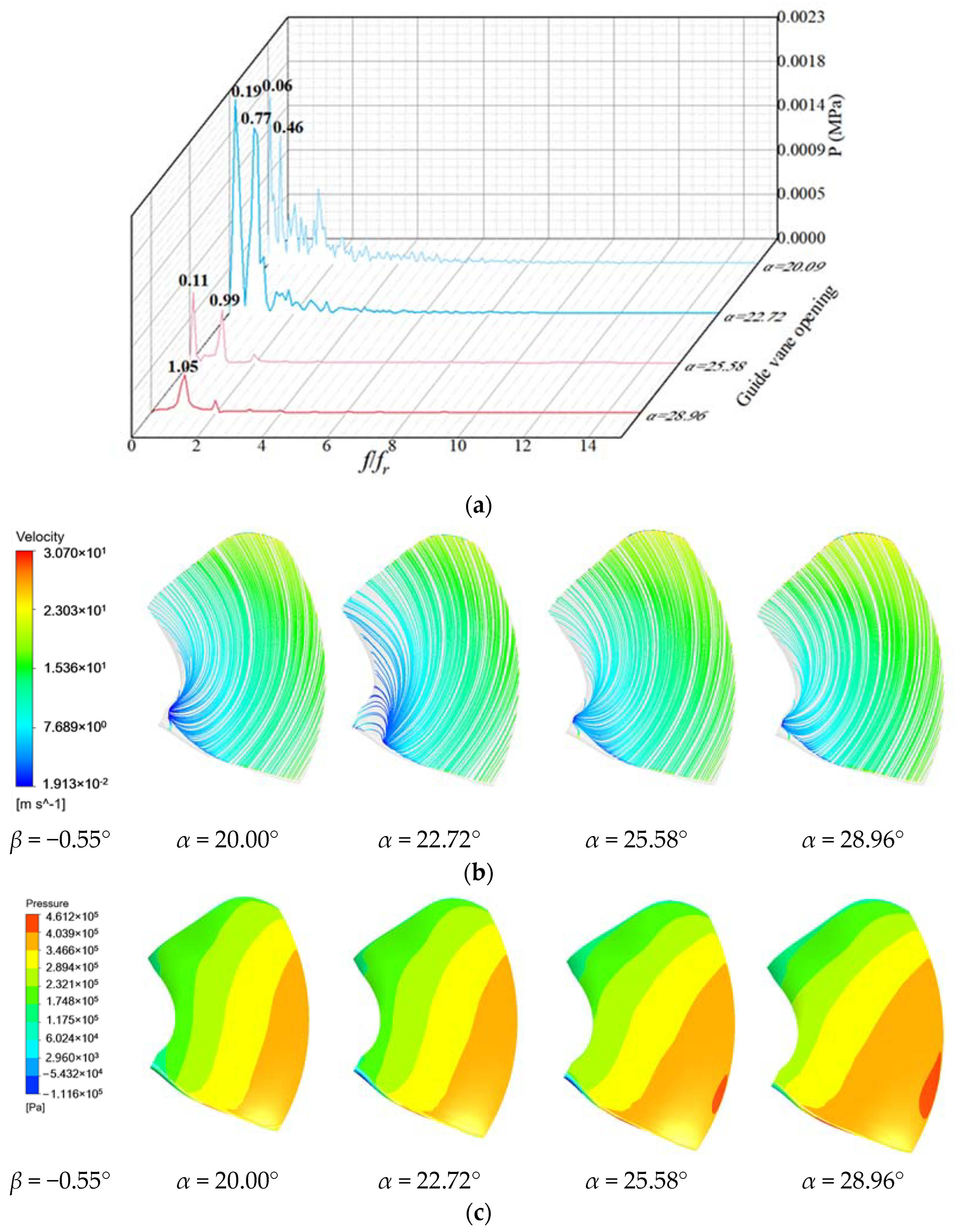
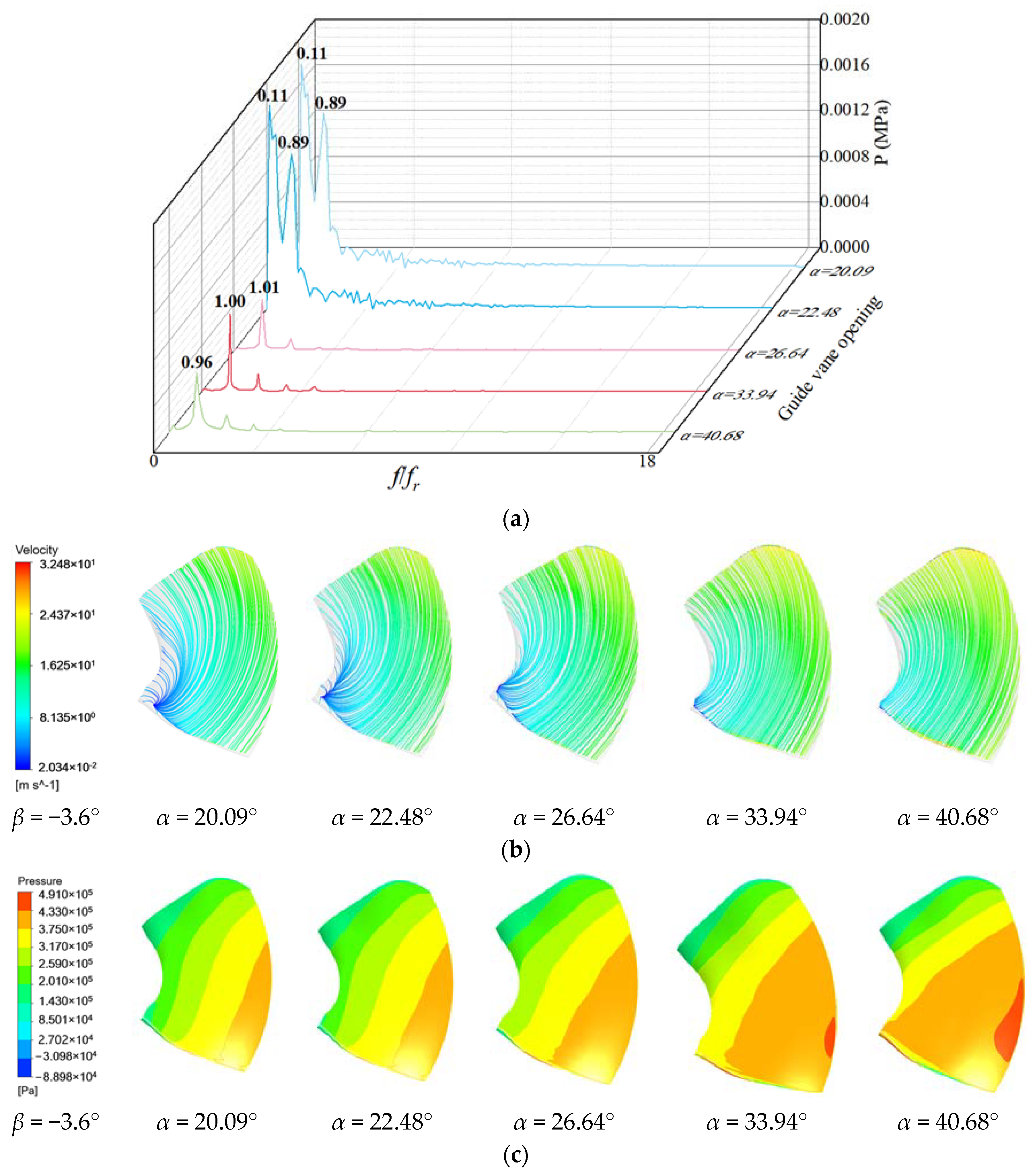
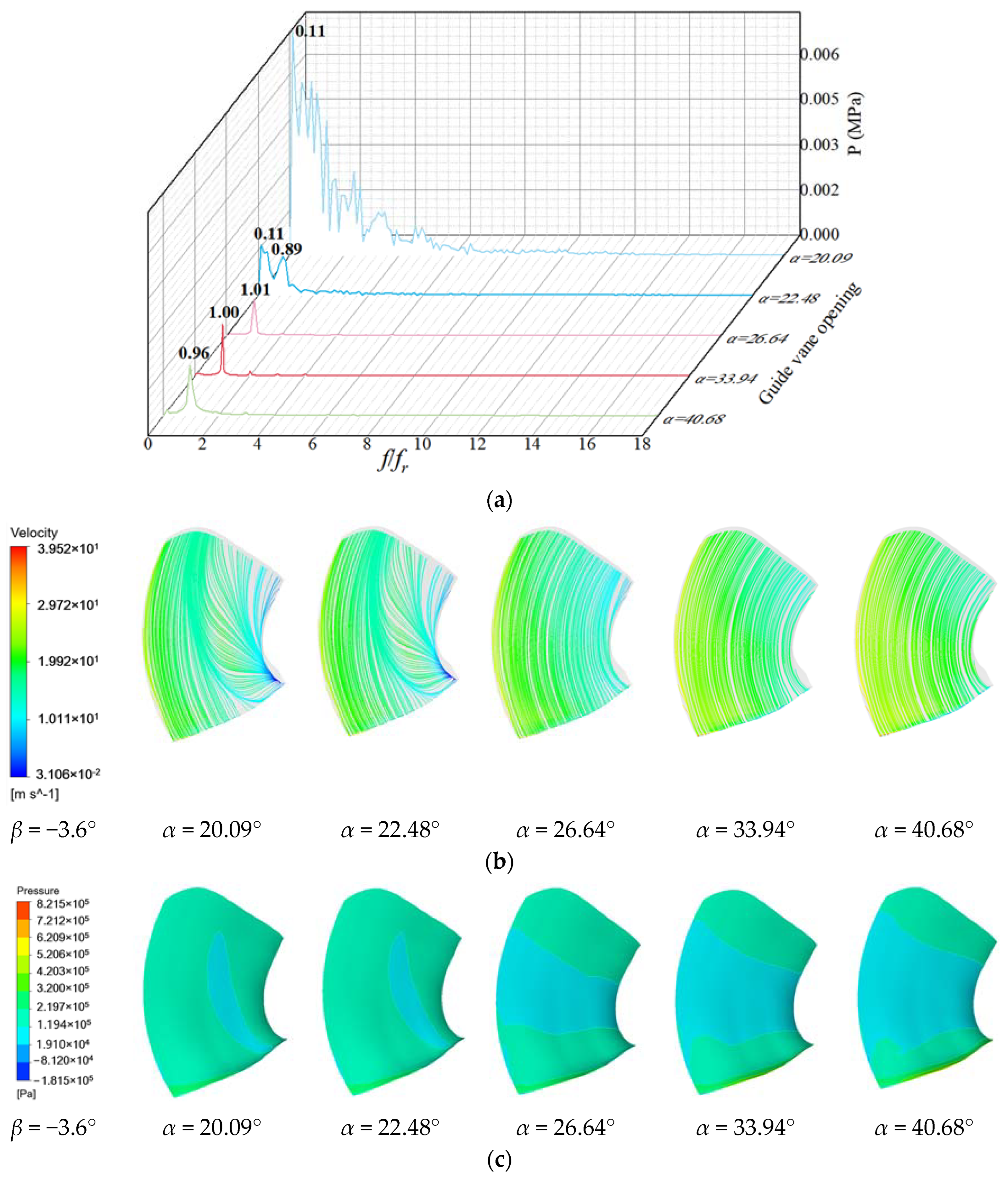
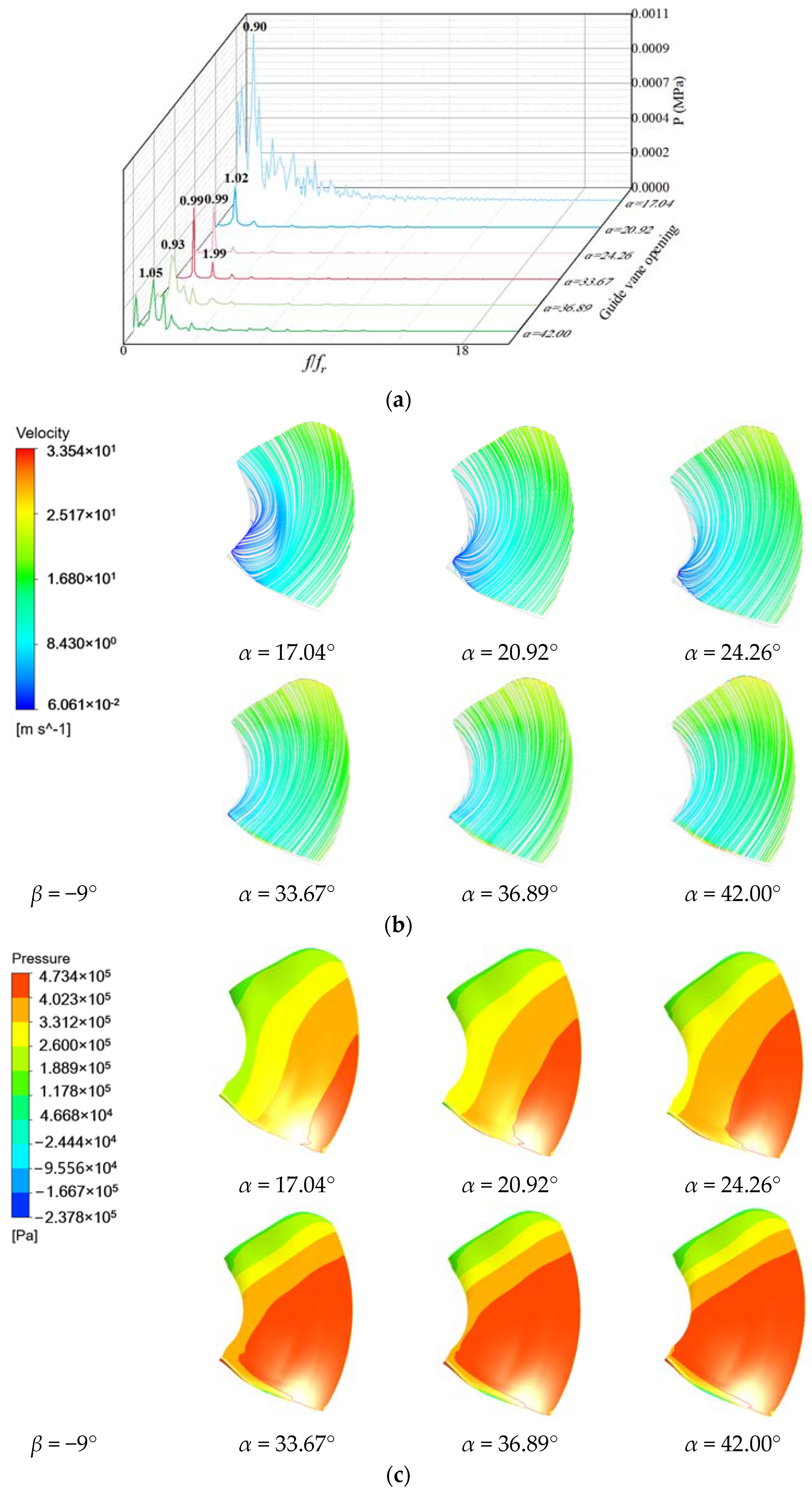
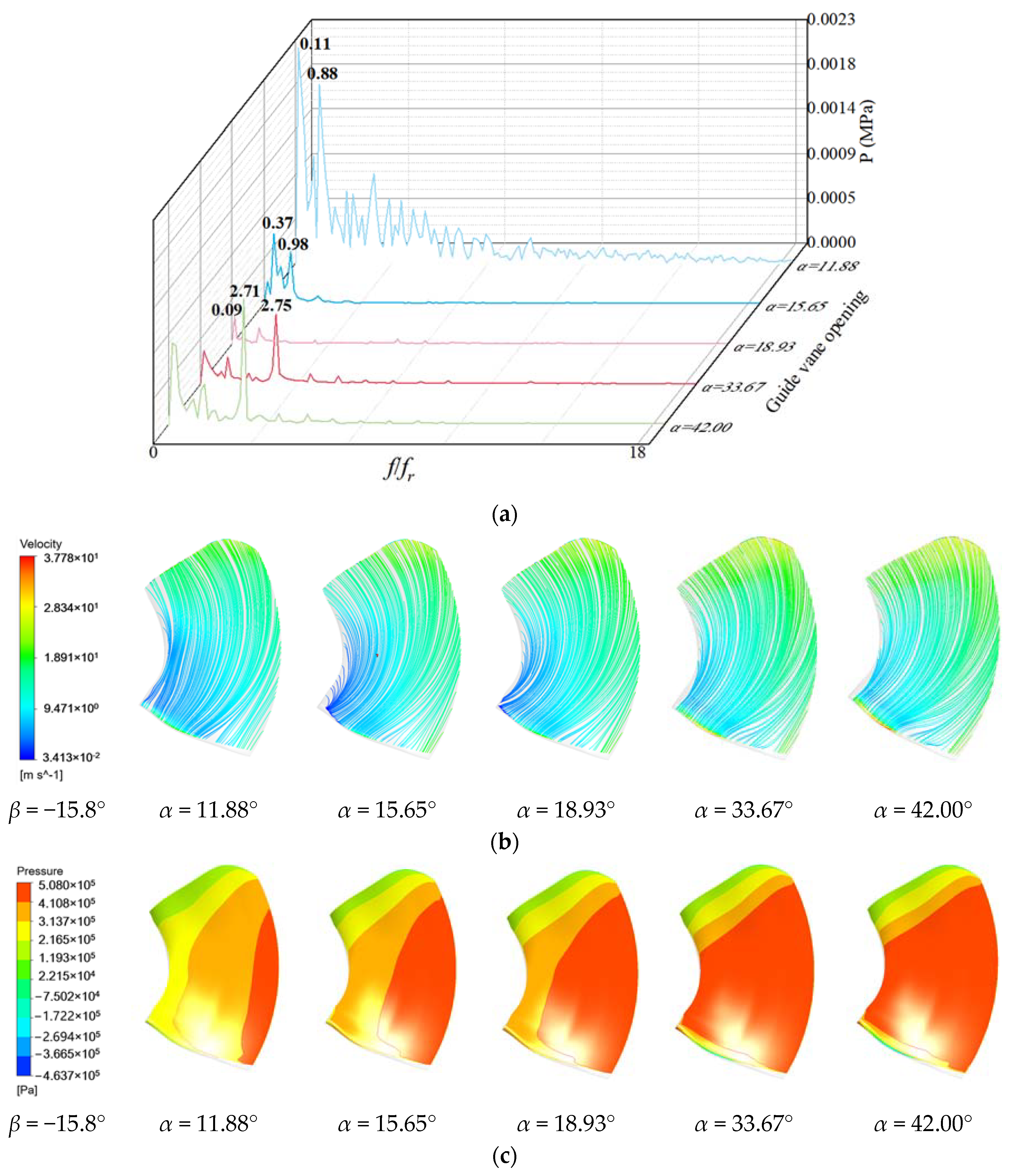
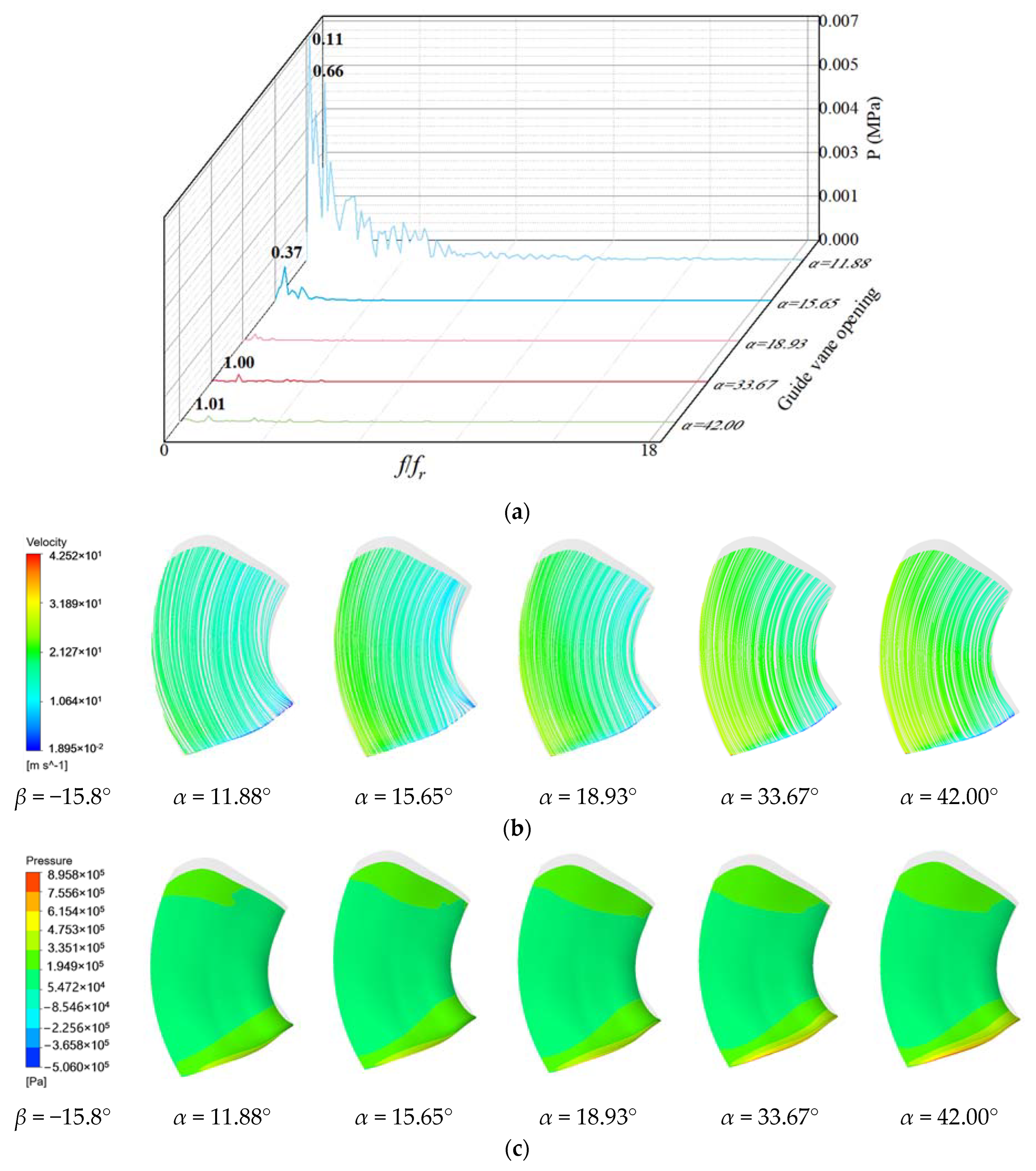
3.1. Flowline and Pressure Pulsation Comparison of Runner Blade
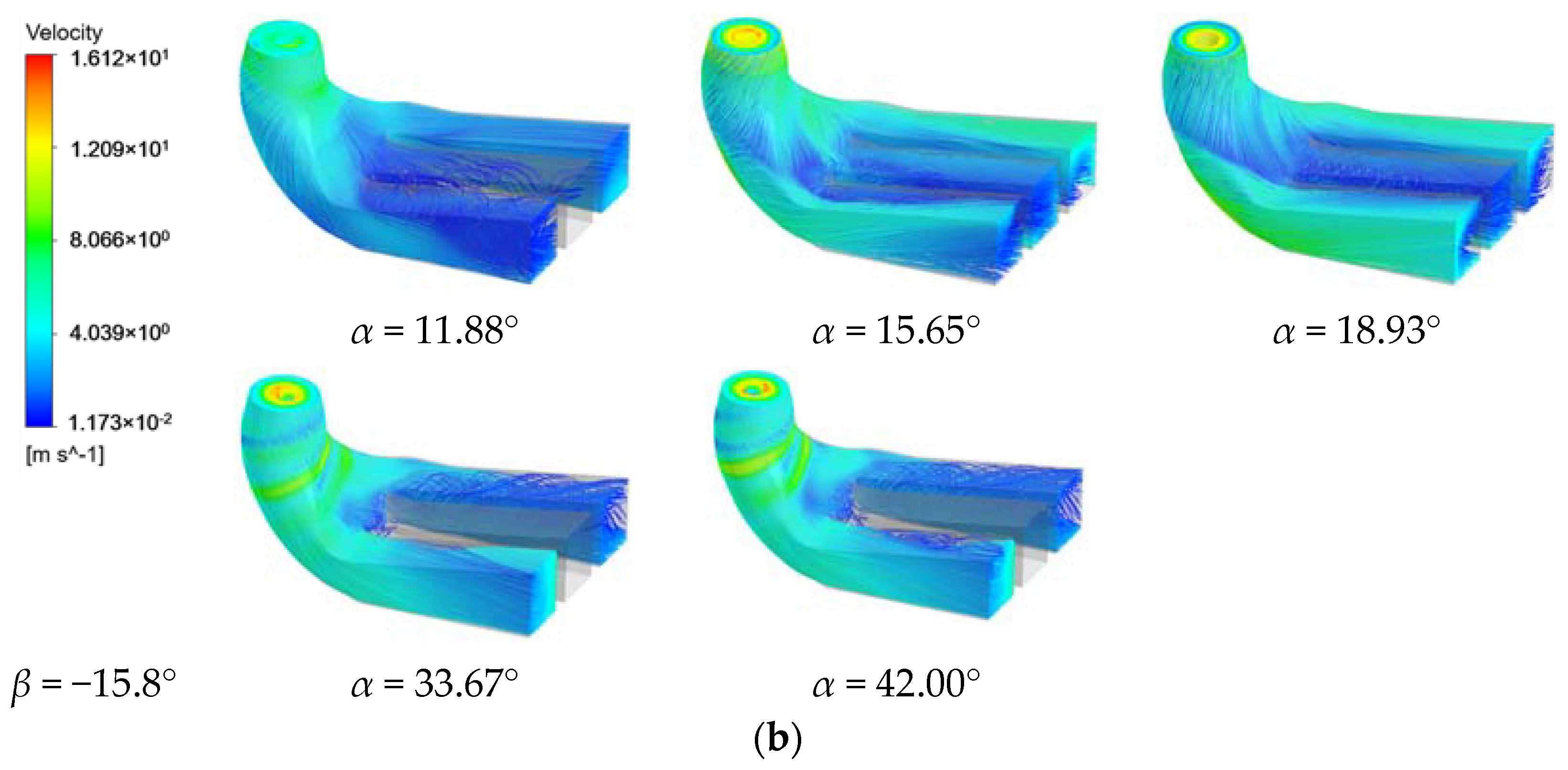
3.2. Flowline and Pressure Pulsation Comparison of Draft Tube
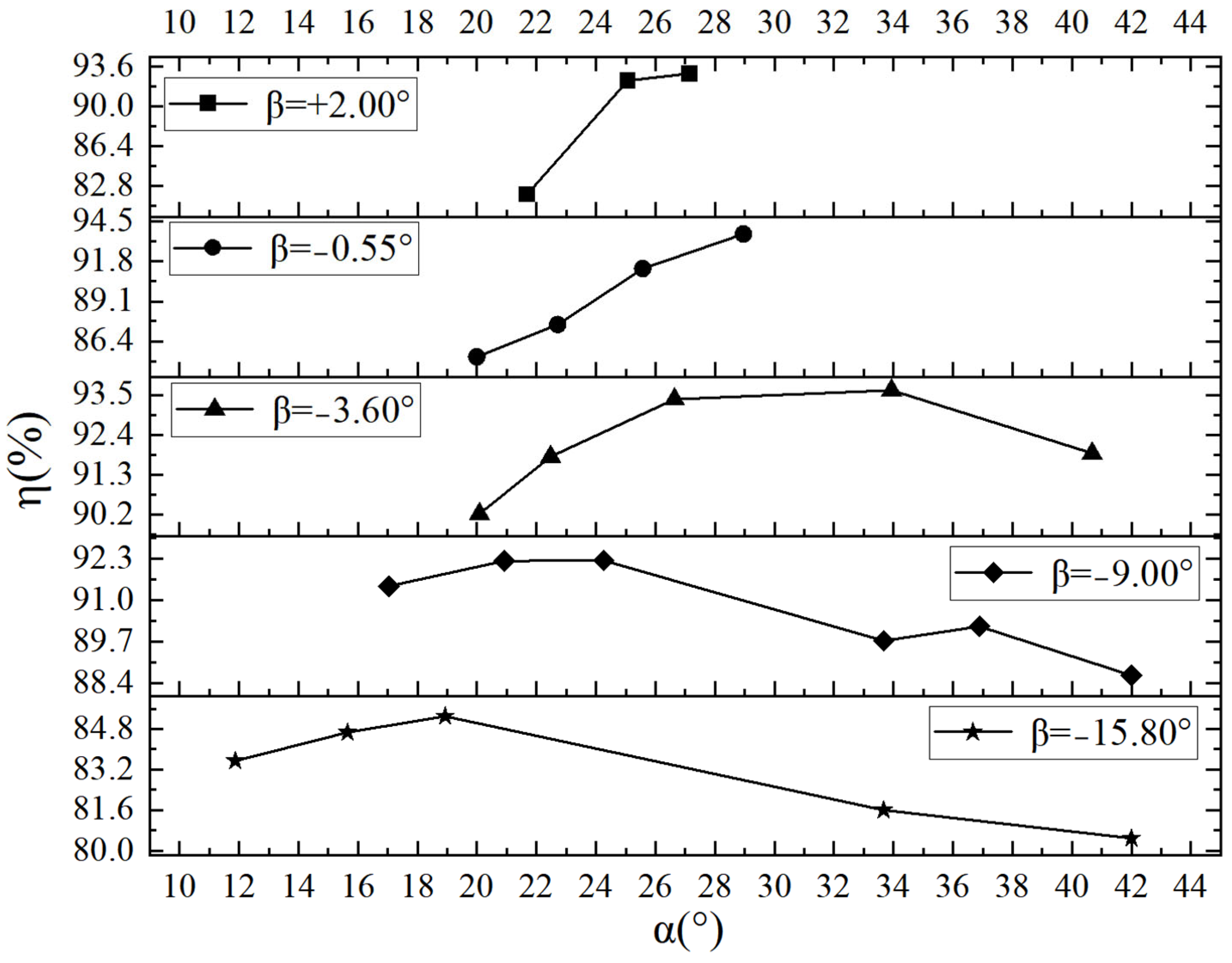
3.3. Efficiency Comparison
3.4. Differential Number of Flow Heterogeneity
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ridha, W.K.M.; Kashyzadeh, K.R.; Ghorbani, S. Common Failures in Hydraulic Kaplan Turbine Blades and Practical Solutions. Materials 2023, 16, 3303. [Google Scholar] [CrossRef] [PubMed]
- Polák, M. A Brief History of the Kaplan Turbine Invention. Energies 2021, 14, 6211. [Google Scholar] [CrossRef]
- Pracheil, B.M.; DeRolph, C.R.; Schramm, M.P.; Bevelhimer, M.S. A fish-eye view of riverine hydropower systems: The current understanding of the biological response to turbine passage. Rev. Fish Biol. Fish. 2016, 26, 153–167. [Google Scholar] [CrossRef]
- Wei, P.; Li, S. Stochastic dynamic analysis of a Kaplan turbine system considering synergistic regulation. Mod. Phys. Lett. B 2021, 35, 2150260. [Google Scholar] [CrossRef]
- Iovănel, R.G.; Dunca, G.; Cervantes, M.J. Study on the Accuracy of RANS Modelling of the Turbulent Flow Developed in a Kaplan Turbine Operated at BEP. Part 2—Pressure Fluctuations. J. Appl. Fluid Mech. 2019, 12, 1463–1473. [Google Scholar] [CrossRef]
- Yen, Y.-H.; ElGammal, T.; Amano, R.S.; Millevolte, J.; Lequesne, B.; Mueller, R.J. Numerical Optimization of Micro Kaplan Hydro Turbine System. In Proceedings of the ASME 2016 Fluids Engineering Division Summer Meeting Collocated with the ASME 2016 Heat Transfer Summer Conference and the ASME 2016 14th International Conference on Nanochannels, Microchannels, and Minichannels, Washington, DC, USA, 10–14 July 2016. [Google Scholar]
- Ma, Y.; Qian, B.; Feng, Z.; Wang, X.; Shi, G.; Liu, Z.; Liu, X. Flow behaviors in a Kaplan turbine runner with different tip clearances. Adv. Mech. Eng. 2021, 13, 16878140211015879. [Google Scholar] [CrossRef]
- Liu, S.; Wu, Y.; Chen, T.; Nishi, M. Development of Numerical Performance Test Stand for a Kaplan Turbine. Int. J. Turbo Jet-Engines 2009, 26, 253–262. [Google Scholar] [CrossRef]
- Jošt, D.; Škerlavaj, A.; Lipej, A. Improvement of Efficiency Prediction for a Kaplan Turbine with Advanced Turbulence Models. Stroj. Vestn.-J. Mech. Eng. 2014, 60, 124–134. [Google Scholar] [CrossRef]
- Wu, Y.; Liu, S.; Dou, H.-S.; Wu, S.; Chen, T. Numerical prediction and similarity study of pressure fluctuation in a prototype Kaplan turbine and the model turbine. Comput. Fluids 2012, 56, 128–142. [Google Scholar] [CrossRef]
- Liu, S.; Shao, J.; Wu, S.; Wu, Y. Numerical simulation of pressure fluctuation in Kaplan turbine. Sci. China Technol. Sci. 2008, 51, 1137–1148. [Google Scholar] [CrossRef]
- Liu, S.; Zhou, D.; Liu, D.; Wu, Y.; Nishi, M. Runaway transient simulation of a model Kaplan turbine. In Proceedings of the 25th IAHR Symposium on Hydraulic Machinery and Systems, Timisoara, Romania, 20–24 September 2010. [Google Scholar] [CrossRef]
- Minakov, A.V.; Platonov, D.V.; Litvinov, I.V.; Shtork, S.I.; Hanjalić, K. Vortex ropes in draft tube of a laboratory Kaplan hydroturbine at low load: An experimental and LES scrutiny of RANS and DES computational models. J. Hydraul. Res. 2017, 55, 668–685. [Google Scholar] [CrossRef]
- Zhang, C.; Tang, H.; Liu, Y.; Chang, J. Study on reverse water hammer of the kaplan turbine. In Proceedings of the 16th Asia and Pacific Division Congress of the International Association of Hydraulic Engineering and Research/3rd IAHR International Symposium on Hydraulic Structures, Nanjing, China, 23 October 2008; pp. 2208–2213. [Google Scholar]
- Mulu, B.; Jonsson, P.; Cervantes, M. Experimental investigation of a Kaplan draft tube—Part I: Best efficiency point. Appl. Energy 2012, 93, 695–706. [Google Scholar] [CrossRef]
- Liu, S.; Mai, J.; Shao, J.; Wu, Y. Pressure pulsation prediction by 3D turbulent unsteady flow simulation through whole flow passage of Kaplan turbine. Eng. Comput. 2009, 26, 1006–1025. [Google Scholar] [CrossRef]
- Dehkharqani, A.S.; Engström, F.; Aidanpää, J.-O.; Cervantes, M.J. Experimental Investigation of a 10 MW Prototype Kaplan Turbine during Start-Up Operation. Energies 2019, 12, 4582. [Google Scholar] [CrossRef]
- Dehkharqani, A.S.; Engström, F.; Aidanpää, J.-O.; Cervantes, M.J. An Indirect Measurement Methodology to Identify Load Fluctuations on Axial Turbine Runner Blades. Sensors 2020, 20, 7220. [Google Scholar] [CrossRef] [PubMed]
- Amiri, K.; Mulu, B.; Raisee, M.; Cervantes, M.J. Unsteady pressure measurements on the runner of a Kaplan turbine during load acceptance and load rejection. J. Hydraul. Res. 2016, 54, 56–73. [Google Scholar] [CrossRef]
- Liu, S.; Li, S.; Wu, Y. Pressure Fluctuation Prediction of a Model Kaplan Turbine by Unsteady Turbulent Flow Simulation. J. Fluids Eng. 2009, 131, 101102. [Google Scholar] [CrossRef]
- Rivetti, A.; Lucino, C.; Liscia, S.; Muguerza, D.; Avellan, F. Pressure pulsation in Kaplan turbines: Prototype-CFD comparison. In Proceedings of the 26th IAHR Symposium on Hydraulic Machinery and Systems, Beijing, China, 19–23 August 2012. [Google Scholar] [CrossRef]
- Mulu, B.G.; Cervantes, M.J.; Devals, C.; Vu, T.C.; Guibault, F. Simulation-based investigation of unsteady flow in near-hub region of a Kaplan Turbine with experimental comparison. Eng. Appl. Comput. Fluid Mech. 2015, 9, 139–156. [Google Scholar] [CrossRef][Green Version]
- Ohiemi, I.E.; Sheng, Y.S.; Singh, P.; Li, Y. Experimental investigation on the effect of axial gap on performance and unsteady pressure pulsations of low head axial flow hydraulic turbine. Flow Meas. Instrum. 2022, 88, 102255. [Google Scholar] [CrossRef]










| Parameters | Values |
|---|---|
| Number of stay vanes Zs | 24 |
| Number of guide vanes Zg | 28 |
| Number of blades Zb | 6 |
| Highest head Hmax | 37.79 m |
| Lowest head Hlow | 12.91 m |
| Runner diameter D | 10.4 m |
| Rated output | 200 MW |
| Rated speed | 68.2 rpm |
| Highest efficiency | 95.67% |
| α (°) | Efficiency of Calculation (%) | Efficiency of Experiment (%) | Error (%) |
|---|---|---|---|
| 20.92 | 92.232 | 92.014 | 0.237 |
| 24.26 | 92.245 | 92.613 | 0.397 |
| 33.67 | 89.731 | 90.089 | 0.396 |
| 36.89 | 90.180 | 89.616 | 0.630 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, D.; Luo, H.; Zhao, W.; Wu, Y.; Zhou, L.; Fan, X.; Wang, Z. Inner Flow Analysis of Kaplan Turbine under Off-Cam Conditions. Energies 2024, 17, 2548. https://doi.org/10.3390/en17112548
Yan D, Luo H, Zhao W, Wu Y, Zhou L, Fan X, Wang Z. Inner Flow Analysis of Kaplan Turbine under Off-Cam Conditions. Energies. 2024; 17(11):2548. https://doi.org/10.3390/en17112548
Chicago/Turabian StyleYan, Dandan, Haiqiang Luo, Weiqiang Zhao, Yibin Wu, Lingjiu Zhou, Xiaofu Fan, and Zhengwei Wang. 2024. "Inner Flow Analysis of Kaplan Turbine under Off-Cam Conditions" Energies 17, no. 11: 2548. https://doi.org/10.3390/en17112548
APA StyleYan, D., Luo, H., Zhao, W., Wu, Y., Zhou, L., Fan, X., & Wang, Z. (2024). Inner Flow Analysis of Kaplan Turbine under Off-Cam Conditions. Energies, 17(11), 2548. https://doi.org/10.3390/en17112548







