Heat Transfer and Fluid Flow Characteristics in a Micro Heat Exchanger Employing Warm Nanofluids for Cooling of Electronic Components
Abstract
1. Introduction
2. Geometry of the Experimental Setup and Measurement Procedure
2.1. Geometry
| Geometric Parameter | Dimension (mm)/Number (-) |
|---|---|
| Heat sink width (W) | 16 |
| Heat sink height (H) | 1.63 |
| Heat sink length (Lmc) | 40 |
| Microchannel width (Wmc) | 0.7 |
| Microchannel height (Hmc) | 1 |
| Half thickness of the solid (es) | 0.35 |
| Thickness of fins (e) | 0.25 |
| Collector tube length (Lc) | 40 |
| Hydraulic diameter (Dh) | 0.8 |
| Collector tube diameter (Dc) | 5 |
| Number of channels (N) | 17 |
2.2. Experimental Apparatus
2.3. Nanofluid Preparation and Calculation of Thermophysical Properties
- Density of nanofluids:
- Specific heat of nanofluids:
- Viscosity of nanofluids:
- Thermal conductivity of nanofluids:where = 3 for the spherical-shaped nanoparticles.
3. Data Reduction in the Experimental Data
3.1. Heat Transfer
3.2. Fluid Flow
3.3. Performance Evaluation Criterion (PEC)
3.4. Uncertainty of the Experimental Data
4. Numerical Approach
4.1. Assumptions and Boundary Conditions
4.2. Governing Equations
- Continuity equation:
- Momentum conservation equation:
- Energy conservation equation:
4.3. Effect of Grid Refinement
5. Results and Discussion
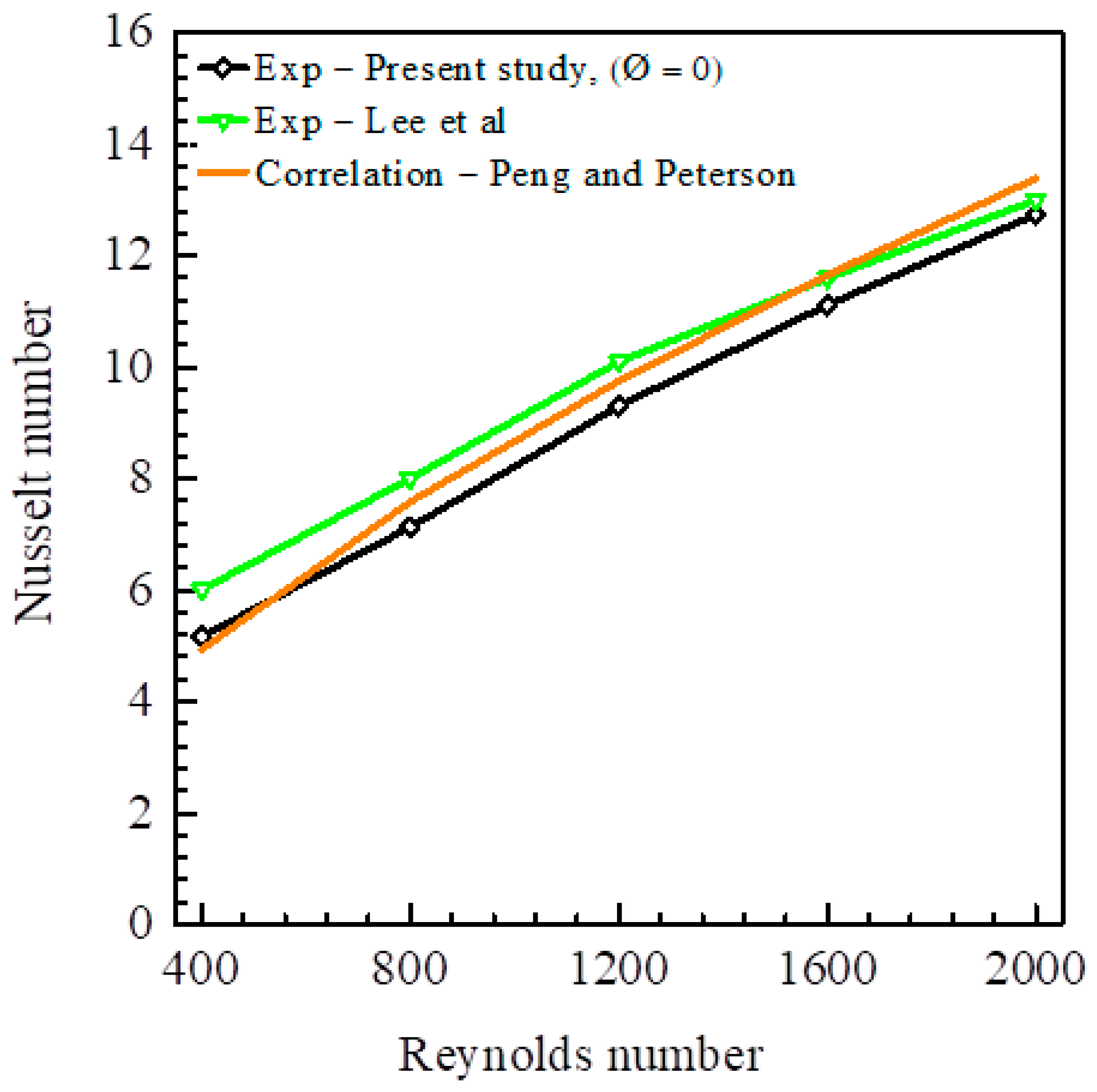
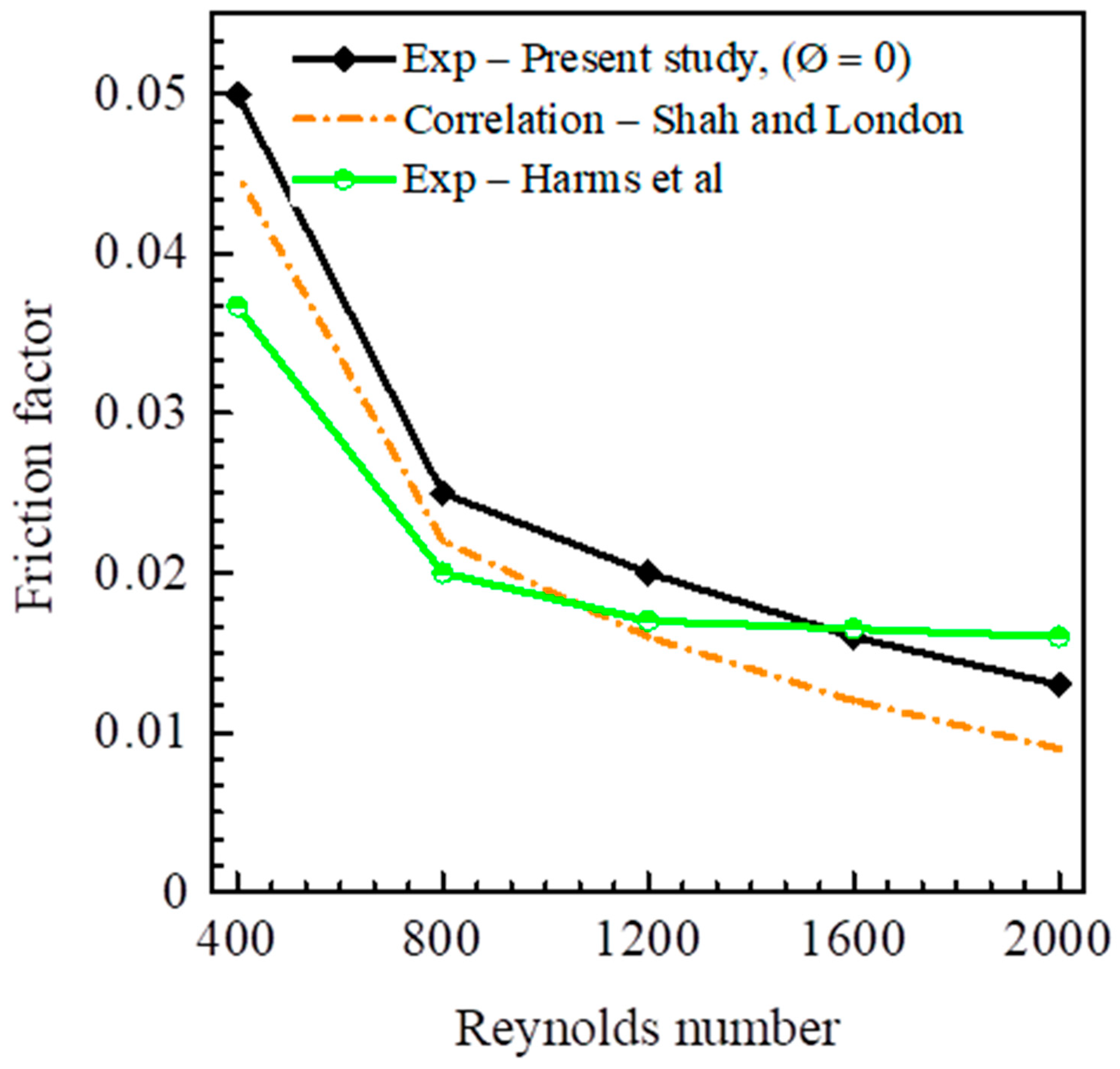
5.1. Validation of Experimental Results
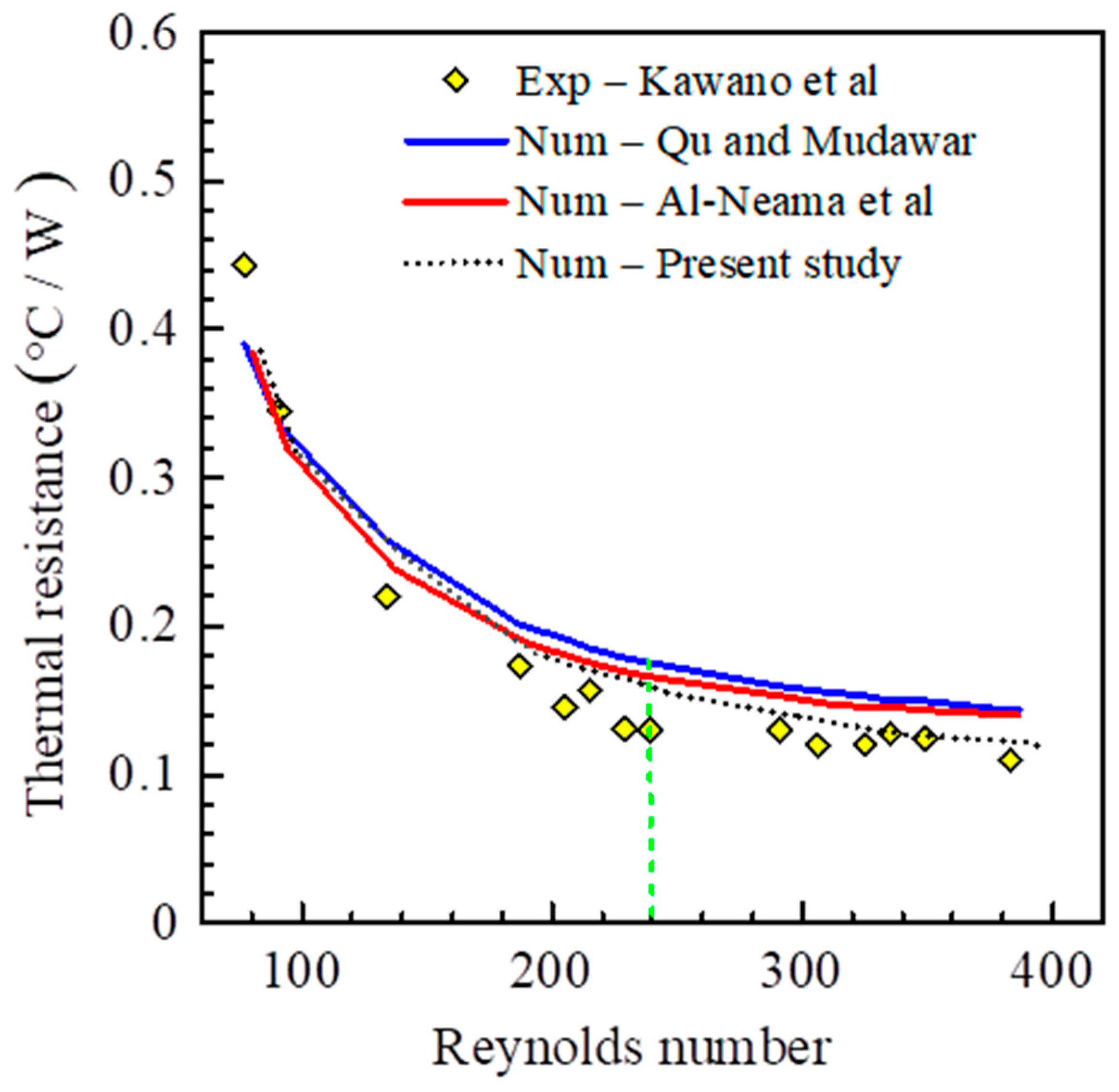
5.2. Validation of the Simulation Code
5.3. Heat Transfer Characteristics
5.4. Pressure Drop Characteristics
5.5. Performance Evaluation Analysis
6. Conclusions
- Both the experimental setup and the numerical simulation tool were validated using pure water, correlations, and experimental data on heat transfer and pressure drops as reported in the literature for laminar flows in rectangular microchannels. The deviations between the Nusselt number and the friction factor from the present work and those from the literature were between 2% and 25%.
- An enhancement in the heat transfer process was obtained with the addition of TiO2 nanoparticles to pure water (i.e., base fluid), on account of the increase in thermal conductivity. At a nanofluid inlet temperature of 55 °C and a nanoparticle concentration of 1%, the Nusselt number increased by 23% to 54% as the Reynolds number varied between 400 and 2000. At a nanoparticle concentration of 5%, the corresponding percentages for Nusselt enhancement were 32% and 63%. The highest value of heat transfer enhancement achieved was 70%, which occurred at a Reynolds number of 2000, a nanoparticle concentration of 5%, and an inlet nanofluid temperature of 35 °C.
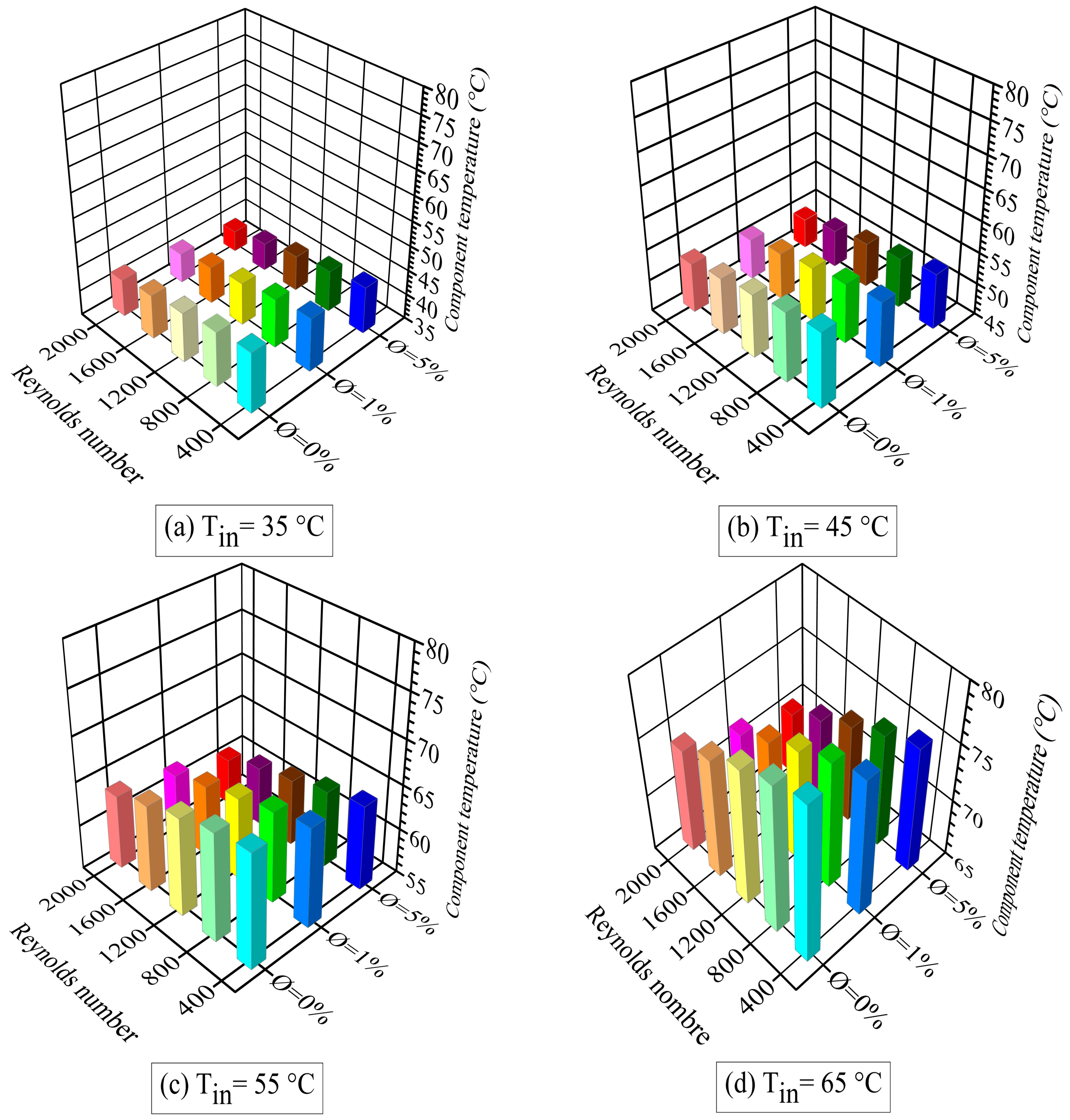
- It was observed that the nanofluid inlet temperature significantly affected the heat transfer. A heat transfer enhancement of about 10% was obtained when the nanofluid inlet temperature decreased from 65 °C to 45 °C.
- The increase in both the Reynolds number and the nanoparticle concentration lowered the temperature of the heating components. This widened the safety margin for the critical temperature limit of 80 °C. However, at an inlet temperature of 65 °C, the operating temperature of the electronic equipment was above the safety temperature limit set at 70 °C, even with the addition of nanoparticles and applying high Reynolds numbers.
- The maximum value of the pressure drop was obtained with nanofluids at a 5% nanoparticle concentration and a Reynolds number of 2000. A pressure drop increase of about 20% was observed when using (TiO2/water) nanofluids instead of base fluid (i.e., pure water).
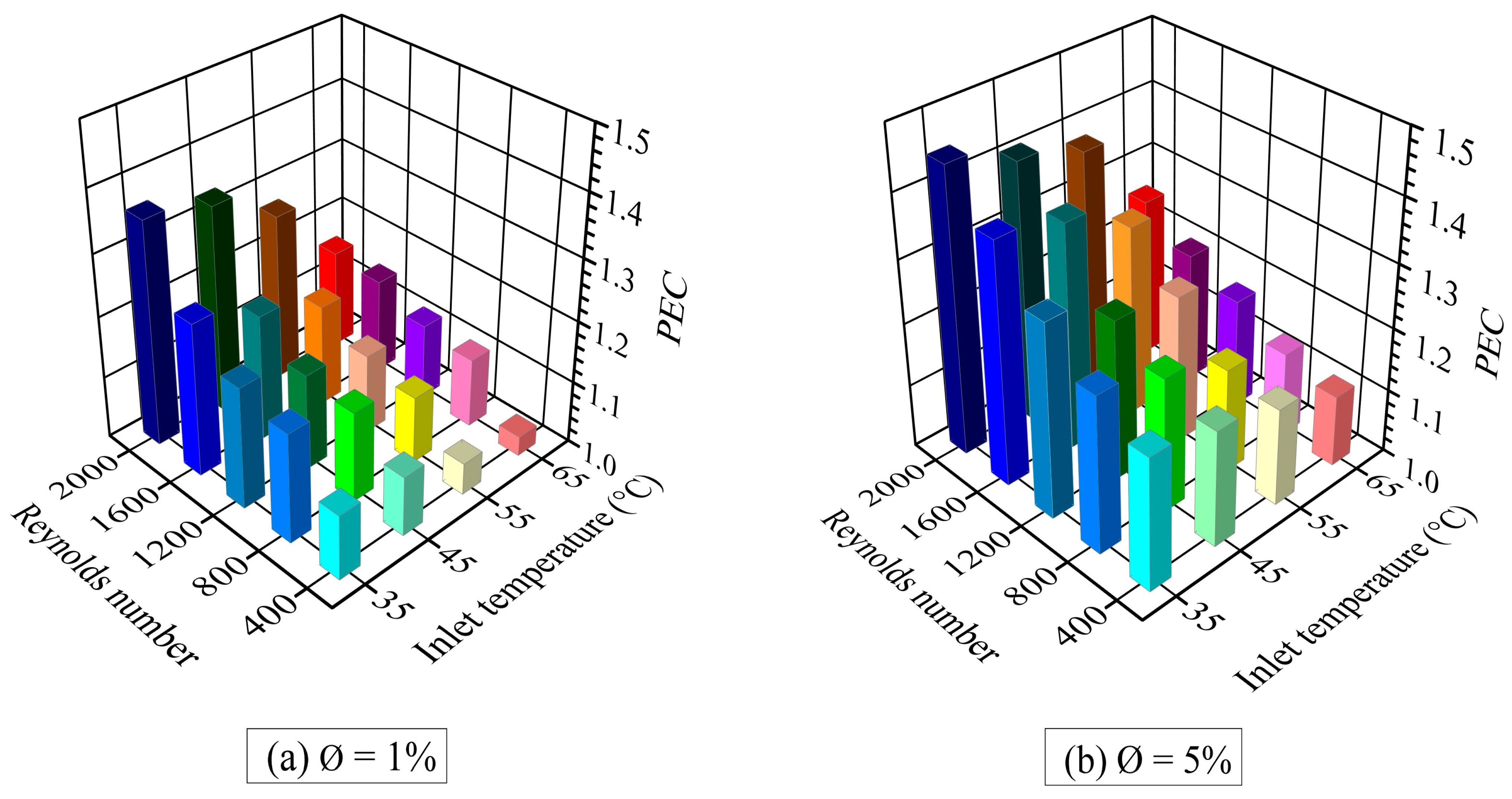
- The PEC values were always greater than the unity for both nanoparticle concentrations. This indicates that adding nanoparticles to cooling water circulating in a micro heat exchanger improves the heat transfer process. At a Reynolds number of 2000 and a nanofluid inlet temperature of 35 °C, PEC values of 1.36 and 1.45 were obtained for nanoparticle concentrations of 1% and 5%, respectively. When the nanofluid inlet temperature increased to 65 °C, the PEC parameter decreased to 1.02–1.10 for both concentrations.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Aeff | Effective heat transfer area (m2) |
| Ac | Collector area (m2) |
| Amc | Cross-sectional area of each flow channel (m2) |
| Atube | Tube area (m2) |
| Cp | Specific heat (J/kg.K) |
| dp | Particle diameter (nm) |
| Dc | Collector diameter (mm) |
| Dh | Hydraulic diameter (mm) |
| e | Thickness of pin fin (mm) |
| es | Thickness of the upper face of the heat sink (mm) |
| fapp | Apparent friction factor |
| h | Convective heat transfer coefficient (W/m2.K) |
| H | Heat sink depth (mm) |
| Hmc | Microchannel depth (mm) |
| k | Thermal conductivity (W/m.K) |
| Kc | Contraction loss coefficient |
| Ke | Expansion loss coefficient |
| K90 | Bend loss coefficient (=1.2) |
| I | Electrical intensity (A) |
| Lc | Collector tube length (mm) |
| L | Heat sink length (mm) |
| m | Mass (kg) |
| N | Number of channels |
| Nu | Nusselt number |
| P | Pressure (Pa) |
| Electric power (W) | |
| Heat transfer rate (W) | |
| Re | Reynolds Number |
| Pr | Prandtl number |
| t | Time (s) |
| T | Temperature (°C) |
| u, v, and w | Velocity in the directions x, y, and z (m/s) |
| Voltage (V) | |
| Volume flow (m3/s) | |
| W | Heat sink width (mm) |
| Power pumping (W) | |
| Symbols | |
| ρ | Density (kg/m3) |
| µ | Dynamic viscosity (kg/m.s) |
| γ | Convergence criterion |
| ϕ | Particle mass fraction (%) |
| λ | Coverage factor |
| α | Channel aspect ratio |
| LMTD | Log-mean temperature difference (°C) |
| PEC | Performance evaluation criterion |
| Subscripts | |
| avg | Average |
| bf | Base fluid |
| c | Collector |
| in | Inlet |
| f | Fluid |
| mc | Microchannel |
| min | Minimum |
| max | Maximum |
| nf | Nanofluid |
| np | Nanoparticle |
| out | Outlet |
| s | Surface |
References
- Zimmermann, S.; Tiwari, M.K.; Meijer, I.; Paredes, S.; Michel, B.; Poulikakos, D. Hot water-cooled electronics: Exergy analysis and waste heat reuse feasibility. Int. J. Heat Mass Transf. 2012, 55, 6391–6399. [Google Scholar] [CrossRef]
- Zhang, H.Y.; Pinjala, D.; Wong, T.N.; Toh, K.C.; Joshi, Y.K. Single-phase liquid cooled microchannel heat sink for electronic packages. Appl. Therm. Eng. 2005, 25, 1472–1487. [Google Scholar] [CrossRef]
- Naphon, P.; Wiriyasart, S. Liquid cooling in the mini-rectangular fin heat sink with and without thermoelectric for CPU. Int. Commun. Heat Mass Transf. 2009, 36, 166–171. [Google Scholar] [CrossRef]
- Tuckerman, D.B.; Pease, R.F.W. High-Performance Heat Sinking for VLSI. IEEE Electron Device Lett. 1981, EDL-2, 126–129. Available online: https://ieeexplore.ieee.org/document/1481851 (accessed on 10 November 2017). [CrossRef]
- Gao, P.; Le Person, S.; Favre-Marinet, M. Scale effects on hydrodynamics and heat transfer in two-dimensional mini and microchannels. Int. J. Therm. Sci. 2002, 41, 1017–1027. [Google Scholar] [CrossRef]
- Mala, G.M.; Li, D. Flow characteristics of water in microtubes. Int. J. Heat Fluid Flow 1999, 20, 142–148. [Google Scholar] [CrossRef]
- Fedorov, A.G.; Viskanta, R. Three-dimensional conjugate heat transfer in the microchannel heat sink for electronic packaging. Int. J. Heat Mass Transf. 2000, 43, 399–415. [Google Scholar] [CrossRef]
- Wu, H.Y.; Cheng, P. Friction factors in smooth trapezoidal silicon microchannels with different aspect ratios. Int. J. Heat Mass Transf. 2003, 46, 2519–2525. [Google Scholar] [CrossRef]
- Colgan, E.G.; Furman, B.; Gaynes, A.; Graham, W.; LaBianca, N.; Magerlein, J.H.; Polastre, R.J.; Rothwell, M.B.; Bezama, R.J. A practical implementation of silicon microchannel coolers for high power chips. IEEE Trans. Compon. Packag. Technol. 2007, 30, 218–225. Available online: https://ieeexplore.ieee.org/document/4214931 (accessed on 29 February 2020). [CrossRef]
- Hong, F.; Cheng, P. Three dimensional numerical analyses and optimization of offset strip-fin microchannel heat sinks. Int. Commun. Heat Mass Transf. 2009, 36, 651–656. [Google Scholar] [CrossRef]
- Foong, A.J.L.; Ramesh, N.; Chandratilleke, T.T. Laminar convective heat transfer in a microchannel with internal longitudinal fins. Int. J. Therm. Sci. 2009, 48, 1908–1913. [Google Scholar] [CrossRef]
- Xie, Y.; Shen, Z.; Zhang, D.; Lan, J. Thermal performance of a water-cooled microchannel heat sink with grooves and obstacles. J. Electron. Packag. Trans. ASME 2014, 136, 021001. [Google Scholar] [CrossRef]
- Gholami, H.; Banaei, M.; Eskandari, A. Investigation of effect of triangular rib in heat transfer of finned rectangular microchannel with extended surfaces. Life Sci. J. 2013, 10, 206–211. Available online: http://www.Lifesciencesite.com (accessed on 18 January 2018).
- Muzychka, Y.S. Constructal design of forced convection cooled microchannel heat sinks and heat exchangers. Int. J. Heat Mass Transf. 2005, 48, 3119–3127. [Google Scholar] [CrossRef]
- Bello-Ochende, T.; Liebenberg, L.; Meyer, J.P. Constructal cooling channels for micro-channel heat sinks. Int. J. Heat Mass Transf. 2007, 50, 4141–4150. [Google Scholar] [CrossRef]
- Salimpour, M.R.; Sharifhasan, M.; Shirani, E. Constructal optimization of the geometry of an array of micro-channels. Int. Commun. Heat Mass Transf. 2011, 38, 93–99. [Google Scholar] [CrossRef]
- Bello-Ochende, T.; Meyer, J.P.; Ighalo, F.U. Combined numerical optimization and constructal theory for the design of microchannel heat sinks. Numer. Heat Transf. Part A Appl. 2010, 58, 882–899. [Google Scholar] [CrossRef]
- Olakoyejo, O.T.; Bello-Ochende, T.; Meyer, J.P. Mathematical optimisation of laminar forced convection heat transfer through a vascularized solid with square channels. Int. J. Heat Mass Transf. 2012, 55, 2402–2411. [Google Scholar] [CrossRef]
- Olakoyejo, O.T.; Bello-Ochende, T.; Meyer, J.P. Constructal conjugate cooling channels with internal heat generation. Int. J. Heat Mass Transf. 2012, 55, 4385–4396. [Google Scholar] [CrossRef]
- Bejan, A. Constructal-theory network of conducting paths for cooling a heat generating volume. Int. J. Heat Mass Transf. 1997, 40, 799–811. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, A.; Kothiyal, A.D.; Bisht, M.S. A review of flow and heat transfer behaviour of nanofluids in micro channel heat sinks. Therm. Sci. Eng. Prog. 2018, 8, 477–493. [Google Scholar] [CrossRef]
- Zimmermann, S.; Meijer, I.; Tiwari, M.K.; Paredes, S.; Michel, B.; Poulikakos, D. Aquasar: A hot water-cooled data center with direct energy reuse. Energy 2012, 43, 237–245. [Google Scholar] [CrossRef]
- Sarafraz, M.M.; Hormozi, F. Experimental study on the thermal performance and efficiency of a copper made thermosyphon heat pipe charged with alumina-glycol based nanofluids. Powder Technol. 2014, 266, 378–387. [Google Scholar] [CrossRef]
- Nojoomizadeh, M.; Karimipour, A. The effects of porosity and permeability on fluid flow and heat transfer of multi walled carbon nano-tubes suspended in oil (MWCNT/Oil nano-fluid) in a microchannel filled with a porous medium. Phys. E Low-Dimens. Syst. Nanostruct. 2016, 84, 423–433. [Google Scholar] [CrossRef]
- Saravanan, K.; Ravishankar, P.; Sivakumar, V.; Velmurugan, K. Review of heat transfer and fluid flow of nanofluids. Renew. Sustain. Energy Rev. 2017, 78, 460–482. [Google Scholar]
- Gupta, S.K.; Gupta, S.; Gupta, T.; Raghav, A.; Singh, A. A review on recent advances and applications of nanofluids in plate heat exchanger. Mater. Today Proc. 2021, 44, 229–241. [Google Scholar] [CrossRef]
- Godson, L.; Raja, B.; Lal, D.M.; Wongwises, S. Enhancement of heat transfer using nanofluids-An overview. Renew. Sustain. Energy Rev. 2010, 14, 629–641. [Google Scholar] [CrossRef]
- Murshed, S.M.S.; Nieto de Castro, C.A.; Lourenço, M.J.V.; Lopes, M.L.M.; Santos, F.J.V. A review of boiling and convective heat transfer with nanofluids. Renew. Sustain. Energy Rev. 2011, 15, 2342–2354. [Google Scholar] [CrossRef]
- Sundar, L.S. Synthesis and characterization of hybrid nanofluids and their usage in different heat exchangers for an improved heat transfer rate: A critical review. Eng. Sci. Technol. Int. J. 2023, 44, 101468. [Google Scholar] [CrossRef]
- Kim, T.I.; Jeong, Y.H.; Chang, S.H. An experimental study on CHF enhancement in flow boiling using Al2O3 nano-fluid. Int. J. Heat Mass Transf. 2010, 53, 1015–1022. [Google Scholar] [CrossRef]
- Wu, J.M.; Zhao, J. A review of nanofluid heat transfer and critical heat flux enhancement—Research gap to engineering application. Prog. Nucl. Energy 2013, 66, 13–24. [Google Scholar] [CrossRef]
- Porgar, S.; Oztop, H.F.; Salehfekr, S. A comprehensive review on thermal conductivity and viscosity of nanofluids and their application in heat exchangers. J. Mol. Liq. 2023, 386, 122213. [Google Scholar] [CrossRef]
- Ahmed, W.; Kazi, S.N.; Chowdhury, Z.Z.; Johan, M.R.B.; Akram, N.; Mujtaba, M.A.; Gul, M.; Oon, C.S. Experimental investigation of convective heat transfer growth on ZnO@TiO2/DW binary composites/hybrid nanofluids in a circular heat exchanger. J. Therm. Anal. Calorim. 2020, 143, 879–898. [Google Scholar] [CrossRef]
- Fang, X.; Chen, Y.; Zhang, H.; Chen, W.; Dong, A.; Wang, R. Heat transfer and critical heat flux of nanofluid boiling: A comprehensive review. Renew. Sustain. Energy Rev. 2016, 62, 924–940. [Google Scholar] [CrossRef]
- Salman, B.H.; Mohammed, H.A.; Munisamy, K.M.; Kherbeet, A.S. Characteristics of heat transfer and fluid flow in microtube and microchannel using conventional fluids and nanofluids: A review. Renew. Sustain. Energy Rev. 2013, 28, 848–880. [Google Scholar] [CrossRef]
- Daungthongsuk, W.; Wongwises, S.A. Critical review of convective heat transfers of nanofluids. Renew. Sustain. Energy Rev. 2007, 11, 797–817. [Google Scholar] [CrossRef]
- Ahmed, W.; Chowdhury, Z.Z.; Kazi, S.N.; Johan, M.R.; Akram, N.; Oon, C.S. Effect of ZnO-water based nanofluids from sonochemical synthesis method on heat transfer in a circular flow passage. Int. Commun. Heat Mass Tranfer 2020, 114, 104591. [Google Scholar] [CrossRef]
- Hussein, A.M.; Sharma, K.V.; Bakar, R.A.; Kadirgama, K. A review of forced convection heat transfer enhancement and hydrodynamic characteristics of a nanofluid. Renew. Sustain. Energy Rev. 2014, 29, 734–743. [Google Scholar] [CrossRef]
- Hussien, A.A.; Abdullah, M.Z.; Al-Nimr, M.A. Single-phase heat transfer enhancement in micro/minichannels using nanofluids: Theory and applications. Appl. Energy 2016, 164, 733–755. [Google Scholar] [CrossRef]
- Kakaç, S.; Pramuanjaroenkij, A. Review of convective heat transfer enhancement with nanofluids. Int. J. Heat Mass Transf. 2009, 52, 3187–3196. [Google Scholar] [CrossRef]
- Yu, W.; France, D.M.; Timofeeva, E.V.; Singh, D.; Routbort, J.L. Comparative review of turbulent heat transfer of nanofluids. Int. J. Heat Mass Transf. 2012, 55, 5380–5396. [Google Scholar] [CrossRef]
- Sundar, L.S.; Singh, M.K. Convective heat transfer and friction factor correlations of nanofluid in a tube and with inserts: A review. Renew. Sustain. Energy Rev. 2013, 20, 23–35. [Google Scholar] [CrossRef]
- Mohammed, H.A.; Bhaskaran, G.; Shuaib, N.H.; Saidur, R. Heat transfer and fluid flow characteristics in microchannels heat exchanger using nanofluids: A review. Renew. Sustain. Energy Rev. 2011, 15, 1502–1512. [Google Scholar] [CrossRef]
- Vanaki, S.M.; Ganesan, P.; Mohammed, H.A. Numerical study of convective heat transfer of nanofluids: A review. Renew. Sustain. Energy Rev. 2016, 54, 1212–1239. [Google Scholar] [CrossRef]
- Sabaghan, A.; Edalatpour, M.; Moghadam, M.C.; Roohi, E.; Niazmand, H. Nanofluid flow and heat transfer in a microchannel with longitudinal vortex generators: Two-phase numerical simulation. Appl. Therm. Eng. 2016, 100, 179–189. [Google Scholar] [CrossRef]
- Lee, J.; Mudawar, I. Assessment of the effectiveness of nanofluids for single-phase and two-phase heat transfer in micro-channels. Int. J. Heat Mass Transf. 2007, 50, 452–463. [Google Scholar] [CrossRef]
- Kalteh, M.; Abbassi, A.; Saffar-Avval, M.; Frijns, A.; Darhuber, A.; Harting, J. Eulerian–Eulerian two-phase numerical simulation of nanofluid laminar forced convection in a microchannel. Int. J. Heat Fluid Flow 2011, 32, 107–116. [Google Scholar] [CrossRef]
- Mohammed, H.A.; Gunnasegaran, P.; Shuaib, N.H. Heat transfer in rectangular microchannels heat sink using nanofluids. Int. Commun. Heat Mass Transf. 2010, 37, 1496–1503. [Google Scholar] [CrossRef]
- Manay, E.; Sahin, B. The effect of microchannel height on performance of nanofluids. Int. J. Heat Mass Transf. 2016, 95, 307–320. [Google Scholar] [CrossRef]
- Martínez, V.A.; Vasco, D.A.; García-Herrera, C.M.; Ortega-Aguilera, R. Numerical study of TiO2-based nanofluids flow in microchannel heat sinks: Effect of the Reynolds number and the microchannel height. Appl. Therm. Eng. 2019, 161, 114130. [Google Scholar] [CrossRef]
- Nitiapiruk, P.; Mahian, O.; Dalkilic, A.S.; Wongwises, S. Performance characteristics of a microchannel heat sink using TiO2/water nanofluid and different thermophysical models. Int. Commun. Heat Mass Transf. 2013, 47, 98–104. [Google Scholar] [CrossRef]
- Heydari, M.; Toghraie, D.; Akbari, O.A. The effect of semi-attached and offset mid-truncated ribs and Water/TiO2 nanofluid on flow and heat transfer properties in a triangular microchannel. Therm. Sci. Eng. Prog. 2017, 2, 140–150. [Google Scholar] [CrossRef]
- Bouanimba, N.; Laid, N.; Zouaghi, R.; Sehili, T. A Comparative Study of the Activity of TiO2 Degussa P25 and Millennium PCs in the Photocatalytic Degradation of Bromothymol Blue. Int. J. Chem. React. Eng. 2017, 16, 20170014. [Google Scholar] [CrossRef]
- Laid, N.; Bouanimba, N.; Ben Ahmede, A.; Toureche, A.; Sehili, T. Characterization of ZnO and TiO2 Nanopowders and their Application for Photocatalytic Water Treatment. Acta Phys. Pol. A 2020, 137, 305–312. [Google Scholar] [CrossRef]
- Ebrahimnia-Bajestan, E.; Charjouei Moghadam, M.; Niazmand, H.; Daungthongsuk, W.; Wongwises, S. Experimental and numerical investigation of nanofluids heat transfer characteristics for application in solar heat exchangers. Int. J. Heat Mass Transf. 2016, 92, 1041–1052. [Google Scholar] [CrossRef]
- Arshad, W.; Ali, H.M. Experimental investigation of heat transfer and pressure drop in a straight minichannel heat sink using TiO2 nanofluid. Int. J. Heat Mass Transf. 2017, 110, 248–256. [Google Scholar] [CrossRef]
- Pak, B.C.; Cho, Y.I. Hydrodynamic and heat transfer study of dispersed fluids with submicron metallic oxide particles. Exp. Heat Transf. 1998, 11, 151–170. [Google Scholar] [CrossRef]
- Xuan, Y.; Roetzel, W. Conceptions for heat transfer correlation of nanofluids. Int. J. Heat Mass Transf. 2000, 43, 3701–3707. [Google Scholar] [CrossRef]
- Rea, U.; McKrell, T.; Hu, L.W.; Buongiorno, J. Laminar convective heat transfers and viscous pressure loss of alumina-water and zirconia-water nanofluids. Int. J. Heat Mass Transf. 2009, 52, 2042–2048. [Google Scholar] [CrossRef]
- Gonçalves, I.; Souza, R.; Coutinho, G.; Miranda, J.; Moita, A.; Pereira, J.E.; Moreira, A.; Lima, R. Thermal Conductivity of Nanofluids: A Review on Prediction Models, Controversies and Challenges. Appl. Sci. 2021, 11, 2525. [Google Scholar] [CrossRef]
- Kandlikar, S.G. Single-phase liquid flow in minichannels and microchannels. In Heat and Fluid Flow in Minichannels and Microchannels; Elsevier: Amsterdam, The Netherlands, 2006; pp. 87–136. [Google Scholar] [CrossRef]
- Idelchik, I.E. Handbook of Hydraulic Resistance; Hemisphere Publishing: New York, NY, USA, 1986. [Google Scholar]
- Zhang, J.; Diao, Y.; Zhao, Y.; Zhang, Y. An experimental investigation of heat transfer enhancement in minichannel: Combination of nanofluid and micro fin structure techniques. Exp. Therm. Fluid Sci. 2017, 81, 21–32. [Google Scholar] [CrossRef]
- Moffat, R.J. Describing the uncertainties in experimental results. Exp. Therm. Fluid Sci. 1988, 1, 3–17. [Google Scholar] [CrossRef]
- Mokrane, M.; Lounis, M.; Announ, M.; Ouali, M.; Djebiret, M.A.; Bourouis, M. Performance analysis of a micro heat exchanger in electronic cooling applications. J. Therm. Eng. 2021, 7, 773–790. Available online: https://jten.yildiz.edu.tr/article/34 (accessed on 2 February 2022). [CrossRef]
- Patankar, S.V. Numerical Heat Transfer and Fluid Flow; CRC Press: Boca Raton, FL, USA, 1980. [Google Scholar] [CrossRef]
- Lee, P.S.; Garimella, S.V.; Liu, D. Investigation of heat transfer in rectangular microchannels. Int. J. Heat Mass Transf. 2005, 48, 1688–1704. [Google Scholar] [CrossRef]
- Peng, X.F.; Peterson, G.P. Convective heat transfer and flow friction for water flow in microchannel structures. Int. J. Heat Mass Transf. 1996, 39, 2599–2608. [Google Scholar] [CrossRef]
- Shah, R.K.; London, A.L. Laminar Flow Forced Convection in Ducts: A Source Book for Compact Heat Exchanger Analytical Data; Academic Press: New York, NY, USA, 1978. [Google Scholar] [CrossRef]
- Harms, T.M.; Kazmierczak, M.J.; Gerner, F.M. Developing convective heat transfer in deep rectangular microchannels. Int. J. Heat Fluid Flow 1999, 20, 149–157. [Google Scholar] [CrossRef]
- Kawano, K.; Sekimura, M.; Minakami, K.; Iwasaki, H.; Ishizuka, M. Development of micro channel heat exchanging. JSME Int. J. Ser. B Fluids Therm. Eng. 2001, 44, 592–598. [Google Scholar] [CrossRef]
- Qu, W.; Mudawar, I. Experimental and numerical study of pressure drop and heat transfer in a single-phase micro-channel heat sink. Int. J. Heat Mass Transf. 2002, 45, 2549–2565. [Google Scholar] [CrossRef]
- Al-Neama, A.F.; Kapur, N.; Summers, J.; Thompson, H.M. An experimental and numerical investigation of the use of liquid flow in serpentine microchannels for microelectronics cooling. Appl. Therm. Eng. 2017, 116, 709–723. [Google Scholar] [CrossRef]
- Kumar, V.; Sarkar, J. Experimental hydrothermal behavior of hybrid nanofluid for various particle ratios and comparison with other fluids in minichannel heat sink. Int. Commun. Heat Mass Transf. 2020, 110, 104397. [Google Scholar] [CrossRef]
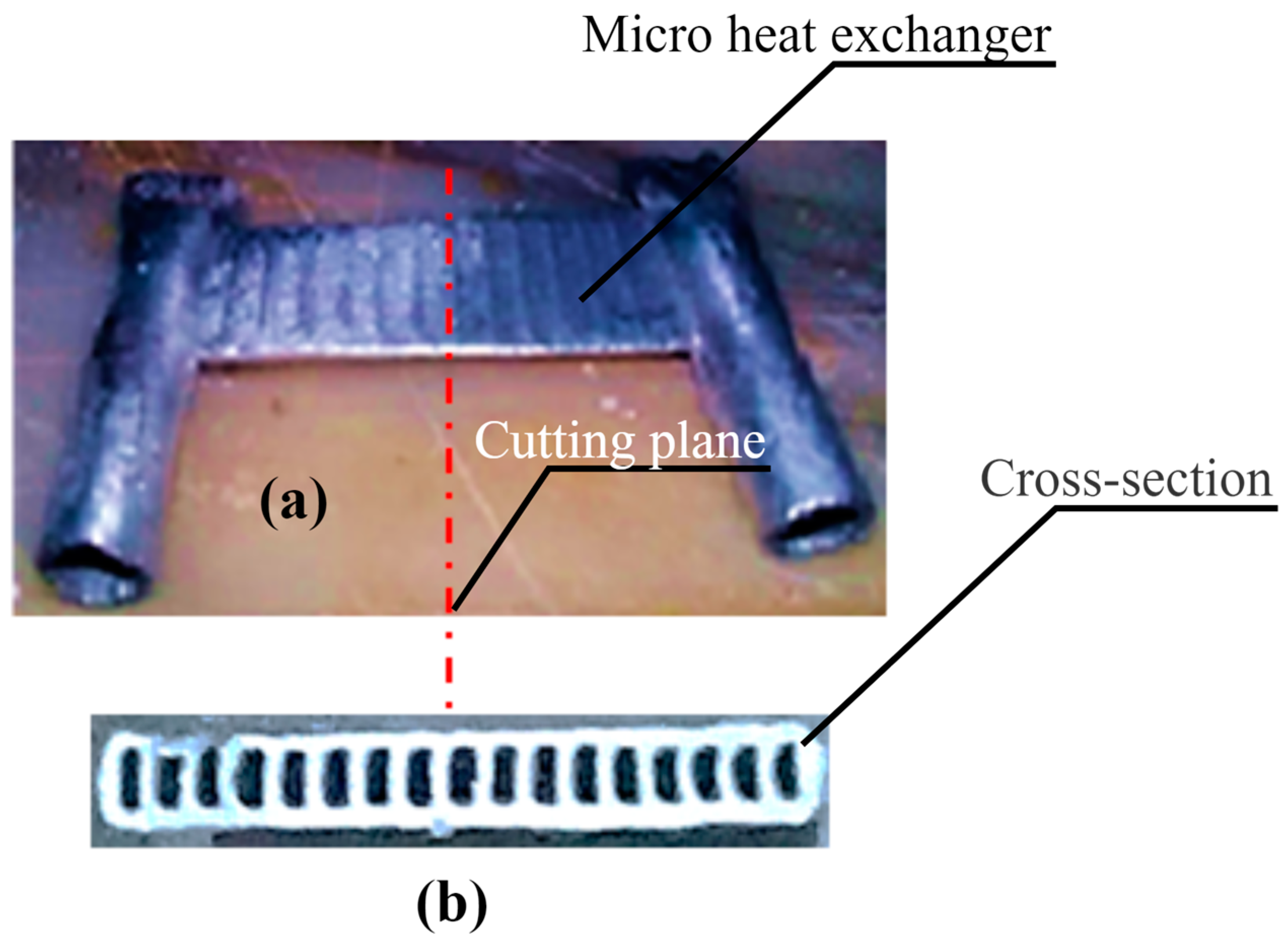
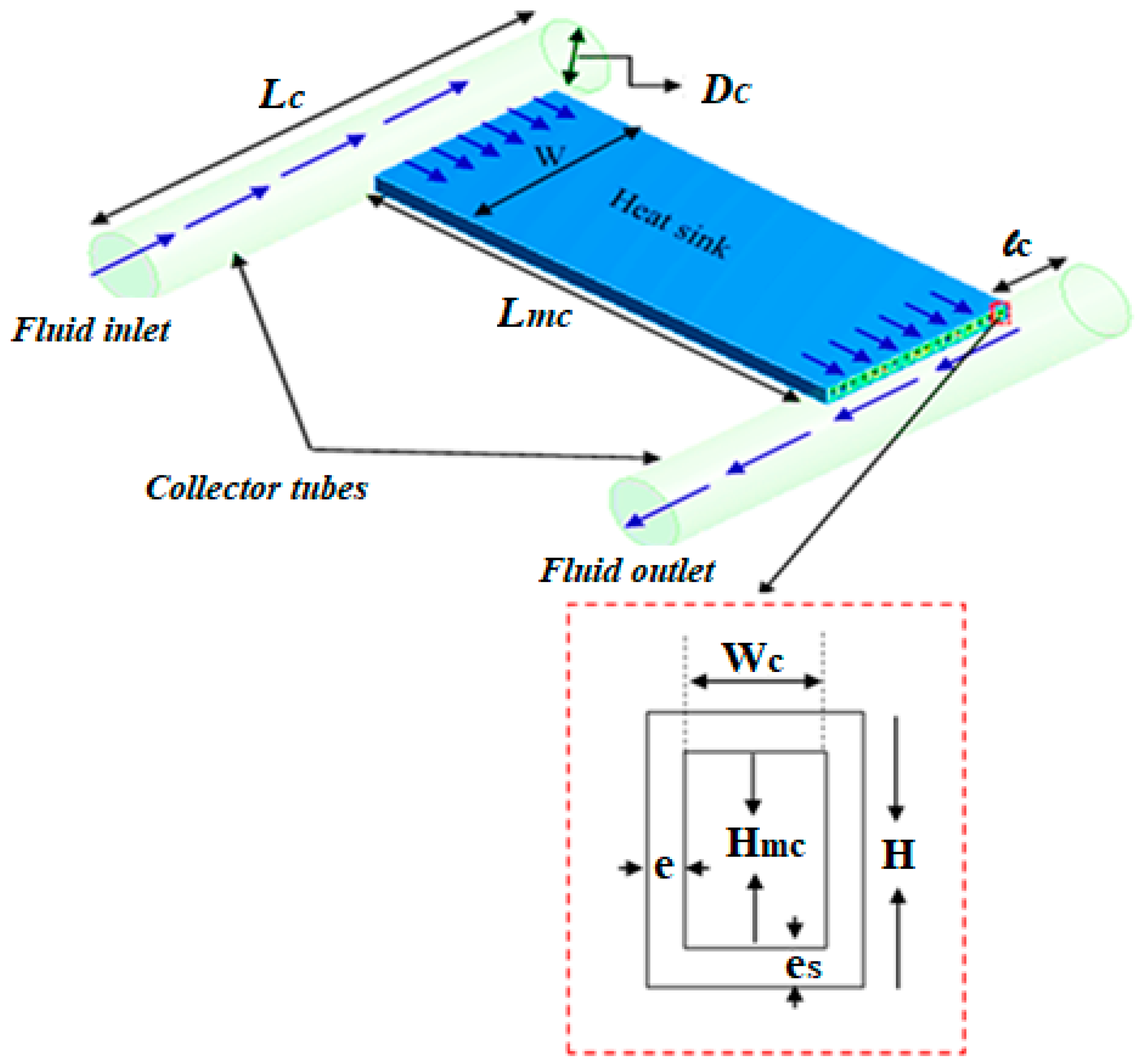


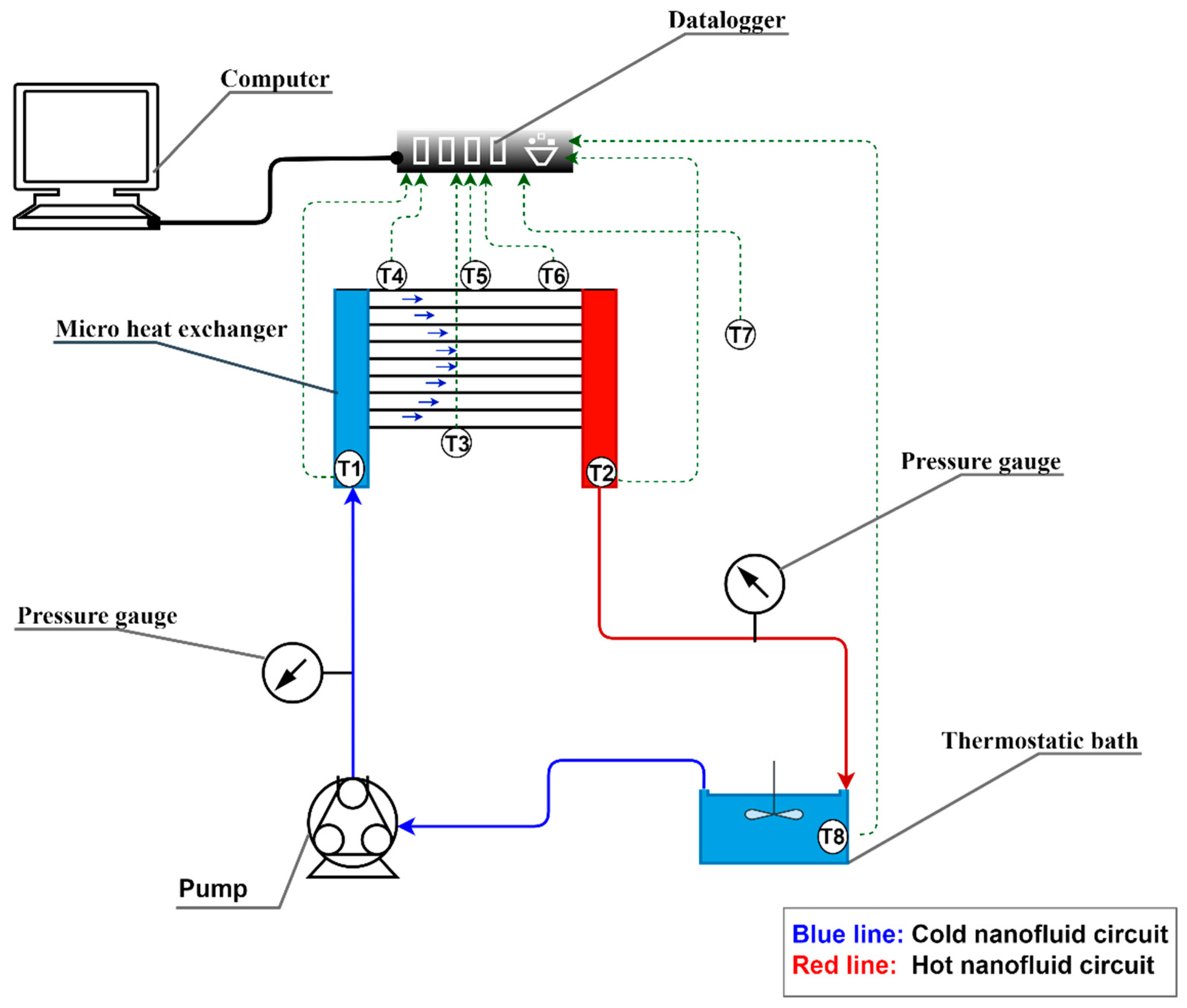







| Properties | TiO2 Nanoparticles |
|---|---|
| Mean diameter, dp | 20 nm |
| Thermal conductivity, k | 8.4 W/m.K |
| Specific heat, Cp | 710 J/kg.K |
| Density, ρ | 4157 kg/m3 |
| Sensor | Uncertainty |
| K-type thermocouple | ±0.1 °C |
| Pressure sensors | ±2.5% FS |
| Peristaltic pump | ±1% |
| Heater power supply voltage and current | 0.01% and 0.1% |
| L (mm) | 2.5 |
| W (mm) | 1.25 |
| Parameter | Uncertainty (%) |
| Re | 1.54 |
| ∆P (Pa) | 0.5 |
| h (W/m2.°C) | 2 |
| Nu | 3 |
| Φ (%) | 1 | 5 | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Tin (°C) | 35 | 45 | 55 | 65 | 35 | 45 | 55 | 65 | |
| Re | |||||||||
| 400 | 26% | 24% | 23% | 21% | 37% | 35% | 32% | 30% | |
| 800 | 35% | 32% | 30% | 28% | 52% | 49% | 47% | 45% | |
| 1200 | 44% | 42% | 40% | 39% | 60% | 57% | 55% | 53% | |
| 2000 | 57% | 56% | 54% | 49% | 70% | 65% | 63% | 59% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mokrane, M.; Bourouis, M. Heat Transfer and Fluid Flow Characteristics in a Micro Heat Exchanger Employing Warm Nanofluids for Cooling of Electronic Components. Energies 2024, 17, 2383. https://doi.org/10.3390/en17102383
Mokrane M, Bourouis M. Heat Transfer and Fluid Flow Characteristics in a Micro Heat Exchanger Employing Warm Nanofluids for Cooling of Electronic Components. Energies. 2024; 17(10):2383. https://doi.org/10.3390/en17102383
Chicago/Turabian StyleMokrane, Mahdi, and Mahmoud Bourouis. 2024. "Heat Transfer and Fluid Flow Characteristics in a Micro Heat Exchanger Employing Warm Nanofluids for Cooling of Electronic Components" Energies 17, no. 10: 2383. https://doi.org/10.3390/en17102383
APA StyleMokrane, M., & Bourouis, M. (2024). Heat Transfer and Fluid Flow Characteristics in a Micro Heat Exchanger Employing Warm Nanofluids for Cooling of Electronic Components. Energies, 17(10), 2383. https://doi.org/10.3390/en17102383







