Computational Particle Fluid Dynamics Simulation on Combustion Characteristics of Blended Fuels of Coal, Biomass, and Oil Sludge in a 130 t h−1 Circulating Fluidized Bed Boiler
Abstract
:1. Introduction
2. CPFD Methods
2.1. Mathematical Models
2.1.1. Governing Equations
2.1.2. Chemical Reaction Models
2.1.3. NOx Models
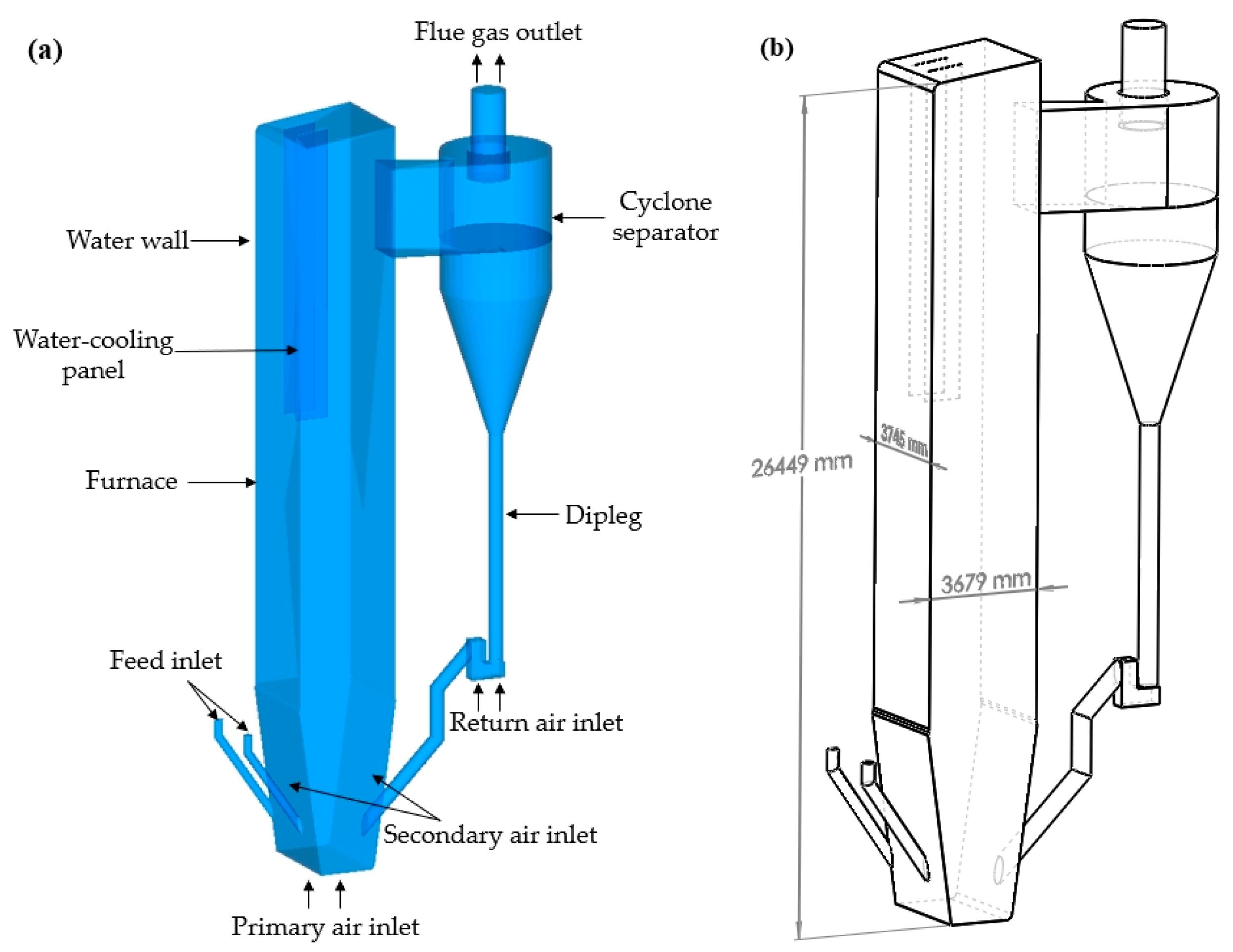
2.2. Computational Geometry and Mesh
2.2.1. Boiler Structure and Geometry
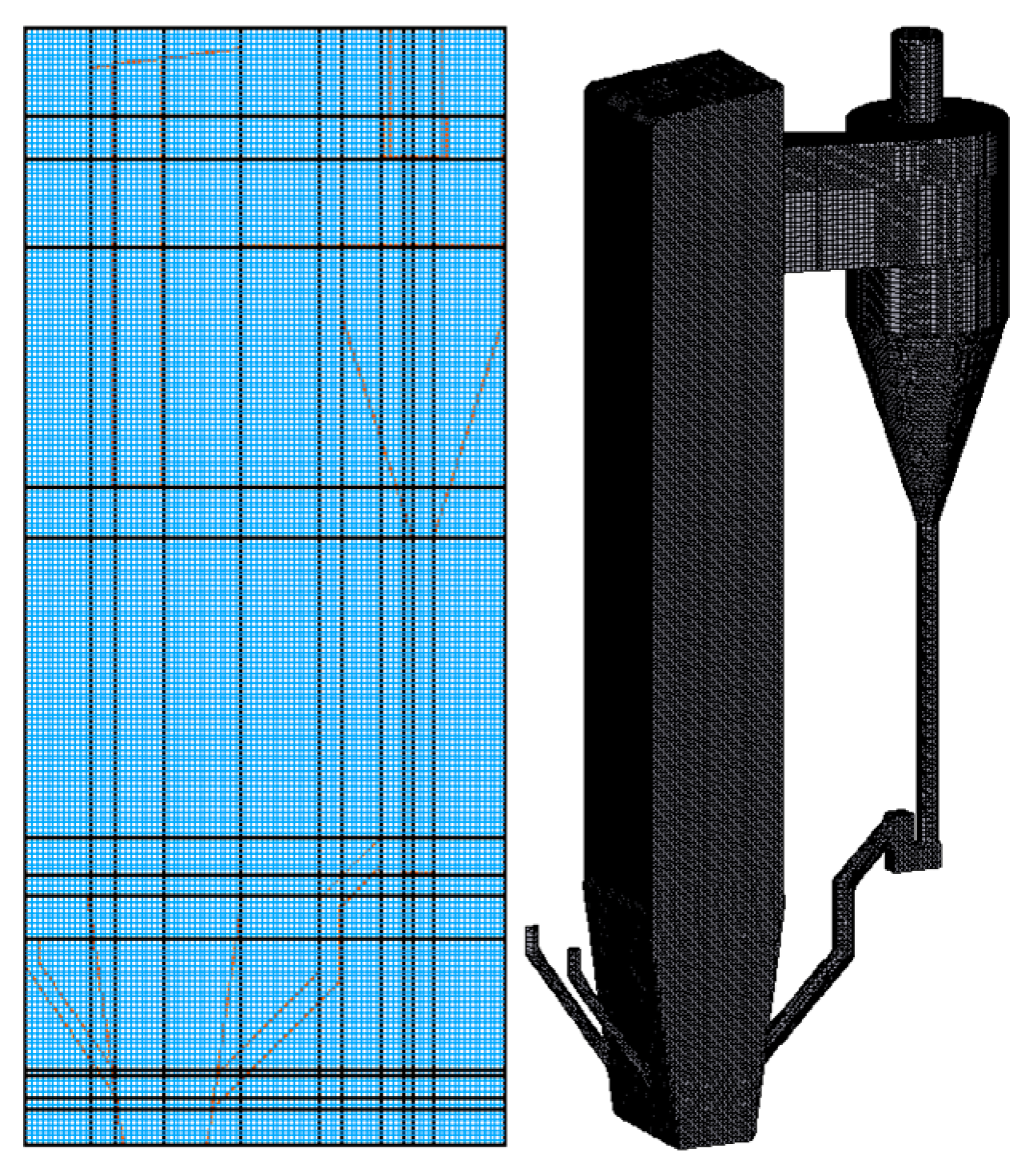
2.2.2. Meshing and Validation
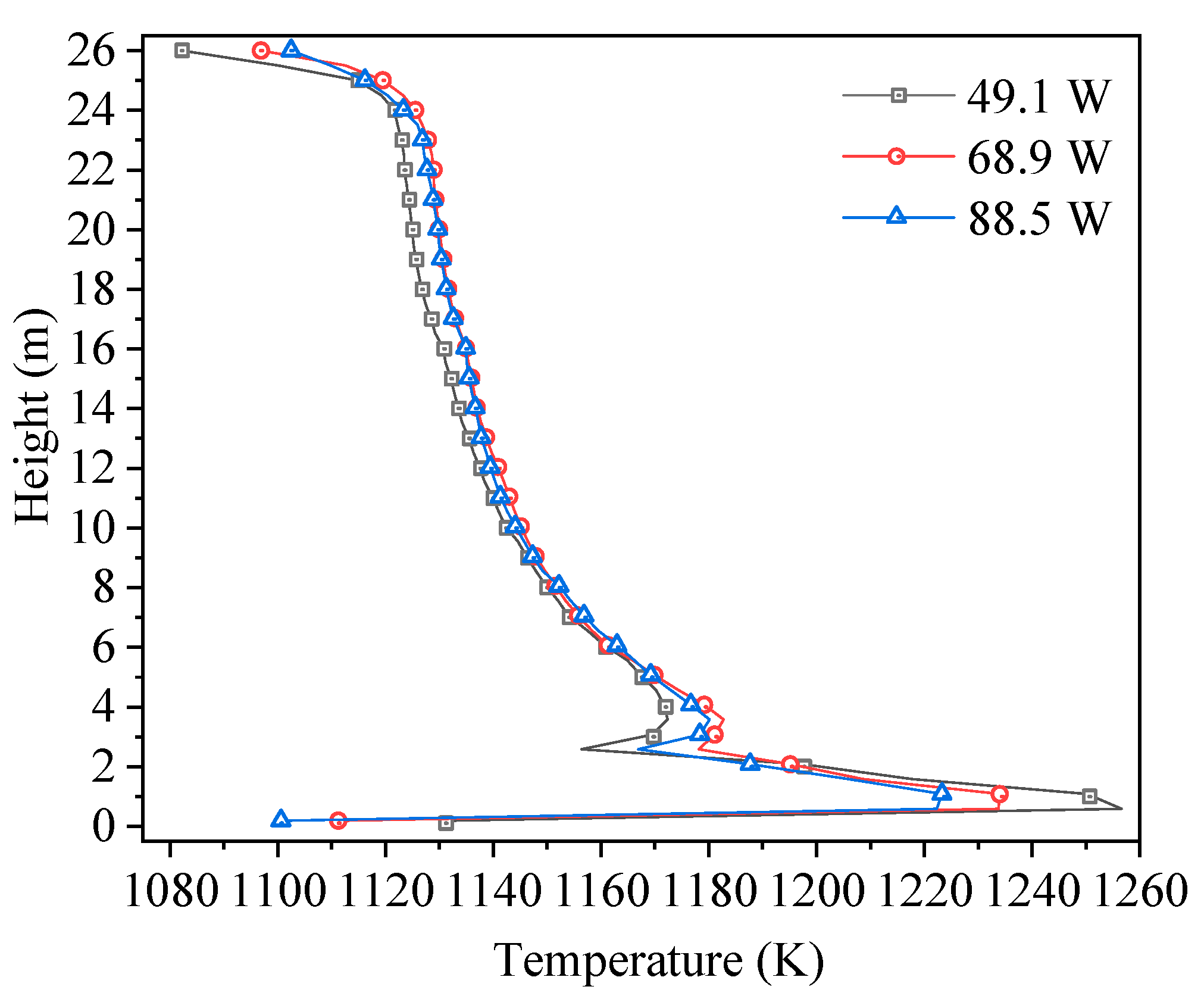
2.2.3. Model Validation
2.3. Materials Property and Simulation Conditions
2.3.1. Fuels and Bed Material
2.3.2. Simulation Conditions
3. Results and Discussion
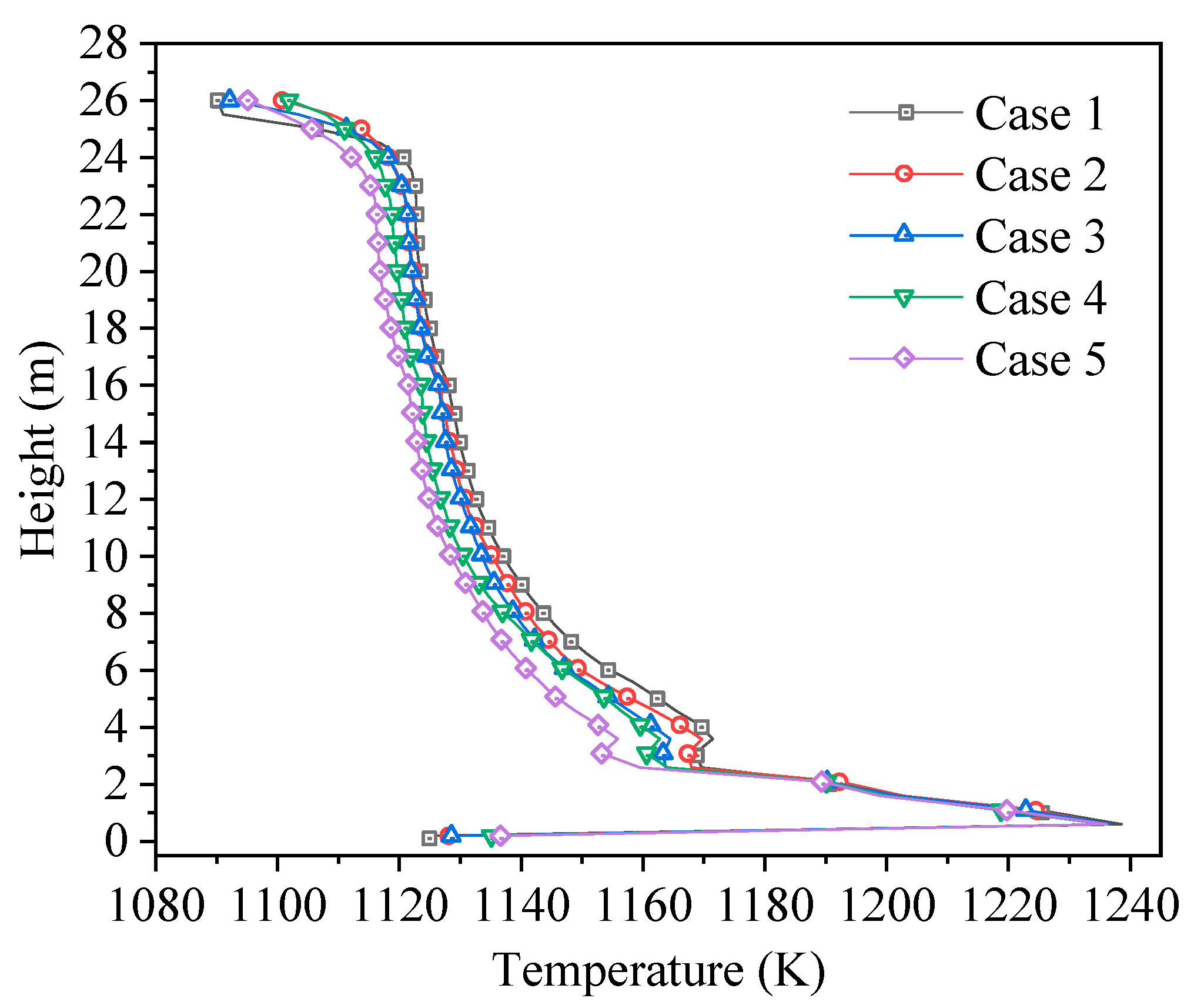
3.1. Co-Combustion of Coal and Biomass
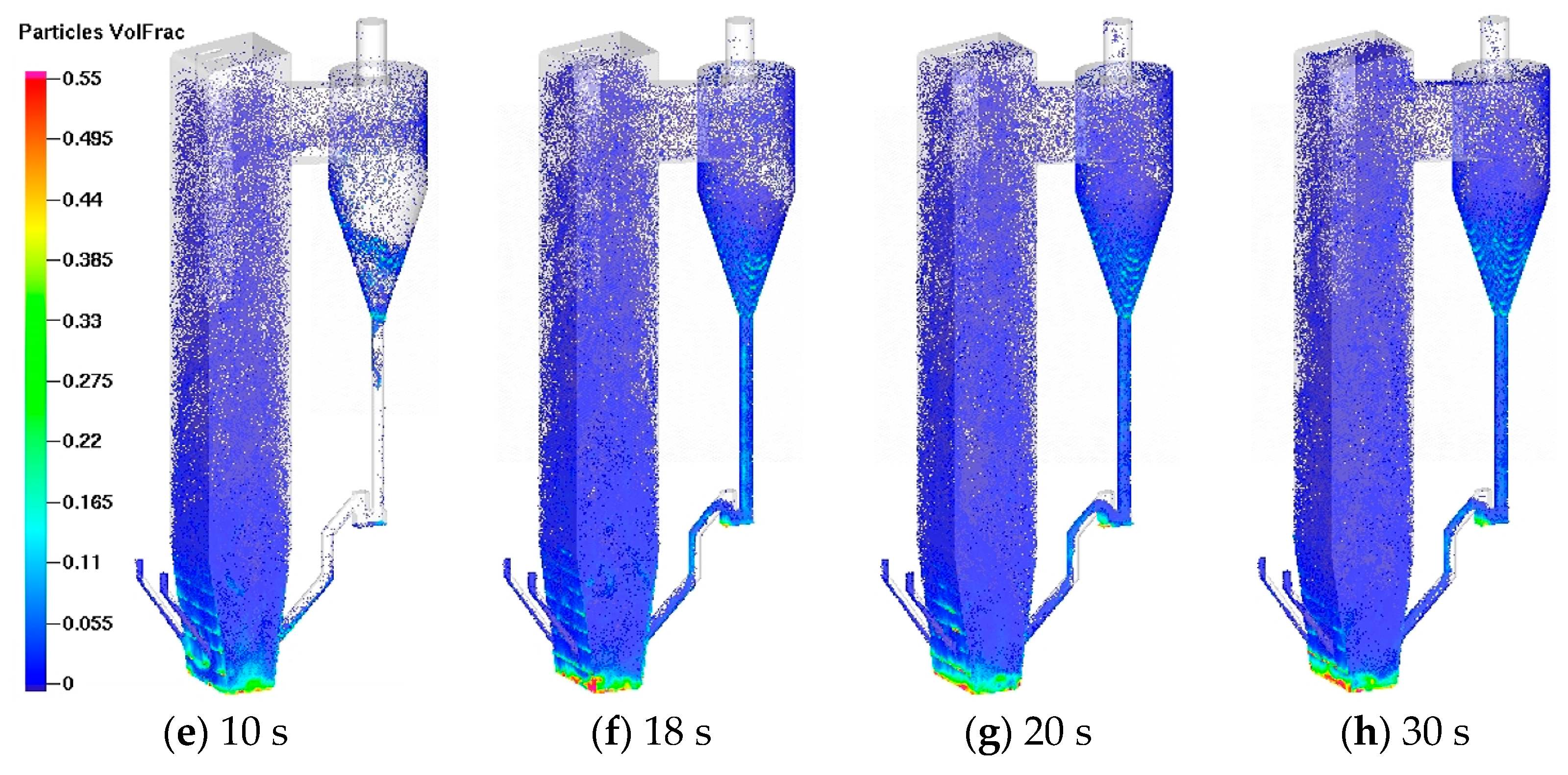
3.1.1. Flow Characteristics
3.1.2. Combustion Characteristics
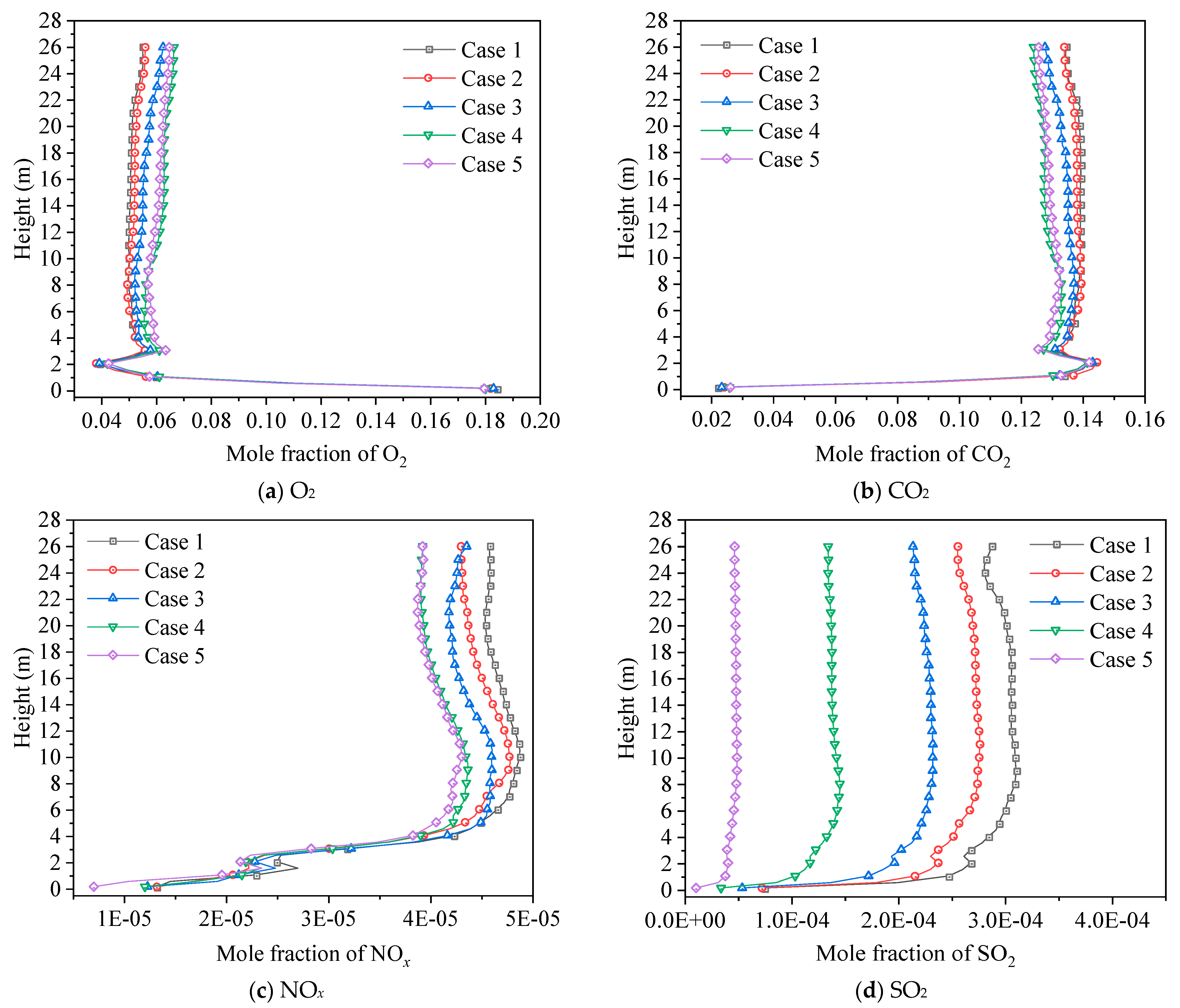
3.1.3. Gas Emission
3.2. Tri-Combustion of Coal, Biomass, and Oil Sludge
3.2.1. Furnace Temperature Distributions
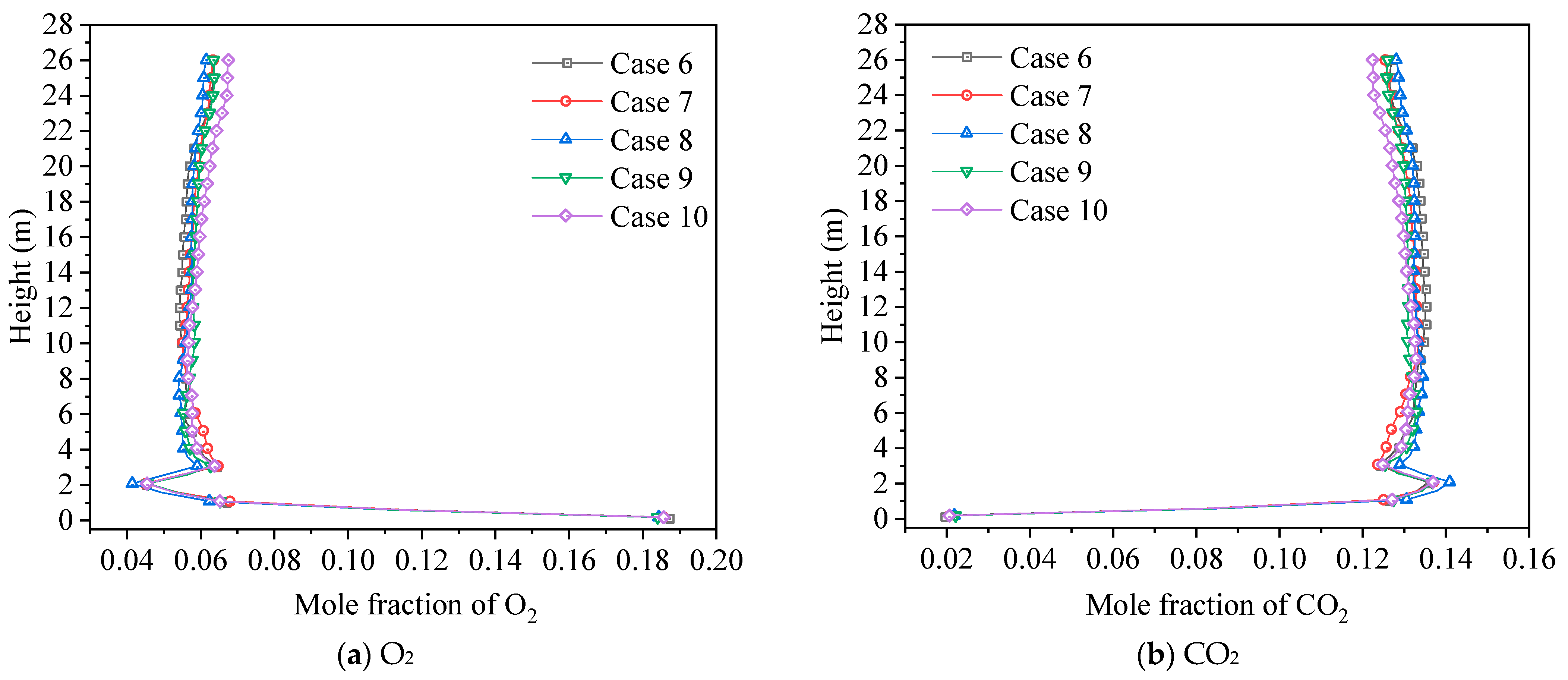
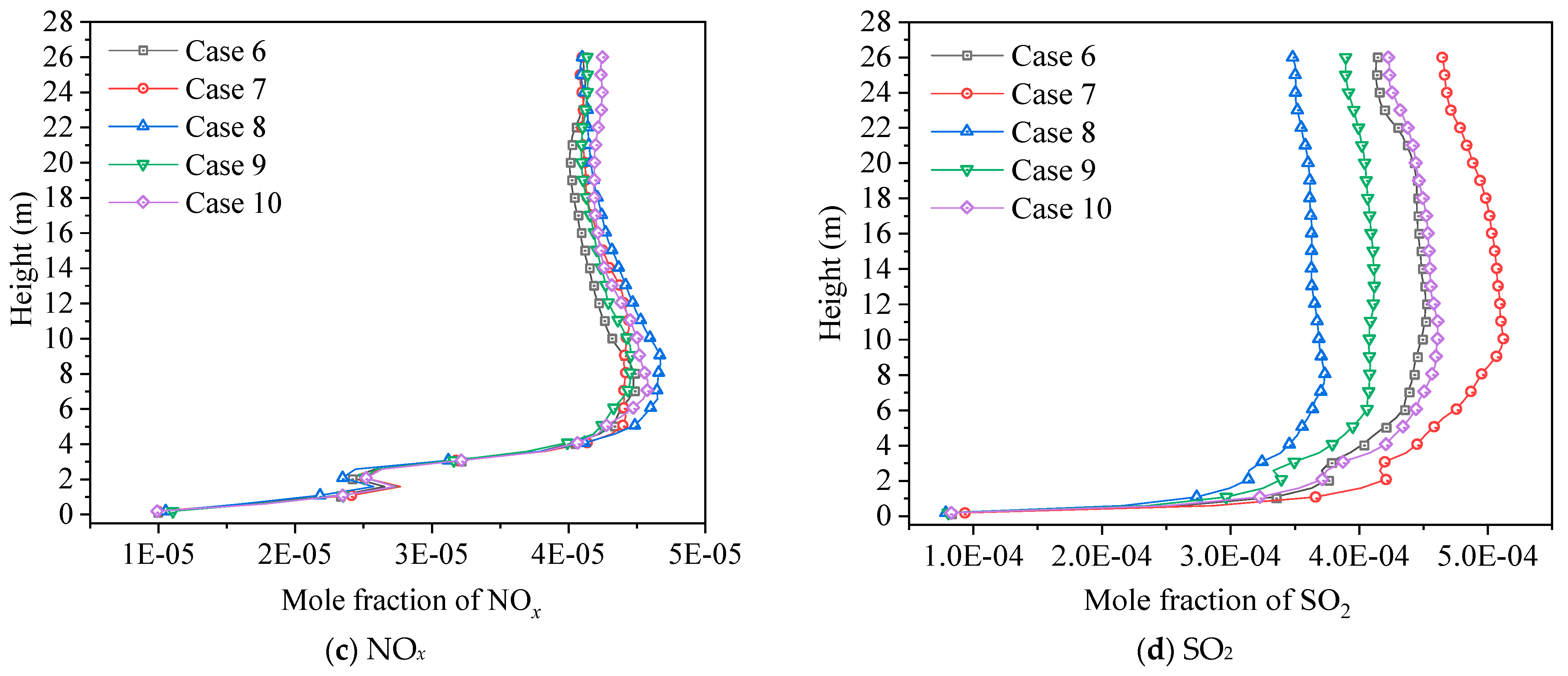
3.2.2. Gas Emission
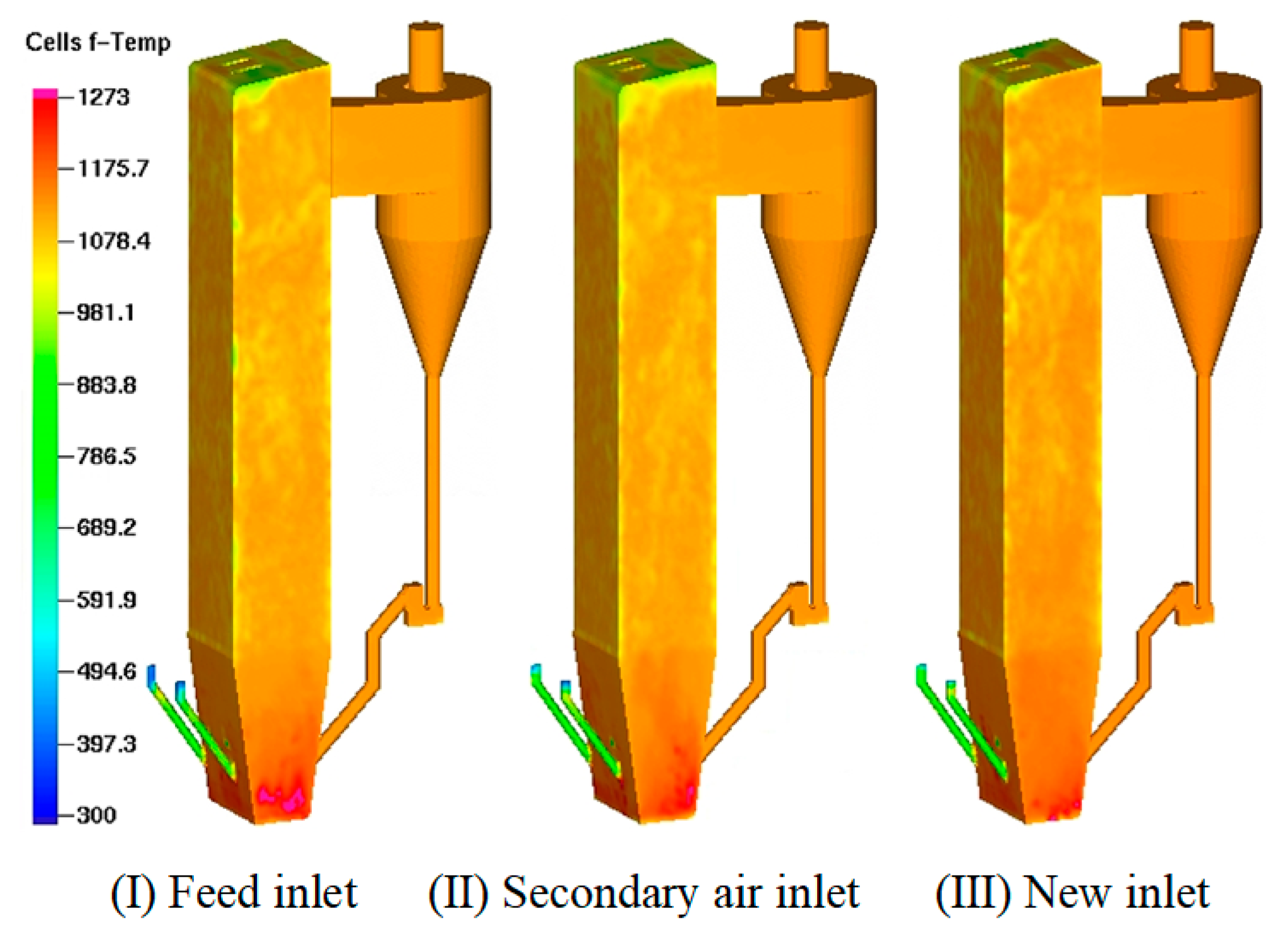
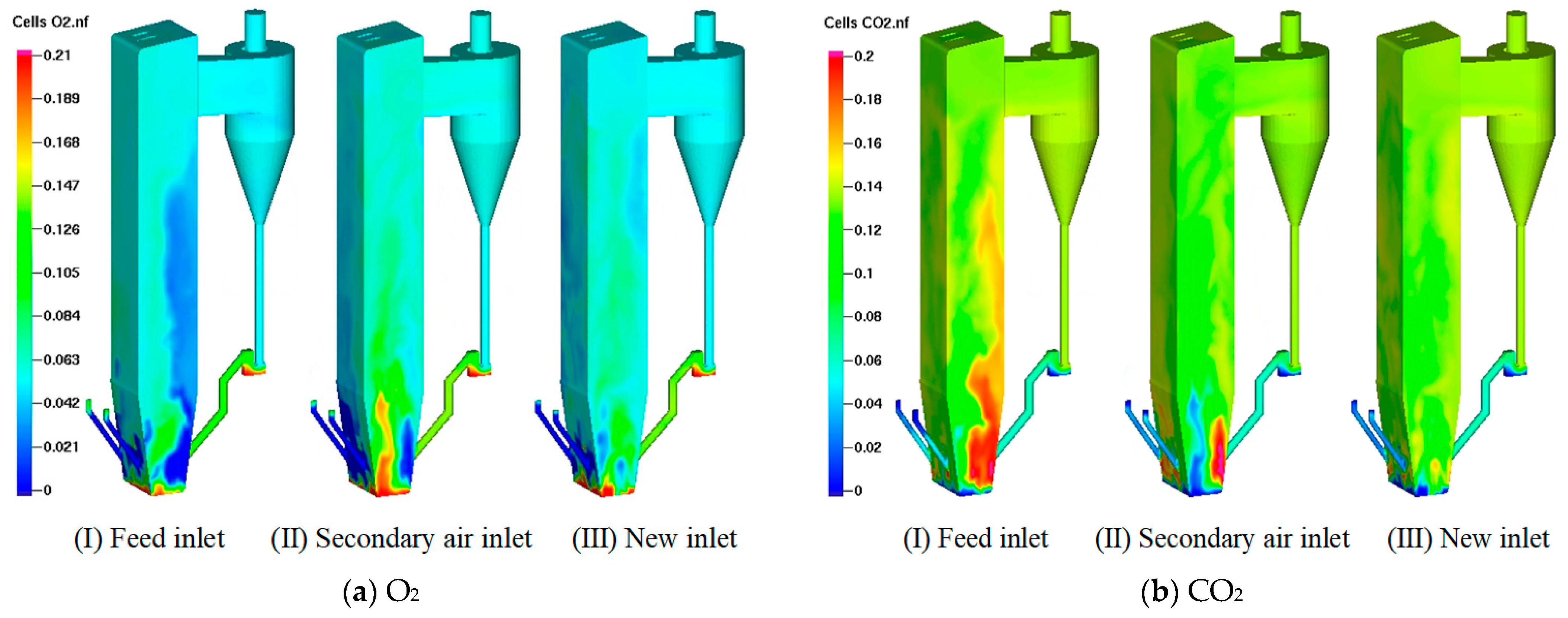
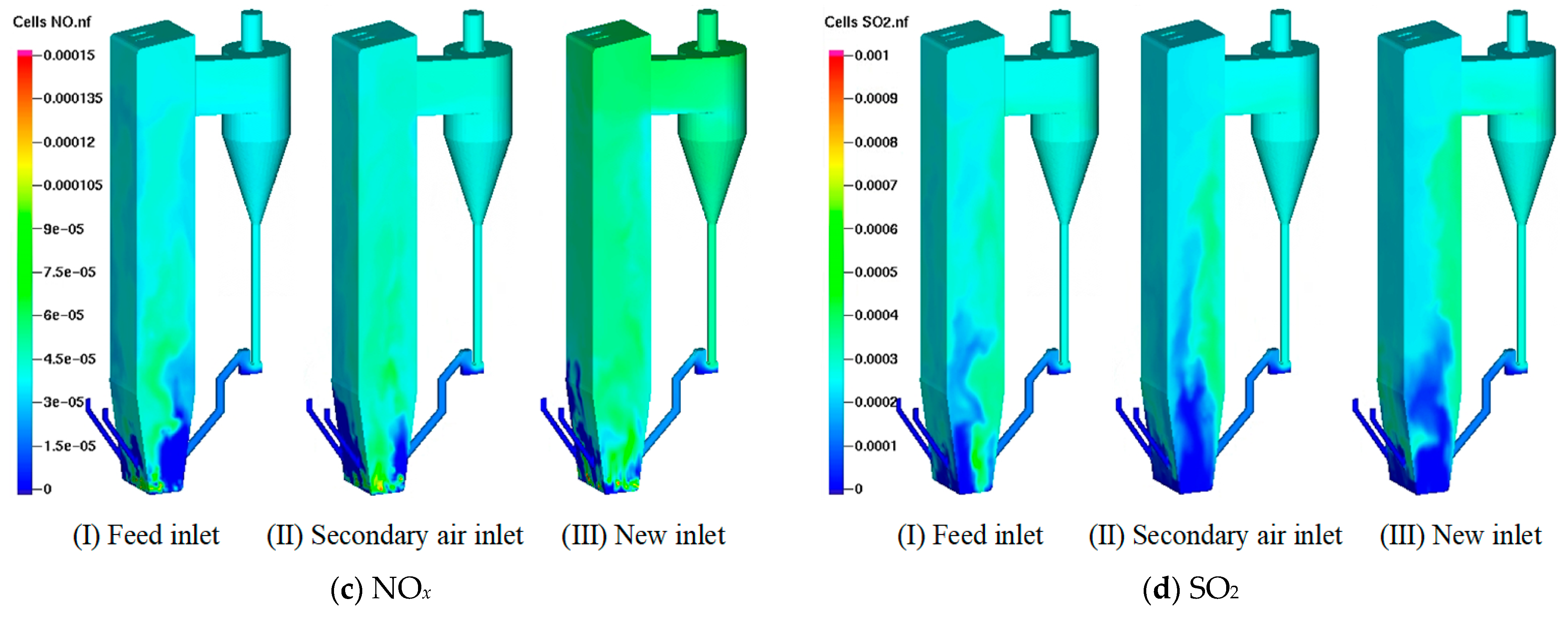
3.3. Effect of Biomass Inlet Position
3.3.1. Furnace Temperature Distributions
3.3.2. Gas Emission
4. Conclusions
- (1)
- After 18 s of simulation, the flow of particles reaches a quasi-steady state. As the water wall continues to absorb heat, the temperature gradually decreases along the height of the furnace. When the biomass blending ratio rises from 40% to 100%, the O2 mole fraction at the furnace outlet increases from 0.0541 to 0.0640, while the CO2 mole fraction decreases from 0.1357 to 0.1267. The average NOx mole fraction in the furnace height direction changes similarly, growing rapidly at first and then gradually decreasing, which could be related to the reduction of NOx to N2. As the biomass blending ratio increases from 40% to 100%, the NOx mole fraction at the furnace outlet decreases from 4.5867 × 10−5 to 3.9096 × 10−5. The SO2 mole fraction drops from 2.8253 × 10−4 to 4.6635 × 10−5.
- (2)
- The change trend of furnace temperature is essentially constant in different cases. When the biomass blending ratio increases from 35% to 50%, the O2 mole fraction at the furnace outlet increases from 0.0606 to 0.0667. CO2 shows the opposite distribution pattern. The NOx mole fraction initially increases rapidly and then gradually decreases, with a range from 4.1173 × 10−5 to 4.2556 × 10−5 at the furnace outlet. The distribution of SO2 along the furnace height is tightly related to the blending ratio of different fuels. As the oil sludge blending ratio rises from 10% to 20%, the mole fraction of SO2 at the furnace outlet increases from 3.5176 × 10−4 to 4.7043 × 10−4.
- (3)
- Compared with feeding biomass fuel through the feed inlet and secondary air inlet, the temperature distribution of the biomass furnace fed from the new inlet is more uniform. The uniformity of O2 and CO2 distribution is that the new inlet has the best uniformity, followed by the secondary air inlet and the feed inlet is the worst. When biomass particles are fed from the feed inlet, secondary air inlet, and new inlet, the mole fractions of NOx at the furnace outlet are 3.9096 × 10−5, 4.1022 × 10−5, and 5.1537 × 10−5, respectively, while the mole fractions of SO2 are 2.5978 × 10−4, 2.5738 × 10−4, and 2.5278 × 10−4, respectively.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Function of the Reynolds number | Interpolation function | ||
| Turbulent mass diffusion | t | Time | |
| Momentum transfer coefficient | Gas phase velocity | ||
| Particle fractional force | Grid cell volume | ||
| F | Momentum exchange rate | vp | Particle velocity |
| hf | Enthalpy of the gas phase | xp | Particle spatial position |
| mp | Particle mass | Mass fraction | |
| Nu | Nusselt number | Gas phase volume fraction | |
| Np | Total number of particles | Gas phase density | |
| Number of real particles | ρp | Particle density | |
| P | Gas phase pressure | Length scale along the x, y, and z directions | |
| Qradi | Radiation heat transfer | Heat of reaction of the gas phase | |
| Qpf | Convective heat transfer | Collision damping time | |
| Qreact | Chemical reaction heat | Stress tensor of the gas phase | |
| Enthalpy diffusion term | τp | Particle normal stress | |
| q | Heat flux | Shear viscosity | |
| Heat transfer rate | Turbulent viscosity |
References
- Jia, W.; Jia, X.; Wu, L.; Guo, Y.; Yang, T.; Wang, E.; Xiao, P. Research on regional differences of the impact of clean energy development on carbon dioxide emission and economic growth. Humanit. Soc. Sci. Commun. 2022, 9, 25. [Google Scholar] [CrossRef]
- Gu, C.; Ye, X.; Cao, Q.; Guan, W.; Peng, C.; Wu, Y.; Zhai, W. System dynamics modelling of urbanization under energy constraints in China. Sci. Rep. 2020, 10, 9956. [Google Scholar] [CrossRef] [PubMed]
- Zou, C.; Ma, F.; Pan, S.; Lin, M.; Zhang, G.; Xiong, B.; Wang, Y.; Liang, Y.; Yang, Z. Earth energy evolution, human development and carbon neutral strategy. Pet. Explor. Dev. 2022, 49, 468–488. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, F.; Wei, H.; Zhang, D.; Miu, C.; Pi, Q.; Luo, R.; Gu, Q. The Integrated Development Practices of Oil, Gas and New Energy in Tarim Oilfield Company toward Carbon Peaking and Carbon Neutrality Goals. Xinjiang Oil Gas 2022, 18, 16–20. [Google Scholar]
- Owusu, P.A.; Asumadu-Sarkodie, S.; Dubey, S. A review of renewable energy sources, sustainability issues and climate change mitigation. Cogent Eng. 2016, 3, 1167990. [Google Scholar] [CrossRef]
- Jana, K.; De, S. Biomass integrated gasification combined cogeneration with or without CO2 capture—A comparative thermodynamic study. Renew. Energy 2014, 72, 243–252. [Google Scholar] [CrossRef]
- Ahmad, A.A.; Zawawi, N.A.; Kasim, F.H.; Inayat, A.; Khasri, A. Assessing the gasification performance of biomass: A review on biomass gasification process conditions, optimization and economic evaluation. Renew. Sustain. Energy Rev. 2016, 53, 1333–1347. [Google Scholar] [CrossRef]
- Demirbas, A. Potential applications of renewable energy sources, biomass combustion problems in boiler power systems and combustion related environmental issues. Prog. Energy Combust. Sci. 2005, 31, 171–192. [Google Scholar] [CrossRef]
- Chen, W.-H.; Lin, B.-J.; Lin, Y.-Y.; Chu, Y.-S.; Ubando, A.T.; Show, P.L.; Ong, H.C.; Chang, J.-S.; Ho, S.-H.; Culaba, A.B.; et al. Progress in biomass torrefaction: Principles, applications and challenges. Prog. Energy Combust. Sci. 2021, 82, 100887. [Google Scholar] [CrossRef]
- Bridgwater, A.V.; Toft, A.J.; Brammer, J.G. A techno-economic comparison of power production by biomass fast pyrolysis with gasification and combustion. Renew. Sustain. Energy Rev. 2002, 6, 181–246. [Google Scholar] [CrossRef]
- Zheng, Y.; Jensen, P.A.; Jensen, A.D.; Sander, B.; Junker, H. Ash transformation during co-firing coal and straw. Fuel 2007, 86, 1008–1020. [Google Scholar] [CrossRef]
- Zhou, W.; Zhao, C.; Duan, L.; Chen, X.; Liu, D. A simulation study of coal combustion under O2/CO2 and O2/RFG atmospheres in circulating fluidized bed. Chem. Eng. J. 2013, 223, 816–823. [Google Scholar] [CrossRef]
- Yang, X.; Luo, Z.; Liu, X.; Yu, C.; Li, Y.a.; Ma, Y. Experimental and numerical investigation of the combustion characteristics and NO emission behaviour during the co-combustion of biomass and coal. Fuel 2021, 287, 119383. [Google Scholar] [CrossRef]
- Karampinis, E.; Nikolopoulos, N.; Nikolopoulos, A.; Grammelis, P.; Kakaras, E. Numerical investigation Greek lignite/cardoon co-firing in a tangentially fired furnace. Appl. Energy 2012, 97, 514–524. [Google Scholar] [CrossRef]
- Guo, F.; Zhong, Z. Co-combustion of anthracite coal and wood pellets: Thermodynamic analysis, combustion efficiency, pollutant emissions and ash slagging. Environ. Pollut. 2018, 239, 21–29. [Google Scholar] [CrossRef] [PubMed]
- Varol, M.; Symonds, R.; Anthony, E.J.; Lu, D.; Jia, L.; Tan, Y. Emissions from co-firing lignite and biomass in an oxy-fired CFBC. Fuel Process. Technol. 2018, 173, 126–133. [Google Scholar] [CrossRef]
- Mazlova, E.A.; Meshcheryakov, S.V. Ecological characteristics of oil sludges. Chem. Technol. Fuels Oils 1999, 35, 49–53. [Google Scholar] [CrossRef]
- Zhao, C.; Li, Y.; Gan, Z.; Nie, M. Method of smoldering combustion for refinery oil sludge treatment. J. Hazard. Mater. 2021, 409, 124995. [Google Scholar] [CrossRef]
- Liu, J.; Jiang, X.; Zhou, L.; Wang, H.; Han, X. Co-firing of oil sludge with coal–water slurry in an industrial internal circulating fluidized bed boiler. J. Hazard. Mater. 2009, 167, 817–823. [Google Scholar] [CrossRef]
- Milićević, A.; Belošević, S.; Crnomarković, N.; Tomanović, I.; Stojanović, A.; Tucaković, D.; Lei, D.; Che, D. Numerical study of co-firing lignite and agricultural biomass in utility boiler under variable operation conditions. Int. J. Heat Mass Transf. 2021, 181, 121728. [Google Scholar] [CrossRef]
- Smith, J.D.; Suo-Antilla, A.; Sreedharan, V. Transient LES based CFD modeling of coal-biomass co-firing combustion. Fuel Process. Technol. 2019, 193, 187–196. [Google Scholar] [CrossRef]
- Loha, C.; Chattopadhyay, H.; Chatterjee, P.K. Three dimensional kinetic modeling of fluidized bed biomass gasification. Chem. Eng. Sci. 2014, 109, 53–64. [Google Scholar] [CrossRef]
- Abbasi, A.; Ege, P.E.; de Lasa, H.I. CPFD simulation of a fast fluidized bed steam coal gasifier feeding section. Chem. Eng. Sci. 2011, 174, 341–350. [Google Scholar] [CrossRef]
- Chang, J.; Ma, X.; Wang, X.; Li, X. CPFD modeling of hydrodynamics, combustion and NOx emissions in an industrial CFB boiler. Particuology 2023, 81, 174–188. [Google Scholar] [CrossRef]
- Chen, X.; Ma, J.; Tian, X.; Wan, J.; Zhao, H. CPFD simulation and optimization of a 50 kWth dual circulating fluidized bed reactor for chemical looping combustion of coal. Int. J. Greenh. Gas Control 2019, 90, 102800. [Google Scholar] [CrossRef]
- Liu, H.; Sun, H.; Bi, Y.; Jia, C.; Zhang, L.; Li, Y.; Qin, H.; Wang, Q. Effect of secondary air on NO emission in a 440 t/h circulating fluidized bed boiler based on CPFD method. Particuology 2023, 83, 18–31. [Google Scholar] [CrossRef]
- Ding, H.; Ouyang, Z.; Su, K.; Zhang, J. Investigation of gas-solid flow characteristics in a novel internal fluidized bed combustor by experiment and CPFD simulation. Adv. Powder Technol. 2023, 34, 103962. [Google Scholar] [CrossRef]
- Yan, J.; Lu, X.; Xue, R.; Lu, J.; Zheng, Y.; Zhang, Y.; Liu, Z. Validation and application of CPFD model in simulating gas-solid flow and combustion of a supercritical CFB boiler with improved inlet boundary conditions. Fuel Process. Technol. 2020, 208, 106512. [Google Scholar] [CrossRef]
- Yang, S.; Fan, F.; Wei, Y.; Hu, J.; Wang, H.; Wu, S. Three-dimensional MP-PIC simulation of the steam gasification of biomass in a spouted bed gasifier. Energy Convers. Manag. 2020, 210, 112689. [Google Scholar] [CrossRef]
- Snider, D.M.; Clark, S.M.; O’Rourke, P.J. Eulerian–Lagrangian method for three-dimensional thermal reacting flow with application to coal gasifiers. Chem. Eng. Sci. 2011, 66, 1285–1295. [Google Scholar] [CrossRef]
- Yang, S.; Zhou, T.; Wei, Y.; Hu, J.; Wang, H. Influence of size-induced segregation on the biomass gasification in bubbling fluidized bed with continuous lognormal particle size distribution. Energy Convers. Manag. 2019, 198, 111848. [Google Scholar] [CrossRef]
- Harris, S.E.; Crighton, D.G. Solitons, solitary waves, and voidage disturbances in gas-fluidized beds. J. Fluid Mech. 2006, 266, 243–276. [Google Scholar] [CrossRef]
- Chang, J.; Ma, X.; Wang, X.; Li, X. Computational particle fluid dynamics modeling and design of in-situ catalytic deNOx in an industrial CFB boiler. Chem. Eng. Sci. 2023, 270, 118502. [Google Scholar] [CrossRef]
- Xu, L.; Cheng, L.; Ji, J.; Wang, Q.; Fang, M. A comprehensive CFD combustion model for supercritical CFB boilers. Particuology 2019, 43, 29–37. [Google Scholar] [CrossRef]
- Snider, D.M. An Incompressible Three-Dimensional Multiphase Particle-in-Cell Model for Dense Particle Flows. J. Comput. Phys. 2001, 170, 523–549. [Google Scholar] [CrossRef]
- Shen, X.; Li, J.; Jia, L.; Wang, Y.; Guo, B.; Qiao, X.; Yang, H.; Zhang, M.; Jin, Y. Numerical simulation of NO and SO2 emission dynamic characteristics during thermal start-up of CFB boiler. Part. Sci. Technol. 2022, 41, 53–63. [Google Scholar] [CrossRef]
- Ji, J.; Cheng, L.; Wei, Y.; Wang, J.; Gao, X.; Fang, M.; Wang, Q. Predictions of NOx/N2O emissions from an ultra-supercritical CFB boiler using a 2-D comprehensive CFD combustion model. Particuology 2020, 49, 77–87. [Google Scholar] [CrossRef]
- Maffei, T.; Frassoldati, A.; Cuoci, A.; Ranzi, E.; Faravelli, T. Predictive one step kinetic model of coal pyrolysis for CFD applications. Proc. Combust. Inst. 2013, 34, 2401–2410. [Google Scholar] [CrossRef]
- Syamlal, M.; Bissett, L.A. METC Gasifier Advanced Simulation (MGAS) Model; U.S. Department of Energy: Washington, DC, USA, 1992. [Google Scholar] [CrossRef]
- Wu, Y.; Liu, D.; Ma, J.; Chen, X. Three-Dimensional Eulerian–Eulerian Simulation of Coal Combustion under Air Atmosphere in a Circulating Fluidized Bed Combustor. Energy Fuels 2017, 31, 7952–7966. [Google Scholar] [CrossRef]
- Kong, D.; Wang, S.; Luo, K.; Hu, C.; Li, D.; Fan, J. Three-dimensional simulation of biomass gasification in a full-loop pilot-scale dual fluidized bed with complex geometric structure. Renew. Energy 2020, 157, 466–481. [Google Scholar] [CrossRef]
- Xie, J.; Zhong, W.; Jin, B.; Shao, Y.; Liu, H. Three-Dimensional Eulerian–Eulerian Modeling of Gaseous Pollutant Emissions from Circulating Fluidized-Bed Combustors. Energy Fuels 2014, 28, 5523–5533. [Google Scholar] [CrossRef]
- Zhou, W.; Zhao, C.S.; Duan, L.B.; Chen, X.P.; Liang, C. Two-dimensional computational fluid dynamics simulation of nitrogen and sulfur oxides emissions in a circulating fluidized bed combustor. Chem. Eng. J. 2011, 173, 564–573. [Google Scholar] [CrossRef]



| Items | Equations |
|---|---|
| Continuity equation (gas phase) | |
| Momentum equation (gas phase) | |
| Species equation | |
| Energy equation | |
| Transport equation (particle phase) | |
| Particle trajectory [30,31] | |
| Wenyu–Ergun drag model | |
| Energy exchange |
| Number | Reaction | Rate Equation | Reaction Rate (mol m−3 s−1) |
|---|---|---|---|
| 1 | H2O(moisture)→H2O(g) | R1 = k1 [H2O] | k1 = 5.13 × 1010 exp(−10,585/T) |
| 2 | Volatile→α1Tar + α2CO + α3CO2 + α4CH4 + α5H2 + α6H2O(g) + α7NH3 + α8HCN + α9H2S | R2 = k2 [Volatile] | k2 = 0.5T exp(−5500/T) |
| 3 | CO + 0.5O2→CO2 | R3 = k3 [CO] [O2] | k3 = 1010 exp(−15,119/T) |
| 4 | CH4 + 2O2→CO2 + 2H2O | R4 = k4 [CH4] [O2] | k4 = 5.01 × 1011 exp(−3430/T) |
| 5 | H2 + 0.5O2→H2O | R5 = k5 [H2]1.5[O2] | k5 = 1.03 × 1014T−1.5 exp(−3430/T) |
| 6 | Tar + O2→CO2 + 2H2O | R6 = k6 [Tar] [O2] | k6 = 3.8 × 107 exp(−6710/T) |
| 7 | C + 0.5O2→CO | R7 = k7 [O2] | k7 = 1.47 × 108θcT exp(−6710/T) |
| 8 | C + H2O→CO + H2 | R8 = k8 [H2O] | k8 = 6.36mcT exp(−13,590/T) |
| 9 | CO + H2→C + H2O | R9 = k9 [H2] [CO] | k9 = 5.218 × 10−4mcT2 exp(−6319/T−17.29) |
| 10 | C + CO2→2CO | R10 = k10 [CO2] | k10 = 6.36mcT exp(−22,645/T) |
| 11 | 2CO→C + CO2 | R11 = k11 [CO] | k11 = 5.218mcT2 exp(−2363/T−20.92) |
| 12 | H2S + 1.5O2→H2O + SO2 | R12 = k12 [H2S] [O2] | k12 = 5.2 × 108 exp(−19,300/RT) |
| Number | Reaction | Rate Equation | Reaction Rate (mol m−3 s−1) |
|---|---|---|---|
| 13 | HCN + 0.5O2→CNO + 0.5H2 | R13 = k13 [O2] [HCN] | k13–14 = 2.14 × 105 exp(−10,000/T) |
| 14 | CNO +0.5O2→NO+ CO | R14 = k14 [O2] [CNO] | |
| 15 | NH3 + 1.25O2→NO + 1.5H2O | R15 = k15 [O2] [NH3] | k15 = 2.73 × 1014 exp(−38,160/T) |
| 16 | NO + CO→0.5N2 + CO2 | R16 = k16 [CO] [NO] | k16 = 2.51 × 1011 exp(−10,000/T) |
| 17 | NO + C→0.5N2 + CO | R17 = k17 [NO] | k17 = 1.17 × 105 exp(−13,221/T) |
| 18 | NH3 + NO + 0.5O2→N2 + 1.5H2O | R18 = k18 ([NO] [O2] [NH3])0.5 | k18 = 1.11 × 1012 exp(−29,400/T) |
| Parameter | Numerical Result | Measured Data | Relative Error |
|---|---|---|---|
| Bed temperature | 922.3 °C | 912.0 °C | 1.13% |
| Bed pressure | 6.15 kPa | 6.32 kPa | 2.69% |
| Outlet gas temperature | 856.6 °C | 851.0 °C | 0.65% |
| Samples | Ultimate Analysis | Proximate Analysis | |||||||
|---|---|---|---|---|---|---|---|---|---|
| C | H | O a | N | S | FC a | M | A | V | |
| Coal | 52.74 | 2.77 | 11.19 | 1.04 | 0.61 | 43.08 | 22.00 | 9.66 | 25.26 |
| Biomass | 43.29 | 5.15 | 37.74 | 0.46 | 0.04 | 16.08 | 6.70 | 6.61 | 70.61 |
| Oil sludge | 21.12 | 3.10 | 0.27 | 0.17 | 0.52 | 2.23 | 41.6 | 33.22 | 22.95 |
| Parameters | Value |
|---|---|
| Initial height of bed (mm) | 500 |
| Coal feed (kg/s) | 2.382 |
| Primary air (kg/s) | 14.5805 |
| Primary air temperature (°C) | 180.0 |
| Primary air pressure (Pa) | 109,030.5 |
| Secondary air (kg/s) | 5.1292 |
| Secondary air temperature (°C) | 170.0 |
| Secondary air pressure (Pa) | 102,634.0 |
| Return air (kg/s) | 0.293 |
| Return air temperature (°C) | 38.0 |
| Return air pressure (Pa) | 113,127.0 |
| Water wall temperature (°C) | 332.0 |
| Particle normal-to-wall retention coefficient | 0.99 |
| Particle tangential-to-wall retention coefficient | 0.3 |
| Radiation emissivity (%) | 70 |
| Time step (s) | 5 × 10−4 |
| Case | Mixing Ratio (Coal/Biomass, wt%) | Feeding Amount (kg/s) | Primary Air Amount (kg/s) | Secondary Air Amount (kg/s) | Return Air Amount (kg/s) |
|---|---|---|---|---|---|
| Case 1 | 60:40 | 2.550 | 14.234 | 5.007 | 0.286 |
| Case 2 | 50:50 | 2.595 | 14.140 | 4.972 | 0.284 |
| Case 3 | 40:60 | 2.642 | 14.041 | 4.940 | 0.282 |
| Case 4 | 20:80 | 2.742 | 13.834 | 4.868 | 0.278 |
| Case 5 | 0:100 | 2.849 | 13.611 | 4.788 | 0.274 |
| Cases | Temperature (K) | O2 | NOx | SO2 |
|---|---|---|---|---|
| Case 1 | 1122.2 | 0.0541 | 4.5867 × 10−5 | 2.8253 × 10−4 |
| Case 2 | 1119.7 | 0.0549 | 4.3185 × 10−5 | 2.5978 × 10−4 |
| Case 3 | 1119.5 | 0.0608 | 4.2585 × 10−5 | 2.1631 × 10−4 |
| Case 4 | 1117.2 | 0.0637 | 3.9170 × 10−5 | 1.3463 × 10−4 |
| Case 5 | 1114.2 | 0.0640 | 3.9096 × 10−5 | 4.6635 × 10−5 |
| Case | Blending Ratio (Coal/Biomass/Oil Sludge, wt%) | Feeding Amount (kg/s) | Primary Air Amount (kg/s) | Secondary Air Amount (kg/s) | Return Air Amount (kg/s) |
|---|---|---|---|---|---|
| Case 6 | 50:35:15 | 2.787 | 14.554 | 5.120 | 0.292 |
| Case 7 | 45:35:20 | 2.886 | 14.658 | 5.156 | 0.295 |
| Case 8 | 45:45:10 | 2.745 | 14.361 | 5.052 | 0.289 |
| Case 9 | 40:45:15 | 2.841 | 14.456 | 5.084 | 0.291 |
| Case 10 | 35:45:20 | 2.945 | 14.559 | 5.122 | 0.293 |
| Cases | Temperature (K) | O2 | NOx | SO2 |
|---|---|---|---|---|
| Case 6 | 1120.4 | 0.0626 | 4.2556 × 10−5 | 4.1735 × 10−4 |
| Case 7 | 1117.9 | 0.0623 | 4.1440 × 10−5 | 4.7043 × 10−4 |
| Case 8 | 1119.7 | 0.0606 | 4.1404 × 10−5 | 3.3041 × 10−4 |
| Case 9 | 1119.6 | 0.0631 | 4.1207 × 10−5 | 3.9482 × 10−4 |
| Case 10 | 1118.6 | 0.0667 | 4.1173 × 10−5 | 4.3007 × 10−5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Chen, X.; Xu, L.; Ma, M.; Huang, X.; Han, F.; Zhou, Y.; Du, C.; Da, Y.; Deng, L. Computational Particle Fluid Dynamics Simulation on Combustion Characteristics of Blended Fuels of Coal, Biomass, and Oil Sludge in a 130 t h−1 Circulating Fluidized Bed Boiler. Energies 2024, 17, 149. https://doi.org/10.3390/en17010149
Wang Y, Chen X, Xu L, Ma M, Huang X, Han F, Zhou Y, Du C, Da Y, Deng L. Computational Particle Fluid Dynamics Simulation on Combustion Characteristics of Blended Fuels of Coal, Biomass, and Oil Sludge in a 130 t h−1 Circulating Fluidized Bed Boiler. Energies. 2024; 17(1):149. https://doi.org/10.3390/en17010149
Chicago/Turabian StyleWang, Yang, Xiangyu Chen, Liping Xu, Mingwei Ma, Xiaole Huang, Feng Han, Yong Zhou, Chen Du, Yaodong Da, and Lei Deng. 2024. "Computational Particle Fluid Dynamics Simulation on Combustion Characteristics of Blended Fuels of Coal, Biomass, and Oil Sludge in a 130 t h−1 Circulating Fluidized Bed Boiler" Energies 17, no. 1: 149. https://doi.org/10.3390/en17010149
APA StyleWang, Y., Chen, X., Xu, L., Ma, M., Huang, X., Han, F., Zhou, Y., Du, C., Da, Y., & Deng, L. (2024). Computational Particle Fluid Dynamics Simulation on Combustion Characteristics of Blended Fuels of Coal, Biomass, and Oil Sludge in a 130 t h−1 Circulating Fluidized Bed Boiler. Energies, 17(1), 149. https://doi.org/10.3390/en17010149







