1. Introduction
In the context of escalating global energy demand and the extensive consumption of fossil fuels, the world is confronted with a severe energy crisis and environmental pollution [
1,
2]. The traditional utilization of fossil energy, due to its low energy conversion efficiency, is no longer the optimal choice. As an effective and promising solution, poly-generation technology can effectively meet a variety of needs, including electricity, cooling, heating, and even fuel and freshwater, in an environmentally friendly manner, which offers the possibility to alleviate the fossil energy crisis [
3]. Therefore, the development and application of poly-generation technology hold significant implications for addressing the current energy crisis and environmental issues [
4].
The typical power generation devices of a poly-generation system mainly include fuel cells, gas turbines, and internal combustion engines. The solid oxide fuel cell (SOFC) is a high-temperature fuel cell with the highest theoretical energy density. The SOFC/gas turbine (GT) hybrid power generation cycle is a highly efficient power generation device [
5]. It has advantages such as being able to burn a variety of fuels, co-producing a variety of products, having a high energy conversion efficiency, and ultra-low pollutant emissions [
6]. In addition, the characteristic of SOFC anode and cathode exhaust separation makes it particularly suitable for coupling with the CO
2 capture system, which can facilitate the poly-generation system in achieving carbon reduction or even zero carbon emissions.
The design and performance research of the combined cooling, heating, and power (CCHP) system based on SOFC/GT is being carried out mainly through numerical simulation methods. Ma et al. [
7] proposed a new type of CCHP system, which uses ammonia-water-mixed working fluid to recover the exhaust heat of SOFC/GT. Under given conditions, the comprehensive energy utilization rate of this system can exceed 80%. Zhao et al. [
8] designed a CCHP system driven by the SOFC/GT hybrid cycle with a coke oven gas as fuel, and studied the effects of fuel flow rate, hydrogen content in the coke oven gas, fuel utilization rate, and SOFC pressure on system performance. The power generation efficiency and comprehensive energy utilization rate of this cold, heat, and electricity cogeneration system can reach 73.32% and 90.03%, respectively. The CCHP system driven by the SOFC/GT hybrid cycle, as a highly efficient energy supply method, has great advantages in energy conservation and environmental protection. However, current research on its performance evaluation, parameter optimization, and other aspects is still insufficient, and further in-depth research needs to be carried out. At the same time, there is an urgent need to explore more efficient and economical system integration methods.
Currently, several nations are grappling with a significant issue: the limited supply of freshwater [
9]. The most common causes of water scarcity are population growth, industrial development, and finite water resources. Brackish water, which is widely distributed in various regions of China [
10], is considered one of the important water resources in semi-arid and arid areas. Its bitter and astringent taste makes it difficult to swallow. The impurities and excessive salts in the water are posing a serious threat to human health. Kidney stones and various types of cancer can be induced by long-term consumption [
11]. Secondary salinization of the soil and a year-by-year decline in crop yields will ensue if brackish water is used for long-term irrigation [
12]. The distillation of brackish water has been considered one of the practical solutions to the world’s freshwater problems [
13]. The optimal technological approach for incorporating heat distillation units into poly-generation power stations is through multi-effect distillation (MED). This method could potentially enhance the overall performance of the unit and its associated costs, despite its high energy consumption [
14].
Utilizing genetic algorithms for multi-objective optimization is an effective approach to find the best solutions when dealing with objectives that are in conflict [
15]. Abdullah et al. [
16] introduced a poly-generation plant for CCHP and freshwater generation and used multi-objective optimization to improve its energy and exergy efficiency from 73.9% to 79% and 71.35% to 73.9%, respectively, and reduce its total cost from 10.62 USD/h to 9.26 USD/h. A poly-generation system was proposed by Hadavi et al. [
17], designed to generate electricity, heating and cooling power, and desalinated water. The system is composed of a biomass gasification system, a solid oxide fuel cell, a gas turbine cycle, Rankine and organic Rankine cycles, an absorption refrigeration system, a multi-effect desalination unit, and a hot water line. A genetic algorithm was employed to optimize energy and exergy efficiencies and to reduce system cost. As a result, energy and exergy efficiencies were improved by 14.94% and 17.93%, respectively.
A novel poly-generation system, which uses biogas as fuel and can provide cooling, heating, electricity, and freshwater, is the aim of this study. The waste heat from the flue gas of the SOFC/GT hybrid power generation subsystem is utilized to desalinate brackish water. A mathematical model of this system is established, and its energy, exergy, and economic characteristics are simulated. The impact of key parameters on system performance is studied. Furthermore, multi-objective optimization research is conducted on this system to seek the maximization of energy utilization rate, exergy efficiency, total energy output, and freshwater production, and the minimization of cost rate.
2. System Description
As depicted in
Figure 1, the poly-generation system proposed in this study, which is based on SOFC/GT and multi-effect distillation, comprises four subsystems: an SOFC/GT hybrid power generation subsystem, a brackish water distillation subsystem, an absorption refrigeration subsystem, and a heating supply subsystem. Currently, the device for the poly-generation system depicted in
Figure 1 does not exist in reality. The feasibility of this poly-generation system will be explored through theoretical calculations in this study.
The SOFC/GT hybrid power generation subsystem primarily consists of an SOFC, an inverter, a combustion chamber, a gas turbine, an air compressor, a biogas compressor, a water pump, a mixer, and three preheaters. Its working principle is as follows: the air, biogas, and water required by the SOFC system are pressurized in the air compressor, biogas compressor, and water pump, respectively, to reach the operating pressure of the SOFC. Before entering the SOFC stack, the air, biogas, and water are heated to the inlet temperature of the SOFC stack. The preheated fuel and the steam generated in the preheater PH-3 are mixed in the mixer to form a gas mixture, and the mixed gas enters the anode of the SOFC to undergo internal reforming and steam displacement reactions, producing hydrogen required for the electrochemical reaction. The preheated air enters the cathode of the SOFC, where the oxygen diffuses to participate in the electrochemical reaction, directly converting the chemical energy of the fuel into electrical energy, and the direct current (DC) generated by the electrochemical reaction is converted into alternating current (AC) by the inverter for external power supply. The residual fuel (natural gas, hydrogen, and carbon monoxide) at the outlet of the SOFC anode and the residual oxygen at the outlet of the cathode undergo a full combustion reaction in the combustion chamber, and the high-temperature and high-pressure gas produced expands in the gas turbine to do work, driving the generator to generate electricity. The exhaust gas from the gas turbine enters the preheaters PH-1, PH-2, and PH-3 in sequence to preheat the air, biogas, and water.
The brackish water distillation subsystem is composed of a heat recovery steam generator and a multi-effect distillation unit with thermal vapor compression (MED-TVC). The MED-TVC primarily includes a thermal vapor compressor, evaporators, a condenser, and flash tanks. The working principle is as follows: the flue gas from the SOFC/GT hybrid power generation subsystem enters the heat recovery steam generator (HRSG) to heat water into saturated steam required for MED-TVC. The generated steam, serving as the motive steam, enters the thermal vapor compressor to entrain some low-temperature and low-pressure steam produced by the last effect evaporator. The mixture then enters the first effect evaporator to heat the sprayed brackish water. The steam inside the tube is condensed into distilled water and enters the flash tank. The small amount of steam flashed out in the flash tank and the secondary steam generated by the evaporation of brackish water enter the next effect evaporator to heat the sprayed brackish water. This process continues until some of the secondary steam produced by the last effect evaporator enters the thermal vapor compressor as the entrained steam. The remaining secondary steam from the last effect evaporator enters the condenser and is condensed into distilled water by the brackish water. A portion of the brackish water entering the condenser is used solely for cooling, while the remainder is sprayed onto each effect evaporator as raw brackish water. The concentrated brackish water produced by the previous effect evaporator enters the next effect evaporator for further evaporation, and ultimately leaves the last effect evaporator in the form of a concentrated liquid. Some of the distilled water produced using MED-TVC enters the heat recovery steam generator and is heated into motive steam, while the rest is pumped into the product water tank to supply fresh water externally.
The absorption refrigeration subsystem primarily comprises an evaporator, an absorber, a generator, a condenser, a solution heat exchanger, a solution pump, and two throttle valves. Its working principle is as follows: the flue gas from the HRSG enters the generator to heat the lithium bromide solution, generating steam, while the lithium bromide solution becomes concentrated. After cooling in the solution heat exchanger and throttling through throttle valve 1, the concentrated lithium bromide solution enters the absorber. The steam generated in the generator condenses into water within the condenser. After throttling through throttle valve 2, it enters the evaporator to absorb heat from the return water of the air conditioning system. Then, it evaporates into steam under low pressure, while the air conditioning return water cools down and becomes air conditioning supply water for external cooling. The steam generated in the evaporator enters the absorber and is absorbed by the lithium bromide solution, which then becomes a dilute solution. After heating in the solution heat exchanger and pressurization by the solution pump, it enters the generator. The cooling water flows through the absorber and the condenser in sequence, carrying away the heat released during the solution dilution process and the steam condensation process.
The heating subsystem consists of heat exchangers, among other components. Its working principle is as follows: backwater enters the heat exchanger H-1 and is heated by the flue gas from the absorption refrigeration subsystem, providing domestic hot water externally.
3. Mathematical Models and Assumptions
An analysis of the poly-generation system proposed in this paper is conducted from the perspectives of energy, exergy, and economics. In the subsequent sections, the energy balance equations, exergy balance equations, and economic models of the system components are established.
In order to facilitate the calculation and analysis of the thermal performance of the novel poly-generation system proposed in this paper, the following assumptions are made:
The working fluid in the poly-generation system is in a steady flow state, and all chemical reactions are in equilibrium [
18].
The composition of air includes nitrogen with a volume fraction of 79% and oxygen with a volume fraction of 21%.
The composition of biogas is a mixture of 60% CH
4 and 40% CO
2 [
19].
The electrochemical oxidation reaction of CO is not considered; only the H
2 generated by the reforming reaction and displacement reaction is considered to participate in the electrochemical oxidation reaction [
20,
21,
22].
The gases at the outlets of both the cathode and anode in the SOFC have identical pressure and temperature parameters [
23], and the SOFC is in a stable working state.
The thermal radiation between the working fluid and the solid structure inside the system is ignored.
The multi-effect distillation unit is in a stable working state.
The temperature difference between adjacent evaporators in the multi-effect distillation device is the same.
The ambient temperature is 303.15 K.
3.1. Mass and Energy Conversion Modeling
3.1.1. Solid Oxide Fuel Cell
In this study, an internal reforming solid oxide fuel cell (IR-SOFC) fueled using biogas is considered. In the SOFC, CH4 and CO undergo reforming reactions and water gas shift reactions with H2O, respectively. O2 gains electrons at the cathode to become O2−, which diffuses to the interface of the electrolyte and anode to undergo an electrochemical reaction with H2.
Water gas shift reaction:
Electrochemical reaction:
The equilibrium constant of the reforming reaction is given by:
where
Kpr is the equilibrium constant of the reforming reaction,
p represents the partial pressures of each gaseous component, and
p0 is the standard atmospheric pressure. m represents the molar flow rates of each component.
The equilibrium constant of the water gas shift reaction is given by:
where
Kps is the equilibrium constant of the water gas shift reaction.
Kps and
Kpr can be calculated using the following empirical formula [
24]:
where
T is the operating temperature of the SOFC, and
Kp is the equilibrium constant of the reforming reaction or water gas shift reaction. When calculating the equilibrium constant of reforming and water gas shift reactions, the coefficients
A,
B,
C,
D, and
E should be selected accordingly [
25,
26].
The steam to carbon ratio (SCR) is the ratio of the molar flow rates of H
2O to CH
4 at the inlet of the SOFC anode, given by:
The relationship between the electrochemical reaction and the water gas shift and reforming reactions can be expressed as:
where
Uf is the fuel utilization rate of the SOFC, and
x,
y,
z are the reaction rates of the reforming, water gas shift, and electrochemical reactions, respectively.
The current density of the SOFC is defined as:
where
ne is the number of electrons transferred in the electrochemical reaction (which is 2 in this case),
F is Faraday’s constant,
Nd is the number of cells, and
S is the reaction area of a single cell.
The power output of the SOFC is given by:
where
V is the actual output voltage of a single cell, and
ηInv is the efficiency of the inverter.
The actual output voltage of a single cell is equal to its reversible electromotive force minus the voltage losses caused by various irreversible processes:
where
Vr is the reversible electromotive force of the SOFC, and
Vact,
Vconc, and
Vohm are the activation polarization, concentration polarization, and ohmic loss, respectively.
The energy conservation equation of the SOFC is given by:
where
m represents the flow rates of the substances,
h represents the enthalpy, LHV represents the lower heating value, and the superscripts in and out represent the states at the inlet and outlet of the SOFC, respectively.
EnSOFC is the energy loss of the SOFC.
3.1.2. SOFC/GT Hybrid Power Generation Subsystem
The energy conservation equation of the combustion chamber is given by:
where the subscripts in and out represent the states at the inlet and outlet of the combustion chamber, respectively.
Enloss is the heat loss of the combustion chamber.
The high-temperature flue gas produced in the combustion chamber enters the gas turbine to do work, and its energy conservation equation is as follows:
where the subscripts in and out represent the states at the inlet and outlet of the gas turbine, respectively.
WT is the output power of the gas turbine.
The relative internal efficiency of the gas turbine is defined as:
where the subscript s represents the state of the fluid at the outlet of the turbine during adiabatic reversible expansion.
The isentropic efficiency of the compressor and water pump is defined as:
where the subscripts in and out represent the states of the fluid at the inlet and outlet of the compressor or water pump, respectively, and the subscript
s represents the state of the fluid at the outlet of the compressor or water pump during adiabatic reversible compression.
The power consumed by the compressor and water pump is given by:
where
hin and
hout represent the enthalpy values at the inlet and outlet of the compressor or water pump, respectively.
The net power output of the SOFC/GT hybrid power generation cycle is defined as:
where
ηele is the efficiency of the generator,
Wnet,SOFC/GT is the net power output of the SOFC/GT hybrid power cycle, and
WCP-1,
WCP-2, and
WP are the power consumed by the air compressor, biogas compressor, and water pump, respectively.
3.1.3. Multi-Effect Distillation Unit
The mathematical model of the thermal vapor compressor can be expressed as [
27]:
where the subscripts m, ev, and s represent the motive steam, ejecting steam, and mixed steam, respectively.
ER,
CR, and
Ra represent the expansion ratio, compression ratio, and entrainment ratio, respectively.
m,
h, and
P represent the mass flow rate, enthalpy, and the pressure of the steam, respectively.
The amount of secondary steam produced by each effect evaporator is given by [
28,
29,
30,
31]:
where
λs is the latent heat of condensation of the mixed steam,
Tf is the temperature of the raw brackish water,
Cp is the specific heat capacity at a constant pressure of water, and the subscripts 1~4 represent the effect number.
D1 is the amount of steam produced by the first effect evaporator.
T1 is the temperature of the concentrated brackish water in the first effect unit, and
λ1 is the latent heat of evaporation of water in the first effect unit.
d1′ is the amount of steam flashed in flash tank 1, and
λ1′ is its corresponding latent heat;
d2 is the amount of steam flashed when the concentrated brackish water from the first effect evaporator enters the second effect evaporator, and
λ2 is its corresponding latent heat.
F1 is the flow rate of the brackish water sprayed in the first effect evaporator, and
B1 is the amount of concentrated brackish water produced by the first effect evaporator.
The gained output ratio is defined as:
where
mW,product is the flow rate of the product freshwater, and
mm is the flow rate of the motive steam.
3.1.4. Lithium Bromide Absorption Refrigeration Unit
The components of the absorption refrigeration subsystem satisfy the mass balance equation for lithium bromide:
where
mj,in and
mk,out represent the flow rate of the lithium bromide solution at the inlet and outlet of a component of the lithium bromide refrigeration cycle, respectively;
Xj,in and
Xk,out represent the concentration of the lithium bromide solution at the inlet and outlet of a component, respectively.
The energy balance equation is given by:
where
Qkj represents the heat absorbed by the lithium bromide solution in a component;
hj,in and
hk,out represent the enthalpy of the lithium bromide solution at the inlet and outlet of a component, respectively.
3.2. Combined Cooling, Heating, and Power
The net power output of the poly-generation system is given by:
where
WP,MED is the power consumed by the pump in the multi-effect distillation subsystem, and
WP,LiBr is the power consumed by the pump in the absorption refrigeration subsystem.
The cooling capacity of the system is given by:
where
mac is the flow rate of the air conditioning supply water,
hR,ac is the enthalpy of the air conditioning return water, and
hS,ac is the enthalpy of the air conditioning supply water.
The heating capacity of the system is given by:
where
mh is the flow rate of the heating water,
hH is the enthalpy of the domestic hot water, and
h0 is the enthalpy of water at the environmental state.
3.3. Exergy Analysis
The exergy at each node of the poly-generation system can be calculated using the following equations:
where the subscript ph refers to physical exergy, ch represents chemical exergy, k denotes the state at each node, and 0 stands for the environmental state;
xi is the volume fraction of the
i-th component fluid;
ei is the specific chemical exergy of the
i-th component fluid; and s represents entropy.
The exergy balance functions for main equipment in the poly-generation system are detailed in
Table 1. In the exergy balance equation, the subscript
des represents exergy loss.
3.4. Economic Analysis
Economic analysis is essential in assessing the performance and reliability of the proposed poly-generation system. In this study, the total expenses (cost per hour) associated with the poly-generation system is given as:
where
fuel represents the fuel cost rate;
cfuel denotes the price of biogas, which is set at 7.36 USD/GJ [
19];
total stands for the total cost rate, while
Zk refers to the investment cost of the
k-th component; the symbol
φ is used to represent the operating and maintenance factor, which is assumed to be 0.06 in this study;
τ indicates the annual system operation hours, which is set to 8000 h; and CRF is the capital recovery factor, defined as follows:
where
N denotes the lifetime of the poly-generation system, which is assumed to be 20 years; the interest rate,
r, is set at 6%.
In the proposed poly-generation system, the SOFC stack is designed with a lifespan of 40,000 h. Throughout the lifecycle of the system, a complete replacement of the SOFC stack is needed three times.
The estimation formulas for the investment costs of the various components of the poly-generation system are shown in
Table 2.
3.5. Performance Evaluation Indicators
This paper selects the net power generation efficiency, comprehensive energy utilization rate, exergy efficiency, total energy output, freshwater production, and the total cost per hour as the performance evaluation indicators for the proposed poly-generation system.
The net power generation efficiency of the poly-generation system is given by:
where
mFuel is the flow rate of biogas, and LHV
Fuel is the lower heating value of biogas.
The total energy output of the poly-generation system is expressed as:
The comprehensive energy utilization rate of the proposed system is defined as:
The exergy efficiency of the whole system is given by:
where
Ein,total is defined as the total exergy entering the system, and
Edes,total is used to denote the total exergy loss within the system.
The models for the poly-generation system that are listed above are deemed suitable only for the study of parameter sensitivity analysis and multi-objective optimization under stable operating conditions. Performance analysis under dynamic conditions is not considered applicable to these models.
4. Results and Discussion
The poly-generation system is simulated using the Engineering Equation Solver (EES) software package V9.446. Furthermore, the multi-objective optimization of this poly-generation system is performed through a co-simulation process involving both EES and MATLAB.
4.1. Model Verification
The SOFC/GT hybrid power generation model employed in this study has been validated in previous research [
6]. To verify the accuracy of the thermal compression multi-effect distillation subsystem model, this study conducted simulation calculations using input parameters from a distillation factory. A comparison between the simulation results and the experimental data is presented in
Table 3. The results obtained align well with the experimental data, thereby validating the accuracy of the model used in this study. This validation underscores the reliability of the findings and discussions presented in this paper, and provides a solid foundation for the subsequent analysis and discussions.
4.2. Simulation Results under the Designed Condition
This study conducted a simulation calculation on the performance of the poly-generation system under the designed condition using the input parameters shown in
Table 4.
The simulation results of this study show that under the input parameters given in
Table 4, the net power output of the innovative poly-generation system is 248.15 kW, the cooling capacity is 21.45 kW, the heating capacity for domestic hot water is 6.87 kW, and the freshwater production is 469.93 kg/h. The system’s net power generation efficiency stands at 61.95%, the comprehensive energy utilization rate is 69.02%, the exergy efficiency is 35.56%, and the cost rate of the entire system is 22.51 USD/h. Compared to the combined cooling, heating, and power (CCHP) system based on solid oxide fuel cell/gas turbine (SOFC/GT) [
6], a noticeable reduction in efficiency is observed in the system presented in this paper due to the incorporation of a multi-effect distillation subsystem. Nevertheless, a substantial amount of fresh water can be produced by this system.
The exergy losses of each component are shown in
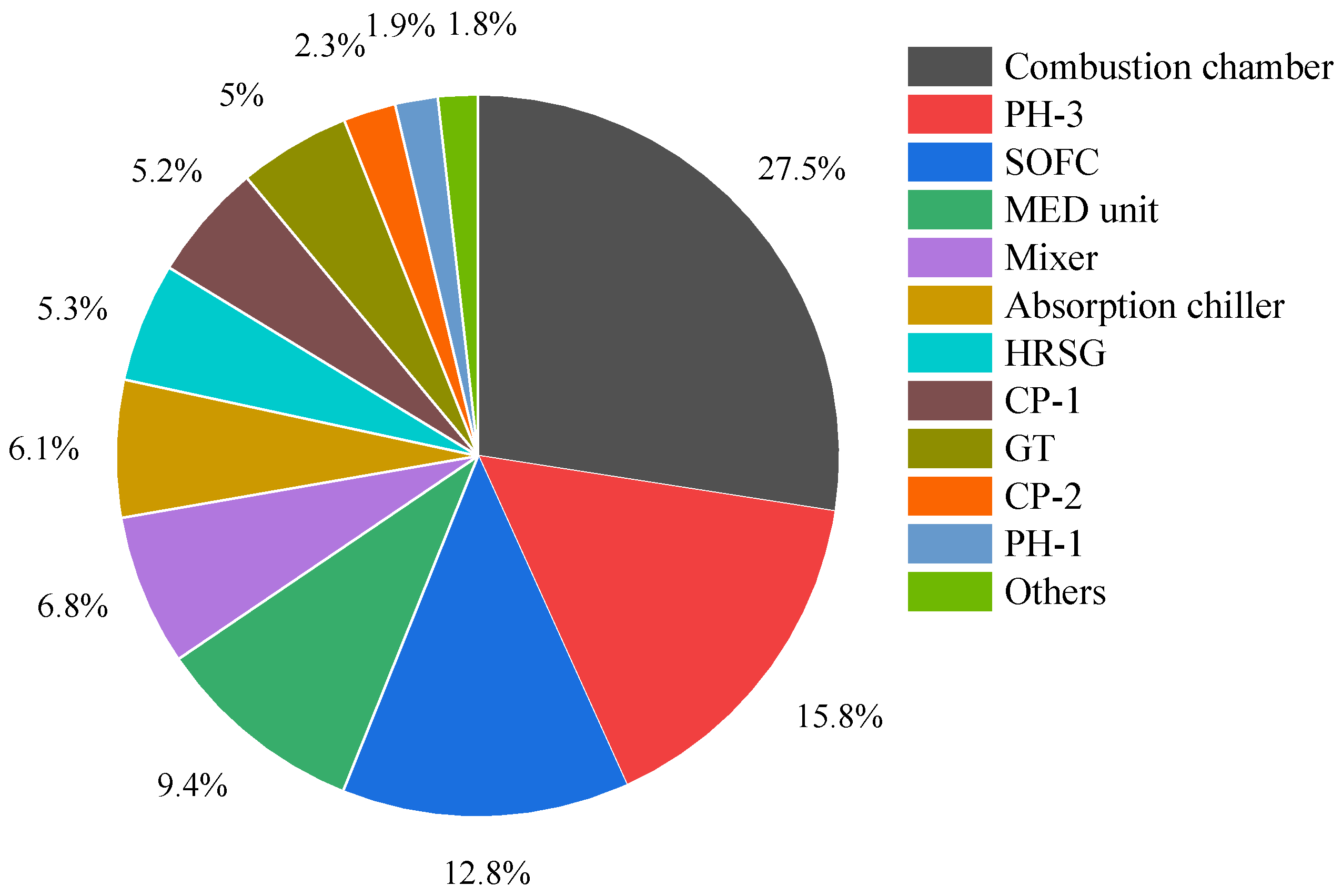
Figure 2. The combustion chamber, preheater PH-3, SOFC, and MED unit have the highest exergy losses. Approximately 27.5% of the total exergy loss in the system occurs in the combustion chamber, mainly due to chemical reactions. The exergy loss of the preheater PH-3 accounts for 15.8% of the total exergy loss in the system. Due to the irreversibility of the chemical reactions and the current conversion process, the SOFC’s loss accounts for 11.03% of the total system loss.
4.3. Parametric Analysis
The performance of the poly-generation system is closely related to the SOFC/GT hybrid power generation subsystem and the thermal compression multi-effect distillation subsystem. This study investigates parameters such as the operating pressure of the SOFC, the air flow rate, the fuel utilization rate of the SOFC, and the compression ratio of the thermal vapor compressor. The impact of variations in these parameters within a reasonable range on the performance of the poly-generation system is analyzed.
4.3.1. Effect of SOFC Pressure
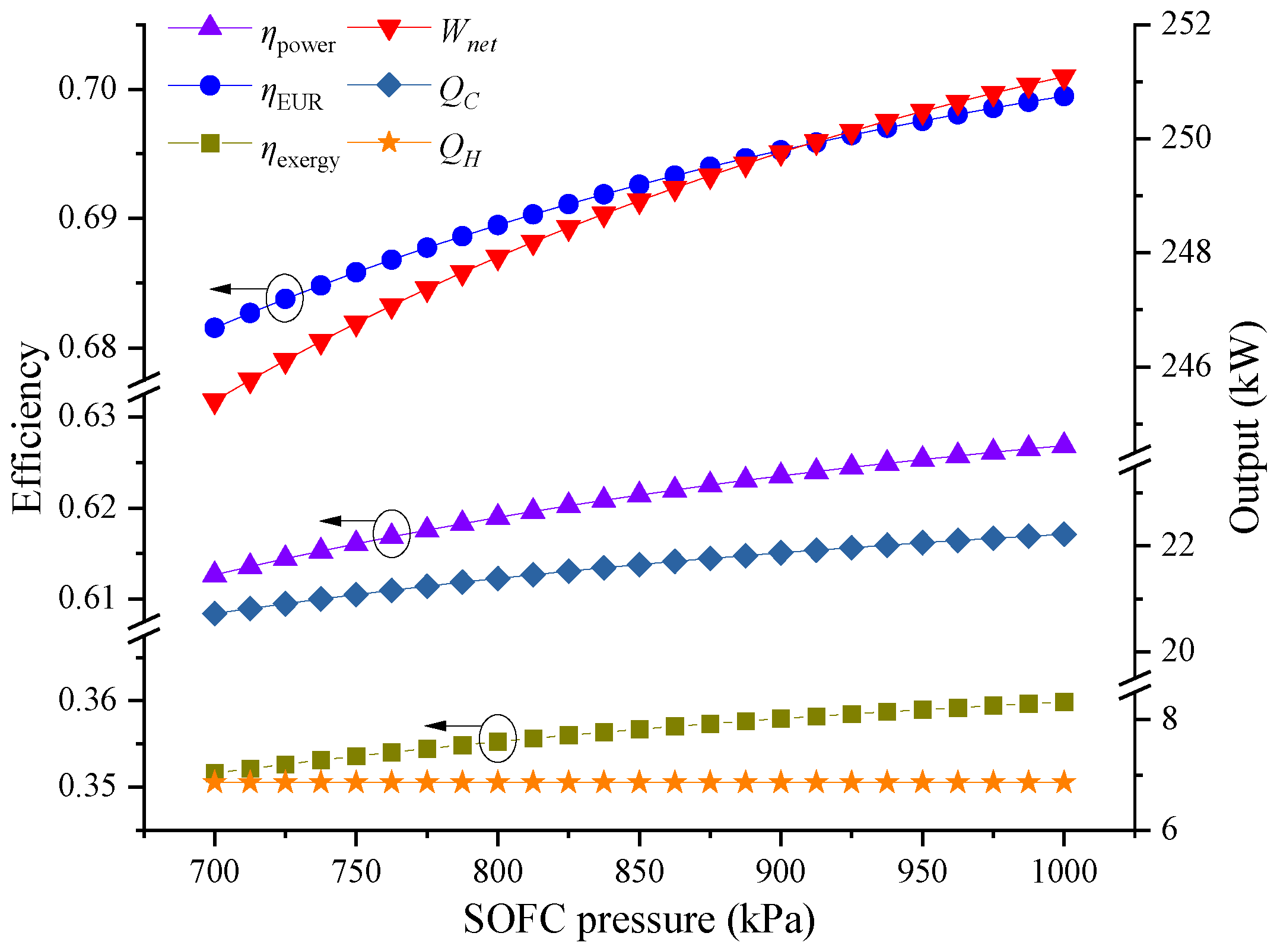
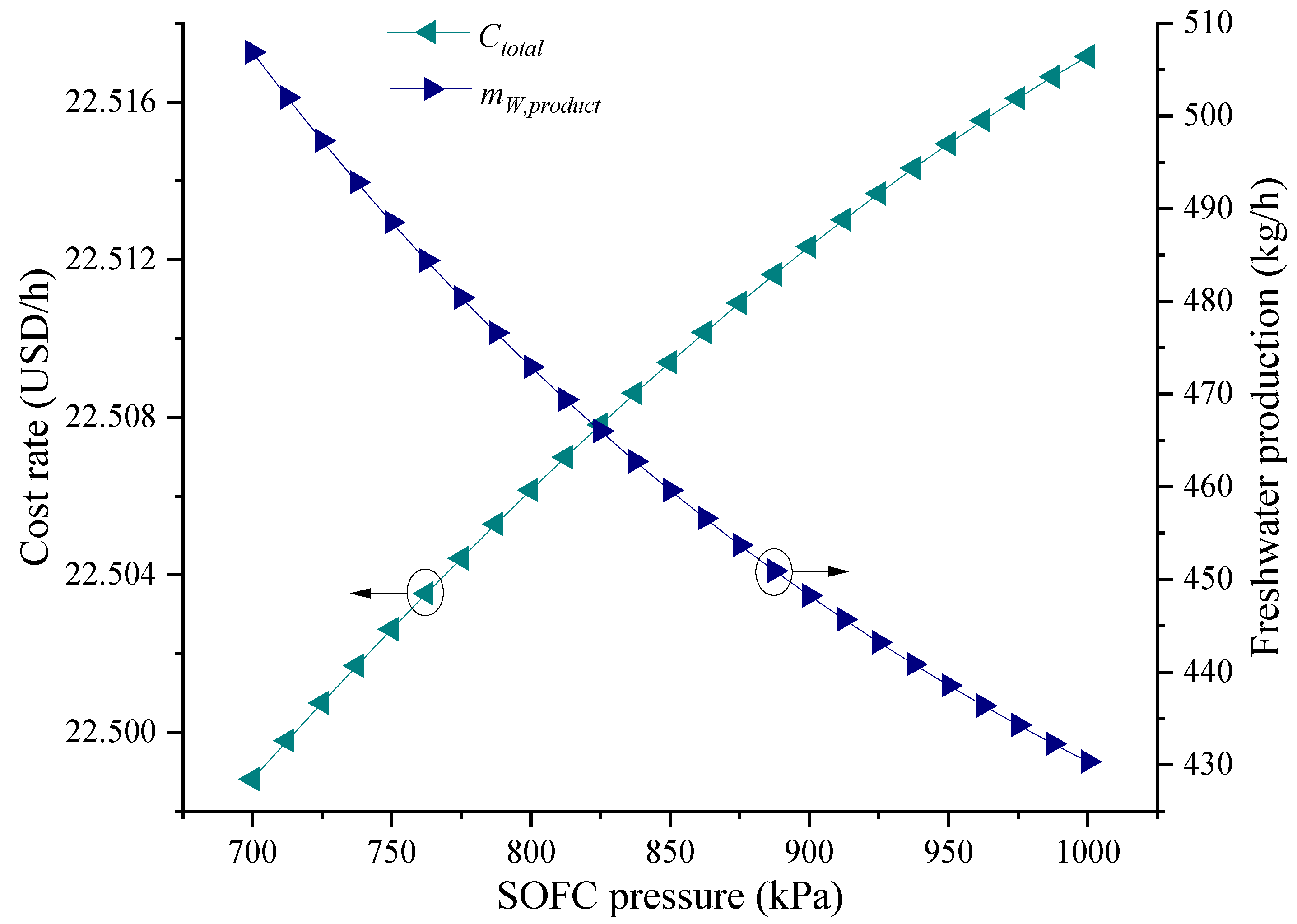
The effects of variations in the operating pressure of the SOFC within a reasonable range on net power output, cooling capacity, heating capacity, net power efficiency, comprehensive energy utilization rate, exergy efficiency, cost rate, and freshwater production are shown in
Figure 3 and
Figure 4.
Increasing the operating pressure of the SOFC leads to an increase in the output voltage of the SOFC, and thus the output power of the SOFC also increases. The increase in the exhaust pressure of the SOFC will also lead to an increase in the output power of the gas turbine. Although increasing the operating pressure of the SOFC will lead to an increase in the power consumption of the compressor and water pump, compared with the power increase of the SOFC and GT, its increase is relatively small. Therefore, the net power output and net power efficiency of the system both increase.
Increasing the operating pressure of the SOFC will cause the exhaust temperature of the SOFC/GT hybrid power generation subsystem to decrease, and the amount of motive steam in the multi-effect distillation subsystem will decrease accordingly, so the freshwater production decreases. Due to the limitation of the condensation water temperature at the inlet of the heat compression steam generator and the pinch temperature difference, the flue gas temperature at the inlet of the absorption refrigeration subsystem increases slightly. Therefore, the cooling capacity of the system increases slightly. Since the heating capacity remains basically unchanged, the net power output and cooling capacity of the system both increase, so the comprehensive energy utilization rate and exergy efficiency of the system increase. When the operating pressure of the SOFC increases, the system’s cost rate slightly increases. The primary reason for this is the increase in the investment cost of the gas turbine due to the increase in its power output.
4.3.2. Effect of Air Flow Rate
The effects of the air flow rate on net power output, cooling capacity, heating capacity, net power efficiency, comprehensive energy utilization rate, exergy efficiency, cost rate, and freshwater production are shown in
Figure 5 and
Figure 6.
Increasing the air flow rate will cause the operating temperature of the SOFC to decrease, which leads to a decrease in the output power of the SOFC. Although an increase in flue gas volume will increase the output power of the gas turbine, the power consumption of the air compressor increases dramatically. Therefore, the net power output and net power efficiency of the system decrease. As the flue gas temperature at the inlet of the heat compression steam generator decreases, the amount of motive steam in the multi-effect distillation subsystem decreases accordingly, so the freshwater production decreases.
Similarly, due to the limitation of the condensation water temperature at the inlet of the heat compression steam generator and the pinch temperature difference, the flue gas temperature at the inlet of the absorption refrigeration subsystem increases. In addition, the flue gas volume also increases, so the cooling capacity and heating capacity of the system also increase. Since the total energy output of the system slightly increases, the comprehensive energy utilization rate increases with the increase in air flow rate. Although the total energy output slightly increases, compared with the decreased power generation, the increased cooling capacity is of low grade, and the converted electricity saving is relatively small compared to the decreased power generation. Therefore, the exergy efficiency of the system decreases with the increase in air flow rate. The increase in the air flow rate causes a decrease in the operating temperature of the SOFC, which leads to a reduction in the investment cost of the SOFC. This results in a decrease in the system’s cost rate.
4.3.3. Effect of Fuel Utilization Rate
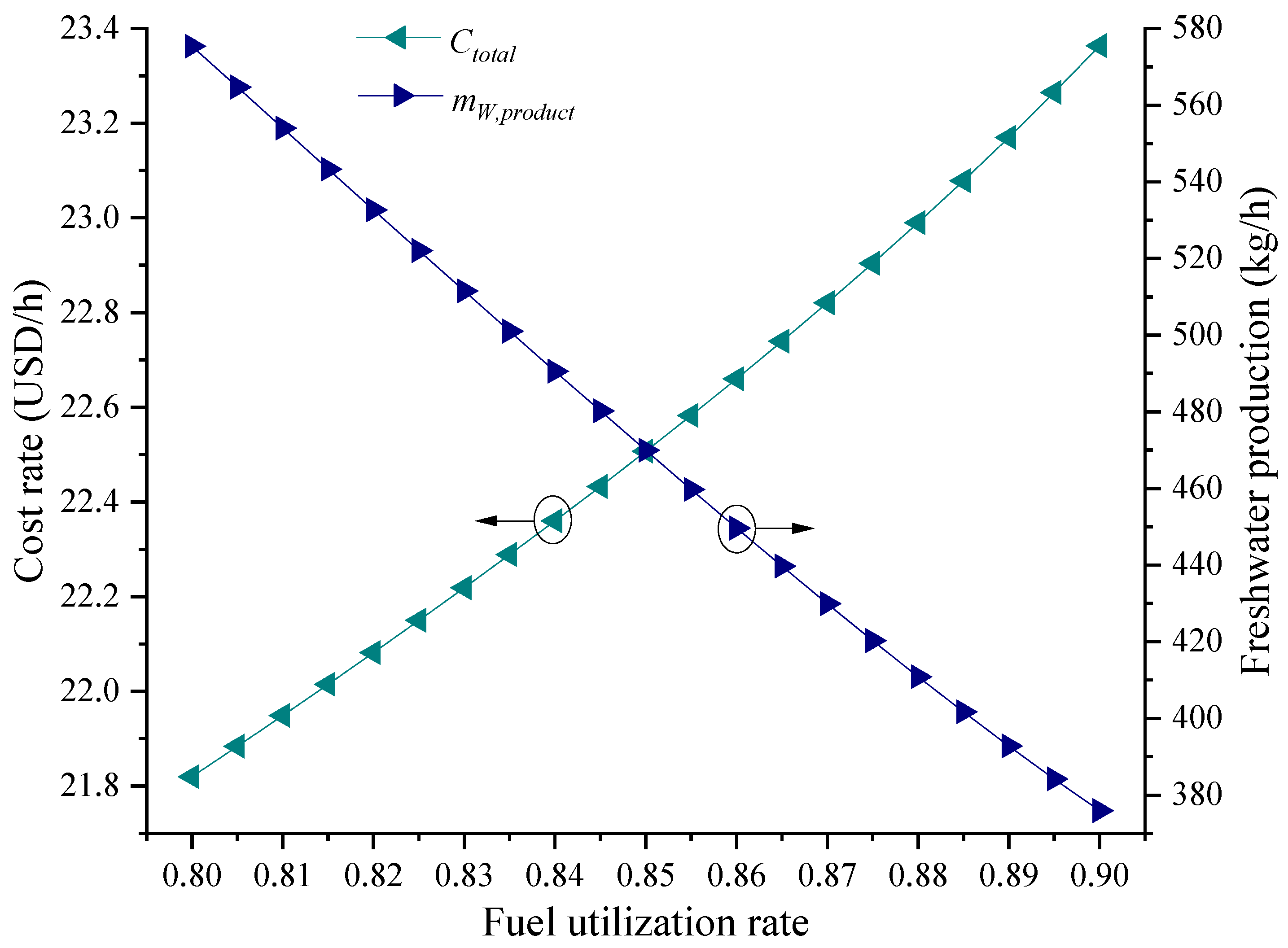
The effects of variations in the fuel utilization rate of the SOFC within a reasonable range on net power output, cooling capacity, heating capacity, net power efficiency, comprehensive energy utilization rate, exergy efficiency, cost rate, and freshwater production are shown in
Figure 7 and
Figure 8.
With the increase in fuel utilization, the amount of H2 participating in electrochemical reactions increases, leading to an increase in current density and a rise in the outlet temperature of the SOFC. This results in a decrease in the reversible cell voltage and a reduction in the output cell voltage. The impact of the increased current density on the power generation of the SOFC is greater than the effect of the reduced output cell voltage, hence the power generation of the SOFC increases.
Due to the improved fuel utilization, the combustion chamber gas temperature decreases, reducing the power generation of the gas turbine. However, the decrease in power generation from the gas turbine is less than the increase from the SOFC, resulting in an increase in net power generation efficiency. At the same time, the heat supply and cooling capacity remain essentially unchanged, the net power generation increases, and the energy comprehensive utilization efficiency and exergy efficiency improve. The decrease in flue gas temperature leads to a decline in freshwater production, while the increase in the operating temperature of the SOFC results in an increase in the cost rate.
4.3.4. Effect of the Compression Ratio of the Thermal Vapor Compressor
Figure 9 illustrates the impact of the thermal vapor compressor’s compression ratio on the cost rate and freshwater production. Given that the pressure of the power steam remains constant, an increase in the thermal vapor compressor’s compression ratio leads to a decrease in the pressure of the entrained steam. This, in turn, results in a reduction in the flow rate of the entrained steam, and subsequently, the amount of steam entering the first-effect evaporator decreases. This causes the secondary steam produced by each evaporator to decrease. Consequently, the freshwater production of the poly-generation system decreases as the thermal vapor compressor’s compression ratio increases. As the compression ratio of the thermal vapor compressor increases, the required heat exchange area for the evaporators decreases. This results in a slight reduction in the cost rate.
4.4. Multi-Objective Optimization
To enhance the comprehensive energy utilization rate, exergy efficiency, total energy output, and freshwater production of the poly-generation system while reducing the cost rate, it is necessary to perform multi-objective optimization on this nonlinear co-generation system. Multi-objective optimization problems are widely present in many complex engineering practical systems, where each objective conflicts with and restricts another, making it impossible to achieve optimality simultaneously, hence there exists more than one solution. Genetic algorithms have loose requirements for objective functions and possess robustness, making them suitable for solving complex multi-objective optimization problems in nonlinear systems and finding Pareto solution sets. The multi-objective optimization research on the poly-generation system is conducted using NSGA-II.
Two optimization schemes to solve the multi-objective optimization problem of the poly-generation system are proposed in this study. Scheme 1 aims to maximize the energy utilization rate and exergy efficiency, and minimize the cost rate. Scheme 2 aims to maximize the total energy output and freshwater production, and minimize the cost rate. The decision variables are the SOFC pressure (
PSOFC), air flow rate (
m8), fuel utilization rate (
Uf), and the compression ratio of the thermal vapor compressor (
CR). The range of values for the decision variables and the input parameters for the optimization program are listed in
Table 5.
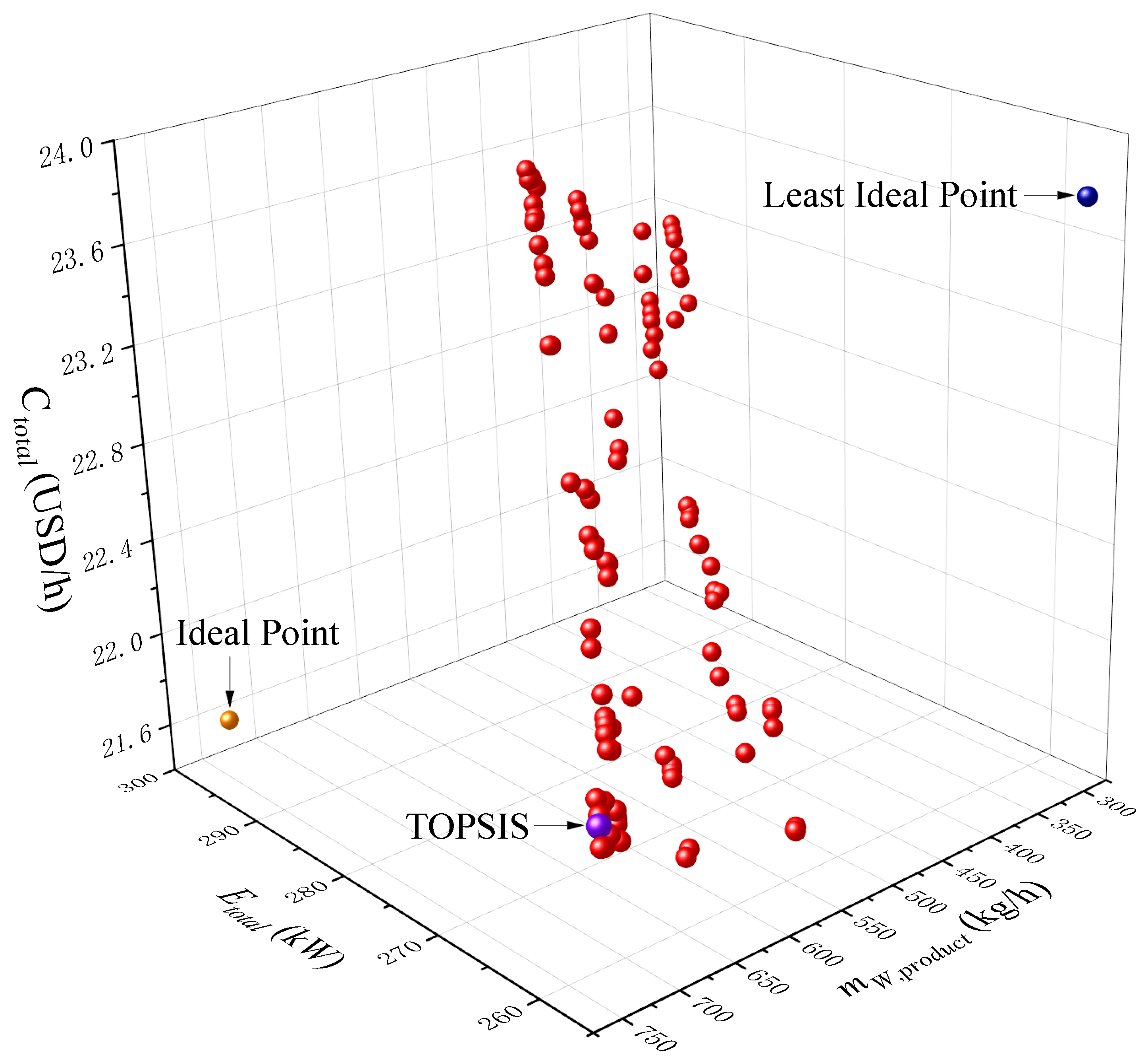
The set of objective function vectors corresponding to the Pareto solution set is called the Pareto frontier, which represents the objective values of all optimal solutions under different weight allocations.
The Pareto frontier obtained from Scheme 1 is shown in
Figure 10.
The three objectives in this study are given equal importance, each having a global weight of 1/3. The decision-making process in this study is conducted using the TOPSIS method. The normalization of the data and the calculation of the Euclidean distance between each point and the ideal point are involved in this process. The optimal solution, denoted as TOPSIS in
Figure 10, provides the best results for all three objectives.
By setting the parameters PSOFC, m8, Uf, and CR to 998.78 kPa, 6.5 mol/s, 89.82%, and 2.81, respectively, the most favorable results for all three objectives are obtained. These results include a comprehensive energy utilization rate of 73.60%, an exergy efficiency of 37.58%, and a cost rate of 23.14 USD/h. Compared to the designed condition, an increase of 4.58% in the comprehensive energy utilization rate and 2.02% in the exergy efficiency are achieved after the optimization of Scheme 1, while the cost rate of the entire system is increased by 0.63 USD/h.
The Pareto frontier obtained from Scheme 2 is shown in
Figure 11.
In Scheme 2, each objective is assigned a weight of 1/3. The optimal solution, referred to as TOPSIS in
Figure 11, yields the most favorable outcomes for all three objectives. By adjusting the parameters
PSOFC,
m8,
Uf, and
CR to 700 kPa, 5.5 mol/s, 80.31%, and 2.0, respectively, the best results for all three objectives are achieved. These include a total energy output of 256.96 kW, a freshwater production rate of 746.31 kg/h, and a cost rate of 22.09 USD/h. In comparison to the designed condition, a reduction of 19.51 kW in the total energy output, an increase of 276.38 kg/h in the freshwater production rate, and a decrease of 0.42 USD/h in the cost rate of the entire system are observed after the optimization of Scheme 2.
5. Conclusions
A novel poly-generation system is proposed, which includes an SOFC/GT hybrid power generation subsystem, a brackish water distillation subsystem, an absorption refrigeration subsystem, and a heating supply subsystem. The performance of this system is investigated using energy, exergy, and economic methods. The NSGA-II and TOPSIS methods are employed to identify the optimal design parameters. The key findings of this study are summarized below:
- (1)
The innovative poly-generation system is indicated by the simulation results of the study under the designed condition to be capable of reaching a net power output of 248.15 kW, a cooling capacity of 21.45 kW, a heating capacity for domestic hot water of 6.87 kW, and a freshwater production of 469.93 kg/h. The efficiency of the system is shown to be 61.95% for net power generation, 69.02% for comprehensive energy utilization rate, and 35.56% for exergy. The cost rate of the whole system is demonstrated to be 22.51 USD/h. Exergy losses occur mainly in the combustion chamber (27.5% of total), preheater PH-3 (15.8% of total), SOFC (11.03% of total), and MED unit. The high exergy loss in the combustion chamber is primarily due to chemical reactions.
- (2)
The net power efficiency, comprehensive energy utilization rate, and exergy efficiency are enhanced by increasing the SOFC pressure or fuel utilization rate. However, the increase in SOFC pressure or fuel utilization rate results in a reduction in freshwater production and an increase in the cost rate.
- (3)
When the air flow rate increases, the comprehensive energy utilization rate also increases. However, the net power efficiency and exergy efficiency decrease. When the air flow rate or the TVC’s compression ratio increase, the cost rate and freshwater production decrease.
- (4)
The objective of Scheme 1 is to optimize energy utilization and exergy efficiency while minimizing cost. This is achieved by setting the parameters PSOFC, m8, Uf, and CR to 998.78 kPa, 6.5 mol/s, 89.82%, and 2.81, respectively. The optimal results include a comprehensive energy utilization rate of 73.60%, an exergy efficiency of 37.58%, and a cost rate of 23.14 USD/h. In comparison to the initial design, the optimization of Scheme 1 results in a 4.58% increase in the comprehensive energy utilization rate and a 2.02% increase in the exergy efficiency. However, the cost rate of the entire system increases by 0.63 USD/h.
- (5)
Scheme 2 is designed to maximize total energy output and freshwater production, and minimize cost. The parameters PSOFC, m8, Uf, and CR are adjusted to 700 kPa, 5.5 mol/s, 80.31%, and 2.0, respectively, to achieve the best results. These results include a total energy output of 256.96 kW, a freshwater production rate of 746.31 kg/h, and a cost rate of 22.09 USD/h. Compared to the initial design, the optimization of Scheme 2 results in a decrease of 19.51 kW in the total energy output, an increase of 276.38 kg/h in the freshwater production rate, and a decrease of USD0.42/h in the cost rate of the entire system.
Author Contributions
Conceptualization, Y.L. and C.T.; methodology, Y.L.; software, Y.L.; validation, Y.L., X.Y. and H.L.; formal analysis, Y.L.; investigation, Y.L.; resources, Y.L.; data curation, Y.L.; writing—original draft preparation, Y.L.; writing—review and editing, Y.L., X.Y., H.L. and C.T.; visualization, Y.L.; supervision, Y.L.; project administration, Y.L.; funding acquisition, Y.L. and C.T. All authors have read and agreed to the published version of the manuscript.
Funding
This research was funded by the Natural Science Foundation of Shandong Province, China (Grant No. ZR2021QE239), and the Doctoral Research Fund of Shandong Jianzhu University (Grant No. X21012Z).
Data Availability Statement
The data are available from the corresponding author upon reasonable request.
Acknowledgments
We appreciate the comments and suggestions from the editor and anonymous reviewers, which helped improve the paper. We would also like to express our gratitude to the Natural Science Foundation of Shandong Province, China, and Shandong Jianzhu University (Jinan, China) for providing funding support.
Conflicts of Interest
The authors declare no conflict of interest.
Nomenclature
| cost rate, USD/h |
| CP-1 | air compressor |
| CP-2 | biogas compressor |
| H-1 | heat exchanger |
| h | enthalpy, J/mol |
| m | molar flowrate, mol/s |
| N | lifetime of the system |
| P | pressure, kPa |
| P-1, P-2 | pumps |
| PH-1···PH-3 | preheaters |
| Q | heat flux, kW |
| r | interest rate |
| S | entropy, J/(mol·K) |
| T | temperature, K |
| W | power, kW |
| Z | purchased equipment cost, USD |
| capital cost rate, USD/hour |
| Subscripts |
| 0 | ambient conditions |
| 1, 2, …, 18 | thermodynamic state points |
| a, b, …, z | thermodynamic state points |
| A | heat transfer area |
| C | cooling |
| ch | chemical exergy |
| des | exergy destruction |
| E | exergy |
| En | energy |
| ele | electrical |
| Eva | evaporator |
| H | heating |
| ph | physical exergy |
| Inv | inverter |
| net | net power generation |
| T | turbine |
| s | isentropic |
| Greek symbols |
| φ | maintenance factor |
| ƞ | efficiency, % |
| Abbreviations |
| AC | alternating current |
| CC | combustion chamber |
| CCHP | combined cooling, heating, and power |
| DC | direct current |
| GT | gas turbine |
| SOFC | solid oxide fuel cell |
| SHX | solution heat exchanger |
| TOPSIS | Technique for Order of Preference by Similarity to Ideal Solution |
References
- Liu, Y.; Han, J.; You, H. Exergoeconomic analysis and multi-objective optimization of a CCHP system based on SOFC/GT and transcritical CO2 power/refrigeration cycles. Appl. Therm. Eng. 2023, 230, 120686. [Google Scholar] [CrossRef]
- Lyu, C.; Xie, Z.; Li, Z. Market supervision, innovation offsets and energy efficiency: Evidence from environmental pollution liability insurance in China. Energy Policy 2022, 171, 113267. [Google Scholar] [CrossRef]
- Jana, K.; Ray, A.; Majoumerd, M.M.; Assadi, M.; De, S. Polygeneration as a future sustainable energy solution—A comprehensive review. Appl. Energy 2017, 202, 88–111. [Google Scholar] [CrossRef]
- You, H.; Han, J.; Liu, Y.; Chen, D. Thermoeconomic assessment and multiobjective optimization of a CCHP and MED hybrid system based on IR-SOFC/MGT. Int. J. Energy Res. 2022, 46, 3155–3172. [Google Scholar] [CrossRef]
- Wang, X.; Mi, X.; Lv, X.; Weng, Y. Fast and stable operation approach of ship solid oxide fuel cell-gas turbine hybrid system under uncertain factors. Int. J. Hydrogen Energy 2022, 47, 21472–21491. [Google Scholar] [CrossRef]
- Liu, Y.; Han, J.; You, H. Performance analysis of a CCHP system based on SOFC/GT/CO2 cycle and ORC with LNG cold energy utilization. Int. J. Hydrogen Energy 2019, 44, 29700–29710. [Google Scholar] [CrossRef]
- Ma, S.; Wang, J.; Yan, Z.; Dai, Y.; Lu, B. Thermodynamic analysis of a new combined cooling, heat and power system driven by solid oxide fuel cell based on ammonia–water mixture. J. Power Sources 2011, 196, 8463–8471. [Google Scholar] [CrossRef]
- Zhao, H.; Jiang, T.; Hou, H. Performance analysis of the SOFC–CCHP system based on H2O/Li–Br absorption refrigeration cycle fueled by coke oven gas. Energy 2015, 91, 983–993. [Google Scholar] [CrossRef]
- Baggio, G.; Qadir, M.; Smakhtin, V. Freshwater availability status across countries for human and ecosystem needs. Sci. Total Environ. 2021, 792, 148230. [Google Scholar] [CrossRef]
- Zhang, P.; Shen, J. Effect of brackish water irrigation on the movement of water and salt in salinized soil. Open Geosci. 2022, 14, 404–413. [Google Scholar] [CrossRef]
- Du, J.R.; Zhang, X.; Feng, X.; Wu, Y.; Cheng, F.; Ali, M.E.A. Desalination of high salinity brackish water by an NF-RO hybrid system. Desalination 2020, 491, 114445. [Google Scholar] [CrossRef]
- Liu, C.; Cui, B.; Zeleke, K.T.; Hu, C.; Wu, H.; Cui, E.; Huang, P.; Gao, F. Risk of Secondary Soil Salinization under Mixed Irrigation Using Brackish Water and Reclaimed Water. Agronomy 2021, 11, 2039. [Google Scholar] [CrossRef]
- Mittal, A.; Brajpuriya, R.; Gupta, R. Solar steam generation using hybrid nanomaterials to address global environmental pollution and water shortage crisis. Mater. Today Sustain. 2023, 21, 100319. [Google Scholar] [CrossRef]
- Hai, T.; Alizadeh, A.A.; Ali, M.A.; Dhahad, H.A.; Goyal, V.; Mohammed Metwally, A.S.; Ullah, M. Machine learning-assisted tri-objective optimization inspired by grey wolf behavior of an enhanced SOFC-based system for power and freshwater production. Int. J. Hydrogen Energy 2023, 48, 25869–25883. [Google Scholar] [CrossRef]
- Bahari, M.; Entezari, A.; Esmaeilion, F.; Ahmadi, A. Systematic analysis and multi-objective optimization of an integrated power and freshwater production cycle. Int. J. Hydrogen Energy 2022, 47, 18831–18856. [Google Scholar] [CrossRef]
- Al-Rashed, A.A.A.A.; Alsarraf, J.; Alnaqi, A.A. Exergy optimization of a novel hydrogen production plant with fuel cell, heat recovery, and MED using NSGAII genetic algorithm. Int. J. Hydrogen Energy 2022, 47, 26673–26686. [Google Scholar] [CrossRef]
- Hadavi, H.; Saifoddin, A.; Amirhaeri, Y.; Pourfayaz, F. Hybrid multi-objective optimization and thermo-economic analysis of a multi-effect desalination unit integrated with a fuel cell-based trigeneration system. J. Clean. Prod. 2023, 407, 137156. [Google Scholar] [CrossRef]
- Emadi, M.A.; Chitgar, N.; Oyewunmi, O.A.; Markides, C.N. Working-fluid selection and thermoeconomic optimisation of a combined cycle cogeneration dual-loop organic Rankine cycle (ORC) system for solid oxide fuel cell (SOFC) waste-heat recovery. Appl. Energy 2020, 261, 114384. [Google Scholar] [CrossRef]
- Zhang, M.; Chen, H.; Zoghi, M.; Habibi, H. Comparison between biogas and pure methane as the fuel of a polygeneration system including a regenerative gas turbine cycle and partial cooling supercritical CO2 Brayton cycle: 4E analysis and tri-objective optimization. Energy 2022, 257, 124695. [Google Scholar] [CrossRef]
- Chitgar, N.; Emadi, M.A.; Chitsaz, A.; Rosen, M.A. Investigation of a novel multigeneration system driven by a SOFC for electricity and fresh water production. Energy Convers. Manag. 2019, 196, 296–310. [Google Scholar] [CrossRef]
- Choudhary, T.; Sanjay. Thermodynamic assessment of advanced SOFC-blade cooled gas turbine hybrid cycle. Int. J. Hydrogen Energy 2017, 42, 10248–10263. [Google Scholar] [CrossRef]
- Mehrpooya, M.; Sadeghzadeh, M.; Rahimi, A.; Pouriman, M. Technical performance analysis of a combined cooling heating and power (CCHP) system based on solid oxide fuel cell (SOFC) technology—A building application. Energy Convers. Manag. 2019, 198, 111767. [Google Scholar] [CrossRef]
- Huang, Y.; Turan, A. Fuel sensitivity and parametric optimization of SOFC-GT hybrid system operational characteristics. Therm. Sci. Eng. Prog. 2019, 14, 100407. [Google Scholar] [CrossRef]
- Sánchez, D.; Chacartegui, R.; Muñoz, A.; Sánchez, T. Thermal and electrochemical model of internal reforming solid oxide fuel cells with tubular geometry. J. Power Sources 2006, 160, 1074–1087. [Google Scholar] [CrossRef]
- Njodzefon, J.C.; Klotz, D.; Kromp, A.; Weber, A.; Ivers-Tiffée, E. Electrochemical Modeling of the Current-Voltage Characteristics of an SOFC in Fuel Cell and Electrolyzer Operation Modes. J. Electrochem. Soc. 2013, 160, F313. [Google Scholar] [CrossRef]
- Akkaya, A.V. Electrochemical model for performance analysis of a tubular SOFC. Int. J. Energy Res. 2007, 31, 79–98. [Google Scholar] [CrossRef]
- Al-Mutaz, I.S.; Wazeer, I. Development of a steady-state mathematical model for MEE-TVC desalination plants. Desalination 2014, 351, 9–18. [Google Scholar] [CrossRef]
- Ahmadi, R.; Pourfatemi, S.M.; Ghaffari, S. Exergoeconomic optimization of hybrid system of GT, SOFC and MED implementing genetic algorithm. Desalination 2017, 411, 76–88. [Google Scholar] [CrossRef]
- Meratizaman, M.; Monadizadeh, S.; Amidpour, M. Introduction of an efficient small-scale freshwater-power generation cycle (SOFC–GT–MED), simulation, parametric study and economic assessment. Desalination 2014, 351, 43–58. [Google Scholar] [CrossRef]
- Ghofrani, I.; Moosavi, A. Brine elimination by hybridization of a novel brine-recycle bubble-column humidification-dehumidification system with a multiple-effect distillation system. Energy Convers. Manag. 2020, 217, 113004. [Google Scholar] [CrossRef]
- Mohammadnia, A.; Asadi, A. A hybrid solid oxide fuel cell-gas turbine fed by the motive steam of a multi-effects desalination-thermo vapor compressor system. Energy Convers. Manag. 2020, 216, 112951. [Google Scholar] [CrossRef]
- Hai, T.; Zoghi, M.; Habibi, H. Comparison between two LiBr–H2O absorption-compression chillers and a simple absorption chiller driven by various solar collectors: Exergy-economic performance and optimization. Energy 2023, 282, 128960. [Google Scholar] [CrossRef]
- Ali, E.; Orfi, J.; AlAnsary, H.; Soukane, S.; Elcik, H.; Alpatova, A.; Ghaffour, N. Cost analysis of multiple effect evaporation and membrane distillation hybrid desalination system. Desalination 2021, 517, 115258. [Google Scholar] [CrossRef]
Figure 1.
(a) The proposed poly-generation system and (b) the multi-effect distillation unit.
Figure 1.
(a) The proposed poly-generation system and (b) the multi-effect distillation unit.
Figure 2.
Exergy loss percentage of components in the proposed poly-generation system.
Figure 2.
Exergy loss percentage of components in the proposed poly-generation system.
Figure 3.
The impact of SOFC pressure on the output of cooling, heating, and power, net power efficiency, comprehensive energy utilization rate, and exergy efficiency.
Figure 3.
The impact of SOFC pressure on the output of cooling, heating, and power, net power efficiency, comprehensive energy utilization rate, and exergy efficiency.
Figure 4.
The impact of SOFC pressure on cost rate and freshwater production.
Figure 4.
The impact of SOFC pressure on cost rate and freshwater production.
Figure 5.
The impact of air flow rate on the output of cooling, heating, and power, net power efficiency, comprehensive energy utilization rate, and exergy efficiency.
Figure 5.
The impact of air flow rate on the output of cooling, heating, and power, net power efficiency, comprehensive energy utilization rate, and exergy efficiency.
Figure 6.
The impact of air flow rate on cost rate and freshwater production.
Figure 6.
The impact of air flow rate on cost rate and freshwater production.
Figure 7.
The impact of fuel utilization rate on the output of cooling, heating, and power, net power efficiency, comprehensive energy utilization rate, and exergy efficiency.
Figure 7.
The impact of fuel utilization rate on the output of cooling, heating, and power, net power efficiency, comprehensive energy utilization rate, and exergy efficiency.
Figure 8.
The impact of fuel utilization rate on cost rate and freshwater production.
Figure 8.
The impact of fuel utilization rate on cost rate and freshwater production.
Figure 9.
The impact of TVC’s compression ratio on cost rate and freshwater production.
Figure 9.
The impact of TVC’s compression ratio on cost rate and freshwater production.
Figure 10.
Pareto optimum frontier of the solutions in Scheme 1.
Figure 10.
Pareto optimum frontier of the solutions in Scheme 1.
Figure 11.
Pareto optimum frontier of the solutions in Scheme 2.
Figure 11.
Pareto optimum frontier of the solutions in Scheme 2.
Table 1.
Exergy balance functions of main equipment.
Table 1.
Exergy balance functions of main equipment.
| Equipment | Exergy Balance Equation |
|---|
| SOFC | |
| Inverter | |
| Mixer | |
| Combustion Chamber | |
| Gas Turbine | |
| CP-1 | |
| CP-2 | |
| P-1 | |
| PH-1 | |
| PH-2 | |
| PH-3 | |
| HRSG | |
| MED-TVC | |
| Absorption Chiller | |
| H-1 | |
Table 2.
Investment cost functions of each type of equipment [
31,
32,
33].
Table 2.
Investment cost functions of each type of equipment [
31,
32,
33].
| Equipment | Investment Cost Function |
|---|
| SOFC | |
| Inverter | |
| Mixer | |
| Combustion chamber | |
| Gas turbine | |
| CP-1 | |
| CP-2 | |
| P-1 | |
| P-2 | |
| PH-1 | |
| PH-2 | |
| PH-3 | |
| HRSG | |
| TVC | |
| Evaporator of MED | |
| Condenser of MED | |
| Generator | |
| Condenser | |
| Absorber | |
| Evaporator | |
| SHX | |
| H-1 | |
Table 3.
Validation of the thermal compression multi-effect distillation model.
Table 3.
Validation of the thermal compression multi-effect distillation model.
| Parameter | Literature Value [27] | Model Result (This Study) | Deviation (%) |
|---|
| Number of effects | 4 | 4 | - |
| Motive steam pressure (kPa) | 2300 | 2300 | - |
| First effect concentrate temperature (K) | 333.25 | 333.25 | - |
| Last effect concentrate temperature (K) | 318.55 | 318.55 | - |
| Temperature drop between adjacent effects (K) | 4.9 | 4.9 | - |
| Spray liquid temperature (K) | 314.65 | 314.65 | - |
| Condenser inlet feed temperature (K) | 304.65 | 304.65 | - |
| Motive steam flow rate (kg/s) | 8.8 | 8.8 | - |
| Entrainment ratio | 1.14 | 1.145 | 0.44 |
| Expansion ratio | - | 240.9 | - |
| Compression ratio | - | 2.657 | - |
| Freshwater production rate (kg/s) | 57.8 | 57.97 | 0.29 |
| Gained output ratio | 6.51 | 6.588 | 1.20 |
Table 4.
Input parameters for the simulation calculation of the poly-generation system.
Table 4.
Input parameters for the simulation calculation of the poly-generation system.
| Parameter | Value | Parameter | Value |
|---|
| Ambient temperature (K) | 303.15 | Inverter efficiency | 95% |
| Ambient pressure (kPa) | 101.325 | Steam/carbon ratio | 2 |
| Air flow rate (mol/s) | 6.12 | Fuel utilization rate | 85% |
| Fuel flow rate (mol/s) | 0.83 | Compressor efficiency | 75% |
| Methane content in biogas | 60% | Pump efficiency | 80% |
| SOFC inlet pressure (kPa) | 810.6 | Number of single cells | 5008 |
| SOFC inlet temperature (K) | 700 | Generator efficiency | 98% |
| Active surface area of single cell (m2) | 0.027 | Combustion chamber efficiency | 95% |
| Motive steam pressure (kPa) | 2300 | Gas turbine efficiency | 85% |
| Motive steam flow rate (kg/s) | 0.022 | Spray liquid temperature (K) | 313.15 |
| Brine concentration (mg/L) | 5000 | Expansion ratio | 240.9 |
| Pinch temperature difference of steam generator (K) | 353.15 | Pinch temperature difference of generator in refrigeration cycle (K) | 333.15 |
| Air conditioning return water temperature (K) | 285.15 | Heating supply water temperature (K) | 333.15 |
| Air conditioning supply water temperature (K) | 280.15 | Heating return water temperature (K) | 313.15 |
Table 5.
Decision variables and optimization program input parameters.
Table 5.
Decision variables and optimization program input parameters.
| Parameter | Value |
|---|
| PSOFC (kPa) | 700~1000 |
| m8 (mol/s) | 5.5~6.5 |
| Uf | 80~90% |
| CR | 2.0~3.0 |
| Population size | 100 |
| Crossover probability | 90% |
| Mutation probability | 10% |
| Termination generation | 200 |
| Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).