Directional Dependency of Relative Permeability in Vugular Porous Medium: Experiment and Numerical Simulation
Abstract
1. Introduction
2. Displacement Experiment for Vugular Porous Media
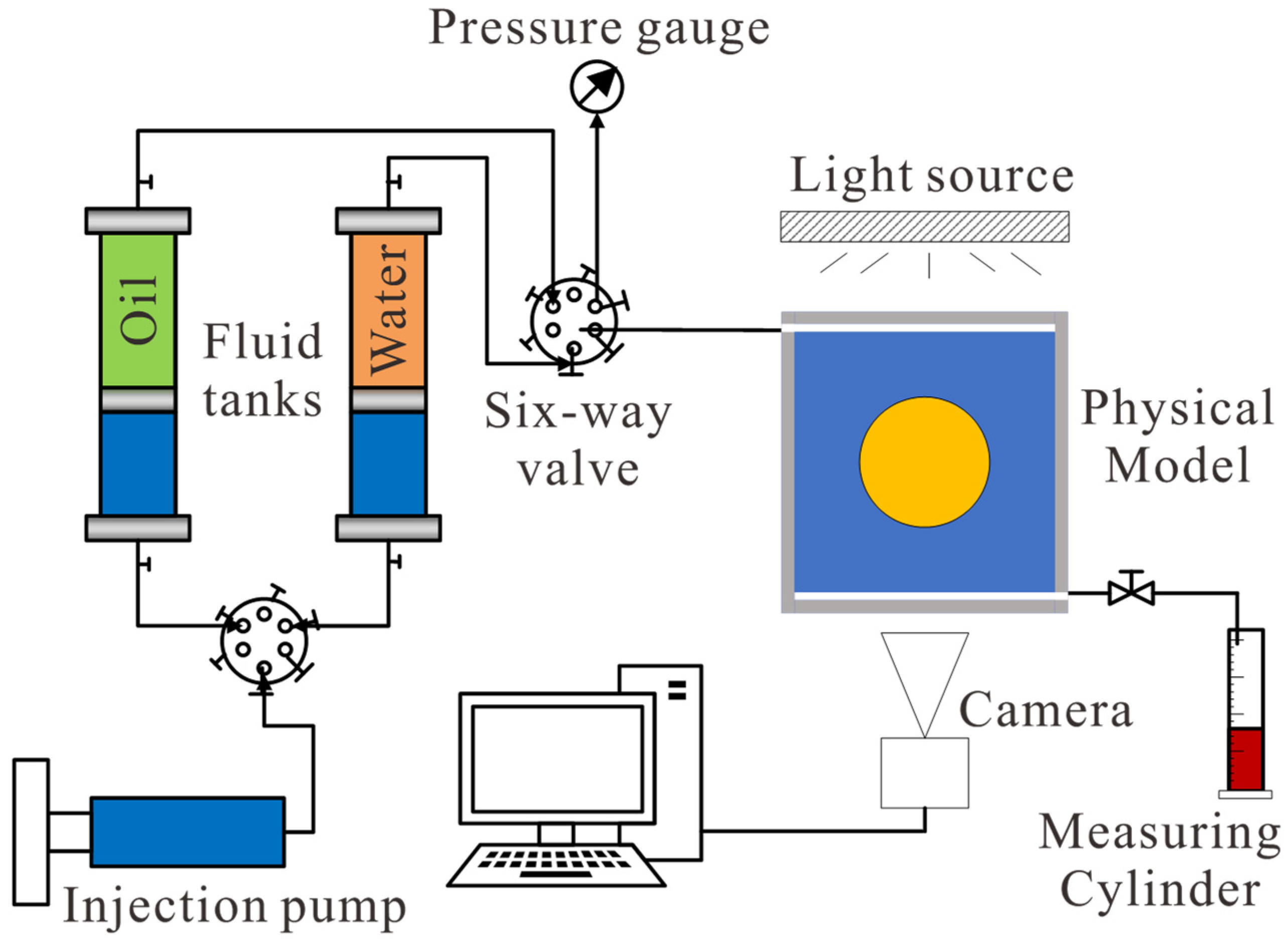
2.1. Physical Model Setup and Fluid Characteristics
2.2. Experiment Setup and Procedure
- (1)
- Fully saturate the model with water, then inject oil from the top of the model until no water is produced.
- (2)
- Fully saturate the injection wellbore with water by opening valves V1 and V2 only.
- (3)
- Inject water into the model at a constant rate of 4.5 mL/min by opening valve V1 and one of the valves V3 or V4. This gives an average flow velocity (Darcy velocity) of 6.48 m/day. As shown in Figure 4, the macroscopic flow direction is across the two sides of the wellbores. In this study, we denote the angle between this flow direction and the horizontal plane as θ. For instance, θ equals to −60° in Figure 4. The injection is continued until the water cut reaches 99%, which is usually achieved after 3–5 PV (pore volume) of water is injected.
- (4)
- During step (3), constantly record phase distribution within the model with a camera, as well as the pressure drop across the model and liquid production.
- (5)
- Change a new sample. Set θ equal to −90° (vertically downward), 0° (horizontal), and 90° (vertically upward), respectively, and repeat step (1)–(4).
2.3. Experiment Results
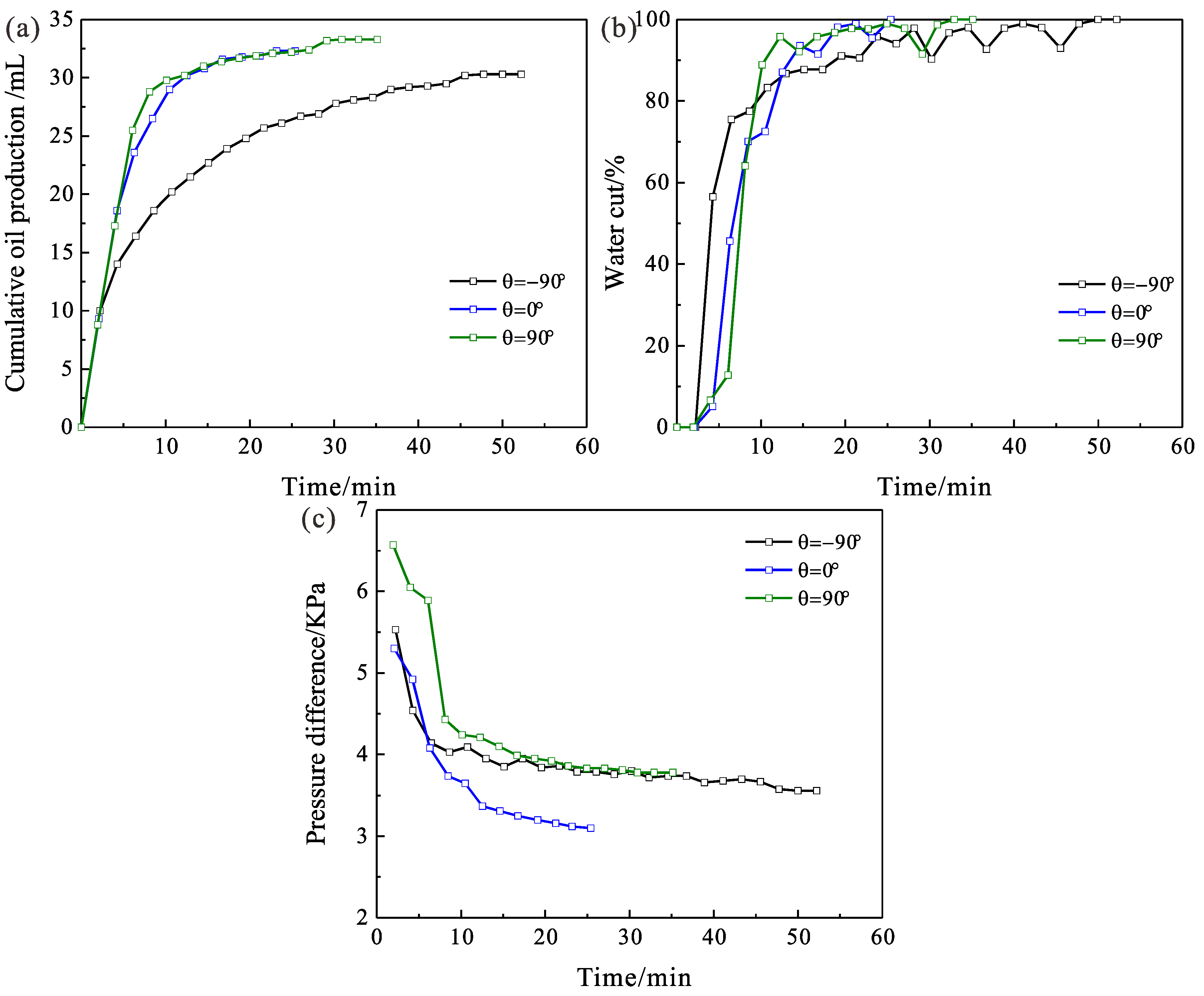
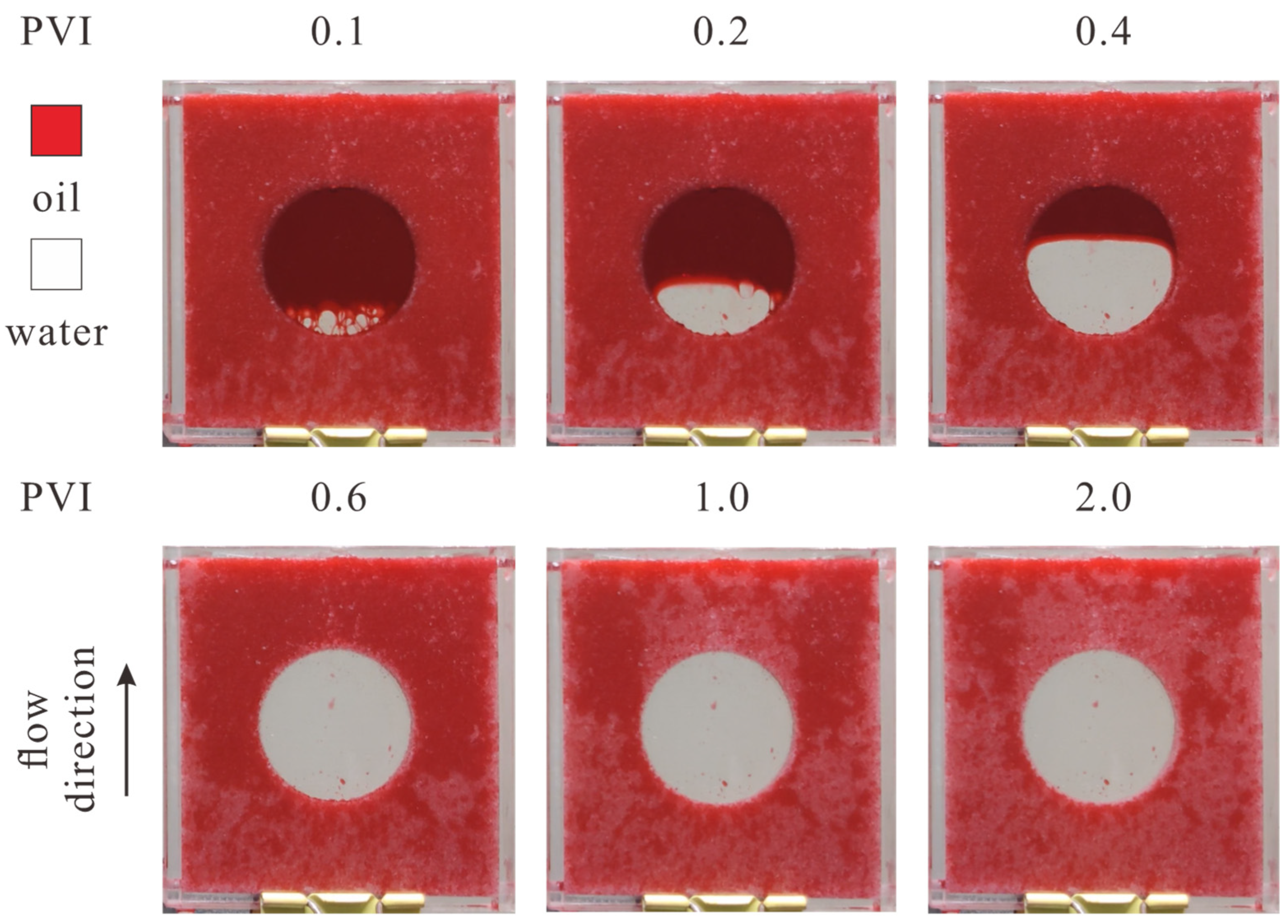
2.3.1. Case 1: Vertically Upwards (θ = 90°)
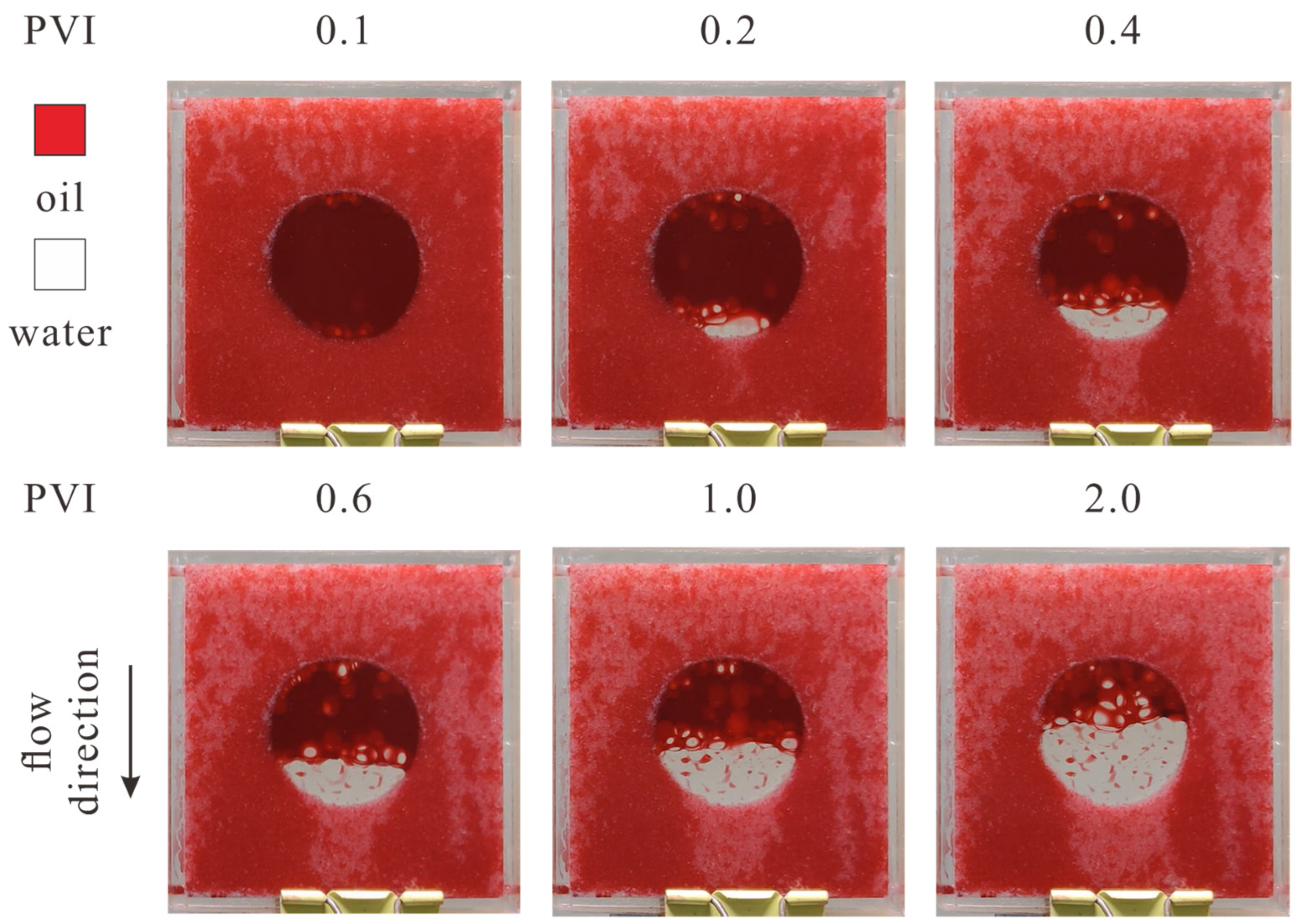
2.3.2. Case 2: Horizontal (θ = 0°)
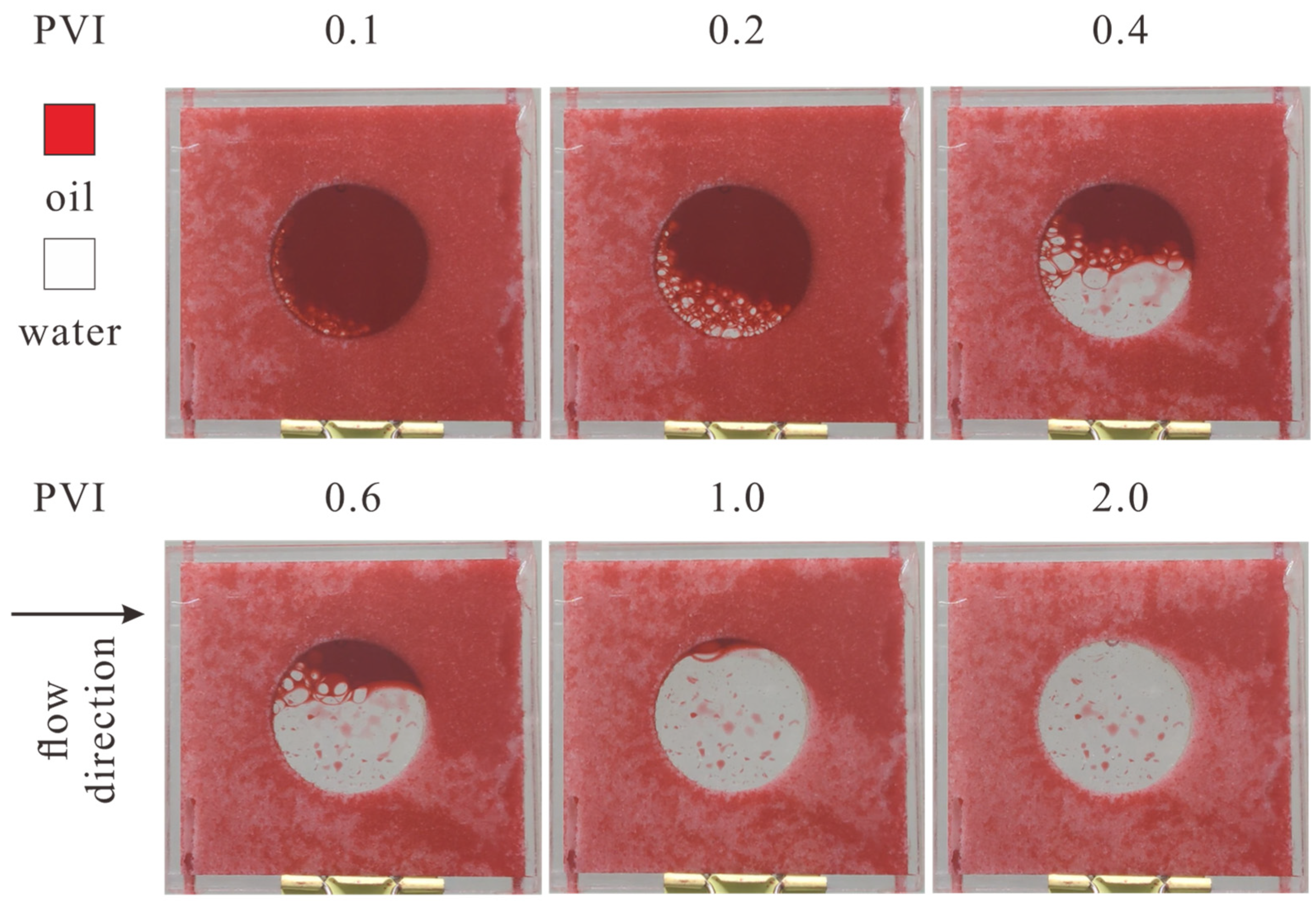
2.3.3. Case 3: Vertically Downwards (θ = −90°)
2.3.4. Discussion
3. Numerical Scheme
3.1. Mathematical Model
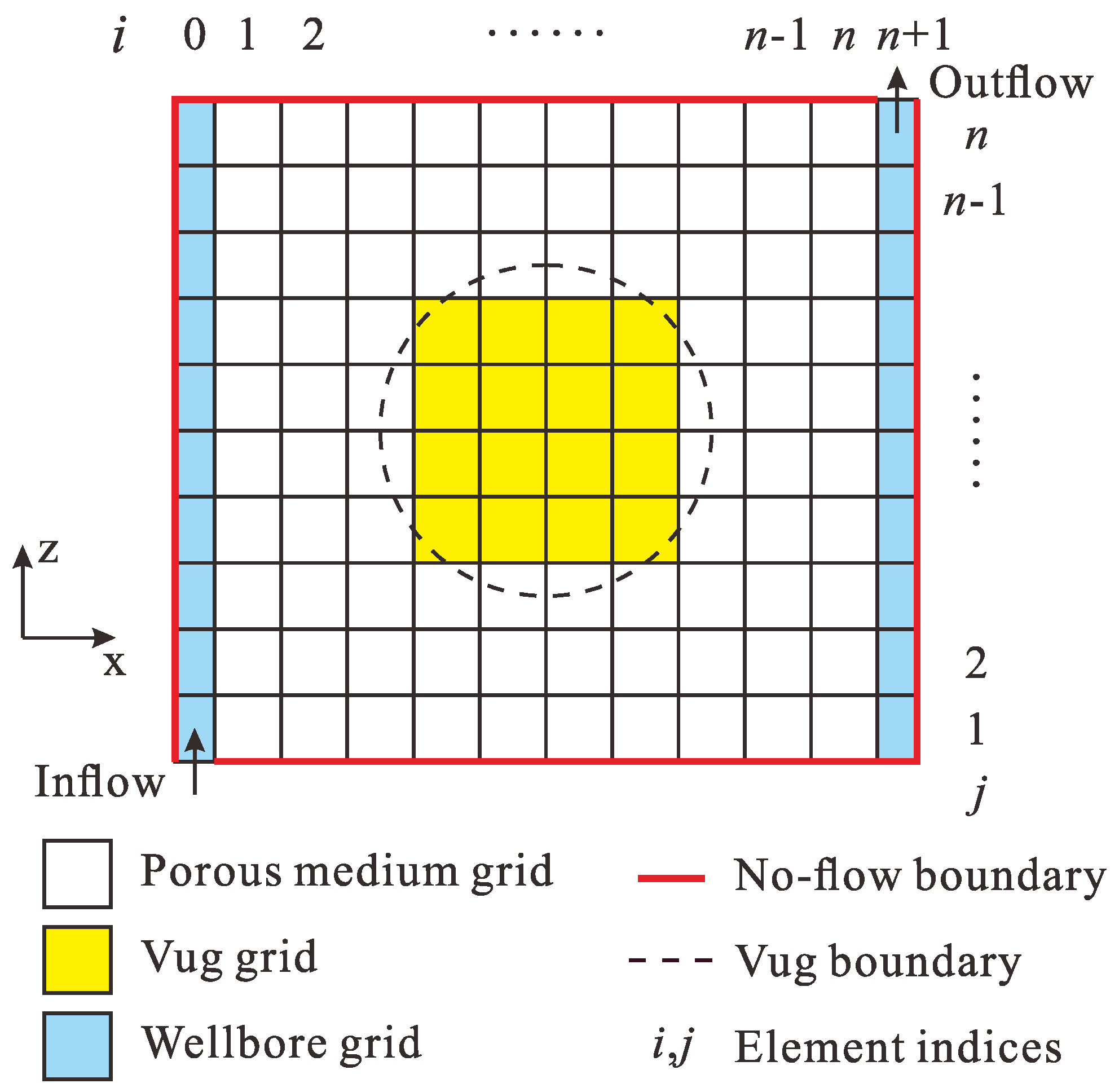
3.2. Model Discretization
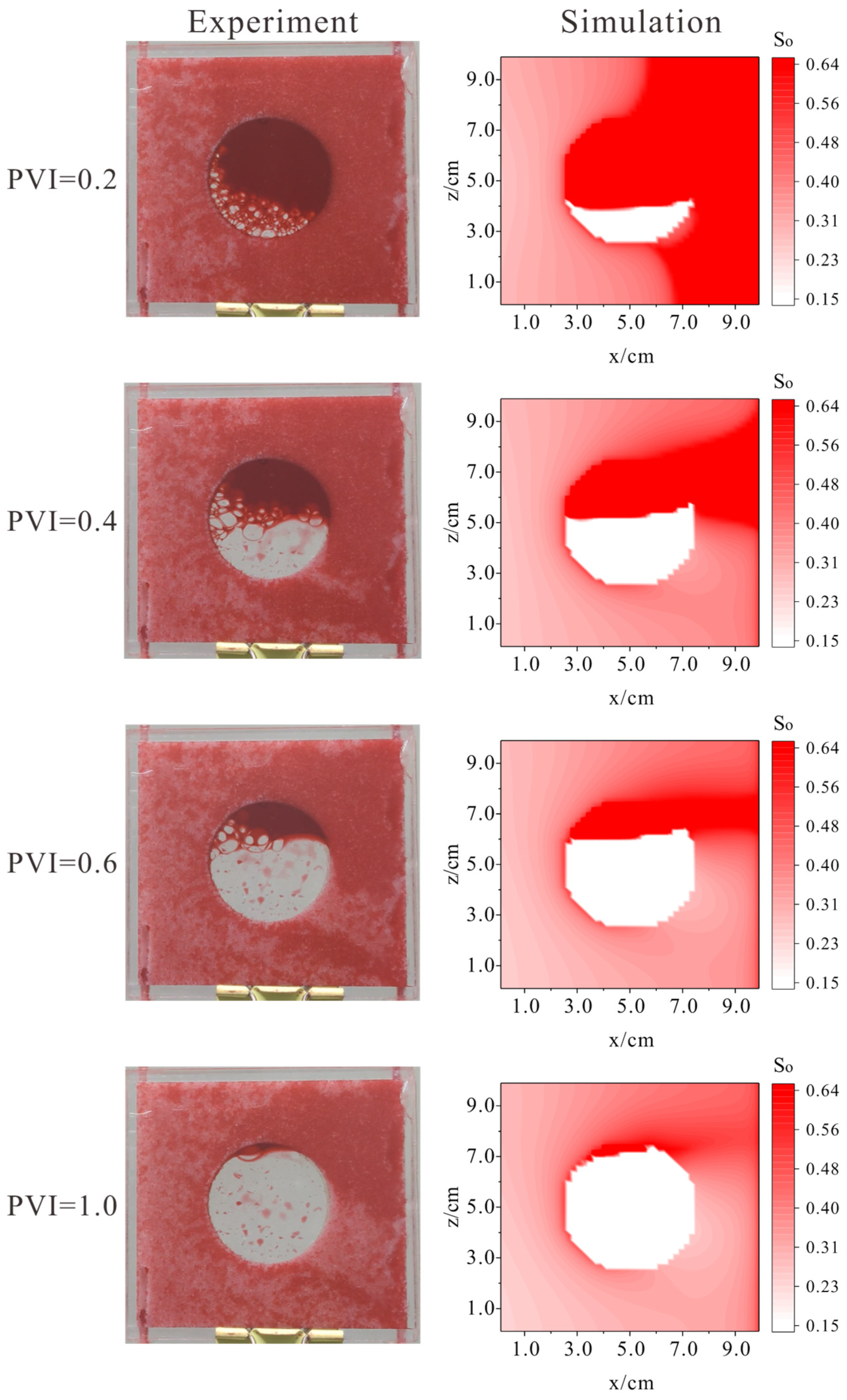
3.3. Model Validation
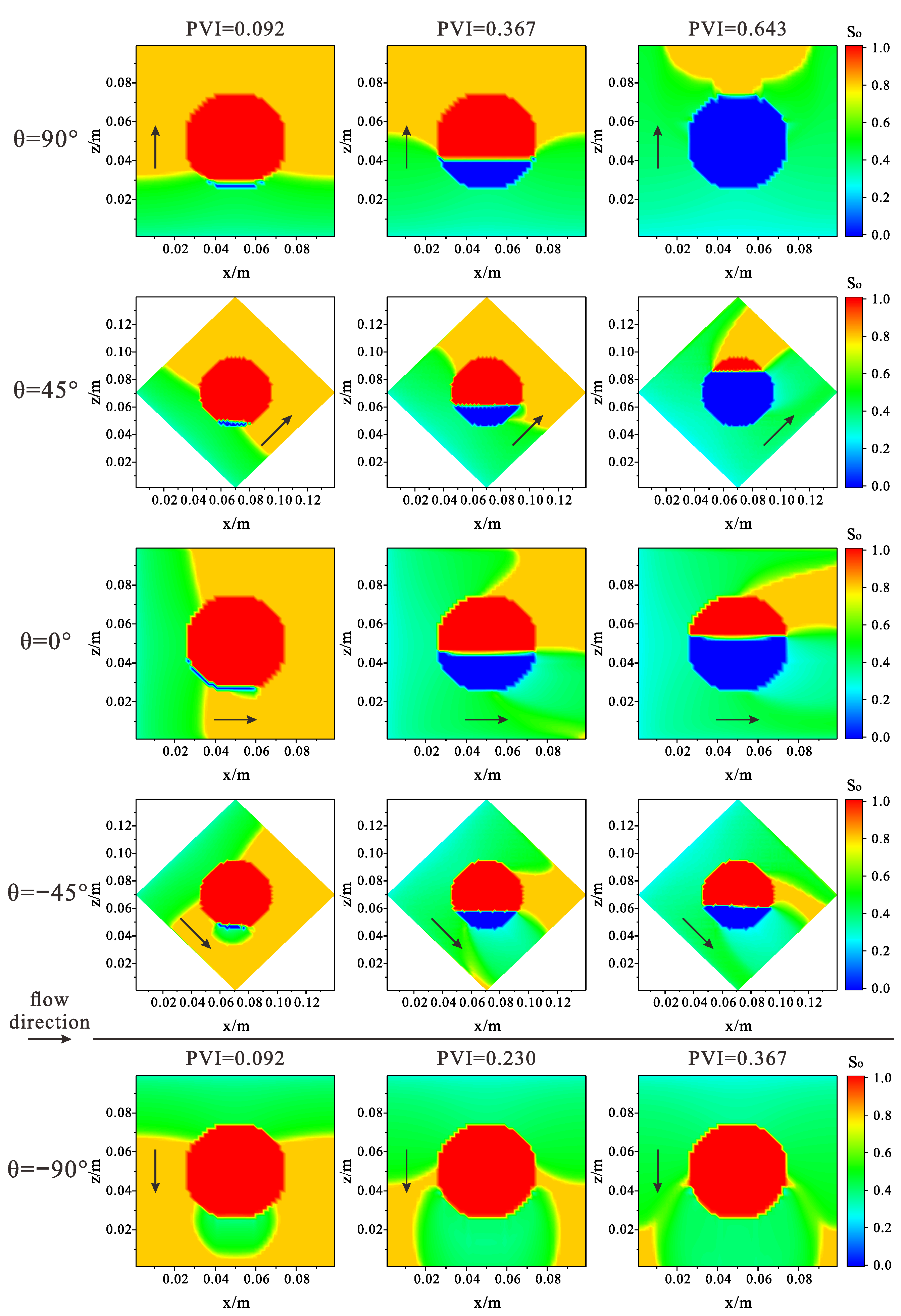
4. Directional Dependent Relative Permeability
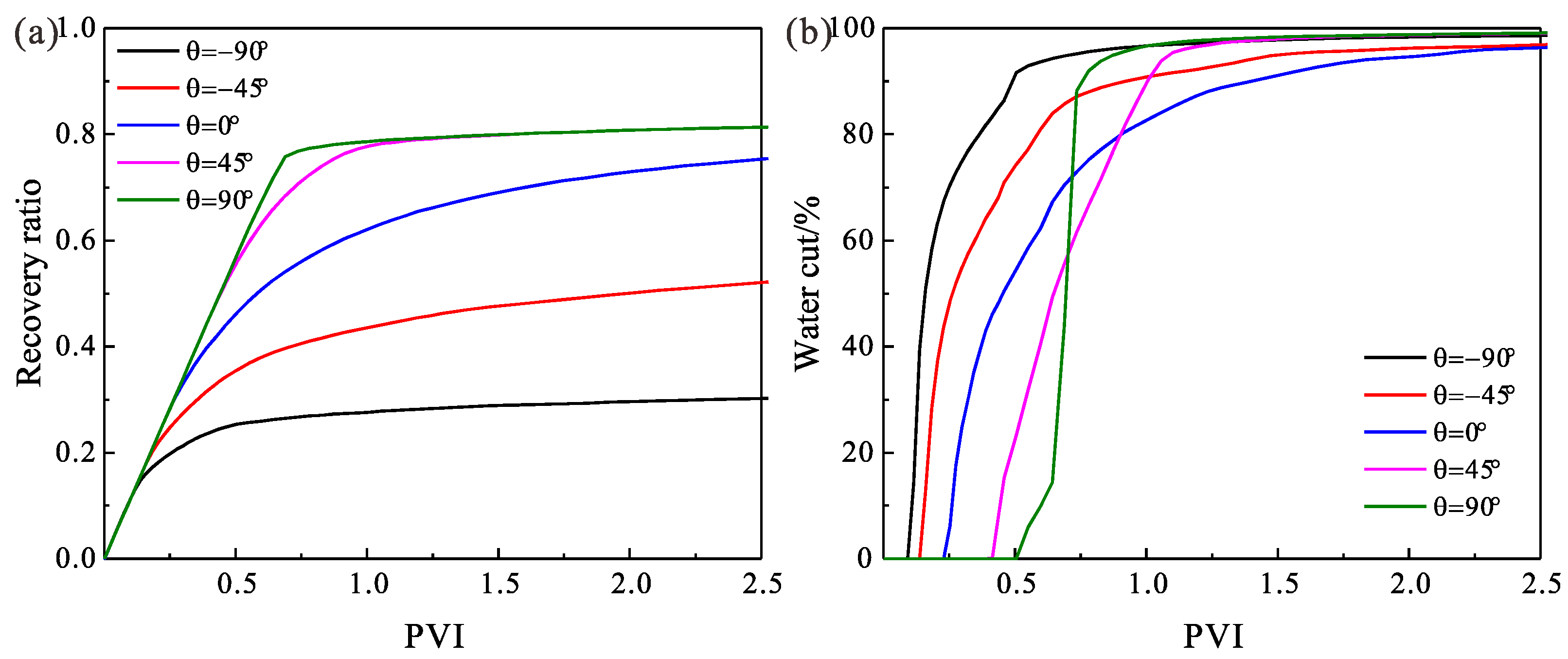
4.1. Numerical Simulation
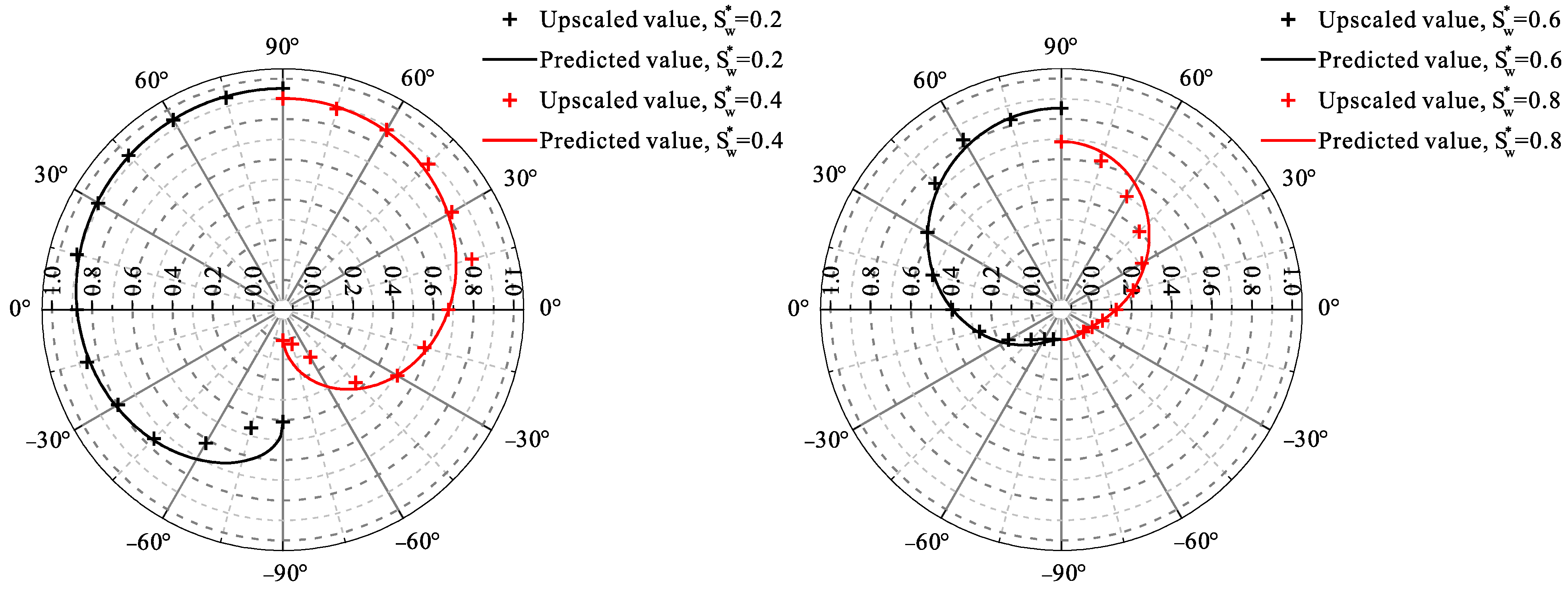
4.2. Transmissibility Weighted Upscaling
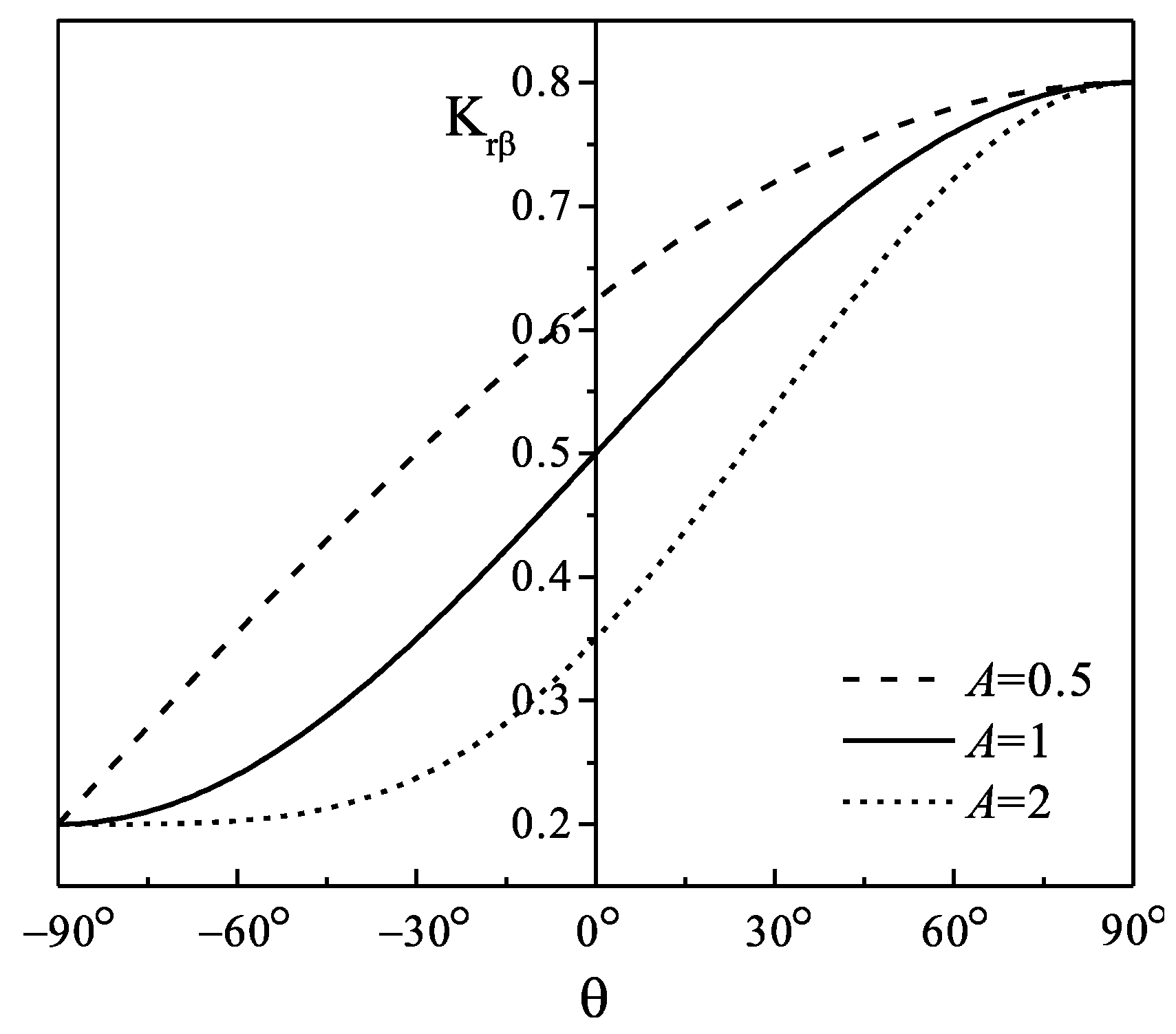
4.3. Directional Relative Permeability Model
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wei, C.; Song, H.; Li, Y.; Zhang, Q.; Song, B.; Wang, J. Production Characteristics with Different Superimposed Modes Using Variogram: A Case Study of a Super-Giant Carbonate Reservoir in the Middle East. Energies 2017, 10, 250. [Google Scholar] [CrossRef]
- Alzayer, H.; Mehran, S. A New Approach to Simulate Near-Miscible Water-Alternating-Gas Injection for Mixed-Wet Reservoirs. In Proceedings of the SPE Kingdom of Saudi Arabia Annual Technical Symposium and Exhibition, Dammam, Saudi Arabia, 23–26 April 2018. [Google Scholar] [CrossRef]
- Tian, F.; Di, Q.; Jin, Q.; Cheng, F.; Zhang, W.; Lin, L.; Wang, Y.; Yang, D.; Niu, C.; Li, Y. Multiscale geological-geophysical characterization of the epigenic origin and deeply buried paleokarst system in tahe oilfield, tarim basin. Mar. Pet. Geol. 2019, 102, 16–32. [Google Scholar] [CrossRef]
- Xie, X.; Weiss, W.W.; Tong, Z.; Morrow, N.R. Improved oil recovery from carbonate reservoirs by chemical stimulation. SPE J. 2005, 10, 276–285. [Google Scholar] [CrossRef]
- Burchette, T.P. Carbonate rocks and petroleum reservoirs; a geological perspective from the industry. Geol. Soc. Lond. Spec. Publ. 2012, 370, 17–37. [Google Scholar] [CrossRef]
- Qing, S.S.; Sloan, R. Quantification of uncertainty in recovery efficiency predictions: Lessons learned from 250 mature carbonate fields. In Proceedings of the SPE Annual Technical Conference and Exhibition, Denver, CO, USA, 5–8 October 2003. [Google Scholar] [CrossRef]
- Agada, S.; Chen, F.; Geiger, S.; Toigulova, G.; Agar, S.; Shekhar, R.; Benson, G.S.; Hehmeyer, O.; Amour, F.; Mutti, M.; et al. Numerical simulation of fluid-flow processes in a 3D high-resolution carbonate reservoir analogue. Pet. Geosci. 2014, 20, 125–142. [Google Scholar] [CrossRef]
- Lucia, F.J. Carbonate Reservoir Characterization: An Integrated Approach, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2007; pp. 29–38. ISBN 978-3-540-72740-8. [Google Scholar] [CrossRef]
- He, J.; Killough, J.E.; Fadlelmula, M.M.; Fraim, M. A unified finite difference model for the simulation of transient flow in naturally fractured carbonate karst reservoirs. In Proceedings of the SPE Reservoir Simulation Symposium, Houston, TX, USA, 23–25 February 2015. [Google Scholar] [CrossRef]
- Popov, P.; Efendiev, Y.; Qin, G. Multiscale modeling and simulations of flows in naturally fractured karst reservoirs. Commun. Comput. Phys. 2009, 6, 162–184. [Google Scholar] [CrossRef]
- Liu, J.; Bodvarsson, G.S.; Wu, Y.S. Analysis of flow behavior in fractured lithophysal reservoirs. J. Contam. Hydrol. 2003, 62–63, 189–211. [Google Scholar] [CrossRef]
- Camacho-Velázquez, R.; Vásquez-Cruz, M.; Castrejón-Aivar, R.; Arana-Ortiz, V. Pressure-transient and decline-curve behavior in naturally fractured vuggy carbonate reservoirs. SPE Res. Eval. Eng. 2005, 8, 95–112. [Google Scholar] [CrossRef]
- Wu, Y.S.; Ehlig-Economides, C.; Qin, G.; Kang, Z.; Zhang, W.; Ajayi, B.; Tao, Q. A Triple-Continuum Pressure-Transient Model for a Naturally Fractured Vuggy Reservoir. In Proceedings of the SPE Annual Technical Conference and Exhibition, Anaheim, CA, USA, 11–14 November 2007. [Google Scholar] [CrossRef]
- Kang, Z.; Wu, Y.S.; Li, J.; Wu, Y.; Zhang, J.; Wang, G. Modeling Multiphase Flow in Naturally Fractured Vuggy Petroleum Reservoirs. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 24–27 September 2006. [Google Scholar] [CrossRef]
- Wu, Y.S.; Di, Y.; Kang, Z.; Fakcharoenphol, P. A multiple-continuum model for simulating single-phase and multiphase flow in naturally fractured vuggy reservoirs. J. Pet. Sci. Eng. 2011, 78, 13–22. [Google Scholar] [CrossRef]
- Yao, J.; Huang, Z.; Li, Y.; Wang, C.; Lv, X. Discrete fracture-vug network model for modeling fluid flow in fractured vuggy porous media. In Proceedings of the International Oil and Gas Conference and Exhibition, Beijing, China, 8–10 June 2010. [Google Scholar] [CrossRef]
- Zhang, N.; Yao, J.; Xue, S.; Huang, Z. Multiscale mixed finite element, discrete fracture–vug model for fluid flow in fractured vuggy porous media. Int. J. Heat Mass Transf. 2016, 96, 396–405. [Google Scholar] [CrossRef]
- Zhang, X.; Huang, Z.; Lei, Q.; Yao, J.; Gong, L.; Sun, S.; Li, Y. Connectivity, permeability and flow channelization in fractured karst reservoirs: A numerical investigation based on a two-dimensional discrete fracture-cave network model. Adv. Water Resour. 2022, 161, 104142. [Google Scholar] [CrossRef]
- Beavers, G.S.; Joseph, D.D. Boundary conditions at a naturally permeable wall. J. Fluid Mech. 1967, 30, 197–207. [Google Scholar] [CrossRef]
- Saffman, P.G. On the boundary condition at the surface of a porous medium. Stud. Appl. Math. 1971, 50, 93–101. [Google Scholar] [CrossRef]
- Chen, J.; Sun, S.; Wang, X. A numerical method for a model of two-phase flow in a coupled free flow and porous media system. J. Comput. Phys. 2014, 268, 1–16. [Google Scholar] [CrossRef]
- Chen, J.; Sun, S.; Chen, Z. Coupling two-phase fluid flow with two-phase darcy flow in anisotropic porous media. Adv. Mech. Eng. 2014, 6, 871021. [Google Scholar] [CrossRef]
- Huang, Z.; Gao, B.; Zhang, X.Y.; Yao, J. On the coupling of two-phase free flow and porous flow. In Proceedings of the 15th European Conference on the Mathematics of Oil Recovery, Amsterdam, The Netherlands, 29 August–1 September 2016. [Google Scholar] [CrossRef]
- Xie, H.; Li, A.; Huang, Z.; Gao, B.; Peng, R. Coupling of two-phase flow in fractured-vuggy reservoir with filling medium. Open Phys. 2017, 15, 12–17. [Google Scholar] [CrossRef]
- Yan, X.; Huang, Z.; Yao, J.; Zhang, Z.; Liu, P.; Li, Y.; Fan, D. Numerical simulation of hydro-mechanical coupling in fractured vuggy porous media using the equivalent continuum model and embedded discrete fracture model. Adv. Water Resour. 2019, 126, 137–154. [Google Scholar] [CrossRef]
- Liu, L.; Huang, Z.; Yao, J.; Di, Y.; Wu, Y. An efficient hybrid model for 3D complex fractured vuggy reservoir simulation. SPE J. 2020, 25, 907–924. [Google Scholar] [CrossRef]
- Liu, L.; Huang, Z.; Yao, J.; Lei, Q.; Di, Y.; Wu, Y.; Zhang, K.; Cui, S. Simulating two-phase flow and geomechanical deformation in fractured karst reservoirs based on a coupled hydro-mechanical model. Int. J. Rock Mech. Min. Sci. 2021, 137, 104543. [Google Scholar] [CrossRef]
- Arbogast, T.; Lehr, H.L. Homogenization of a darcy-stokes system modeling vuggy porous media. Comput. Geosci. 2006, 10, 291–302. [Google Scholar] [CrossRef]
- Arbogast, T.; Brunson, D.S. A computational method for approximating a darcy-stokes system governing a vuggy porous medium. Comput. Geosci. 2007, 11, 207–218. [Google Scholar] [CrossRef]
- Huang, Z.; Yao, J.; Li, Y.; Wang, C.; Lv, X. Numerical calculation of equivalent permeability tensor for fractured vuggy porous media based on homogenization theory. Commun. Comput. Phys. 2011, 9, 180–204. [Google Scholar] [CrossRef]
- Popov, P.; Qin, G.; Bi, L.; Efendiev, Y.; Kang, Z.; Li, J. Multiphysics and Multiscale Methods for Modeling Fluid Flow Through Naturally Fractured Vuggy Carbonate Reservoirs. SPE Res. Eval. Eng. 2009, 12, 218–231. [Google Scholar] [CrossRef]
- Qin, G.; Bi, L.; Popov, P.; Efendiev, Y.; Espedal, M.S. An efficient upscaling process based on a unified fine-scale multi-physics model for flow simulation in naturally fracture carbonate karst reservoirs. In Proceedings of the International Oil and Gas Conference and Exhibition, Beijing, China, 8–10 June 2010. [Google Scholar] [CrossRef]
- Golfier, F.; Lasseux, D.; Quintard, M. Investigation of the effective permeability of vuggy or fractured porous media from a darcy-brinkman approach. Comput. Geosci. 2015, 19, 63–78. [Google Scholar] [CrossRef]
- Pal, M. A unified approach to simulation and upscaling of single-phase flow through vuggy carbonates. Int. J. Numer. Methods Fluids 2012, 69, 1096–1123. [Google Scholar] [CrossRef]
- Li, Y.; Yao, J.; Li, Y.; Yin, C.; Pan, B.; Lee, J.; Dong, M. An equivalent continuum approach for modeling two-phase flow in fractured-vuggy media. Int. J. Multiscale Comput. Eng. 2017, 15, 79–98. [Google Scholar] [CrossRef]
- Huang, Z.; Yao, J.; Wang, Y. An efficient numerical model for immiscible two-phase flow in fractured karst reservoirs. Commun. Comput. Phys. 2013, 13, 540–558. [Google Scholar] [CrossRef]
- Wang, L.; Golfier, F.; Tinet, A.; Chen, W.; Vuik, C. An efficient adaptive implicit scheme with equivalent continuum approach for two-phase flow in fractured vuggy porous media. Adv. Water Resour. 2022, 163, 104186. [Google Scholar] [CrossRef]
- Pairoys, F.; Lasseux, D.; Bertin, H. An experimental and numerical investigation of water-oil flow in vugular porous media. In Proceedings of the International Symposium of the Society of Core Analysis, Pau, France, 21–24 September 2003. [Google Scholar]
- Wang, J.; Liu, H.; Ning, Z.; Zhang, H.; Hong, C. Experiments on water flooding in fractured-vuggy cells in fractured-vuggy reservoirs. Pet. Explor. Dev. 2014, 41, 74–81. [Google Scholar] [CrossRef]
- Yuan, D.; Hou, J.; Song, Z.; Wang, Y.; Luo, M.; Zheng, Z. Residual oil distribution characteristic of fractured-cavity carbonate reservoir after water flooding and enhanced oil recovery by N2 flooding of fractured-cavity carbonate reservoir. J. Pet. Sci. Eng. 2015, 129, 15–22. [Google Scholar] [CrossRef]
- Wang, Y.; Hou, J.; Tang, Y.; Song, Z. Effect of vug filling on oil-displacement efficiency in carbonate fractured-vuggy reservoir by natural bottom-water drive: A conceptual model experiment. J. Pet. Sci. Eng. 2019, 174, 1113–1126. [Google Scholar] [CrossRef]
- Yang, W.; Zhang, D.; Lei, G. Experimental study on multiphase flow in fracture-vug medium using 3D printing technology and visualization techniques. J. Pet. Sci. Eng. 2020, 193, 107394. [Google Scholar] [CrossRef]
- Lu, G.; Zhang, L.; Liu, Q.; Xu, Q.; Zhao, Y.; Li, X.; Deng, G.; Wang, Y. Experiment analysis of remaining oil distribution and potential tapping for fractured-vuggy reservoir. J. Pet. Sci. Eng. 2022, 208, 109544. [Google Scholar] [CrossRef]
- Krotkiewski, M.; Ligaarden, I.S.; Lie, K.; Schmid, D.W. On the importance of the stokes-brinkman equations for computing effective permeability in karst reservoirs. Commun. Comput. Phys. 2011, 10, 1315–1332. [Google Scholar] [CrossRef]
- Zhou, D.; Fayers, F.J.; Orr Jr, F.M. Scaling of multiphase flow in simple heterogeneous porous media. In Proceedings of the SPE/DOE Improved Oil Recovery Symposium, Tulsa, OK, USA, 17–20 April 1994. [Google Scholar] [CrossRef]
- Durlofsky, L.J. Numerical calculation of equivalent grid-block permeability tensors for heterogeneous porous media. Water Resour. Res. 1991, 27, 699–708. [Google Scholar] [CrossRef]
- Durlofsky, L.J. Coarse scale models of two phase flow in heterogeneous reservoirs: Volume averaged equations and their relationship to existing upscaling techniques. Comput. Geosci. 1998, 2, 73–92. [Google Scholar] [CrossRef]
- Chen, Y.; Li, Y. Local-global two-phase upscaling of flow and transport in heterogeneous formations. Multiscale Model. Simul. 2009, 8, 125–153. [Google Scholar] [CrossRef]
- Darman, N.H.; Sorbie, K.S.; Pickup, G.E. Development of pseudo functions for gravity-dominated immiscible gas displacements. In Proceedings of the SPE Reservoir Simulation Symposium, Houston, TX, USA, 14–17 February 1999. [Google Scholar] [CrossRef]
- Darman, N.H.; Pickup, G.E.; Sorbie, K.S. A comparison of two-phase dynamic upscaling methods based on fluid potentials. Comput. Geosci. 2002, 6, 5–27. [Google Scholar] [CrossRef]
- Azoug, Y.; Tiab, D. The performance of pseudofunctions in the upscaling process. In Proceedings of the SPE Production and Operations Symposium, Oklahoma City, OK, USA, 22–25 March 2003. [Google Scholar] [CrossRef]
- Hashemi, A.; Shadizadeh, S.R.; Zargar, G. Upscaling of relative permeability using pseudo functions. Energy Sources Part A Recovery Util. Environ. Eff. 2014, 36, 2227–2237. [Google Scholar] [CrossRef]










| Parameter | Description | Value | Unit |
|---|---|---|---|
| K | Absolute permeability | 2.4 × 10−11 | m2 |
| ϕ | Porosity | 0.315 | - |
| Swi | Irreducible water saturation | 0.358 | - |
| kro(Swi) | Oil phase relative permeability at irreducible water saturation | 1.0 | - |
| Sor | Residual oil saturation | 0.15 | - |
| krw(Sor) | Water phase relative permeability at residual oil saturation | 0.4 | - |
| λ | Shape factor for Brooks-Corey relative permeability model Equations (7)–(9) | 3.0 | - |
| PD | Pore entry pressure | 0.6 | KPa |
| ρo | Oil density | 840 | kg/m3 |
| μo | Oil viscosity | 18.0 | mpa·s |
| ρw | Water density | 1000 | kg/m3 |
| μw | Water viscosity | 1.0 | mpa·s |
| Parameter | Description | Value | Unit |
|---|---|---|---|
| K | Absolute permeability | 1.0 × 10−11 | m2 |
| ϕ | Porosity | 0.3 | - |
| Swi | Irreducible water saturation | 0.2 | - |
| kro(Swi) | Oil phase relative permeability at irreducible water saturation | 1.0 | - |
| Sor | Residual oil saturation | 0.2 | - |
| krw(Sor) | Water phase relative permeability at residual oil saturation | 0.6 | - |
| λ | Shape factor for Brooks-Corey relative permeability model Equations (7)–(9) | 3.0 | - |
| PD | Pore entry pressure | 0 | KPa |
| ρo | Oil density | 800 | kg/m3 |
| μo | Oil viscosity | 5.884 | mpa·s |
| ρw | Water density | 1000 | kg/m3 |
| μw | Water viscosity | 1.0 | mpa·s |
| Q | Injection rate | 2.0 | ml/min |
| Gr | Gravity number | 0.1 | - |
| Ca | Capillary number | 0.0 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, S.; Di, Y.; Guo, W. Directional Dependency of Relative Permeability in Vugular Porous Medium: Experiment and Numerical Simulation. Energies 2023, 16, 3041. https://doi.org/10.3390/en16073041
Song S, Di Y, Guo W. Directional Dependency of Relative Permeability in Vugular Porous Medium: Experiment and Numerical Simulation. Energies. 2023; 16(7):3041. https://doi.org/10.3390/en16073041
Chicago/Turabian StyleSong, Shihan, Yuan Di, and Wanjiang Guo. 2023. "Directional Dependency of Relative Permeability in Vugular Porous Medium: Experiment and Numerical Simulation" Energies 16, no. 7: 3041. https://doi.org/10.3390/en16073041
APA StyleSong, S., Di, Y., & Guo, W. (2023). Directional Dependency of Relative Permeability in Vugular Porous Medium: Experiment and Numerical Simulation. Energies, 16(7), 3041. https://doi.org/10.3390/en16073041






