Permeability Evolution of Bituminous Coal and Its Dynamic Control, a Case Study from the Southeastern Ordos Basin, China
Abstract
:1. Introduction
2. Samples and Methods
2.1. Samples and Experiments
- (1)
- The confining pressure was held constant at 2.1 MPa unlike the other three cycling experiments which had confining pressures of 4.1 MPa, 5.3 MPa, and 6.3 MPa. Firstly, the experiment was carried out with He. The inlet gas pressure was gradually increased to test the steady flow rate of He at each experimental pressure point, and the permeability of He was then calculated. The above experiment was then repeated with CO2 gas on the same sample, and the permeability of CO2 was calculated.
- (2)
- The effective stress for the particular experiment was set constantly at 2 MPa (the effective stresses of the other three cyclic experiments were 3 MPa, 4 MPa, and 5 MPa). The inlet pressure and the confining pressure were increased at the same time, and the experiments with He and CO2 were performed successively, followed by the measurement of the gas permeability at each pressure point;
- (3)
- X-ray CT scans were performed on the coal samples before and after the four cycles of experiments in order to investigate the development of fractures in the coal rock.
2.2. Permeability Variation Calculations
3. Results
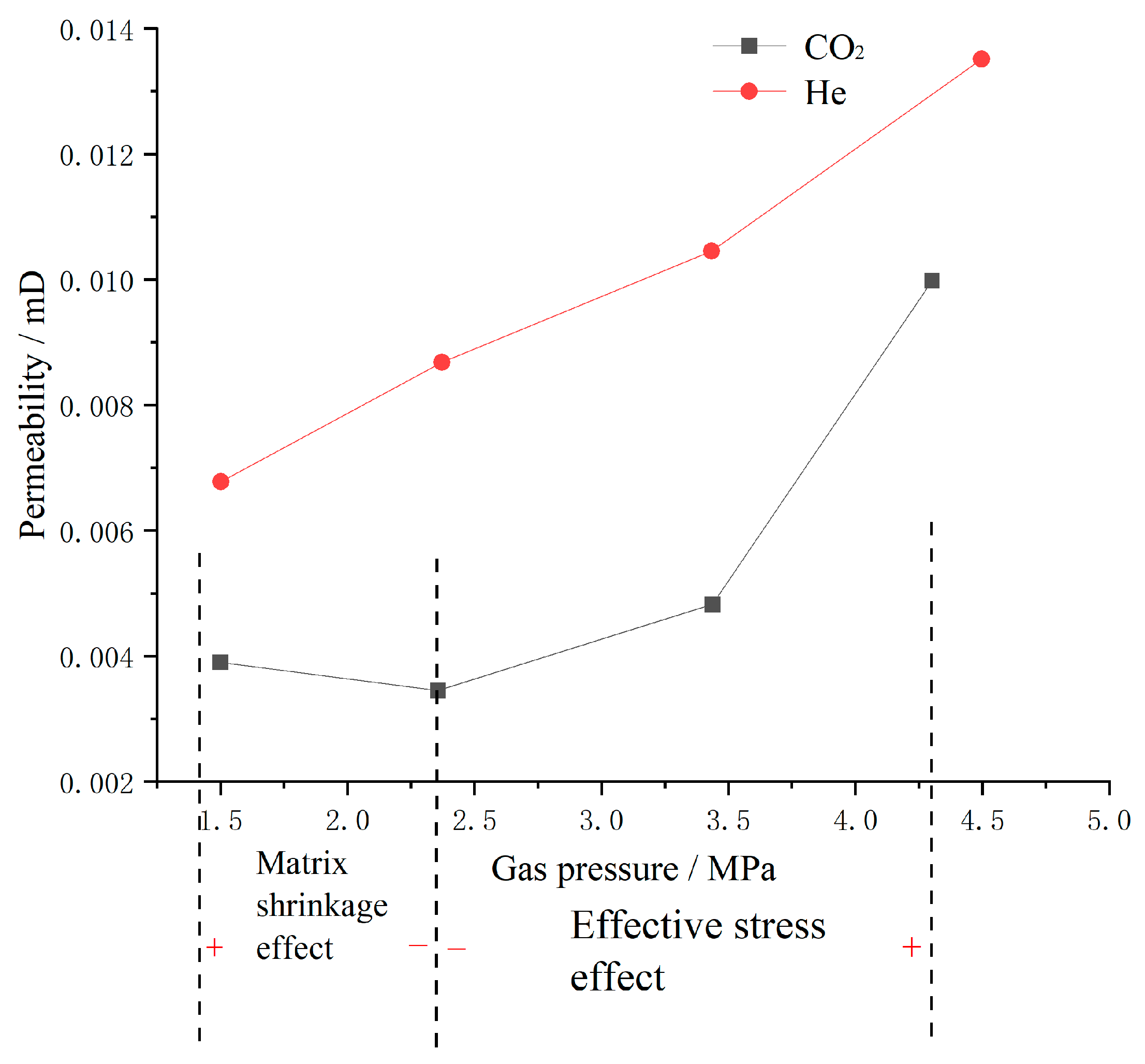
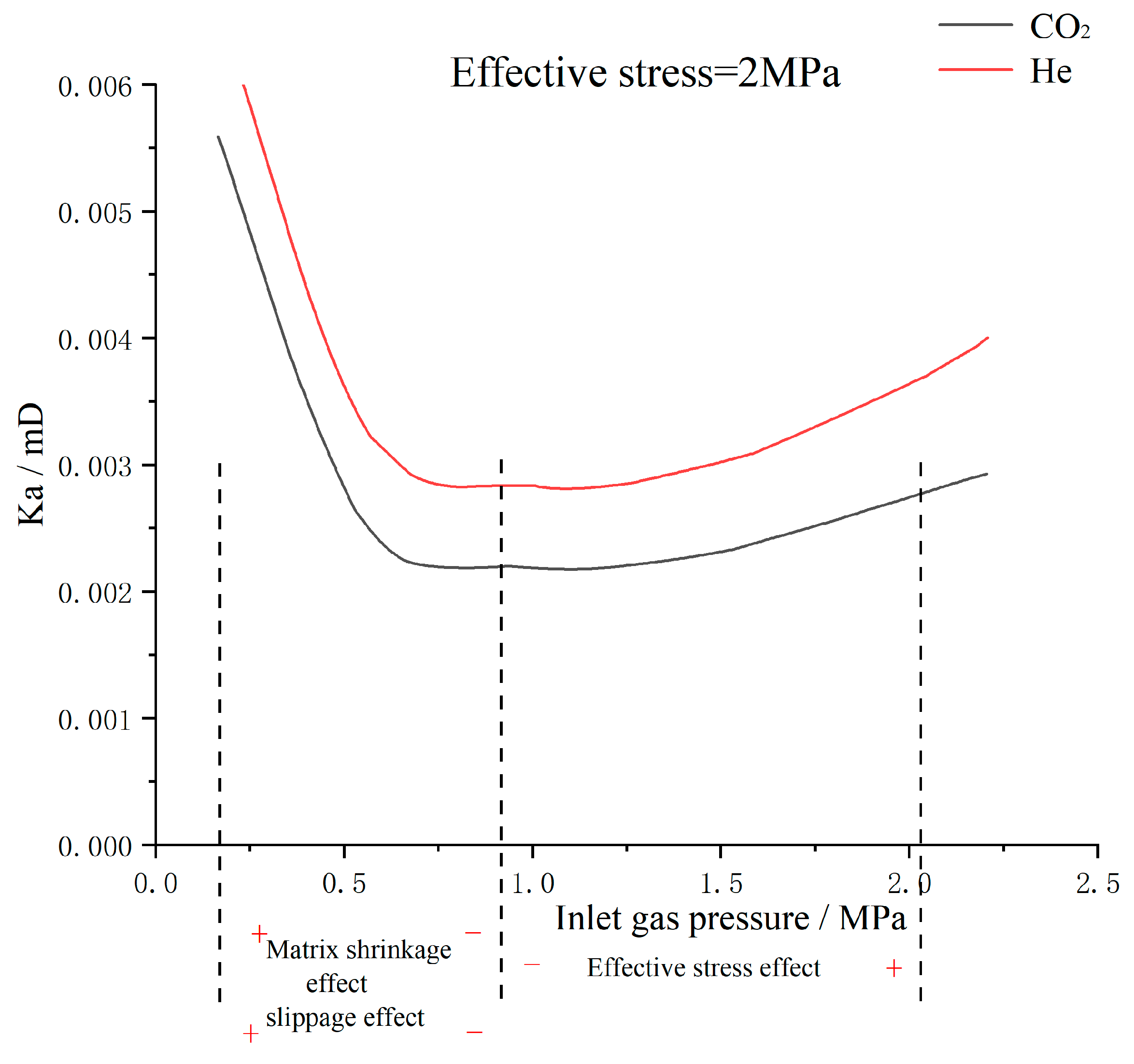
3.1. Coal Fracture and Permeability Evolution Characteristics
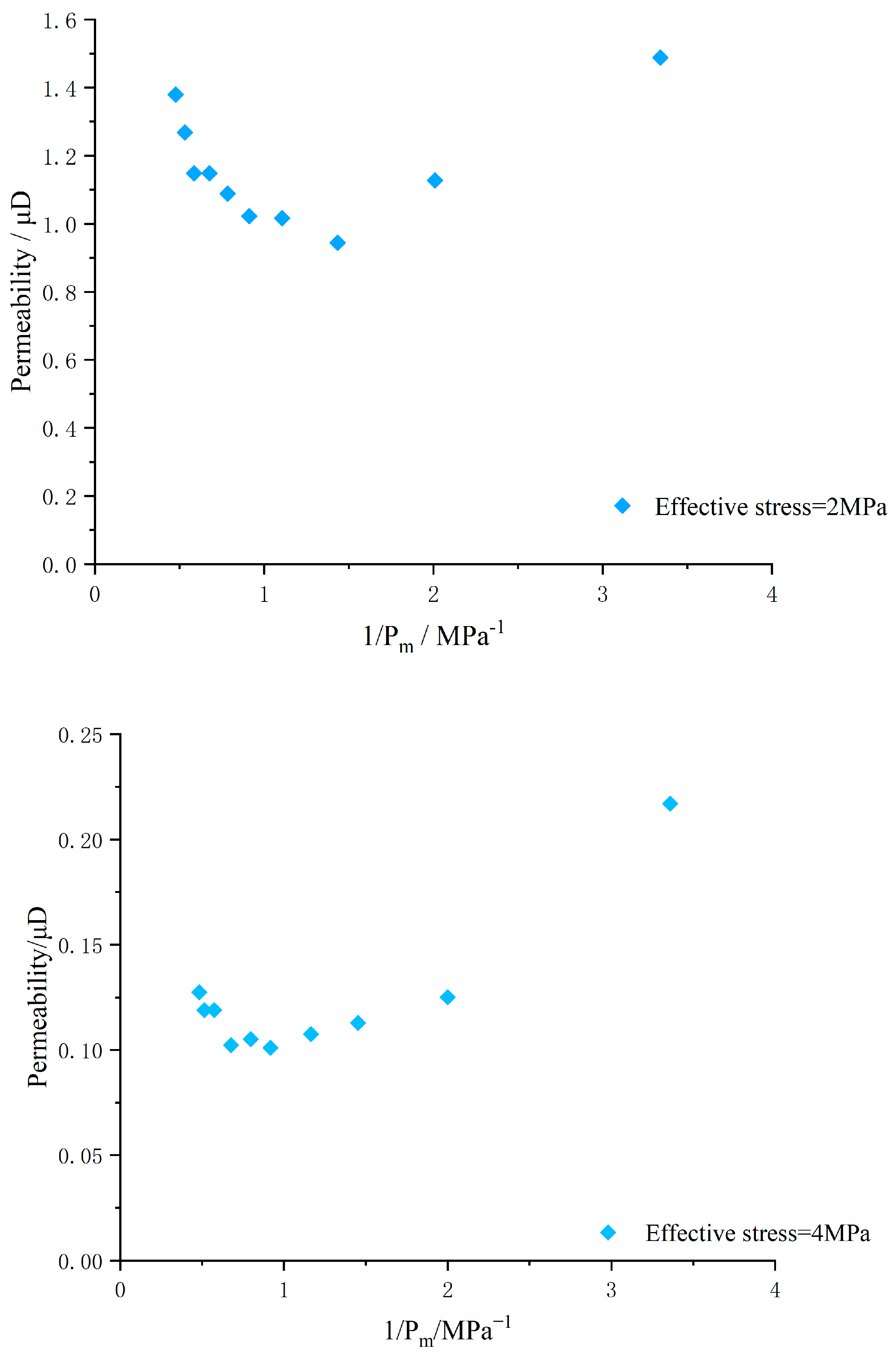
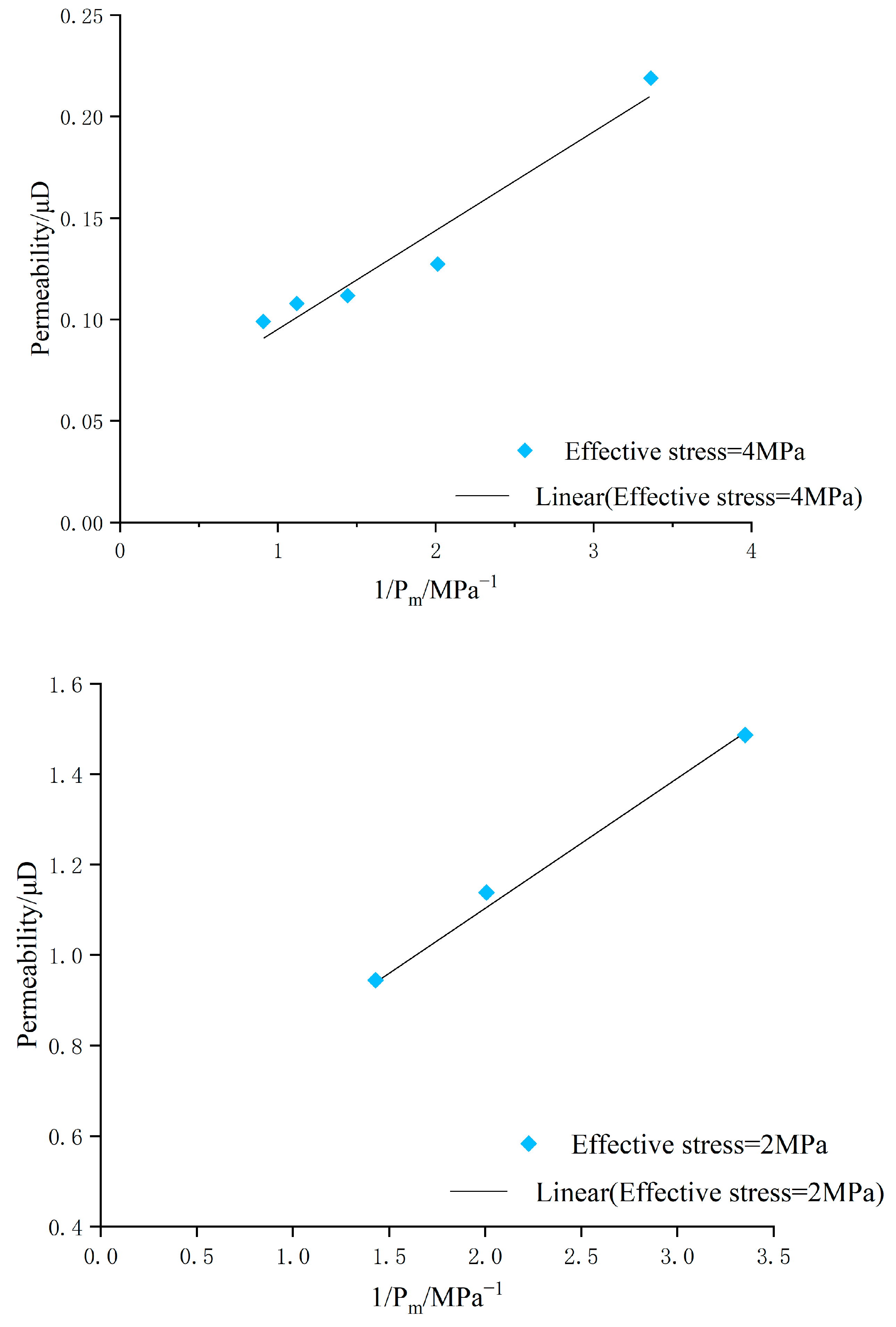
3.2. Factors Influencing Permeability Evolution
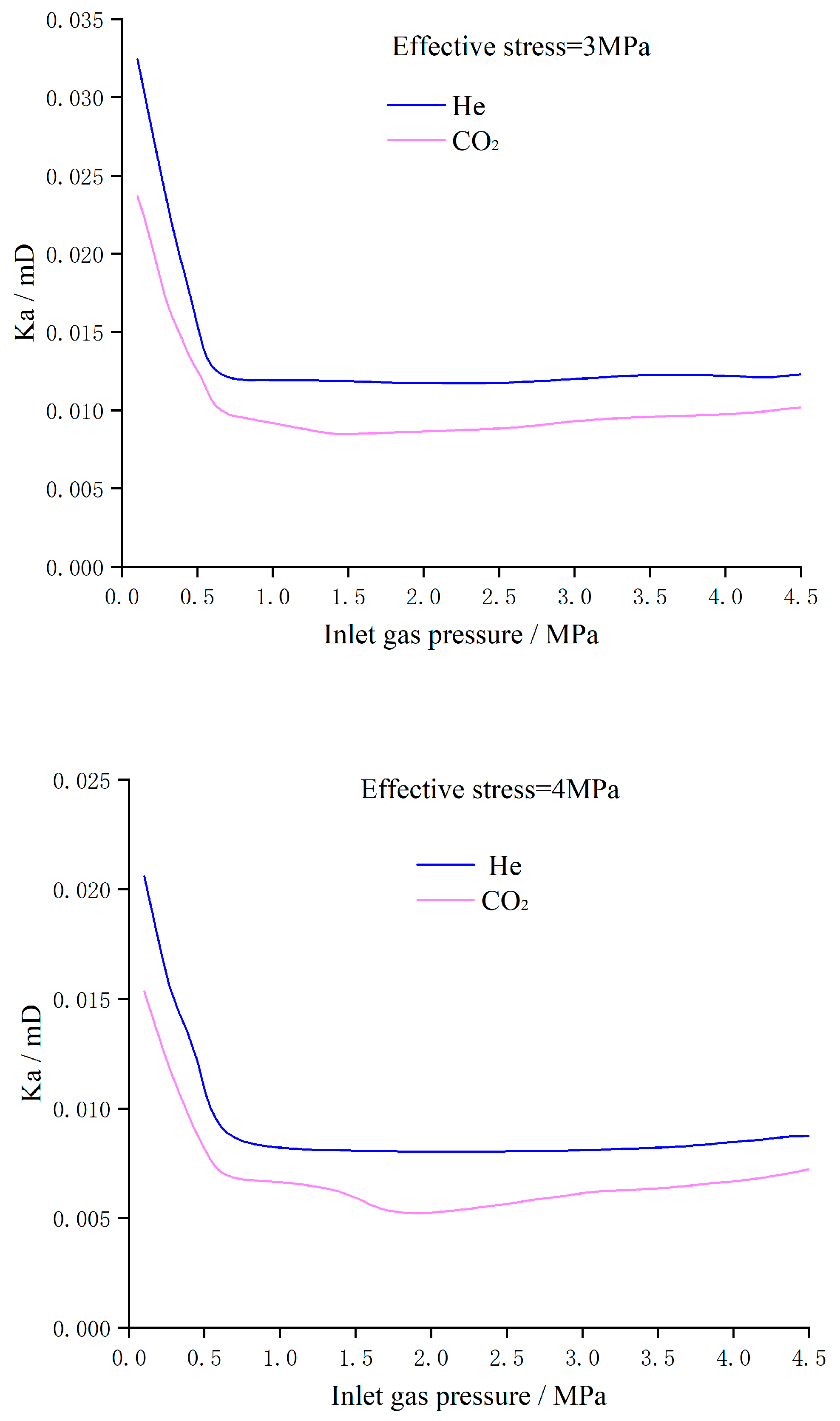
3.2.1. Effective Stress Effect
3.2.2. Gas Slippage Effect
3.2.3. Matrix Shrinkage Effect
3.2.4. Comprehensive Control of Permeability Variation
4. Discussion
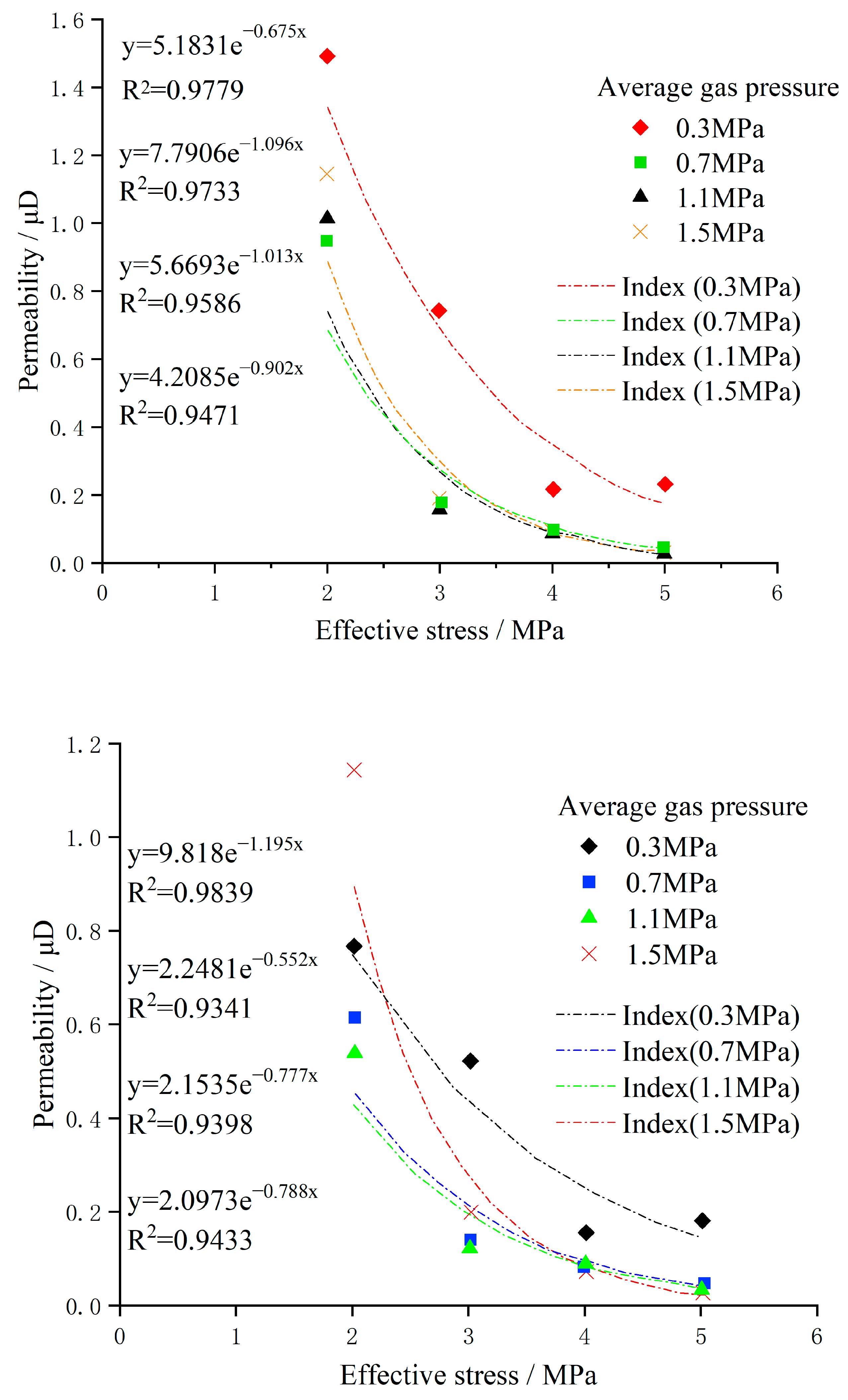
4.1. Model of Effective Stress Controlling Permeability
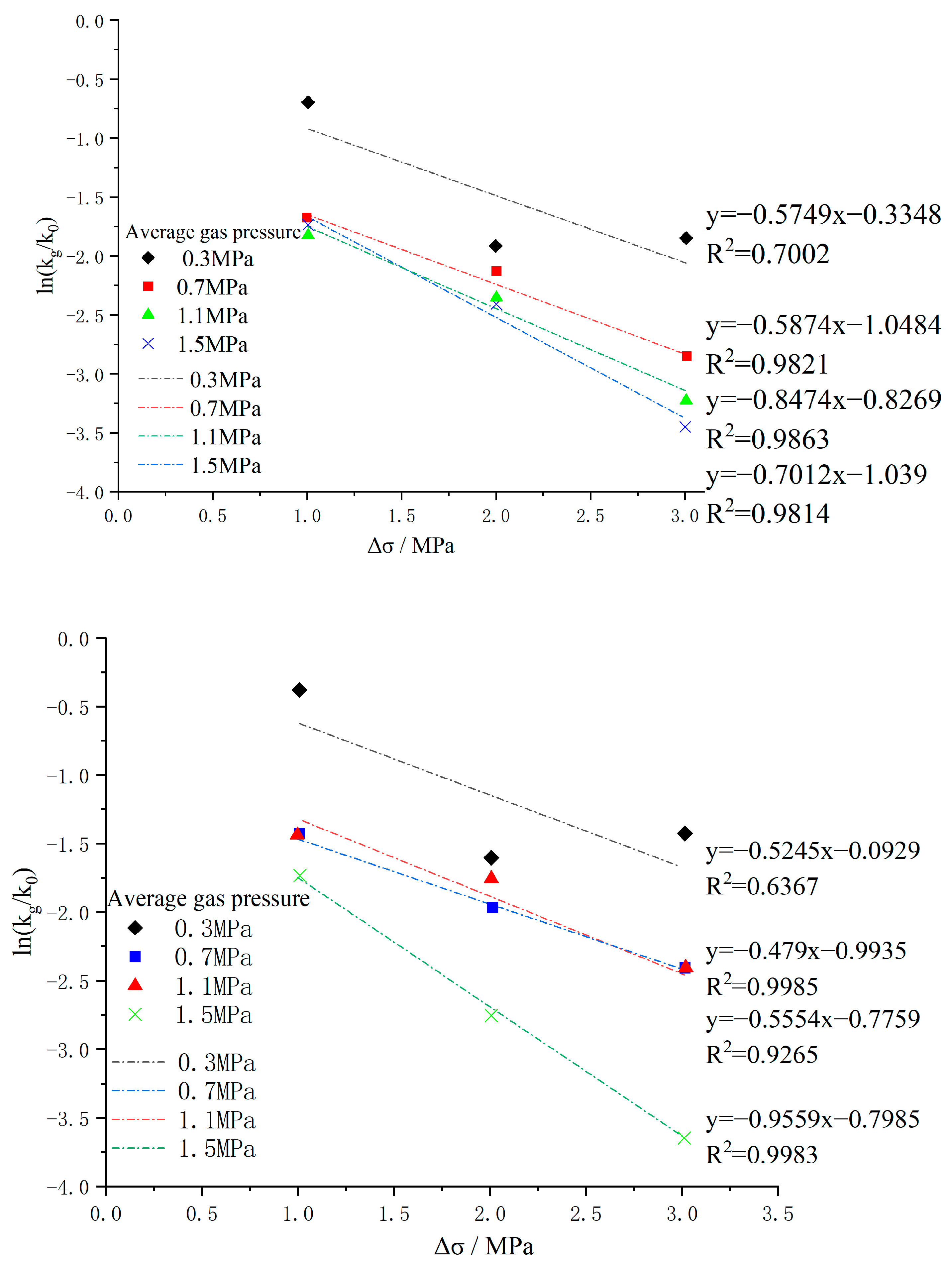
4.2. Model of Gas Slippage Effect and Effective Stress-Controlled Permeability
4.3. Model for Controlling Permeability by Slippage Effect and Matrix Shrinkage Effect
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bai, M.; Elsworth, D. Coupled processes in subsurface deformation. ASCE Press Flow Transp. 2000, 336–337. [Google Scholar]
- Elsworth, D.; Bai, M. Flow-deformation response of dual porosity media. Geotech. Eng. 1992, 118, 107–124. [Google Scholar] [CrossRef]
- Sun, F.; Liu, D.; Cai, Y.; Qiu, Y. Coal rank-pressure coupling control mechanism on gas adsorption/desorption in coalbed methane reservoirs. Energy 2023, 270, 126849. [Google Scholar] [CrossRef]
- Sun, F.; Liu, D.; Cai, Y.; Qiu, Y. A micro-macro coupled permeability model for gas transport in coalbed methane reservoirs. Energy 2023, 284, 128604. [Google Scholar] [CrossRef]
- Liu, D.; Yao, Y.; Chang, Y. Measurement of adsorption phase densities with respect to different pressure: Potential application for determination of free and adsorbed methane in coalbed methane reservoir. Chem. Eng. J. 2022, 446 Pt 3, 137103. [Google Scholar] [CrossRef]
- Harpalani, S.; Schraufnagel, R.A. Shrinkage of coal matrix with release of gas and its impact on permeability of coal. Fuel 1990, 69, 551–556. [Google Scholar] [CrossRef]
- Seidle, J.P.; Huitt, L.G. Experimental measurement of coal matrix shrinkage due to gas desorption and implications for cleat permeability increases. In Proceedings of the International Meeting on Petroleum Engineering, v 2. Richardson: Soc of Petroleum Engineers, Beijing, China, 14–17 November 1995; pp. 575–582. [Google Scholar]
- Bustin, R.M. Importance of fabric and composition on the stress sensitivity of permeability in some coals, northern Sydney basin: Relevance to coalbed methane exploitation. AAPG Bull. 1997, 81, 1894–1908. [Google Scholar]
- Fu, X.H.; Li, D.H.; Qin, Y.; Song, X. Experimental study on the effect of coal matrix shrinkage on permeability. J. China Univ. Min. Technol. 2002, 31, 129–131. [Google Scholar]
- Fu, X.H.; Qin, Y.; Zhang, W.H. Analysis of the coupling relationship between the mechanical effects of high rank coal matrix and coal reservoir permeability. J. Univ. Geol. 2003, 9, 373–377. [Google Scholar]
- Somerton, W.H.; Soylemezoglu, I.M.; Dudley, R.C. Effect of stress on permeability of coal. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1975, 12, 129–145. [Google Scholar] [CrossRef]
- Clarkson, C.R.; Jordan, C.L.; Gierhart, R.; Seidle, J.P. Production data analysis of CBM wells. Soc. Pet. Eng. 2007. [Google Scholar] [CrossRef]
- Qu, P.; Shen, R.C.; Yuan, J.P. Theoretical study on stress sensitivity of coal reservoirs. Pet. Drill. Tech. 2007, 35, 68–71. [Google Scholar]
- Palmer, I.; Mansoori, J. How permeability depends on stress and pore pressure in coalbeds: A New Model. Spe Reservoir. Eval. Eng. 1998, 1, 539–544. [Google Scholar] [CrossRef]
- Shi, J.Q.; Durucan, S. Drawdown induced changes in permeability of coalbeds: A New Interpretation of the Reservoir Response to Primary Recovery. Transp. Porous Media 2004, 56, 1–16. [Google Scholar] [CrossRef]
- Li, Q.M. Research on the Seepage Characteristics of Coal and Rock Damage under Multiple Protection Conditions and the Rationality Evaluation of Mining Deployment; Chongqing University: Chongqing, China, 2019. [Google Scholar]
- Shao, Z.B.; Zhang, H.; Zhong, Y.; Li, G.F.; She, J.P.; Li, Z.Z. Study on liquid phase detention and stress sensitive superimposed damage of tight sandstone gas reservoirs with multiple fractures: Taking the tight sandstone of Penglaizhen formation in western Sichuan as an example. J. Xi’an Pet. Univ. 2015, 30, 59–62. [Google Scholar]
- Meng, Z.P.; Hou, Q.L. Experimental analysis of stress sensitivity and influencing factors in coal reservoirs. J. Coal Sci. 2012, 37, 430–437. [Google Scholar]
- Yang, M.P.; Li, Y.; Li, Z.P. Experimental study on stress sensitivity of gas reservoirs rocks containing bound water. Nat. Gas Geosci. 2004, 15, 227–229. [Google Scholar]
- Chen, Z.H.; Wang, Y.B.; Guo, K. Study on stress sensitivity of high rank coalbed methane reservoirs. J. Geol. 2008, 82, 1390–1395. [Google Scholar]
- Li, X.C.; Kang, Y.L.; Luo, P.Y. The effect of stress on the width and permeability of coal and rock fractures. Coal Field Geol. Explor. 2009, 37, 29–37. [Google Scholar]
- Fu, Y.K.; Xie, B.J.; Wang, Q.F. Dynamic mechanical constitutive model of coal. J. Coal Sci. 2013, 38, 1769–1774. [Google Scholar]
- Liu, X.H.; Zhang, R.; Liu, J.F. Experimental study on coal rock impact dynamics under different strain rates. J. Coal Sci. 2012, 1528–1534. [Google Scholar]
- Wei, J.P.; Li, M.Z.; Wang, D.K.; Qin, H.J. Experimental study on sensitivity of coal sample permeability to confining pressure. Coal Sci. Technol. 2014, 42, 76–80. [Google Scholar]
- Shang, H.B.; Jin, D.W.; Zhang, T.J.; Tang, J. Evolution law of permeability characteristics of fractured coal under triaxial stress. J. Coal Sci. 2019, 044, 1066–1075. [Google Scholar]
- Wang, D.K.; Zeng, F.C.; Wei, J.P.; Zhang, H.T.; Wu, Y.; Wei, Q. Quantitative analysis of fracture dynamic evolution in coal subjected to uniaxial and triaxial compression loads based on industrial CT and fractal theory. J. Pet. Sci. Eng. 2021, 196, 108051. [Google Scholar] [CrossRef]
- Shi, J.; Feng, Z.; Zhou, D.; Meng, Q. Analysis of the permeability evolution law of in situ steam pyrolysis of bituminous coal combing with in situ CT technology. Energy 2023, 263, 126009. [Google Scholar] [CrossRef]
- He, J.; Li, H.; Yang, W.; Lu, J.; Lu, Y.; Liu, T.; Shi, S. Experimental study on erosion mechanism and pore structure evolution of bituminous and anthracite coal under matrix acidification and its significance to coalbed methane recovery. Energy 2023, 283, 128485. [Google Scholar] [CrossRef]
- Li, X.; Tian, J.; Ju, Y.; Chen, Y. Permeability variations of lignite and bituminous coals under elevated pyrolysis temperatures (35–600 °C): An experimental study. Energy 2022, 254, 124187. [Google Scholar] [CrossRef]
- Li, Y.; Ji, H.; Li, G.; Hu, S.; Liu, X. Effect of supercritical CO2 transient high-pressure fracturing on bituminous coal microstructure. Energy 2023, 282, 128975. [Google Scholar] [CrossRef]
- Huang, H.; Wang, E. A laboratory investigation of the impact of solvent treatment on the permeability of bituminous coal from Western Canada with a focus on microbial in-situ processing of coals. Energy 2020, 210, 118542. [Google Scholar] [CrossRef]
- Wang, D.K.; Tian, X.R.; Wei, J.P.; Zhang, H.T.; Yao, B.H.; Zhang, H.; Chen, C.Y. Fracture evolution and nonlinear seepage characteristic of gas-bearing coal using X-ray computed tomography and the lattice Boltzmann method. J. Pet. Sci. Eng. 2022, 211, 110144. [Google Scholar] [CrossRef]
- Wu, Y.; Wang, D.K.; Wang, L.; Shang, Z.; Zhu, C.; Wei, J.; Yuan, A.; Zhang, H.; Zeng, F. An analysis of the meso-structural damage evolution of coal using X-ray CT and a gray-scale level co-occurrence matrix method. Int. J. Rock Mech. Min. Sci. 2022, 152, 105062. [Google Scholar] [CrossRef]
- Wu, Y.; Wang, D.K.; Wang Wei, J.P.; Yao, B.; Zhang, H.; Fu, J.; Zeng, F. Damage constitutive model of gas-bearing coal using industrial CT scanning technology. J. Nat. Gas Sci. Eng. 2022, 101, 104543. [Google Scholar] [CrossRef]
- Song, B.; Zhai, X.; Ma, T.; Wang, B.; Hao, L.; Zhou, Y. Effect of water immersion on pore structure of bituminous coal with different metamorphic degrees. Energy 2023, 274, 127449. [Google Scholar] [CrossRef]
- Su, E.; Liang, Y.; Chen, X.; Wang, Z.; Ni, X.; Zou, Q.; Chen, H.; Wei, J. Relationship between pore structure and mechanical properties of bituminous coal under sub-critical and super-critical CO2 treatment. Energy 2023, 280, 128155. [Google Scholar] [CrossRef]
- Liu, D.M.; Jia, Q.F.; Cai, Y.D.; Gao, C.J.; Qiu, F.; Zhao, Z.; Chen, S.Y. A new insight into coalbed methane occurrence and accumulation in the Qinshui Basin, China. Gondwana Res. 2022, 111, 280–297. [Google Scholar] [CrossRef]
- Liu, D.M.; Yao, Y.B.; Wang, H. Structural compartmentalization and its relationships with gas accumulation and gas production in the Zhengzhuang Field, southern Qinshui Basin. Int. J. Coal Geol. 2022, 259, 104055. [Google Scholar] [CrossRef]
- Liu, D.M.; Yao, Y.B.; Yuan, X.H.; Yang, Y.H. Experimental evaluation of the dynamic water-blocking effect in coalbed methane reservoir. J. Pet. Sci. Eng. 2022, 217, 110887. [Google Scholar] [CrossRef]
- Randolph, P.L.; Soeder, D.J.; Chowdiah, P. Porosity and permeability of tight sands. In Spe Unconventional Gas Recovery Symposium; OnePetro: Richardson, TX, USA, 1984. [Google Scholar]
- Zhao, Y.S.; Hu, Y.Q.; Wei, J.P.; Yang, D. The experimental approach to effective stress law of coal mass by effect of methane. Transp. Porous Media 2003, 53, 235–244. [Google Scholar]
- Li, J.Q.; Liu, D.M.; Yao, Y.B.; Cai, Y.D.; Wang, L. The control effect of gas slippage and effective stress on the gas phase permeability of coal and rock. Nat. Gas Geosci. 2013, 24, 1074–1078. [Google Scholar]
- Shi, J.; Durucan, S. A model for changes in coalbed permeability during primary and enhanced methane recovery. SPE Reserv. Eval. Eng. 2005, 8, 291–299. [Google Scholar] [CrossRef]
- Klinkenberg, L.J. The permeability of porous media to liquids and gases. Drill. Prod. Pract. 1941, 2, 200–213. [Google Scholar] [CrossRef]
- Li, J.; Liu, D.; Yao, Y.; Cai, Y.; Chen, Y. Evaluation and modeling of gas permeability changes in anthracite coals. Fuel 2013, 111, 606–612. [Google Scholar] [CrossRef]

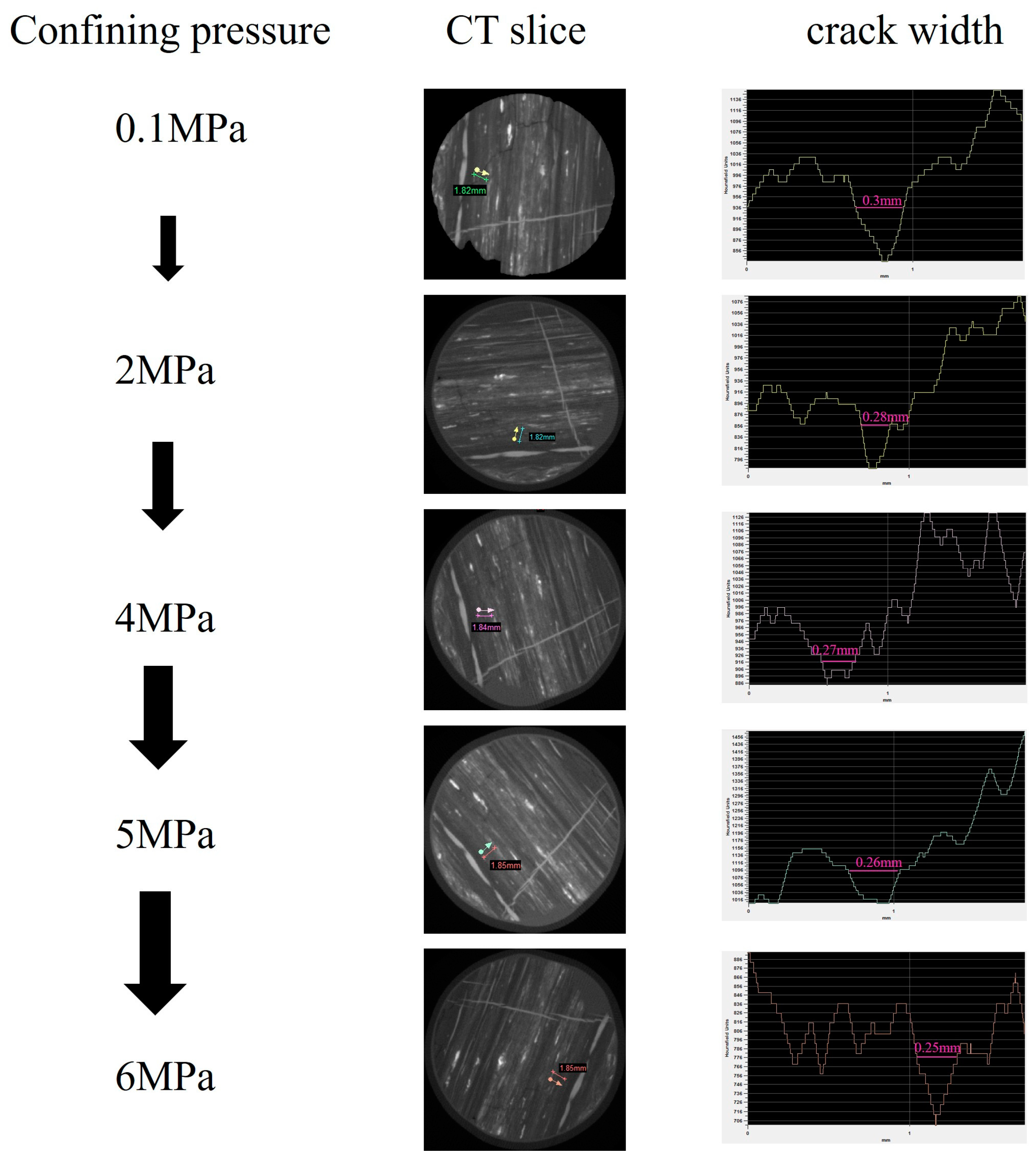

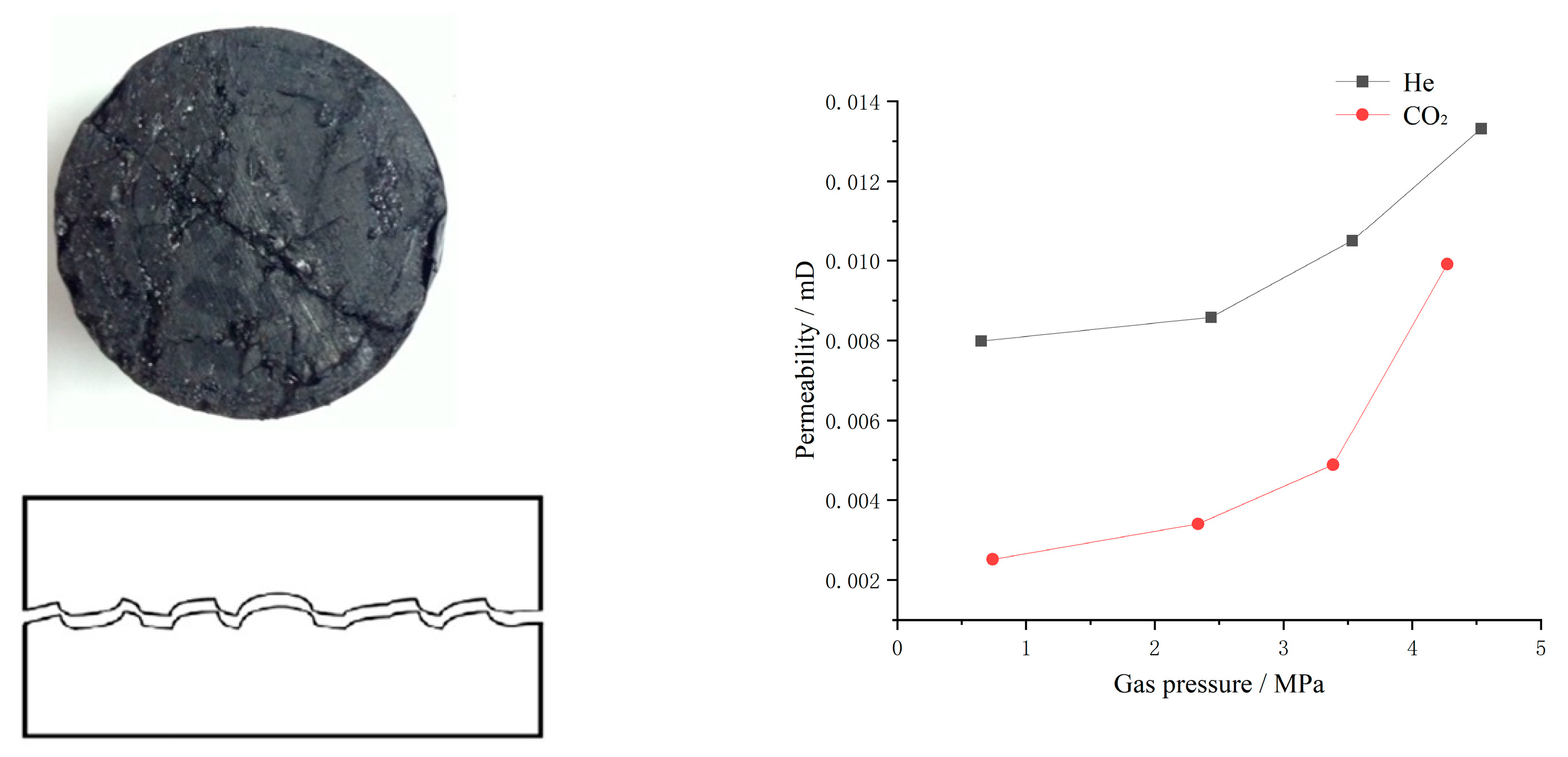
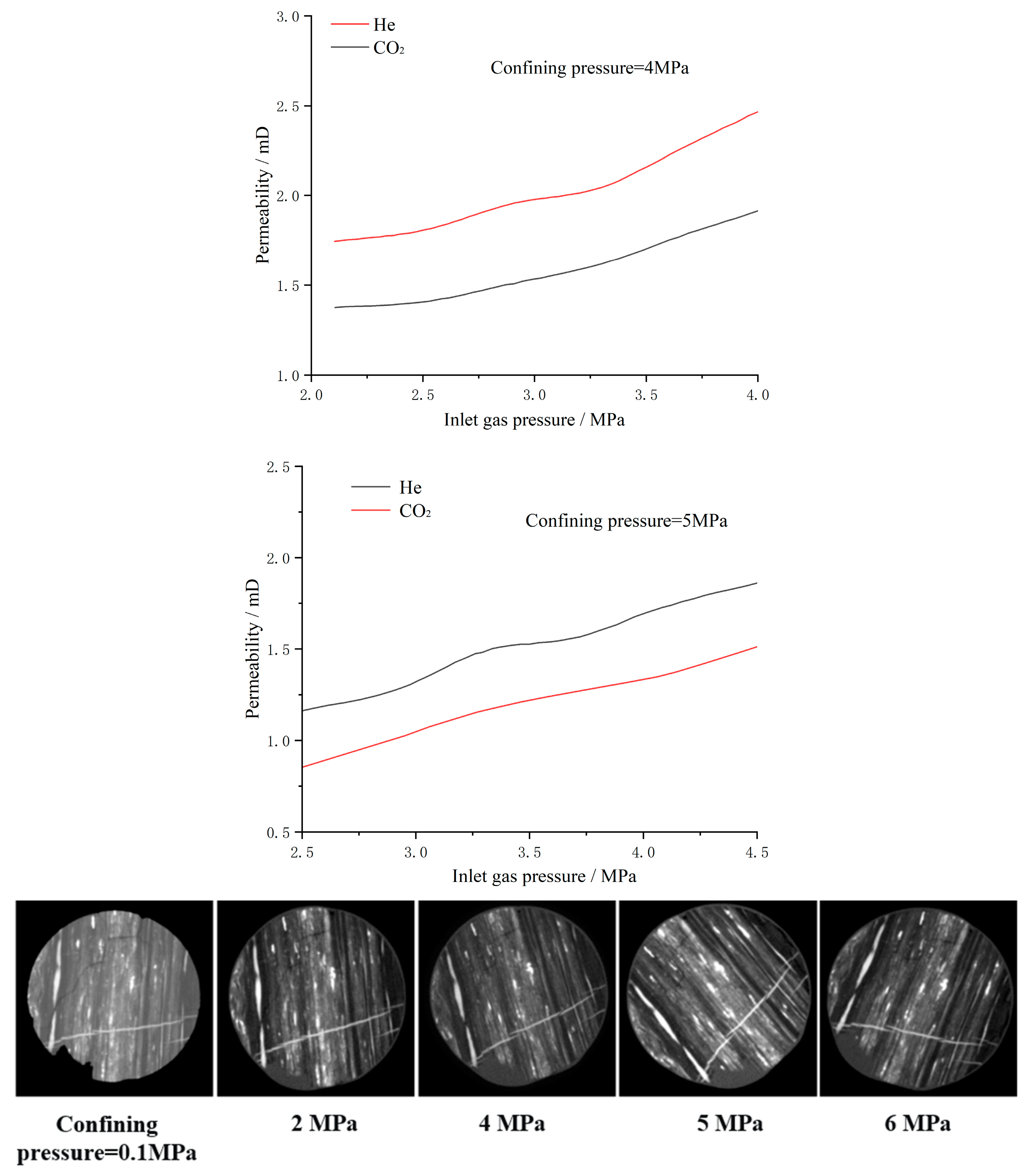

| Sampling Point | Seam No. | Sample Number | Serial Number | Length/cm | Diameter/cm | Ro (%) | Initial Permeability ×10−3 μm2 | |
|---|---|---|---|---|---|---|---|---|
| Han Cheng | Xiang Shan | 5 | XS5 | 1 | 3.44 | 2.53 | 2.45 | 0.04 |
| XS5-1 | 6 | 2.52 | 2.54 | 2.45 | -- | |||
| Xiayu Kou | 11 | XYK11 | 2 | 2.83 | 2.55 | 1.70 | 3.76 | |
| Sangshu Ping | 3 | SSP3-1 | 3 | 2.61 | 2.54 | 2.24 | 0.26 | |
| He Yang | He Yan 2# | 5 | HYR5-1 | 4 | 4.13 | 2.51 | 2.69 | 0.66 |
| Cheng Cheng | San yan Bridge | 5 | SYQ5-1 | 5 | 5.23 | 2.54 | 2.40 | 0.66 |
| Average Gas Pressure pm/MPa | Fitting Relationship between Permeability and Effective Stress | R2 | Cleat Compression Coefficient c/MPa−1 | Initial Effective Stress σ0/MPa | Initial Permeability k0/μD | Permeability Model for Effective Stress Control ke/μD |
|---|---|---|---|---|---|---|
| 0.3 (He) | y = 5.1831 × 10−0.675x | 0.878 | 0.192 | 2 | 1.48 | ke = 1.48 × 10−0.575(σ−2) |
| 0.3 (CO2) | y = 2.2481 × 10−0.552x | 0.828 | 0.175 | 2 | 0.77 | ke = 0.77 × 10−0.525(σ−2) |
| 0.7 (He) | y = 4.2085 × 10−0.902x | 0.922 | 0.196 | 2 | 0.95 | ke = 0.95 × 10−0.587(σ−2) |
| 0.7 (CO2) | y = 2.1535 × 10−0.777x | 0.911 | 0.185 | 2 | 0.61 | ke = 0.61 × 10−0.555(σ−2) |
| 1.1 (He) | y = 5.6693 × 10−1.013x | 0.937 | 0.233 | 2 | 1.02 | ke = 1.02 × 10−0.701(σ−2) |
| 1.1 (CO2) | y = 2.0973 × 10−0.788x | 0.931 | 0.158 | 2 | 0.55 | ke = 0.55 × 10−0.479(σ−2) |
| 1.5 (He) | y = 7.9706 × 10−1.096x | 0.964 | 0.283 | 2 | 1.14 | ke = 1.14 × 10−0.847(σ−2) |
| 1.5 (CO2) | y = 9.818 × 10−1.195x | 0.974 | 0.319 | 2 | 1.14 | ke = 1.14 × 10−0.956(σ−2) |
| Effective Stress σe/MPa | Fitting Relationship between Permeability and Reciprocal Gas Pressure | R2 | Kjeldahl Permeability k0/μD | Slippage Factor b/MPa | Permeability Model Controlled by Slippage Effect ∆ksli/μD |
|---|---|---|---|---|---|
| 2 | y = 0.2777x + 0.5637 | 0.997 | 0.5637 | 0.4926 | ∆ksli = 0.2777/pm |
| 4 | y = 0.0486x + 0.0475 | 0.922 | 0.0475 | 1.023 | ∆ksli = 0.0486/pm |
| Effective Stress σe/MPa | Kjeldahl Permeability k0/μD | Slippage Factor /MPa | Permeability Model Controlled by Slippage Effect ∆ksli/μD | Permeability Model Controlled by Matrix Shrinkage Effect ∆kshr/μD |
|---|---|---|---|---|
| 2 | 0.5637 | 0.0794 | ∆ksli = 0.0447/pm | ∆kshr = kgC − 0.5637 − 0.0447/pm |
| 4 | 0.0475 | 0.1648 | ∆ksli = 0.0078/pm | ∆kshr = kgC − 0.0475 − 0.0078/pm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, Y.; Chang, D.; Sun, F.; Abuduerxiti, A.; Cai, Y. Permeability Evolution of Bituminous Coal and Its Dynamic Control, a Case Study from the Southeastern Ordos Basin, China. Energies 2023, 16, 8046. https://doi.org/10.3390/en16248046
Qiu Y, Chang D, Sun F, Abuduerxiti A, Cai Y. Permeability Evolution of Bituminous Coal and Its Dynamic Control, a Case Study from the Southeastern Ordos Basin, China. Energies. 2023; 16(24):8046. https://doi.org/10.3390/en16248046
Chicago/Turabian StyleQiu, Yongkai, Dingjun Chang, Fengrui Sun, Abulaitijiang Abuduerxiti, and Yidong Cai. 2023. "Permeability Evolution of Bituminous Coal and Its Dynamic Control, a Case Study from the Southeastern Ordos Basin, China" Energies 16, no. 24: 8046. https://doi.org/10.3390/en16248046
APA StyleQiu, Y., Chang, D., Sun, F., Abuduerxiti, A., & Cai, Y. (2023). Permeability Evolution of Bituminous Coal and Its Dynamic Control, a Case Study from the Southeastern Ordos Basin, China. Energies, 16(24), 8046. https://doi.org/10.3390/en16248046












