An Investigation on the Effect of Watertightness Criteria on the Structural Assessment of Prestressed Concrete Substructures of Floating Wind Turbines
Abstract
:1. Introduction
2. Design Basis for the Concrete Floater
2.1. Design Philosophy and Guidelines
2.2. Metocean Data and the Design Load Case
2.3. Mounted Wind Turbine
2.4. Material and Safety Factors
2.5. Design Load Cases
2.5.1. Ultimate Limit States
2.5.2. Fatigue Limit States
2.5.3. Serviceability Limit States
3. Analysis Methods
3.1. Dynamic Analysis of the Floating Wind Turbine
3.2. Structural Assessment
3.2.1. Ultimate Limit States
3.2.2. Fatigue Limit States
3.2.3. Serviceability Limit States and the Design of the Prestressing Steel
4. Design of the Concrete Floater
4.1. Floater Geometries
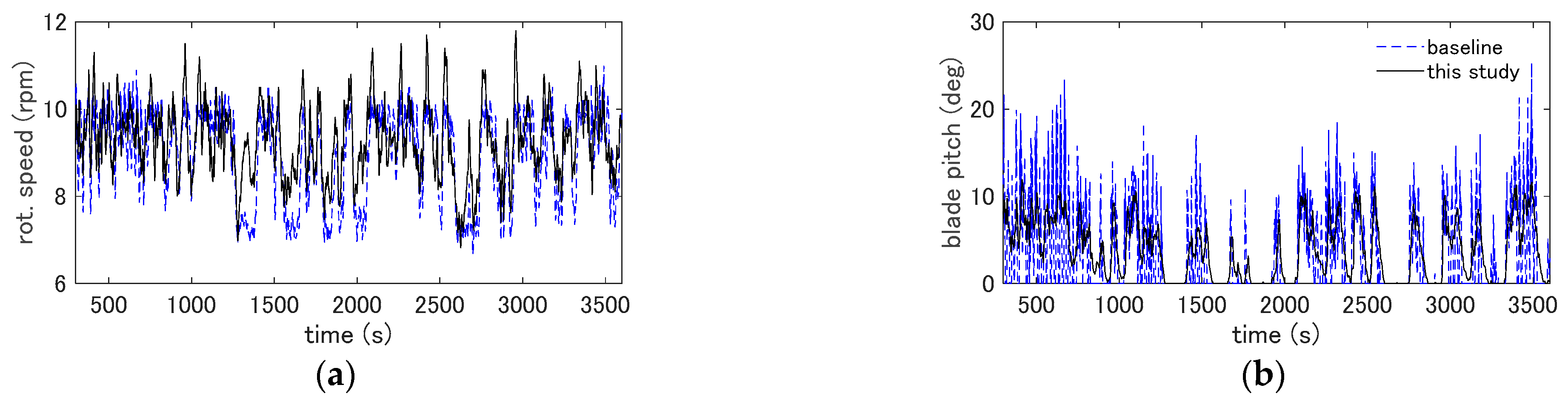
4.2. Dynamic Response
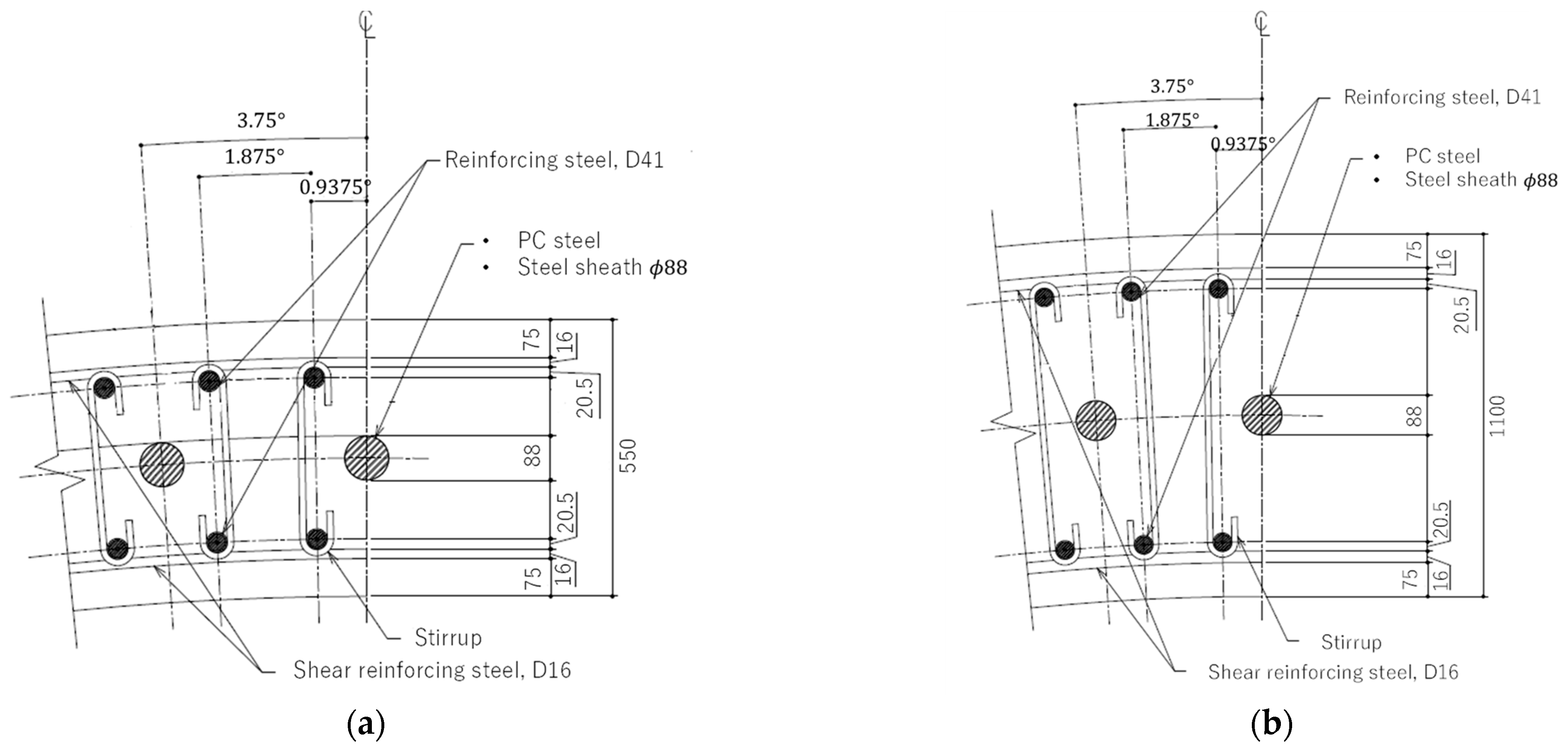
4.3. Structural Design of the Floater Members
5. Effect of Prestressing Design on Structural Assessment Results
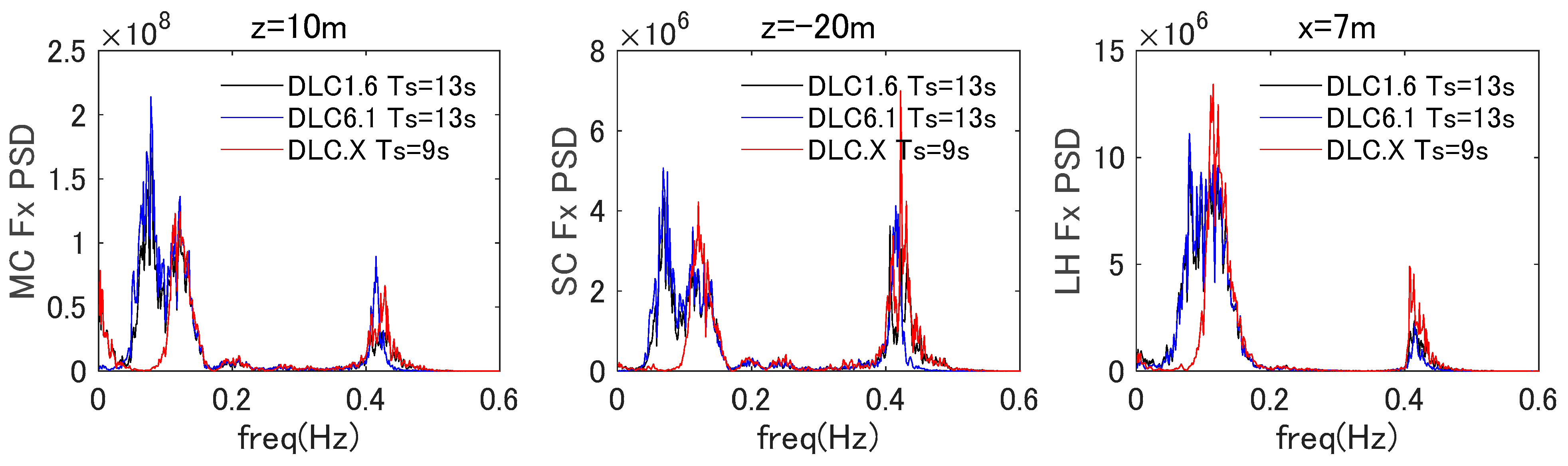
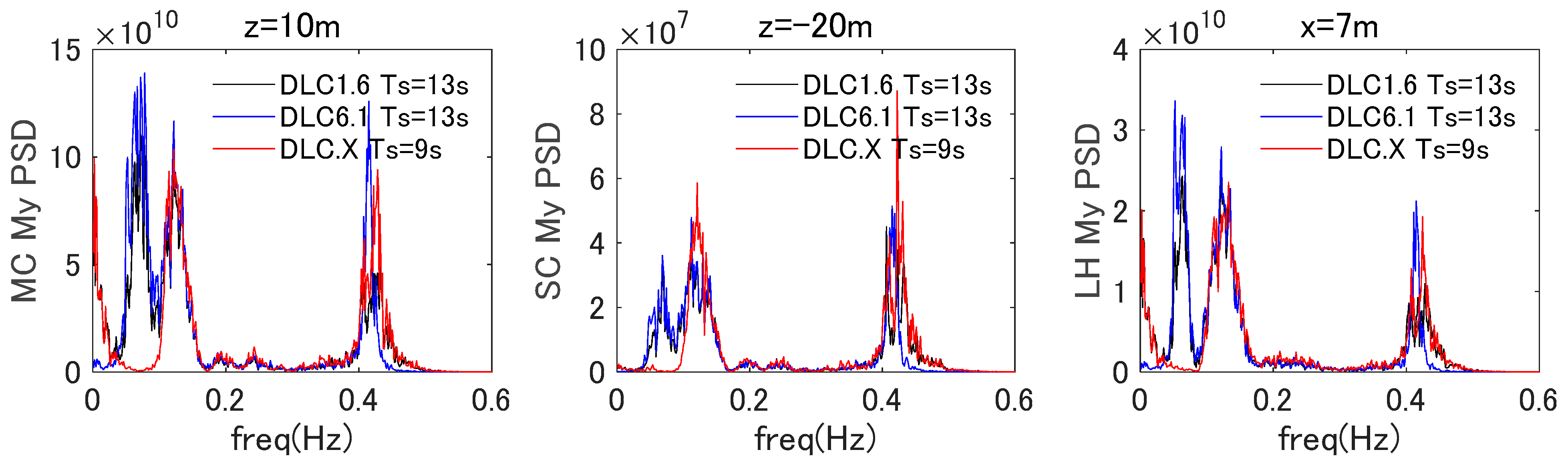
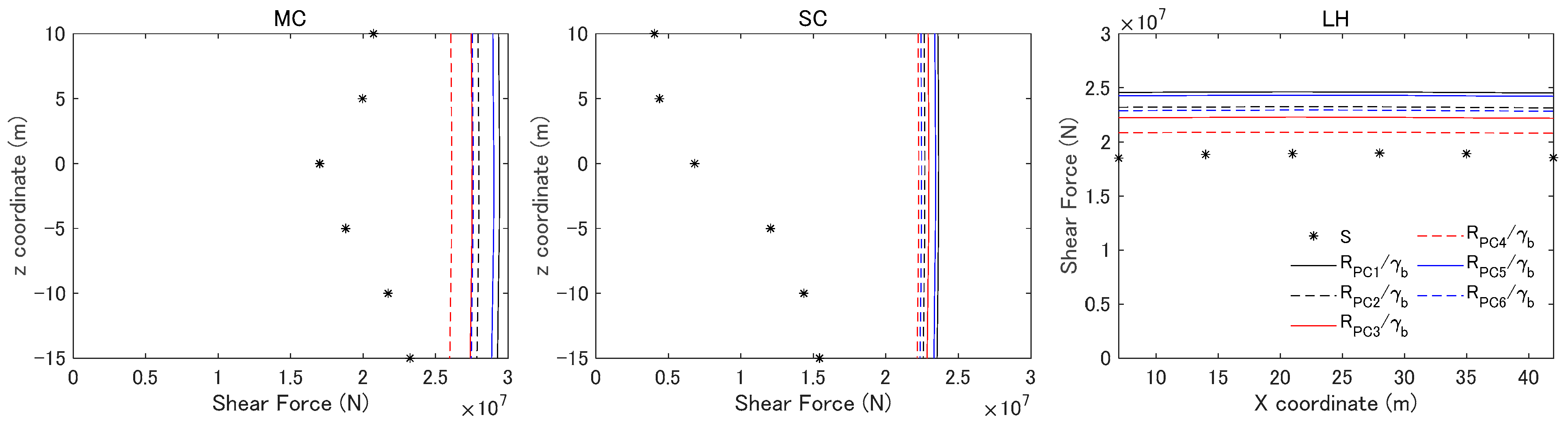
5.1. Ultimate Limit States
5.2. Fatigue Limit States
6. Conclusions
- The different assessment conditions for watertightness resulted in different designs of prestressing tension, which also caused differences in the design margins for the ultimate limit states as well as the SN diagram used for the assessment of the fatigue limit states.
- The maximum stress acting on the member of the floating wind turbine differed by about 7.6 to 16.2% for the 50-year and 1-year environmental conditions. The small difference between the stress for the two return periods can be attributed to the large component of the wind turbine thrust in the variable loads.
- When the partial load factor of 1.0 is used, the structural requirement in the prestressing design caused larger differences compared to the return period of the loading conditions in the watertightness assessment. The use of the partial load factor of 0.5 for the prestressing design also caused a large difference in the results of the structural assessment, indicating the necessity of further investigation of the determination of the partial load factor for floating offshore wind turbines.
Funding
Data Availability Statement
Conflicts of Interest
References
- EN 1992-1-1; Eurocode 2: Design of Concrete Structures—Part 1-1: General Ruels and Rules for Buildings. British Standards Institution: London, UK, 2005.
- Japan Society of Civil Engineers. Standard Specifications for Concrete Structures [Design]; Japan Society of Civil Engineers: Tokyo, Japan, 2017. [Google Scholar]
- DNVGL-ST-C502; Offshore Concrete Structures. DNV GL: Calgary, Canada, 2018.
- Viselli, A.M.; Goupee, A.J.; Dagher, H.J.; Allen, C.K. Design and model confirmation of the intermediate scale VolturnUS floating wind turbine subjected to its extreme design conditions offshore. Wind Energy 2016, 19, 1161–1177. [Google Scholar] [CrossRef]
- Pegalajar-Jurado, A.; Bredmose, H.; Borg, M.; Straume, J.G.; Landbø, T.; Andersen, H.S.; Yu, W.; Müller, K.; Lemmer, F. State-of-the-art model for the LIFES50+ OO-Star Wind Floater Semi 10MW floating wind turbine. J. Phys. Conf. Ser. 2018, 1104, 012024. [Google Scholar] [CrossRef]
- Campos, A.; Molins, C.; Gironella, X.; Trubat, P. Spar concrete monolithic design for offshore wind turbines. Marit. Eng. 2016, 169, 49–63. [Google Scholar] [CrossRef]
- Walia, D.; Schunemann, P.; Kuhl, M.; Adam, F.; Hartmann, H.; Großmann, J.; Ritschel, U. Prestressed ultra high performance concrete members for a TLP substructure for floating wind turbines. In Proceedings of the 27th International Ocean and Polar Engineering Conference, San Francisco, CA, USA, 25–30 June 2017. [Google Scholar]
- IEC61400-3-1; Design Requirements for Fixed Offshore Wind Turbines. International Electrotechnical Commission: Geneva, Switzerland, 2019.
- Ishihara, T.; Shimada, K.; Imakita, A. Metocean design condition for “Fukushima FORWARD” project. In Proceedings of the Grand Renewable Energy 2014, Tokyo, Japan, 30 July–1 August 2014. [Google Scholar]
- New Energy and Industrial Technology Development Organization. Accomplishment Report/Next Generation Floating Offshore Wind Power Generation System Demonstration Study (Semi-Sub Type); New Energy and Industrial Technology Development Organization: Tokyo, Japan, 2016. [Google Scholar]
- NeoWinds. Available online: https://appwdc1.infoc.nedo.go.jp/Nedo_Webgis/top.html (accessed on 23 October 2023).
- Bak, C.; Zahle, F.; Bitsche, R.; Kim, T.; Yde, A.; Henriksen, L.C.; Natarajan, A.; Hansen, M.H. Description of the DTU 10MW Reference Wind Turbine; DTU Wind Energy Report I-0092; Technical University of Denmark: Roskilde, Denmark, 2013. [Google Scholar]
- Hansen, M.H.; Henriksen, L.C. Basic DTU Wind Energy Controller; DTU Wind Energy Report; Technical University of Denmark: Roskilde, Denmark, 2013. [Google Scholar]
- Oh, S.; Lemmer, F.; Raach, S. A study on the effect of wind turbine controller on the sectional forces of a semi-sub floater. In Proceedings of the 43th Wind Energy Symposium, Tokyo, Japan, 18–19 November 2021. [Google Scholar]
- Lemmer, F.; Yu, W.; Schlipf, D.; Cheng, P.W. Robust gain scheduling baseline controller for floating offshore wind turbines. Wind Energy 2020, 23, 17–30. [Google Scholar] [CrossRef]
- Oh, S.; Ishii, K.; Iijima, K.; Suzuki, H. Implementation of potential flow hydrodynamics to time-domain analysis of flexible platforms of floating offshore wind turbines. J. Phys. Conf. Ser. 2019, 1356, 012041. [Google Scholar] [CrossRef]
- Jonkman, J.M. The New Modularization Framework for the FAST Wind Turbine CAE Tool. In Proceedings of the 51st AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, Dallas, TX, USA, 7–10 January 2013. [Google Scholar]
- Oh, S. Comparison of concrete and steel semi-submersible floaters for 10MW wind turbines. J. Phys. Conf. Ser. 2021, 2018, 012029. [Google Scholar] [CrossRef]








| Water Depth | 200 m | Reference turbulence | 0.12% |
| 10 min average wind speed, 50-year return period | 48.3 m/s (at 60 m height) | Significant wave height, 50-year return period | 11.71 m |
| Wind shear at extreme condition | 0.1 | Significant wave period, 50-year return period | 13.0 s |
| Annual mean wind speed | 7.34 m/s | Significant wave height, 1-year return period | 7.1 m |
| Wind shear at normal condition | 0.078 | Significant wave period, 1-year return period | 10.5 s |
| Weibull parameters for the long-term wind distribution | 1.87, 8.27 m/s |
| Hub height | 119 m | Hub mass | 105,520 m |
| Nacelle mass | 446,036 kg | Hub COG | (−7.073, 0, 119.0) |
| Nacelle COG | (2.687, 0, 118.08) | Tower mass | 628,442 kg |
| Blade mass (3 Blades) | 132148 kg | Tower COG | (0, 0, 63.56) |
| Height | Diameter (m) | Thickness (m) |
|---|---|---|
| 115.63 | 5.8 | 0.022 |
| 92 | 5.8 | 0.024 |
| 80.5 | 6.0 | 0.026 |
| 69 | 6.4 | 0.028 |
| 57.5 | 6.6 | 0.030 |
| 46 | 6.9 | 0.032 |
| 34.5 | 7.2 | 0.034 |
| 25 | 7.5 | 0.045 |
| 21 | 9.2 | 0.050 |
| 17 | 11 | 0.050 |
| 10 | 12 | 0.050 |
| Mean Wind Speed (m/s) | Turbulence Intensity (%) | Significant Wave Height (m) | Significant Wave Period (s) | |
|---|---|---|---|---|
| DLC. 1.6 | 11.4 | 14.9 | 11.7 | 12.0 |
| 13.0 | ||||
| 14.0 | 13.5 | 14.0 | ||
| DLC. 6.1 | 51.7 | 10.3 | 11.7 | 12.0 |
| 13.0 | ||||
| 14.0 |
| Mean Wind Speed (m/s) | Turbulence Intensity (%) | Significant Wave Height (m) | Significant Wave Period (s) |
|---|---|---|---|
| 11.40 | 14.90 | 7.0 | 8 |
| 9 | |||
| 15.00 | 13.48 | 10 |
| Material | Diameter | Wall Thickness | |
|---|---|---|---|
| MC | Concrete | 14 m | 0.55 m |
| SC | Concrete | 12 m | 0.55 m |
| LH | Concrete | 9.5 m | 1.1 m |
| Brace (Horizontal) | Steel | 3.5 m | 0.05 m |
| Brace (Diagonal) | Steel | 2.5 m | 0.04 m |
| Horizontal distance of anchor to platform centerline | 800 m | Mooring line weight in water | 224.789 kg/m |
| Initial line length | 850 m | Mooring line axial stiffness | 1,110,000,000 N |
| Reinforcing steel | D19@300, SD345 | Prestressing steel | 7 SWPR7BL, |
| Covering depth for reinforcing steel (mm) | 75 (MC, SC, LH) | Covering depth for PC steel (mm) | 231 (MC, SC) 506 (LH) |
| Load Case Return Period | Load Factor | Water Requirement | MC | SC | LH | Case Name | |||
|---|---|---|---|---|---|---|---|---|---|
| (N/mm2) | (N) | (N/mm2) | (N) | (N/mm2) | (N) | ||||
| 50 years (DLC 1.6 /DLC 6.1) | 1.0 | Fully Compressed | 7.22 | 2.47 × 108 | 3.90 | 1.13 × 108 | 8.81 | 3.76 × 108 | PC1 |
| Partially Compressed | 1.54 × 108 | 3.46 × 107 | 2.64 × 108 | PC2 | |||||
| 0.5 | Fully Compressed | 3.61 | 1.23 × 108 | 1.95 | 5.67 × 107 | 4.41 | 1.88 × 108 | PC3 | |
| Partially Compressed | 3.07 × 107 | 0 | 7.70 × 107 | PC4 | |||||
| 1-year (DLC X) | 1.0 | Fully Compressed | 6.46 | 2.21 × 108 | 3.27 | 9.51 × 107 | 8.14 | 3.51 × 108 | PC5 |
| Partially Compressed | 1.28 × 108 | 1.62 × 107 | 2.40 × 108 | PC6 | |||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oh, S. An Investigation on the Effect of Watertightness Criteria on the Structural Assessment of Prestressed Concrete Substructures of Floating Wind Turbines. Energies 2023, 16, 7992. https://doi.org/10.3390/en16247992
Oh S. An Investigation on the Effect of Watertightness Criteria on the Structural Assessment of Prestressed Concrete Substructures of Floating Wind Turbines. Energies. 2023; 16(24):7992. https://doi.org/10.3390/en16247992
Chicago/Turabian StyleOh, Sho. 2023. "An Investigation on the Effect of Watertightness Criteria on the Structural Assessment of Prestressed Concrete Substructures of Floating Wind Turbines" Energies 16, no. 24: 7992. https://doi.org/10.3390/en16247992
APA StyleOh, S. (2023). An Investigation on the Effect of Watertightness Criteria on the Structural Assessment of Prestressed Concrete Substructures of Floating Wind Turbines. Energies, 16(24), 7992. https://doi.org/10.3390/en16247992





