Zero-Voltage-Switching Analysis Model of the Triple-Active-Bridge Converter
Abstract
:1. Introduction
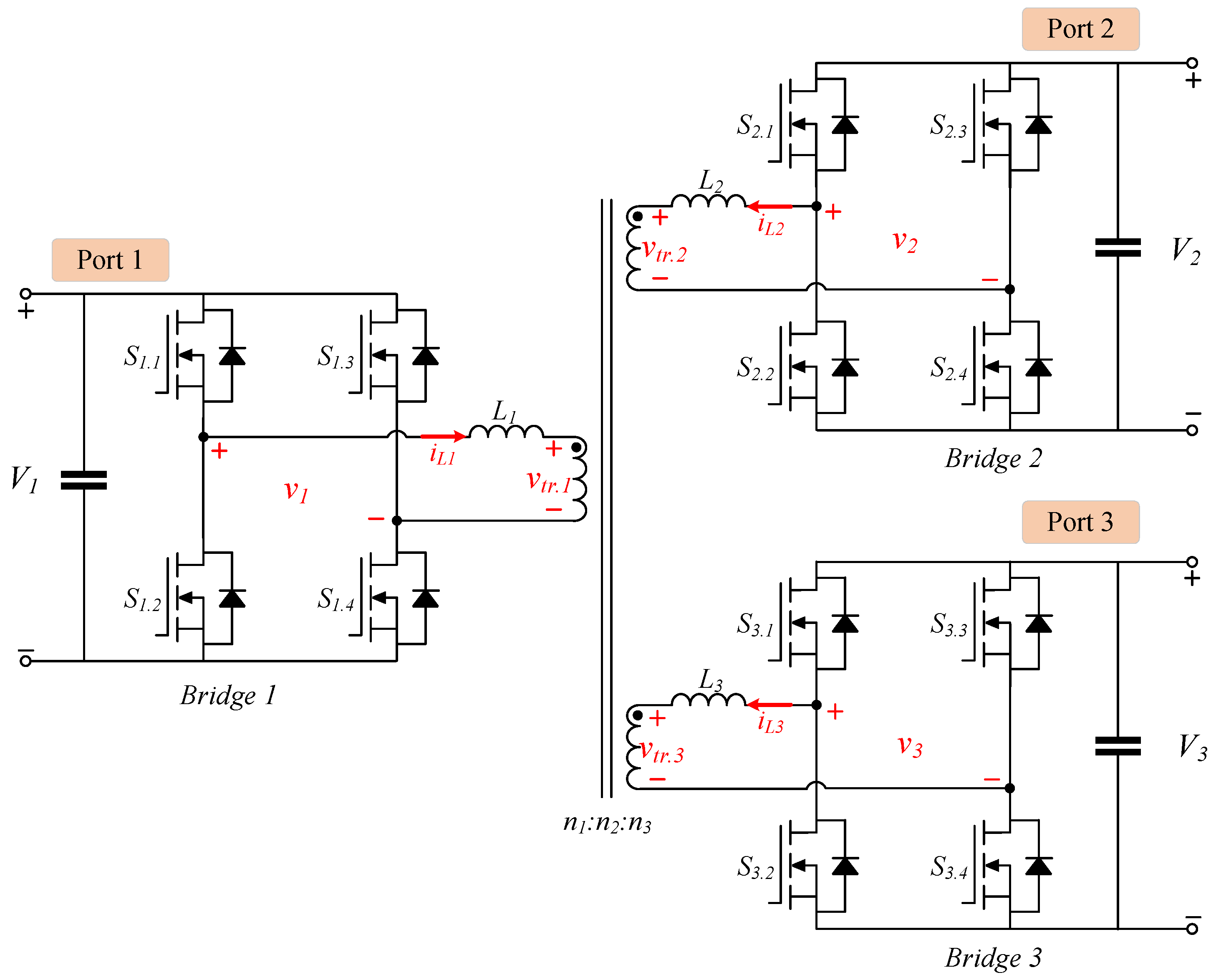
2. Operation and the GHA Modeling of the TAB Converter
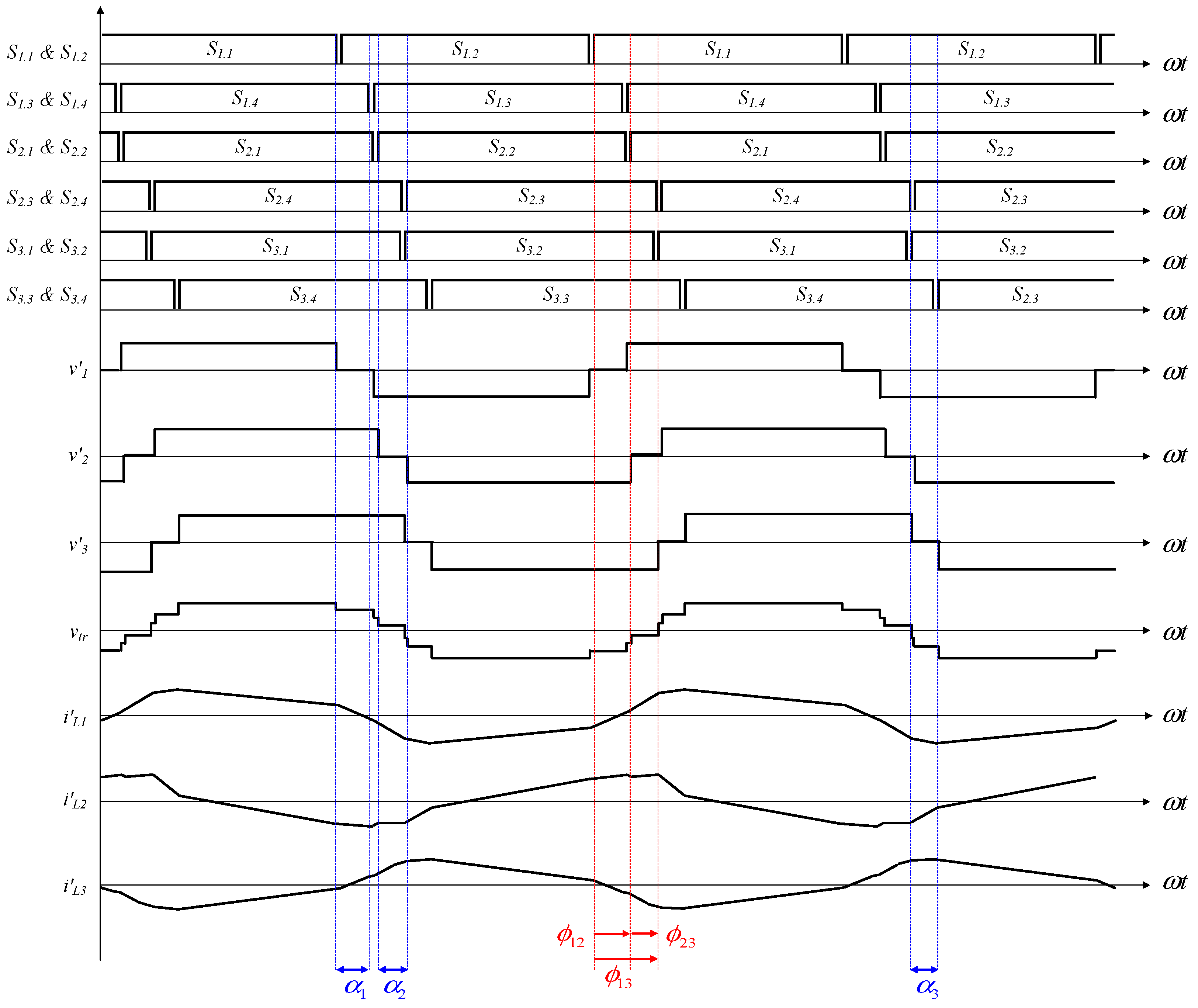
2.1. Operating Principles
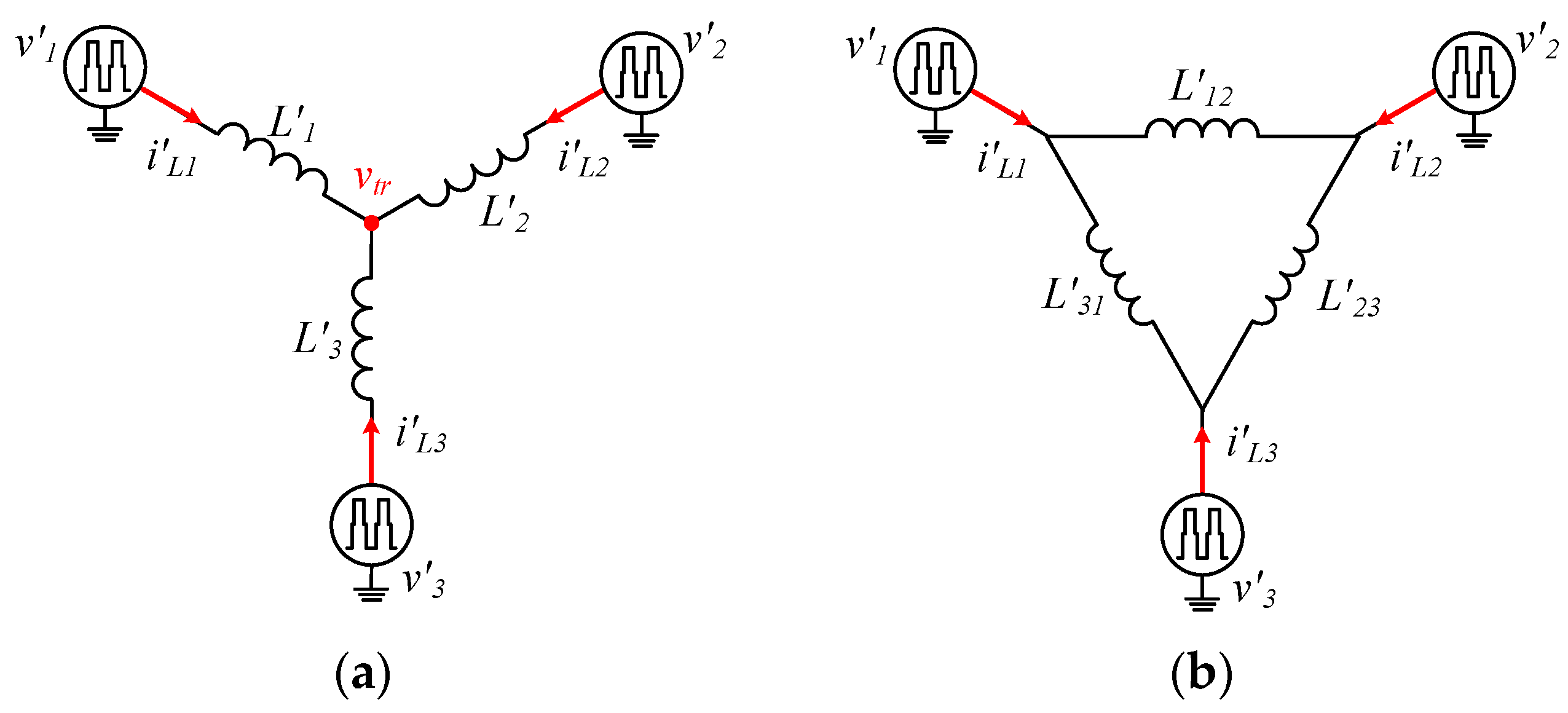
2.2. GHA Modeling
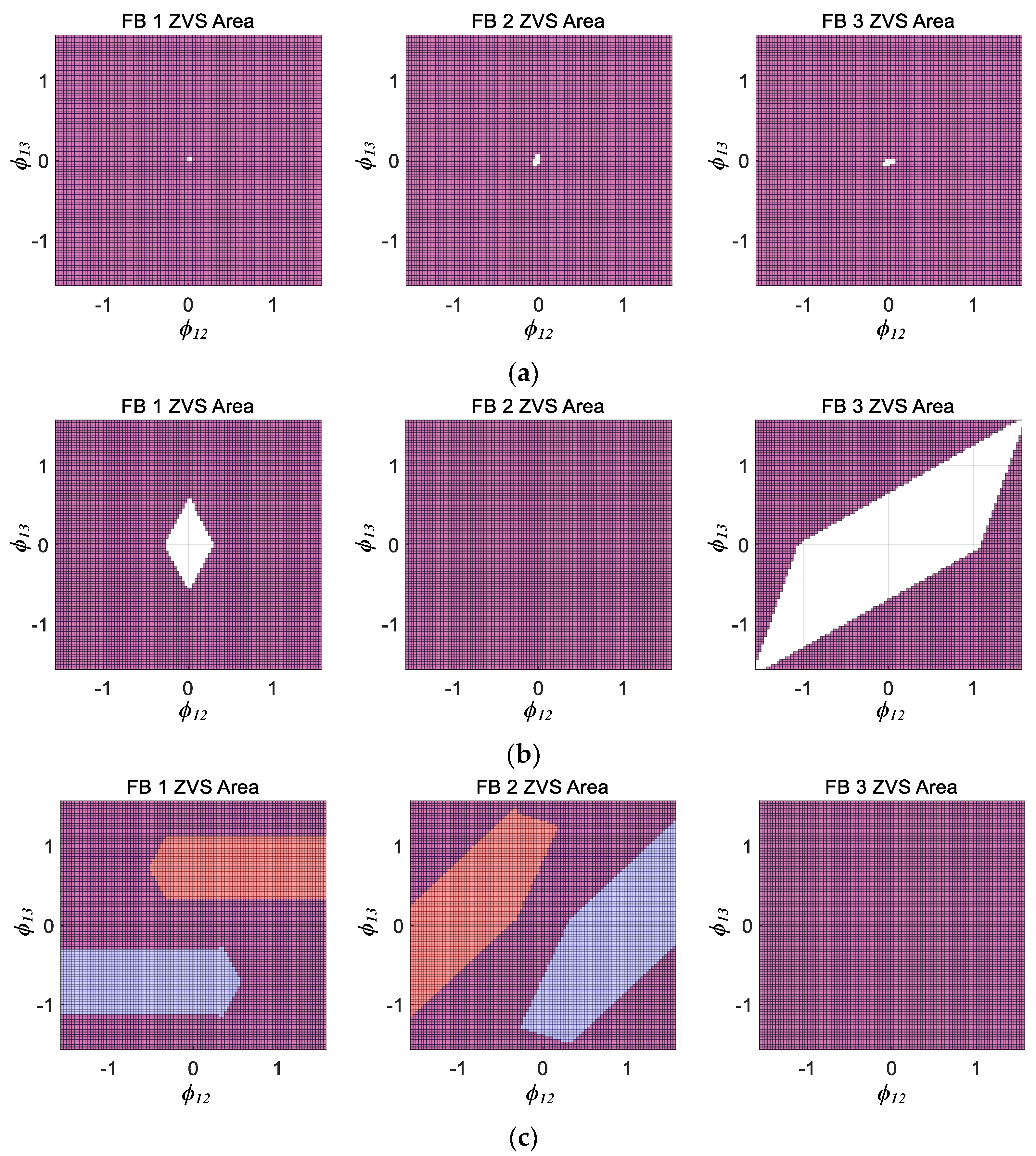
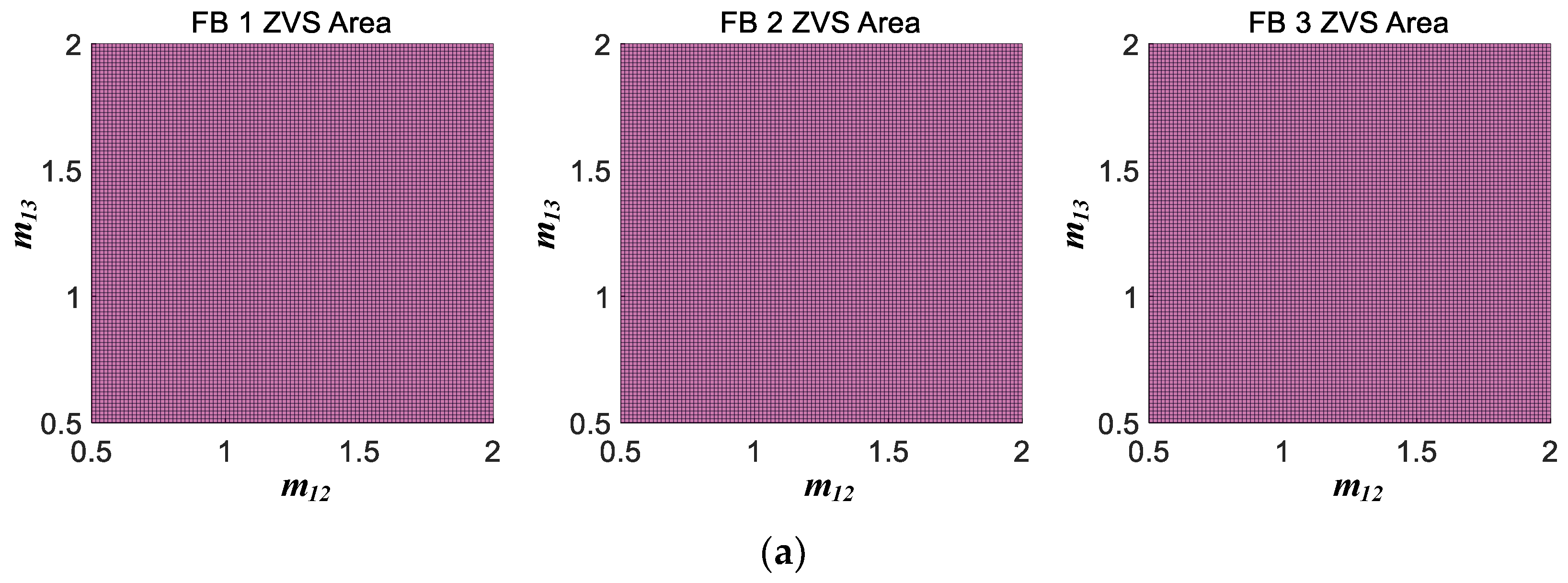
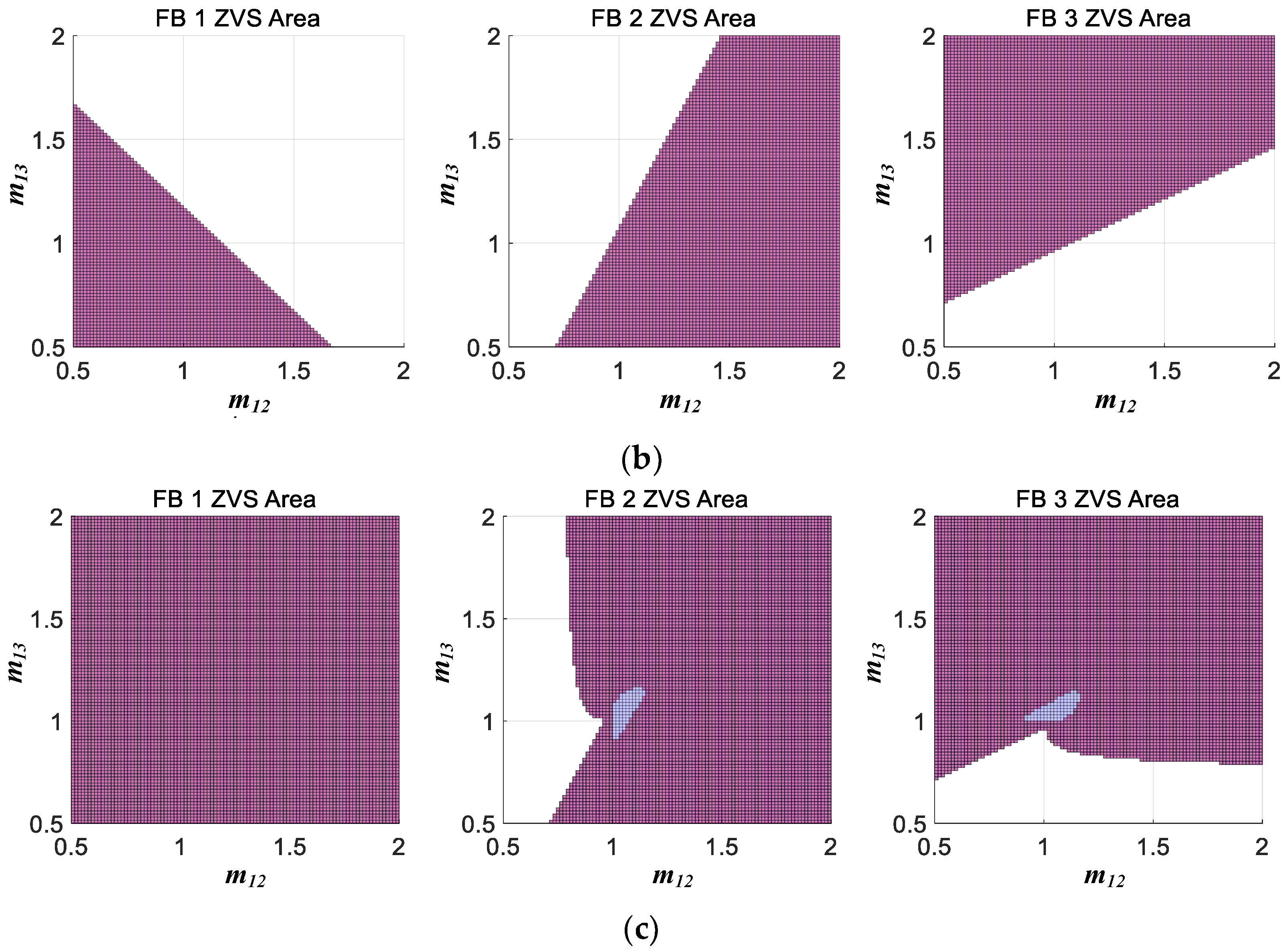
3. Zero Voltage Switching
3.1. Without Inner Phase Shift (αx = 0)
3.2. With Inner Phase Shift (αx ≠ 0)
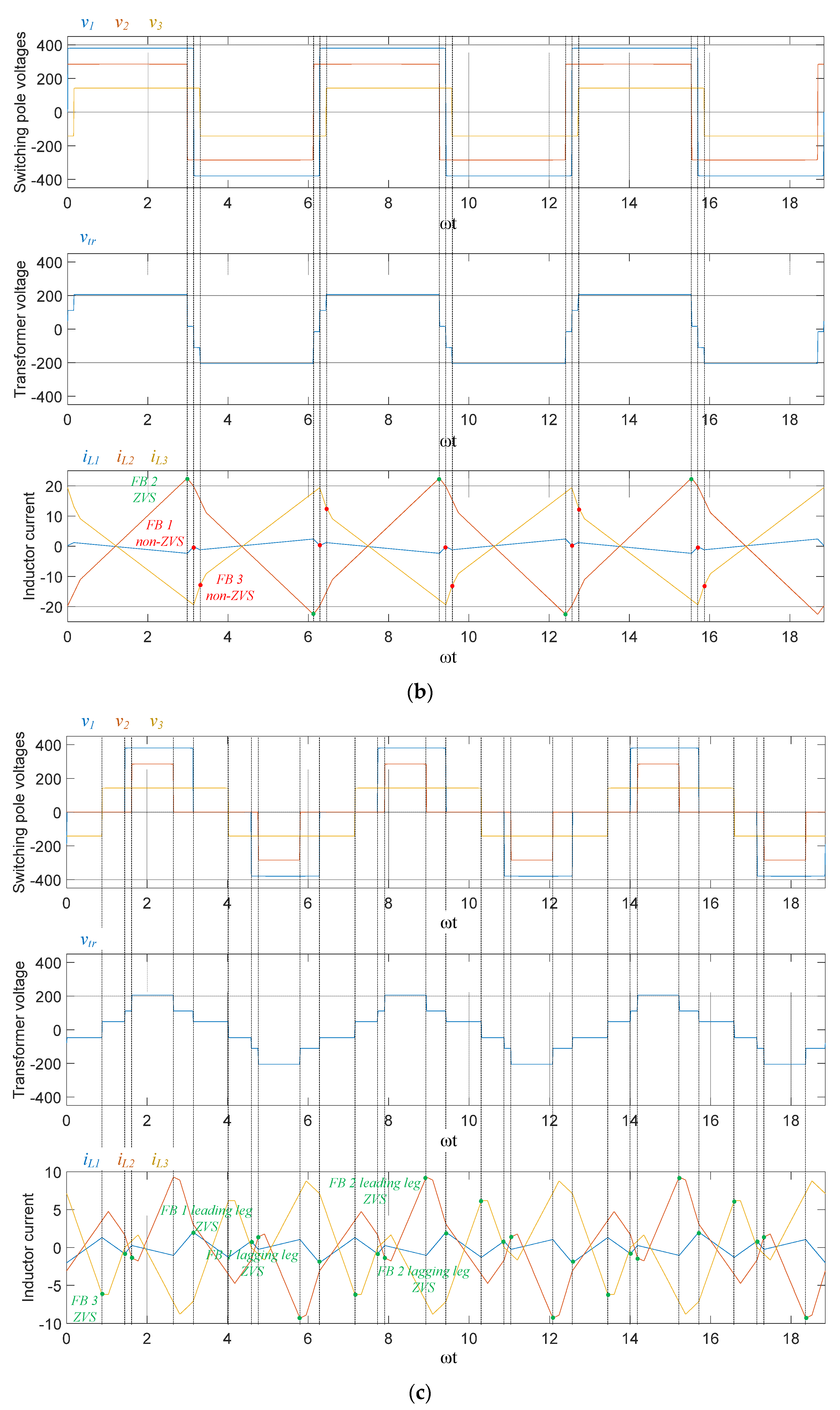
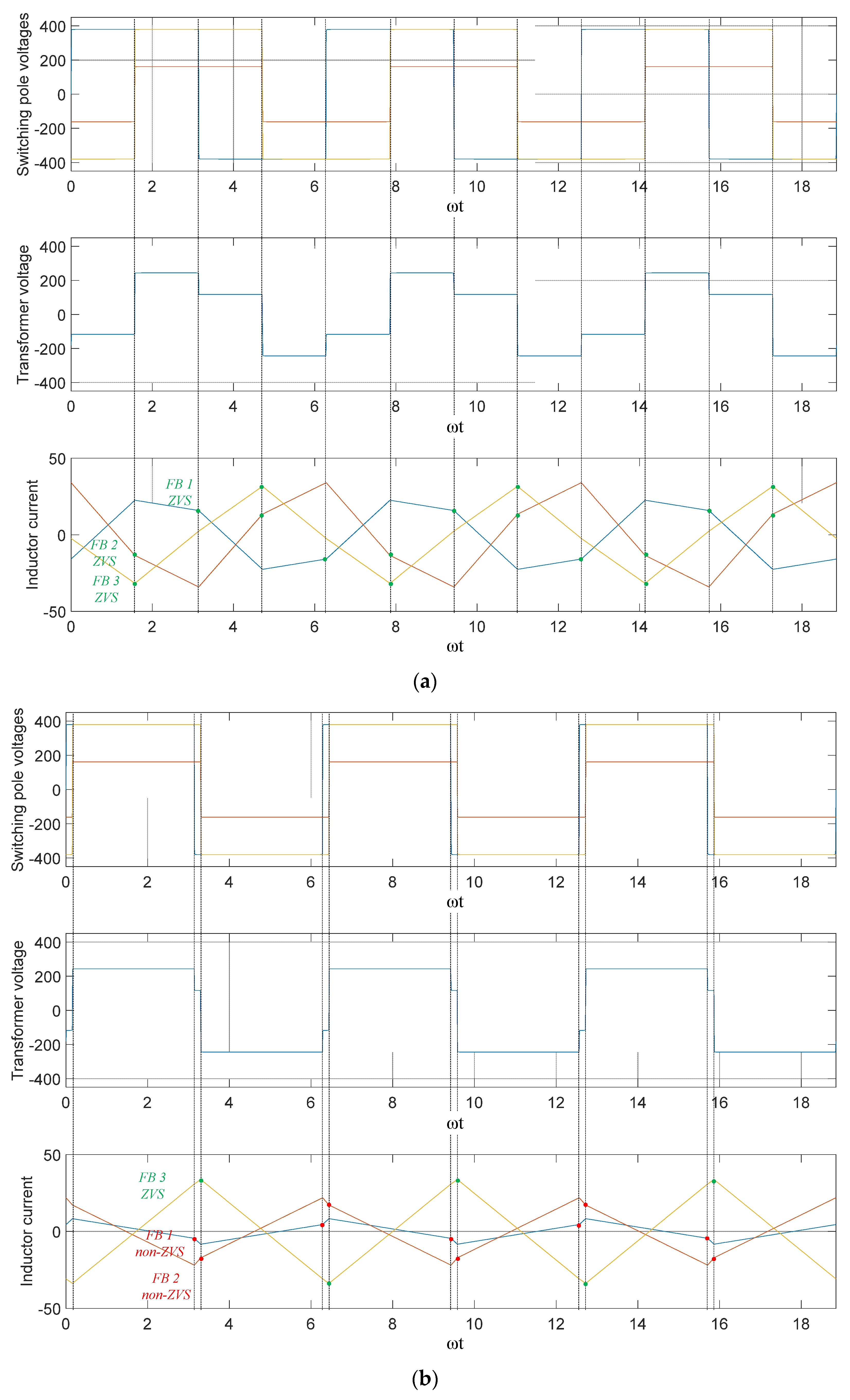
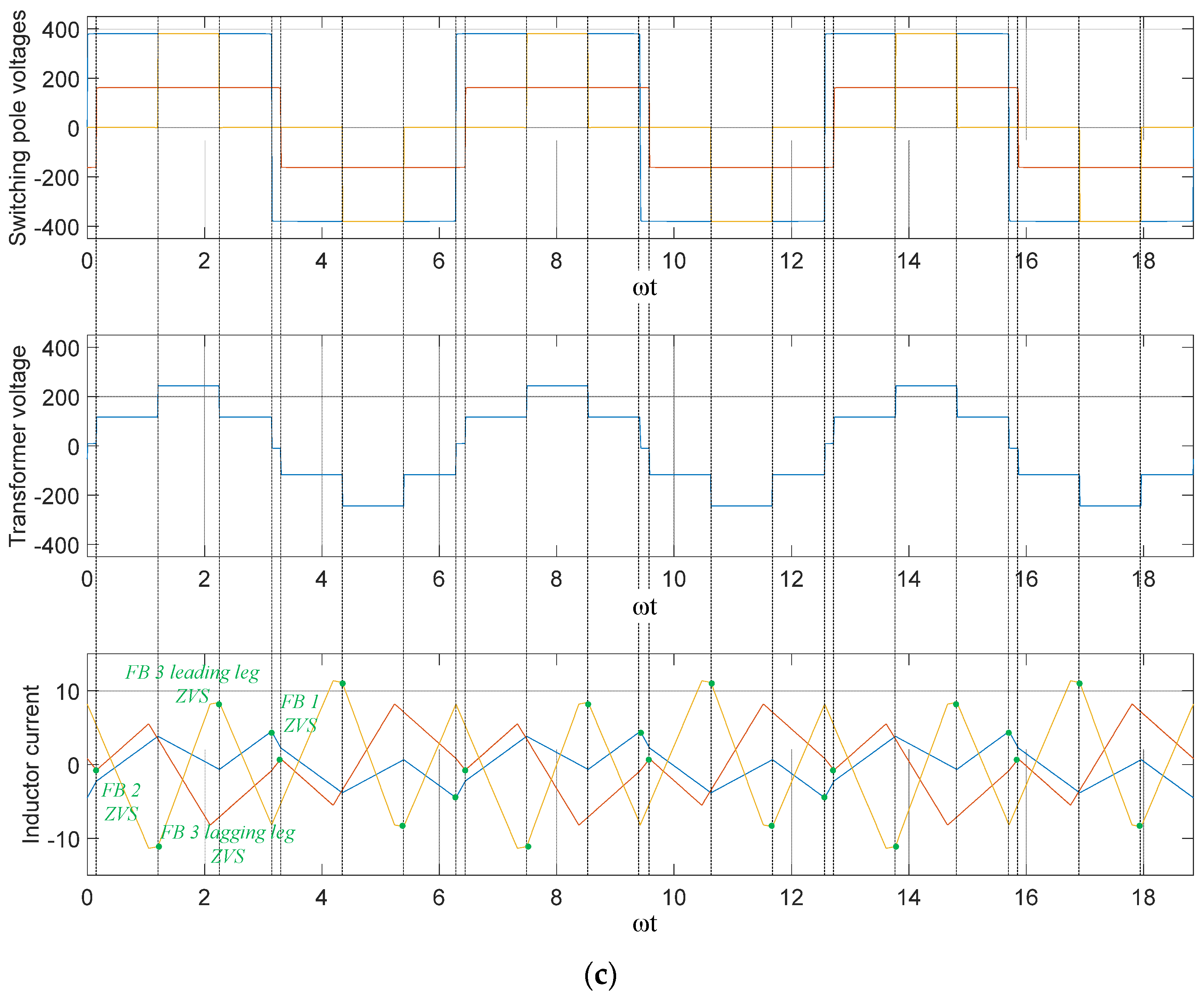
4. Simulation and Experimental Results
4.1. Case A (Variable: ϕ)
4.2. Case B (Variable: m)
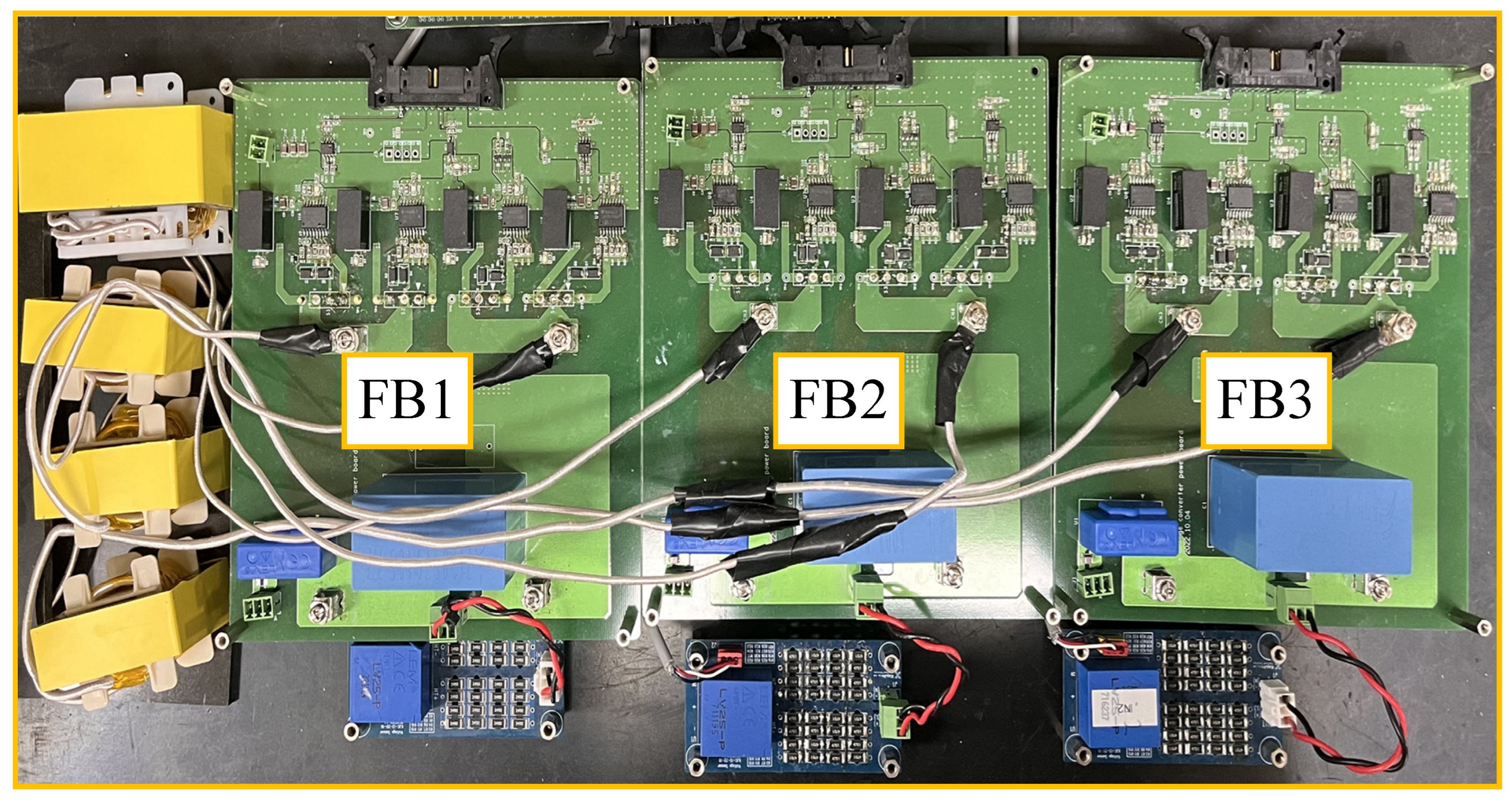
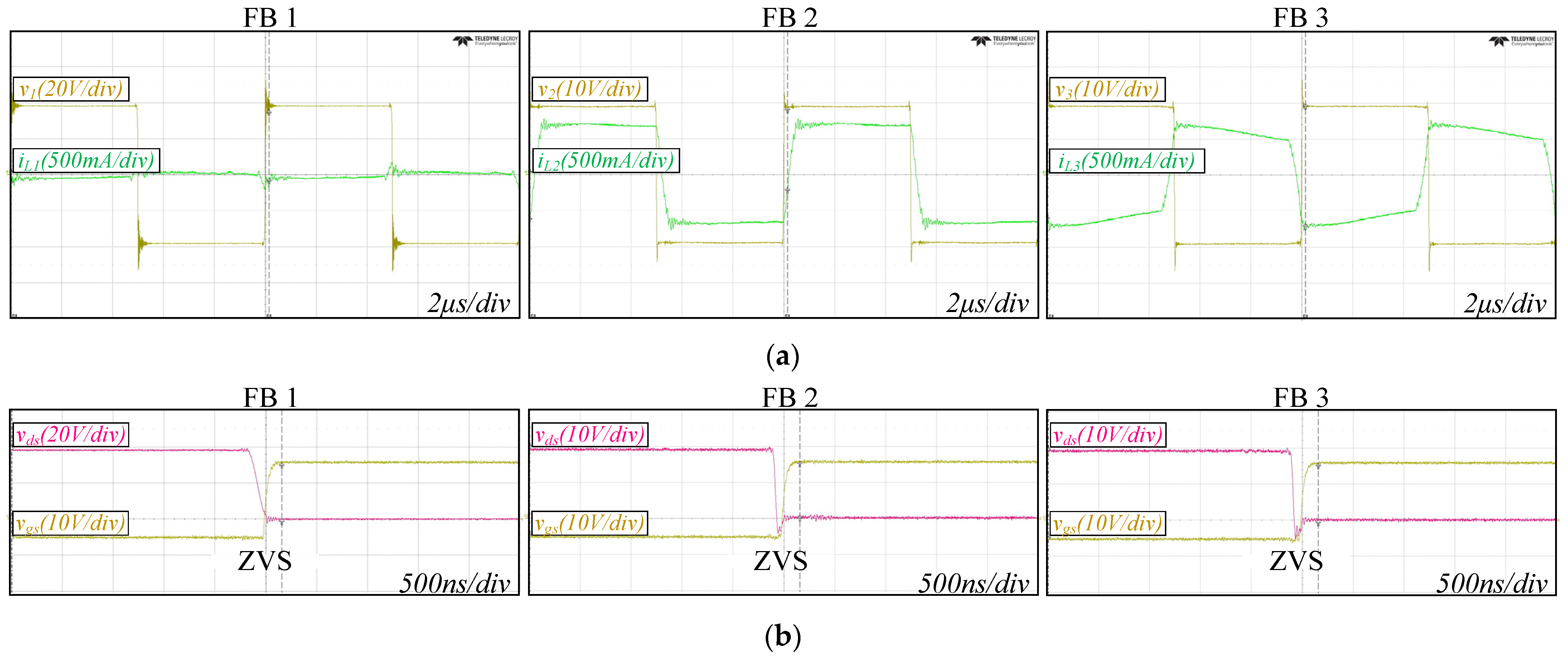
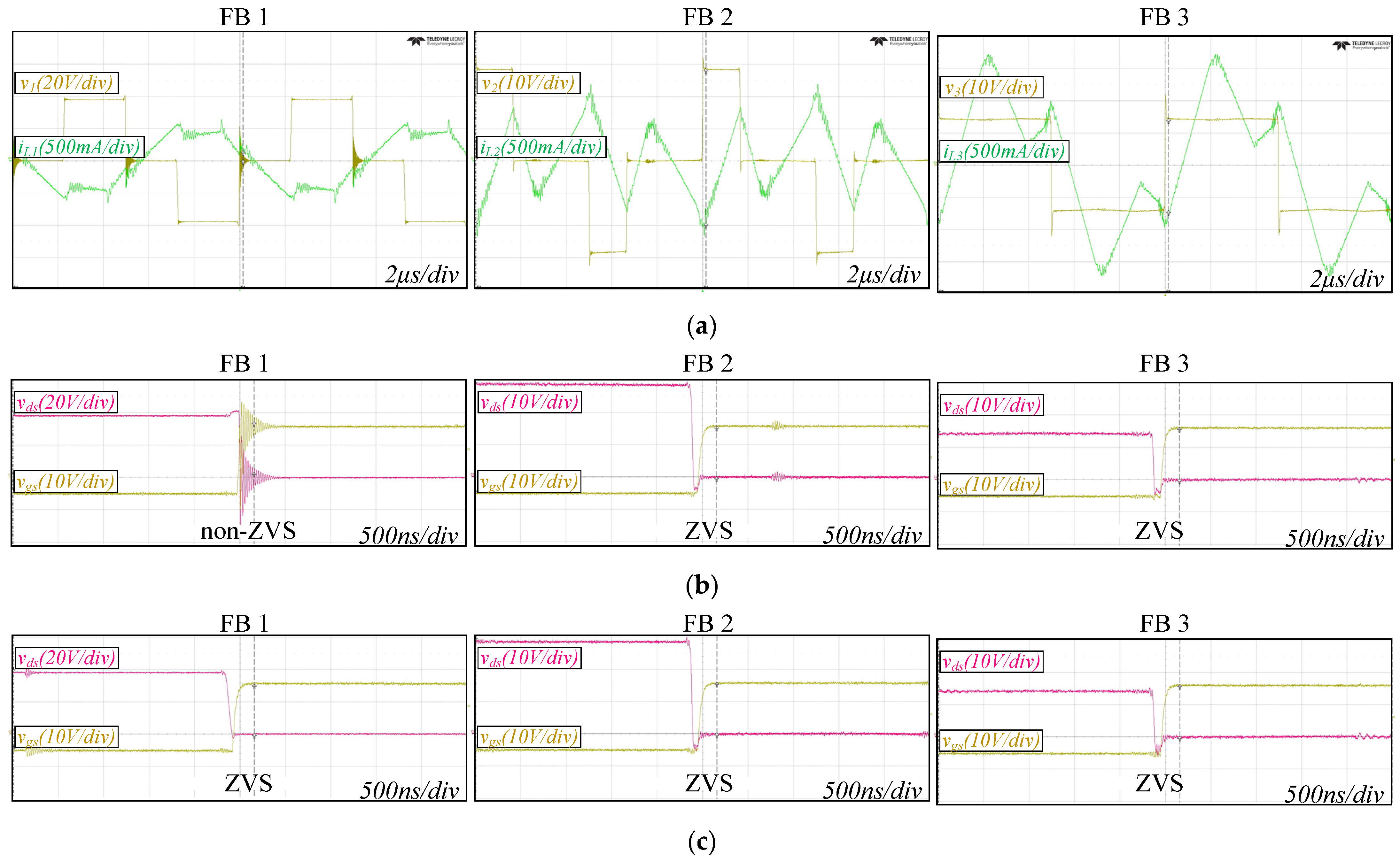
4.3. Prototype Experiment Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Dincer, I. Renewable energy and sustainable development: A crucial review. Renew. Sustain. Energy Rev. 2000, 4, 157–175. [Google Scholar] [CrossRef]
- Fuente, D.V.; Rodríguez, C.L.T.; Garcerá, G.; Figueres, E.; Gonzalez, R.O. Photovoltaic Power System with Battery Backup with Grid-Connection and Islanded Operation Capabilities. IEEE Trans. Ind. Electron. 2013, 60, 1571–1581. [Google Scholar] [CrossRef]
- Hadjipaschalis, I.; Poullikkas, A.; Efthimiou, V. Overview of current and future energy storage technologies for electric power applications. Renew. Sustain. Energy Rev. 2009, 13, 1513–1522. [Google Scholar] [CrossRef]
- Bhattacharjee, K.; Kutkut, N.; Batarseh, I. Review of Multiport Converters for Solar and Energy Storage Integration. IEEE Trans. Power Electron. 2019, 34, 1431–1445. [Google Scholar] [CrossRef]
- Litran, S.P.; Duran, E.; Semiao, J.; Diaz-Martin, C. Multiple-Output DC–DC Converters: Applications and Solutions. Electronics 2022, 11, 1258. [Google Scholar] [CrossRef]
- Jiang, W.; Fahimi, B. Multiport Power Electronic Interface—Concept, Modeling, and Design. IEEE Trans. Power Electron. 2011, 26, 1890–1900. [Google Scholar] [CrossRef]
- Farhangi, B.; Toliyat, H.A. Modeling and Analyzing Multiport Isolation Transformer Capacitive Components for Onboard Vehicular Power Conditioners. IEEE Trans. Ind. Electron. 2015, 62, 3134–3142. [Google Scholar] [CrossRef]
- Wheeler, P.; Bozhko, S. The More Electric Aircraft: Technology and challenges. IEEE Electrif. Mag. 2014, 2, 6–12. [Google Scholar] [CrossRef]
- Neubert, M.; Gorodnichev, A.; Gottschlich, J.; De Doncker, R.W. Performance analysis of a triple-active bridge converter for interconnection of future dc-grids. In Proceedings of the 2016 IEEE Energy Conversion Congress and Exposition (ECCE), Milwaukee, WI, USA, 18–22 September 2016. [Google Scholar]
- Purgat, P.; Mackay, L.; Schulz, M.; Han, Y.; Qin, Z.; Marz, M.; Bauer, P. Design of a Power Flow Control Converter for Bipolar Meshed LVDC Distribution Grids. In Proceedings of the 2018 IEEE 18th International Power Electronics and Motion Control Conference (PEMC), Budapest, Hungary, 26–30 August 2018. [Google Scholar]
- Zhao, C.; Kolar, J.W. A novel three-phase three-port UPS employing a single high-frequency isolation transformer. In Proceedings of the 2004 IEEE 35th Annual Power Electronics Specialists Conference (IEEE Cat. No.04CH37551), Aachen, Germany, 15 November 2004. [Google Scholar]
- Koohi, P.; Watson, A.J.; Clare, J.C.; Soerio, T.B.; Wheeler, P.W. A Survey on Multi-Active Bridge DC-DC Converters: Power Flow Decoupling Techniques, Applications, and Challenges. Energies 2023, 16, 5927. [Google Scholar] [CrossRef]
- De Doncker, R.W.A.A.; Divan, D.M.; Kheraluwala, M.H. A three-phase soft-switched high-power-density DC/DC converter for high-power applications. IEEE Trans. Ind. Appl. 1991, 27, 63–73. [Google Scholar] [CrossRef]
- Michon, M.; Duarte, J.L.; Hendrix, M.; Simoes, M.G. A three-port bi-directional converter for hybrid fuel cell systems. In Proceedings of the 2004 IEEE 35th Annual Power Electronics Specialists Conference (IEEE Cat. No.04CH37551), Aachen, Germany, 20–25 June 2004. [Google Scholar]
- Bal, S.; Yelaverthi, D.B.; Rathore, A.K.; Srinivasan, D. Improved Modulation Strategy Using Dual Phase Shift Modulation for Active Commutated Current-Fed Dual Active Bridge. IEEE Trans. Power Electron. 2018, 33, 7359–7375. [Google Scholar] [CrossRef]
- Dai, P.; Liu, S.; Fang, S.; Gong, Z. Optimal Asymmetric Duty Modulation for Dual Active Bridge Converters with DC Blocking Capacitors. Energies 2023, 16, 6674. [Google Scholar] [CrossRef]
- Huang, J.; Wang, Y.; Li, Z.; Lei, W. Unified Triple-Phase-Shift Control to Minimize Current Stress and Achieve Full Soft-Switching of Isolated Bidirectional DC–DC Converter. IEEE Trans. Ind. Electron. 2016, 63, 4169–4179. [Google Scholar] [CrossRef]
- Oggier, G.; García, G.O.; Oliva, A.R. Modulation strategy to operate the dual active bridge DC-DC converter under soft switching in the whole operating range. IEEE Trans. Power Electron. 2011, 26, 1228–1236. [Google Scholar] [CrossRef]
- Oggier, G.G.; GarcÍa, G.O.; Oliva, A.R. Switching Control Strategy to Minimize Dual Active Bridge Converter Losses. IEEE Trans. Power Electron. 2009, 24, 1826–1838. [Google Scholar] [CrossRef]
- Krismer, F.; Kolar, J.W. Closed Form Solution for Minimum Conduction Loss Modulation of DAB Converters. IEEE Trans. Power Electron. 2012, 27, 174–188. [Google Scholar] [CrossRef]
- Everts, J. Closed-Form Solution for Efficient ZVS Modulation of DAB Converters. IEEE Trans. Power Electron. 2017, 32, 7561–7576. [Google Scholar] [CrossRef]
- Jiang, L.; Costinett, D. A triple active bridge DC-DC converter capable of achieving full-range ZVS. In Proceedings of the 2016 IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 20–24 March 2016. [Google Scholar]
- Yan, Y.; Gui, H.; Bai, H. Complete ZVS Analysis in Dual Active Bridge. IEEE Trans. Power Electron. 2021, 36, 1247–1252. [Google Scholar] [CrossRef]
- Kasper, M.; Burkart, R.M.; Deboy, G.; Kolar, J.W. ZVS of Power MOSFETs Revisited. IEEE Trans. Power Electron. 2016, 31, 8063–8067. [Google Scholar] [CrossRef]
- Purgat, P.; Bandyopadhyay, S.; Qin, Z.; Bauer, P. Zero Voltage Switching Criteria of Triple Active Bridge Converter. IEEE Trans. Power Electron. 2021, 36, 5425–5439. [Google Scholar] [CrossRef]
- Shen, Z.; Burgos, R.; Boroyevich, D.; Wang, F. Soft-switching capability analysis of a dual active bridge dc-dc converter. In Proceedings of the 2009 IEEE Electric Ship Technologies Symposium, Baltimore, MD, USA, 20–22 April 2009. [Google Scholar]






| Case | Switches | Energy Difference between the Two Voltage Sources | Conditions for ZVS Operation |
|---|---|---|---|
| A | Sx.1 and Sx.4 | or | |
| A′ | Sx.2 and Sx.3 | or | |
| B | Sx.1 | or | |
| B′ | Sx.2 | or | |
| C | Sx.3 | or | |
| C′ | Sx.4 | or |
| Leading Leg | Lagging Leg | Switching Pole Voltage |
|---|---|---|
| 1 | −1 | Vx |
| −1 | −1 | 0 |
| −1 | 1 | −Vx |
| 1 | 1 | 0 |
| MOSFET | Sx.1~Sx.4 | C3M0060065D |
|---|---|---|
| Switching frequency | fsw | 100 kHz |
| Turn ratio | n1:n2:n3 | 2:1:1 |
| FB 1 series inductance | L1 | 33.3 μH |
| FB 2 and 3 series inductance | L2 and L3 | 8.3 μH |
| Parasitic capacitance | Coss.x | 80 pF |
| FB 1 input voltage | V1 | 38 V |
| Gate-Source voltage | Vgs | −5/+15 V |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Byun, H.-J.; Park, J.-M.; Yi, J.; Won, C.-Y. Zero-Voltage-Switching Analysis Model of the Triple-Active-Bridge Converter. Energies 2023, 16, 7763. https://doi.org/10.3390/en16237763
Byun H-J, Park J-M, Yi J, Won C-Y. Zero-Voltage-Switching Analysis Model of the Triple-Active-Bridge Converter. Energies. 2023; 16(23):7763. https://doi.org/10.3390/en16237763
Chicago/Turabian StyleByun, Hyung-Jun, Jung-Min Park, Junsin Yi, and Chung-Yuen Won. 2023. "Zero-Voltage-Switching Analysis Model of the Triple-Active-Bridge Converter" Energies 16, no. 23: 7763. https://doi.org/10.3390/en16237763
APA StyleByun, H.-J., Park, J.-M., Yi, J., & Won, C.-Y. (2023). Zero-Voltage-Switching Analysis Model of the Triple-Active-Bridge Converter. Energies, 16(23), 7763. https://doi.org/10.3390/en16237763






