Numerical Simulation of Immersed Liquid Cooling System for Lithium-Ion Battery Thermal Management System of New Energy Vehicles
Abstract
:1. Introduction
2. Methodology
2.1. Lithium-Ion Battery Heat Generation Model
- I—current during charging and discharging, A;
- Ro—internal ohmic resistance of battery, Ω;
- n—the number of batteries;
- m—the mass of each battery, kg;
- Q—total chemical reaction heat, J;
- M—molar mass, kg/mol;
- F—Faraday constant, 6485.4 C/mol;
- Rp—internal polarization resistance of battery, Ω.
2.2. Physical Model
2.3. Governing Equations
2.4. The Grid Independent Analysis of Numerical Simulations
3. Numerical Investigations
3.1. Effect of Different Coolants
3.2. Effect of Different Discharge Rates
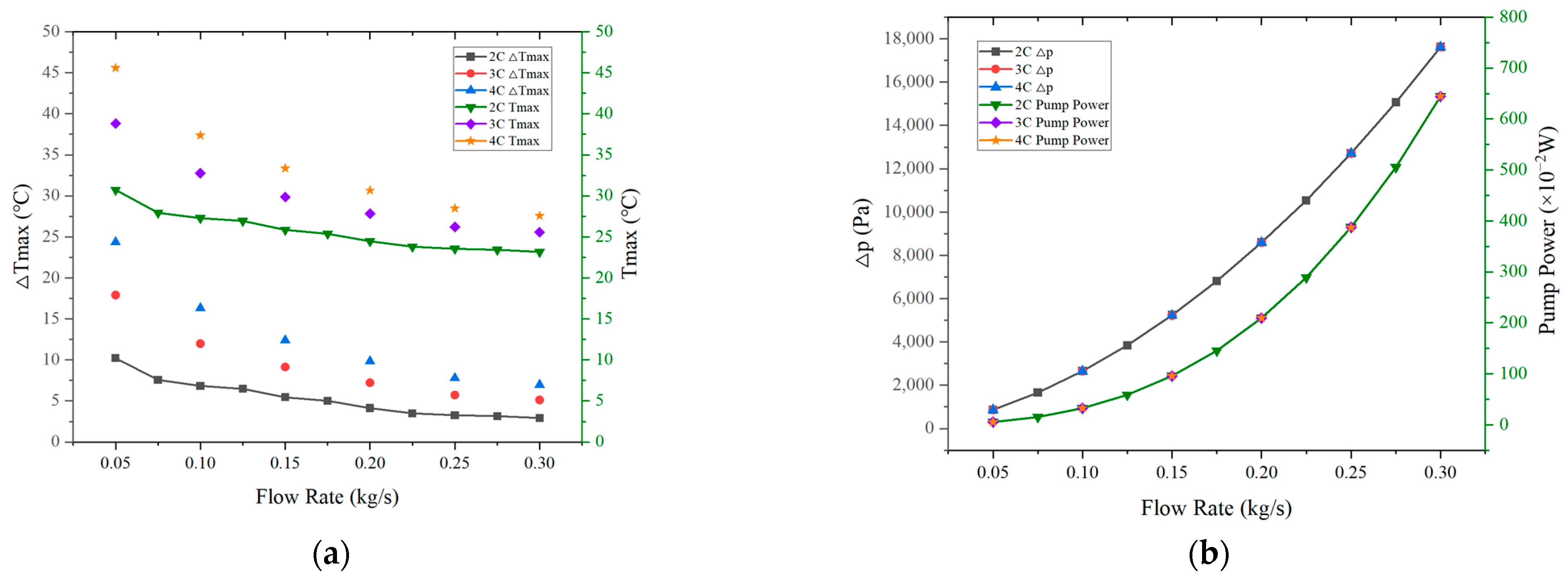
3.3. Effect of Different Flow Rates
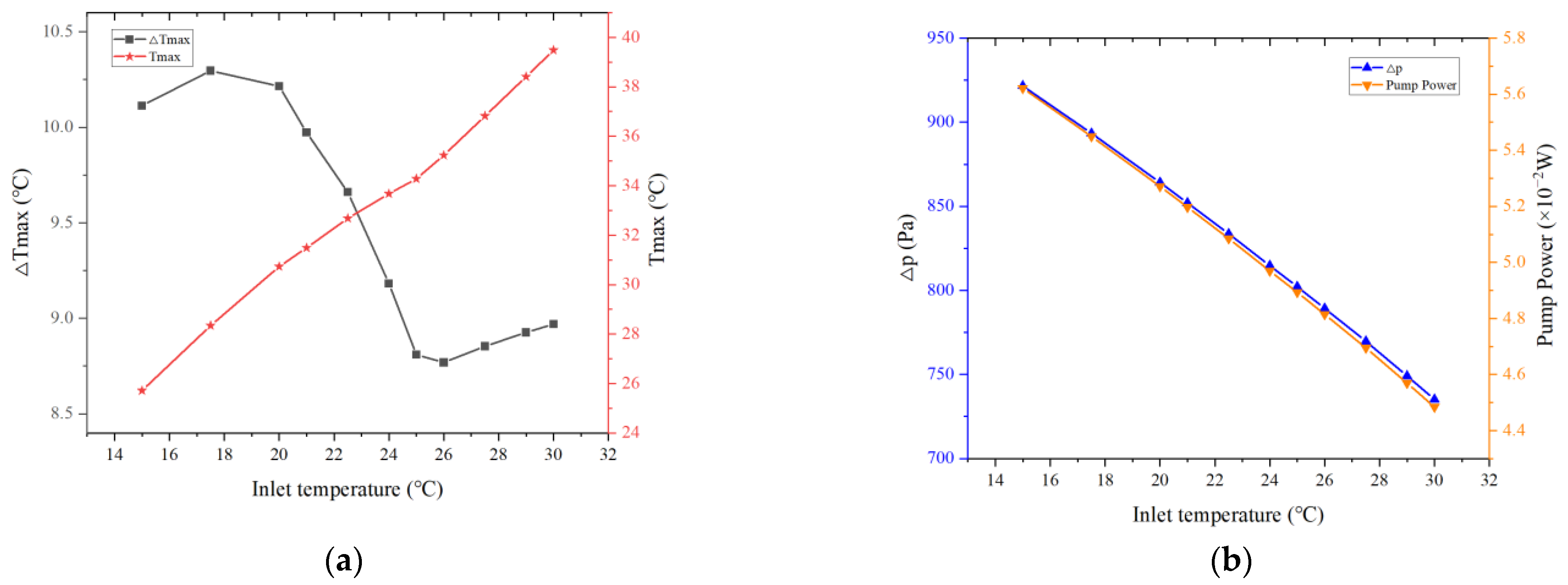
3.4. Effect of Different Inlet Temperatures
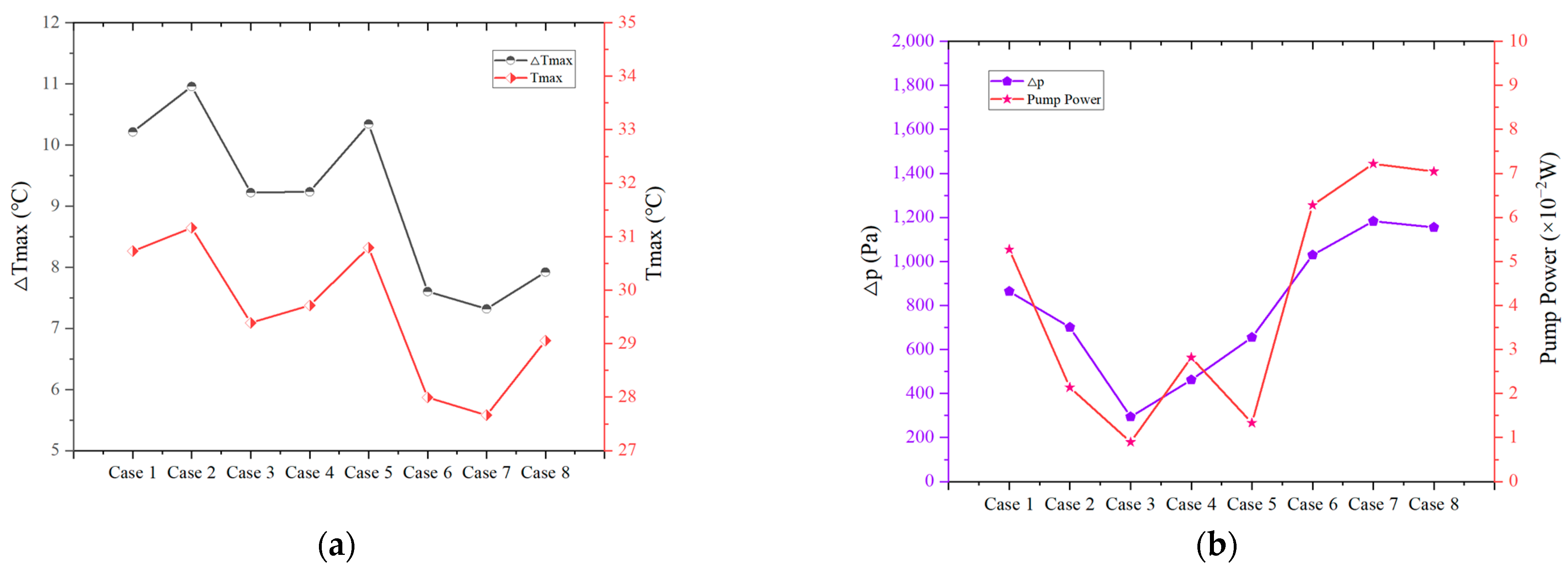
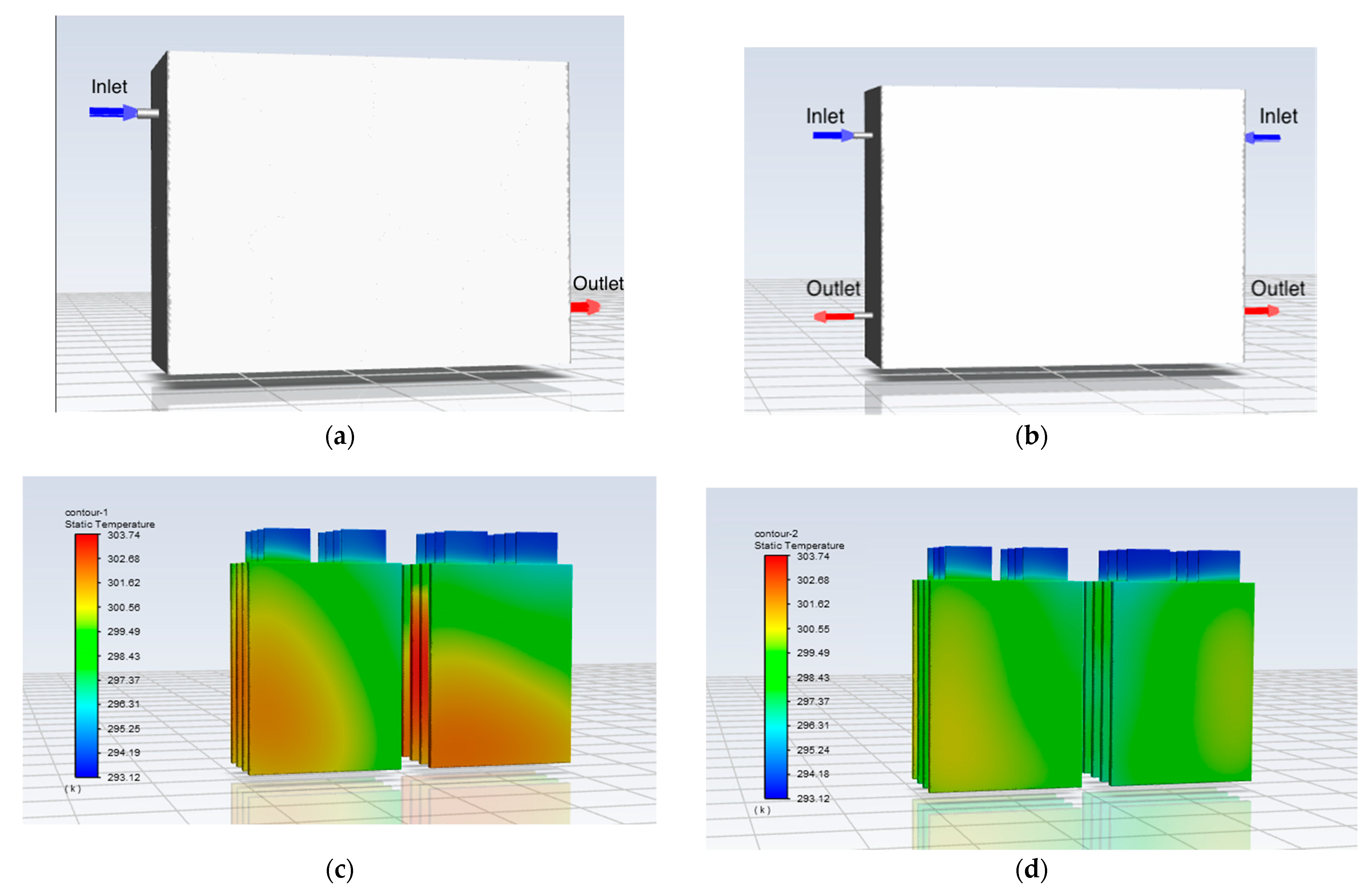
3.5. Effect of Different Inlet and Outlet Settings
4. Conclusions
- (1)
- Among the five coolants studied, W-E in water-based fluids has the best cooling effect, but its electric conductivity is high and requires special considerations to avoid electric leakage. Except for water-based fluids, PAO in hydrocarbon has the best cooling performance and requires the smallest pump power under the same conditions because of its higher specific heat capacity, higher thermal conductivity, and lower viscosity.
- (2)
- As the discharge rate increases, the heat production of the battery pack increases, Tmax and ΔTmax in the battery pack increase, and Δp and the required pump power remain basically unchanged.
- (3)
- Tmax and ΔTmax in the battery pack decrease as the inlet mass flow rate increases, but the method that only relies on increasing the inlet mass flow rate to control Tmax and ΔTmax is limited. Both Δp and the required pump power increase as the inlet mass flow rate increases, and the required pump power increases faster than Δp under the same mass flow rate gradient.
- (4)
- The inlet temperature of the coolant will affect physical properties such as density, specific heat capacity, thermal conductivity, and viscosity. Choosing the appropriate coolant temperature can not only decrease ΔTmax in the battery pack, but also decrease Δp and the required pump power of the system. This paper recommends that the inlet temperature of the coolant AC-110 should be in 25~27 °C.
- (5)
- For different inlet and outlet settings, The temperature in Case 3 (2 inlets and 2 outlets) and Case 7 (2 inlets and 2 outlets) are low, indicating that uniform distribution is very important. And, the 2 inlet and 2 outlet setting used in Case 7 has the best cooling effect. Compared with Case 1, Tmax decreases by 11.3% and ΔTmax decreases by 28.7%; however, Δp increases by 37.7% and the required pump power increases by 38.0%. Therefore, different inlet and outlet settings need to be considered comprehensively.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lyu, Y.; Siddique, A.R.M.; Majid, S.H.; Biglarbegian, M.; Gadsden, S.A.; Mahmud, S. Electric vehicle battery thermal management system with thermoelectric cooling. Energy Rep. 2019, 5, 822–827. [Google Scholar] [CrossRef]
- Al-Zareer, M.; Dincer, I.; Rosen, M.A. A novel phase change based cooling system for prismatic lithium ion batteries. Int. J. Refrig. 2018, 86, 203–217. [Google Scholar] [CrossRef]
- Kim, G.-H.; Pesaran, A. Battery Thermal Management System Design Modeling; National Renewable Energy Laboratory (NREL): Golden, CO, USA, 2006. [Google Scholar]
- Yu, X.; Lu, Z.; Zhang, L.; Wei, L.; Cui, X.; Jin, L. Experimental study on transient thermal characteristics of stagger-arranged lithium-ion battery pack with air cooling strategy. Int. J. Heat Mass Transf. 2019, 143, 118576. [Google Scholar] [CrossRef]
- Qian, Z.; Li, Y.; Rao, Z. Thermal Performance of Lithium-Ion Battery Thermal Management System by Using Mini-Channel Cooling. Energy Convers. Manag. 2016, 126, 622–631. [Google Scholar] [CrossRef]
- Wu, W.; Wang, S.; Wu, W.; Chen, K.; Hong, S.; Lai, Y. A Critical Review of Battery Thermal Performance and Liquid Based Battery Thermal Management. Energy Convers. Manag. 2019, 182, 262–281. [Google Scholar] [CrossRef]
- Wu, S.; Lao, L.; Wu, L.; Liu, L.; Lin, C.; Zhang, Q. Effect analysis on integration efficiency and safety performance of a battery thermal management system based on direct contact liquid cooling. Appl. Therm. Eng. 2022, 201, 117788. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, X.; Meng, H.; Guo, L.; Zhang, Z. Numerical analysis of the thermal performance of a liquid cooling battery module based on the gradient ratio of a liquid cooling battery module based on the gradient ratio flow velocity and gradient increment tube diameter. Int. J. Heat Mass Transf. 2021, 175, 121338. [Google Scholar] [CrossRef]
- Safdari, M.; Ahmadi, R.; Sadeghzadeh, S. Numerical Investigation on PCM Encapsulation Shape Used in the Passive-Active Battery Thermal Management. Energy 2020, 193, 116840. [Google Scholar] [CrossRef]
- Cao, J.; Ling, Z.; Fang, X.; Zhang, Z. Delayed Liquid Cooling Strategy with Phase Change Material to Achieve High Temperature Uniformity of Li-Ion Battery under High-Rate Discharge. J. Power Sources 2020, 450, 227673. [Google Scholar] [CrossRef]
- Zhang, W.; Qiu, J.; Yin, X.; Wang, D. A novel heat pipe assisted separation type battery thermal management system based on phase change material. Appl. Therm. Eng. 2019, 165, 114571. [Google Scholar] [CrossRef]
- Luo, B. Research of Electric Vehicle Liquid Cooling System Which Directly Contact with Battery Pack. Master’s Thesis, University of Technology, Guangzhou, China, 2016. [Google Scholar]
- Jinqiang, Z.; Haimin, W.; Nan, L. Temperature field characteristics of a small NCM811 traction battery module cooled by insulating oil immersion. Energy Storage Sci. Technol. 2022, 11, 2612–2619. [Google Scholar]
- Le, Q.; Shi, Q.; Liu, Q.; Yao, X.; Ju, X.; Xu, C. Numerical investigation on manifold immersion cooling scheme for lithium ion battery thermal management application. Int. J. Heat Mass Transf. 2022, 190, 122750. [Google Scholar] [CrossRef]
- Wang, Y.; Rao, Z.; Liu, S.; Li, X.; Li, H.; Xiong, R. Evaluating the performance of liquid immersing preheating system for lithium-ion battery pack. Appl. Therm. Eng. 2021, 190, 116811. [Google Scholar] [CrossRef]
- Patil, M.S.; Seo, J.-H.; Lee, M.-Y. A Novel Dielectric Fluid Immersion Cooling Technology for Li-Ion Battery Thermal Management. Energy Convers. Manag. 2021, 229, 113715. [Google Scholar] [CrossRef]
- Al-Zareer, M.; Dincer, I.; Rosen, M.A. Heat and Mass Transfer Modeling and Assessment of a New Battery Cooling System. Int. J. Heat Mass Transf. 2018, 126, 765–778. [Google Scholar] [CrossRef]
- Al-Zareer, M.; Dincer, I.; Rosen, M.A. Electrochemical Modeling and Performance Evaluation of a New Ammonia-Based Battery Thermal Management System for Electric and Hybrid Electric Vehicles. Electrochim. Acta 2017, 247, 171–182. [Google Scholar] [CrossRef]
- Li, X.; Huang, Q.; Deng, J.; Zhang, G.; Zhong, Z.; He, F. Evaluation of Lithium Battery Thermal Management Using Sealant Made of Boron Nitride and Silicone. J. Power Sources 2020, 451, 227820. [Google Scholar] [CrossRef]
- Zeng, S.; Wu, W.; Liu, J.; Wang, S.; Ye, S.; Feng, Z. A review of research on immersion cooling technology for lithium-ion batteries. Energy Storage Sci. Technol. 2023, 5, 2888. [Google Scholar]
- Bernardi, D.; Pawlikowski, E.; Newman, J. A general energy balance for battery systems. J. Electrochem. Soc. 1985, 132, 5–12. [Google Scholar] [CrossRef]
- AmpCool Dielectric Coolant|Engineered Fluids. Available online: https://www.engineeredfluids.com/ampcool (accessed on 1 August 2023).
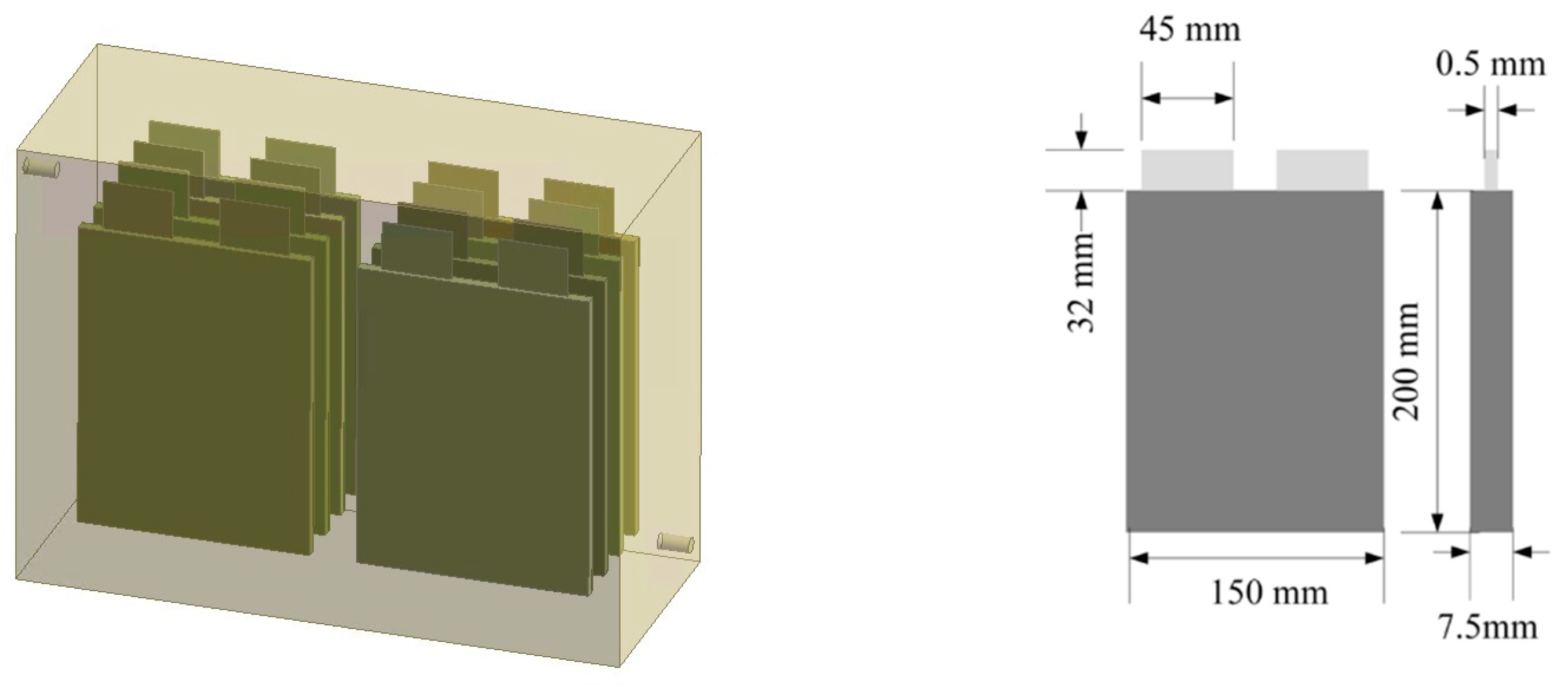
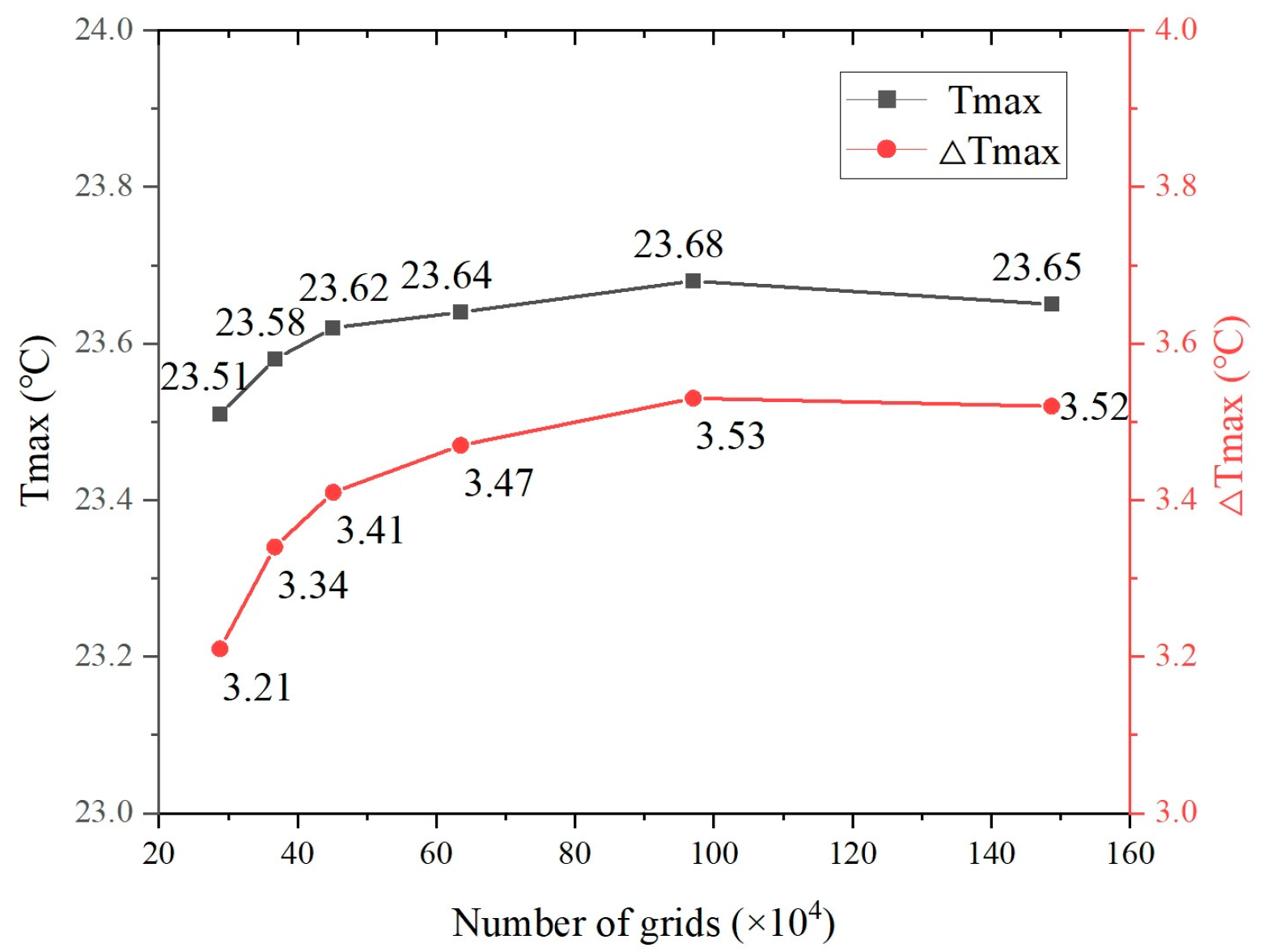
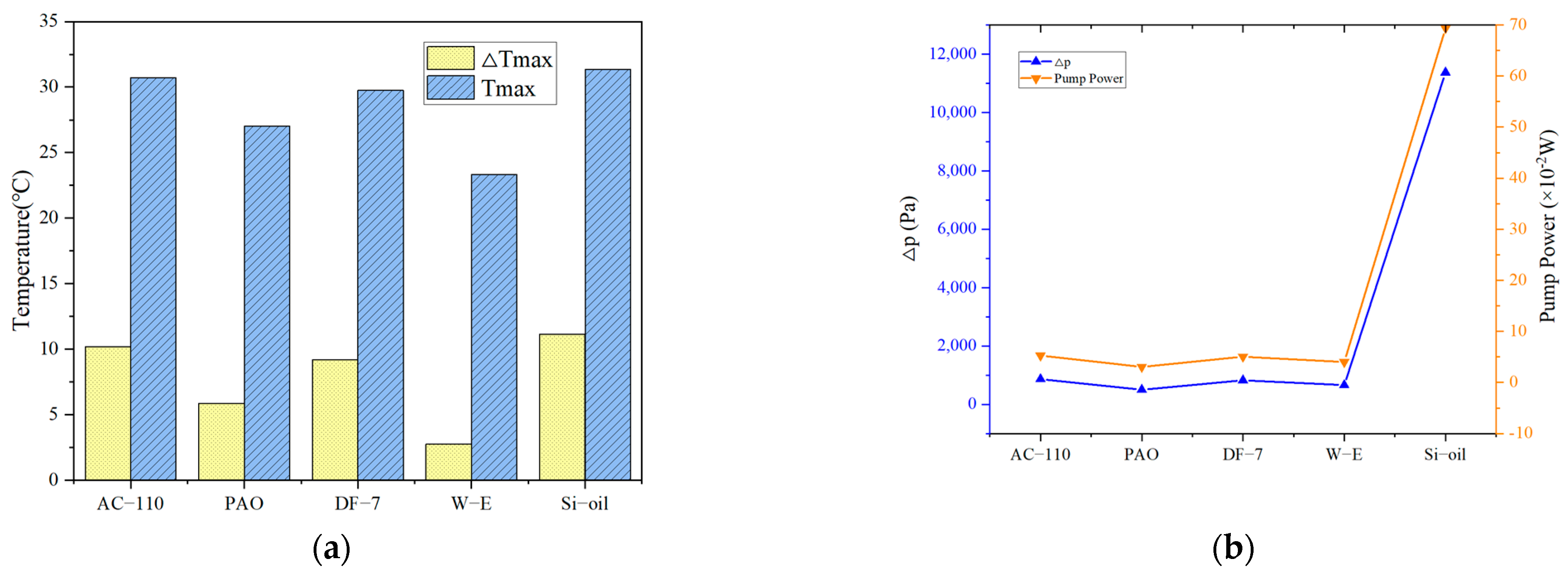
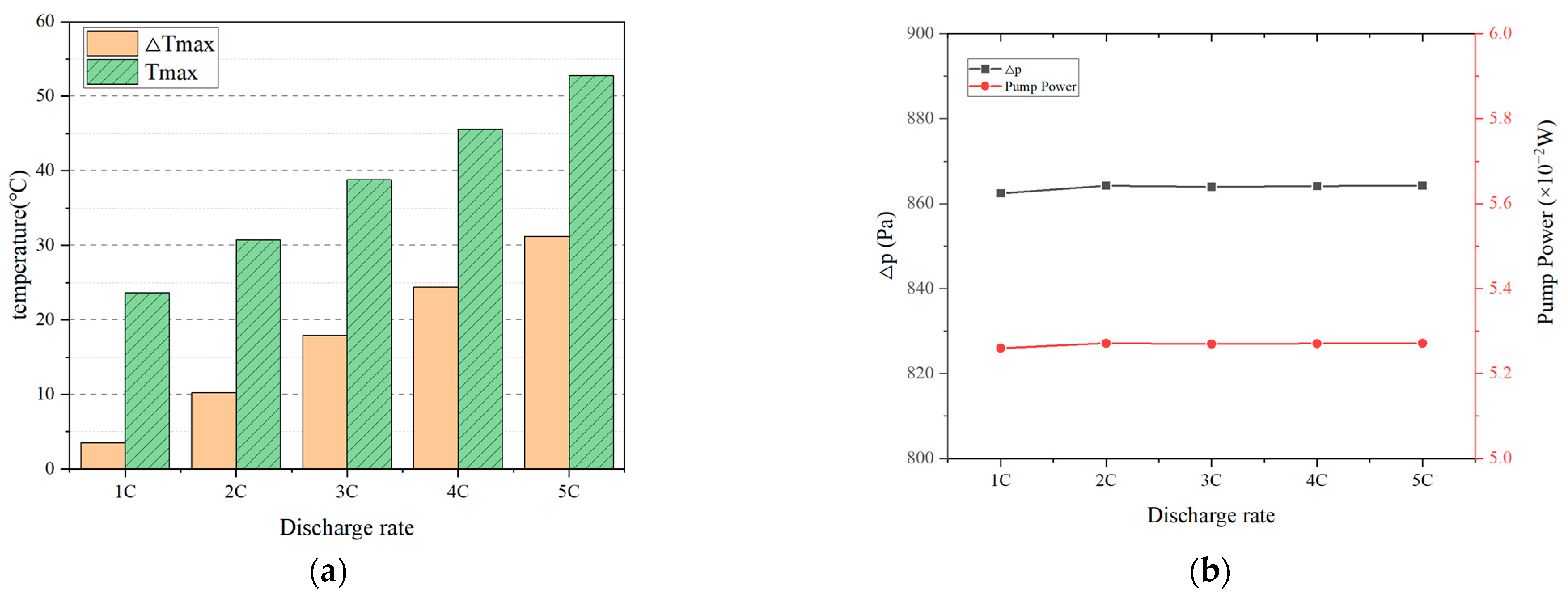
| Type | Item | Density kg/m3 | Kinematic Viscosity cSt | Heat Capacity J/(kg·K) | Thermal Conductivity W/(m·K) | Dynamic Viscosity kg/(m·s) |
|---|---|---|---|---|---|---|
| Hydrocarbons | PAO | 800 | 5.1 | 2241 | 0.14 | 0.004080 |
| Hydrocarbons | AC-110 | 820 | 24.6 | 2060 | 0.14 | 0.020172 |
| Esters | DF-7 | 920 | 16.4 | 1907 | 0.13 | 0.015088 |
| Silicone oils | Si-oil | 970 | 994.2 | 1370 | 0.15 | 0.964374 |
| Water-based | W-E | 1082 | 4.5 | 3260 | 0.402 | 0.004869 |
| Discharge Rate | Thermal Power W | Volume of One Cell m3 | Volumetric Heat Source W/m3 |
|---|---|---|---|
| 1C | 6.51 | 0.00022644 | 28,749 |
| 2C | 19.17 | 84,658 | |
| 3C | 33.60 | 148,384 | |
| 4C | 45.71 | 201,864 | |
| 5C | 58.51 | 258,391 |
| Inlet Temperature °C | Density kg/m3 | Kinematic Viscosity cSt | Heat Capacity J/(kg·K) | Thermal Conductivity W/(m·K) | Dynamic Viscosity kg/(m·s) |
|---|---|---|---|---|---|
| 15 | 820 | 28.725 | 2060 | 0.14 | 0.023555 |
| 17.5 | 26.663 | 2060 | 0.021863 | ||
| 20 | 24.600 | 2060 | 0.020172 | ||
| 22.5 | 22.539 | 2079 | 0.018482 | ||
| 25 | 20.478 | 2098 | 0.016792 | ||
| 27.5 | 18.416 | 2117 | 0.015101 | ||
| 30 | 16.355 | 2136 | 0.013411 |
| Inlet Velocity m/s | Single Inlet Area m2 | Inlet Flow Rate kg/s | ||
|---|---|---|---|---|
| Case 1 | 1 inlet, 1 outlet | 0.777 | 7.85 × 10−5 | 0.05 |
| Case 2 | 2 inlets, 1 outlet | 0.3885 | ||
| Case 3 | 2 inlets, 2 outlets | 0.3885 | ||
| Case 4 | 1 inlet, 2 outlets | 0.777 | ||
| Case 5 | 3 inlets, 1 outlet | 0.259 | ||
| Case 6 | 2 inlets, 1 outlet | 0.777 | 3.925 × 10−5 | |
| Case 7 | 2 inlets, 2 outlets | 3.925 × 10−5 | ||
| Case 8 | 3 inlets, 1 outlet | 2.627 × 10−5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fu, P.; Fang, L.; Jiao, S.; Sun, J.; Xin, Z. Numerical Simulation of Immersed Liquid Cooling System for Lithium-Ion Battery Thermal Management System of New Energy Vehicles. Energies 2023, 16, 7673. https://doi.org/10.3390/en16227673
Fu P, Fang L, Jiao S, Sun J, Xin Z. Numerical Simulation of Immersed Liquid Cooling System for Lithium-Ion Battery Thermal Management System of New Energy Vehicles. Energies. 2023; 16(22):7673. https://doi.org/10.3390/en16227673
Chicago/Turabian StyleFu, Ping, Liwei Fang, Shouyi Jiao, Jian Sun, and Zhicheng Xin. 2023. "Numerical Simulation of Immersed Liquid Cooling System for Lithium-Ion Battery Thermal Management System of New Energy Vehicles" Energies 16, no. 22: 7673. https://doi.org/10.3390/en16227673
APA StyleFu, P., Fang, L., Jiao, S., Sun, J., & Xin, Z. (2023). Numerical Simulation of Immersed Liquid Cooling System for Lithium-Ion Battery Thermal Management System of New Energy Vehicles. Energies, 16(22), 7673. https://doi.org/10.3390/en16227673






