Study on the Evolution Law of Wellbore Stability Interface during Drilling of Offshore Gas Hydrate Reservoirs
Abstract
:1. Introduction
2. Wellbore Stability Model in Gas Hydrate Reservoir Drilling
2.1. Mechanical Model of Wellbore Stability
2.2. Multiphase Flow Model in Hydrate Reservoir
2.3. Multiphase Flow Model in the Wellbore
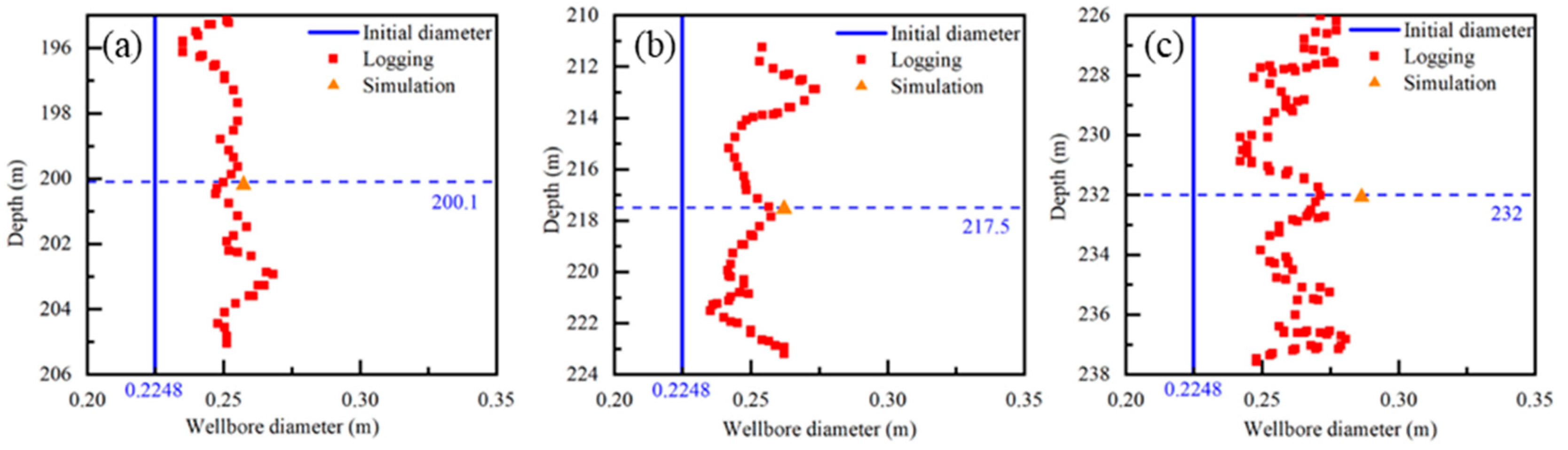
3. Solution and Verification of the Wellbore Stability Model
4. Results and Discussion
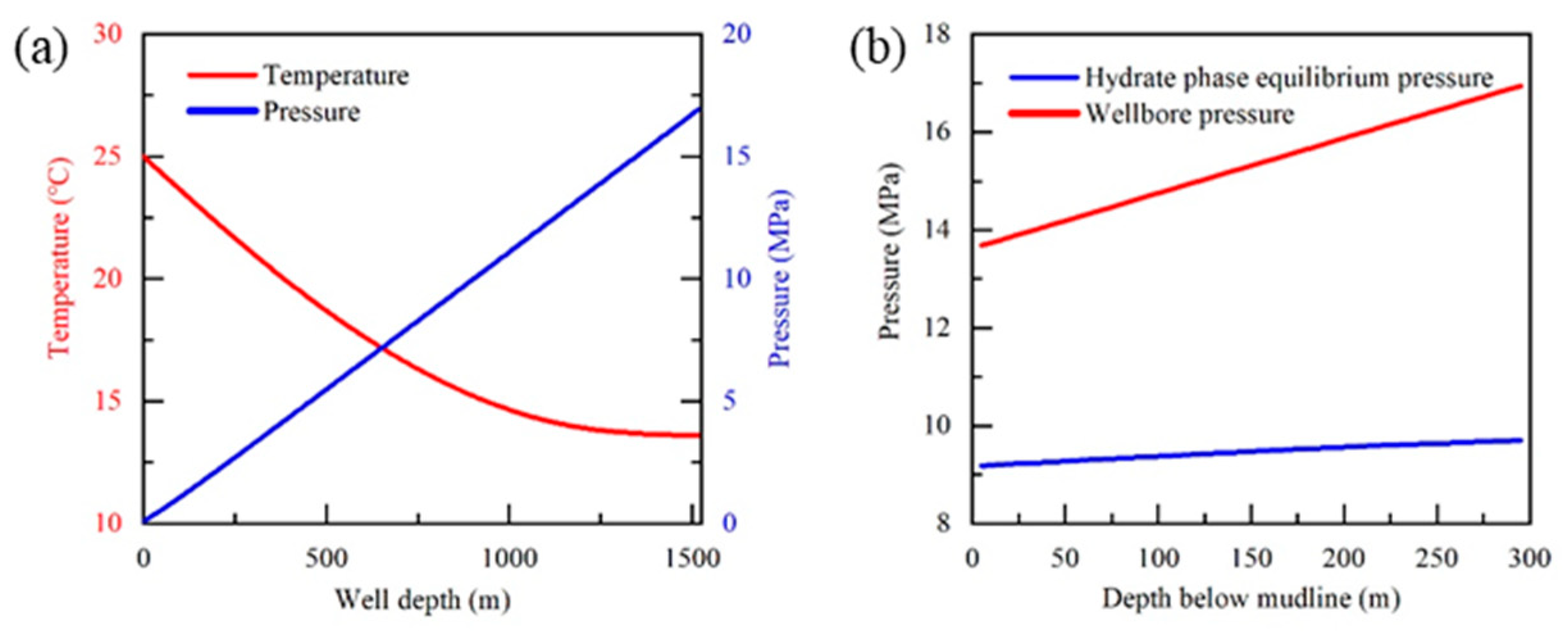
4.1. Distribution of the Wellbore Temperature and Pressure Field
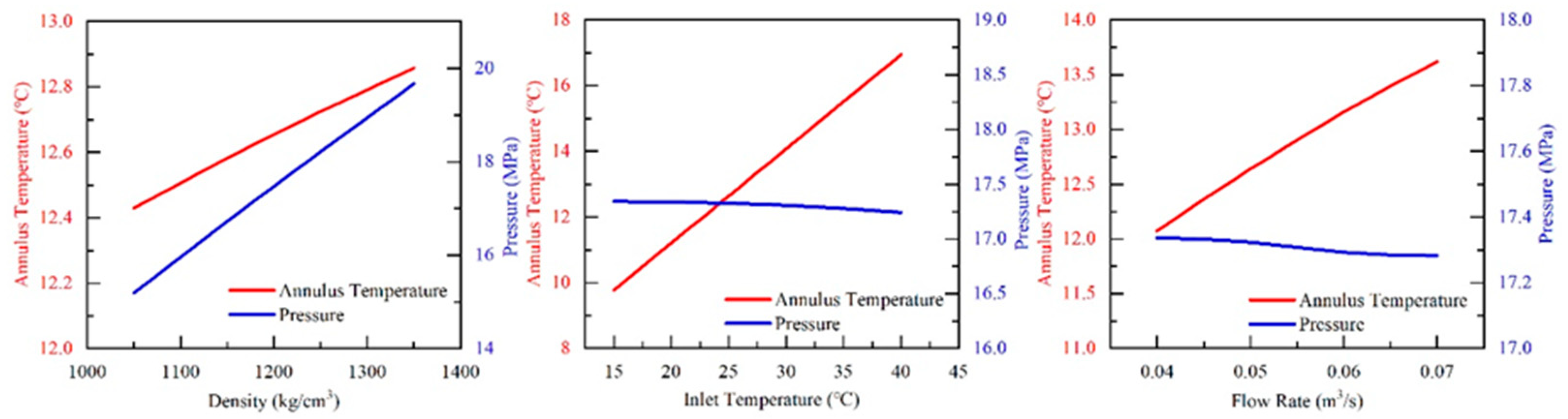
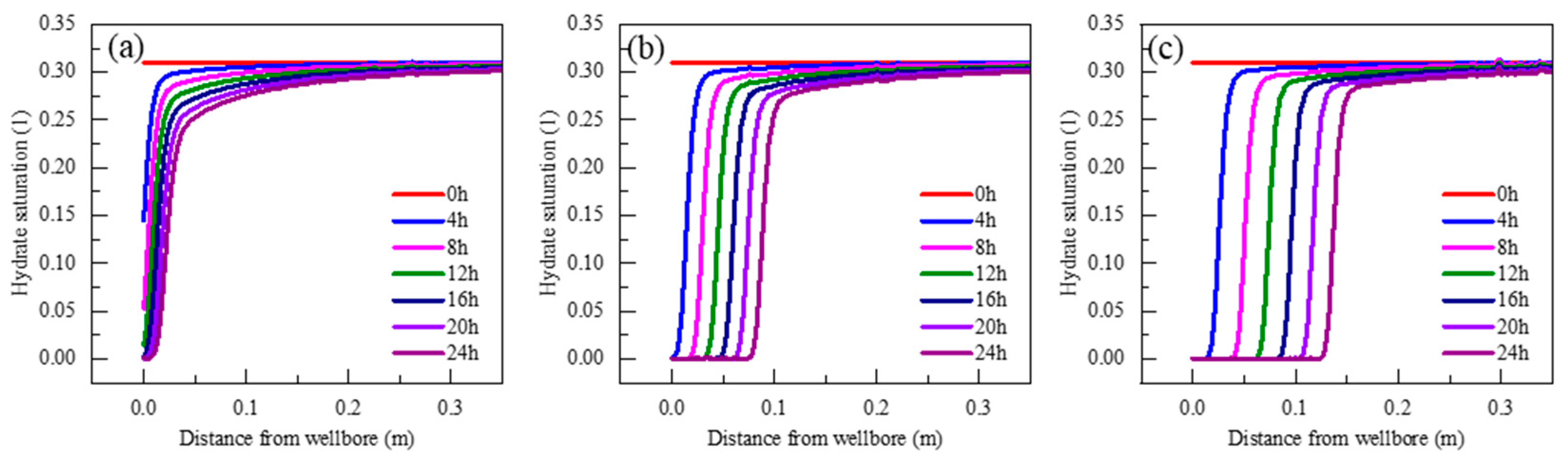
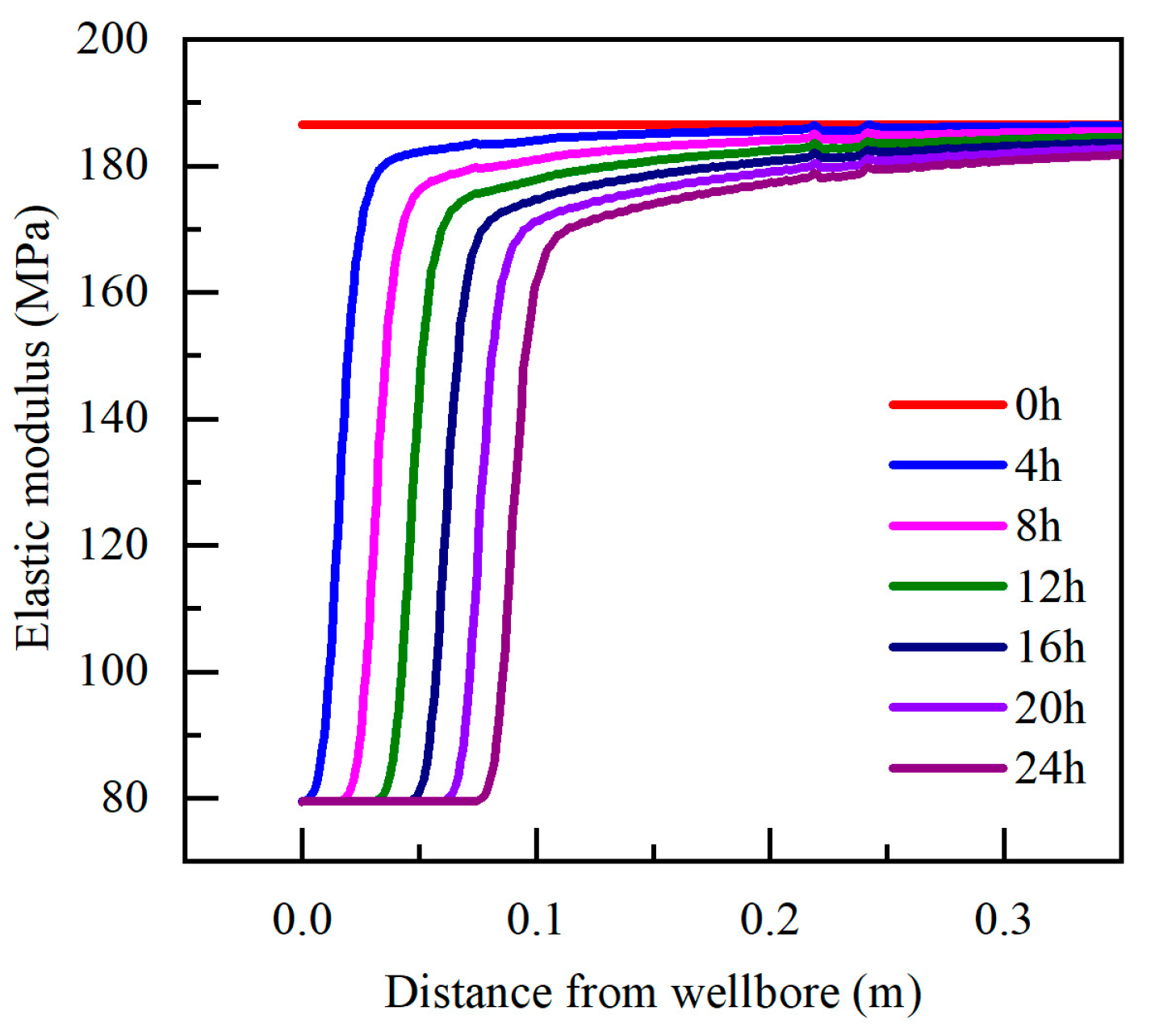
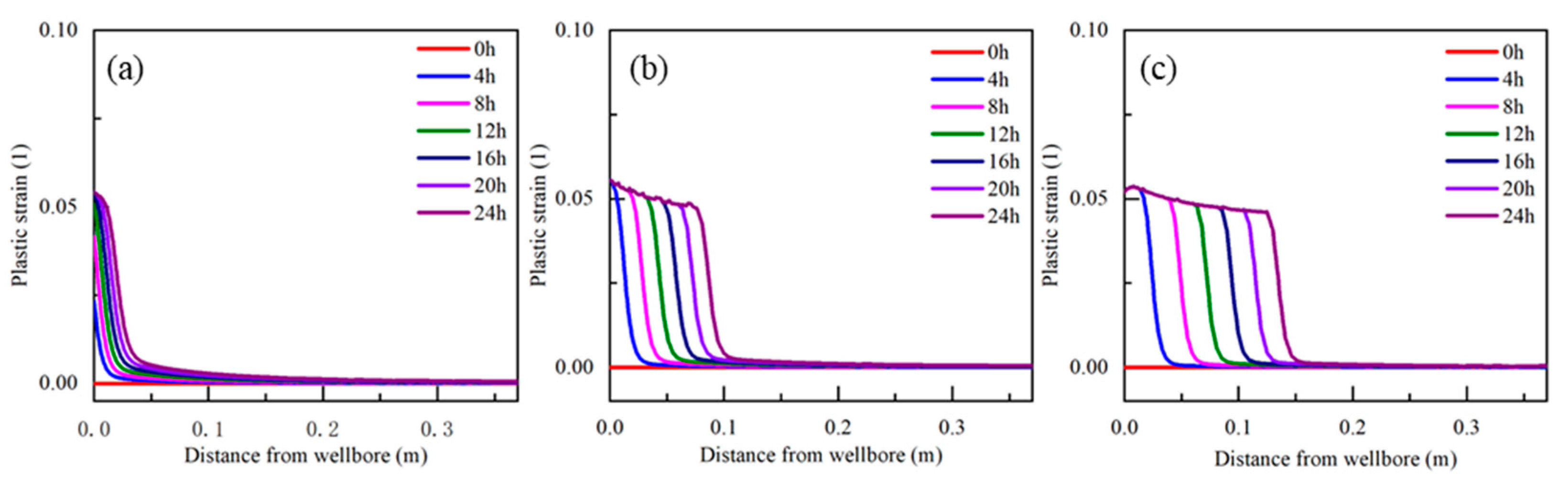
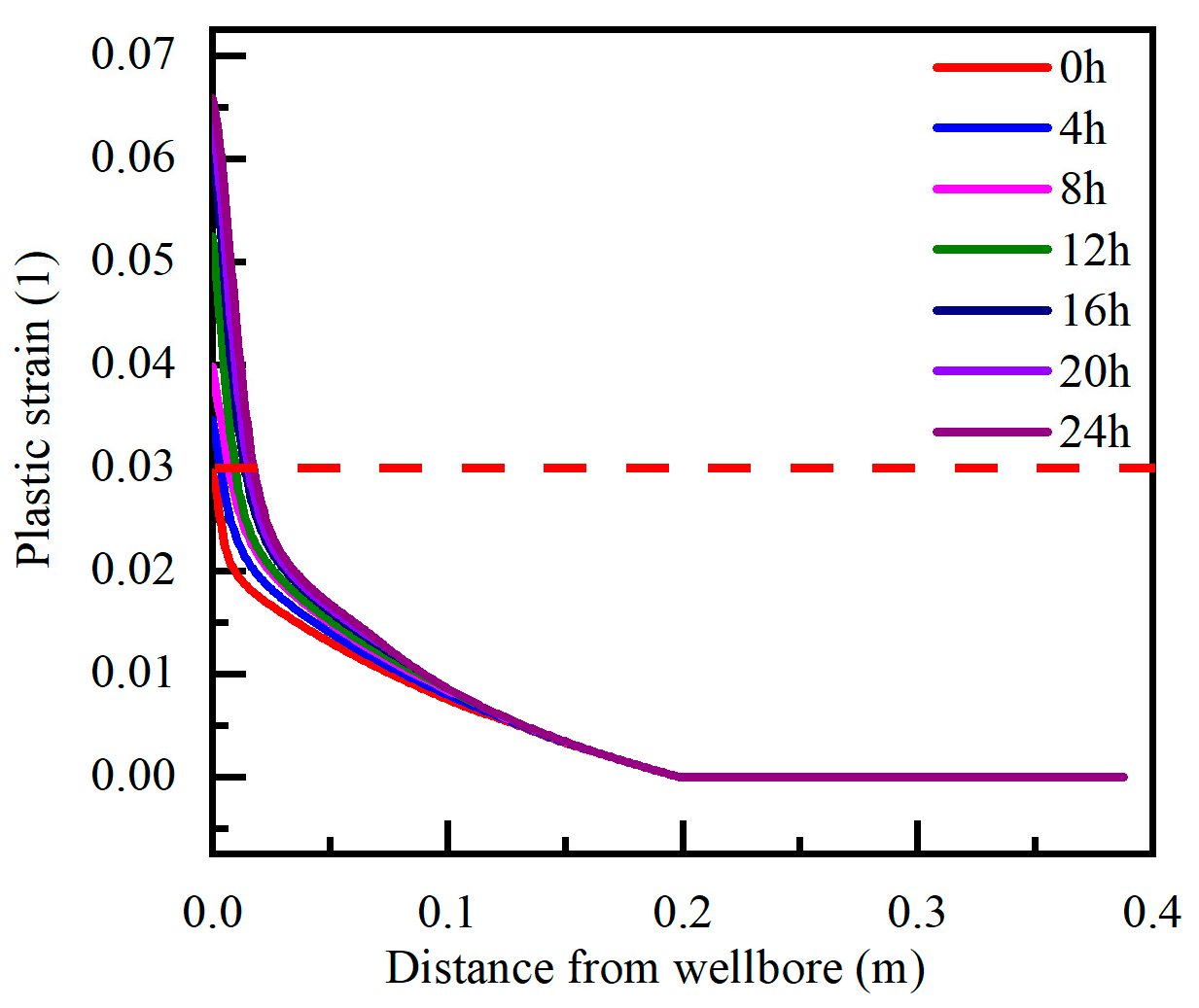
4.2. Influence of Temperature Disturbance on Wellbore Stability
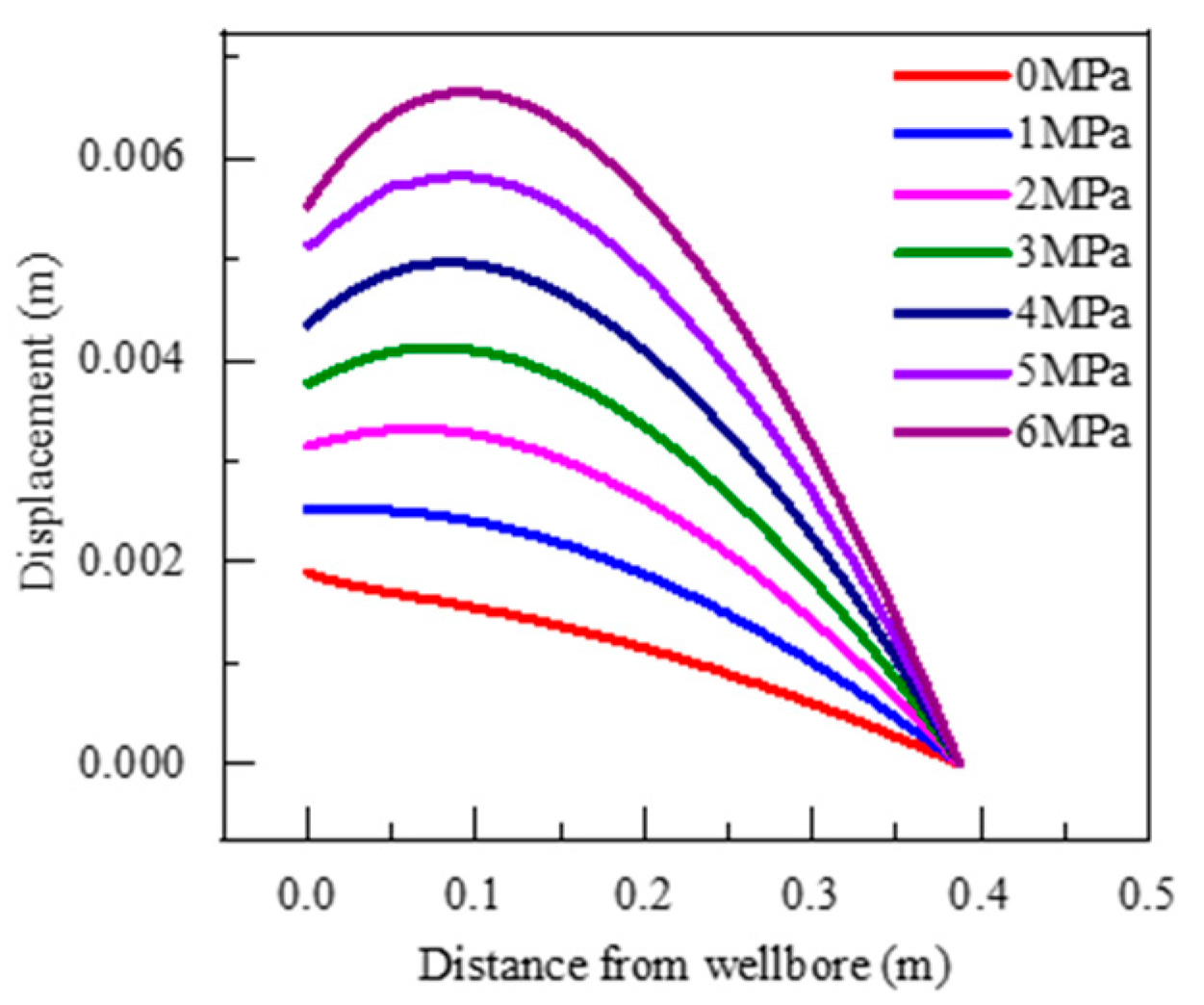
4.3. Influence of Pressure Disturbance on Wellbore Stability
5. Conclusions
- (1)
- Under the condition of deep-water drilling, owing to the low temperature of the seawater section and shallow formation, if the drilling speed, circulating flow rate, sea surface mud pit temperature and other parameters are properly controlled, the temperature of the drilling fluid in the shallow layer of the wellbore can be maintained at a level lower than the formation temperature.
- (2)
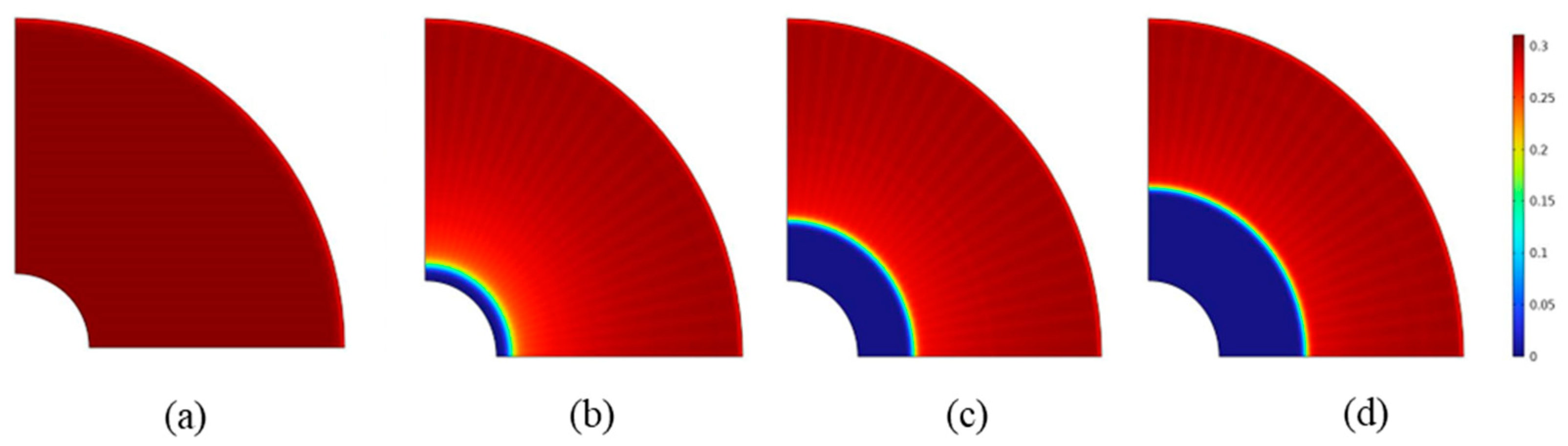
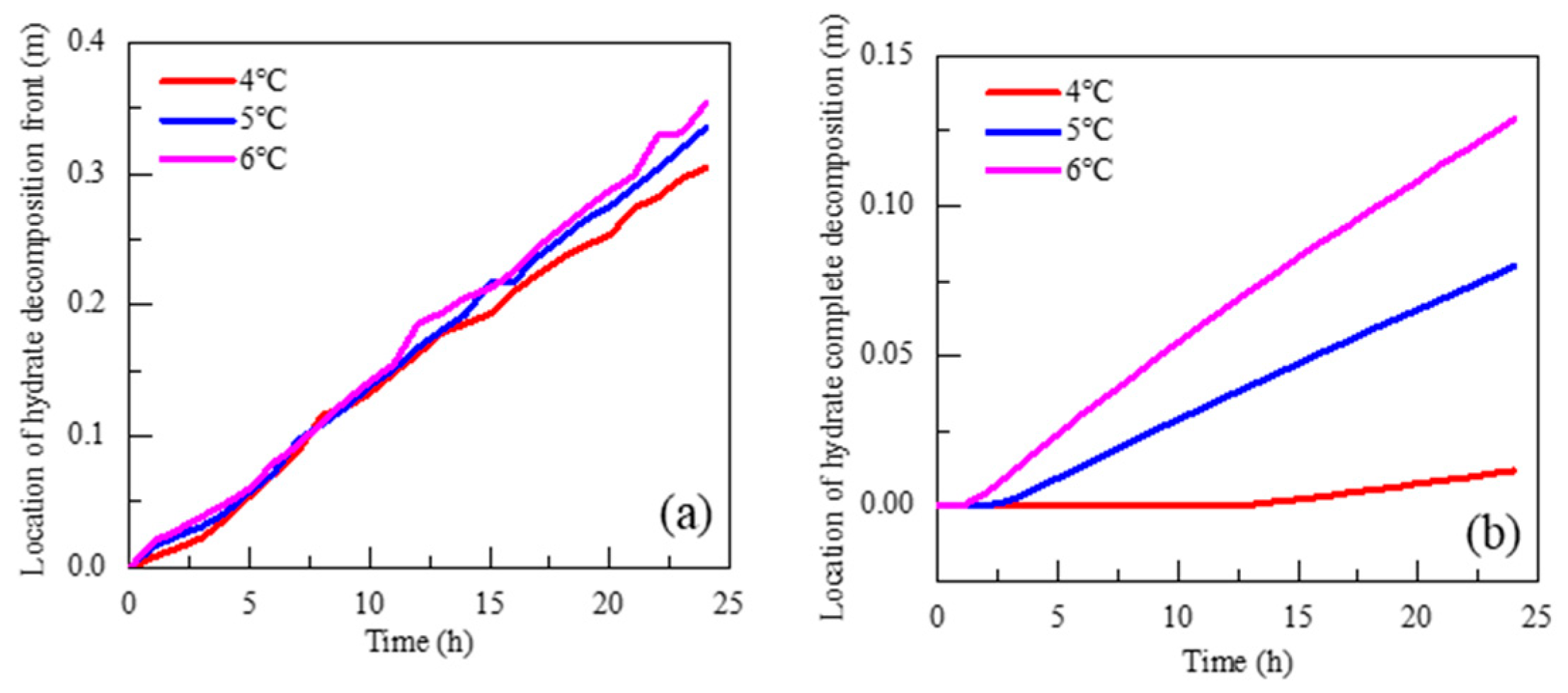
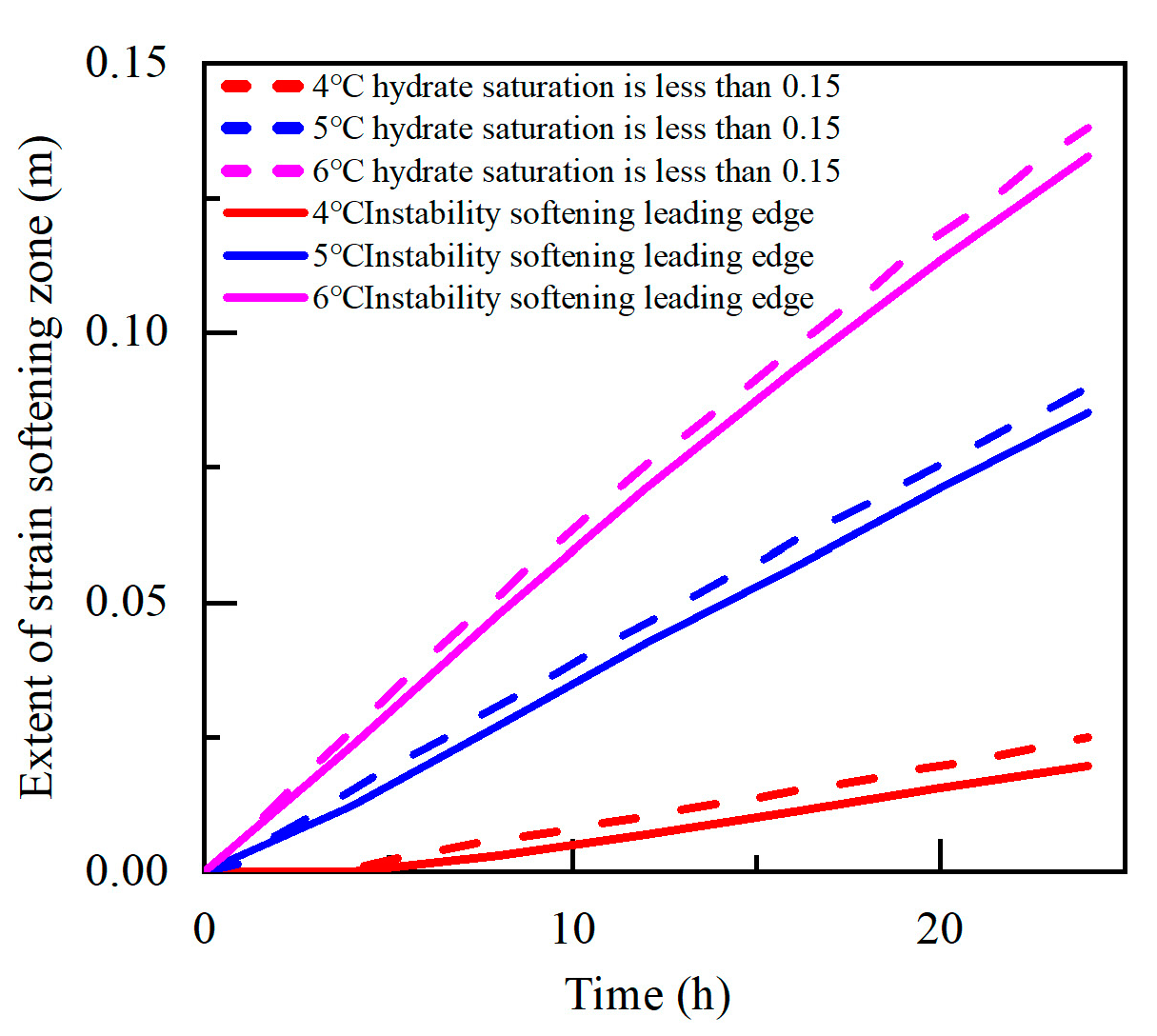
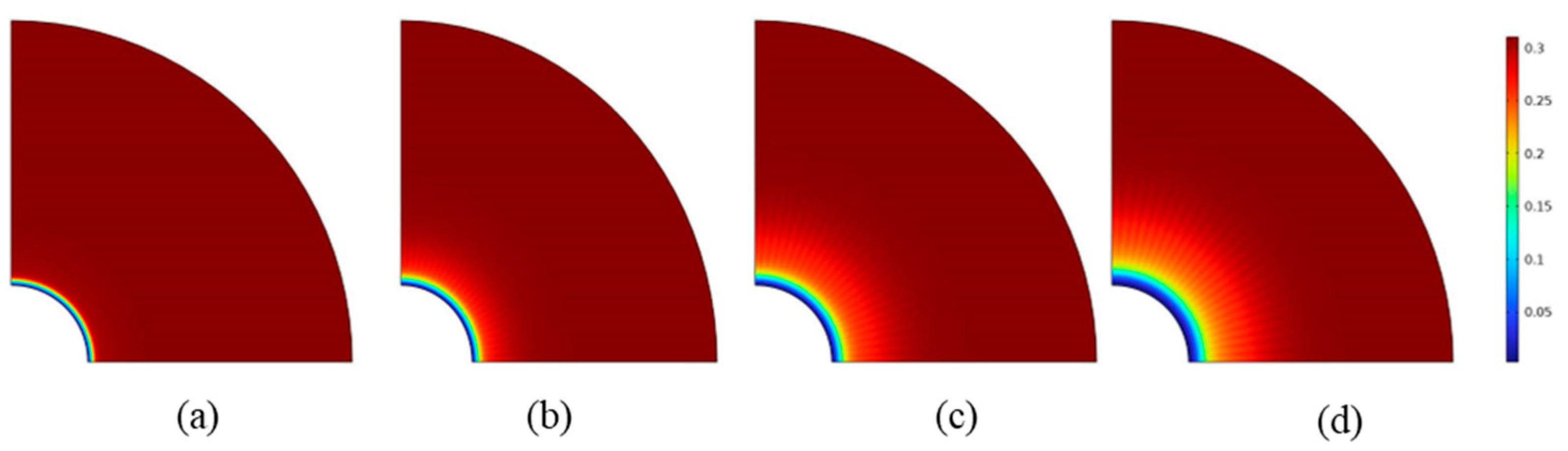
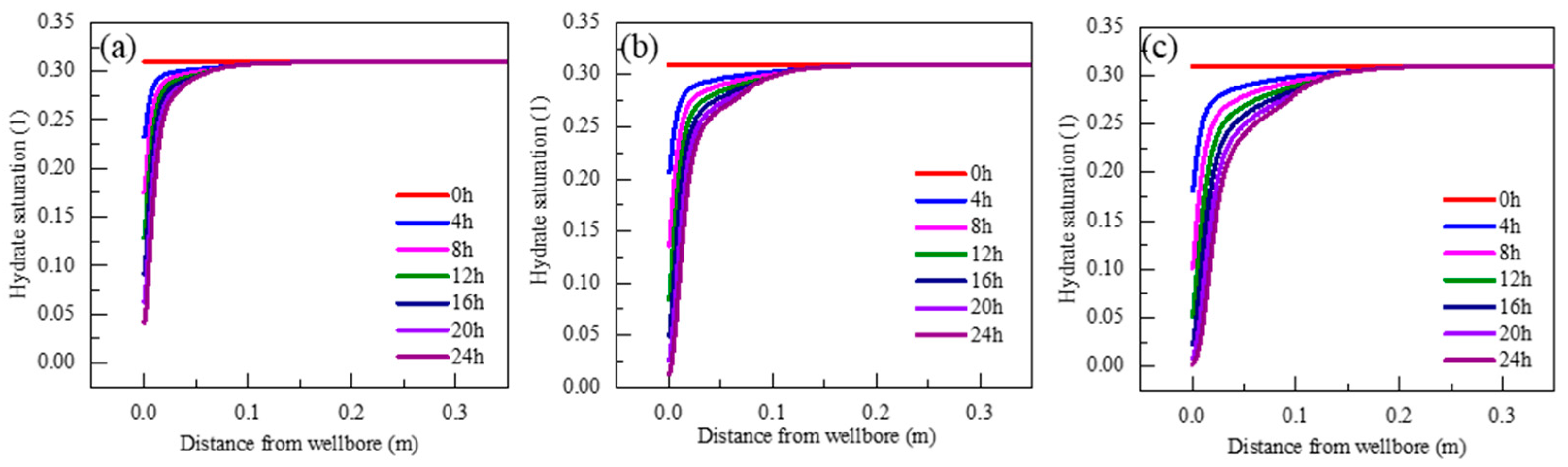
- The increase in wellbore temperature results in the rapid decomposition of hydrates near the wellbore interface, thus causing wellbore instability. In the process of hydrate decomposition disturbed by temperature, the extension speed of the hydrate decomposition front is approximately linear, which is less affected by the temperature difference, and the decomposition speed is slow. The decomposition rate of the hydrate near the borehole wall is high, and its interfacial migration speed significantly accelerates with an increase in temperature difference.
- (3)
- Under the conditions of drilling parameters simulated in this study, the interface with hydrate saturation greater than 0.15 has good consistency with the unstable interface, which can be used to predict the stability of wellbores. If the saturation of the hydrate reservoir is low, the drilling process risks wellbore instability.
- (4)
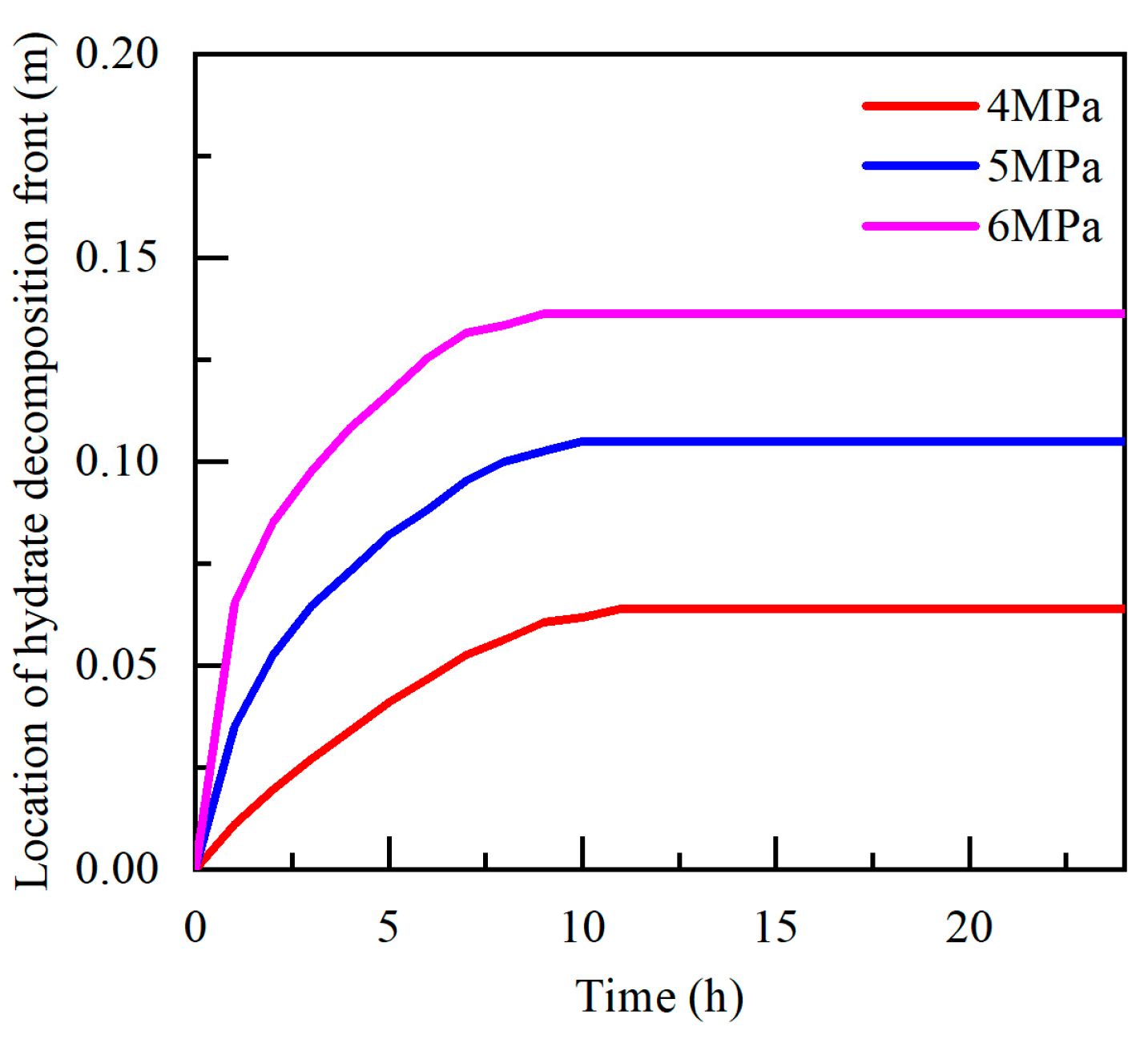
- A decrease in wellbore pressure directly results in wellbore instability. Under a decrease in pressure, hydrate decomposition is slow, and the instability area gradually expands over time. The pressure increase is more conducive to maintaining the stability of the near–wellbore interface. During the drilling process, properly increasing the bottom hole pressure is conducive to maintaining the stability of the well wall during the drilling process of the hydrate reservoir.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Kvenvolden, K.A. A Review of the Geochemistry of Methane in Natural Gas Hydrate; Elsevier Ltd: Oxford, UK, 1995; Volume 23, pp. 997–1008. [Google Scholar]
- Makogon, Y.F. Natural gas hydrates—A promising source of energy. J. Nat. Gas Sci. Eng. 2010, 2, 49–59. [Google Scholar] [CrossRef]
- Yin, Z.; Linga, P. Methane hydrates: A future clean energy resource. Chin. J. Chem. Eng. 2019, 27, 2026–2036. [Google Scholar] [CrossRef]
- Chong, Z.R.; Yang, S.H.B.; Babu, P.; Linga, P.; Li, X. Review of natural gas hydrates as an energy resource: Prospects and challenges. Appl. Energy 2016, 162, 1633–1652. [Google Scholar] [CrossRef]
- Li, X.; Xu, C.; Zhang, Y.; Ruan, X.; Li, G.; Wang, Y. Investigation into gas production from natural gas hydrate: A review. Appl. Energy 2016, 172, 286–322. [Google Scholar] [CrossRef]
- Makogon, Y.F.; Holditch, S.A.; Makogon, T.Y. Natural gas-hydrates—A potential energy source for the 21st Century. J. Pet. Sci. Eng. 2007, 56, 14–31. [Google Scholar] [CrossRef]
- Rutqvist, J.; Moridis, G.J.; Grover, T.; Silpngarmlert, S.; Collett, T.S.; Holdich, S.A. Coupled multiphase fluid flow and wellbore stability analysis associated with gas production from oceanic hydrate-bearing sediments. J. Pet. Sci. Eng. 2012, 92, 65–81. [Google Scholar] [CrossRef]
- Johnson, J.E.; MacLeod, D.R.; Phillips, S.C.; Phillips, M.P.; Divins, D.L. Primary deposition and early diagenetic effects on the high saturation accumulation of gas hydrate in a silt dominated reservoir in the Gulf of Mexico. Mar. Geol. 2022, 444, 106718. [Google Scholar] [CrossRef]
- Moridis, G.J.; Kim, J.; Reagan, M.T.; Kim, S.-J. System response during short- and long-term gas production from a gas hydrate deposit at the site of a planned field test in the Ulleung basin of the Korean East Sea. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 5–8 May 2014. [Google Scholar]
- Moridis, G.J.; Reagan, M.T.; Queiruga, A.F.; Boswell, R. Evaluation of the performance of the oceanic hydrate accumulation at site NGHP-02-09 in the Krishna-Godavari Basin during a production test and during single and multi-well production scenarios. Mar. Pet. Geol. 2019, 108, 660–696. [Google Scholar] [CrossRef]
- Qiu, K.; Yamamoto, K.; Birchwood, R.; Chen, Y. Well-integrity evaluation for methane-hydrate production in the deepwater Nankai Trough. SPE Drill. Complet. 2015, 30, 52–67. [Google Scholar] [CrossRef]
- Bazaluk, O.; Sai, K.; Lozynskyi, V.; Petlovanyi, M.; Saik, P. Research into Dissociation Zones of Gas Hydrate Deposits with a Heterogeneous Structure in the Black Sea. Energies 2021, 14, 1345. [Google Scholar] [CrossRef]
- Liao, Y.Q.; Sun, X.H.; Sun, B.J.; Wang, Z.Y.; Zhang, J.B.; Lou, W.Q. Wellhead backpressure control strategies and outflow response characteristics for gas kick during managed pressure drilling. J. Nat. Gas Sci. Eng. 2020, 75, 103164. [Google Scholar] [CrossRef]
- Wang, Z.; Zhao, Y.; Sun, B.; Chen, L.; Zhang, J.; Wang, X. Modeling of Hydrate Blockage in Gas-Dominated Systems. Energy Fuels 2016, 30, 4653–4666. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, J.; Sun, B.; Chen, L.; Zhao, Y.; Fu, W. A new hydrate deposition prediction model for gas-dominated systems with free water. Chem. Eng. Sci. 2017, 163, 145–154. [Google Scholar] [CrossRef]
- Boswell, R.; Collett, T.; Frye, M.; Shedd, W.; McConnell, D.; Shelander, D. Subsurface gas hydrates in the northern Gulf of Mexico. Mar. Pet. Geol. 2012, 34, 4–30. [Google Scholar] [CrossRef]
- McConnell, D.R.; Zhang, Z.; Boswell, R. Review of progress in evaluating gas hydrate drilling hazards. Mar. Pet. Geol. 2012, 34, 209–223. [Google Scholar] [CrossRef]
- Kim, J.; Moridis, G.J.; Rutqvist, J. Coupled flow and geomechanical analysis for gas production in the Prudhoe Bay Unit L-106 well Unit C gas hydrate deposit in Alaska. J. Pet. Sci. Eng. 2012, 92, 143–157. [Google Scholar] [CrossRef]
- Hoseinpour, M.; Riahi, M.A. Determination of the mud weight window, optimum drilling trajectory, and wellbore stability using geomechanical parameters in one of the Iranian hydrocarbon reservoirs. J. Pet. Explor. Prod. Technol. 2022, 12, 63–82. [Google Scholar] [CrossRef]
- Liu, C.; Han, Y.; Phan, D.T.; Abousleiman, Y.N. Stress solutions for short- and long-term wellbore stability analysis. J. Nat. Gas Sci. Eng. 2022, 105, 104693. [Google Scholar] [CrossRef]
- Petlovanyi, M.; Lozynskyi, V.; Saik, P.; Sai, K. Predicting the Producing Well Stability in the Place of its Curving at the Underground Coal Seams Gasification; EDP Sciences: Berdiansk, Ukraine, 2019. [Google Scholar]
- Freij-Ayoub, R.; Tan, C.; Clennell, B.; Tohidi, B.; Yang, J. A wellbore stability model for hydrate bearing sediments. J. Pet. Sci. Eng. 2007, 57, 209–220. [Google Scholar] [CrossRef]
- Cheng, Y.; Li, L.; Mahmood, S.; Cui, Q. Fluid-solid coupling model for studying wellbore instability in drilling of gas hydrate bearing sediments. Appl. Math. Mech. 2013, 34, 1421–1432. [Google Scholar] [CrossRef]
- Wang, X.; Sun, B.; Gao, Y.; Wang, Z.; Li, H.; Chen, Y. Numerical simulation of the stability of hydrate layer during well cementing in deep-water region. J. Pet. Sci. Eng. 2019, 176, 893–905. [Google Scholar] [CrossRef]
- Sun, J.; Ning, F.; Lei, H.; Gai, X.; Sánchez, M.; Lu, J.; Li, Y.; Liu, L.; Liu, C.; Wu, N.; et al. Wellbore stability analysis during drilling through marine gas hydrate-bearing sediments in Shenhu area: A case study. J. Pet. Sci. Eng. 2018, 170, 345–367. [Google Scholar] [CrossRef]
- Li, Y.; Cheng, Y.; Yan, C.; Song, L.; Liu, H.; Tian, W.; Ren, X. Mechanical study on the wellbore stability of horizontal wells in natural gas hydrate reservoirs. J. Nat. Gas Sci. Eng. 2020, 79, 103359. [Google Scholar] [CrossRef]
- Liao, Y.Q.; Wang, Z.Y.; Chao, M.Z.; Sun, X.H.; Wang, J.T.; Zhou, B.Y.; Sun, B.J. Coupled wellbore-reservoir heat and mass transfer model for horizontal drilling through hydrate reservoir and application in wellbore stability analysis. J. Nat. Gas Sci. Eng. 2021, 95, 104216. [Google Scholar] [CrossRef]
- Cheng, W.; Ning, F.; Sun, J.; Liu, Z.; Jiang, G.; Li, X. A porothermoelastic wellbore stability model for riserless drilling through gas hydrate-bearing sediments in the Shenhu area of the South China Sea. J. Nat. Gas Sci. Eng. 2019, 72, 103036. [Google Scholar] [CrossRef]
- Gurtin, M.E. On a framework for small-deformation viscoplasticity: Free energy, microforces, strain gradients. Int. J. Plasticity 2003, 19, 47–90. [Google Scholar] [CrossRef]
- Zhang, H.W.; Xie, Z.Q.; Chen, B.S.; Xing, H.L. A finite element model for 2D elastic–plastic contact analysis of multiple Cosserat materials. Eur. J. Mech.-A/Solids 2012, 31, 139–151. [Google Scholar] [CrossRef]
- Bishop, A.W.; Blight, G.E. Some aspects of effective stress in saturated and partly saturated soils. Géotechnique 1963, 13, 177–197. [Google Scholar] [CrossRef]
- Yoneda, J.; Masui, A.; Konno, Y.; Jin, Y.; Egawa, K.; Kida, M.; Ito, T.; Nagao, J.; Tenma, N. Mechanical properties of hydrate-bearing turbidite reservoir in the first gas production test site of the Eastern Nankai Trough. Mar. Pet. Geol. 2015, 66, 471–486. [Google Scholar] [CrossRef]
- Wu, Y.; Liao, J.; Zhang, W.; Cui, J. Characterization of stress–dilatancy behavior for methane hydrate-bearing sediments. J. Nat. Gas Sci. Eng. 2021, 92, 104000. [Google Scholar] [CrossRef]
- Hyodo, M.; Yoneda, J.; Yoshimoto, N.; Nakata, Y. Mechanical and dissociation properties of methane hydrate-bearing sand in deep seabed. Soils Found. 2013, 53, 299–314. [Google Scholar] [CrossRef]
- Li, Z.D.; Tian, X.; Li, Z.; Xu, J.Z.; Zhang, H.X.; Wang, D.J. Experimental study on growth characteristics of pore-scale methane hydrate. Energy Rep. 2020, 6, 933–943. [Google Scholar] [CrossRef]
- Hardwick, J.S.; Mathias, S.A. Masuda’s sandstone core hydrate dissociation experiment revisited. Chem. Eng. Sci. 2018, 175, 98–109. [Google Scholar] [CrossRef]
- Parker, J.C.; Lenhard, R.J.; Kuppusamy, T. A parametric model for constitutive properties governing multiphase flow in porous media. Water Resour. Res. 1987, 23, 618–624. [Google Scholar] [CrossRef]
- Levitus, S.; Boyer, T.P. World Ocean Atlas 1994. Volume 4. Temperature. In United States. 1994; Web. Available online: https://www.osti.gov/biblio/137203 (accessed on 8 November 2023).
- COMSOL Multiphysics®, v. 6.0. cn.comsol.com; COMSOL AB: Stockholm, Sweden, 2021. [Google Scholar]
- Kim, H.C.; Bishnoi, P.R.; Heidemann, R.A.; Rizvi, S.S.H. Kinetics of methane hydrate decomposition. Chem. Eng. Sci. 1987, 42, 1645–1653. [Google Scholar] [CrossRef]
- Liao, B.; Wang, J.; Sun, J.; Lv, K.; Liu, L.; Wang, Q.; Wang, R.; Lv, X.; Wang, Y.; Chen, Z. Microscopic insights into synergism effect of different hydrate inhibitors on methane hydrate formation: Experiments and molecular dynamics simulations. Fuel 2023, 340, 127488. [Google Scholar] [CrossRef]
- Dickens, G.; Hunt, M.Q. Methane hydrate stability in seawate. Geophys. Res. Lett. 1994, 19, 2115–2118. [Google Scholar] [CrossRef]


| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Sea water depth | 1225.23 m | Wellbore diameter | 311.15 mm |
| Hydrate reservoir depth | 1433.03–1478.63 m | Drilling fluid density | 1190 kg/m3 |
| Porosity | 0.45 | Drilling rate | 40 m/h |
| Average permeability | 10 mD | Output volume | 50 L/s |
| Mudline temperature | 4 °C | Wellhead temperature | 25 °C |
| Inside diameter of riser | 339.7 mm | Geothermal gradient | 0.0469 °C/m |
| Parameter | Value |
|---|---|
| Depth below mudline | 255 m |
| Pore pressure of the hydrate layer | 15.25 MPa |
| Initial temperature of hydrate layer | 17.37 °C |
| Hydrate layer saturation | 0.31 |
| Drilling fluid pressure in the well | 16.49 MPa |
| Drilling fluid temperature in the well | 15.35 °C |
| Vertical initial geostress | 19.65 MPa |
| Horizontal initial geostress | 17.74 MPa |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Sun, B.; Ma, B.; Li, H.; Liu, H.; Cai, D.; Wang, X.; Li, X. Study on the Evolution Law of Wellbore Stability Interface during Drilling of Offshore Gas Hydrate Reservoirs. Energies 2023, 16, 7585. https://doi.org/10.3390/en16227585
Li X, Sun B, Ma B, Li H, Liu H, Cai D, Wang X, Li X. Study on the Evolution Law of Wellbore Stability Interface during Drilling of Offshore Gas Hydrate Reservoirs. Energies. 2023; 16(22):7585. https://doi.org/10.3390/en16227585
Chicago/Turabian StyleLi, Xuefeng, Baojiang Sun, Baojin Ma, Hao Li, Huaqing Liu, Dejun Cai, Xiansi Wang, and Xiangpeng Li. 2023. "Study on the Evolution Law of Wellbore Stability Interface during Drilling of Offshore Gas Hydrate Reservoirs" Energies 16, no. 22: 7585. https://doi.org/10.3390/en16227585
APA StyleLi, X., Sun, B., Ma, B., Li, H., Liu, H., Cai, D., Wang, X., & Li, X. (2023). Study on the Evolution Law of Wellbore Stability Interface during Drilling of Offshore Gas Hydrate Reservoirs. Energies, 16(22), 7585. https://doi.org/10.3390/en16227585





