Double-Coupling Resonant Network for Dynamic IPT Systems Used in EV Charging Applications
Abstract
:1. Introduction
2. Single-Coupling Resonant Networks for DIPT Systems
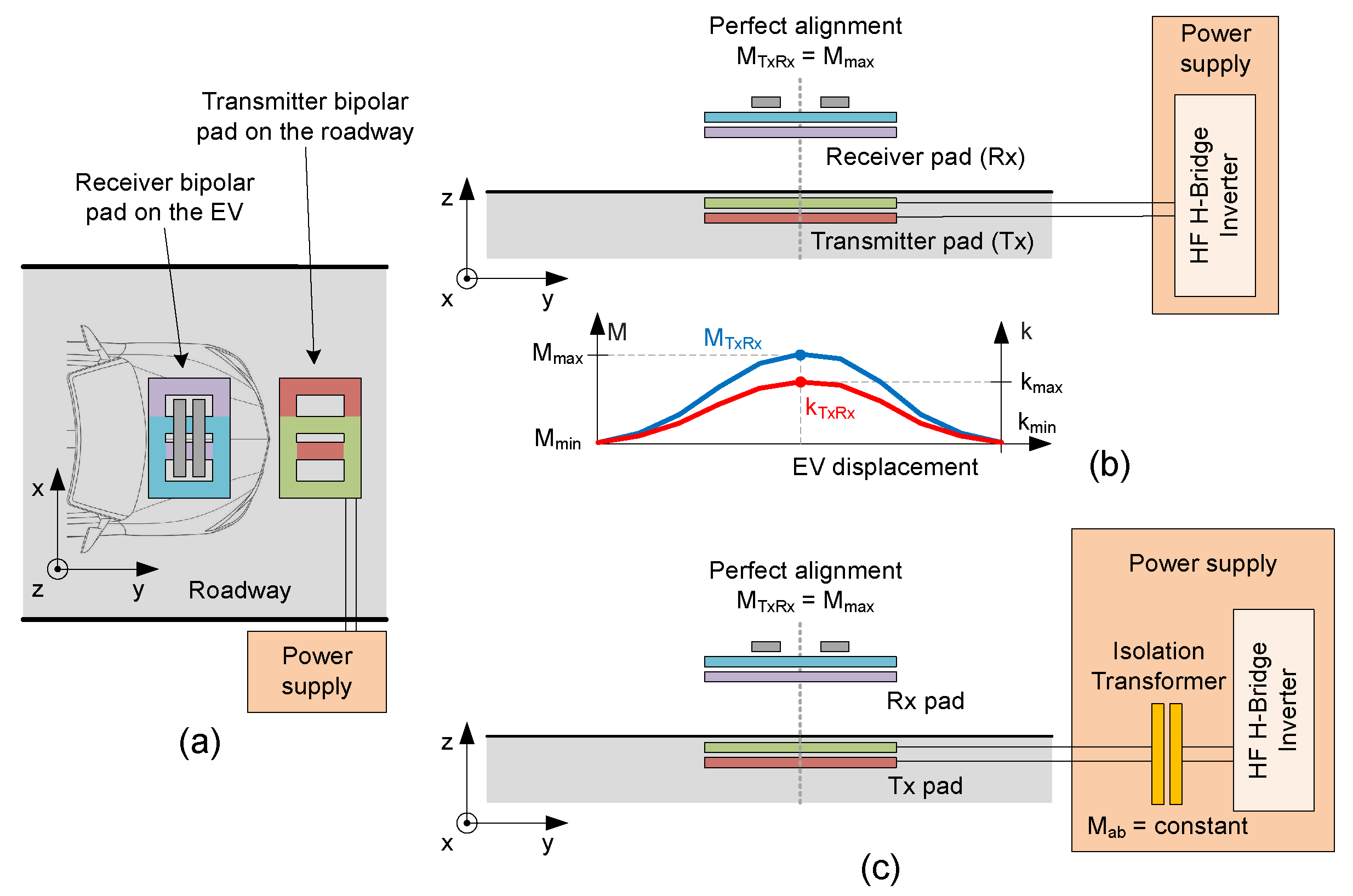
2.1. DIPT System Description
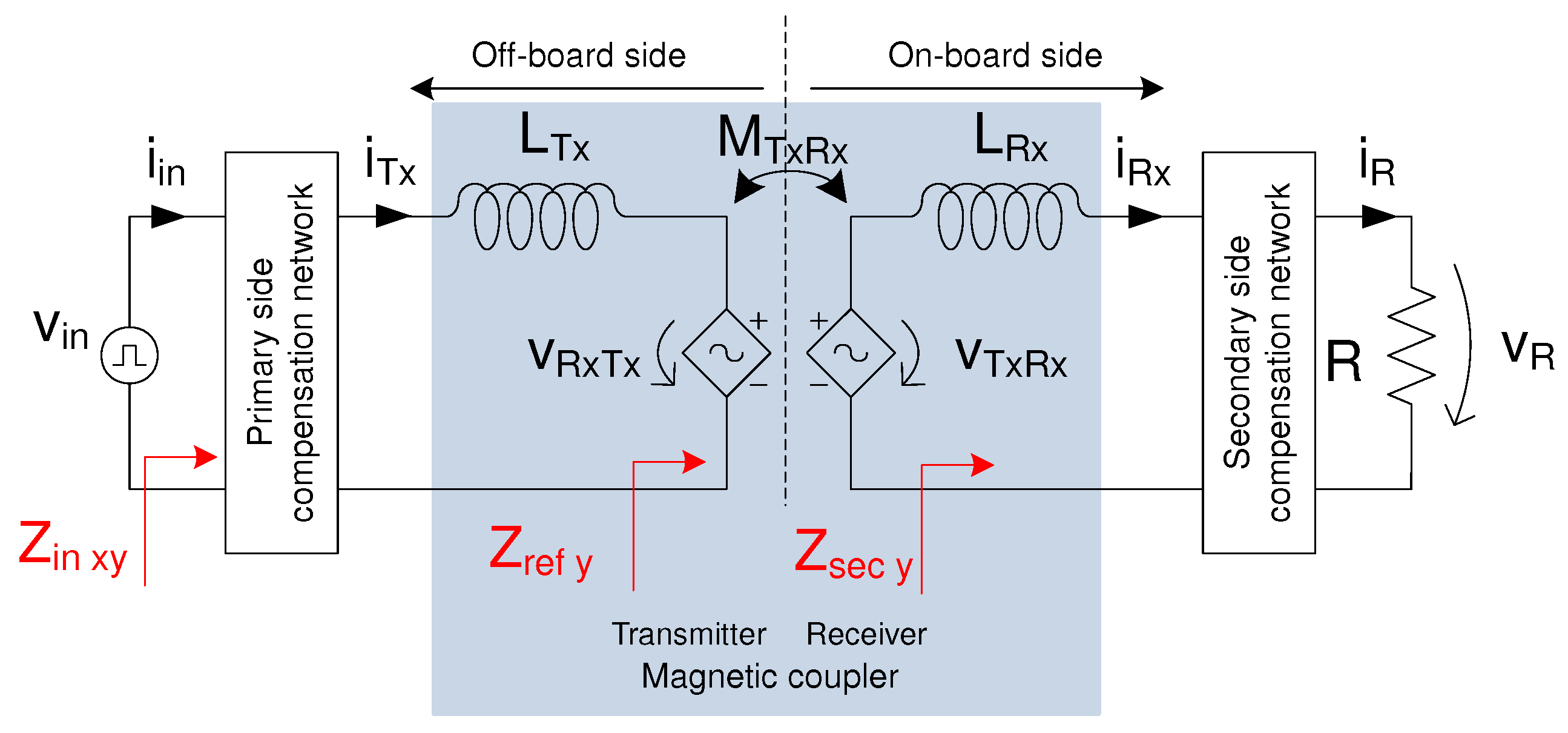
2.2. Circuit Considerations and Definitions
2.3. Impedance Definitions and Resonant Operation
2.3.1. Secondary-Side Topologies
2.3.2. Primary-Side Topologies
2.4. Impact of Compensation Networks in DIPT Systems
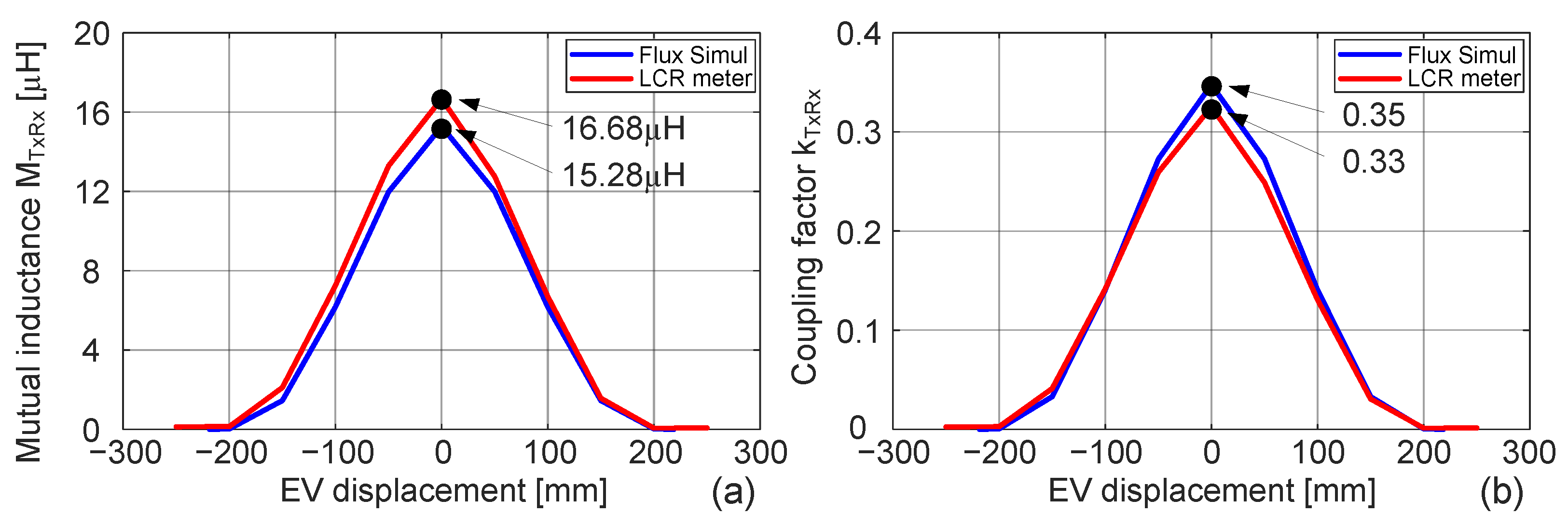
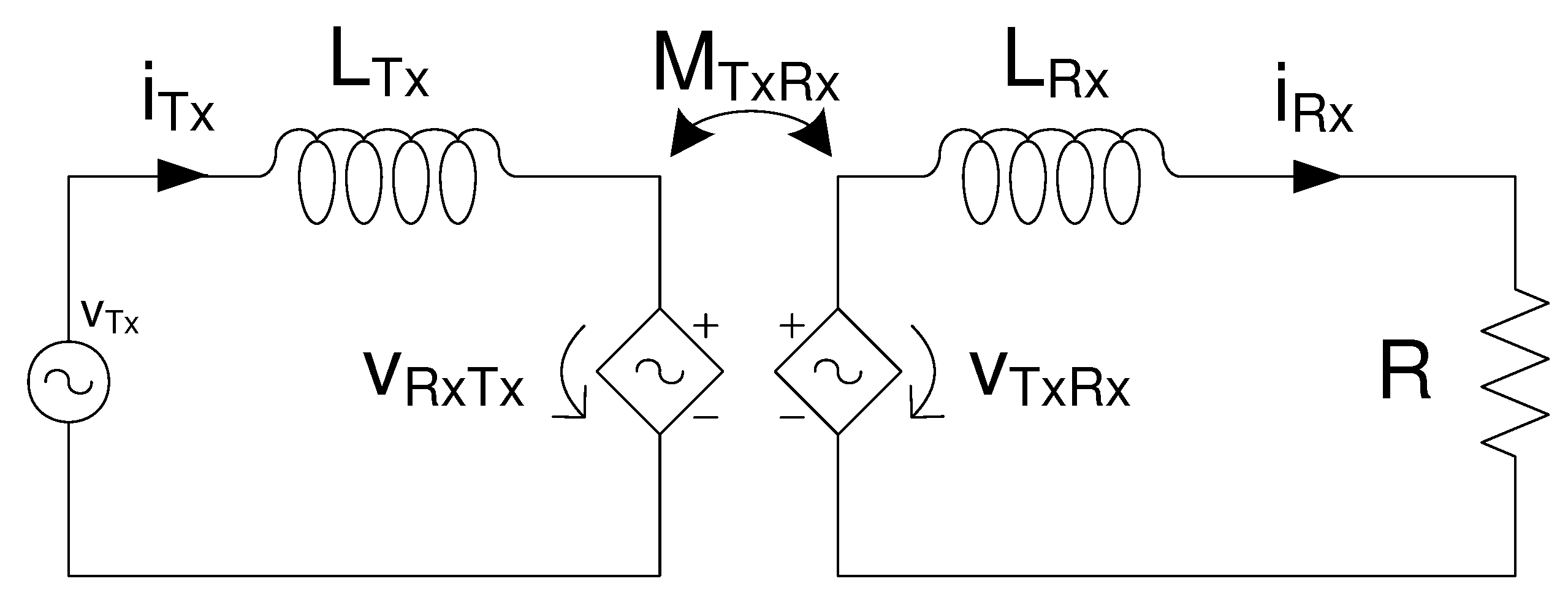
2.4.1. MC Characterization
2.4.2. Assessment of Compensation Network Behavior
3. Double-Coupling Resonant Network for DIPT System
3.1. Circuital Analysis
3.2. Load, No-Load, and Coupling Impact for the SSS Configuration
3.2.1. No-Coupling Mode
- (i)
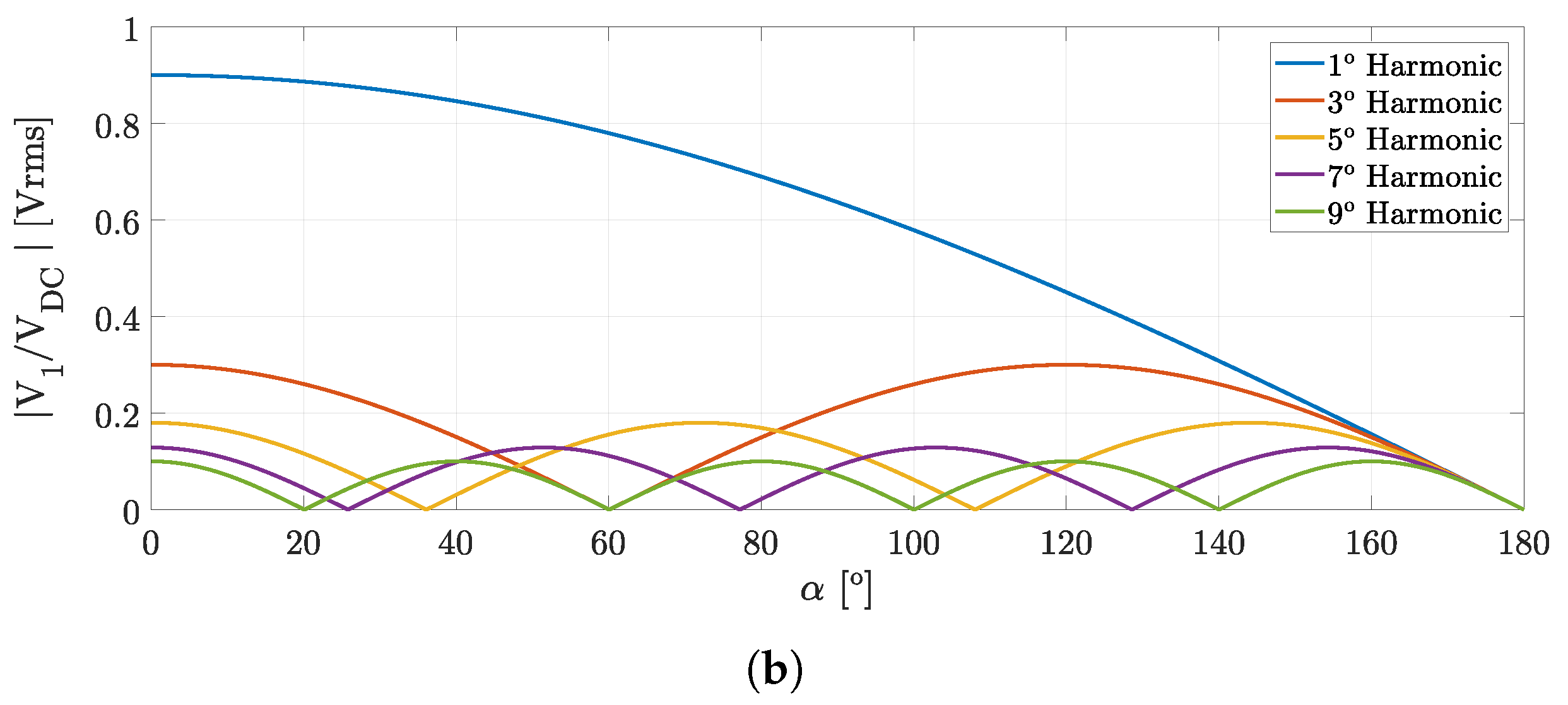
- The use of an MC1 with larger self-inductance values results in higher values at the harmonic frequencies, and consequently, it reduces the corresponding current component values;
- (ii)
- The increase of moves the inflection point closer to the harmonic frequencies. Therefore, high coupled MC1 will exhibit larger values due to the harmonic values, especially the 3rd and 5th components.
3.2.2. Coupling Mode
- Current source characteristics in the intermediary network ();
- Load-independent voltage source characteristics between output/input voltages ();
- Load/no-load: limits ;
- Coupling/no-coupling: limits ;
- coupling and load-independence.
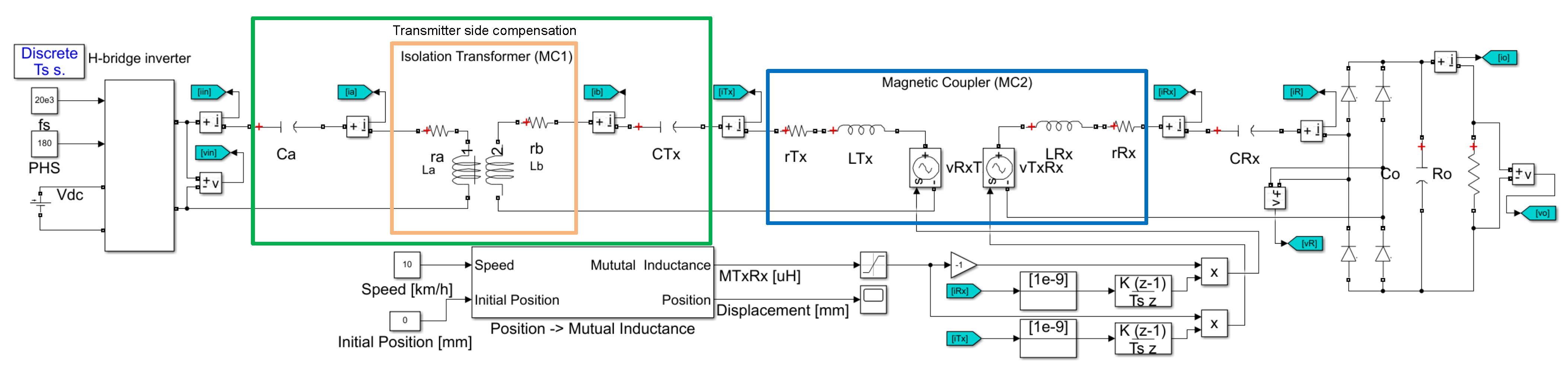
4. MC Model and Simulation Results
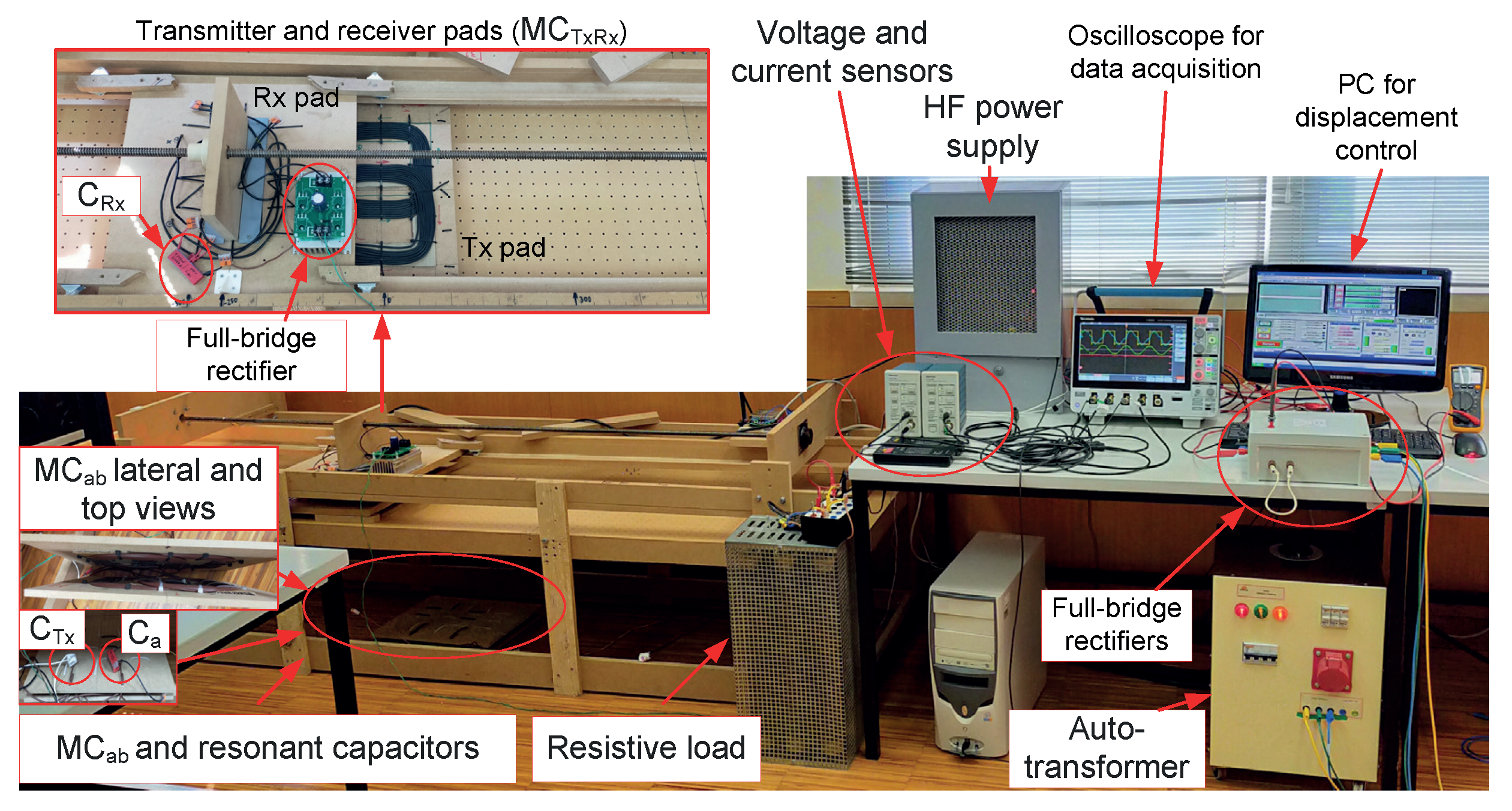
5. Experimental Validation
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| AC | Alternate Current |
| BPP | Bipolar Pad |
| CC | Constant Current |
| CV | Constant Voltage |
| DC | Direct Current |
| DIPT | Dynamic Inductive Power Transfer |
| EV | Electric Vehicle |
| FEA | Finite Element Analysis |
| FHA | First Harmonic Approximation |
| HF | High-Frequency |
| IPT | Inductive Power Transfer |
| MC | Magnetic Coupler |
| P | Parallel |
| PP | Parallel-Parallel |
| PS | Parallel-Series |
| SIPT | Static Inductive Power Transfer |
| S | Series |
| SP | Series-Parallel |
| SS | Series-Series |
| SSS | Series-Series-Series |
| THD | Total Harmonic Distortion |
| WPT | Wireless Power Transfer |
| ZCS | Zero Current Switching |
| ZPA | Zero Phase Angle |
| ZVS | Zero Voltage Switching |
References
- Khalid, M.R.; Khan, I.A.; Hameed, S.; Asghar, M.S.J.; Ro, J.-S. A Comprehensive Review on Structural Topologies, Power Levels, Energy Storage Systems, and Standards for Electric Vehicle Charging Stations and Their Impacts on Grid. IEEE Access 2021, 9, 128069–128094. [Google Scholar] [CrossRef]
- Panchal, C.; Stegen, S.; Lu, J. Review of static and dynamic wireless electric vehicle charging system. Eng. Sci. Technol. Int. J. 2018, 21, 922–937. [Google Scholar] [CrossRef]
- Marques, E.G.; Mendes, A.M.S. Optimization of transmitter magnetic structures for roadway applications. In Proceedings of the 2017 IEEE Applied Power Electronics Conference and Exposition (APEC), Tampa, FL, USA, 26–30 March 2017; pp. 959–965. [Google Scholar] [CrossRef]
- Marques, E.G.; Mendes, A.M.S.; Perdigão, M.S.; Costa, V.S. Design Methodology of a Three Coil IPT System with Parameters Identification for EVs. IEEE Trans. Veh. Technol. 2021, 70, 7509–7521. [Google Scholar] [CrossRef]
- Nagendra, G.R.; Chen, L.; Covic, G.A.; Boys, J.T. Detection of EVs on IPT Highways. IEEE J. Emerg. Sel. Top. Power Electron. 2014, 2, 584–597. [Google Scholar] [CrossRef]
- Covic, G.A.; Boys, J.T. Inductive Power Transfer. Proc. IEEE 2013, 101, 1276–1289. [Google Scholar] [CrossRef]
- Ramezani, A.; Narimani, M. A Dynamic Wireless Charging System with a Robust Output Voltage Respect to Misalignment. In Proceedings of the 2019 IEEE PELS Workshop on Emerging Technologies: Wireless Power Transfer (WoW), London, UK, 18–21 June 2019; pp. 292–296. [Google Scholar] [CrossRef]
- Tavakoli, R.; Pantic, Z. ANN-based algorithm for estimation and compensation of lateral misalignment in dynamic wireless power transfer systems for EV charging. In Proceedings of the 2017 IEEE Energy Conversion Congress and Exposition (ECCE), Cincinnati, OH, USA, 1–5 October 2017; pp. 2602–2609. [Google Scholar] [CrossRef]
- Barsari, V.Z.; Thrimawithana, D.J.; Covic, G.A. An Inductive Coupler Array for In-Motion Wireless Charging of Electric Vehicles. IEEE Trans. Power Electron. 2021, 36, 9854–9863. [Google Scholar] [CrossRef]
- Karakitsios, I.; Palaiogiannis, F.; Markou, A.; Hatziargyriou, N.D. Optimizing the Energy Transfer, With a High System Efficiency in Dynamic Inductive Charging of EVs. IEEE Trans. Veh. Technol. 2018, 67, 4728–4742. [Google Scholar] [CrossRef]
- Jeong, S.Y.; Park, J.H.; Hong, G.P.; Rim, C.T. Autotuning Control System by Variation of Self-Inductance for Dynamic Wireless EV Charging with Small Air Gap. IEEE Trans. Power Electron. 2019, 34, 5165–5174. [Google Scholar] [CrossRef]
- Gati, E.; Kampitsis, G.; Manias, S. Variable Frequency Controller for Inductive Power Transfer in Dynamic Conditions. IEEE Trans. Power Electron. 2017, 32, 1684–1696. [Google Scholar] [CrossRef]
- Kamineni, A.; Covic, G.A.; Boys, J.T. Interoperable EV detection for dynamic wireless charging with existing hardware and free resonance. In Proceedings of the 2016 IEEE PELS Workshop on Emerging Technologies: Wireless Power Transfer (WoW), Knoxville, TN, USA, 4–6 October 2016; pp. 169–173. [Google Scholar] [CrossRef]
- Wang, C.H.; Stielau, O.H.; Covic, G.A. Design considerations for a contactless electric vehicle battery charger. IEEE Trans. Ind. Electron. 2005, 52, 1308–1314. [Google Scholar] [CrossRef]
- Shevchenko, V.; Husev, O.; Strzelecki, R.; Pakhaliuk, B.; Poliakov, N.; Strzelecka, N. Compensation Topologies in IPT Systems: Standards, Requirements, Classification, Analysis, Comparison and Application. IEEE Access 2019, 7, 120559–120580. [Google Scholar] [CrossRef]
- Patil, D.; McDonough, M.K.; Miller, J.M.; Fahimi, B.; Balsara, P.T. Wireless Power Transfer for Vehicular Applications: Overview and Challenges. IEEE Trans. Transp. Electrif. 2018, 4, 3–37. [Google Scholar] [CrossRef]
- Sonapreetha, M.R.; Jeong, S.Y.; Choi, S.Y.; Rim, C.T. Dual-purpose non-overlapped coil sets as foreign object and vehicle location detections for wireless stationary EV chargers. In Proceedings of the 2015 IEEE PELS Workshop on Emerging Technologies: Wireless Power (2015 WoW), Daejeon, Republic of Korea, 5–6 June 2015; pp. 1–7. [Google Scholar] [CrossRef]
- Wang, H.; Pratik, U.; Jovicic, A.; Hasan, N.; Pantic, Z. Dynamic Wireless Charging of Medium Power and Speed Electric Vehicles. IEEE Trans. Veh. Technol. 2021, 70, 12552–12566. [Google Scholar] [CrossRef]
- Barsari, V.Z.; Thrimawithana, D.J.; Covic, G.A.; Kim, S. A Switchable Inductively Coupled Connector for IPT Roadway Applications. In Proceedings of the 2020 IEEE PELS Workshop on Emerging Technologies: Wireless Power Transfer (WoW), Seoul, Republic of Korea, 15–19 November 2020; pp. 35–39. [Google Scholar] [CrossRef]
- Chen, L.; Nagendra, G.R.; Boys, J.T.; Covic, G.A. Double-Coupled Systems for IPT Roadway Applications. IEEE J. Emerg. Sel. Top. Power Electron. 2015, 3, 37–49. [Google Scholar] [CrossRef]
- Wireless Power Transfer for Light-Duty Plug-In/Electric Vehicles and Alignment Methodology; SAE International: Warrendale, PA, USA, 2016.
- Feng, H.; Cai, T.; Duan, S.; Zhao, J.; Zhang, X.; Chen, C. An LCC-Compensated Resonant Converter Optimized for Robust Reaction to Large Coupling Variation in Dynamic Wireless Power Transfer. IEEE Trans. Ind. Electron. 2016, 63, 6591–6601. [Google Scholar] [CrossRef]
- Marques, E.G.; Costa, V.S.; Torres, M.; Rios, B.; Mendes, A.M.S.; Perdigão, M.S. Double Coupling IPT Systems for EV Charging Applications. In Proceedings of the 2021 IEEE Vehicle Power and Propulsion Conference (VPPC), Gijon, Spain, 25–28 October 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Wu, H.H.; Gilchrist, A.; Sealy, K.D.; Bronson, D. A High Efficiency 5 kW Inductive Charger for EVs Using Dual Side Control. IEEE Trans. Ind. Inform. 2012, 8, 585–595. [Google Scholar] [CrossRef]
- Ahmad, A.; Alam, M.S.; Chabaan, R. A Comprehensive Review of Wireless Charging Technologies for Electric Vehicles. IEEE Trans. Transp. Electrif. 2018, 4, 38–63. [Google Scholar] [CrossRef]
- Navidi, T.; Cao, Y.; Krein, P.T. Analysis of wireless and catenary power transfer systems for electric vehicle range extension on rural highways. In Proceedings of the 2016 IEEE Power and Energy Conference at Illinois (PECI), Urbana, IL, USA, 19–20 February 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Stielau, O.H.; Covic, G.A. Design of loosely coupled inductive power transfer systems. In Proceedings of the 2000 International Conference on Power System Technology (PowerCon 2000), Proceedings (Cat. No.00EX409), Perth, Australia, 4–7 December 2000; Volume 1, pp. 85–90. [Google Scholar] [CrossRef]
- Wang, C.H.; Covic, G.A.; Stielau, O.H. Power transfer capability and bifurcation phenomena of loosely coupled inductive power transfer systems. IEEE Trans. Ind. Electron. 2004, 51, 148–157. [Google Scholar] [CrossRef]
- Wu, H.H.; Gilchrist, A.; Sealy, K.; Israelsen, P.; Muhs, J. Design of Symmetric Voltage Cancellation Control for LCL converters in Inductive Power Transfer Systems. In Proceedings of the 2011 IEEE International Electric Machines & Drives Conference (IEMDC), Niagara Falls, ON, Canada, 15–18 May 2011; pp. 866–871. [Google Scholar] [CrossRef]







| Type | Energy Efficiency [%] | Energy Density [Wh/kg] | Power Density [W/kg] | Life Cycles | Self-Discharge |
|---|---|---|---|---|---|
| Pb-Acid | 70–80 | 20–35 | 25 | 200–2000 | Low |
| Ni-Cd | 60–90 | 40–60 | 140–180 | 500–2000 | Low |
| Ni-MH | 50–80 | 60–80 | 220 | <3000 | High |
| Li-Ion | 70–85 | 100–200 | 360 | 500–2000 | Medium |
| Li-Polymer | 70 | 200 | 250–1000 | >1200 | Medium |
| Company | Output Power [kW] | Application | Vehicle Type |
|---|---|---|---|
| Witricity | 11 | Static | EVs |
| Brusa | 11 | Static | EVs |
| ENRX | 100 | Static | EVs and Buses |
| ENRX | 180 | Dynamic | Buses |
| WAVE | 250 | Static | Buses |
| Research Groups and Universities | |||
| University of Coimbra/IT | 1–3 | Static/Dynamic | EVs |
| University of Auckland | 1–11 | Static/Dynamic | EVs |
| ETH | 50 | Static | Buses |
| ORLN | 120 | Static | EVs and Buses |
| KAIST | 180 | Dynamic | Buses |
| Generic | Tuned Conditions (at Secondary Side Resonance) | ||||
|---|---|---|---|---|---|
| Real | Imag | Real | Imag | Observations | |
| 0 |
- Voltage source characteristics - Null reflected reactance - Primary-side compensation - not affected | ||||
| - Current source characteristics - Reflected reactance - dependent - Primary-side compensation - affected by | |||||
| Real | Imag | Observations | |
|---|---|---|---|
| - Voltage source characteristics - No-load/no-coupling: - Load/coupling: limits at high values - coupling- and load-independent | |||
| - Current source characteristics - Load/No-load: limits - Coupling/no-Coupling: limits - varies with load and coupling - load-independent at | |||
| - Needs input choke inductor - Voltage source characteristics - No-load/no-coupling: - Load/coupling: limits - varies with load and coupling | |||
| - Needs input choke inductor - Current source characteristics - No-load/no-coupling: - Load/coupling: limits - varies with load and coupling | |||
| - Additional in the resonant network - Voltage source characteristics - No-load/no-coupling: - Load/coupling: limits - coupling- and load-independent | |||
| - Additional in the resonant network - Current source characteristics - No-load/no-coupling: limits - Load/coupling: limits - varies with load and coupling | |||
| Parameter\Topology | SS | SP | PS | PP | LCL-S | LCL-P | SSS | |
|---|---|---|---|---|---|---|---|---|
| Independence on and | Transmitter | Yes | No | No | No | Yes | No | Yes |
| Receiver | Yes | No | Yes | No | Yes | No | Yes | |
| Zero coupling allowance | Not allowed | Allowed | Not allowed | Not allowed | Allowed | Allowed | Allowed | |
| Total impedance | Decreases with displacement | Decreases with displacement but limited to value different from zero. | Increases with displacement | Increases with displacement | Increases with displacement | Increases slightly with displacement | Increases with displacement | |
| Load independent output at resonance | Voltage and current | Voltage and current | Only voltage | Only current | Only voltage | Only current | Voltage and current | |
| Resonance affected by | Coupling | No | Yes | Yes | Yes | No | Yes | No |
| Load | No | Yes, but load independent at | Yes | Yes | No | Yes | No | |
| Other advantages | Not suitable for DIPT. | (1) Requires a smaller receiver coil self-inductance than SS. (2) The parallel resonant circuit supplies the stable current. | Not suitable for DIPT. | Not suitable for DIPT. | Current source characteristics at the transmitter pad input. | (1) Galvanic isolation. (2) Constant intermediate coil current. | ||
| Other drawbacks | Lacking DC component blocking. | Additional inductor relatively to classical topologies. | Requires additional isolation transformer and resonant capacitor. | |||||
| Parameter | Values |
|---|---|
| 100 V | |
| 20 kHz | |
| H; H; H | |
| H; H; H] | |
| Resonant capacitors | F nF F |
| F | |
| Theoretical Results | Simulation Results | Experimental Results | Error Simul. vs. Theor. | Error Exp. vs. Simul. | ||
|---|---|---|---|---|---|---|
| Full-coupling and full-load condition | 16.36 A | 15.18 A | 14.58 A | 7.2% | 3.9% | |
| 25.43 A | 22.94 A | 23.96 A | 9.8% | 4.5% | ||
| 48.33 V | 47.52 V | 41.60 V | 1.7% | 12.5% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Costa, V.S.; Mendes, A.M.S.; Marques, E.; Perdigão, M.S. Double-Coupling Resonant Network for Dynamic IPT Systems Used in EV Charging Applications. Energies 2023, 16, 7269. https://doi.org/10.3390/en16217269
Costa VS, Mendes AMS, Marques E, Perdigão MS. Double-Coupling Resonant Network for Dynamic IPT Systems Used in EV Charging Applications. Energies. 2023; 16(21):7269. https://doi.org/10.3390/en16217269
Chicago/Turabian StyleCosta, Valter S., André M. S. Mendes, Emanuel Marques, and Marina S. Perdigão. 2023. "Double-Coupling Resonant Network for Dynamic IPT Systems Used in EV Charging Applications" Energies 16, no. 21: 7269. https://doi.org/10.3390/en16217269
APA StyleCosta, V. S., Mendes, A. M. S., Marques, E., & Perdigão, M. S. (2023). Double-Coupling Resonant Network for Dynamic IPT Systems Used in EV Charging Applications. Energies, 16(21), 7269. https://doi.org/10.3390/en16217269







