Fractal Characteristics of the Low-Gas Permeability Area of a Fully Mechanized Up-Dip Working Face under Different Dip Angles of Rock Strata
Abstract
:1. Introduction
2. Two-Dimensional Simulation Experiment
2.1. Experiment Background
2.2. Experiment Design and Preparation
2.3. Fractal Dimension of Overburden Fractures
3. Results and Analysis
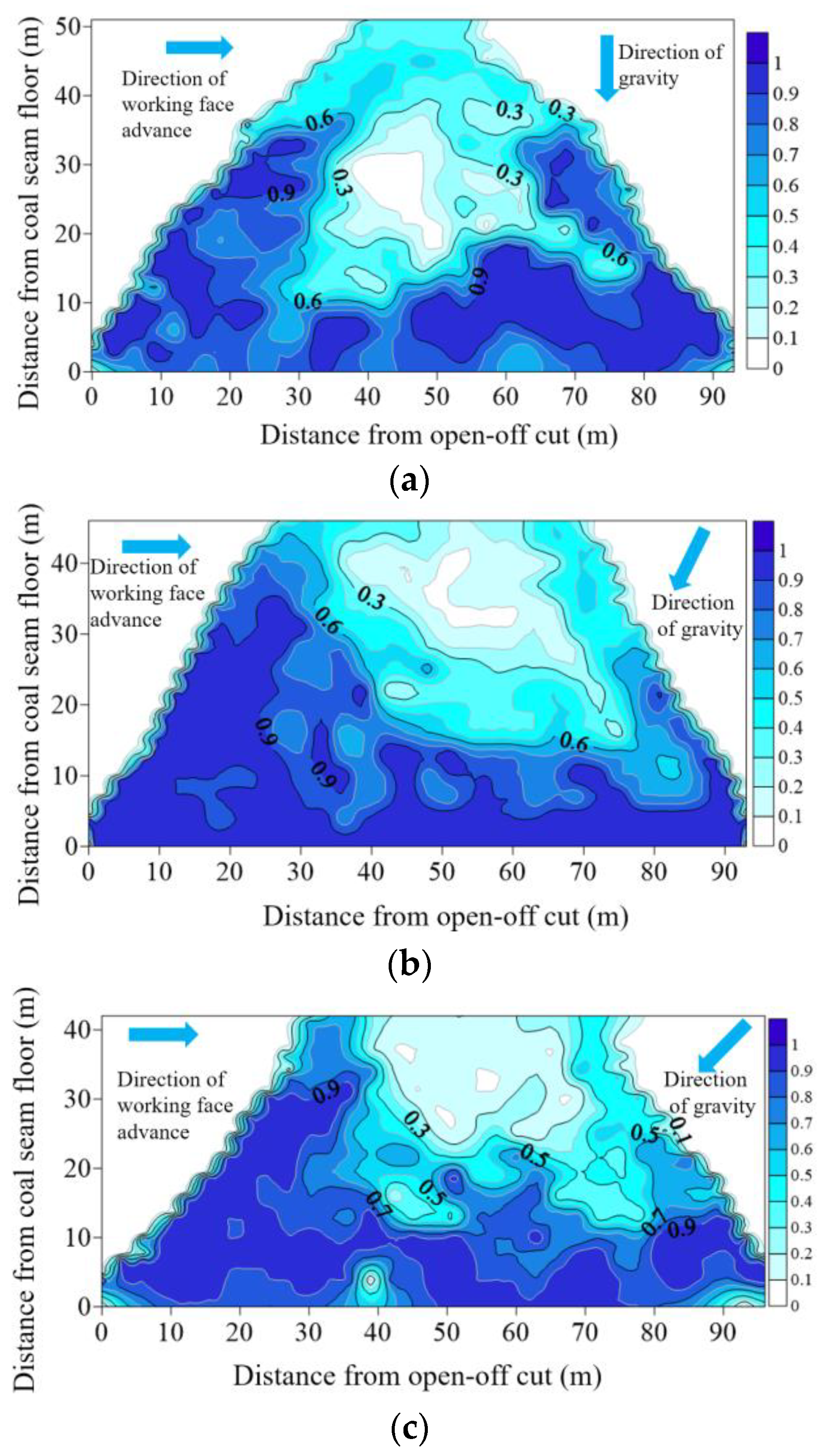
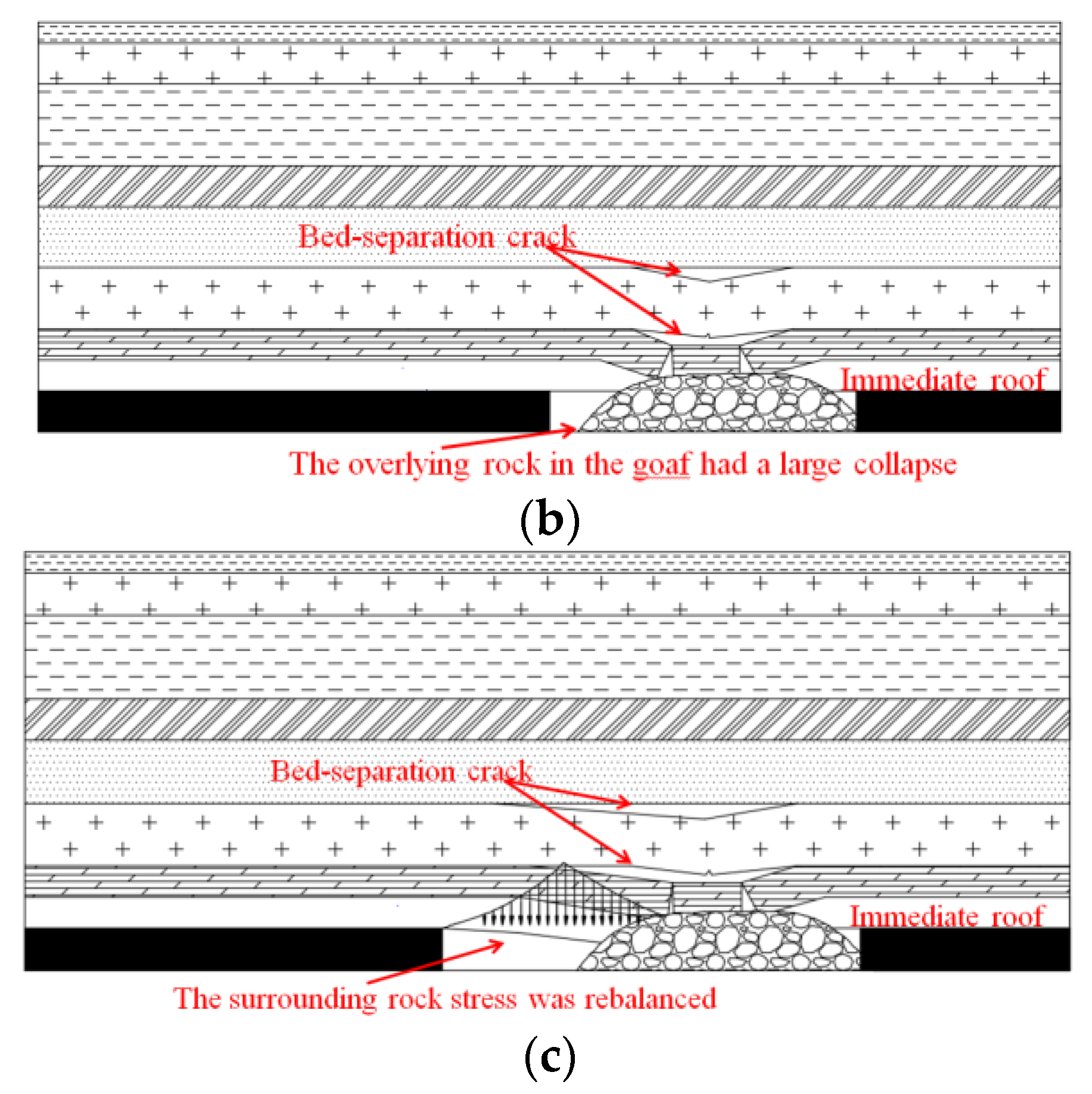
3.1. Distribution Rule of Overburden Penetration in Goaf
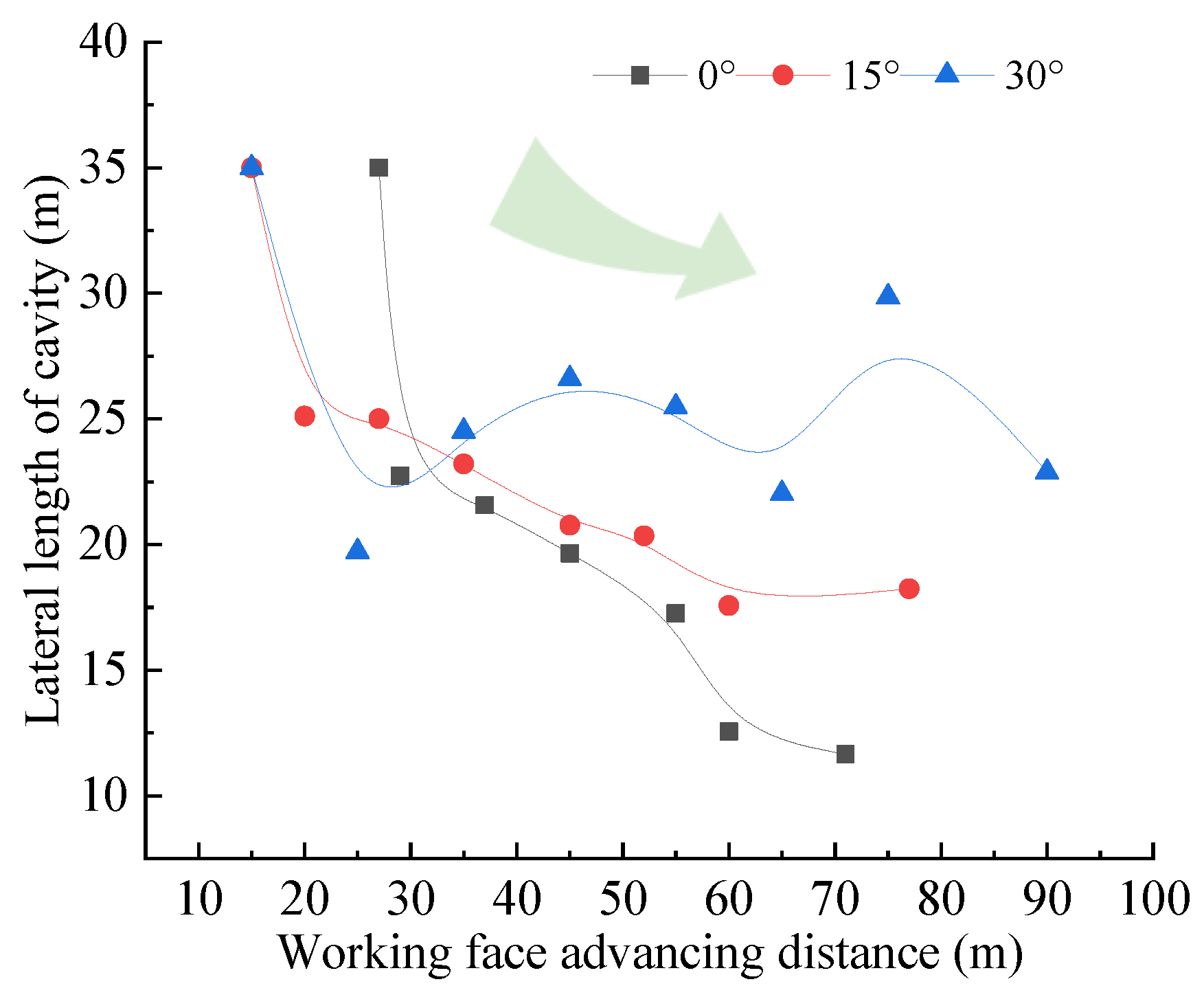
3.2. Evolution Rule of Cavity in Goaf
3.3. Periodic Weighing Rule of the Working Face
3.4. Morphological Distribution Characteristics of the Low-Gas Permeability Area
4. Discussion
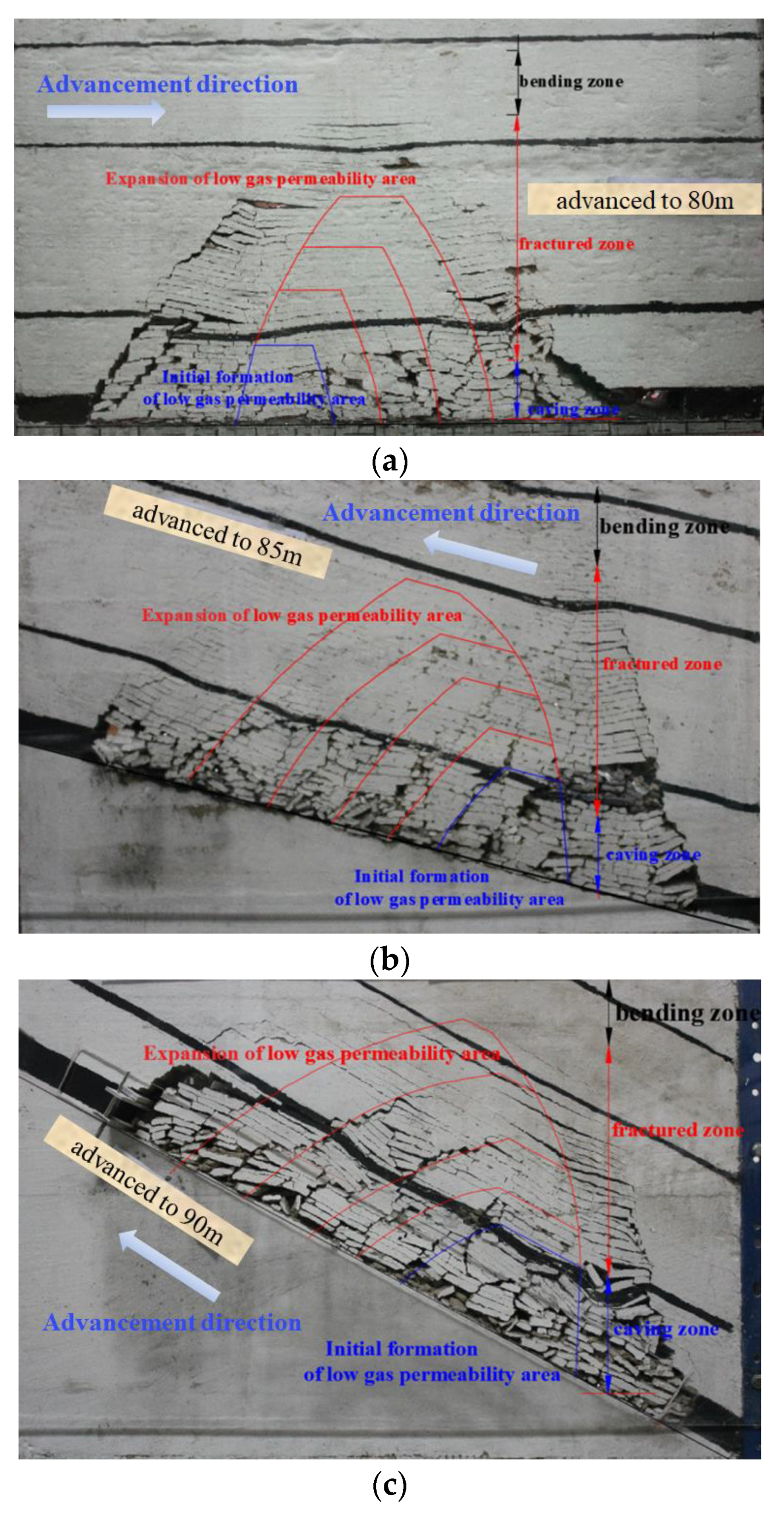
4.1. Evolutionary Law of the Fractal Dimension of the Low-Gas Permeability Area
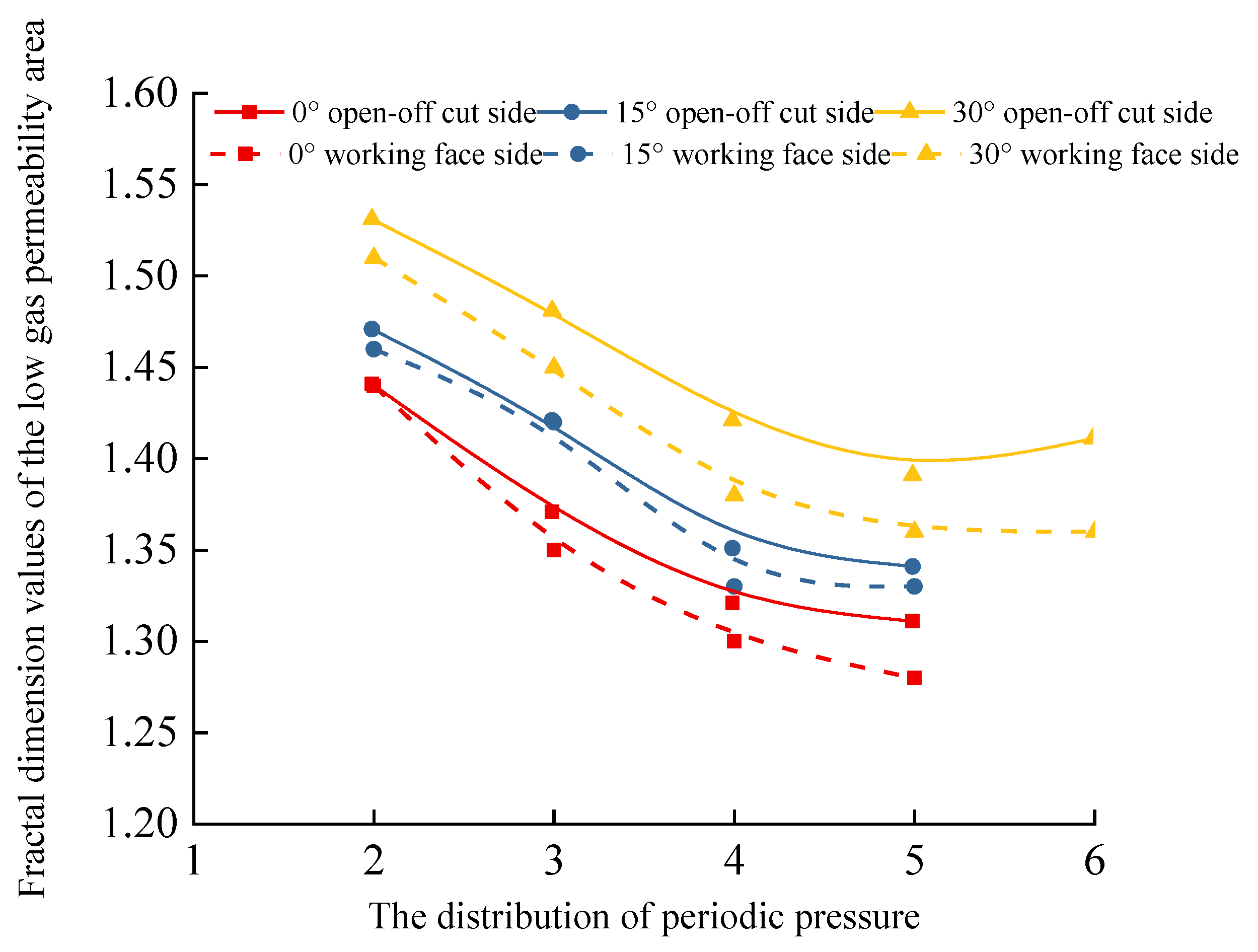
4.1.1. Periodic Pressure Effect of the Fractal Dimension of the Low-Gas Permeability Area
4.1.2. The Fractal Dimension of the Boundaries of the Low-Gas Permeability Area
4.1.3. Gradient Evolutionary Effect of the Fractal Dimension of the Low-Gas Permeability Area
4.2. Construction of a Fractal Dimensional Control Model for the Low-Gas Permeability Area
5. Field Testing
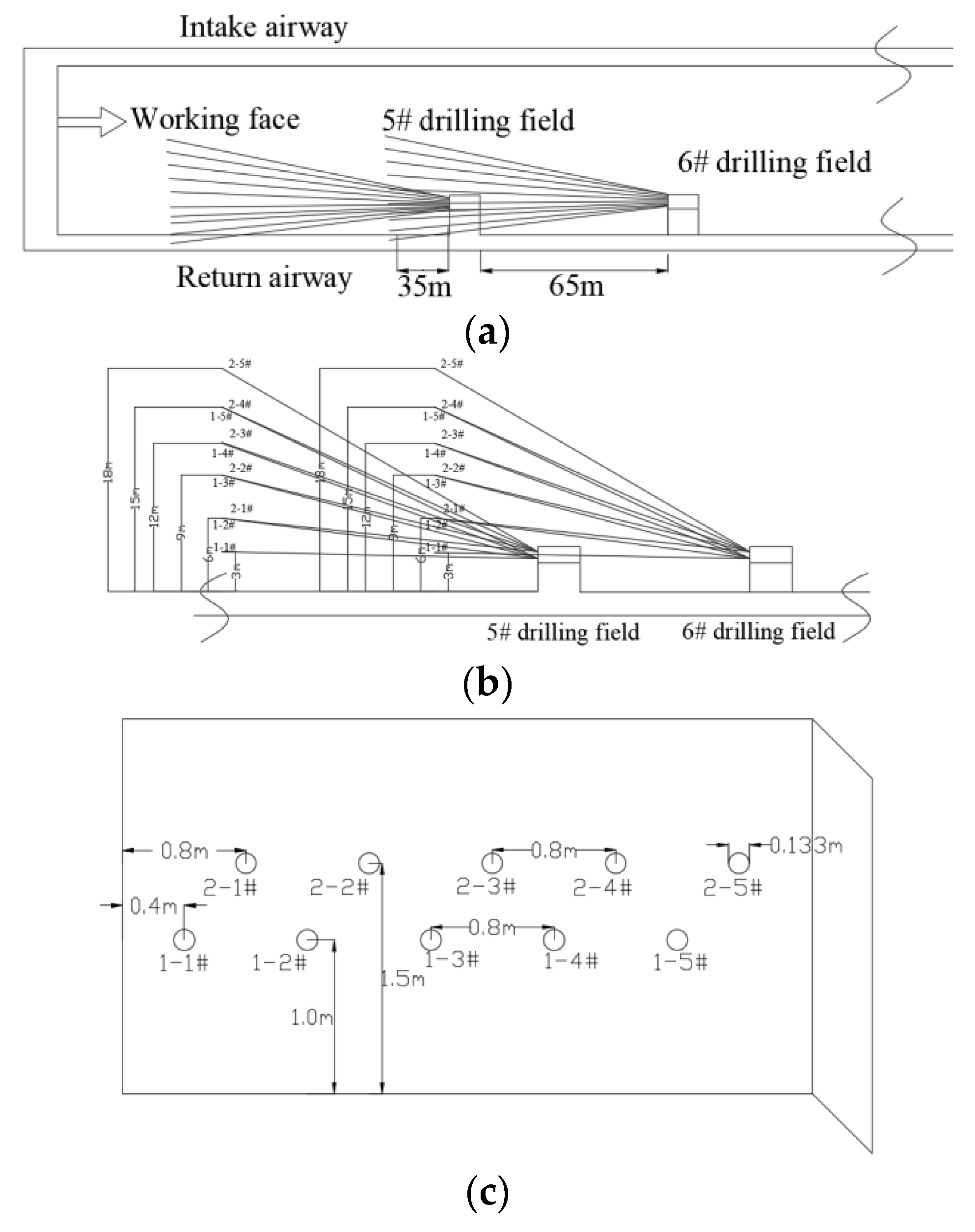
5.1. The Parameters of High-Level Boreholes
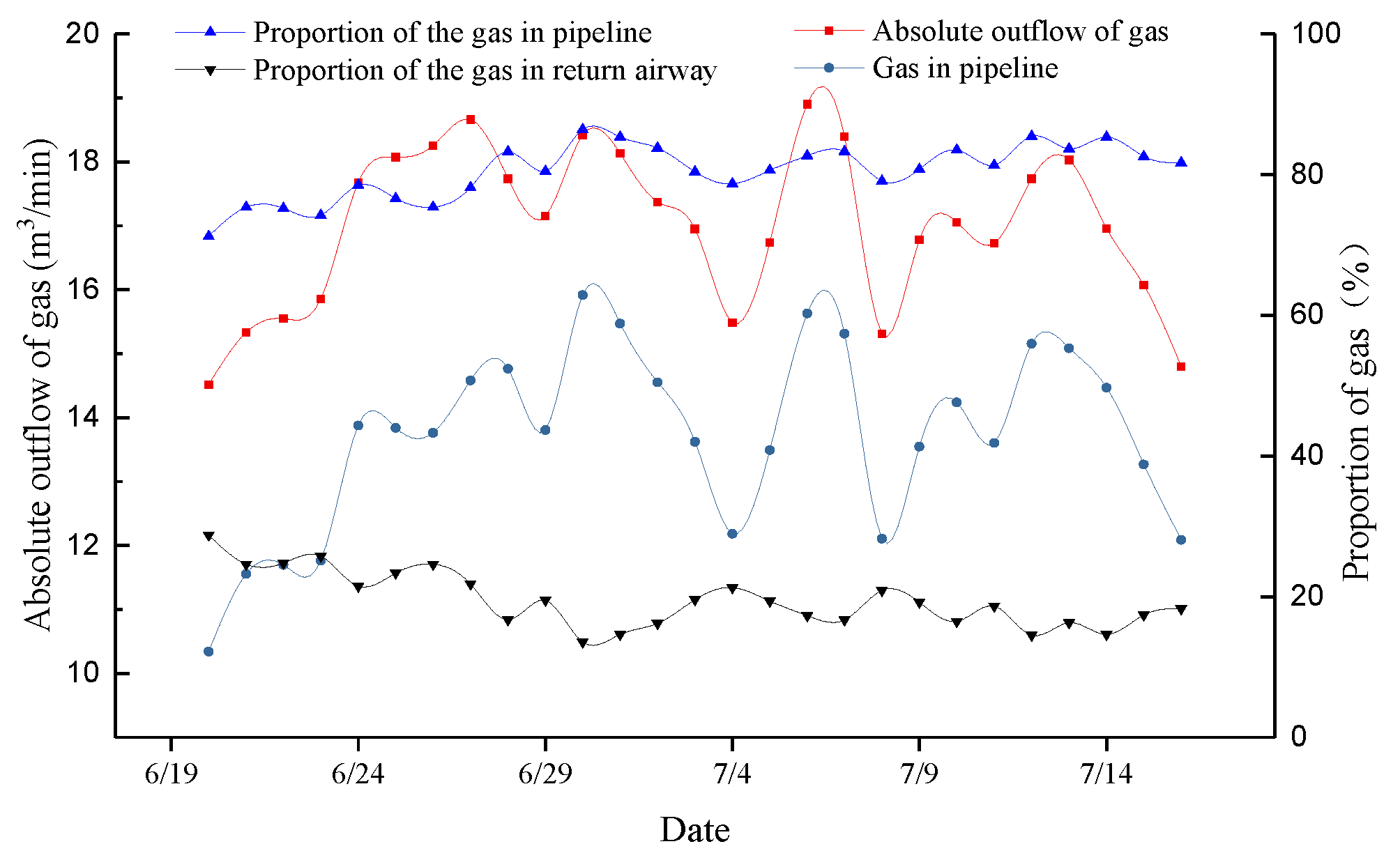
5.2. Analysis of the Effect of High-Level Drilling Field
5.3. The Practical Effect of Gas Extraction in the High-Level Drilling Field
6. Conclusions
- (1)
- Under the mining condition where the slope is upward, as the rock strata’s dip angle increases, the area of the overburden rock with high penetration in the goaf shifts gradually from a symmetrical form to an asymmetrical one on the side of the open-off cut. The overall trend towards a decrease in the lateral length of the cavity in the goaf area persists. The slumping effect affects the longitudinal width. There is a reverse growth evidenced in the early stage of mining that surpasses the height of mining before declining to the point of disappearance.
- (2)
- As the dip angle of the rock strata increases (0° < 15° < 30°), so does the fractal dimension of the low-gas permeability area. Periodic pressure causes the fractal dimension to gradually decrease until it stabilizes at 1.24, 1.27, and 1.34. Additionally, the fractal dimension of the low-gas permeability area is smaller on the working face side than on the side of the open-off cut. The gradient evolution rule suggests that the area of low gas permeability can be further classified into three zones: the strongly disturbed, the moderately disturbed, and the low disturbed areas.
- (3)
- A fractal dimension control equation for the low-gas permeability area affected by the dip angle of rock strata has been established using the theory of fracture elliptical paraboloid zone mining. This equation provides a framework for analyzing the development of overburden fractures in low-gas permeability areas and for establishing an accurate pressure relief gas extraction system.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhao, P.X.; Kang, X.P.; Li, S.G.; Lin, H.F.; Gan, L.J.; An, X.B. Optimization of “hole-drift” collaborative drainage layout parameters and high efficient drainage in pressure relief gas migration area. Coal Sci. Technol. 2022, 50, 137–146. [Google Scholar]
- Ding, Y.; Zhu, B.; Li, S.G.; Lin, H.F.; Wei, Z.Y.; Li, L.M.; Long, H.; Yi, Y. Accurate identification and efficient drainage of relieved methane in goaf of high outburst mine. Coal Sci. Technol. 2021, 46, 3565–3577. [Google Scholar]
- Zhao, P.X.; Liu, L.D.; Li, S.G.; Xu, P.Y.; Li, G.; Wei, Z.Y.; Jia, Y.Y. Dip angle effect of evolution coal seam in overburden low-gas permeability area of fully mechanized mining face. Coal Sci. Technol. 2021, 49, 65–72. [Google Scholar]
- Kashnikov, Y.A.; Musikhin, V.V.; Lyskov, I.A. Radar interferometry-based determination of ground surface subsidence under mineral mining. J. Min. Sci. 2012, 48, 649–655. [Google Scholar] [CrossRef]
- Sahu, P.; Lokhande, R.D.; Pradhan, M.; Jade, R. The influence of geotechnical parameters on sinkhole subsidence and its model development for underground coal mines in central India. J. Min. Sci. 2021, 57, 220–228. [Google Scholar] [CrossRef]
- Zhang, J.X.; Shi, X.C. Failure of overburden rock under large mining height in shallow buried goaf area. Chin. J. Geol. Hazard Control. 2019, 30, 92–97. [Google Scholar]
- Cheng, J.G. Numerical simulation research on “Three Zones” distribution rule in overlying strata of fully mechanized working face in upper protective seam with god-side entry retaining. Min. Saf. Environ. Prot. 2015, 42, 31–34+56. [Google Scholar]
- Li, X.; Jiao, Y.Y.; Zhou, J.P.; Zhang, X.F.; Wang, C. Study on overlying strata movement and ground subsidence in deep coal mining. Saf. Environ. Eng. 2022, 29, 32–38+56. [Google Scholar]
- Guo, D.; Wang, N.; Fan, L.; Lu, Z.; Li, K. Study on new type of roadway side support technology in coal mines. J. Min. Sci. 2020, 56, 603–615. [Google Scholar] [CrossRef]
- Khurshid, I.; Fujii, Y. Geomechanical analysis of formation deformation and permeability enhancement due to low-temperature CO2 injection in subsurface oil reservoirs. J. Pet. Explor. Prod. Technol. 2021, 11, 1–9. [Google Scholar] [CrossRef]
- Khurshid, I.; Afgan, I. Geochemical investigation of CO2 injection in oil and gas reservoirs of middle east to estimate the formation damage and related oil recovery. Energies 2021, 14, 7676. [Google Scholar] [CrossRef]
- Khurshid, I.; Afgan, I. Investigation of water composition on formation damage and related energy recovery from geothermal reservoirs: Geochemical and geomechanics insights. Energies 2021, 14, 7415. [Google Scholar] [CrossRef]
- Wang, D.K.; Wei, Q.; Wei, J.P.; Bai, X.; Yu, C.; Zhang, P. Fractal characteristics of fracture structure and fractal seepage model of coal. J. China Univ. Min. Technol. 2020, 49, 103–109+122. [Google Scholar]
- Qian, M.G.; Xu, J.L. Study on the “O-shape” circle distribution characteristics of mining-induced fractures in the overlaying strata. J. China Coal Soc. 1998, 23, 466–469. [Google Scholar]
- Yuan, L.; Guo, H.; Shen, B.T.; Qu, Q.D.; Xue, J.H. Circular overlying zone at longwall panel for efficient methane capture of mutiple coal seams with low permeability. J. China Coal Soc. 2011, 36, 357–365. [Google Scholar]
- Li, S.G.; Lin, H.F.; Zhao, P.X.; Xiao, P.; Pan, H.Y. Dynamic evolution of mining fissure elliptic paraboloid zone and extraction coal and gas. J. China Coal Soc. 2014, 39, 1455–1462. [Google Scholar]
- Husain, A.; Reddy, J.; Bisht, D.; Sajid, M. Fractal dimension of coastline of Australia. Sci. Rep. 2021, 11, 6304. [Google Scholar] [CrossRef] [PubMed]
- Cui, B.; Huang, P.; Xie, W. Fractal dimension characteristics of wind speed time series under typhoon climate. J. Wind. Eng. Ind. Aerodyn. 2022, 229, 105144. [Google Scholar] [CrossRef]
- Wu, M.; Li, H.; Wang, L.; Yang, X.; Dai, C.; Yang, N.; Li, J.; Wang, Y.; Yu, M. μCT quantitative assessment of the pore–fracture structures and permeability behaviors of long-flame coal treated by infrared rapid heating. Energy 2023, 274, 127308. [Google Scholar] [CrossRef]
- Cui, H.; Zhu, S.; Wang, J.; Gao, Y.; Wan, C.; Tong, H. Physical Properties, Pore-Throat Structure, Fractal Characteristics and Their Effects on the Gas-Bearing Capacity of Tight Sandstone: A Case Study from the Northern Tianhuan Depression, Ordos Basin, China. Nat. Resour. Res. 2022, 31, 1559–1584. [Google Scholar] [CrossRef]
- Xie, H.P.; Yu, G.M.; Yang, L.; Zhang, Y.Z. Fractal fracture network of mining-induced rock mass. Chin. J. Rock Mech. Eng. 1999, 2, 29–33. [Google Scholar]
- Yu, G.M.; Xie, H.P.; Zhou, H.W.; Zhang, Y.Z.; Yang, L. Experimental study on distribution law and fractal property of mining-induced fracture in structured rock mass. J. Exp. Mech. 1998, 13, 145–153. [Google Scholar]
- Zhao, P.X.; Zhuo, R.S.; Li, S.G.; Lin, H.F.; Shu, C.M.; Lai, W.B.; Jia, Y.Y.; Suo, L. Fractal characteristics of gas migration channels at different mining heights. Fuel 2020, 271, 117479. [Google Scholar] [CrossRef]
- Miao, T.J.; Yang, S.S.; Long, Z.C.; Yu, B.M. Fractal analysis of permeability of dual-porosity media embedded with random fractures. Int. J. Heat Mass Transf. 2015, 88, 814–821. [Google Scholar] [CrossRef]
- Liang, T.; Liu, X.L.; Wang, S.J. Fractal study on the crack network evolution and permeability change in mining rock mass. J. China Coal Soc. 2019, 44, 3729–3739. [Google Scholar]
- Gou, R.T.; Jiang, C.Y.; Liu, Y.; Wang, C.; Li, Y.L. Study on fractal characteristics of evolution of mining-induced fissures in karst landform. Energies 2022, 15, 5372. [Google Scholar] [CrossRef]
- Liu, C.; Xue, J.H.; Yu, G.F.; Cheng, X.Y. Fractal characterization for the mining crack evolution process of overlying strata based on microseismic monitoring technology. Int. J. Min. Sci. Technol. 2016, 2, 295–299. [Google Scholar] [CrossRef]
- Miao, K.J.; Tu, S.H.; Tu, H.S.; Liu, X.; Li, W.L.; Zhao, H.B.; Tang, L.; Ma, J.Y.; Li, Y. Research on Fractal Evolution Characteristics and Safe Mining Technology of Overburden Fissures under Gully Water Body. Fractal Fract. 2022, 6, 486. [Google Scholar] [CrossRef]
- Lu, F.C.; Zhang, X.B. Research on porosity distribution laws of goaf in inclined extra-thick coal seam. J. Saf. Sci. Technol. 2020, 16, 119–124. [Google Scholar]
- Feng, J.Y.; Liu, X.H.; Yu, Z.Q. Numerical simulation study on the mining-induced fracture evolution of steep coal seam. J. China Coal Soc. 2017, 42, 1971–1978. [Google Scholar]
- Li, S.G.; Qin, W.B.; Li, Z.L.; Ding, Y.; Lin, H.F. Research on fractal characterization of mined crack network evolution repeated coal mining. J. Liaoning Tech. Univ. 2016, 35, 1384–1389. [Google Scholar]
- Cao, J.; Shi, S.T. Evolution law of mining-induced fractures in shallow buried close coal seams mining. Geotech. Geol. Eng. 2023, 4, 2665–2676. [Google Scholar] [CrossRef]
- Xue, D.J.; Zhou, H.W.; Ren, W.G.; Zhang, B.F.; Liu, Y.Q.; Zhao, Y.F. Stepped shearing-induced failure mechanism and cracks propagation of overlying thin bedrocks in shallow deep coal seams mining. J. China Coal Soc. 2015, 40, 1746–1752. [Google Scholar]
- Li, D.P.; Zhou, H.W.; Xue, D.J.; Yi, H.Y.; Gao, H.L. Relationship between percolation and fractal properties of mining-induced crack network in coal and rock masses. Rock Soil Mech. 2015, 36, 1135–1140. [Google Scholar]
- Li, H.Y.; Wang, W.H.; Qi, Q.X.; Zhang, L. Study on fissure development rule of overlying strata influenced by mining based on fractal theory. J. China Coal Soc. 2014, 39, 1023–1030. [Google Scholar]
- Wang, G.Y.; Yu, G.M.; Yu, Y.J.; Lu, S.B.; Kang, Y. Study on Cracks Fractal Evolution Laws of Mining Rock Mass. J. Min. Saf. Eng. 2012, 29, 859–863. [Google Scholar]
- Zhao, Y.X.; Ling, C.W.; Liu, B.; He, X. Fracture evolution and energy dissipation of overlying strata in shallow-buried underground mining with ultra-high working face. J. Min. Saf. Eng. 2021, 38, 9–18+30. [Google Scholar]
- Wang, D.K.; Zeng, F.C.; Wang, J.G.; Wei, J.P.; Jiang, Z.G.; Wang, X.B.; Zhang, P.; Yu, C. Dynamic evolution characteristics and fractal law of loaded coal fractures by micro industrial CT. Chin. J. Rock Mech. Eng. 2020, 39, 1165–1174. [Google Scholar]
- Zou, E.L.; Li, Z.H.; Ma, J.; He, X.; Geng, Q. Study on the quantitative analysis of dynamic evolution of mining fracture and its application. Min. Res. Dev. 2023, 7, 101–107. [Google Scholar]








| Order Number | Lithology | Bulk Density/kN·m−3 | Elastic Modulus/GPa | Compressive Strength/MPa | Poisson Ratio |
|---|---|---|---|---|---|
| 1 | mudstone | 20.8 | 20 | 20.5 | 0.195 |
| 2 | sandy mudstone | 26.4 | 56 | 48.8 | 0.278 |
| 3 | medium sandstone | 26.6 | 50 | 65.1 | 0.28 |
| 4 | fine sandstone | 26.2 | 43 | 69.0 | 0.26 |
| 5 | siltstone | 26.0 | 54 | 58.5 | 0.253 |
| 6 | limestone | 26.5 | 47 | 91.2 | 0.23 |
| 7 | AL mudstone | 13.0 | 40 | 16.0 | 0.25 |
| 8 | coal | 14.6 | 14 | 13.5 | 0.275 |
| Order Number | Lithology | Thickness/m | Order Number | Lithology | Thickness/m |
|---|---|---|---|---|---|
| 1 | sandy mudstone | 2.4 | 16 | 13# coal | 0.5 |
| 2 | AL mudstone | 0.2 | 17 | K3 limestone | 3.0 |
| 3 | 15# coal | 5.1 | 18 | sandy mudstone | 1.0 |
| 4 | mudstone | 0.5 | 19 | fine sandstone | 0.8 |
| 5 | medium sandstone | 7.1 | 20 | AL mudstone | 0.5 |
| 6 | siltstone | 3.0 | 21 | fine sandstone | 2.2 |
| 7 | sandy mudstone | 3.7 | 22 | sandy mudstone | 4.5 |
| 8 | 10# coal | 0.8 | 23 | 12# coal | 1.0 |
| 9 | siltstone | 1.0 | 24 | sandy mudstone | 3.4 |
| 10 | mudstone | 1.7 | 25 | siltstone | 0.9 |
| 11 | 14# coal | 0.8 | 26 | 11# coal | 0.3 |
| 12 | K2 limestone | 5.5 | 27 | K4 limestone | 4.65 |
| 13 | siltstone | 2.8 | 28 | sandy mudstone | 7.75 |
| 14 | fine sandstone | 6.1 | 29 | fine sandstone | 4.0 |
| 15 | sandy mudstone | 2.0 | 30 | sandy mudstone | 2.7 |
| Geometry Ratio | Bulk Density Ratio | Stress Ratio | Time Ratio | Strength Ratio | Poisson Ratio |
|---|---|---|---|---|---|
| 1:100 | 1:1.5 | 1:150 | 1:10 | 1:150 | 1:1 |
| The Dip Angle of the Rock Strata | Mathematical Expressions for Curve Fitting | R2 |
|---|---|---|
| 0° | 0.878 | |
| 15° | 0.853 | |
| 30° | 0.960 |
| The Dip Angle of the Rock Strata | Mathematical Expressions for Curve Fitting | R2 |
|---|---|---|
| 0° | 0.928 | |
| 15° | 0.961 | |
| 30° | 0.933 |
| No. | Distance to the Floor (m) | Depth (m) | Angle with the Centerline of the Roadway | Final Position (m) | Diameter (m) |
|---|---|---|---|---|---|
| 1-1# | 1.0 | 100.04 | left to 3.5° | 3 | 0.133 |
| 1-2# | 1.0 | 100.07 | left to 1.8° | 6 | 0.133 |
| 1-3# | 1.0 | 100.11 | 0° | 9 | 0.133 |
| 1-4# | 1.0 | 100.15 | right to 1.7° | 12 | 0.133 |
| 1-5# | 1.0 | 100.20 | right to 3.5° | 15 | 0.133 |
| 2-1# | 1.5 | 100.09 | left to 2.4° | 6 | 0.133 |
| 2-2# | 1.5 | 100.13 | left to 0.6° | 9 | 0.133 |
| 2-3# | 1.5 | 100.18 | right to 1.1° | 12 | 0.133 |
| 2-4# | 1.5 | 100.25 | right to 2.9° | 15 | 0.133 |
| 2-5# | 1.5 | 100.31 | right to 4.6° | 18 | 0.133 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, S.; Liu, L.; Zhao, P.; Jia, Y.; Lin, H. Fractal Characteristics of the Low-Gas Permeability Area of a Fully Mechanized Up-Dip Working Face under Different Dip Angles of Rock Strata. Energies 2023, 16, 7055. https://doi.org/10.3390/en16207055
Li S, Liu L, Zhao P, Jia Y, Lin H. Fractal Characteristics of the Low-Gas Permeability Area of a Fully Mechanized Up-Dip Working Face under Different Dip Angles of Rock Strata. Energies. 2023; 16(20):7055. https://doi.org/10.3390/en16207055
Chicago/Turabian StyleLi, Shugang, Lidong Liu, Pengxiang Zhao, Yongyong Jia, and Haifei Lin. 2023. "Fractal Characteristics of the Low-Gas Permeability Area of a Fully Mechanized Up-Dip Working Face under Different Dip Angles of Rock Strata" Energies 16, no. 20: 7055. https://doi.org/10.3390/en16207055
APA StyleLi, S., Liu, L., Zhao, P., Jia, Y., & Lin, H. (2023). Fractal Characteristics of the Low-Gas Permeability Area of a Fully Mechanized Up-Dip Working Face under Different Dip Angles of Rock Strata. Energies, 16(20), 7055. https://doi.org/10.3390/en16207055






